Numerical Simulation of the Cold-Start Process of Polymer Electrolyte Fuel Cell

Abstract

In this study, the cold-start process of a polymer electrolyte fuel cell has been numerically investigated under various ambient temperatures and operating currents, ranging from subzero to 283 K. The water desorbed from the electrolyte, when the cell temperature is below the freezing point, is assumed to exist in a state of either supercooled water or ice. The evolution of cell voltage, temperature, membrane water content, and the averaged volume fraction of supercooled water or ice in the catalyst layer and gas diffusion layer are presented. The results indicate that the cold-start process may fail due to ice blocking of the cathode catalyst layer when the desorbed water is in the form of ice and the ambient temperature is sufficiently low. However, when the desorbed water is in a supercooled state, it can diffuse from the cathode catalyst layer to the cathode gas diffusion layer, avoiding water clogging and enabling a successful cold-start process. During the cold-start process, as the ice undergoes a melting process, the membrane water content inside the membrane would increase rapidly, and a larger operation current with anode gas humidification is helpful to the cold-start process.

1. Introduction

Polymer electrolyte fuel cell (PEFC) stands out as a highly promising power supply in the automotive industry, owing to its exceptional attributes, such as high-power density, remarkable efficiency, low operating temperature, and complete lack of emissions. Despite advancements, successfully initiating a startup in subfreezing temperatures, commonly known as a “cold-start,” remains a significant technical challenge and a key obstacle in the commercialization of PEFC [1]. During the cold-start process, there is a possibility of product water freezing inside the cell, potentially covering the triple-phase boundary and clogging the flow of reactants from the gas channels (GCs) to the catalyst layers (CLs). When the CLs are completely blocked by ice, the electrochemical reaction is halted due to gas starvation [2]. Repeated failures may cause irreversible losses in cell performance. Even worse, the ice formation within the PEFC can lead to the destruction of the cell’s internal structure, owing to the interplay of multiple dynamic factors [3,4]. Therefore, a better understanding of cold-start characteristics is of great importance for the development of PEFC.

Over the past decade, numerous experimental and numerical studies have provided comprehensive insights into the mass and heat transfer mechanism inside the cell. Extensive efforts have been implemented for elucidating the cold-start characteristics and startup strategies from various aspects. The majority of these studies have investigated the ice formation and growth properties within the PEFC under various cold-start conditions [5,6]. Based on the experimental evidence, researchers have developed a non-isothermal and multiphase model to predict the electrochemical, mass, and heat transfer processes occurring inside the PEFC during the cold-start process [7]. Water vapor was generated in CL and rapidly equilibrated with ionomer/membrane water. When the water vapor became locally saturated, it underwent de-sublimation, leading to the formation of ice [8,9,10,11,12,13,14]. These models reveal the performance of cell voltage, temperature, and ice volume fraction as functions of time. The cell voltage initially rises as a result of membrane hydration. In a failed cold start process, the cell voltage drops rapidly during the last few seconds when the ice volume fraction in cathode CL approaches unity. The gas supply is blocked. Based on the fundamental research mentioned above, a series of startup strategies and optimized cell designs are proposed. Micro-porous layer (MPL) is used to optimize the ice storage ability of the electrode into MPL for enhancement of cold-start performance. A proper humidification strategy is also regarded as an important issue because of its notable influence not only on water evaporation but also on the water transfer in porous electrode [15].

Recently, significant advancements in in situ visualization and detection methods have enabled researchers to conduct visualization experiments using optical microscopes [16,17], X-rays [18,19], and neutron imaging [20,21,22,23] to gain a better understanding of water behavior within the PEFC during the cold-start process. In all cases, the generation of product water was observed in CL, gas diffusion layer (GDL), and even GC in the state of supercooled rather than ice, particularly between −2 °C and −20 °C. Therefore, it can be inferred that the water inside the PEFC was initially in a supercooled state, and the formation of the water pathway from the cathode CL towards the flow channel likely occurred gradually. After successfully establishing the water pathway, the supercooled water could efficiently transport along this preferred route, enabling the cell to operate continuously for extended periods. This observation suggests that the presence of supercooled water could potentially enhance the cold-start process. In addition to the proper humidification strategy, liquid water management strategies are also possibly employed for the reinforcement of the cold-start capability [24]. However, supercooled water is thermodynamically unstable. The freezing of supercooled water, characterized by considerable randomness, can be initiated either by the activation of a phase transition or the presence of a nucleation site. In more recent studies, the impact of supercooled water has been taken into account in numerical models. Specifically, researchers have examined the influence of MPL hydrophobicity on output performance and ice/water distribution under various startup conditions [25]. In our recent research [26], we developed a model to simulate the stochastic freezing process of supercooled water by incorporating a freezing probability function. This allowed us to comprehensively investigate the cell cold-start processes under various initial and operating conditions. The results indicate that the failure of the cell cold-start process is primarily attributed to anode dehydration when the cell operates with a relatively high current density. However, when the cell starts with a relatively high initial membrane water content, the failure is dominated by the pore blockage of cathode CL [27]. Moreover, because of the stochastic freezing behavior of supercooled water, the failure mode becomes uncertain under certain cold-start conditions with specific initial membrane water content and current load. The outcome predominantly relies on the stochastic occurrence of the freezing process.

As mentioned above, the cold-start performance of PEFC has been extensively explored in the literature. However, the water production and phase transition mechanisms inside the cell and corresponding effects on the cell cold-start capability are considered to be immature and need to be further consummated [25]. The conventional phase transition system, which only includes water vapor, ice, and membrane water (water dissolved in the electrolyte), is not rigorous anymore, and is possibly unable to predict the water transport inside the cell. In this study, utilizing the numerical model introduced in [26], we conducted numerical simulations of the cold-start process under various operating conditions, ranging in temperature from subzero to above zero. The phase transition processes among water vapor, liquid water, ice, and membrane water are considered comprehensively. Throughout the operation of PEFC, the product water is assumed to be in the “dissolved” phase, which is considered membrane water. When the cell temperature is below the freezing point, the water desorbed out of the membrane is presumed to exist in a state of either supercooled water or ice. The evolution of cell performance, membrane water, ice, and water inside the cell are intensively studied. Considering the scarcity of the cold-start research with consideration of the cell temperature ranging from subzero to above zero, this study qualitatively analyzes the influence of the phase state of water on the fuel cell cold-start performance. The ice melting and water transport mechanism during the cold-start process is further investigated and discussed, which will offer valuable insights for designing an effective cold-start strategy for PEFC. The numerical model is introduced in the Section 2. The detailed resultant modeling prediction and proper discussions are given in the Section 3, followed by the main conclusions in the Section 4.

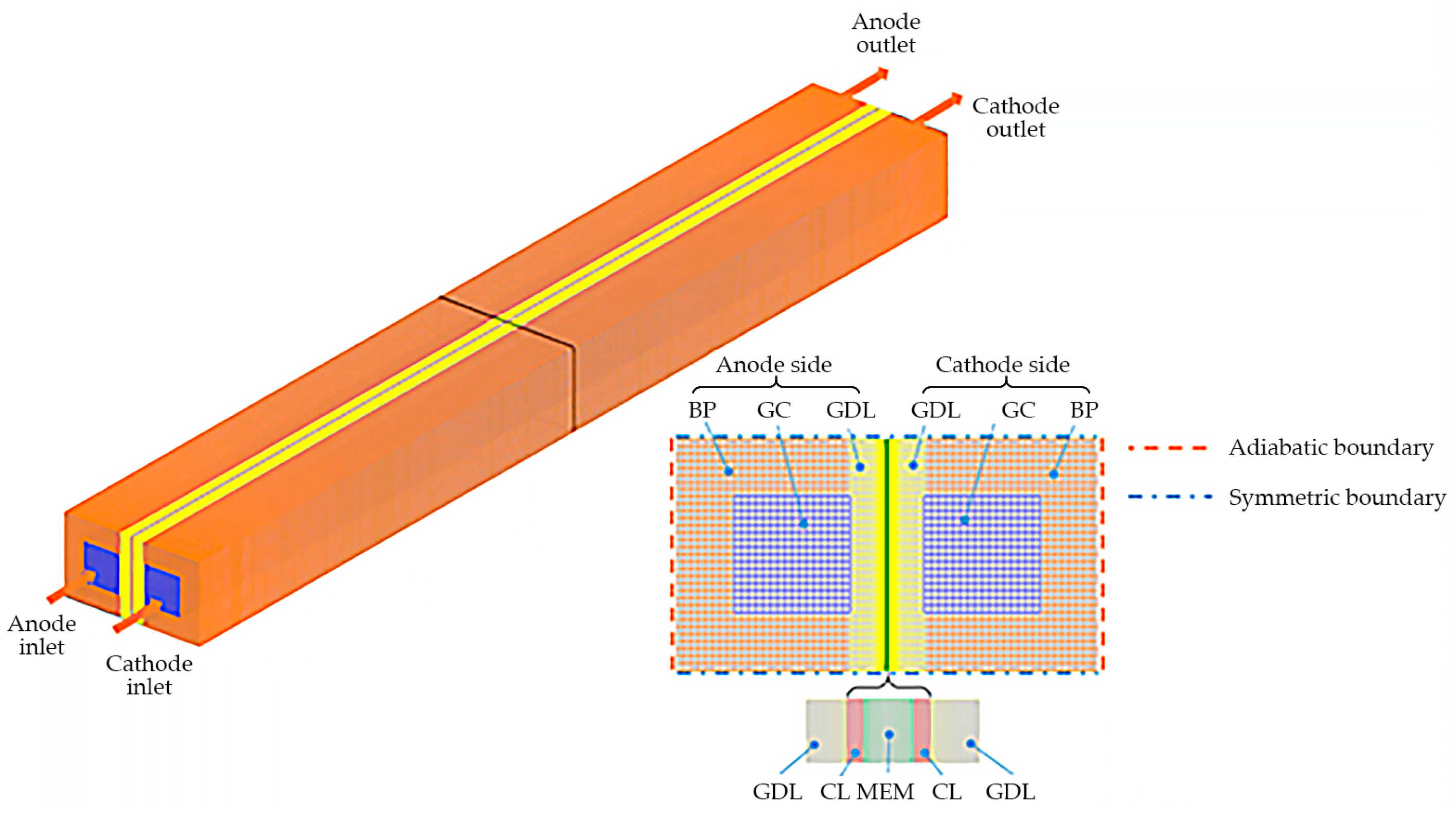
2. Numerical Model

In this study, as illustrated in Figure 1, the PEFC with a single GC is considered. It incorporates all the essential components, including bipolar plates (BPs), gas diffusion layers (GDLs), catalyst layers (CLs), and membrane (MEM). The cell geometry and material parameters are provided in

Table 1. Geometry and material parameters of the cell.

Description	Symbol	Value	Unit
Length, width, height/rib of GC	-	50/1.0/1.0/1.0	mm
Thickness of BP/GDL/CL/MEM	$\mathit{\delta }$	2.0/0.2/0.01/0.03	mm
Density of BP/GDL/CL/MEM	$\mathit{\rho }$	2230/1230/1230/1980	kg m−3
Specific heat capacities of BP/GDL/CL/MEM	${\mathit{C}}_{\mathit{p}}$	1071/462/2683/833	J kg−1 K−1
Thermal conductivity of BP/GDL/CL/MEM	$\mathit{k}$	20/1.5/1.2/0.95	W m−1 K−1
Electronic conductivity of BP/GDL/MPL/CL	${\mathit{\kappa }}_{\mathit{s}}$	20,000/300/300/300	S m−1
Porosity of GDL/CL	$\mathit{\epsilon }$	0.78/0.43	-
Contact angle in pore GDL/CL	$\mathit{\theta }$	140/100	$°$
Pore radii of GDL/CL	${\mathit{r}}_{\mathit{porous}}$	3.89 × 10−5/1.2 × 10−8	m
Permeability of GDL/CL	$\mathit{K}$	10−12/10−13	m2
Ionomer volume fraction in CL	$\mathit{\omega }$	0.15	-
Equivalent weight of MEM	$\mathit{E}\mathit{W}$	1100	kg kmol−1
Density of liquid water/ice	$\mathit{\rho }$	998.2/920	kg m−3
Dynamic viscosity of water	$\mathit{\mu }$	1.01 × 10−3	Pa s
Specific heat capacities of liquid water/ice	${\mathit{C}}_{\mathit{p}}$	4182/2050	J kg−1 K−1
Thermal conductivity of liquid water/ice	$\mathit{k}$	0.6/0.22	W m−1 K−1

. A comprehensive account of the cold-start PEFC model has been presented in our previous papers [26]. For the sake of completeness, a concise overview of the numerical model is also provided here, along with the following specific assumptions:

• The porous components of the PEFC are isotropic and homogeneous.
• The gas mixture is incompressible and the gas flow is laminar due to the small pressure gradient and a low flow Reynolds number.
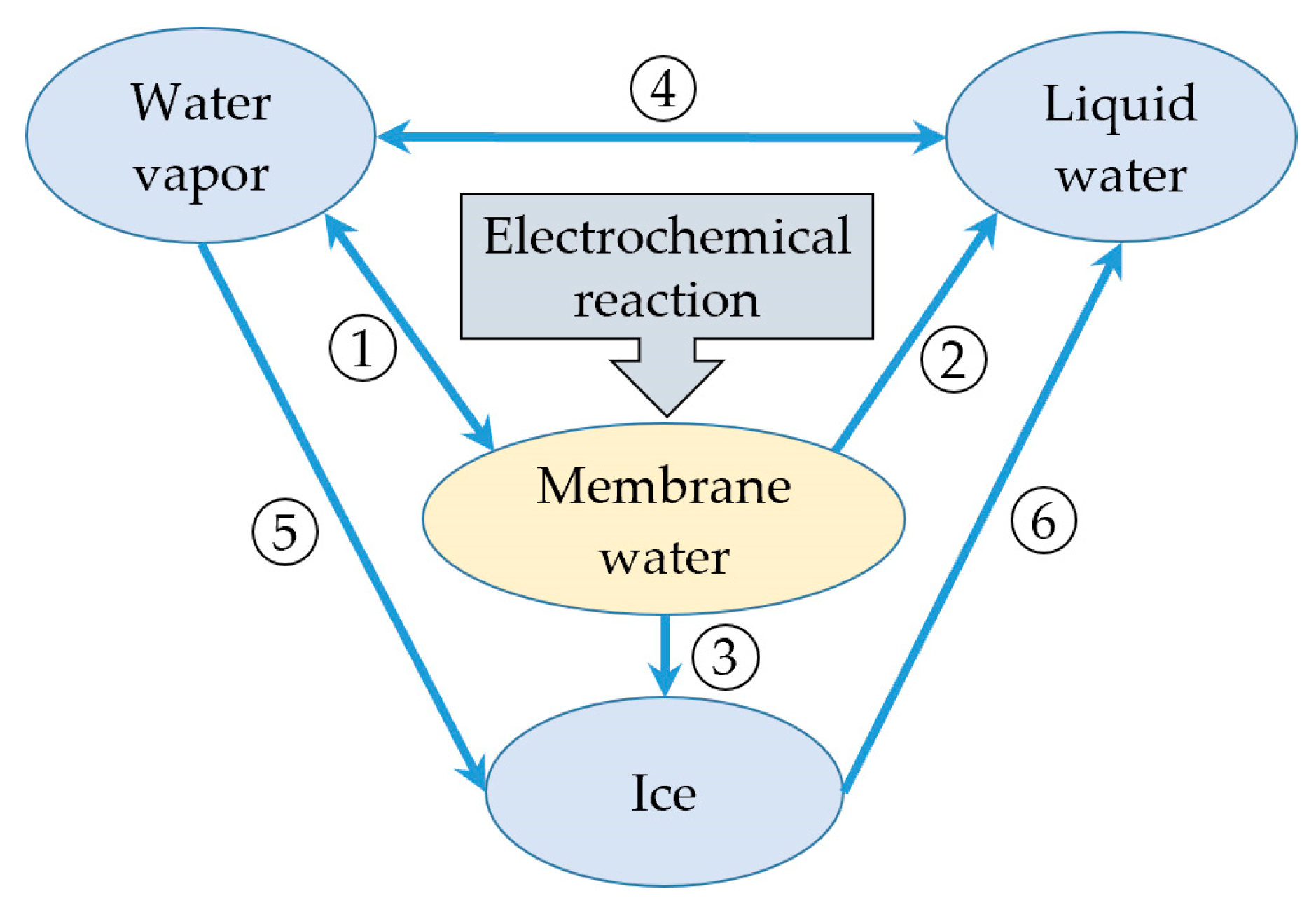
• The product water in the cathode CL resulting from the electrochemical reaction is considered to be membrane water, which can convert to vapor. The presence of liquid water or ice is taken into account when the membrane water content becomes saturated. However, the icing phenomenon of supercooled water is not considered in the model.
• The membrane water diffusion is considered, which is driven by the concentration gradient of membrane water content in MEM and is independent of the hydraulic pressure gradient.
• The electrochemical double-layer effect in CLs and the gravitational effect are ignored.

2.1. Governing Equations

Based on the given assumptions above, conservation equations of mass, momentum, species, water in different states, charge, and energy are included in this transient multiphase model. These equations are listed as follows:

Mass conservation is:

$$\frac{\partial }{\partial \mathit{t}}\left[\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right){\mathit{\rho }}_{\mathit{g}}\right]+\nabla \cdot \left({\mathit{\rho }}_{\mathit{g}}{\mathit{u}}_{\mathit{g}}\right)={\mathit{S}}_{\mathit{m}}$$

(1)

where $\mathit{\epsilon }$ is the porosity of CLs and GDLs, ${\mathit{s}}_{\mathit{l}}$ and ${\mathit{s}}_{\mathit{i}}$ indicate the volume fraction of liquid water and ice in the porous pore, ${\mathit{u}}_{\mathit{g}}$ denotes the gas flow velocity, and ${\mathit{\rho }}_{\mathit{g}}$ is the density of the gas mixture. The expression is as follows:

$${\mathit{\rho }}_{\mathit{g}}=\frac{1}{{\displaystyle \sum _{\mathit{n}}{\mathit{Y}}_{\mathit{n}}/{\mathit{\rho }}_{\mathit{n}}}}$$

(2)

where ${\mathit{Y}}_{\mathit{n}}$ is the mass fraction of each species (including H₂, O₂, and H₂O) in the gas mixture. ${\mathit{S}}_{\mathit{m}}$ is the mass source term, which is comprised of the consumption of hydrogen and oxygen, and the phase transition between vapor and membrane water, liquid water, and ice.

Momentum conservation is as follows:

$$\begin{array}{l}\frac{\partial }{\partial \mathit{t}}\left(\frac{{\mathit{\rho }}_{\mathit{g}}{\mathit{u}}_{\mathit{g}}}{\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}\right)+\nabla \cdot \left(\frac{{\mathit{\rho }}_{\mathit{g}}{\mathit{u}}_{\mathit{g}}{\mathit{u}}_{\mathit{g}}}{{\mathit{\epsilon }}^2{\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}^2}\right)=-\nabla {\mathit{p}}_{\mathit{g}}-\frac{2}{3}{\mathit{\mu }}_{\mathit{g}}\nabla \left(\nabla \cdot \left(\frac{{\mathit{u}}_{\mathit{g}}}{\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}\right)\right)\\ +{\mathit{\mu }}_{\mathit{g}}\nabla \cdot \left(\nabla \left(\frac{{\mathit{u}}_{\mathit{g}}}{\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}\right)+\nabla {\left(\frac{{\mathit{u}}_{\mathit{g}}}{\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}\right)}^{\mathit{t}\mathit{r}}\right)+{\mathit{S}}_{\mathit{u}}\end{array}$$

(3)

where ${\mathit{p}}_{\mathit{g}}$ and ${\mathit{\mu }}_{\mathit{g}}$ denote the pressure and dynamic viscosity of the gas mixture, and where ${\mathit{S}}_{\mathit{u}}$ is the source term of the momentum, which is zero in the GCs. However, in the porous GDLs and CLs, according to the Darcy law, ${\mathit{S}}_{\mathit{u}}$ can be expressed as:

$${\mathit{S}}_{\mathit{u}}=-\frac{{\mathit{\mu }}_{\mathit{g}}}{{\mathit{K}}_{\mathit{g}}}{\mathit{u}}_{\mathit{g}}$$

(4)

Here, ${\mathit{K}}_{\mathit{g}}={\mathit{K}}_0{\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)}^4$ is the permeability of the gas mixture, and ${\mathit{K}}_0$ is the intrinsic permeability of the porous media.

Gas species conservation is as follows:

$$\frac{\partial }{\partial \mathit{t}}\left[\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right){\mathit{\rho }}_{\mathit{g}}{\mathit{Y}}_{\mathit{n}}\right]+\nabla \cdot \left({\mathit{\rho }}_{\mathit{g}}{\mathit{u}}_{\mathit{g}}{\mathit{Y}}_{\mathit{n}}\right)=\nabla \cdot \left({\mathit{\rho }}_{\mathit{g}}{\mathit{D}}_{\mathit{n}}^{\mathit{e}\mathit{f}\mathit{f}}\nabla {\mathit{Y}}_{\mathit{n}}\right)+{\mathit{S}}_{\mathit{n}}$$

(5)

where ${\mathit{D}}_{\mathit{n}}^{\mathit{e}\mathit{f}\mathit{f}}$ is the effective diffusivity. It is corrected by porosity and volume fractions of liquid water and ice as follows:

$${\mathit{D}}_{\mathit{n}}^{\mathit{eff}}={\left[\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{i}}-{\mathit{s}}_{\mathit{l}}\right)\right]}^{1.5}{\mathit{D}}_{\mathit{n}}$$

(6)

where ${\mathit{D}}_{\mathit{n}}$ is the mass diffusivity for each gas species. ${\mathit{S}}_{\mathit{n}}$ is the source term of each species.

Membrane water conservation is as follows:

$$\frac{{\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}}{\mathit{E}\mathit{W}}\frac{\partial \left(\mathit{\omega }\mathit{\lambda }\right)}{\partial \mathit{t}}=\frac{{\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}}{\mathit{E}\mathit{W}}\nabla \cdot \left({\mathit{\omega }}^{1.5}{\mathit{D}}_{\mathit{m}\mathit{w}}\nabla \mathit{\lambda }\right)+{\mathit{S}}_{\mathit{m}\mathit{w}}$$

(7)

where $\mathit{\lambda }$ indicates the membrane water content; $\mathit{E}\mathit{W}$ and ${\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}$ denote the equivalent weight and the density of MEM, respectively; and $\mathit{\omega }$ is the ionomer volume fraction in CLs, which is set to be 1.0 in membrane. ${\mathit{D}}_{\mathit{m}\mathit{w}}$ is the membrane water diffusivity, which depends on temperature and the membrane water content $\mathit{\lambda }$:

$${\mathit{D}}_{\mathit{m}\mathit{w}}=\{\begin{array}{cc}3.1\times {10}^{-7}\mathit{\lambda }\left[\mathit{exp}\left(0.28\mathit{\lambda }\right)-1\right]\mathit{exp}\left(-\frac{2436}{\mathit{T}}\right)& 0<\mathit{\lambda }\le 3\\ 4.17\times {10}^{-8}\mathit{\lambda }\left[161\mathit{exp}\left(-\mathit{\lambda }\right)+1\right]\mathit{exp}\left(-\frac{2436}{\mathit{T}}\right)& \mathit{\lambda }>3\end{array}$$

(8)

${\mathit{S}}_{\mathit{m}\mathit{w}}$ is the source term of membrane water. It is comprised of the electroosmosis and phase transition between vapor, liquid water, ice, and membrane water in porous CLs. Despite that, in the membrane, ${\mathit{S}}_{\mathit{m}\mathit{w}}$ is contributed by the electroosmosis only.

Liquid water conservation is as follows:

$$\frac{\partial \left(\mathit{\epsilon }{\mathit{\rho }}_{\mathit{l}}{\mathit{s}}_{\mathit{l}}\right)}{\partial \mathit{t}}+\nabla \cdot \left(\frac{{\mathit{K}}_{\mathit{l}}{\mathit{\mu }}_{\mathit{g}}}{{\mathit{K}}_{\mathit{g}}{\mathit{\mu }}_{\mathit{l}}}{\mathit{\rho }}_{\mathit{l}}{\mathit{u}}_{\mathit{g}}\right)=\nabla \cdot \left(-\frac{{\mathit{\rho }}_{\mathit{l}}{\mathit{K}}_{\mathit{l}}}{{\mathit{\mu }}_{\mathit{l}}}\frac{\mathit{d}{\mathit{p}}_{\mathit{l}}}{\mathit{d}{\mathit{s}}_{\mathit{l}}}\nabla {\mathit{s}}_{\mathit{l}}\right)+{\mathit{S}}_{\mathit{l}}$$

(9)

where ${\mathit{\rho }}_{\mathit{l}}$ and ${\mathit{\mu }}_{\mathit{l}}$ indicate the density and dynamic viscosity of the liquid water. ${\mathit{K}}_{\mathit{l}}={\mathit{K}}_0{\mathit{s}}_{\mathit{l}}^4{\left(1-{\mathit{s}}_{\mathit{i}}\right)}^4$ is the permeability of liquid water in the porous GDLs and CLs. ${\mathit{p}}_{\mathit{l}}$ is the pressure attributed to the surface tension, which can be expressed as:

$${\mathit{p}}_{\mathit{l}}=\{\begin{array}{cc}\frac{\mathit{\sigma }\mathit{cos}\mathit{\theta }}{{\left({\mathit{K}}_0/\mathit{\epsilon }\right)}^{0.5}}\left[1.417\left(1-{\mathit{s}}_{\mathit{l}}\right)-2.12{\left(1-{\mathit{s}}_{\mathit{l}}\right)}^2+1.263{\left(1-{\mathit{s}}_{\mathit{l}}\right)}^3\right]& \mathit{\theta }\le {90}^{°}\\ \frac{\mathit{\sigma }\mathit{cos}\mathit{\theta }}{{\left({\mathit{K}}_0/\mathit{\epsilon }\right)}^{0.5}}\left[1.417{\mathit{s}}_{\mathit{l}}-2.12{\mathit{s}}_{\mathit{l}}^2+1.263{\mathit{s}}_{\mathit{l}}^3\right]& \mathit{\theta }>{90}^{°}\end{array}$$

(10)

Here, $\mathit{\theta }$ is the contact angle in pore GDLs and CLs, and $\mathit{\sigma }$ is the surface tension coefficient, which can be expressed as a function of temperature $\mathit{T}$ [28], which is:

$$\mathit{\sigma }=\{\begin{array}{ll}0.1218-0.0001676\mathit{T}& \mathit{for}273.15\le \mathit{T}<373.15\\ 0.1130-0.0001354\mathit{T}& \mathit{for}240\le \mathit{T}<273.15\end{array}$$

(11)

It is worth noting that liquid water would exist in the form of supercooled water when the cell temperature is below the freezing point. The source term ${\mathit{S}}_{\mathit{l}}$ includes the phase transition between vapor, ice, membrane water, and liquid water in porous CLs. However, in GDLs, ${\mathit{S}}_{\mathit{l}}$ specifically denotes the phase transition between liquid water and vapor as well as ice.

Ice conservation is as follows:

$$\frac{\partial \left(\mathit{\epsilon }{\mathit{s}}_{\mathit{i}}{\mathit{\rho }}_{\mathit{i}}\right)}{\partial \mathit{t}}={\mathit{S}}_{\mathit{i}}$$

(12)

where ${\mathit{\rho }}_{\mathit{i}}$ is the density of ice, and ${\mathit{S}}_{\mathit{i}}$ is the source term contributed by the phase transition between liquid water, vapor, membrane water, and ice.

Charge conservation is as follows:

$$\nabla \cdot \left({\mathit{\kappa }}_{\mathit{s}}^{\mathit{e}\mathit{f}\mathit{f}}\nabla {\mathit{\varphi }}_{\mathit{s}}\right)+{\mathit{S}}_{\mathit{s}}=0$$

(13)

$$\nabla \cdot \left({\mathit{\kappa }}_{\mathit{e}}^{\mathit{e}\mathit{f}\mathit{f}}\nabla {\mathit{\varphi }}_{\mathit{e}}\right)+{\mathit{S}}_{\mathit{e}}=0$$

(14)

where ${\mathit{\varphi }}_{\mathit{s}}$ and ${\mathit{\varphi }}_{\mathit{e}}$ denote the potential of the solid phase and electrolyte, ${\mathit{\kappa }}_{\mathit{s}}^{\mathit{e}\mathit{f}\mathit{f}}$ is effective electronic conductivity, which is a constant, and ${\mathit{\kappa }}_{\mathit{e}}^{\mathit{e}\mathit{f}\mathit{f}}$ is ionic conductivity, which can be expressed as [11]:

$${\mathit{\kappa }}_{\mathit{e}}^{\mathit{e}\mathit{f}\mathit{f}}={\mathit{\omega }}^{1.5}\left(0.5139\mathit{\lambda }-0.326\right)\times \mathit{exp}\left[1268\times \left(\frac{1}{303.15}-\frac{1}{\mathit{T}}\right)\right]$$

(15)

The source term ${\mathit{S}}_{\mathit{e}}$ and ${\mathit{S}}_{\mathit{s}}$ correspond to the hydrogen oxidation reaction in the anode CL and the oxygen reduction reaction in the cathode CL, respectively. Under facile and sluggish kinetics, the electrochemical reaction rates on the anode and cathode side follow the linearized Butler–Volmer equation and the Tafel equation, respectively [29]:

$${\mathit{j}}_{\mathit{an}}={\mathit{i}}_{\mathit{an}}^{\mathit{ref}}\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right){\left(\frac{{\mathit{C}}_{{\mathit{H}}_2}}{{\mathit{C}}_{{\mathit{H}}_2}^{\mathit{ref}}}\right)}^{0.5}\left(\frac{{\mathit{\alpha }}_{\mathit{an}}+{\mathit{\alpha }}_{\mathit{ca}}}{\mathit{R}\mathit{T}}\right)\mathit{F}{\mathit{\eta }}_{\mathit{an}}$$

(16)

$${\mathit{j}}_{\mathit{ca}}={\mathit{i}}_{\mathit{ca}}^{\mathit{ref}}\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right){\left(\frac{{\mathit{C}}_{{\mathit{O}}_2}}{{\mathit{C}}_{{\mathit{O}}_2}^{\mathit{ref}}}\right)}^{0.75}\mathit{exp}\left(-\frac{{\mathit{\alpha }}_{\mathit{ca}}\mathit{F}}{\mathit{R}\mathit{T}}{\mathit{\eta }}_{\mathit{ca}}\right)$$

(17)

Here, ${\mathit{i}}_{\mathit{an}}^{\mathit{ref}}$ and ${\mathit{i}}_{\mathit{ca}}^{\mathit{ref}}$ are the reference exchange current densities, ${\mathit{C}}_{{\mathit{H}}_2}^{\mathit{ref}}$ and ${\mathit{C}}_{{\mathit{O}}_2}^{\mathit{ref}}$ are reference molar concentrations, ${\mathit{\alpha }}_{\mathit{an}}$ and ${\mathit{\alpha }}_{\mathit{ca}}$ are the anodic and cathodic transfer coefficients, respectively, $\mathit{F}$ is Faraday’s constant, and $\mathit{R}$ is the universal gas constant. The local over-potential ${\mathit{\eta }}_{\mathit{an}}$ and ${\mathit{\eta }}_{\mathit{ca}}$ are:

$${\mathit{\eta }}_{\mathit{an}}={\mathit{\varphi }}_{\mathit{s}}-{\mathit{\varphi }}_{\mathit{e}},{\mathit{\eta }}_{\mathit{ca}}={\mathit{\varphi }}_{\mathit{s}}-{\mathit{\varphi }}_{\mathit{e}}-{\mathit{V}}_{\mathit{open}}$$

(18)

where the thermodynamic equilibrium potential ${\mathit{V}}_{\mathit{open}}$ is expressed as [10,11]:

$${\mathit{V}}_{\mathit{open}}=1.23-0.0009\times \left(\mathit{T}-298.15\right)$$

(19)

Energy conservation is as follows:

$$\frac{\partial }{\partial \mathit{t}}\left[{\left(\mathit{\rho }{\mathit{C}}_{\mathit{p}}\right)}_{\mathit{f}\mathit{l},\mathit{s}\mathit{l}}^{\mathit{e}\mathit{f}\mathit{f}}\mathit{T}\right]+\nabla \cdot \left[{\left(\mathit{\rho }{\mathit{C}}_{\mathit{p}}\right)}_{\mathit{f}\mathit{l}}^{\mathit{e}\mathit{f}\mathit{f}}{\mathit{u}}_{\mathit{g}}\mathit{T}\right]=\nabla \cdot \left({\mathit{k}}_{\mathit{f}\mathit{l},\mathit{s}\mathit{l}}^{\mathit{e}\mathit{f}\mathit{f}}\nabla \mathit{T}\right)+{\mathit{S}}_{\mathit{T}}$$

(20)

where ${\left(\mathit{\rho }{\mathit{C}}_{\mathit{p}}\right)}_{\mathit{f}\mathit{l},\mathit{s}\mathit{l}}^{\mathit{e}\mathit{f}\mathit{f}}$ represents the effective volumetric heat capacities, and ${\mathit{k}}_{\mathit{f}\mathit{l},\mathit{s}\mathit{l}}^{\mathit{e}\mathit{f}\mathit{f}}$ represents thermal conductivity of fluid and solid phase, respectively. The source term ${\mathit{S}}_{\mathit{T}}$ is comprised of the entropy change of the cell overall reaction, the Ohmic heat, and the latent heat attributed to the phase transition process in the CLs. In the GDLs, ${\mathit{S}}_{\mathit{T}}$ includes the Ohmic heat and the latent heat. Additionally, in the BPs, ${\mathit{S}}_{\mathit{T}}$ is solely contributed by the Ohmic heat. In this study, while the swelling of the membrane caused by water sorption is an endothermic process, the associated “swelling energy” is found to be negligible, and it can be safely disregarded [30]. Hence, it is reasonable to assume that the membrane water present in the “dissolved” phase is effectively identical to liquid water. Based on this assumption, the product water is also in the liquid phase, and the entropy change of the overall reaction is defined based on the liquid water product.

2.2. Water Phase Transition

In this study, during the cold start process, as mentioned in the previous literature [26], water is considered to exist in four states: vapor phase, liquid phase (supercooled water at subzero temperatures), membrane water, or ice. According to the previous assumption, membrane water is generated in the cathode CL during the cell operating period due to the electrochemical reaction. The phase transition between the membrane water and the water vapor is taken into account, as indicated by ① in Figure 2. This signifies the hydration/dehydration process of the electrolyte, which can be expressed as follows:

$${\mathit{S}}_{\mathit{m}\mathit{w}\leftrightarrow \mathit{v}}={\mathit{\alpha }}_{\mathit{m}\mathit{w}-\mathit{v}}\frac{{\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}}{\mathit{E}\mathit{W}}\left(\mathit{\lambda }-{\mathit{\lambda }}_{\mathit{e}\mathit{q}\mathit{u}\mathit{i}\mathit{l}}\right)\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)$$

(21)

Here, $\mathit{\lambda }$ is defined as the membrane water content, ${\mathit{\alpha }}_{\mathit{m}\mathit{w}-\mathit{v}}$ is the phase transition rate, and ${\mathit{\lambda }}_{\mathit{e}\mathit{q}\mathit{u}\mathit{i}\mathit{l}}$ is the equilibrium membrane water content. It can be expressed as a function of the water activity $\mathit{a}$ with the following relationship [29]:

$${\mathit{\lambda }}_{\mathit{e}\mathit{q}\mathit{u}\mathit{i}\mathit{l}}=\{\begin{array}{ll}0.043+17.81\mathit{a}-39.85{\mathit{a}}^2+36.0{\mathit{a}}^3& \mathit{a}\le 1\\ 14.0+1.4(\mathit{a}-1)& 1<\mathit{a}\end{array}$$

(22)

where the water activity $\mathit{a}$ is expressed as:

$$\mathit{a}=\frac{{\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}}{{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}}+2{\mathit{s}}_{\mathit{l}}$$

(23)

where ${\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}$ indicates the partial pressure of water vapor in the gas mixture, and ${\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}$ is the saturation vapor pressure over liquid water. During the initial cold start process, the membrane water content $\mathit{\lambda }$ gradually increases, leading to a corresponding rise in the partial pressure of the water vapor. As mentioned earlier, in this study, the icing phenomenon of supercooled water is not taken into consideration. When the membrane water reaches saturation, the water desorbed from the electrolyte exists as either liquid water or ice, as labeled with ② or ③ in Figure 2, respectively, and can be expressed as follows:

$${\mathit{S}}_{\mathit{m}\mathit{w}\to \mathit{l}}=\{\begin{array}{ll}{\mathit{\alpha }}_{\mathit{m}\mathit{w}\to \mathit{l}}\frac{{\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}}{\mathit{E}\mathit{W}}\left(\mathit{\lambda }-{\mathit{\lambda }}_{\mathit{e}\mathit{q}\mathit{u}\mathit{i}\mathit{l}}\right)& \mathit{a}\ge 1\\ 0& \mathit{a}<1\end{array},{\mathit{S}}_{\mathit{m}\mathit{w}\to \mathit{i}}=\{\begin{array}{ll}{\mathit{\alpha }}_{\mathit{m}\mathit{w}\to \mathit{i}}\frac{{\mathit{\rho }}_{\mathit{m}\mathit{e}\mathit{m}}}{\mathit{E}\mathit{W}}\left(\mathit{\lambda }-{\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}\right)& \mathit{\lambda }\ge {\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}\\ 0& \mathit{\lambda }<{\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}\end{array}$$

(24)

Here, ${\mathit{\alpha }}_{\mathit{m}\mathit{w}\to \mathit{l}}$ and ${\mathit{\alpha }}_{\mathit{m}\mathit{w}\to \mathit{i}}$ are defined as the desorption rate, and ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ is the saturated membrane water content, which denotes the maximum membrane water content in the electrolyte. When the membrane water desorbed from the electrolyte is assumed to be in the liquid phase (supercooled water below the freezing point), the saturation of membrane water occurs at ${\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}={\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}$. Based on Equations (22) and (23), the water activity value $\mathit{a}$ is $2{\mathit{s}}_{\mathit{l}}+1.0$, corresponding to a saturated membrane water content ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ of $14.0+2.8{\mathit{s}}_{\mathit{l}}$. When the membrane water desorbed from the electrolyte is assumed to be ice below the freezing point, its content would approach the maximum value with ${\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}={\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}$. ${\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}$ denotes the saturation vapor pressure over ice, which is smaller than ${\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}$ [31]. Consequently, according to Equation (23), the water activity $\mathit{a}$ is less than 1.0. The saturated membrane water content ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ will be lower than 14.0. When the temperature approaches zero, a is close to $1.0$. ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ tends to be 14.0, which is consistent with that presented in the previous literature [32]. It is worth noting that Equations (22) and (23) present a general model to calculate the saturated membrane water content no matter whether the water desorbed from the electrolyte is ice or supercooled water. Based on this model, in the following simulation, the influence of ice melting on the cell membrane water content during the cold-start process can be considered. Additionally, the phase transition process between water vapor and liquid water (condensation/evaporation), labeled as ④ in Figure 2, is incorporated with the following expression:

$${\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}=\{\begin{array}{ll}{\mathit{\alpha }}_{\mathit{c}\mathit{o}\mathit{n}}\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)\frac{{\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}-{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}}{\mathit{R}\mathit{T}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}& {\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}\ge {\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}\\ {\mathit{\alpha }}_{\mathit{e}\mathit{v}\mathit{a}\mathit{p}}\mathit{\epsilon }{\mathit{s}}_{\mathit{l}}\frac{{\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}-{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}}{\mathit{R}\mathit{T}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}& {\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}<{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}\end{array}$$

(25)

where ${\mathit{\alpha }}_{\mathit{c}\mathit{o}\mathit{n}}$ and ${\mathit{\alpha }}_{\mathit{e}\mathit{v}\mathit{a}\mathit{p}}$ are the phase transition rate of condensation and evaporation, and ${\mathit{M}}_{{\mathit{H}}_2\mathit{O}}$ is the molecular weight of water. The de-sublimate process between water vapor and ice is considered, labeled as ⑤ in Figure 2, while ignoring the sublimation of ice. The corresponding source term can be expressed as:

$${\mathit{S}}_{\mathit{v}\to \mathit{i}}=\{\begin{array}{ll}{\mathit{\alpha }}_{\mathit{d}\mathit{e}-\mathit{s}\mathit{u}\mathit{b}}\mathit{\epsilon }\left(1-{\mathit{s}}_{\mathit{l}}-{\mathit{s}}_{\mathit{i}}\right)\frac{{\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}-{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}}{\mathit{R}\mathit{T}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}& {\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}\ge {\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}\\ 0& {\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}<{\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}\end{array}$$

(26)

where ${\mathit{\alpha }}_{\mathit{d}\mathit{e}-\mathit{s}\mathit{u}\mathit{b}}$ is the de-sublimation rate. Due to the exothermic nature of the electrochemical reaction in the PEFC, the cell temperature increases during operation.

When the cell temperature rises above the freezing point, ${\mathit{T}}_{\mathit{f}}$, the ice within the cell starts to melt. The freezing temperature can be calculated as follows [31]:

$${\mathit{T}}_{\mathit{f}}=273.15\times \left(1+7.602\times {10}^{-7}\frac{\mathit{cos}\mathit{\theta }}{3.336{\mathit{\rho }}_{\mathit{i}}{\mathit{r}}_{\mathit{porous}}}\right)$$

(27)

where ${\mathit{r}}_{\mathit{porous}}$ is the pore radius of porous CLs and GDLs. The melting process labeled with ⑥ can be expressed as:

$${\mathit{S}}_{\mathit{i}\to \mathit{l}}={\mathit{\alpha }}_{\mathit{m}\mathit{e}\mathit{l}\mathit{t}\mathit{i}\mathit{n}\mathit{g}}\mathit{\epsilon }{\mathit{s}}_{\mathit{i}}{\mathit{\rho }}_{\mathit{i}}$$

(28)

where ${\mathit{\alpha }}_{\mathit{m}\mathit{e}\mathit{l}\mathit{t}\mathit{i}\mathit{n}\mathit{g}}$ is the melting rate from ice to liquid water. Note that this study does not consider the freezing phenomenon of supercooled water. The phase transition from liquid water to ice, as shown in Figure 2, is disregarded here. Clearly, the source terms (${\mathit{S}}_{\mathit{m}}$, ${\mathit{S}}_{{\mathit{H}}_2\mathit{O}}$, ${\mathit{S}}_{\mathit{m}\mathit{w}}$, ${\mathit{S}}_{\mathit{l}}$, and ${\mathit{S}}_{\mathit{i}}$) in the conservation equations incorporate the phase transition term (${\mathit{S}}_{\mathit{m}\mathit{w}\leftrightarrow \mathit{v}}$, ${\mathit{S}}_{\mathit{m}\mathit{w}\to \mathit{l}}$ ${\mathit{S}}_{\mathit{m}\mathit{w}\to \mathit{i}}$, ${\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}$, ${\mathit{S}}_{\mathit{v}\to \mathit{i}}$ or ${\mathit{S}}_{\mathit{i}\to \mathit{l}}$). Detailed expressions for each source term can be found in

Table 2. List of source terms.

Sources	Expression	Application Domain
${\mathit{S}}_{\mathit{m}}$	$-{\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}-{\mathit{S}}_{\mathit{v}\to \mathit{i}}+{\mathit{S}}_{\mathit{mw}\leftrightarrow \mathit{v}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}-\left({\mathit{j}}_{\mathit{an}}/2\mathit{F}\right){\mathit{M}}_{{\mathit{H}}_2}$	anode CL
	$-{\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}-{\mathit{S}}_{\mathit{v}\to \mathit{i}}+{\mathit{S}}_{\mathit{mw}\leftrightarrow \mathit{v}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}-\left({\mathit{j}}_{\mathit{ca}}/4\mathit{F}\right){\mathit{M}}_{{\mathit{O}}_2}$	cathode CL
	$-{\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}-{\mathit{S}}_{\mathit{v}\to \mathit{i}}$	GDLs
${\mathit{S}}_{\mathit{u}}$	$-\left({\mathit{\mu }}_{\mathit{g}}/{\mathit{K}}_{\mathit{g}}\right){\mathit{u}}_{\mathit{g}}$	CLs and GDLs
${\mathit{S}}_{\mathit{n}}$	${\mathit{S}}_{{\mathit{H}}_2}=-\left({\mathit{j}}_{\mathit{an}}/2\mathit{F}\right){\mathit{M}}_{{\mathit{H}}_2}$	anode CL
	${\mathit{S}}_{{\mathit{O}}_2}=-\left({\mathit{j}}_{\mathit{ca}}/4\mathit{F}\right){\mathit{M}}_{{\mathit{O}}_2}$	cathode CL
	${\mathit{S}}_{{\mathit{H}}_2\mathit{O}}=-{\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}-{\mathit{S}}_{\mathit{v}\to \mathit{i}}$	GDLs
${\mathit{S}}_{\mathit{mw}}$	$\nabla \cdot \left[\left(\mathit{\lambda }/8.8\mathit{F}\right){\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}\nabla {\mathit{\varphi }}_{\mathit{e}}\right]-{\mathit{S}}_{\mathit{mw}\leftrightarrow \mathit{v}}-{\mathit{S}}_{\mathit{mw}\to \mathit{l}}-{\mathit{S}}_{\mathit{mw}\to \mathit{i}}$ ${\mathit{j}}_{\mathit{ca}}/2\mathit{F}+\nabla \cdot \left[\left(\mathit{\lambda }/8.8\mathit{F}\right){\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}\nabla {\mathit{\varphi }}_{\mathit{e}}\right]-{\mathit{S}}_{\mathit{mw}\leftrightarrow \mathit{v}}-{\mathit{S}}_{\mathit{mw}\to \mathit{l}}-{\mathit{S}}_{\mathit{mw}\to \mathit{i}}$ $\nabla \cdot \left[\left(\mathit{\lambda }/8.8\mathit{F}\right){\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}\nabla {\mathit{\varphi }}_{\mathit{e}}\right]$	anode CL cathode CL membrane
${\mathit{S}}_{\mathit{l}}$	${\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}+{\mathit{S}}_{\mathit{i}\to \mathit{l}}+{\mathit{S}}_{\mathit{mw}\leftrightarrow \mathit{l}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}$	CLs
	${\mathit{S}}_{\mathit{v}\leftrightarrow \mathit{l}}+{\mathit{S}}_{\mathit{i}\to \mathit{l}}$	GDLs
${\mathit{S}}_{\mathit{i}}$	${\mathit{S}}_{\mathit{v}\to \mathit{i}}-{\mathit{S}}_{\mathit{i}\to \mathit{l}}+{\mathit{S}}_{\mathit{mw}\to \mathit{i}}{\mathit{M}}_{{\mathit{H}}_2\mathit{O}}$	CLs
	${\mathit{S}}_{\mathit{v}\to \mathit{i}}-{\mathit{S}}_{\mathit{i}\to \mathit{l}}$	GDLs
${\mathit{S}}_{\mathit{e}}$, ${\mathit{S}}_{\mathit{s}}$	${\mathit{S}}_{\mathit{e}}={\mathit{j}}_{\mathit{an}}$, ${\mathit{S}}_{\mathit{s}}=-{\mathit{j}}_{\mathit{an}}$	Anode CL
	${\mathit{S}}_{\mathit{e}}=-{\mathit{j}}_{\mathit{ca}}$, ${\mathit{S}}_{\mathit{s}}={\mathit{j}}_{\mathit{ca}}$	Cathode CL
${\mathit{S}}_{\mathit{T}}$	${\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{e}}\right|}^2+{\mathit{\kappa }}_{\mathit{s}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{s}}\right|}^2+{\mathit{j}}_{\mathit{an}}\left|{\mathit{\eta }}_{\mathit{an}}\right|+{\mathit{S}}_{\mathit{pc}}$	anode CL
	${\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{e}}\right|}^2+{\mathit{\kappa }}_{\mathit{s}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{s}}\right|}^2+{\mathit{j}}_{\mathit{ca}}\left|{\mathit{\eta }}_{\mathit{ca}}\right|-{\mathit{j}}_{\mathit{ca}}\mathit{T}\left(\mathit{\Delta }\mathit{S}/2\mathit{F}\right)+{\mathit{S}}_{\mathit{pc}}$	cathode CL
	${\mathit{\kappa }}_{\mathit{e}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{e}}\right|}^2$	membrane
	${\mathit{\kappa }}_{\mathit{s}}^{\mathit{eff}}{\left|\nabla {\mathit{\varphi }}_{\mathit{s}}\right|}^2+{\mathit{S}}_{\mathit{pc}}$	GDLs
	$\mathit{\lambda }=14.0$	BPs

, which also presents the corresponding application domain for each term. ${\mathit{S}}_{\mathit{pc}}$ denotes the latent heat function during the phase transition process.

2.3. Boundary Conditions and Numerical Implementation

Figure 1 illustrates the application of symmetric boundary conditions on both the top and bottom surfaces of the computational domain. The end surfaces of both the anode and cathode BPs (left and right surfaces of the computational domain) are considered adiabatic. Additionally, for the galvanostatic condition, a constant current density, $\mathit{I}$, is applied at the end surface of the cathode BP, while the potential of the solid phase ${\mathit{\varphi }}_{\mathit{s}}$ is set to 0 at the end surface of the anode BP. The mass flow rate and mole fraction of each gas species, such as H₂, O₂, and H₂O, at both the anode and the cathode inlets of the GC, are determined based on their respective stoichiometric ratios and the operating current density $\mathit{I}$. The corresponding gas temperature is assumed to be equal to the ambient temperature, and the relative humidity is set to zero. A pressure outlet condition, representing the operating pressure of the PEFC, is applied at the outlets of the GCs. Since the co- and counter-flow configurations, as well as the airflow rate, have been shown not to significantly affect ice accumulation behavior [7], this study only considers the co-flow configuration and ignores variations in the stoichiometric ratios of gas.

The governing equations are discretized and solved using the commercially available computational fluid dynamics program FLUENT version 22. The source terms listed in Table 2 are implemented via a set of user-defined functions (UDFs). Spatial discretization is performed using the second-order upwind scheme, and convergence is accelerated using the algebraic multigrid (AMG) method. A grid independence study is conducted, and the simulation employs a total of 3,350,000 structured mesh elements, refined in MEM, CLs, and GDLs. The simulations are performed on a supercomputer equipped with 256 CPUs clocked at 2.35 GHz. The time step $\mathit{\Delta }\mathit{t}$ is set to be 10⁻⁴ s. Convergence criteria for the flow field and all species residuals are set to 10⁻⁶.

3. Results and Discussion

To analyze the cold-start behavior of a PEFC under various operating conditions, we defined five simulation cases differing in ambient temperature (i.e., 258 K and 263 K), state of water (i.e., supercooled water or ice), and operating current density (i.e., 0.3 A cm⁻² and 0.6 A cm⁻²). Specific details are provided in

Table 3. Simulation cases.

Case No.	Ambient Temperature (K)	Water State	Current Density (A cm−2)
1	258	Ice	0.3
2	258	Supercooled water	0.3
3	263	Ice	0.3
4	263	Supercooled water	0.3
5	263	ice	0.6

. The initial membrane water content λ in the electrolyte phase is assumed to be 5.0. The stoichiometric ratios of dry gas are set to 2.0 for both the anode and cathode, with zero relative humidity. Pressures at the outlets of both the anode and cathode GCs are maintained at 1.0 atm. As our focus is on the cold-start process, the simulation is terminated either when the cold-start process fails (cell voltage drops suddenly) or when it succeeds (cell temperature reaches above 283 K).

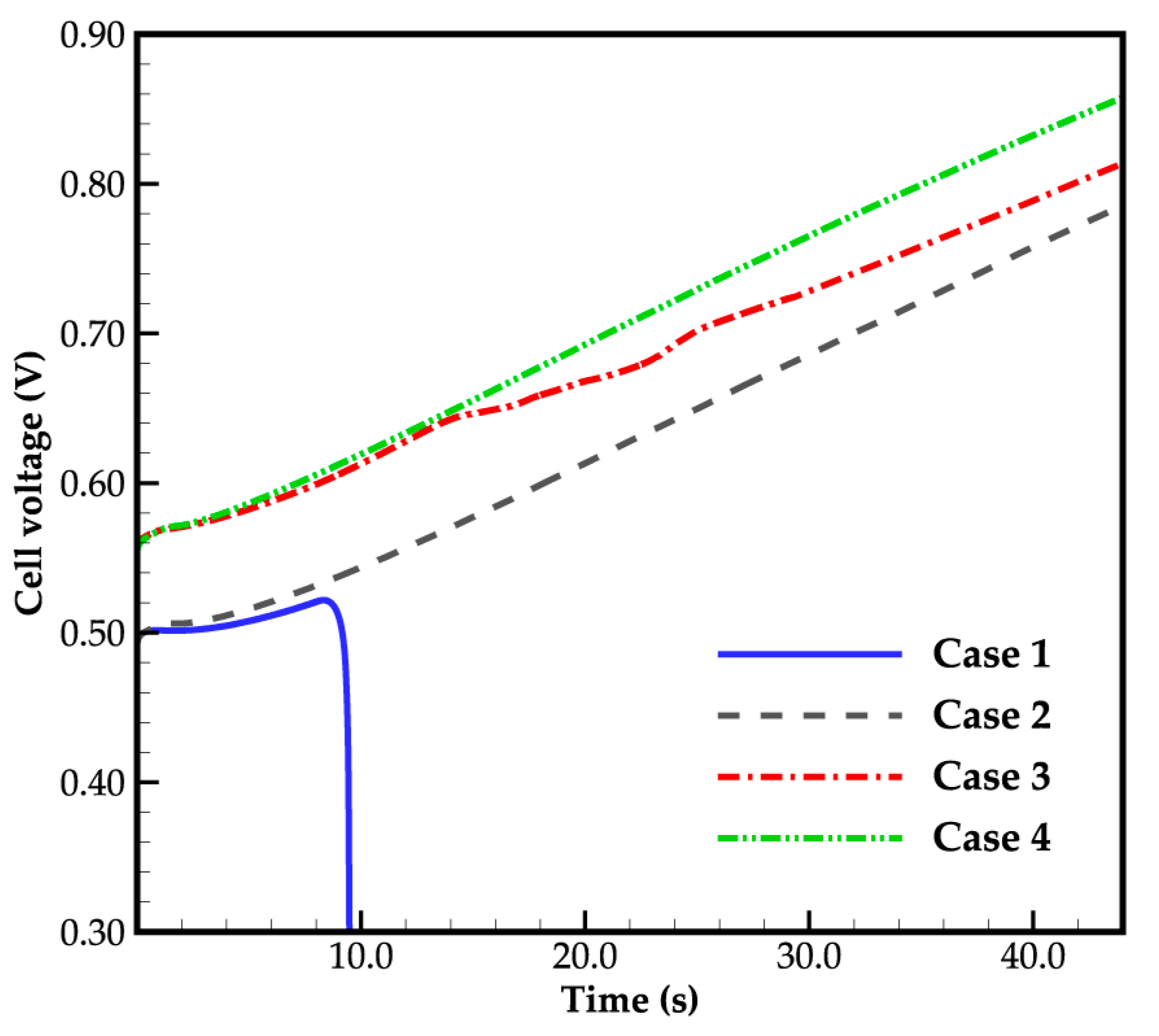
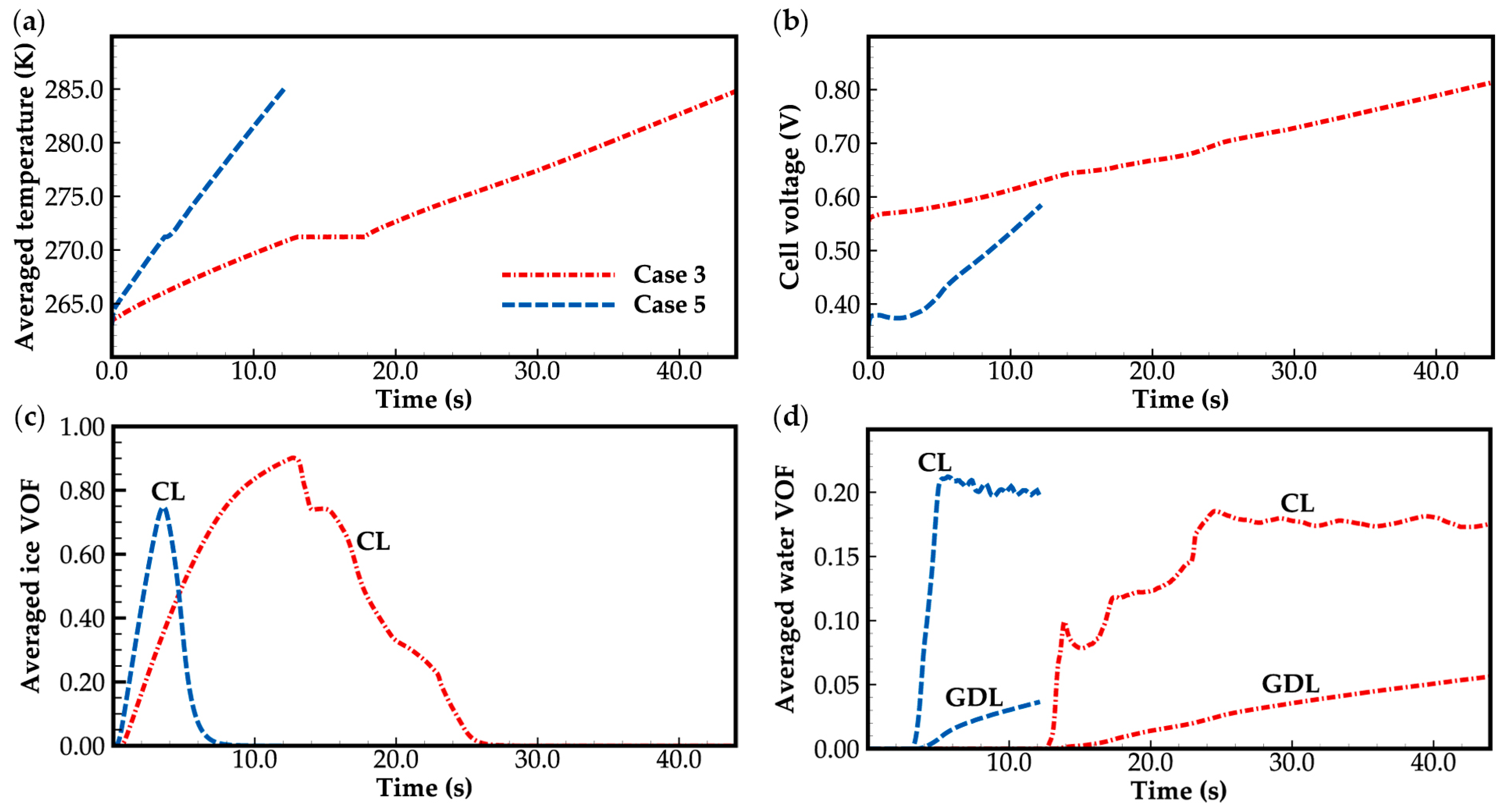
3.1. Effect of Ambient Temperature on Cold-Start Process

Figure 3 depicts the evolution curves of cell voltage for different cases, clearly illustrating the impact of ambient temperature on the initial cell voltage. With decreasing ambient temperature from 263 K to 258 K, the electrochemical reaction rate weakens, as indicated by Equations (16) and (17), leading to a reduction in initial cell voltage from 0.554 V to 0.492 V. However, as the cell continues to operate, the cell voltage gradually increases.

Furthermore, in Figure 3, it can be found that in case 1, where the ambient temperature is 258 K and the water desorbed from the electrolyte is in a state of ice, the cell voltage increases gradually as it runs, and starts to drop after 8.3 s of startup, resulting in a failed cold-start process of the cell. In case 2, when the water desorbed from the electrolyte is in the supercooled water state, the situation differs significantly. The cell voltage continues to rise, and the cold-start process appears to be successful. Similarly, in case 3 and case 4, with an ambient temperature of 263 K, the cold-start process is also successful, regardless of whether the water desorbed from the electrolyte is in the form of ice or supercooled water. Additionally, the cell voltage in case 3 is lower than that in case 4, and the gap increases when the cell averaged temperature rises above the freezing point. This will be discussed in detail later.

Overall, the evolution of cell voltage during the cold-start process under various operating conditions is influenced by the cell temperature, distribution of ice or supercooled water, and the content of membrane water. The following sections will provide a detailed analysis of these factors. Before that, it is worth noting that in the industry test, it is customary to set the cold-start temperature below 243 (or even lower). However, in Figure 3, the cell cold-start fails when the cold-start temperature is 258 K and the corresponding water desorbed from the electrolyte is ice. Therefore, the case of cold-start with lower temperature is not further discussed.

Figure 4 displays the evolution curves of cell averaged temperature and the averaged volume of fraction (VOF) of ice and water in CLs and GDLs for case 1 and case 3. In case 1, when the cell averaged temperature remains below the freezing point, the water desorbed from the electrolyte is assumed to be kept in a state of ice. As shown in Figure 4a,b, ice accumulates in the cathode CL as the cell continues to operate, and the cell averaged temperature increases at the same time. No ice is observed in the anode side and the cathode GDL. In case 1, combing Figure 3 and Figure 4, when the averaged ice VOF reaches close to 1.0, the cell voltage drops suddenly. The cell averaged temperature only increases to 264.2 K, which is far below the freezing point of 271.2 K defined in Equation (27). This indicates that the failure of the cold-start process is attributed to the clogging of gas transportation caused by ice occupying the pores in the cathode CL. This can also be observed in Figure 5a, where the ice VOF in the middle of cathode CL increases as the cell operating time extends. The cell voltage keeps dropping when the ice VOF is close to 1.0. Notably, the ice VOF under the rib remains consistently higher than under the GC, contributing to the hindrance in gas transport. However, in case 3, as depicted in Figure 4a,b, unlike case 1, the cell averaged temperature approaches the freezing point when the averaged ice VOF reaches close to 1.0. This indicates that the ice accumulated in the pores of the cathode CL undergoes melting. The waste heat released during cell operation is absorbed by the phase transition process. The cell averaged temperature is maintained at the freezing point, and the water in the cathode CL tends to be expelled into the cathode GDL due to the presence of surface tension. Therefore, the averaged ice VOF in cathode CL decreases, and the corresponding averaged water VOF in the cathode CL and GDL rises, as illustrated in Figure 4b,c. As a result, the transportation of gas is not clogged, allowing the cell to continue operating. This indicates that the success of the cell cold-start process relies on the cell temperature approaching the freezing point before the ice generated by the electrochemical reaction completely blocks gas transport in the cathode CL.

In this study, it is worth noting that the temperature of the inlet gas is set to be the ambient temperature, which is considerably lower than the freezing point. Throughout the cold-start process, the temperature of the cell inlet region remains below the freezing point. Consequently, in case 3, the ice located in the inlet region is the last to melt, as depicted in Figure 5b at t = 22 s. The corresponding averaged ice VOF in cathode CL drops to ${\mathit{s}}_{\mathit{i}}=0.268$. This phenomenon aligns with observations in the previous literature [7]. Complete melting of ice in the cell occurs when the cell averaged temperature is almost 4.5 K above the freezing point 271.2 K. After this, the water VOF under the rib continues to be larger than that under the GC, as illustrated in Figure 5b at t = 30 s and t = 42 s.

Figure 6 illustrates the evolution curves of cell averaged temperature and supercooled water/water in cathode CL and GDL for case 2 and case 4. In both cases, the water desorbed from the electrolyte is assumed to be in a state of supercooled water when the cell temperature is below the freezing point. As illustrated in Figure 6a, throughout the cold-start process, the cell averaged temperature shows a linear growth with time. Water in the cathode CL can be expelled into the cathode GDL. The transportation of gas in the cathode side remains unimpeded. The cell cold-start process is consistently successful, regardless of whether the ambient temperature is 258 K or 263 K. In Figure 6b, the evolution curves of averaged VOF of supercooled water/water in cathode CL exhibit two distinct stages during the cold-start period. In the first 10 s, a rapid growth rate of supercooled water/water is observed because the vapor saturation pressure is low due to the low cell temperature, and most of the product water precipitates in the cathode CL. However, as the cell temperature increases, the vapor saturation pressure also increases significantly, allowing more product water to be stored in the vapor phase. Consequently, the growth rate of supercooled water/water in cathode CL slows down, and the averaged VOF of water exhibits fluctuations. According to Equations (9) and (10), we know that the transport of water in CL and GDL is primarily driven by the additional pressure caused by surface tension. As the water VOF increases, the effective diffusivity of water $(-\mathit{\rho }{\mathit{K}}_{\mathit{l}}/{\mathit{\mu }}_{\mathit{l}})\cdot (\mathit{d}{\mathit{p}}_{\mathit{l}}/\mathit{d}{\mathit{s}}_{\mathit{l}})$ will grow rapidly. In this study, the initial averaged water VOF in the GDLs, treated as the residual water, is assumed to be 0.0075. The corresponding effective diffusivity of water is 7.26 × 10⁻¹⁰ kg m⁻¹s⁻¹. It is much lower than 3.53 × 10⁻⁸ kg m⁻¹s⁻¹, where the averaged water VOF ${\mathit{s}}_{\mathit{l}}$ in GDL is around 0.02 (at time t = 10 s). This indicates that the diffusing ability of water in CL and GDL will be enhanced swiftly with the increase in the water VOF.

Figure 7 displays the distribution of water VOF across the cathode CL and GDL in case 2. Notably, the water VOF under the rib is higher than under the GC, e.g., at t = 32.3 s. As the cell continues to operate, water accumulates in both the cathode CL and GDL. When the water VOF reaches a relatively high value, water under the rib experiences breakthrough and spreads in the cathode GDL earlier than the water under the GC. Subsequently, water in the cathode CL rapidly spreads to the cathode GDL, e.g., at t = 37.9 s and 38.9 s. This leads to a decrease in the averaged water VOF in the cathode CL, e.g., at t = 39.1 s in Figure 7. Consequently, the effective diffusivity of water decreases. The water accumulation process repeats, and when the water VOF approaches a relatively high value, water under the rib breakthrough occurs again, leading to its spread in the cathode GDL. This phenomenon accounts for the fluctuations observed in the evolution curve of averaged water VOF in the cathode CL, as illustrated in Figure 6b. However, as listed in Table 1, the porosity and thickness of cathode GDL are much larger than those of cathode CL. Therefore, as illustrated in Figure 6b, no obvious fluctuation can be observed during the evolution of the averaged water VOF in cathode GDL. It is important to note that the diffusion of water in real porous media is closely related to the structure and spatial distribution of the pores. Therefore, the transportation of water in the cathode CL and GDL during the cold-start process of a real PEFC is expected to be more complex.

Furthermore, in Figure 6b, it can also be observed that in case 2, the averaged water VOF in both CL and GDL are higher compared to those in case 4. In this study, for both cases 2 and 4, the initial membrane water content $\mathit{\lambda }$ is set to 5.0, and the operating current density is 0.3 A cm⁻². However, in case 2, the ambient temperature is 258 K, which is lower than 263 K in case 4. According to Equation (8), the diffusion coefficient of membrane water in case 2 is smaller than that in case 4. The corresponding back diffusion of membrane water from the cathode CL to the MEM and anode CL in case 2 may be weaker than that in case 4. This can be observed obviously in Figure 8. As a result, the product water absorbed by the membrane and the anode CL in case 2 may be less than that in case 4, despite the membrane water content in the cathode CL almost approaching saturation ($\mathit{\lambda }=14.0$). Consequently, in case 2, more product water will precipitate into the cathode CL and diffuse into the cathode GDL. A similar phenomenon can also be observed in case 1, where the ambient temperature is 258 K. It is lower than 263 K in case 4. As depicted in Figure 4b, compared to case 3, more ice precipitates in the cathode CL in case 1 and acts to hinder gas transportation. This is also one of the reasons for the cell cold-start failure in case 1. Therefore, it can be concluded that during the cold-start process, the membrane water diffusion rate will be slower if the cell temperature is lower. This will lead to a decrease in the water storage capacity of the membrane, and more ice or supercooled water will be precipitated. If the desorbed membrane water is in an ice state, the lower the startup temperature, the more possible it is that cold-start failure will occur due to the ice blockage.

Figure 8 shows the evolution curves of cell averaged membrane water content. It is evident that the averaged membrane water contents in the cathode CL reach saturation ($\mathit{\lambda }=14.0$) rapidly during the initial cold-start stage, while the averaged membrane water content in the MEM and anode CL decreases. This suggests that the product water is effectively absorbed by the cathode CL electrolyte. Conversely, the water absorption by MEM and anode CL is not significant due to sluggish water diffusion through the MEM at temperatures below the freezing point, as mentioned before. In this study, the anode CL becomes dry due to the inlet gas having zero relative humidity. Nonetheless, as the cell continues to operate, the averaged membrane water content in the MEM and anode CL gradually increases. According to Equation (15), the ionic conductivity of the electrolyte tends to increase, leading to an increase in cell voltage, as illustrated in Figure 3. It is worth noting that if the initial membrane water content is too low or the drying effect of the anode gas on the anode CL is too strong, it may lead to dehydration of the anode CL during the initial cold-start stage. This may also lead to failure of the cell cold-start process.

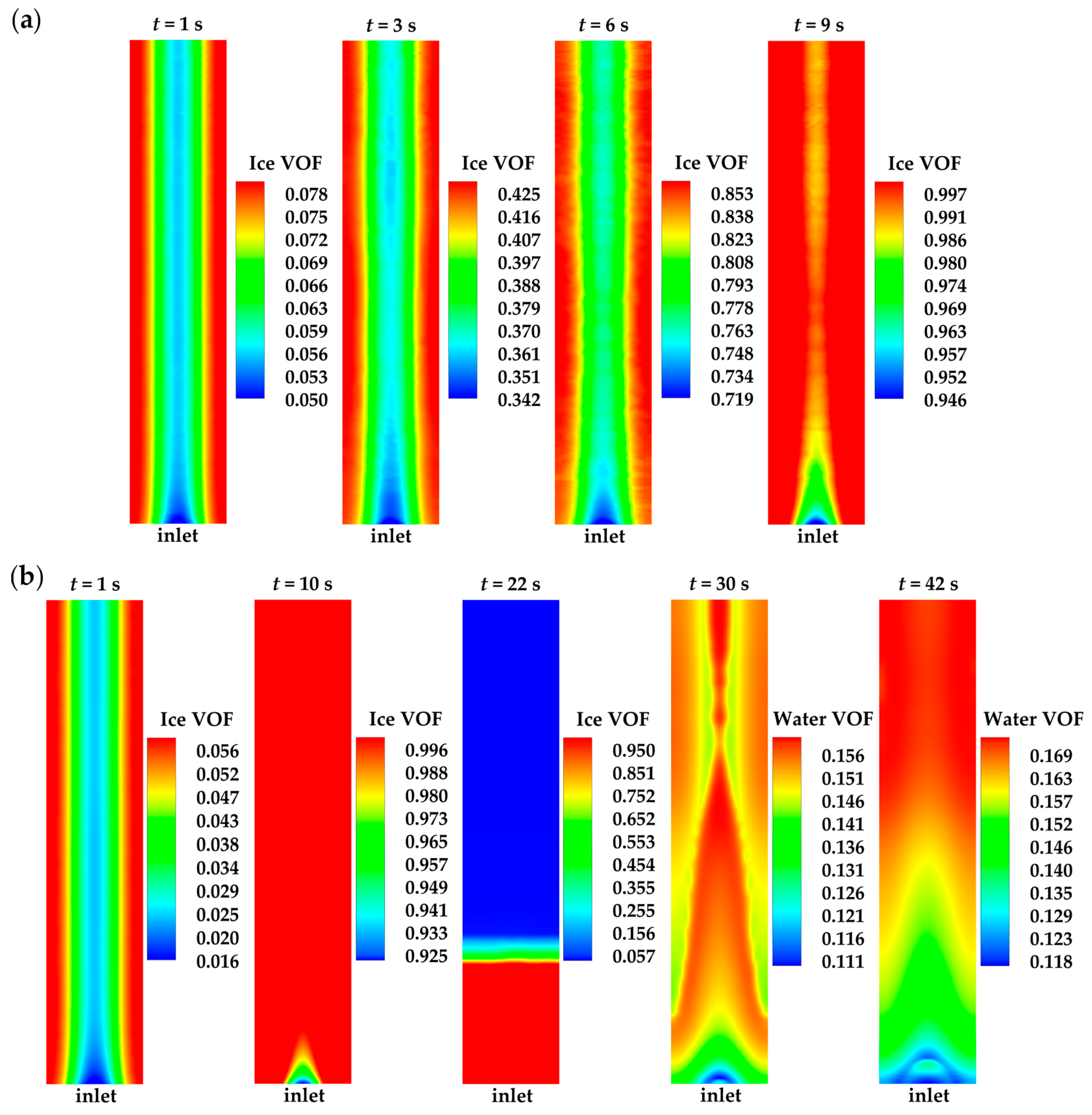
3.2. Effect of the State of Desorbed Water on Cold-Start Process

Based on the previous section, we know that when the water desorbed from the electrolyte is in the ice state and the ambient temperature is low (i.e., 258 K), the cold-start process fails, as illustrated in Figure 3. However, when the ambient temperature is slightly higher (i.e., 263 K), regardless of whether the water desorbed from the electrolyte is in the supercooled water state or the ice state, the cold-start process is successful. In this section, we will conduct an in-depth examination of case 3 and case 4 with an ambient temperature of 263 K. The primary concern will be the impact of the state of the desorbed water on the cell cold-start process. As evident from the source terms in the energy conservation Equation (20), which is listed in Table 2, heat generation is influenced by factors such as entropic heat of reactions, irreversibility of electrochemical reactions, ohmic resistance, and phase transition. In case 3, when the cell averaged temperature falls below the freezing point, the water desorbed from the electrolyte transforms into ice. This is an exothermic process, meaning it releases heat. As a result, the averaged temperature of the cell in case 3 is slightly higher than in case 4, as depicted in Figure 9a. When the cell averaged temperature rises above the freezing point, the situation is reversed. The ice-melting phase transition process comes into play. It is an endothermic process and causes the cell averaged temperature in case 3 to be lower than in case 4. Consequently, the cell voltage in case 3 consistently remains smaller than that in case 4, as illustrated in Figure 9b or Figure 3.

Figure 10 displays the evolution curves of averaged membrane water content in case 3. As a comparison, the corresponding curves in case 4 shown in Figure 8 are also given again. Similar to Figure 8, during the initial cold-start stage, the product water is efficiently absorbed by the cathode electrolyte, resulting in a rapid increase in membrane water content within the cathode CL. However, in case 3, the water desorbed from the electrolyte exists in the form of ice. If the cell temperature is below freezing point (t < 13 s in Figure 10), the saturated membrane water content ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$, which can be attained with the condition ${\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}={\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{i}}$ and increases with rising cell temperature [26], is lower than that in case 4, as depicted in Figure 10c. This leads to a lower membrane water content in MEM compared to that in case 4, as shown in Figure 10b. Afterward, as the cell temperature in case 3 reaches the freezing point, ${\mathit{p}}_{\mathit{v}\mathit{a}\mathit{p}}$ approaches ${\mathit{p}}_{\mathit{s}\mathit{a}\mathit{t},\mathit{l}}$ and the value of ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ becomes close to 14. However, due to the presence of the ice melting process, the cell temperature in case 3 is depicted as lower than that in case 4, as shown in Figure 9a. Consequently, the humidity of the cathode gas in case 3 is also lower than that in case 4, resulting in less water being expelled from the cell by the gas. As a result, the averaged membrane water contents in the cathode CL and MEM are higher in case 3 compared to those in case 4, as illustrated in Figure 10b,c. As the cell continues to operate, its temperature increases. Once the cell temperature surpasses the freezing point and the ice completely melts, the evolution of averaged membrane water content in cathode CL and MEM in case 3 tends to align with that observed in case 4.

It is worth noting that in case 3, the cell exhibits a distinct behavior during the cold-start process compared to that in case 4. When the cell temperature reaches the freezing point, in case 3, the averaged membrane water content remains high, resulting in relatively low membrane resistance (or high ionic conductivity). However, at the same time, the cell temperature rises at a slower pace due to the endothermic process of ice melting. Consequently, the electrochemical performance of the cell during the cold-start process in case 3 is relatively poor compared to case 4. Specifically, the cell voltage in case 3 is lower than that in case 4, as illustrated in Figure 3.

3.3. Effect of Current Density on Cold-Start Process

Figure 11 shows the evolution curves of cell averaged temperature, cell voltage, and averaged VOF of ice (or water) in case 5. For comparison, corresponding curves in case 3 are also presented. As the cell operation current increases from 0.3 A cm⁻² to 0.6 A cm⁻² in case 5, the cell voltage decreases. However, according to the source terms in the energy conservation Equation (20), more heat will be generated during the cold-start process. Compared to case 3, case 5 shows a significantly higher rate of increase in the cell averaged temperature, as shown in Figure 11a. The corresponding growth rate of cell voltage is also faster, as illustrated in Figure 11b. Consequently, the averaged VOF of ice accumulated in cathode CL before melting is smaller than that in case 3, and the corresponding ice melting process is faster, as illustrated in Figure 11c. This facilitates the cold-start process of the cell. In Figure 11d, since the water in the cathode CL cannot be transported to the GDL in time, in case 5, the averaged VOF of water in the cathode CL is higher than that in case 3.

Figure 12 shows the evolution curves of averaged membrane water content in case 5. As a comparison, the corresponding curves in case 3, shown in Figure 10, are given again. During the initial cold-start stage, the averaged membrane water content within the cathode CL increases quickly, and the saturated membrane water content ${\mathit{\lambda }}_{\mathit{s}\mathit{a}\mathit{t}}$ approaches the maximum more rapidly than that in case 3. Meanwhile, as illustrated in Figure 12a,b, the averaged membrane water content in anode CL and MEM in case 5 decreases drastically and becomes lower than that in case 3. This indicates that the membrane water in anode CL and MEM is carried to the cathode CL due to the strong electroosmosis effect at high operation current. The anode CL may become dehydrated during the cold-start process. This results in a decrease in ionic conductivity in anode CL and membrane, and may also lead to a failure of the cold-start process. Moreover, in case 5, as shown in Figure 12b, during the cold-start process, though the averaged membrane water content in MEM shows an overall downward trend due to the strong electroosmosis, it can also be found that the average membrane water content in MEM tends to rise as ice melts. This is consistent with that observed in case 3. It can be concluded that this phenomenon is induced by the coupling effect of constant water generation inside the cell (due to the electrochemical reaction) and the constant humidity of the gas (due to constant temperature) when the ice inside the cell melts.

In Figure 12c, it can also be found that the averaged membrane water content in cathode CL reaches its peak in both case 3 and case 5 when the ice in the cathode CL is almost completely melted. After that, it gradually decreases. The corresponding value of the maximum in case 5 is higher than that in case 3. Physically, this is attributed to the fact that in case 5, the water VOF in cathode CL is higher than that in case 3, as illustrated in Figure 11d. According to Equations (22) and (23), we know that the membrane water content in case 5 would be greater than that in case 3. It is worth noting that in case 5, though the membrane water content in cathode CL arrives at saturation, the membrane water content in anode CL is relatively low. This reminds us that the cold-start process could fail due to the dehydration of anode CL, and it can be improved by anode humidification.

4. Conclusions

In this paper, we present a transient three-dimensional multiphase model designed to simulate the cold-start process of a PEFC from subzero temperatures. The product water resulting from electrochemical reactions is assumed to dissolve in the electrolyte and to be treated as membrane water. Two possible phase states for the water desorbed from the electrolyte are considered when the cell temperature is below freezing point: either supercooled water or ice. We try to disclose the influence of the phase state of water on the fuel cell cold-start process. To ensure comprehensive analysis, we conduct numerical simulations with varying ambient temperatures and operating currents. For the sake of brevity, when the cell temperature rises beyond the freezing point, specifically 283 K, the cold-start process is assumed to be successful and the simulation is ceased. The results show that the cell cold-start fails when the cold-start temperature is 258 K and the water desorbed from the electrolyte is ice. Therefore, the case of cold-start with a lower temperature is not further discussed. The major conclusions drawn from the simulation results are as follows:

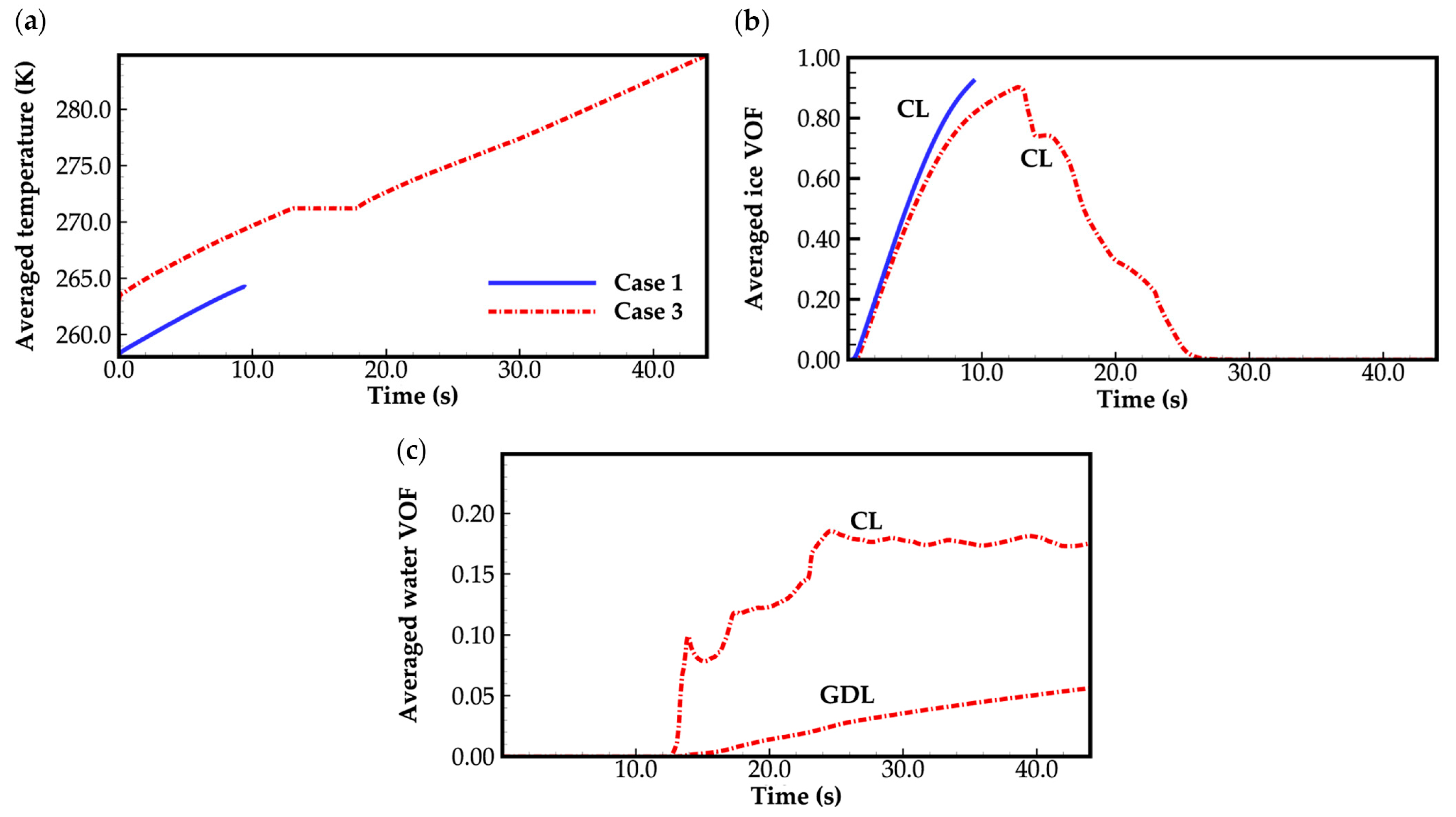
• The model successfully captures the evolution of cell voltage, temperature, membrane water content, and the averaged volume fraction of supercooled water or ice during the cold-start process. In cases where the water desorbed from the electrolyte is in the form of ice and the ambient temperature is sufficiently low, the cold-start process may fail due to ice blockage in the cathode CL. However, when the water desorbed from the electrolyte exists as supercooled water, it can diffuse from the cathode CL to the GDL, preventing clogging of the cathode CL and enabling a successful cold-start process.
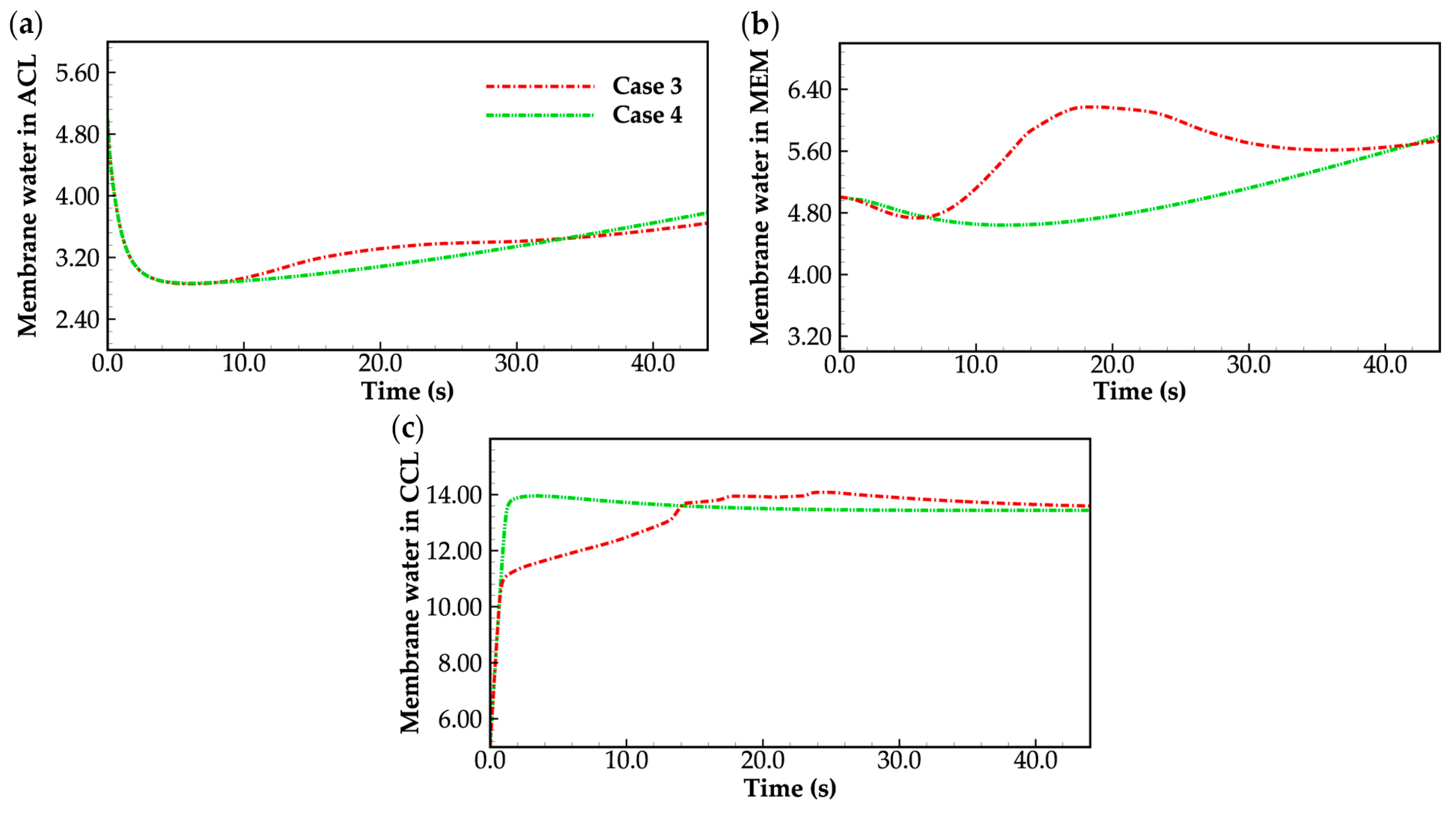
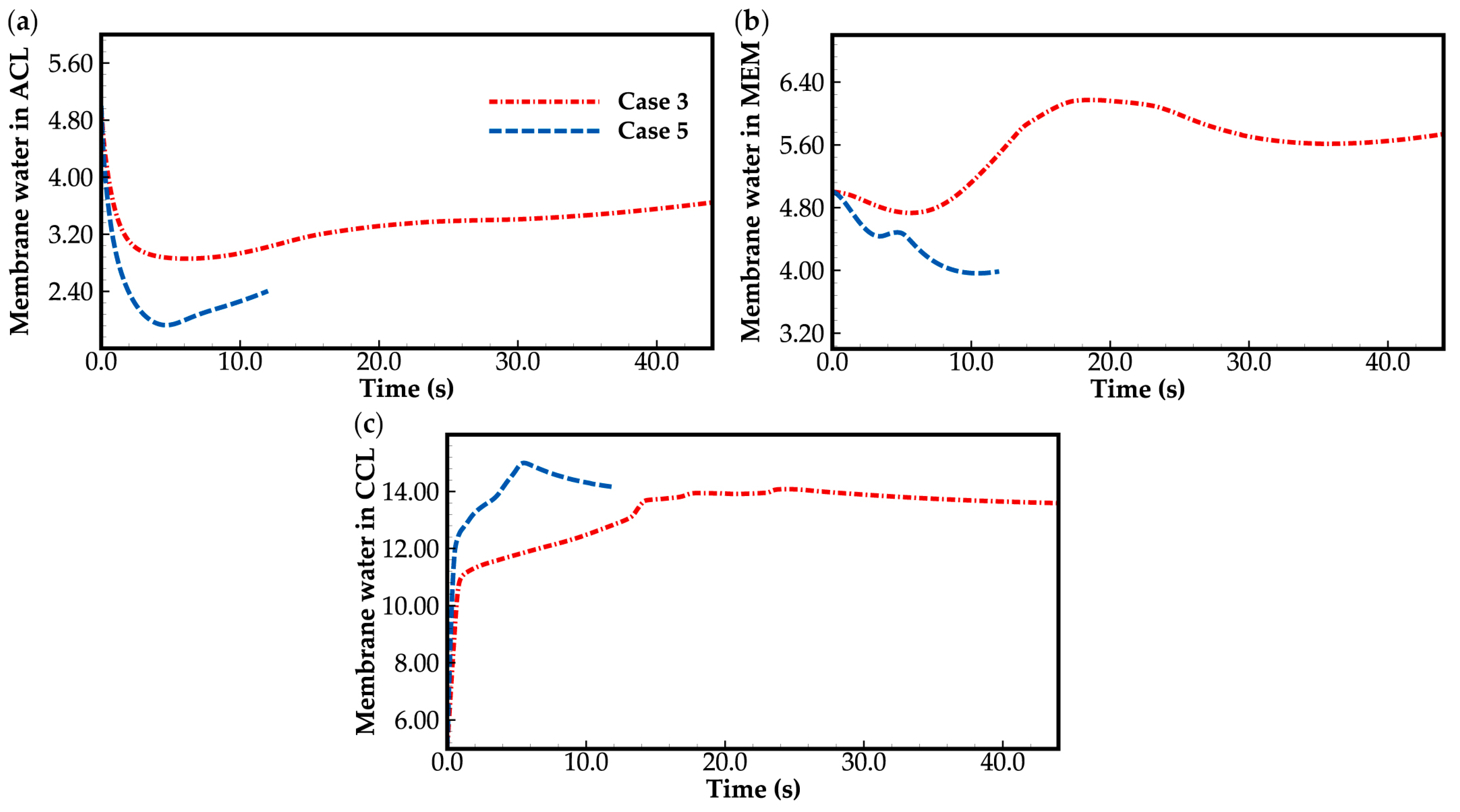
• During the initial stage of the cold-start process, the membrane water content in the cathode CL increases and reaches saturation rapidly, while the membrane water contents in the anode CL and MEM exhibit a decreasing trend. As the cell continues to operate, the membrane water contents in the anode CL and MEM gradually start to increase. Consequently, if the initial membrane water content is relatively low, it may lead to dehydration of the anode CL during the initial cold-start stage.
• In contrast to the cold-start process where the water desorbed from the electrolyte exists in a supercooled state, when the water desorbed from the electrolyte is in the form of ice, it is found that the membrane will become wetter due to the melting process of ice within the cell. We expect this phenomenon to be verified by the cell impedance test in the following study.
• In the case that the anode CL does not dehydrate (e.g., anode humidification), a larger operation current is beneficial to the cold-start process. This is due to the large ohmic heat it causes, which is helpful to increase the rate of cell temperature rise.