Temperature Distribution in a Finite-Length Cylindrical Channel Filled with Biomass Transported by Electrically Heated Auger
Abstract
:1. Introduction
2. Mathematical Formulation of the Problem
3. Analytical Determination of the Solution of the Problem
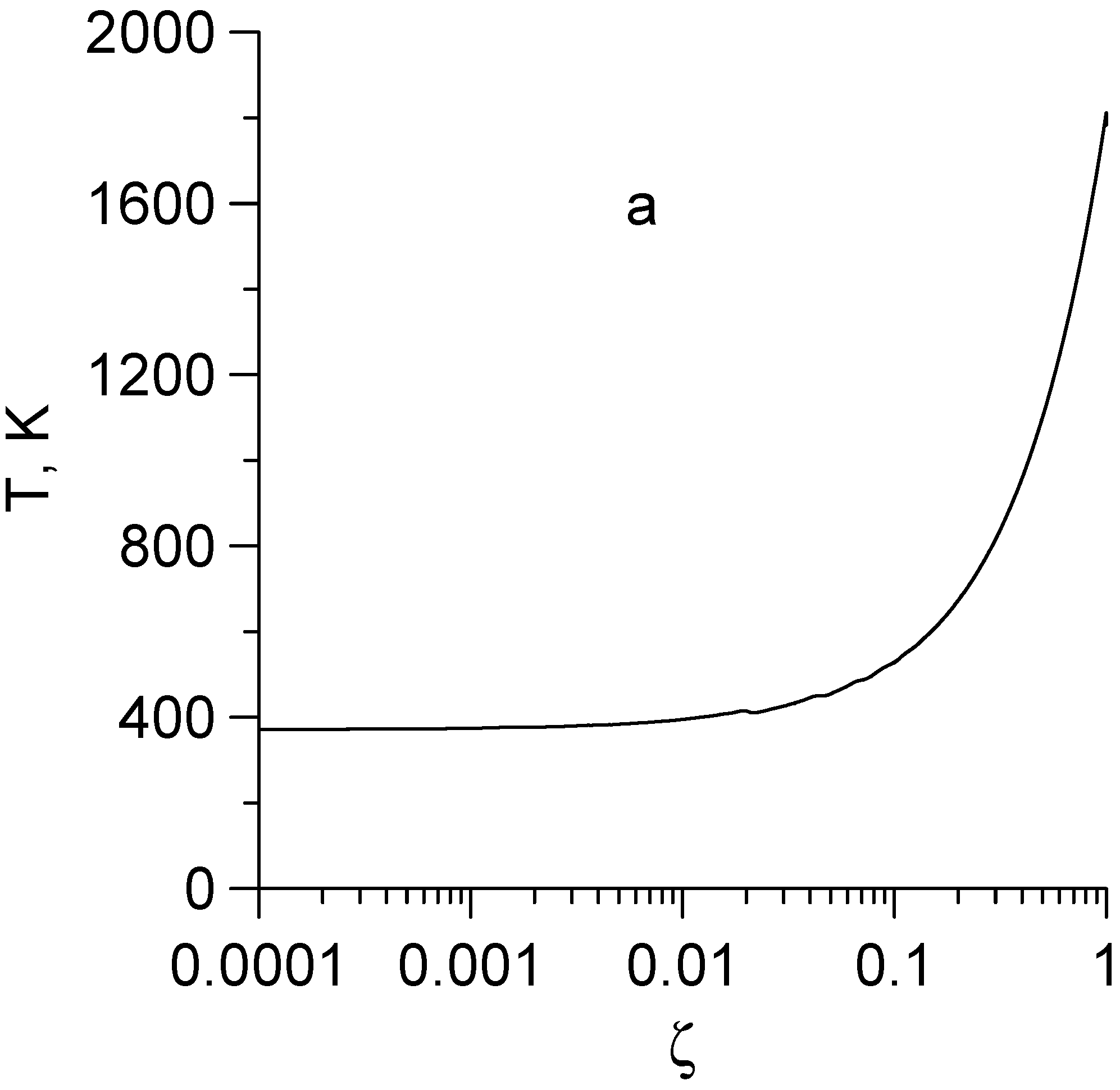
4. Analysis of the Numerical Results
5. Conclusions
- The non-stationary temperature field in a reactor of finite length has a short-term transition period beyond which the temperature is quasi-stationary. The quasi-stationary part of the solution against a background of constant temperature contains quasi-harmonic oscillations, the amplitude of which is determined by the rotation period of the screw and the superposition of heat flows reflected on the thermally insulated side surfaces and on the flat surfaces of the reactor inlet and outlet, where heat exchange with the external environment takes place. The amplitude of these oscillations is very weak, of the order of 0.5 K, but these oscillations reflect the specifics of the screw action.
- Globally, the temperature is characterized by its increase along the screw axis in the direction of biomass linear movement. This effect, known from the experimental and theoretical literature [19,23,24], received a mathematical justification here. In addition, due to the movement of biomass, the solution of the problem has the character of a boundary layer, as a result of which the axial dependence of the temperature in the channel at a distance of the order of 1/b = 2a/v0 from its entrance and exit has a slightly different form than on the main part of the reactor.
- Taking into account the speed of biomass movement leads to the emergence of a large parameter β = bL (of the order of 103), which allows approximately summing a weakly convergent series by trigonometric functions and thereby regularizing solutions containing the exponent eβ multiplied by these series. Failure to use this procedure prevents numerical calculations. However, it should be noted that this is not the only method of regularization; other methods are also possible.
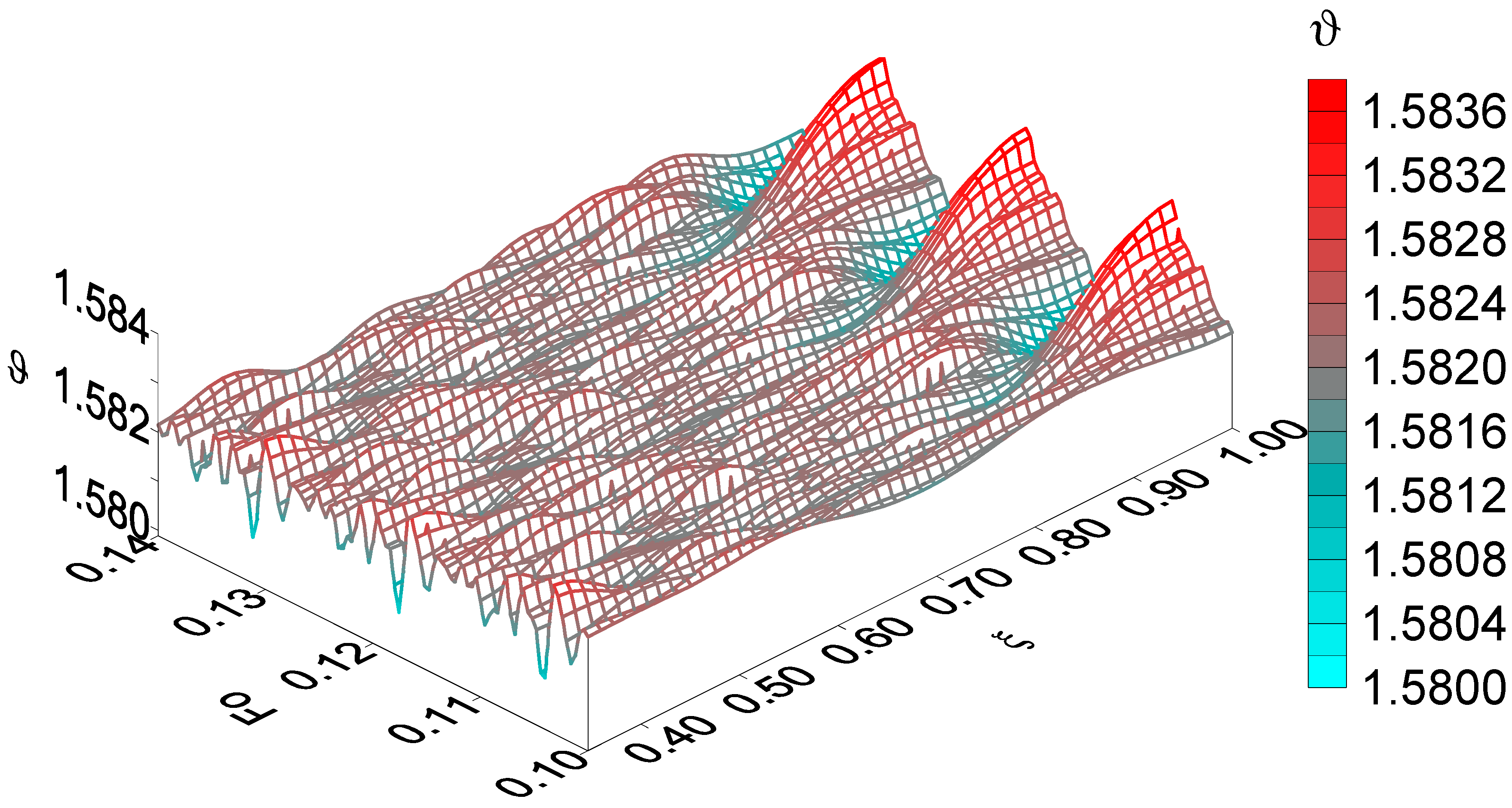
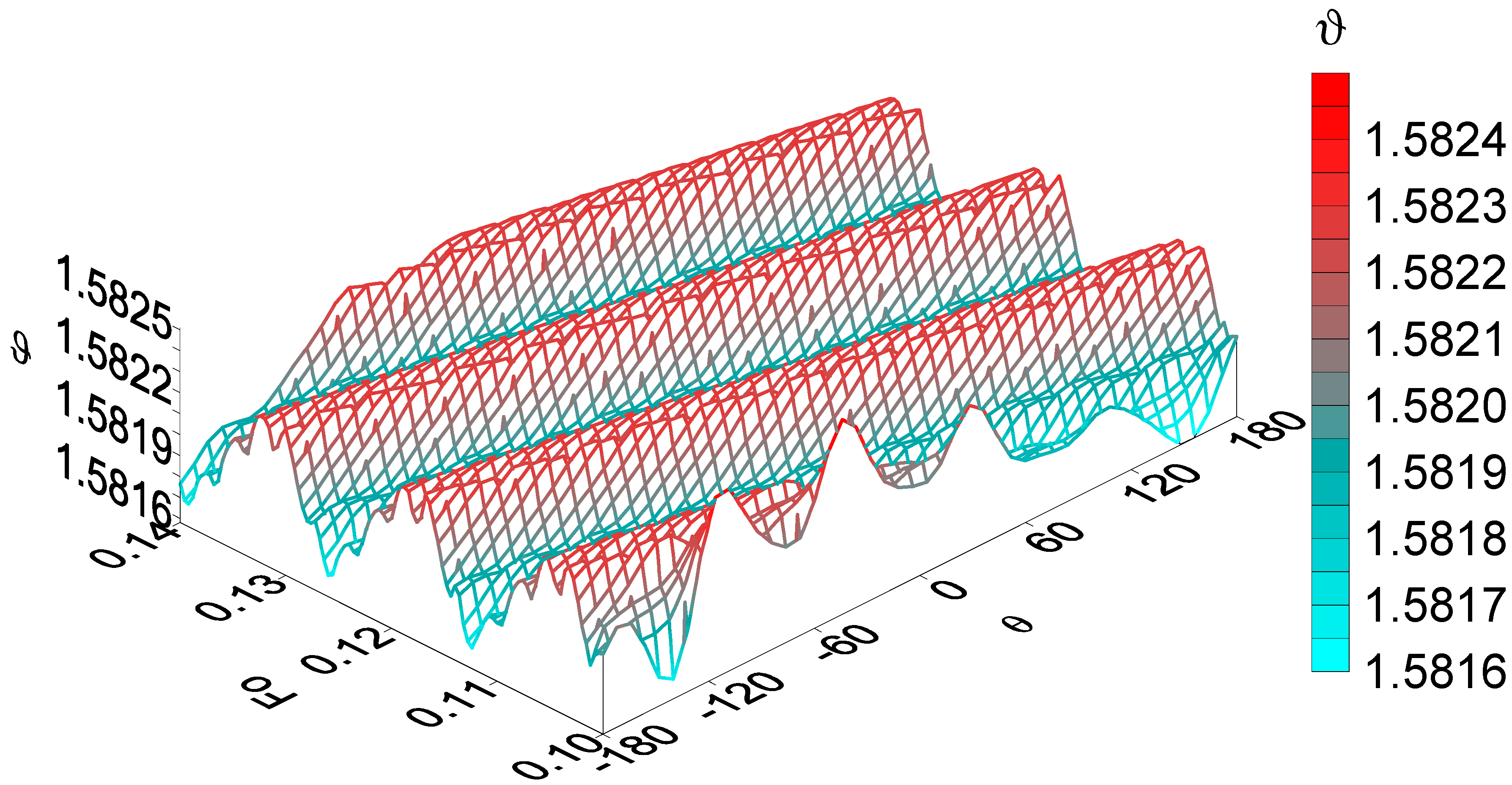
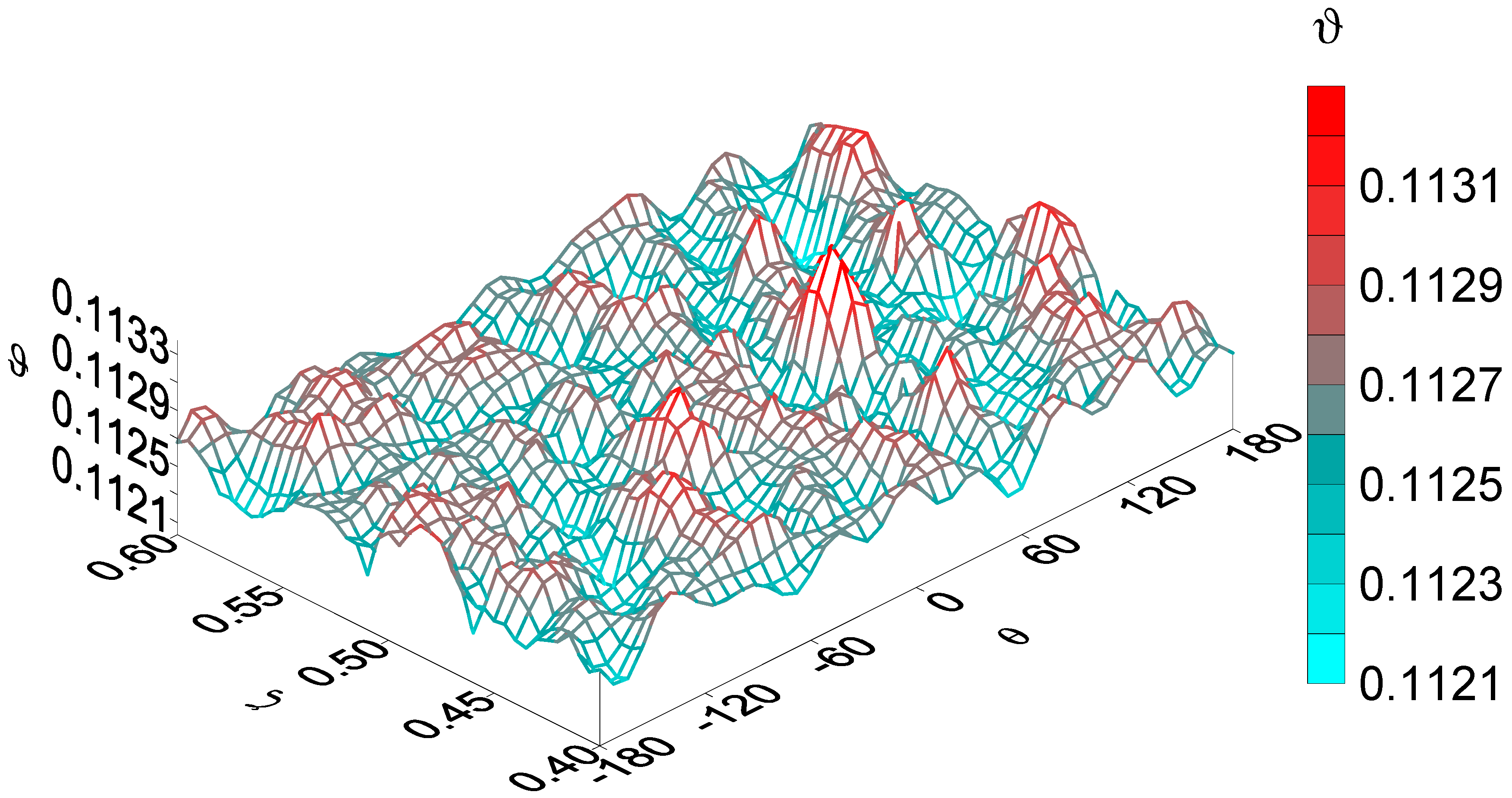
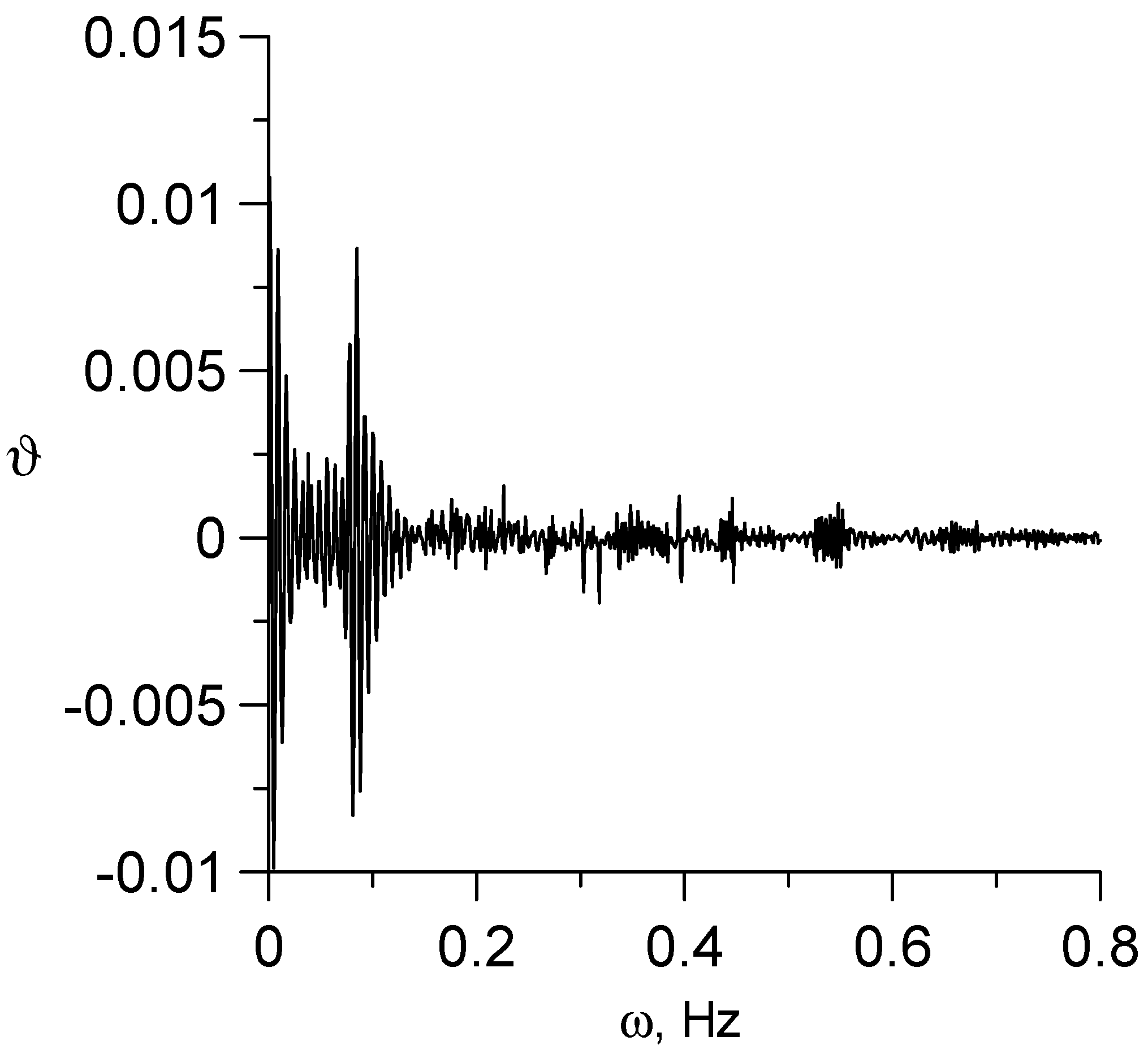
- This paper provides a detailed analysis of the sensitivity function ϑ, which depends only on the geometric and physico-mechanical parameters of the biomass and screw. It is shown that the change in time of this function is reflected in the form of the periodic appearance of spikes in the temperature amplitude. These spikes are arranged in space and time so that they reflect the shape of the screw. In particular, it was established that the temperature distribution in the radial direction is most sensitive to the geometry of the screw surface and its rotation frequency in those places of the filled reactor that are near the edge blades of the screw. The temperature has the same regular character in the angular direction. The most complex is the temperature field in the axial direction as a consequence of the complex superposition of heat flows.
- It is shown that as in the case of an infinitely long channel, for the temperature field in a channel of finite length, there is a condition of spatio-temporal resonance of the temperature field, under which, when the rotation frequency passes through a certain value associated with a constant speed of biomass movement, or vice versa, when the speed of biomass movement passes through a fixed value related to the angular speed of rotation of the screw, the temperature undergoes a sharp increase in the amplitude of oscillations and a sharp change in the phase of oscillations. However, due to interference effects in the case of a channel of finite length, this process is more complex in nature.
- Until now, practically nothing was known about the temperature field in such problems. We limited ourselves to a linear non-connected model of thermal conductivity of a moving substance with a moving heat source of complex shape. We tried to show what kind of spatial–dynamic distribution of the temperature field can be expected in a heating regime close to the one in which the experimentally observed biomass pyrolysis process takes place. The question of the adequacy of such a model for the problem of biomass processing can be solved by experimental measurements of the temperature field in space and time, for which, we hope, the results obtained here can be useful. Experience shows that it seems that the applicability of our results has limitations to the sterilization or pasteurization of food products. Unfortunately, corresponding temperature measurements are currently missing from the literature.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Campuzano, F.; Brown, R.C.; Martinez, J.D. Auger reactors for pyrolysis of biomass and wastes. Renew. Sustain. Energy Rev. 2019, 102, 372–409. [Google Scholar] [CrossRef]
- Makavana, J.M.; Sarsavadia, P.N.; Chauhan, P.M. Effect of pyrolysis temperature and residence time on bio-char obtained from pyrolysis of shredded cotton stalk. Int. Res. J. Pure Appl. Chem. 2020, 21, 10–28. [Google Scholar] [CrossRef]
- Solar, J.; de Marco, I.; Caballero, B.M.; Lopez-Urionabarrenechea, A.; Rodriguez, N.; Agirre, I.; Adrados, A. Influence of temperature and residence time in the pyrolysis of woody biomass waste in a continuous screw reactor. Biomass Bioenergy 2016, 95, 416–423. [Google Scholar] [CrossRef]
- Yu, Y.; Yang, Y.; Cheng, Z.; Blanco, P.H.; Liu, R.; Bridgwater, A.V.; Cai, J. Pyrolysis of rice husk and corn stalk in auger reactor: Part 1. Characterization of char and gas at various temperatures. Energy Fuels 2016, 30, 10568–10574. [Google Scholar] [CrossRef]
- Piersa, P.; Unyay, H.; Szufa, S.; Lewandowska, W.; Modrzewski, R.; Slęzak, R.; Ledakowicz, S. An extensive review and comparison of modern biomass torrefaction reactors vs. biomass pyrolysis—Part 1. Energies 2022, 15, 2227. [Google Scholar] [CrossRef]
- Slęzak, R.; Unyay, H.; Szufa, S.; Ledakowicz, S. An extensive review and comparison of modern biomass torrefaction reactors vs. biomass pyrolysis—Part 2. Energies 2023, 16, 2212. [Google Scholar] [CrossRef]
- Musii, R.; Pukach, P.; Kohut, I.; Vovk, M.; Šlahor, L. Determination and analysis of Joule’s heat and temperature in an electrically conductive plate element subject to short-term induction heating by a non-stationary electromagnetic field. Energies 2022, 15, 5250. [Google Scholar] [CrossRef]
- SAFESTERIL: ETIA ECOTECHNOLOGIES a Branch of the ETIA Group. Available online: https://safesteril.etia-group.com (accessed on 22 June 2023).
- Ledakowicz, S.; Stolarek, P.; Malinowski, A.; Lepez, O. Thermochemical treatment of sewage sludge by integration of drying and pyrolysis/autogasification. Renew. Sustain. Energy Rev. 2019, 104, 319–327. [Google Scholar] [CrossRef]
- Ledakowicz, S.; Piddubniak, O. Analysis of non-stationary temperature field generated by a shaftless screw conveyor heated by Joule–Lenz effect. Chem. Process Eng. 2021, 42, 119–137. [Google Scholar] [CrossRef]
- Piddubniak, O.P.; Piddubniak, N.G. Non-stationary temperature distribution in a thermally insulated concentric cylindrical channel with biomass moving under the influence of rotation of electrically heated helix. Math. Methods Physicomech. Fields 2021, 64, 107–123. (In Ukrainian) [Google Scholar] [CrossRef]
- Piddubniak, O.; Ledakowicz, S. Modeling of heat transfer from an electrically heated rotating helix in a circular cylindrical channel filled with a biomass moving at a constant velocity. Therm. Sci. Eng. Prog. 2022, 30, 101265. [Google Scholar] [CrossRef]
- Ledakowicz, S.; Piddubniak, O. The non-stationary heat transport inside a shafted screw conveyor filled with homogeneous biomass heated electrically. Energies 2022, 15, 6164. [Google Scholar] [CrossRef]
- Carslaw, H.S.; Jaeger, J.C. Conduction of Heat in Solids; Clarendon Press: Oxford, UK, 1959. [Google Scholar]
- Krein, S.G. (Ed.) Functional Analysis; Wolters-Noorhoff Publ.: Groningen, The Netherlands, 1972. [Google Scholar]
- Tsoy, P.V. Methods for Calculating Heat Transfer Problems; Energoatomizdat: Moscow, Russia, 1984. (In Russian) [Google Scholar]
- Luikov, A.V. Analytical Heat Diffusion Theory; Academic Press: New York, NY, USA, 1968. [Google Scholar]
- Korn, G.A.; Korn, T.U. Mathematical Handbook for Scientists and Engineers: Definitions, Theorems and Formulas for References and Review; Dover Publ., Inc.: Mineola, NY, USA, 2000. [Google Scholar]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products; Elsevier Inc.: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Nachenius, R.W.; Van De Wardt, T.A.; Ronsse, F.; Prins, W. Residence time distributions of coarse biomass particles in a screw conveyor reactor. Fuel Process. Technol. 2015, 130, 87–95. [Google Scholar] [CrossRef]
- Shi, X.; Ronsse, F.; Roegiers, J.; Pieters, J.G. 3D Eulerian–Eulerian modeling of a screw reactor for biomass thermochemical conversion. Part 2: Slow pyrolysis for char production. Renew. Energy 2019, 143, 1477–1487. [Google Scholar] [CrossRef]
- Fernández-Díaza, J.M.; Menéndez-Pérezb, C.O. A common framework for modified Regula Falsi methods and new methods of this kind. Math. Comput. Simul. 2023, 205, 678–696. [Google Scholar] [CrossRef]
- Dahlquist, G.; Björck, Å. Numerical Methods in Scientific Computing; SIAM: Philadelphia, PA, USA, 2008; Volume 1. [Google Scholar]
- Luz, F.C.; Cordiner, S.; Manni, A.; Mulone, V.; Rocco, V. Biomass fast pyrolysis in a shaftless screw reactor: A 1-D numerical model. Energy 2018, 157, 792–805. [Google Scholar] [CrossRef]
- Yang, H.; Kudo, S.; Kuo, H.; Norinaga, K.; Mori, A.; Mašek, O.; Hayashi, J. Estimation of enthalpy of bio-oil vapor and heat required for pyrolysis of biomass. Energy Fuels 2013, 27, 2675–2686. [Google Scholar] [CrossRef]
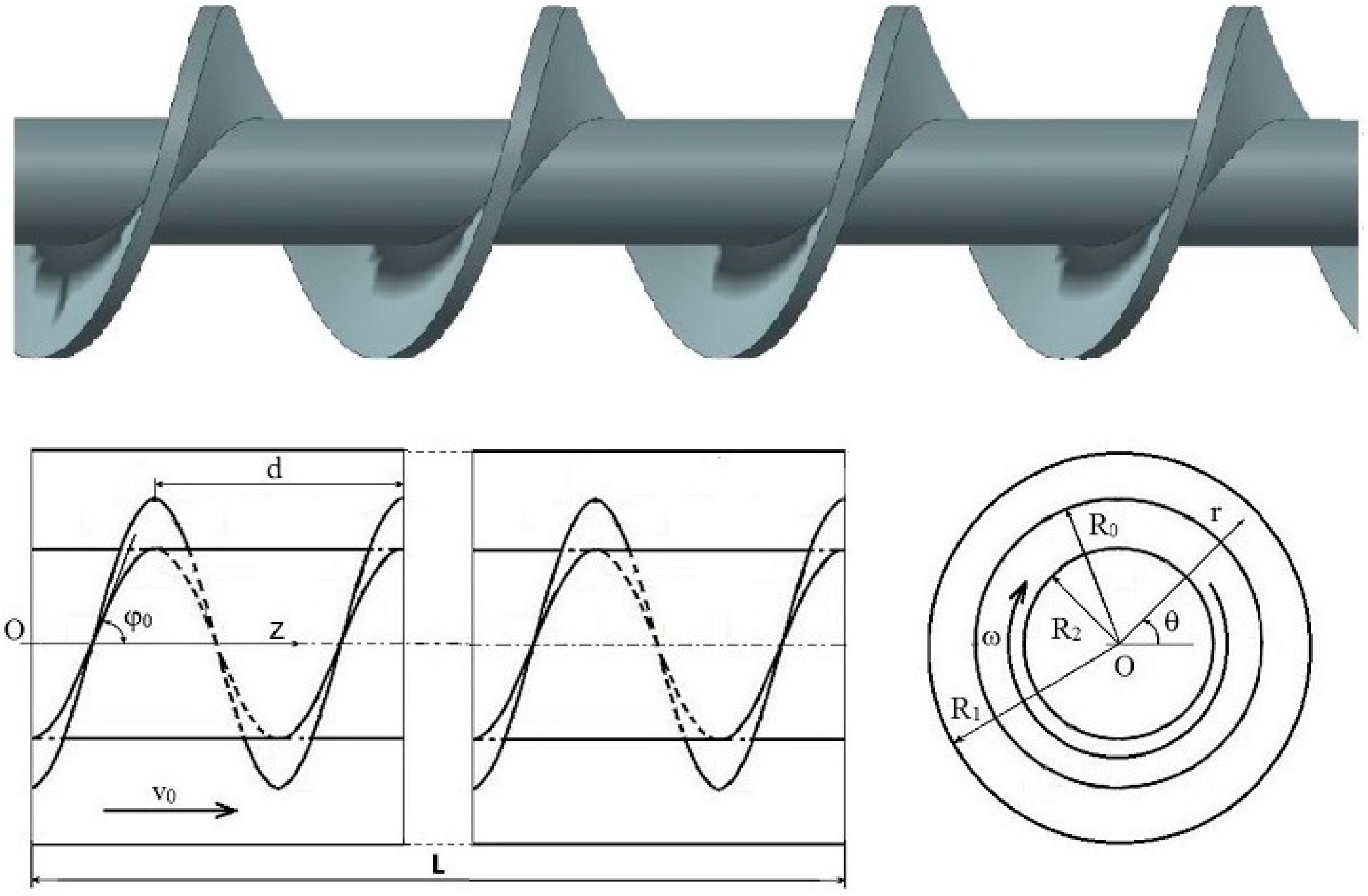









| T0 | 293.15 K | ρ0 | 5.44 × 10−8 Ohm |
| R0 | 0.025 m | cp | 1502 J/(kg K) ** |
| R2 | 0.009 m | λ | 0.35 W/(m K) |
| R1 | 0.026 m | h | 10 W/(m2 K) *** |
| L | 1.64 m | ρ | 551 kg/m3 |
| h1 | 0.003 m | v0 | 5.888 × 10−4 m/s **** |
| ε | 0.962 | a | 4.23 × 10−7 m2/s |
| ε0 | 0.346 | β | 1142 |
| φ0 | 73.68 * | Nu | 46.86 |
| δ | 0.016 | Fo0 | 0.0135 |
| ω | 0.292 Hz | Fov | 0.0145 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ledakowicz, S.; Piddubniak, O. Temperature Distribution in a Finite-Length Cylindrical Channel Filled with Biomass Transported by Electrically Heated Auger. Energies 2023, 16, 6260. https://doi.org/10.3390/en16176260
Ledakowicz S, Piddubniak O. Temperature Distribution in a Finite-Length Cylindrical Channel Filled with Biomass Transported by Electrically Heated Auger. Energies. 2023; 16(17):6260. https://doi.org/10.3390/en16176260
Chicago/Turabian StyleLedakowicz, Stanisław, and Olexa Piddubniak. 2023. "Temperature Distribution in a Finite-Length Cylindrical Channel Filled with Biomass Transported by Electrically Heated Auger" Energies 16, no. 17: 6260. https://doi.org/10.3390/en16176260





