Numerical Evaluation of Heat Transfer and Conversion Efficiency by Tube Design and Flow Configuration for a Compact Steam-Methane Reformer
Abstract
:1. Introduction
2. Numerical Methods
2.1. Reformer Geometry and Operating Conditions
2.2. Numerical Models
2.3. Validation of Mesh Sensitivity
2.4. Validation of Numerical Models
3. Results and Discussion
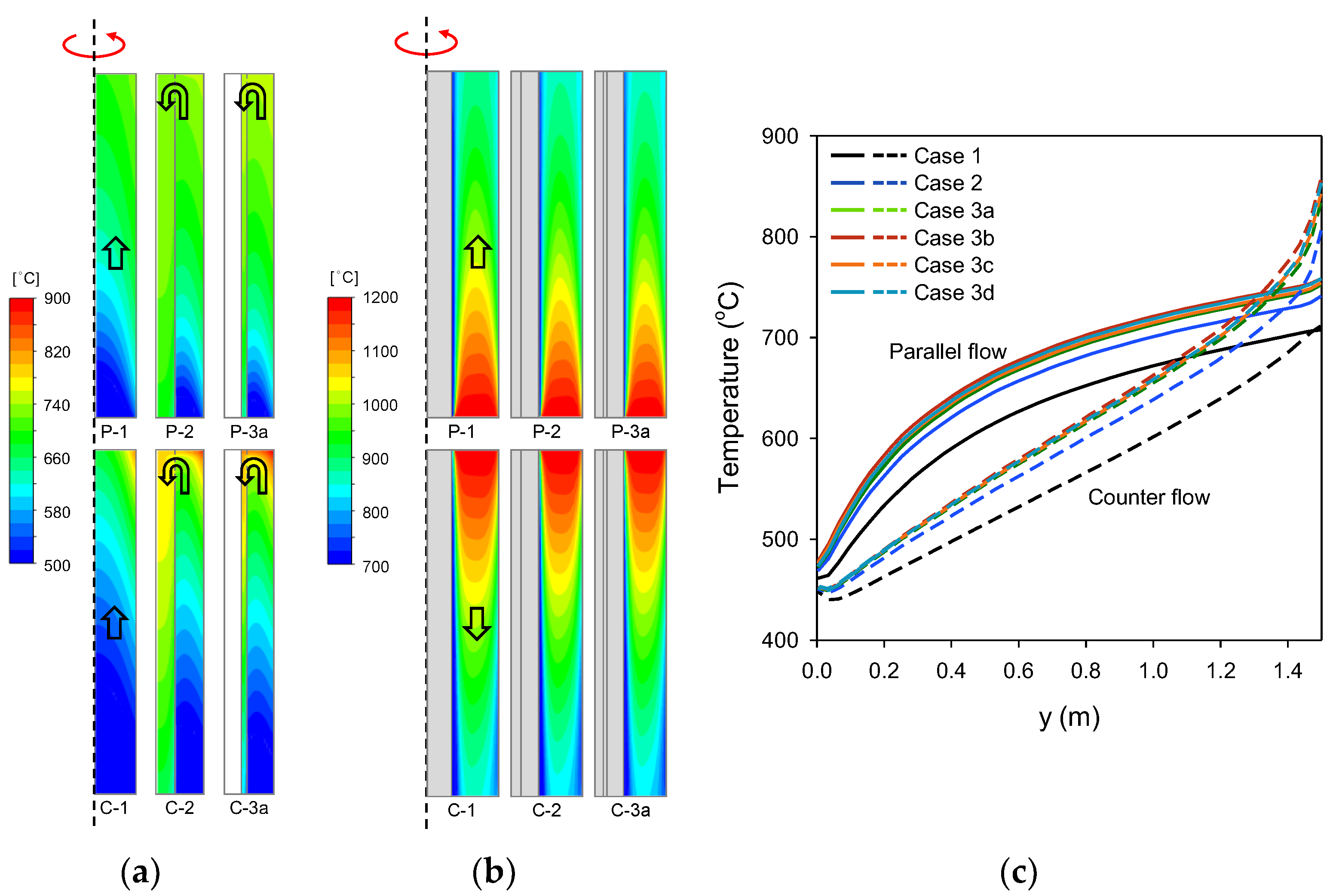
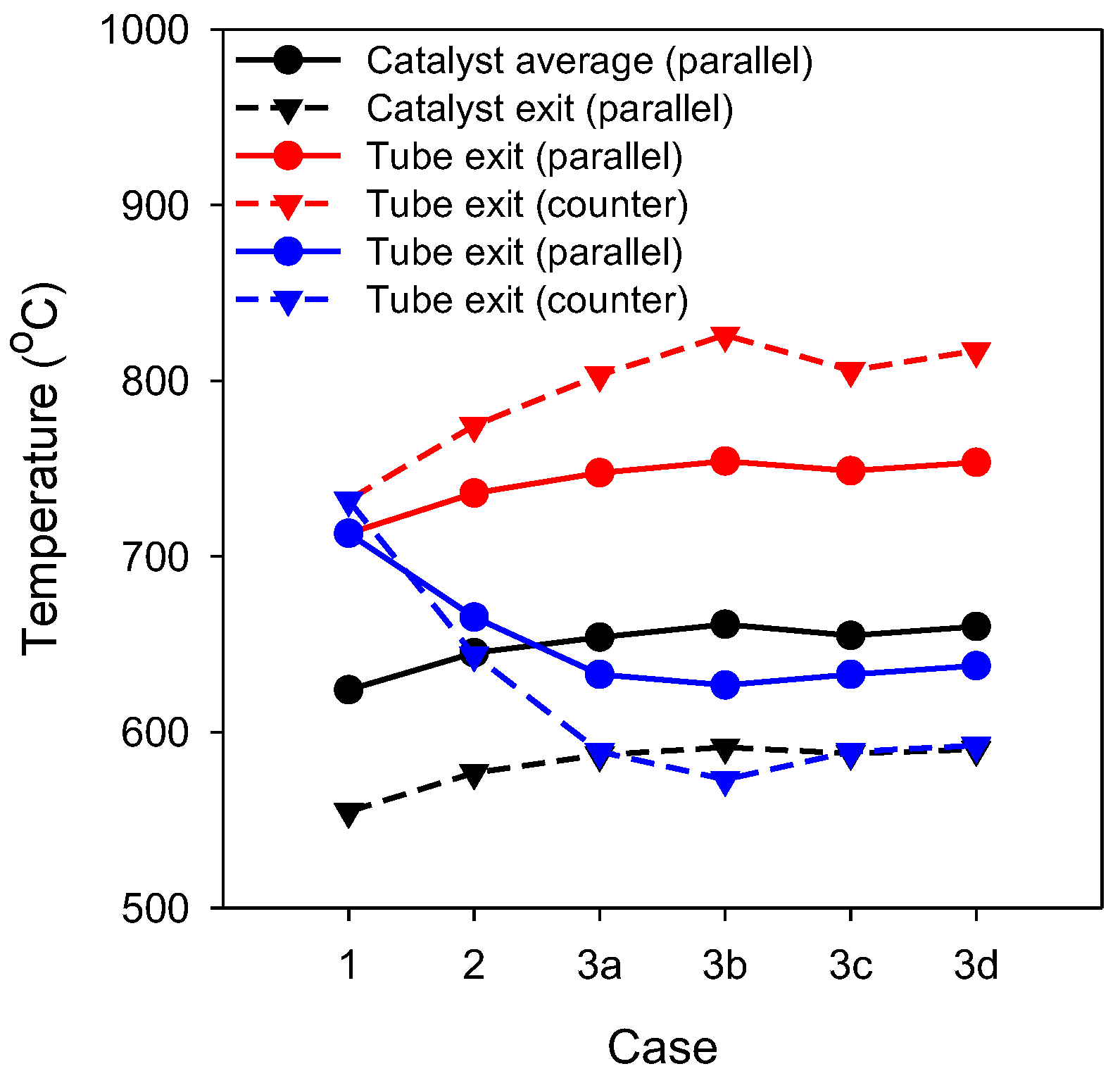
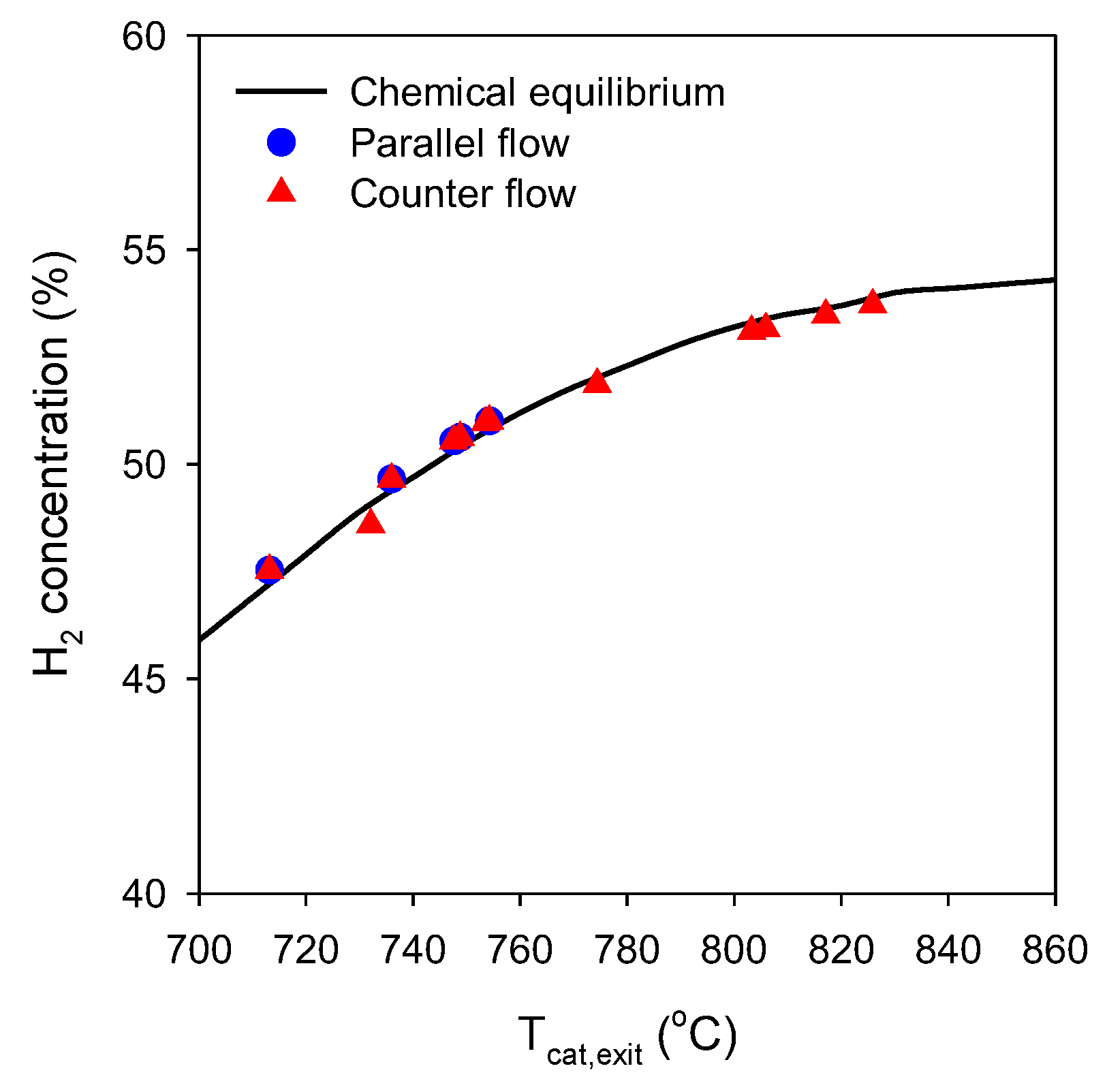
3.1. Analysis of Temperature and Methane Conversion
3.2. Analysis of Heat Transfer
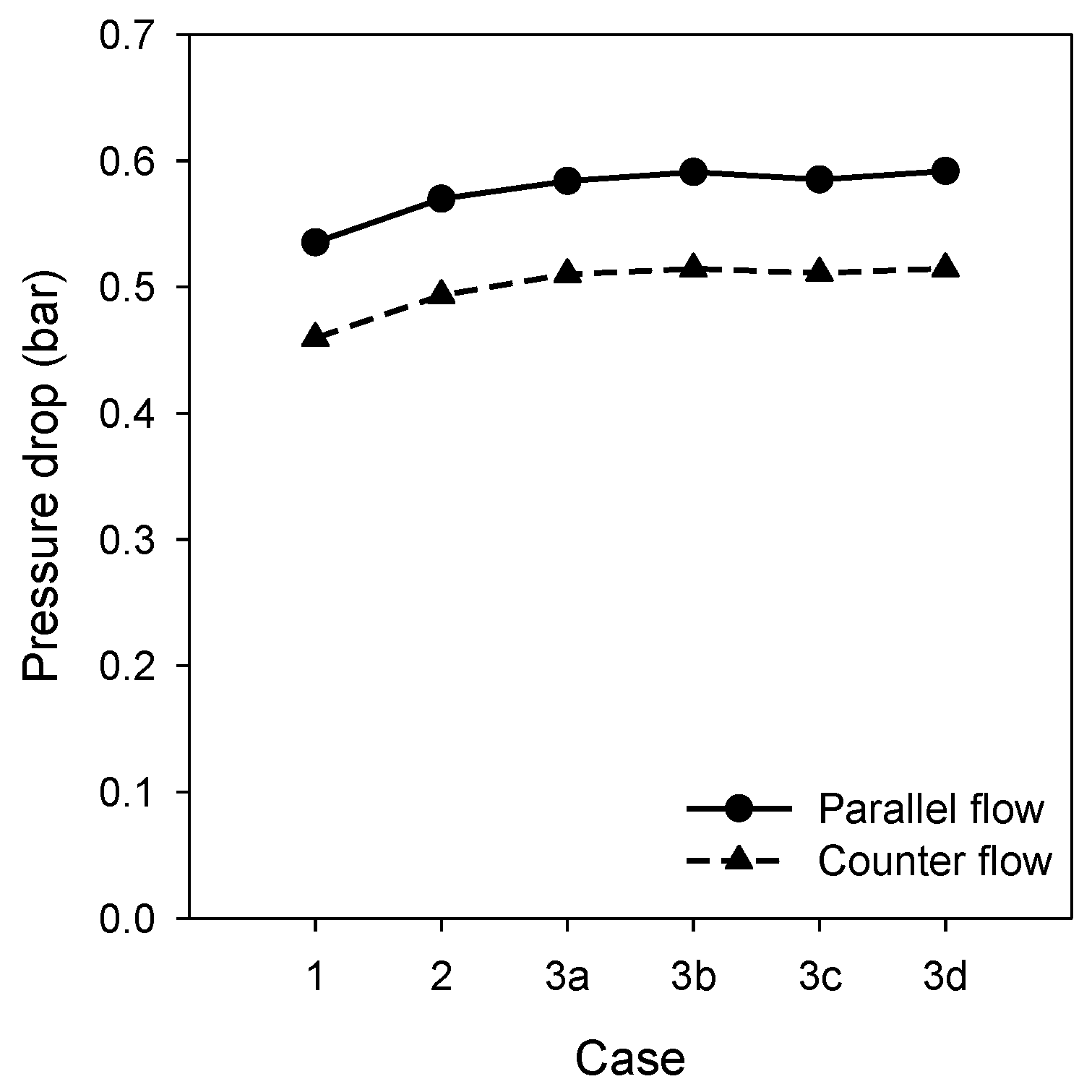
3.3. Pressure Drop in the Reformer Tube
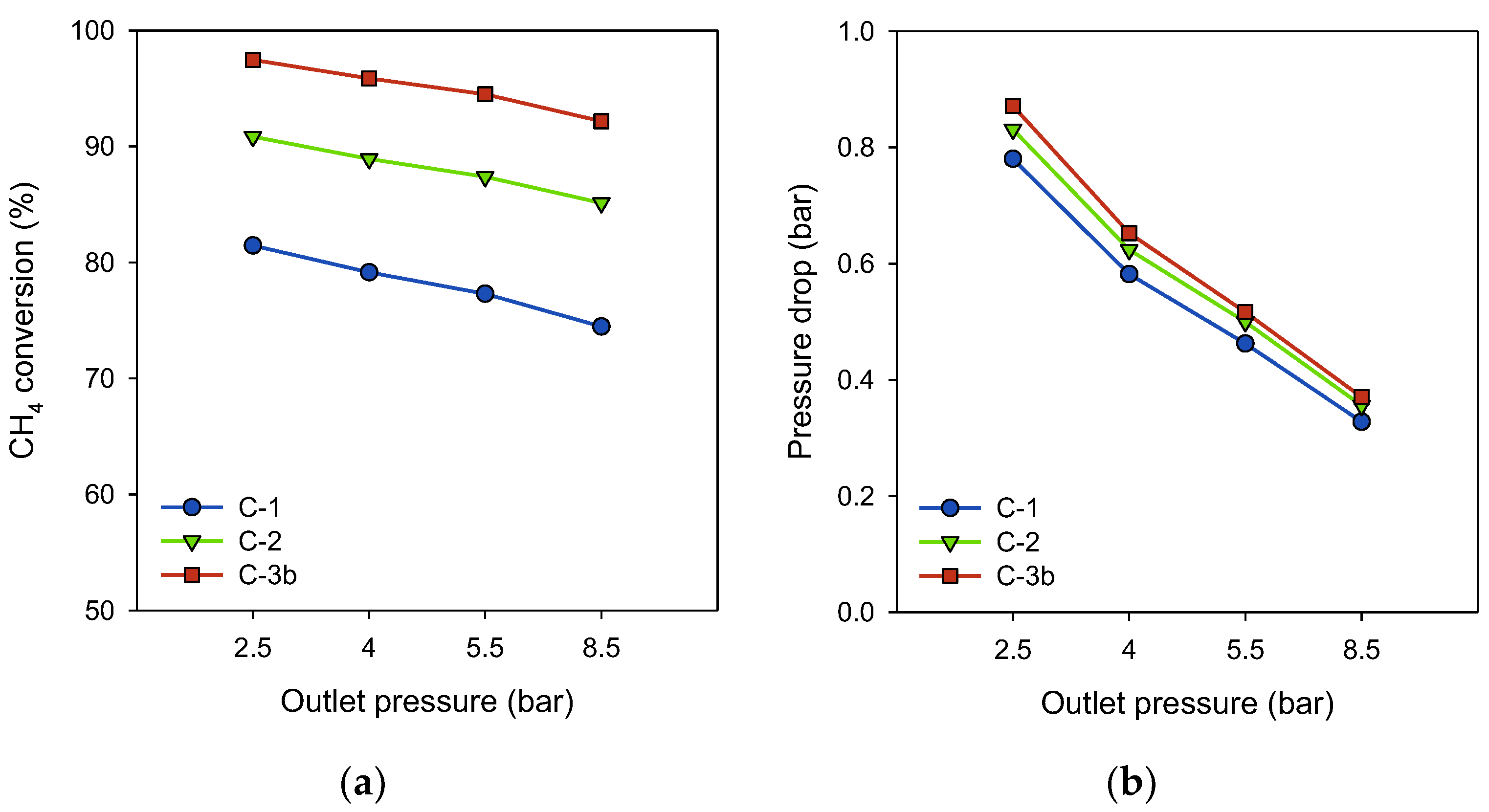
3.4. Influence of Reformate Pressure on Methane Conversion and Pressure Loss
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols | Greek | ||
| A | Area (m2) | Porosity of catalyst | |
| C | Model constant | Rate of dissipation | |
| D | Diameter (mm) | Heat transfer efficiency | |
| Dp | Particle size of catalyst (mm) | Viscosity (kPa·s) | |
| G | Generation of turbulence kinetic energy | Gas superficial velocity (m/s) | |
| k | Turbulence kinetic energy | Density of porous media (kg/m3) | |
| ki | Rate coefficient of reaction i(kmol, bar, kgcat, h) | Turbulent Prandtl number | |
| Ki | Equilibrium constant for reaction i | ||
| KCH4, KCO, KH2 | Adsorption constant (bar−1) | Subscripts | |
| KH2O | Dissociative adsorption constant | avg | Average |
| L | Depth of porous media (m) | b | Buoyancy |
| M | Molecular weight | cat | Catalyst |
| Pressure drop (kPa) | exit | Exit | |
| p | Partial pressure (bar) | i | Index of reforming reaction |
| ri | Rate of reaction i (kmol/kgcat·h) | t | Turbulence |
| T | Temperature (°C) | tube | Reforming tube |
| u | Velocity (m/s) | ||
| x | Mass fraction | ||
References
- Staffell, I.; Scamman, D.; Abad, A.V.; Balcombe, P.; Dodds, P.E.; Ekins, P.; Shah, N.; Ward, K.R. The role of hydrogen and fuel cells in the global energy system. Energy Environ. Sci. 2019, 12, 463–491. [Google Scholar] [CrossRef]
- Younas, M.; Shafique, S.; Hafeez, A.; Javed, F.; Rehman, F. An overview of hydrogen production: Current status, potential, and challenges. Fuel 2022, 316, 123317. [Google Scholar] [CrossRef]
- Birol, F. The Future of Hydrogen: Seizing Today’s Opportunities; International Energy Agency: Paris, France, 2019; Available online: https://doi.org/10.1787/1e0514c4-en (accessed on 18 June 2019).
- Chen, L.; Qi, Z.; Zhang, S.; Su, J.; Somorjai, G.A. Catalytic hydrogen production from methane: A review on recent progress and prospect. Catalysts 2020, 10, 858. [Google Scholar] [CrossRef]
- Ogden, J.M. Review of Small Stationary Reformers for Hydrogen Production; IEA/H2/TR-02/002; International Energy Agency: Paris, France, 2001. [Google Scholar]
- Zhao, X.; Joseph, B.; Kuhn, J.; Ozcan, S. Biogas reforming to syngas: A review. IScience 2020, 23, 101082. [Google Scholar] [CrossRef]
- Barbarias, I.; Lopez, G.; Artetxe, M.; Arregi, A.; Bilbao, J.; Olazar, M. Valorisation of different waste plastics by pyrolysis and in-line catalytic steam reforming for hydrogen production. Energy Convers. Manag. 2018, 156, 575–584. [Google Scholar] [CrossRef]
- Nobandegani, M.S.; Birjandi, M.R.S.; Darbandi, T.; Khalilipour, M.M.; Shahraki, F.; Mohebbi-Kalhori, D. An industrial Steam Methane Reformer optimization using response surface methodology. J. Nat. Gas Sci. Eng. 2016, 36, 540–549. [Google Scholar] [CrossRef]
- Yuan, Q.; Gu, R.; Ding, J.; Lu, J. Heat transfer and energy storage performance of steam methane reforming in a tubular reactor. Appl. Therm. Eng. 2017, 125, 633–643. [Google Scholar] [CrossRef]
- Li, P.; Chen, L.; Xia, S.; Zhang, L. Maximum hydrogen production rate optimization for tubular steam methane reforming reactor. Int. J. Chem. React. Eng. 2019, 17, 20180191. [Google Scholar] [CrossRef]
- Jo, T.; Koo, B.; Lee, Y.; So, H.; Lee, D. Numerical and experimental study on the thermal characteristics of a steam reformer. J. Mech. Sci. Technol. 2018, 32, 679–687. [Google Scholar] [CrossRef]
- Yeh, C.L. Numerical investigation of the effects of steam mole fraction and the inlet velocity of reforming reactants on an industrial-scale steam methane reformer. Energies 2018, 11, 2082. [Google Scholar] [CrossRef]
- Rosen, M.A. Thermodynamic investigation of hydrogen production by steam-methane reforming. Int. J. Hydrogen Energy 1991, 16, 207–217. [Google Scholar] [CrossRef]
- Peng, X.D. Analysis of the thermal efficiency limit of the steam methane reforming process. Ind. Eng. Chem. Res. 2012, 51, 16385–16392. [Google Scholar] [CrossRef]
- Hajjaji, N.; Pons, M.N.; Houas, A.; Renaudin, V. Exergy analysis: An efficient tool for understanding and improving hydrogen production via the steam methane reforming process. Energy Policy 2012, 42, 392–399. [Google Scholar] [CrossRef]
- De Falco, M.; Santoro, G.; Capocelli, M.; Caputo, G.; Giaconia, A. Hydrogen production by solar steam methane reforming with molten salts as energy carriers: Experimental and modelling analysis. Int. J. Hydrogen Energy 2021, 46, 10682–10696. [Google Scholar] [CrossRef]
- Shagdar, E.; Lougou, B.G.; Shuai, Y.; Ganbold, E.; Chinonso, O.P.; Tan, H. Process analysis of solar steam reforming of methane for producing low-carbon hydrogen. RSC Adv. 2020, 10, 12582–12597. [Google Scholar] [CrossRef] [PubMed]
- Engel, S.; Liesche, G.; Sundmacher, K.; Janiga, G.; Thévenin, D. Optimal tube bundle arrangements in side-fired methane steam reforming furnaces. Front. Energy Res. 2020, 8, 583346. [Google Scholar] [CrossRef]
- Shin, G.; Yun, J.; Yu, S. Thermal design of methane steam reformer with low-temperature non-reactive heat source for high efficiency engine-hybrid stationary fuel cell system. Int. J. Hydrogen Energy 2017, 42, 14697–14707. [Google Scholar] [CrossRef]
- Yun, J.; Kim, Y.; Yu, S. Interactive heat transfer characteristics of 5 kW class shell-and-tube methane steam reformer with intermediate temperature heat source. Int. J. Hydrogen Energy 2020, 45, 21767–21778. [Google Scholar] [CrossRef]
- Rohini, A.K.; Choi, S.H.; Lee, H.J. Numerical parametric study on the burner arrangement design for hydrogen production in a steam methane reformer. Int. J. Energy Res. 2021, 45, 16006–16026. [Google Scholar] [CrossRef]
- Halder Topsoe. Bayonet Reformer (SMR-b). Available online: https://www.topsoe.com/our-resources/knowledge/our-products/equipment/bayonet-reformer-smr-b (accessed on 4 October 2023).
- Ma, T.; Zeng, M.; Ji, Y.; Zhu, H.; Wang, Q. Investigation of a novel bayonet tube high temperature heat exchanger with inner and outer fins. Int. J. Hydrogen Energy 2011, 36, 3757–3768. [Google Scholar] [CrossRef]
- Gao, Q.; Zhang, P.; Peng, W.; Chen, S.; Zhao, G. Structural design simulation of bayonet heat exchanger for sulfuric acid decomposition. Energies 2021, 14, 422. [Google Scholar] [CrossRef]
- Lee, J.; Cho, H.; Kim, M.; Hall, S.; Moon, I. Double-tube reactor design and process optimization for on-site steam methane reforming processes. Ind. Eng. Chem. Res. 2020, 59, 18028–18038. [Google Scholar] [CrossRef]
- Minette, F.; De Almeida, L.C.; Feinstein, J.; De Wilde, J. Structured ZoneFlow™-Bayonet steam reforming reactor for reduced firing and steam export: Pressure drop and heat transfer modelling and evaluation of the reactor performance. Chem. Eng. J. Adv. 2022, 10, 100258. [Google Scholar] [CrossRef]
- Upadhyay, M.; Lee, H.; Kim, A.; Lee, S.H.; Lim, H. CFD simulation of methane steam reforming in a membrane reactor: Performance characteristics over range of operating window. Int. J. Hydrogen Energy 2021, 46, 30402–30411. [Google Scholar] [CrossRef]
- Lao, L.; Aguirre, A.; Tran, A.; Wu, Z.; Durand, H.; Christofides, P.D. CFD modeling and control of a steam methane reforming reactor. Chem. Eng. Sci. 2016, 148, 78–92. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Fluent User Guide; ANSYS Inc.: Cannonsburg, PA, USA, 2016. [Google Scholar]
- Xu, J.; Froment, G.F. Methane steam reforming, methanation and water-gas shift: I. Intrinsic kinetics. AIChE J. 1989, 35, 88–96. [Google Scholar] [CrossRef]
- Wesenberg, M.H.; Svendsen, H.F. Mass and heat transfer limitations in a heterogeneous model of a gas-heated steam reformer. Ind. Eng. Chem. Res. 2007, 46, 667–676. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. Lectures in Mathematical Models of Turbulence; Academic Press: London, UK, 1972. [Google Scholar]
- Smith, T.F.; Shen, Z.F.; Friedman, J.N. Evaluation of coefficients for the weighted sum of gray gases model. J. Heat Transf. 1982, 104, 602–608. [Google Scholar] [CrossRef]








| Case | 1 | 2 | 3a | 3b | 3c | 3d | |
|---|---|---|---|---|---|---|---|
| Diameter (mm) | D1 | 331 | 331 | 331 | 331 | 331 | 331 |
| D2o | 115.1 | 129.7 | 133.9 | 145.9 | 135.3 | 133.9 | |
| D2i | 96.4 | 111.0 | 115.2 | 127.2 | 116.6 | 115.2 | |
| D3o | - | 55 | 63 | 83 | 63 | 63 | |
| D3i | 55 | 75 | 55 | 55 | |||
| D4 | - | - | 40 | 60 | 40 | 40 | |
| Fin thickness (mm) | - | - | - | - | 5 | 5 | |
| Outer surface area, A2o (m2) | 0.542 | 0.611 | 0.631 | 0.688 | 0.638 | 0.631 | |
| Height (mm) | 1500 | ||||||
| Catalyst cross-sectional area (mm2) | 7300 | ||||||
| Operating Condition | Value | |
|---|---|---|
| Reforming gas | Inlet temperature (°C) | 500 |
| Tube exit pressure (bar, abs) | 6.5 (2.5–8.5) | |
| Mass flow rate (kg/s) | 0.0084 | |
| Steam-to-carbon ratio | 3 | |
| Heat source | Inlet temperature (°C) | 1200 |
| Pressure (bar, abs) | 1 | |
| Mass flow rate (kg/s) | 0.0328 | |
| Composition (mol.%) | 9.6% O2, 10.9% CO2, 12.4% H2O, and 67.1% N2 | |
| Wall | Parameter | Value |
|---|---|---|
| Outer wall of heat source | Heat transfer | Adiabatic |
| Emissivity | 0.7 | |
| Reformer tube | Emissivity | 0.65 |
| Thermal conductivity (W/m·K) | 23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Koo, Y.; Kang, S.; Ra, H.; Yoon, S.; Ryu, C. Numerical Evaluation of Heat Transfer and Conversion Efficiency by Tube Design and Flow Configuration for a Compact Steam-Methane Reformer. Energies 2023, 16, 7475. https://doi.org/10.3390/en16227475
Koo Y, Kang S, Ra H, Yoon S, Ryu C. Numerical Evaluation of Heat Transfer and Conversion Efficiency by Tube Design and Flow Configuration for a Compact Steam-Methane Reformer. Energies. 2023; 16(22):7475. https://doi.org/10.3390/en16227475
Chicago/Turabian StyleKoo, Yunha, Seoyoung Kang, Howon Ra, Sungmin Yoon, and Changkook Ryu. 2023. "Numerical Evaluation of Heat Transfer and Conversion Efficiency by Tube Design and Flow Configuration for a Compact Steam-Methane Reformer" Energies 16, no. 22: 7475. https://doi.org/10.3390/en16227475
APA StyleKoo, Y., Kang, S., Ra, H., Yoon, S., & Ryu, C. (2023). Numerical Evaluation of Heat Transfer and Conversion Efficiency by Tube Design and Flow Configuration for a Compact Steam-Methane Reformer. Energies, 16(22), 7475. https://doi.org/10.3390/en16227475







