Pressure Drop Characteristics of Subcooled Water in a Hypervapotron under High and Non-Uniform Heat Fluxes
Abstract
:1. Introduction
2. Experimental Descriptions
2.1. Test Loop
2.2. Test Section
2.3. Experimental Conditions
2.4. Data Reduction
2.5. Heat Balance Test
2.6. Uncertainty Analysis
3. Effect of System Parameters
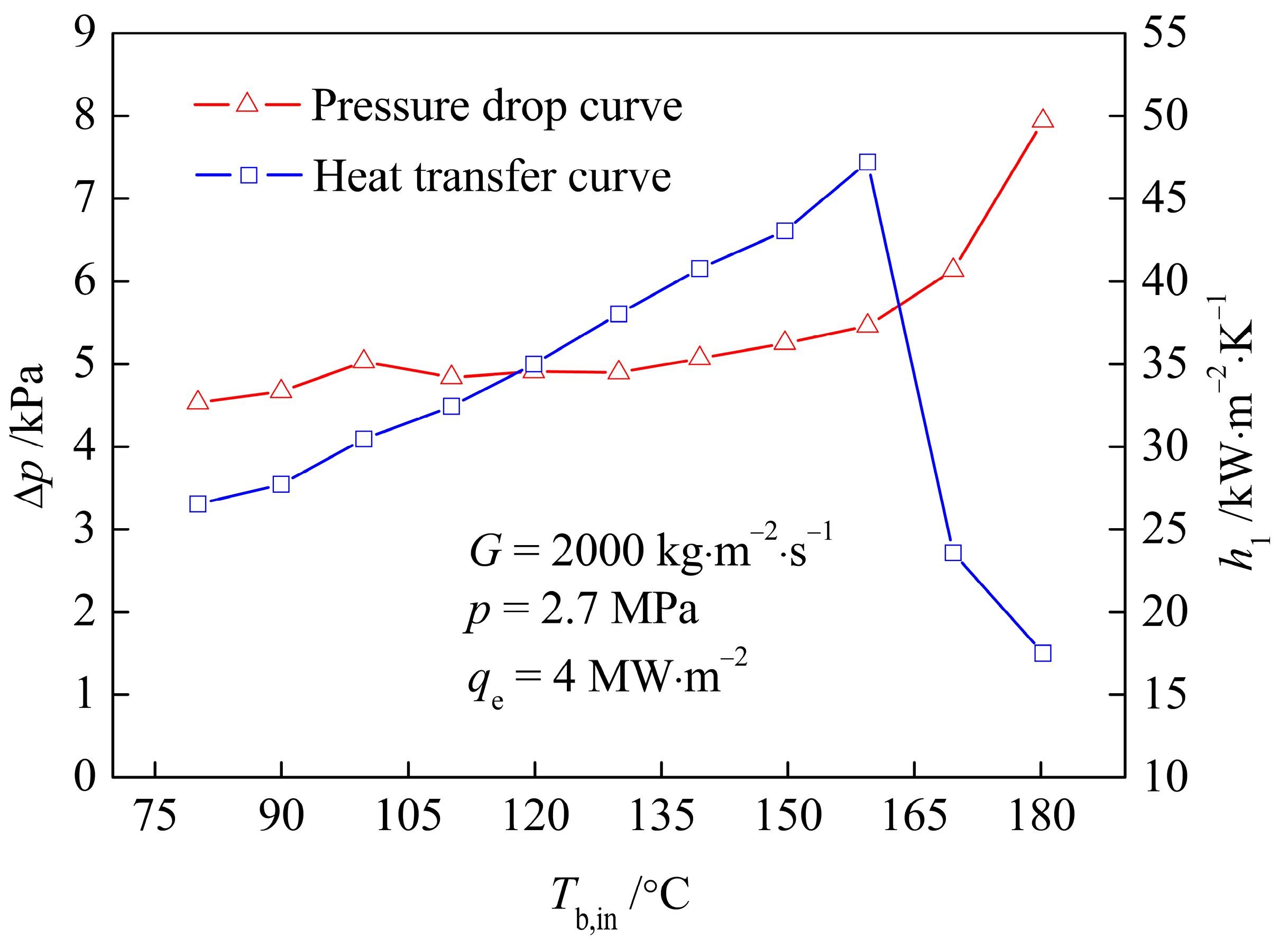
3.1. Typical Working Condition
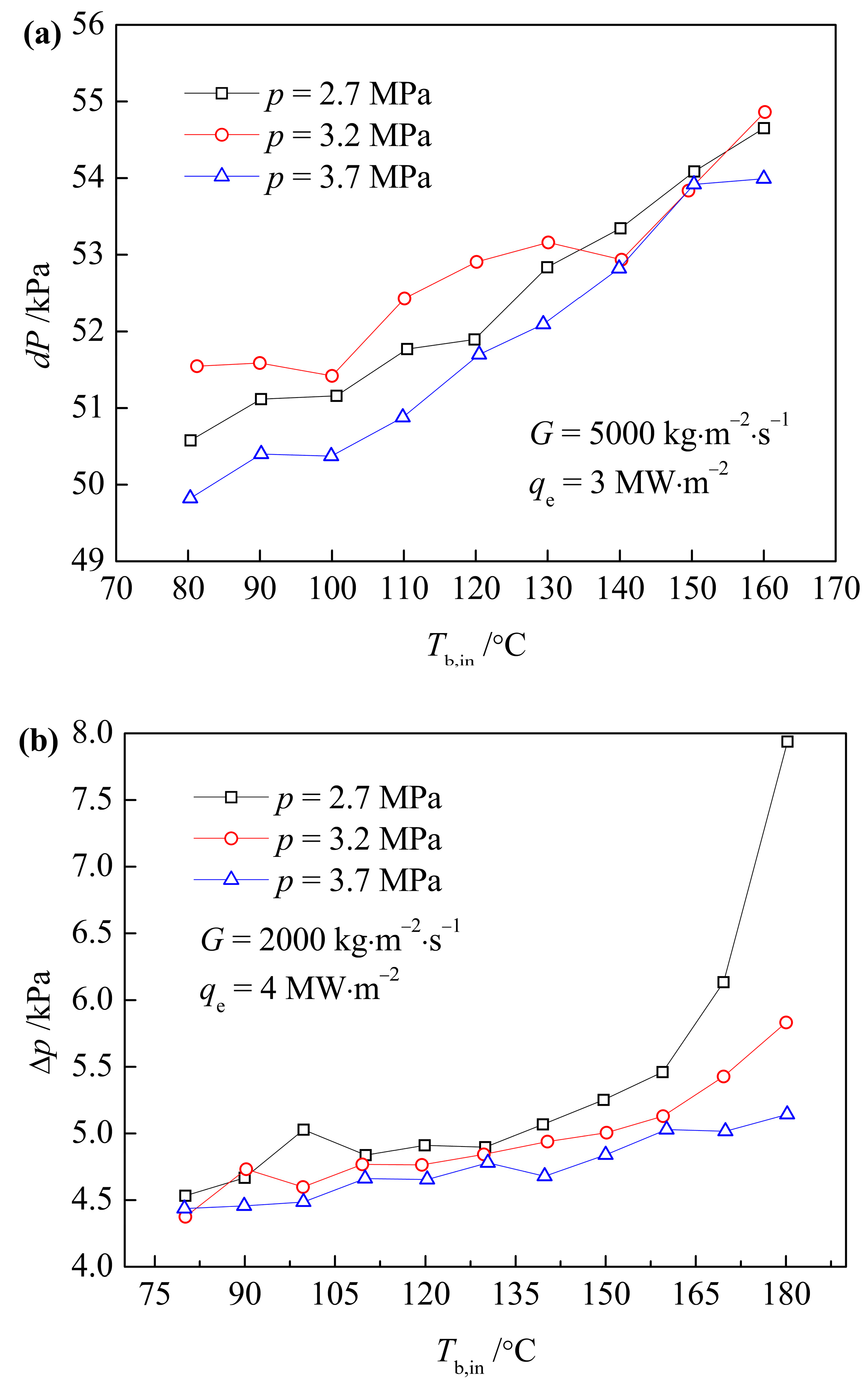
3.2. Effect of Pressure
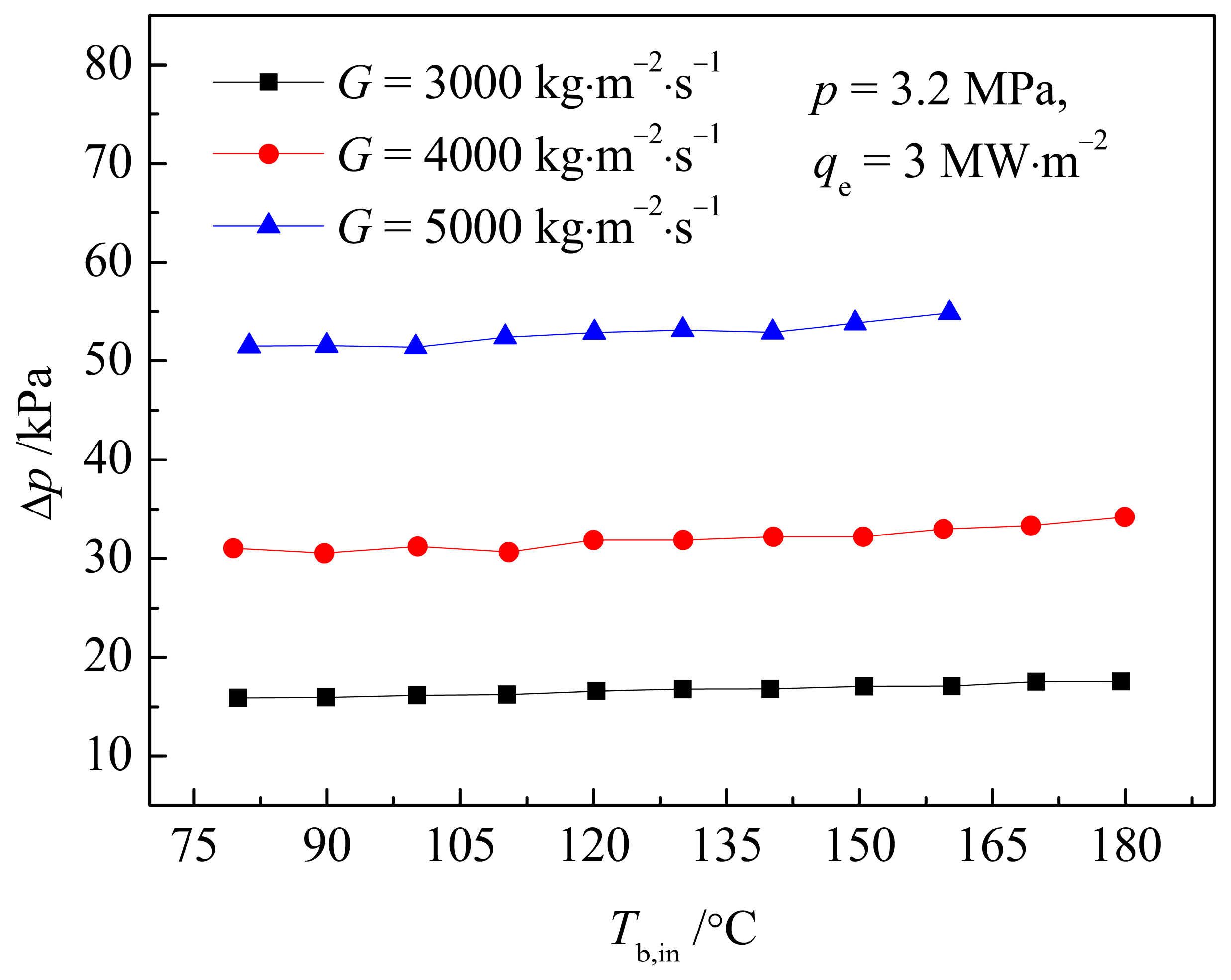
3.3. Effect of Mass Flux
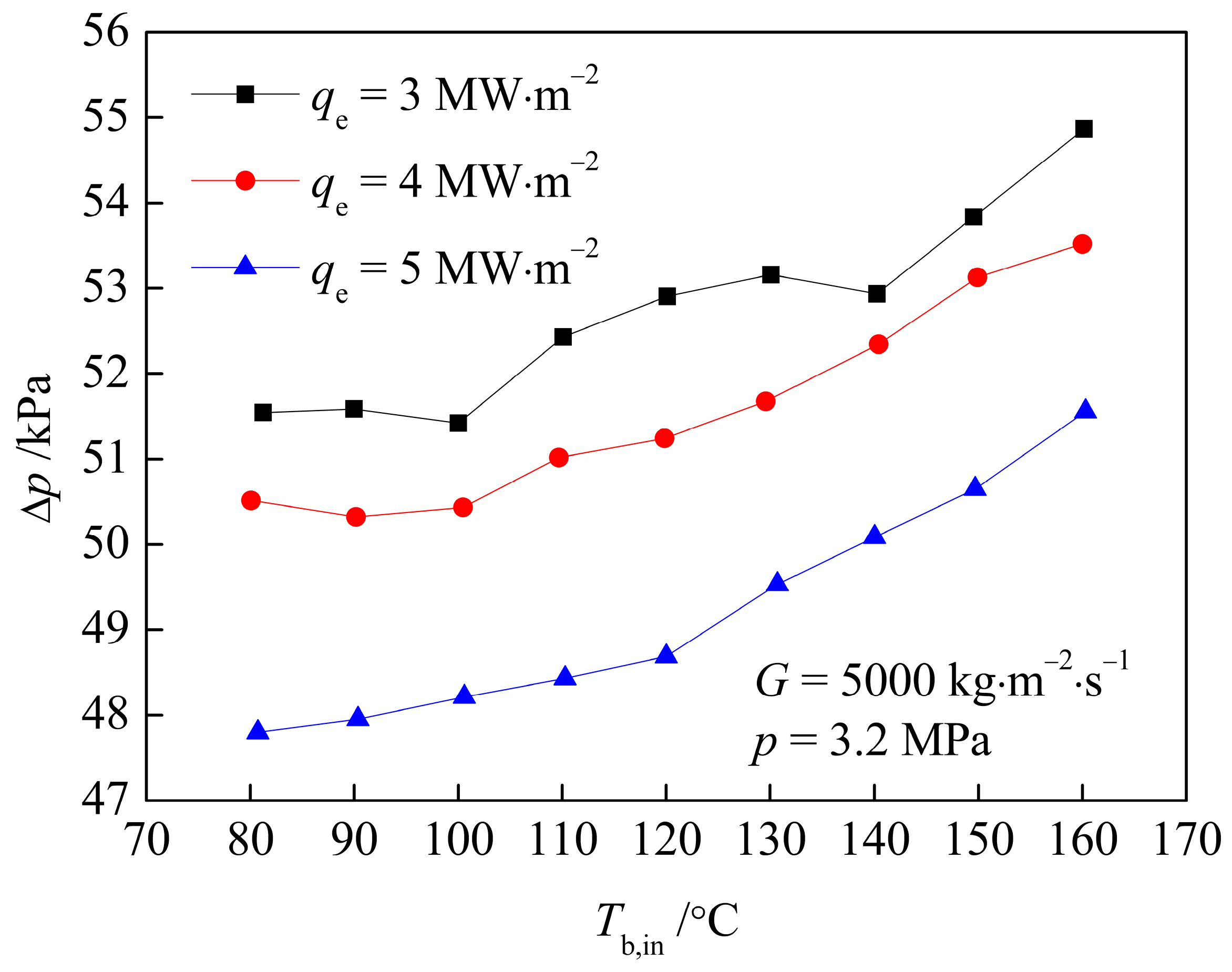
3.4. Effect of Heat Flux
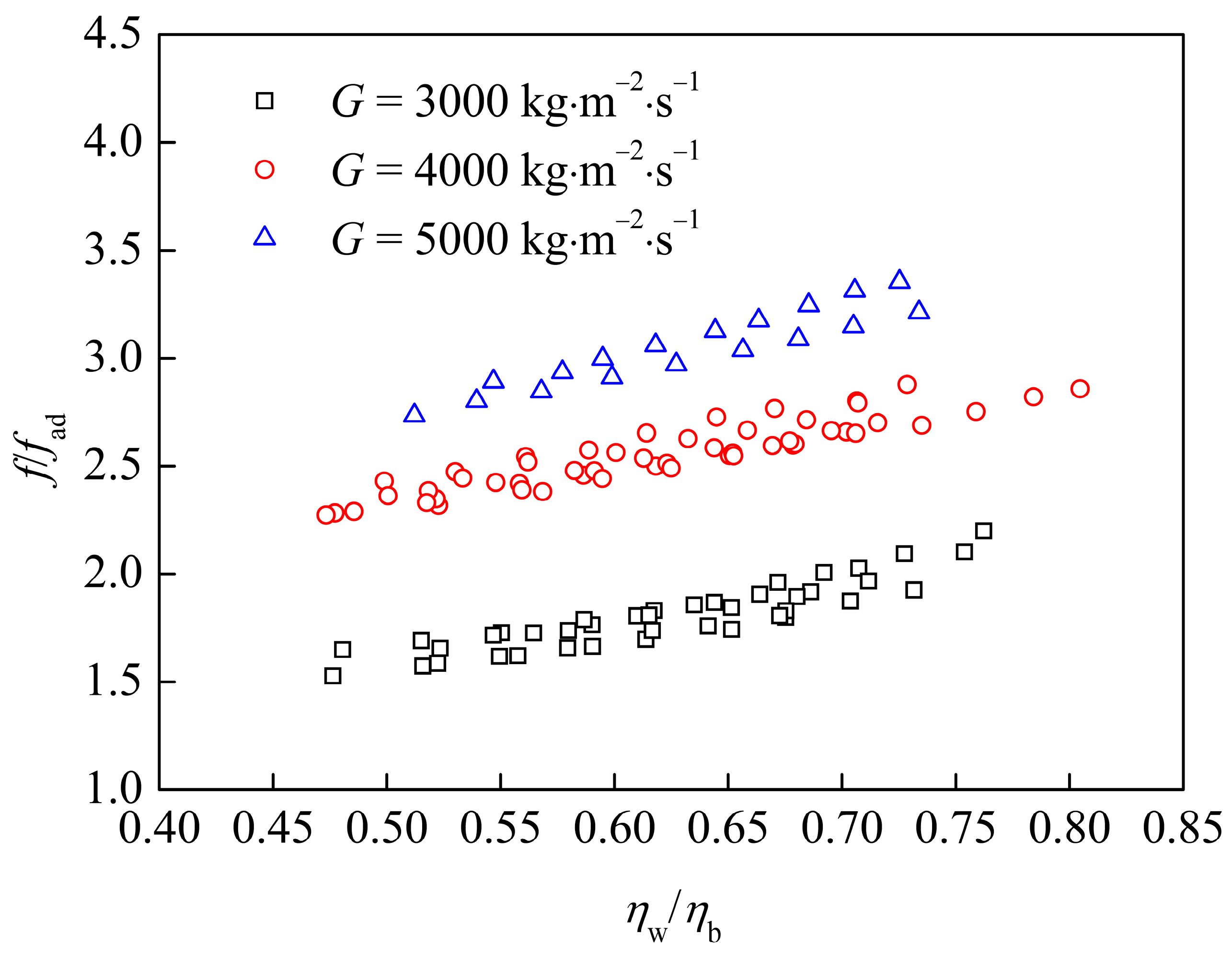
4. Evaluation and Analysis of Pressure Drop Correlations
5. Conclusions
- (1)
- The pressure drop in the hypervapotron is different from that in the ordinary straight channel. Because the thick-walled side of the hypervapotron is densely covered with a transverse fin structure, even the bulk fluid is in a SP state, and there will be fluid heated to generate bubbles in the grooves of the thick-wall side. Meanwhile, the fluid near the thin-wall side is pure liquid phase. Therefore, as Tb,in rises, it may lead to the increase in bubbles on the thick-wall side and the increase in ∆p; while the dynamic viscosity of the fluid near the thin-wall side decreases and ∆p decreases. From the pressure drop curve, ∆p rises with the increasing Tb,in, which indicates that under the working conditions of this test, the increasing effect of the thick-wall side is greater than the weakening effect of the thin-wall side on the pressure drop in the process of Tb,in rises.
- (2)
- The ∆p curve can be divided into an SP region and a subcooled boiling region. In the SP region, ∆p does not change significantly with Tb,in; while in the subcooled boiling region, especially in the fully developed subcooled boiling region with a low subcooling degree, ∆p increase rapidly with the increase in Tb,in. It should be noted that since the thin-wall side is always in a SP flow state under the operating conditions of this test, even if the thick-wall side has entered the subcooled boiling region, the ∆p curve does not increase rapidly. With the further increase in the thermodynamic dryness, the slope of the ∆p curve will increase significantly.
- (3)
- In terms of the influence of system parameters, in the SP region, the influence of p on ∆p is not obvious, but under the condition of lower pressure, it will enter the subcooled boiling region earlier, and the ∆p curve will first start to rise; a higher G leads to a greater ∆p; in the SP region, a higher q leads to a smaller ∆p, because a higher q makes a higher wall temperature, which leads to a higher fluid temperature near the wall surface, so it creates a lower dynamic viscosity, and a smaller ∆p.
- (4)
- Through the analysis of the experimental data, a new pressure drop correlation for subcooled water in an unilateral heated hypervapotron under high heat fluxes and high mass fluxes is proposed. The new correlation has a high prediction accuracy for the test data, the MRE and RMSE are 0.72% and 4.33%, respectively. It can be used for the SP region and the PB region.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area [m2] |
| AF | flow area [m2] |
| Dh | hydraulic diameter [m] |
| f | friction factor [-] |
| G | mass flux [kg·m−2·s−1] |
| h | heat transfer coefficient [kw·m−2·K−1] |
| H | enthalpy [kJ·kg−1] |
| I | current [A] |
| k | thermal efficiency [%] |
| L | length [m] |
| Lh | heated length of tube [m] |
| M | mass flow [kg·m−2·s−1] |
| p | pressure [MPa] |
| ∆p | pressure drop [MPa] |
| Pw | wetting perimeter [m] |
| q | heat flux [kW·m−2] |
| Q | power [kW] |
| r | pressure drop ratio [-] |
| Re | Reynolds number |
| T | temperature [°C] |
| U | voltage drop [V] |
| W | width [m] |
| dynamic viscosity [Pa·s] | |
| density [kg·m−3] | |
| Subscripts | |
| ad | adiabatic |
| b | bulk |
| e | equivalent |
| cal | calculated |
| exp | experimental |
| eff | effective |
| f | frictional |
| g | gas |
| HV | hypervapotron |
| i | inside of the test section |
| in | inlet of the test section |
| l | liquid |
| mea | measure |
| o | outside of the test section |
| out | outlet of the test section |
| sat | saturation |
| sb | subcooled |
| t | thick |
| tp | two-phase |
| w | wall |
| Abbreviations | |
| CHF | critical heat flux |
| ITER | International Thermonuclear Experimental Reactor |
| MRE | mean relative error |
| ONB | onset of nucleate boiling |
| PB | partially boiling |
| RMSE | root mean square error |
| SP | single-phase |
References
- Pascal-Ribot, S.; Saroli, A.F.; Grandotto, M.; Spitz, P.; Escourbiac, F. 3D numerical simulations of hypervapotron cooling concept. Fusion Eng. Des. 2007, 82, 1781–1785. [Google Scholar] [CrossRef]
- Chen, P.; Newell, T.A.; Jones, B.G. Heat transfer characteristics in subcooled flow boiling with hypervapotron. Ann. Nucl. Energy 2008, 35, 1159–1166. [Google Scholar] [CrossRef]
- Escourbiac, F.; Schlosser, J.; Merola, M.; Vastra, I.B. Experimental optimisation of a hypervapotron (R) concept for ITER plasma facing components. Fusion Eng. Des. 2003, 66–68, 301–304. [Google Scholar] [CrossRef]
- Hirai, T.; Bao, L.; Barabash, V.; Carrat, R.; Chappuis, P.; Eaton, R.; Edwards, P.; Escourbiac, F.; Gicquel, S.; Komarov, V.; et al. Hypervapotron heat sinks in ITER plasma-facing components—Process qualifications and production control toward series production. Fusion Eng. Des. 2023, 189, 113454. [Google Scholar] [CrossRef]
- Chen, Z.; Li, Q.; Peng, W.; Wei, R.; Wang, W.; Jiang, K.; Wang, J.; Pan, Z.; Xie, C.; Geng, X.; et al. Study of W/Cu flat-type mock-ups with novel hypervapotron cooling structure for CFETR divertor. Fusion Eng. Des. 2021, 172, 112919. [Google Scholar] [CrossRef]
- Lim, J.H.; Park, M. Forced convective and sub-cooled flow boiling heat transfer in a hypervapotron heat sink under one-side heating condition. Int. J. Heat Mass Transf. 2022, 186, 122523. [Google Scholar] [CrossRef]
- Lim, J.H.; Park, M. Effect of Hypervapotron Fin Angle on Subcooled Flow Boiling Heat Transfer Performance Under One-Side High-Heat Load Condition. Fusion Sci. Technol. Int. J. Am. Nucl. Soc. 2022, 78, 395–413. [Google Scholar] [CrossRef]
- Lim, J.H.; Park, M. Flow Boiling Burnout in a Hypervapotron Channel Under High Heat Flux and High Sub-Cooling Conditions. J. Fusion Energy 2022, 41, 4. [Google Scholar] [CrossRef]
- Chen, X.; Qin, S.; Wang, Q.; Cao, J. Fatigue life prediction of hypervapotron for CFETR divertor. Fusion Eng. Des. 2022, 174, 112985. [Google Scholar] [CrossRef]
- Cao, J.; Qin, S.; Wang, Q.; Chen, X.; Feng, X. Reliability research of hypervapotron under steady-state thermal load. Fusion Eng. Des. 2022, 180, 113191. [Google Scholar] [CrossRef]
- Youchison, D.L.; Ulrickson, M.A.; Bullock, J.H. Effects of hypervapotron geometry on thermalhydraulic performance. IEEE Trans. Plasma Sci. 2012, 40, 653–658. [Google Scholar] [CrossRef]
- Youchison, D.L.; Ulrickson, M.A.; Bullock, J.H. Thermalhydraulic optimization of hypervapotron geometries for first wall applications. In Proceedings of the 2011 IEEE/NPSS 24th Symposium on Fusion Engineering, Chicago, IL, USA, 26–30 June 2011. [Google Scholar] [CrossRef]
- Escourbiac, F.; Bobin-Vastra, I.; Kuznetsov, V.; Missirlian, M.; Schedler, B.; Schlosser, J. A mature industrial solution for ITER divertor plasma facing components: Hypervapotron cooling concept adapted to Tore Supra flat tile technology. Fusion Eng. Des. 2005, 75–79, 387–390. [Google Scholar] [CrossRef]
- Falter, H.D.; Thompson, E. Performance of hypervapotron beam-stopping elements at jet. Fusion Technol. 1996, 29, 584–595. [Google Scholar] [CrossRef]
- Lim, J.H.; Park, M.; Shin, S.M.; Chung, S.S. Exploring the onset of nucleate boiling with hypervapotron channel for tokamak cooling system application. Appl. Therm. Eng. 2022, 209, 118334. [Google Scholar] [CrossRef]
- Baxi, C.B. Comparison of swirl tube and hypervapotron for cooling of ITER divertor. In Proceedings of the 16th International Symposium on Fusion Engineering, Champaign, IL, USA, 30 September–5 October 1995; IEEE: Champaign, IL, USA, 1995; pp. 186–189. [Google Scholar]
- Cattadori, G.; Gaspari, G.P.; Celata, G.P.; Cumo, M.; Mariani, A.; Zummo, G. Hypervapotron technique in subcooled flow boiling CHF. Exp. Therm. Fluid Sci. 1993, 7, 230–240. [Google Scholar] [CrossRef]
- Chen, P.; Jones, B.G.; Newell, T.A. An Experimental Investigation of Sub-Cooled Flow Boiling in Hypervapotron Cooling Configuration. In Proceedings of the 12th International Conference on Nuclear Engineering, Arlington, VA, USA, 25–29 April 2004. [Google Scholar]
- Chen, P.; Wu, W.; Jones, B.G.; Newell, T.A. Heat Transfer for Subcooled Flow Boiling in Hypervapotron Configuration. Nucl. Technol. 2006, 164, 89–96. [Google Scholar] [CrossRef]
- Lemmon, E.; Huber, M.; Mclinden, M. NIST Standard Reference Database 23: Reference Fluid Thermodynamic and Transport Properties-REFPROP, Version 9.1; NIST: Gaithersburg, MD, USA, 2013.
- Zhu, G.; Bi, Q.; Yan, J.; Wang, T.; Zhao, J. Heat transfer characteristics of subcooled water in a hypervapotron under high mass fluxes and high heat fluxes. Int. J. Heat Mass Transf. 2019, 129, 580–590. [Google Scholar] [CrossRef]
- Coleman, H.W.; Steele, W.G. Engineering application of experimental uncertainty analysis. AIAA Journal 1995, 33, 1888–1896. [Google Scholar] [CrossRef]
- Celata, G.P.; Cumo, M.; Mariani, A. Experimental evaluation of the onset of subcooled flow boiling at high liquid velocity and subcooling. Int. J. Heat Mass Transf. 1997, 40, 2879–2885. [Google Scholar] [CrossRef]
- Hoffman, M.A.; Wong, C.F. Prediction of pressure drops in forced convection subcooled boiling water flows. Int. J. Heat Mass Transf. 1992, 35, 3291–3299. [Google Scholar] [CrossRef]
- Sieder, E.N.; Tate, G.E. Heat transfer and pressure drop of liquids in tubes. Ind. Eng. Chem. 1936, 28, 1429–1435. [Google Scholar] [CrossRef]
- Tong, W.; Bergles, A.E.; Jensen, M.K. Pressure drop with highly subcooled flow boiling in small-diameter tubes. Exp. Therm. Fluid Sci. 1997, 15, 202–212. [Google Scholar] [CrossRef]
- Tarasova, N.V.; Leontév, A.I. Hydraulic resistance during flow of water in heated pipes at supercritical pressures. High Temp. 1968, 6, 721–722. [Google Scholar]
- Owens, J.R.W.L.; Schrock, V.E. Local Pressure Gradients for Subcooled Boiling of Water in Vertical Tubes; ASME paper 60-WA-249; ASME: New York, NY, USA, 1960. [Google Scholar]
- Dormer, J.R.T.; Bergles, A.E. Pressure Drop with Surface Boiling in Small-Diameter Tubes; MIT Report, No.8767-31. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1964. [Google Scholar]
- Baburajan, P.K.; Bisht, G.S.; Gupta, S.K.; Prabhu, S.V. Measurement of subcooled boiling pressure drop and local heat transfer coefficient in horizontal tube under LPLF conditions. Nucl. Eng. Des. 2013, 255, 169–179. [Google Scholar] [CrossRef]
- Kim, S.M.; Mudawar, I. Consolidated method to predicting pressure drop and heat transfer coefficient for both subcooled and saturated flow boiling in micro-channel heat sinks. Int. J. Heat Mass Transf. 2012, 55, 3720–3731. [Google Scholar] [CrossRef]
- Tarasova, N.V.; Leontiev, A.; Hlopushin, V.I.; Orlov, V.M. Pressure drop of boiling subcooled water and steam-water mixture flowing in heated channels. In Proceedings of the 3rd International Heat and Transfer Conference, Chicago, CA, USA, 7–12 August 1966; pp. 178–183. [Google Scholar]
- Hahne, E.; Spindler, K.; Skok, H. A new pressure drop correlation for subcooled flow boiling of refrigerants. Int. J. Heat Mass Transf. 1993, 36, 4267–4274. [Google Scholar] [CrossRef]
- Yan, J.; Guo, P.; Bi, Q.; Liu, Z.; Zhang, Q.; Yang, Z. Pressure drop for highly subcooled water flow boiling under high heat and mass fluxes. Appl. Therm. Eng. 2017, 124, 1061–1074. [Google Scholar] [CrossRef]

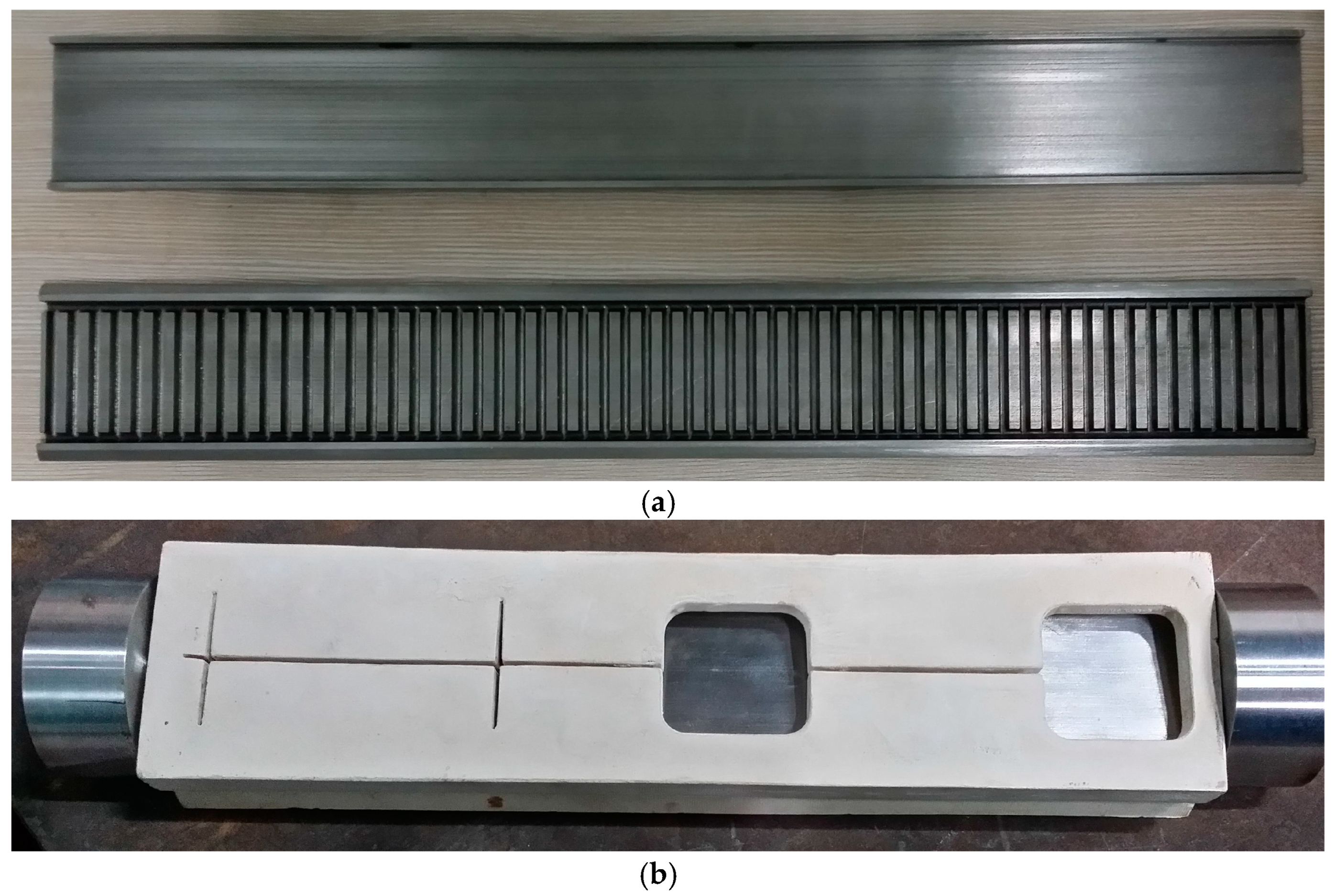
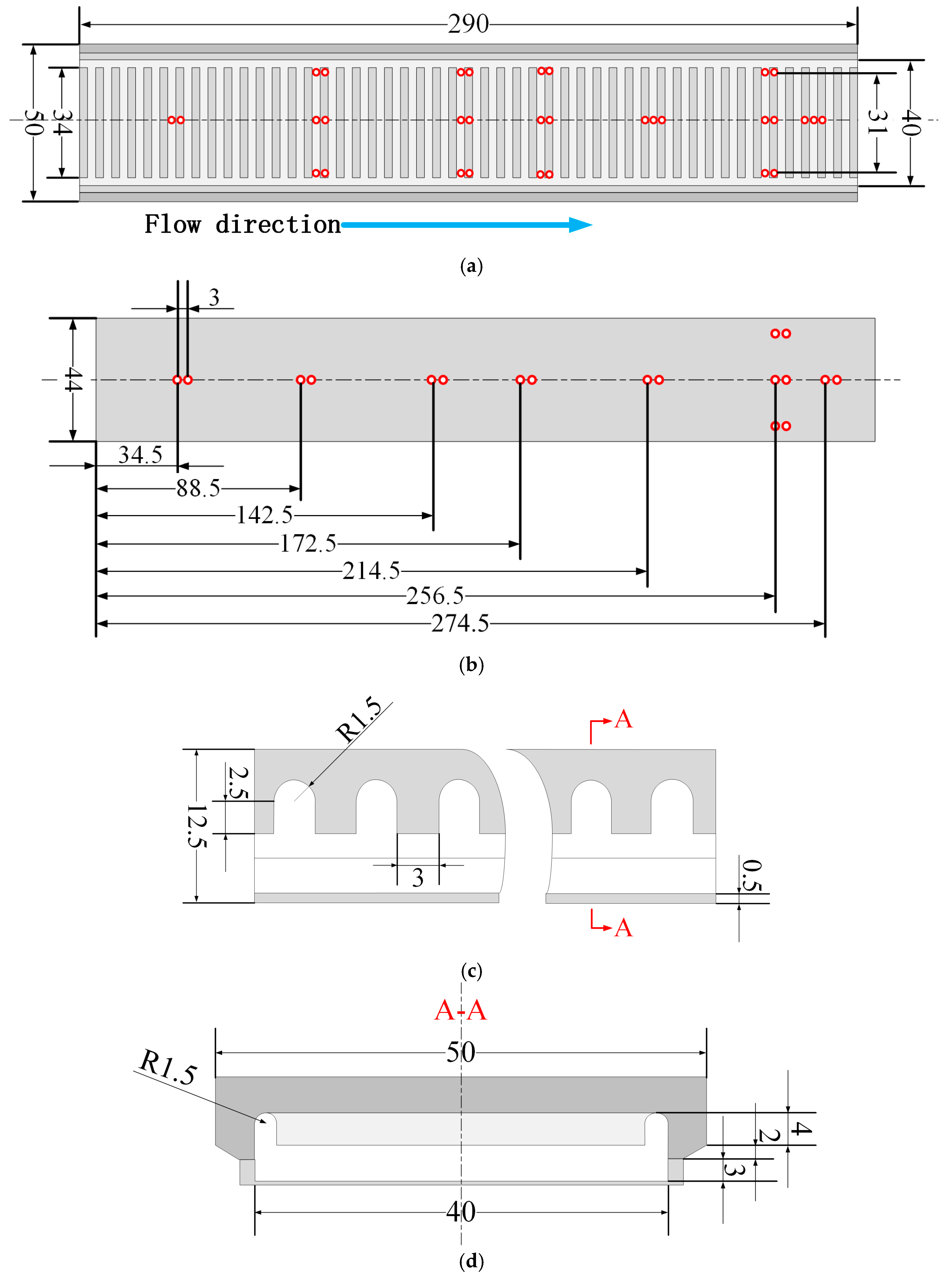



| Experimental Conditions | Characteristics |
|---|---|
| Test section | Hypervapotron |
| Material | 321 Stainless Steel |
| Heating mode | Electrically heated |
| Effective flowing channel size | 40 mm × 7.29 mm |
| Effective heating length | 290 mm |
| Inlet pressure | 2.7–3.7 MPa |
| Mass flux | 2000–5000 kg·m−2·s−1 |
| The effective radiating heat flux | 0–5 MW·m−2 |
| Inlet bulk temperature | 40–230 °C |
| Parameters | Units | Uncertainties (%) |
|---|---|---|
| Pressure | MPa | ±0.25 |
| Mass flux | kg·m−2·s−1 | ±0.25 |
| Heat flux | MW·m−2 | ±4.9 |
| Fluid temperature | °C | ±0.5 |
| Outer wall temperature | °C | ±0.4 |
| Pressure drop | kPa | ±5.5 |
| Authors | Correlations | P/MPa | G/(kg∙m−2∙s−1) | q/(MW∙m−2) |
|---|---|---|---|---|
| Celata [23] | f/fad = (ηw/ηb)0.25 | 1~2.5 | 5000~10,000 | <14 |
| Hoffman [24] | f/fad = (ηw/ηb)0.3 | 0.2~2.8 | 2500~10,000 | <9.7 |
| Sieder [25] | f/fad = (ηw/ηb)0.14 | Not mentioned | 25~387 | <0.013 |
| Tong [26] | f/fad = (ηw/ηb)0.163 | 0.4~1.6 | 25,000~45,000 | <80 |
| Tarasova [27] | f/fad = (ηw/ηb)0.22 | 22.6~26.5 | 2000, 5000 | 0.58~1.32 |
| Owens [28] | f/fad = (ηw/ηb)0.4 | 0.34~2.76 | 1143~5322 | 0.675–4 |
| Dormer [29] | f/fad = (ηw/ηb)0.35 | 0.2~0.55 | 1500~15,000 | <17.35 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, G.; Mei, G.; Yan, J.; Tian, S. Pressure Drop Characteristics of Subcooled Water in a Hypervapotron under High and Non-Uniform Heat Fluxes. Energies 2023, 16, 8121. https://doi.org/10.3390/en16248121
Zhu G, Mei G, Yan J, Tian S. Pressure Drop Characteristics of Subcooled Water in a Hypervapotron under High and Non-Uniform Heat Fluxes. Energies. 2023; 16(24):8121. https://doi.org/10.3390/en16248121
Chicago/Turabian StyleZhu, Ge, Ge Mei, Jianguo Yan, and Shujian Tian. 2023. "Pressure Drop Characteristics of Subcooled Water in a Hypervapotron under High and Non-Uniform Heat Fluxes" Energies 16, no. 24: 8121. https://doi.org/10.3390/en16248121
APA StyleZhu, G., Mei, G., Yan, J., & Tian, S. (2023). Pressure Drop Characteristics of Subcooled Water in a Hypervapotron under High and Non-Uniform Heat Fluxes. Energies, 16(24), 8121. https://doi.org/10.3390/en16248121





