Study on the Mechanism of Gas Intrusion and Its Transportation in a Wellbore under Shut-in Conditions
Abstract
1. Introduction
2. Visualization of Gas Intrusion
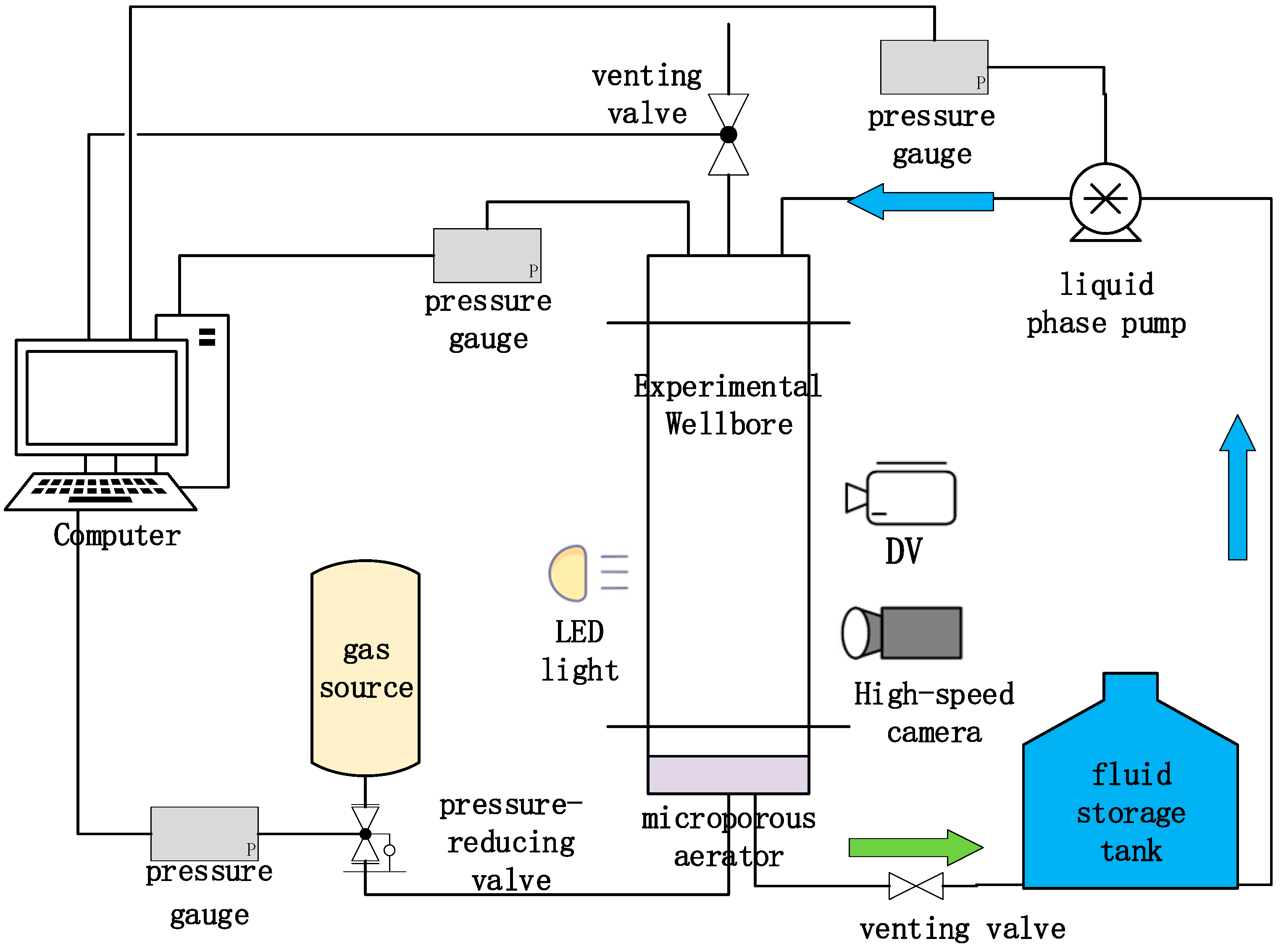
2.1. Experimental System
2.2. Experimental Materials
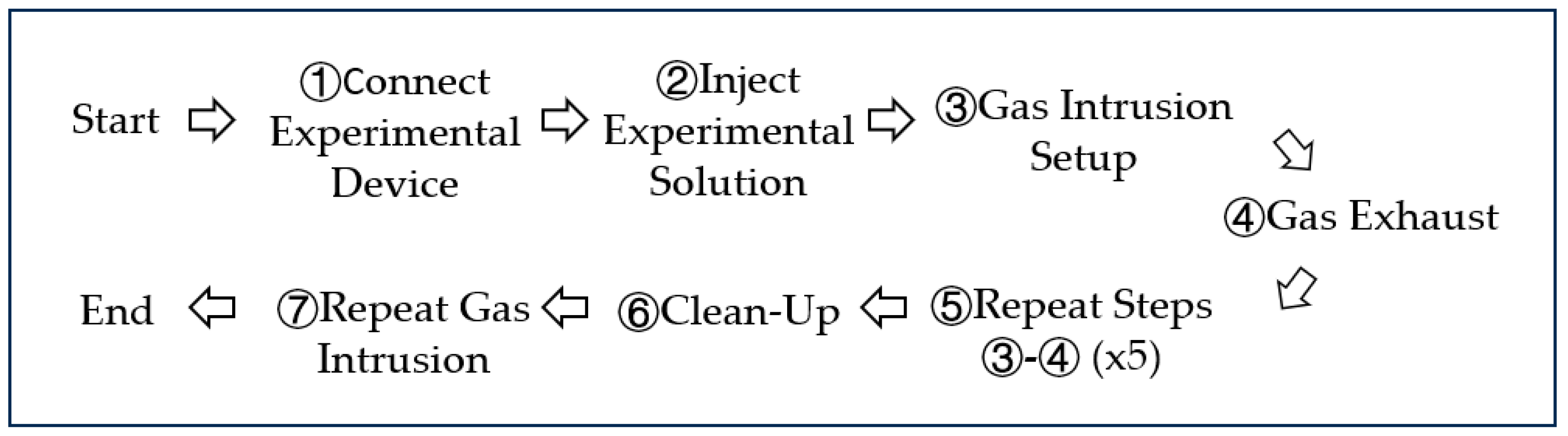
2.3. Experimental Procedure
- (1)
- Simulation of gas intrusion into the wellbore under shut-in conditions
- (2)
- Obtaining intrusive-gas transportation velocity within the wellbore under shut-in conditions
3. Results and Analysis
3.1. Simulation of Gas Intrusion into the Wellbore under Shutdown Conditions
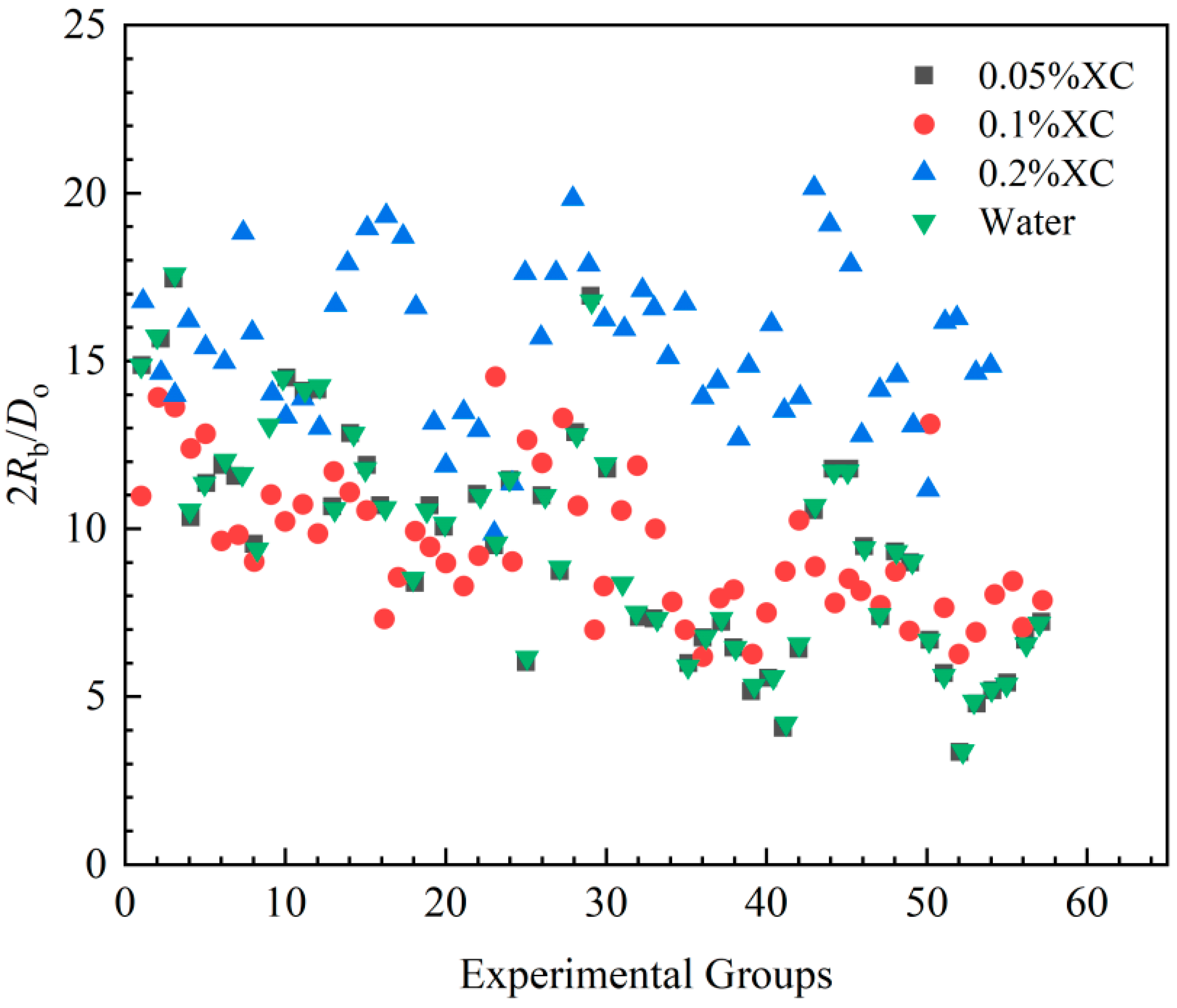
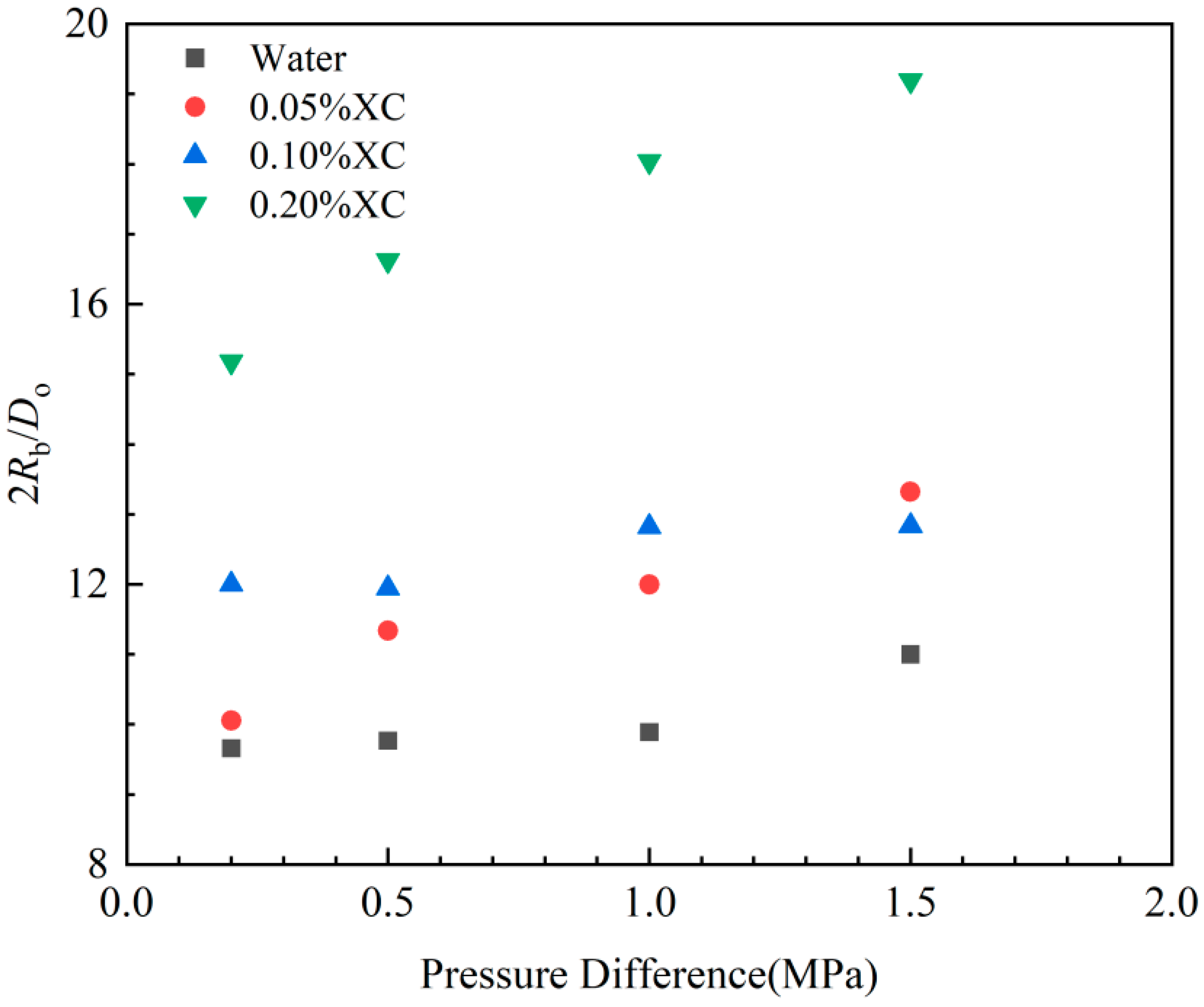
3.1.1. Variations in the Size of the Intrusion Bubbles
3.1.2. Modeling Gas Intrusion Rate under Shut-in Well Conditions
3.2. Simulation of Intrusive-Gas Transportation Velocity in Wellbore under Shut-in Conditions
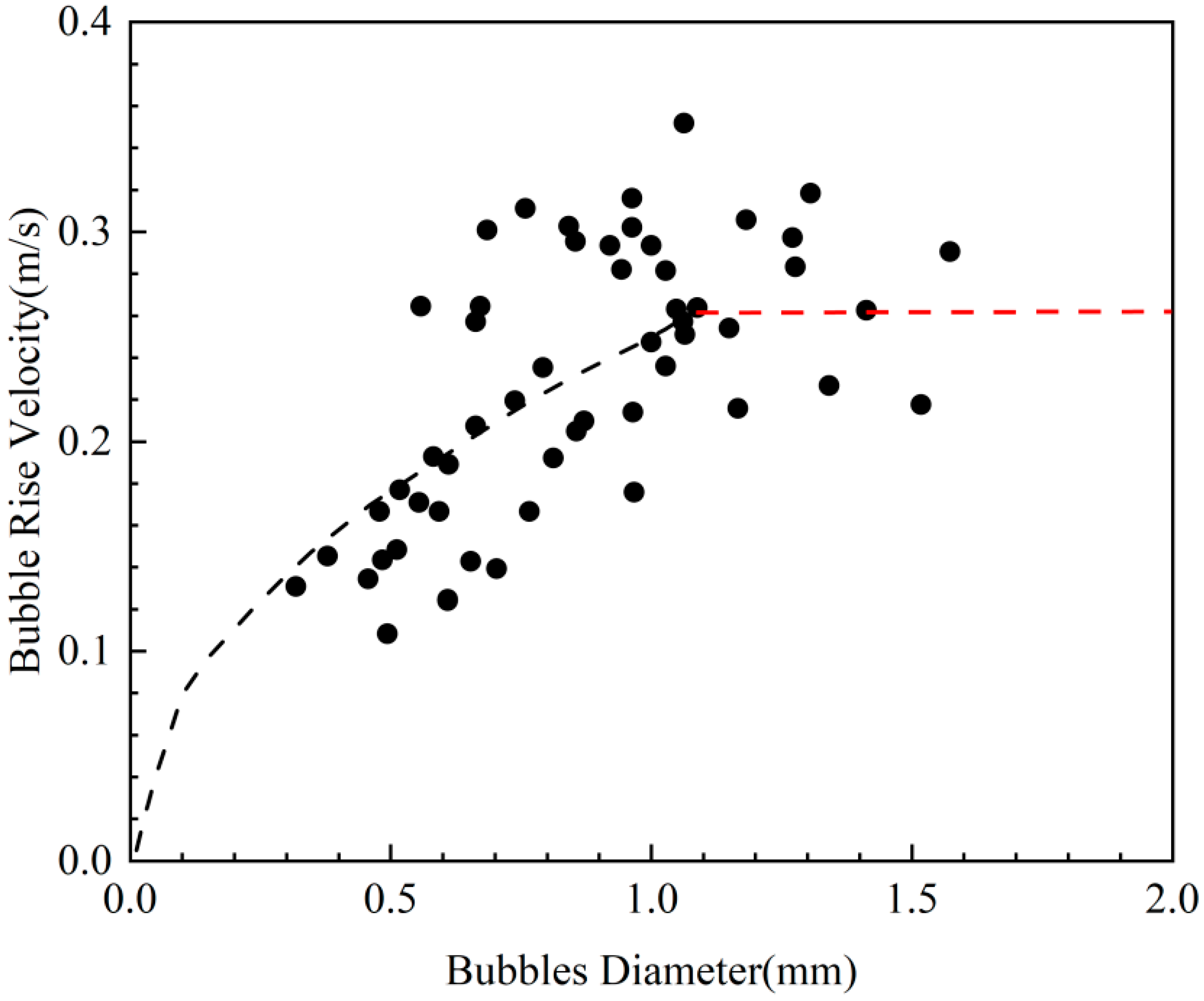
3.2.1. Single-Bubble Transport Experiment
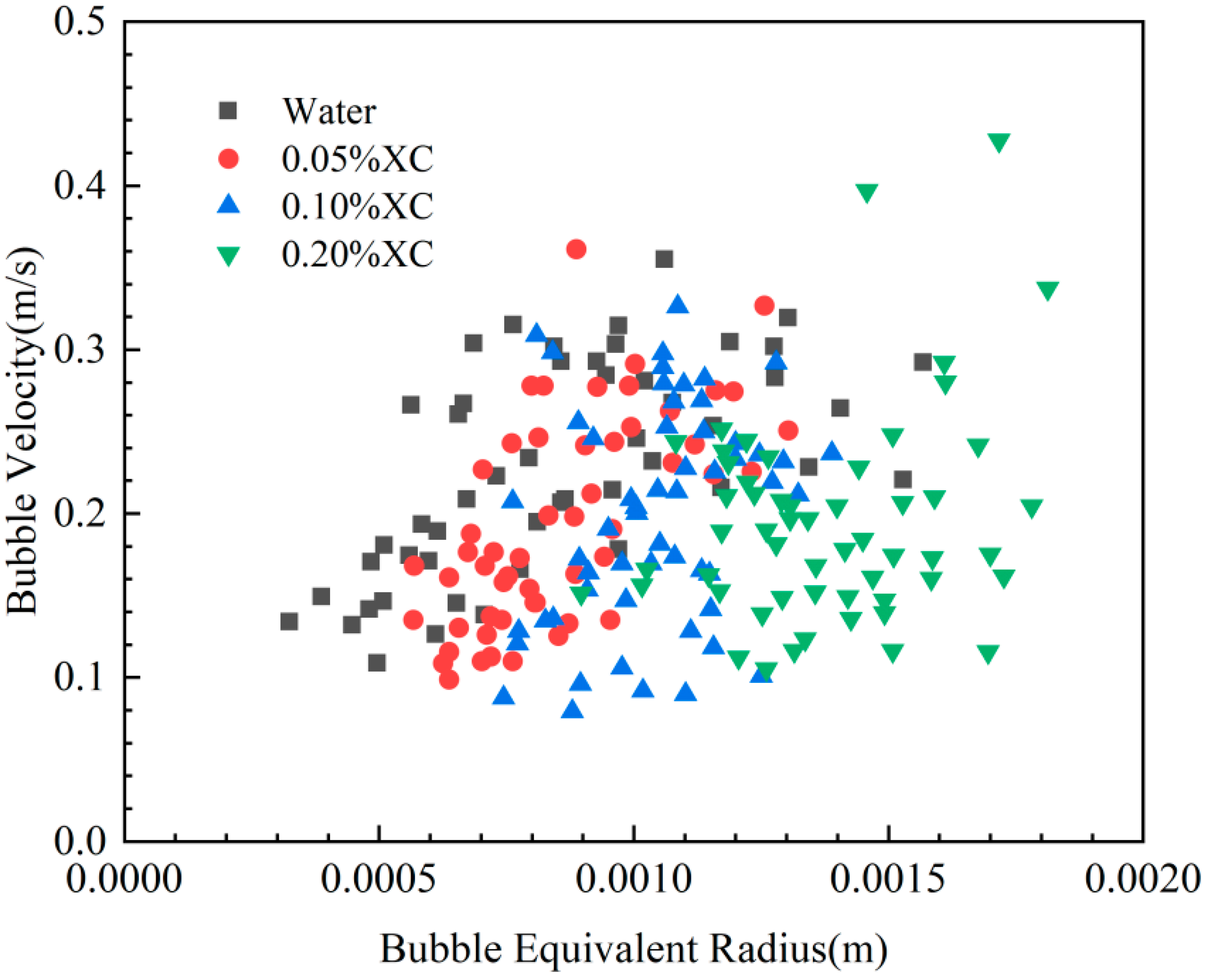
3.2.2. Experiments on the Transport of Bubble Populations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yin, H.; Si, M.; Li, Q.; Zhang, J.; Dai, L. Kick Risk Forecasting and Evaluating during Drilling Based on Autoregressive Integrated Moving Average Model. Energies 2019, 12, 3540. [Google Scholar] [CrossRef]
- Rommetveit, R.; Vefring, E.H. Comparison of results from an advanced gas kick simulator with surface and downhole data from scale gas kick experiments in an inclined well. In Proceedings of the SPE Annual Technical Conference and Exhibition, SPE 22558, Dallas, TX, USA, 6–9 October 1991. [Google Scholar]
- Stefan, M.; Samuel, G.; Azar, J. Modeling of pressure buildup on a kicking well and its practical application. In Proceedings of the Permian Basin Oil and Gas Recovery Conference, Midland, TX, USA, 27–29 March 1996. [Google Scholar]
- Davies, R.M.; Taylor, G. The mechanics of large bubbles rising through extended liquids and through liquids in tubes. Dyn. Curved Front. 1988, 200, 377–392. [Google Scholar]
- Harmathy, T.Z. Velocity of large drops and bubbles in media of infinite or restricted extent. Aiche J. 1960, 6, 281–288. [Google Scholar] [CrossRef]
- Wallis, G.B. One Dimensional Two-Phase Flows; McGraw-Hill: New York, NY, USA, 1969; pp. 97–112. [Google Scholar]
- Fan, W.Y. Study of Bubble Behaviors and Flow Field around Moving Bubble in Non-Newtonian Fluid; Tianjin University: Tianjin, China, 2008. [Google Scholar]
- Wang, T.; Li, H.X.; Li, Y. Numerical Investigation on Coaxial Coalescence of Two Gas Bubbles. J. Xi’an Jiaotong Univ. 2013, 47, 1–6. [Google Scholar]
- Liu, Y.P.; Wang, P.Y.; Lin, S.N. Correlation of Position of Taylor Bubble Formation in Cryogenic Tube. J. Shanghai Jiaotong Univ. 2013, 47, 1509–1514+1519. [Google Scholar]
- Ulaganathan, V.; Krzan, M.; Lotfi, M.; Dukhin, S.; Kovalchuk, V.; Javadi, A.; Gunes, D.; Gehin-Delval, C.; Malysa, K.; Miller, R. Influence of β-lactoglobulin and its surfactant mixtures on velocity of the rising bubbles. Colloids Surf. A Physicochem. Eng. Asp. 2014, 460, 361–368. [Google Scholar] [CrossRef]
- Azzopardi, B.J.; Pioli, L.; Abdulkareem, L.A. The properties of large bubbles rising in very viscous liquids in vertical columns. Int. J. Multiph. Flow 2014, 67, 160–173. [Google Scholar] [CrossRef]
- Keshavarzi, G.; Pawell, R.S.; Barber, T.J.; Yeoh, G.H. Transient analysis of a single rising bubble used for numerical validation for multiphase flow. Chem. Eng. Sci. 2014, 112, 25–34. [Google Scholar] [CrossRef]
- Cano-Lozano, J.C.; Bolaños-Jiménez, R.; Gutiérrez-Montes, C.; Martínez-Bazán, C. The use of volume of fluid technique to analyze multiphase flows: Specific case of bubble rising in still liquids. Appl. Math. Model. 2015, 39, 3290–3305. [Google Scholar] [CrossRef]
- Yan, X.; Zheng, K.; Jia, Y.; Miao, Z.; Wang, L.; Cao, Y.; Liu, J. Drag Coefficient Prediction of a Single Bubble Rising in Liquids. Ind. Eng. Chem. Res. 2018, 57, 5385–5393. [Google Scholar] [CrossRef]
- Khodayar, J.; Davoudian, S.H. Surface Wettability Effect on the Rising of a Bubble Attached to a Vertical Wall. Int. J. Multiph. Flow 2018, 109, 178–190. [Google Scholar]
- Du, J.; Zhao, C.; Bo, H.; Ren, X. The Modeling of Bubble Lift-Off Diameter in Vertical Subcooled Boiling Flow. Energies 2022, 15, 6857. [Google Scholar] [CrossRef]
- Mangani, F.; Soligo, G. Influence of density and viscosity on deformation, breakage, and coalescence of bubbles in turbulence. Phys. Rev. Fluids 2022, 7, 34. [Google Scholar] [CrossRef]
- He, H.; Liu, Z.; Ji, J.; Li, S. Analysis of Interaction and Flow Pattern of Multiple Bubbles in Shear-Thinning Viscoelastic Fluids. Energies 2023, 16, 5345. [Google Scholar] [CrossRef]
- Guo, Y.L. Study on Gas Invasion Mechanism and Wellbore Pressure during Well Shut-In in Deepwater Drilling. Ph.D. Thesis, China University of Petroleum, Qingdao, China, 2018. [Google Scholar]
- Zhang, J.; Du, D.; Hou, J. Seepage Mechanics of Oil and Gas Reservoirs, 2nd ed.; China University of Petroleum Press: Dongying, China, 2009. (In Chinese) [Google Scholar]
- Sun, B.; Guo, Y.; Wang, Z.; Yang, X.; Gong, P.; Wang, J.; Wang, N. Experimental study on the drag coefficient of single bubbles rising in static non-Newtonian fluids in wellbore. J. Nat. Gas Sci. Eng. 2015, 26, 867–872. [Google Scholar] [CrossRef]






| Type | Consistency Factor K (Pa·s−n) | Fluidity Index n | Density (kg·m−3) | Surface Tension (N·m−1) |
|---|---|---|---|---|
| Water | 0.01 | 1 | 1000 | 72.35 |
| 0.05% XC | 0.07224 | 0.51458 | 1000 | 73.67 |
| 0.1% XC | 0.12377 | 0.48544 | 1000 | 74.24 |
| 0.2% XC | 0.34337 | 0.43297 | 1000 | 75.05 |
| 0.4% XC | 1.28381 | 0.34793 | 1000 | 76.75 |
| Type | Range of Bubble Equivalent Radius (mm) | Bubble Velocity Range (mm/s) | ||
|---|---|---|---|---|
| Single Bubble | Bubble Group | Single Bubble | Bubble Group | |
| Water | 0.5–8 | 0.32–1.6 | 170–267 | 110–330 |
| 0.05% XC | 2.7–6.9 | 0.56–1.29 | 213–303 | 100–360 |
| 0.1% XC | 2–9.8 | 0.74–1.43 | 116–259 | 77.9–321 |
| 0.2% XC | 1.9–9.4 | 0.89–1.81 | 95–231 | 106–428 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, H.; Xiang, M.; Lin, Z.; Yang, J.; Wang, X.; Liu, X.; Wang, Z. Study on the Mechanism of Gas Intrusion and Its Transportation in a Wellbore under Shut-in Conditions. Energies 2024, 17, 242. https://doi.org/10.3390/en17010242
Zhu H, Xiang M, Lin Z, Yang J, Wang X, Liu X, Wang Z. Study on the Mechanism of Gas Intrusion and Its Transportation in a Wellbore under Shut-in Conditions. Energies. 2024; 17(1):242. https://doi.org/10.3390/en17010242
Chicago/Turabian StyleZhu, Haifeng, Ming Xiang, Zhiqiang Lin, Jicheng Yang, Xuerui Wang, Xueqi Liu, and Zhiyuan Wang. 2024. "Study on the Mechanism of Gas Intrusion and Its Transportation in a Wellbore under Shut-in Conditions" Energies 17, no. 1: 242. https://doi.org/10.3390/en17010242
APA StyleZhu, H., Xiang, M., Lin, Z., Yang, J., Wang, X., Liu, X., & Wang, Z. (2024). Study on the Mechanism of Gas Intrusion and Its Transportation in a Wellbore under Shut-in Conditions. Energies, 17(1), 242. https://doi.org/10.3390/en17010242







