Effects of Hydrogen Addition on the Thermal Performance and Emissions of Biomass Syngas Combustion in a Horizontal Boiler
Abstract
:1. Introduction
2. Modeling and Solution
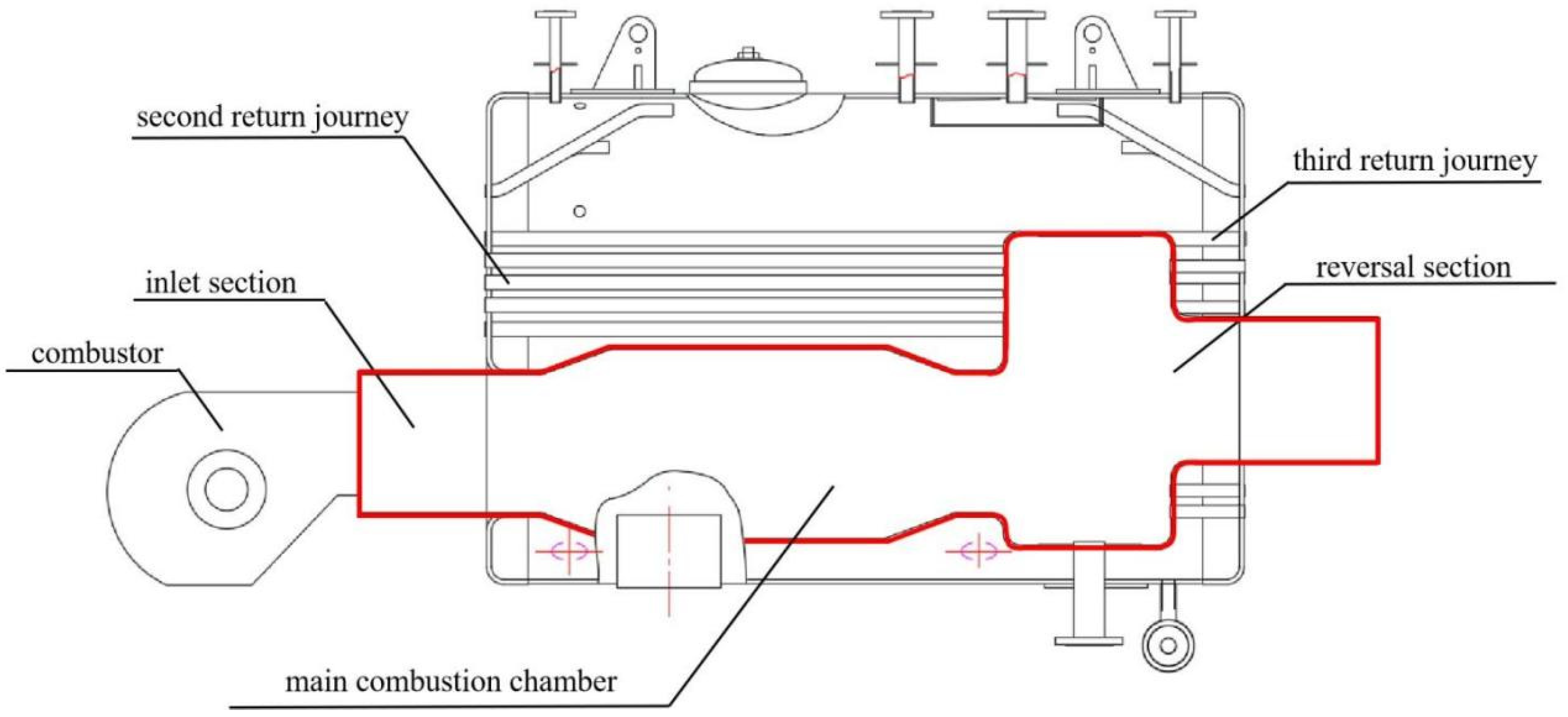
2.1. Geometric Model
2.2. Mathematic Models
2.2.1. Governing Equations
2.2.2. Turbulence Model
2.2.3. Combustion Model
2.2.4. Radiation Model
2.2.5. NOx Generation Model
2.2.6. Field Synergy Principle
2.3. Boundary Conditions and Parameters
2.4. Biogas Parameters
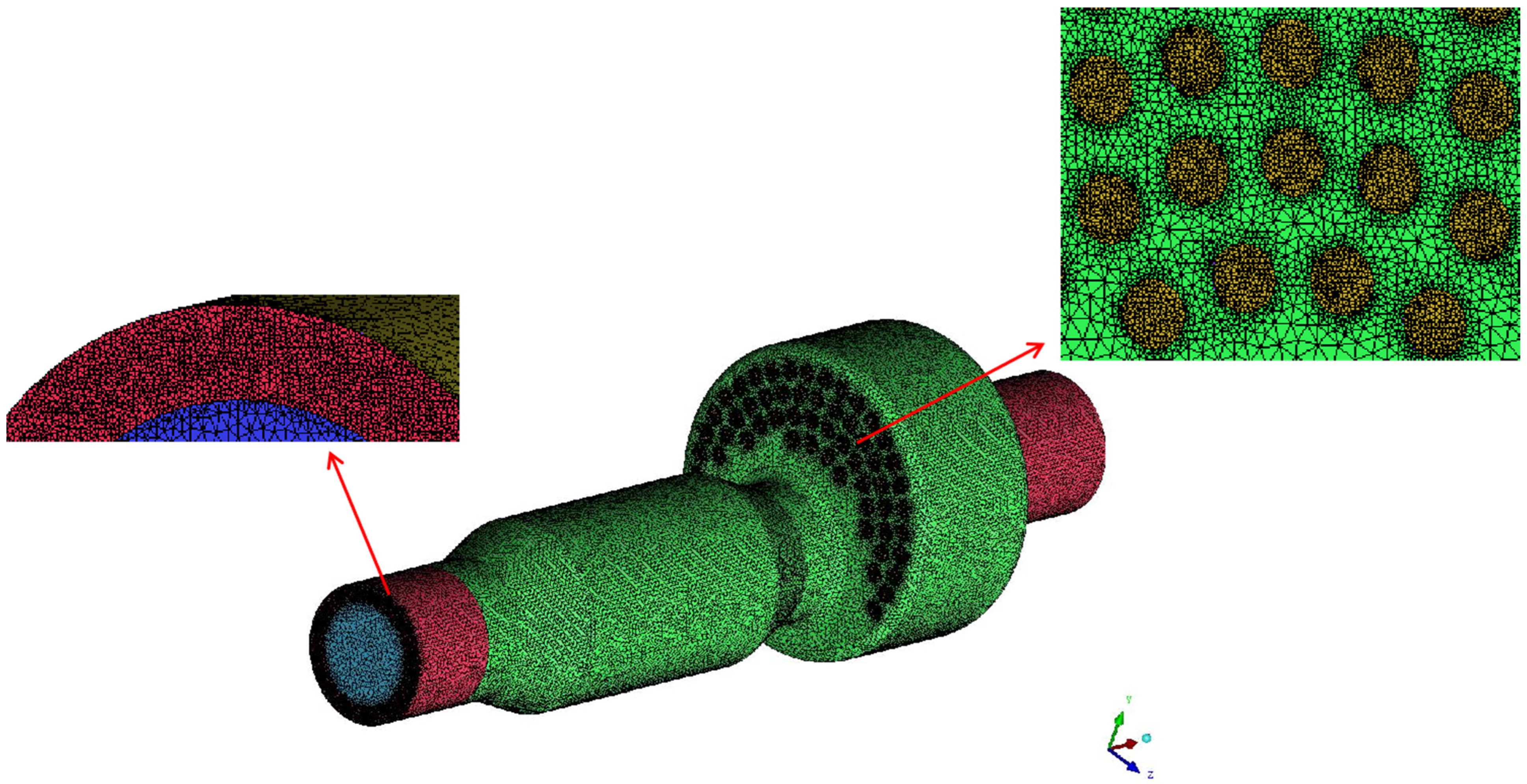
2.5. Grid Independence
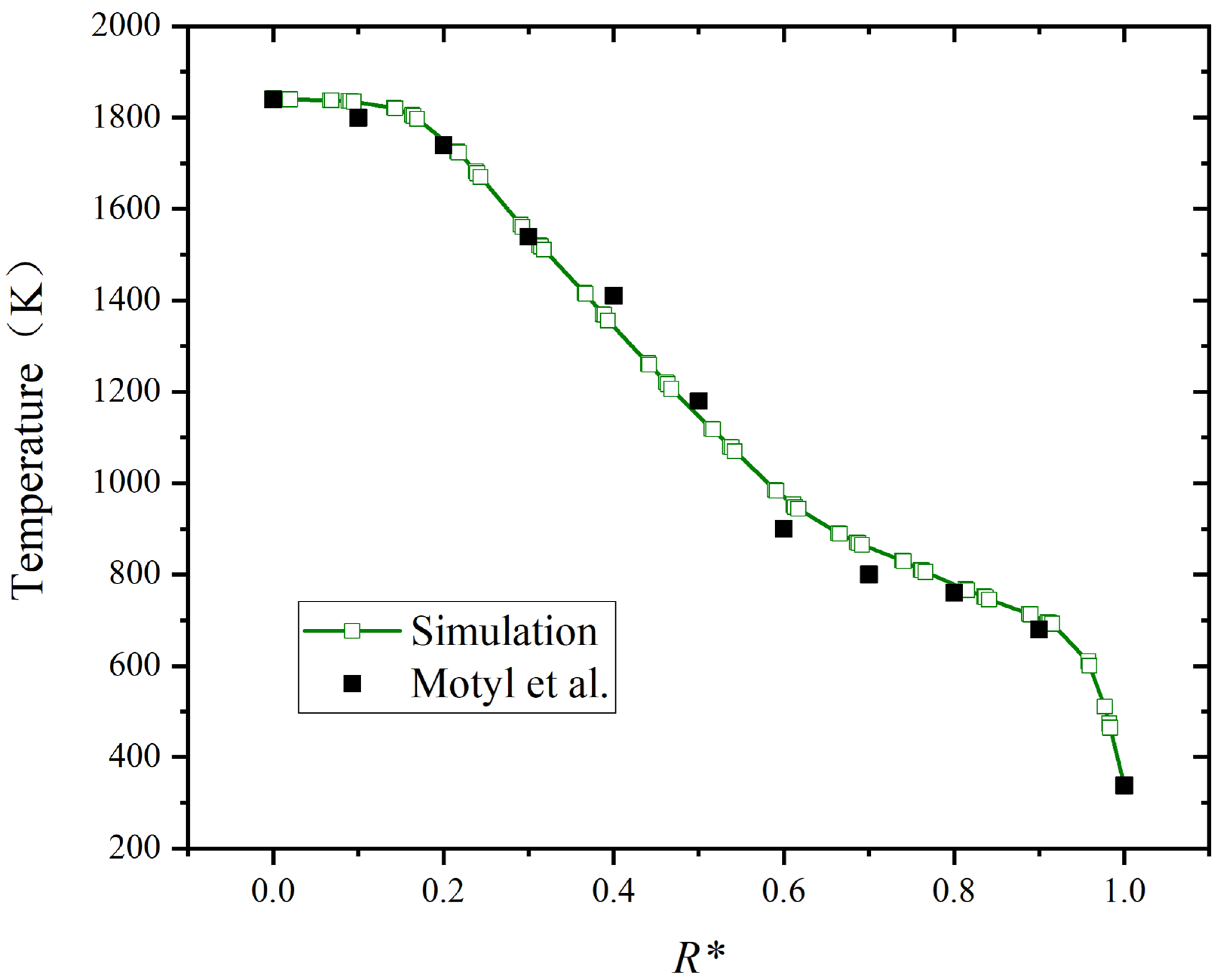
2.6. Numerical Validation
3. Results and Discussion
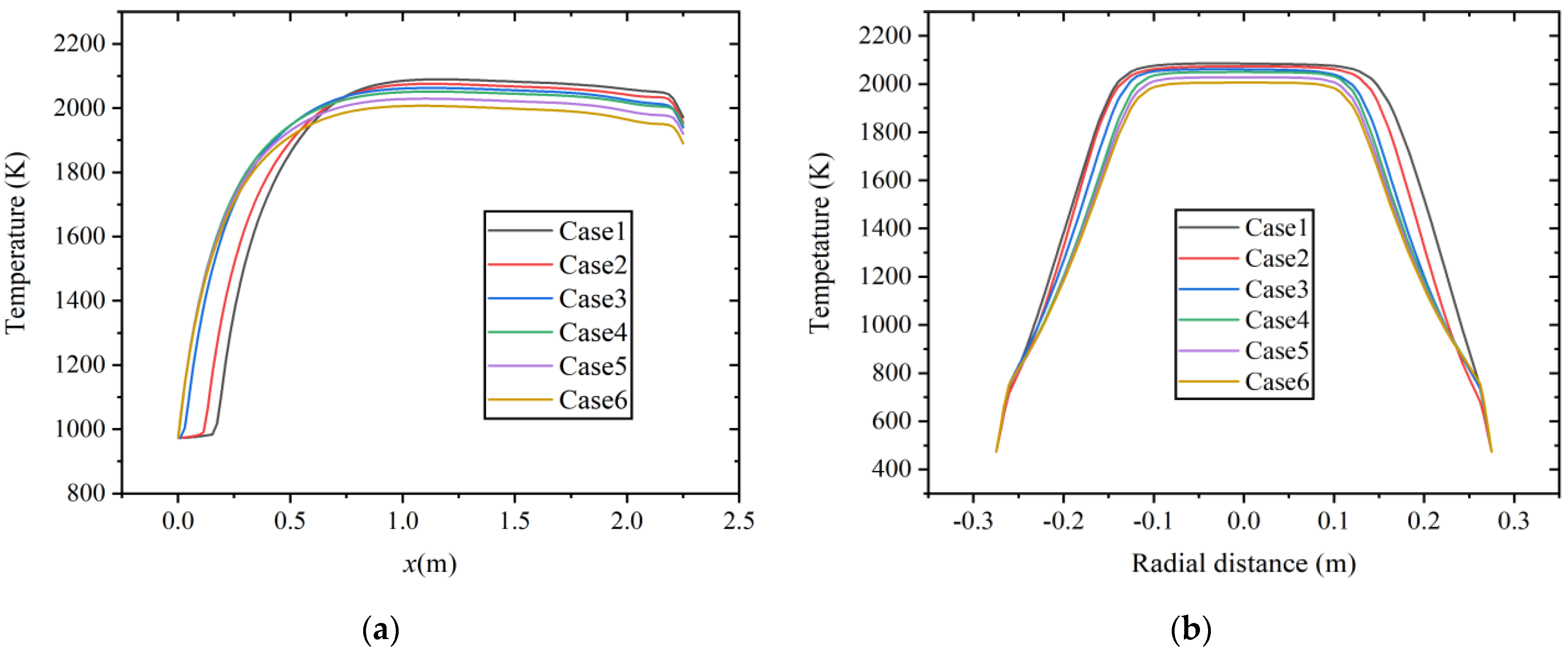
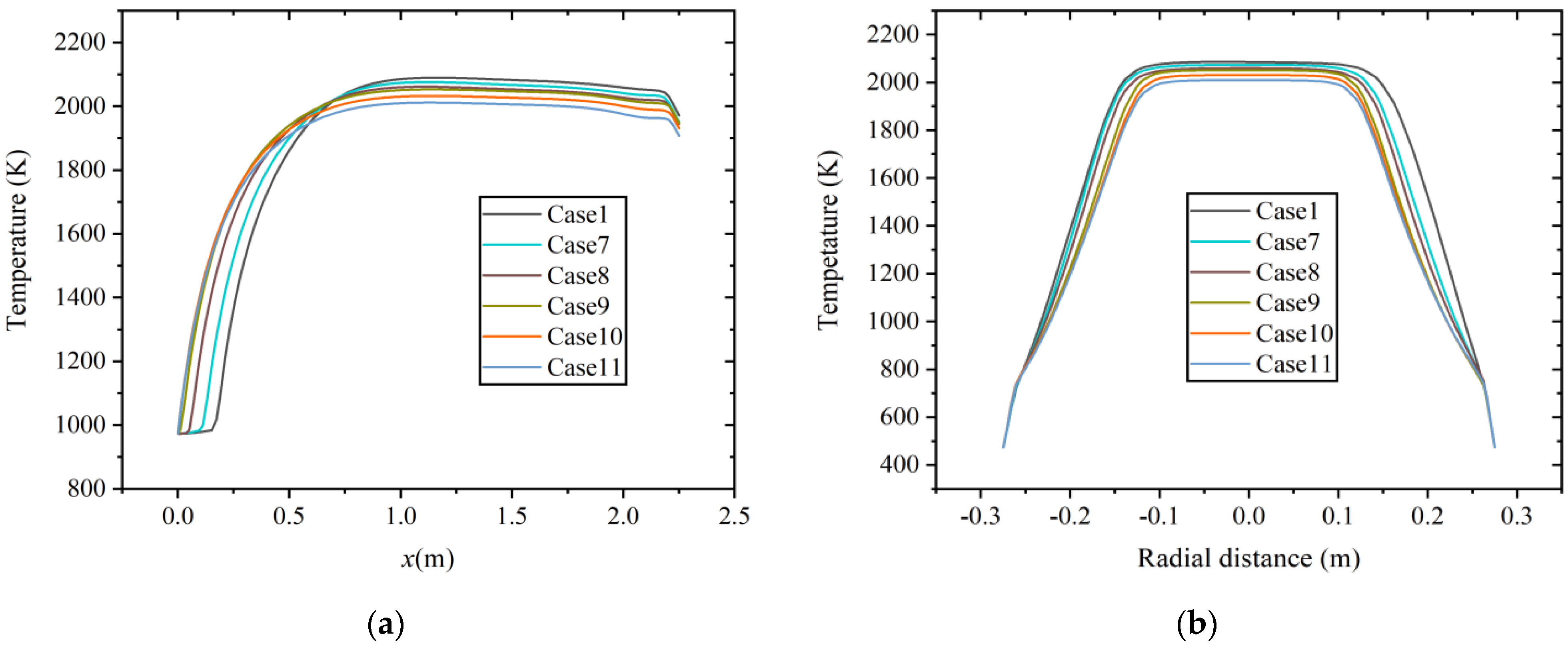
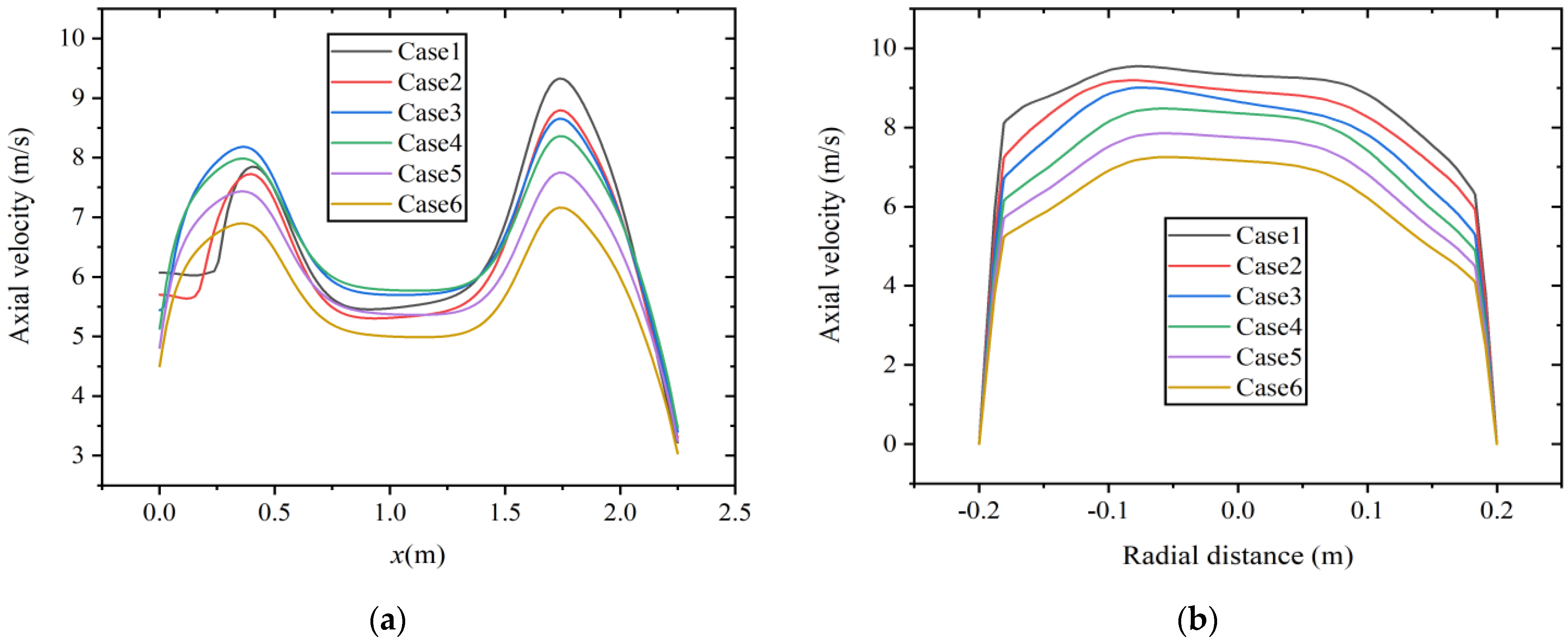
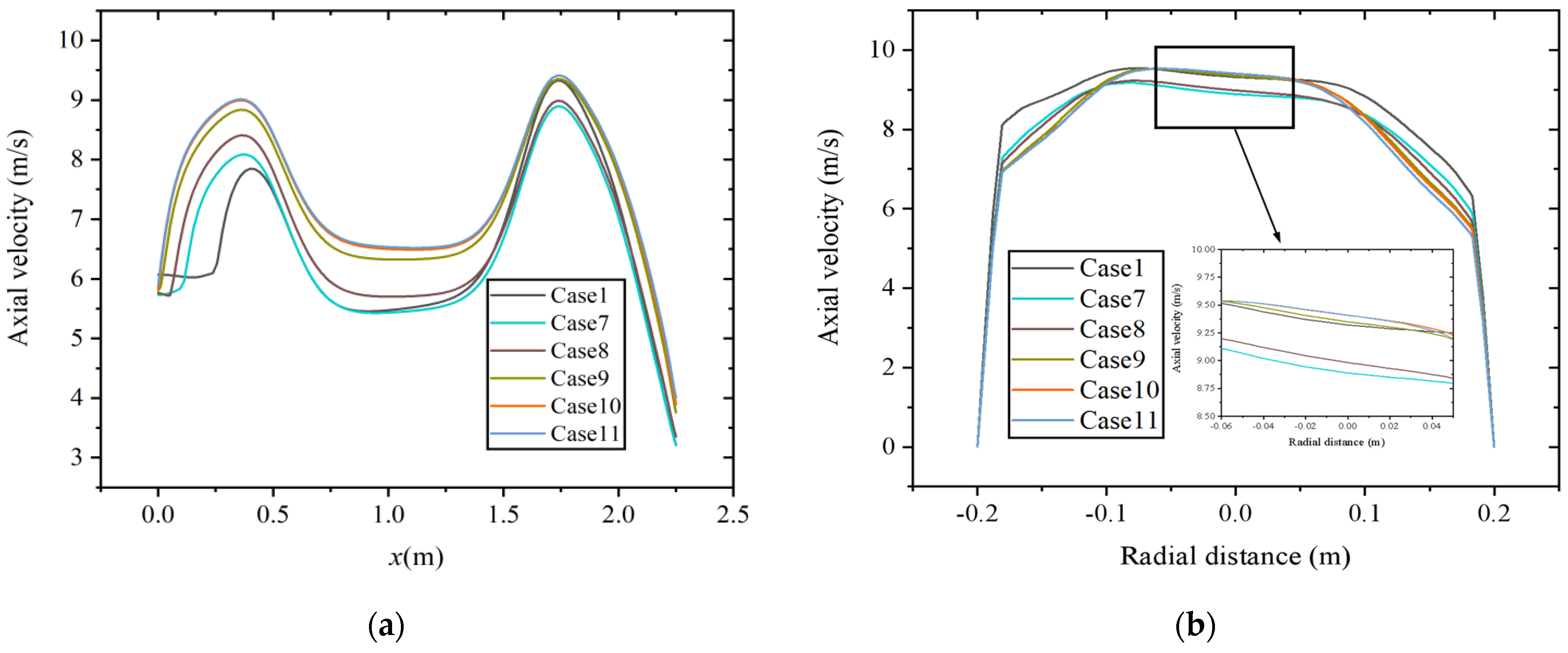
3.1. Temperature Field and Velocity Field
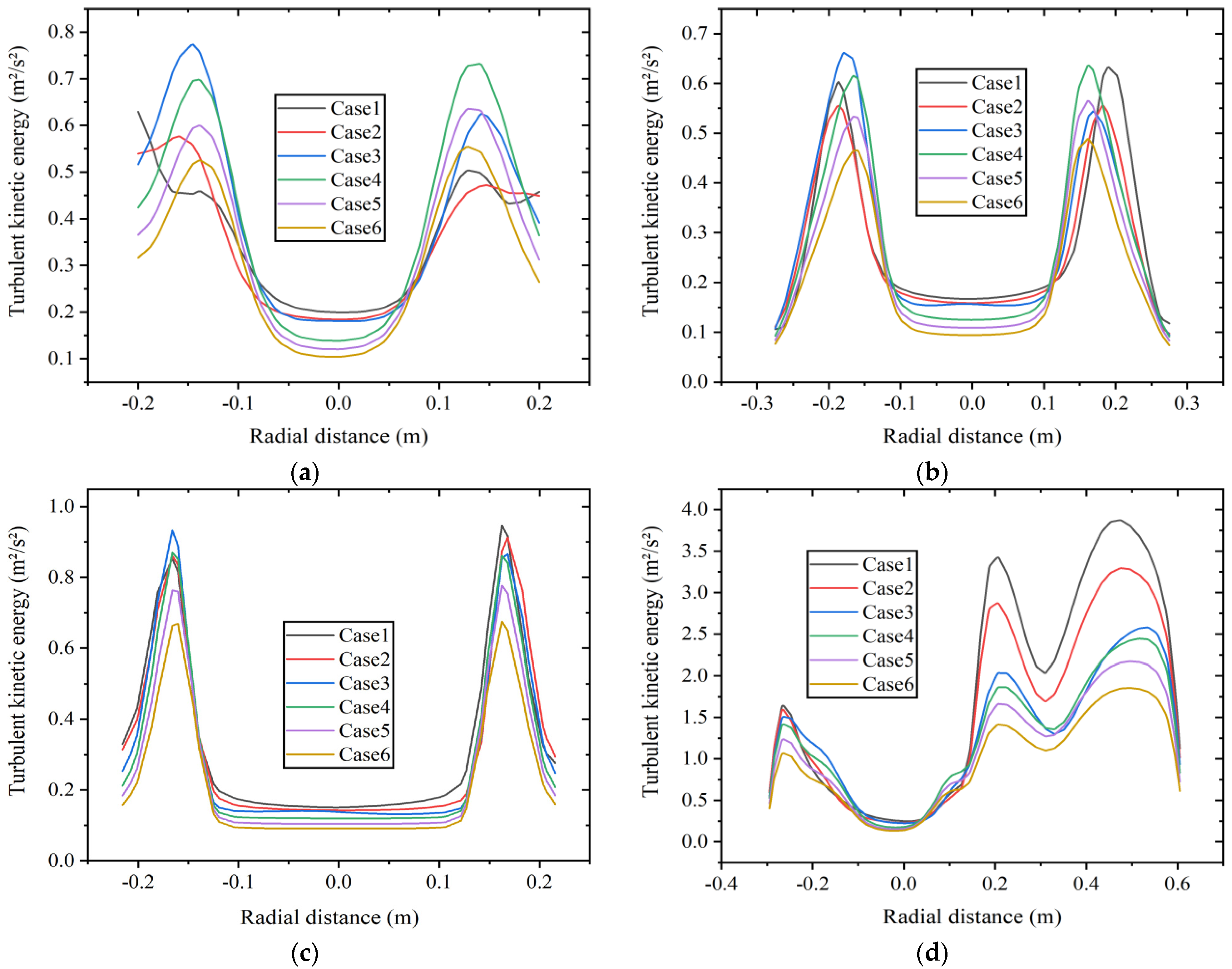
3.2. Turbulent Kinetic Energy
3.3. Field Synergy
3.4. Pollutant Emissions
4. Conclusions
- Temperature and velocity fields are significantly affected due to the addition of hydrogen. The temperature in the combustion chamber shows a decreasing trend as the hydrogen addition ratios increase and the temperature peak moves towards the inlet direction. The reduction in combustion temperature reduces the emission of thermal NOx, resulting in a significant reduction in the overall NOx emission. In addition, hydrogen addition effectively reduces the emissions of pollutants CO and CO2.
- The variation of hydrogen content significantly affects the velocity field and TKE distribution in the combustion chamber. Compared to other positions, the TKE at the position of the reversal section is greater, and the fluid instability is stronger. At a constant fuel volume flow rate, with the increase in hydrogen content, the TKE at the reversal section shows a decreasing trend, which is positively correlated with the trend of velocity changing with hydrogen content at this point. At constant heat input, the average TKE is highest at 5% hydrogen content.
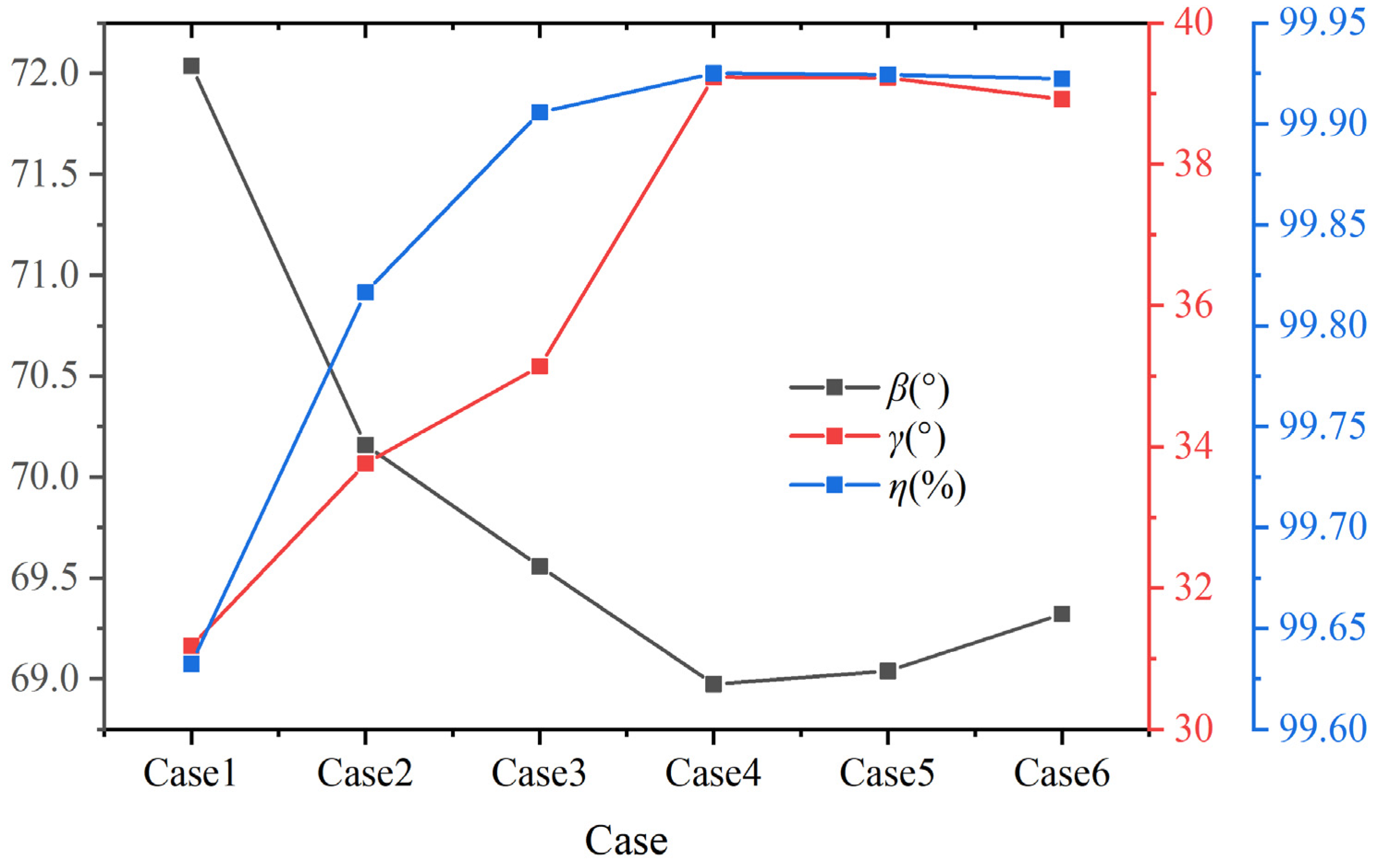
- As the hydrogen content increases, the volume average synergy angle β initially decreases and subsequently increases, while the synergy angle γ and combustion efficiency first increases and then decreases. Hydrogen addition can optimize the heat transfer performance of biomass syngas combustion and improve the comprehensive heat transfer performance, but excessive hydrogen addition may reduce its positive effect. The addition of hydrogen has a slight impact on combustion efficiency, and the deviation between the maximum and minimum values does not exceed 0.3%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Correction Statement
Nomenclature
| linear-anisotropic phase function coefficient | contribution of pulsating expansion associated to the compressible turbulence, kg·m−1s−2 | ||
| specific heat capacity at constant pressure, J/(kg·K) | absorption coefficient | ||
| methane | synergy angle | ||
| carbon monoxide | density, kg/m3 | ||
| carbon dioxide | dynamic viscosity, Pa·s | ||
| strain rate of the flow | turbulent viscosity, m2s | ||
| source terms, kg·m−1s−3 | characteristic time | ||
| source terms, kg·m−1s−3 | viscous stress, Pa | ||
| volumetric stress, Pa | Stephen Boltzmann constant | ||
| incident radiation | turbulent Prandtl number of dissipation rate | ||
| turbulent kinetic energy caused by average velocity gradient, kg·m−1s−2 | turbulent Prandtl number of turbulent flow energy | ||
| hydrogen | scattering coefficient | ||
| turbulent kinetic energy, m2s−2 | combustion efficiency | ||
| mass flow, kg/s | expansion parameter | ||
| nitrogen | turbulent dissipation rate, m2s−3 | ||
| oxides of nitrogen | Levi-Civita symbol in three dimensions | ||
| pressure, Pa | thermal conductivity, W/(m·K) | ||
| heat flux density, W/m2 | characteristic length | ||
| heat effect of chemical reaction of a certain substance, J/m3 | |||
| Schmidt number | |||
| temperature, K | |||
| temperature gradient | Subscripts | ||
| velocity gradient | in | inlet | |
| velocity on the j component, m/s | out | outlet | |
| molecular kinematic viscosity, m2s | r | radiation | |
| chemical reaction rate, kg/(m3·s) | w | wall | |
References
- Wasif, M.Z.; Avik, S.; Zahoor, A.; Quande, Q.; Haider, Z.S.A. Effects of biomass energy consumption on environmental quality: The role of education and technology in Asia-Pacific Economic Cooperation countries. Renew. Sust. Energy Rev. 2021, 142, 110868. [Google Scholar]
- Boyle, G. Renewable Energy, Power for a Sustainable Future; Oxford University Press: Oxford, UK, 1996. [Google Scholar]
- Grammelis, P.; Skodras, G.; Kakaras, E. Effects of biomass co-firing with coal on ash properties. Part I: Characterisation and PSD. Fuel 2006, 85, 2310–2315. [Google Scholar] [CrossRef]
- Dasappa, S. Potential of biomass energy for electricity generation in sub-Saharan Africa. Energy Sustain. Dev. 2011, 15, 203–213. [Google Scholar] [CrossRef]
- Xiao, L.; Ha, Y.; Meng, F.; Liang, J.; Wang, P. Research and application progress on biomass gasification technologies. Mod. Chem. Ind. 2020, 40, 68–72. [Google Scholar]
- Cao, Y.; Wang, Y.; Riley, T.J.; Pan, W.P. A novel biomass air gasification process for producing tar-free higher heating value fuel gas. Fuel Process Technol. 2006, 87, 343–353. [Google Scholar] [CrossRef]
- Liu, A.; Fan, R.; Liu, Q.; Xi, L.; Zeng, W. Numerical and Experimental Study on Combustion Characteristics of Micro-Gas Turbine Biogas Combustor. Energies 2022, 15, 8302. [Google Scholar] [CrossRef]
- Hanaoka, T.; Inoue, S.; Uno, S.; Ogi, T.; Minowa, T. Effect of woody biomass components on air-steam gasification. Biomass Bioenergy 2005, 28, 69–76. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, G.; Wang, F.; Deng, H.; Wen, X. Experimental and model analyses of laminar combustion characteristics of variable composition CO/H2/CH4 mixtures at high N2 and CO2 concentrations. Int. J. Energy Res. 2020, 44, 7507–7524. [Google Scholar] [CrossRef]
- Wang, F.; Chen, Y.; Zhang, D.; Weng, X.; Zhang, A. Effect of hydrogen addition on the laminar combustion characteristics of biomass syngas. J. Saf. Environ. 2023, 23, 943–951. [Google Scholar]
- Liu, X. Study on the Chemical Reaction Mechanism of Biogas and Simulation of in Cylinder Combustion Process; Harbin Institute of Technology: Harbin, China, 2016. [Google Scholar]
- Khaleghi, M.; Hosseini, E.S.; Wahid, M. Experimental and numerical investigations of biogas vortex combustion. Proc. Inst. Mech. Eng. Part A J. Power Energy 2015, 229, 662–676. [Google Scholar] [CrossRef]
- Ilker, Y.; Yakup, C.; Bugrahan, A. Effect of N2 dilution on combustion instabilities and emissions in biogas flame. Fuel 2022, 308, 121943. [Google Scholar]
- Hu, Z.; Zhang, X. Experimental study on flame stability of biogas/hydrogen combustion. Int. J. Hydrogen Energy 2018, 44, 5607–5614. [Google Scholar] [CrossRef]
- Mordaunt, J.C.; Pierce, C.W. Design and preliminary results of an atmospheric-pressure model gas turbine combustor utilizing varying CO2 doping concentration in CH4 to emulate biogas combustion. Fuel 2014, 124, 258–268. [Google Scholar] [CrossRef]
- Xie, Y.; Qin, C.; Chen, Z.; Duan, P.; Guo, S. The impact of hydrogen addition to natural gas on flame stability. Int. J. Hydrogen Energy 2022, 47, 35851–35863. [Google Scholar] [CrossRef]
- Khare, R.; Vlavakis, P.; Von, L.T.; Loukou, A.; Khoseavi, M. Experimental investigation of the effect of hydrogen addition on the sooting limit and structure of methane/air laminar counterflow diffusion flames. Fuel 2022, 324, 124506. [Google Scholar] [CrossRef]
- Xu, C.; Wang, Q.; Li, X.; Liu, K.; Liu, W. Effect of hydrogen addition on the laminar burning velocity of n-decane/air mixtures: Experimental and numerical study. Int. J. Hydrogen Energy 2022, 47, 19263–19274. [Google Scholar] [CrossRef]
- Antonio, M.; Mario, M.; Andrea, U. Use of biogas containing CH4, H2 and CO2 in controlled auto-ignition engines to reduce NOx emissions. Fuel 2021, 301, 120925. [Google Scholar]
- Zhen, H.; Leung, C.; Cheung, C. Effects of hydrogen addition on the characteristics of a biogas diffusion flame. Int. J. Hydrogen Energy 2013, 38, 6874–6881. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, J.; Ju, D.; Huang, Z.; Han, D. Analysis of exergy losses in laminar premixed flames of methane/hydrogen blends. Int. J. Hydrogen Energy 2019, 44, 24043–24053. [Google Scholar] [CrossRef]
- Zhao, T.; Zhang, J.; Ju, D.; Huang, Z.; Han, D. Exergy losses in premixed flames of dimethyl ether and hydrogen blends. Front. Energy 2019, 13, 658–666. [Google Scholar] [CrossRef]
- China Hydrogen Energy Alliance. White Paper on China’s Hydrogen Energy and Fuel Cell Industry 2019 [EB/OL]. Available online: https://www.ligongku.com/resource/94551 (accessed on 24 May 2024).
- Sun, D.; Yan, W. Research on the Development Status and Whole Industry Chain Construction of Natural Gas Hydrogen Blended Power Generation. China Offshore Oil Gas 2023, 35, 189–197. [Google Scholar]
- Xi, X.; Tian, C.; Kuanliang, W. Effects of hydrogen addition on oblique detonations in methane–air mixtures. Int. J. Hydrogen Energy 2022, 47, 8621–8629. [Google Scholar] [CrossRef]
- Fu, Z.; Sui, L.; Lu, J.; Liu, J.; Weng, P. Investigation on effects of hydrogen addition to the thermal performance of a traditional counter-flow combustor. Energy 2023, 262, 125465. [Google Scholar] [CrossRef]
- Shih, H.; Liu, C. A computational study on the combustion of hydrogen/methane blended fuels for a micro gas turbines. Int. J. Hydrogen Energy 2014, 39, 15103–15115. [Google Scholar] [CrossRef]
- Meziane, S.; Bentebbiche, A. Numerical study of blended fuel natural gas-hydrogen combustion in rich/quench/lean combustor of a micro gas turbine. Int. J. Hydrogen Energy 2019, 44, 15610–15621. [Google Scholar] [CrossRef]
- Verma, S.; Das, L.; Kaushik, S.; Tyagi, S. An experimental investigation of exergetic performance and emission characteristics of hydrogen supplemented biogas-diesel dual fuel engine. Int. J. Hydrogen Energy 2018, 43, 2452–2468. [Google Scholar] [CrossRef]
- Porpatham, E.; Ramesh, A.; Nagalingam, B. Effect of swirl on the performance and combustion of a biogas fuelled spark ignition engine. Energy Convers. Manag. 2013, 76, 463–471. [Google Scholar] [CrossRef]
- Bora, B.J.; Saha, U.K.; Chatterjee, S.; Veer, V. Effect of compression ratio on performance, combustion and emission characteristics of a dual fuel diesel engine run on raw biogas. Energy Convers. Manag. 2014, 87, 1000–1009. [Google Scholar] [CrossRef]
- Yi, O.; Tang, K.; Yang, X.; Zou, H.; Chu, G. Evaluation of various turbulence models for numerical simulation of a multiphase system in a rotating packed bed. Comput. Fluids 2019, 194, 104296. [Google Scholar]
- Wang, Y.; Xu, Z.; Liu, J.; Zhang, A.; Xu, Z.; Meng, D.; Zhao, J. Study on flow field of electrochemical machining for large size blade. Int. J. Mech. Sci. 2021, 190, 106018. [Google Scholar] [CrossRef]
- Li, J.; Wang, D.; Zhu, D.; He, B. Analysis of the flow field in counter-rotating electrochemical machining. J. Mater. Process. Technol. 2020, 275, 116323. [Google Scholar] [CrossRef]
- Magnussen, B.F.; Hjertager, B.H. On mathematical modeling of turbulent combustion with special emphasis on soot formation and combustion. Symp. Combust. 1977, 16, 719–729. [Google Scholar] [CrossRef]
- Spalding, D.B. Mixing and chemical reaction in steady confined turbulent flames. Symp. Combust. 1971, 13, 649–657. [Google Scholar] [CrossRef]
- Magnussen, B. On the structure of turbulence and a generalized eddy dissipation concept for chemical reaction in turbulent flow. Otolaryngol.-Head Neck Surg. Off. J. Am. Acad. Otolaryngol.-Head Neck Surg. 1981, 140, 433–434. [Google Scholar]
- Fu, Z.; Gao, H.; Zeng, Z.; Liu, J.; Zhu, Q. Generation characteristics of thermal NOx in a double-swirler annular combustor under various inlet conditions. Energy 2020, 200, 117487. [Google Scholar] [CrossRef]
- Yao, P.; Zhai, Y.; Li, Z.; Shen, X.; Wang, H. Thermal performance analysis of multi-objective optimized microchannels with triangular cavity and rib based on field synergy principle. Case Stud. Therm. Eng. 2021, 25, 100963. [Google Scholar]
- Porpatham, E.; Ramesh, A.; Nagalingam, B. Effect of compression ratio on the performance and combustion of a biogas fuelled spark ignition engine. Fuel 2012, 95, 247–256. [Google Scholar] [CrossRef]
- Henriksen, U.; Ahrenfeldt, J.; Jensen, T.K.; Gobel, B.; Bentzen, J.D. The design, construction and operation of a 75 kW two-stage gasifier. Energy 2006, 31, 1542–1553. [Google Scholar] [CrossRef]
- Motyl, P.; Król, D.; Poskrobko, S.; Juszczak, M. Numerical Modelling and Experimental Verification of the Low-Emission Biomass Combustion Process in a Domestic Boiler with Flue Gas Flow around the Combustion Chamber. Energies 2020, 13, 5837. [Google Scholar] [CrossRef]
- Liu, W.; Liu, Z.; Ma, L. Application of a multi-field synergy principle in the performance evaluation of convective heat transfer enhancement in a tube. Chin. Sci. Bull. 2012, 57, 1600–1607. [Google Scholar] [CrossRef]
- E, J.; Zuo, W.; Liu, H.; Peng, Q. Field synergy analysis of the micro-cylindrical combustor with a step. Appl. Therm. Eng. 2016, 93, 83–89. [Google Scholar]
- Du Toit, M.; Engelbrecht, N.; Oelofse, S.P.; Bessarabov, D. Performance evaluation and emissions reduction of a micro gas turbine via the co-combustion of H2/CH4/CO2 fuel blends. Sustain. Energy Technol. Assess. 2020, 39, 100718. [Google Scholar] [CrossRef]
- Li, Y. Research and Numerical Simulation on the Combustion Characteristics of Biomass Gasification Gas; Zhejiang University: Hangzhou, China, 2019. [Google Scholar]








| Reaction | Reaction | Reaction | |||
|---|---|---|---|---|---|
| 1 | O + H2⇔H + OH | 19 | 2OH(+M)⇔H2O2(+M) | 37 | CH3 + CH2O⇔HCO + CH4 |
| 2 | O + CH3⇔H + CH2O | 20 | 2OH⇔O + H2O | 38 | HCO + H2O⇔H + CO + H2O |
| 3 | O + CH4⇔OH + CH3 | 21 | OH + HO2⇔O2 + H2O | 39 | HCO + M⇔H + CO + M |
| 4 | O + CO(+M)⇔CO2(+M) | 22 | OH + CH4⇔CH3 + H2O | 40 | HCO + O2⇔HO2 + CO |
| 5 | O + CH2O⇔OH + HCO | 23 | OH + CO⇔H + CO2 | 41 | N + NO⇔N2 + O |
| 6 | O2 + CO⇔O + CO2 | 24 | 2HO2⇔O2 + H2O2 | 42 | N + O2⇔NO + O |
| 7 | O2 + CH2O⇔HO2 + HCO | 25 | 2HO2⇔O2 + H2O2 | 43 | N + OH⇔NO + H |
| 8 | H + O2 + M⇔HO2 + M | 26 | HO2 + CH3⇔O2 + CH4 | 44 | HO2 + NO⇔NO2 + OH |
| 9 | H + 2O2⇔HO2 + O2 | 27 | HO2 + CH3⇔OH + CH3O | 45 | NO + O + M⇔NO2 + M |
| 10 | H + O2 + H2O⇔HO2 + H2O | 28 | HO2 + CO⇔OH + CO2 | 46 | NO2 + O⇔NO + O2 |
| 11 | H + O2 + N2⇔HO2 + N2 | 29 | HO2 + CH2O⇔HCO + H2O2 | 47 | NO2 + H⇔NO + OH |
| 12 | H + O2⇔O + OH | 30 | CH + CO2⇔HCO + CO | 48 | NNH + O⇔NH + NO |
| 13 | H + HO2⇔O2 + H2 | 31 | CH2 + O2⇔OH + H + CO | 49 | H + NO + M⇔HNO + M |
| 14 | H + H2O2⇔HO2 + H2 | 32 | CH2(S) + CO2⇔CO + CH2O | 50 | HNO + H⇔H2 + NO |
| 15 | H + CH3(+M)⇔CH4(+M) | 33 | CH3 + O2⇔O + CH3O | 51 | N + CO2⇔NO + CO |
| 16 | H + CH4⇔CH3 + H2 | 34 | CH3 + O2⇔OH + CH2O | 52 | O + CH3⇔H + H2 + CO |
| 17 | H + CH2O⇔HCO + H2 | 35 | CH3 + H2O2⇔HO2 + CH4 | 53 | OH + HO2⇔O2 + H2O |
| 18 | OH + H2⇔H + H2O | 36 | 2CH3(+M)⇔C2H6(+M) | 54 | CH2 + O2⇔2H + CO2 |
| Section | Parameter | Symbol | Value | Unit |
|---|---|---|---|---|
| Fuel-inlet | Fuel temperature | Tf,in | 973 | K |
| Air-inlet | Air temperature | Tair,in | 343 | K |
| Wall (in contact with water) | Water temperature | Tw | 473 | K |
| Wall (not in contact with water) | Heat flux density | qw | 1000 | W/m2 |
| Outlet | Pressure | Pout | 50 | Pa |
| Case | Fuel Composition (vol%) | LHV (kJ/Nm3) | Heat Input (kW) | Fuel Volumetric Flow (Nm3/h) | ||||
|---|---|---|---|---|---|---|---|---|
| CH4 | CO | H2 | CO2 | N2 | ||||
| 1 | 6 | 24 | 5 | 15 | 50 | 5734 | 777.78 | 488.31 |
| 2 | 5 | 20 | 10 | 15 | 50 | 5409 | 733.68 | |
| 3 | 4 | 16 | 15 | 15 | 50 | 5083 | 689.59 | |
| 4 | 3 | 12 | 20 | 15 | 50 | 4758 | 645.49 | |
| 5 | 2 | 8 | 25 | 15 | 50 | 4433 | 601.39 | |
| 6 | 1 | 4 | 30 | 15 | 50 | 4108 | 557.29 | |
| Case | Fuel Composition(vol%) | LHV (kJ/Nm3) | Heat Input (kW) | Fuel Mass Flow (kg/s) | ||||
|---|---|---|---|---|---|---|---|---|
| CH4 | CO | H2 | CO2 | N2 | ||||
| 1 | 6 | 24 | 5 | 15 | 50 | 5734 | 777.78 | 0.0486 |
| 7 | 5 | 20 | 10 | 15 | 50 | 5409 | 0.0493 | |
| 8 | 4 | 16 | 15 | 15 | 50 | 5083 | 0.0502 | |
| 9 | 3 | 12 | 20 | 15 | 50 | 4758 | 0.0512 | |
| 10 | 2 | 8 | 25 | 15 | 50 | 4433 | 0.0523 | |
| 11 | 1 | 4 | 30 | 15 | 50 | 4108 | 0.0536 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suxing, S.; Yu, X.; Li, J.; Liu, X.; Sui, L.; Zhang, J.; Fu, Z.; Shao, Y. Effects of Hydrogen Addition on the Thermal Performance and Emissions of Biomass Syngas Combustion in a Horizontal Boiler. Energies 2024, 17, 2632. https://doi.org/10.3390/en17112632
Suxing S, Yu X, Li J, Liu X, Sui L, Zhang J, Fu Z, Shao Y. Effects of Hydrogen Addition on the Thermal Performance and Emissions of Biomass Syngas Combustion in a Horizontal Boiler. Energies. 2024; 17(11):2632. https://doi.org/10.3390/en17112632
Chicago/Turabian StyleSuxing, Shengnan, Xiao Yu, Jinze Li, Xuelai Liu, Lichao Sui, Jingkui Zhang, Zaiguo Fu, and Yan Shao. 2024. "Effects of Hydrogen Addition on the Thermal Performance and Emissions of Biomass Syngas Combustion in a Horizontal Boiler" Energies 17, no. 11: 2632. https://doi.org/10.3390/en17112632
APA StyleSuxing, S., Yu, X., Li, J., Liu, X., Sui, L., Zhang, J., Fu, Z., & Shao, Y. (2024). Effects of Hydrogen Addition on the Thermal Performance and Emissions of Biomass Syngas Combustion in a Horizontal Boiler. Energies, 17(11), 2632. https://doi.org/10.3390/en17112632






