Energy, Economic and Environmental (3E) Analysis for an Optimal CSP Technology Integration in Morocco
Abstract
:1. Introduction
2. Materials and Methods
2.1. Field of Study
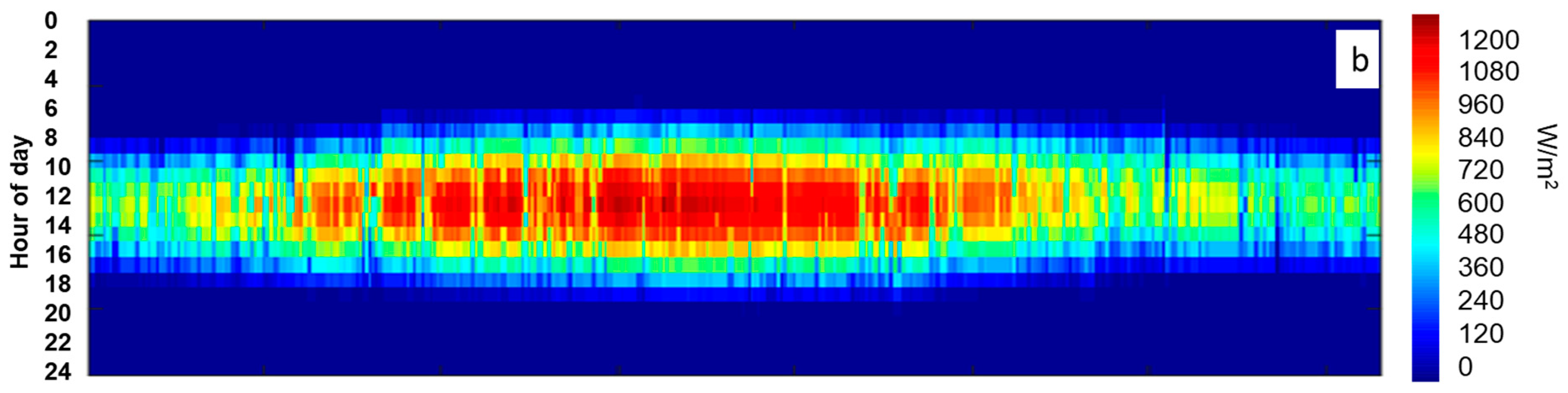
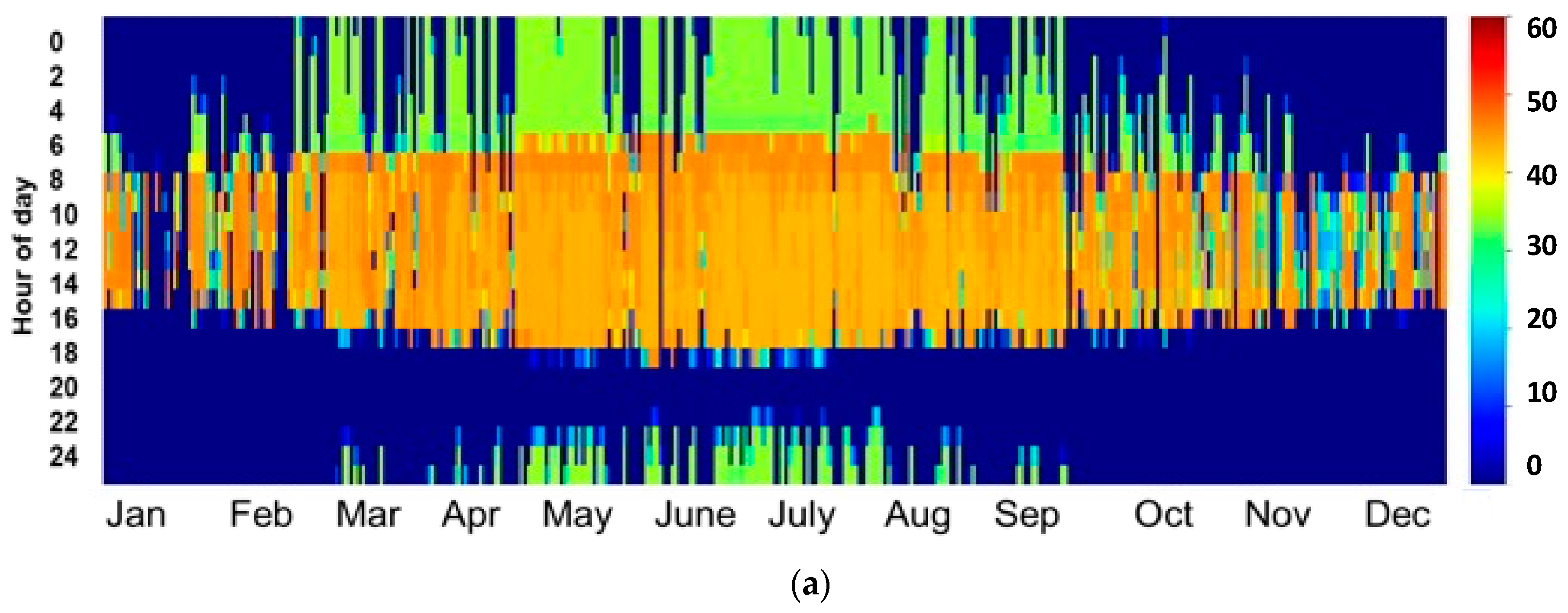
2.2. Solar Resource Assessment
3. Model Description, Experimental Validation and Plant Optimization
3.1. Model Validation
3.2. Case Study: Andasol
3.3. Economical Parameters
3.4. Power Plant Sizing and Optimization
3.5. Heat Transfer Fluid Descriptions
3.5.1. Therminol VP-1
3.5.2. DOWTHERM A
3.5.3. Hitec-Salt
3.5.4. Solar Salt
3.5.5. Syltherm 800
3.6. Selecting the Best Heat Transfer Fluid
4. Results and Discussion
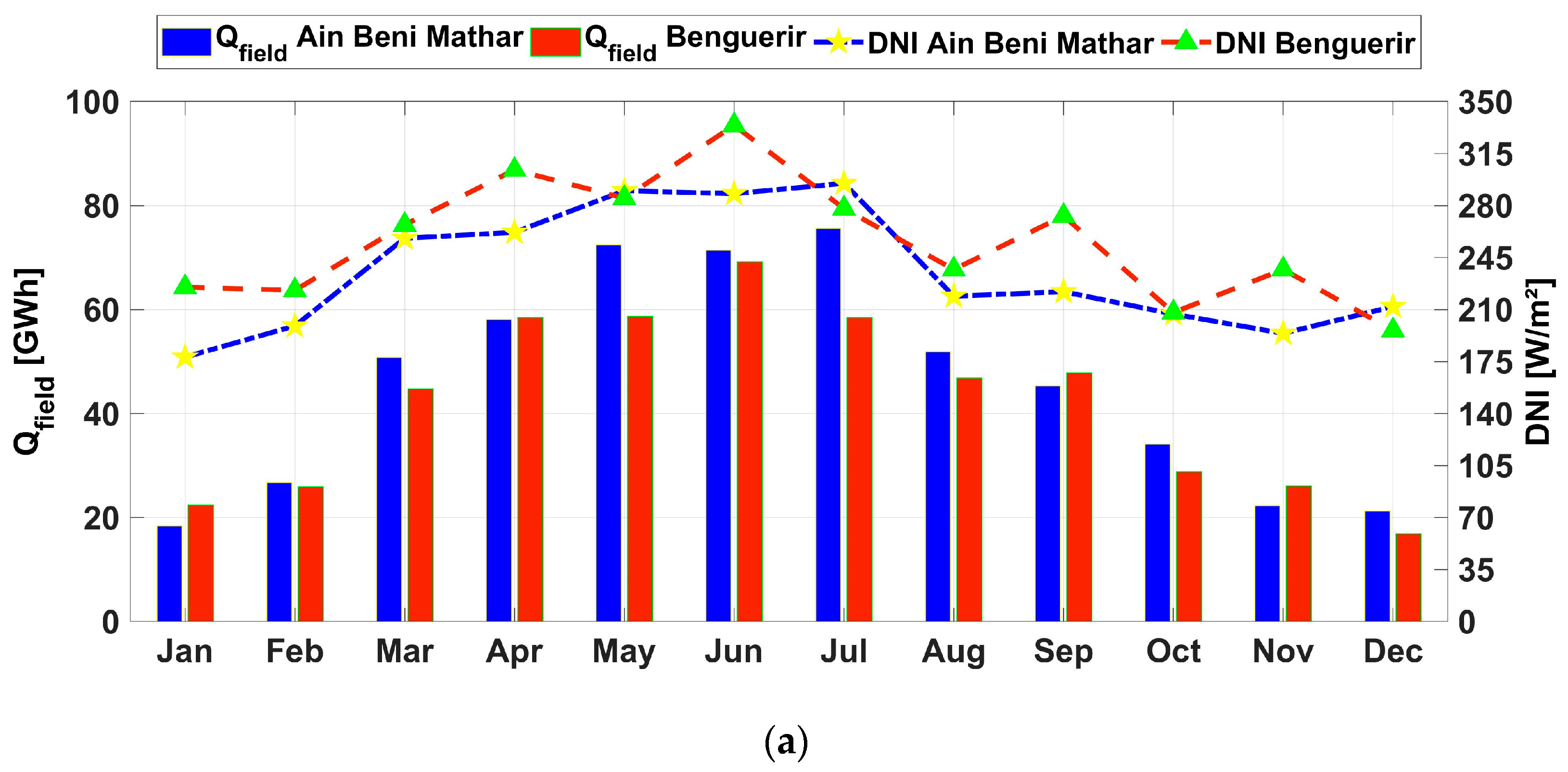
4.1. Yield and Performance Analysis
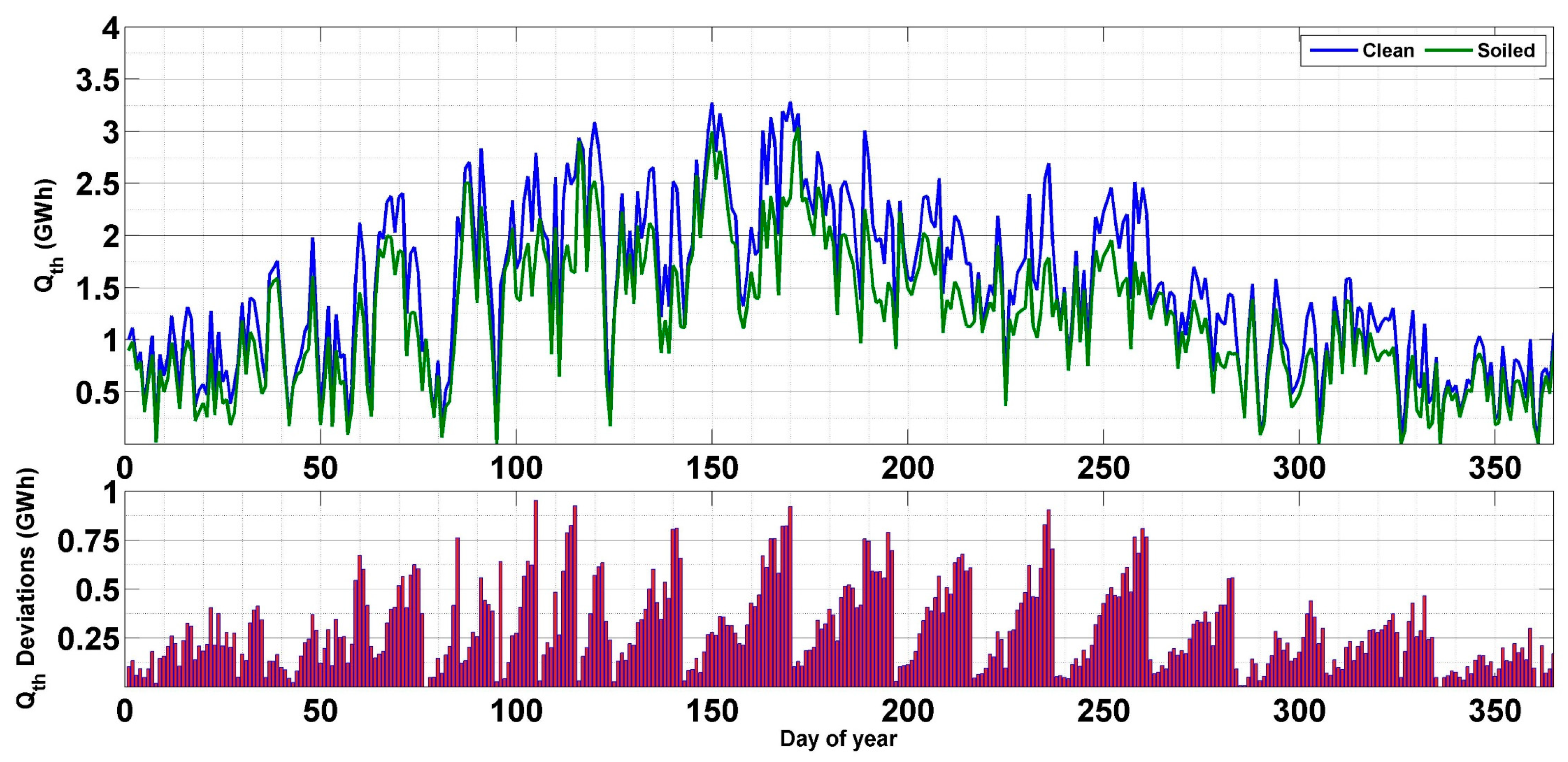
4.2. Soiling Effect
5. Conclusions
- ▪
- For optimal operation from a techno-economic point of view, the configuration of the 50 MWe power plant at Ain Beni Mathar should have an SM of 2.5 and a TES of 7.5 h. As for Benguerir, the SM and the TES should be 7.5 h and 2.5, respectively.
- ▪
- Morocco has great potential for CSP applications, where the optimized plant configurations can produce daily thermal energy reaching 360 MWhth at Ain Beni Mathar and approximately 300 MWhth at Benguerir.
- ▪
- The daily electrical production from the proposed configurations can reach 8.47 GWhe for Ani Beni Mathar and 8.2 GWhe for Benguerir, with DNI values of 427 W/m2 and 433 W/m2, respectively.
- ▪
- The thermal storage system is considered very reliable, where it enables the plant to keep producing electricity at a medium load for up to 9 h (from 19:00 to 5:00) after sunset at Benguerir and up to 8 h (from 19:00 to 5:00) at Ain Beni Mathar.
- ▪
- For Ain Beni Mathar, the most efficient production was recorded during July, where the monthly thermal efficiency was around 56%, and the total efficiency was around 16% for a DNI average of 295 W/m2. Regarding Benguerir, June was the most efficient month with a DNI of 334 W/m2 and thermal and electrical efficiency of 58% and 19%, respectively.
- ▪
- Soiling is a highly efficiency-limiting factor, where the daily drop in the mirror reflectivity can reach 38% after only 2 weeks of exposition without cleaning during the dry period of the year.
- ▪
- Technically, soiling causes annual thermal and electrical loses of 109 GWhth and 30.3 GWhe. This represents 20% and 17% losses in annual production under the nominal conditions.
- ▪
- From an economic point of view, soiling causes a significant increase in the LCOE where the value jumps from 0.1465 €/kWh to 0.1771 €/kWh, which represents a rise of 20% due to soiling.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| DNI | Direct normal irradiance (W/m2) |
| GHI | Global horizontal irradiance (W/m2) |
| IAM | Incidence angle modifier (Deg°) |
| DHI | Diffuse horizontal irradiance (W/m2) |
| Wel | Electrical output (Whe) |
| Qth | Thermal output (Whth) |
| ηth | Optical efficiency (%) |
| ηtot | Total efficiency (%) |
| LCOE | Levelized cost of electricity (€/Wh) |
| CSP | Concentrated solar power plant |
| HTF | Heat transfer fluid |
| TES | Thermal energy storage |
| SM | Solar Multiple |
| N-loops | Number of loops |
References
- Kanwal, S.; Mehran, M.T.; Hassan, M.; Anwar, M.; Naqvi, S.R.; Khoja, A.H. An integrated future approach for the energy security of Pakistan: Replacement of fossil fuels with syngas for better environment and socio-economic development. Renew. Sustain. Energy Rev. 2022, 156, 111978. [Google Scholar] [CrossRef]
- Dincer, I.; Acar, C. Review and evaluation of hydrogen production methods for better sustainability. Int. J. Hydrogen Energy 2014, 40, 11094–11111. [Google Scholar] [CrossRef]
- Pudukudy, M.; Yaakob, Z.; Mohammad, M.; Narayanan, B.; Sopian, K. Renewable hydrogen economy in Asia—Opportunities and challenges: An overview. Renew. Sustain. Energy Rev. 2014, 30, 743–757. [Google Scholar] [CrossRef]
- REN21. Renewables 2020 Global Status Report; 2020. Available online: https://www.ren21.net/wp-content/uploads/2019/05/gsr_2020_full_report_en.pdf (accessed on 6 June 2024).
- Yu, Q.; Hao, Y.; Ali, K.; Hua, Q.; Sun, L. Techno-economic analysis of hydrogen pipeline network in China based on levelized cost of transportation. Energy Convers. Manag. 2024, 301, 118025. [Google Scholar] [CrossRef]
- Amrani, S.-E.; Merrouni, A.A.; Touili, S.; Ouali, H.A.L.; Dekhissi, H. An AHP-GIS combination for site suitability analysis of hydrogen production units from CSP & PV solar power plants in Morocco. Int. J. Hydrogen Energy 2024, 56, 369–382. [Google Scholar] [CrossRef]
- IRENA. Renewable Power Generation Costs in 2019; International Renewable Energy Agency: Abu Dhabi, United Arab Emirates, 2020. [Google Scholar]
- IRENA. Concentrating Solar Power Technology Brief; IRENA: Abu Dhabi, United Arab Emirates, 2012; Available online: https://www.irena.org/publications/2013/Jan/Concentrated-Solar-Power (accessed on 6 June 2024).
- Elmohlawy, A.E.; Ochkov, V.F.; Kazandzhan, B.I. Thermal performance analysis of a concentrated solar power system (CSP) integrated with natural gas combined cycle (NGCC) power plant. Case Stud. Therm. Eng. 2019, 14, 100458. [Google Scholar] [CrossRef]
- Calderón, A.; Palacios, A.; Barreneche, C.; Segarra, M.; Prieto, C.; Rodriguez-Sanchez, A.; Fernández, A.I. High temperature systems using solid particles as TES and HTF material: A review. Appl. Energy 2018, 213, 100–111. [Google Scholar] [CrossRef]
- IRENA. Concentrating Solar Power: Renewable Energytechnologies: Cost Analysis Series; Elsevier: Amsterdam, The Netherlands, 2020; Volume 1: PowerSector. [Google Scholar] [CrossRef]
- IRENA. Technology Roadmap Concentrating Solar Power; IRENA: Abu Dhabi, United Arab Emirates, 2010. [Google Scholar]
- Fernández, A.G.; Gomez-Vidal, J.; Oró, E.; Kruizenga, A.; Solé, A.; Cabeza, L.F. Mainstreaming commercial CSP systems: A technology review. Renew. Energy 2019, 140, 152–176. [Google Scholar] [CrossRef]
- Vignarooban, K.; Xu, X.; Arvay, A.; Hsu, K.; Kannan, A. Heat transfer fluids for concentrating solar power systems—A review. Appl. Energy 2015, 146, 383–396. [Google Scholar] [CrossRef]
- Benoit, H.; Spreafico, L.; Gauthier, D.; Flamant, G. Review of heat transfer fluids in tube-receivers used in concentrating solar thermal systems: Properties and heat transfer coefficients. Renew. Sustain. Energy Rev. 2016, 55, 298–315. [Google Scholar] [CrossRef]
- Sau, S.; Corsaro, N.; Crescenzi, T.; D’ottavi, C.; Liberatore, R.; Licoccia, S.; Russo, V.; Tarquini, P.; Tizzoni, A. Techno-economic comparison between CSP plants presenting two different heat transfer fluids. Appl. Energy 2016, 168, 96–109. [Google Scholar] [CrossRef]
- Xu, X.; Guo, P.; Liu, W.; Yang, W. Entropy generation and Carnot efficiency comparisons of high temperature heat transfer fluid candidates for CSP plants. Int. J. Hydrogen Energy 2017, 42, 20316–20323. [Google Scholar] [CrossRef]
- Peiró, G.; Gasia, J.; Miró, L.; Prieto, C.; Cabeza, L.F. Influence of the heat transfer fluid in a CSP plant molten salts charging process. Renew. Energy 2017, 113, 148–158. [Google Scholar] [CrossRef]
- Conroy, T.; Collins, M.N.; Fisher, J.; Grimes, R. Thermohydraulic analysis of single phase heat transfer fluids in CSP solar receivers. Renew. Energy 2018, 129, 150–167. [Google Scholar] [CrossRef]
- Polimeni, S.; Binotti, M.; Moretti, L.; Manzolini, G. Comparison of sodium and KCl-MgCl2 as heat transfer fluids in CSP solar tower with sCO2 power cycles. Sol. Energy 2018, 162, 510–524. [Google Scholar] [CrossRef]
- Wette, J.; Sutter, F.; Fernández-García, A. Evaluation of anti-soiling coatings for CSP reflectors under realistic outdoor conditions. Sol. Energy 2019, 191, 574–584. [Google Scholar] [CrossRef]
- Conceição, R.; Merrouni, A.A.; Lopes, D.; Alae, A.; Silva, H.G.; Bennouna, E.G.; Collares-Pereira, M.; Ghennioui, A. A comparative study of soiling on solar mirrors in Portugal and Morocco: Preliminary results for the dry season. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2019; Volume 2126, p. 220001. [Google Scholar]
- Merrouni, A.A.; Ghennioui, A.; Wolfertstetter, F.; Mezrhab, A. The Uncertainty of the HelioClim-3 DNI Data under Moroccan Climate; AIP Publishing: Abu Dhabi, United Arab Emirates, 2017; p. 140002. [Google Scholar] [CrossRef]
- Wilcox, S.M.; McCormack, P. Implementing Best Practices for Data Quality Assessment of the National Renewable Energy Laboratory’s Solar Resource and Meteorological Assessment Project: Preprint; p. 10. Available online: https://www.nrel.gov/docs/fy11osti/50897.pdf (accessed on 6 June 2024).
- Merrouni, A.A.; Elalaoui, F.E.; Ghennioui, A.; Mezrhab, A.; Mezrhab, A. A GIS-AHP combination for the sites assessment of large-scale CSP plants with dry and wet cooling systems. Case study: Eastern Morocco. Sol. Energy 2018, 166, 2–12. [Google Scholar] [CrossRef]
- Mouaky, A.; Merrouni, A.A.; Laadel, N.E.; Bennouna, E.G. Simulation and experimental validation of a parabolic trough plant for solar thermal applications under the semi-arid climate conditions. Sol. Energy 2019, 194, 969–985. [Google Scholar] [CrossRef]
- Qoaider, L.; Liqreina, A. Optimization of dry cooled parabolic trough (CSP) plants for the desert regions of the Middle East and North Africa (MENA). Sol. Energy 2015, 122, 976–985. [Google Scholar] [CrossRef]
- Bouhal, T.; Agrouaz, Y.; Kousksou, T.; Allouhi, A.; El Rhafiki, T.; Jamil, A.; Bakkas, M. Technical feasibility of a sustainable Concentrated Solar Power in Morocco through an energy analysis. Renew. Sustain. Energy Rev. 2018, 81, 1087–1095. [Google Scholar] [CrossRef]
- Liqreina, A.; Qoaider, L. Dry cooling of concentrating solar power (CSP) plants, an economic competitive option for the desert regions of the MENA region. Sol. Energy 2014, 103, 417–424. [Google Scholar] [CrossRef]
- Mwesigye, A.; Yılmaz, I.H.; Meyer, J.P. Numerical analysis of the thermal and thermodynamic performance of a parabolic trough solar collector using SWCNTs-Therminol®VP-1 nanofluid. Renew. Energy 2018, 119, 844–862. [Google Scholar] [CrossRef]
- Merrouni, A.A.; Ouali, H.A.L.; Moussaoui, M.A.; Mezrhab, A. Analysis and comparaison of different Heat Transfer Fluids for a 1MWe Parabolic Trough Collector. In Proceedings of the 2016 International Conference on Electrical and Information Technologies (ICEIT), Tangiers, Morocco, 4–7 May 2016; pp. 510–515. [Google Scholar]
- Coco-Enríquez, L.; Muñoz-Antón, J.; Martínez-Val, J. Dual Loop line-focusing solar power plants with supercritical Brayton power cycles. Int. J. Hydrogen Energy 2017, 42, 17664–17680. [Google Scholar] [CrossRef]
- Calvet, N.; Gomez, J.C.; Faik, A.; Roddatis, V.V.; Meffre, A.; Glatzmaier, G.C.; Doppiu, S.; Py, X. Compatibility of a post-industrial ceramic with nitrate molten salts for use as filler material in a thermocline storage system. Appl. Energy 2013, 109, 387–393. [Google Scholar] [CrossRef]
- Raade, J.W.; Padowitz, D. Development of Molten Salt Heat Transfer Fluid with Low Melting Point and High Thermal Stability. J. Sol. Energy Eng. 2011, 133, 031013. [Google Scholar] [CrossRef]
- Delise, T.; Tizzoni, A.; Ferrara, M.; Corsaro, N.; D’ottavi, C.; Sau, S.; Licoccia, S. Thermophysical, environmental, and compatibility properties of nitrate and nitrite containing molten salts for medium temperature CSP applications: A critical review. J. Eur. Ceram. Soc. 2019, 39, 92–99. [Google Scholar] [CrossRef]
- Bellmann, P.; Wolfertstetter, F.; Conceição, R.; Silva, H.G. Comparative modeling of optical soiling losses for CSP and PV energy systems. Sol. Energy 2020, 197, 229–237. [Google Scholar] [CrossRef]
- Ilse, K.; Micheli, L.; Figgis, B.W.; Lange, K.; Daßler, D.; Hanifi, H.; Wolfertstetter, F.; Naumann, V.; Hagendorf, C.; Gottschalg, R.; et al. Techno-Economic Assessment of Soiling Losses and Mitigation Strategies for Solar Power Generation. Joule 2019, 3, 2303–2321. [Google Scholar] [CrossRef]
- Conceição, R.; Silva, H.G.; Collares-Pereira, M. CSP mirror soiling characterization and modeling. Sol. Energy Mater. Sol. Cells 2018, 185, 233–239. [Google Scholar] [CrossRef]
- Merrouni, A.A.; Mezrhab, A.; Ghennioui, A.; Naimi, Z. Measurement, comparison and monitoring of solar mirror’s specular reflectivity using two different Reflectometers. Energy Procedia 2017, 119, 433–445. [Google Scholar] [CrossRef]
- Conceição, R.; González-Aguilar, J.; Merrouni, A.A.; Romero, M. Soiling effect in solar energy conversion systems: A review. Renew. Sustain. Energy Rev. 2022, 162, 112434. [Google Scholar] [CrossRef]
- Wolfertstetter, F.; Pottler, K.; Geuder, N.; Affolter, R.; Merrouni, A.; Mezrhab, A.; Pitz-Paal, R. Monitoring of Mirror and Sensor Soiling with TraCS for Improved Quality of Ground based Irradiance Measurements. Energy Procedia 2014, 49, 2422–2432. [Google Scholar] [CrossRef]
- Lopes, D.; Conceição, R.; Silva, H.G.; Aranzabe, E.; Pérez, G.; Collares-Pereira, M. Anti-soiling coating performance assessment on the reduction of soiling effect in second-surface solar mirror. Sol. Energy 2019, 194, 478–484. [Google Scholar] [CrossRef]
- Conceição, R.; Silva, H.G.; Mirão, J.; Gostein, M.; Fialho, L.; Narvarte, L.; Collares-Pereira, M. Saharan dust transport to Europe and its impact on photovoltaic performance: A case study of soiling in Portugal. Sol. Energy 2018, 160, 94–102. [Google Scholar] [CrossRef]















| Plant Characteristics | Value | Unit |
|---|---|---|
| Nominal capacity | 50 | MW |
| Storage medium | 60% NaNO3/40% KNO3 | - |
| Thermal storage capacity | 964.01 | MWhth |
| HTF | Dowtherm A | - |
| Land use | 477 | acres |
| Full load hours of storage | 7.5 | h |
| Number of collectors | 624 | - |
| Number of loops | 156 | - |
| Parameter | Value | Unit |
|---|---|---|
| Plant lifetime | 25 | (years) |
| Interest rate | 5.4 | % |
| Solar field | ||
| Specific cost | 242 | €/m2 |
| Specific O&M | 4 | €/m2 per year |
| Specific replacement cost | 0.20 | % per year |
| Specific insurance cost | 1 | % per year |
| Power block | ||
| Specific cost | 1 275 | €/kW |
| Specific O&M | 2.5 | €/MWh |
| Specific replacement cost | 0.20 | % per year |
| Specific insurance cost | 1 | % per year |
| Land cost | 1 | €/m2 |
| LCOE (€/kWh) | SOLAR MULTIPLE | ||||||||||
| 1 | 1.25 | 1.5 | 1.75 | 2 | 2.25 | 2.5 | 2.75 | 3 | |||
| Ain Beni Mathar | TES (h) | 0 h | 0.204 | 0.1846 | 0.1805 | 0.1818 | 0.1865 | 0.1935 | 0.202 | 0.2117 | 0.2219 |
| 1.5 h | 0.212 | 0.1836 | 0.1738 | 0.1718 | 0.1733 | 0.1775 | 0.1837 | 0.191 | 0.1993 | ||
| 3 h | 0.2271 | 0.1937 | 0.1777 | 0.1713 | 0.1705 | 0.1725 | 0.1762 | 0.1811 | 0.1872 | ||
| 4.5 h | 0.2424 | 0.2052 | 0.1852 | 0.1743 | 0.1703 | 0.1705 | 0.1727 | 0.1761 | 0.1805 | ||
| 6 h | 0.2577 | 0.2168 | 0.1944 | 0.18 | 0.1728 | 0.1708 | 0.1716 | 0.1739 | 0.1774 | ||
| 7.5 h | 0.2733 | 0.2284 | 0.2039 | 0.1873 | 0.1778 | 0.1741 | 0.1736 | 0.1749 | 0.1773 | ||
| 9 h | 0.289 | 0.2401 | 0.2135 | 0.1953 | 0.1846 | 0.18 | 0.1787 | 0.1792 | 0.1811 | ||
| 10.5 h | 0.3048 | 0.2519 | 0.2231 | 0.2034 | 0.1917 | 0.1864 | 0.1847 | 0.1849 | 0.1865 | ||
| N-loops | 77 | 97 | 116 | 136 | 155 | 174 | 194 | 213 | 232 | ||
| LCOE (€/kWh) | SOLAR MULTIPLE | ||||||||||
| 1 | 1.25 | 1.5 | 1.75 | 2 | 2.25 | 2.5 | 2.75 | 3 | |||
| Benguerir | TES (h) | 0 h | 0.1748 | 0.1611 | 0.1596 | 0.1631 | 0.1692 | 0.1775 | 0.1868 | 0.1966 | 0.2068 |
| 1.5 h | 0.1819 | 0.1595 | 0.1533 | 0.1537 | 0.157 | 0.1627 | 0.1699 | 0.1778 | 0.1864 | ||
| 3 h | 0.1949 | 0.1672 | 0.155 | 0.1521 | 0.1532 | 0.1564 | 0.1613 | 0.1675 | 0.1743 | ||
| 4.5 h | 0.208 | 0.177 | 0.1603 | 0.1532 | 0.1521 | 0.1535 | 0.1565 | 0.1609 | 0.1663 | ||
| 6 h | 0.2211 | 0.1869 | 0.1675 | 0.1569 | 0.153 | 0.1529 | 0.1547 | 0.1576 | 0.1616 | ||
| 7.5 h | 0.2343 | 0.1969 | 0.1756 | 0.1625 | 0.1566 | 0.1547 | 0.1554 | 0.1576 | 0.1607 | ||
| 9 h | 0.2476 | 0.207 | 0.1838 | 0.1693 | 0.1623 | 0.1596 | 0.1595 | 0.161 | 0.1636 | ||
| 10.5 h | 0.261 | 0.2171 | 0.192 | 0.1763 | 0.1685 | 0.1653 | 0.1648 | 0.166 | 0.1685 | ||
| N-loops | 75 | 94 | 113 | 132 | 150 | 169 | 188 | 207 | 226 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ammari, N.; Alami Merrouni, A.; Mendyl, A.; Chaabelasri, E.; Weidinger, T. Energy, Economic and Environmental (3E) Analysis for an Optimal CSP Technology Integration in Morocco. Energies 2024, 17, 3020. https://doi.org/10.3390/en17123020
Ammari N, Alami Merrouni A, Mendyl A, Chaabelasri E, Weidinger T. Energy, Economic and Environmental (3E) Analysis for an Optimal CSP Technology Integration in Morocco. Energies. 2024; 17(12):3020. https://doi.org/10.3390/en17123020
Chicago/Turabian StyleAmmari, Nabil, Ahmed Alami Merrouni, Abderrahmane Mendyl, Elmiloud Chaabelasri, and Tamás Weidinger. 2024. "Energy, Economic and Environmental (3E) Analysis for an Optimal CSP Technology Integration in Morocco" Energies 17, no. 12: 3020. https://doi.org/10.3390/en17123020
APA StyleAmmari, N., Alami Merrouni, A., Mendyl, A., Chaabelasri, E., & Weidinger, T. (2024). Energy, Economic and Environmental (3E) Analysis for an Optimal CSP Technology Integration in Morocco. Energies, 17(12), 3020. https://doi.org/10.3390/en17123020









