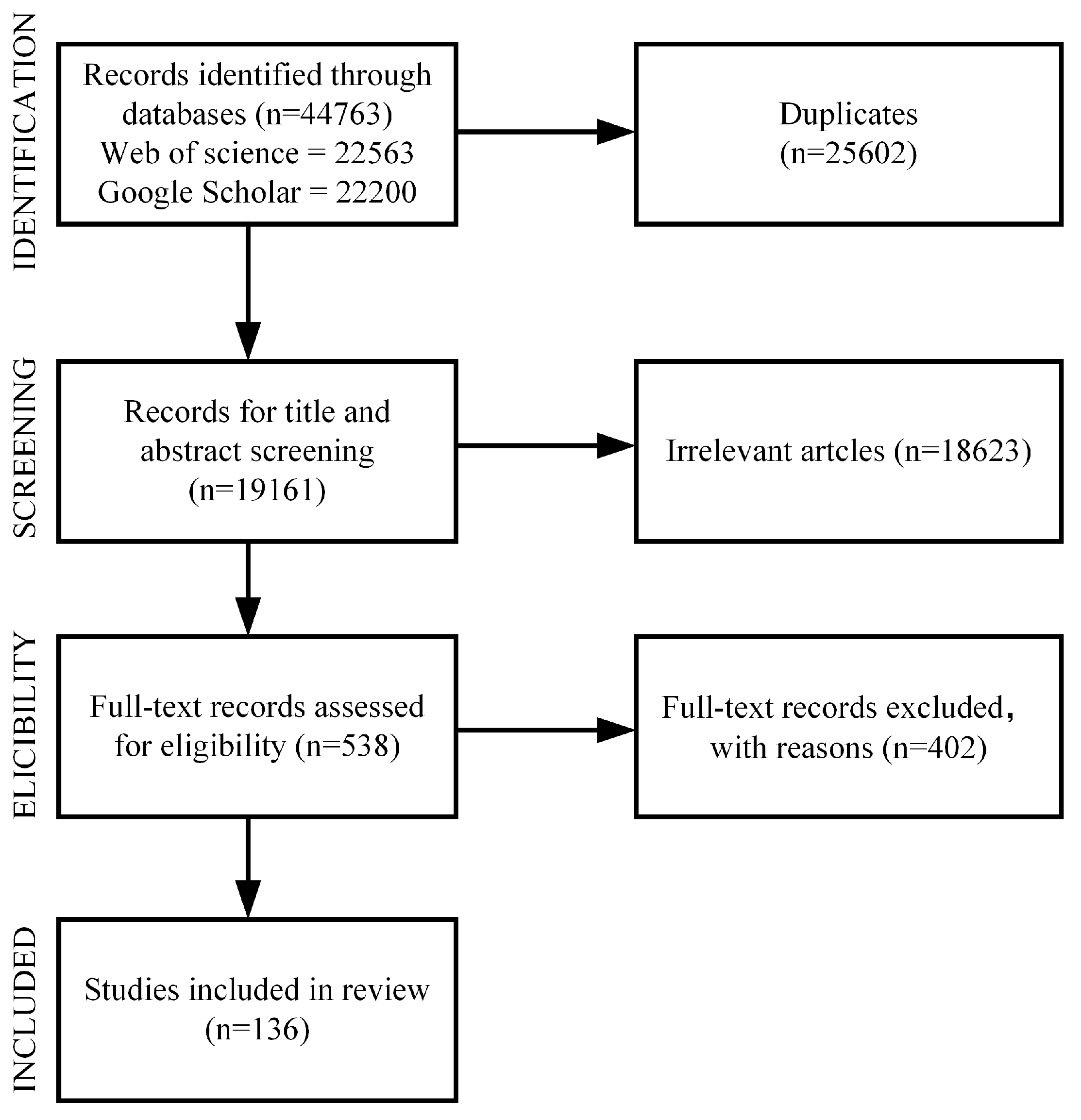
Comprehensive Review of Bearing Currents in Electrical Machines: Mechanisms, Impacts, and Mitigation Techniques
Abstract
1. Introduction
2. Bearing Current in Conventional Sine Wave Power Supply Mode
2.1. Phenomenological Principle
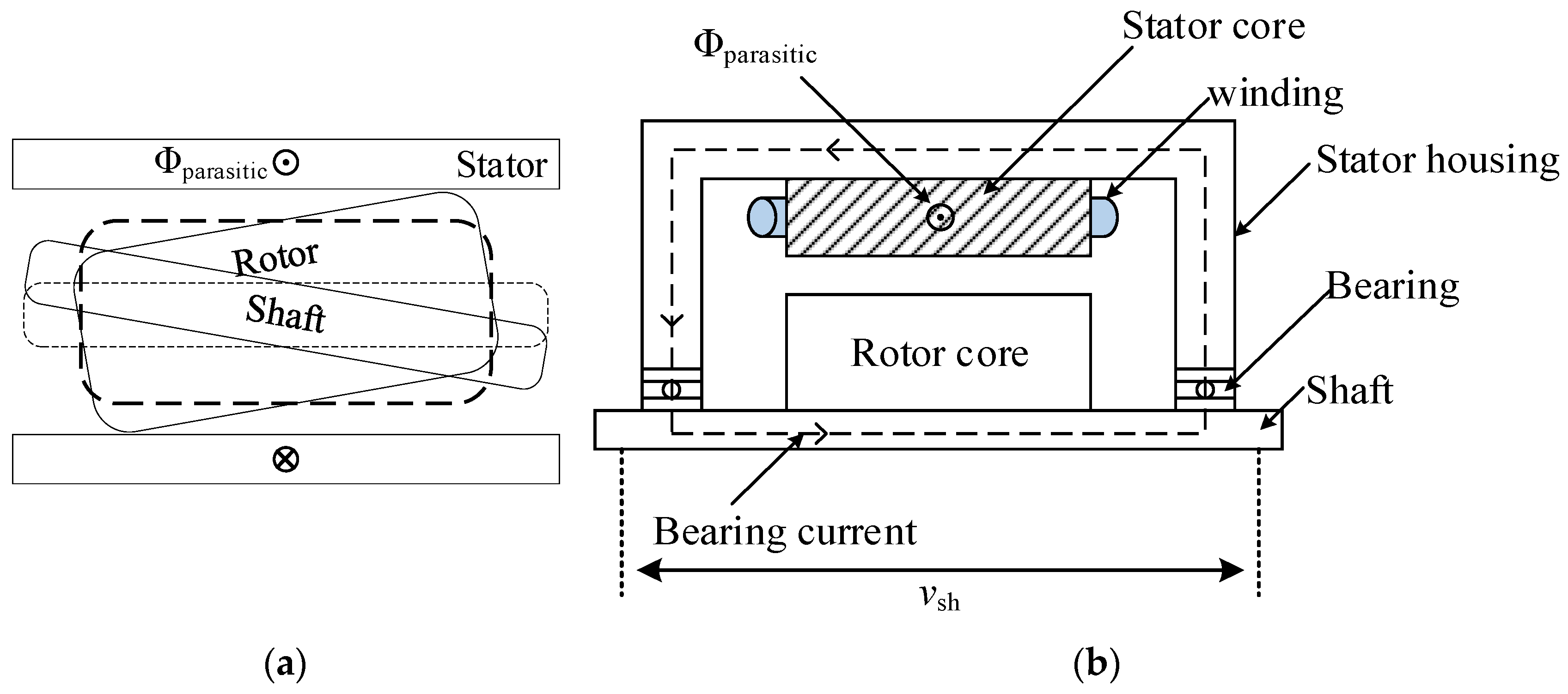
- The voltage induced at both ends of the shaft due to the tangential magnetic flux.
- 2.
- The voltage induced in the bearing due to the axial magnetic flux.
- 3.
- Shaft-to-ground voltage due to electrostatic effect.
2.2. The Harm Caused by Bearing Current and Its Solution
3. Bearing Current Phenomenon Caused by PWM Inverter
3.1. Phenomenon Principle
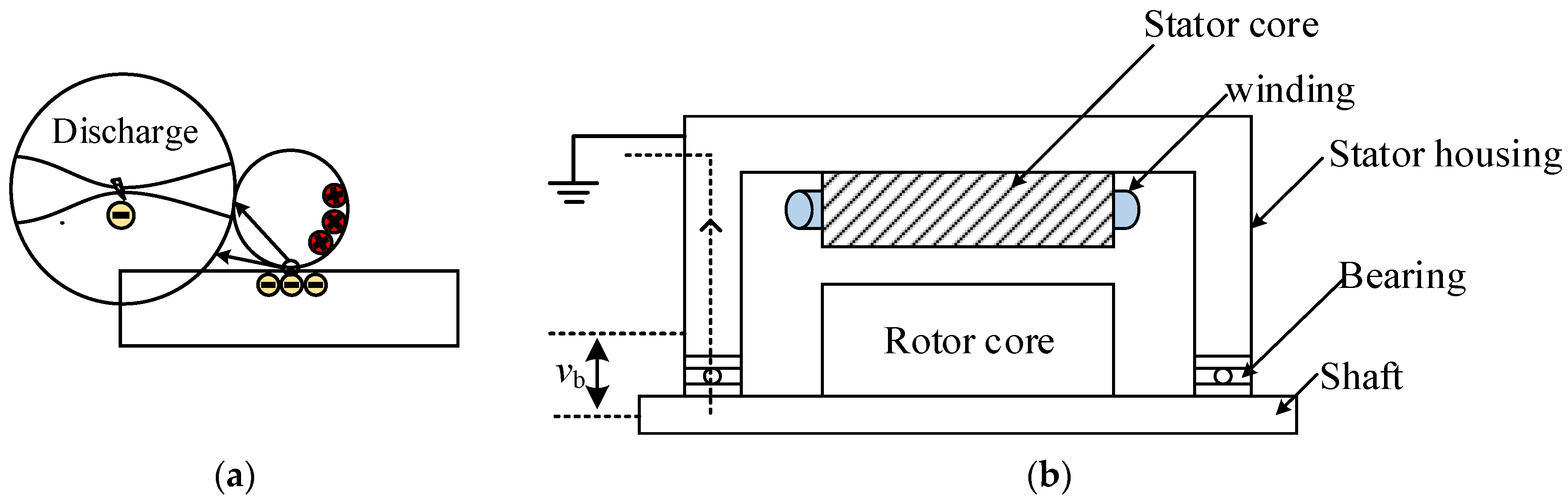
- Electrical discharge machining bearing current;
- 2.
- High-frequency circulating bearing current.
- 3.
- Rotor-to-ground bearing current.
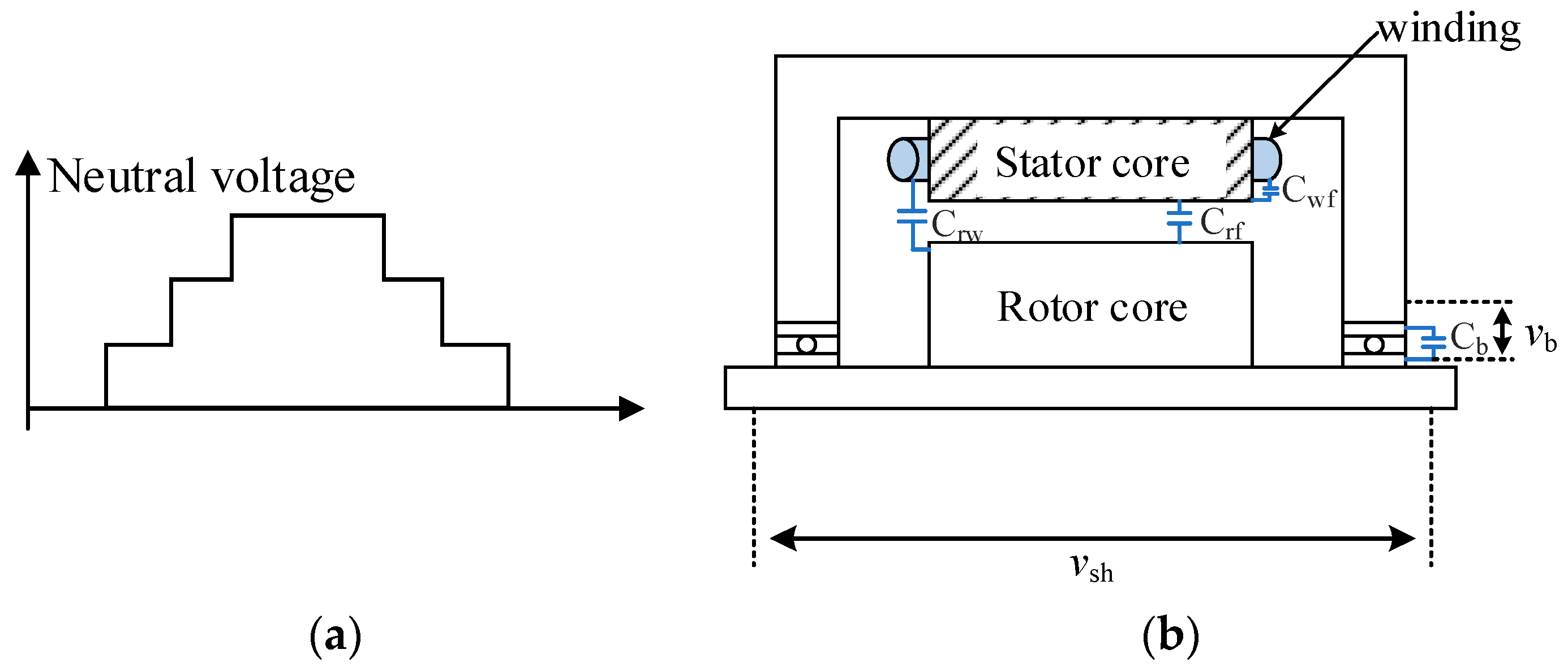
3.2. Mathematical Model
3.3. Bearing Current Solutions
3.3.1. Solution to Suppress Bearing Current from the Inverter Side
3.3.2. Solutions for Suppressing Bearing Currents from Transmission Cables
3.3.3. Solutions to Suppress Bearing Current from the Motor Side
3.4. Prediction of Bearing Currents
4. Common-Mode Voltage Suppression Scheme
4.1. Mathematical Model of Common-Mode Voltage of Three-Phase Motor
4.1.1. Common-Mode Voltage Suppression Based on SVPWM
4.1.2. Common-Mode Voltage Suppression Based on CBPWM
4.2. Multi-Phase Motor Common-Mode Voltage Prediction of Bearing Currents
5. Discussions
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hadden, T.; Jiang, J.W.; Bilgin, B.; Yang, Y.; Sathyan, A.; Dadkhah, H.; Emadi, A. A Review of Shaft Voltages and Bearing Currents in EV and HEV Motors. In Proceedings of the IECON 2016—42nd Annual Conference of the IEEE Industrial Electronics Society, Florence, Italy, 23–26 October 2016; pp. 1578–1583. [Google Scholar]
- Kudelina, K.; Vaimann, T.; Rassolkin, A.; Kallaste, A.; Asad, B.; Demidova, G. Induction Motor Bearing Currents–Causes and Damages. In Proceedings of the 2021 28th International Workshop on Electric Drives: Improving Reliability of Electric Drives (IWED), Moscow, Russia, 27 January 2021; pp. 1–5. [Google Scholar] [CrossRef]
- Liu, R.; Ma, X.; Ren, X.; Cao, J.; Niu, S. Comparative Analysis of Bearing Current in Wind Turbine Generators. Energies 2018, 11, 1305. [Google Scholar] [CrossRef]
- Whittle, M.; Trevelyan, J.; Tavner, P.J. Bearing Currents in Wind Turbine Generators. J. Renew. Sustain. Energy 2013, 5, 053128. [Google Scholar] [CrossRef]
- de Azevedo, H.D.M.; Araújo, A.M.; Bouchonneau, N. A Review of Wind Turbine Bearing Condition Monitoring: State of the Art and Challenges. Renew. Sustain. Energy Rev. 2016, 56, 368–379. [Google Scholar] [CrossRef]
- Bell, R.N.; Mcwilliams, D.W.; O’donnell, P.; Singh, C.; Wells, S.J. Report of Large Motor Reliability Survey of Industrial and Commercial Installations I. IEEE Trans. Ind. Appl. 1985, 21, 853–864. [Google Scholar]
- Bell, R.N.; Heising, C.R.; O’donnell, P.; Singh, C.; Wells, S.J. Report of Large Motor Reliability Survey of Industrial and Commercial Installations II. IEEE Trans. Ind. Appl. 1985, 21, 865–872. [Google Scholar]
- O’donnell, P.; Heising, C.; Singh, C.; Wells, S.J. Report of Large Motor Reliability Survey of Industrial and Commercial Installations III. IEEE Trans. Ind. Appl. 1987, 23, 153–158. [Google Scholar]
- Shami, U.T.; Akagi, H. Experimental Discussions on a Shaft End-to-End Voltage Appearing in an Inverter-Driven Motor. IEEE Trans. Power Electron. 2009, 24, 1532–1540. [Google Scholar] [CrossRef]
- Alger, P.L.; Samson, H.W. Shaft Currents in Electric Machines. Trans. Am. Inst. Electr. Eng. 2009, XLIII, 235–245. [Google Scholar] [CrossRef]
- IEC 60034-17; Cage Induction Motors When Fed From Converters Application Guide. International Electrotechnical Commission: Geneva, Switzerland, 2007.
- NEMA MG-1 Specification 31, Section IV; Motors and Generators. NEMA: Rosslyn, VA, USA, 2021.
- Costello, M.J. Shaft Voltages and Rotating Machinery. IEEE Trans. Ind. Appl. 1993, 29, 419–426. [Google Scholar] [CrossRef]
- Busse, D.F.; Erdman, J.M.; Kerkman, R.J.; Schlegel, D.W.; Skibinski, G.L. An Evaluation of the Electrostatic Shielded Induction Motor: A Solution for Rotor Shaft Voltage Buildup and Bearing Current. IEEE Trans. Ind. Appl. 1997, 33, 1563–1570. [Google Scholar] [CrossRef]
- Xie, G.; Luo, J.; Guo, D.; Liu, S.; Li, G. Damages on the Lubricated Surfaces in Bearings under the Influence of Weak Electrical Currents. Sci. China Technol. Sci. 2013, 56, 2979–2987. [Google Scholar] [CrossRef]
- Boyanton, H.E.; Hodges, G. Bearing Fluting [Motors]. IEEE Ind. Appl. Mag. 2002, 8, 53–57. [Google Scholar] [CrossRef]
- Nippes, P.I. Early Warning of Developing Problems in Rotating Machinery as Provided by Monitoring Shaft Voltages and Grounding Currents. IEEE Trans. Energy Convers. 2004, 19, 340–345. [Google Scholar] [CrossRef]
- Sunahara, K.; Ishida, Y.; Yamashita, S.; Yamamoto, M.; Nishikawa, H.; Matsuda, K.; Kaneta, M. Preliminary Measurements of Electrical Micropitting in Grease-Lubricated Point Contacts. Tribol. Trans. 2011, 54, 730–735. [Google Scholar] [CrossRef]
- Robak, S.; Wasilewski, J.; Dawidowski, P.; Szewczyk, M. Variable Speed Drive (VSD)–towards Modern Industry and Electric Power Systems. Rzegląd Elektrotechniczny 2016, 92, 207–210. [Google Scholar] [CrossRef][Green Version]
- McLean, G.W. Review of Recent Progress in Linear Motors. IEE Proc. B (Electr. Power Appl.) 1988, 135, 380–416. [Google Scholar] [CrossRef]
- Gieras, J.F.; Piech, Z.J.; Tomczuk, B. Linear Synchronous Motors. In Transportation and Automation Systems; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Kumar, A.B.; Narayanan, G. Variable-Switching Frequency PWM Technique for Induction Motor Drive to Spread Acoustic Noise Spectrum with Reduced Current Ripple. IEEE Trans. Ind. Appl. 2016, 52, 3927–3938. [Google Scholar] [CrossRef]
- Sahu, A.K.; Emadi, A.; Bilgin, B. Noise and Vibration in Switched Reluctance Motors: A Review on Structural Materials, Vibration Dampers, Acoustic Impedance, and Noise Masking Methods. IEEE Access 2023, 11, 27702–27718. [Google Scholar] [CrossRef]
- Abad, G.; Rodriguez, M.Á.; Poza, J. Two-Level VSC Based Predictive Direct Torque Control of the Doubly Fed Induction Machine With Reduced Torque and Flux Ripples at Low Constant Switching Frequency. IEEE Trans. Power Electron. 2008, 23, 1050–1061. [Google Scholar] [CrossRef]
- Erdman, J.M.; Kerkman, R.J.; Schlegel, D.W.; Skibinski, G.L. Effect of PWM Inverters on AC Motor Bearing Currents and Shaft Voltages. IEEE Trans. Ind. Appl. 1996, 32, 250–259. [Google Scholar] [CrossRef]
- Chen, S.; Lipo, T.A. Circulating Type Motor Bearing Current in Inverter Drives. IEEE Ind. Appl. Mag. 1998, 4, 32–38. [Google Scholar] [CrossRef]
- Busse, D.; Erdman, J.; Kerkman, R.J.; Schlegel, D.; Skibinski, G. System Electrical Parameters and Their Effects on Bearing Currents. IEEE Trans. Ind. Appl. 1997, 33, 577–584. [Google Scholar] [CrossRef]
- Busse, D.; Erdman, J.; Kerkman, R.J.; Schlegel, D.; Skibinski, G. Bearing Currents and Their Relationship to PWM Drives. IEEE Trans. Power Electron. 1997, 12, 243–252. [Google Scholar] [CrossRef]
- Guzinski, J. Common-Mode Voltage and Bearing Currents in PWM Inverters: Causes, Effects and Prevention. In Power Electronics for Renewable Energy Systems, Transportation and Industrial Applications; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2014; pp. 664–694. ISBN 978-1-118-75552-5. [Google Scholar]
- Chen, S.; Lipo, T.A.; Fitzgerald, D. Modeling of Motor Bearing Currents in PWM Inverter Drives. IEEE Trans. Ind. Appl. 1996, 32, 1365–1370. [Google Scholar] [CrossRef]
- Chen, S.; Lipo, T.A.; Fitzgerald, D. Source of Induction Motor Bearing Currents Caused by PWM Inverters. IEEE Trans. Energy Convers. 1996, 11, 25–32. [Google Scholar] [CrossRef]
- Binder, A.; Muetze, A. Scaling Effects of Inverter-Induced Bearing Currents in AC Machines. IEEE Trans. Ind. Appl. 2008, 44, 769–776. [Google Scholar] [CrossRef]
- Collin, R.; Yokochi, A.; von Jouanne, A. Novel Characterization of Si- and SiC-Based PWM Inverter Bearing Currents Using Probability Density Functions. Energies 2022, 15, 3043. [Google Scholar] [CrossRef]
- Costabile, G.; De Vivo, B.; Egiziano, L.; Tucci, V.; Vitelli, M.; Beneduce, L.; Iovieno, S.; Masucci, A. An Accurate Evaluation of Electric Discharge Machining Bearings Currents in Inverter-Driven Induction Motors. In Proceedings of the 2007 European Conference on Power Electronics and Applications, Aalborg, Denmark, 2–5 September 2007; pp. 1–8. [Google Scholar]
- Quintero, D.R.; Mejia, W.; Rosero, J.A. Good Practice for Electric Discharge Machining (EDM) Bearing Currents Measurement in the Induction Motor and Drives System. In Proceedings of the 2013 International Electric Machines & Drives Conference, Chicago, IL, USA, 12–15 May 2013; pp. 1384–1390. [Google Scholar]
- Muetze, A.; Niskanen, V.; Ahola, J. On Radio-Frequency-Based Detection of High-Frequency Circulating Bearing Current Flow. IEEE Trans. Ind. Appl. 2014, 50, 2592–2601. [Google Scholar] [CrossRef]
- Magdun, O.; Gemeinder, Y.; Binder, A. Rotor Impedance of the High Frequency Circulating Bearing Current Path in Inverter-Fed AC Machines. In Proceedings of the 2013 IEEE Energy Conversion Congress and Exposition, Denver, CO, USA, 15–19 September 2013; pp. 3512–3519. [Google Scholar]
- Jaeger, C.; Grinbaum, I.; Smajic, J. Numerical Simulation and Measurement of Common-Mode and Circulating Bearing Currents. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; pp. 486–491. [Google Scholar]
- Muetze, A.; Binder, A. Calculation of Circulating Bearing Currents in Machines of Inverter-Based Drive Systems. IEEE Trans. Ind. Electron. 2007, 54, 932–938. [Google Scholar] [CrossRef]
- Schuster, M.; Binder, A. Comparison of Different Inverter-Fed AC Motor Types Regarding Common-Mode Bearing Currents. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 2762–2768. [Google Scholar]
- Weicker, M.; Binder, A. Characteristic Parameters for Electrical Bearing Damage. In Proceedings of the 2022 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Sorrento, Italy, 22–24 June 2022; pp. 785–790. [Google Scholar]
- Safdarzadeh, O.; Weicker, M.; Binder, A. Measuring of Electrical Currents, Voltage and Resistance of an Axial Bearing. In Proceedings of the 2021 International Aegean Conference on Electrical Machines and Power Electronics (ACEMP) & 2021 International Conference on Optimization of Electrical and Electronic Equipment (OPTIM), Brasov, Romania, 2–3 September 2021; pp. 376–382. [Google Scholar]
- Zhu, W.; De Gaetano, D.; Chen, X.; Jewell, G.W.; Hu, Y. A Review of Modeling and Mitigation Techniques for Bearing Currents in Electrical Machines With Variable-Frequency Drives. IEEE Access 2022, 10, 125279–125297. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A. Calculation of Motor Capacitances for Prediction of the Voltage Across the Bearings in Machines of Inverter-Based Drive Systems. IEEE Trans. Ind. Appl. 2007, 43, 665–672. [Google Scholar] [CrossRef]
- Han, P.; Heins, G.; Patterson, D.; Thiele, M.; Ionel, D.M. Modeling of Bearing Voltage in Electric Machines Based on Electromagnetic FEA and Measured Bearing Capacitance. IEEE Trans. Ind. Appl. 2021, 57, 4765–4775. [Google Scholar] [CrossRef]
- Liu, Y.; Cao, J.; Song, Y.; Xu, G.; Li, L. Research on Bearing Current Detection Method of High-Speed Motor Driven by PWM Inverter. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–6. [Google Scholar]
- Kindl, V.; Skala, B.; Pechanek, R.; Byrtus, M.; Hruska, K. Calculation of Induction Machine Parasitic Capacitances Using Finite Element Method. In Proceedings of the 2016 ELEKTRO, Strbske Pleso, Slovakia, 16–18 May 2016; pp. 176–179. [Google Scholar]
- Jaritz, M.; Jaeger, C.; Bucher, M.; Smajic, J.; Vukovic, D.; Blume, S. An Improved Model for Circulating Bearing Currents in Inverter-Fed AC Machines. In Proceedings of the 2019 IEEE International Conference on Industrial Technology (ICIT), Melbourne, VIC, Australia, 13–15 February 2019; pp. 225–230. [Google Scholar]
- Bubert, A.; Zhang, J.; De Doncker, R.W. Modeling and Measurement of Capacitive and Inductive Bearing Current in Electrical Machines. In Proceedings of the 2017 Brazilian Power Electronics Conference (COBEP), Juiz de Fora, Brazil, 19–22 November 2017; pp. 1–6. [Google Scholar]
- Li, J.; Liu, R.; Zheng, B.; Zhang, Y. The Effects of End Part of Winding on Parasitic Capacitances of Induction Motor Fed by PWM Inverter. In Proceedings of the 2012 15th International Conference on Electrical Machines and Systems (ICEMS), Sapporo, Japan, 21–24 October 2012; pp. 1–5. [Google Scholar]
- Wu, J.; Zhuo, L.; Pei, J.; Li, Y.; Xie, H.; Wu, J.; Liu, H. Modularized and Parametric Modeling Technology for Finite Element Simulations of Underground Engineering under Complicated Geological Conditions. CMES 2024, 140, 621–645. [Google Scholar] [CrossRef]
- Kongar-Syuryun, C.; Klyuev, R.; Golik, V.; Oganesyan, A.; Solovykh, D.; Khayrutdinov, M.; Adigamov, D. Principles of Sustainable Development of Georesources as a Way to Reduce Urban Vulnerability. Urban Sci. 2024, 8, 44. [Google Scholar] [CrossRef]
- Gonda, A.; Capan, R.; Bechev, D.; Sauer, B. The Influence of Lubricant Conductivity on Bearing Currents in the Case of Rolling Bearing Greases. Lubricants 2019, 7, 108. [Google Scholar] [CrossRef]
- Jammoul, S.; Pusca, R.; Romary, R.; Lefevre, G.; Voyer, N.; Mariani, G.B. Analysis of Capacitive Discharges in Motor Bearings under Different Operation Conditions. IET Electr. Power Appl. 2024, 18, 952–961. [Google Scholar] [CrossRef]
- Magdun, O.; Binder, A. Calculation of Roller and Ball Bearing Capacitances and Prediction of EDM Currents. In Proceedings of the 2009 35th Annual Conference of IEEE Industrial Electronics, Porto, Portugal, 3–5 November 2009; pp. 1051–1056. [Google Scholar]
- Wang, Z.; Mao, S.; Tian, H.; Su, B.; Cui, Y. Simulation Analysis and Experimental Research on Electric Thermal Coupling of Current Bearing. Lubricants 2024, 12, 73. [Google Scholar] [CrossRef]
- Punga, F.; Hess, W. Bearing Currents. Elektrotechnik Maschinenbau 1907, 25, 615–618. [Google Scholar]
- Im, J.-H.; Kang, J.-K.; Lee, Y.-K.; Hur, J. Shaft Voltage Elimination Method to Reduce Bearing Faults in Dual Three-Phase Motor. IEEE Access 2022, 10, 81042–81053. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Güleç, M.; Sergeant, P. Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review. Machines 2023, 11, 550. [Google Scholar] [CrossRef]
- He, F.; Xie, G.; Luo, J. Electrical Bearing Failures in Electric Vehicles. Friction 2020, 8, 4–28. [Google Scholar] [CrossRef]
- Ma, J.; Xue, Y.; Han, Q.; Li, X.; Yu, C. Motor Bearing Damage Induced by Bearing Current: A Review. Machines 2022, 10, 1167. [Google Scholar] [CrossRef]
- Julian, A.L.; Oriti, G.; Lipo, T.A. Elimination of Common-Mode Voltage in Three-Phase Sinusoidal Power Converters. IEEE Trans. Power Electron. 1999, 14, 982–989. [Google Scholar] [CrossRef]
- von Jauanne, A.; Zhang, H. A Dual-Bridge Inverter Approach to Eliminating Common-Mode Voltages and Bearing and Leakage Currents. IEEE Trans. Power Electron. 1999, 14, 43–48. [Google Scholar] [CrossRef]
- Chandrashekar, S.M.; Ramachandran, A.; Reddy, M.C. Simulation and Experimental Measurement of Shaft Voltage, Bearing Current in Induction Motor Drive. In Proceedings of the 2017 IEEE International Conference on Power, Control, Signals and Instrumentation Engineering (ICPCSI), Chennai, India, 21–22 September 2017; pp. 732–737. [Google Scholar]
- Bharatiraja, C.; Selvaraj, R.; Chelliah, T.R.; Munda, J.L.; Tariq, M.; Maswood, A.I. Design and Implementation of Fourth Arm for Elimination of Bearing Current in NPC-MLI-Fed Induction Motor Drive. IEEE Trans. Ind. Appl. 2018, 54, 745–754. [Google Scholar] [CrossRef]
- Turzynski, M.; Chrzan, P.J. Reducing Common-Mode Voltage and Bearing Currents in Quasi-Resonant DC-Link Inverter. IEEE Trans. Power Electron. 2020, 35, 9553–9562. [Google Scholar] [CrossRef]
- Akagi, H.; Tamura, S. A Passive EMI Filter for Eliminating Both Bearing Current and Ground Leakage Current From an Inverter-Driven Motor. IEEE Trans. Power Electron. 2006, 21, 1459–1469. [Google Scholar] [CrossRef]
- Jiang, Y.; Wu, W.; He, Y.; Chung, H.S.-H.; Blaabjerg, F. New Passive Filter Design Method for Overvoltage Suppression and Bearing Currents Mitigation in a Long Cable Based PWM Inverter-Fed Motor Drive System. IEEE Trans. Power Electron. 2017, 32, 7882–7893. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A. Experimental Evaluation of Mitigation Techniques for Bearing Currents in Inverter-Supplied Drive-Systems–Investigations on Induction Motors up to 500 kW. In Proceedings of the IEEE International Electric Machines and Drives Conference, 2003. IEMDC’03, Madison, WI, USA, 1–4 June 2003; Volume 3, pp. 1859–1865. [Google Scholar]
- Anagha, E.R.; Nisha, P.V.; Sindhu, T.K. Design of an Active EMI Filter for Bearing Current Elimination in VFD. In Proceedings of the 2018 IEEE International Symposium on Electromagnetic Compatibility and 2018 IEEE Asia-Pacific Symposium on Electromagnetic Compatibility (EMC/APEMC), Singapore, 14–18 May 2018; pp. 131–134. [Google Scholar]
- Mechlinski, M.; Schröder, S.; Shen, J.; De Doncker, R.W. Grounding Concept and Common-Mode Filter Design Methodology for Transformerless MV Drives to Prevent Bearing Current Issues. IEEE Trans. Ind. Appl. 2017, 53, 5393–5404. [Google Scholar] [CrossRef]
- Bartolucci, E.J.; Finke, B.H. Cable Design for PWM Variable-Speed AC Drives. IEEE Trans. Ind. Appl. 2001, 37, 415–422. [Google Scholar] [CrossRef]
- Vostrov, K.; Petrov, I.; Abramenko, V.; Pyrhönen, J. Grounded Electrodes Approach to Reduce Bearing Currents: Robustness Analysis for Open-Slots Machines. In Proceedings of the 2024 IEEE 33rd International Symposium on Industrial Electronics (ISIE), Ulsan, Republic of Korea, 18–21 June 2024; pp. 1–7. [Google Scholar]
- Vostrov, K.; Pyrhönen, J.; Niemelä, M.; Lindh, P.; Ahola, J. On the Application of Extended Grounded Slot Electrodes to Reduce Noncirculating Bearing Currents. IEEE Trans. Ind. Electron. 2023, 70, 2286–2295. [Google Scholar] [CrossRef]
- De Gaetano, D.; Zhu, W.; Sun, X.; Chen, X.; Griffo, A.; Jewell, G.W. Novel Stator Slot Opening to Reduce Electrical Machine Bearing Currents. IEEE Trans. Magn. 2023, 59, 1–5. [Google Scholar] [CrossRef]
- Vostrov, K.; Pyrhönen, J.; Niemelä, M.; Ahola, J.; Lindh, P. Mitigating Noncirculating Bearing Currents by a Correct Stator Magnetic Circuit and Winding Design. IEEE Trans. Ind. Electron. 2021, 68, 3805–3812. [Google Scholar] [CrossRef]
- Scheuermann, S.; Brodatzki, M.; Doppelbauer, M. Investigation of Electrostatic Shieldings in Traction Motors to Mitigate Capacitive Bearing Currents. In Proceedings of the 2024 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Napoli, Italy, 19–21 June 2024; pp. 1087–1092. [Google Scholar]
- Vostrov, K.; Pyrhönen, J.; Lindh, P.; Niemelä, M.; Ahola, J. Mitigation of Inverter-Induced Noncirculating Bearing Currents by Introducing Grounded Electrodes into Stator Slot Openings. IEEE Trans. Ind. Electron. 2021, 68, 11752–11760. [Google Scholar] [CrossRef]
- Yang, M.; Cheng, Y.; Du, B.; Li, Y.; Wang, S.; Cui, S. Research on Analysis and Suppression Methods of the Bearing Current for Electric Vehicle Motor Driven by SiC Inverter. Energies 2024, 17, 1109. [Google Scholar] [CrossRef]
- Chen, S.; Lipo, T.A. Bearing Currents and Shaft Voltages of an Induction Motor under Hard- and Soft-Switching Inverter Excitation. IEEE Trans. Ind. Appl. 1998, 34, 1042–1048. [Google Scholar] [CrossRef]
- Ludois, D.C.; Reed, J.K. Brushless Mitigation of Bearing Currents in Electric Machines Via Capacitively Coupled Shunting. IEEE Trans. Ind. Appl. 2015, 51, 3783–3790. [Google Scholar] [CrossRef]
- Vostrov, K. Reduction of Non-Circulating Bearing Currents by Electrical Machine Design. Ph.D. Thesis, Lappeenranta-Lahti University of Technology LUT, Lappeenranta, Finland, 2023. ISBN 978-952-335-961-1. [Google Scholar]
- Sanchez, L.J.; Hager, C.H., Jr.; Evans, R.D. Rolling Element Bearing Damage in the Presence of Applied Electric Current. Tribol. Trans. 2024, 67, 602–613. [Google Scholar] [CrossRef]
- Aqil, M.; Im, J.-H.; Hur, J. Application of Perovskite Layer to Rotor for Enhanced Stator-Rotor Capacitance for PMSM Shaft Voltage Reduction. Energies 2020, 13, 5762. [Google Scholar] [CrossRef]
- Lee, S.J.; Choi, J.; Lim, G.C.; Kim, S.M.; Kim, E.-K.; Ha, J.-I. Analysis and Mitigation of Bearing Current in Inverter-Fed IPMSMs Drive System. IEEE Trans. Ind. Electron. 2024, 1–11. [Google Scholar] [CrossRef]
- Kalaiselvi, J.; Srinivas, S. Bearing Currents and Shaft Voltage Reduction in Dual-Inverter-Fed Open-End Winding Induction Motor With Reduced CMV PWM Methods. IEEE Trans. Ind. Electron. 2015, 62, 144–152. [Google Scholar] [CrossRef]
- Cheng, Y.; Yang, M.; Peng, H.; Du, B.; Zhao, T.; Cui, S. Research on Suppression Method of Bearing Current for Electric Vehicle Motor Driven by SiC Inverter. In Proceedings of the IECON 2023—49th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 16–19 October 2023; pp. 1–6. [Google Scholar]
- Magdun, O.; Gemeinder, Y.; Binder, A. Investigation of Influence of Bearing Load and Bearing Temperature on EDM Bearing Currents. In Proceedings of the 2010 IEEE Energy Conversion Congress and Exposition, Atlanta, GA, USA, 12–16 September 2010; pp. 2733–2738. [Google Scholar]
- Muetze, A.; Tamminen, J.; Ahola, J. Influence of Motor Operating Parameters on Discharge Bearing Current Activity. IEEE Trans. Ind. Appl. 2011, 47, 1767–1777. [Google Scholar] [CrossRef]
- Tischmacher, H.; Gattermann, S. Bearing Currents in Converter Operation. In Proceedings of the The XIX International Conference on Electrical Machines–ICEM 2010, Rome, Italy, 6–8 September 2010; pp. 1–8. [Google Scholar]
- Immovilli, F.; Bellini, A.; Rubini, R.; Tassoni, C. Diagnosis of Bearing Faults in Induction Machines by Vibration or Current Signals: A Critical Comparison. IEEE Trans. Ind. Appl. 2010, 46, 1350–1359. [Google Scholar] [CrossRef]
- Prudhom, A.; Antonino-Daviu, J.; Razik, H.; Climente-Alarcon, V. Time-Frequency Vibration Analysis for the Detection of Motor Damages Caused by Bearing Currents. Mech. Syst. Signal Process. 2017, 84, 747–762. [Google Scholar] [CrossRef]
- Schoen, R.R.; Habetler, T.G.; Kamran, F.; Bartfield, R.G. Motor Bearing Damage Detection Using Stator Current Monitoring. IEEE Trans. Ind. Appl. 1995, 31, 1274–1279. [Google Scholar] [CrossRef]
- Singleton, R.K.; Strangas, E.G.; Aviyente, S. The Use of Bearing Currents and Vibrations in Lifetime Estimation of Bearings. IEEE Trans. Ind. Inf. 2017, 13, 1301–1309. [Google Scholar] [CrossRef]
- Afshar, M.; Rajabioun, R.; Akin, B. Current-Driven Deep Learning for Enhanced Motor Bearing Prognostics. IEEE Trans. Ind. Appl. 2024, 1–10. [Google Scholar] [CrossRef]
- Guan, B.; Bao, X.; Qiu, H.; Yang, D. Enhancing Bearing Fault Diagnosis Using Motor Current Signals: A Novel Approach Combining Time Shifting and CausalConvNets. Measurement 2024, 226, 114049. [Google Scholar] [CrossRef]
- de Araújo, V.G.; Bissiriou, A.O.-S.; Villanueva, J.M.M.; Villarreal, E.R.L.; Salazar, A.O.; de Teixeira, R.A.; Fonsêca, D.A.d.M. Monitoring and Diagnosing Faults in Induction Motors’ Three-Phase Systems Using NARX Neural Network. Energies 2024, 17, 4609. [Google Scholar] [CrossRef]
- Senanayaka, J.S.L.; Kandukuri, S.T.; Khang, H.V.; Robbersmyr, K.G. Early Detection and Classification of Bearing Faults Using Support Vector Machine Algorithm. In Proceedings of the 2017 IEEE Workshop on Electrical Machines Design, Control and Diagnosis (WEMDCD), Nottingham, UK, 20–21 April 2017; pp. 250–255. [Google Scholar]
- Roy, S.S.; Dey, S.; Chatterjee, S. Autocorrelation Aided Random Forest Classifier-Based Bearing Fault Detection Framework. IEEE Sens. J. 2020, 20, 10792–10800. [Google Scholar] [CrossRef]
- Ozcan, I.H.; Devecioglu, O.C.; Ince, T.; Eren, L.; Askar, M. Enhanced Bearing Fault Detection Using Multichannel, Multilevel 1D CNN Classifier. Electr. Eng. 2022, 104, 435–447. [Google Scholar] [CrossRef]
- Liu, Z.; Cheng, X.; Peng, X.; Wang, P.; Zhao, X.; Liu, J.; Jiang, D.; Qu, R. A Review of Common-Mode Voltage Suppression Methods in Wind Power Generation. Renew. Sustain. Energy Rev. 2024, 203, 114773. [Google Scholar] [CrossRef]
- Wu, M.; Xue, C.; Li, Y.W.; Yang, K. A Generalized Selective Harmonic Elimination PWM Formulation With Common-Mode Voltage Reduction Ability for Multilevel Converters. IEEE Trans. Power Electron. 2021, 36, 10753–10765. [Google Scholar] [CrossRef]
- Patel, H.S.; Hoft, R.G. Generalized Techniques of Harmonic Elimination and Voltage Control in Thyristor Inverters: Part I–Harmonic Elimination. IEEE Trans. Ind. Appl. 1973, IA-9, 310–317. [Google Scholar] [CrossRef]
- Yun, S.-W.; Baik, J.-H.; Kim, D.-S.; Yoo, J.-Y. A New Active Zero State PWM Algorithm for Reducing the Number of Switchings. J. Power Electron. 2017, 17, 88–95. [Google Scholar] [CrossRef]
- Baik, J.-H.; Yun, S.-W.; Kim, D.-S.; Kwon, C.-K.; Yoo, J.-Y. EMI Noise Reduction with New Active Zero State PWM for Integrated Dynamic Brake Systems. J. Power Electron. 2018, 18, 923–930. [Google Scholar] [CrossRef]
- Chen, H.; Zhao, H. Review on Pulse-Width Modulation Strategies for Common-Mode Voltage Reduction in Three-Phase Voltage-Source Inverters. IET Power Electron. 2016, 9, 2611–2620. [Google Scholar] [CrossRef]
- Zhang, M.; Atkinson, D.J.; Ji, B.; Armstrong, M.; Ma, M. A Near-State Three-Dimensional Space Vector Modulation for a Three-Phase Four-Leg Voltage Source Inverter. IEEE Trans. Power Electron. 2014, 29, 5715–5726. [Google Scholar] [CrossRef]
- Un, E.; Hava, A.M. A Near-State PWM Method With Reduced Switching Losses and Reduced Common-Mode Voltage for Three-Phase Voltage Source Inverters. IEEE Trans. Ind. Appl. 2009, 45, 782–793. [Google Scholar] [CrossRef]
- Hava, A.M.; Ün, E. Performance Analysis of Reduced Common-Mode Voltage PWM Methods and Comparison With Standard PWM Methods for Three-Phase Voltage-Source Inverters. IEEE Trans. Power Electron. 2009, 24, 241–252. [Google Scholar] [CrossRef]
- Cacciato, M.; Consoli, A.; Scarcella, G.; Testa, A. Reduction of Common-Mode Currents in PWM Inverter Motor Drives. IEEE Trans. Ind. Appl. 1999, 35, 469–476. [Google Scholar] [CrossRef]
- Baik, J.; Yun, S.; Kim, D.; Kwon, C.; Yoo, J. Remote-State PWM with Minimum RMS Torque Ripple and Reduced Common-Mode Voltage for Three-Phase VSI-Fed BLAC Motor Drives. Electronics 2020, 9, 586. [Google Scholar] [CrossRef]
- Guo, L.; Jin, N.; Gan, C.; Luo, K. Hybrid Voltage Vector Preselection-Based Model Predictive Control for Two-Level Voltage Source Inverters to Reduce the Common-Mode Voltage. IEEE Trans. Ind. Electron. 2020, 67, 4680–4691. [Google Scholar] [CrossRef]
- Samanes, J.; Gubia, E.; Juankorena, X.; Girones, C. Common-Mode and Phase-to-Ground Voltage Reduction in Back-to-Back Power Converters With Discontinuous PWM. IEEE Trans. Ind. Electron. 2020, 67, 7499–7508. [Google Scholar] [CrossRef]
- Lee, H.-D.; Sul, S.-K. Common Mode Voltage Reduction Method Modifying the Distribution of Zero Voltage Vector in PWM Converter/Inverter System. In Proceedings of the Conference Record of the 1999 IEEE Industry Applications Conference, Thirty-Forth IAS Annual Meeting (Cat. No.99CH36370), Phoenix, AZ, USA, 3–7 October 1999; Volume 3, pp. 1596–1601. [Google Scholar]
- Shen, Z.; Jiang, D.; Zou, J.; Liu, Z.; Ma, C. Current-Balance Mode-Based Unified Common-Mode Voltage Elimination Scheme for Dual Three-Phase Motor Drive System. IEEE Trans. Ind. Electron. 2022, 69, 12575–12586. [Google Scholar] [CrossRef]
- Kimball, J.W.; Zawodniok, M. Reducing Common-Mode Voltage in Three-Phase Sine-Triangle PWM With Interleaved Carriers. IEEE Trans. Power Electron. 2011, 26, 2229–2236. [Google Scholar] [CrossRef]
- Jiang, D.; Shen, Z.; Wang, F. Common-Mode Voltage Reduction for Paralleled Inverters. IEEE Trans. Power Electron. 2018, 33, 3961–3974. [Google Scholar] [CrossRef]
- Xiong, W.; Sun, Y.; Su, M.; Zhang, J.; Liu, Y.; Yang, J. Carrier-Based Modulation Strategies With Reduced Common-Mode Voltage for Five-Phase Voltage Source Inverters. IEEE Trans. Power Electron. 2018, 33, 2381–2394. [Google Scholar] [CrossRef]
- Nguyen, N.-V.; Doi, M.-V. Carrier Based PWM Control of 3-Level NPC Inverter with DC Neutral Point Balancing and Common Mode Voltage Reduction. In Proceedings of the 2019 10th International Conference on Power Electronics and ECCE Asia (ICPE 2019–ECCE Asia), Busan, Republic of Korea, 27–30 May 2019; pp. 1213–1220. [Google Scholar]
- Xu, X.; Zheng, Z.; Wang, K.; Yang, B.; Li, Y. A Comprehensive Study of Common Mode Voltage Reduction and Neutral Point Potential Balance for a Back-to-Back Three-Level NPC Converter. IEEE Trans. Power Electron. 2020, 35, 7910–7920. [Google Scholar] [CrossRef]
- Liu, P.; Duan, S.; Yao, C.; Chen, C. A Double Modulation Wave CBPWM Strategy Providing Neutral-Point Voltage Oscillation Elimination and CMV Reduction for Three-Level NPC Inverters. IEEE Trans. Ind. Electron. 2018, 65, 16–26. [Google Scholar] [CrossRef]
- Turzyński, M.; Musznicki, P. A Review of Reduction Methods of Impact of Common-Mode Voltage on Electric Drives. Energies 2021, 14, 4003. [Google Scholar] [CrossRef]
- Kwak, S.; Mun, S. Model Predictive Control Methods to Reduce Common-Mode Voltage for Three-Phase Voltage Source Inverters. IEEE Trans. Power Electron. 2015, 30, 5019–5035. [Google Scholar] [CrossRef]
- Gao, H.; Guo, J.; Hou, Z.; Zhang, B.; Dong, Y. Fault Diagnosis Method of Six-Phase Permanent Magnet Synchronous Motor Based on Vector Space Decoupling. Electronics 2022, 11, 1229. [Google Scholar] [CrossRef]
- Zhao, Y.; Lipo, T.A. Space Vector PWM Control of Dual Three-Phase Induction Machine Using Vector Space Decomposition. IEEE Trans. Ind. Appl. 1995, 31, 1100–1109. [Google Scholar] [CrossRef]
- Wang, Z.; Wang, Y.; Chen, J.; Hu, Y. Decoupled Vector Space Decomposition Based Space Vector Modulation for Dual Three-Phase Three-Level Motor Drives. IEEE Trans. Power Electron. 2018, 33, 10683–10697. [Google Scholar] [CrossRef]
- Marouani, K.; Baghli, L.; Hadiouche, D.; Kheloui, A.; Rezzoug, A. A New PWM Strategy Based on a 24-Sector Vector Space Decomposition for a Six-Phase VSI-Fed Dual Stator Induction Motor. IEEE Trans. Ind. Electron. 2008, 55, 1910–1920. [Google Scholar] [CrossRef]
- Grandi, G.; Serra, G.; Tani, A. Space Vector Modulation of a Six-Phase VSI Based on Three-Phase Decomposition. In Proceedings of the Automation and Motion 2008 International Symposium on Power Electronics, Electrical Drives, Ischia, Italy, 11–13 June 2008; pp. 674–679. [Google Scholar]
- Abu-Seif, M.A.; Ahmed, M.; Metwly, M.Y.; Abdel-Khalik, A.S.; Hamad, M.S.; Ahmed, S.; Elmalhy, N. Data-Driven-Based Vector Space Decomposition Modeling of Multiphase Induction Machines. IEEE Trans. Energy Convers. 2023, 38, 2061–2074. [Google Scholar] [CrossRef]
- Alcaide, A.M.; Wang, X.; Yan, H.; Leon, J.I.; Monopoli, V.G.; Buticchi, G.; Vazquez, S.; Liserre, M.; Franquelo, L.G. Common-Mode Voltage Mitigation of Dual Three-Phase Voltage Source Inverters in a Motor Drive Application. IEEE Access 2021, 9, 67477–67487. [Google Scholar] [CrossRef]
- Mansuri, A.; Maurya, R.; Suhel, S.M.; Iqbal, A. Novel SV-PWM Strategies for Six-Phase Induction Motor Drive With Reduced Switching Losses and Common-Mode Voltage. IEEE Trans. Power Electron. 2024, 39, 14–24. [Google Scholar] [CrossRef]
- Zhao, L.; Huang, S.; Gao, Y.; Zheng, J. A Common-Mode Voltage Suppression Strategy Based on Double Zero-Sequence Injection PWM for Two-Level Six-Phase VSIs. Energies 2022, 15, 6242. [Google Scholar] [CrossRef]
- Liu, Z.; Zheng, Z.; Sudhoff, S.D.; Gu, C.; Li, Y. Reduction of Common-Mode Voltage in Multiphase Two-Level Inverters Using SPWM With Phase-Shifted Carriers. IEEE Trans. Power Electron. 2016, 31, 6631–6645. [Google Scholar] [CrossRef]
- Liu, Z.; Zheng, Z.; Peng, Z.; Li, Y.; Hao, L. A Sawtooth Carrier-Based PWM for Asymmetrical Six-Phase Inverters With Improved Common-Mode Voltage Performance. IEEE Trans. Power Electron. 2018, 33, 9444–9458. [Google Scholar] [CrossRef]
- Fangke, L.; Haifeng, L.; Yongdong, L.; Jianyun, C. A Modulation Method to Eliminate Common-Mode Voltage of Dual Three-Phase Motor. In Proceedings of the 2019 IEEE Transportation Electrification Conference and Expo (ITEC), Detroit, MI, USA, 19–21 June 2019; pp. 1–5. [Google Scholar]
- Shen, Z.; Jiang, D.; Liu, Z.; Ye, D.; Li, J. Common-Mode Voltage Elimination for Dual Two-Level Inverter-Fed Asymmetrical Six-Phase PMSM. IEEE Trans. Power Electron. 2020, 35, 3828–3840. [Google Scholar] [CrossRef]

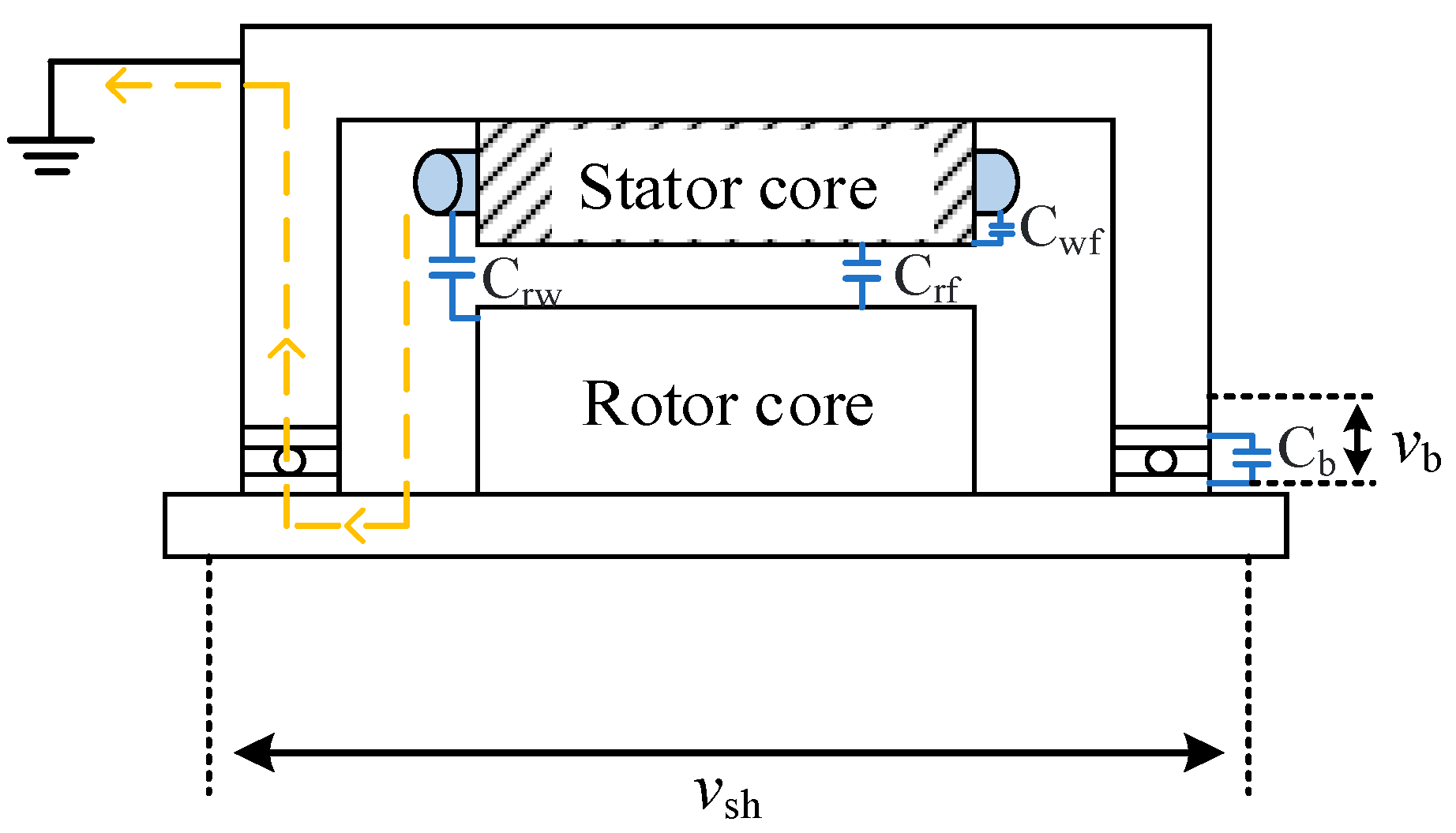
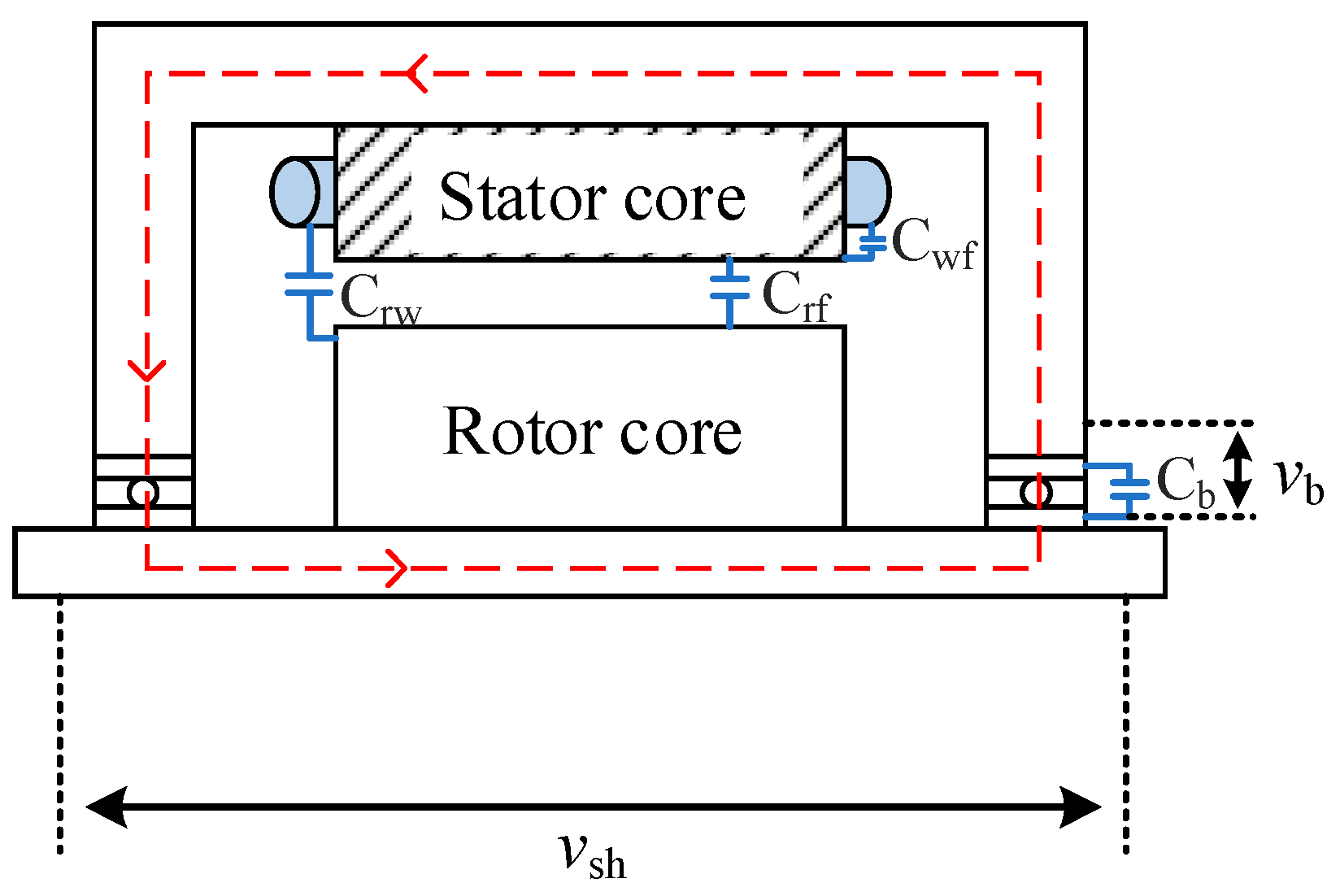
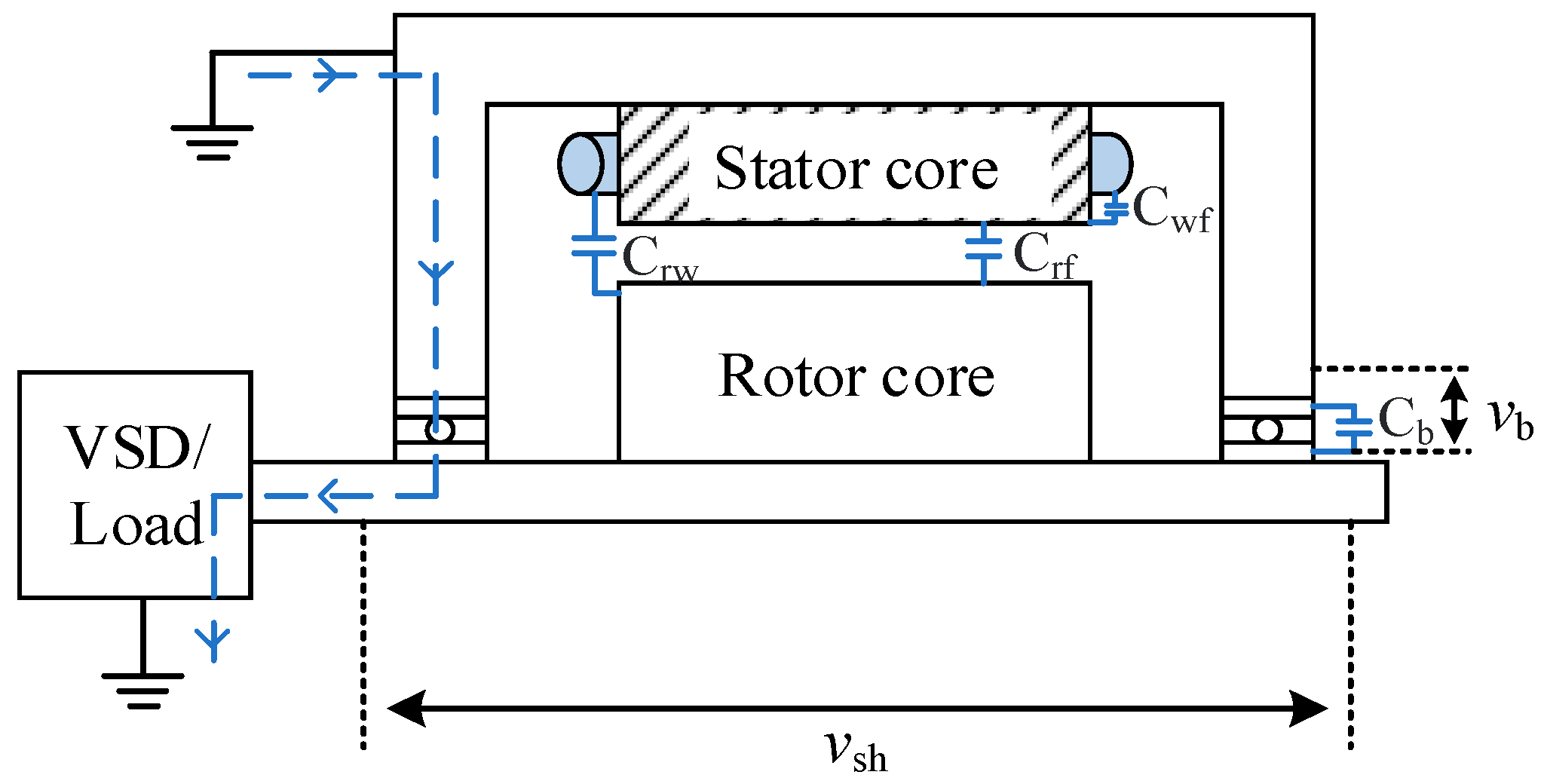

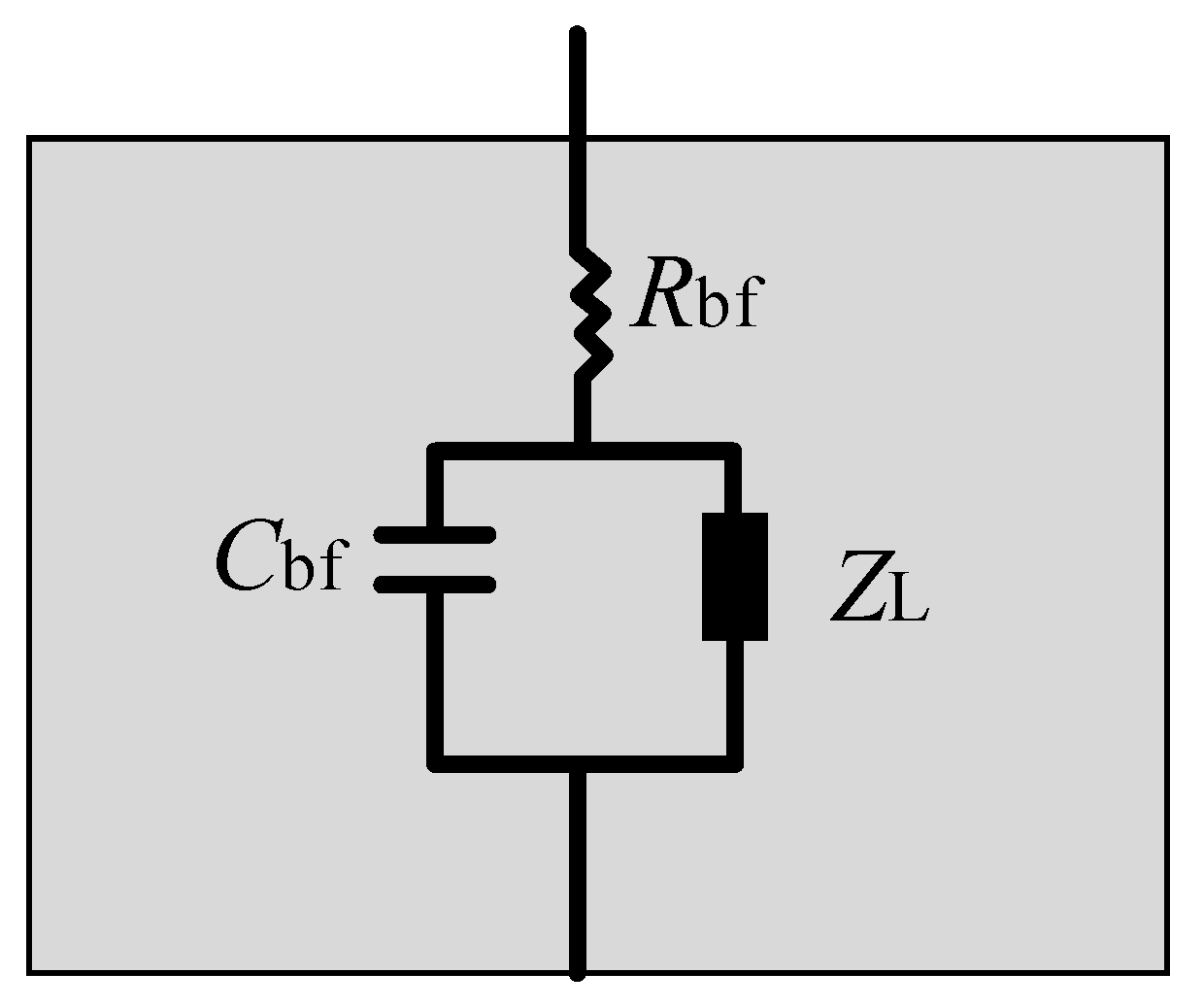
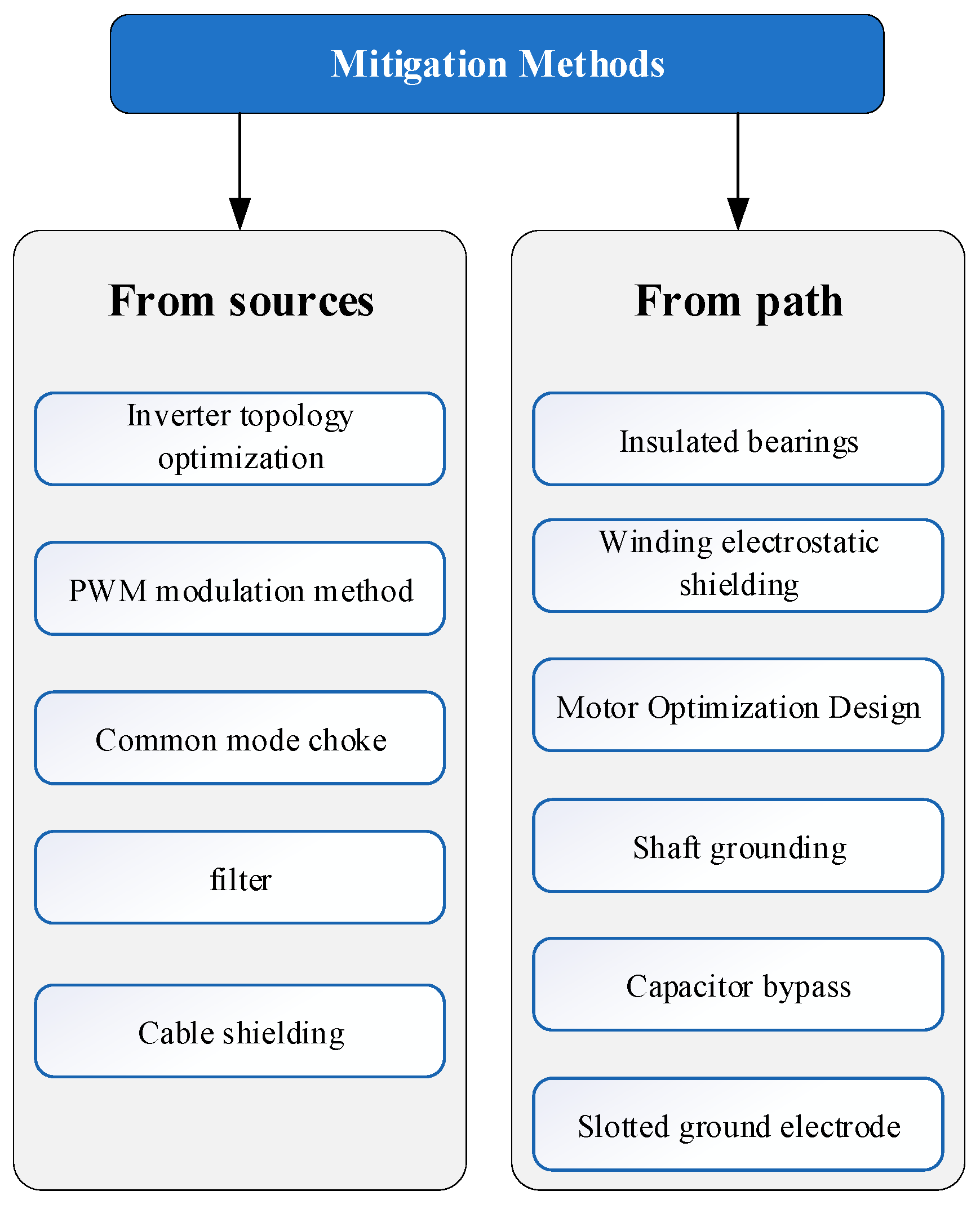

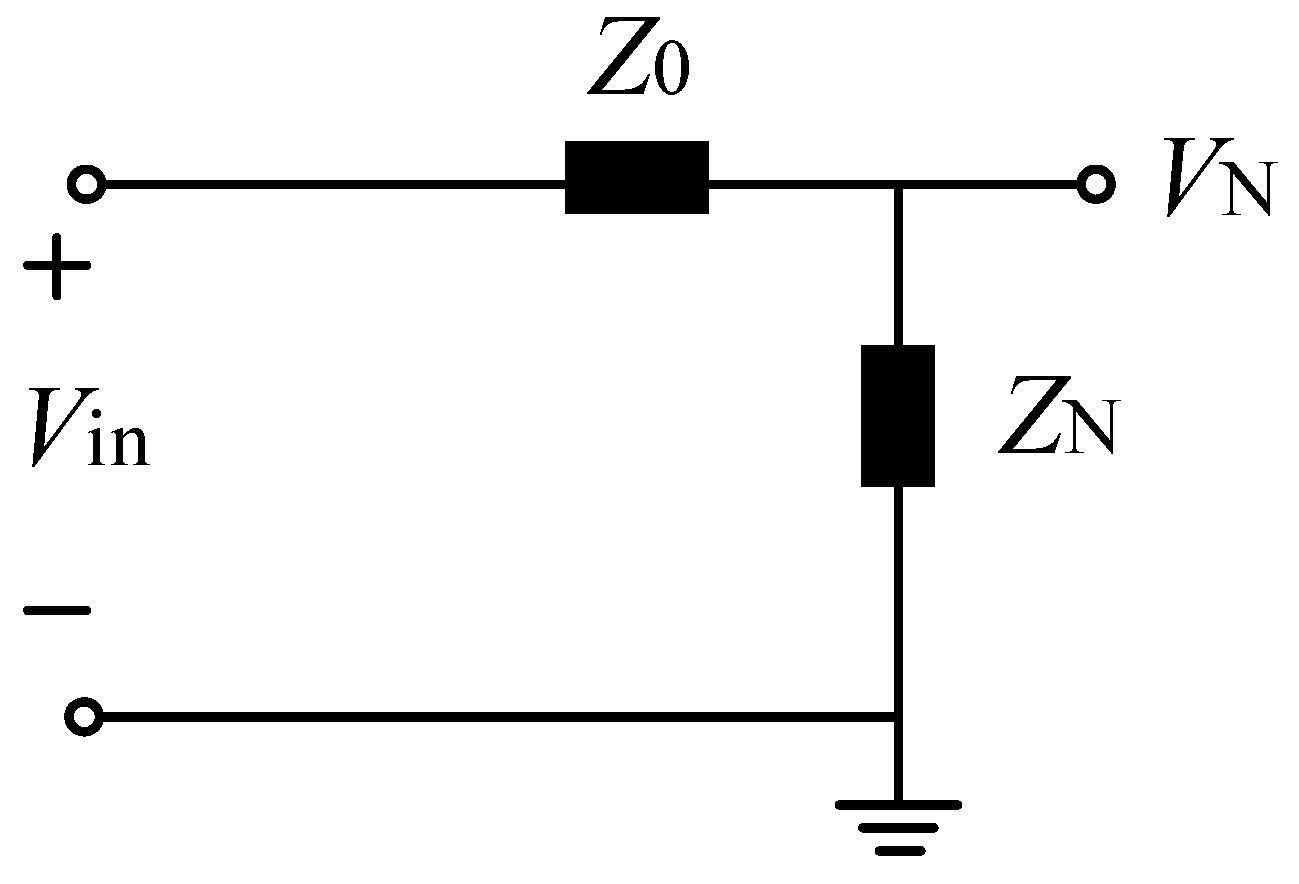
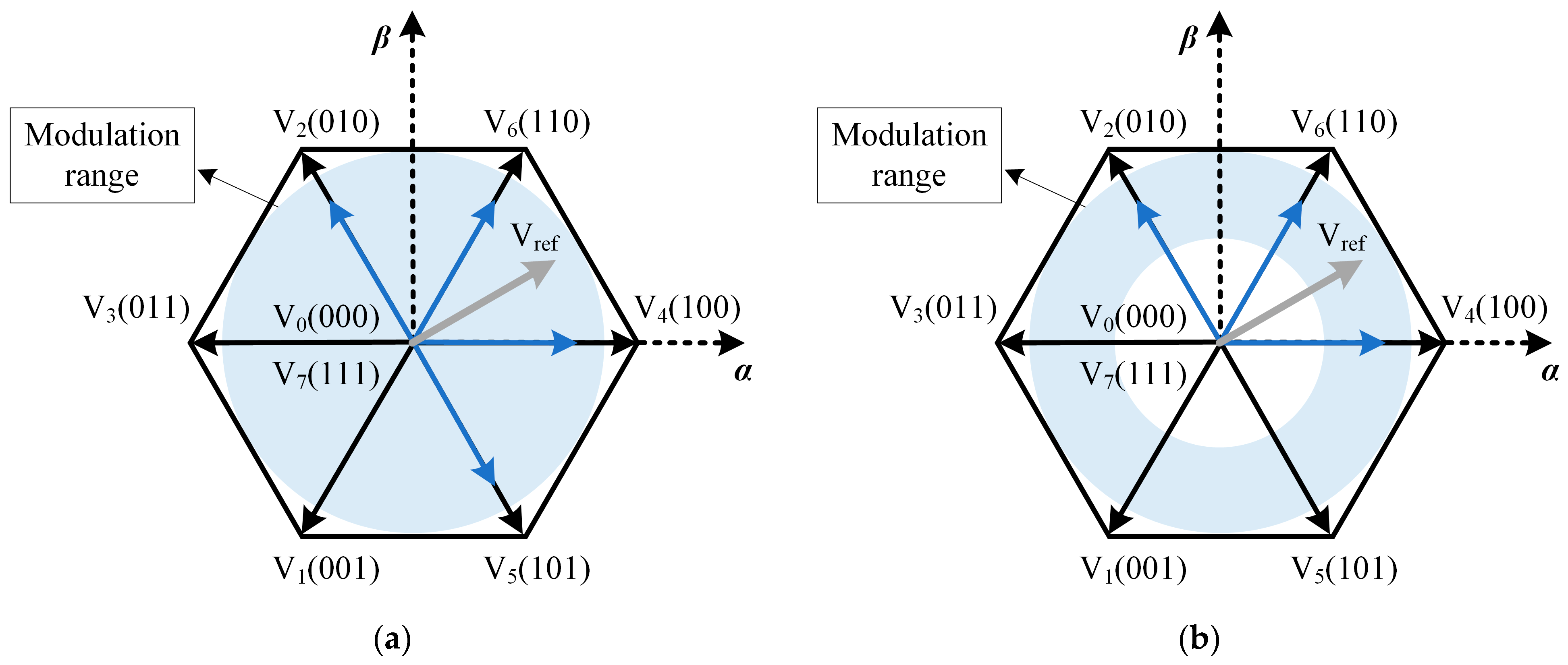
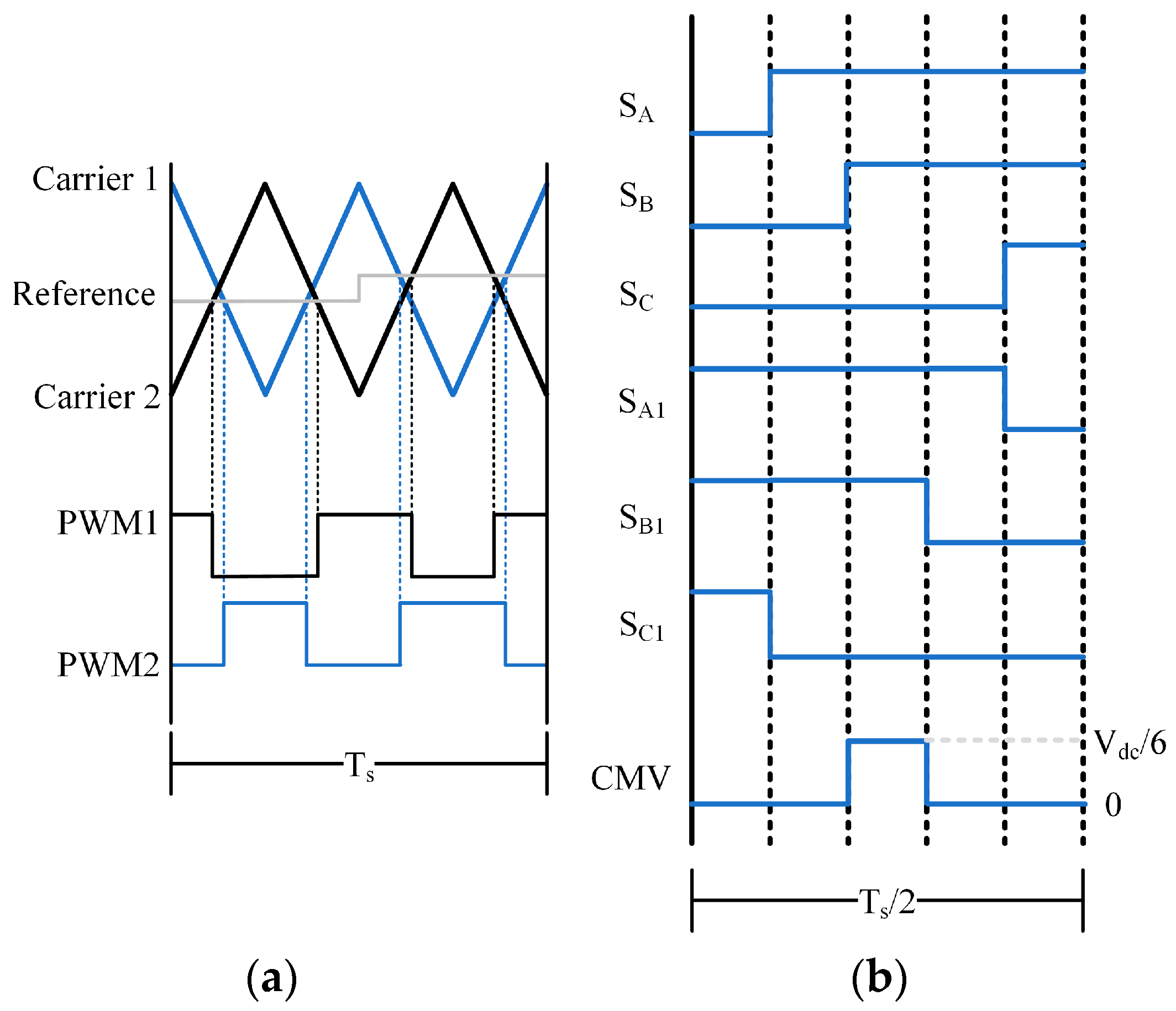

| Method | Advantages | Disadvantages | Application Scenarios | Error Range | Calculation Time |
|---|---|---|---|---|---|
| Analytical Method | Fast calculation speed, suitable for initial design or simple geometries. | Limited accuracy for complex structures, unable to fully consider parasitic effects. | Used for quick estimation of simple motor geometries. | Large | Fast |
| Two-Dimensional Finite Element Method | Balances accuracy and efficiency, suitable for planar symmetrical structures. | Only applicable to 2D structures, unable to capture complete 3D interactions, such as edge effects of windings. | Suitable for moderately complex planar symmetrical designs. | Medium | Medium |
| Three-Dimensional Finite Element Method | High accuracy, suitable for complex and asymmetrical structures. | Time-consuming with large computational demands. | Suitable for final verification of high-precision complex motor designs. | Small | Slow |
| Aspect | Inverter Hardware Optimization | Inverter Control Mode Optimization |
|---|---|---|
| Implementation Difficulty | High, involves hardware replacement and complex circuit optimization | Low, mainly depends on software adjustment, fewer hardware requirements |
| Implementation Cost | High, requires hardware replacement | Low, only involves control software |
| Effectiveness and Longevity | High, through hardware optimization, long-term effects | Medium, depends on control algorithms, may have environmental variability |
| Effect on Suppressing Bearing Current | Strong, optimized hardware circuits can significantly reduce bearing current | Medium, optimized control mode can smooth bearing currents, but with limited results |
| System Compatibility | Requires redesigning several subsystems, lower compatibility | High, can adapt to existing systems quickly, no major upgrades needed |
| Maintenance and Upgrade Difficulty | High, expanding and optimizing require hardware maintenance and upgrades | Low, optimization can be achieved through software upgrades |
| Aspect | Inverter hardware optimization | Inverter control mode optimization |
| Method | EDM Current | Circulating Bearing Current | Shaft-to-Ground Current |
|---|---|---|---|
| Passive Filter | No significant impact | Reduces by 30–50% | No significant impact |
| Active Filter | Reduces | Significantly reduces | Significantly reduces |
| Common-Mode Choke | No significant impact | Reduces | No significant impact |
| Shielded Cable | No significant impact | May increase | Reduces |
| Method | EDM current | Circulating bearing current | Shaft-to-ground current |
| Passive Filter | No significant impact | Reduces by 30–50% | No significant impact |
| Active Filter (ACC) | Reduces | Significantly reduces | Significantly reduces |
| Solution | Description | Advantages | Disadvantages | References |
|---|---|---|---|---|
| Insulated bearings | Use insulated bearings at the non-drive end to block the current path. | Effectively blocks the circulating current path and reduces the risk of bearing current. | The price is high, installation and maintenance are complicated, and mechanical performance may be affected. | [27,30] |
| Shaft grounding brushes | Install grounding brushes on the shaft to provide a low impedance path to shunt bearing current. | Economical and effective, suitable for most application scenarios, simple to install. | The bristles may wear out and need to be replaced regularly, and the life span is limited. | [27] |
| Capacitor bypass | Connect capacitors in parallel at both ends of the bearing to provide a low-impedance path to shunt the high-frequency current. | Effectively reduces high-frequency current, low cost. | The capacitance value needs to be selected accurately, which is not suitable for all scenarios. | [81] |
| Winding electrostatic shielding | Add shielding layers to the windings to reduce the capacitive coupling of the windings to the bearings. | Effectively reduces bearing voltage, suitable for high-frequency scenarios. | Increases design complexity and cost, and the design needs to be optimized to reduce losses. | [77,82] |
| Common-mode filters | Add common-mode filters to the inverter output to reduce the common-mode voltage amplitude and dv/dt. | Reduce the generation of bearing current from the source and protect the overall system. | The filter design is complex, the cost is high, and it takes up a lot of space. | [71,79] |
| Active filters | Through the control algorithm inside the filter, the active filter generates a compensation signal that is the opposite of the undesirable waveform in the power supply. | The active filter can dynamically adjust the compensation strategy as needed to adapt to the current demand under different loads and working conditions, and has strong adaptability. | Active filters require the design and implementation of more complex control algorithms and circuits, so the system structure is more complex. | [70] |
| Passive filters | A circuit composed of passive components (such as resistors, inductors, and capacitors) to filter out high-frequency noise and harmonics in the power supply. | Passive filters are simple to design and generally less expensive than active filters and other complex mitigation methods. | Passive filters have limited effectiveness in suppressing harmonics, especially over a wide frequency spectrum. | [67,68] |
| Lubricant improvements | Use high-impedance grease to increase the breakdown voltage of the lubricating film and reduce the possibility of arc discharge. | Easy to use, improves bearing life, suitable for most scenarios. | The high impedance of grease may reduce other properties and require frequent replacement. | [83,84] |
| Inverter topology optimization | A method for suppressing motor bearing current by optimizing the design of the inverter involves adjusting the output characteristics and control strategy of the inverter. | The topology can be adjusted according to actual needs to better adapt to different working environments and load conditions. | Optimizing inverter topology involves complex circuit design and control algorithms, which may require modifications to existing systems. | [65,66] |
| PWM modulation optimization | Optimize PWM modulation strategies, such as reducing switching frequency or using a three-level inverter to reduce dv/dt. | The generation of high-frequency current can be fundamentally reduced. | May reduce system efficiency and increase inverter complexity and cost. | [85,86,87] |
| Stator slot grounding electrodes | This way, by connecting the stator slots to the ground, it is possible to introduce the current in the motor to the ground effectively. | The design of the grounding electrode is relatively simple and does not require additional complex control systems or equipment. | Improper grounding design may cause changes in the stator electromagnetic field, thus affecting the operating efficiency and performance of the motor. | [73,74,78] |
| Motor design optimization | Optimize motor design through finite element analysis, such as improving the stator winding arrangement and the stator slotting method to reduce parasitic capacitance. | Improve overall motor performance and reduce parasitic capacitance coupling. | The design is complex and needs to be optimized for each application scenario, resulting in high R&D costs. | [75,76] |
| Method | Typical Methods | CMV Suppression Effect | Loss | Efficiency | Applicability |
|---|---|---|---|---|---|
| Switching State Selection | - AZS-PWM - NS-PWM | Reduces common-mode voltage to Vdc/6 | Relatively low | High | Suitable for two-level and three-level inverters, and applications with relatively low performance requirements |
| Vector Synthesis | - Virtual Space Vector PWM | Reduces common-mode voltage to Vdc/6 | Higher | Lower | Suitable for two-level and three-level inverters, and scenarios requiring higher output performance |
| Switching State Action Time | - Adjust Zero Vector Action Time | Reduces common-mode voltage amplitude | Medium | Relatively high | Back-to-back system or parallel inverter system |
| Switching State Action Sequence | - Reorder Switching Sequence | Completely eliminates common-mode voltage | Low | Medium | Back-to-back system or parallel inverter system |
| Method | Typical Methods | Complexity | CMV Suppression Effect | Features and Applicability |
|---|---|---|---|---|
| Carrier Adjustment | Carrier phase shift, changing the direction of the carrier, the angle of the carrier, and the peak position of the carrier | Low | Partial suppression | The suppression effect is good, but the harmonics are large; it is suitable for parallel and back-to-back inverters. |
| Modulation Wave Adjustment | Zero-sequence injection, dual modulation wave, wave decomposition | High | Partial suppression | Zero-sequence voltage injection is flexible and has a wide range of applications; dual modulation waves are suitable for high-performance applications; but the control is complex, and the loss increases. |
| Voltage Vector | CMV |
| 000 | −1/2 × Udc |
| 001 | −1/6 × Udc |
| 011 | 1/6 × Udc |
| 010 | 1/6 × Udc |
| 110 | 1/6 × Udc |
| 100 | −1/6 × Udc |
| 101 | 1/6 × Udc |
| 111 | 1/2 × Udc |
| Voltage vector | CMV |
|---|---|
| v00 | 1/2 × Udc |
| v77 | −1/2 × Udc |
| v37, v57, v67, v73, v75, v76 | 1/3 × Udc |
| v01, v02, v04, v10, v20, v40 | −1/3 × Udc |
| v35, v56, v63, v17, v27, v47 v71, v72, v74, v36, v53, v65 v33, v55, v66 | 1/6 × Udc |
| v14, v21, v42, v03, v05, v06 v30, v50, v60, v12, v24, v41 v11, v22, v44 | −1/6 × Udc |
| v07, v70, v16, v25, v34, v43 v52, v61, v15, v23, v31, v46 v54, v62, v13, v26, v32, v45 v51, v64 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pei, T.; Zhang, H.; Hua, W.; Zhang, F. Comprehensive Review of Bearing Currents in Electrical Machines: Mechanisms, Impacts, and Mitigation Techniques. Energies 2025, 18, 517. https://doi.org/10.3390/en18030517
Pei T, Zhang H, Hua W, Zhang F. Comprehensive Review of Bearing Currents in Electrical Machines: Mechanisms, Impacts, and Mitigation Techniques. Energies. 2025; 18(3):517. https://doi.org/10.3390/en18030517
Chicago/Turabian StylePei, Tianyi, Hengliang Zhang, Wei Hua, and Fengyu Zhang. 2025. "Comprehensive Review of Bearing Currents in Electrical Machines: Mechanisms, Impacts, and Mitigation Techniques" Energies 18, no. 3: 517. https://doi.org/10.3390/en18030517
APA StylePei, T., Zhang, H., Hua, W., & Zhang, F. (2025). Comprehensive Review of Bearing Currents in Electrical Machines: Mechanisms, Impacts, and Mitigation Techniques. Energies, 18(3), 517. https://doi.org/10.3390/en18030517







