A Method for the Modular Power Flow Analysis of Extensive Distribution Grids
Abstract
:1. Introduction
- The validation of limit compliance: The conventional static load models do not allow for validating compliance with the voltage and current limits within the system parts they represent. For instance, in an MV-level study, limit compliance within the LV level cannot be validated if the LV subsystems are not explicitly modelled but represented by conventional static load models.
- Inaccuracy in load model parametrization: The parameters of the conventional static load models are identified based on simplified aggregation methods that neglect effects such as losses and spatial voltage variations. Depending on the system’s characteristics, this can lead to significant inaccuracies, especially if voltage-dependent elements such as Volt/var-controlled PV inverters are connected.
- Redundant computational effort in repeated simulations: Many studies require multiple simulations of the same distribution system while varying only a small set of parameters, such as in n-1 security analysis (where a single element is taken out of service), static voltage stability analysis (where active or reactive power injections at specific nodes are incremented), and control parametrization studies (where the parameters of a controller are varied). The conventional approach necessitates recalculating the entire studied system part for each simulation run, even when certain subsystems remain unchanged, leading to unnecessary computational overhead.
- The extended static load model allows for validating compliance with the voltage and current limits within the system part it represents.
- The component-based parameter identification method enables the accurate aggregation of system parts including voltage-dependent loads by considering the effects of the intermediate network, i.e., network losses and spatial voltage variations.
- The modular power flow approach provides a systematic and computationally practicable methodology for analyzing extensive distribution systems. In studies involving repeated simulations, this approach allows for the aggregation of subsystems that remain unchanged during the study, ensuring that only system parts subject to parameter variations are recalculated, thereby reducing computational overhead.
2. Materials and Methods
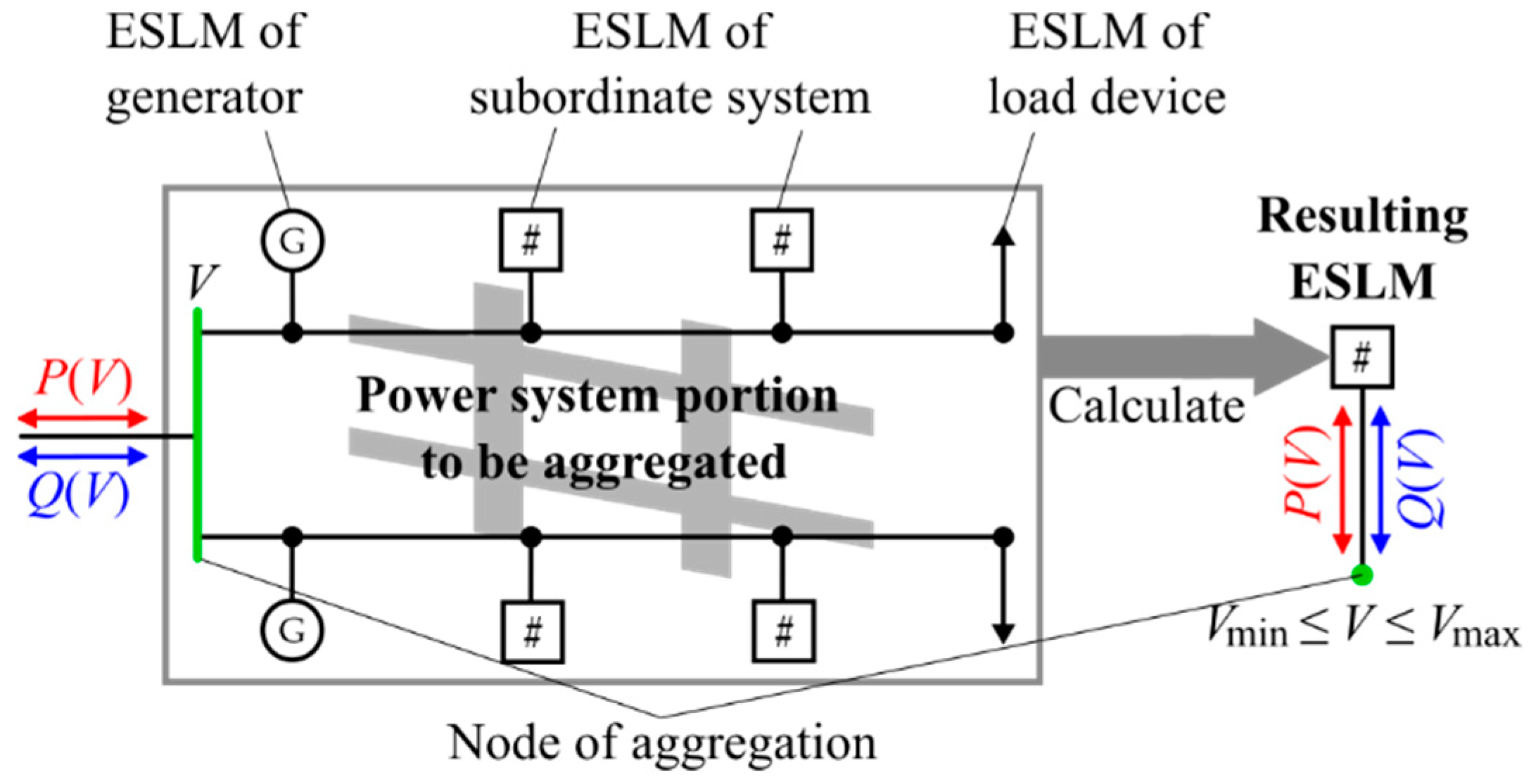
2.1. Extended Static Load Modeling
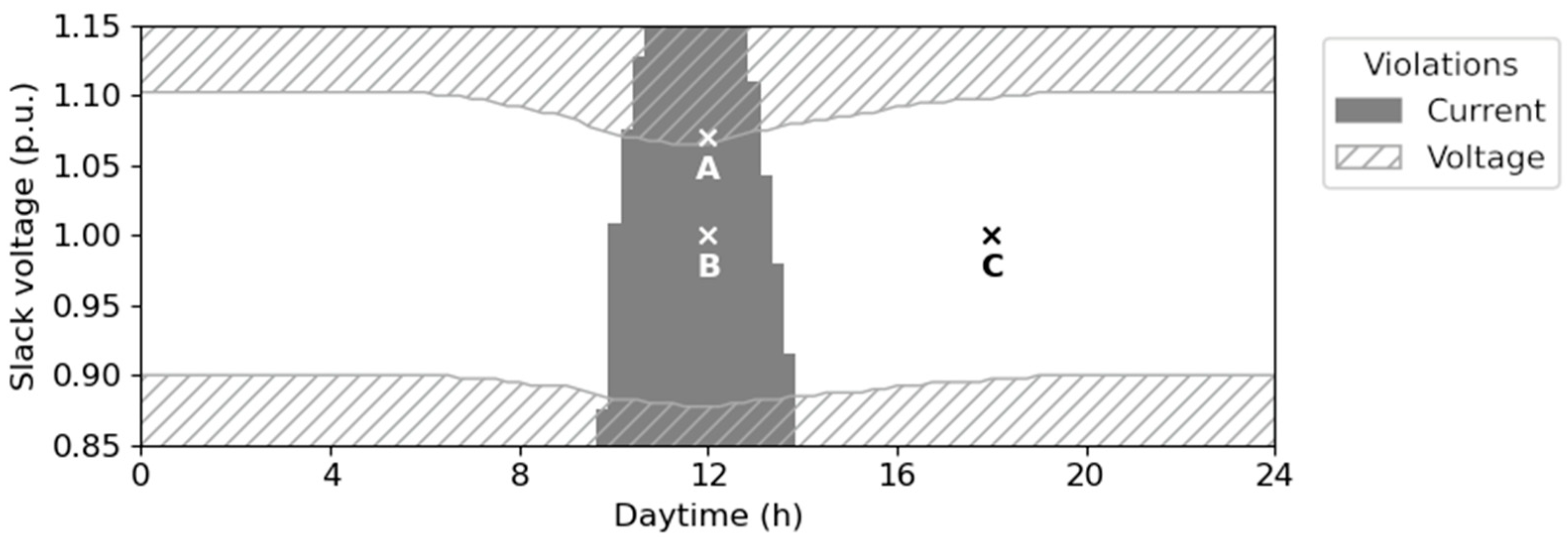
2.1.1. Boundary Voltage Limits
2.1.2. Extended Static Load Model
2.1.3. Component-Based Identification of Model Parameters
- 4.
- Connect the slack node element to the node of aggregation.
- 5.
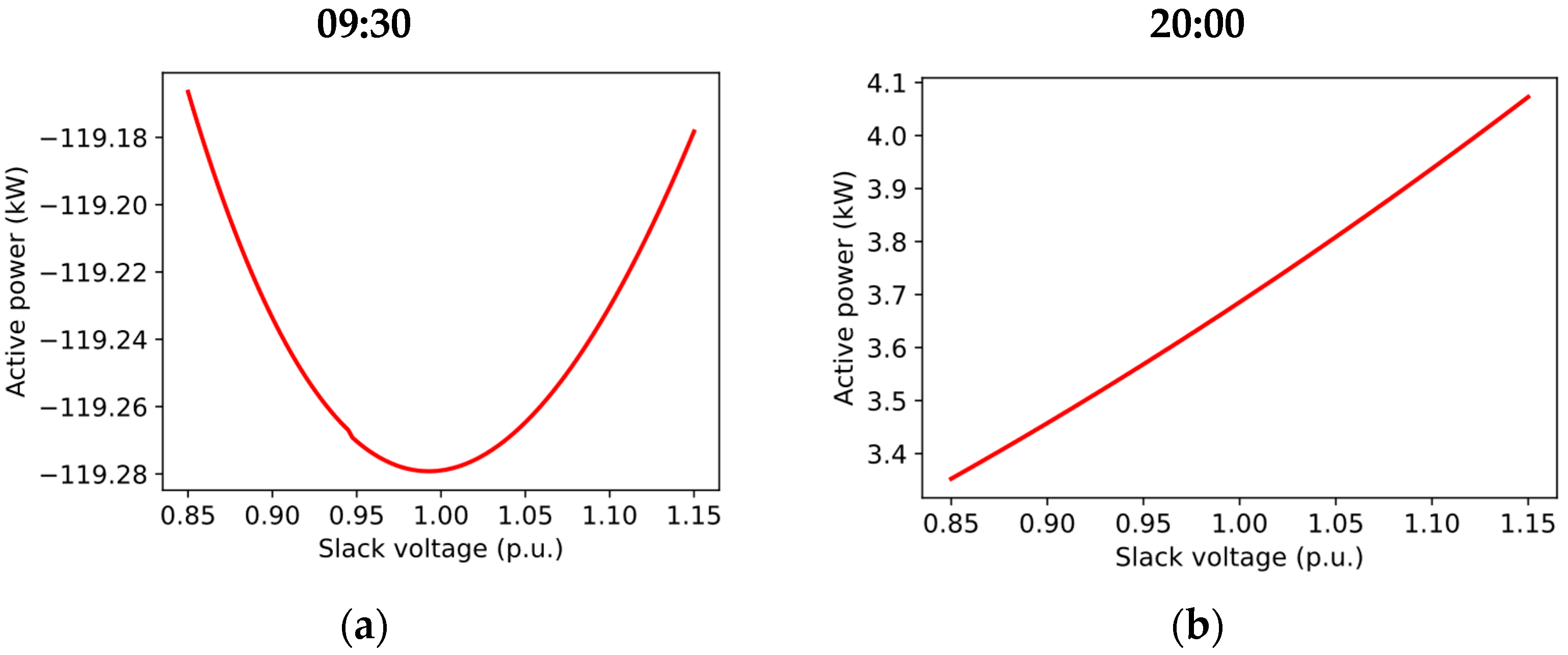
- Define the slack voltage range of interest, e.g., from 0.9 to 1.1 p.u., and the corresponding voltage resolution, e.g., 0.0025 p.u. steps.
- 6.
- Repeat load flow simulations for all defined slack voltage values and record the active and reactive power provided by the slack node element as functions of the slack voltage. Furthermore, record all slack voltage values that do not provoke violations of the
- internal current limits, i.e., current limits of the system portion to be aggregated, or
- external voltage limits, i.e., BVLs of subordinate ESLMs.
2.2. Modular Power Flow Analysis
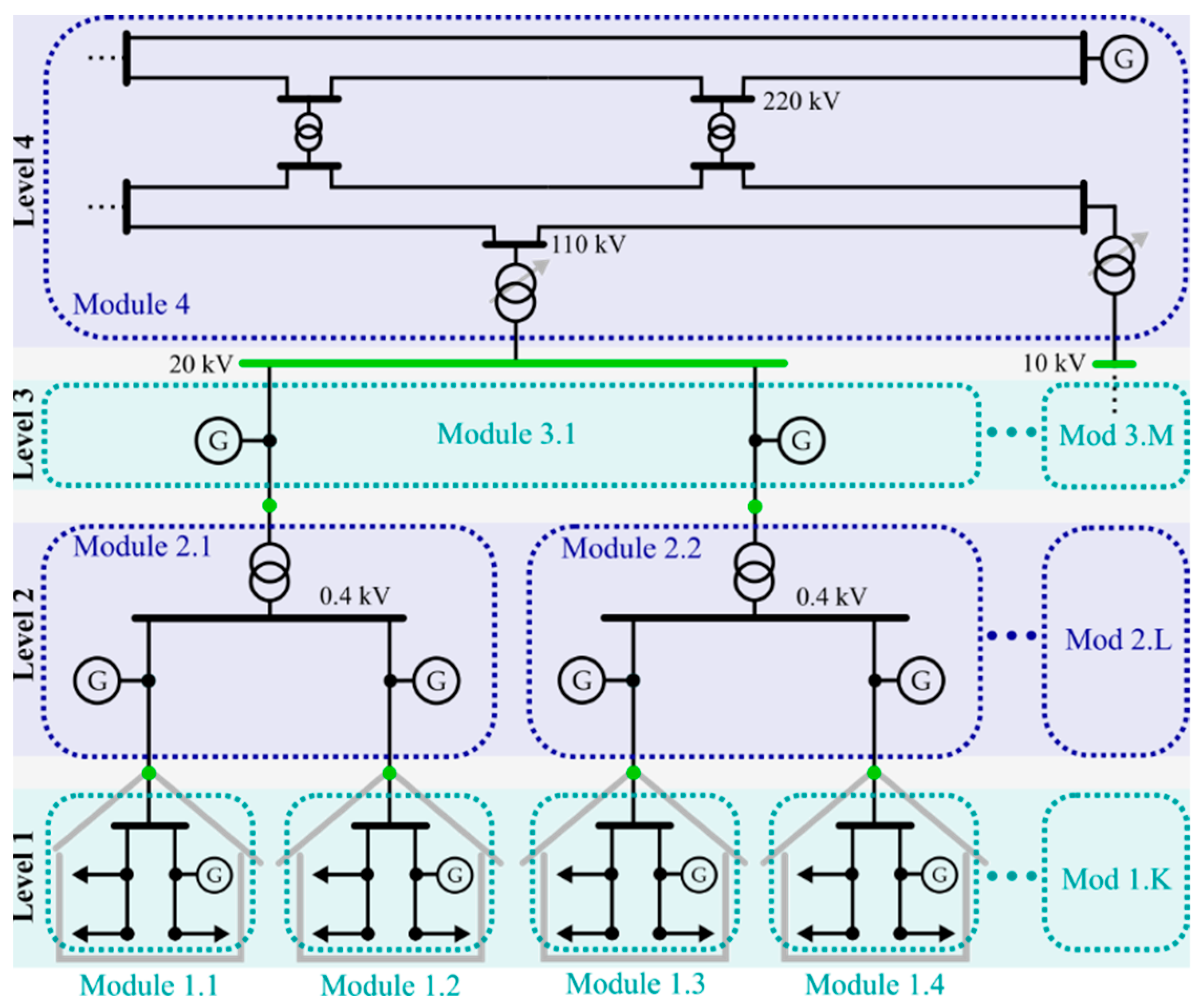
2.2.1. Modularization of the Power System
2.2.2. Simulation Procedure
2.2.3. Scope of Applicability
- Power system structure—Each power system can be divided into arbitrary modules interconnected through single or multiple nodes, although its partitioning along multiple nodes involves significant challenges, as subordinate system parts connected via multiple nodes cannot be accurately represented by conventional and extended static load models (e.g., linear, exponential, or polynomial). This limitation arises because the power flow through each interconnection node depends not only on the voltage magnitudes at all interconnection nodes but also on the phase angle differences between them. Equation (6) illustrates these dependencies for a module with two connection nodes to the superordinate grid without considering the frequency.
- State variables—Some power system elements that are relevant for power flow analysis have state variables, such as the tap positions of OLTCs and the state of charge of storage systems. In QSTS power flow simulations, these state variables are initialized for the first instance of time and permanently updated according to the power flow results. As the power flow results depend on the used slack voltage, the state variables depend on the complete time and slack voltage history, and consequently, they cannot be described as algebraic functions of (V, t). Hence, the modular approach does not apply to system portions that contain elements with state variables. However, the state variables are often neglected in studies that are not explicitly dedicated to their investigation in order to facilitate the analysis and enable parallel computation. For instance, the time-dependent states of OLTCs are frequently neglected, e.g., by modeling the tap changers as continuous elements that keep the voltage at a specific setpoint, or by initializing the tap position with the same value for each instance of time. Storage units are often represented by node elements with predefined load profiles instead of modeling their state of charge.
2.3. Application Example
2.3.1. Test System Description
2.3.2. Methodology
3. Results
3.1. Aggregation of the LV Level
3.2. Aggregation of the MV Level
3.3. Calculation Accuracy at the MV Level
4. Discussions
4.1. Accurate Computation of Power Flows
4.2. Validation of Compliance with Actual Limits
4.3. Systematization of Power Flow Studies
4.4. Computational Effort and Performance
4.5. Limitations
4.6. Future Work
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BVL | Boundary voltage limit |
| CP | Customer plant |
| DG | Distributed generator |
| DP | Delivery point |
| DTR | Distribution transformer |
| ESLM | Extended static load model |
| HV | High voltage |
| LV | Low voltage |
| MV | Medium voltage |
| OLTC | On-load tap changer |
| PV | Photovoltaic |
| QSTS | Quasi-static time-series |
| STR | Supplying transformer |
Appendix A

References
- Martinez, J.A.; Mahseredjian, J. Load Flow Calculations in Distribution Systems with Distributed Resources. A Review. In Proceedings of the 2011 IEEE Power and Energy Society General Meeting, Detroit, MI, USA, 24–28 July 2011; pp. 1–8. [Google Scholar]
- Price, W.W.; Chiang, H.-D.; Clark, H.K.; Concordia, C.; Lee, D.C.; Hsu, J.C.; Ihara, S.; King, C.A.; Lin, C.J.; Mansour, Y.; et al. Load Representation for Dynamic Performance Analysis (of Power Systems). IEEE Trans. Power Syst. 1993, 8, 472–482. [Google Scholar] [CrossRef]
- Arif, A.; Wang, Z.; Wang, J.; Mather, B.; Bashualdo, H.; Zhao, D. Load Modeling—A Review. IEEE Trans. Smart Grid 2018, 9, 5986–5999. [Google Scholar] [CrossRef]
- Modelling and Aggregation of Loads in Flexible Power Networks. Available online: https://e-cigre.org/publication/566-modelling-and-aggregation-of-loads-in-flexible-power-networks (accessed on 5 May 2022).
- Standard Load Models for Power Flow and Dynamic Performance Simulation. IEEE Trans. Power Syst. 1995, 10, 1302–1313. [CrossRef]
- Schultis, D.-L.; Petrusic, S.; Ilo, A. Modelling of Low-Voltage Grids with High PV Share and Q(U)-Control. CIRED Open Access Proc. J. 2021, 2020, 501–504. [Google Scholar] [CrossRef]
- IEEE Std 2781-2022; IEEE Guide for Load Modeling and Simulations for Power Systems. IEEE: Piscataway, NJ, USA, 2022; pp. 1–88. [CrossRef]
- Ren, H.; Schulz, N.N.; Krishnan, V.; Zhang, Y. Online Static Load Model Estimation in Distribution Systems. In Proceedings of the 2019 IEEE 28th International Symposium on Industrial Electronics (ISIE), Vancouver, BC, Canada, 12–14 June 2019; pp. 153–158. [Google Scholar]
- Wang, C.; Wang, Z.; Wang, J.; Zhao, D. Robust Time-Varying Parameter Identification for Composite Load Modeling. IEEE Trans. Smart Grid 2019, 10, 967–979. [Google Scholar] [CrossRef]
- Ballanti, A.; Ochoa, L.F. Initial Assessment of Voltage-Led Demand Response from UK Residential Loads. In Proceedings of the 2015 IEEE Power & Energy Society Innovative Smart Grid Technologies Conference (ISGT), Washington, DC, USA, 18–20 February 2015; pp. 1–5. [Google Scholar]
- Lamberti, F.; Dong, C.; Calderaro, V.; Ochoa, L.F. Estimating the Load Response to Voltage Changes at UK Primary Substations. In Proceedings of the IEEE PES ISGT Europe 2013, Lyngby, Denmark, 6–9 October 2013; pp. 1–5. [Google Scholar]
- Ballanti, A.; Ochoa, L.F. Voltage-Led Load Management in Whole Distribution Networks. IEEE Trans. Power Syst. 2018, 33, 1544–1554. [Google Scholar] [CrossRef]
- Schultis, D.-L.; Ilo, A. Adaption of the Current Load Model to Consider Residential Customers Having Turned to LED Lighting. In Proceedings of the 2019 IEEE PES Asia-Pacific Power and Energy Engineering Conference (APPEEC), Macao, China, 1–4 December 2019; pp. 1–5. [Google Scholar]
- Collin, A.J.; Tsagarakis, G.; Kiprakis, A.E.; McLaughlin, S. Development of Low-Voltage Load Models for the Residential Load Sector. IEEE Trans. Power Syst. 2014, 29, 2180–2188. [Google Scholar] [CrossRef]
- Collin, A.J.; Acosta, J.L.; Hayes, B.P.; Djokic, S.Z. Component-Based Aggregate Load Models for Combined Power Flow and Harmonic Analysis. In Proceedings of the 7th Mediterranean Conference and Exhibition on Power Generation, Transmission, Distribution and Energy Conversion (MedPower 2010), Agia Napa, Cyprus, 7–10 November 2010; pp. 1–10. [Google Scholar]
- Tang, Y.; Zhang, H.-B.; Zhang, D.-X.; Hou, J.-X. A Synthesis Load Model with Distribution Network for Power Transmission System Simulation and Its Validation. In Proceedings of the 2009 IEEE Power & Energy Society General Meeting, Calgary, AB, Canada, 26–30 July 2009; pp. 1–7. [Google Scholar]
- Chen, Q.; Ju, P.; Shi, K.Q.; Tang, Y.; Shao, Z.Y.; Yang, W.Y. Parameter Estimation and Comparison of the Load Models with Considering Distribution Network Directly or Indirectly. Int. J. Electr. Power Energy Syst. 2010, 32, 965–968. [Google Scholar] [CrossRef]
- Wang, Q.; Zhao, B.; Ren, B.; Li, Q.; Hao, J.; Lan, T.; Luo, P. Synthetic Load Modelling Considering the Influence of Distributed Generation. Energy Rep. 2023, 9, 662–669. [Google Scholar] [CrossRef]
- Wu, P.; Zhang, X.; Lu, C.; Wang, Y.; Ye, H.; Ling, X. A Composite Load Model Aggregation Method and Its Equivalent Error Analysis. Int. J. Electr. Power Energy Syst. 2023, 150, 109098. [Google Scholar] [CrossRef]
- Joshi, K.; Pindoriya, N. Advances in Distribution System Analysis with Distributed Resources: Survey with a Case Study. Sustain. Energy Grids Netw. 2018, 15, 86–100. [Google Scholar] [CrossRef]
- Power Distribution in Europe—Facts & Figures. Available online: https://www3.eurelectric.org/powerdistributionineurope/ (accessed on 4 October 2023).
- Turiman, M.S.; Sarmin, M.K.N.M.; Saadun, N.; Chong, L.C.; Ali, H.; Mohammad, Q. Analysis of High Penetration Level of Distributed Generation at Medium Voltage Levels of Distribution Networks. In Proceedings of the 2022 IEEE International Conference on Power Systems Technology (POWERCON), Kuala Lumpur, Malaysia, 12–14 September 2022; pp. 1–6. [Google Scholar]
- Arshad, A.; Lehtonen, M. Multi-Agent System Based Distributed Voltage Control in Medium Voltage Distribution Systems. In Proceedings of the 2016 17th International Scientific Conference on Electric Power Engineering (EPE), Prague, Czech Republic, 16–18 May 2016; pp. 1–6. [Google Scholar]
- Jayasekara, N.; Masoum, M.A.S.; Wolfs, P.J. Optimal Operation of Distributed Energy Storage Systems to Improve Distribution Network Load and Generation Hosting Capability. IEEE Trans. Sustain. Energy 2016, 7, 250–261. [Google Scholar] [CrossRef]
- OVE EN 50160; Voltage Characteristics of Electricity Supplied by Public Electricity Networks. Austrian Standards: Vienna, Austria, 2020. Available online: https://www.austrian-standards.at/de/shop/ove-en-50160-2020-12-01~p2557383 (accessed on 25 June 2024).
- Bolgaryn, R.; Scheidler, A.; Braun, M. Combined Planning of Medium and Low Voltage Grids. In Proceedings of the 2019 IEEE Milan PowerTech, Milan, Italy, 23–27 June 2019; pp. 1–6. [Google Scholar]
- Schultis, D.-L.; Ilo, A. Increasing the Utilization of Existing Infrastructures by Using the Newly Introduced Boundary Voltage Limits. Energies 2021, 14, 5106. [Google Scholar] [CrossRef]
- Meinecke, S.; Sarajlić, D.; Drauz, S.R.; Klettke, A.; Lauven, L.-P.; Rehtanz, C.; Moser, A.; Braun, M. SimBench—A Benchmark Dataset of Electric Power Systems to Compare Innovative Solutions Based on Power Flow Analysis. Energies 2020, 13, 3290. [Google Scholar] [CrossRef]
- Meinecke, S.; Bornhorst, N.; Lauven, L.-P.; Menke, J.-H.; Braun, M.; Drauz, S.; Spalthoff, C.; Cronbach, D.; Kneiske, T.; Klettke, A.; et al. SimBench—Dokumentation. Available online: https://simbench.de/wp-content/uploads/2021/09/simbench_documentation_de_1.1.0.pdf (accessed on 11 February 2025).
- Obilor, E.I.; Amadi, E. Test for Significance of Pearson’s Correlation Coefficient (r). Int. J. Innov. Math. Stat. Energy Policies 2018, 6, 11–23. [Google Scholar]
- Brunner, H.; Korner, C.; Wieland, T.; Brandl, S.; Ortner, M. Methods and Future Scenarios for Strategic Grid Development of Full Low and Medium Voltage DSO Supply Areas. IET Conf. Proc. 2023, 2023, 2872–2876. [Google Scholar] [CrossRef]




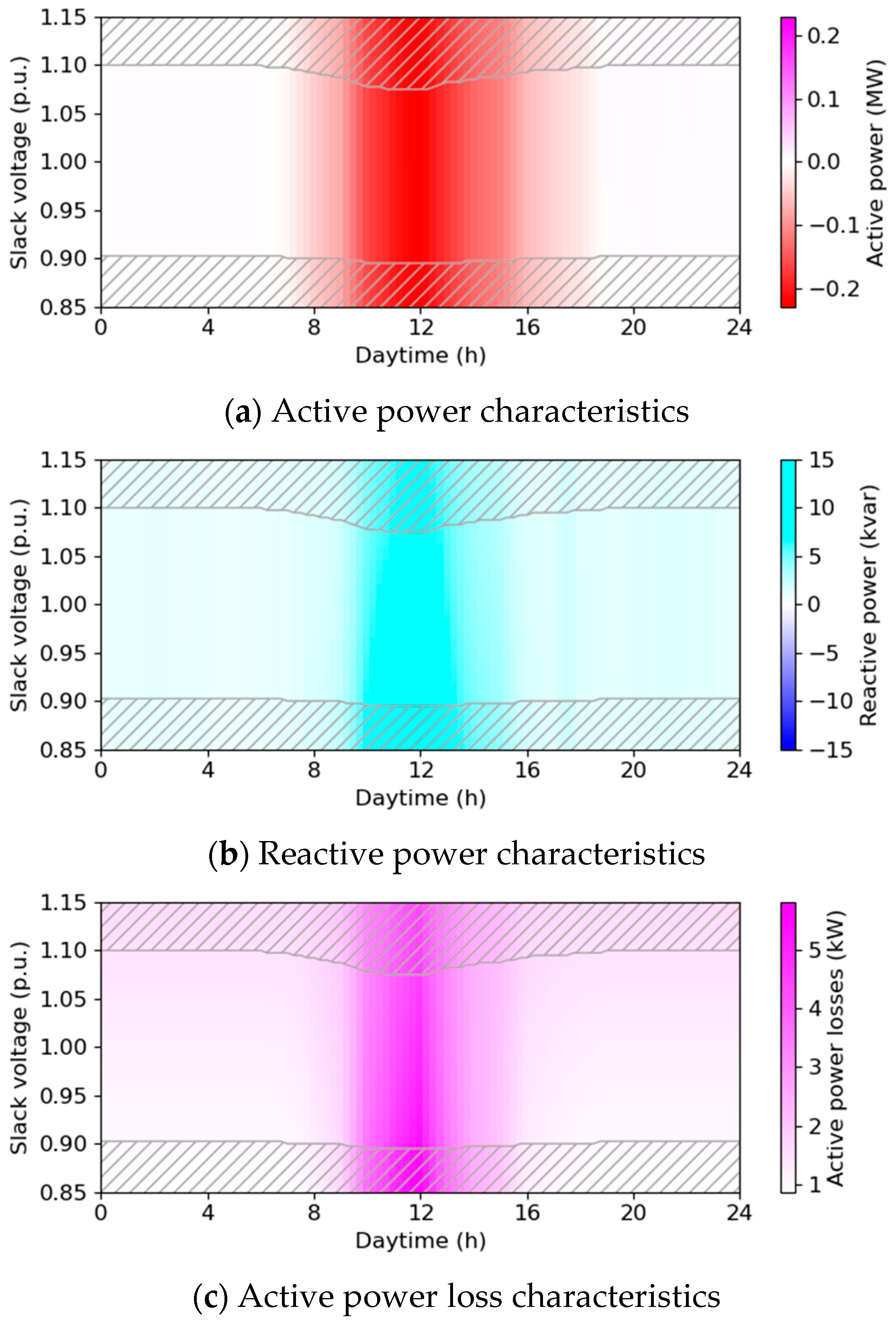
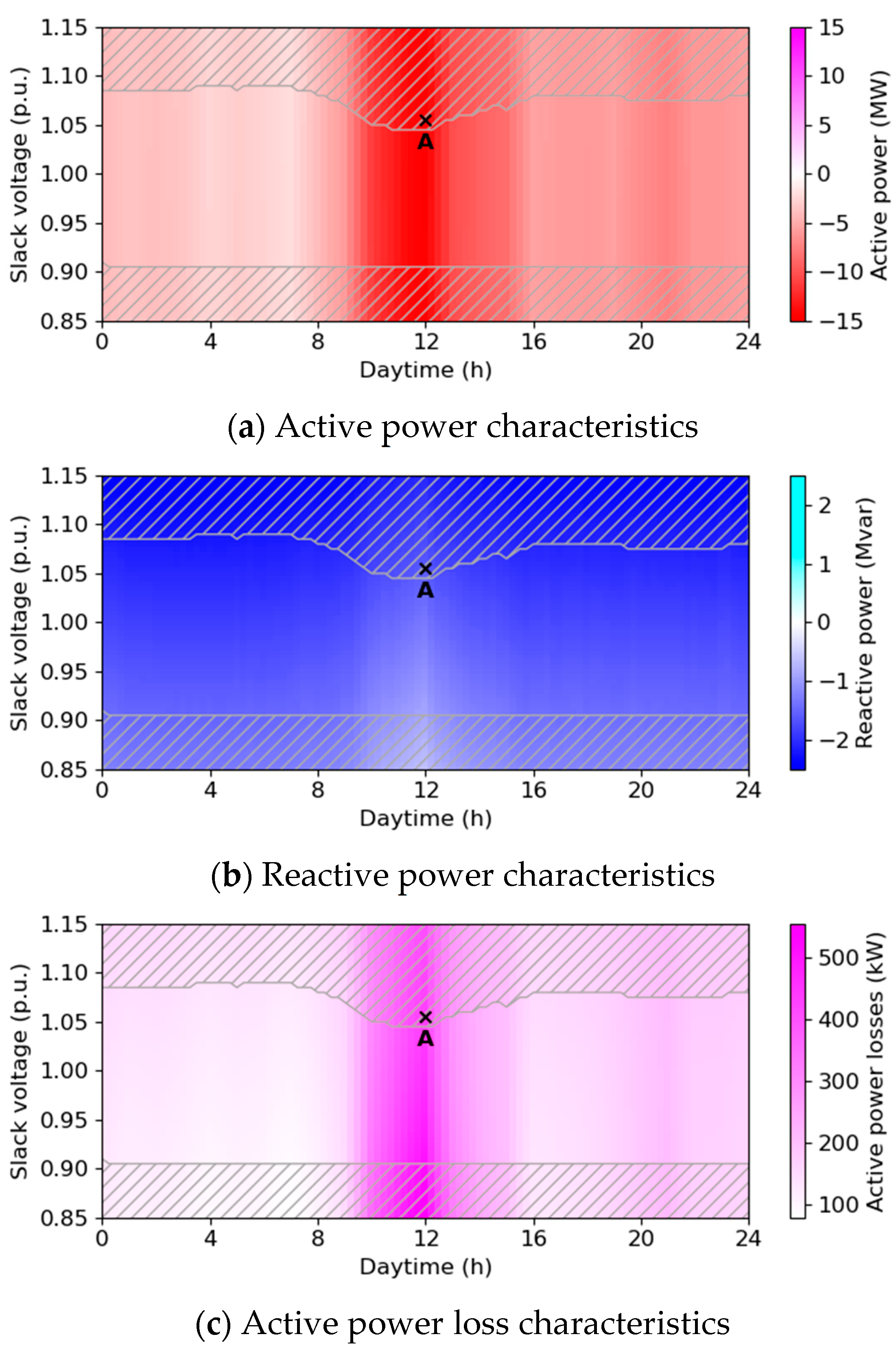



| (p.u.) | (p.u.) | (p.u.) | (kW) | (kvar) | (kW) |
|---|---|---|---|---|---|
| 0.858 | 1.075 | 1.100 | −228.77 | 8.24 | 4.46 |
| 1.095 | −228.75 | 8.31 | 4.48 | ||
| … | … | … | … | ||
| 0.905 | −227.86 | 11.53 | 5.37 | ||
| 0.900 | −227.82 | 11.64 | 5.41 |
| Type | DTR Rating (kVA) | No. Feeders | Max. Feeder Length (m) | Number of CPs |
|---|---|---|---|---|
| Small rural | 160 or 250 | 4 | 245.34 | 13 |
| Mid-size rural | 250 | 4 | 564.69 | 99 |
| Large rural | 400 | 9 | 479.34 | 118 |
| Semiurban | 400 | 3 | 228.98 | 41 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schultis, D.-L.; Korner, C. A Method for the Modular Power Flow Analysis of Extensive Distribution Grids. Energies 2025, 18, 1559. https://doi.org/10.3390/en18061559
Schultis D-L, Korner C. A Method for the Modular Power Flow Analysis of Extensive Distribution Grids. Energies. 2025; 18(6):1559. https://doi.org/10.3390/en18061559
Chicago/Turabian StyleSchultis, Daniel-Leon, and Clemens Korner. 2025. "A Method for the Modular Power Flow Analysis of Extensive Distribution Grids" Energies 18, no. 6: 1559. https://doi.org/10.3390/en18061559
APA StyleSchultis, D.-L., & Korner, C. (2025). A Method for the Modular Power Flow Analysis of Extensive Distribution Grids. Energies, 18(6), 1559. https://doi.org/10.3390/en18061559







