Study on the Stabilization Mechanism of Gas Injection Interface in Fractured-Vuggy Reservoirs
Abstract
1. Introduction
2. Experimental Materials and Procedure
2.1. Visual Physical Model of Cracks and Holes
- (1)
- System Integration: capable of synchronously simulating gas injection-water injection (edge/bottom water) collaborative displacement processes and conducting simulation experiments for different fracture-cavity filling degrees;
- (2)
- Advanced Observation: through 10 visualization observation windows symmetrically distributed on both sides, real-time capture of dynamic migration characteristics of oil–gas–water three-phase interfaces is enabled;
- (3)
- Parameter Controllability: equipped with a precision temperature-pressure control system to accurately regulate parameters such as formation dip angle, water intrusion position, and water intrusion multiples;
- (4)
- Detection Reliability: utilizing a dual-color tracer system (Sudan Red for crude oil staining and Methylene Blue for injected water staining) combined with high-resolution image acquisition technology, ensuring three-phase interface recognition accuracy at the millimeter level.
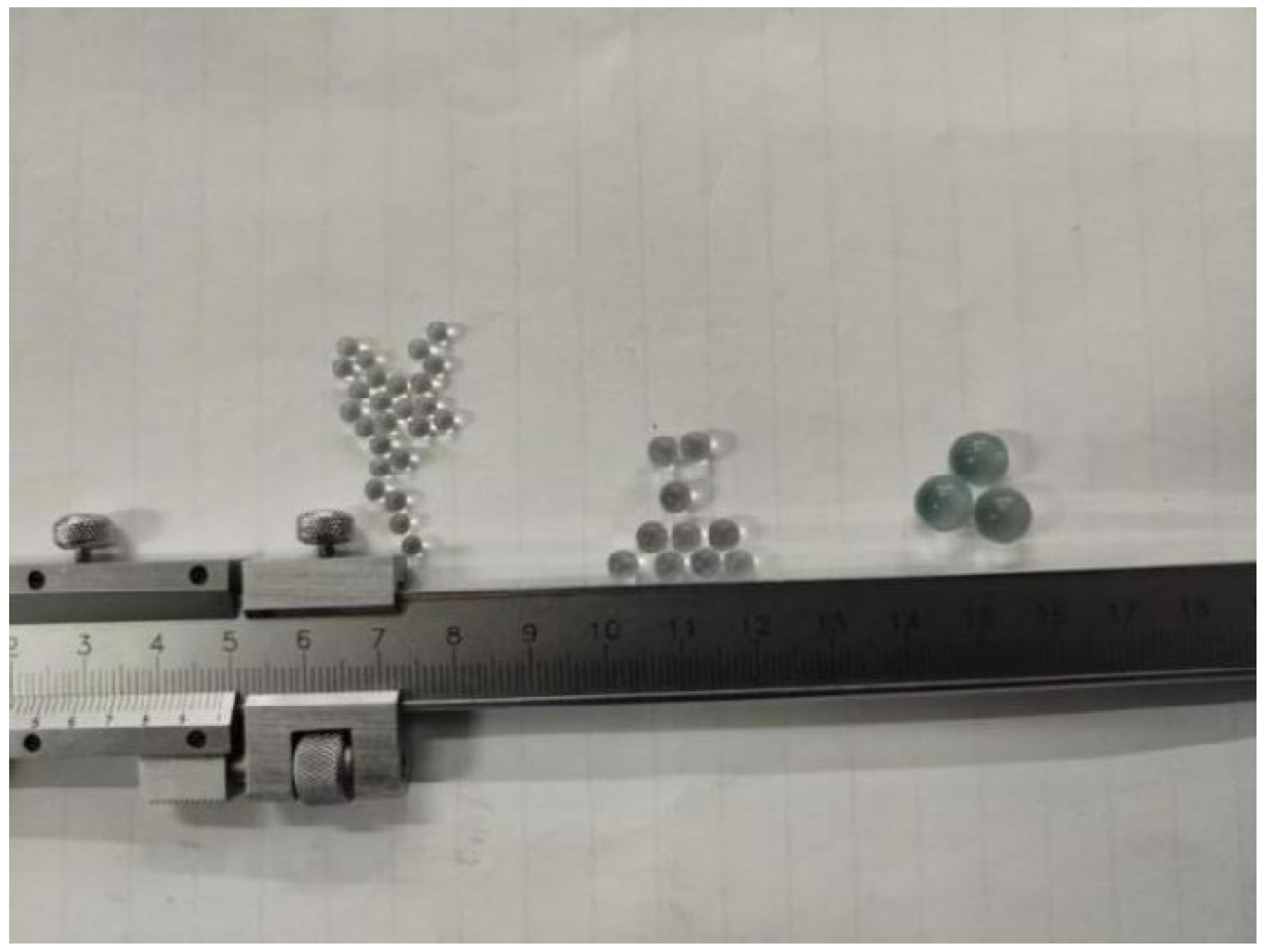
2.2. Filling Medium
2.3. Experimental Procedure
- (1)
- The experiments were carried out at 20 °C and atmospheric pressure.
- (2)
- After the glass ball is loaded into the 3D visual fracture and hole physical model, change the inclination of the model, and then wholly saturate the oil.
- (3)
- Slow water flooding was carried out from the bottom at a displacement pressure of 150 psi. When the water cut of the fluid at the outlet end was close to 99%, nitrogen gas injection was started from the top, and a video camera was used to take pictures and record the interface changes.
- (4)
- The experiment is stopped when the production well no longer produces oil.
2.4. Comparison of Experimental Schemes
2.5. Fracture-Vuggy Reservoir Simulation
3. Results and Discussion
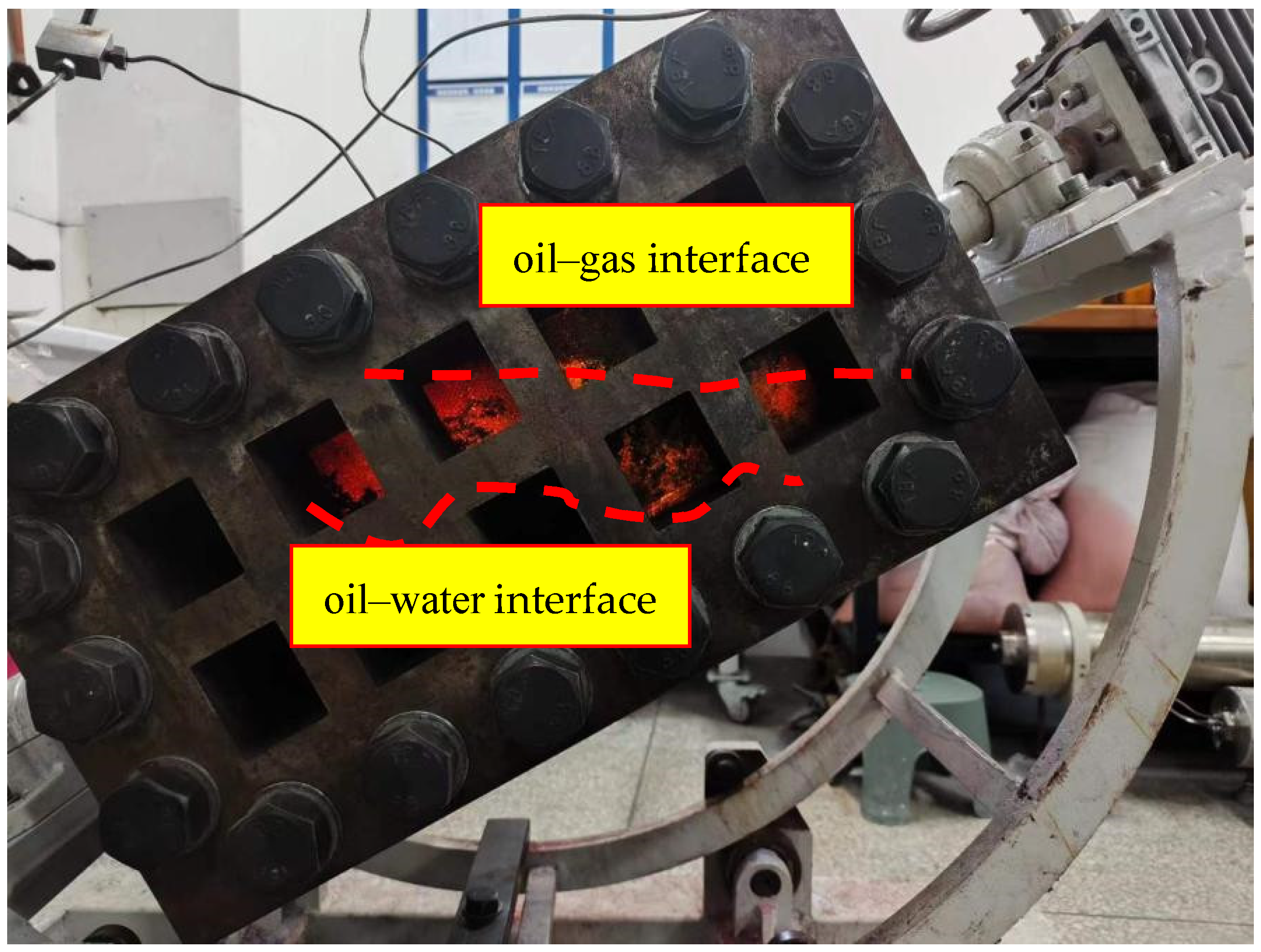
3.1. Oil–Water Interface Characteristics of Fractured-Vuggy Reservoirs
3.2. Gas Injection from the Top After Water Flooding
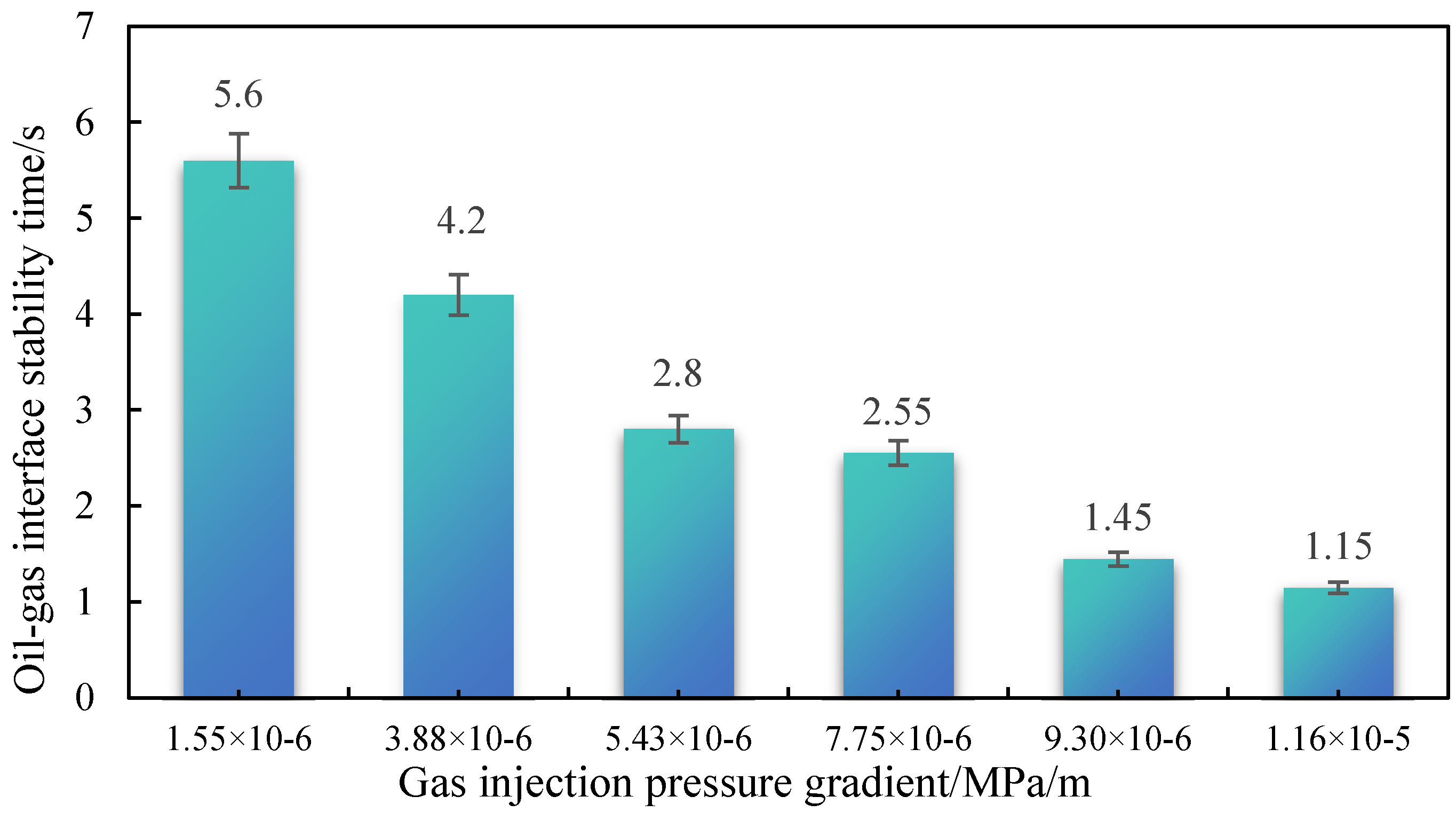
3.3. Stability Characterization of the Gas Injection Interface
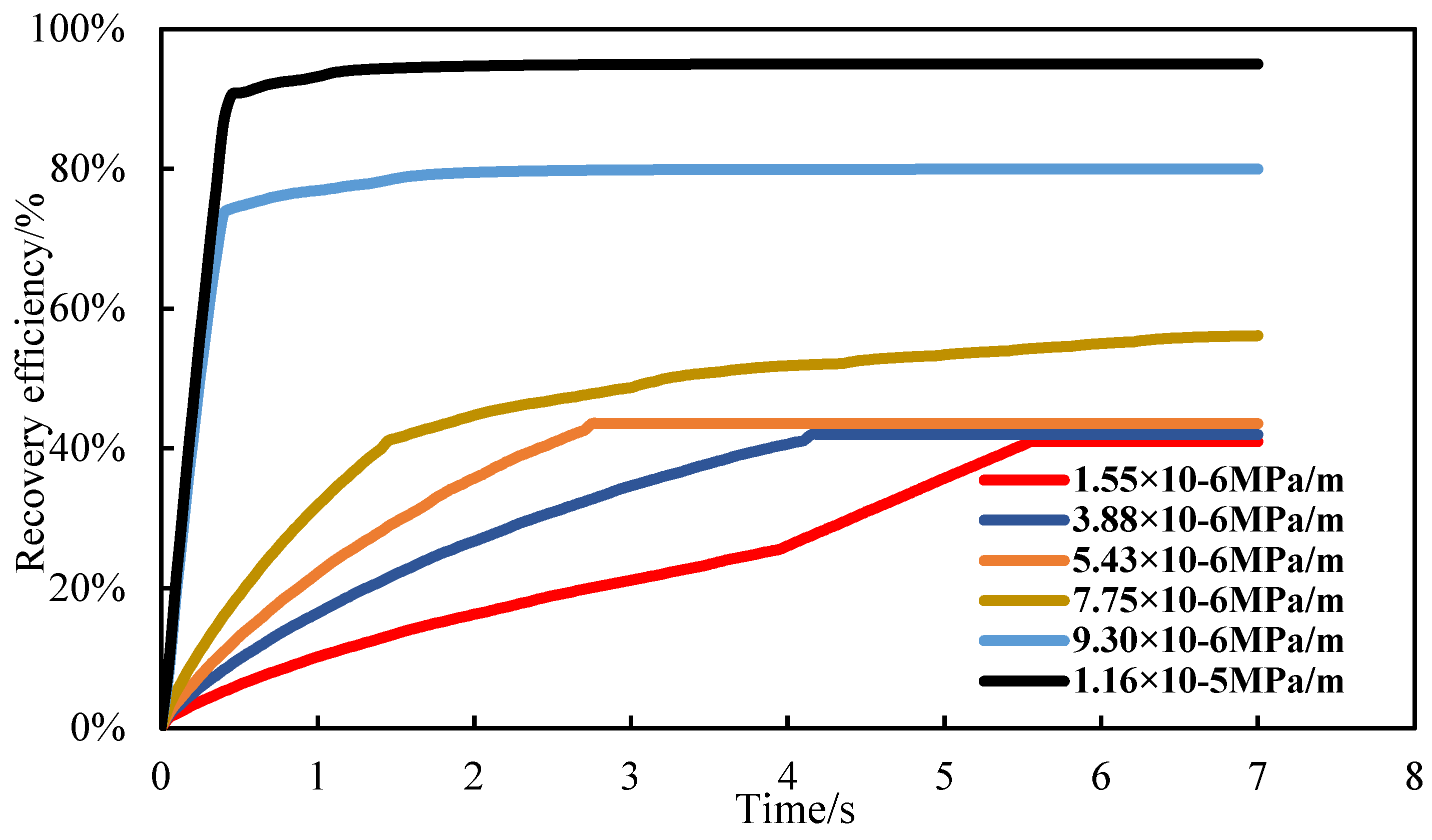
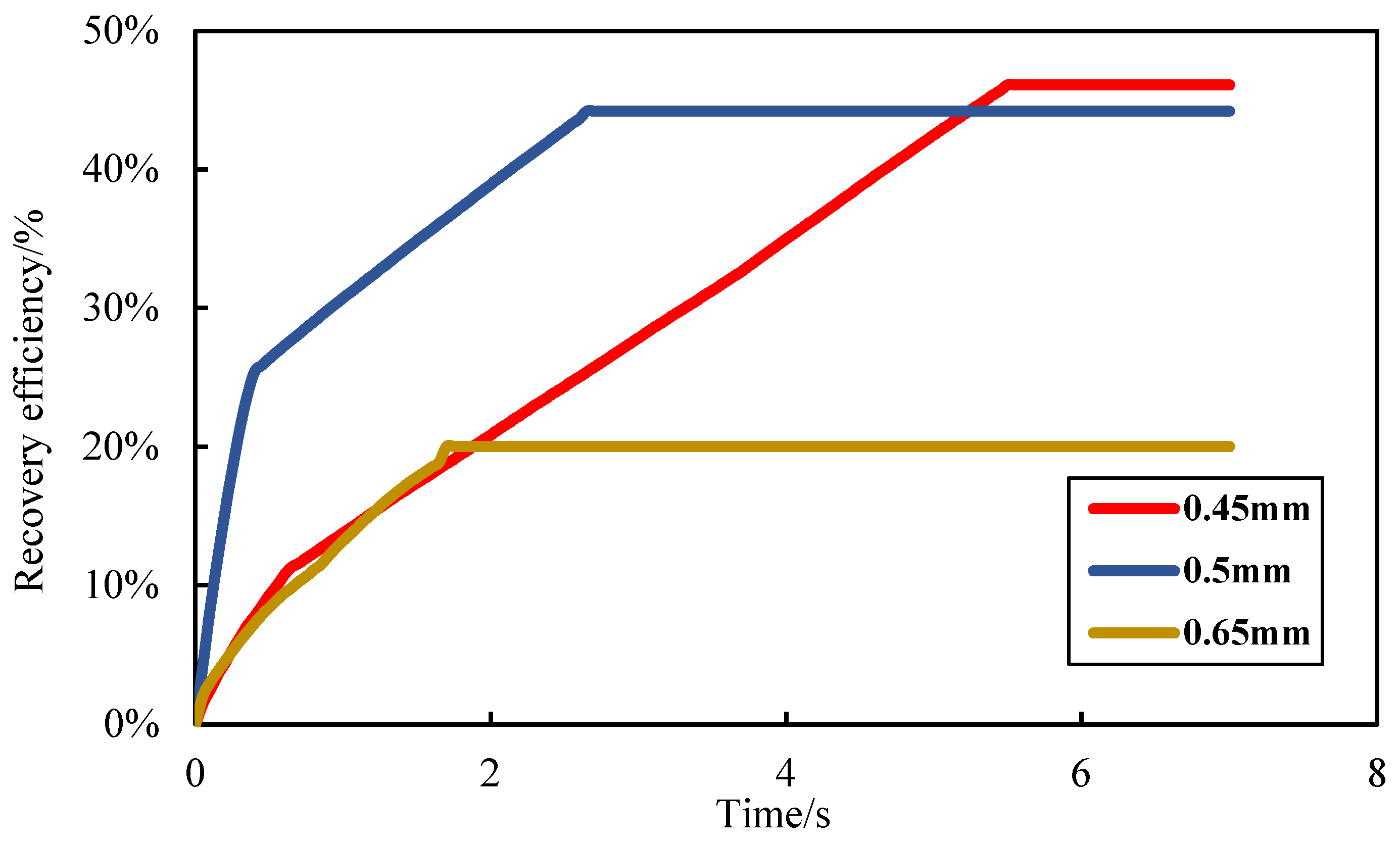
3.4. Numerical Simulation Analysis of Interface Stability Factors
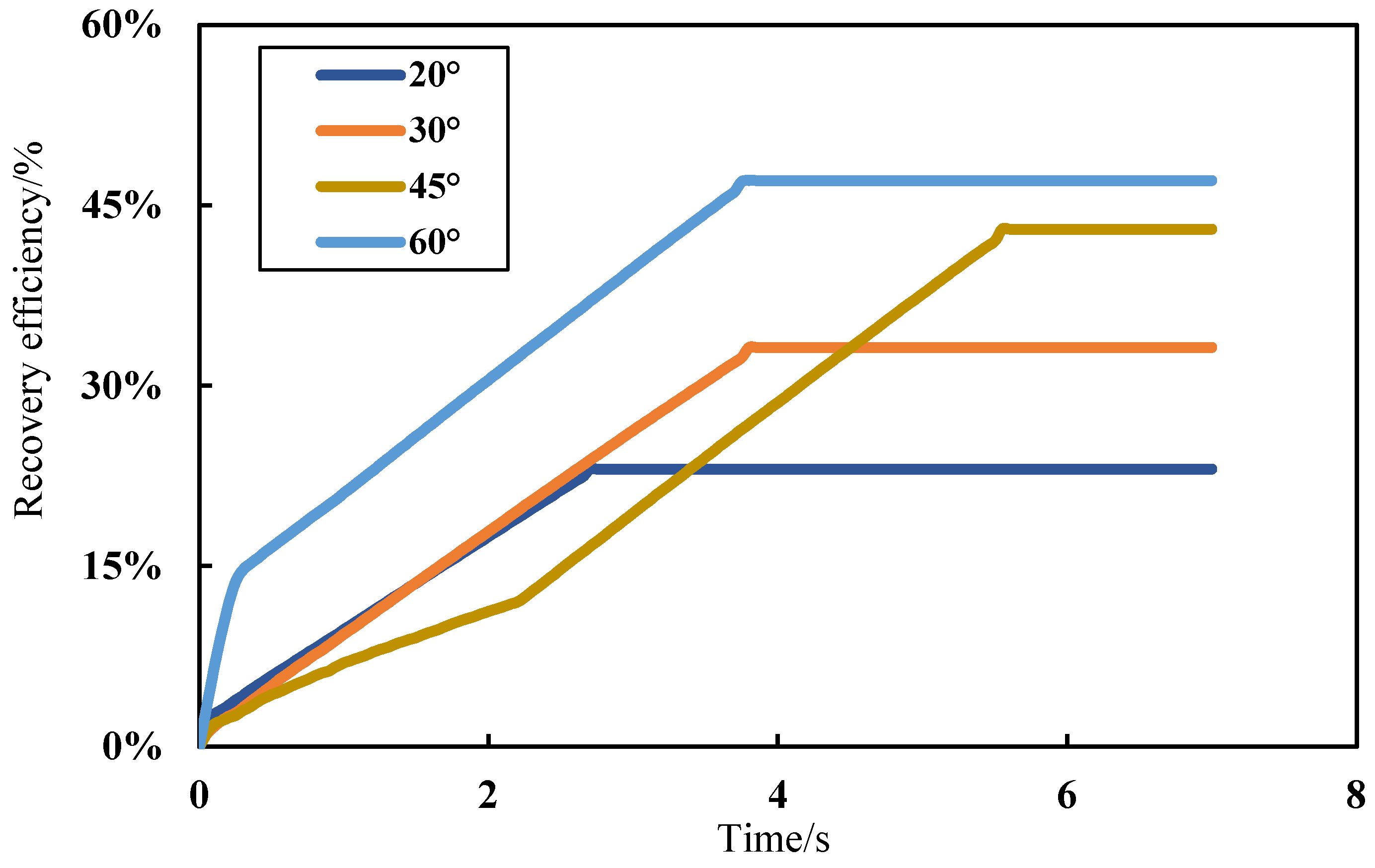
3.4.1. Dip Angle
3.4.2. Oil/Water Viscosity Ratio
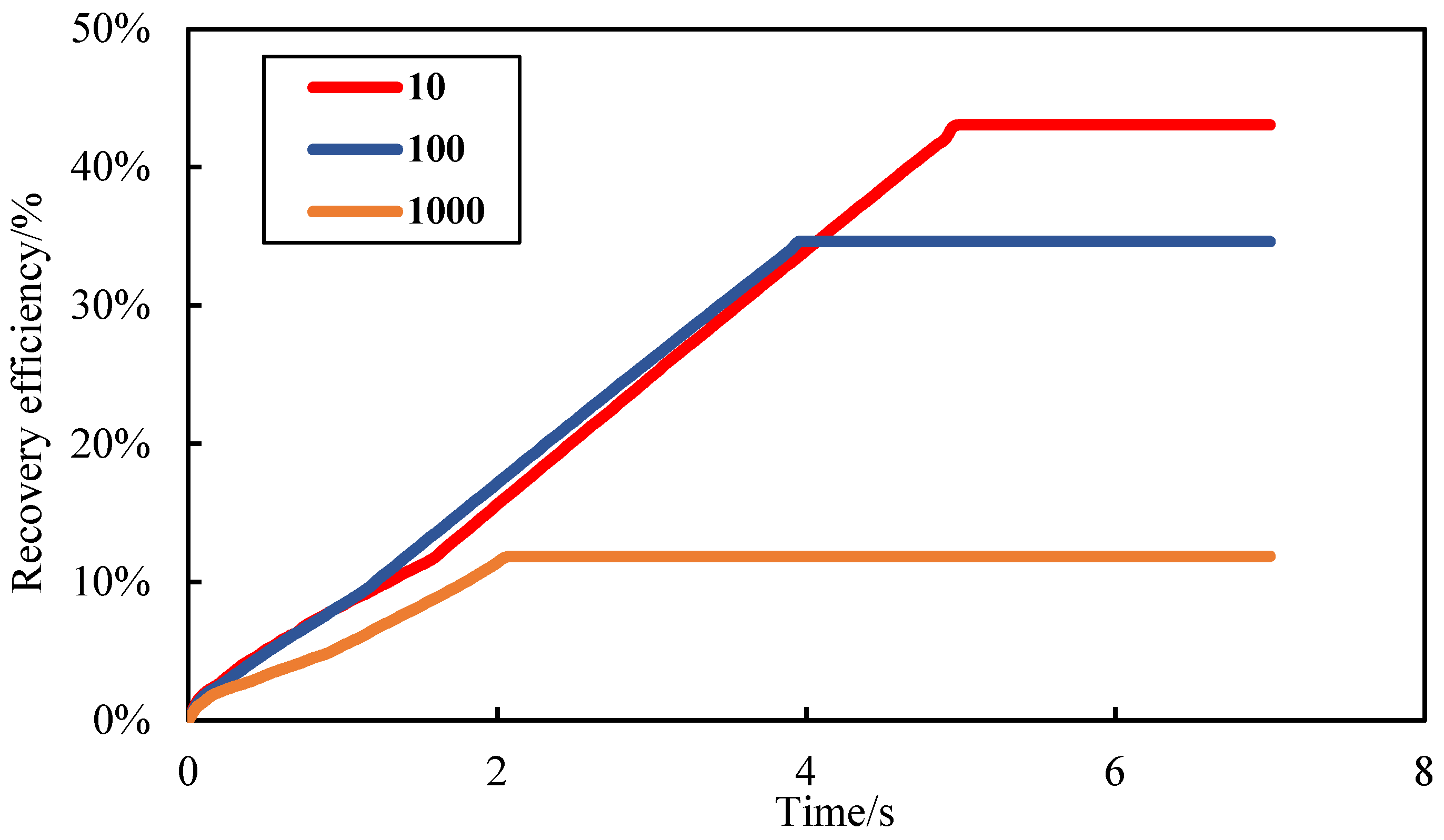
3.4.3. Gas Injection Pressure Gradient
3.4.4. Particle Diameter
4. Conclusions
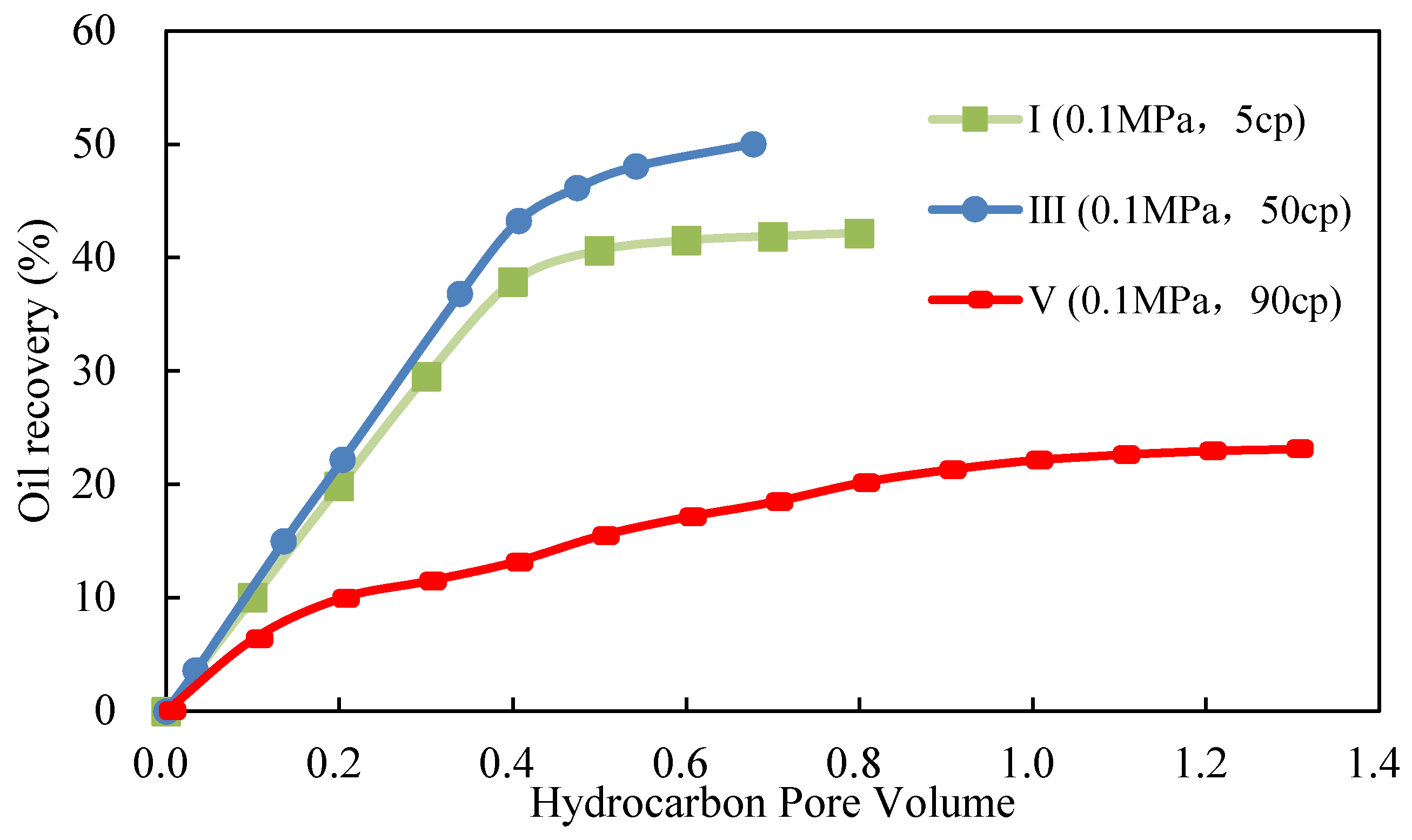
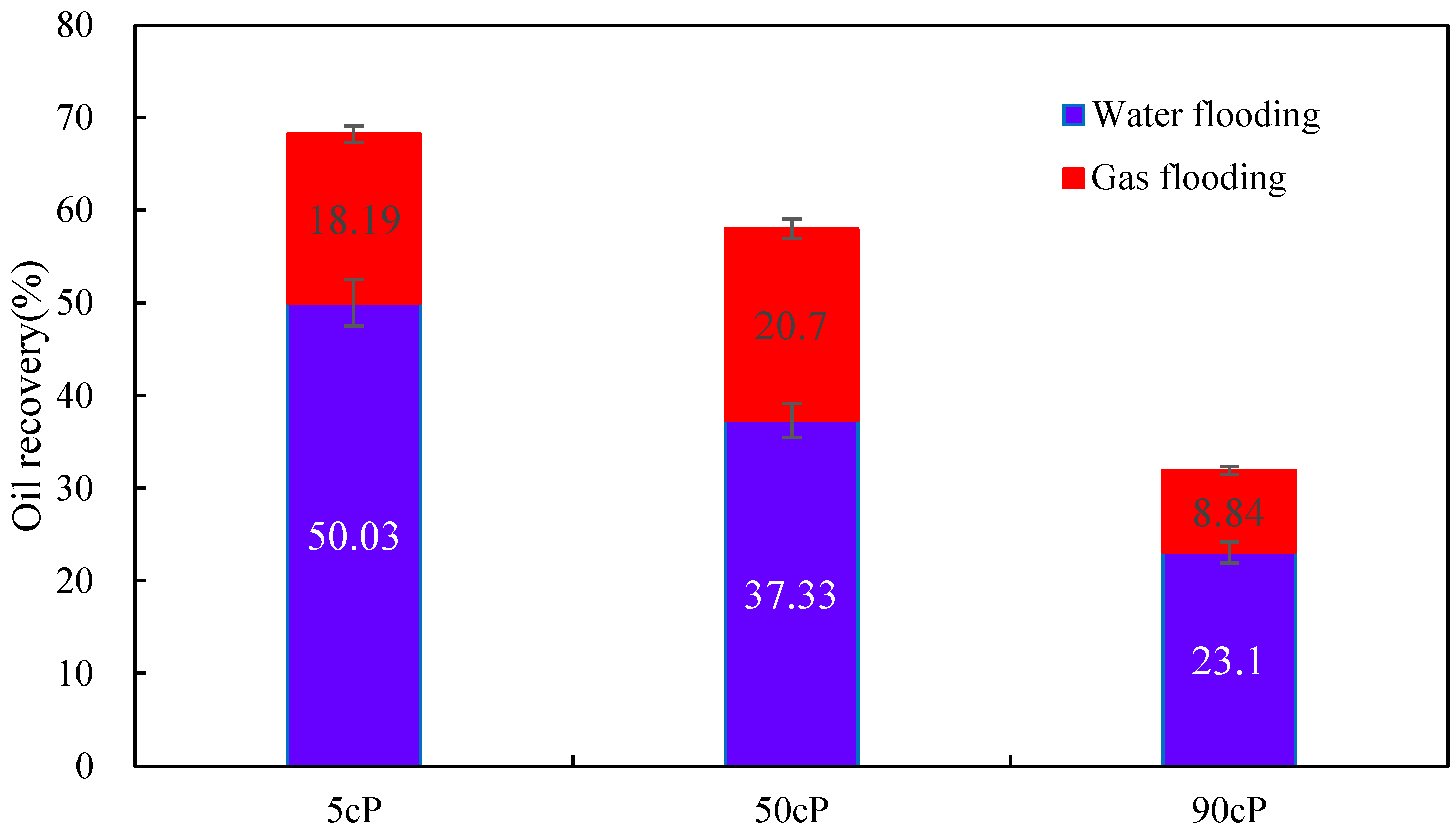
- The viscosity of crude oil has a great influence on the degree of recovery. Under the condition of strong edge water, top gas injection can effectively inhibit the edge water energy and achieve the effect of bidirectional flooding. Meanwhile, increasing the viscosity of crude oil will make the stability of the fluid interface worse. When crude oil viscosity increased from 5 cP to 90 cP, the oil recovery decreased by 36.28%.
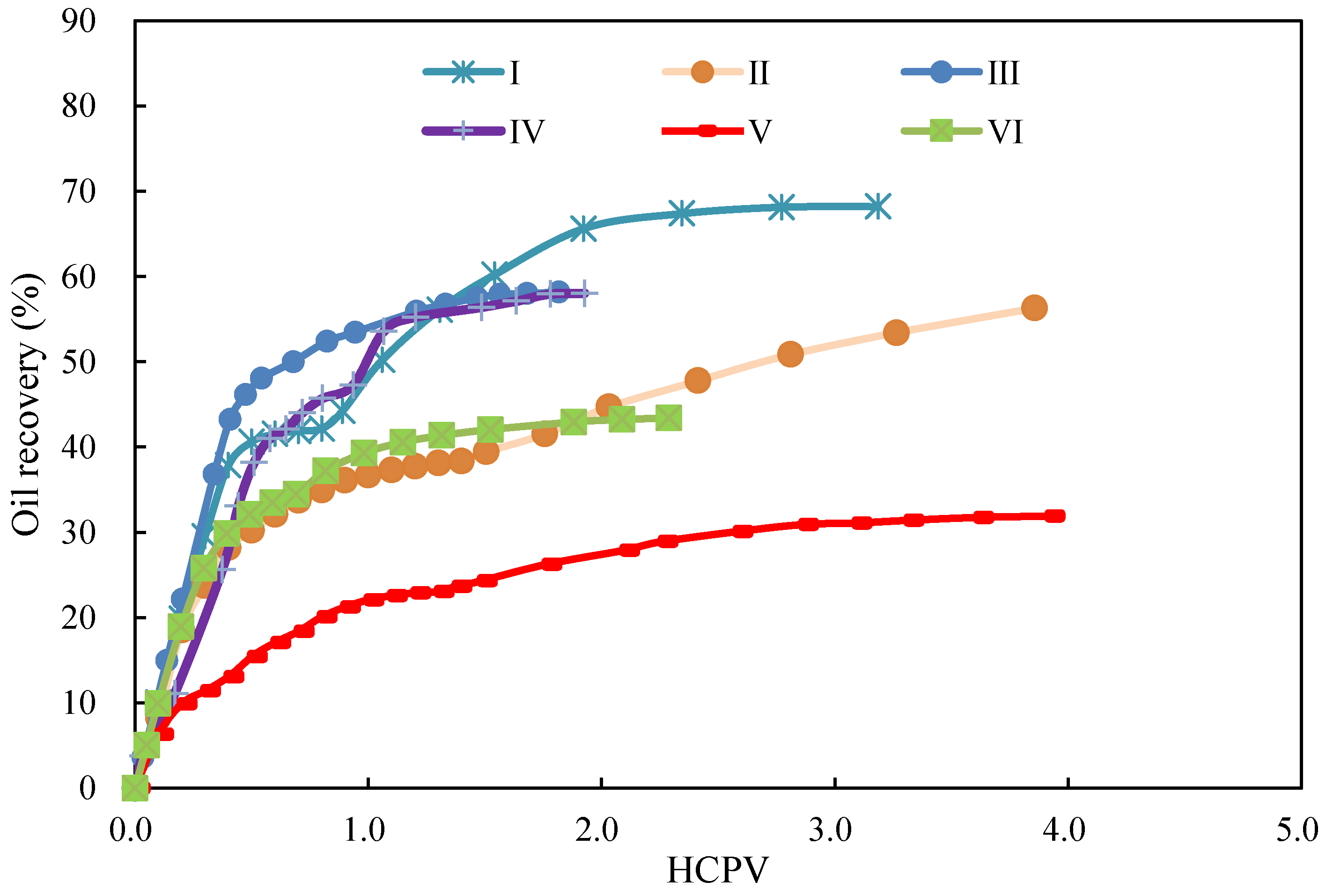
- Gas drive speed has little influence on the gas–water drive process at low viscosity, but with the increase of viscosity, the influence of gas drive speed on the recovery degree becomes more obvious: gas and water break earlier, and the corresponding oil phase recovery degree is lower.
- The finite element simulation reveals the key mechanisms affecting the stability of the oil–gas–water interface: the increase of the formation dip promotes the formation of a stable gas cap at the high part of the structure by strengthening the gravitational differentiation, inhibits the viscous finger-in caused by the difference in oil-temperament, and drives the attic oil to accumulate in the direction of the production well. Comprehensive optimization of the inclination angle, viscosity ratio and gas injection speed can achieve the synergistic improvement of the stability of the gas drive front and the recovery efficiency.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PV | Pore volume |
| N2 | Nitrogen |
| CP | Centipoise viscosity |
References
- Meng, Q.; Xu, S.; Cai, J. Microscopic studies of immiscible displacement behavior in interconnected fractures and cavities. ASME J. Energy Resour. Technol. 2019, 141, 092901. [Google Scholar] [CrossRef]
- Li, M.; Wang, Q.; Yao, C.; Chen, F.; Wang, Q.; Zhang, J. Optimization of development strategies and injection-production parameters in a fractured-vuggy carbonate reservoir by considering the effect of karst patterns: Taking c oilfield in the tarim basin as an example. Energies 2025, 18, 319. [Google Scholar] [CrossRef]
- Chen, L.; Jiang, Z.; Sun, C.; Ma, B.; Su, Z.; Wan, X.; Han, J.; Wu, G. An overview of the differential carbonate reservoir characteristic and exploitation challenge in the Tarim Basin (NW China). Energies 2023, 16, 5586. [Google Scholar] [CrossRef]
- Ma, X. Research on Well Failure Management of Water Injection Replacement Wells in Seam-Hole Carbonate Reservoirs. Master’s Thesis, Southwest Petroleum University, Chengdu, China, 2015. [Google Scholar]
- Fang, B.; Jiao, Y.; Zhang, Q.; Tian, Y.; Li, B.; Yu, W. New method for calculating rock compressibility, dynamic reserves, and aquifer size for fractured–vuggy reservoirs with bottom aquifer. Processes 2025, 13, 684. [Google Scholar] [CrossRef]
- Geng, J.; Xiao, L.; Yue, P.; Xiao, Y.; Yang, H.; Qu, S.; Wang, X. Evaluation method of water injection development effects in fractured vuggy carbonate reservoirs: Case study of fi7 strike-slip fault zone. Energies 2024, 17, 5611. [Google Scholar] [CrossRef]
- Cruz, H.J.; Islas, J.R.; Perez, R.C. Oil displacement by water in vuggy fractured porous media. In Proceedings of the SPE Latin American and Caribbean Petroleum Engineering Conference, Buenos Aires, Argentina, 1–3 April 2001. [Google Scholar]
- Kang, Z.H. Simulation and experimental study on the mechanism of water-driven oil in slit-hole carbonate reservoirs. Oil. Gas. Geol. 2006, 2, 87–90. [Google Scholar]
- Wang, L.; Do, Z.; Lin, T. Physical simulation study on visualization of water injection and oil drive in seam-hole reservoirs. J. Southwest Pet. Univ. Sci. Technol. Ed. 2011, 121, 15–16. [Google Scholar]
- Zhang, D.; Yao, J.; Gao, C.H. Physical simulation of water injection development in a seam-hole unit. J. China Univ. Pet. Ed. Nat. Sci. 2012, 36, 130–135. [Google Scholar]
- Wang, J.; Qi, X.; Liu, X.Q. Mechanism of residual oil formation in water-driven slit-hole type reservoirs and mechanism of oil enhancement by switching water injection. Pet. Explor. Dev. 2022, 49, 965–976. [Google Scholar]
- Yang, X.W.; Wang, R.J.; Deng, X.L.; Yao, C. Theoretical exploration of water injection and gravity oil drive in ultra-deep fracture-controlled seam-hole carbonate reservoirs. Pet. Explor. Dev. 2022, 49, 116–124. [Google Scholar] [CrossRef]
- Qian, Z.; Mao, Z. Experiment on water plugging by rubber particles regulating dissection in a single set of seam-hole type reservoir between wells. Lithol. Reserv. 2023, 35, 161–168. [Google Scholar]
- He, J.; Li, A.; Wu, S.; Tang, R.; Lv, D.; Li, Y.; Li, X. Experimental investigation on injection and production pattern in fractured-vuggy carbonate reservoirs. Energies 2020, 13, 603. [Google Scholar] [CrossRef]
- Chen, H.L.; Lucas, L.R. Laboratory monitoring of surfactant imbibition using computerized tomography. In Proceedings of the SPE International Petroleum Conference and Exhibition, International Petroleum Conference and Exhibition, Villahermosa, Mexico, 17–19 February 2020. [Google Scholar]
- Gillespie, J.M.; Stephens, M.J.; Chang, W. Mapping aquifer salinity gradients and effects of oil field produced water disposal using geophysical logs: Elk Hills, Buena Vista and Coles Levee Oil Fields, San Joaquin Valley, California. PLoS ONE 2022, 17, e0263477. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.H.; Wang, X. Feasibility study and parameter optimization of co2 injection technology in heavy oil field. In Proceedings of the 16th International Conference on Applied Energy (ICAE 2024), Singapore, 1–5 January 2025. [Google Scholar]
- Brown, G.; Carvalho, V.; Wray, A.; Sanchez, A.; Gutierrez, G. Lickline with fiber-optic distributed temperature monitoring for water-injection and gas lift systems optimization in Mexico. In Proceedings of the 9th Latin American and Caribbean Petroleum Engineering Conference, Rio de Janeiro, Brazil, 10–13 April 2005. [Google Scholar]
- Guo, P.; Yuan, H.; Li, X.H. Micro-visualization modeling of the gas drive mechanism in carbonate reservoirs. J. China Univ. Pet. Ed. Nat. Sci. 2012, 36, 89–93. [Google Scholar]
- Hui, J.; Liu, X.L.; Wang, Y. Study on the mechanism of gas injection and oil replacement in Tahe oilfield seam-hole type reservoir. Oil Drill. Prod. Technol. 2013, 36, 55–57. [Google Scholar]
- Qin, S.Y. Study on the Mechanism of N2 Replacement Oil Injection for Enhanced Recovery in Tahe Oilfield Seam Hole Reservoir. Master’s Thesis, Southwest Petroleum University, Chengdu, China, 2014. [Google Scholar]
- Hou, J.; Zhang, L.; Li, H. Influencing factors of nitrogen drive for enhanced recovery in carbonate slit-hole type reservoirs. Oil. Gas. Geol. 2015, 22, 64–68. [Google Scholar]
- Yang, J.B.; Hou, J.R. Experiment of nitrogen injection for enhanced recovery in karst reservoirs of slit-hole carbonate reservoirs. Oil. Gas. Geol. 2019, 26, 107–114. [Google Scholar]
- Chen, F.F.; Peng, D.B.; Wang, N.; Wang, Z.Z.; Zeng, Q.X. Water Injection and Gas Injection to Enhance Oil Recovery in Yingmai 2 Fracture-Vuggy Reservoir. J. Southwest Pet. Univ. 2024, 46, 149–158. [Google Scholar]
- Fu, Q.L.; Qun, Y.W. Establishment of stability evaluation criteria for the oil–oil-gas interface during gas-cap gravity flooding in oil reservoirs with strong edge-water drive. ACS Omega 2023, 8, 14915–14925. [Google Scholar]
- Su, Z.; Lin, J.; Bai, M.X.; Wu, D.S.; Liu, L.; Zhu, J.H. Inter-well connectivity analysis in carbonate fracture-vuggy reservoir in natural energy development stage. J. Shenzhen Univ. Sci. Eng. 2020, 37, 645–652. [Google Scholar] [CrossRef]
- Wang, Y.Z.; Cao, R.Y.; Jia, P. Prediction method of oil-water interface between injection and extraction wells in fracture-controlled seam-hole type reservoir. J. Shenzhen Univ. Sci. Eng. 2024, 41, 152–162. [Google Scholar]
- Yang, T.; Zhong, H.; Xia, H.; Zhao, X. Mechanism of residual oil mobilization after water flooding based on microscopic flow characteristics. Reserv. Eval. Dev. 2020, 10, 46–52. [Google Scholar]
- Zhu, G.; Yao, J.; Zhang, L.; Sun, H.; Li, A.; Zhang, K. Pore-scale investigation of residual oil distributions and formation mechanisms at the extra-high water-cut stage. Chin. Sci. Bull. 2017, 62, 2553–2563. [Google Scholar]











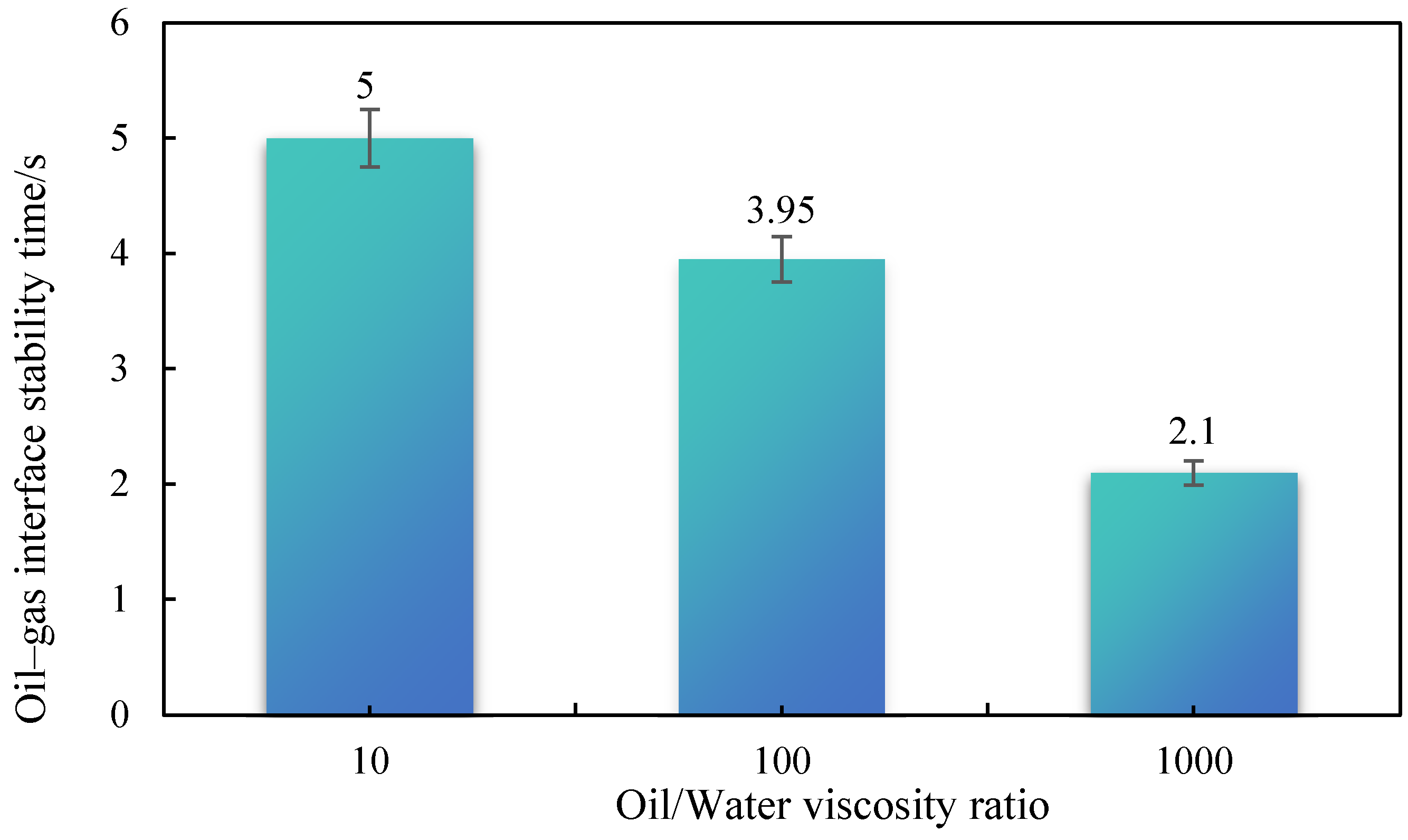

| No. | Diameter (mm) | Stratigraphic Inclination (°) | Centipoise Viscosity (cP) | Gas Drive Speed (MPa) |
|---|---|---|---|---|
| I | 2 | 30 | 5 | 0.1 |
| II | 2 | 30 | 5 | 0.25 |
| III | 2 | 30 | 50 | 0.1 |
| IV | 2 | 30 | 50 | 0.25 |
| V | 2 | 30 | 90 | 0.1 |
| VI | 2 | 30 | 90 | 0.25 |
| Two-Dimensional Model Parameter | |||
|---|---|---|---|
| Length (m) | 12.9 | Initial pressure (MPa) | 0.1 |
| Width (m) | 4.8 | Temperature (°C) | 20 |
| Particle diameter (mm) | 0.4 | Water injection pressure (Psi) | 150 |
| Model inclination (°) | 30 | N2 end pressure (MPa | 0.25 |
| Filling degree | 23.4% | Export production rate (m/s) | 5 |
| No. | Dip Angle (°) | Viscosity (c) | Gas Drive Velocity (MPa) | Recovery (%) | Interfacial Stability |
|---|---|---|---|---|---|
| I | 30 | 5 | 0.1 | 68.22 | Stable |
| II | 30 | 5 | 0.25 | 62.27 | Stable |
| III | 30 | 50 | 0.1 | 58.03 | Relatively stable |
| IV | 30 | 50 | 0.25 | 58.17 | Relatively stable |
| V | 30 | 90 | 0.1 | 31.94 | unstable |
| VI | 30 | 90 | 0.25 | 43.46 | unstable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, Y.; Liu, X.; Yang, Z.; Sun, Y.; Chen, C.; Sun, L. Study on the Stabilization Mechanism of Gas Injection Interface in Fractured-Vuggy Reservoirs. Energies 2025, 18, 1996. https://doi.org/10.3390/en18081996
Pan Y, Liu X, Yang Z, Sun Y, Chen C, Sun L. Study on the Stabilization Mechanism of Gas Injection Interface in Fractured-Vuggy Reservoirs. Energies. 2025; 18(8):1996. https://doi.org/10.3390/en18081996
Chicago/Turabian StylePan, Yi, Xinyu Liu, Zhicheng Yang, Yang Sun, Chong Chen, and Lei Sun. 2025. "Study on the Stabilization Mechanism of Gas Injection Interface in Fractured-Vuggy Reservoirs" Energies 18, no. 8: 1996. https://doi.org/10.3390/en18081996
APA StylePan, Y., Liu, X., Yang, Z., Sun, Y., Chen, C., & Sun, L. (2025). Study on the Stabilization Mechanism of Gas Injection Interface in Fractured-Vuggy Reservoirs. Energies, 18(8), 1996. https://doi.org/10.3390/en18081996





