Figure 1.
(a): Geometric model of the composite laminated shallow shell with elastic restraint; (b): geometric model of the composite laminated shallow shell with various curvature types.
Figure 1.
(a): Geometric model of the composite laminated shallow shell with elastic restraint; (b): geometric model of the composite laminated shallow shell with various curvature types.
Figure 2.
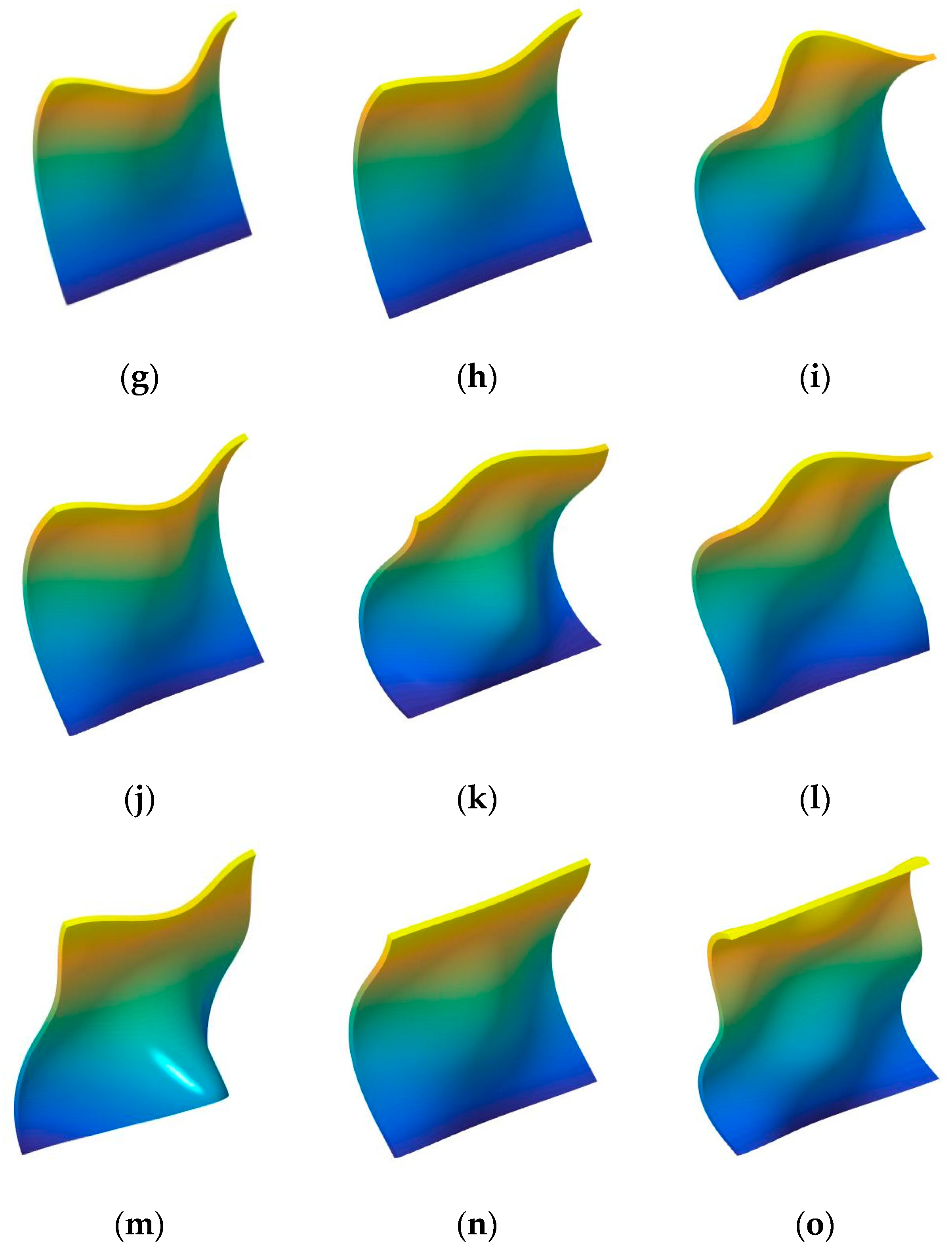
The mode shapes for the composite laminated shallow cylindrical shell with various boundary conditions. (a) C-C,(1,1); (b) C-C,(1,2); (c) C-C,(1,3); (d) F-C,(1,1); (e) F-C,(1,2); (f) F-C,(1,3); (g) F-F,(1,1); (h) F-F,(1,2); (i) F-F,(1,3); (j) F-S,(1,1); (k) F-S,(1,2); (l) F-S,(1,3); (m) S-C,(1,1); (n) S-C,(1,2); (o) S-C,(1,3).
Figure 2.
The mode shapes for the composite laminated shallow cylindrical shell with various boundary conditions. (a) C-C,(1,1); (b) C-C,(1,2); (c) C-C,(1,3); (d) F-C,(1,1); (e) F-C,(1,2); (f) F-C,(1,3); (g) F-F,(1,1); (h) F-F,(1,2); (i) F-F,(1,3); (j) F-S,(1,1); (k) F-S,(1,2); (l) F-S,(1,3); (m) S-C,(1,1); (n) S-C,(1,2); (o) S-C,(1,3).
Figure 3.
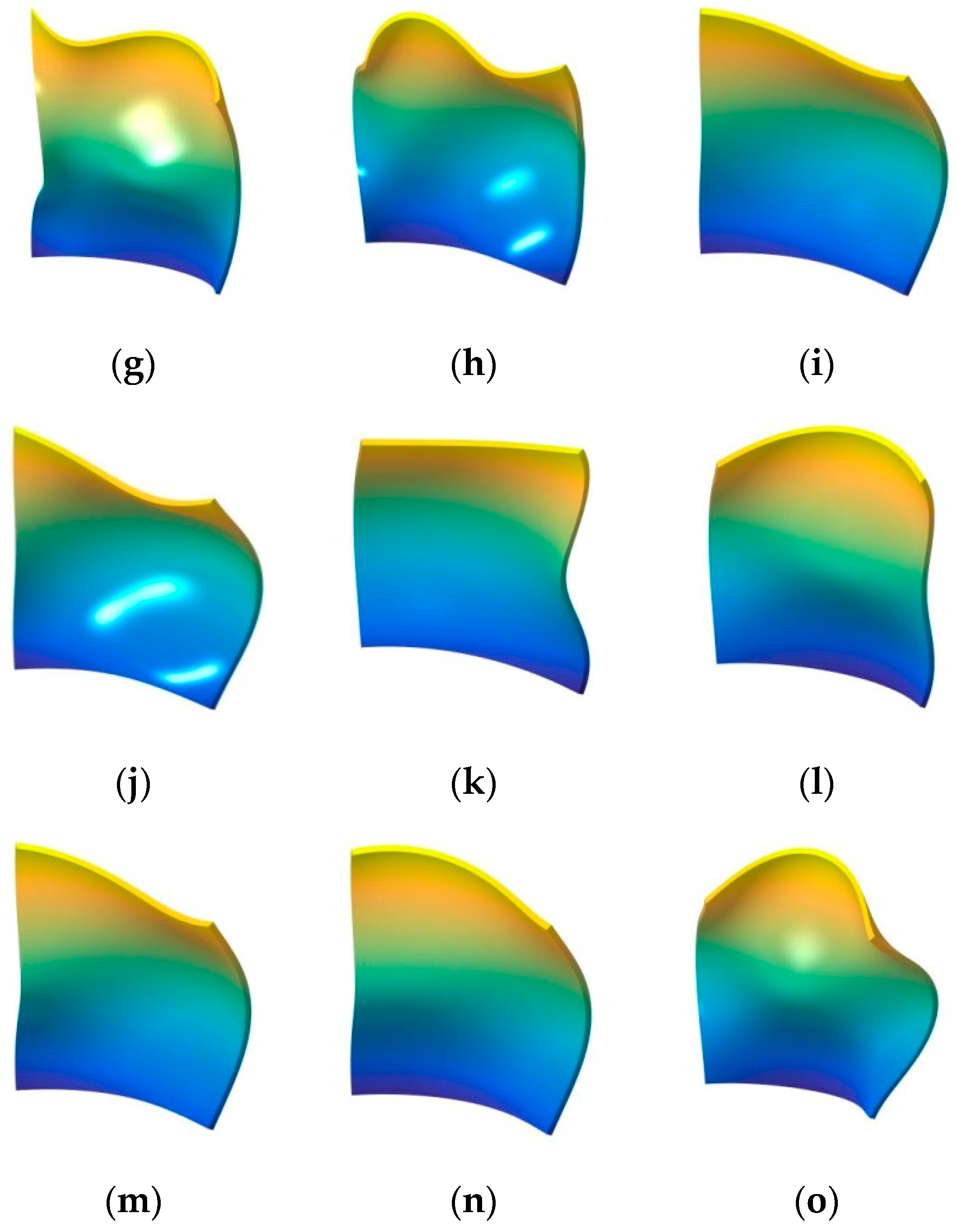
The mode shapes for the composite laminated shallow spherical shell with various boundary conditions. (a) C-C,(1,1); (b) C-C,(1,2); (c) C-C,(1,3); (d) F-C,(1,1); (e) F-C,(1,2); (f) F-C,(1,3); (g) F-F,(1,1); (h) F-F,(1,2); (i) F-F,(1,3); (j) F-S,(1,1); (k) F-S,(1,2); (l) F-S,(1,3); (m) S-C,(1,1); (n) S-C,(1,2); (o) S-C,(1,3).
Figure 3.
The mode shapes for the composite laminated shallow spherical shell with various boundary conditions. (a) C-C,(1,1); (b) C-C,(1,2); (c) C-C,(1,3); (d) F-C,(1,1); (e) F-C,(1,2); (f) F-C,(1,3); (g) F-F,(1,1); (h) F-F,(1,2); (i) F-F,(1,3); (j) F-S,(1,1); (k) F-S,(1,2); (l) F-S,(1,3); (m) S-C,(1,1); (n) S-C,(1,2); (o) S-C,(1,3).
Figure 4.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow shells with the SD-SD boundary condition with respect to various length to radius ratios Lx/Rx and length to thickness ratios Lx/h by first-order shear deformation shell theory (FSDT). (a) Cylindrical shell, 0°/90°/90°/0°; (b) cylindrical shell, 0°/90°; (c) cylindrical shell, 90°/0°; (d) spherical shell, 0°/90°/90°/0°; (e) spherical shell, 0°/90°; (f) spherical shell, 90°/0°; (g) hyperbolic paraboloidal, 0°/90°/90°/0°; (h) hyperbolic paraboloidal, 0°/90°; (i) hyperbolic paraboloidal, 90°/0°.
Figure 4.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow shells with the SD-SD boundary condition with respect to various length to radius ratios Lx/Rx and length to thickness ratios Lx/h by first-order shear deformation shell theory (FSDT). (a) Cylindrical shell, 0°/90°/90°/0°; (b) cylindrical shell, 0°/90°; (c) cylindrical shell, 90°/0°; (d) spherical shell, 0°/90°/90°/0°; (e) spherical shell, 0°/90°; (f) spherical shell, 90°/0°; (g) hyperbolic paraboloidal, 0°/90°/90°/0°; (h) hyperbolic paraboloidal, 0°/90°; (i) hyperbolic paraboloidal, 90°/0°.
Figure 5.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow shells with the SD-SD boundary conditions with respect to various length to radius ratios Lx/Rx and length to thickness ratios Lx/h by classical shell theory (CST). (a) Cylindrical shell, 0°/90°/90°/0°; (b) cylindrical shell, 0°/90°; (c) cylindrical shell, 90°/0°; (d) spherical shell, 0°/90°/90°/0°; (e) spherical shell, 0°/90°; (f) spherical shell, 90°/0°; (g) hyperbolic paraboloidal, 0°/90°/90°/0°; (h) hyperbolic paraboloidal, 0°/90°; (i) hyperbolic paraboloidal, 90°/0°.
Figure 5.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow shells with the SD-SD boundary conditions with respect to various length to radius ratios Lx/Rx and length to thickness ratios Lx/h by classical shell theory (CST). (a) Cylindrical shell, 0°/90°/90°/0°; (b) cylindrical shell, 0°/90°; (c) cylindrical shell, 90°/0°; (d) spherical shell, 0°/90°/90°/0°; (e) spherical shell, 0°/90°; (f) spherical shell, 90°/0°; (g) hyperbolic paraboloidal, 0°/90°/90°/0°; (h) hyperbolic paraboloidal, 0°/90°; (i) hyperbolic paraboloidal, 90°/0°.
Figure 6.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow spherical shells with various boundary conditions with respect to diverse length to radius ratios Lx/Rx and modulus ratios E1/E2 by FSDT and CST. (a) FSDT: SD-SD; (b) FSDT: C-C; (c) CST: SD-SD; (d) CST: C-C.
Figure 6.
Variation laws of the fundamental frequency parameter Ω for composite laminated shallow spherical shells with various boundary conditions with respect to diverse length to radius ratios Lx/Rx and modulus ratios E1/E2 by FSDT and CST. (a) FSDT: SD-SD; (b) FSDT: C-C; (c) CST: SD-SD; (d) CST: C-C.
Figure 7.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Ku by FSDT. (a) m = 1; (b) m = 2.
Figure 7.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Ku by FSDT. (a) m = 1; (b) m = 2.
Figure 8.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kv by FSDT. (a) m = 1; (b) m = 2.
Figure 8.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kv by FSDT. (a) m = 1; (b) m = 2.
Figure 9.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kw by FSDT. (a) m = 1; (b) m = 2.
Figure 9.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kw by FSDT. (a) m = 1; (b) m = 2.
Figure 10.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kϕx by FSDT. (a) m = 1; (b) m = 2.
Figure 10.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kϕx by FSDT. (a) m = 1; (b) m = 2.
Figure 11.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kϕy by FSDT. (a) m = 1; (b) m = 2.
Figure 11.
Variation laws of the frequency parameter Ω for composite shallow [0°/90°] shells with various stiffness constant Kϕy by FSDT. (a) m = 1; (b) m = 2.
Table 1.
The fundamental frequency parameter Ω for the composite shallow shell with the SD-SD boundary condition by first-order shear deformation shell theory (FSDT). WBM, wave-based method.
Table 1.
The fundamental frequency parameter Ω for the composite shallow shell with the SD-SD boundary condition by first-order shear deformation shell theory (FSDT). WBM, wave-based method.
| Rx/Ry | Ry/Ly | 0°/90°/90°/0° |
|---|
| WBM | Ref. [13] | Error | Ref. [22] | Error |
|---|
| 1 | 2 | 12.3093 | 12.5718 | −2.09% | 12.3633 | −0.44% |
| 5 | 11.1495 | 11.2522 | −0.91% | 11.2135 | −0.57% |
| 10 | 10.9672 | 11.0428 | −0.69% | 11.0329 | −0.60% |
| 0 | 2 | 11.2142 | 11.3342 | −1.06% | 11.2756 | −0.54% |
| 5 | 10.9562 | 11.0316 | −0.68% | 11.0217 | −0.59% |
| 10 | 10.9562 | 10.9867 | −0.28% | 10.9842 | −0.25% |
| −1 | 2 | 10.3671 | 10.7031 | −3.14% | 10.4300 | −0.60% |
| 5 | 10.8169 | 10.9273 | −1.01% | 10.8826 | −0.60% |
| 10 | 10.8831 | 10.9605 | −0.71% | 10.9493 | −0.60% |
| Rx/Ry | Ry/Ly | 0°/90° |
| 1 | 2 | 10.0265 | 10.2492 | −2.17% | 10.0998 | −0.73% |
| 5 | 8.3845 | 8.5084 | −1.46% | 8.4783 | −1.11% |
| 10 | 8.1132 | 8.2190 | −1.29% | 8.2111 | −1.19% |
| 0 | 2 | 8.6166 | 8.7523 | −1.55% | 8.7075 | −1.04% |
| 5 | 8.1475 | 8.2445 | −1.18% | 8.2458 | −1.19% |
| 10 | 8.0644 | 8.1592 | −1.16% | 8.1636 | −1.22% |
| −1 | 2 | 7.8626 | 8.0831 | −2.73% | 7.9596 | −1.22% |
| 5 | 8.0549 | 8.1538 | −1.21% | 8.1546 | −1.22% |
| 10 | 8.0537 | 8.1448 | −1.12% | 8.1534 | −1.22% |
| Rx/Ry | Ry/Ly | 90°/0° |
| 1 | 2 | 10.0261 | 10.2492 | −2.18% | 10.0998 | −0.73% |
| 5 | 8.3843 | 8.5084 | −1.46% | 8.4783 | −1.11% |
| 10 | 8.1131 | 8.2190 | −1.29% | 8.2111 | −1.19% |
| 0 | 2 | 8.3533 | 8.5784 | −2.62% | 8.4421 | −1.05% |
| 5 | 8.1474 | 8.1774 | −0.37% | 8.1448 | 0.03% |
| 10 | 8.0644 | 8.1259 | −0.76% | 8.1136 | −0.61% |
| −1 | 2 | 7.4152 | 7.7739 | −4.61% | 7.5071 | −1.22% |
| 5 | 7.8606 | 8.0223 | −2.02% | 7.9581 | −1.22% |
| 10 | 7.9554 | 8.0785 | −1.52% | 8.0540 | −1.22% |
Table 2.
The fundamental frequency parameter Ω for composite shallow shell with the SD-SD boundary condition by classical shell theory (CST) theory.
Table 2.
The fundamental frequency parameter Ω for composite shallow shell with the SD-SD boundary condition by classical shell theory (CST) theory.
| Rx/Ry | Ry/Ly | 0°/90°/90°/0° |
|---|
| WBM | Ref. [13] | Error | Ref. [22] | Error |
|---|
| 1 | 2 | 66.52832 | 66.5774 | −0.07% | 66.5285 | 0.00% |
| 5 | 29.29062 | 29.309 | −0.06% | 29.2906 | 0.00% |
| 10 | 18.12154 | 18.129 | −0.04% | 18.1215 | 0.00% |
| 0 | 2 | 35.10566 | 35.1838 | −0.22% | 35.1622 | −0.16% |
| 5 | 18.08579 | 18.1107 | −0.14% | 18.1038 | −0.10% |
| 10 | 13.96209 | 13.9703 | −0.06% | 13.9681 | −0.04% |
| −1 | 2 | 11.67421 | 11.9776 | −2.60% | 11.6742 | 0.00% |
| 5 | 12.1784 | 12.2279 | −0.41% | 12.1783 | 0.00% |
| 10 | 12.25251 | 12.2649 | −0.10% | 12.2525 | 0.00% |
| Rx/Ry | Ry/Ly | 0°/90° |
| 1 | 2 | 65.98726 | 66.0139 | −0.04% | 65.98717 | 0.00% |
| 5 | 27.95602 | 27.9666 | −0.04% | 27.95599 | 0.00% |
| 10 | 15.85265 | 15.8573 | −0.03% | 15.85258 | 0.00% |
| 0 | 2 | 28.16678 | 28.2471 | −0.28% | 28.16667 | 0.00% |
| 5 | 15.84446 | 15.8484 | −0.02% | 15.81926 | 0.16% |
| 10 | 10.85982 | 10.8616 | −0.02% | 10.85171 | 0.07% |
| −1 | 2 | 8.121357 | 8.37737 | −3.06% | 8.17396 | −0.64% |
| 5 | 8.487776 | 8.54161 | −0.63% | 8.51081 | −0.27% |
| 10 | 8.545494 | 8.56847 | −0.27% | 8.55691 | −0.13% |
| Rx/Ry | Ry/Ly | 90°/0° |
| 1 | 2 | 65.98726 | 66.0139 | −0.04% | 66.0139 | −0.04% |
| 5 | 27.95602 | 27.9666 | −0.04% | 27.9666 | −0.04% |
| 10 | 15.85265 | 15.8573 | −0.03% | 15.8573 | −0.03% |
| 0 | 2 | 27.692 | 27.827 | −0.49% | 27.69195 | 0.00% |
| 5 | 15.81913 | 15.8342 | −0.10% | 15.84456 | −0.16% |
| 10 | 10.85163 | 10.8567 | −0.05% | 10.85977 | −0.08% |
| −1 | 2 | 8.173862 | 8.34143 | −2.05% | 8.12143 | 0.64% |
| 5 | 8.510863 | 8.52632 | −0.18% | 8.48796 | 0.27% |
| 10 | 8.557038 | 8.55594 | 0.01% | 8.54535 | 0.14% |
Table 3.
The fundamental frequency parameter Ω for a composite plate with the SD-SD boundary condition with variety theory.
Table 3.
The fundamental frequency parameter Ω for a composite plate with the SD-SD boundary condition with variety theory.
| Lamination Theory |
|---|
| Ly/h | 0°/90° | | 0°/90°/90°/0° |
|---|
| FSDT | CST | FSDT | CST |
|---|
| Ref. [13] | WBM | Ref. [13] | WBM | Ref. [13] | WBM | Ref. [13] | WBM |
|---|
| 100 | 8.56394 | 8.55196 | 8.56847 | 8.56858 | 12.26147 | 12.26147 | 12.37733 | 12.27746 |
| 20 | 8.44807 | 8.44807 | 8.55811 | 8.55808 | 11.90100 | 11.90100 | 12.27733 | 12.27731 |
| 10 | 8.11956 | 8.11956 | 8.52569 | 8.52570 | 10.97163 | 10.97163 | 12.27733 | 12.27733 |
| 5 | 7.14661 | 7.14661 | 8.39526 | 8.39527 | 8.77840 | 8.77841 | 12.27733 | 12.27734 |
Table 4.
The fundamental frequency parameter Ω for a composite plate with the SD-SD boundary condition by variety theory.
Table 4.
The fundamental frequency parameter Ω for a composite plate with the SD-SD boundary condition by variety theory.
| Lamination Theory |
|---|
| Ly//h | 0°/90° |
|---|
| FSDT | CST |
|---|
| Ref. [13] | WBM | Ref. [13] | WBM |
|---|
| 100 | 9.6873 | 9.6873 | 9.696 | 9.6961 |
| 10 | 8.9001 | 8.9001 | 9.6436 | 9.6436 |
| Ly//h | 0°/90°/0° |
| 100 | 15.183 | 15.1834 | 15.228 | 15.2278 |
| 10 | 12.163 | 12.1629 | 15.228 | 15.2278 |
| Ly//h | 0°/90°/90°/0° |
| 100 | 15.184 | 15.1839 | 15.228 | 15.2278 |
| 10 | 12.226 | 12.2272 | 15.228 | 15.2278 |
Table 5.
The fundamental frequency parameter Ω for two types of the layered composite shallow cylindrical shell with various boundary conditions, theories, and radii.
Table 5.
The fundamental frequency parameter Ω for two types of the layered composite shallow cylindrical shell with various boundary conditions, theories, and radii.
| Lamination Schemes | Theory | R | | Boundary Conditions |
|---|
| | F-F | F-S | F-C | S-S | S-C | C-C |
|---|
| 0°/90° | CST | 20 | Ref. [13] | 6.128 | 6.489 | 7.008 | 9.56 | 12.136 | 15.757 |
| WBM | 6.147 | 6.376 | 7.257 | 9.633 | 12.236 | 15.895 |
| Error | 0.31% | −1.74% | 3.55% | 0.77% | 0.82% | 0.88% |
| 5 | Ref. [13] | 6.096 | 6.444 | 7.014 | 9.598 | 12.154 | 15.747 |
| WBM | 6.070 | 6.433 | 7.231 | 9.630 | 12.225 | 15.861 |
| Error | −0.42% | −0.16% | 3.10% | 0.34% | 0.58% | 0.72% |
| FSDT | 20 | Ref. [13] | 5.763 | 6.087 | 6.535 | 8.894 | 10.609 | 12.623 |
| WBM | 5.778 | 6.007 | 6.532 | 8.803 | 10.555 | 12.621 |
| Error | 0.26% | −1.32% | −0.05% | −1.03% | −0.50% | −0.01% |
| 5 | Ref. [13] | 5.716 | 6.030 | 6.524 | 8.931 | 10.647 | 12.663 |
| WBM | 5.850 | 5.994 | 6.502 | 8.826 | 10.582 | 12.651 |
| Error | 2.34% | −0.59% | −0.34% | −1.17% | −0.61% | −0.10% |
| 0°/90°/0° | CST | 20 | Ref. [13] | 3.902 | 4.484 | 6.866 | 15.106 | 22.557 | 32.091 |
| WBM | 3.966 | 4.501 | 6.891 | 15.229 | 22.214 | 32.385 |
| Error | 1.63% | 0.39% | 0.36% | 0.82% | −1.52% | 0.92% |
| 5 | Ref. [13] | 3.894 | 4.472 | 6.901 | 15.136 | 22.560 | 32.062 |
| WBM | 3.966 | 4.475 | 6.917 | 15.253 | 22.214 | 32.352 |
| Error | 1.84% | 0.07% | 0.23% | 0.77% | −1.53% | 0.90% |
| FSDT | 20 | Ref. [13] | 3.787 | 4.318 | 6.146 | 12.166 | 14.250 | 16.385 |
| WBM | 3.796 | 4.312 | 6.146 | 12.104 | 14.218 | 16.384 |
| Error | 0.23% | −0.14% | 0.00% | −0.51% | −0.22% | 0.00% |
| 5 | Ref. [13] | 3.773 | 4.301 | 6.176 | 12.212 | 14.284 | 16.408 |
| WBM | 3.910 | 4.392 | 6.170 | 12.148 | 14.250 | 16.406 |
| Error | 3.62% | 2.11% | −0.09% | −0.53% | −0.24% | −0.01% |
Table 6.
The fundamental frequency parameter Ω for two types of the layered composite shallow spherical shell with various boundary conditions, theories, and radii.
Table 6.
The fundamental frequency parameter Ω for two types of the layered composite shallow spherical shell with various boundary conditions, theories, and radii.
| Lamination Schemes | Theory | R | | Boundary Conditions |
|---|
| | F-F | F-S | F-C | S-S | S-C | C-C |
|---|
| 0°/90° | CST | 20 | Ref. [13] | 6.132 | 6.493 | 7.002 | 9.588 | 12.165 | 15.822 |
| WBM | 6.360 | 6.360 | 7.066 | 9.663 | 12.274 | 15.975 |
| Error | 3.71% | −2.05% | 0.91% | 0.78% | 0.90% | 0.97% |
| 5 | Ref. [13] | 6.162 | 6.51 | 6.971 | 9.903 | 12.465 | 16.82 |
| WBM | 6.250 | 6.482 | 6.940 | 9.945 | 12.560 | 16.990 |
| Error | 1.43% | −0.43% | −0.44% | 0.42% | 0.77% | 1.01% |
| FSDT | 20 | Ref. [13] | 5.768 | 6.093 | 6.535 | 8.922 | 10.64 | 12.713 |
| WBM | 5.764 | 6.076 | 6.532 | 8.833 | 10.590 | 12.714 |
| Error | −0.07% | −0.27% | −0.04% | −1.00% | −0.47% | 0.01% |
| 5 | Ref. [13] | 5.787 | 6.105 | 6.511 | 9.247 | 11.004 | 14.081 |
| WBM | 5.765 | 6.073 | 6.493 | 9.146 | 10.946 | 14.078 |
| Error | −0.38% | −0.53% | −0.27% | −1.09% | −0.53% | −0.02% |
| 0°/90°/0° | CST | 20 | Ref. [13] | 3.909 | 4.49 | 6.863 | 15.116 | 22.562 | 32.136 |
| WBM | 3.924 | 4.512 | 6.888 | 15.236 | 22.214 | 32.430 |
| Error | 0.38% | 0.49% | 0.36% | 0.79% | −1.54% | 0.91% |
| 5 | Ref. [13] | 4.009 | 4.562 | 6.861 | 15.29 | 22.64 | 32.785 |
| WBM | 4.010 | 4.599 | 6.909 | 15.356 | 22.215 | 33.051 |
| Error | 0.02% | 0.81% | 0.70% | 0.43% | −1.88% | 0.81% |
| FSDT | 20 | Ref. [13] | 3.794 | 4.325 | 6.146 | 12.178 | 14.264 | 16.487 |
| WBM | 3.794 | 4.319 | 6.146 | 12.114 | 14.231 | 16.486 |
| Error | 0.00% | −0.14% | −0.01% | −0.52% | −0.23% | −0.01% |
| 5 | Ref. [13] | 3.891 | 4.397 | 6.163 | 12.394 | 14.499 | 17.959 |
| WBM | 3.884 | 4.369 | 6.163 | 12.312 | 14.454 | 17.951 |
| Error | −0.18% | −0.63% | 0.00% | −0.66% | −0.31% | −0.04% |
Table 7.
The fundamental frequency parameter Ω for three types of layered composite shallow cylindrical shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
Table 7.
The fundamental frequency parameter Ω for three types of layered composite shallow cylindrical shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
| Lx/h | Lx/Rx | Lamination Schemes |
|---|
| 0°/90°/90°/0° | 0°/90° | 90°/0° |
|---|
| FSDT | CST | FSDT | CST | FSDT | CST |
|---|
| 100 | 0 | 12.2531 | 12.2775 | 8.5520 | 8.5686 | 8.5520 | 8.5686 |
| 0.1 | 13.9407 | 13.9621 | 10.8462 | 10.8598 | 10.8382 | 10.8516 |
| 0.2 | 18.0687 | 18.0858 | 15.8342 | 15.8445 | 15.8090 | 15.8192 |
| 0.5 | 35.0954 | 35.1057 | 28.1209 | 28.1668 | 27.6433 | 27.6921 |
| 20 | 0 | 11.8617 | 12.2773 | 8.3908 | 8.5581 | 8.3908 | 8.5581 |
| 0.1 | 11.9222 | 12.3350 | 8.5016 | 8.6680 | 8.4735 | 8.6386 |
| 0.2 | 12.1011 | 12.2811 | 8.8000 | 8.9626 | 8.7410 | 8.9010 |
| 0.5 | 13.2636 | 13.6185 | 10.6002 | 10.7427 | 10.4142 | 10.5501 |
| 10 | 0 | 10.9053 | 12.2773 | 8.0202 | 8.5257 | 8.0202 | 8.5257 |
| 0.1 | 10.9125 | 12.2803 | 8.0644 | 8.5724 | 8.0149 | 8.5146 |
| 0.2 | 10.9338 | 12.2894 | 8.1475 | 8.6547 | 8.0476 | 8.5383 |
| 0.5 | 11.0809 | 12.3534 | 8.6166 | 9.1042 | 8.3533 | 8.8006 |
Table 8.
The fundamental frequency parameter Ω for three types of layered composite shallow spherical shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
Table 8.
The fundamental frequency parameter Ω for three types of layered composite shallow spherical shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
| Lx/h | Lx/Rx | Lamination Schemes |
|---|
| 0°/90°/90°/0° | 0°/90° | 90°/0° |
|---|
| FSDT | CST | FSDT | CST | FSDT | CST |
|---|
| 100 | 0 | 12.2531 | 12.2775 | 8.5520 | 8.5686 | 8.5520 | 8.5686 |
| 0.1 | 18.1044 | 18.1215 | 15.8423 | 15.8526 | 15.8423 | 15.8526 |
| 0.2 | 29.2786 | 29.2906 | 27.9478 | 27.9560 | 27.9478 | 27.9560 |
| 0.5 | 66.5196 | 66.5283 | 65.9775 | 65.9873 | 65.9775 | 65.9873 |
| 20 | 0 | 11.8617 | 12.2773 | 8.3908 | 8.5581 | 8.3908 | 8.5581 |
| 0.1 | 12.1375 | 12.5436 | 8.7873 | 8.9489 | 8.7873 | 8.9489 |
| 0.2 | 12.9248 | 13.3057 | 9.8753 | 10.0240 | 9.8753 | 10.0240 |
| 0.5 | 17.3215 | 17.6007 | 15.3115 | 15.4258 | 15.3114 | 15.4258 |
| 10 | 0 | 10.9053 | 12.2773 | 8.0202 | 8.5257 | 8.0202 | 8.5257 |
| 0.1 | 10.9672 | 12.3284 | 8.1132 | 8.6132 | 8.1131 | 8.6132 |
| 0.2 | 11.1495 | 12.4794 | 8.3845 | 8.8692 | 8.3843 | 8.8692 |
| 0.5 | 12.3093 | 13.4531 | 10.0265 | 10.4333 | 10.0261 | 10.4333 |
Table 9.
The fundamental frequency parameter Ω for three types of layered composite shallow hyperbolic paraboloidal shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
Table 9.
The fundamental frequency parameter Ω for three types of layered composite shallow hyperbolic paraboloidal shells for variety theories, length to thickness ratios Lx/h, and length to radius ratios Lx/Rx with the SD-SD boundary condition.
| Lx/h | Lx/Rx | Lamination Schemes |
|---|
| 0°/90°/90°/0° | 0°/90° | 90°/0° |
|---|
| FSDT | CST | FSDT | CST | FSDT | CST |
|---|
| 100 | 0 | 12.2531 | 12.2775 | 8.5520 | 8.5686 | 8.5520 | 8.5686 |
| 0.1 | 12.2283 | 12.2525 | 8.5404 | 8.5570 | 8.5289 | 8.5454 |
| 0.2 | 12.1543 | 12.1783 | 8.4944 | 8.5109 | 8.4716 | 8.4877 |
| 0.5 | 11.6512 | 11.6742 | 8.1583 | 8.1739 | 8.1059 | 8.1213 |
| 20 | 0 | 11.8617 | 12.2773 | 8.3908 | 8.5581 | 8.3908 | 8.5581 |
| 0.1 | 11.8377 | 12.2524 | 8.4015 | 8.5698 | 8.3464 | 8.5120 |
| 0.2 | 11.7659 | 12.1778 | 8.3783 | 8.5466 | 8.2693 | 8.4325 |
| 0.5 | 11.2784 | 11.6712 | 8.1079 | 8.2715 | 7.8571 | 8.0092 |
| 10 | 0 | 10.9053 | 12.2773 | 8.0202 | 8.5257 | 8.0202 | 8.5257 |
| 0.1 | 10.8831 | 12.2519 | 8.0537 | 8.5664 | 7.9554 | 8.4514 |
| 0.2 | 10.8169 | 12.1761 | 8.0549 | 8.5724 | 7.8606 | 8.3452 |
| 0.5 | 10.3671 | 11.6619 | 7.8626 | 8.6024 | 7.4152 | 7.8559 |
Table 10.
The fundamental frequency parameter Ω for composite shallow [0°/90°] spherical shells for variety length to radius ratios Lx/Rx, modulus ratios E1/E2, and boundary conditions by FSDT.
Table 10.
The fundamental frequency parameter Ω for composite shallow [0°/90°] spherical shells for variety length to radius ratios Lx/Rx, modulus ratios E1/E2, and boundary conditions by FSDT.
| Boundary Conditions | Lx/Rx | E1/E2 |
|---|
| 5 | 10 | 15 | 25 | 40 |
|---|
| SD-SD | 0.1 | 6.8768 | 7.5476 | 8.1132 | 9.0869 | 10.3019 |
| 0.2 | 7.1668 | 7.8326 | 8.3845 | 9.3295 | 11.1241 |
| 0.5 | 8.8937 | 9.5415 | 10.0265 | 10.8234 | 11.8112 |
| F-F | 0.1 | 3.9347 | 4.5645 | 5.0554 | 5.8596 | 6.8237 |
| 0.2 | 3.9482 | 4.5782 | 5.0664 | 5.8626 | 6.8150 |
| 0.5 | 4.0430 | 4.6793 | 5.1581 | 5.9229 | 6.8248 |
| C-C | 0.1 | 4.2046 | 5.0239 | 5.6852 | 6.7780 | 8.0950 |
| 0.2 | 5.0485 | 6.3327 | 7.3725 | 9.0778 | 11.1241 |
| 0.5 | 9.0303 | 12.0219 | 14.4032 | 18.2531 | 22.2144 |
Table 11.
The fundamental frequency parameter Ω for composite shallow [0°/90°] spherical shells for variety length to radius ratios Lx/Rx, modulus ratios E1/E2, and boundary conditions by CST.
Table 11.
The fundamental frequency parameter Ω for composite shallow [0°/90°] spherical shells for variety length to radius ratios Lx/Rx, modulus ratios E1/E2, and boundary conditions by CST.
| Boundary Conditions | Lx/Rx | E1/E2 |
|---|
| 5 | 10 | 15 | 25 | 40 |
|---|
| SD-SD | 0.1 | 7.2637 | 7.9884 | 8.6201 | 9.7202 | 11.1634 |
| 0.2 | 7.5404 | 8.2592 | 8.8759 | 9.9450 | 11.3972 |
| 0.5 | 9.0807 | 9.8983 | 10.4389 | 11.3430 | 12.5272 |
| C-C | 0.1 | 4.2891 | 5.1468 | 5.8463 | 7.0198 | 8.4698 |
| 0.2 | 5.1199 | 6.4318 | 7.4987 | 9.2602 | 11.3972 |
| 0.5 | 9.0807 | 12.0872 | 14.4816 | 18.3556 | 22.2167 |
Table 12.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Ku boundary conditions by FSDT.
Table 12.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Ku boundary conditions by FSDT.
| Stiffness | Plate | Cylindrical Shell | Spherical Shell | Hyperbolic Paraboloidal |
|---|
| m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 |
|---|
| 100 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 101 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 102 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 103 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 104 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 105 | 6.0999 | 11.8764 | 6.1713 | 11.9614 | 6.0843 | 12.1226 | 5.8619 | 7.0016 |
| 106 | 6.0999 | 11.8766 | 6.1716 | 11.9615 | 6.0847 | 12.1228 | 5.8619 | 7.0016 |
| 107 | 6.1000 | 11.8780 | 6.1739 | 11.9629 | 6.0884 | 12.1251 | 5.8619 | 7.0016 |
| 108 | 6.1009 | 11.8911 | 6.1960 | 11.9763 | 6.1241 | 12.1468 | 5.8600 | 7.0017 |
| 109 | 6.1070 | 11.9770 | 6.3472 | 12.0653 | 6.3634 | 12.2935 | 5.6539 | 7.0042 |
| 1010 | 6.1187 | 12.1169 | 6.6216 | 12.2166 | 6.7778 | 12.5456 | 7.0567 | 7.4902 |
| 1011 | 6.1223 | 12.1553 | 6.7039 | 12.2594 | 6.8965 | 12.6167 | 7.0752 | 7.3410 |
| 1012 | 6.1228 | 12.1598 | 6.7137 | 12.2645 | 6.9106 | 12.6251 | 7.0754 | 7.3395 |
Table 13.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kv boundary conditions by FSDT.
Table 13.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kv boundary conditions by FSDT.
| Stiffness | Plate | Cylindrical Shell | Spherical Shell | Hyperbolic Paraboloidal |
|---|
| m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 |
|---|
| 100 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 101 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 102 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 103 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 104 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 105 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0844 | 12.1226 | 5.8619 | 7.0016 |
| 106 | 6.1000 | 11.8765 | 6.1715 | 11.9614 | 6.0849 | 12.1228 | 5.8619 | 7.0016 |
| 107 | 6.1012 | 11.8772 | 6.1731 | 11.9621 | 6.0907 | 12.1251 | 5.8619 | 7.0016 |
| 108 | 6.1122 | 11.8844 | 6.1890 | 11.9686 | 6.1466 | 12.1476 | 5.8619 | 7.0016 |
| 109 | 6.2016 | 11.9437 | 6.3170 | 12.0234 | 6.5714 | 12.3419 | 5.8600 | 7.0016 |
| 1010 | 6.4698 | 12.1403 | 6.6986 | 12.2091 | 7.5921 | 13.0667 | 5.9118 | 7.0022 |
| 1011 | 6.6018 | 12.2486 | 6.8848 | 12.3139 | 7.9735 | 13.4990 | 5.9009 | 7.0021 |
| 1012 | 6.6204 | 12.2645 | 6.9109 | 12.3294 | 8.0215 | 13.5632 | 5.9008 | 7.0021 |
Table 14.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kw boundary conditions by FSDT.
Table 14.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kw boundary conditions by FSDT.
| Stiffness | Plate | Cylindrical Shell | Spherical Shell | Hyperbolic Paraboloidal |
|---|
| m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 |
|---|
| 100 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 101 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 102 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 103 | 6.0999 | 11.8765 | 6.1713 | 11.9614 | 6.0843 | 12.1226 | 5.8619 | 7.0016 |
| 104 | 6.1002 | 11.8766 | 6.1716 | 11.9616 | 6.0846 | 12.1227 | 5.8619 | 7.0016 |
| 105 | 6.1031 | 11.8786 | 6.1745 | 11.9635 | 6.0876 | 12.1245 | 5.8619 | 7.0016 |
| 106 | 6.1315 | 11.8980 | 6.2027 | 11.9826 | 6.1172 | 12.1424 | 5.8605 | 7.0024 |
| 107 | 6.3925 | 12.0948 | 6.4630 | 12.1764 | 6.3902 | 12.3238 | 6.4573 | 7.0719 |
| 108 | 7.7073 | 14.0587 | 7.7845 | 14.1180 | 7.8101 | 14.1629 | 7.7352 | 9.1254 |
| 109 | 8.7004 | 19.8103 | 8.7935 | 19.8887 | 19.8721 | 21.5398 | 8.7320 | 9.1254 |
| 1010 | 8.8380 | 21.1601 | 8.9336 | 21.2291 | 21.1699 | 21.6778 | 8.8733 | 9.1254 |
| 1011 | 8.8521 | 21.2786 | 8.9478 | 21.3223 | 21.2528 | 21.7339 | 8.8433 | 9.1254 |
| 1012 | 8.8535 | 21.2894 | 8.9493 | 21.3296 | 21.2599 | 21.7405 | 8.8434 | 9.1254 |
Table 15.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kϕx boundary conditions by FSDT.
Table 15.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kϕx boundary conditions by FSDT.
| Stiffness | Plate | Cylindrical Shell | Spherical Shell | Hyperbolic Paraboloidal |
|---|
| m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 |
|---|
| 100 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 101 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 102 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 103 | 6.0999 | 11.8762 | 6.1713 | 11.9611 | 6.0843 | 12.1223 | 5.8619 | 7.0016 |
| 104 | 6.0997 | 11.8739 | 6.1711 | 11.9588 | 6.0840 | 12.1200 | 5.8619 | 7.0016 |
| 105 | 6.0974 | 11.8507 | 6.1688 | 11.9354 | 6.0812 | 12.0971 | 5.8619 | 7.0013 |
| 106 | 6.0711 | 11.5921 | 6.1418 | 11.6754 | 6.0485 | 11.8418 | 5.8619 | 6.9694 |
| 107 | 6.5494 | 9.0772 | 6.6282 | 9.1053 | 6.6639 | 9.0215 | 5.8621 | 9.1254 |
| 108 | 6.2722 | 14.4763 | 6.3469 | 14.5752 | 6.3025 | 14.7114 | 5.8621 | 8.9458 |
| 109 | 6.2620 | 14.2671 | 6.3365 | 14.3650 | 6.2894 | 14.5019 | 5.8621 | 8.9351 |
| 1010 | 6.2611 | 14.2479 | 6.3356 | 14.3457 | 6.2882 | 14.4828 | 5.8621 | 8.9350 |
| 1011 | 6.2610 | 14.2460 | 6.3355 | 14.3438 | 6.2881 | 14.4808 | 5.8621 | 8.9350 |
| 1012 | 6.2609 | 14.2458 | 6.3355 | 14.3436 | 6.2880 | 14.4807 | 5.8621 | 8.9350 |
Table 16.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kϕy boundary conditions by FSDT.
Table 16.
The frequency parameter Ω for composite shallow [0°/90°] shells with S-Kϕy boundary conditions by FSDT.
| Stiffness | Plate | Cylindrical Shell | Spherical Shell | Hyperbolic Paraboloidal |
|---|
| m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 | m = 1 | m = 2 |
|---|
| 100 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 101 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 102 | 6.0999 | 11.8764 | 6.1713 | 11.9613 | 6.0843 | 12.1225 | 5.8619 | 7.0016 |
| 103 | 6.0997 | 11.8763 | 6.1711 | 11.9612 | 6.0841 | 12.1225 | 5.8619 | 7.0016 |
| 104 | 6.0977 | 11.8755 | 6.1691 | 11.9604 | 6.0820 | 12.1217 | 5.8619 | 7.0016 |
| 105 | 6.0780 | 11.8669 | 6.1485 | 11.9521 | 6.0611 | 12.1145 | 5.8610 | 7.0017 |
| 106 | 5.8434 | 11.7713 | 5.9050 | 11.8600 | 5.8139 | 12.0349 | 5.7743 | 7.0070 |
| 107 | 5.7759 | 9.0847 | 9.1053 | 9.2703 | 9.0215 | 9.4964 | 7.1004 | 9.1254 |
| 108 | 7.5204 | 12.8896 | 7.6516 | 12.9518 | 7.6426 | 12.9930 | 7.1247 | 9.4010 |
| 109 | 7.4432 | 12.8039 | 7.5710 | 12.8671 | 7.5546 | 12.9172 | 7.1250 | 9.3841 |
| 1010 | 7.4360 | 12.7961 | 7.5634 | 12.8594 | 7.5463 | 12.9103 | 7.1250 | 9.3840 |
| 1011 | 7.4352 | 12.7954 | 7.5627 | 12.8587 | 7.5455 | 12.9096 | 7.1250 | 9.3840 |
| 1012 | 7.4352 | 12.7953 | 7.5626 | 12.8586 | 7.5454 | 12.9095 | 7.1250 | 9.3840 |