Numerical and Experimental Investigation of Morphological Modification on Fused Silica Using CO2 Laser Ablation
Abstract
:1. Introduction
2. Materials and Methods
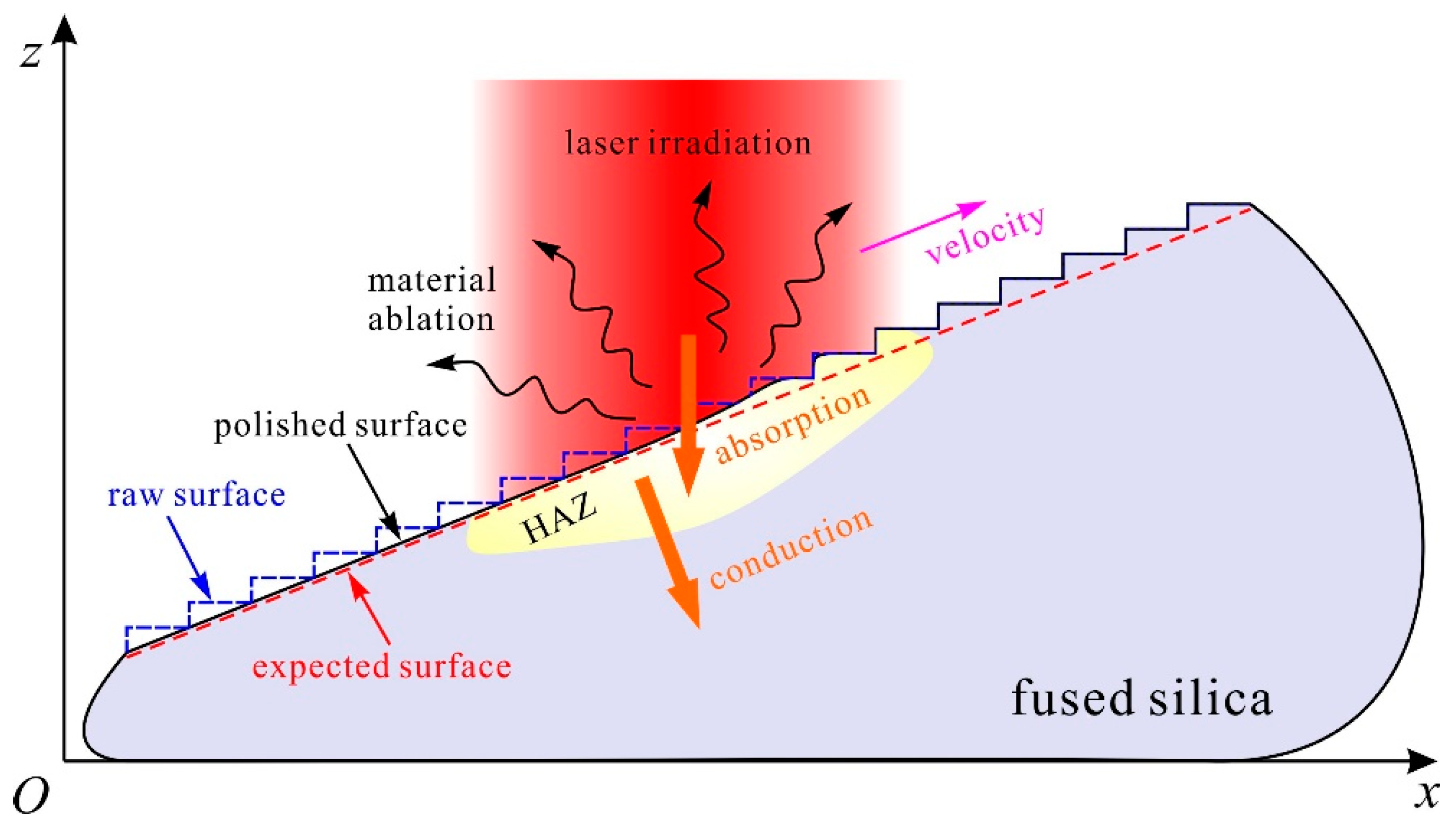
2.1. Numerical Model of CO2 Laser Ablation
2.2. Experimental Setup
3. Results and Discussion
3.1. Prediction of Morphological Modification
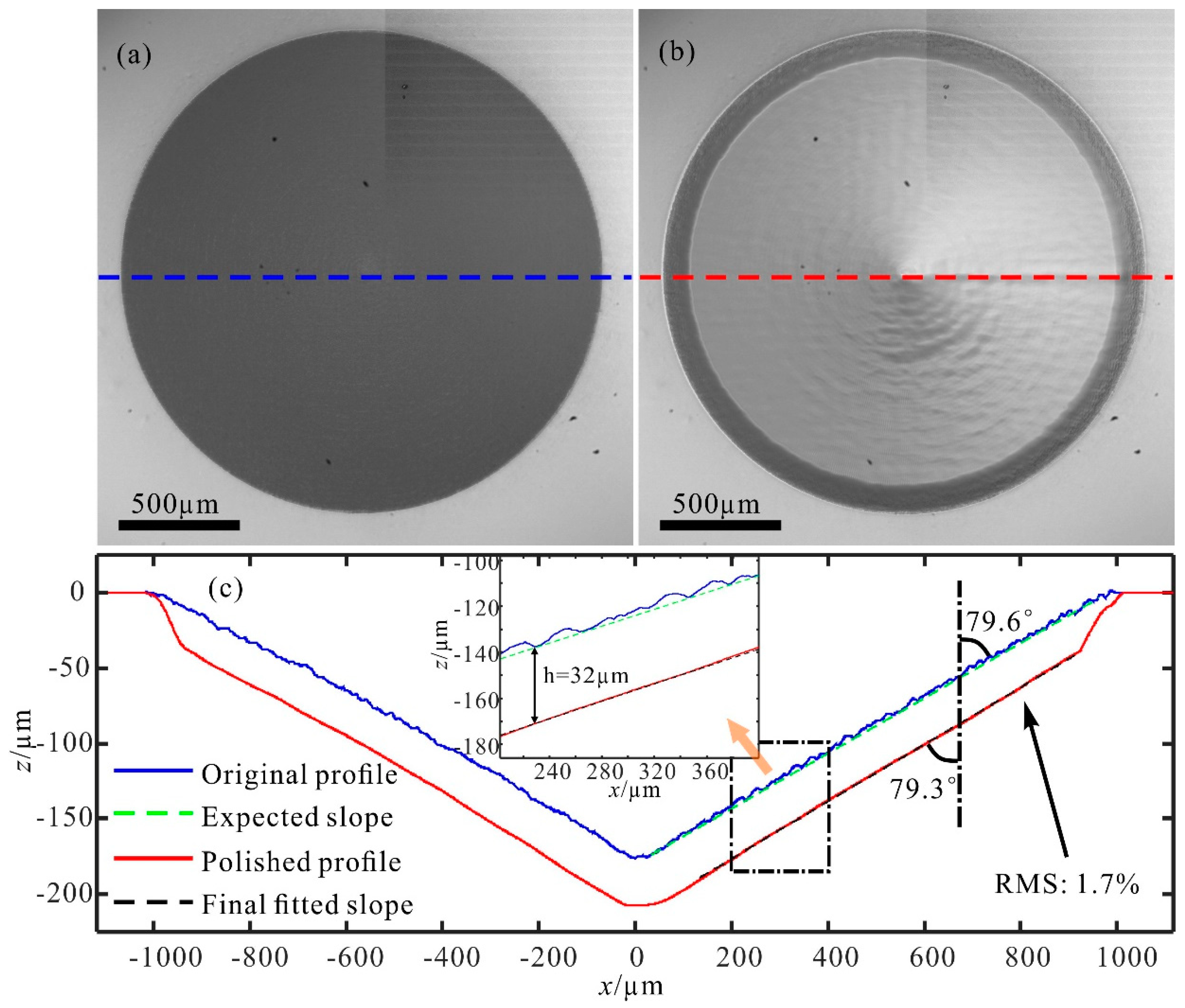
3.2. Experimental Verification
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sugioka, K. Progress in ultrafast laser processing and future prospects. Nanophotonics 2017, 6. [Google Scholar] [CrossRef] [Green Version]
- Phillips, K.C.; Gandhi, H.H.; Mazur, E.; Sundaram, S.K. Ultrafast laser processing of materials: A review. Adv. Opt. Photon. 2015, 7, 684–712. [Google Scholar] [CrossRef]
- Asri, R.I.M.; Harun, W.S.W.; Samykano, M.; Lah, N.A.C.; Ghani, S.A.C.; Tarlochan, F.; Raza, M.R. Corrosion and surface modification on biocompatible metals: A review. Mater. Sci. Eng. C 2017, 77, 1261–1274. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tian, Y.S.; Chen, C.Z.; Li, S.T.; Huo, Q.H. Research progress on laser surface modification of titanium alloys. Appl. Surf. Sci. 2005, 242, 177–184. [Google Scholar] [CrossRef]
- Weingarten, C.; Schmickler, A.; Willenborg, E.; Wissenbach, K.; Poprawe, R. Laser polishing and laser shape correction of optical glass. J. Laser Appl. 2017, 29. [Google Scholar] [CrossRef] [Green Version]
- Weingarten, C.; Uluz, E.; Schmickler, A.; Braun, K.; Willenborg, E.; Temmler, A.; Heidrich, S. Glass processing with pulsed CO2 laser radiation. Appl. Opt. 2017, 56, 777–783. [Google Scholar] [CrossRef] [PubMed]
- Shen, N.; Matthews, M.J.; Fair, J.E.; Britten, J.A.; Nguyen, H.T.; Cooke, D.; Elhadj, S.; Yang, S.T. Laser smoothing of sub-micron grooves in hydroxyl-rich fused silica. Appl. Surf. Sci. 2010, 256, 4031–4037. [Google Scholar] [CrossRef] [Green Version]
- Wlodarczyk, K.L.; Mendez, E.; Baker, H.J.; McBride, R.; Hall, D.R. Laser smoothing of binary gratings and multilevel etched structures in fused silica. Appl. Opt. 2010, 49, 1997–2005. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wlodarczyk, K.L. Surface Deformation Mechanisms in Laser Smoothing and Micromachining of Optical Glasses. Ph.D. Thesis, Heriot-Watt University, Edinburgh, UK, 2011. [Google Scholar]
- Nowak, K.M.; Baker, H.J.; Hall, D.R. Analytical model for CO(2) laser ablation of fused quartz. Appl. Opt. 2015, 54, 8653–8663. [Google Scholar] [CrossRef] [PubMed]
- Lane, D.W. The optical properties and laser irradiation of some common glasses. J. Phys. D Appl. Phys. 1990, 23, 1727–1734. [Google Scholar] [CrossRef]
- Lukasz, W.K.; Neil, M.W.; Paul, H.D. Laser processing of borofloat 33 glass. In Proceedings of the 16th International Symposium on Laser Precision Microfabrication (LPM), Kokura, Japan, 26–29 May 2015. [Google Scholar]
- Markillie, G.A.; Baker, H.J.; Villarreal, F.J.; Hall, D.R. Effect of vaporization and melt ejection on laser machining of silica glass micro-optical components. Appl. Opt. 2002, 41, 5660–5667. [Google Scholar] [CrossRef] [PubMed]
- Exarhos, G.J.; Bass, I.L.; Gruzdev, V.E.; Guss, G.M.; Nostrand, M.J.; Menapace, J.A.; Ristau, D.; Wegner, P.J.; Soileau, M.J. An improved method of mitigating laser-induced surface damage growth in fused silica using a rastered pulsed CO2 laser. In Proceedings of the Annual Symposium on Optical Materials for High Power Lasers, Boulder, CO, USA, 2 December 2010. [Google Scholar]
- Schrage, R.W. A Theoretical Study of Interphase Mass Transfer; Columbia University Press: New York, NY, USA, 1953. [Google Scholar]
- Schick, H.L. A Thermodynamic Analysis of the High-Temperature Vaporization Properties of Silica. Chem. Rev. 1960, 60, 331–362. [Google Scholar] [CrossRef]
- Elhadj, S.; Matthews, M.J.; Yang, S.T.; Cooke, D.J. Evaporation kinetics of laser heated silica in reactive and inert gases based on near-equilibrium dynamics. Opt. Express 2012, 20, 1575–1587. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhou, L.; Jiang, Y.E.; Zhang, P.; Fan, W.; Li, X.C. Directly writing binary multi-sector phase plates on fused silica using femtosecond laser. High Power Laser Sci. 2018, 6, e6. [Google Scholar] [CrossRef] [Green Version]
- Exarhos, G.J.; Feit, M.D.; Gruzdev, V.E.; Matthews, M.J.; Soules, T.F.; Menapace, J.A.; Ristau, D.; Stolken, J.S.; Vignes, R.M.; Soileau, M.J.; et al. Densification and residual stress induced by CO2 laser-based mitigation of SiO2 surfaces. In Proceedings of the Laser Damage Symposium XLII: Annual Symposium on Optical Materials for High Power Lasers, Boulder, CO, USA, 30 November 2010. [Google Scholar]
- Vignes, R.M.; Soules, T.F.; Stolken, J.S.; Settgast, R.R.; Elhadj, S.; Matthews, M.J.; Mauro, J. Thermomechanical Modeling of Laser-Induced Structural Relaxation and Deformation of Glass: Volume Changes in Fused Silica at High Temperatures. J. Am. Ceram. Soc. 2013, 96, 137–145. [Google Scholar] [CrossRef]
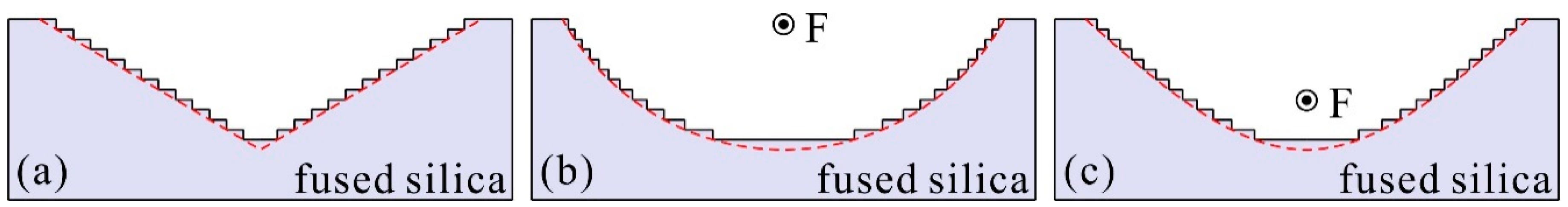





| Parameter | Value |
|---|---|
| pulse energy E | 0.5–5 MJ |
| pulse repetition frequency fr | 0.5–2 kHz |
| pulse duration τ | 10–100 µs |
| diameter of focus spot 2ω0 | 120 µm@1/e2 |
| interval between the adjacent spots di | 10–120 µm |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, L.; Jiang, Y.; Zhang, P.; Wei, H.; Fan, W.; Li, X.; Zhu, J. Numerical and Experimental Investigation of Morphological Modification on Fused Silica Using CO2 Laser Ablation. Materials 2019, 12, 4109. https://doi.org/10.3390/ma12244109
Zhou L, Jiang Y, Zhang P, Wei H, Fan W, Li X, Zhu J. Numerical and Experimental Investigation of Morphological Modification on Fused Silica Using CO2 Laser Ablation. Materials. 2019; 12(24):4109. https://doi.org/10.3390/ma12244109
Chicago/Turabian StyleZhou, Li, Youen Jiang, Peng Zhang, Hui Wei, Wei Fan, Xuechun Li, and Jianqiang Zhu. 2019. "Numerical and Experimental Investigation of Morphological Modification on Fused Silica Using CO2 Laser Ablation" Materials 12, no. 24: 4109. https://doi.org/10.3390/ma12244109
APA StyleZhou, L., Jiang, Y., Zhang, P., Wei, H., Fan, W., Li, X., & Zhu, J. (2019). Numerical and Experimental Investigation of Morphological Modification on Fused Silica Using CO2 Laser Ablation. Materials, 12(24), 4109. https://doi.org/10.3390/ma12244109






