Experimental Study on Chloride Ion Diffusion in Concrete under Uniaxial and Biaxial Sustained Stress
Abstract
:1. Introduction
2. Experimental Program
2.1. Materials and Specimen Preparation
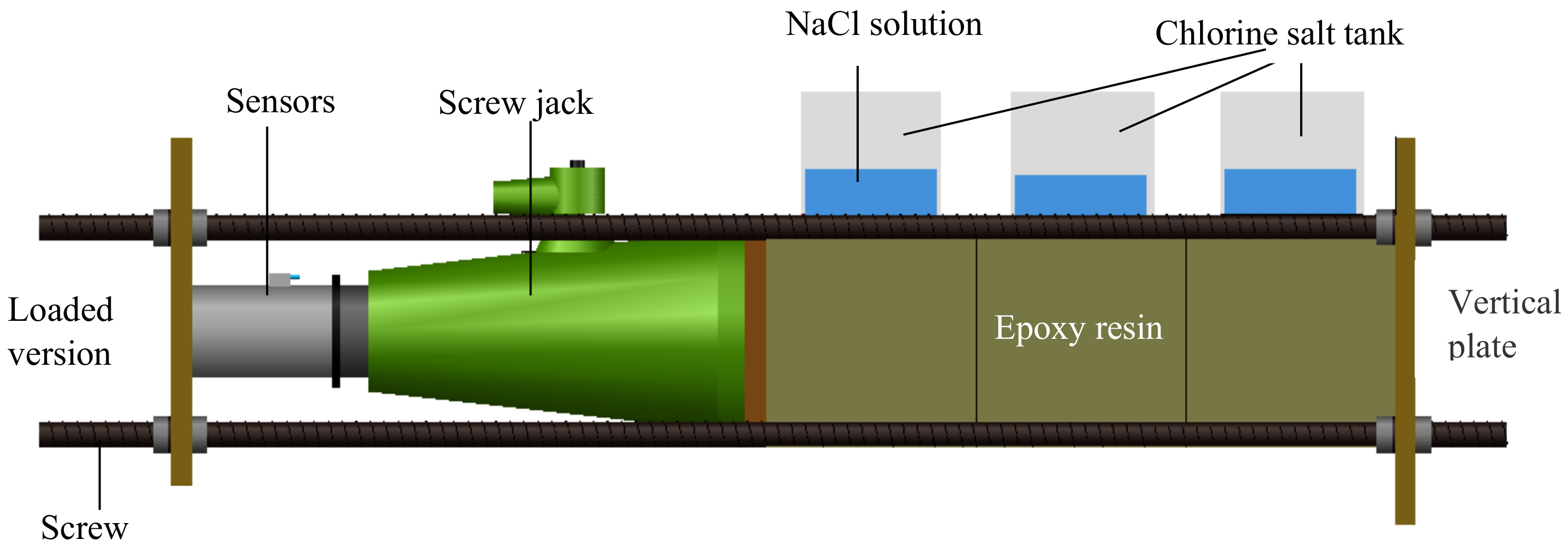
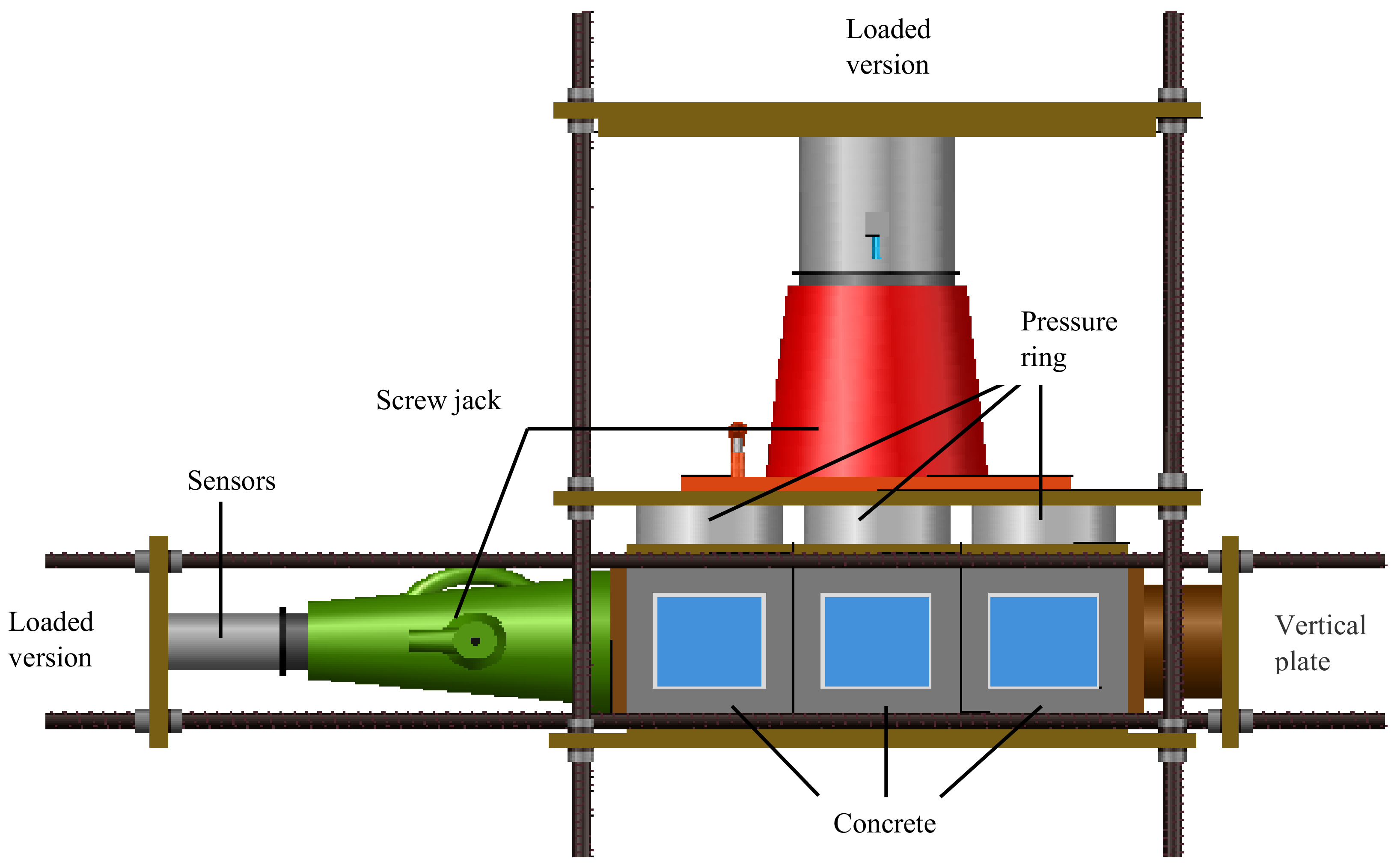
2.2. Experimental and Test Arrangement
2.3. Diffusion Experiment and Determination of Chloride Concentration
3. Test Results and Discussion
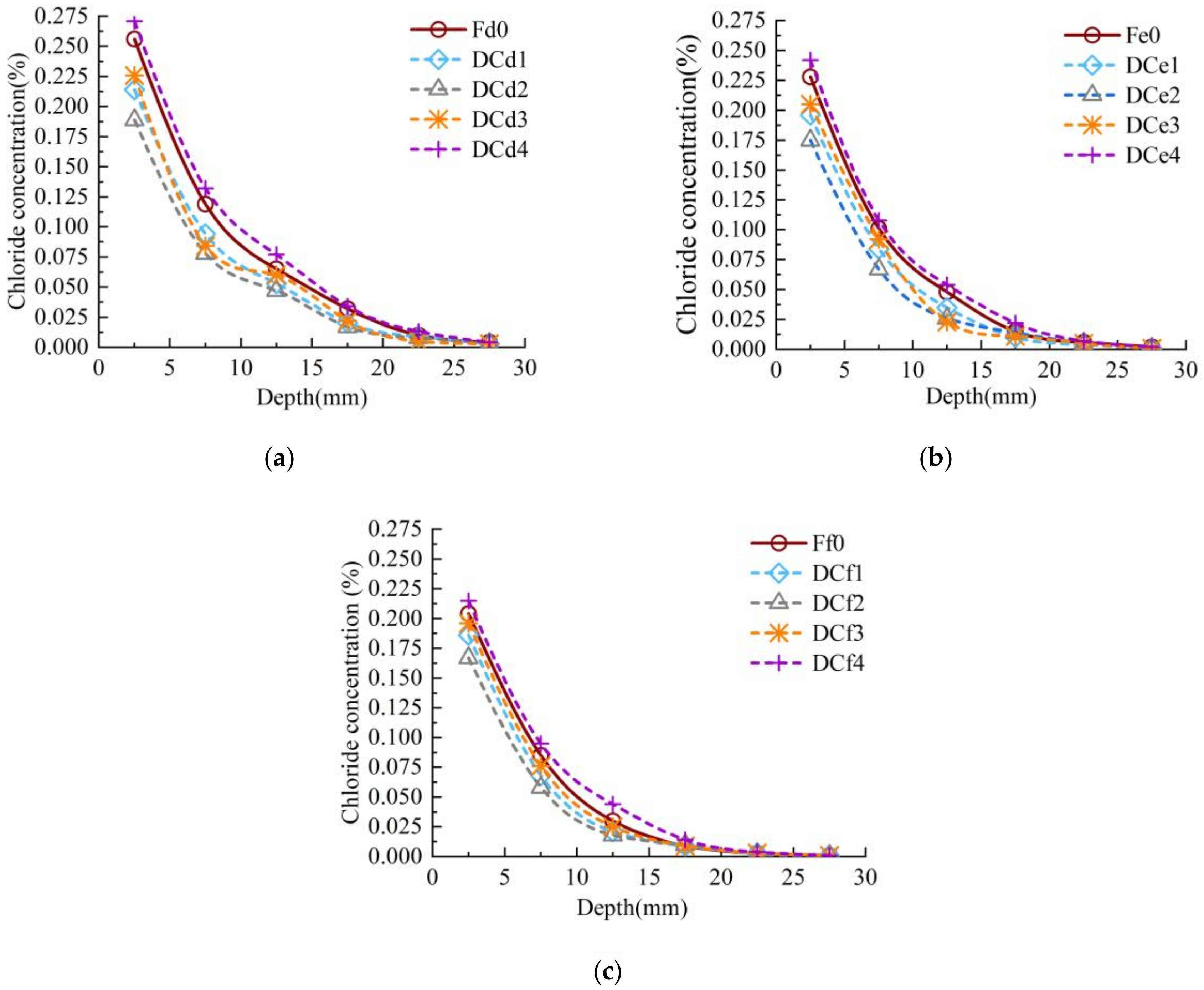
3.1. Distribution of Chloride Concentration under Different Axial Compressive Stresses
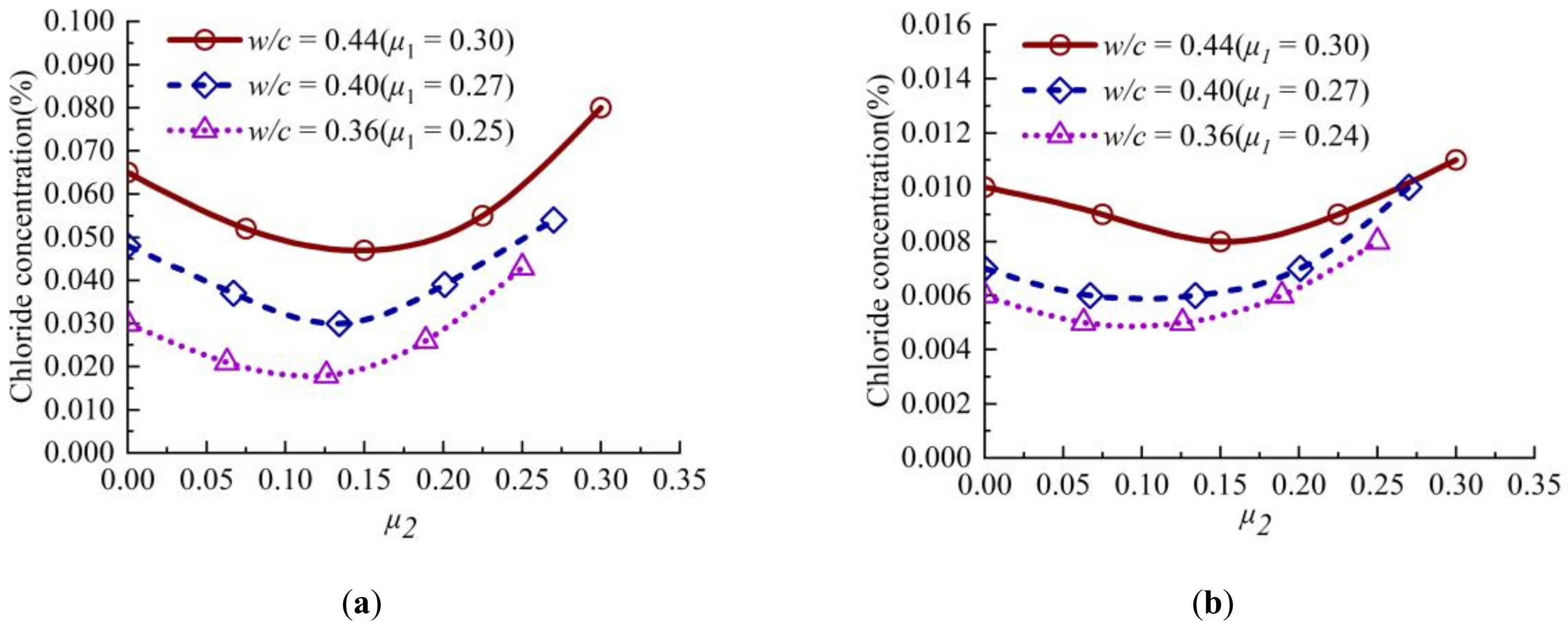
3.2. Distribution of Chloride Concentration under Different Lateral Compressive Stresses
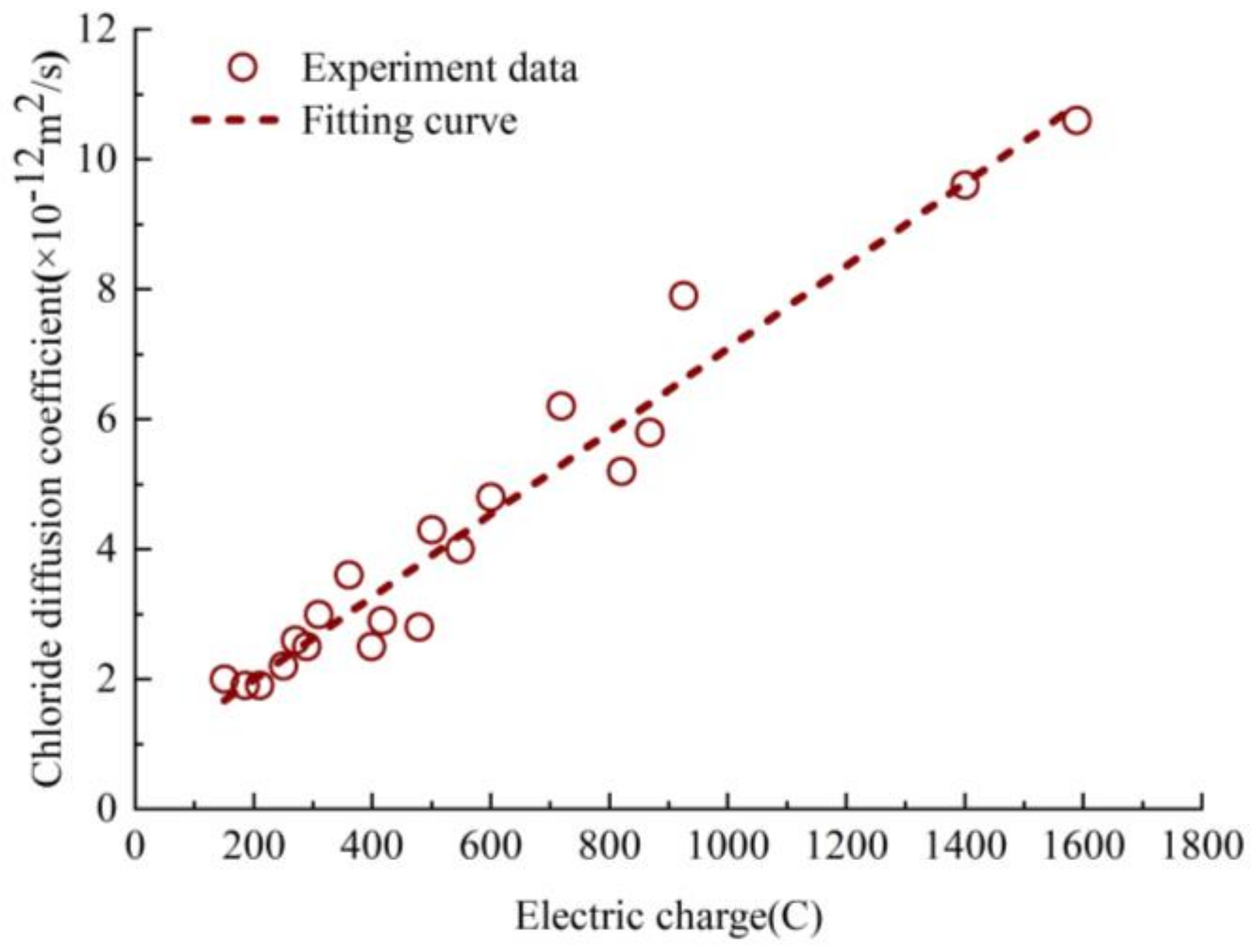
4. Chloride Diffusion Coefficient under Different Stress States
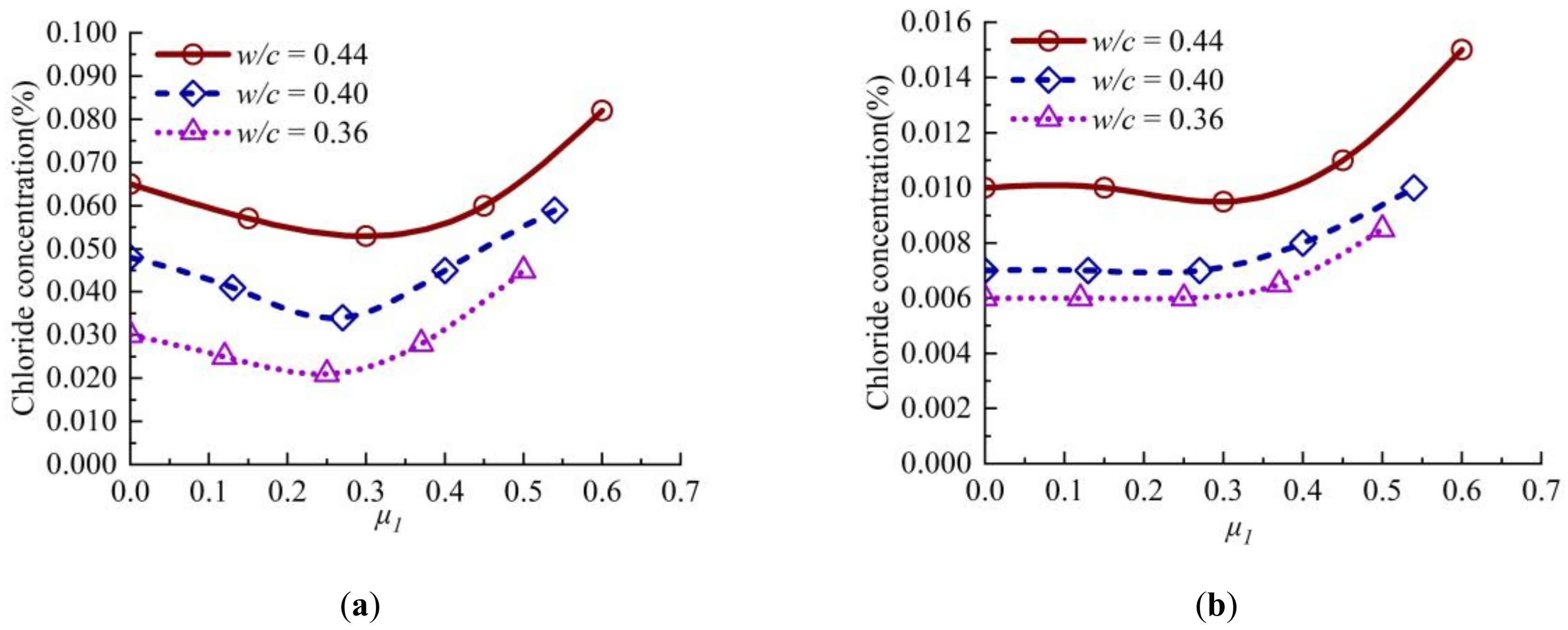
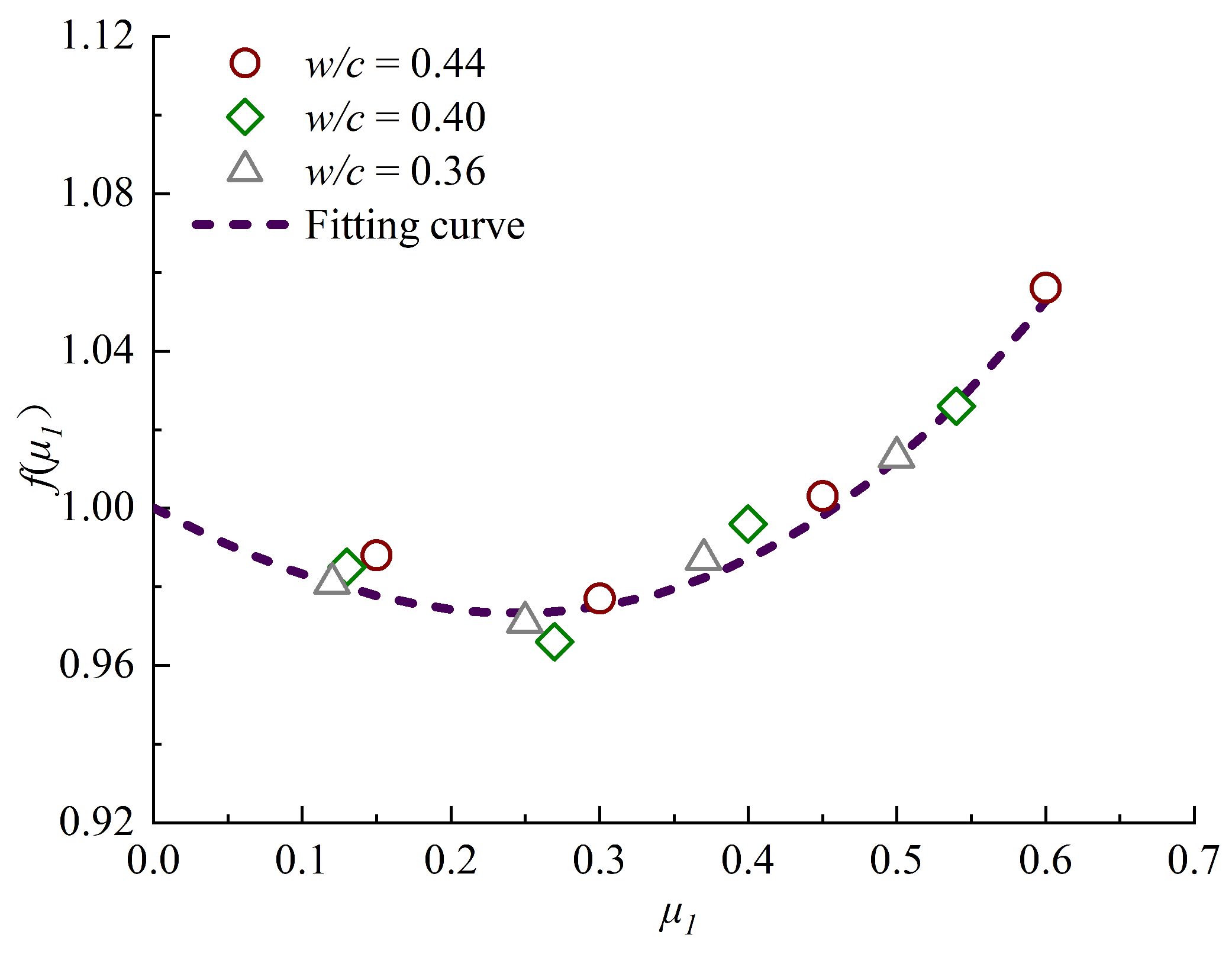
4.1. Stress Level Function under Uniaxial Sustained Stress
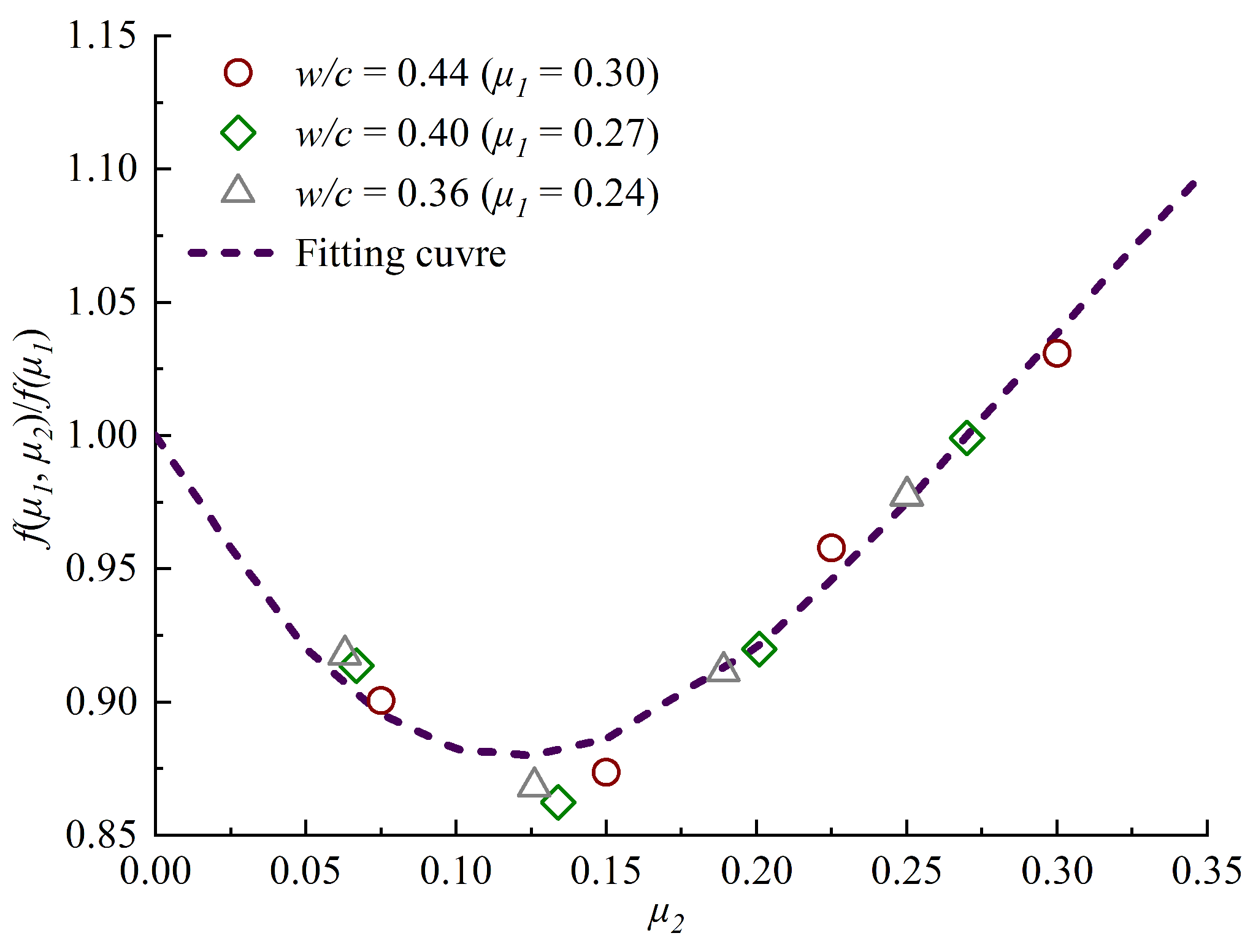
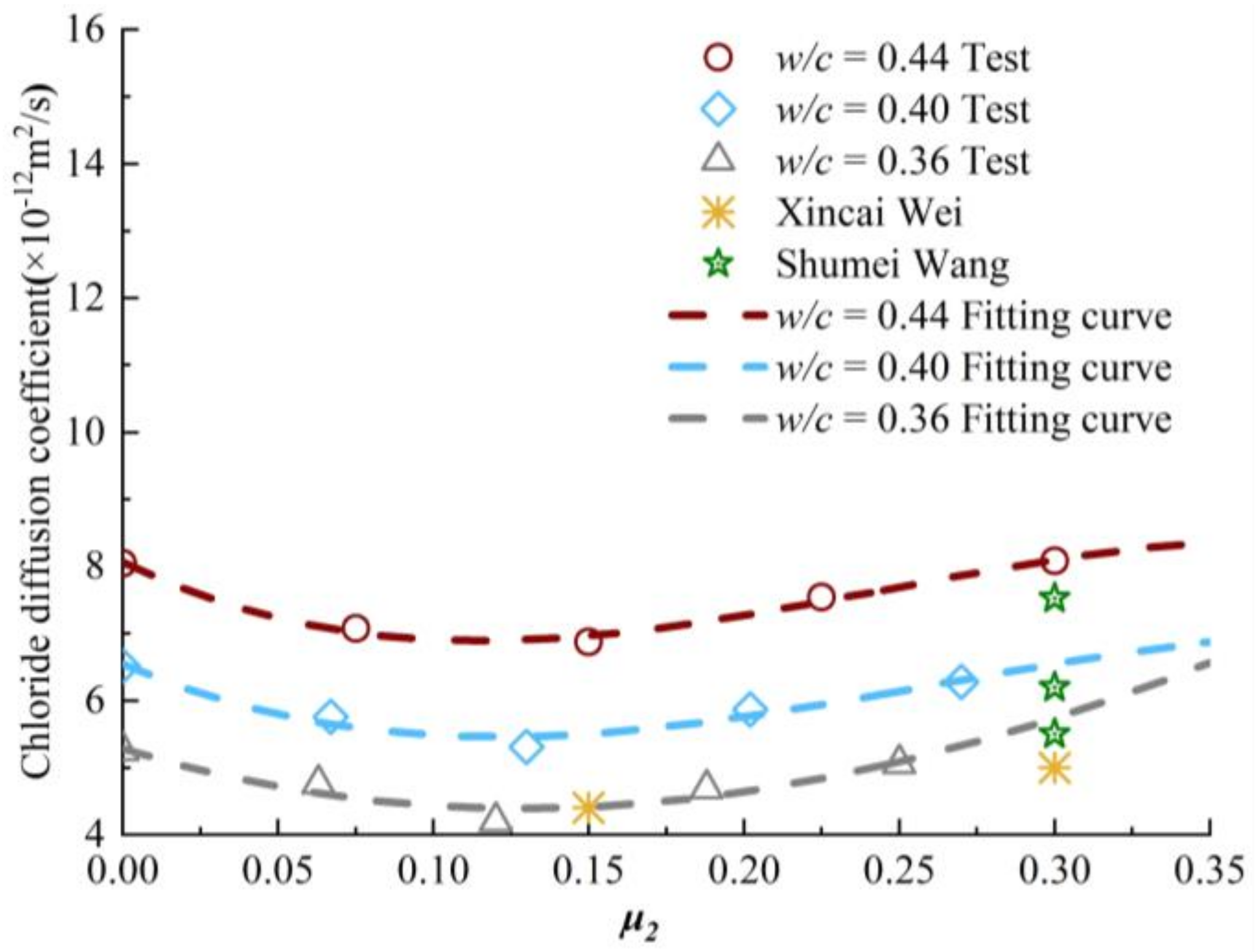
4.2. Stress Level Function under Biaxial Sustained Compressive Stress
5. Conclusions and Comments
- The existence of sustained compressive stress changes the diffusion process of chloride ions from environmental solution into concrete. It is found that the maximum chloride concentration of concrete specimens under axial sustained compressive stress of 0.3·fc are about 35% lower than that those of under no stress. The highest concentration chloride in the concretes with the axial and lateral sustained compressive stress of 0.3·fc and 0.15·fc are respectively reduced by about 50% compared with those of without stress. In addition, the diffusion process and concentration of chloride ions will increase when the sustained compressive stress is higher than 0.3fc. For biaxial concrete with constant axial sustained compressive stress, the chloride concentration distribution with lateral sustained compressive stress greater than 0.15·fc is 1.27-1.1 times that of lateral sustained compressive stress. With the increase of sustained compressive stress, the diffusion distribution of chloride ions will be further enhanced.
- Under the same sustained compressive stress, the chloride concentration of C45 concrete specimens is higher than that of C55 concrete specimen. This is used for the higher w/c ratio produces lower grade concrete will leads to the concrete structure is not dense and is more susceptible to the influence of sustained compressive stress. Therefore, concrete specimens with higher w/c ratio have higher permeability. The chloride diffusion coefficient model, considering the effect of uniaxial and biaxial sustained compressive stress of concrete, is established. The model is helpful to predict the durability of concrete structures in chlorine-laden environment.
- It is recommended that further research to measure the cracks in concrete with sustained stress states during the experiment. The pore size distribution of concrete under sustained stress and crack depth, so as to expand and improve the model potentially.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Peng, J.X.; Hu, S.W.; Zhang, J.R.; Cai, C.S.; Li, L.Y. Influence of cracks on chloride diffusivity in concrete: A five-phase mesoscale model approach. Constr. Build. Mater. 2019, 197, 587–596. [Google Scholar] [CrossRef]
- Xu, F.; Yang, Z.Q.; Liu, W.Q.; Wang, S.G.; Zhang, H.G. Experimental investigation on the effect of sulfate attack on chloride diffusivity of cracked concrete subjected to composite solution. Constr. Build. Mater. 2020, 237, 117643. [Google Scholar] [CrossRef]
- Peng, J.X.; Tang, H.; Zhang, J.R.; Cai, C.S. Numerical simulation on carbonation depth of concrete structures considering time- and temperature-dependent carbonation process. Adv. Mater. Sci. Eng. 2018, 1–16, in press. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.M.; Peng, J.X.; Zhang, J.R.; Cai, C.S. A new method for estimating the scale of fluctuation in reliability assessment of reinforced concrete structures considering spatial variability. Adv. Struct. Eng. 2018, 21, 1951–1962. [Google Scholar] [CrossRef]
- Ma, Y.F.; Guo, Z.Z.; Wang, L.; Zhang, J.R. Probabilistic life prediction for reinforced concrete structures subjected to seasonal corrosion fatigue damage. J. Struct. Eng. 2020, 146, 04020117. [Google Scholar] [CrossRef]
- Dai, L.Z.; Bian, H.B.; Wang, L.; Michel, P.-F.; Zhang, J.R. Prestress loss diagnostics in pre-tensioned concrete structures with corrosive cracking. J. Struct. Eng. 2020, 146, 04020013. [Google Scholar] [CrossRef]
- Wang, J.; Ng, P.-L.; Su, H.; Du, S.J. Influence of the coupled time and concrete stress effects on instantaneous chloride diffusion coefficient. Constr. Build. Mater. 2020, 237, 117645. [Google Scholar] [CrossRef]
- Peng, J.X.; Tang, H.; Zhang, J.R. Structural behavior of corroded reinforced concrete beams strengthened with steel plate. J. Perform. Constr. Fac. 2017, 31, 1–13. [Google Scholar] [CrossRef]
- Samaha, H.R.; Hover, K.C. Influence of microcracking on the mass transport properties of concrete. ACI Mater. J. 1992, 89, 416–424. [Google Scholar]
- Wang, H.L.; Dai, J.G.; Sun, X.Y.; Zhang, X.L. Time-dependent and stress-dependent chloride diffusivity of concrete subjected to sustained compressive loading. J. Mater. Civ. Eng. 2016, 28, 1–9. [Google Scholar] [CrossRef]
- Lim, C.C.; Gowripalan, N.; Sirivivatnanon, V. Microcracking and chloride permeability of concrete under uniaxial compression. Cem. Concr. Compos. 2000, 22, 353–360. [Google Scholar] [CrossRef]
- Lawler, J.S.; Zampini, D.; Shah, S.P. Permeability of cracked hybrid fiber-reinforced mortar under load. ACI Mater. J. 2002, 99, 379–385. [Google Scholar]
- Shuo, Y.; Hao, J. Modeling of the corrosion-induced crack in concrete contained transverse crack subject to chloride ion penetration. Constr. Build. Mater. 2020, 258, 119645. [Google Scholar]
- Tang, H.; Peng, J.X.; Zhang, J.R. Influence of Further corrosion on structural behavior of corroded and strengthened RC beams. J. Perform. Constr. Fac. 2020, 33, 1–13. [Google Scholar]
- Wei, X.C. The Research of the Effect of Two-Way Load, Freezing-Thawing Cycles and Curing Age on Chloride Permeability of Concrete. Master’s Thesis, Dalian University of Technology, Dalian, China, 10 June 2014. [Google Scholar]
- Yan, B.L.; Jiang, L.Z.; Xiao, Z.M.; Liu, C.; Zhang, Q.Y.; Chen, P.; Huo, C.M.; Xi, J.S.; Song, L.C.; Wang, X.; et al. Common Portland Cement; GB175-2007; CABR: Beijing, China, 2007; pp. 3–6. [Google Scholar]
- Matthias, Q.; Manfred, C. Concrete under biaxial dynamic compressive loading. Procedia Eng. 2017, 210, 24–31. [Google Scholar]
- Zhou, J.J.; Pan, J.L.; Zhang, L.; Zhao, J.; Li, Z.J. Experimental study on mechanical behavior of high-strength high-performance concrete under biaxial loading. Constr. Build. Mater. 2020, 258, 119681. [Google Scholar] [CrossRef]
- Li, J.Y.; Xu, C.H.; Mi, S.D.; Li, G.S.; Fan, W.D.; Weng, Y.F.; Huang, X.H.; Tu, L.Q.; Xiong, J.B. Technical Code for Concrete Test and Detection of Waterway Engineering; JTS/T 236-2019; MCC: Beijing, China, 2019; pp. 227–231. [Google Scholar]
- Wang, L.; Dai, L.Z.; Bian, H.B.; Ma, Y.F.; Zhang, J.R. Concrete cracking prediction under combined prestress and strand corrosion. Struct. Infrastruct. E. 2019, 15, 285–295. [Google Scholar] [CrossRef]
- Yang, Y.M.; Peng, J.X.; Liu, X.H.; Cai, C.S.; Zhang, J.R. Probability analysis of web cracking of corroded prestressed concrete box-girder bridges considering aleatory and epistemic uncertainties. Eng. Struct. 2020, 111486, in press. [Google Scholar] [CrossRef]
- Zacchei, E.; Nogueira, C.G. Chloride diffusion assessment in RC structures considering the stress-strain state effects and crack width influences. Constr. Build. Mater. 2019, 201, 100–109. [Google Scholar] [CrossRef]
- Wang, L.C.; Bao, J.W. Investigation on chloride penetration into unsaturated concrete under short-term sustained tensile loading. Mater. Struct. 2017, 227, 1–15. [Google Scholar] [CrossRef]
- Xu, J.; Li, F.M.; Zhao, J.; Huang, L. Model of time-dependent and stress-dependent chloride penetration of concrete under sustained axial pressure in the marine environment. Constr. Build. Mater. 2018, 170, 207–216. [Google Scholar] [CrossRef]
- Khan, I.M. Comparison of diffusion and chloride ion penetration of high-performance concrete. Ksce. J. Civ. Eng. 2012, 16, 779–784. [Google Scholar] [CrossRef]
- Wang, Y.Z.; Zhou, H.F. Chlorine ion diffusion experiment in loaded concrete under salt spray environment. J. Mater. Sci. Eng. 2013, 5, 25–30. [Google Scholar] [CrossRef]
- Wang, W.J.; Wu, J.; Wang, Z.; Wang, Z.; Wu, G.Z.; Yue, A.Y. Chloride diffusion coefficient of recycled aggregate concrete under compressive loading. Mater. Struct. 2016, 49, 4729–4736. [Google Scholar]
- Li, H.M.; Wu, J.; Song, Y.J.; Wang, Z. Effect of external loads on chloride diffusion coefficient of concrete with fly ash and blast furnace slag. J. Mater. Civ. Eng. 2014, 26, 1–6. [Google Scholar]
- Wang, S.M. Influence of Two-Way Load on Chloride Permeability of High-Performance Concrete. Master’s Thesis, Dalian University of Technology, Dalian, China, 9 December 2011. [Google Scholar]
- Hu, Y.J.; Du, Y.L.; Zhang, W. Evaluation of relationship between innitial current, conductance, chloride diffusion coefficient and charge passed of concrete. Adv. Mat. Res. 2012, 399–401, 1200–1203. [Google Scholar] [CrossRef]
- Liu, Q.F.; Li, L.Y.; Easterbrook, D.; Yang, J. Multi-phase modelling of ionic transport in concrete when subjected to an externally applied electric field. Eng. Struct. 2012, 42, 201–213. [Google Scholar] [CrossRef]
- Yang, C.C.; Cho, S.W. The relationship between chloride migration rate for concrete and electrical current in steady state using the accelerated chloride migration test. Mater. Struct. 2004, 37, 456–463. [Google Scholar] [CrossRef]


| Ingredients | SiO2 | Al2O3 | CaO | MgO | SO2 | Loss on Ignition |
|---|---|---|---|---|---|---|
| Mass Percent (%) | 22.45 | 6.64 | 63.31 | 2.63 | 2.38 | 1.89 |
| Type | Unit Content (kg/m3) | |||||
|---|---|---|---|---|---|---|
| w/c | Water | Cement | Fine Aggregate | Coarse Aggregate | SP | |
| C45 | 0.44 | 180 | 409.09 | 543.27 | 1267.64 | 3.40 |
| C50 | 0.40 | 180 | 450.00 | 531.00 | 1239.00 | 3.15 |
| C55 | 0.36 | 180 | 500.00 | 516.00 | 1204.00 | 3 |
| Specimen | w/c | fc (MPa) | σcx (MPa) |
|---|---|---|---|
| DCd1 | 0.44 | 47.21 | 7.08(0.15fc) |
| DCd2 | 0.44 | 47.21 | 14.16(0.30fc) |
| DCd3 | 0.44 | 47.21 | 21.24(0.45fc) |
| DCd4 | 0.44 | 47.21 | 28.33(0.60fc) |
| DCe1 | 0.40 | 52.68 | 7.08(0.13fc) |
| DCe2 | 0.40 | 52.68 | 14.16(0.27fc) |
| DCe3 | 0.40 | 52.68 | 21.24(0.40fc) |
| DCe4 | 0.40 | 52.68 | 28.33(0.54fc) |
| DCf1 | 0.36 | 56.78 | 7.08(0.12fc) |
| DCf2 | 0.36 | 56.78 | 14.16(0.25fc) |
| DCf3 | 0.36 | 56.78 | 21.24(0.37fc) |
| DCf4 | 0.36 | 56.78 | 28.33(0.50fc) |
| Specimen | w/c | σcx/σcy | fc (MPa) | σcx (MPa) | σcy (MPa) |
|---|---|---|---|---|---|
| ECd1 | 0.44 | 1:0.25 | 47.21 | 14.16(0.30fc) | 3.54(0.075fc) |
| ECd2 | 0.44 | 1:0.5 | 47.21 | 14.16(0.30fc) | 7.08(0.150fc) |
| ECd3 | 0.44 | 1:0.75 | 47.21 | 14.16(0.30fc) | 10.63(0.225fc) |
| ECd4 | 0.44 | 1:1 | 47.21 | 14.16(0.30fc) | 14.16(0.300fc) |
| ECe1 | 0.40 | 1:0.25 | 52.68 | 14.16(0.27fc) | 3.54(0.067fc) |
| ECe2 | 0.40 | 1:0.5 | 52.68 | 14.16(0.27fc) | 7.08(0.134fc) |
| ECe3 | 0.40 | 1:0.75 | 52.68 | 14.16(0.27fc) | 10.63(0.201fc) |
| ECe4 | 0.40 | 1:1 | 52.68 | 14.16(0.27fc) | 14.16(0.270fc) |
| ECf1 | 0.36 | 1:0.25 | 56.78 | 14.16(0.25fc) | 3.54(0.063fc) |
| ECf2 | 0.36 | 1:0.5 | 56.78 | 14.16(0.25fc) | 7.08(0.126fc) |
| ECf3 | 0.36 | 1:0.75 | 56.78 | 14.16(0.25fc) | 10.63(0.189fc) |
| ECf4 | 0.36 | 1:1 | 56.78 | 14.16(0.25fc) | 14.16(0.250fc) |
| Specimen | Dc (×10−12 m2/s) | Specimen | Dc (×10−12 m2/s) | Specimen | Dc (×10−12 m2/s) |
|---|---|---|---|---|---|
| Fd0 | 8.05 | Fe0 | 6.52 | Ff0 | 5.25 |
| DCd1 | 7.95 | DCe1 | 6.42 | DCf1 | 5.15 |
| DCd2 | 7.86 | DCe2 | 6.30 | DCf2 | 5.10 |
| DCd3 | 8.07 | DCe3 | 6.49 | DCf3 | 5.18 |
| DCd4 | 8.50 | DCe4 | 6.69 | DCf4 | 5.32 |
| Specimen | Dc (×10−12 m2/s) | Specimen | Dc (×10−12 m2/s) | Specimen | Dc (×10−12 m2/s) |
|---|---|---|---|---|---|
| Fd0 | 8.05 | Fe0 | 6.52 | Ff0 | 5.25 |
| ECd1 | 7.08 | ECe1 | 5.73 | ECf1 | 4.70 |
| ECd2 | 6.87 | ECe2 | 5.41 | ECf2 | 4.43 |
| ECd3 | 7.54 | ECe3 | 5.81 | ECf3 | 4.61 |
| ECd4 | 8.08 | ECe4 | 6.28 | ECf4 | 5.00 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, X.; Peng, J.; Cai, C.S.; Zhang, J. Experimental Study on Chloride Ion Diffusion in Concrete under Uniaxial and Biaxial Sustained Stress. Materials 2020, 13, 5717. https://doi.org/10.3390/ma13245717
Cheng X, Peng J, Cai CS, Zhang J. Experimental Study on Chloride Ion Diffusion in Concrete under Uniaxial and Biaxial Sustained Stress. Materials. 2020; 13(24):5717. https://doi.org/10.3390/ma13245717
Chicago/Turabian StyleCheng, Xiaokang, Jianxin Peng, C.S. Cai, and Jianren Zhang. 2020. "Experimental Study on Chloride Ion Diffusion in Concrete under Uniaxial and Biaxial Sustained Stress" Materials 13, no. 24: 5717. https://doi.org/10.3390/ma13245717
APA StyleCheng, X., Peng, J., Cai, C. S., & Zhang, J. (2020). Experimental Study on Chloride Ion Diffusion in Concrete under Uniaxial and Biaxial Sustained Stress. Materials, 13(24), 5717. https://doi.org/10.3390/ma13245717




