Properties of Rubberized Engineered Cementitious Composites Containing Nano-Silica
Abstract
:1. Introduction
2. Experimental Program
2.1. Material Properties
2.2. Rubberized ECC Mixtures Proportions Using RSM
2.3. Specimens Preparation and Test Procedures
3. Results and Discussions
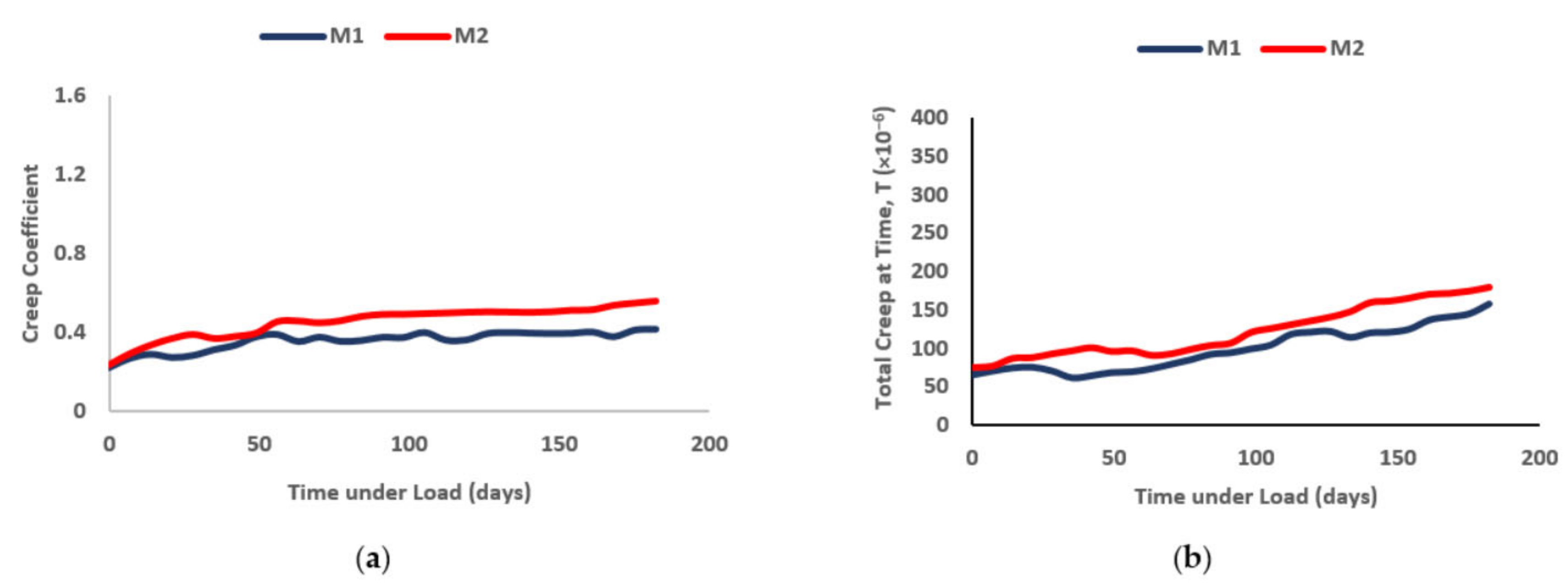
3.1. Creep Test Results
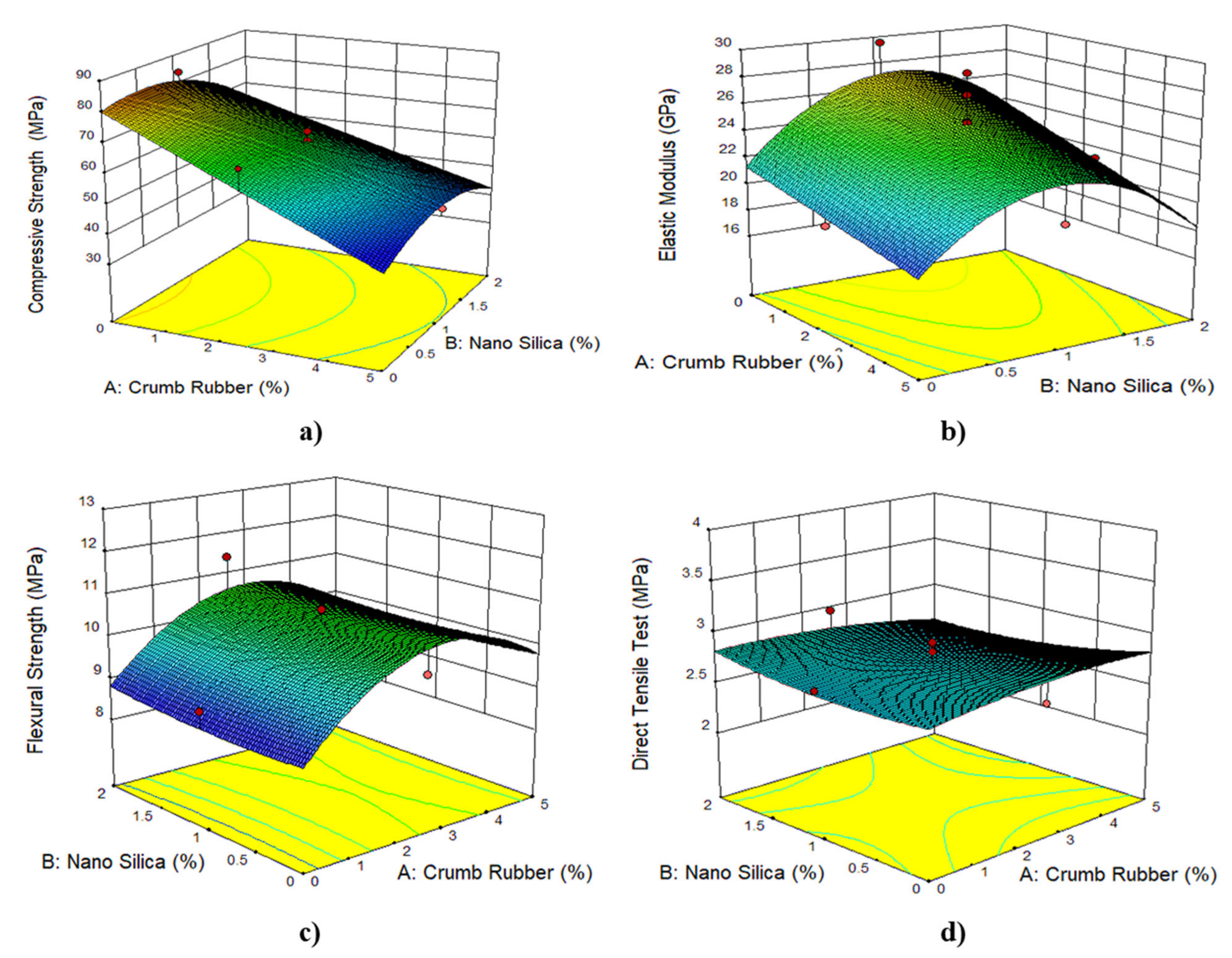
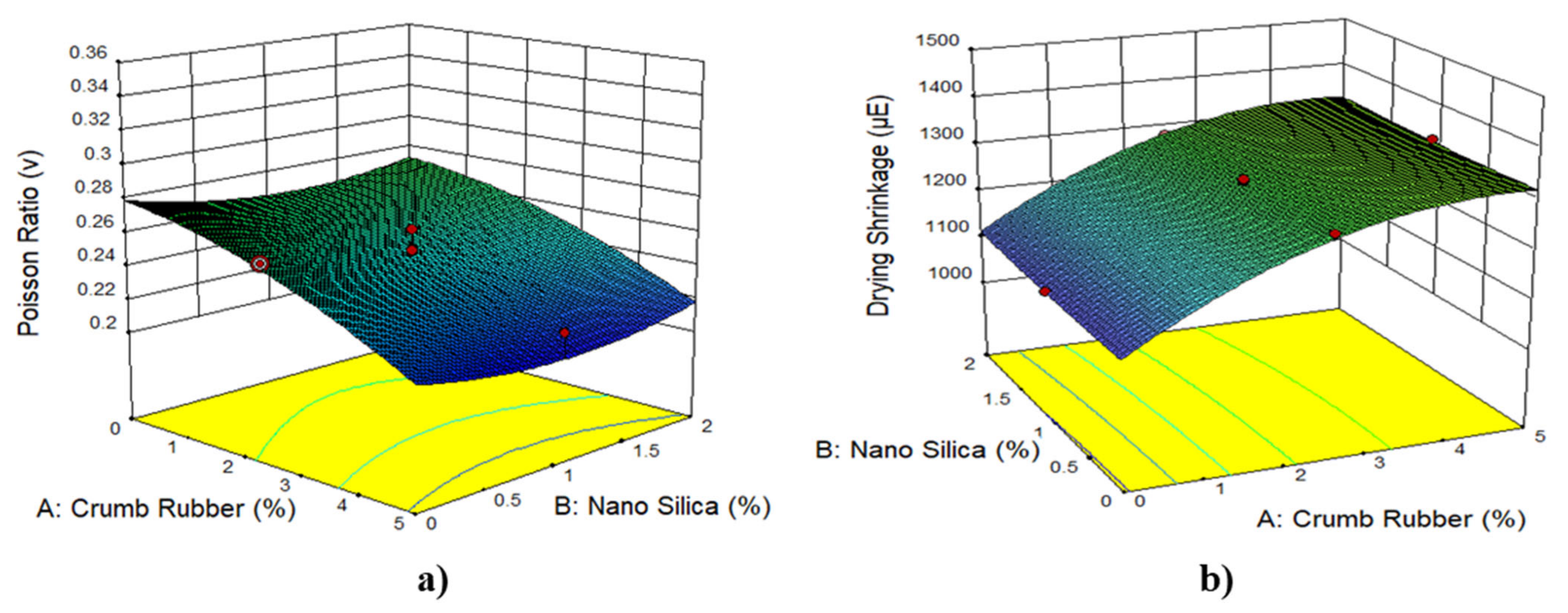
3.2. Response Surface Methodology (RSM)
3.3. Analysis of Variance (ANOVA)
3.4. Multi-Objective Optimization
3.5. Model Validation
4. Conclusions
- Incorporation of nano-silica can offset the adverse effects of crumb rubber content on the properties of rubberized ECC.
- The reduction in the elastic modulus of rubberized ECC is attributed to the elastic properties of the crumb rubber particles, which act as tiny springs inside the hardened cement matrix. However, this adverse effect has been offset by adding nano-silica which restricted the internal structure of the rubberized ECC.
- The developed quadratic equations can be used to predict the responses. All the responses are within the 5% significance level, where the probability value (p-value) is less than 0.05, clearly indicating that the models are significant with an outstanding 95% confidence level. The developed models using the RSM can provide reliable and accurate responses and thus can be used to predict the strength of the rubberized ECC containing NS.
- The optimum mixture of rubberized ECC mixture achieved using RSM is highly reliable.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Thomas, B.S.; Gupta, R.C.; Panicker, V.J. Recycling of waste tire rubber as aggregate in concrete: Durability-related performance. J. Clean. Prod. 2016, 112, 504–513. [Google Scholar] [CrossRef]
- Kumar, T. Waste Tyre Management in Malaysia. Ph.D. Thesis, Putra University, Seri Kembangan, Malaysia, 2006. [Google Scholar]
- Al-Fakih, A.; Mohammed, B.S.; Liew, M.S.; Alaloul, W.S.; Adamu, M.; Khed, V.C.; Dahim, M.A.; Al-Mattarneh, H. Mechanical behavior of rubberized interlocking bricks for masonry structural applications. Int. J. Civ. Eng. Technol. 2018, 9, 185–193. [Google Scholar]
- Bulei, C.; Todor, M.; Heput, T.; Kiss, I. Directions for material recovery of used tires and their use in the production of new products intended for the industry of civil construction and pavements. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018; p. 012064. [Google Scholar]
- Al-Fakih, A.; Mohammed, B.S.; Liew, M.S.; Alaloul, W.S. Physical properties of the rubberized interlocking masonry brick. Int. J. Civ. Eng. Technol. 2018, 9, 656–664. [Google Scholar]
- Mohammed, B.S. Structural behavior and m–k value of composite slab utilizing concrete containing crumb rubber. Constr. Build. Mater. 2010, 24, 1214–1221. [Google Scholar] [CrossRef]
- Khan, S.; Singh, A. Behavior of Crumb Rubber Concrete. Int. J. Res. Eng. 2018, 8, 86–92. [Google Scholar]
- Mohammed, B.S.; Awang, A.B.; Wong, S.S.; Nhavene, C.P. Properties of nano silica modified rubbercrete. J. Clean. Prod. 2016, 119, 66–75. [Google Scholar] [CrossRef]
- Li, G.; Garrick, G.; Eggers, J.; Abadie, C.; Stubblefield, M.A.; Pang, S.-S. Waste tire fiber modified concrete. Compos. Part B Eng. 2004, 35, 305–312. [Google Scholar] [CrossRef]
- Mohammed, B.S.; Anwar Hossain, K.M.; Eng Swee, J.T.; Wong, G.; Abdullahi, M. Properties of crumb rubber hollow concrete block. J. Clean. Prod. 2012, 23, 57–67. [Google Scholar] [CrossRef]
- Onuaguluchi, O.; Panesar, D.K. Hardened properties of concrete mixtures containing pre-coated crumb rubber and silica fume. J. Cleaner Prod. 2014, 82, 125–131. [Google Scholar] [CrossRef]
- Shu, X.; Huang, B. Recycling of waste tire rubber in asphalt and portland cement concrete: An overview. Constr. Build. Mater. 2014, 67, 217–224. [Google Scholar] [CrossRef]
- Demir, F.; Yesilata, B.; Turgut, P.; Bulut, H.; Isiker, Y. Investigation of the effects of pH, aging and scrap tire content on the dissolution behaviors of new scrap tire-concrete mixture structures. J. Clean. Prod. 2015, 93, 38–46. [Google Scholar] [CrossRef]
- Mohammed, B.S.; Adamu, M.; Shafiq, N. A review on the effect of crumb rubber on the properties of rubbercrete. Int. J. Civ. Eng. Technol. 2017, 8, 599–615. [Google Scholar]
- Al-Fakih, A.; Mohammed, B.S.; Wahab, M.M.A.; Liew, M.S.; Mugahed Amran, Y.H.; Alyousef, R.; Alabduljabbar, H. Characteristic compressive strength correlation of rubberized concrete interlocking masonry wall. Structures 2020, 26, 169–184. [Google Scholar] [CrossRef]
- Al-Fakih, A.; Wahab, M.M.A.; Mohammed, B.S.; Liew, M.S.; Wan Abdullah Zawawi, N.A.; As’ad, S. Experimental study on axial compressive behavior of rubberized interlocking masonry walls. J. Build. Eng. 2020, 29, 101107. [Google Scholar] [CrossRef]
- Chou, L.H.; Lu, C.-K.; Chang, J.-R.; Lee, M.T. Use of waste rubber as concrete additive. Waste Manag. Res. 2007, 25, 68–76. [Google Scholar] [CrossRef]
- Khed, V.C.; Mohammed, B.S.; Nuruddin, M.F. Effects of different crumb rubber sizes on the flowability and compressive strength of hybrid fibre reinforced ECC. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2018. [Google Scholar] [CrossRef]
- Kanda, T.; Li, V.C. New micromechanics design theory for pseudostrain hardening cementitious composite. J. Eng. Mech. 1999, 125, 373–381. [Google Scholar] [CrossRef] [Green Version]
- Maalej, M.; Quek, S.T.; Ahmed, S.F.U.; Zhang, J.; Lin, V.W.J.; Leong, K.S. Review of potential structural applications of hybrid fiber Engineered Cementitious Composites. Constr. Build. Mater. 2012, 36, 216–227. [Google Scholar] [CrossRef]
- Phan, L. Fire Performance of High Strength Concrete: A Report of the State-of-the-Art; National Institute of Standards and Technology, Office of Applied Economics, Building and Fire Research Laboratory: Gaithersburg, MD, USA, 1996. [Google Scholar]
- Anderberg, Y. Spalling phenomena of HPC and OC. In NIST Workshop on Fire Performance of High Strength Concrete in Gaithersburg; U.S. Government Printing Office: North Capitol, WA, USA, 1997; pp. 69–73. [Google Scholar]
- Phan, L.T.; Lawson, J.R.; Davis, F.L. Effects of elevated temperature exposure on heating characteristics, spalling, and residual properties of high performance concrete. Mater. Struct. 2001, 34, 83–91. [Google Scholar] [CrossRef]
- Şahmaran, M.; Özbay, E.; Yücel, H.E.; Lachemi, M.; Li, V.C. Effect of Fly Ash and PVA Fiber on Microstructural Damage and Residual Properties of Engineered Cementitious Composites Exposed to High Temperatures. J. Mater. Civ. Eng. 2011, 23, 1735–1745. [Google Scholar] [CrossRef]
- Hernández-Olivares, F.; Barluenga, G. Fire performance of recycled rubber-filled high-strength concrete. Cem. Concr. Res. 2004, 34, 109–117. [Google Scholar] [CrossRef]
- ASTM C150/C150M-19a, Standard Specification for Portland Cement; ASTM International: West Conshohocken, PA, USA, 2019.
- ASTM C618-19, Standard Specification for Coal Fly Ash and Raw or Calcined Natural Pozzolan for Use in Concrete; ASTM International: West Conshohocken, PA, USA, 2019.
- ASTM C136/C136M-14, Standard Test Method for Sieve Analysis of Fine and Coarse Aggregates; ASTM International: West Conshohocken, PA, USA, 2014.
- Adamu, M.; Mohammed, B.S.; Shahir Liew, M. Mechanical properties and performance of high volume fly ash roller compacted concrete containing crumb rubber and nano silica. Constr. Build. Mater. 2018, 171, 521–538. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments; John Wiley & Sons: Hoboken, NJ, USA, 2017. [Google Scholar]
- ASTM C109/C109M-16a, Standard Test Method for Compressive Strength of Hydraulic Cement Mortars (Using 2-in. or [50-mm] Cube Specimens); ASTM International: West Conshohocken, PA, USA, 2016.
- Rokugo, K. Recommendations for Design and Construction of High Performance Fiber Reinforced Cement Composites with Multiple Fine Cracks (HPFRCC); Japan Society of Civil Engineers, Gifu University: Gifu, Japan, 2008. [Google Scholar]
- ASTM C293/C293M-16, Standard Test Method for Flexural Strength of Concrete (Using Simple Beam with Center-Point Loading); ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM C469/C469M-14, Standard Test Method for Static Modulus of Elasticity and Poisson’s Ratio of Concrete in Compression. ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM C512/C512M-15, Standard Test Method for Creep of Concrete in Compression; ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM C490/C490M-17, Standard Practice for Use of Apparatus for the Determination of Length Change of Hardened Cement Paste, Mortar, and Concrete; ASTM International: West Conshohocken, PA, USA, 2017.
- Mohammed, B.S.; Fang, O.C.; Anwar Hossain, K.M.; Lachemi, M. Mix proportioning of concrete containing paper mill residuals using response surface methodology. Constr. Build. Mater. 2012, 35, 63–68. [Google Scholar] [CrossRef]

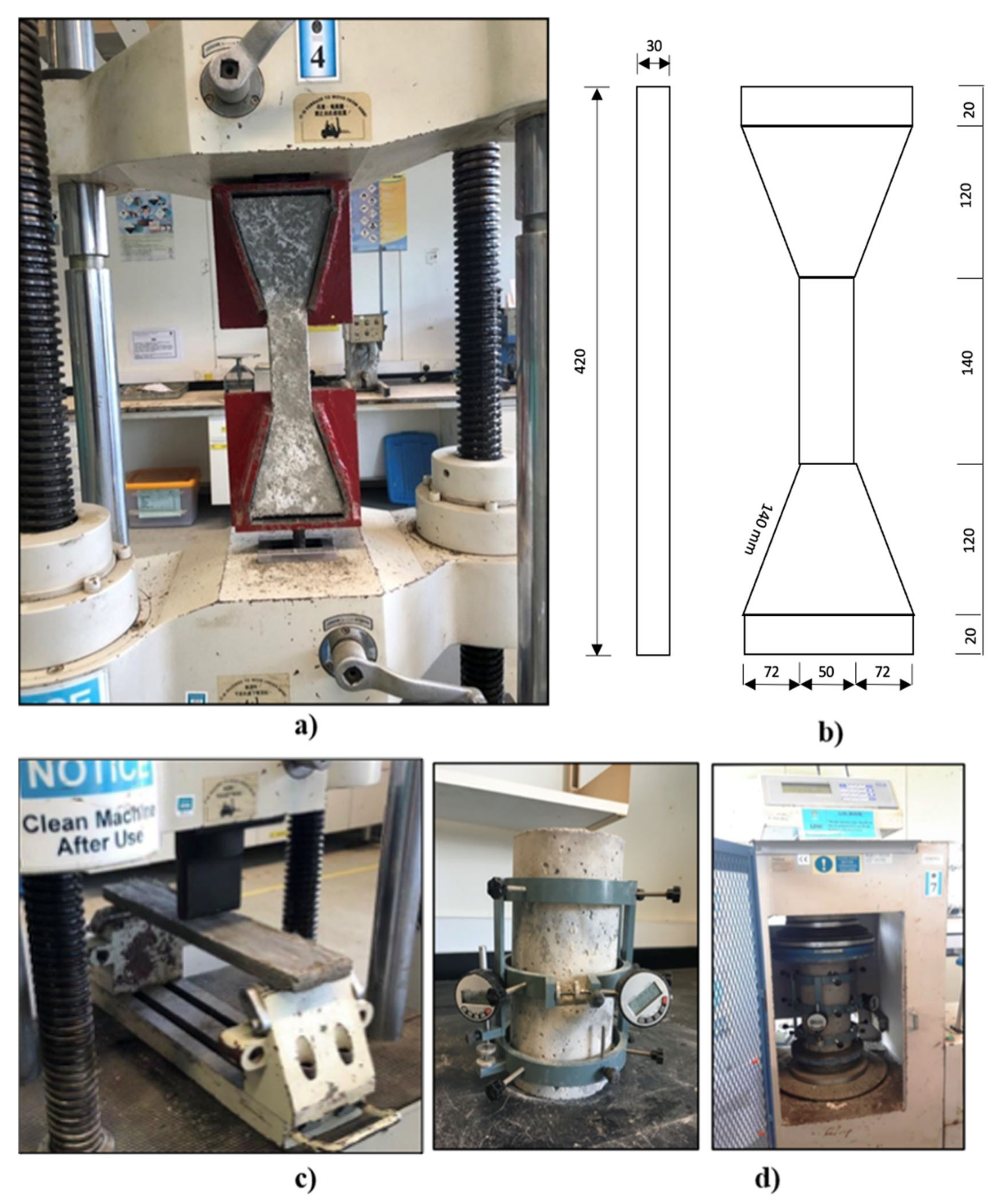



| Configuration | Cement (%) | Fly Ash (%) |
|---|---|---|
| SiO2 (%) | 25.21 | 58.35 |
| Al2O3 (%) | 4.59 | 20.96 |
| Fe2O3 (%) | 2.99 | 4.9 |
| CaO (%) | 62.85 | 9.79 |
| MgO (%) | 1.70 | 1.99 |
| Na2O (%) | 0.98 | 2.41 |
| K2O (%) | 1.68 | 1.60 |
| Specific gravity (g/cm3) | 3.15 | 2.38 |
| Loss on ignition (%) | 2.2 | 1.25 |
| Item | Unit | Quality |
|---|---|---|
| Appearance | - | High-dispersive white powder |
| Hear reduction | % | ≤3 |
| Ignition loss | % | ≤6 |
| SiO2 | % | ≥99.8 |
| Specific surface area | m2/g | 100 ± 25 |
| pH value | - | 6.5–7.5 |
| Surface density | g/mL | ≤0.15 |
| Dispensability (CCl4) | % | ≥80 |
| Oil-absorbed value | mL/100 g | ≥250 |
| Average particle size | nm | 10−25 |
| Hydrophobicity | - | Strong |
| Fiber Type | Specific Gravity (g/cm3) | Elastic Modulus (GPa) | Length (mm) | Diameter (μm) | Aspect Ratio (L/d) | Tensile Strength (MPa) |
|---|---|---|---|---|---|---|
| PVA Fiber | 1.3 | 41 | 12 | 40 | 462 | 1600 |
| Mix | Factors (%) | Quantities (kg/m3) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| w/cm | CR | FA | PVA | NS | OPC | FA | Fine Aggregate | CR | PVA | NS | Water | |
| M1 | 0.15 | 0 | 0 | 1 | 0 | 260.00 | 0.00 | 210.60 | 0.00 | 5.85 | 0.00 | 39.00 |
| M2 | 0.15 | 2.5 | 50 | 1 | 1 | 130.00 | 130.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M3 | 0.15 | 0 | 50 | 0 | 2 | 130.00 | 130.00 | 210.60 | 0.00 | 0.00 | 5.20 | 39.00 |
| M4 | 0.15 | 5 | 25 | 1 | 1 | 195.00 | 65.00 | 200.07 | 3.75 | 5.85 | 2.60 | 39.00 |
| M5 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M6 | 0.15 | 0 | 0 | 2 | 2 | 260.00 | 0.00 | 210.60 | 0.00 | 11.70 | 5.20 | 39.00 |
| M7 | 0.15 | 2.5 | 0 | 1 | 1 | 260.00 | 0.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M8 | 0.15 | 0 | 50 | 2 | 2 | 130.00 | 130.00 | 210.60 | 0.00 | 11.70 | 5.20 | 39.00 |
| M9 | 0.15 | 5 | 50 | 0 | 0 | 130.00 | 130.00 | 200.07 | 3.75 | 0.00 | 0.00 | 39.00 |
| M10 | 0.15 | 0 | 25 | 1 | 1 | 195.00 | 65.00 | 210.60 | 0.00 | 5.85 | 2.60 | 39.00 |
| M11 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M12 | 0.15 | 0 | 50 | 0 | 0 | 130.00 | 130.00 | 210.60 | 0.00 | 0.00 | 0.00 | 39.00 |
| M13 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M14 | 0.15 | 0 | 0 | 2 | 0 | 260.00 | 0.00 | 210.60 | 0.00 | 11.70 | 0.00 | 39.00 |
| M15 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M16 | 0.15 | 5 | 50 | 2 | 0 | 130.00 | 130.00 | 200.07 | 3.75 | 11.70 | 0.00 | 39.00 |
| M17 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M18 | 0.15 | 2.5 | 25 | 1 | 0 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 0.00 | 39.00 |
| M19 | 0.15 | 5 | 50 | 2 | 2 | 130.00 | 130.00 | 200.07 | 3.75 | 11.70 | 5.20 | 39.00 |
| M20 | 0.15 | 2.5 | 25 | 1 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 2.60 | 39.00 |
| M21 | 0.15 | 0 | 50 | 2 | 0 | 130.00 | 130.00 | 210.60 | 0.00 | 11.70 | 0.00 | 39.00 |
| M22 | 0.15 | 2.5 | 25 | 2 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 11.70 | 2.60 | 39.00 |
| M23 | 0.15 | 2.5 | 25 | 1 | 2 | 195.00 | 65.00 | 205.34 | 1.89 | 5.85 | 5.20 | 39.00 |
| M24 | 0.15 | 5 | 0 | 0 | 0 | 260.00 | 0.00 | 200.07 | 3.75 | 0.00 | 0.00 | 39.00 |
| M25 | 0.15 | 5 | 50 | 0 | 2 | 130.00 | 130.00 | 200.07 | 3.75 | 0.00 | 5.20 | 39.00 |
| M26 | 0.15 | 5 | 0 | 0 | 2 | 260.00 | 0.00 | 200.07 | 3.75 | 0.00 | 5.20 | 39.00 |
| M27 | 0.15 | 0 | 0 | 0 | 2 | 260.00 | 0.00 | 210.60 | 0.00 | 0.00 | 5.20 | 39.00 |
| M28 | 0.15 | 5 | 0 | 2 | 2 | 260.00 | 0.00 | 200.07 | 3.75 | 11.70 | 5.20 | 39.00 |
| M29 | 0.15 | 2.5 | 25 | 0 | 1 | 195.00 | 65.00 | 205.34 | 1.89 | 0.00 | 2.60 | 39.00 |
| M30 | 0.15 | 5 | 0 | 2 | 0 | 260.00 | 0.00 | 200.07 | 3.75 | 11.70 | 0.00 | 39.00 |
| Mix | Factors (%) | Responses | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| A: CR | B: NS | C: FA | D: PVA | CS (MPa) | ME (GPa) | DT (MPa) | DS (µE) | FS (MPa) | ||
| M1 | 0 | 0 | 0 | 1 | 87.17 | 21.92 | 0.31 | 2.63 | 1103.70 | 8.95 |
| M2 | 2.5 | 1 | 50 | 1 | 57.11 | 29.65 | 0.26 | 2.82 | 1267.80 | 10.75 |
| M3 | 0 | 2 | 50 | 0 | 69.91 | 27.14 | 0.34 | 3.13 | 1150.20 | 8.80 |
| M4 | 5 | 1 | 25 | 1 | 48.17 | 20.17 | 0.22 | 2.58 | 1323.90 | 9.50 |
| M5 | 2.5 | 1 | 25 | 1 | 64.29 | 25.26 | 0.26 | 2.70 | 1260.30 | 10.75 |
| M6 | 0 | 2 | 0 | 2 | 82.44 | 24.75 | 0.24 | 3.40 | 1204.50 | 10.20 |
| M7 | 2.5 | 1 | 0 | 1 | 65.05 | 28.78 | 0.27 | 2.76 | 1284.40 | 10.35 |
| M8 | 0 | 2 | 50 | 2 | 83.42 | 23.19 | 0.24 | 3.36 | 1203.70 | 9.95 |
| M9 | 5 | 0 | 50 | 0 | 52.44 | 21.67 | 0.21 | 2.91 | 1402.90 | 10.25 |
| M10 | 0 | 1 | 25 | 1 | 83.57 | 29.57 | 0.25 | 2.73 | 1101.30 | 9.01 |
| M11 | 2.5 | 1 | 25 | 1 | 65.22 | 27.21 | 0.24 | 2.91 | 1281.30 | 10.10 |
| M12 | 0 | 0 | 50 | 0 | 83.80 | 21.78 | 0.33 | 3.74 | 1124.80 | 8.89 |
| M13 | 2.5 | 1 | 25 | 1 | 60.45 | 22.90 | 0.24 | 2.82 | 1279.20 | 10.05 |
| M14 | 0 | 0 | 0 | 2 | 86.81 | 26.35 | 0.29 | 2.59 | 1203.50 | 9.87 |
| M15 | 2.5 | 1 | 25 | 1 | 63.07 | 28.78 | 0.25 | 2.50 | 1283.30 | 10.15 |
| M16 | 5 | 0 | 50 | 2 | 44.96 | 20.29 | 0.21 | 3.81 | 1401.30 | 11.71 |
| M17 | 2.5 | 1 | 25 | 1 | 68.45 | 25.18 | 0.25 | 2.72 | 1280.10 | 10.25 |
| M18 | 2.5 | 0 | 25 | 1 | 67.63 | 19.24 | 0.26 | 2.62 | 1281.30 | 9.95 |
| M19 | 5 | 2 | 50 | 2 | 54.86 | 20.11 | 0.21 | 3.81 | 1459.20 | 10.05 |
| M20 | 2.5 | 1 | 25 | 1 | 59.10 | 25.23 | 0.25 | 2.89 | 1254.10 | 10.25 |
| M21 | 0 | 0 | 50 | 2 | 80.36 | 23.32 | 0.24 | 2.99 | 1203.50 | 10.20 |
| M22 | 2.5 | 1 | 25 | 2 | 85.68 | 18.11 | 0.23 | 2.97 | 1361.10 | 12.20 |
| M23 | 2.5 | 2 | 25 | 1 | 48.69 | 21.21 | 0.25 | 2.97 | 1281.90 | 11.40 |
| M24 | 5 | 0 | 0 | 0 | 57.66 | 19.21 | 0.21 | 2.81 | 1399.80 | 9.95 |
| M25 | 5 | 2 | 50 | 0 | 48.93 | 18.61 | 0.23 | 2.70 | 1403.30 | 10.05 |
| M26 | 5 | 2 | 0 | 0 | 51.17 | 20.98 | 0.22 | 2.39 | 1402.40 | 10.70 |
| M27 | 0 | 2 | 0 | 0 | 69.55 | 23.04 | 0.35 | 2.92 | 1250.30 | 8.90 |
| M28 | 5 | 2 | 0 | 2 | 59.10 | 19.24 | 0.27 | 3.19 | 1401.90 | 11.35 |
| M29 | 2.5 | 1 | 25 | 0 | 81.63 | 22.37 | 0.24 | 3.12 | 1358.80 | 11.10 |
| M30 | 5 | 0 | 0 | 2 | 48.09 | 17.93 | 0.29 | 3.55 | 1424.30 | 10.990 |
| Response | Factors | Squares Sum | D.F. | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|---|
| Compressive strength | Model | 5108.15 | 14 | 364.87 | 16.36 | <0.0001 |
| A | 4169.69 | 1 | 4169.69 | 186.94 | <0.0001 | |
| B | 187.27 | 1 | 187.27 | 8.40 | 0.0110 | |
| C | 131.61 | 1 | 131.61 | 5.90 | 0.0282 | |
| D | 6.04 | 1 | 6.04 | 0.27 | 0.6105 | |
| AB | 230.35 | 1 | 230.35 | 10.33 | 0.0058 | |
| AC | 8.81 | 1 | 8.81 | 0.40 | 0.5391 | |
| AD | 9.81 | 1 | 9.81 | 0.44 | 0.5173 | |
| BC | 59.18 | 1 | 59.18 | 2.65 | 0.1241 | |
| BD | 294.85 | 1 | 294.85 | 13.22 | 0.0024 | |
| CD | 5.48 | 1 | 5.48 | 0.25 | 0.6274 | |
| A2 | 0.0055 | 1 | 0.0055 | 0.00025 | 0.9877 | |
| B2 | 156.03 | 1 | 156.03 | 7.00 | 0.0184 | |
| C2 | 59.79 | 1 | 59.79 | 2.68 | 0.1224 | |
| D2 | 655.05 | 1 | 655.05 | 29.37 | <0.0001 | |
| Lack of Fit | 277.67 | 10 | 27.77 | 2.44 | 0.0984 | |
| Elastic modulus | Model | 272.60 | 14 | 19.47 | 3.15 | <0.0001 |
| A | 78.20 | 1 | 78.20 | 12.65 | 0.0029 | |
| B | 6.47 | 1 | 6.47 | 1.05 | 0.3224 | |
| C | 3.43 | 1 | 3.43 | 0.56 | 0.4676 | |
| D | 0.18 | 1 | 0.18 | 0.028 | 0.8686 | |
| AB | 5.24 | 1 | 5.24 | 0.85 | 0.3718 | |
| AC | 0.017 | 1 | 0.017 | 0.0027 | 0.9592 | |
| AD | 3.65 | 1 | 3.65 | 0.59 | 0.4544 | |
| BC | 1.54 | 1 | 1.54 | 0.25 | 0.6250 | |
| BD | 2.94 | 1 | 2.94 | 0.47 | 0.5013 | |
| CD | 2.64 | 1 | 2.64 | 0.43 | 0.5234 | |
| A2 | 0.00096 | 1 | 0.00096 | 0.00016 | 0.9902 | |
| B2 | 57.78 | 1 | 57.78 | 9.35 | 0.0080 | |
| C2 | 49.70 | 1 | 49.70 | 8.04 | 0.0125 | |
| D2 | 30.42 | 1 | 30.42 | 4.92 | 0.0424 | |
| Lack of Fit | 72.46 | 10 | 7.25 | 1.79 | 0.2707 | |
| Poisson’s ratio | Model | 0.037 | 14 | 0.002659 | 16.31 | <0.0001 |
| A | 0.015 | 1 | 0.015000 | 91.89 | <0.0001 | |
| B | 0.000043 | 1 | 0.000043 | 0.26 | 0.6155 | |
| C | 0.002041 | 1 | 0.002041 | 12.52 | 0.0030 | |
| D | 0.002748 | 1 | 0.002748 | 16.86 | 0.0009 | |
| AB | 0.000039 | 1 | 0.000039 | 0.24 | 0.6332 | |
| AC | 0.000294 | 1 | 0.000294 | 1.80 | 0.1995 | |
| AD | 0.010 | 1 | 0.010000 | 63.48 | <0.0001 | |
| BC | 0.000311 | 1 | 0.000311 | 1.91 | 0.1876 | |
| BD | 0.000867 | 1 | 0.000867 | 5.32 | 0.0358 | |
| CD | 0.001820 | 1 | 0.001820 | 11.17 | 0.0045 | |
| A2 | 0.000248 | 1 | 0.000248 | 1.52 | 0.2360 | |
| B2 | 0.000294 | 1 | 0.000294 | 1.81 | 0.1989 | |
| C2 | 0.001141 | 1 | 0.001141 | 7.00 | 0.0183 | |
| D2 | 0.000117 | 1 | 0.000117 | 0.72 | 0.4104 | |
| Lack of Fit | 0.002191 | 10 | 0.000219 | 4.31 | 0.1603 | |
| Direct tensile strength | Model | 3.53 | 14 | 0.25 | 5.28 | <0.0001 |
| A | 0.0068 | 1 | 0.0068 | 0.14 | 0.7116 | |
| B | 0.0081 | 1 | 0.0081 | 0.17 | 0.6857 | |
| C | 0.3 | 1 | 0.3 | 6.32 | 0.0238 | |
| D | 0.36 | 1 | 0.36 | 7.44 | 0.0156 | |
| AB | 0.089 | 1 | 0.089 | 1.88 | 0.1910 | |
| AC | 0.0033 | 1 | 0.0033 | 0.070 | 0.7949 | |
| AD | 0.99 | 1 | 0.99 | 20.86 | 0.0004 | |
| BC | 0.0015 | 1 | 0.0015 | 0.031 | 0.8615 | |
| BD | 0.31 | 1 | 0.31 | 6.60 | 0.0214 | |
| CD | 0.0016 | 1 | 0.0016 | 0.034 | 0.8556 | |
| A2 | 0.011 | 1 | 0.011 | 0.23 | 0.6399 | |
| B2 | 0.015 | 1 | 0.015 | 0.32 | 0.5790 | |
| C2 | 0.013 | 1 | 0.013 | 0.28 | 0.6039 | |
| D2 | 0.34 | 1 | 0.34 | 7.18 | 0.0171 | |
| Lack of Fit | 0.60 | 10 | 0.060 | 2.56 | 0.1551 | |
| Drying shrinkage | Model | 279.40 | 14 | 19,957.70 | 61.52 | <0.0001 |
| A | 208.50 | 1 | 208.50 | 642.82 | <0.0001 | |
| B | 768.45 | 1 | 768.45 | 2.37 | 0.1446 | |
| C | 1195.33 | 1 | 1195.33 | 3.68 | 0.0741 | |
| D | 1271.57 | 1 | 1271.57 | 3.92 | 0.0664 | |
| AB | 107.75 | 1 | 107.75 | 0.33 | 0.5730 | |
| AC | 2626.96 | 1 | 2626.96 | 8.1 | 0.0123 | |
| AD | 0.15 | 1 | 0.15 | 0.000454 | 0.9833 | |
| BC | 138.08 | 1 | 138.08 | 0.43 | 0.5240 | |
| BD | 56.32 | 1 | 56.32 | 0.17 | 0.6828 | |
| CD | 2513.31 | 1 | 2513.31 | 7.75 | 0.0139 | |
| A2 | 11,086.53 | 1 | 11,086.53 | 34.17 | <0.0001 | |
| B2 | 50.78 | 1 | 50.78 | 0.16 | 0.6979 | |
| C2 | 3.23 | 1 | 3.23 | 0.009950 | 0.9219 | |
| D2 | 24,209.25 | 1 | 24,209.25 | 74.62 | <0.0001 | |
| Lack of Fit | 4084.39 | 10 | 408.44 | 2.61 | 0.1505 | |
| Flexural strength | Model | 272.60 | 14 | 19.47 | 3.15 | <0.0001 |
| A | 78.20 | 1 | 78.20 | 12.65 | 0.0029 | |
| B | 6.47 | 1 | 6.47 | 1.05 | 0.3224 | |
| C | 3.43 | 1 | 3.43 | 0.56 | 0.4676 | |
| D | 0.18 | 1 | 0.18 | 0.028 | 0.8686 | |
| AB | 5.24 | 1 | 5.24 | 0.85 | 0.3718 | |
| AC | 0.017 | 1 | 0.017 | 0.002700 | 0.9592 | |
| AD | 3.65 | 1 | 3.65 | 0.59 | 0.4544 | |
| BC | 1.54 | 1 | 1.54 | 0.25 | 0.6250 | |
| BD | 2.94 | 1 | 2.94 | 0.47 | 0.5013 | |
| CD | 2.64 | 1 | 2.64 | 0.43 | 0.5234 | |
| A2 | 0.000964 | 1 | 0.000964 | 0.000156 | 0.9902 | |
| B2 | 57.78 | 1 | 57.78 | 9.35 | 0.0080 | |
| C2 | 49.70 | 1 | 49.70 | 8.04 | 0.0125 | |
| D2 | 30.42 | 1 | 30.42 | 4.92 | 0.0424 | |
| Lack of Fit | 72.46 | 10 | 7.25 | 1.79 | 0.2707 |
| Model | Compressive Strength | Modulus Elasticity | Poisson’s Ratio | Direct Tensile | Dry Shrinkage | Flexural Strength |
|---|---|---|---|---|---|---|
| R2 | 0.939 | 0.946 | 0.938 | 0.931 | 0.983 | 0.944 |
| Adj. R2 | 0.881 | 0.909 | 0.881 | 0.874 | 0.967 | 0.898 |
| Pred R2 | 0.696 | 0.284 | 0.591 | 0.086 | 0.863 | 0.201 |
| AP | 14.62 | 6.73 | 15.93 | 9.11 | 27.11 | 10.44 |
| S.D. | 4.72 | 2.49 | 0.013 | 0.218 | 18.01 | 0.461 |
| μ | 65.96 | 23.11 | 0.255 | 2.97 | 1287.9 | 10.22 |
| C.V.% | 7.16 | 10.76 | 5.01 | 7.36 | 1.40 | 4.52 |
| Factors and Responses | Notation | Target | Lower Limit | Upper Limit |
|---|---|---|---|---|
| Crumb rubber, % | A | In range | 0 | 5 |
| Nano-silica, % | B | In range | 0 | 2 |
| Fly ash, % | C | In range | 0 | 50 |
| PVA fiber, % | D | In range | 0 | 2 |
| Compressive strength, MPa | CS | Target | 50 | 50 |
| Flexural strength, MPa | FS | Maximize | 8.8 | 12.2 |
| Direct tensile strength, MPa | DT | Maximize | 2.394 | 3.812 |
| Elastic modulus, GPa | ME | Maximize | 17.93 | 29.65 |
| Poisson’s ratio | Minimize | 0.2089 | 0.347 | |
| Drying shrinkage, E | DS | Minimize | 1101.29 | 1459.24 |
| CR, % | NS, % | FA, % | PVA Fiber, % | CS, MPa | FS, MPa | DT, MPa | ME, GPa | DS, μE | Desirability, % | |
|---|---|---|---|---|---|---|---|---|---|---|
| 3.49 | 1.345 | 42.14 | 1.245 | 50.00 | 10.601 | 2.941 | 22.062 | 0.240 | 1301.16 | 1.0 |
| 4.43 | 1.085 | 43.51 | 1.079 | 49.10 | 10.059 | 2.853 | 25.973 | 0.218 | 1323.86 | 1.0 |
| 4.70 | 0.118 | 47.03 | 1.975 | 48.80 | 11.352 | 3.574 | 19.91 | 0.222 | 1427.21 | 1.0 |
| CR, % | NS, % | FA, % | PVA Fiber, % | Results & Error | CS, MPa | FS, MPa | DT, MPa | ME, GPa | DS, μE | |
|---|---|---|---|---|---|---|---|---|---|---|
| 3.49 | 1.345 | 42.14 | 1.245 | Predicted | 50.00 | 10.601 | 2.941 | 22.062 | 0.240 | 1301.16 |
| Experimental | 47.2 | 10.38 | 2.665 | 20.38 | 0.231 | 1226.96 | ||||
| Error, % | 5.60 | 2.08 | 9.38 | 7.62 | 3.75 | 5.70 | ||||
| 4.437 | 1.085 | 43.51 | 1.079 | Predicted | 49.1 | 10.059 | 2.853 | 25.973 | 0.218 | 1323.86 |
| Experimental | 45.76 | 9.62 | 2.748 | 23.61 | 0.200 | 1209.64 | ||||
| Error, % | 6.8 | 4.36 | 3.68 | 9.09 | 8.25 | 8.627 | ||||
| 4.703 | 0.118 | 47.03 | 1.975 | Predicted | 48.8 | 11.352 | 3.574 | 19.91 | 0.222 | 1427.21 |
| Experimental | 46.22 | 10.91 | 3.341 | 19.37 | 0.204 | 1349.77 | ||||
| Error, % | 5.28 | 3.89 | 6.52 | 2.71 | 8.10 | 5.43 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Loganathan, R.; Mohammed, B.S. Properties of Rubberized Engineered Cementitious Composites Containing Nano-Silica. Materials 2021, 14, 3765. https://doi.org/10.3390/ma14133765
Loganathan R, Mohammed BS. Properties of Rubberized Engineered Cementitious Composites Containing Nano-Silica. Materials. 2021; 14(13):3765. https://doi.org/10.3390/ma14133765
Chicago/Turabian StyleLoganathan, Rubendran, and Bashar S. Mohammed. 2021. "Properties of Rubberized Engineered Cementitious Composites Containing Nano-Silica" Materials 14, no. 13: 3765. https://doi.org/10.3390/ma14133765
APA StyleLoganathan, R., & Mohammed, B. S. (2021). Properties of Rubberized Engineered Cementitious Composites Containing Nano-Silica. Materials, 14(13), 3765. https://doi.org/10.3390/ma14133765







