A FEM-Based Optimization Method for Driving Frequency of Contactless Magnetoelastic Torque Sensors in Steel Shafts
Abstract
:1. Introduction
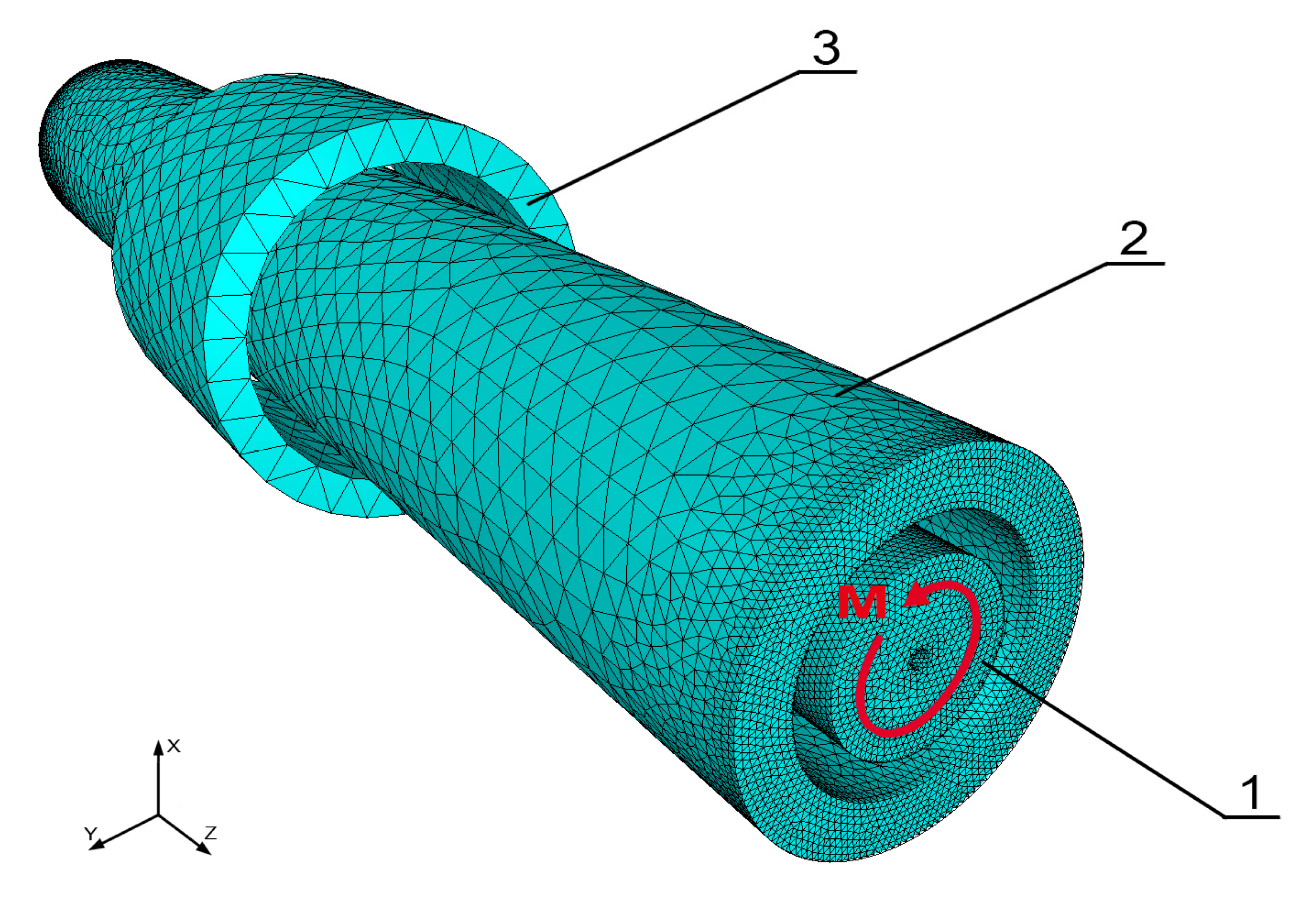
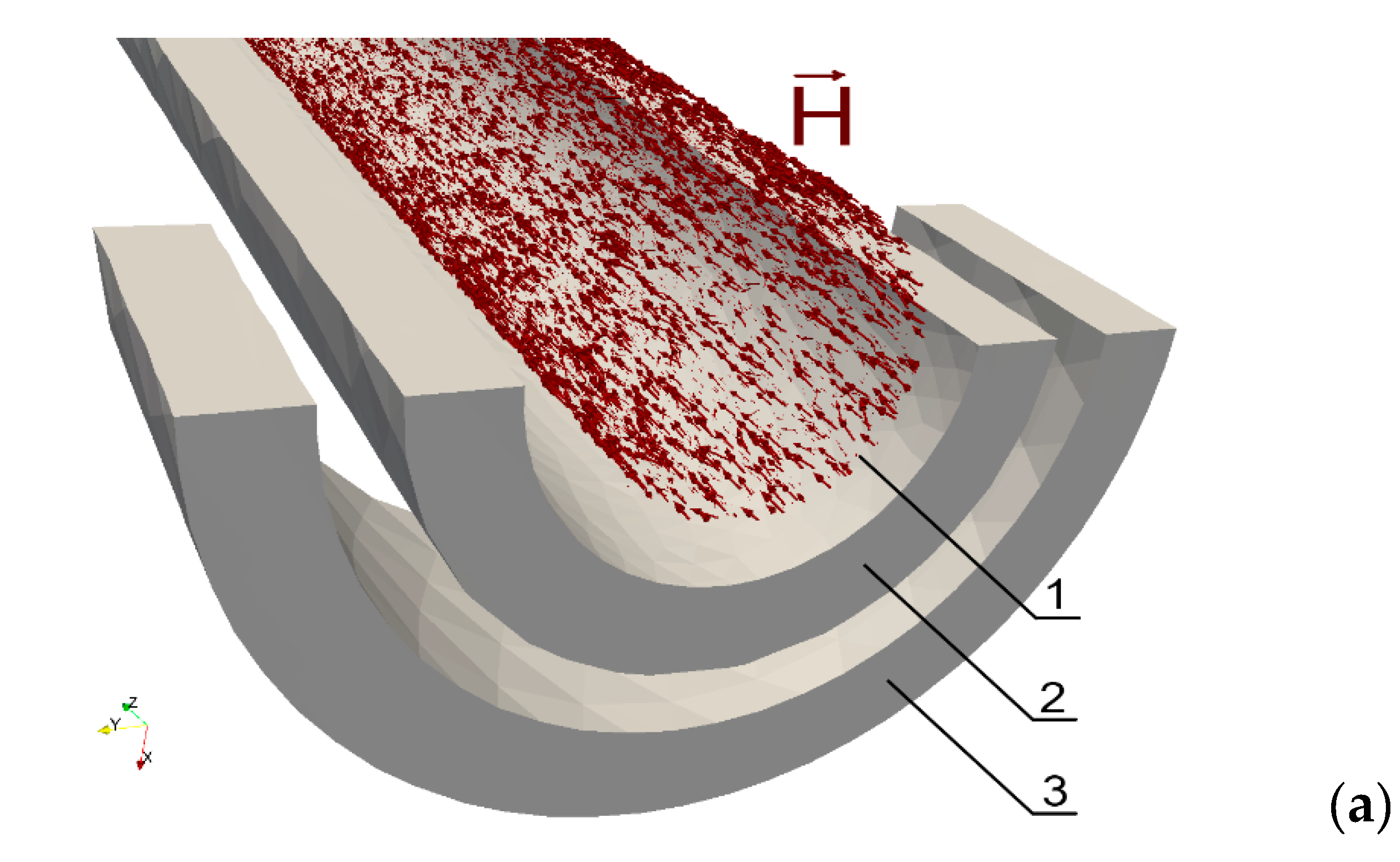
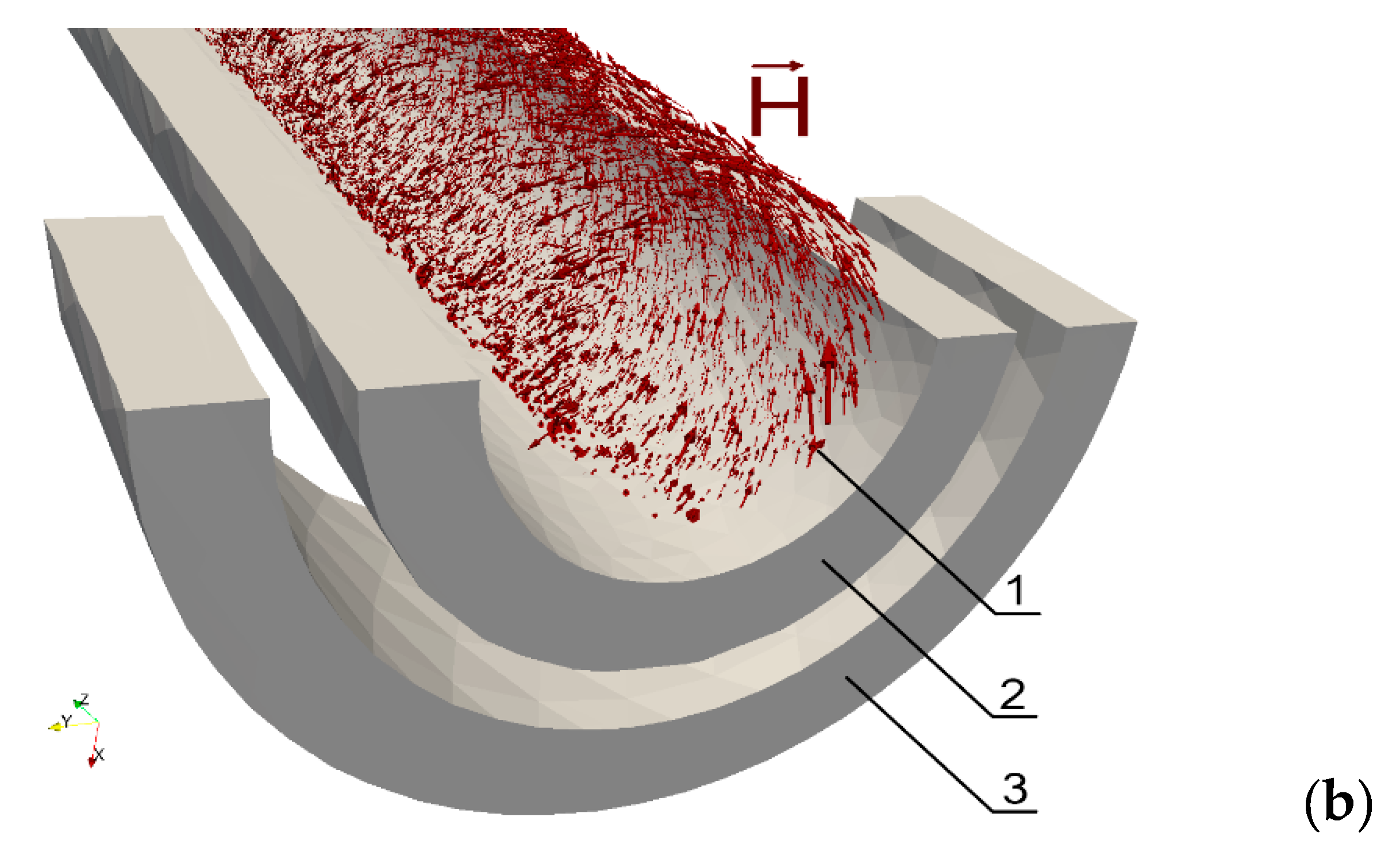
2. Finite Element Model for Development of Contactless Torque Sensors Utilizing Ferromagnetic Construction Steels
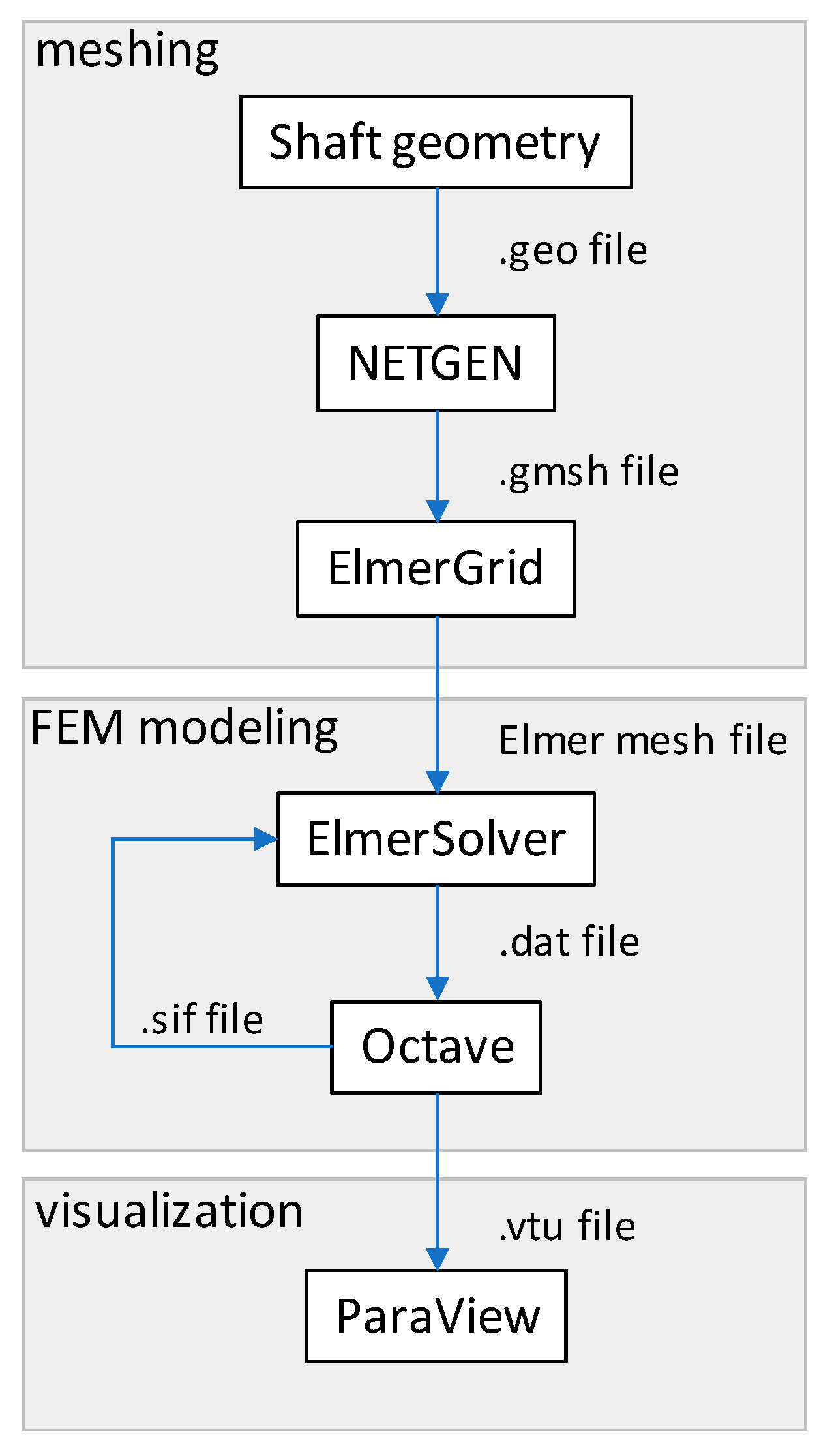
3. Implementation of Proposed Model in Open-Source FEM-Oriented Software
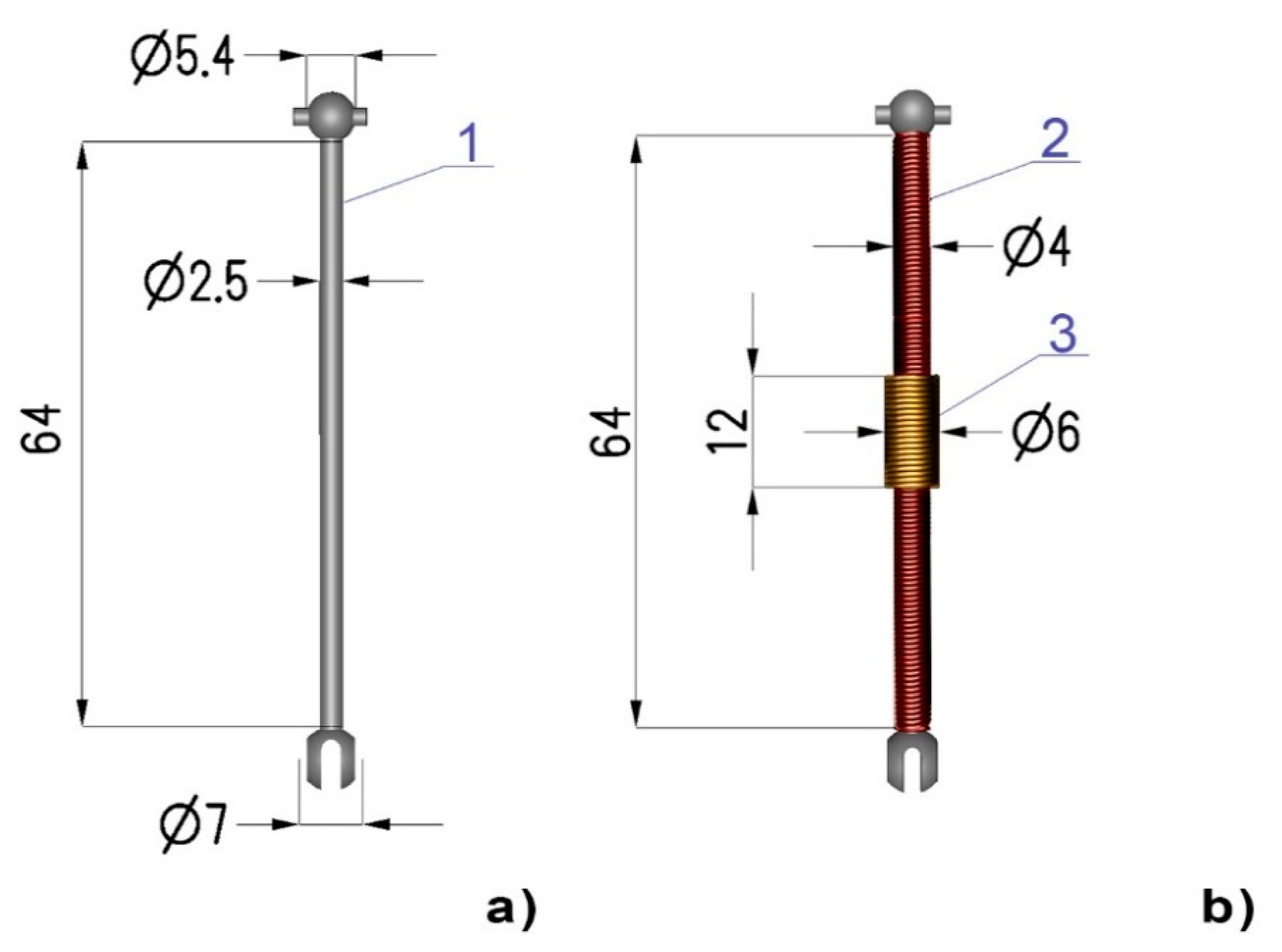
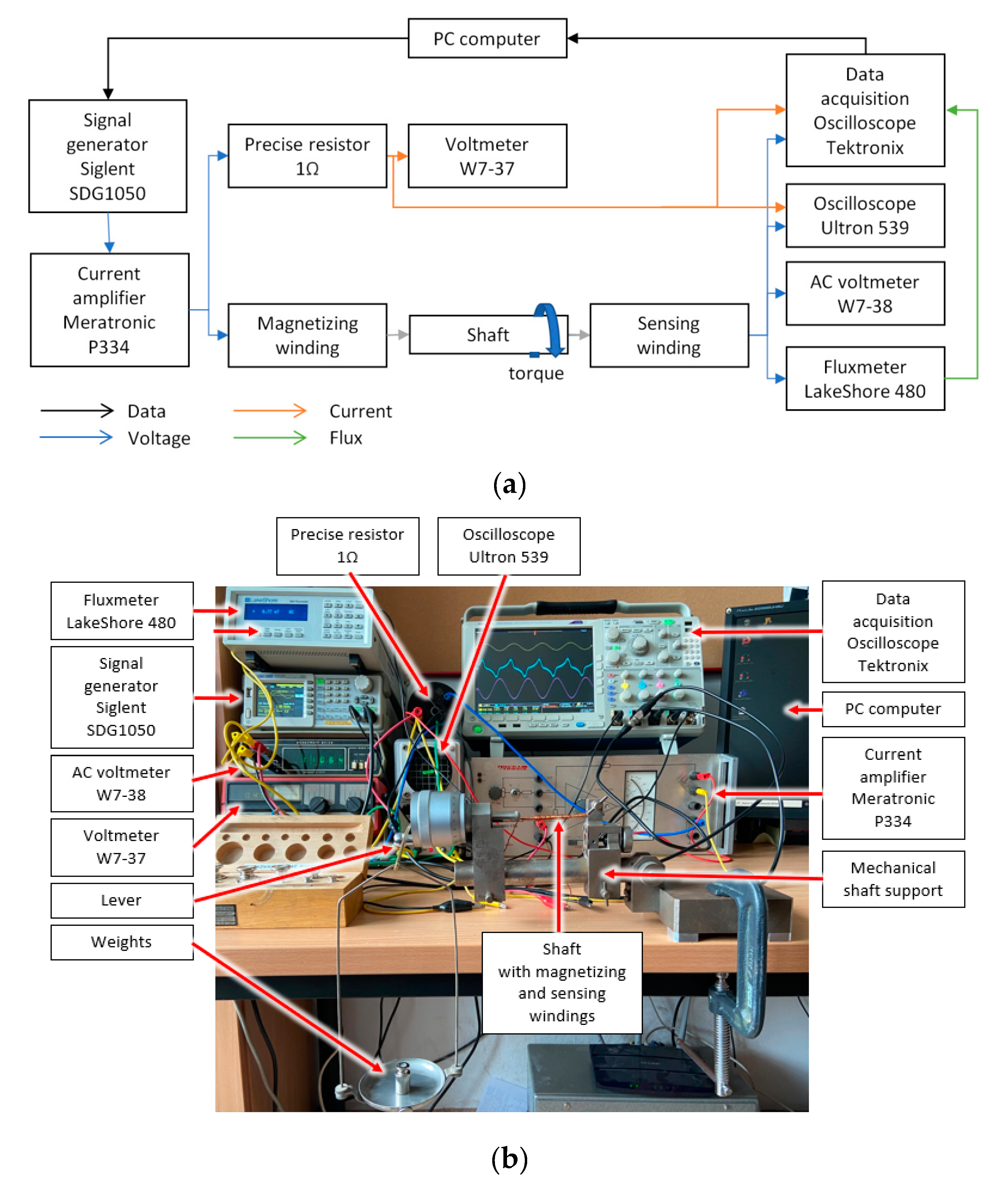
4. Experimental Validation of Proposed Model
- -
- SDG1050 signal generator (Siglent, Helmond, The Netherlands);
- -
- P334 current amplifier (Meratronic, Warsaw, Poland);
- -
- Precise resistor 1 kΩ (INCO, Warsaw, Poland);
- -
- Voltmeter W7-37 (Petersburg, Russia);
- -
- Fluxmeter LakeShore 480 (Lake Shore Cryotronics, Inc., Westerville, OH, USA);
- -
- AC voltmeter W7-38 (Leningrad, USSR);
- -
- Oscilloscope Ultron 539 (Munchen, Germany);
- -
- Oscilloscope Tektronix MDO4024C (Beaverton, OR, USA);
- -
- PC computer (X-KOM, Warsaw, Poland).
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Džapo, H.; Stare, Z.; Bobanac, N. Digital Measuring System for Monitoring Motor Shaft Parameters on Ships. IEEE Trans. Instrum. Meas. 2009, 58, 3702–3712. [Google Scholar] [CrossRef]
- Bonisławski, M.; Hołub, M.; Borkowski, T.; Kowalak, P. A Novel Telemetry System for Real Time, Ship Main Propulsion Power Measurement. Sensors 2019, 19, 4771. [Google Scholar] [CrossRef] [Green Version]
- Hammons, T.J.; Chanal, L. Measurement of torque in steam turbine-generator shafts following severe disturbances on the electrical supply system-analysis and implementation. IEEE Trans. Energy Convers. 1991, 6, 193–203. [Google Scholar] [CrossRef]
- Zhang, H.; Wenske, J.; Reuter, A.; Neshati, M. Proposals for a practical calibration method for mechanical torque measurement on the wind turbine drive train under test on a test bench. Wind Energy 2020, 23, 1048–1062. [Google Scholar] [CrossRef]
- Zhang, H.; De Luna, R.O.; Pilas, M.; Wenske, J. A study of mechanical torque measurement on the wind turbine drive train-ways and feasibilities. Wind Energy 2018, 21, 1406–1422. [Google Scholar] [CrossRef]
- Khan, H.; D’Imperio, M.; Cannella, F.; Caldwell, D.G.; Cuschieri, A.; Semini, C. Towards Scalable Strain Gauge-Based Joint Torque Sensors. Sensors 2017, 17, 1905. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Joubair, A.; Zhao, L.F.; Bigras, P.; Bonev, I.A. Use of a Force-Torque Sensor for Self-Calibration of a 6-DOF Medical Robot. Sensors 2016, 16, 798. [Google Scholar] [CrossRef] [Green Version]
- Tang, T.; Lin, H.-C.; Zhao, Y.; Chen, W.; Tomizuka, M. Autonomous alignment of peg and hole by force/torque measurement for robotic assembly. In Proceedings of the 2016 IEEE International Conference on Automation Science and Engineering (CASE), Fort Worth, TX, USA, 21–25 August 2016. [Google Scholar]
- O’Neill, J.J.; Stephens, T.K.; Kowalewski, T.M. Evaluation of Torque Measurement Surrogates as Applied to Grip Torque and Jaw Angle Estimation of Robotic Surgical Tools. IEEE Robot. Autom. Lett. 2018, 3, 3027–3034. [Google Scholar] [CrossRef]
- Jung, B.-J.; Kim, B.; Koo, J.C.; Choi, H.R.; Moon, H. Joint Torque Sensor Embedded in Harmonic Drive Using Order Tracking Method for Robotic Application. IEEE/ASME Trans. Mechatron. 2017, 22, 1594–1599. [Google Scholar] [CrossRef]
- Fleming, W. Automotive torque measurement: A summary of seven different methods. In Proceedings of the 32nd IEEE Vehicular Technology Conference, San Diego, CA, USA, 23–26 March 1982; pp. 71–78. [Google Scholar]
- Gao, B.; Chen, H.; Ma, Y.; Sanada, K. Design of nonlinear shaft torque observer for trucks with Automated Manual Transmission. Mechatronics 2011, 21, 1034–1042. [Google Scholar] [CrossRef]
- Kim, W.; Kang, C.M.; Son, Y.-S.; Chung, C.C. Nonlinear Steering Wheel Angle Control Using Self-Aligning Torque with Torque and Angle Sensors for Electrical Power Steering of Lateral Control System in Autonomous Vehicles. Sensors 2018, 18, 4384. [Google Scholar] [CrossRef] [Green Version]
- Bitar, S.; Probst, J.S.; Garshelis, I.J. Development of a Magnetoelastic Torque Sensor for Formula 1 and CHAMP Car Racing Applications. SAE Tech. Pap. Ser. 2000, 1, 85. [Google Scholar] [CrossRef]
- Stahl, K.; Höhn, B.-R.; Otto, H.-P.; Bäumler, R.; Hasl, C. Electro Mechanically Actuated Torque Sensing Multi-Disc. Clutch for Automatic Transmissions–Electro Mechanic Powershift Clutch; Springer: Berlin, Germany, 2013; pp. 85–104. [Google Scholar]
- Zhao, Z.; Chen, H.; Yang, Y. Fuzzy Determination of Target Shifting Time and Torque Control of Shifting Phase for Dry Dual Clutch Transmission. Math. Probl. Eng. 2014, 2014, 347490. [Google Scholar] [CrossRef]
- Zhao, Z.-G.; Chen, H.-J.; Yang, Y.-Y.; He, L. Torque coordinating robust control of shifting process for dry dual clutch transmission equipped in a hybrid car. Veh. Syst. Dyn. 2015, 53, 1269–1295. [Google Scholar] [CrossRef]
- Petreus, D.; Farca, C.; Dobra, P.; Moga, D. Torque measurement system design. In Proceedings of the 2008 IEEE International Conference on Automation, Quality and Testing, Robotics, Pasadena, CA, USA, 19–23 May 2008; pp. 174–177. [Google Scholar]
- Jin, H.; Lu, W.-Y. Strain Measurement of Microsamples Using Laser Interferometry. In Proceedings of the ASME 2006 International Mechanical Engineering Congress and Exposition, Chicago, IL, USA, 5–10 November 2006; pp. 563–567. [Google Scholar]
- Zupan, M.; Hemker, K.J. Application of fourier analysis to the laser based interferometric strain/displacement gage. Exp. Mech. 2002, 42, 214–220. [Google Scholar] [CrossRef] [Green Version]
- Zi, X.; Geng, S.; Zhao, S.; Shu, F. Measurement of short shaft power based on a digital speckle correlation method. Meas. Sci. Technol. 2015, 26, 045001. [Google Scholar] [CrossRef]
- Zi, X.; Geng, S.; Zhao, S.; Wang, Y.; Qin, W. Research on Principle of Saft Dynamic Torque Measurement Based on Digital Industrial Photogrammetry Technology. Chin. J. Lasers 2015, 42, 208002. [Google Scholar] [CrossRef]
- Kruger, L.; Swart, P.L.; Chtcherbakov, A.A.; van Wyk, A.J. Non-contact torsion sensor using fibre Bragg gratings. Meas. Sci. Technol. 2004, 15, 1448–1452. [Google Scholar] [CrossRef]
- Wang, Y.; Liang, L.; Yuan, Y.; Xu, G.; Liu, F. A Two Fiber Bragg Gratings Sensing System to Monitor the Torque of Rotating Shaft. Sensors 2016, 16, 138. [Google Scholar] [CrossRef] [Green Version]
- Silva, D.; Mendes, J.C.; Pereira, A.B.; Gégot, F.; Alves, L.N. Measuring Torque and Temperature in a Rotating Shaft Using Commercial SAW Sensors. Sensors 2017, 17, 1547. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bieńkowski, A.; Szewczyk, R.; Salach, J. Industrial Application of Magnetoelastic Force and Torque Sensors. Acta Phys. Pol. A 2010, 118, 1008–1009. [Google Scholar] [CrossRef]
- Bieńkowski, A.; Szewczyk, R. The possibility of utilizing the high permeability magnetic materials in construction of magnetoelastic stress and force sensors. Sens. Actuators A Phys. 2004, 113, 270–276. [Google Scholar] [CrossRef]
- Dahle, O. The pressductor and the torductor—Two heavy-duty transducers based on magnetic stress sensitivity. IEEE Trans. Commun. Electron. 1964, 83, 752–758. [Google Scholar] [CrossRef]
- Salach, J.; Bieńkowski, A.; Szewczyk, R. The ring-shaped magnetoelastic torque sensors utilizing soft amorphous magnetic materials. J. Magn. Magn. Mater. 2007, 316, e607–e609. [Google Scholar] [CrossRef]
- Jackiewicz, D. Stress assessment in steel truss structures on the basis of magnetoelastic effects. Adv. Mechatron. Solut. 2016, 393, 467–472. [Google Scholar]
- Jackiewicz, D.; Kachniarz, M.; Bieńkowski, A.; Szewczyk, R. Possibilities of Application of the Magnetoelastic Effect for Stress Assessment in Construction Elements Made of Steel Considering Rayleigh Region. Adv. Intell. Syst. Comput. 2017, 543, 689–697. [Google Scholar] [CrossRef]
- Jackiewicz, D.; Nowicki, M.; Szewczyk, R.; Bienkowski, A.; Kachniarz, M.; Salach, J.; Kaminski, M. Application of Magnetoelastic Effects for Stress Assesment and Risk Mitigation in Constructions. J. Eng. Stud. Res. 2015, 21, 65–70. [Google Scholar] [CrossRef]
- Bieńkowski, A. Magnetoelastic Villari effect in Mn–Zn ferrites. J. Magn. Magn. Mater. 2000, 215–216, 231–233. [Google Scholar] [CrossRef]
- Dapino, M.; Smith, R.; Flatau, A. Structural magnetic strain model for magnetostrictive transducers. IEEE Trans. Magn. 2000, 36, 545–556. [Google Scholar] [CrossRef] [Green Version]
- Ostaszewska-Liżewska, A.; Szewczyk, R.; Råback, P.; Malinen, M. Modelling the Characteristics of Ring-Shaped Magnetoelastic Force Sensor in Mohri’s Configuration. Sensors 2020, 20, 266. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Szewczyk, R. Generalization of the Model of Magnetoelastic Effect: 3D Mechanical Stress Dependence of Magnetic Permeability Tensor in Soft Magnetic Materials. Materials 2020, 13, 4070. [Google Scholar] [CrossRef]
- Aydin, U.; Rasilo, P.; Martin, F.; Belahcen, A.; Daniel, L.; Haavisto, A.; Arkkio, A. Effect of multi-axial stress on iron losses of electrical steel sheets. J. Magn. Magn. Mater. 2019, 469, 19–27. [Google Scholar] [CrossRef]
- Sablik, M. Modeling stress dependence of magnetic properties for NDE of steels. Nondestruct. Test. Eval. 1989, 5, 49–65. [Google Scholar] [CrossRef]
- Kaido, C.; Hirose, N.; Iwasa, S.; Hayashi, T.; Waki, Y. Stress Dependence of Magnetic Properties in Non-oriented Electrical Steel Sheets. J. Magn. Soc. Jpn. 2010, 34, 140–145. [Google Scholar] [CrossRef]
- Timoshenko, S.; Goodier, J.N. Theory of Elasticity; McGraw-Hill: New York, NY, USA, 1970. [Google Scholar]
- Mohri, K. Review on recent advances in the field of amorphous-metal sensors and transducers. IEEE Trans. Magn. 1984, 20, 942–947. [Google Scholar] [CrossRef]
- Kwun, H. Investigation of the dependence of Barkhausen noise on stress and the angle between the stress and magnetization directions. J. Magn. Magn. Mater. 1985, 49, 235–240. [Google Scholar] [CrossRef]
- Stuelpnagel, J. On the Parametrization of the Three-Dimensional Rotation Group. SIAM Rev. 1964, 6, 422–430. [Google Scholar] [CrossRef] [Green Version]
- Available online: https://linuxmint.com/ (accessed on 8 April 2020).
- GNU Octave (Version 5.2.0). Available online: https://octave.org/doc/v5.2.0/ (accessed on 25 April 2020).
- Netgen/NGSolve. Available online: https://ngsolve.org/ (accessed on 23 November 2020).
- Elmer. Available online: https://www.csc.fi/web/elmer (accessed on 1 November 2020).
- Paraview. Available online: https://www.paraview.org/ (accessed on 1 November 2020).
- Salach, J.; Bienskowski, A.; Szewczyk, R. Magnetoelastic, ring-shaped torque sensors with the uniform stress distribution. J. Autom. Mob. Robot. Intell. Syst. 2013, 1, 66–68. [Google Scholar]
- Nowak, P.; Nowicki, M.; Juś, A.; Szewczyk, R. Utilization of Eddy Current Tomography in Automotive Industry. Acta Phys. Pol. A 2017, 131, 1168–1170. [Google Scholar] [CrossRef]
- Silveyra, J.M.; Garrido, J.M.C. On the modelling of the anhysteretic magnetization of homogeneous soft magnetic materials. J. Magn. Magn. Mater. 2021, in press. [Google Scholar]



| Torque [Nm] | 0 | 1.3 | ||
|---|---|---|---|---|
| Frequency [Hz] | Voltage [mV] | Flux Density [mT] | Voltage [mV] | Flux Density [mT] |
| 50 | 11.2 | 101.57 | 9.54 | 86.6 |
| 60 | 13.29 | 100.59 | 11.38 | 85.91 |
| 70 | 15.36 | 99.55 | 13.17 | 85.24 |
| 80 | 17.38 | 98.63 | 14.9 | 84.6 |
| 90 | 19.39 | 97.79 | 16.68 | 83.92 |
| 100 | 21.37 | 96.94 | 18.4 | 83.28 |
| 120 | 25.24 | 95.42 | 21.78 | 82.07 |
| 140 | 29 | 93.93 | 25 | 80.88 |
| 160 | 32.64 | 92.47 | 28.2 | 79.71 |
| 180 | 36.16 | 91.06 | 31.3 | 78.59 |
| 200 | 39.57 | 89.7 | 34.2 | 77.48 |
| 250 | 47.6 | 86.5 | 41.4 | 74.98 |
| 300 | 55.25 | 83.63 | 48.1 | 72.63 |
| 350 | 62.37 | 81.01 | 54.44 | 70.52 |
| 400 | 69.12 | 78.64 | 60.42 | 68.55 |
| 450 | 75.49 | 76.43 | 66.1 | 66.73 |
| 500 | 81.57 | 74.37 | 71.5 | 65.04 |
| 600 | 92.8 | 70.69 | 81.6 | 61.98 |
| 700 | 103.26 | 67.45 | 90.9 | 59.26 |
| 800 | 112.8 | 64.57 | 99.54 | 56.82 |
| 900 | 121.7 | 61.99 | 107.5 | 54.62 |
| 1000 | 130.1 | 59.66 | 115 | 52.64 |
| 1200 | 145.5 | 55.64 | 128.8 | 49.16 |
| 1400 | 159.5 | 52.28 | 141.2 | 46.22 |
| 1600 | 172.3 | 49.44 | 152.6 | 43.72 |
| 1800 | 184.3 | 47.02 | 163.2 | 41.57 |
| 2000 | 195.6 | 44.92 | 173.2 | 39.7 |
| 2500 | 221.9 | 40.74 | 196.1 | 35.96 |
| 3000 | 245.7 | 37.6 | 217 | 33.14 |
| 3500 | 268 | 35.15 | 236.5 | 30.94 |
| 4000 | 289 | 33.18 | 254.6 | 29.176 |
| 4500 | 309.3 | 31.55 | 272.2 | 27.72 |
| 5000 | 328.7 | 30.18 | 289 | 26.49 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ostaszewska-Liżewska, A.; Nowicki, M.; Szewczyk, R.; Malinen, M. A FEM-Based Optimization Method for Driving Frequency of Contactless Magnetoelastic Torque Sensors in Steel Shafts. Materials 2021, 14, 4996. https://doi.org/10.3390/ma14174996
Ostaszewska-Liżewska A, Nowicki M, Szewczyk R, Malinen M. A FEM-Based Optimization Method for Driving Frequency of Contactless Magnetoelastic Torque Sensors in Steel Shafts. Materials. 2021; 14(17):4996. https://doi.org/10.3390/ma14174996
Chicago/Turabian StyleOstaszewska-Liżewska, Anna, Michał Nowicki, Roman Szewczyk, and Mika Malinen. 2021. "A FEM-Based Optimization Method for Driving Frequency of Contactless Magnetoelastic Torque Sensors in Steel Shafts" Materials 14, no. 17: 4996. https://doi.org/10.3390/ma14174996
APA StyleOstaszewska-Liżewska, A., Nowicki, M., Szewczyk, R., & Malinen, M. (2021). A FEM-Based Optimization Method for Driving Frequency of Contactless Magnetoelastic Torque Sensors in Steel Shafts. Materials, 14(17), 4996. https://doi.org/10.3390/ma14174996







