Modeling of the Effective Permittivity of Open-Cell Ceramic Foams Inspired by Platonic Solids
Abstract
:1. Introduction
- Relations, such as the EMA relations [4,5] or probability distribution relations of the micromechanical bounds (e.g., Wiener bounds) [4], have been derived. Phenomenologically, these relations consider inclusions to be spherical (or quasi-spherical, e.g., ellipsoids) particles that are embedded in a continuous medium. Reliable predictions can only be obtained if the real microstructure resembles features of the one from which the relation had been derived.
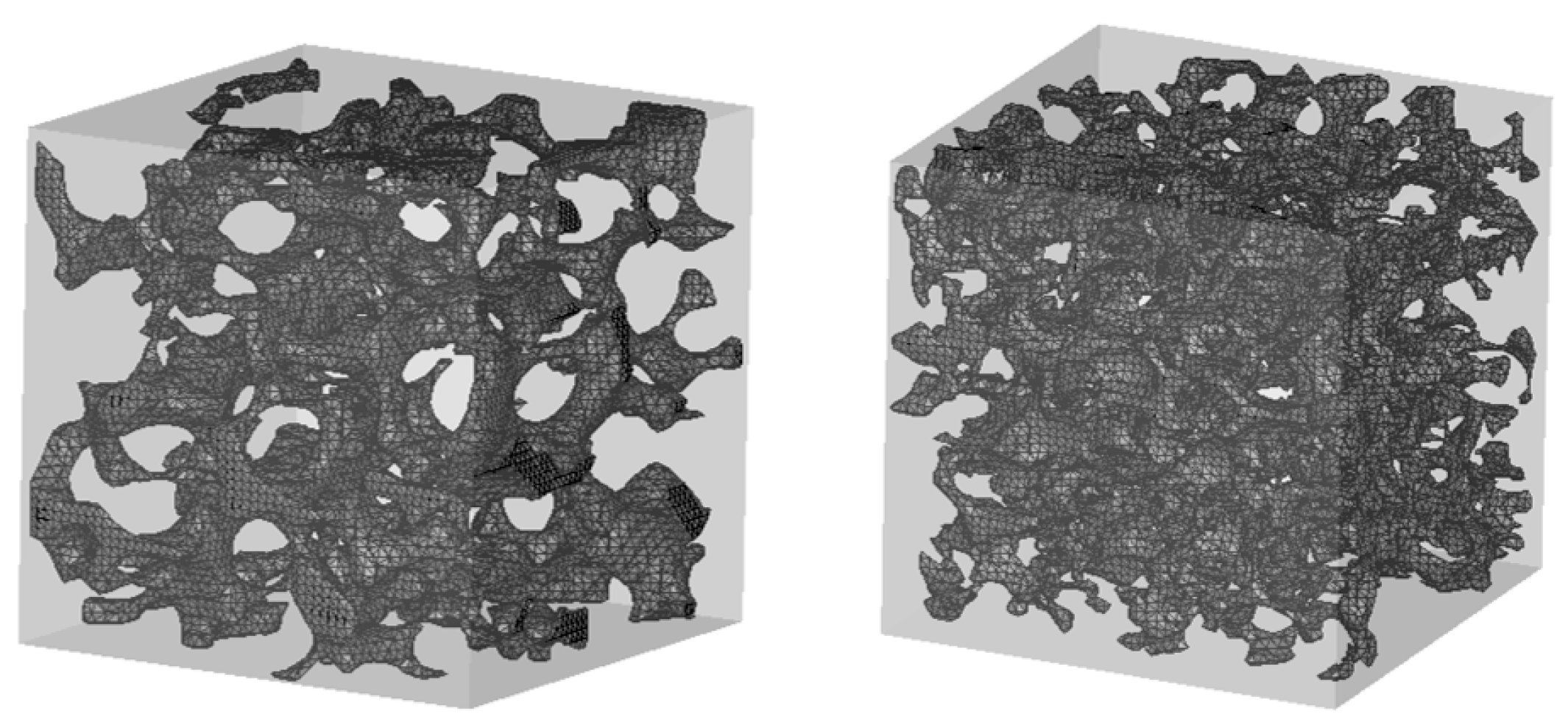
- Numerical approaches consider the real 3D structure of the skeleton, which is, for example, reconstructed from tomographic scans. The real foam structures are subsequently recreated in a 3D simulation environment [6] to help perform numerical electromagnetic calculations to obtain their scattering parameters [7] and the corresponding . Here, the accuracy depends on the spatial resolution of the scanned 3D volume and the refinement of the simulation mesh. The superiority of this method for estimating the comes at the expense of imaging and simulation costs.
2. Materials and Methods
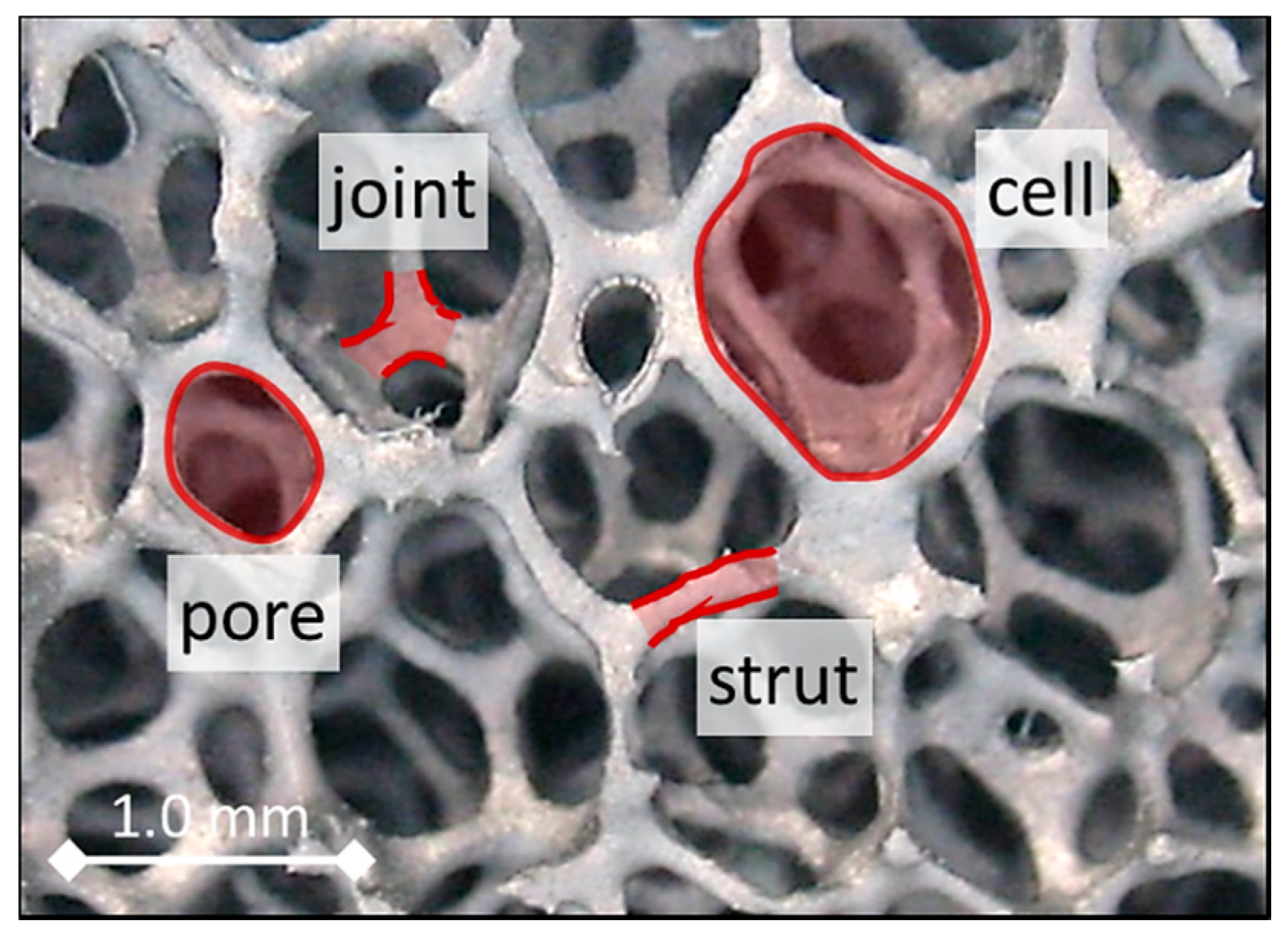
2.1. Sintered Open-Cell Ceramic Foams
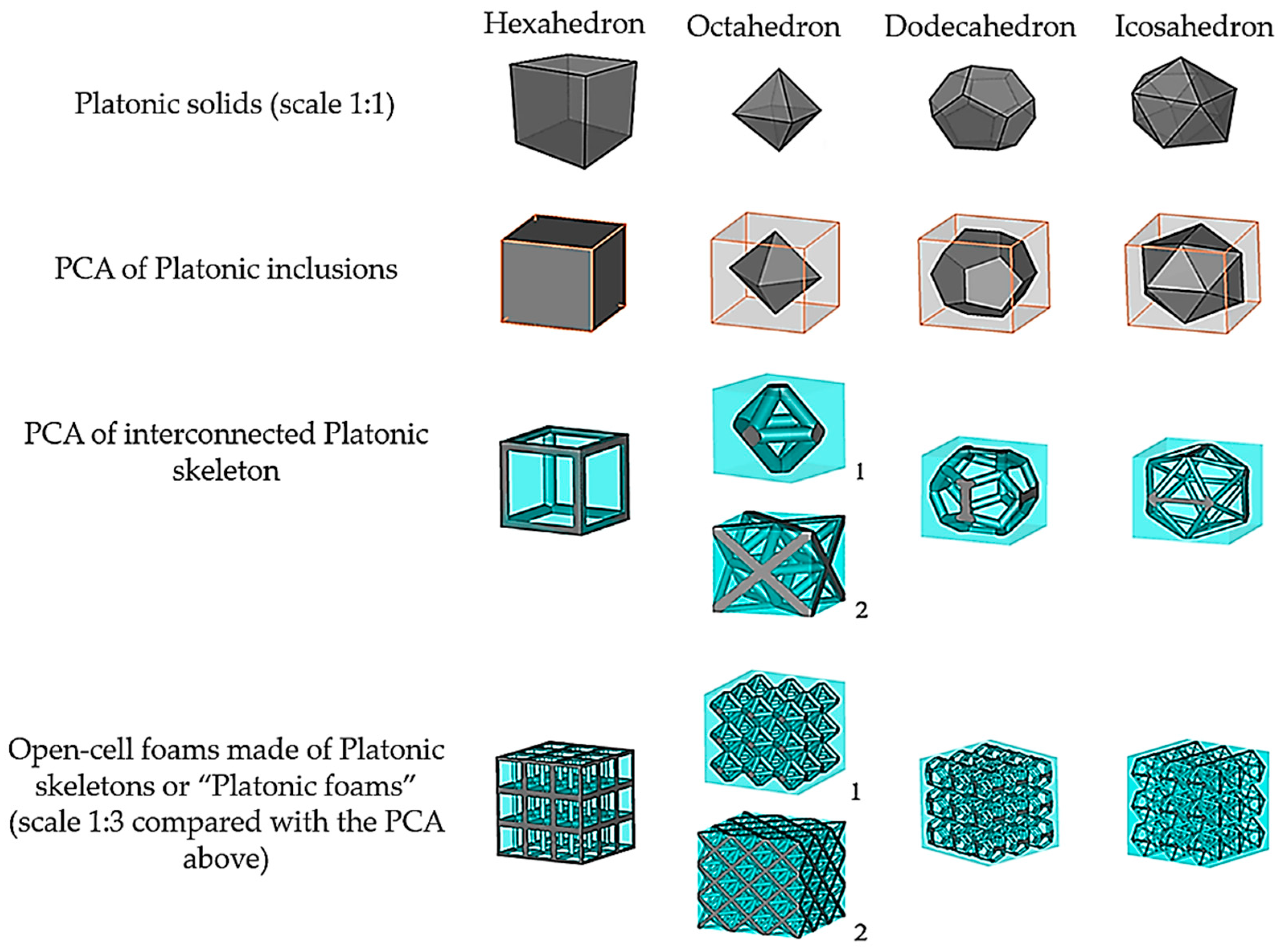
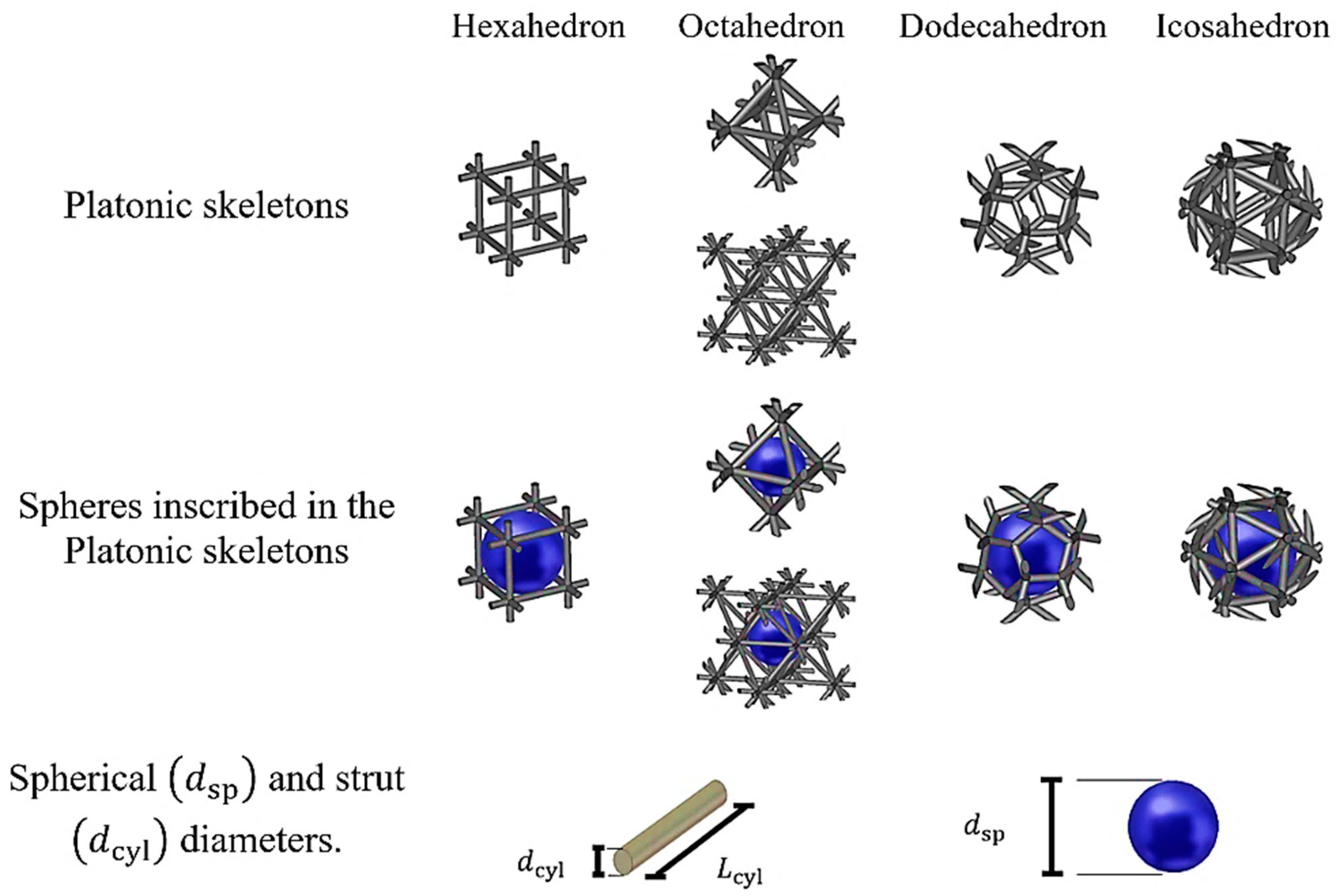
2.2. Open-Cell Foams Modeled with Platonic Skeletons
2.3. Calculation of the Effective Permittivities
- with ;
- with ;
- with ;
- with (corresponding to the air permittivity);
- with (as an alternative to air).
2.4. Expressions for Discussion of Results
- Skeleton: with , continuous medium: with.
- Skeleton: with , continuous medium: with.
- Skeleton: with , continuous medium: with.
- Skeleton: with , continuous medium: with.
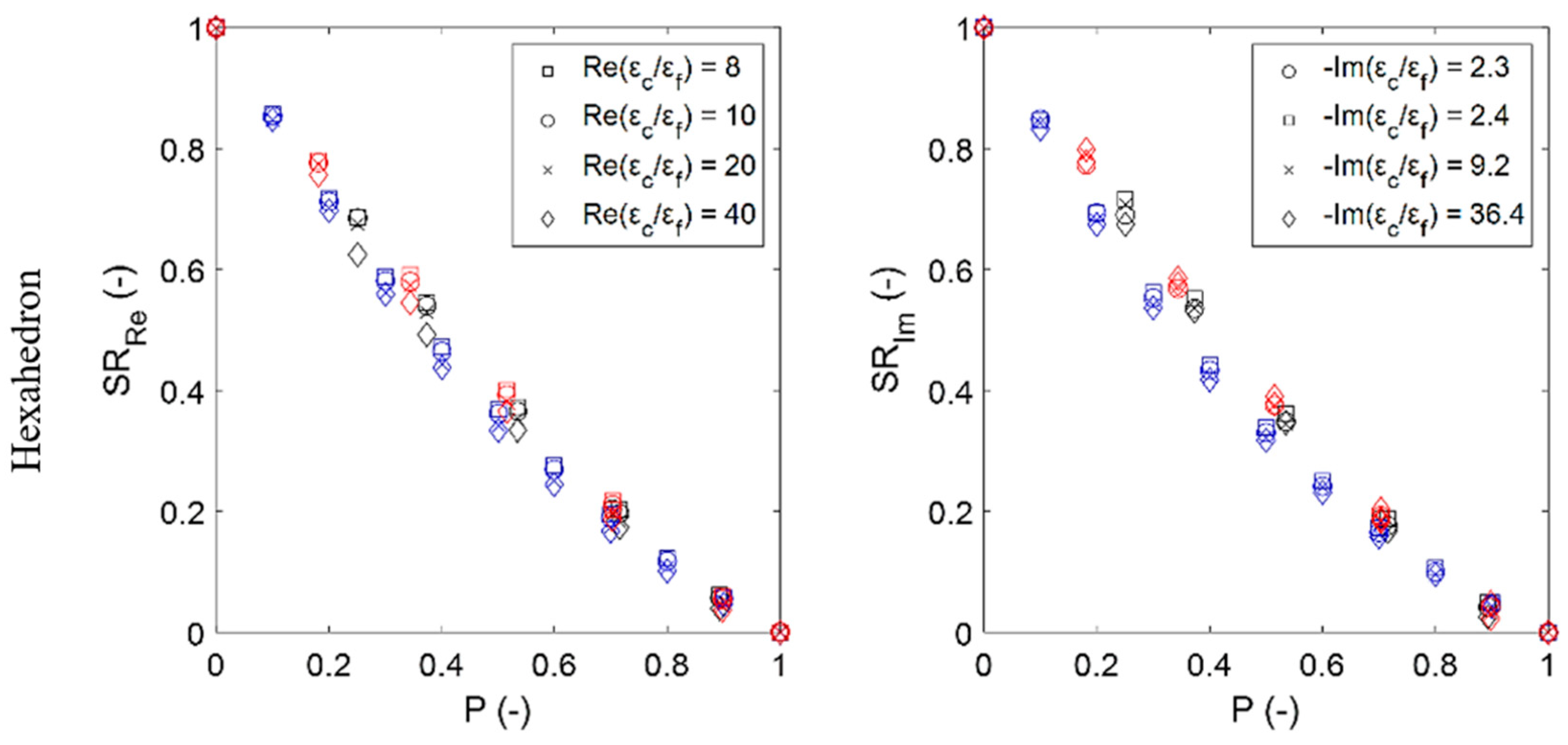
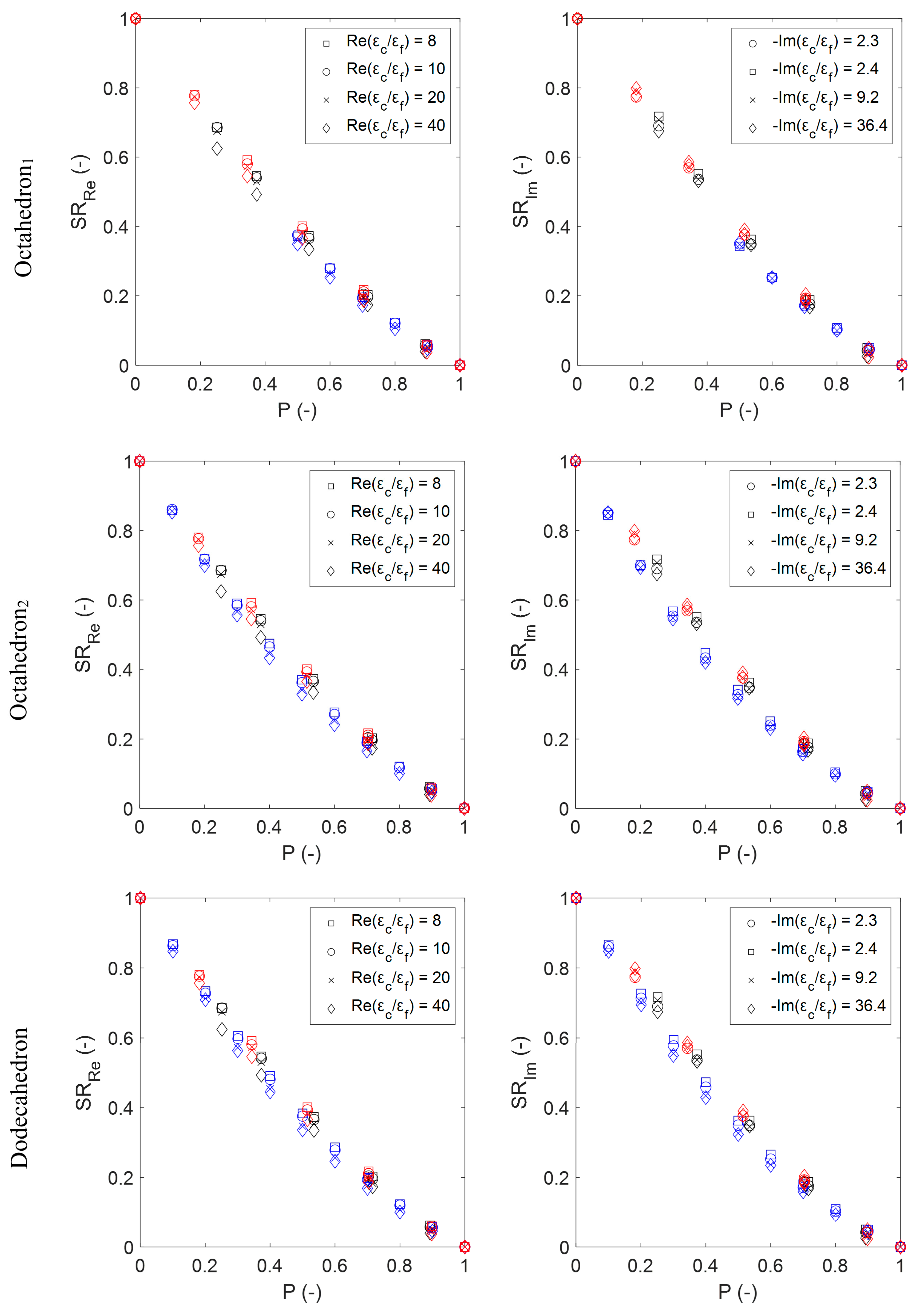
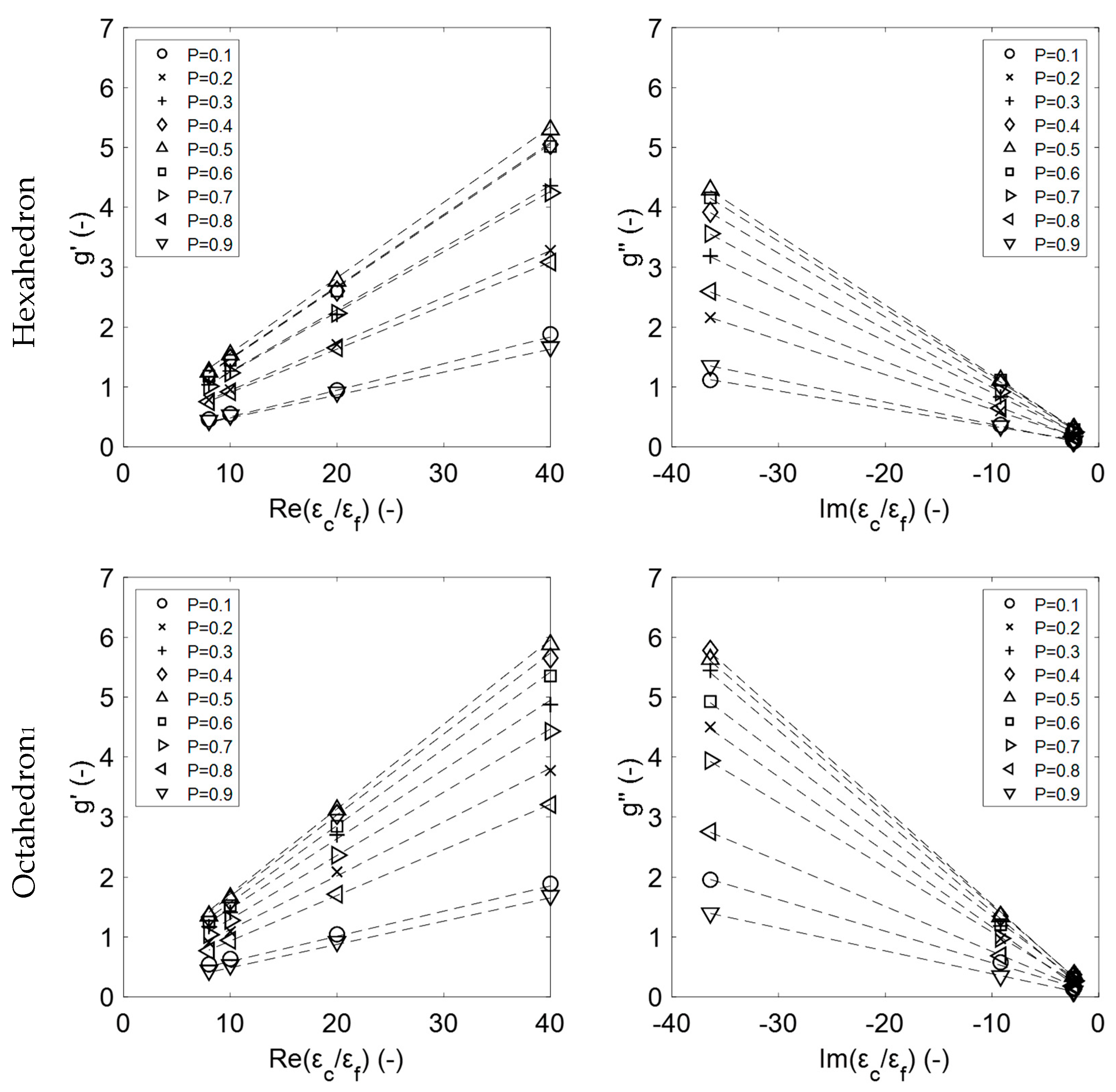
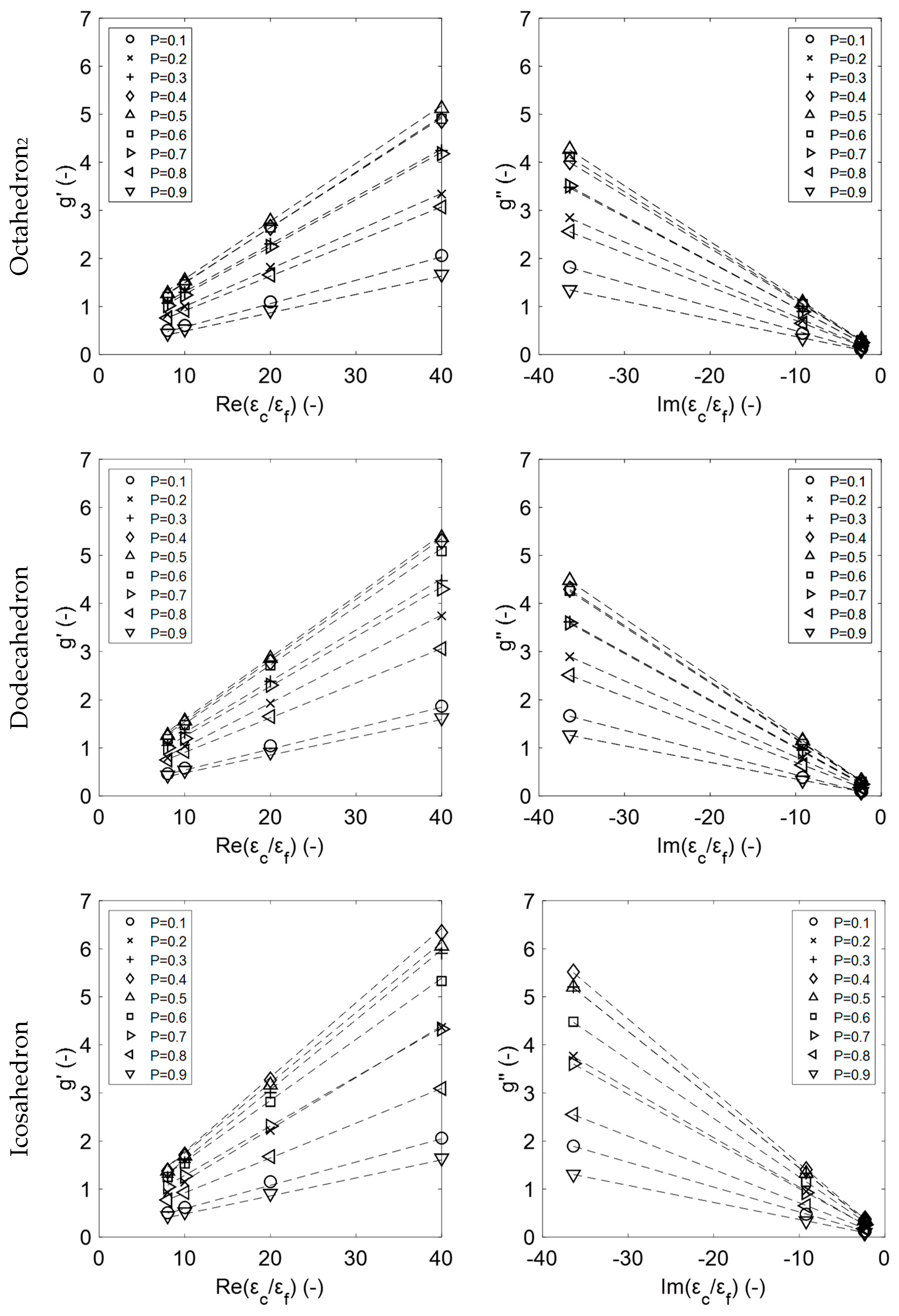
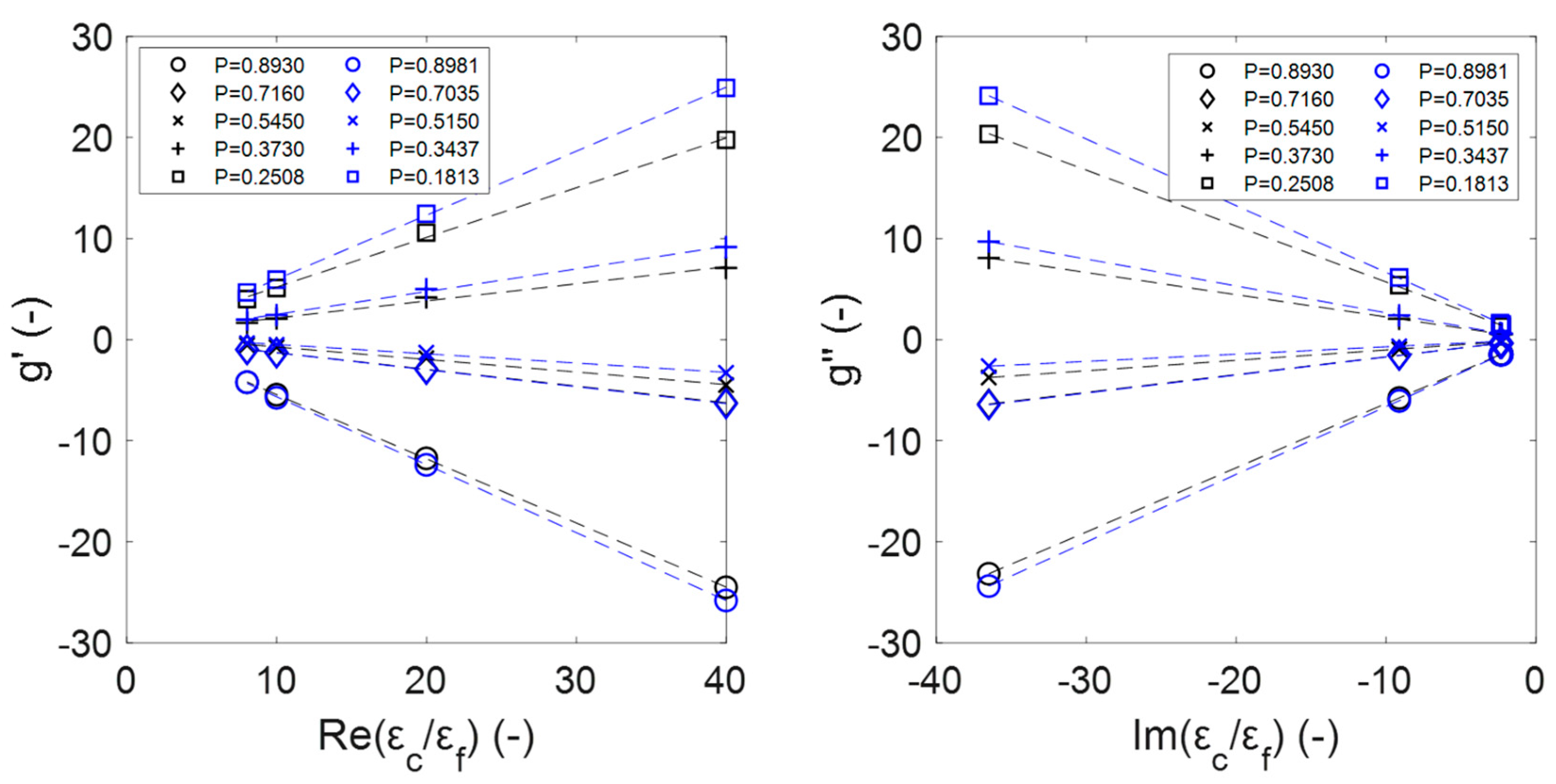
3. Results
4. Discussion
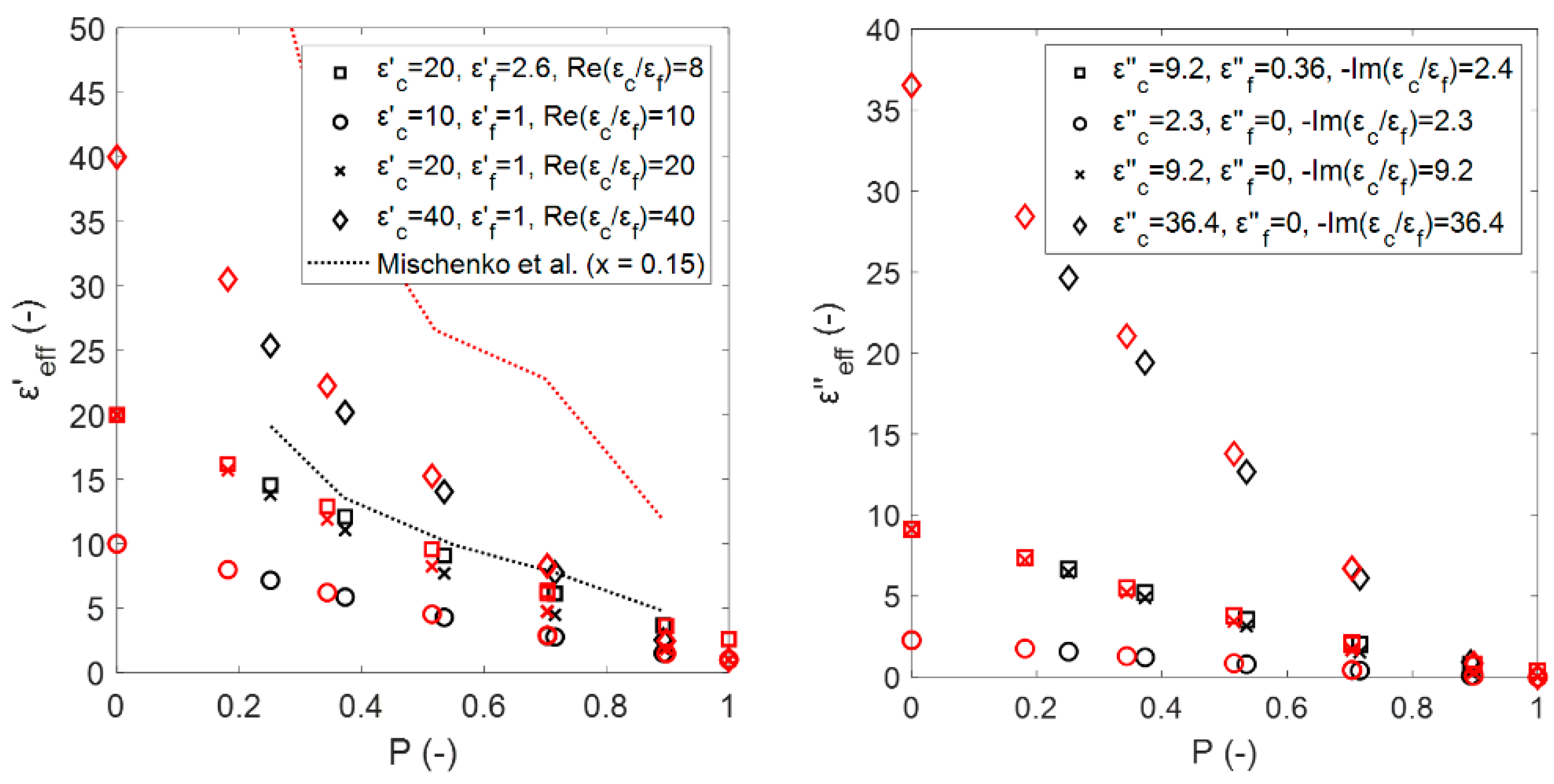
4.1. Analysis of the Effective Medium Approximation Applied to Sintered Foams
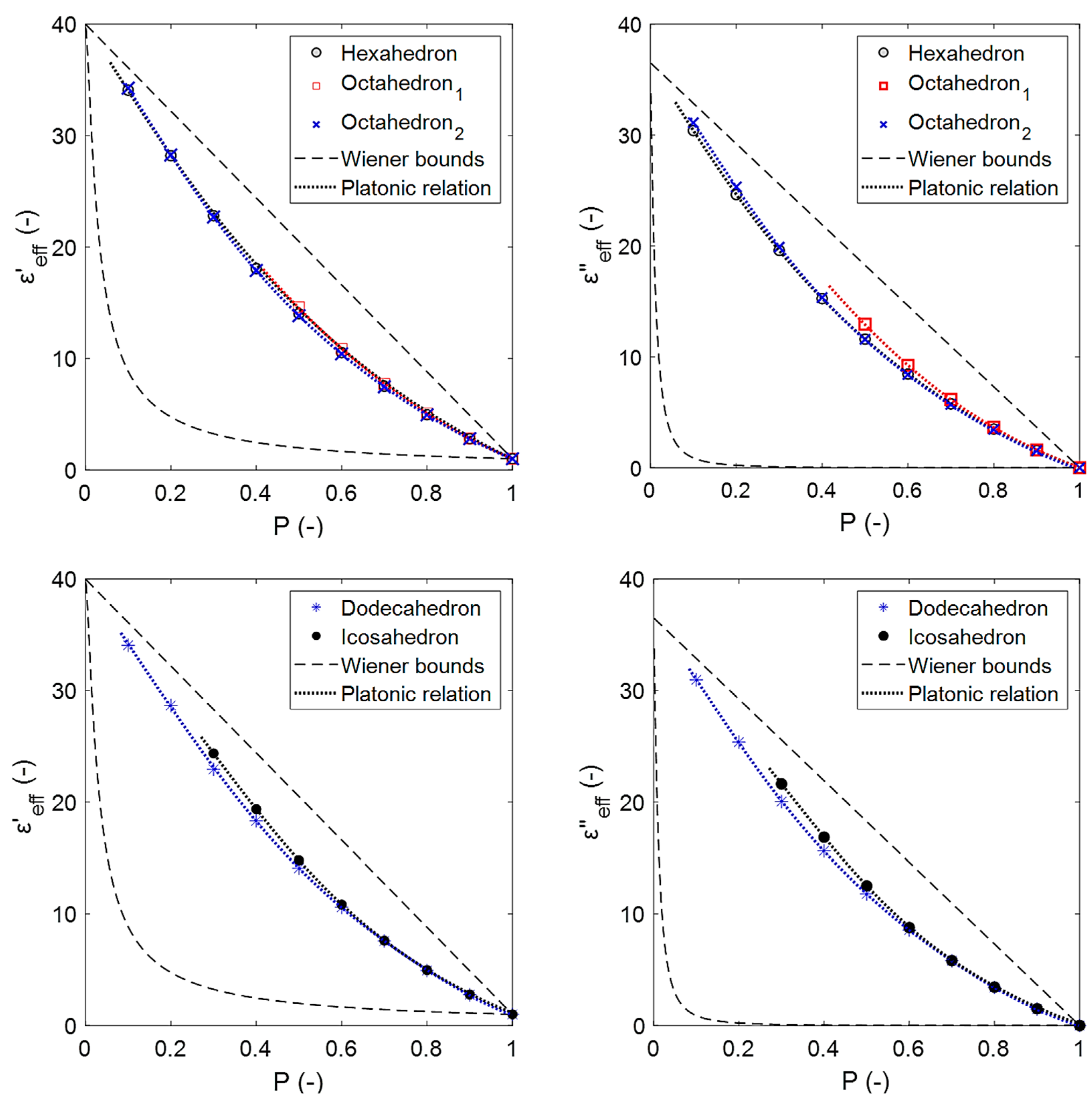
4.2. Comparison of Effective Permittivity Estimates with Mixing Relations from the Literature
4.3. Comparison of Effective Permittivity Estimates from Sintered and Platonic Foams
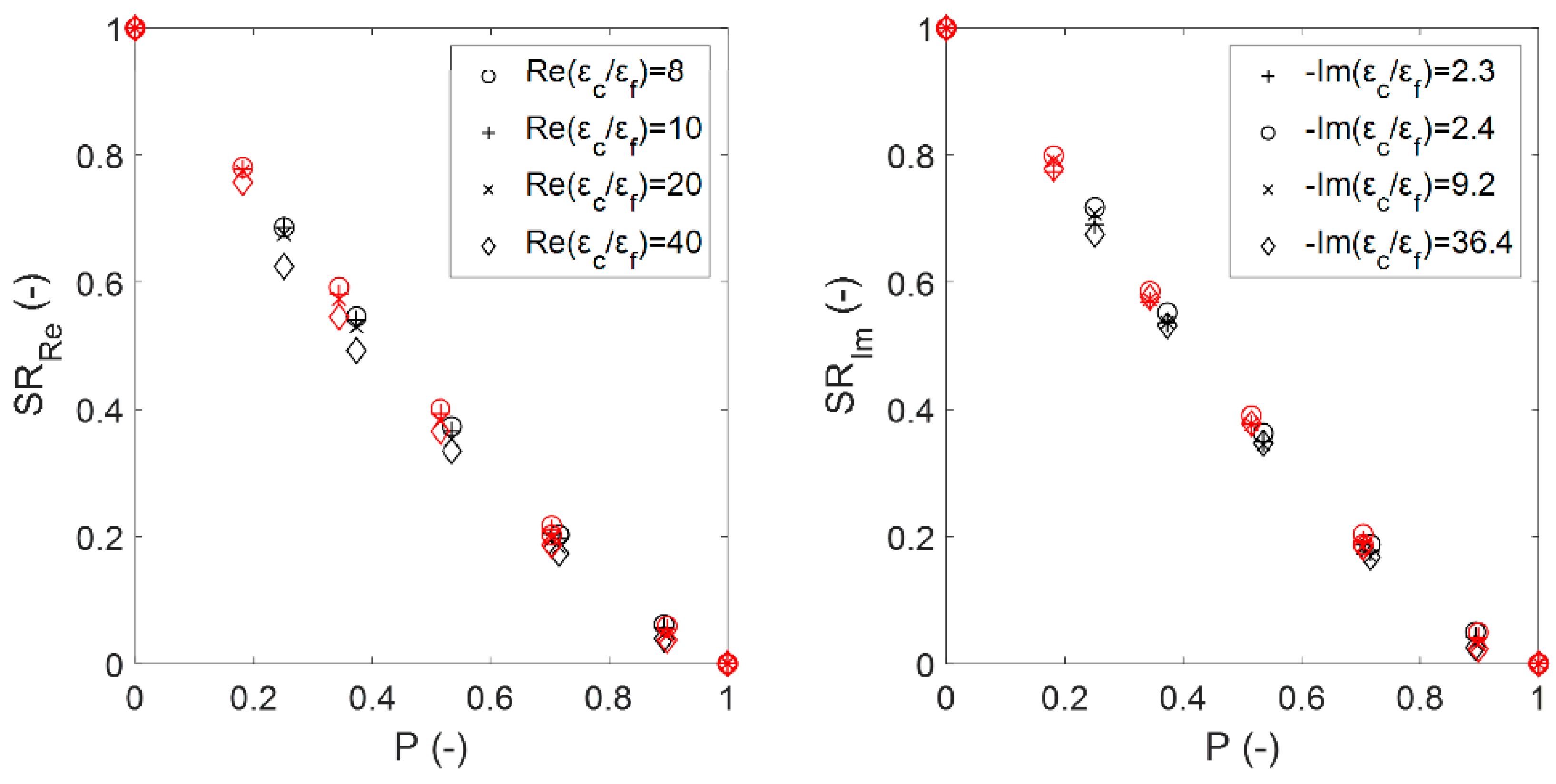
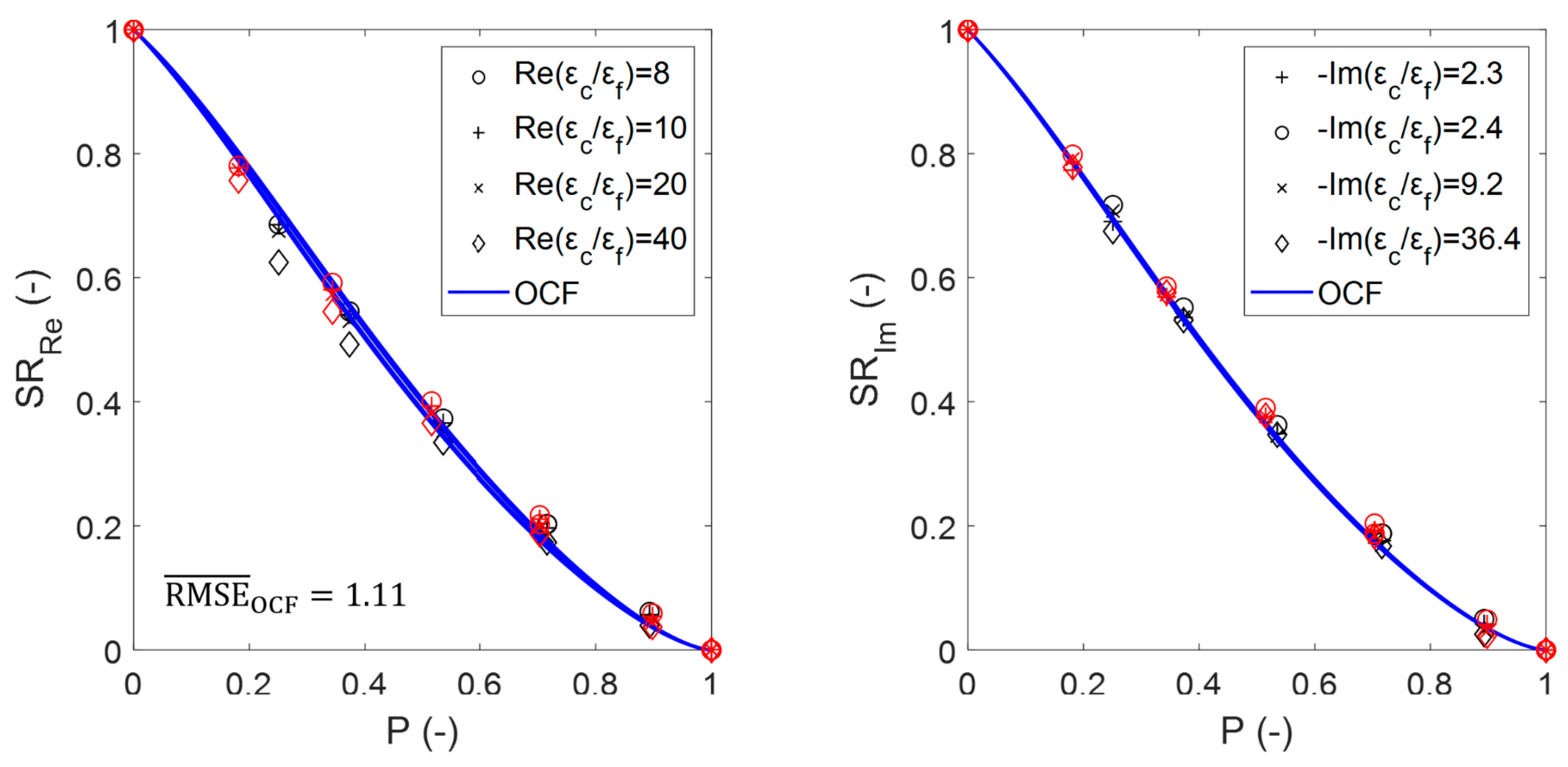
4.4. Analysis of Proposed Relations for the Estimation of the Permittivity of Platonic and Sintered Foams
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
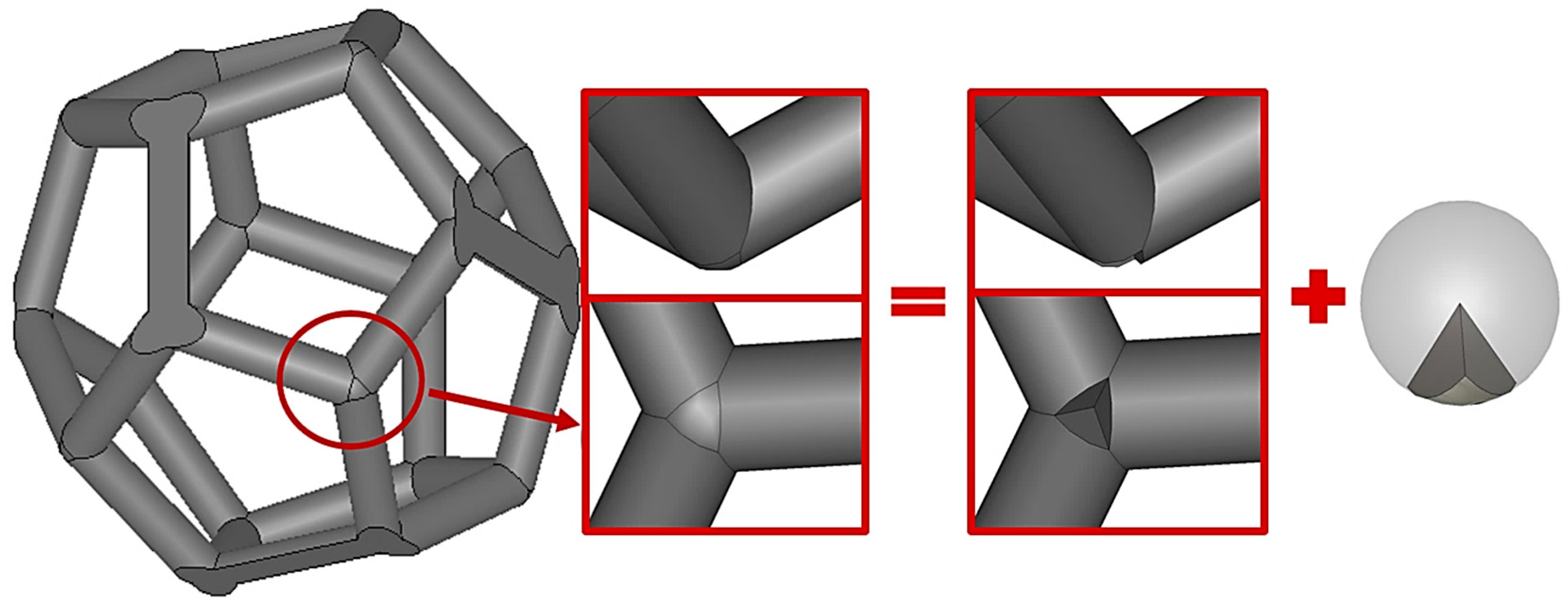
Appendix A.1. Skeletal Volume Calculation Sequence for Platonic Foams
- Calculate the volume of all cylinders at each intersection: .
- Calculate the volumes of two intersecting cylinders at : .
- Calculate the sum of the volumes of : .
- Calculate the volume of the total solid by using the inclusion-exclusion principle: − + .
- Calculate the percentage of enclosed in the PCA. Use symmetry (e.g., hexahedral or octahedral skeleton) or modeling software (e.g., computer-aided design).
- Calculate the total polyhedron volume inside the PCA: .
- Calculate the volume of all inserted spherical triangle segments: , where is the spherical excess, which is solved by L’Huilier’s theorem [28], and is the number of segments added to the skeleton.
- Calculate the volume of the Platonic skeleton: .
Appendix A.2. Example of Skeletal Volume Calculation of Hexahedral Foams
- (by symmetry, the whole solid can be arranged to give the PCA)
- (for the hexahedral foam, a non-spherical pyramid segment is needed)
Appendix A.3. Deriving the Permittivity Mixture-Relation for Platonic Foams
Appendix A.4. Extending the Proposed Permittivity Mixture Relation for Sintered Foams
Appendix A.5. Tables and Figures
| Platonic Skeleton | Volume of the Platonic skeleton | |
|---|---|---|
| Hexahedron | ||
| Octahedron1 | ||
| Octahedron2 | ||
| Dodecahedron | ||
| Icosahedron |
| Variable | ||||||
|---|---|---|---|---|---|---|
| Hexahedron | ||||||
| 0.5229 | −0.9951 | 2.4460 | −4.7673 | 3.9566 | −1.1630 | |
| 0.2775 | 4.7271 | −14.1449 | 15.0204 | −6.6742 | 0.7939 | |
| 0.2889 | −0.0356 | 0.8263 | −3.3594 | 3.3200 | −1.0402 | |
| −1.2278 | 10.1408 | −36.4260 | 63.3016 | −52.0799 | 16.2911 | |
| Octahedron1 | ||||||
| 0.4008 | 0.6699 | −3.2970 | 3.9169 | −2.2435 | 0.5528 | |
| 2.1613 | −7.0162 | 17.1386 | −28.2326 | 23.5875 | −7.6392 | |
| 0.3209 | 4.0884 | −19.4308 | 33.9140 | −27.2604 | 8.3683 | |
| −2.0185 | 26.2707 | −105.0485 | 185.5296 | −151.8010 | 47.0699 | |
| Octahedron2 | ||||||
| 0.6546 | −2.1904 | 6.0160 | −9.7020 | 7.2485 | −2.0266 | |
| 0.3698 | 7.6377 | −26.3063 | 34.1470 | −20.6464 | 4.7987 | |
| 0.7183 | −2.8553 | 8.1037 | −12.6555 | 9.2101 | −2.5214 | |
| 0.1978 | −2.4611 | 7.5172 | −10.0237 | 6.2255 | −1.4557 | |
| Dodecahedron | ||||||
| 0.4617 | 0.3546 | −3.9082 | 7.6496 | −7.0236 | 2.4661 | |
| 1.4799 | −10.1720 | 50.1347 | −109.2526 | 103.7717 | −35.9641 | |
| 0.6052 | −1.7991 | 4.9208 | −8.1320 | 5.9922 | −1.5869 | |
| 0.1169 | −3.0237 | 12.1921 | −20.3098 | 15.4249 | −4.4004 | |
| Icosahedron | ||||||
| 0.2213 | 4.1649 | −17.8917 | 29.0420 | −21.8497 | 6.3132 | |
| 2.4320 | −21.2239 | 84.7916 | −152.1911 | 124.4845 | −38.2943 | |
| 0.3270 | 2.8913 | −12.9887 | 20.3933 | −14.6653 | 4.0423 | |
| −0.1270 | 0.6904 | −2.6267 | 4.5291 | −3.4173 | 0.9512 | |

References
- Wan, T.; Liu, Y.; Zhou, C.; Chen, X.; Li, Y. Fabrication, properties, and applications of open-cell aluminum foams: A review. J. Mater. Sci. Technol. 2020, 62, 11–24. [Google Scholar] [CrossRef]
- Zalucky, J.; Schubert, M.; Lange, R.; Hampel, U. Dynamic Liquid–Solid Mass Transfer in Solid Foam Packed Reactors at Trickle and Pulse Flow. Ind. Eng. Chem. Res. 2017, 56, 13190–13205. [Google Scholar] [CrossRef]
- Bracconi, M.; Ambrosetti, M.; Maestri, M.; Groppi, G.; Tronconi, E. A fundamental investigation of gas/solid mass transfer in open-cell foams using a combined experimental and CFD approach. Chem. Eng. J. 2018, 352, 558–571. [Google Scholar] [CrossRef]
- Pabst, W.; Hříbalová, S. Describing the Effective Conductivity of Two-Phase and Multiphase Materials via Weighted Means of Bounds and General Power Means. JOM 2019, 71, 4005–4014. [Google Scholar] [CrossRef]
- Torquato, S.; Haslach, H. Random Heterogeneous Materials: Microstructure and Macroscopic Properties. Appl. Mech. Rev. 2002, 55, B62–B63. [Google Scholar] [CrossRef]
- Diani, A.; Bodla, K.K.; Rossetto, L.; Garimella, S.V. Numerical Analysis of Air Flow through Metal Foams. Energy Procedia 2014, 45, 645–652. [Google Scholar] [CrossRef]
- Pickles, A.J.; Steer, M.B. Effective Permittivity of 3-D Periodic Composites With Regular and Irregular Inclusions. IEEE Access 2013, 1, 523–536. [Google Scholar] [CrossRef]
- Pabst, W.; Gregorová, E.; Uhlířová, T. Microstructure characterization via stereological relations—A shortcut for beginners. Mater. Charact. 2015, 105, 1–12. [Google Scholar] [CrossRef]
- Lorensen, W.E.; Cline, H.E. Marching cubes: A high resolution 3D surface construction algorithm. Proc. Siggraph 1987, 21, 163–169. [Google Scholar] [CrossRef]
- Hernandez, J.N.C.; Link, G.; Soldatov, S.; Füssel, A.; Schubert, M.; Hampel, U. Experimental and numerical analysis of the complex permittivity of open-cell ceramic foams. Ceram. Int. 2020, 46, 26829–26840. [Google Scholar] [CrossRef]
- Rueden, C.T.; Schindelin, J.; Hiner, M.C.; Dezonia, B.E.; Walter, A.E.; Arena, E.T.; Eliceiri, K.W. ImageJ2: ImageJ for the next generation of scientific image data. BMC Bioinform. 2017, 18, 529. [Google Scholar] [CrossRef] [PubMed]
- Ollion, J.; Cochennec, J.; Loll, F.; Escudé, C.; Boudier, T. TANGO: A generic tool for high-throughput 3D image analysis for studying nuclear organization. Bioinformatics 2013, 29, 1840–1841. [Google Scholar] [CrossRef]
- Dougherty, R.; Kunzelmann, K.-H. Computing Local Thickness of 3D Structures with ImageJ. Microsc. Microanal. 2007, 13, 1678–1679. [Google Scholar] [CrossRef]
- Atiyah, M.; Sutcliffe, P. Polyhedra in Physics, Chemistry and Geometry. Milan J. Math. 2003, 71, 33–58. [Google Scholar] [CrossRef]
- Numan, A.B.; Sharawi, M.S. Extraction of Material Parameters for Metamaterials Using a Full-Wave Simulator [Education Column]. IEEE Antennas Propag. Mag. 2013, 55, 202–211. [Google Scholar] [CrossRef]
- Hernandez, J.N.C.; Lecrivain, G.; Schubert, M.; Hampel, U. Droplet Retention Time and Pressure Drop in SiSiC Open-Cell Foams Used as Droplet Separation Devices: A Numerical Approach. Ind. Eng. Chem. Res. 2019, 59, 4093–4107. [Google Scholar] [CrossRef]
- Mishchenko, M.I.; Dlugach, J.M.; Liu, L. Applicability of the effective-medium approximation to heterogeneous aerosol particles. J. Quant. Spectrosc. Radiat. Transf. 2016, 178, 284–294. [Google Scholar] [CrossRef] [Green Version]
- Baker-Jarvis, J.; Janezic, M.; Riddle, B.; Johnk, R.; Holloway, C.; Geyer, R.; Grosvenor, C. Measuring the Permittivity and Permeability of Lossy Materials: Solids, Liquids, Metals, and negative-Index Materials, 1st ed.; National Institute of Standards and Technology, Technology Administration, U.S. Department of Commerce, NIST: Boulder, CO, USA, 2005; pp. 1–160. [Google Scholar]
- MATLAB 2020, version 9.8.0.1323502, R2020a; The Mathworks Inc.: Natick, MA, USA, 2020.
- MATLAB. 2021, Fit a Model to Complex-Valued Data. Available online: https://www.mathworks.com/help/optim/ug/fit-model-to-complex-data.html (accessed on 25 November 2021).
- Camerucci, M.A.; Urretavizcaya, G.; Castro, M.; Cavalieri, A. Electrical properties and thermal expansion of cordierite and cordierite-mullite materials. J. Eur. Ceram. Soc. 2001, 21, 2917–2923. [Google Scholar] [CrossRef]
- Westphal, W.; Sils, A. Dielectric Constant and Loss Data; MIT Technical Report AFML TR 72; Wright Patterson: Dayton, OH, USA, 1972; Volume 39, pp. 1–71. [Google Scholar]
- Ratanadecho, P.; Aoki, K.; Akahori, M. The characteristics of microwave melting of frozen packed beds using a rectangular waveguide. IEEE Trans. Microw. Theory Tech. 2002, 50, 1495–1502. [Google Scholar] [CrossRef]
- He, X.; Zhou, J.; Jin, L.; Long, X.; Wu, H.; Xu, L.; Gong, Y.; Zhou, W. Improved Dielectric Properties of Thermoplastic Polyurethane Elastomer Filled with Core–Shell Structured PDA@TiC Particles. Materials 2020, 13, 3341. [Google Scholar] [CrossRef]
- McKeen, L.W. Chapter 1—Introduction to Plastics and Polymers. In Plastics Design Library, Film Properties of Plastics and Elastomers, 3rd ed.; William Andrew Publishing: Norwich, NY, USA, 2012; pp. 1–18. ISBN 9781455725519. [Google Scholar] [CrossRef]
- Moore, M. Symmetrical intersections of right circular cylinders. Math. Gaz. 1974, 58, 181–185. [Google Scholar] [CrossRef]
- Strzebonski. Cylindrical Algebraic Decomposition. From MathWorld—A Wolfram Web Resour. Creat. by Eric W. Weisstein. (n.d.). Available online: https://mathworld.wolfram.com/CylindricalAlgebraicDecomposition.html (accessed on 18 May 2021).
- Weisstein, E.W. L’Huilier’s Theorem. From MathWorld—A Wolfram Web Resour. (n.d.). Available online: https://mathworld.wolfram.com/LHuiliersTheorem.html (accessed on 18 May 2021).
- Sihvola, A.; Yla-Oijala, P.; Jarvenpaa, S.; Avelin, J. Polarizabilities of Platonic Solids. IEEE Trans. Antennas Propag. 2004, 52, 2226–2233. [Google Scholar] [CrossRef]
- Sihvola, A. Dielectric Polarization and Particle Shape Effects. J. Nanomater. 2007, 2007, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Martínez, E.S.; Calleja, R.D.; Gunßer, W. Complex polarizability as used to analyze dielectric relaxation measurements. Colloid Polym. Sci. 1992, 270, 146–153. [Google Scholar] [CrossRef]
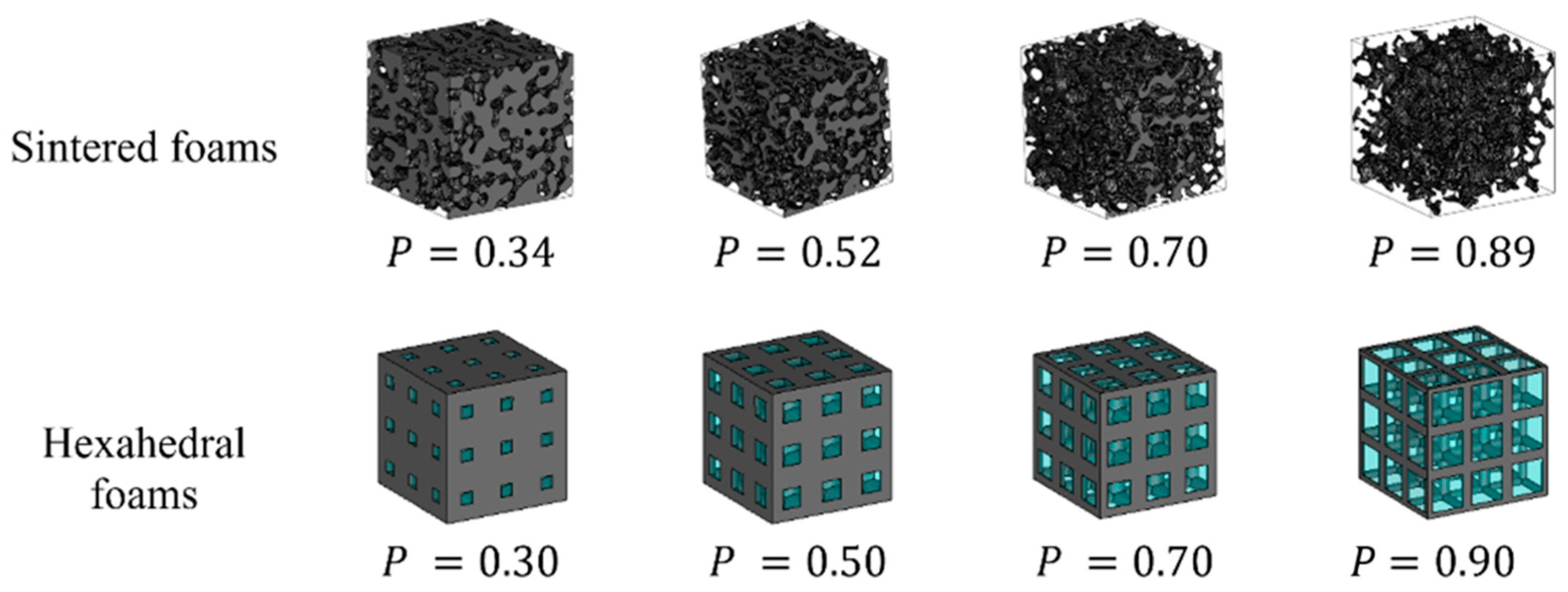





| 20 ppi | 0.89 | 0.72 | 0.55 | 0.37 | 0.25 | |
| (mm) | 2.65 ± 0.51 | 2.10 ± 0.49 | 1.85 ± 0.33 | 1.58 ± 0.28 | 1.33 ± 0.44 | |
| (mm) | 0.64 ± 0.20 | 0.95 ± 0.21 | 1.03 ± 0.42 | 1.41 ± 0.52 | 1.93 ± 0.23 | |
| 30 ppi | 0.89 | 0.70 | 0.52 | 0.34 | 0.18 | |
| (mm) | 1.68 ± 0.54 | 1.22 ± 0.20 | 1.13 ± 0.19 | 0.92 ± 0.14 | 0.70 ± 0.17 | |
| (mm) | 0.37 ± 0.12 | 0.53 ± 0.13 | 0.70 ± 0.30 | 0.84 ± 0.20 | 1.22 ± 0.26 |
| Platonic Skeleton | Porosity | Limits for Strut Diameter and Porosity |
|---|---|---|
| Hexahedron | ||
| Octahedron1 | ||
| Octahedron2 | ||
| Dodecahedron | ||
| Icosahedron |
| EMA Relations | Expression |
|---|---|
| Maxwell Garnett (M-G) (Maxwell-type relation, non-symmetric) [5] | |
| Bruggeman–Landauer (B-L) (self-consistent relation, symmetric) [5] | |
| Differential effective medium (DEM) (Bruggeman relation, non-symmetric) [5] | |
| Probability distribution relations | |
| Weighted arithmetic (Ar) mean of upper and lower Wiener bounds [4] | |
| Weighted harmonic (Hr) mean of upper and lower Wiener bounds [4] | |
| Weighted geometric (Ge) mean of upper and lower Wiener bounds [4] | |
| General sigmoidal (Sg) mean of upper and lower Wiener bounds [4] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Camacho Hernandez, J.N.; Link, G.; Schubert, M.; Hampel, U. Modeling of the Effective Permittivity of Open-Cell Ceramic Foams Inspired by Platonic Solids. Materials 2021, 14, 7446. https://doi.org/10.3390/ma14237446
Camacho Hernandez JN, Link G, Schubert M, Hampel U. Modeling of the Effective Permittivity of Open-Cell Ceramic Foams Inspired by Platonic Solids. Materials. 2021; 14(23):7446. https://doi.org/10.3390/ma14237446
Chicago/Turabian StyleCamacho Hernandez, Jesus Nain, Guido Link, Markus Schubert, and Uwe Hampel. 2021. "Modeling of the Effective Permittivity of Open-Cell Ceramic Foams Inspired by Platonic Solids" Materials 14, no. 23: 7446. https://doi.org/10.3390/ma14237446






