Laboratory Study on the Stability of Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression
Abstract
:1. Introduction
2. Shakedown Theory
2.1. Principles of Shakedown Theory
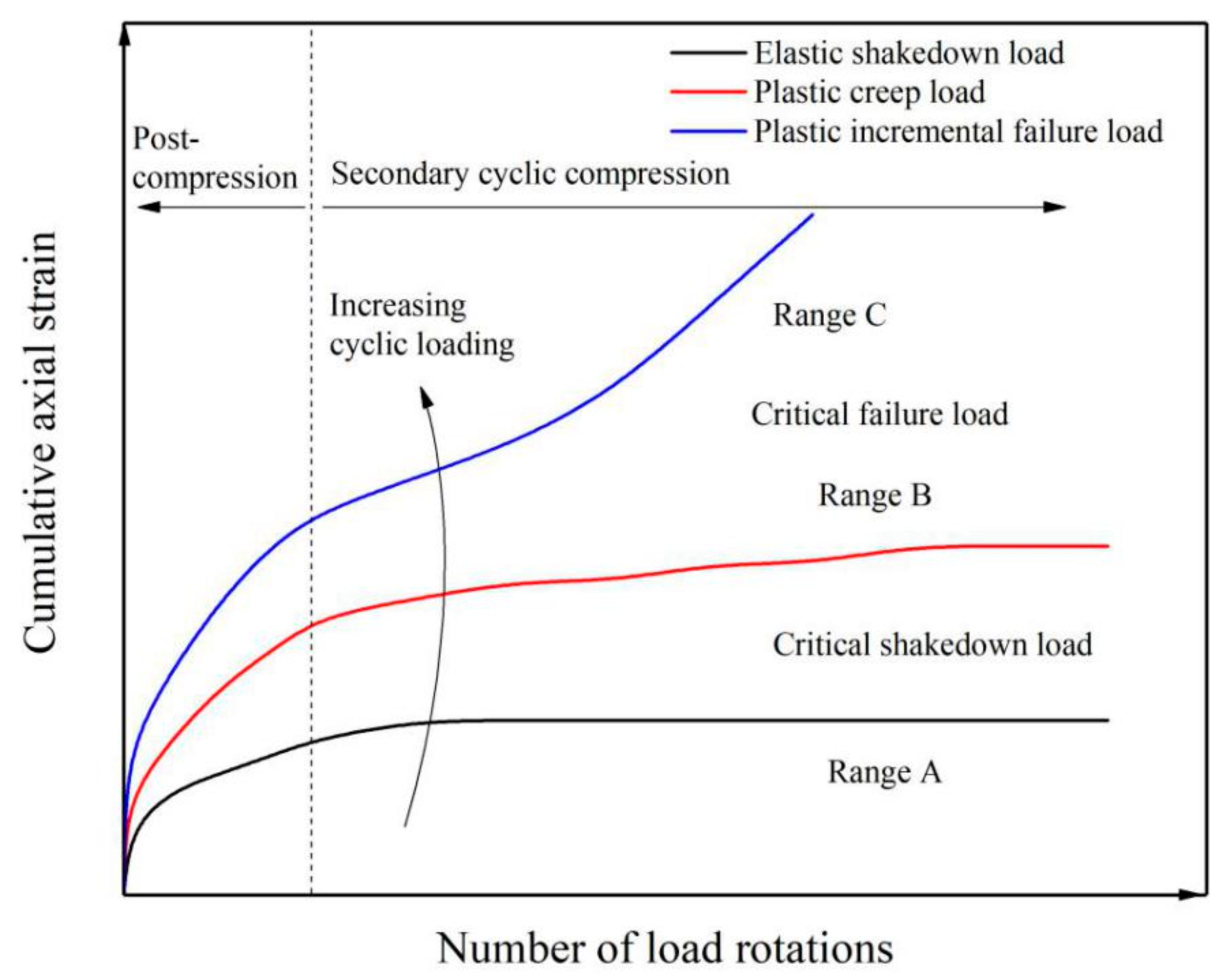
2.2. Stability Test and Behavior Evaluation
- Range A: , the material is in the elastic stability state.
- Range B: , the material is in the plastic creep state.
- Range C: , the material is in the incremental plastic failure state.
- Range A: , the specimen is in the elastic stability state.
- Range B: , the specimen is in the plastic creep state.
- Range C: , the specimen is in the incremental plastic failure state.
3. Raw Materials and Specimen Preparation
3.1. Raw Materials
- Fine aggregate: crushed granite crushed stone particles with particle size below 4.75 mm used in the Guigang Expressway in Guangxi.
3.2. Specimen Preparation Method
- Take 5000 g of crushed stone mixture according to intended gradation.
- Add water to the 5000 g crushed stone mixture according to the best moisture content of 4.1% and mix evenly. Seal with plastic and maintain for 12 h.
- Place the crushed stone mixture in two layers and compact each layer for 4 min.
4. Experimental Program
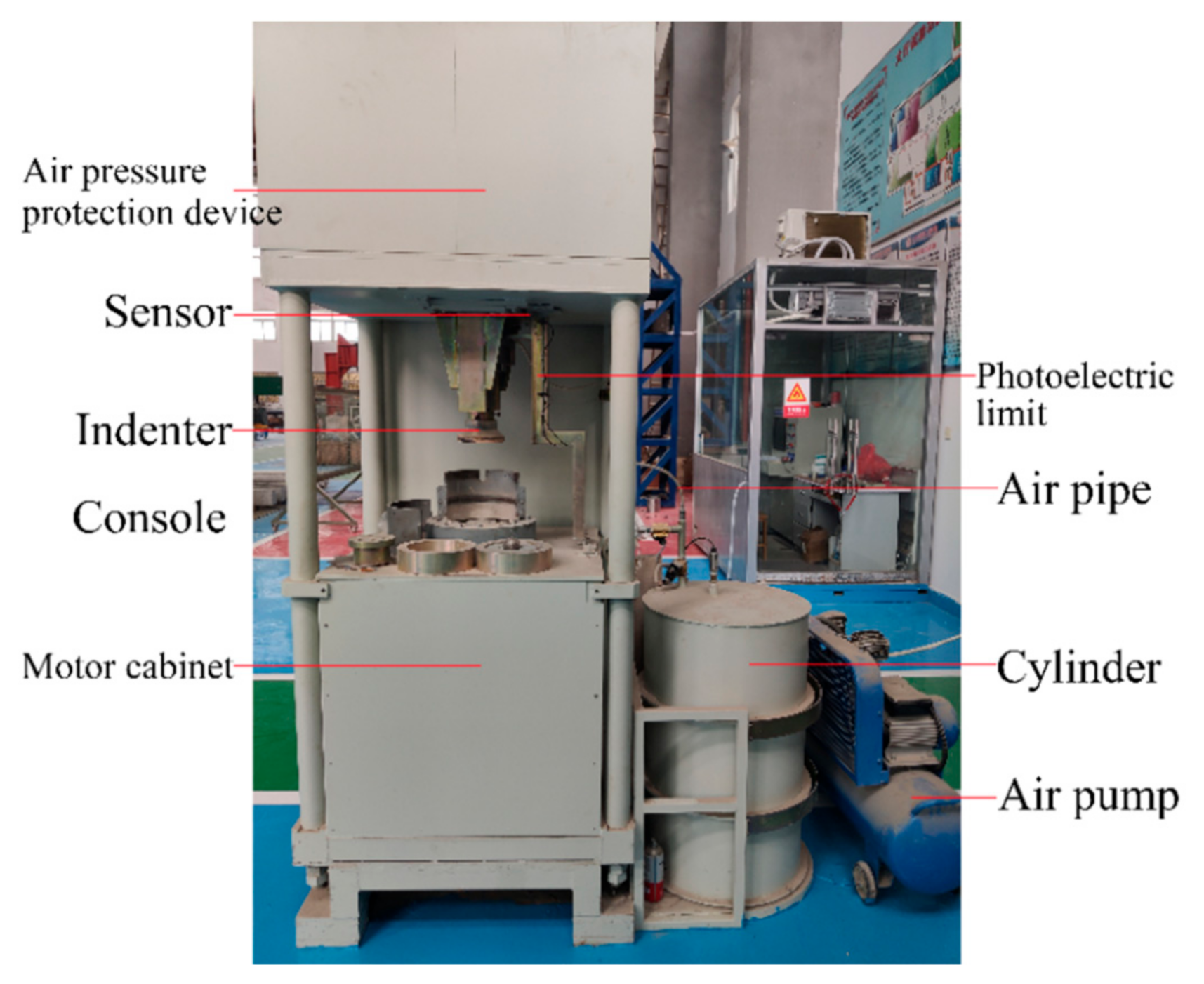
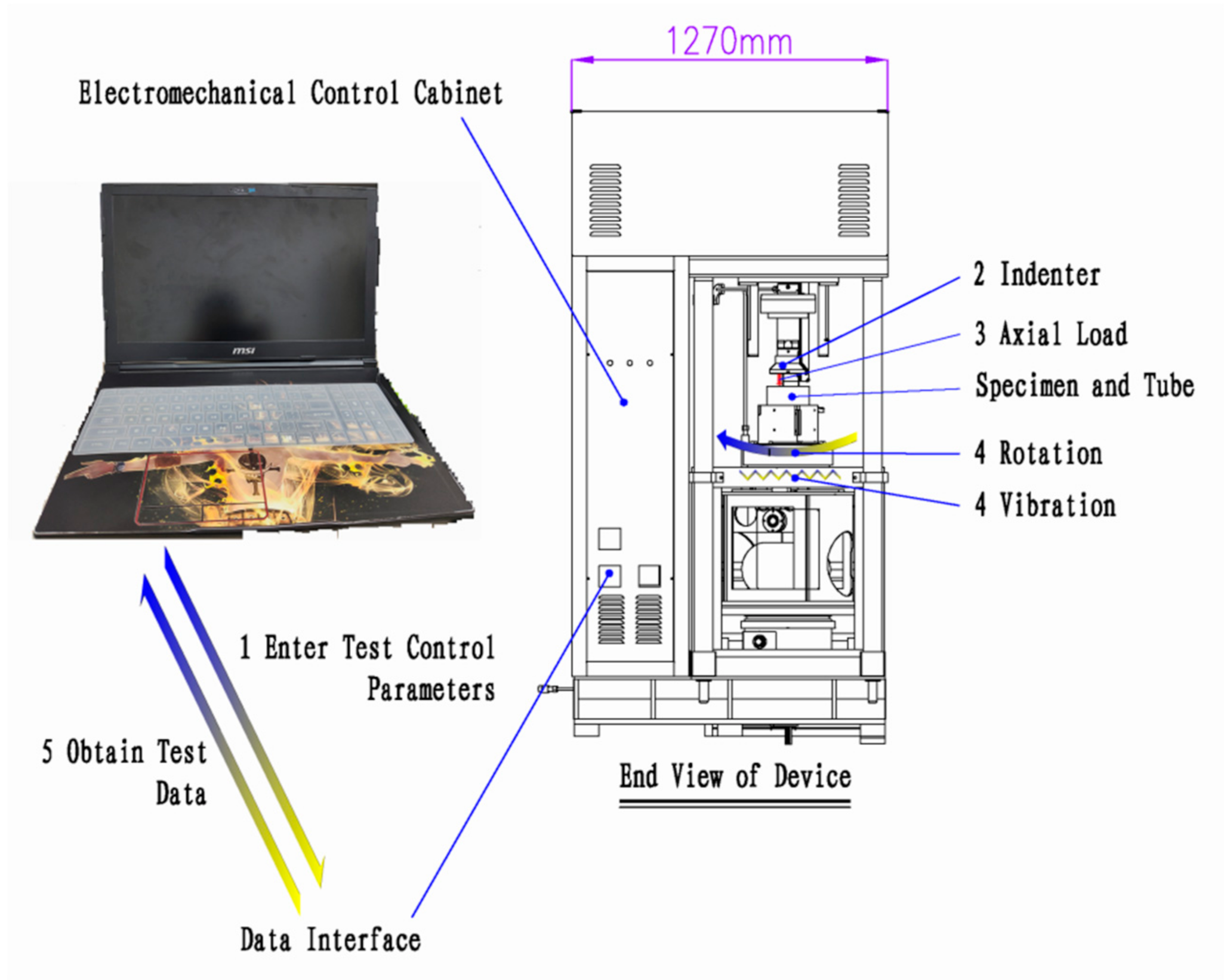
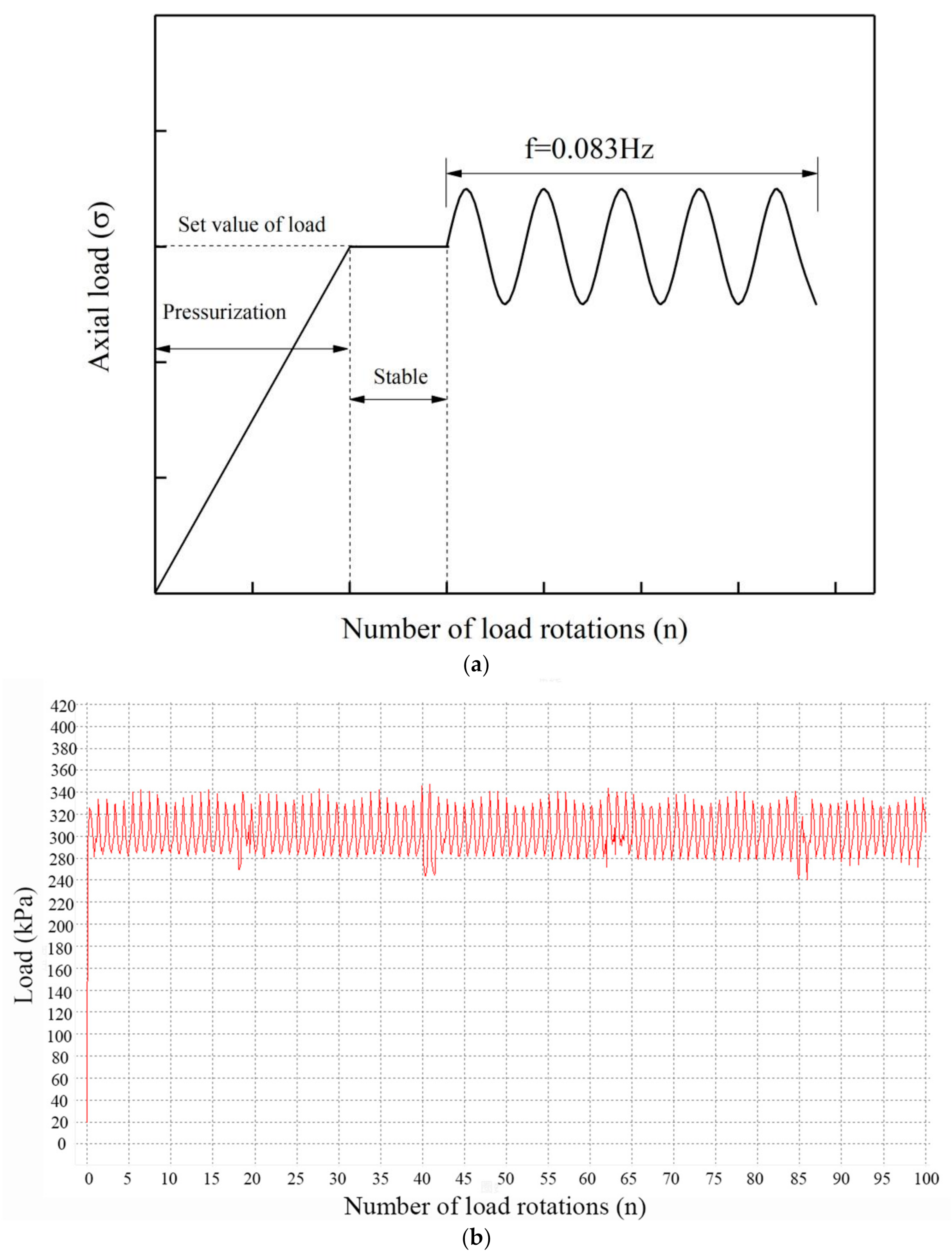
4.1. Loading Method of Cyclic Rotating Axial Compression
4.2. Influence of Gradation on Stability
4.3. Influence of Skeleton Structure Performance on Long-Term Stability Based on CBR Values
5. Analysis of Test Results
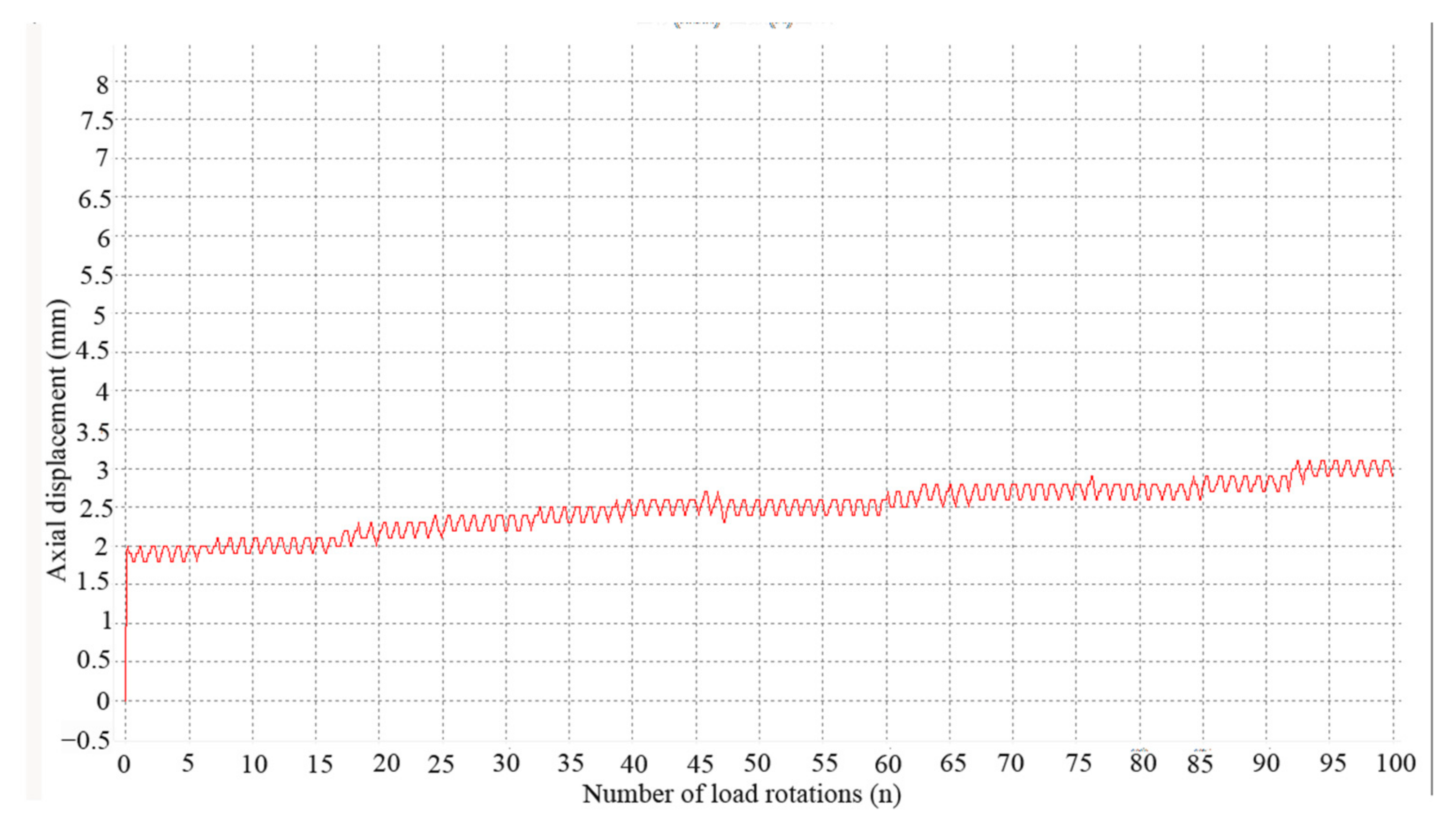
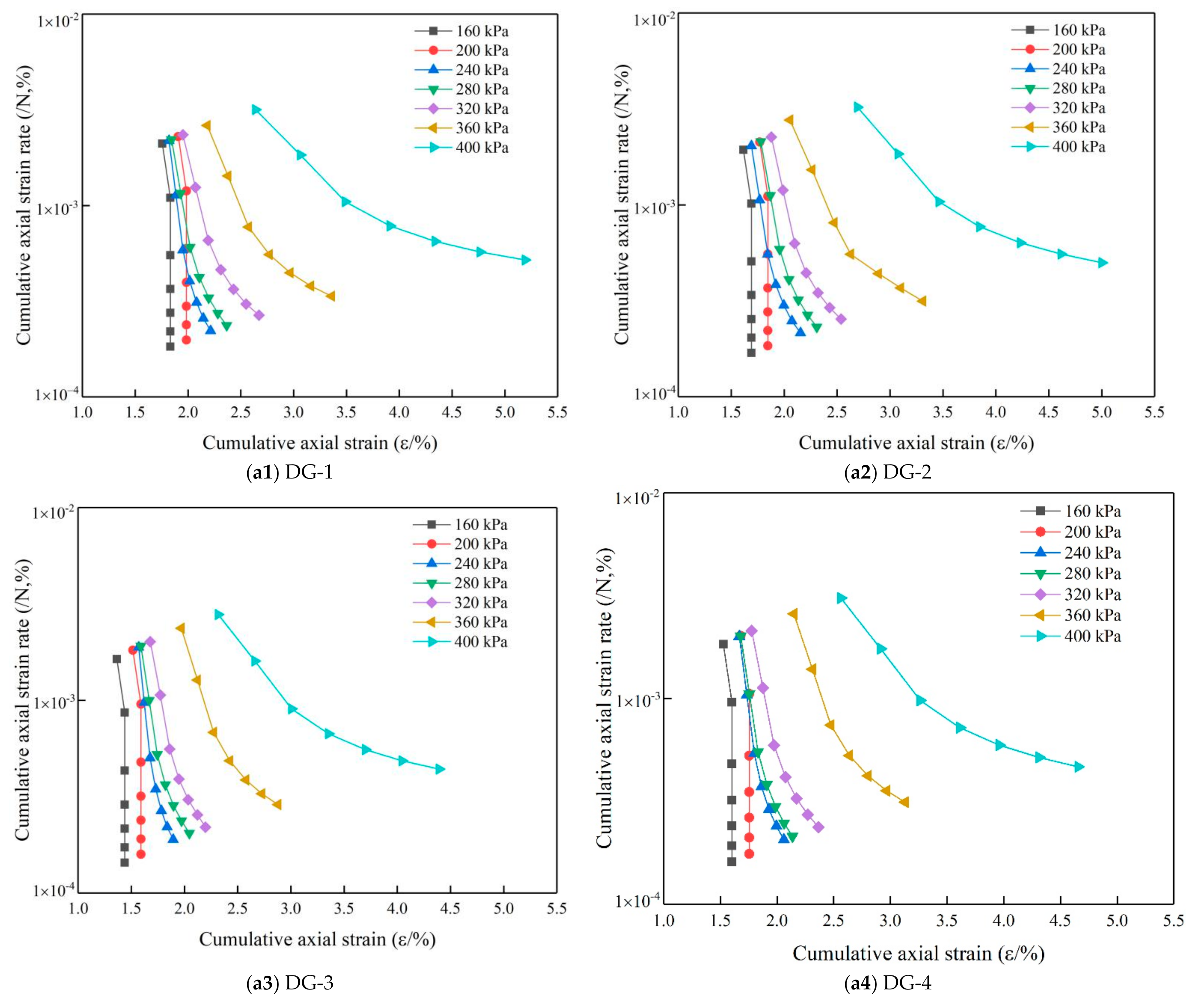
5.1. Deformation Relationship for Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression
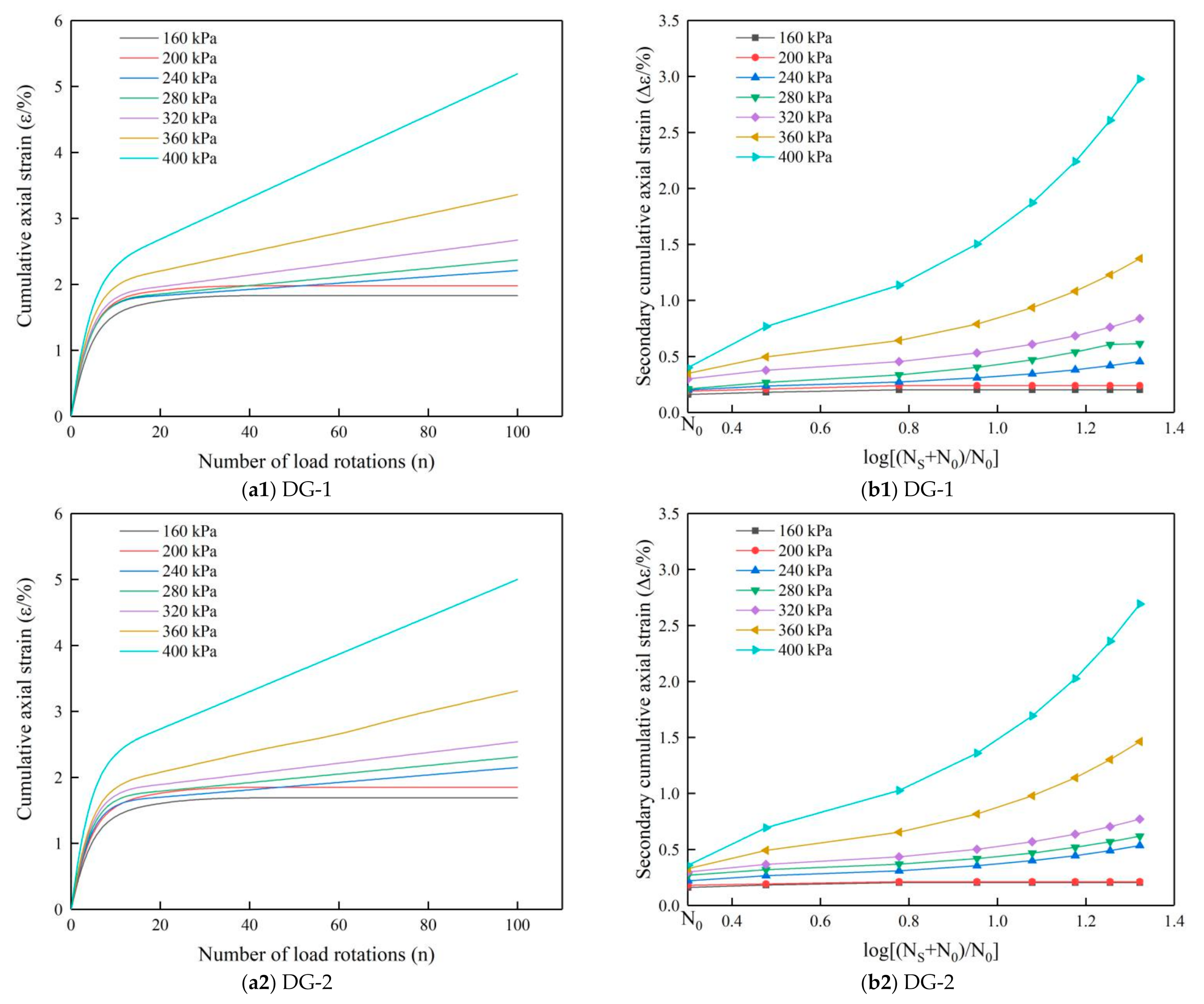
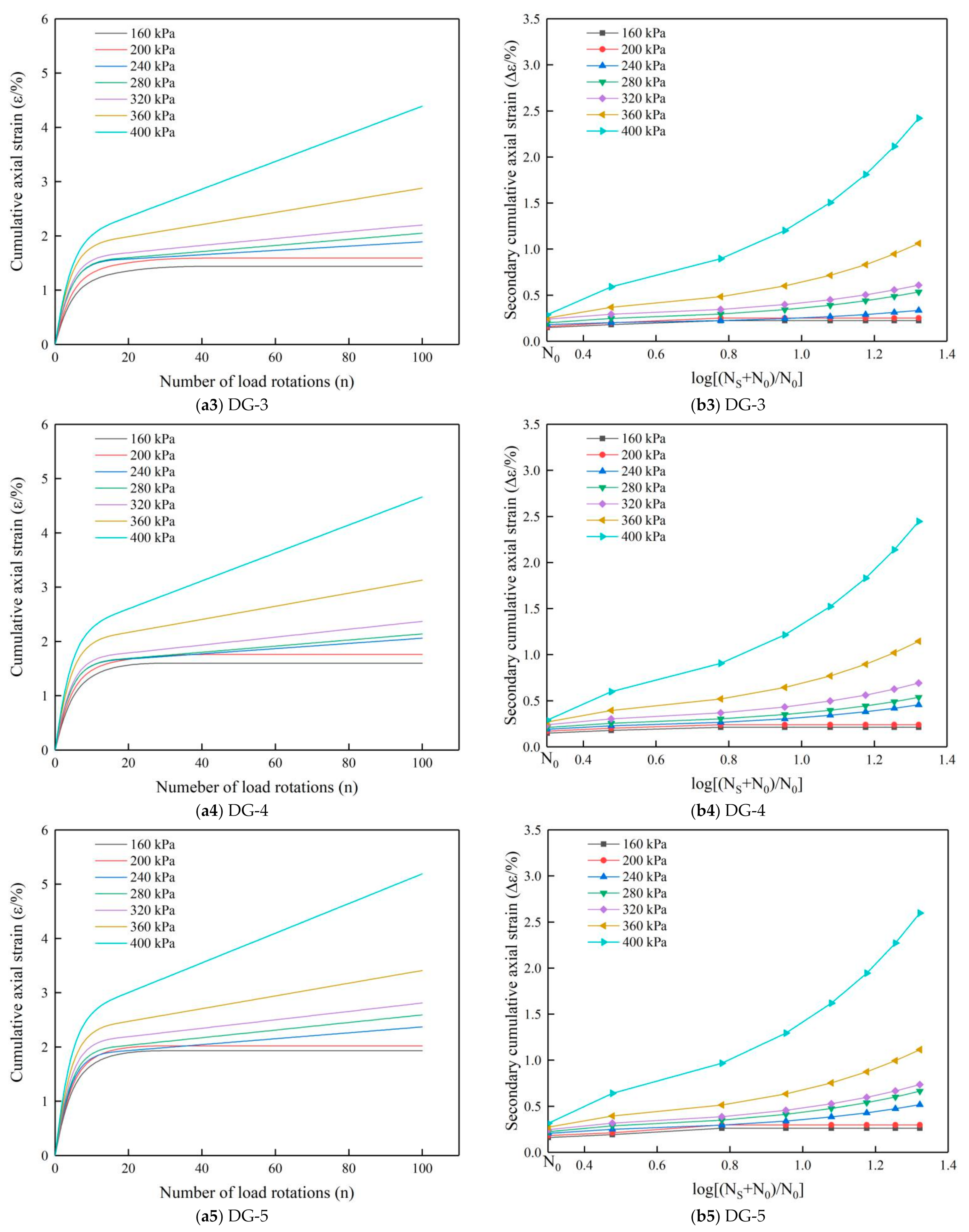
5.2. Influence of Gradation on Shakedown Stability
5.2.1. Stability Analysis of Specimens with Different Gradations
- Range A: , the specimen is in the elastic stability state.
- Range B: , the specimen is in the plastic creep state.
- Range C: , the specimen is in the incremental plastic failure state.
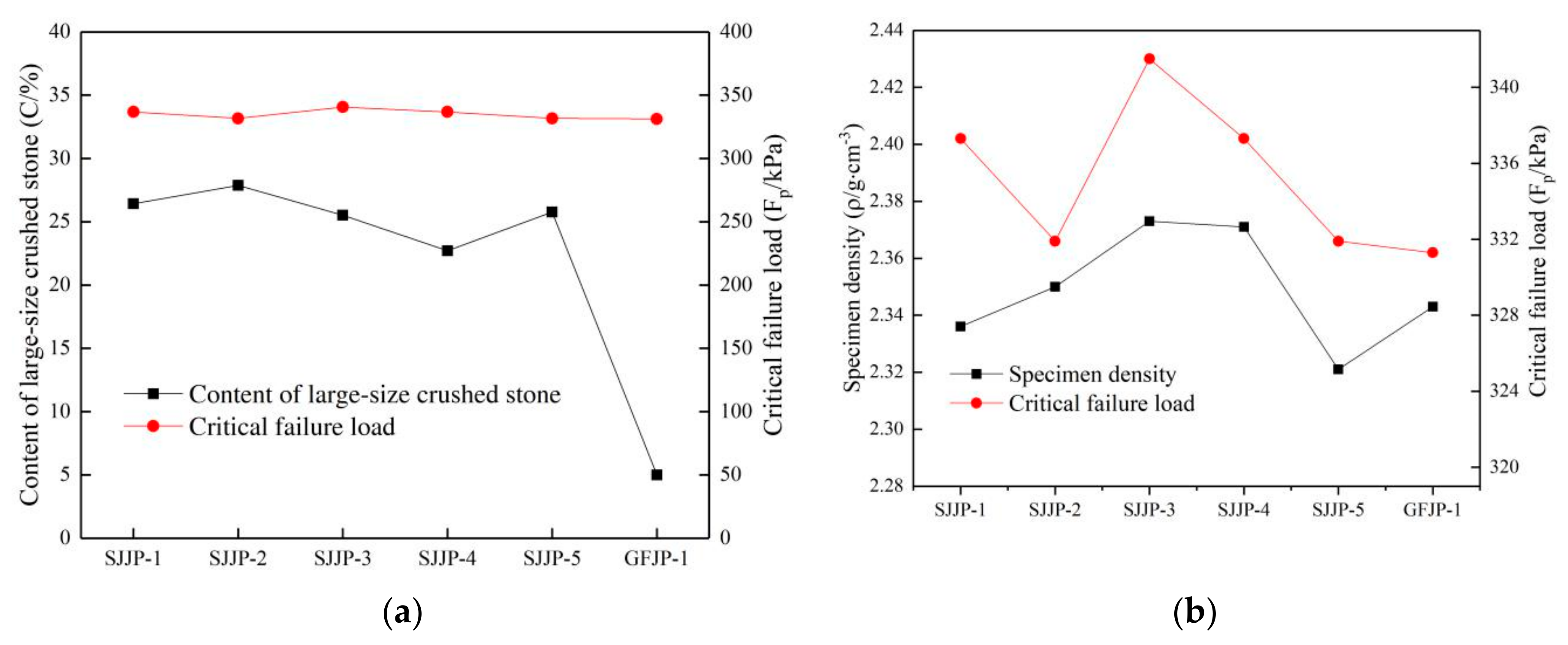
5.2.2. Effect of Content and Density of Large-Size Crushed Stone on Critical Load
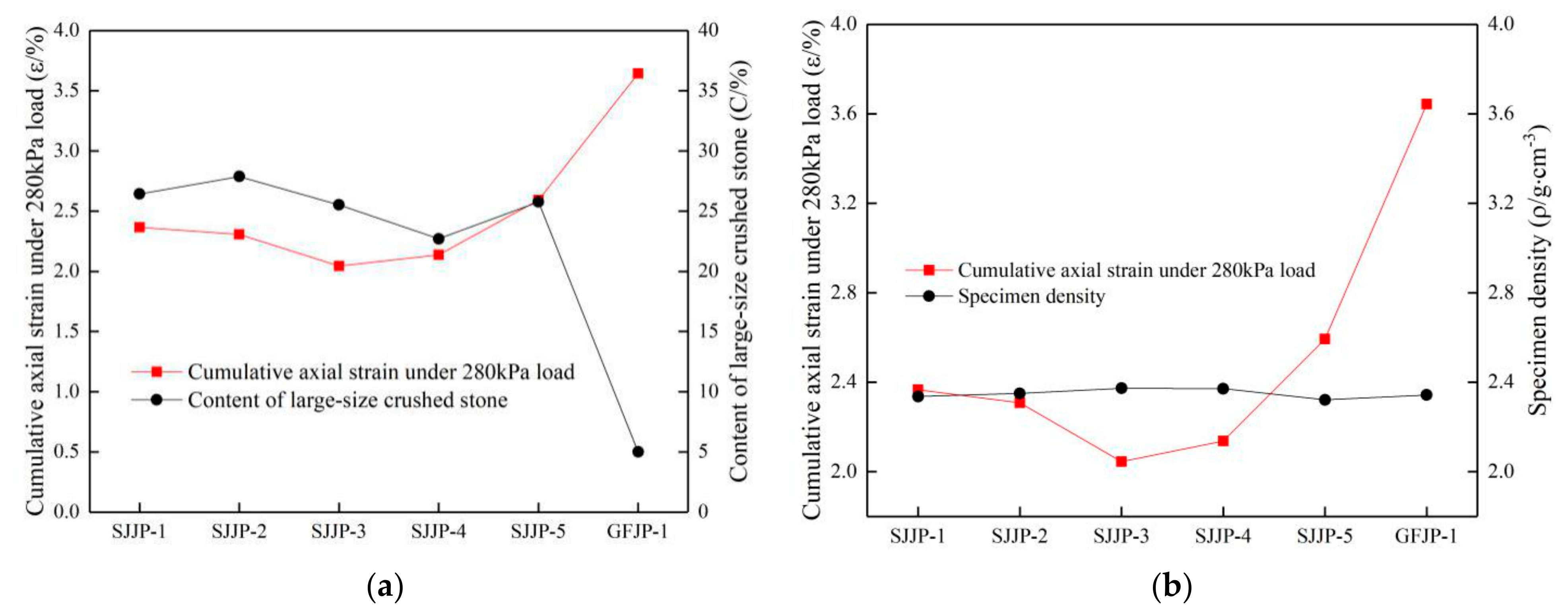
5.2.3. Effect of Content and Density of Large-Size Crushed Stone on Cumulative Axial Strain
5.2.4. Regression Analysis of the Joint Effect of Content and Density of Large-Size Crushed Stone
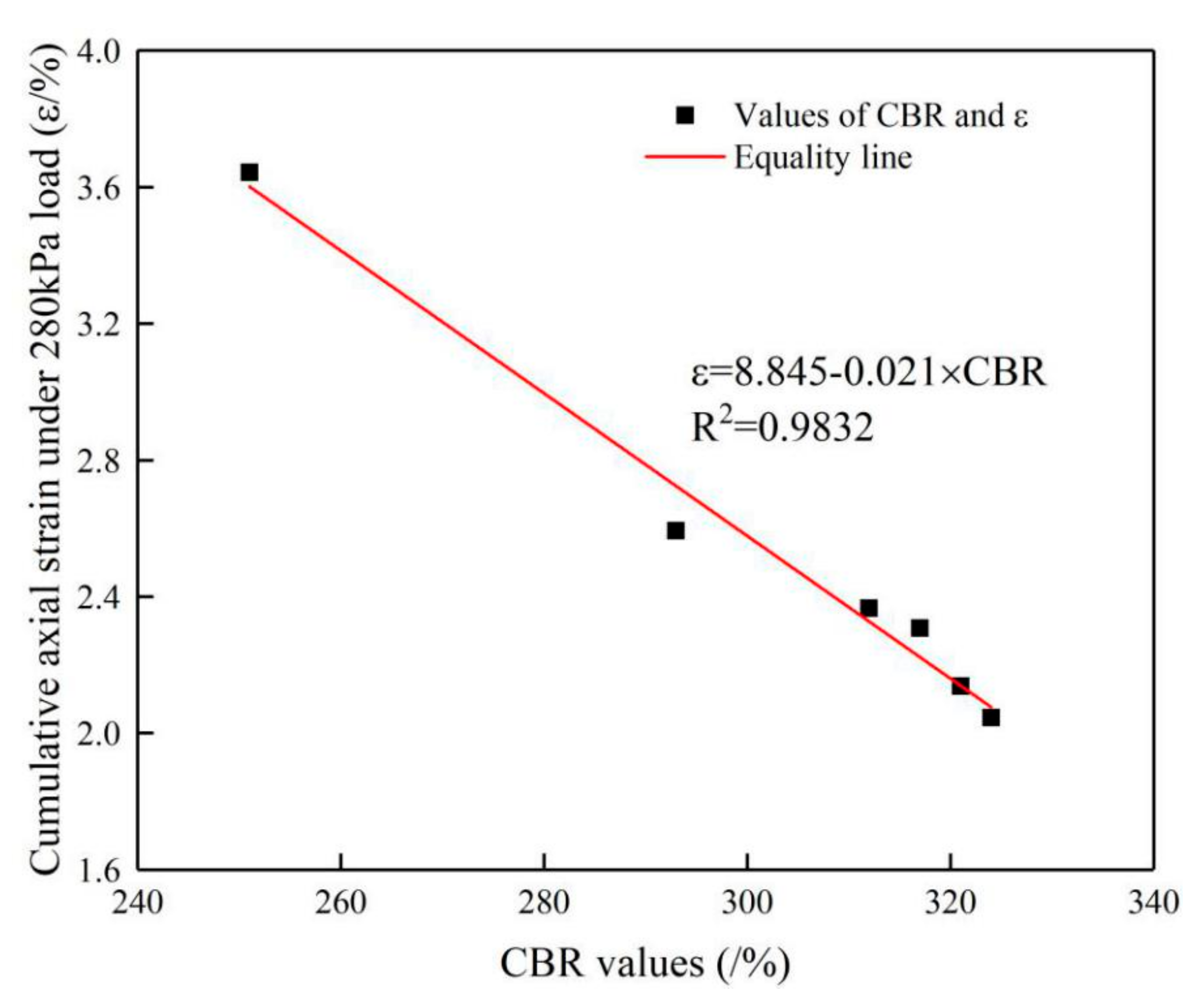
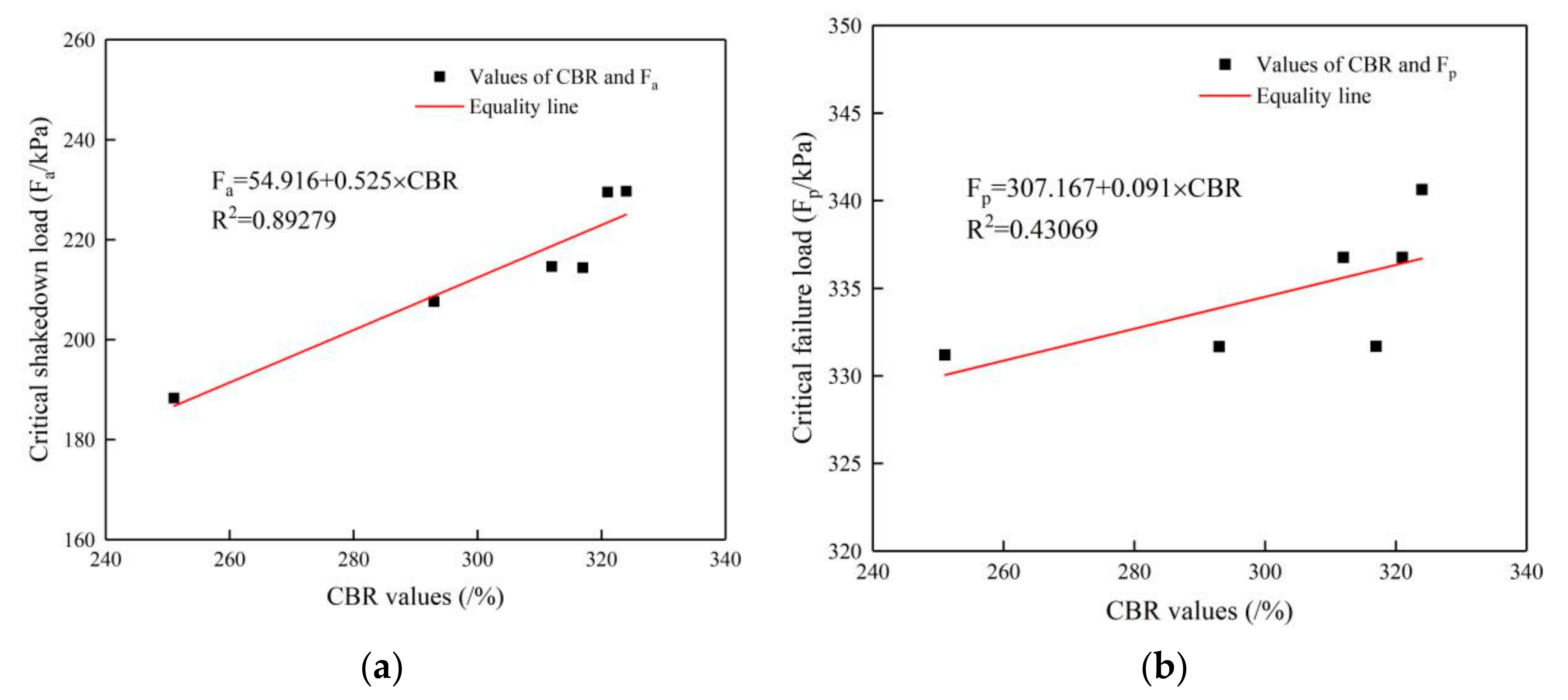
5.3. Relationship between CBR Values, Strain, and Critical Load
5.3.1. Relationship between CBR Values and Cumulative Axial Strain of Specimen
5.3.2. Relationship between CBR Values and Critical Load
6. Conclusions
- In the first part of the research, the cumulative axial strain of 126 crushed stone specimens under cyclic loading was analyzed. The cumulative axial strain of large-size graded crushed stone specimen will eventually become stable and no longer increase under a cyclic rotating axial compression of 200 kPa. When the load is between 240 and 320 kPa, the cumulative axial strain of large-size graded crushed stone will still increase slowly after elastic deformations, but the strain rate will decrease rapidly. When the load is increased to 360 kPa, the cumulative axial strain of the large-size graded crushed stone increases rapidly during the entire process of cyclic loading, and the strain rate is maintained at a large value. It is observed that the deformation relationship and strain values of the specimens under cyclic rotating axial compression are similar to those in existing literature. Therefore, it is feasible to apply cyclic rotating axial compression to study the long-term stability of large-size graded crushed stone used in road base.
- In the second part, the critical load of graded crushed stone specimens under cyclic loading was calculated based on the shakedown theory and the new calculation method. The maximum critical shakedown load of large-size graded crushed stone was 229.655 kPa and the lowest was 207.619 kPa, respectively. The critical shakedown load of the conventional-size graded crushed stone was 188.276 kPa, making the critical shakedown load of the large-size graded crushed stone at least 10% higher than that of the conventional-size graded crushed stone. This indicates that the large-size graded crushed stone can be used in engineering applications, and it deforms less. The analysis clearly showed that the content of the large-size crushed stone, density, and skeleton structure performance influence the critical shakedown load. On the contrary, there is no significant difference between the critical failure load of the large-size graded crushed stone and that of the conventional-size graded crushed stone, with both being between 330 and 345 kPa.
- In the third part, the relationship between the cumulative axial strain and gradation of graded crushed stone was analyzed. When the content of large-size graded crushed stone with a size over 26.5 mm was between 22% and 28%, the higher the density was, and the larger the stiffness, the better the skeleton structure performance and the load bearing capacity were. Therefore, the content of the large-size crushed stone in the large-size graded crushed stone mixture should be controlled between 22% and 26%, and the density should be as high as possible.
- In addition, the skeleton structure performance of crushed stone influences the stability behavior of crushed stone specimens under cyclic loading was observed. The CBR values of 30 specimens and the deformation data of 126 specimens under cyclic loading test showed that there is a linear relationship between CBR values and cumulative axial strain and critical shakedown load of crushed stone specimens. The linear correlation coefficient between CBR values and critical shakedown load was 0.89279 and cumulative axial strain of specimens under a 280 kPa load cyclic loading was 0.9832, respectively.
- In short, this study proposed the method is using the vibration and rotary compaction method for preparation of specimens of crushed stone and testing their stability under cyclic rotating axial compression in the road material vibration rotatory compaction device. The method achieved simultaneous testing under axial pressure and shear stress using the device. The test data of 126 crushed stone specimens show that this is a simple and practical method for testing the stability of granular materials under cyclic loading, and it enables analyzing the correlations between deformation behavior and gradation parameters. Therefore, this method could be used for investigating the stability of granular materials under a cycling load.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lekarp, F.; Dawson, A. Modelling permanent deformation behaviour of unbound granular materials. Constr. Build. Mater. 1998, 12, 9–18. [Google Scholar] [CrossRef]
- Rahman, M.S.; Erlingsson, S. A model for predicting permanent deformation of unbound granular materials. Road Mater. Pavement Des. 2015, 16, 653–673. [Google Scholar] [CrossRef]
- Rahman, M.S.; Erlingsson, S. Predicting permanent deformation behaviour of unbound granular materials. Int. J. Pavement Eng. 2015, 16, 587–601. [Google Scholar] [CrossRef]
- Lekarp, F.; Isacsson, U.; Dawson, A. State of the art I:Resilient response of unbound aggregates. J. Transp. Eng. 2000, 126, 66–75. [Google Scholar] [CrossRef] [Green Version]
- Lekarp, F.; Isacsson, U.; Dawson, A. State of the art II:Permanent strain response of unbound aggregates. J. Transp. Eng. 2000, 126, 76–83. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.F.; Xu, M.; Song, E.X.; Cao, G.X. Large Scale Triaxial Testing on Mechanical Properties of Broken Limestone Under Various Stress Paths. Eng. Mech. 2012, 29, 195–201. [Google Scholar] [CrossRef]
- Fu, Q.L. Research on Composition Design Parameters and Method of Open-Graded Large Stone Asphalt Mixes. Ph.D. Thesis, Chang’an University of Technology, Xi’an, China, 2011. [Google Scholar] [CrossRef]
- Fu, Q.L.; Wei, J.G.; Zhou, X.Z. Evaluation of low temperature performance of open-graded large stone asphalt mixes. J. Build. Mater. 2020, 23, 896–903. [Google Scholar] [CrossRef]
- Dave, E.V.; Buttlar, W.G. Thermal reflective cracking of asphalt concrete overlays. Int. J. Pavement Eng. 2010, 11, 477–488. [Google Scholar] [CrossRef]
- Li, C.; Wang, F.; Deng, X.; Li, Y.; Zhao, S. Testing and prediction of the strength development of recycled-aggregate concrete with large particle natural aggregate. Materials 2019, 12, 1891. [Google Scholar] [CrossRef] [Green Version]
- Gu, F.; Zhang, Y.; Luo, X.; Sahin, H.; Lytton, R.L. Characterization and prediction of permanent deformation properties of unbound granular materials for Pavement ME Design. Constr. Build. Mater. 2017, 155, 584–592. [Google Scholar] [CrossRef] [Green Version]
- Zhang, J.Q. Study on Shakedown Behavior of Granular Material Used in Pavement and Shakedown Analysis of Pavement Structures. Ph.D. Thesis, South China University of Technology, Guangzhou, China, 2012. [Google Scholar]
- Werkmeister, S. Shakedown analysis of unbound granular materials using accelerated pavements test results from New Zealand’s CAPTIF facility. In Proceedings of the Geoshanghai International Conference, Shanghai, China, 6–8 June 2006; pp. 220–228. [Google Scholar]
- Werkmeister, S. Permanent Deformation Behaviour of Unbound Granular Materials in Pavement Constructions. Ph.D. Thesis, Dresden University of Technology, Dresden, Germany, 2003. [Google Scholar]
- Xiao, Y.; Zheng, K.; Chen, L.; Mao, J. Shakedown analysis of cyclic plastic deformation characteristics of unbound granular materials under moving wheel loads. Constr. Build. Mater. 2018, 167, 457–472. [Google Scholar] [CrossRef]
- Wichtmann, T.; Rondón, H.A.; Niemunis, A.; Triantafyllidis, T.; Lizcano, A. Prediction of permanent deformations in pavementsusing a high-cycle accumulation model. J. Geotech. Geoenviron. Eng. 2010, 136, 728–740. [Google Scholar] [CrossRef] [Green Version]
- Sas, W.; Głuchowski, A.; Gabryś, K.; Soból, E.; Szymański, A. Deformation Behavior of Recycled Concrete Aggregate during Cyclic and Dynamic Loading Laboratory Tests. Materials 2016, 9, 780. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Jiang, H.; Bian, X.; Jiang, J.; Chen, Y. Dynamic performance of high-speed railway formation with the rise of water table. Eng. Geol. 2016, 206, 18–32. [Google Scholar] [CrossRef]
- Chen, Q.; Abu-Farsakh, M.; Voyiadjis, G.Z.; Souci, G. Shakedown analysis of geogrid-reinforced granular base material. J. Mater. Civ. Eng. 2013, 337, 337–346. [Google Scholar] [CrossRef]
- Abu-Farsakh, M.Y.; Chen, Q. Evaluation of geogrid base reinforcement in flexible pavement using cyclic plate load testing. Int. J. Pavement Eng. 2011, 12, 275–288. [Google Scholar] [CrossRef]
- Chen, Z.D.; Yuan, W.J.; Gao, C.H. Research on design method of multilevel dense built gradation. China J. Highw. Transp. 2006, 19, 32–37. [Google Scholar] [CrossRef]
- Peng, B. Gradation design method based on method of I change. J. Wuhan Univ. Technol. 2005, 29, 751–754. [Google Scholar] [CrossRef]
- Sharp, R.W.; Booker, J.R. Shakedown of Pavements under Moving Surface Loads. J. Transp. Eng. 1984, 110, 1–14. [Google Scholar] [CrossRef]
- Sharp, R.W. Shakedown Analysis and the Design of Pavement under Moving Surface Load. Ph.D. Thesis, University of Sydney, Sydney, Australia, 1983. [Google Scholar]
- Dawson, A.R.; Mundy, M.J.; Huhtala, M. European Research into Granular Material for Pavement Bases and Subbases. Transp. Res. Rec. 2000, 1721, 91–99. [Google Scholar] [CrossRef]
- Zhou, Y.M.; Tan, Z.M.; Liu, S.W.; Niu, K.M. Analysis of Near-corner Stresses in Concrete Pavement Structure. Eng. Mech. 2010, 27, 105–110. [Google Scholar] [CrossRef] [PubMed]
- Werkmeister, S.; Dawson, A.R.; Wellner, F. Permanent Deformation Behavior of Granular Materials and the Shakedown Concept. Road Mater. Pavement Des. 2005, 6, 31–51. [Google Scholar] [CrossRef]
- Chen, W.B.; Feng, W.Q.; Yin, J.H.; Borana, L.; Chen, R.P. Characterization of permanent axial strain of granular materials subjected to cyclic loading based on shakedown theory. Constr. Build. Mater. 2019, 198, 751–761. [Google Scholar] [CrossRef]
- Tao, M.; Mohammad, L.N.; Nazzal, M.D.; Zhang, Z.; Wu, Z. Application of Shakedown Theory in Characterizing Traditional and Recycled Pavement Base Materials. J. Transp. Eng. 2010, 136, 214–222. [Google Scholar] [CrossRef]
- Pérez, I.; Medina, L.; Romana, M.G. Permanent deformation models for a granular material used in road pavements. Constr. Build. Mater. 2006, 20, 790–800. [Google Scholar] [CrossRef] [Green Version]
- Yin, J.H. Non-linear creep of soils in oedometer tests. Geotechnique 1999, 49, 699–707. [Google Scholar] [CrossRef]
- Test Methods of Aggregate for Highway Engineering. In JTGE42-2005, Occupation Standard of the People’s Republic of China; China Communications Press: Beijing, China, 2005.
- Xiao, J.; Zhang, D.; Wei, K.; Luo, Z. Shakedown behaviors of railway ballast under cyclic loading. Constr. Build. Mater. 2017, 155, 1206–1214. [Google Scholar] [CrossRef]
- Ge, H.; Sha, A.; Han, Z.; Xiong, X. Three-dimensional Characterization of Morphology and Abrasion Decay Laws for Coarse Aggregates. Constr. Build. Mater. 2018, 188, 56–67. [Google Scholar] [CrossRef]
- Ardah, A.; Chen, Q.M.; Abu-Farsakh, M.Y. Evaluating the Performance of Very Weak Subgrade Soils Treated/stablized with Cementitious Materials for Sustainable Pavements. Transp. Geotech. 2017, 11, 107–119. [Google Scholar] [CrossRef]
- Pouranian, M.R.; Haddock, J.E. Determination of voids in the mineral aggregate and aggregate skeleton characteristics of asphalt mixtures using a linear-mixture packing model. Constr. Build. Mater. 2018, 188, 292–304. [Google Scholar] [CrossRef]
- Zhang, J.W.; Wang, H.L.; Chen, S.J.; Li, Y.L. Bearing Deformation Characteristics of Large-Size Broken Rock. Journal of China Coal Society. J. China Coal Soc. 2018, 43, 1000–1007. [Google Scholar] [CrossRef]
- Li, J.; Yu, J.; Xie, J.; Ye, Q. Performance Degradation of Large-Sized Asphalt Mixture Specimen under Heavy Load and Its Affecting Factors Using Multifunctional Pavement Material Tester. Materials 2019, 12, 3814. [Google Scholar] [CrossRef] [PubMed] [Green Version]







| Aggregate (mm) | Crushing Value (%) | Apparent Density (g/cm3) | Dry Density (g/cm3) | Gross Relative Volumetric Density | Water Absorption Rate (%) | Needle Flake Content (%) |
|---|---|---|---|---|---|---|
| 19–53 | 21.57 | 2.729 | 2.672 | 2.646 | 1.24 | 7.30 |
| 9.5–19 | 14.08 | 2.753 | 2.691 | 2.662 | 1.32 | 6.58 |
| 4.75–9.5 | 2.735 | 2.702 | 2.684 | 0.70 | 6.89 | |
| 0–4.75 | 2.720 | 2.705 | 2.694 | 0.92 | ||
| Specification requirements | ≤28 | Measured data | Measured data | Measured data | Measured data | ≤15 |
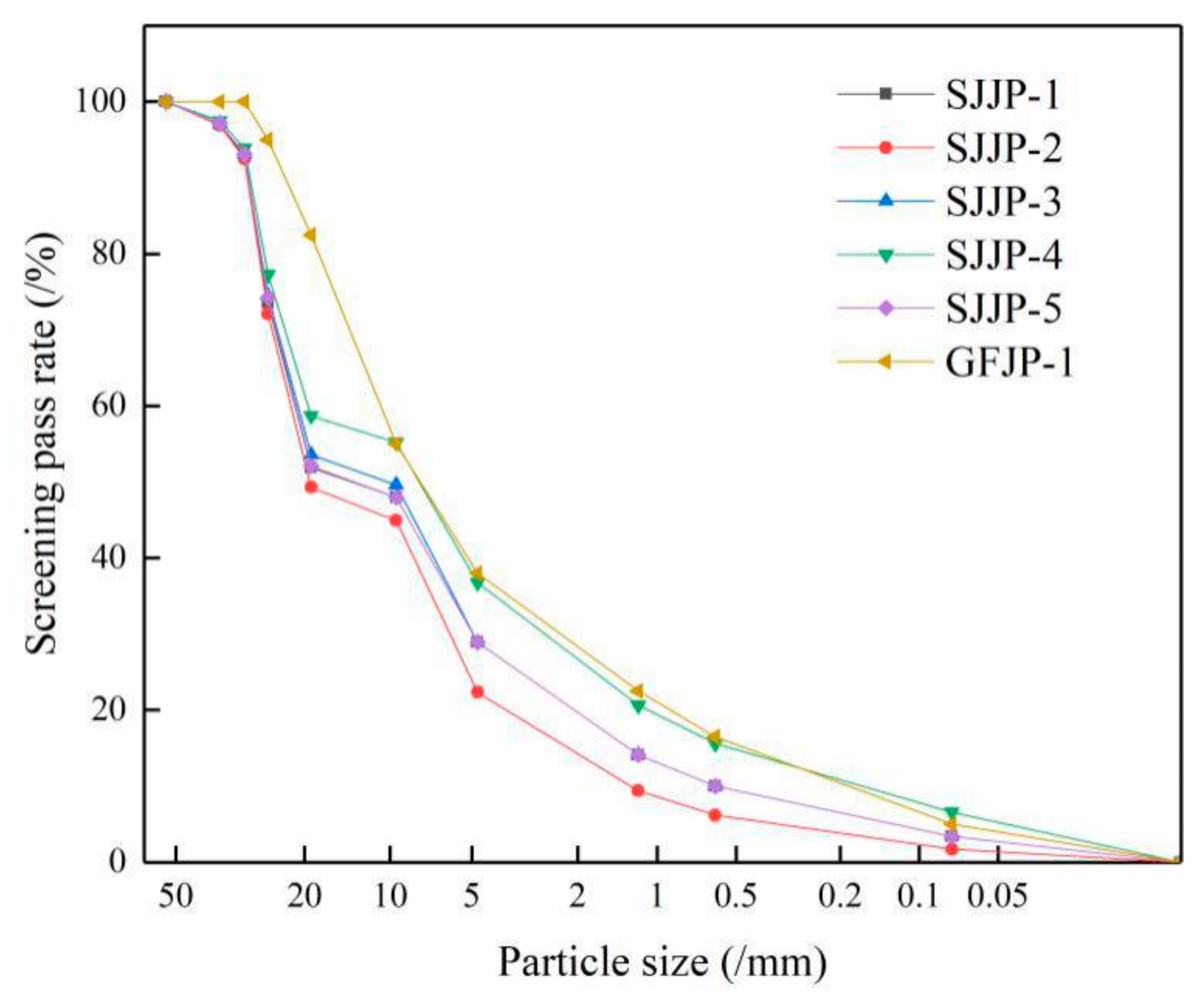
| Gradation Group | Mass Percentage Passing through Screen Hole (mm)/% | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 53 | 37.5 | 31.5 | 26.5 | 19 | 9.5 | 4.75 | 1.18 | 0.6 | 0.075 | |
| DG-1 | 100 | 97.05 | 92.87 | 73.57 | 51.93 | 48.00 | 28.92 | 14.13 | 9.98 | 3.43 |
| DG-2 | 100 | 96.89 | 92.47 | 72.12 | 49.29 | 44.96 | 22.35 | 9.41 | 6.18 | 1.70 |
| DG-3 | 100 | 97.15 | 93.11 | 74.48 | 53.58 | 49.62 | 28.92 | 14.13 | 9.98 | 3.43 |
| DG-4 | 100 | 97.47 | 93.87 | 77.30 | 58.71 | 55.19 | 36.77 | 20.63 | 15.59 | 6.58 |
| DG-5 | 100 | 97.15 | 93.10 | 74.23 | 52.09 | 47.98 | 28.92 | 14.13 | 9.98 | 3.43 |
| SG-1 | 100 | 100 | 100 | 95.00 | 82.50 | 55.00 | 38.00 | 22.50 | 16.50 | 5.00 |
| Gradation Group | 1/ns (160 kPa) | Behavior | 1/ns (200 kPa) | Behavior | 1/ns (240 kPa) | Behavior | 1/ns (280 kPa) | Behavior | 1/ns (320 kPa) | Behavior | 1/ns (360 kPa) | Behavior | 1/ns (400 kPa) | Behavior |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| DG-1 | 0.058 | A | 0.058 | A | 0.173 | B | 0.289 | B | 0.289 | B | 0.635 | C | 1.847 | C |
| DG-2 | 0.058 | A | 0.058 | A | 0.175 | B | 0.291 | B | 0.349 | B | 0.640 | C | 1.803 | C |
| DG-3 | 0.057 | A | 0.057 | A | 0.115 | B | 0.229 | B | 0.286 | B | 0.573 | C | 1.604 | C |
| DG-4 | 0.058 | A | 0.058 | A | 0.115 | B | 0.173 | B | 0.289 | B | 0.635 | C | 1.617 | C |
| DG-5 | 0.056 | A | 0.084 | A | 0.168 | B | 0.280 | B | 0.336 | B | 0.672 | C | 1.793 | C |
| SG-1 | 0.059 | A | 0.117 | B | 0.117 | B | 0.235 | B | 0.352 | B | 0.645 | C | 2.462 | C |
| Gradation Group | Critical Shakedown Load (kPa) | Critical Failure Load (kPa) |
|---|---|---|
| DG-1 | 214.609 | 336.763 |
| DG-2 | 214.359 | 331.684 |
| DG-3 | 229.655 | 340.627 |
| DG-4 | 229.474 | 336.763 |
| DG-5 | 207.619 | 331.667 |
| SG-1 | 188.276 | 331.195 |
| Gradation Group | Content of Large-Size Crushed Stone with Size over 26.5 mm (%) | Density (g/cm3) |
|---|---|---|
| DG-1 | 26.43 | 2.336 |
| DG-2 | 27.88 | 2.350 |
| DG-3 | 25.52 | 2.373 |
| DG-4 | 22.70 | 2.371 |
| DG-5 | 25.77 | 2.321 |
| SG-1 | 5.00 | 2.343 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, B.; Yang, T.; Qin, H.; Liu, Q. Laboratory Study on the Stability of Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression. Materials 2021, 14, 1584. https://doi.org/10.3390/ma14071584
Tan B, Yang T, Qin H, Liu Q. Laboratory Study on the Stability of Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression. Materials. 2021; 14(7):1584. https://doi.org/10.3390/ma14071584
Chicago/Turabian StyleTan, Bo, Tao Yang, Heying Qin, and Qi Liu. 2021. "Laboratory Study on the Stability of Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression" Materials 14, no. 7: 1584. https://doi.org/10.3390/ma14071584
APA StyleTan, B., Yang, T., Qin, H., & Liu, Q. (2021). Laboratory Study on the Stability of Large-Size Graded Crushed Stone under Cyclic Rotating Axial Compression. Materials, 14(7), 1584. https://doi.org/10.3390/ma14071584






