Laboratory Investigation and Numerical Modelling of Concrete Reinforced with Recycled Steel Fibers
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
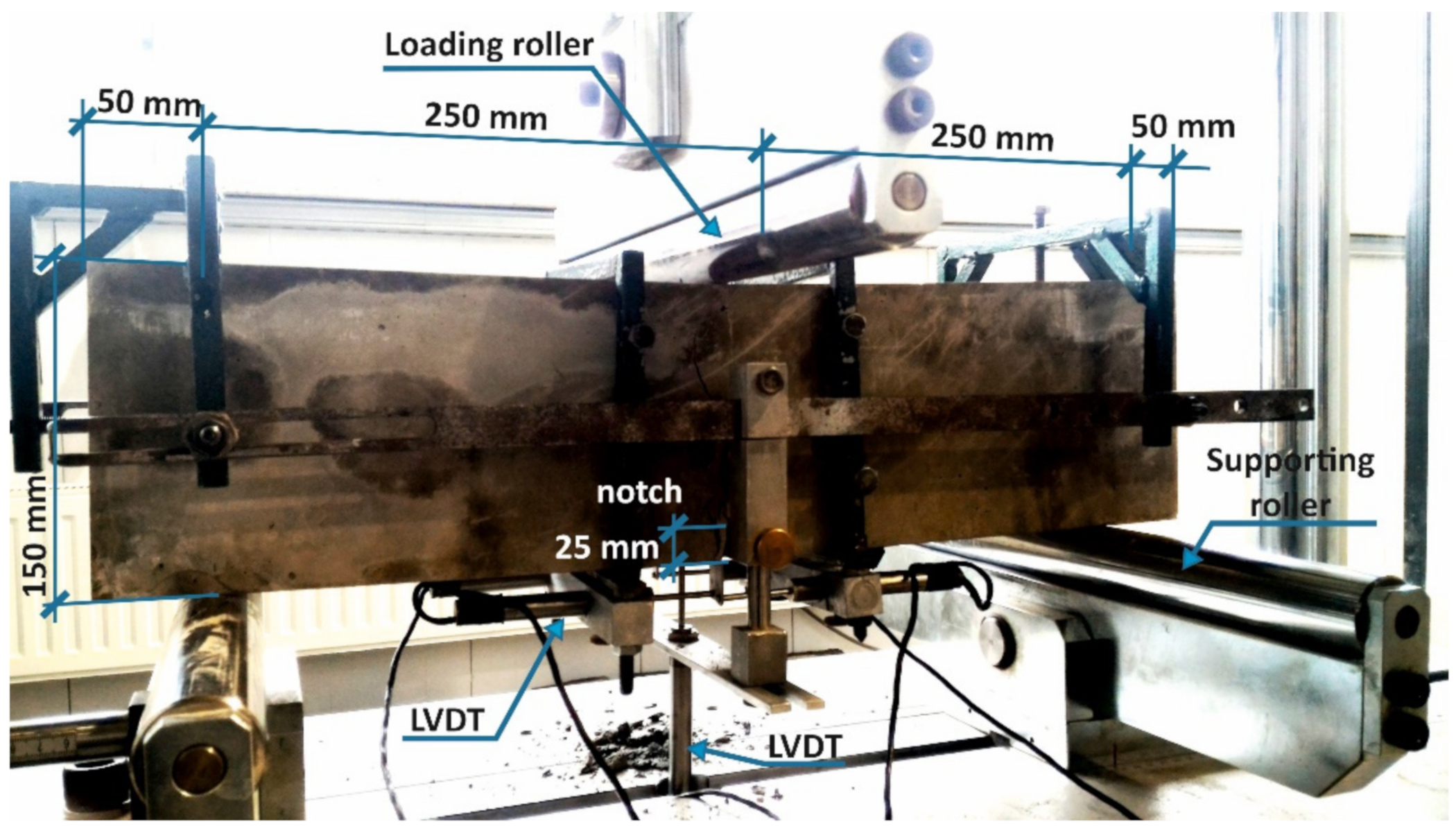
2.2. Experimental Procedure
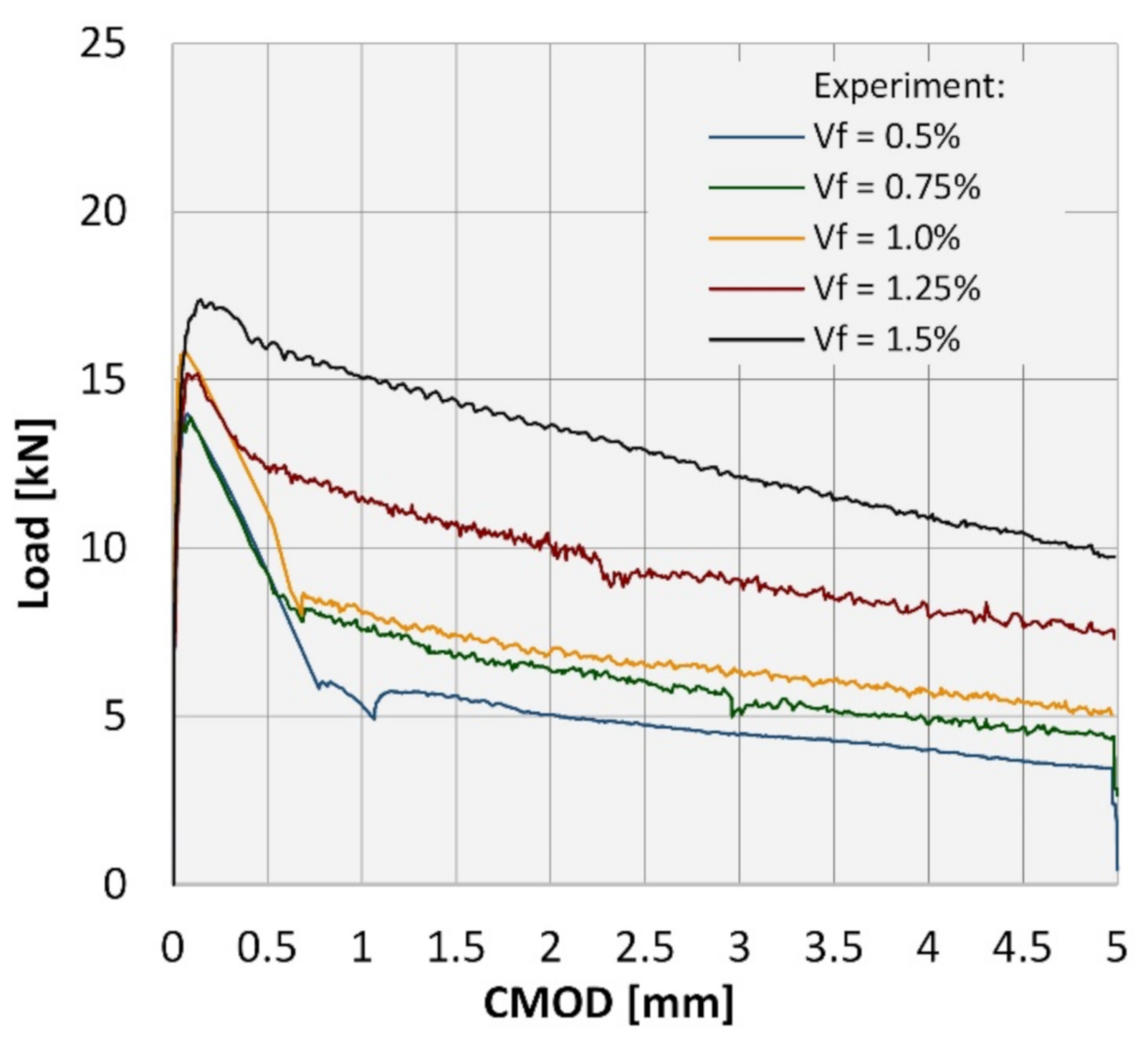
2.3. Experimental Results
3. Numerical Modelling
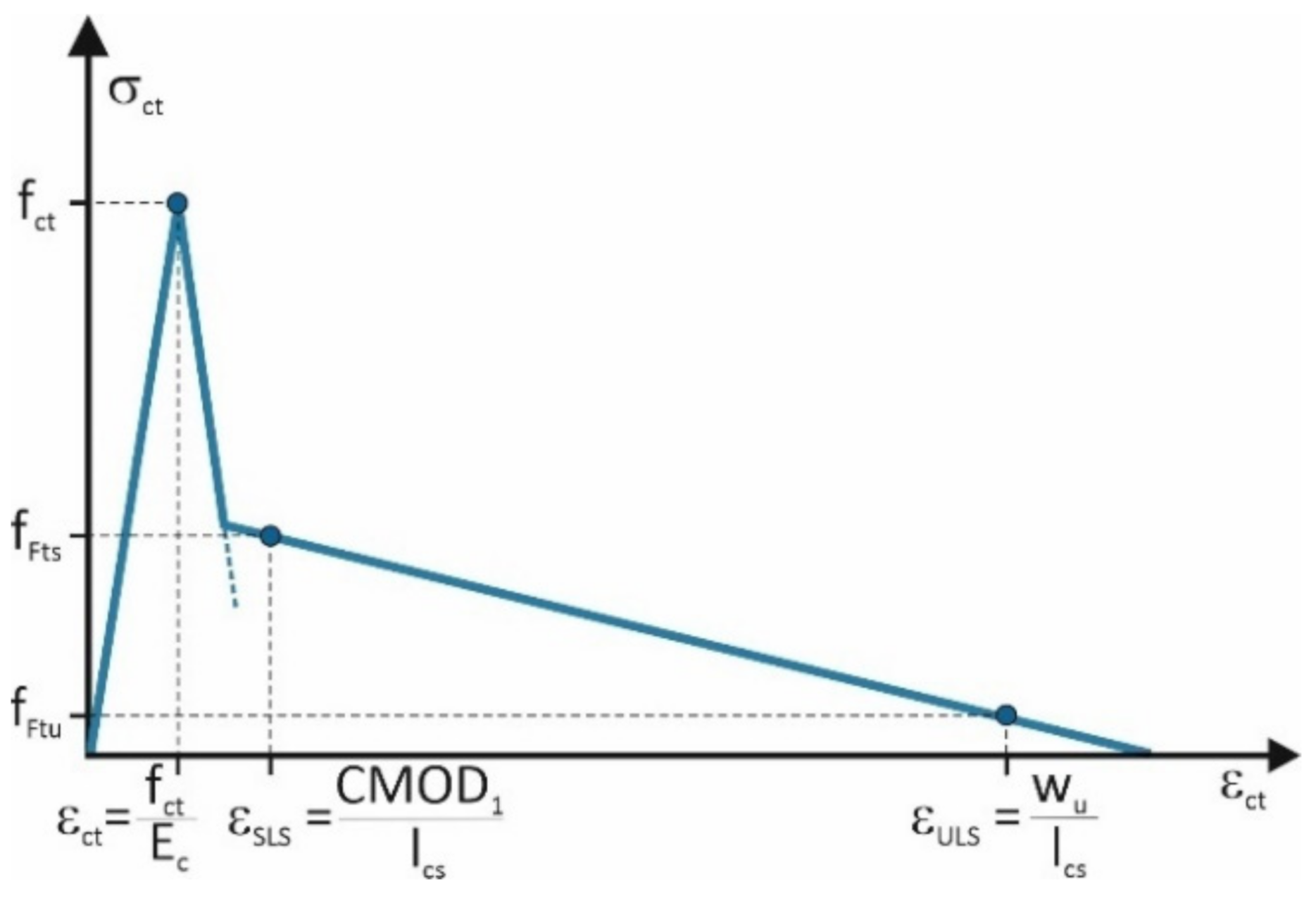
3.1. Material Model
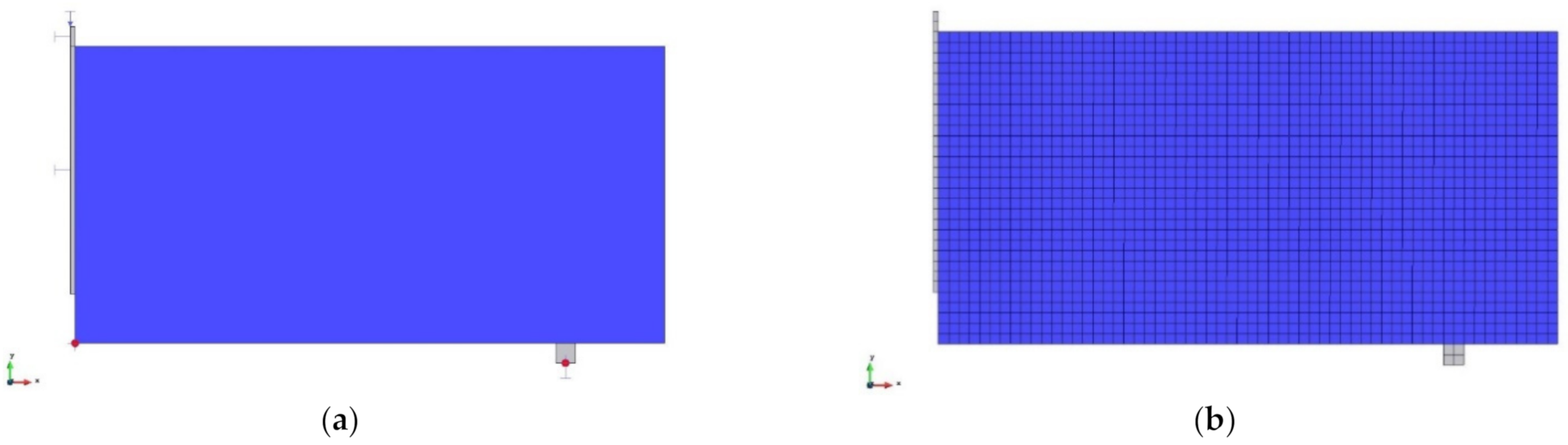
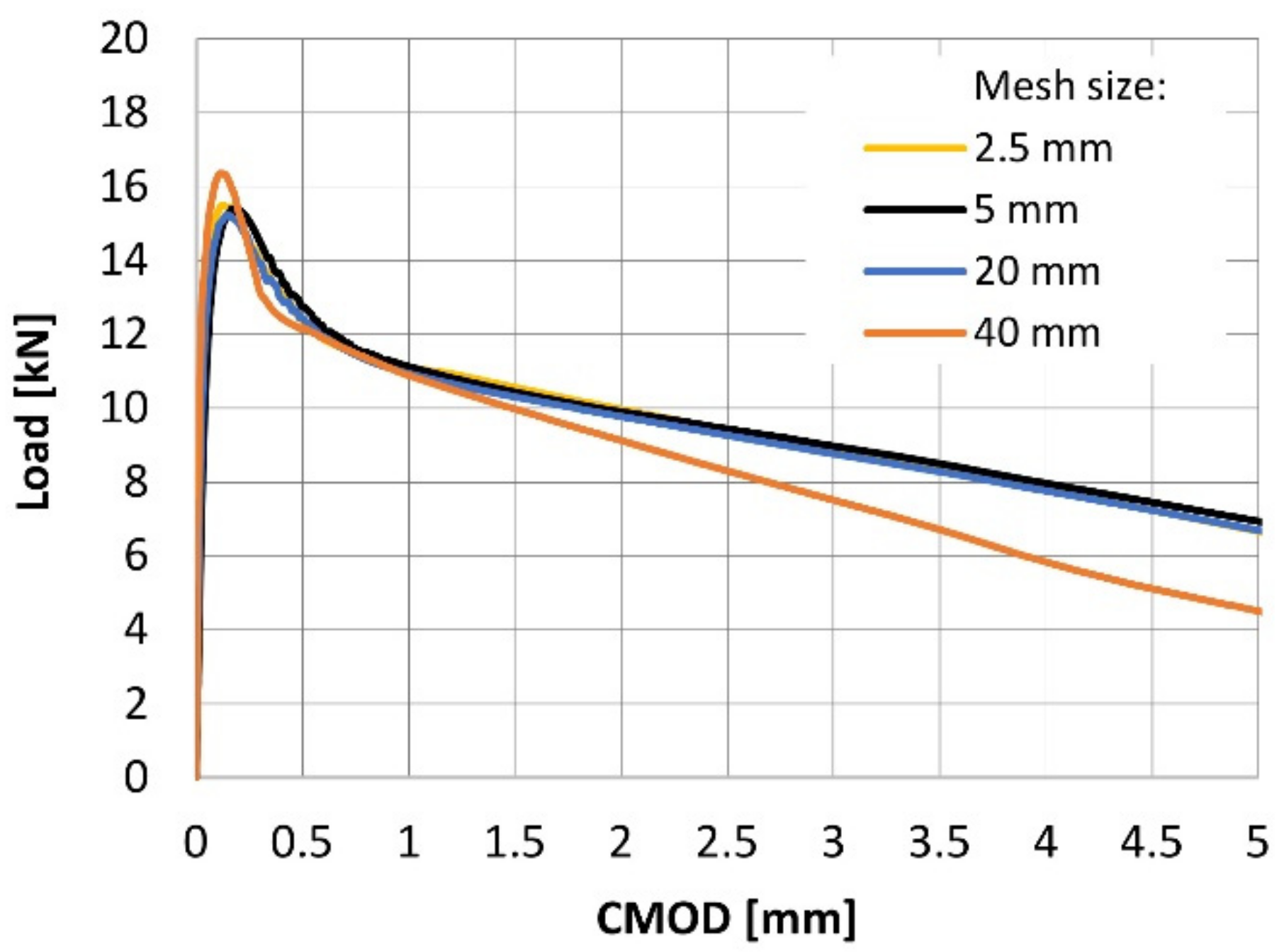
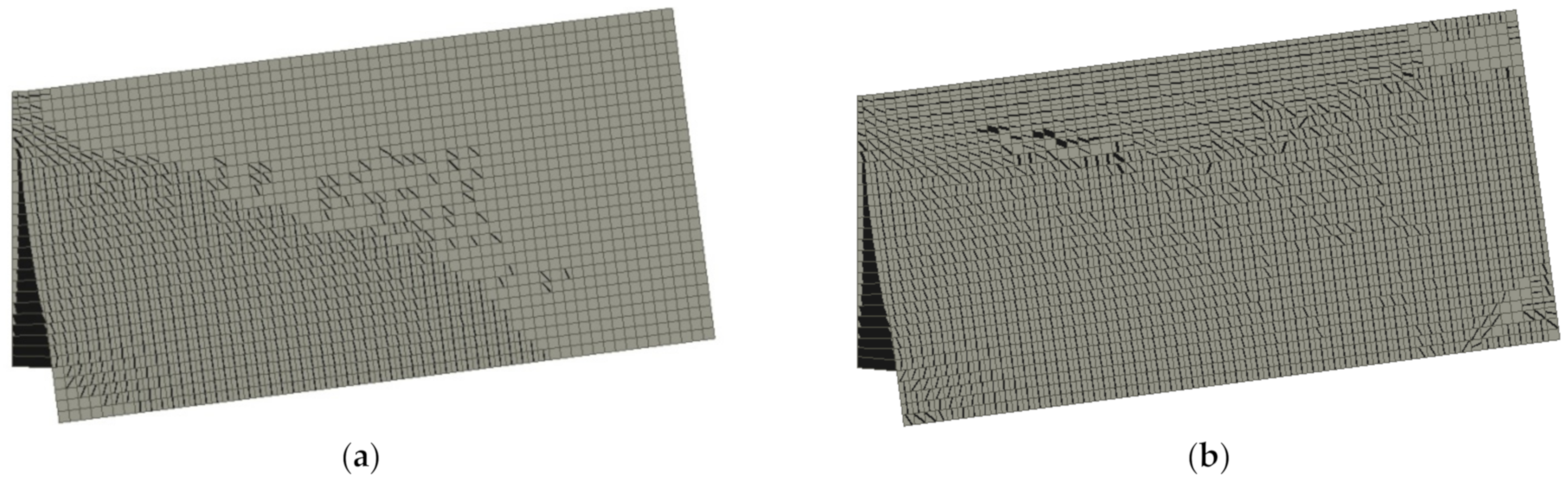
3.2. Numerical Simulation
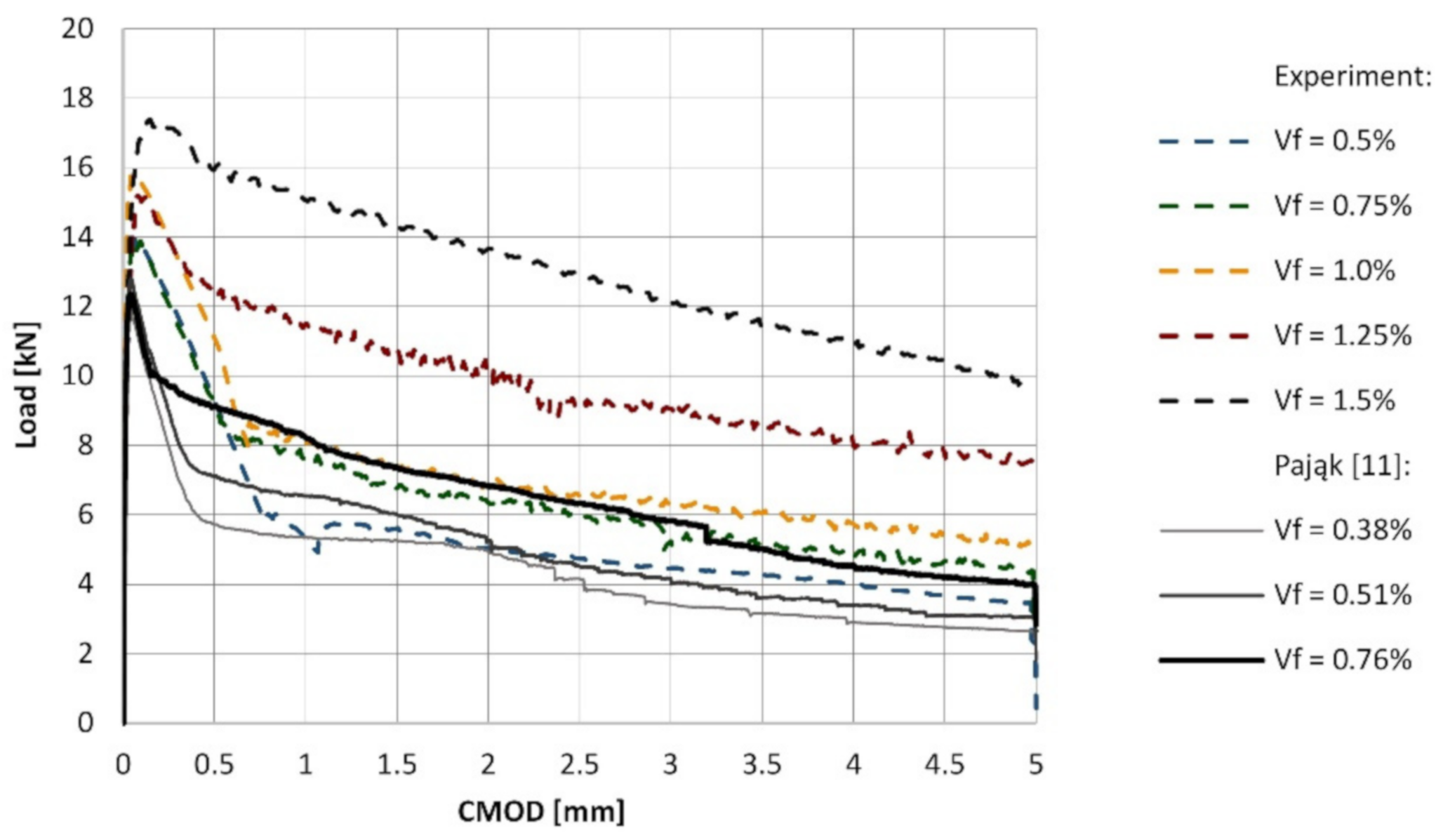
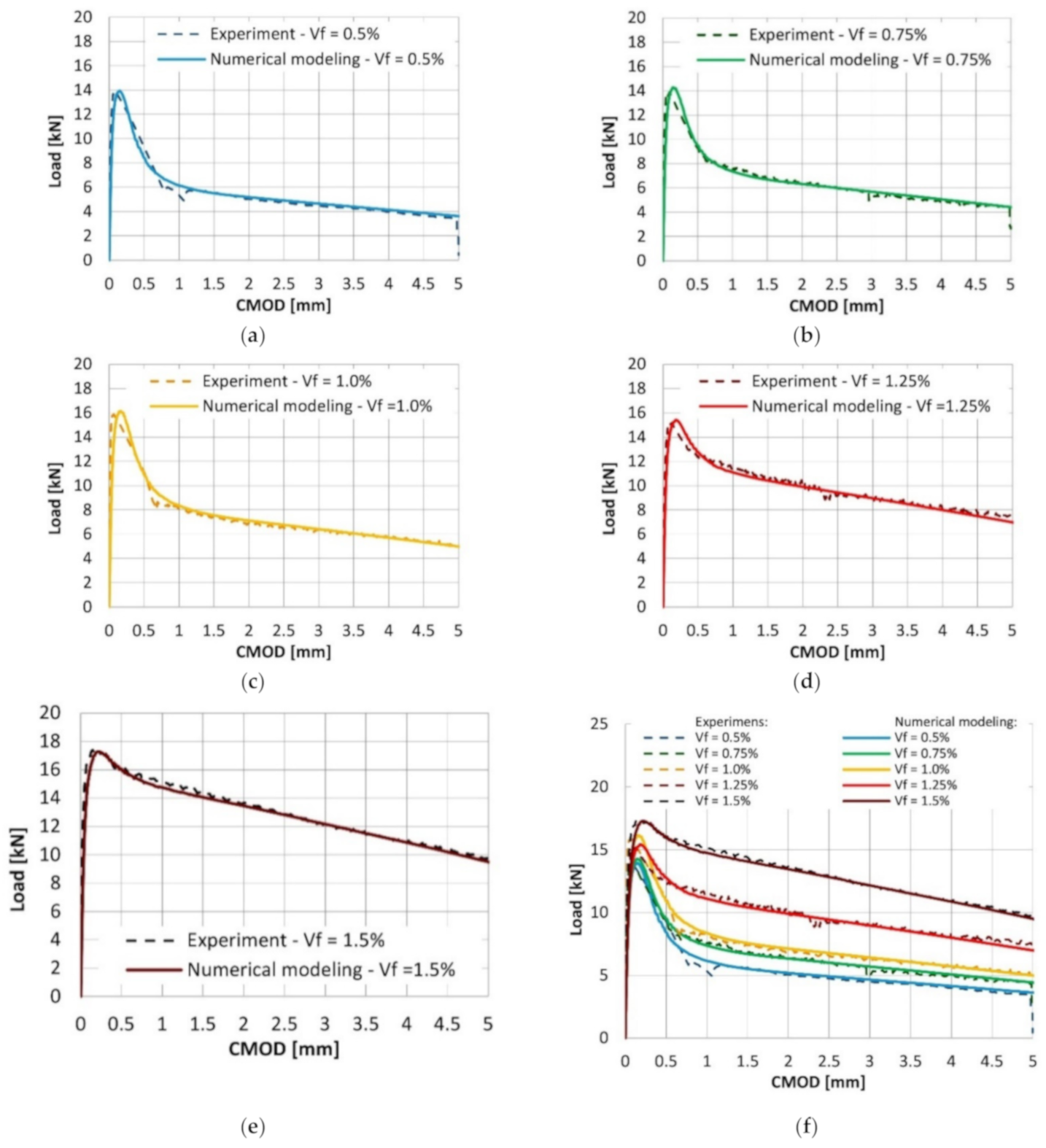
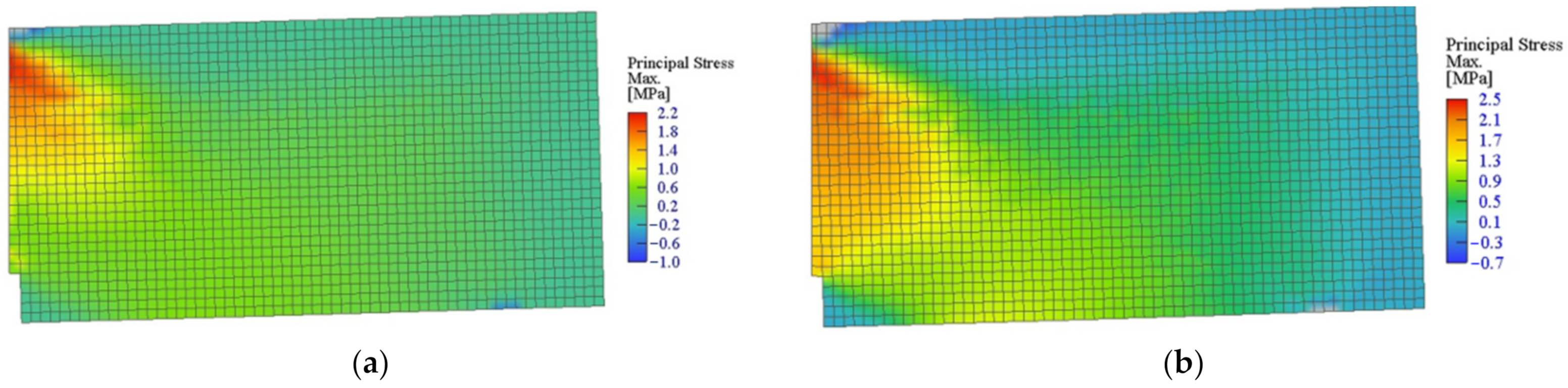
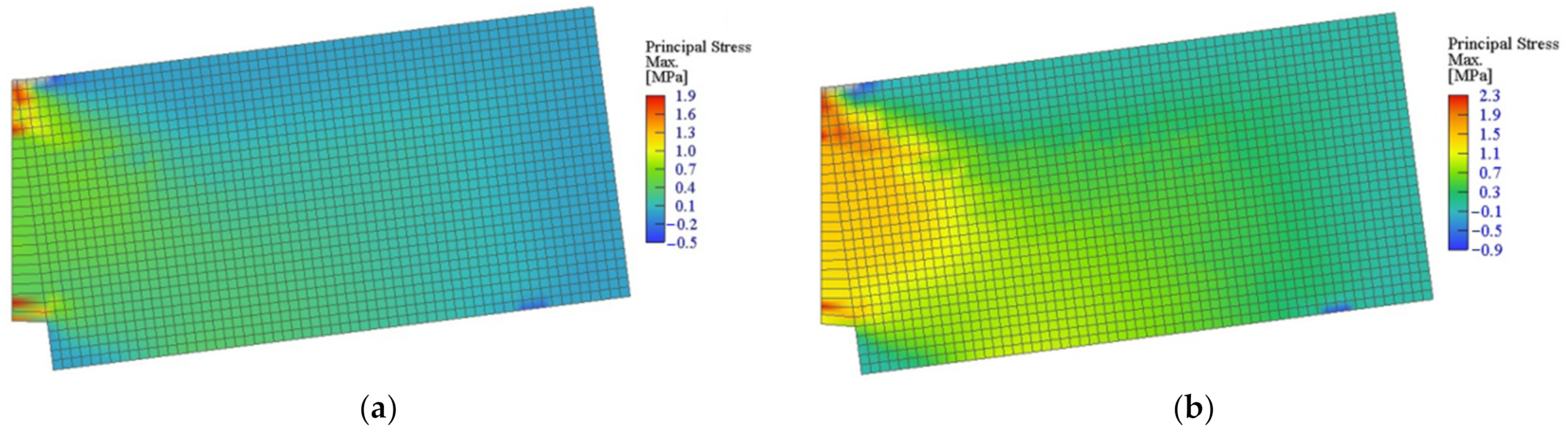
3.3. Results from Numerical Modelling
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Seitl, S.; Viszlay, V.; Domski, J.; Katzer, J. Fracture Mechanical Properties of Cement Based Composites with Various Amount of Waste Aggregates. Procedia Eng. 2017, 190, 345–351. [Google Scholar] [CrossRef]
- Hoffmann, C.; Schubert, S.; Leemann, A.; Motavalli, M. Recycled concrete and mixed rubble as aggregates: Influence of variations in composition on the concrete properties and their use as structural material. Constr. Build. Mater. 2012, 35, 701–709. [Google Scholar] [CrossRef]
- Di Prisco, M.; Colombo, M.; Dozio, D. Fibre-reinforced concrete in fib Model Code 2010: Principles, models and test validation. Struct. Concr. 2013, 14, 342–361. [Google Scholar] [CrossRef]
- Chalioris, C.E. Analytical approach for the evaluation of minimum fibre factor required for steel fibrous concrete beams under combined shear and flexure. Constr. Build. Mater. 2013, 43, 317–336. [Google Scholar] [CrossRef]
- Kytinou, V.K.; Chalioris, C.E.; Karayannis, C.G.; Elenas, A. Effect of steel fibers on the hysteretic performance of concrete beams with steel reinforcement-tests and analysis. Materials 2020, 13, 2923. [Google Scholar] [CrossRef]
- Bayard, O.; Plé, O. Fracture mechanics of reactive powder concrete: Material modelling and experimental investigations. Eng. Fract. Mech. 2003, 70, 839–851. [Google Scholar] [CrossRef]
- Paul, S.C.; van Zijl, G.P.A.G.; Šavija, B. Effect of fibers on durability of concrete: A practical review. Materials 2020, 13, 4562. [Google Scholar] [CrossRef] [PubMed]
- Ju, Y.; Liu, J.; Liu, H.; Tian, K.; Ge, Z. On the thermal spalling mechanism of reactive powder concrete exposed to high temperature: Numerical and experimental studies. Int. J. Heat Mass Transf. 2016, 98, 493–507. [Google Scholar] [CrossRef]
- Bragov, A.M.; Petrov, Y.V.; Karihaloo, B.L.; Konstantinov, A.Y.; Lamzin, D.A.; Lomunov, A.K.; Smirnov, I.V. Dynamic strengths and toughness of an ultra-high performance fibre reinforced concrete. Eng. Fract. Mech. 2013, 110, 477–488. [Google Scholar] [CrossRef]
- Brandt, A.M. Fibre reinforced cement-based (FRC) composites after over 40 years of development in building and civil engineering. Compos. Struct. 2008, 86, 3–9. [Google Scholar] [CrossRef]
- Pająk, M.; Ponikiewski, T. Flexural behavior of self-compacting concrete reinforced with different types of steel fibers. Constr. Build. Mater. 2013, 47, 397–408. [Google Scholar] [CrossRef]
- Domski, J.; Katzer, J.; Zakrzewski, M.; Ponikiewski, T. Comparison of the mechanical characteristics of engineered and waste steel fiber used as reinforcement for concrete. J. Clean. Prod. 2017, 158, 18–28. [Google Scholar] [CrossRef]
- Yoo, D.Y.; Kim, M.J.; Kim, S.W.; Park, J.J. Development of cost effective ultra-high-performance fiber-reinforced concrete using single and hybrid steel fibers. Constr. Build. Mater. 2017, 150, 383–394. [Google Scholar] [CrossRef]
- Aiello, M.A.; Leuzzi, F.; Centonze, G.; Maffezzoli, A. Use of steel fibres recovered from waste tyres as reinforcement in concrete: Pull-out behaviour, compressive and flexural strength. Waste Manag. 2009, 29, 1960–1970. [Google Scholar] [CrossRef] [PubMed]
- Leone, M.; Centonze, G.; Colonna, D.; Micelli, F.; Aiello, M.A. Fiber-reinforced concrete with low content of recycled steel fiber: Shear behaviour. Constr. Build. Mater. 2018, 161, 141–155. [Google Scholar] [CrossRef]
- Groli, G.; Pérez Caldentey, A.; Soto, A.G. Cracking performance of SCC reinforced with recycled fibres—An experimental study. Struct. Concr. 2014, 15, 136–153. [Google Scholar] [CrossRef]
- Caggiano, A.; Folino, P.; Lima, C.; Martinelli, E.; Pepe, M. On the mechanical response of Hybrid Fiber Reinforced Concrete with Recycled and Industrial Steel Fibers. Constr. Build. Mater. 2017, 147, 286–295. [Google Scholar] [CrossRef]
- Pająk, M. Concrete reinforced with various amounts of steel fibers reclaimed from end-of-life tires. MATEC Web Conf. 2019, 262, 06008. [Google Scholar] [CrossRef]
- Pająk, M. Application of fibers from end-of-life tires as a self-compacting concrete reinforcement—an experimental study. Archit. Civ. Eng. Environ. 2018, 11, 105–113. [Google Scholar] [CrossRef]
- Taerwe, L.; Stijn, M. Fib Model Code for Concrete Structures 2010; Ernst & Sohn, Wiley: Berlin, Germany, 2013. [Google Scholar]
- Atena Studio, version 5.4.1. Software for Technical Computation; Cervenka Consulting: Prague, Czech Republic, 2018.
- Funari, M.F.; Spadea, S.; Fabbrocino, F.; Luciano, R. A moving interface finite element formulation to predict dynamic edge debonding in FRP-strengthened concrete beams in service conditions. Fibers 2020, 8, 42. [Google Scholar] [CrossRef]
- Nonato Da Silva, C.A.; Ciambella, J.; Barros, J.A.O.; dos Santos Valente, T.D.; Costa, I.G. A multiscale model for optimizing the flexural capacity of FRC structural elements. Compos. Part B Eng. 2020, 200, 108325. [Google Scholar] [CrossRef]
- Domski, J.; Zakrzewski, M.; Laskowska-Bury, J. Bending moment of the waste fine aggregate concrete beams. Rocz. Ochr. Sr. 2019, 21, 1505–1514. [Google Scholar]
- EN. 1766 Eurocode: Products and Systems for the Protection and Repair of Concrete Structures—Test Methods—Reference Concretes for Testing; European Commission Standards; European Union: Brussels, Belgium, 2017.
- BS:EN. 1008 Mixing Water for Concrete. Specification for Sampling, Testing and Assessing the Suitability of Water, Including Water Recovered from Processes in the Concrete Industry, as Mixing Water for Concrete; British Standards Institution: London, UK, 2002.
- EN. 12350-4 Testing Fresh Concrete. Degree of Compactability; European Commission Standards; European Union: Brussels, Belgium, 2009.
- EN. 14651 Test Method for Metallic Fibered Concrete—Measuring the Flexural Tensile Strength (Limit or Proportionality (LOP), Residual); European Commission Standards; European Union: Brussels, Belgium, 2007.
- EN. 206-1 Concrete—Part 1: Specification, Performance, Production and Conformity; European Commission Standards; European Union: Brussels, Belgium, 2000.
- Cervenka, V.; Jendele, L.; Cervenka, J. ATENA Program Documentation. Part 1—Theory; Cervenka Consulting: Prague, Czech Republic, 2018. [Google Scholar]







| Cement CEM I 42.5 R (kg/m3) | Water (kg/m3) | Aggregate (kg/m3) | Fiber Reinforcement Ratio | w/c |
|---|---|---|---|---|
| 350 | 192.5 | 1840 | 0.5%; 0.75%; 1.0%; 1.25%; 1.5% | 0.55 |
| Parameter | Average (mm) | Standard Deviation (mm) | Median (mm) | Minimum Value (mm) | Maximum Value (mm) |
|---|---|---|---|---|---|
| Diameter | 0.306 | 0.183 | 0.250 | 0.050 | 1.350 |
| Length | 13.29 | 5.80 | 12.57 | 2.90 | 27.90 |
| Mix | S (mm) | Degree of Compactability c | Fresh Concrete Consistency Class |
|---|---|---|---|
| 0.5% | 25 | 1.07 | C3 |
| 0.75% | 20 | 1.05 | C3 |
| 1.0% | 35 | 1.10 | C3 |
| 1.25% | 45 | 1.13 | C2 |
| 1.5% | 45 | 1.13 | C2 |
| Mix | Compressive Strength (Cylinders) (MPa) | Limit of Proportionality (MPa) | Residual Flexural Tensile Strength (MPa) | fR.3/fR.1 | fR.1/fLOP | |||
|---|---|---|---|---|---|---|---|---|
| fLOP | fR.1 | fR.2 | fR.3 | fR.4 | ||||
| 0.5% | 39.88 | 4.31 | 2.97 | 1.79 | 1.52 | 1.36 | 0.51 | 0.69 |
| 0.75% | 40.29 | 4.33 | 2.94 | 2.17 | 1.93 | 1.65 | 0.66 | 0.68 |
| 1.0% | 41.48 | 5.03 | 3.55 | 2.37 | 2.09 | 1.94 | 0.59 | 0.71 |
| 1.25% | 40.08 | 4.47 | 3.97 | 3.43 | 3.03 | 2.72 | 0.76 | 0.89 |
| 1.5% | 42.79 | 4.85 | 5.13 | 4.60 | 4.13 | 3.67 | 0.81 | 1.06 |
| Mix | Data from Laboratory Investigation | Basic Material Model | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Compressive Strength fcm (MPa) | Residual Flexural Tensile Strength (MPa) | Tensile Strength (MPa) | Flexural Tensile Strength (MPa) | Tensile Strain | |||||
| fR.1 | fR.3 | fctm | fFts | fftu | εct | εSLS | εULS | ||
| 0.5% | 39.88 | 2.97 | 1.52 | 3.50 | 1.34 | 0.16 | 0.0719 | 4 | 20 |
| 0.75% | 40.29 | 2.94 | 1.93 | 3.53 | 1.32 | 0.38 | 0.0721 | 4 | 20 |
| 1.0% | 41.48 | 3.55 | 2.09 | 3.59 | 1.60 | 0.34 | 0.0728 | 4 | 20 |
| 1.25% | 40.08 | 3.97 | 3.03 | 3.51 | 1.79 | 0.72 | 0.0720 | 4 | 20 |
| 1.5% | 42.79 | 5.13 | 4.13 | 3.67 | 2.31 | 1.04 | 0.0736 | 4 | 20 |
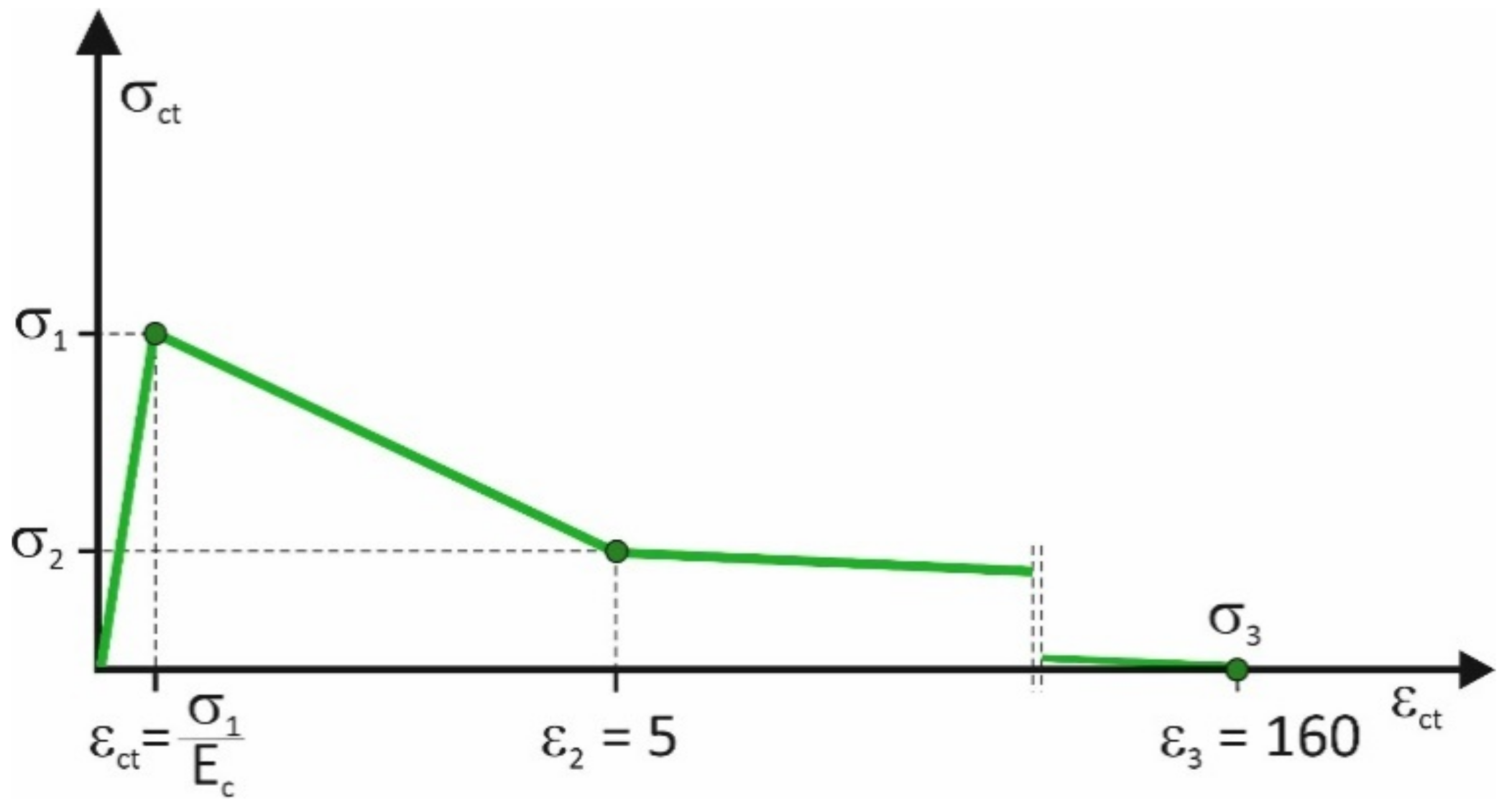
| Mix | Data for the Final Material Model | Final Material Model | ||||||
|---|---|---|---|---|---|---|---|---|
| Limit of Proportionality fL (MPa) | Residual Flexural Tensile Strength for CMOD = 2.5 mm (MPa) fR.3 | Tensile Stress (MPa) | Tensile Strain | |||||
| σ1 | σ2 | σ3 | ε1 | ε2 | ε3 | |||
| 0.5% | 4.28 | 1.52 | 1.96 | 0.58 | 0 | 0.0603 | 5 | 160 |
| 0.75% | 4.33 | 1.93 | 1.98 | 0.72 | 0 | 0.0608 | 5 | 160 |
| 1.0% | 5.04 | 2.09 | 2.30 | 0.81 | 0 | 0.0701 | 5 | 160 |
| 1.25% | 4.47 | 3.03 | 2.04 | 1.16 | 0 | 0.0628 | 5 | 160 |
| 1.5% | 4.86 | 4.13 | 2.22 | 1.58 | 0 | 0.0668 | 5 | 160 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pająk, M.; Krystek, M.; Zakrzewski, M.; Domski, J. Laboratory Investigation and Numerical Modelling of Concrete Reinforced with Recycled Steel Fibers. Materials 2021, 14, 1828. https://doi.org/10.3390/ma14081828
Pająk M, Krystek M, Zakrzewski M, Domski J. Laboratory Investigation and Numerical Modelling of Concrete Reinforced with Recycled Steel Fibers. Materials. 2021; 14(8):1828. https://doi.org/10.3390/ma14081828
Chicago/Turabian StylePająk, Małgorzata, Małgorzata Krystek, Mateusz Zakrzewski, and Jacek Domski. 2021. "Laboratory Investigation and Numerical Modelling of Concrete Reinforced with Recycled Steel Fibers" Materials 14, no. 8: 1828. https://doi.org/10.3390/ma14081828
APA StylePająk, M., Krystek, M., Zakrzewski, M., & Domski, J. (2021). Laboratory Investigation and Numerical Modelling of Concrete Reinforced with Recycled Steel Fibers. Materials, 14(8), 1828. https://doi.org/10.3390/ma14081828







