A New Type of Hierarchical Honeycomb in-Plane Impact Study
Abstract
:1. Introduction
2. Materials and Methods
2.1. Theoretical Method of Second-Order Hierarchical Honeycomb (SHH) Static Failure Stress during Impact in the x and z Directions
2.2. Theoretical Method of SHH Dynamic Failure Stress during Impact in the x and z Directions
3. Results and Discussion
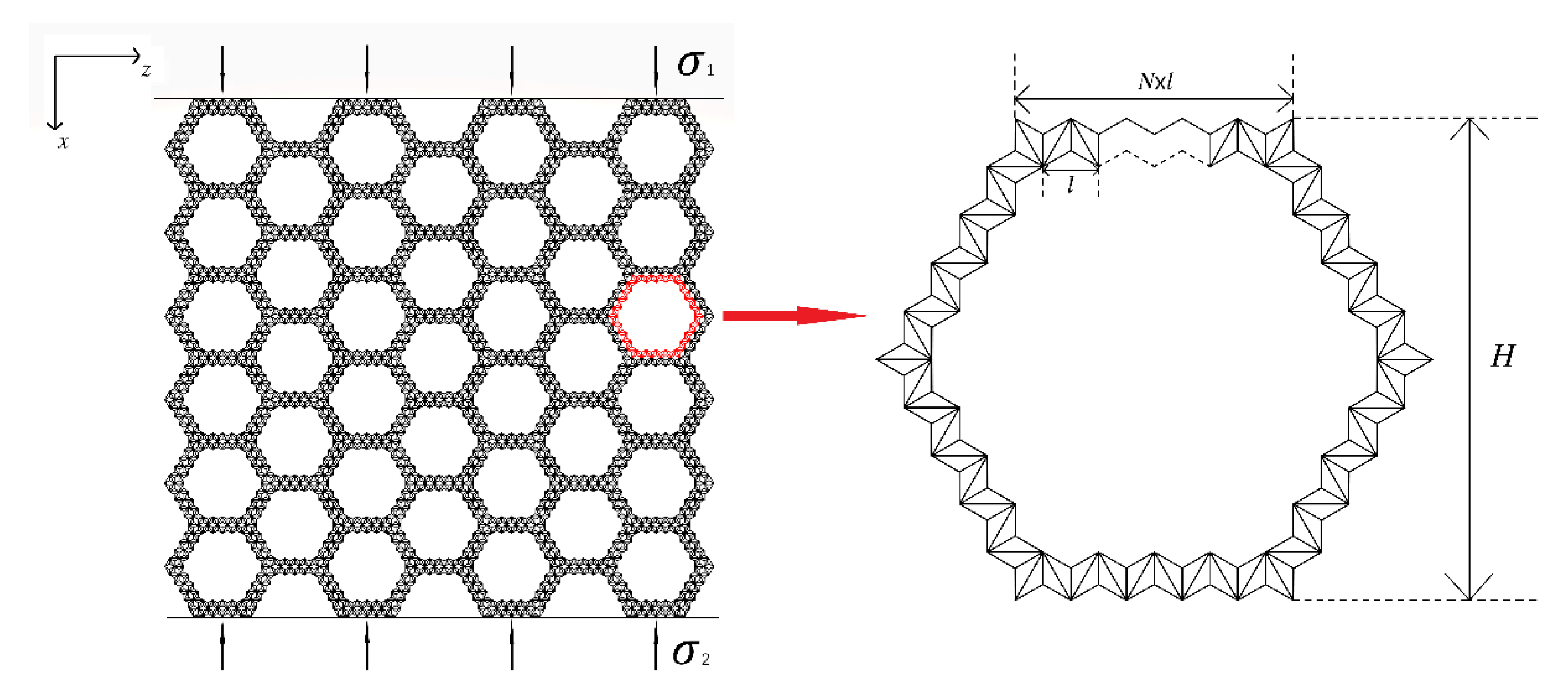
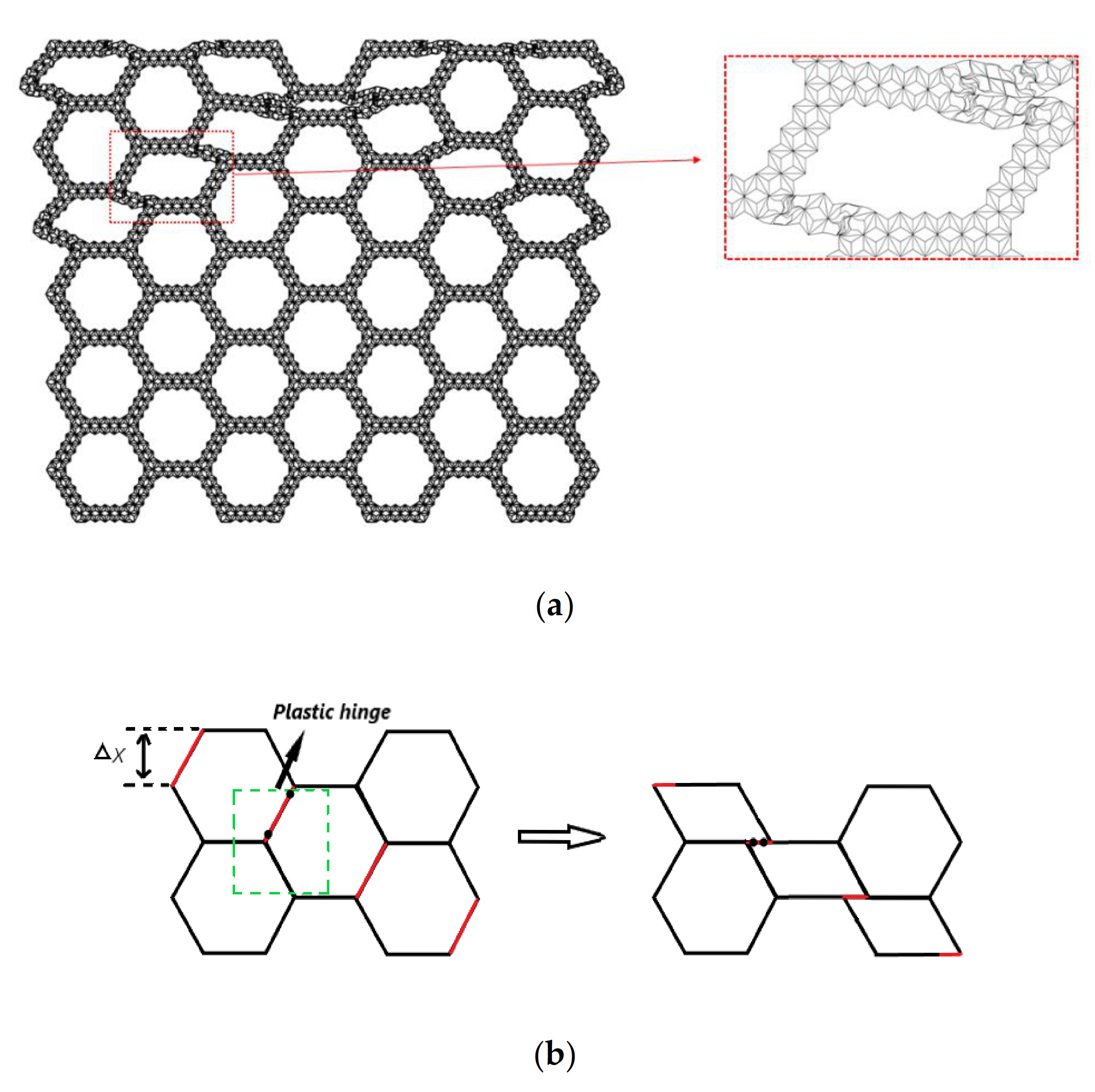
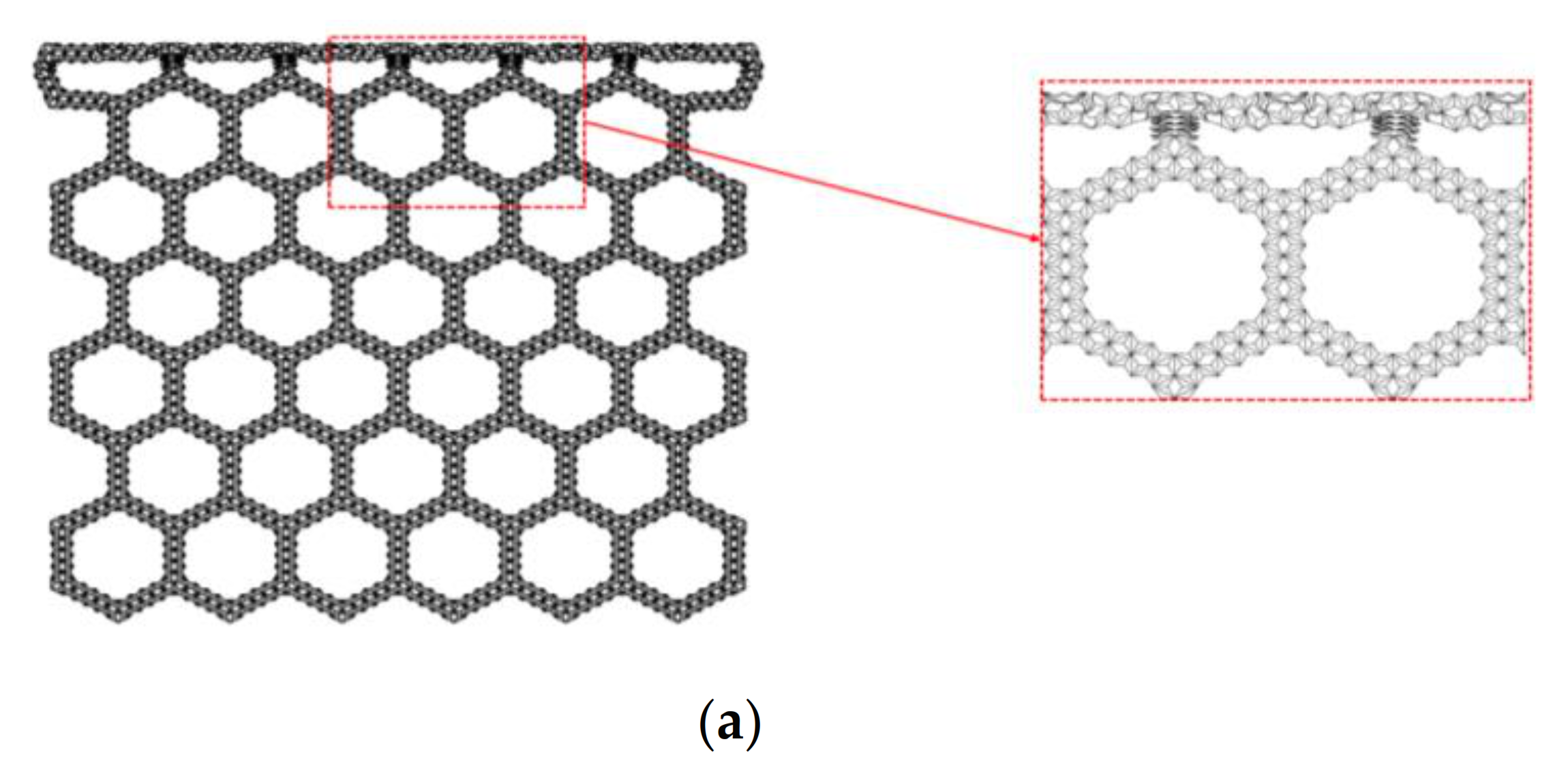
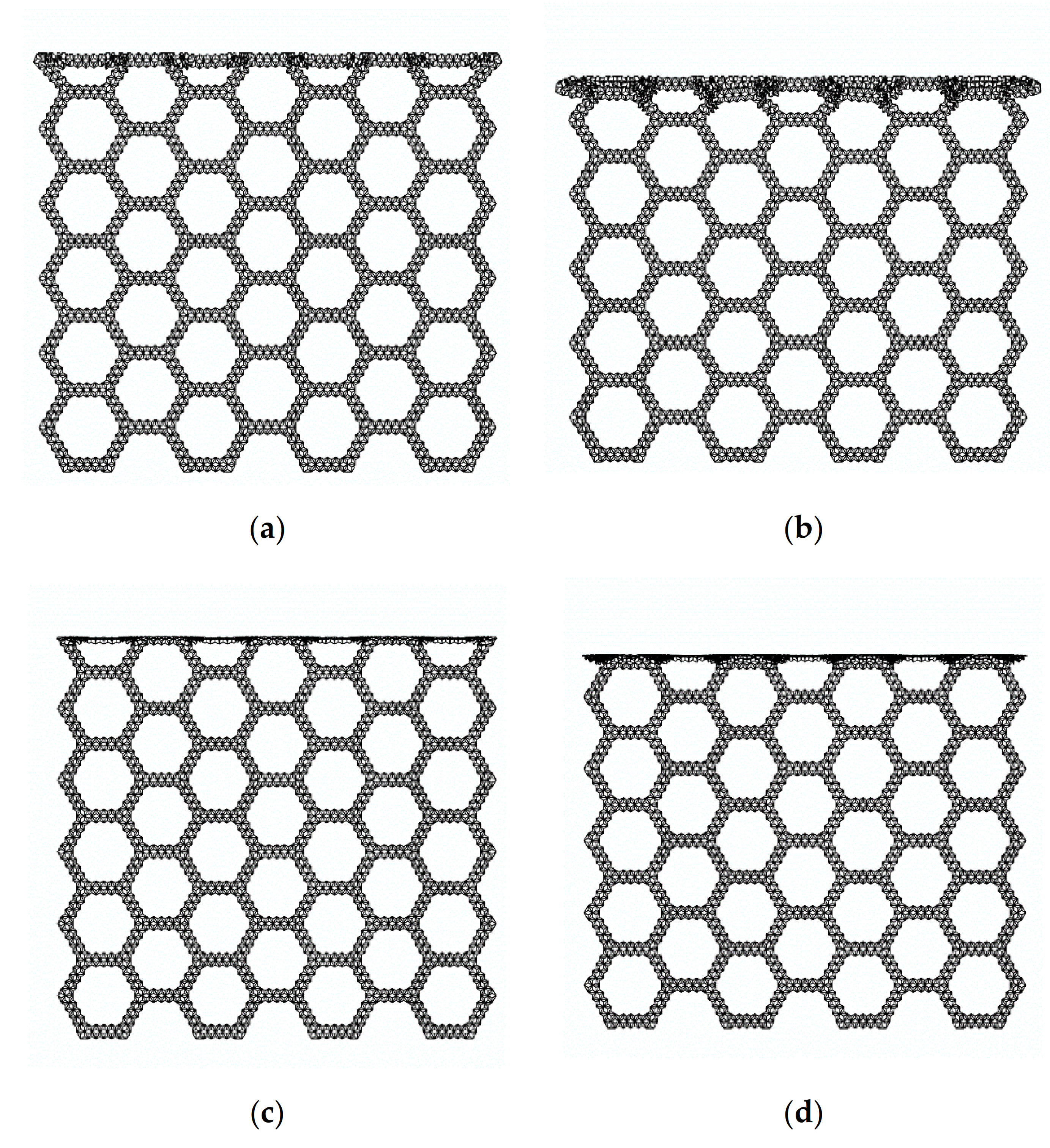
3.1. Second-Order Hierarchical Honeycomb (SHH) and Finite Element Modeling
3.2. Quasi-Static Collapse
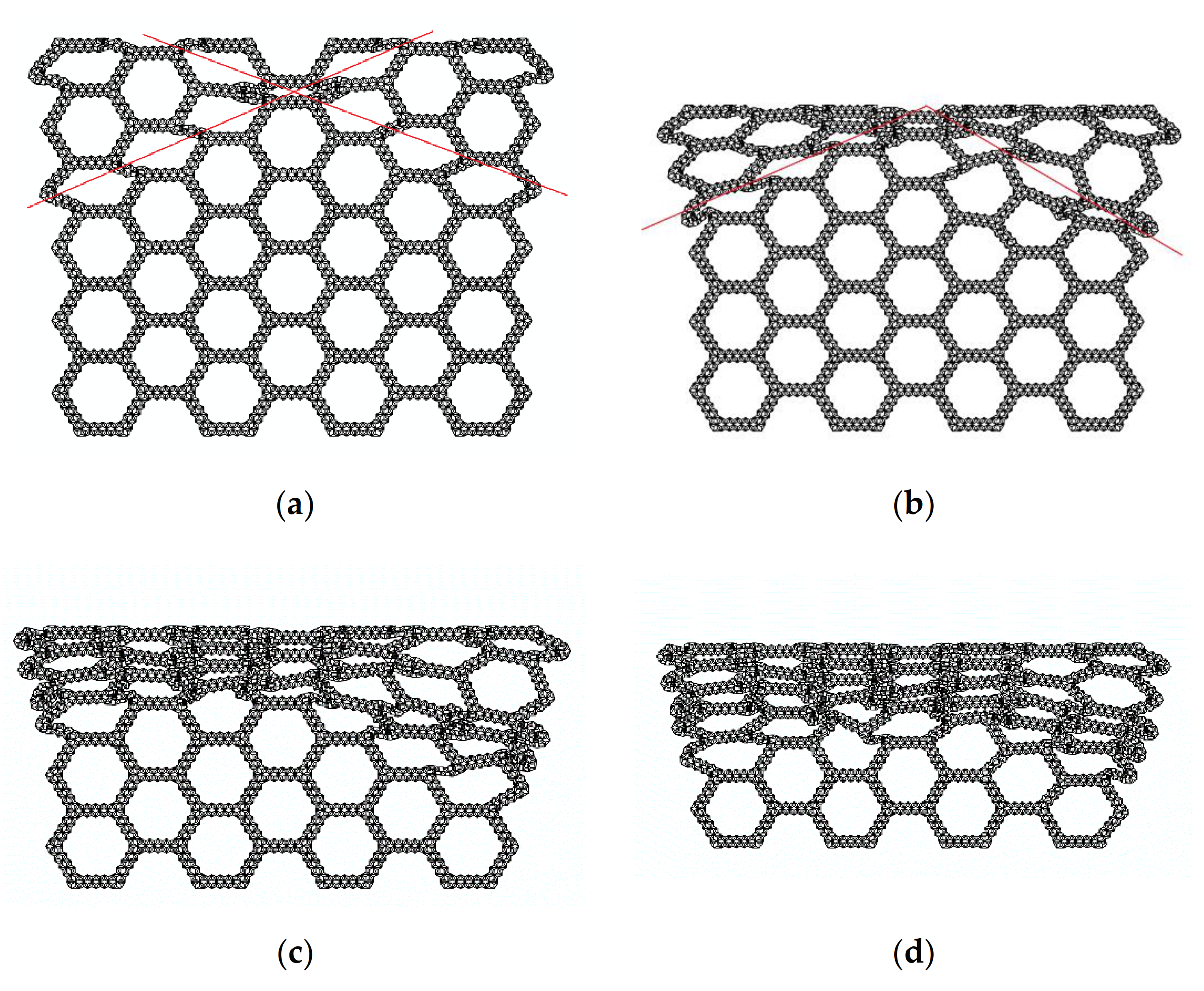
3.2.1. Uniaxial Compression in the Direction
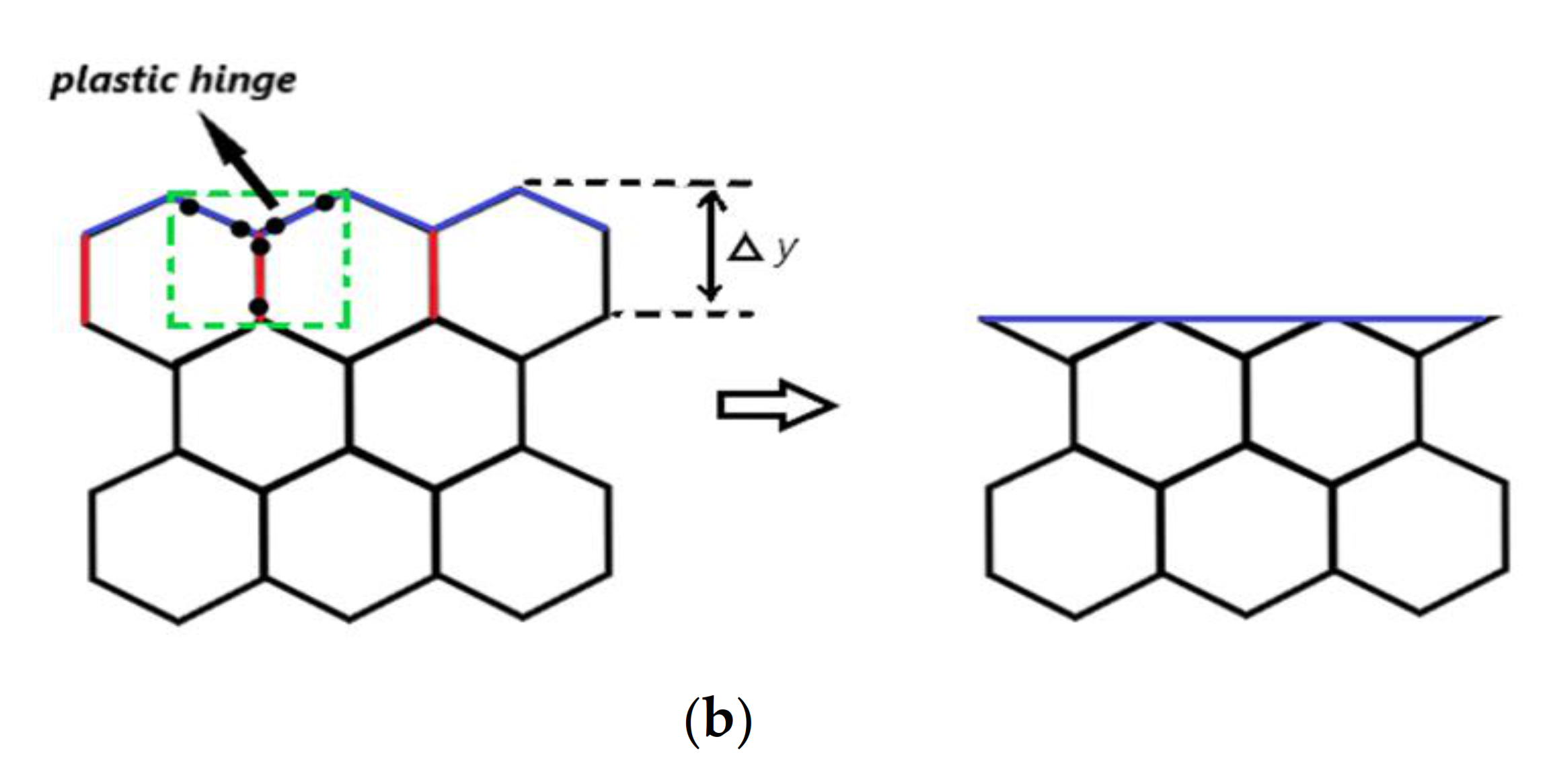
3.2.2. Collapse Model of SHH
3.2.3. Augmented Double Arrow Honeycomb’s (ADAH’s) FE Model
3.2.4. Theoretical Model of the ADAH
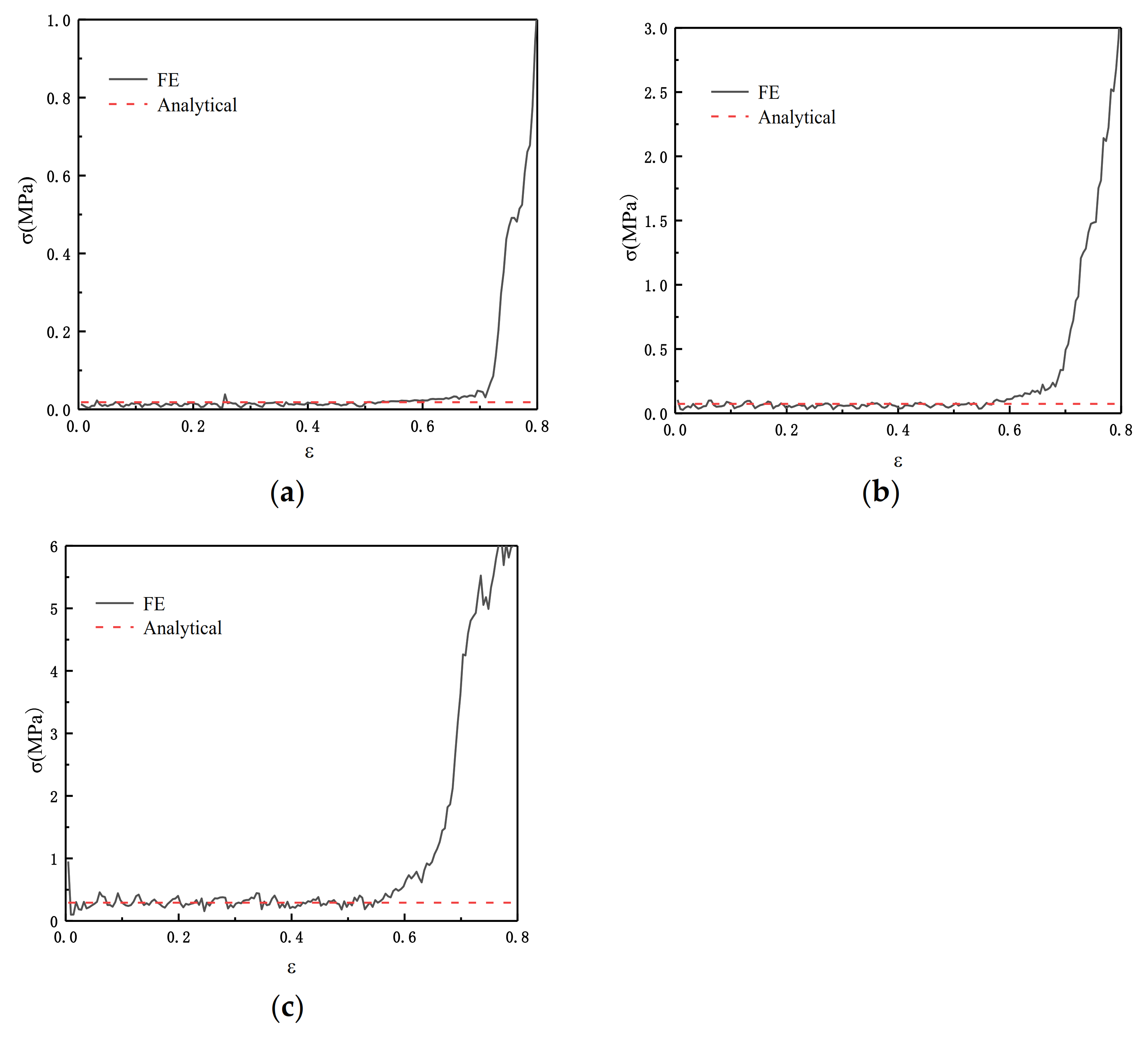
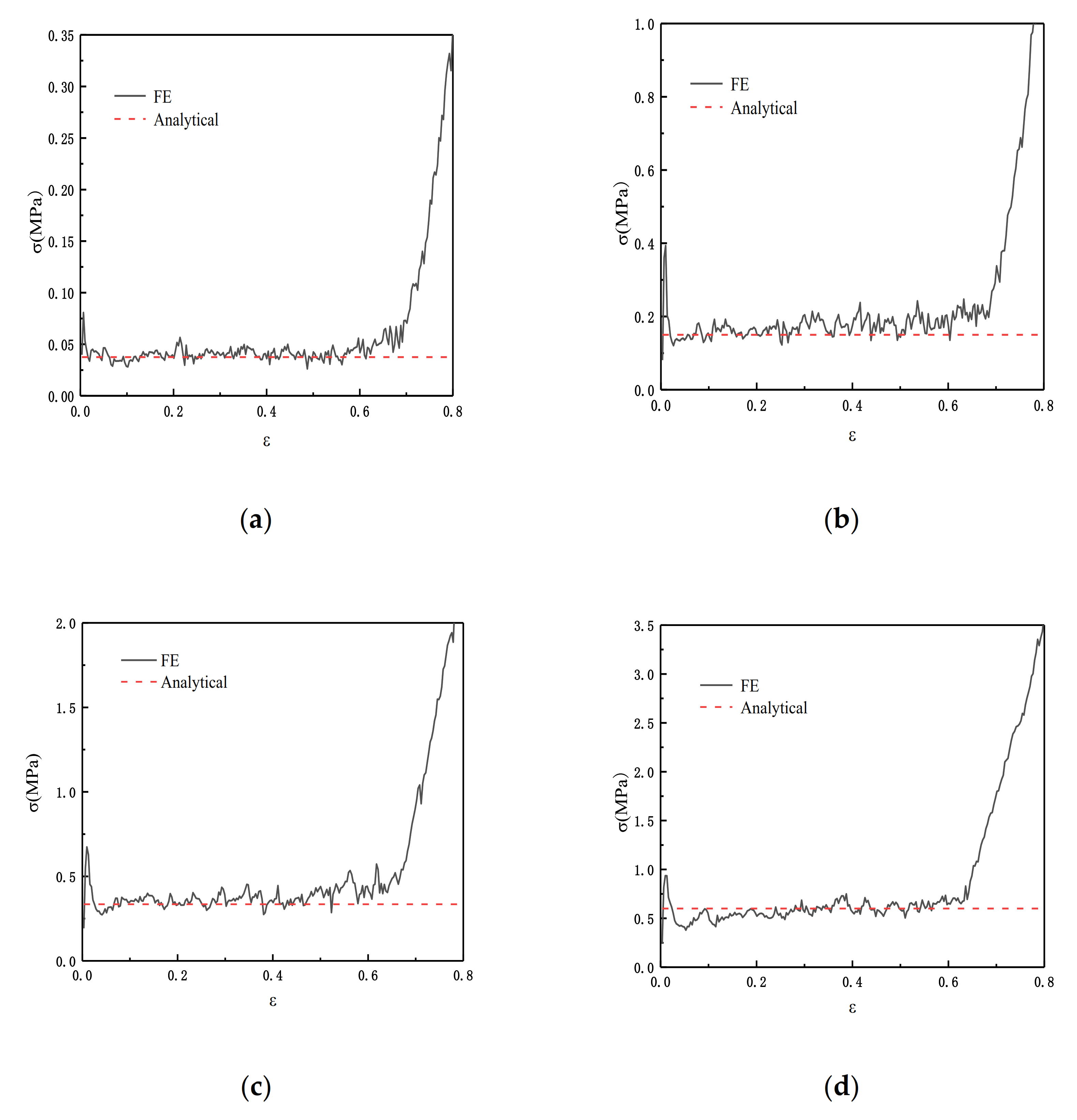
3.2.5. Comparison of Theoretical Analysis of the ADAH with the Finite Element Simulation
3.2.6. The Failure Stress of the SHH Structure
3.3. Uniaxial Compression in the Direction
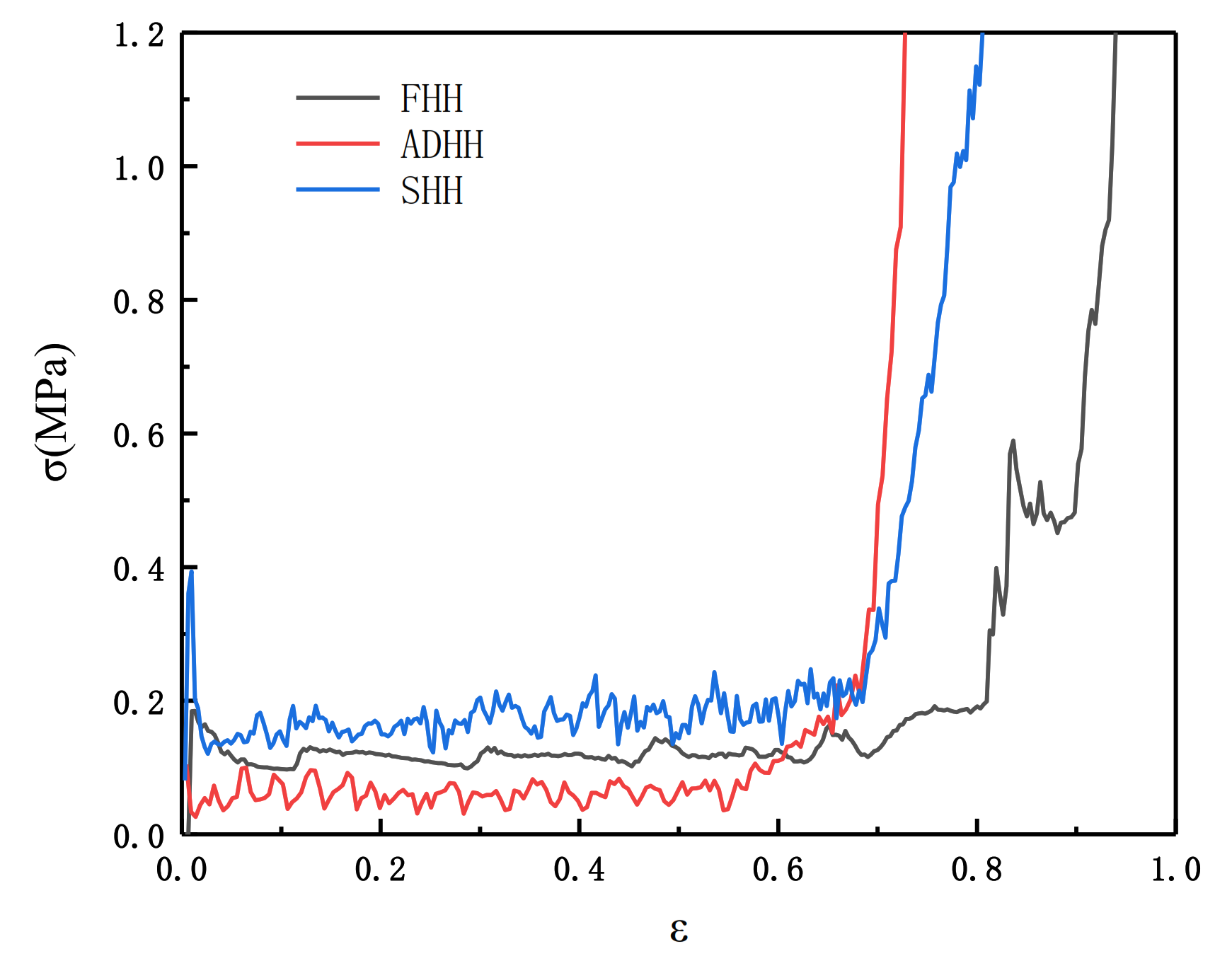
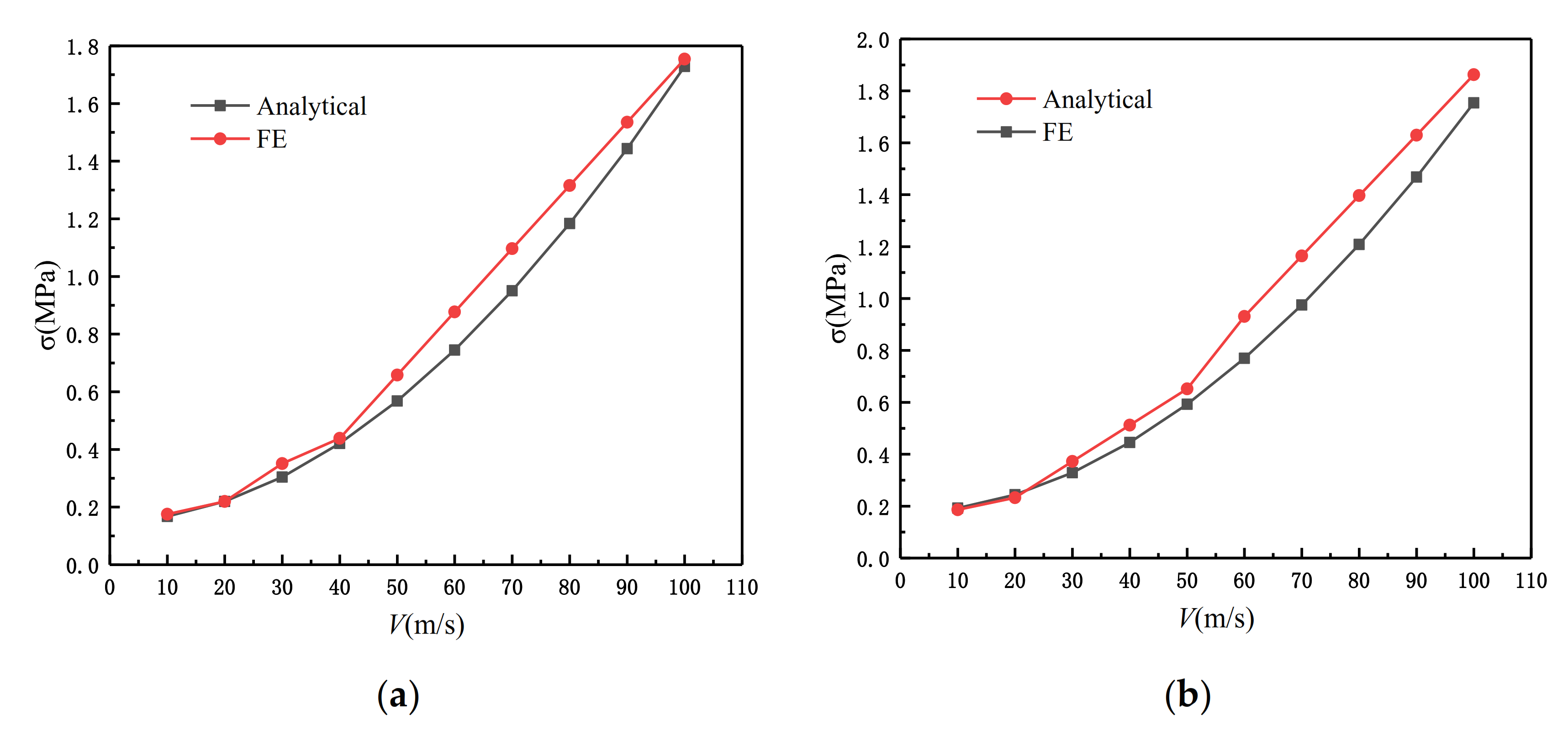
3.4. Dynamic Damage Response
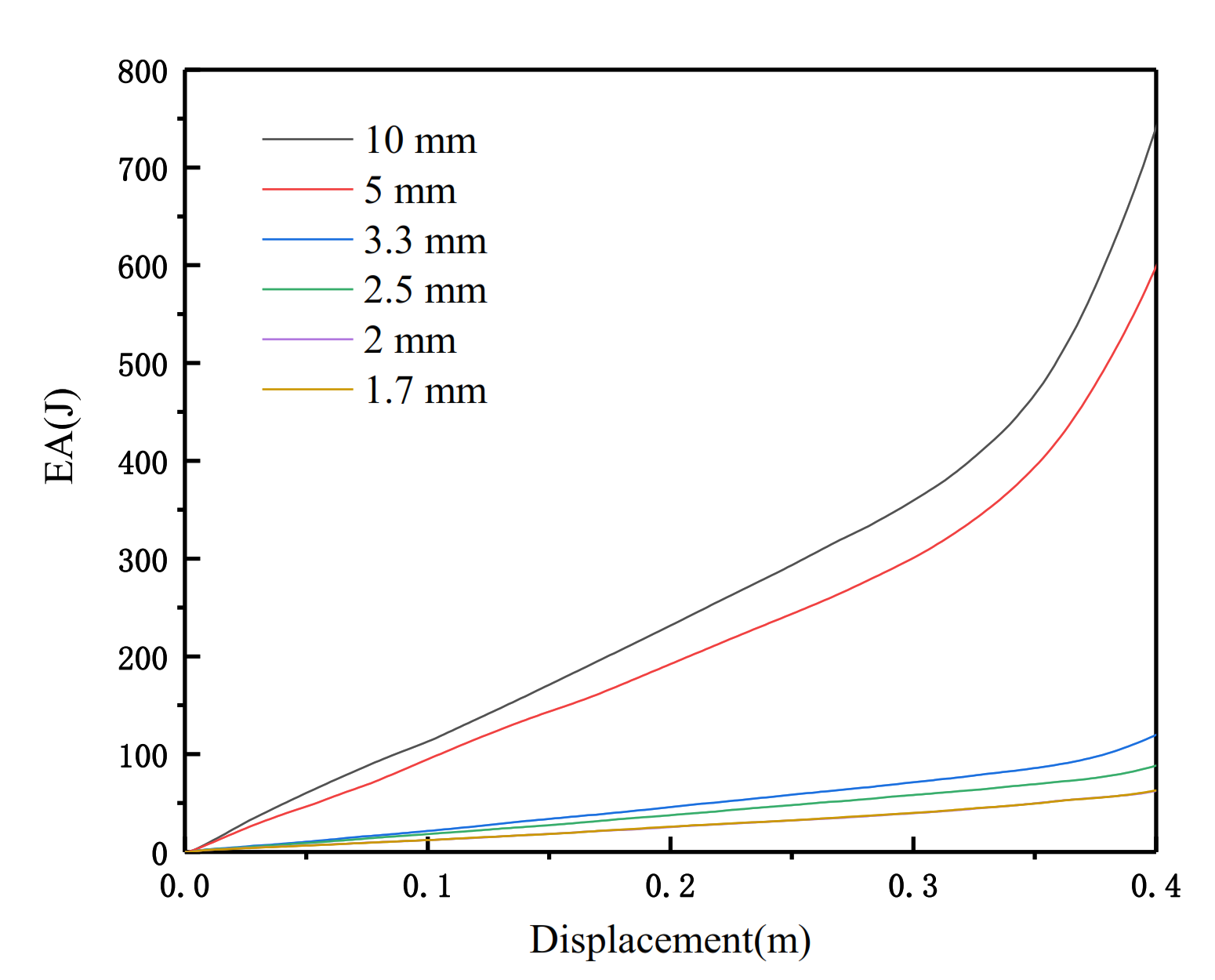
3.5. The Influence of the Number of Substructures on the SHH Failure Stress
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tomasz, W. Crushing analysis of metal honeycombs. Pergamon 1983, 1, 157–174. [Google Scholar] [CrossRef]
- Talukdar, D.; Dutta, K. A simplified thermomechanical approach to visualize hexagonal honeycomb construction. SN Appl. Sci. 2019, 1, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Hu, L.L.; Yu, T.X. Mechanical behavior of hexagonal honeycombs under low-velocity impact—Theory and simulations. Int. J. Solids Struct. 2013, 50, 3152–3165. [Google Scholar] [CrossRef] [Green Version]
- Liu, L.; Zhang, Y.; Sheng, J.Q.; Wei, Y.M.; Liu, R.M.; Yu, W.; Qi, Y.H. Gradient honeycomb absorber with high power handling capability. Appl. Phys. A Mater. Sci. Process. 2021, 127, 129. [Google Scholar] [CrossRef]
- Shang, L.; Wu, Y.; Fang, Y.; Li, Y. High Temperature Mechanical Properties of a Vented Ti-6Al-4V Honeycomb Sandwich Panel. Materials 2020, 13, 3008. [Google Scholar] [CrossRef]
- Hu, L.; Zha, J.; Kan, F.; Long, H.; Chen, Y. Research on a Five-Axis Machining Center Worktable with Bionic Honeycomb Lightweight Structure. Materials 2020, 14, 74. [Google Scholar] [CrossRef] [PubMed]
- He, C.; Chen, J.; Wu, Z.; Xie, J.; Zu, Q.; Lu, Y. Simulated effect on the compressive and shear mechanical properties of bionic integrated honeycomb plates. Mater. Sci. Eng. C 2015, 50, 286–293. [Google Scholar] [CrossRef]
- Du, J.; Hao, P. Investigation on Microstructure of Beetle Elytra and Energy Absorption Properties of Bio-Inspired Honeycomb Thin-Walled Structure under Axial Dynamic Crushing. Nanomaterials 2018, 8, 667. [Google Scholar] [CrossRef] [Green Version]
- Majewski, A.; Krystofiak, T.; Smardzewski, J. Mechanical Properties of Corner Joints Made of Honeycomb Panels with Double Arrow-Shaped Auxetic Cores. Materials 2020, 13, 4212. [Google Scholar] [CrossRef]
- Qiu, X.; Zhang, J.; Yu, T. Collapse of periodic planar lattices under uniaxial compression, part I: Quasi-static strength predicted by limit analysis. Int. J. Impact Eng. 2009, 36, 1223–1230. [Google Scholar] [CrossRef]
- Niu, B.; Wang, B. Directional mechanical properties and wave propagation directionality of Kagome honeycomb structures. Eur. J. Mech. A 2016, 57, 45–58. [Google Scholar] [CrossRef]
- Zhang, Y.; Yan, L.; Zhang, C.; Guo, S. Low-velocity impact response of tube-reinforced honeycomb sandwich structure. Thin-Walled Struct. 2021, 158, 107188. [Google Scholar] [CrossRef]
- Hu, L.; Yu, T. Dynamic crushing strength of hexagonal honeycombs. Int. J. Impact Eng. 2010, 37, 467–474. [Google Scholar] [CrossRef]
- Choi, J.B.; Lakes, R.S. Fracture toughness of re-entrant foam materials with a negative Poisson’s ratio: Experiment and analysis. Int. J. Fract. 1996, 80, 73–83. [Google Scholar] [CrossRef] [Green Version]
- Imbalzano, G.; Tran, P.; Ngo, T.D.; Lee, P.V. Three-dimensional modelling of auxetic sandwich panels for localised impact resistance. J. Sandw. Struct. Mater. 2017, 19, 291–316. [Google Scholar] [CrossRef]
- Imbalzano, G.; Tran, P.; Ngo, T.D.; Lee, P.V. A numerical study of auxetic composite panels under blast loadings. Compos. Struct. 2016, 135, 339–352. [Google Scholar] [CrossRef]
- Zhao, X.; Gao, Q.; Wang, L.; Yu, Q.; Ma, Z. Dynamic crushing of double-arrowed auxetic structure under impact loading. Mater. Des. 2018, 160, 527–537. [Google Scholar] [CrossRef]
- Lethbridge, Z.A.; Williams, J.J.; Walton, R.I.; Smith, C.W.; Hooper, R.M.; Evans, K.E. Direct, static measurement of single-crystal Young’s moduli of the zeolite natrolite: Comparison with dynamic studies and simulations. Acta Mater. 2006, 54, 2533–2545. [Google Scholar] [CrossRef]
- Gao, Q.; Liao, W.-H.; Wang, L. On the low-velocity impact responses of auxetic double arrowed honeycomb. Aerosp. Sci. Technol. 2020, 98, 105698. [Google Scholar] [CrossRef]
- Li, X.; Wang, Q.; Yang, Z.; Lu, Z. Novel auxetic structures with enhanced mechanical properties. Extreme Mech. Lett. 2019, 27, 59–65. [Google Scholar] [CrossRef]
- Qiao, J.; Chen, C.Q. Analyses on the In-Plane Impact Resistance of Auxetic Double Arrowhead Honeycombs. J. Appl. Mech. 2015, 82, 73–83. [Google Scholar] [CrossRef]
- Qiao, J.; Chen, C. In-plane crushing of a hierarchical honeycomb. Int. J. Solids Struct. 2016, 85–86, 57–66. [Google Scholar] [CrossRef]
- Chen, Y.; Jia, Z.; Wang, L. Hierarchical honeycomb lattice metamaterials with improved thermal resistance and mechanical properties. Compos. Struct. 2016, 152, 395–402. [Google Scholar] [CrossRef] [Green Version]
- Ryvkin, M.; Shraga, R. Fracture toughness of hierarchical self-similar honeycombs. Int. J. Solids Struct. 2018, 152–153, 151–160. [Google Scholar] [CrossRef]
- Sun, Y.; Wang, B.; Pugno, N.; Wang, B.; Ding, Q. In-plane stiffness of the anisotropic multifunctional hierarchical honeycombs. Compos. Struct. 2015, 131, 616–624. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, T.; Xu, X.; Hu, Z. Out-of-plane mechanical behaviors of a side hierarchical honeycomb. Mech. Mater. 2020, 140, 103227. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, T.; Ren, H.; Maskery, I.; Ashcroft, I. Dynamic compressive response of additively manufactured AlSi10Mg alloy hierarchical honeycomb structures. Compos. Struct. 2018, 195, 45–59. [Google Scholar] [CrossRef]
- He, Q.; Feng, J.; Zhou, H. A numerical study on the in-plane dynamic crushing of self-similar hierarchical honeycombs. Mech. Mater. 2019, 138, 103151. [Google Scholar] [CrossRef]
- Tan, H.; He, Z.; Li, E.; Tan, X.; Cheng, A.; Li, Q. Energy absorption characteristics of three-layered sandwich panels with graded re-entrant hierarchical honeycombs cores. Aerosp. Sci. Technol. 2020, 106, 106073. [Google Scholar] [CrossRef]
- Tan, H.; He, Z.; Li, K.; Li, E.; Cheng, A.; Xu, B. In-plane crashworthiness of re-entrant hierarchical honeycombs with negative Poisson’s ratio. Compos. Struct. 2019, 229, 111415. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, H.; Zhang, C.; Wang, P.; Meng, L.; Wang, Z. A New Type of Hierarchical Honeycomb in-Plane Impact Study. Materials 2021, 14, 1917. https://doi.org/10.3390/ma14081917
Song H, Zhang C, Wang P, Meng L, Wang Z. A New Type of Hierarchical Honeycomb in-Plane Impact Study. Materials. 2021; 14(8):1917. https://doi.org/10.3390/ma14081917
Chicago/Turabian StyleSong, Hang, Chenyang Zhang, Pengyu Wang, Lingzhi Meng, and Zhenqing Wang. 2021. "A New Type of Hierarchical Honeycomb in-Plane Impact Study" Materials 14, no. 8: 1917. https://doi.org/10.3390/ma14081917




