Simulation-Based Process Design for Asymmetric Single-Point Incremental Forming of Individual Titanium Alloy Hip Cup Prosthesis
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material and Experimental Characterization
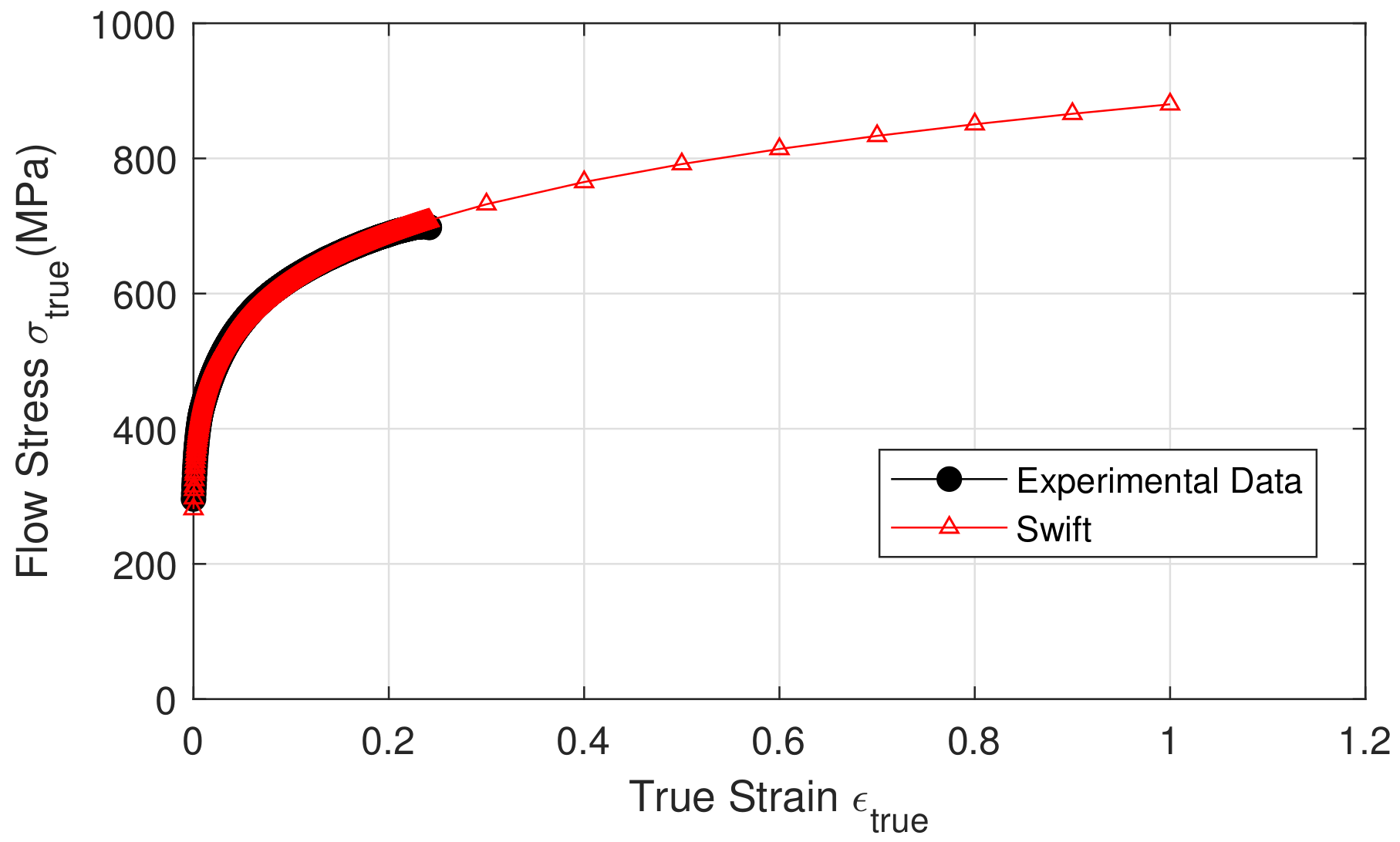
- Swift (or Krupkowski) model:where and n define hardening constants. As a result, this work hardening law was tailored to the upper reaches of the cold deformation domain and can also be used for hot deformation.
- Johnson–Cook failure criterion
2.2. Forming Strategy
- Step 1 allows for the creation of a cloud of points that define the pelvic shape. This geometry was larger than the portion that had to be constructed.
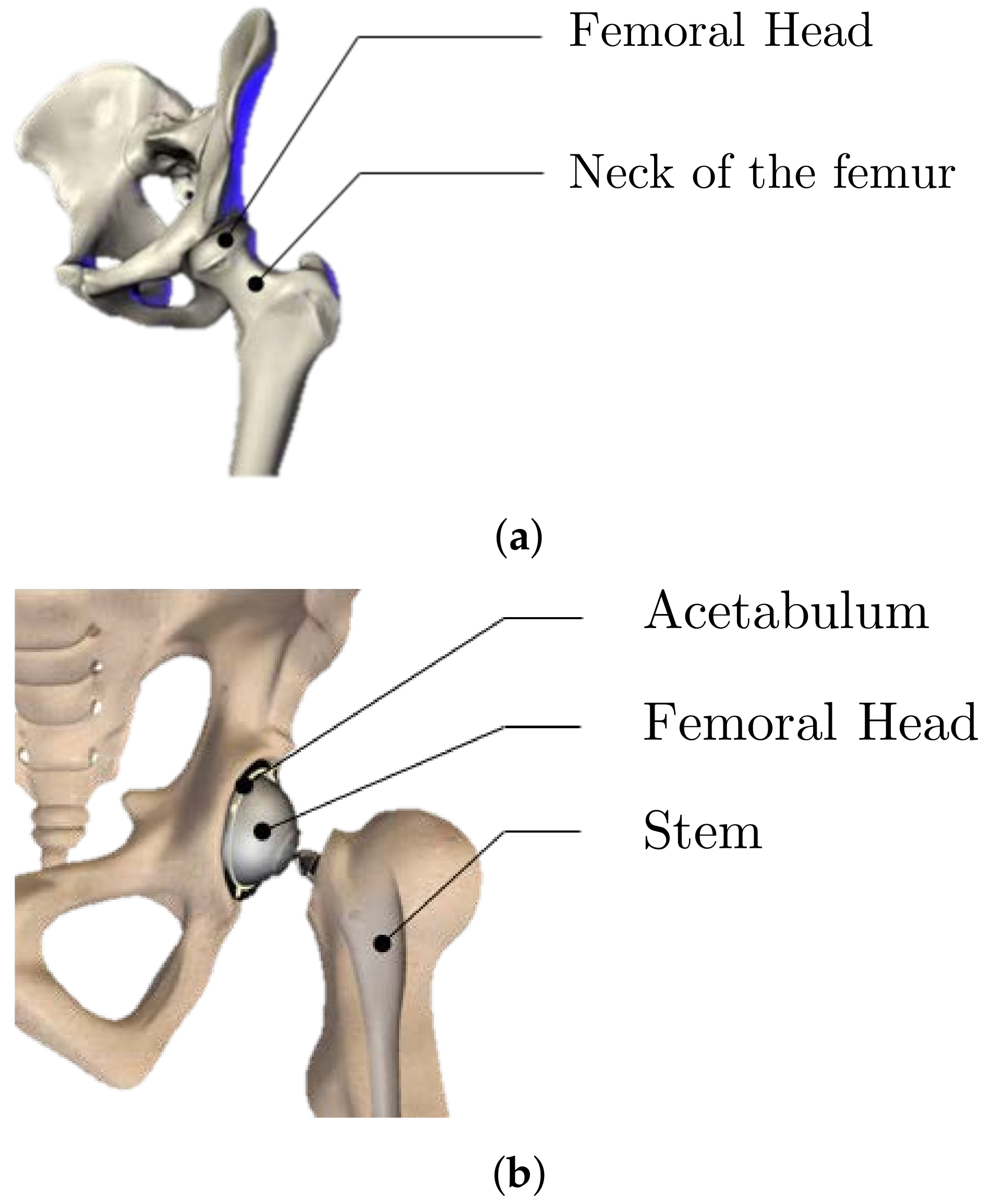
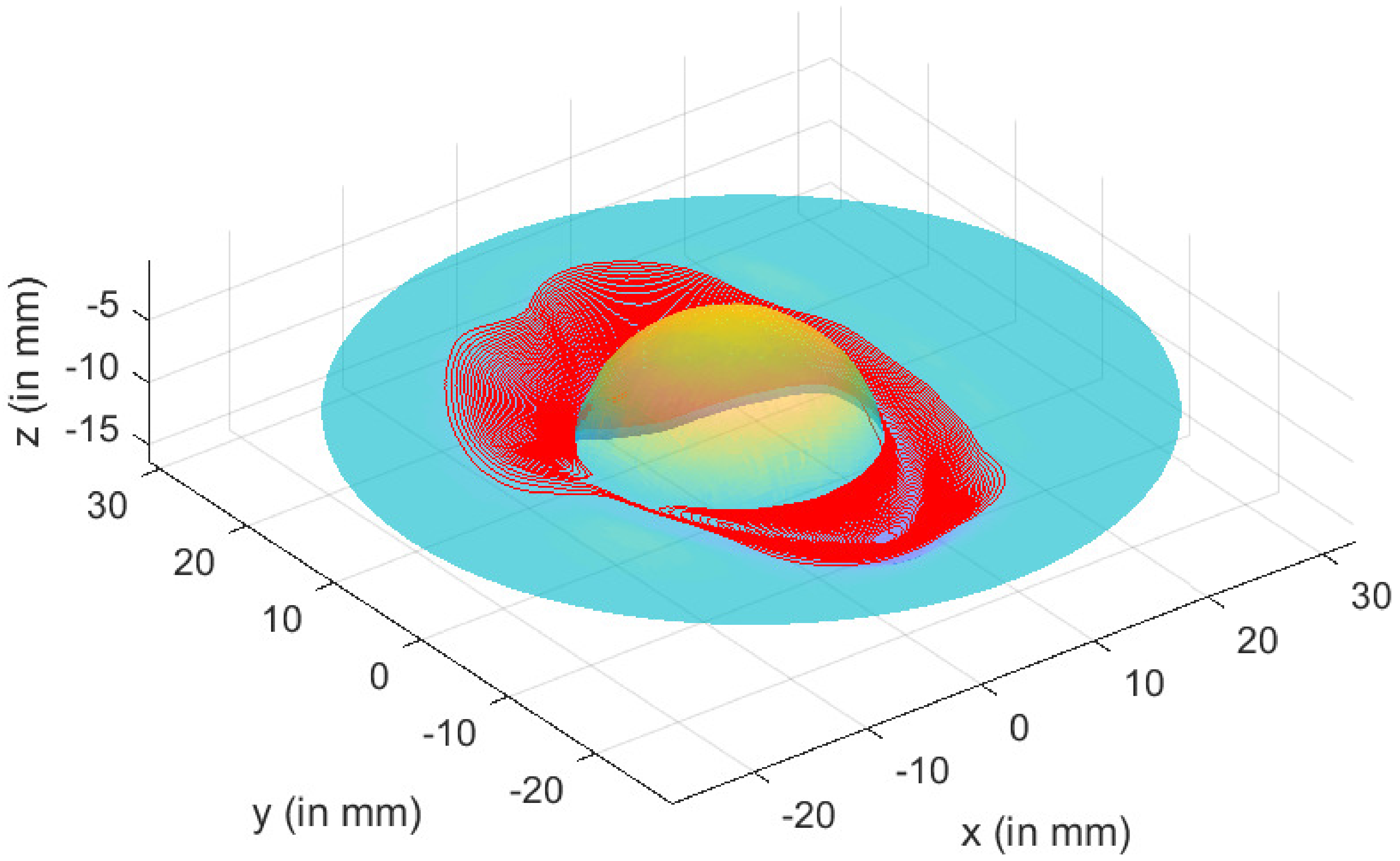
- Step 2 specifies the cup’s geometry before it is constructed. Escobar et al. [5,6] presented a detailed description of the technique for obtaining this geometry. Initially, the fascia lunatic area is resected. The target region is fitted with a first sphere that is positioned so that the radius is the largest without entering the bone. As a result, the approximation sphere is better fitted. Then, to produce the outside contour, a second sphere is created around the first sphere. The inner component is produced by replacing the part’s core with a 20 mm diameter hemisphere. The final shape of the hip cup prosthesis is a titanium part with a large diameter of 100 mm, thickness of 1 mm, and a hemispherical region of 20 mm in the middle area with a wall angle of 70 to 75 in the outer zone (cf. Figure 6).
- During Step 3, the inner half of the cup is constructed using a preforming process. In the middle of the hip cup, a standardized geometry is defined. This procedure is perfectly suited to this situation.
- Step 4: The incremental forming procedure is utilized in Step 4 to build the cup’s outer section. At room temperature, titanium used to produce the hip cup had poor formability. Previous research demonstrated that forming direct parts with a wall angle larger than 40 at ambient temperature is unfeasible according to Saidi et al. [14]. The hip cup prosthesis is intended to be formed in multiple steps by increasing the wall angle.
2.3. Numerical Simulation
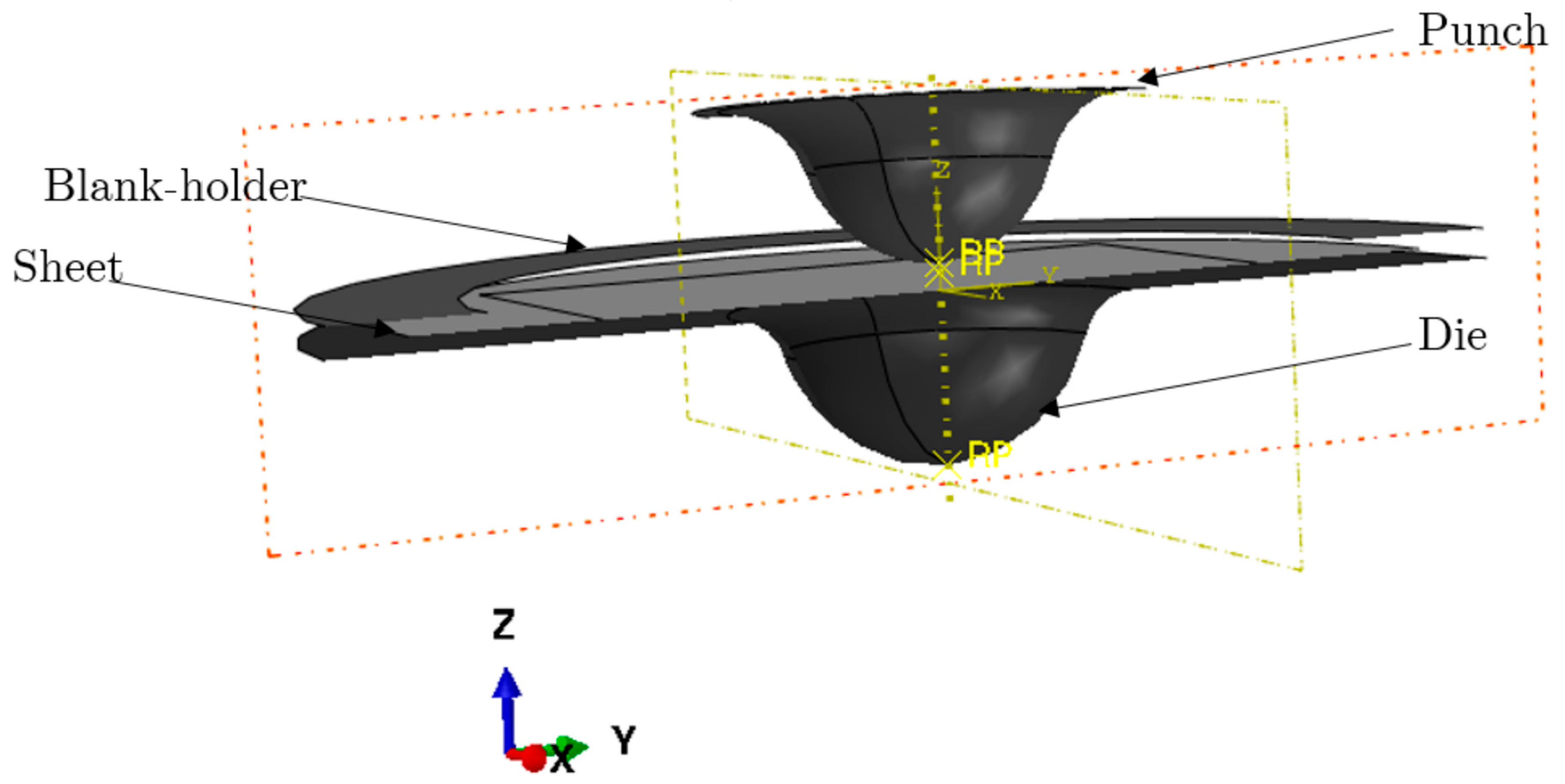
- PreformingTo model the stamping of the inner portion, a numerical simulation of the sheet metal stamping process was first performed. Figure 9 depicts the punch and die. They are supposed to be rigid, and rigid surfaces are used to model them.During this simulation, the sheet was first placed on the die by being merely subjected to gravity, formed using the punch action, and released. Interaction parameters between contact zone and sheet were defined using the Coulomb friction model. Quadrilateral components with reduced integration S4R that were chosen to mesh the part were used.
- Incremental formingThe SPIF process was numerically simulated using ABAQUS FE software. The dynamic displacement explicit solver was used to model the finite elastoviscoplastic deformation of a thin titanium sheet with contact friction. Large-strain formulation and linear four-node coupled stress/displacement shell elements (S4RT) with reduced integration were employed. The sheet’s mesh was partitioned into three zone divisions to minimize computational calculations (cf. Figure 10):sweep mesh (finite elements with a size of 10 × 0.5 mm) in the clamping zone;circular fine mesh (finite elements with a size of 0.5 × 0.5 mm) in the useful zone;fine mesh (finite elements with a size of 0.25 × 0.25 mm) in the critical zone.The part is created in the second stage using the tool trajectory shown in Figure 7. The forming tool follows a specified tool path to form the sheet metal in a series of incremental steps until the desired depth is reached. For incremental forming, tools (die and punch) are regarded to be rigid analytical surfaces. The punch is transformed into a diameter–diameter ball (dp = 0.4 mm) and is inflicted by displacement. The blank holder, modeled by rigid surfaces, is the stationary portion. However, the die on which the sheet sits was fixed according to the (x,y) plane and followed the tool’s movement in z, and surface-to-surface contact between punch and sheet. The sheet was tightly secured to the fixture (the blank holder) and was experimentally supported on it. The sides of the initial sheet were set in all directions from a numerical standpoint and for each simulation (cf. Figure 10).The chosen tool path for this study was composed of rotational movements in the horizontal plane combined with small steps in the vertical direction (Z = 0.2 mm) after every round. The feed speed of mm/min was chosen.One of the most critical concerns for accurately simulating the incremental forming process is modeling the interaction between tool and sheet. Coulomb’s friction model, which is described as follows, defines the interaction qualities between sheet and contact region with the spherical punch:where (f) is frictional shear stress, [36] is the coefficient of friction, and N is the normal contact pressure.The viscoplastic material of Grade 2 titanium was defined using the experimental tensile test data presented in Section 3.1. The Swift model was selected to extrapolate data even though shaping processes need huge plastic deformations. The Hill48 yield model was also incorporated. The Johnson–Cook damage model was chosen because of its ability to take into account the triaxiality in the material modeling during numerical simulation to predict failures.
3. Results and Discussion
3.1. Experimental Results
3.2. Numerical Results
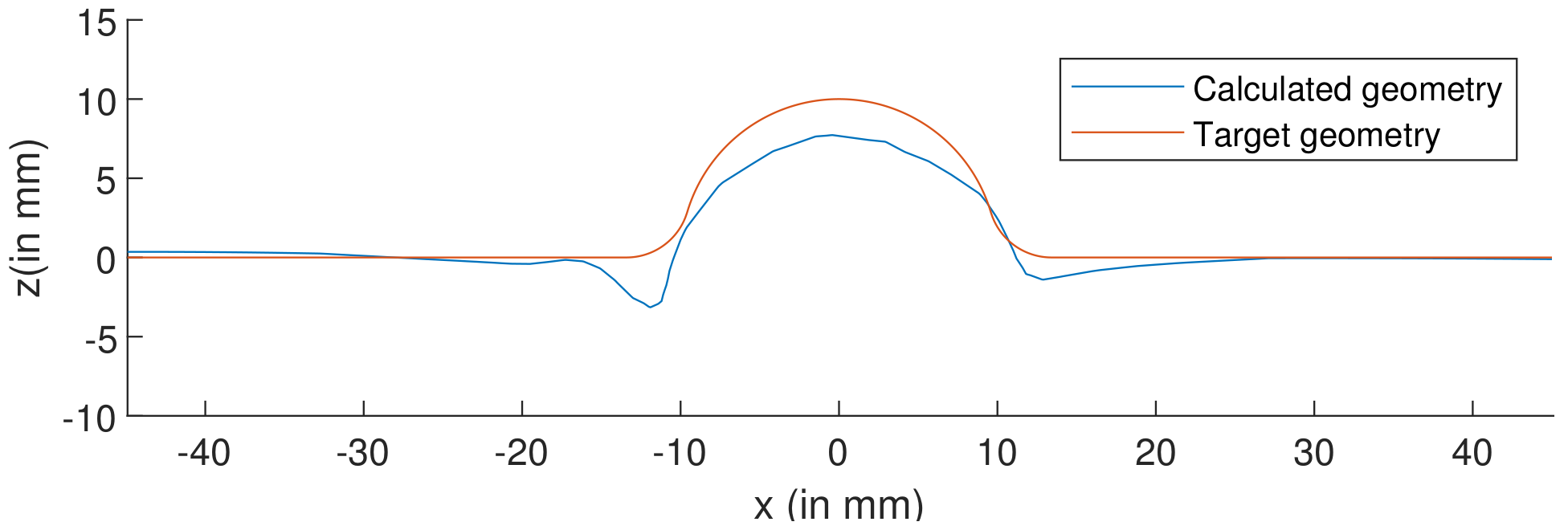
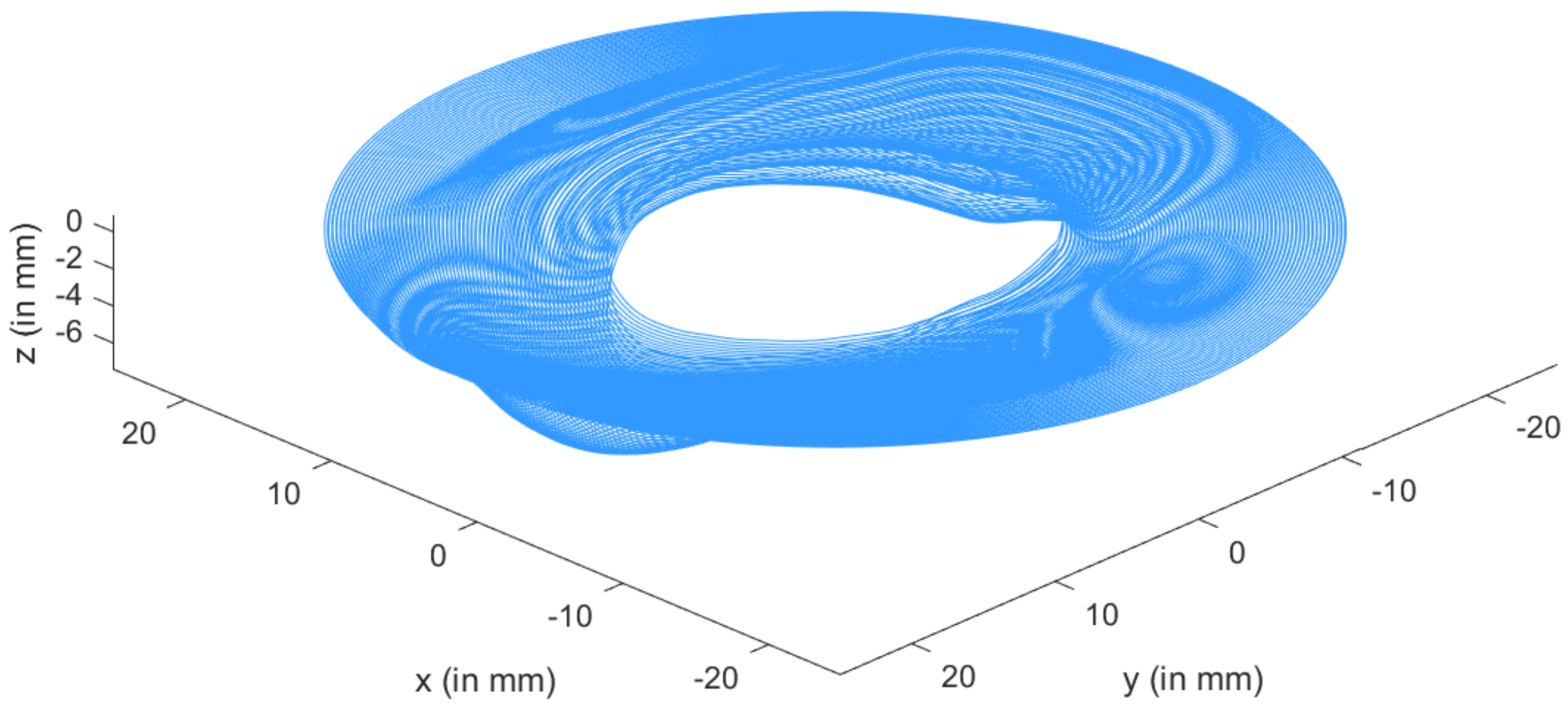
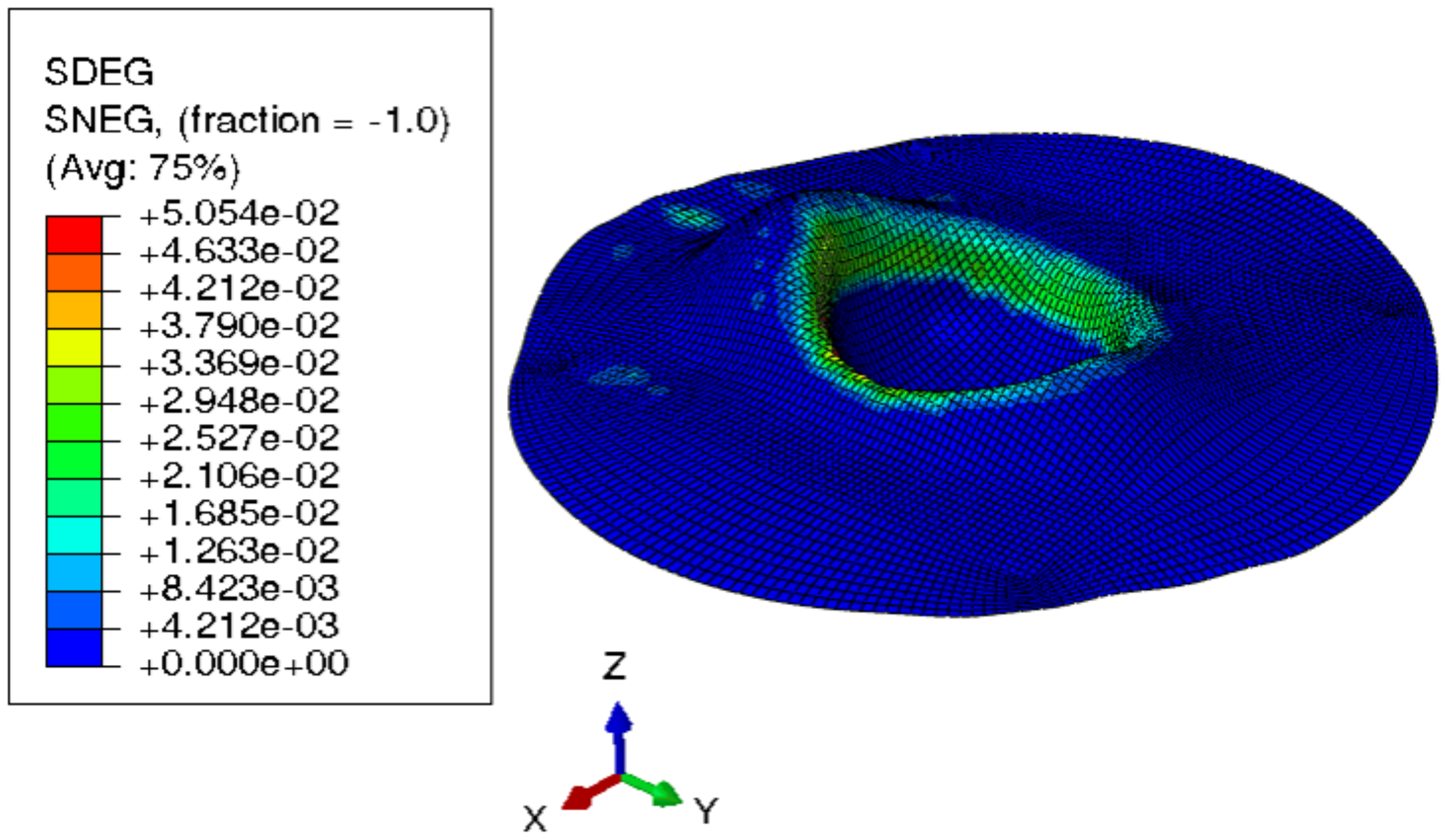
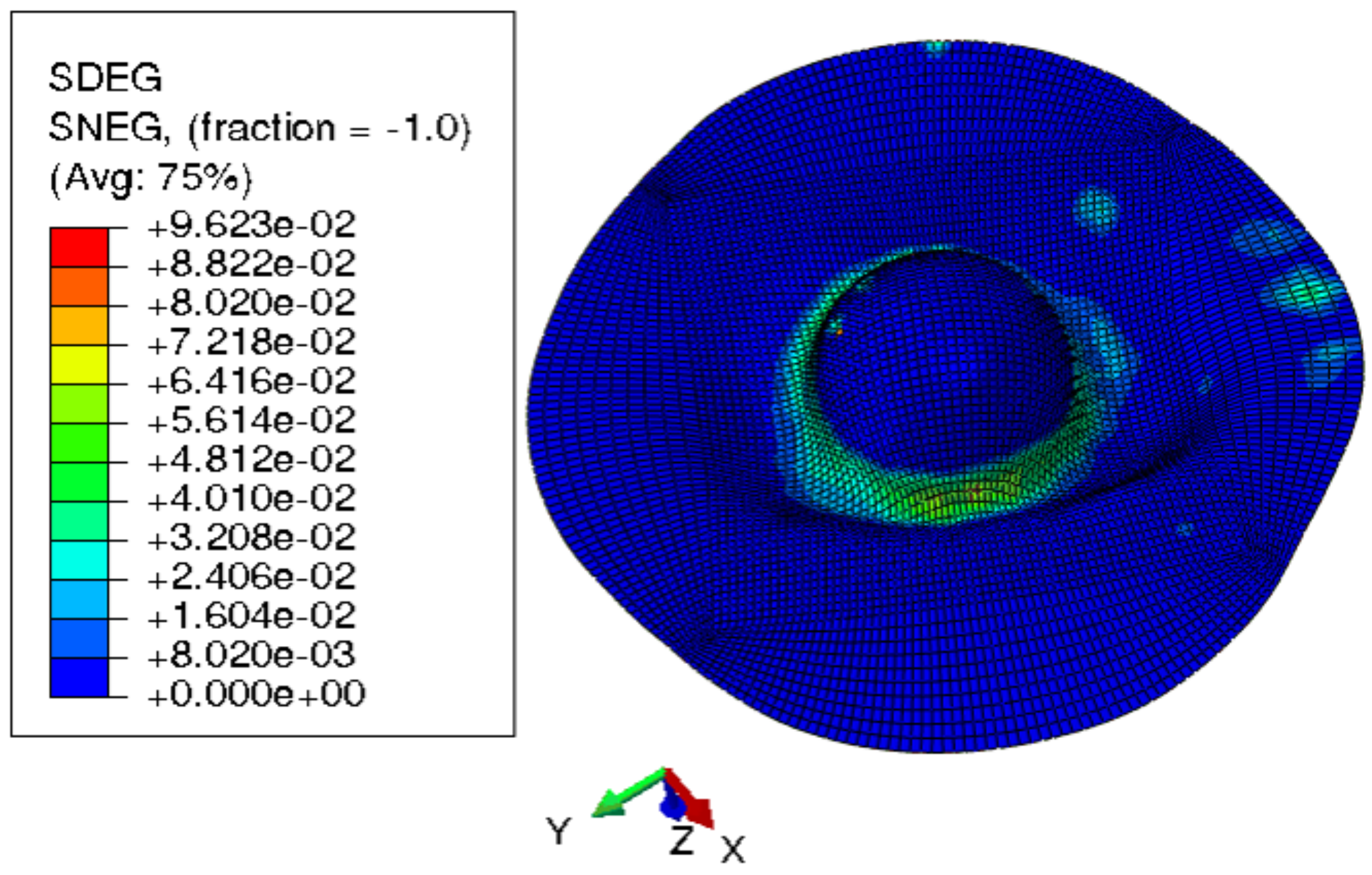
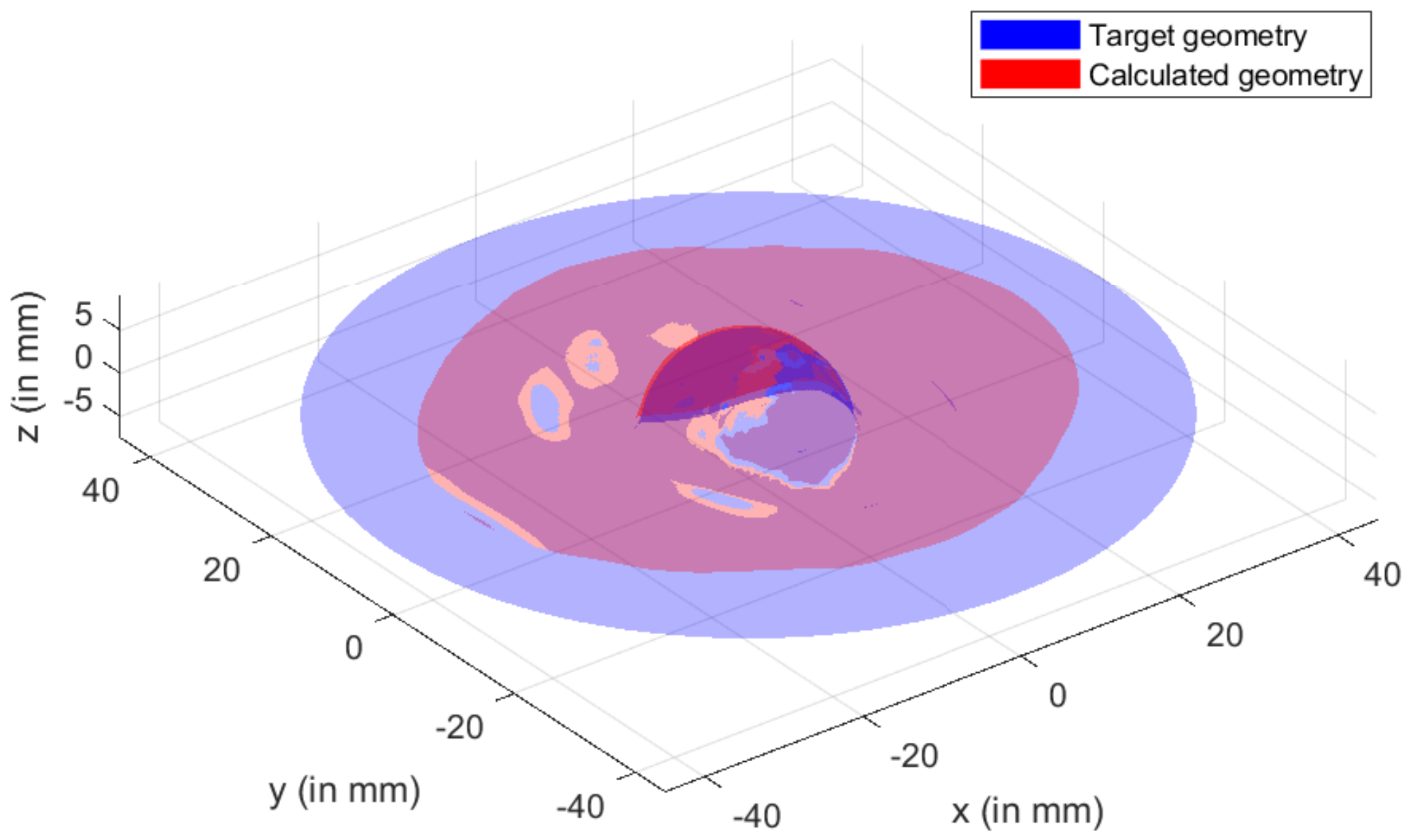
- PreformingFigure 15 shows the visual inspection of standardized human hip cup’s generated components. At room temperature, simulating the preforming process yielded satisfactory results, with a 20% reduction in blank thickness, which is tolerable thickness. Figure 15 also depicts homogeneous thickness distribution.Regarding the forming process at room temperature, Figure 16 displays the isovalues of the scalar damage parameter described by stiffness degradation SDEG. indicates that the appropriate elements failed, and a fracture formed. Damage parameter SDEG was substantially lower than zero in these data, confirming the earlier result that the inner section forms at Ti = 20 C.We estimated the root mean square error (MSE) of altitude z of each point on the X–Y plane to compare theoretical and numerical profiles. The largest distance between two orthogonal locations on the surface is termed the error. As seen in Figure 17, the error was 2.38, which is small when compared to the sheet’s diameter, indicating excellent conformance.
- Incremental forming


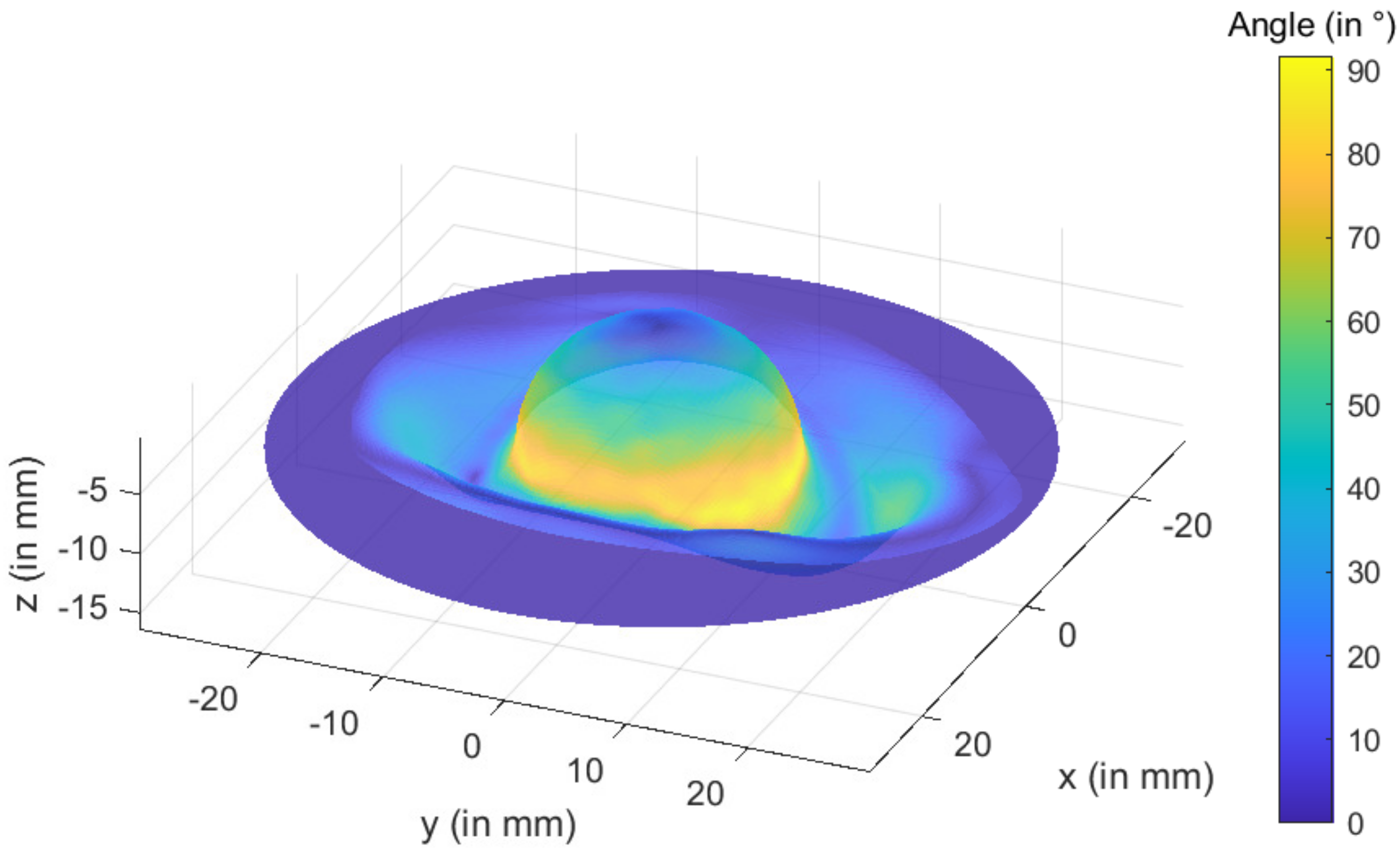
3.2.1. One-Step Forming
3.2.2. Multistep Forming
4. Conclusions
- The SPIF of the acetabular utilizing titanium sheets is a realistic technique that demonstrates the potential for real-world medical use.
- Multistep manufacturing improves geometry accuracy.
- Preliminary findings are promising, and the procedure appears to be suitable for the installation of a hip prosthesis.
- To optimize these parameters for experimental investigation, more work is needed to examine the influence of the majority of process factors on component formability.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Harhash, M.; Palkowski, H. Incremental sheet forming of steel/polymer/steel sandwich composites. J. Mater. Res. Technol. 2021, 13, 417–430. [Google Scholar] [CrossRef]
- Jeswiet, J.; Micari, F.; Hirt, G.; Bramley, A.; Duflou, J.; Allwood, J. Asymmetric single point incremental forming of sheet metal. CIRP Ann. 2005, 54, 88–114. [Google Scholar] [CrossRef]
- Duflou, J.; Verbert, J.; Belkassem, B.; Gu, J.; Sol, H.; Henrard, C.; Habraken, A. Process window enhancement for single point incremental forming through multi-step toolpaths. CIRP Ann. 2008, 57, 253–256. [Google Scholar] [CrossRef] [Green Version]
- Lu, B.; Ou, H.; Shi, S.; Long, H.; Chen, J. Titanium based cranial reconstruction using incremental sheet forming. Int. J. Mater. Form. 2016, 9, 361–370. [Google Scholar] [CrossRef] [Green Version]
- Behrens, B.; Escobar, S.; Niemeier, H.; Vucetic, M.; Bouguecha, A.; Lucas, K.; Nolte, I.; Wefstaedt, P.; Lerch, M.; Stukenborg-Colsman, C.; et al. Design and manufacturing of a human standardised hip cup out of titanium: Konstruktion und Fertigung einer menschlichen standardisierten Hüftpfannenprothese aus Titan. Mater. Und Werkst. 2016, 47, 608–622. [Google Scholar] [CrossRef]
- Escobar, S.B.; Bouguecha, A.; Almohallami, A.; Niemeier, H.; Lucas, K.; Stukenborg-Colsman, C.; Nolte, I.; Wefstaedt, P.; Behrens, B.A. The Customized Artificial Hip Cup: Design and Manufacturing of an Innovative Prosthesis. In Biomedical Technology; Springer: Berlin/Heidelberg, Germany, 2015; pp. 55–68. [Google Scholar]
- Malhotra, R.; Cao, J.; Ren, F.; Kiridena, V.; Cedric Xia, Z.; Reddy, N. Improvement of geometric accuracy in incremental forming by using a squeezing toolpath strategy with two forming tools. J. Manuf. Sci. Eng. 2011, 133, 061019. [Google Scholar] [CrossRef]
- Asgar, J.; Lingam, R.; Reddy, V. Tool path influence on electric pulse aided deformation during incremental sheet metal forming. In AIP Conference Proceedings; American Institute of Physics: College Park, MA, USA, 2013; Volume 1567, pp. 840–843. [Google Scholar]
- Verbert, J.; Behera, A.K.; Lauwers, B.; Duflou, J.R. Multivariate adaptive regression splines as a tool to improve the accuracy of parts produced by FSPIF. In Key Engineering Materials; Trans Tech Publications Ltd.: Bach, Switzerland, 2011; Volume 473, pp. 841–846. [Google Scholar]
- Hussain, G.; Lin, G.; Hayat, N. Improving profile accuracy in SPIF process through statistical optimization of forming parameters. J. Mech. Sci. Technol. 2011, 25, 177–182. [Google Scholar] [CrossRef]
- Ambrogio, G.; Cozza, V.; Filice, L.; Micari, F. An analytical model for improving precision in single point incremental forming. J. Mater. Process. Technol. 2007, 191, 92–95. [Google Scholar] [CrossRef]
- Micari, F.; Ambrogio, G.; Filice, L. Shape and dimensional accuracy in single point incremental forming: State of the art and future trends. J. Mater. Process. Technol. 2007, 191, 390–395. [Google Scholar] [CrossRef]
- Essa, K.; Hartley, P. An assessment of various process strategies for improving precision in single point incremental forming. Int. J. Mater. Form. 2011, 4, 401–412. [Google Scholar] [CrossRef]
- Saidi, B.; Moreau, L.G.; Mhemed, S.; Cherouat, A.; Adragna, P.A.; Nasri, R. Hot incremental forming of titanium human skull prosthesis by using cartridge heaters: A reverse engineering approach. Int. J. Adv. Manuf. Technol. 2019, 101, 873–880. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud Moreau, L.; Cherouat, A.; Nasri, R. Experimental and numerical study on warm single-point incremental sheet forming (WSPIF) of titanium alloy Ti–6Al–4V, using cartridge heaters. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 534. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud Moreau, L.; Cherouat, A.; Nasri, R. Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process. J. Manuf. Mater. Process. 2021, 5, 122. [Google Scholar] [CrossRef]
- Iseki, H. An approximate deformation analysis and FEM analysis for the incremental bulging of sheet metal using a spherical roller. J. Mater. Process. Technol. 2001, 111, 150–154. [Google Scholar] [CrossRef]
- Haque, M.Z.; Yoon, J.W. Stress based prediction of formability and failure in incremental sheet forming. Int. J. Mater. Form. 2016, 9, 413–421. [Google Scholar] [CrossRef]
- Huang, Y.; Cao, J.; Smith, S.; Woody, B.; Ziegert, J.; Li, M. Studies of size effect on the formability of a domed part in incremental forming. In Proceedings of the International Manufacturing Science and Engineering Conference, Evanston, IL, USA, 7–10 October 2008; Volume 48517, pp. 645–654. [Google Scholar]
- Besson, J. Continuum models of ductile fracture: A review. Int. J. Damage Mech. 2010, 19, 3–52. [Google Scholar] [CrossRef] [Green Version]
- Malhotra, R.; Xue, L.; Belytschko, T.; Cao, J. Mechanics of fracture in single point incremental forming. J. Mater. Process. Technol. 2012, 212, 1573–1590. [Google Scholar] [CrossRef]
- Guzmán, C.F.; Yuan, S.; Duchêne, L.; Flores, E.I.S.; Habraken, A.M. Damage prediction in single point incremental forming using an extended Gurson model. Int. J. Solids Struct. 2018, 151, 45–56. [Google Scholar] [CrossRef] [Green Version]
- Park, N.; Huh, H.; Lim, S.J.; Lou, Y.; Kang, Y.S.; Seo, M.H. Fracture-based forming limit criteria for anisotropic materials in sheet metal forming. Int. J. Plast. 2017, 96, 1–35. [Google Scholar] [CrossRef]
- Park, N.; Huh, H.; Yoon, J.W. Anisotropic fracture forming limit diagram considering non-directionality of the equi-biaxial fracture strain. Int. J. Solids Struct. 2018, 151, 181–194. [Google Scholar] [CrossRef]
- Hill, R. A theory of the yielding and plastic flow of anisotropic metals. Proc. R. Soc. London Ser. A Math. Phys. Sci. 1948, 193, 281–297. [Google Scholar]
- Yue, Z.; Chu, X.; Gao, J. Numerical simulation of incremental sheet forming with considering yield surface distortion. Int. J. Adv. Manuf. Technol. 2017, 92, 1761–1768. [Google Scholar] [CrossRef]
- Nieh, T.; Wang, J.; Hsiung, L.; Wadsworth, J.; Sikka, V. Low temperature superplasticity in a TiAl alloy with a metastable microstructure. Scr. Mater. 1997, 37, 773–779. [Google Scholar] [CrossRef]
- Lemaitre, J. How to use damage mechanics. Nucl. Eng. Des. 1984, 80, 233–245. [Google Scholar] [CrossRef]
- Lemaitre, J. A three-dimensional ductile damage model applied to deep-drawing forming limits. In Mechanical Behaviour of Materials; Elsevier: Amsterdam, The Netherlands, 1984; pp. 1047–1053. [Google Scholar]
- Murugesan, M.; Jung, D.W. Johnson Cook material and failure model parameters estimation of AISI-1045 medium carbon steel for metal forming applications. Materials 2019, 12, 609. [Google Scholar] [CrossRef] [Green Version]
- Mirone, G.; Corallo, D. A local viewpoint for evaluating the influence of stress triaxiality and Lode angle on ductile failure and hardening. Int. J. Plast. 2010, 26, 348–371. [Google Scholar] [CrossRef]
- Cao, T.S.; Gachet, J.M.; Montmitonnet, P.; Bouchard, P.O. A Lode-dependent enhanced Lemaitre model for ductile fracture prediction at low stress triaxiality. Eng. Fract. Mech. 2014, 124, 80–96. [Google Scholar] [CrossRef]
- Cao, T.S.; Maire, E.; Verdu, C.; Bobadilla, C.; Lasne, P.; Montmitonnet, P.; Bouchard, P.O. Characterization of ductile damage for a high carbon steel using 3D X-ray micro-tomography and mechanical tests–Application to the identification of a shear modified GTN model. Comput. Mater. Sci. 2014, 84, 175–187. [Google Scholar] [CrossRef]
- Bao, Y.; Wierzbicki, T. On the cut-off value of negative triaxiality for fracture. Eng. Fract. Mech. 2005, 72, 1049–1069. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud-Moreau, L.; Cherouat, A.; Nasri, R. Experimental and numerical study on optimization of the single point incremental forming of AINSI 304L stainless steel sheet. In Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2017; Volume 896, p. 012039. [Google Scholar]
- Saidi, B.; Giraud-Moreau, L.; Cherouat, A. Optimization of the single point incremental forming process for titanium sheets by using response surface. In Proceedings of the MATEC Web of Conferences; EDP Sciences: Les Ulis, France, 2016; Volume 80, p. 10011. [Google Scholar]
- Odenberger, E.L.; Hertzman, J.; Thilderkvist, P.; Merklein, M.; Kuppert, A.; Stöhr, T.; Lechler, J.; Oldenburg, M. Thermo-mechanical sheet metal forming of aero engine components in Ti-6Al-4V–PART 1: Material characterisation. Int. J. Mater. Form. 2013, 6, 391–402. [Google Scholar] [CrossRef]
- Duflou, J.R.; Behera, A.K.; Vanhove, H.; Bertol, L.S. Manufacture of accurate titanium cranio-facial implants with high forming angle using single point incremental forming. In Key Engineering Materials; Trans Tech Publications Ltd.: Bach, Switzerland, 2013; Volume 549, pp. 223–230. [Google Scholar]
- Bouguecha, A.; Elgaly, I.; Stukenborg-Colsman, C.; Lerch, M.; Nolte, I.; Wefstaedt, P.; Matthias, T.; Behrens, B.A. Numerical investigations of the strain-adaptive bone remodeling in the prosthetic pelvis. In XII Mediterranean Conference on Medical and Biological Engineering and Computing 2010; Springer: Berlin/Heidelberg, Germany, 2010; pp. 562–565. [Google Scholar]

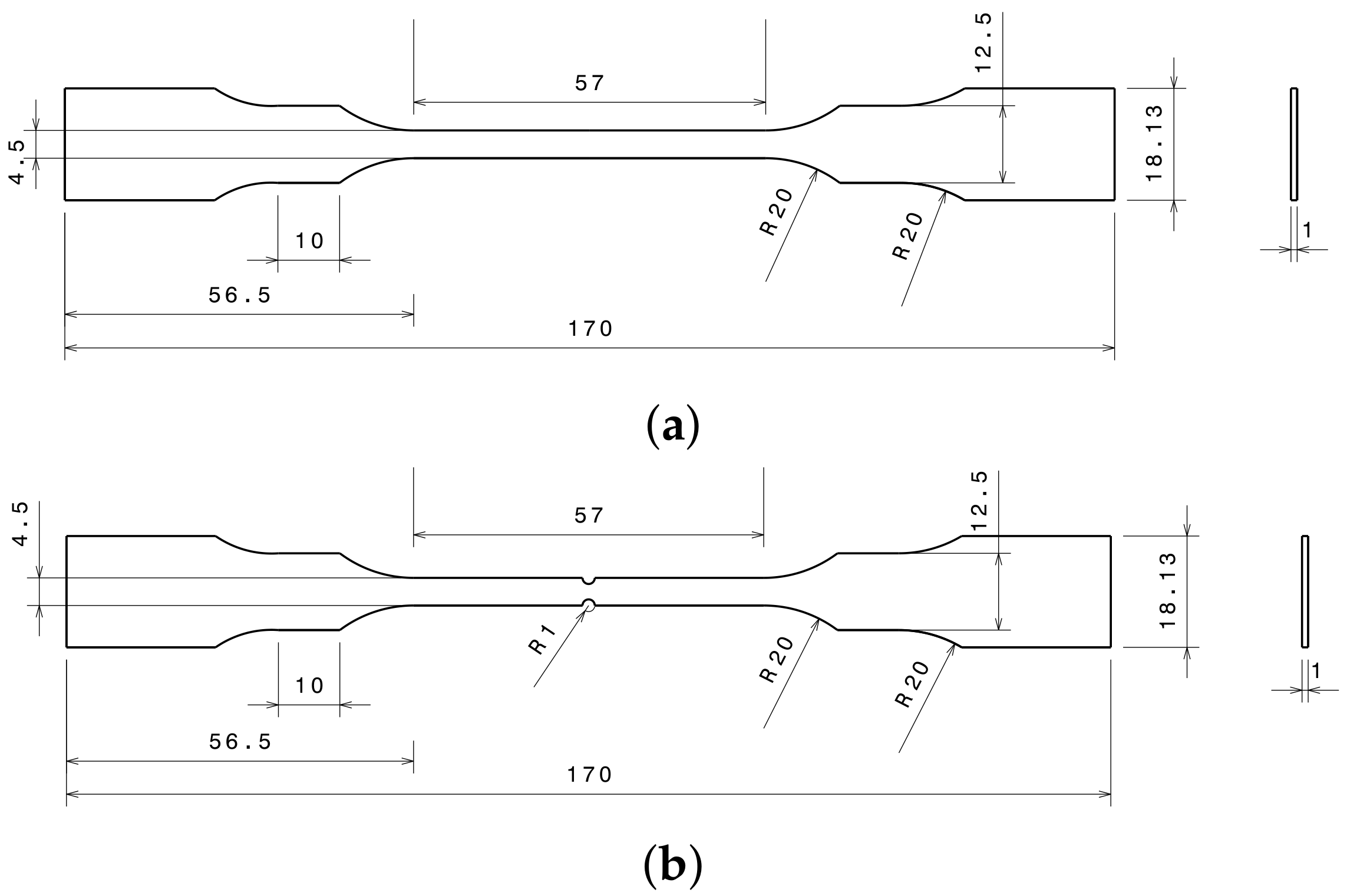









| Chemical Composition (wt%) | Used Sample | ASTM F67 |
|---|---|---|
| Titanium | 49.7% | 32.5% |
| Iron | 20% | 30% |
| Oxygen | 18% | 25% |
| Carbon | 6% | 8% |
| Nitrogen | 5% | 3% |
| Hydrogen | 1.3% | 1.5% |
| Material | Notch Radius R (in mm) | Gauge Length (in mm) |
|---|---|---|
| Rolling Direction | 0 | 57 |
| 0.5 | 56 | |
| 1 | 55 | |
| 1.5 | 54 |
| Rolling Angle | E (GPa) | Rm (MPa) | Re (MPa) | A |
|---|---|---|---|---|
| 0 | 111.92 ± 0.29 | 288.50 ± 4.53 | 422.05 ± 5.00 | 32.25% ± 1.71% |
| 45 | 111.90 ± 0.18 | 288.75 ± 41.0 | 368.45 ± 6.29 | 35.90% ± 0.84% |
| 90 | 112.02 ± 0.17 | 325.25 ± 51.6 | 373.12 ± 5.68 | 35.25% ± 2.50% |
| Material Characteristics | K[MPa] | n | |
|---|---|---|---|
| T40 | 880 | 0.0578 | 0.153 |
| Orientation () | r |
|---|---|
| 0 | 5.43 |
| 45 | 4.57 |
| 90 | 4.1 |
| Hill48 Coefficients | Values |
|---|---|
| G | 0.31 |
| H | 1.68 |
| F | 0.41 |
| L = M = N | 1.83 |
| Material Parameters of Grade 2 Titanium | Values |
|---|---|
| −1.157 | |
| 1.685 | |
| −0.583 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frikha, S.; Giraud-Moreau, L.; Bouguecha, A.; Haddar, M. Simulation-Based Process Design for Asymmetric Single-Point Incremental Forming of Individual Titanium Alloy Hip Cup Prosthesis. Materials 2022, 15, 3442. https://doi.org/10.3390/ma15103442
Frikha S, Giraud-Moreau L, Bouguecha A, Haddar M. Simulation-Based Process Design for Asymmetric Single-Point Incremental Forming of Individual Titanium Alloy Hip Cup Prosthesis. Materials. 2022; 15(10):3442. https://doi.org/10.3390/ma15103442
Chicago/Turabian StyleFrikha, Sirine, Laurence Giraud-Moreau, Anas Bouguecha, and Mohamed Haddar. 2022. "Simulation-Based Process Design for Asymmetric Single-Point Incremental Forming of Individual Titanium Alloy Hip Cup Prosthesis" Materials 15, no. 10: 3442. https://doi.org/10.3390/ma15103442
APA StyleFrikha, S., Giraud-Moreau, L., Bouguecha, A., & Haddar, M. (2022). Simulation-Based Process Design for Asymmetric Single-Point Incremental Forming of Individual Titanium Alloy Hip Cup Prosthesis. Materials, 15(10), 3442. https://doi.org/10.3390/ma15103442







