Experimental Investigation on Seepage Characteristics of Clay–Structure Interface after Shear Deformation
Abstract
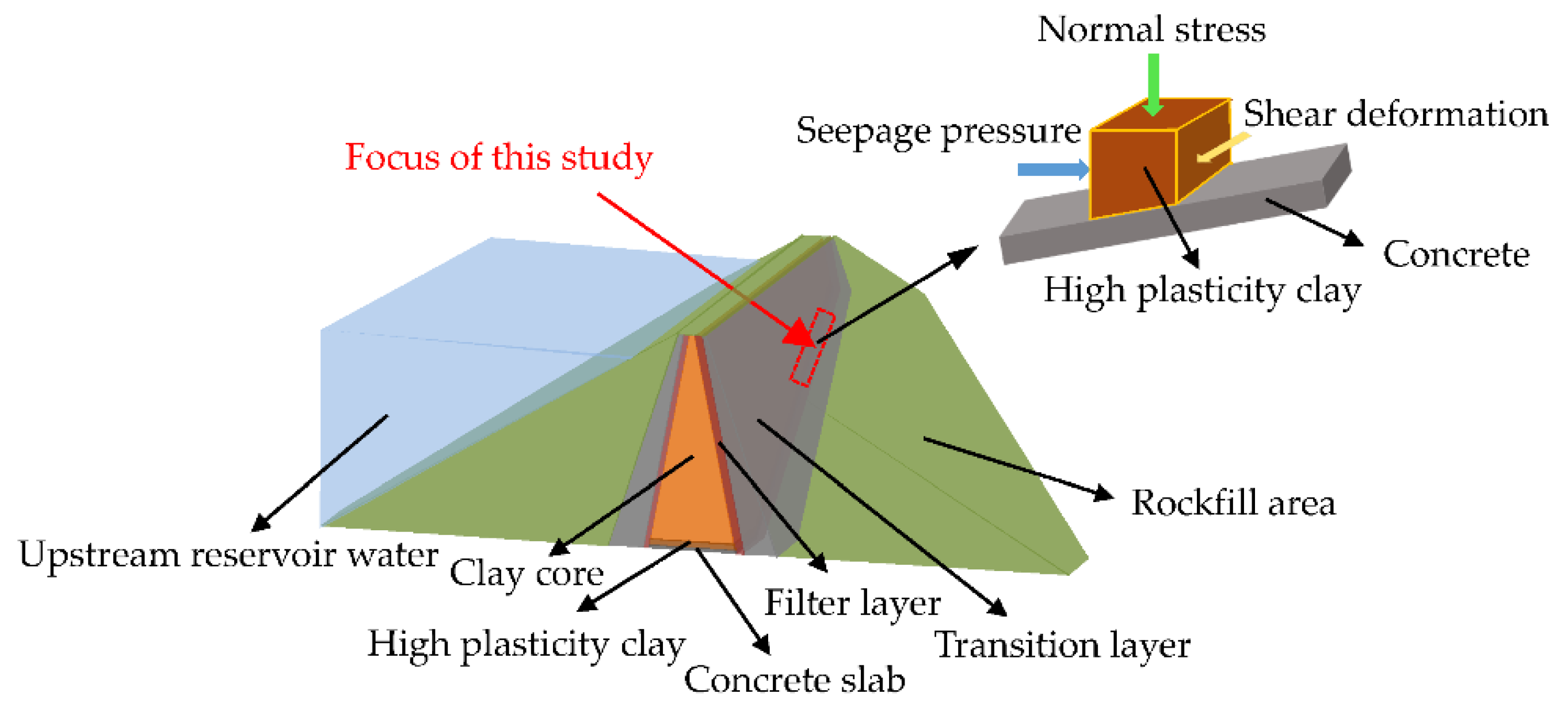
:1. Introduction
2. Materials and Methods
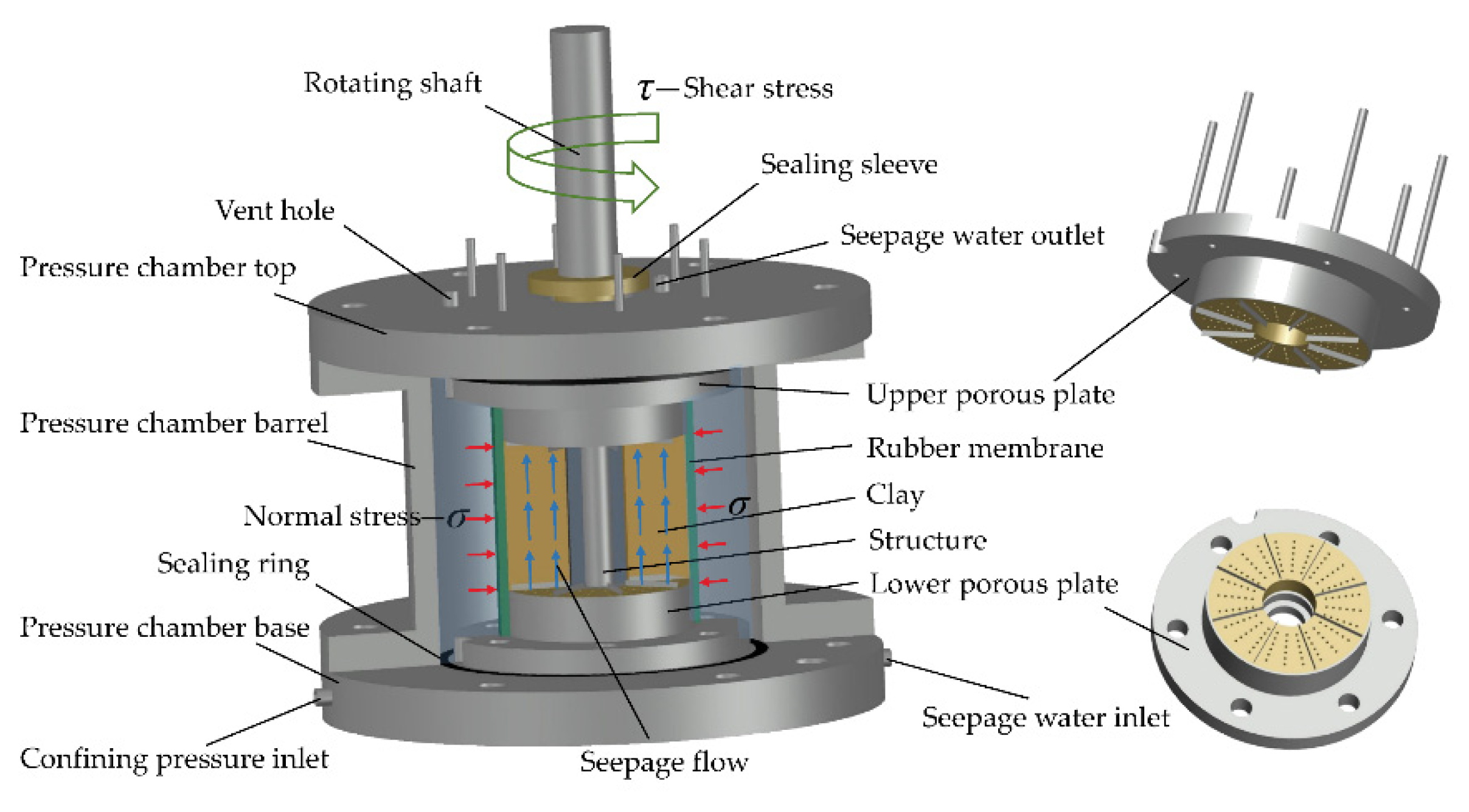
2.1. Shear-Seepage Test System
2.2. Testing Materials
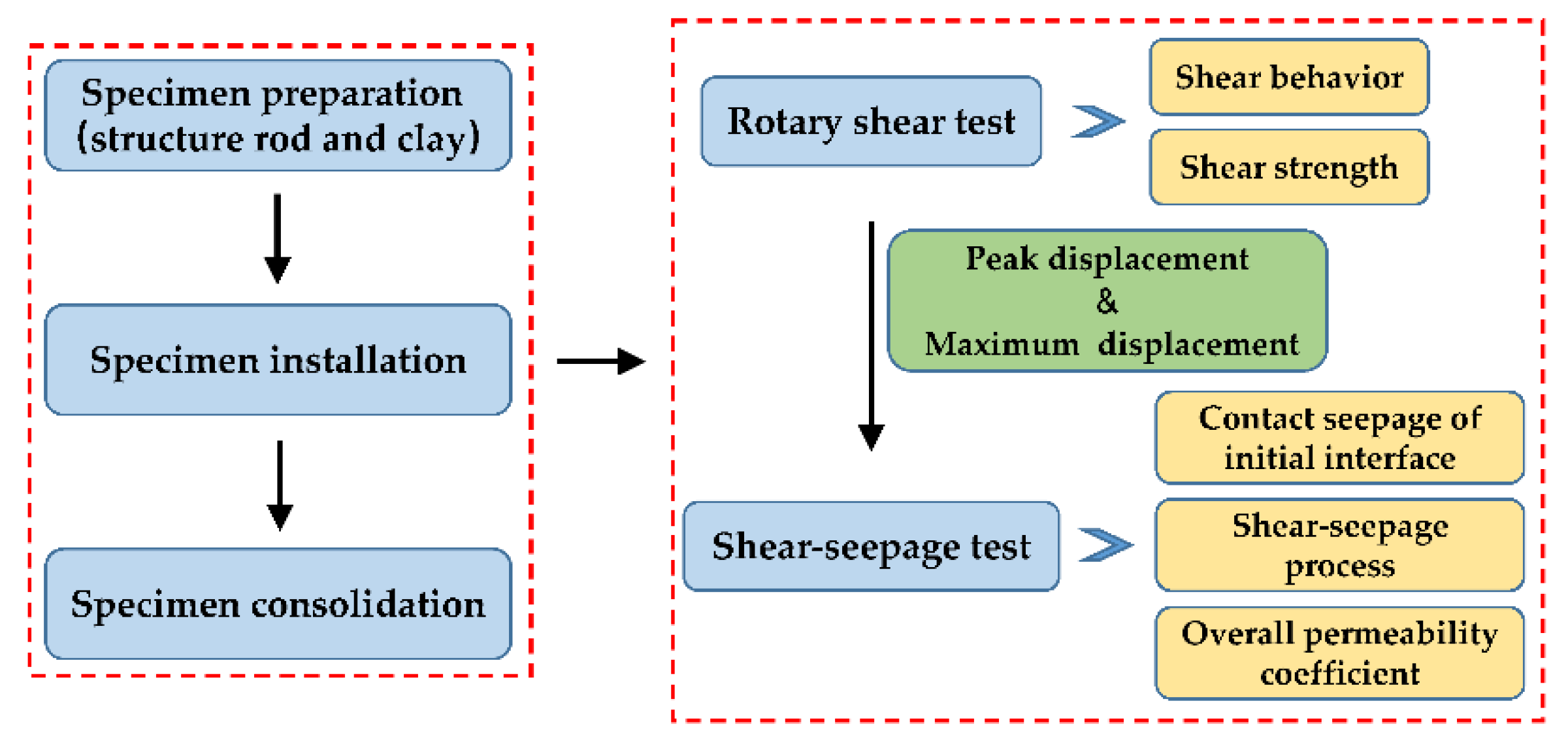
2.3. Testing Methods
- Specimen preparation. Firstly, the clay soil was dried, crushed, and sieved. The clay and water were mixed according to the designed dry density of 1.83 g/cm3 and water content of 15.6%, then the prepared clay soil was stored for 24 h. Secondly, the prepared structure rod was placed at the center of the lower porous plate and the specimen was compacted directly on the lower porous plate to reduce the disturbance to the specimen, which could ensure that the clay soil adheres to the structure rod. The clay was evenly divided into two layers and compacted, and the target compaction degree of each layer was 98%. The joint surface of the two layers was fully shaved to facilitate the close combination of the soil. Thirdly, the upper porous plate was pressed on the soil, and the rubber membrane was wrapped on the upper porous plate, clay–structure specimen, and lower porous plate. Finally, the specimen was saturated in a vacuum saturated bucket for 24 h.
- Specimen consolidation. Firstly, fill all pipes with airless water and install the pressure chamber. Secondly, inject distilled water into the pressure chamber from the confining pressure inlet until it is full, and then block the vent hole. Finally, apply the specified consolidation pressure to the water in the pressure chamber by using the GDS pressure-volume controller, and open the seepage water outlet and the seepage water inlet to drain the pore water of the specimen. The volume change in this stage was measured by the variation of pore water. When high consolidation pressure was needed, a hierarchical loading method was adopted. When the volume change of the GDS pressure-volume controller was less than 1 mm3/min, or no pore water was discharged, the specimen consolidation was considered complete.
- Shear-seepage test. The shear-seepage test mainly included two processes: seepage process and shear process, which were carried out alternately. During the seepage process, open the seepage water inlet and outlet and apply the seepage pressure. The upward seepage water flowed into the specimen and discharged from the seepage water outlet. When the specimen seepage outflow was equal to outflow, the seepage flow was considered to be stable. Then, we recorded the inlet and outlet hydraulic heads and the inlet and outlet seepage discharge, and calculated the overall permeability coefficient of the specimen (defined by Equation (1)). The whole seepage process was no less than 6 h. After the current seepage process was completed, we closed the seepage pressure system and left the specimen for several hours to ensure that the residual water at the clay–structure interface was fully discharged. During the shear process, we applied torque to the rotating shaft and produce large shear deformation at the clay–structure interface. When the shear deformation reached the specified displacement, we stopped shearing. After that, we repeated the above seepage process and measured the overall permeability coefficient of specimen under different shear deformation conditions. Specimens should be changed under different normal stress. The experiment was performed in the laboratory at room temperature of approximately 20 °C. The experimental flowchart is shown in Figure 6.
2.4. Testing Schemes
2.5. Overall Permeability Coefficient
3. Results
3.1. Mechanical Behavior Analysis
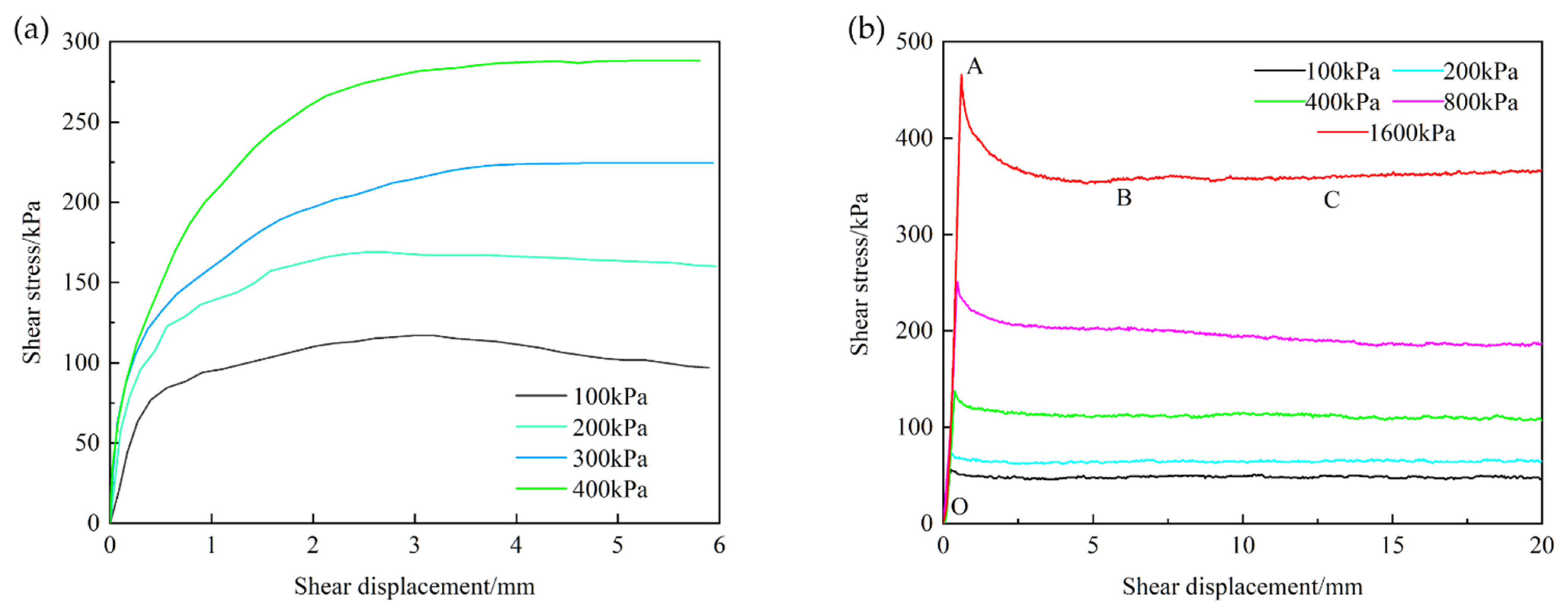
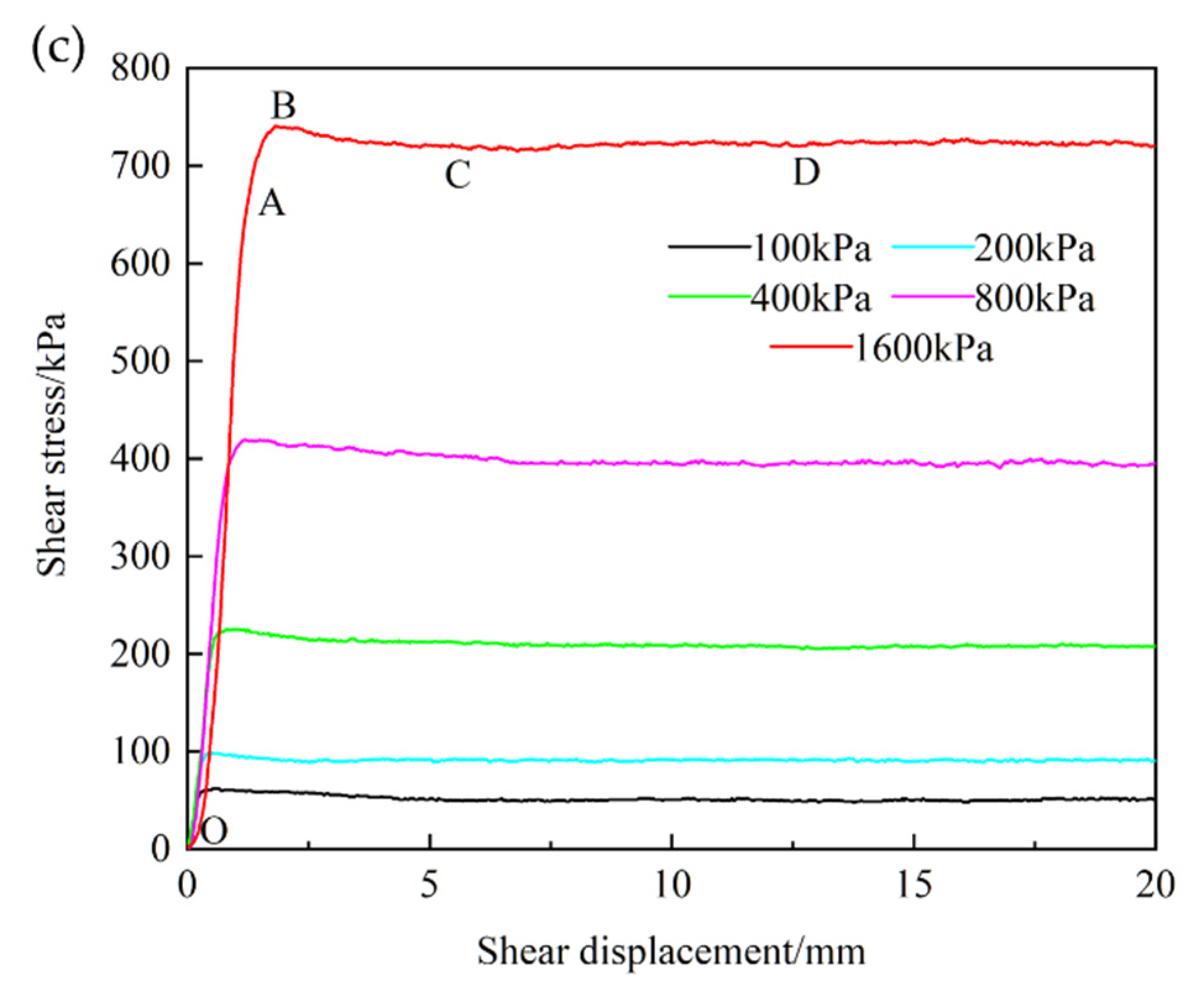
3.1.1. Shear Behavior
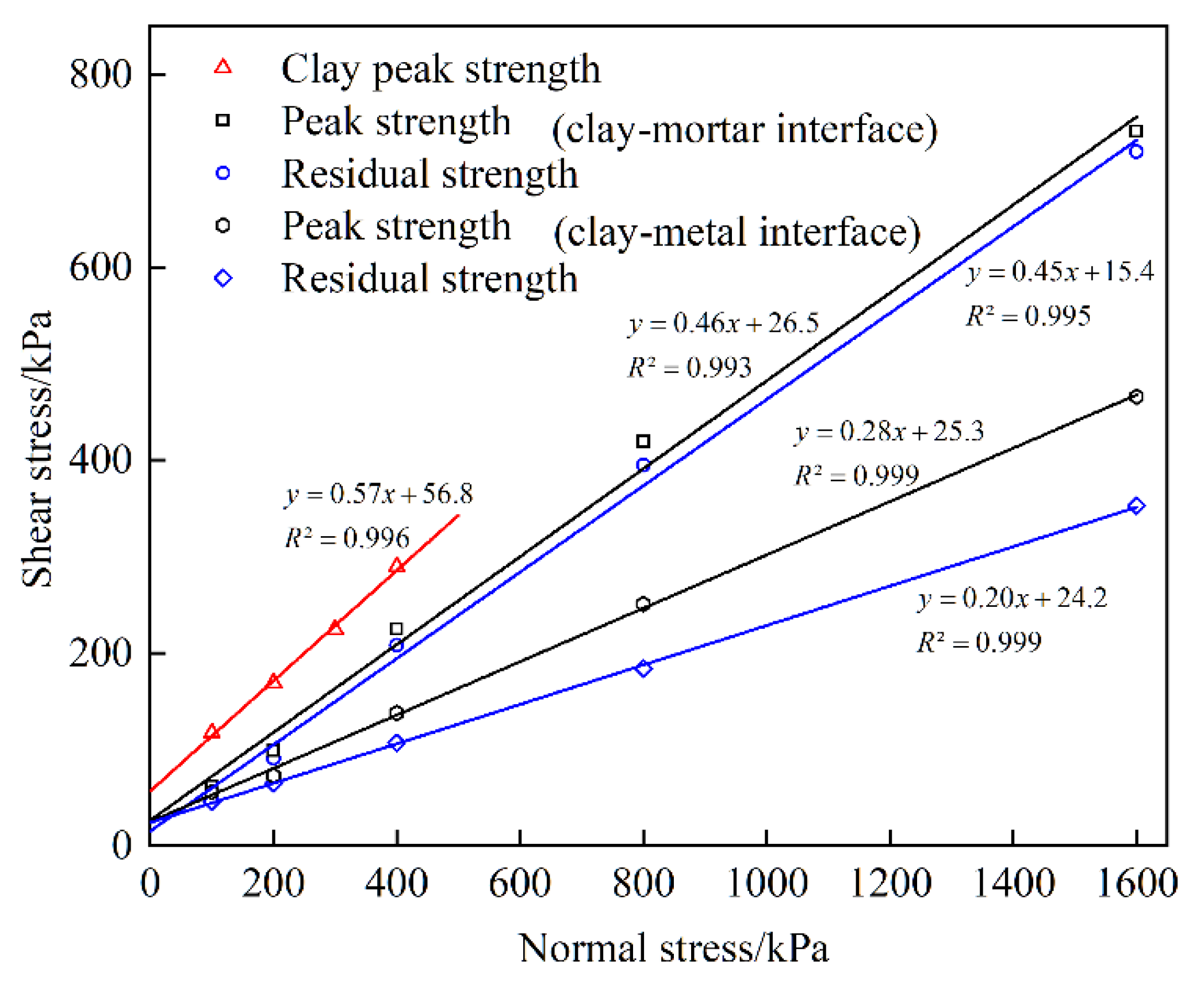
3.1.2. Shear Strength
3.2. Seepage Behavior Analysis
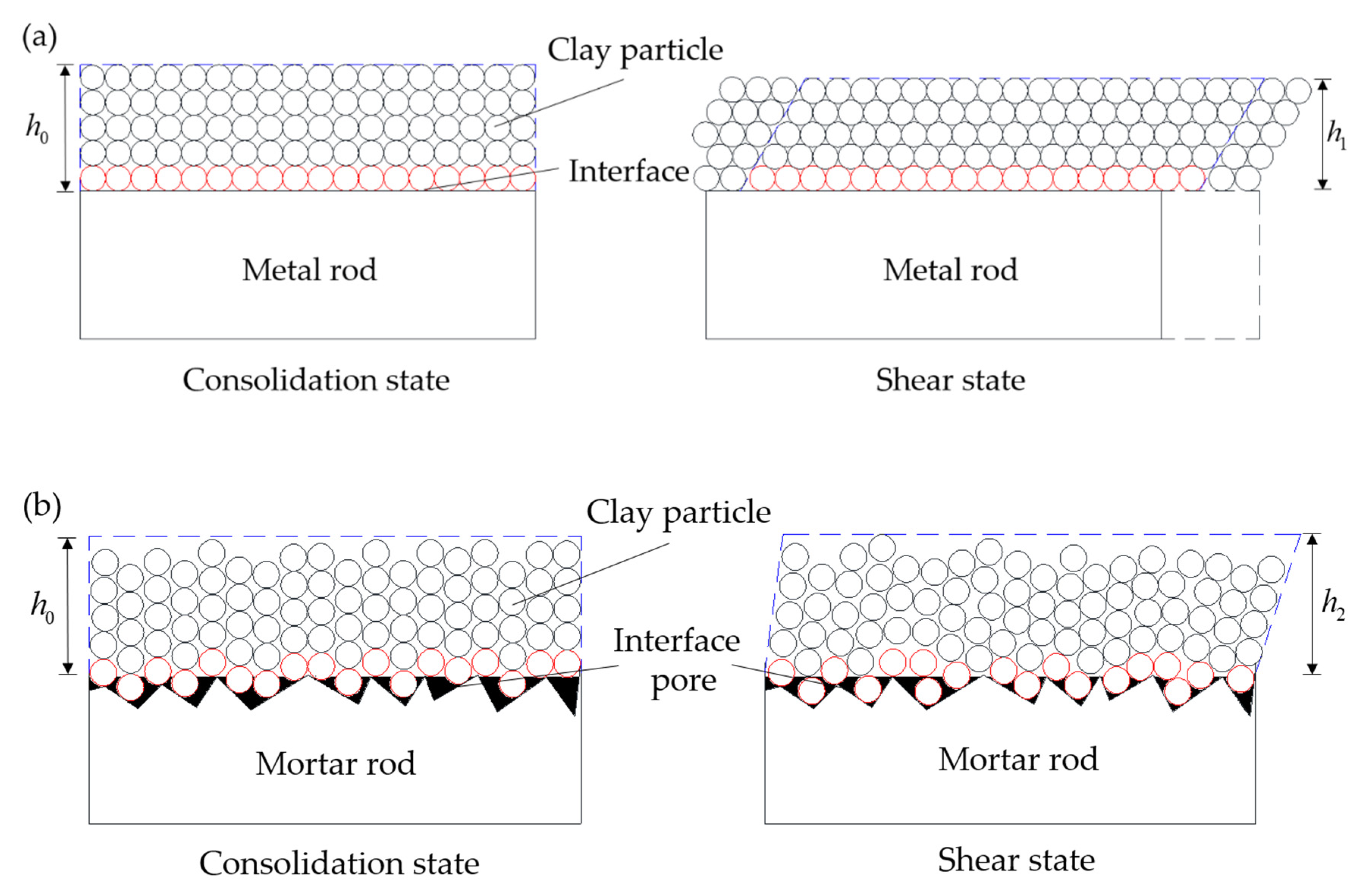
3.2.1. Contact Seepage of Initial Interface
3.2.2. Shear-Seepage Process
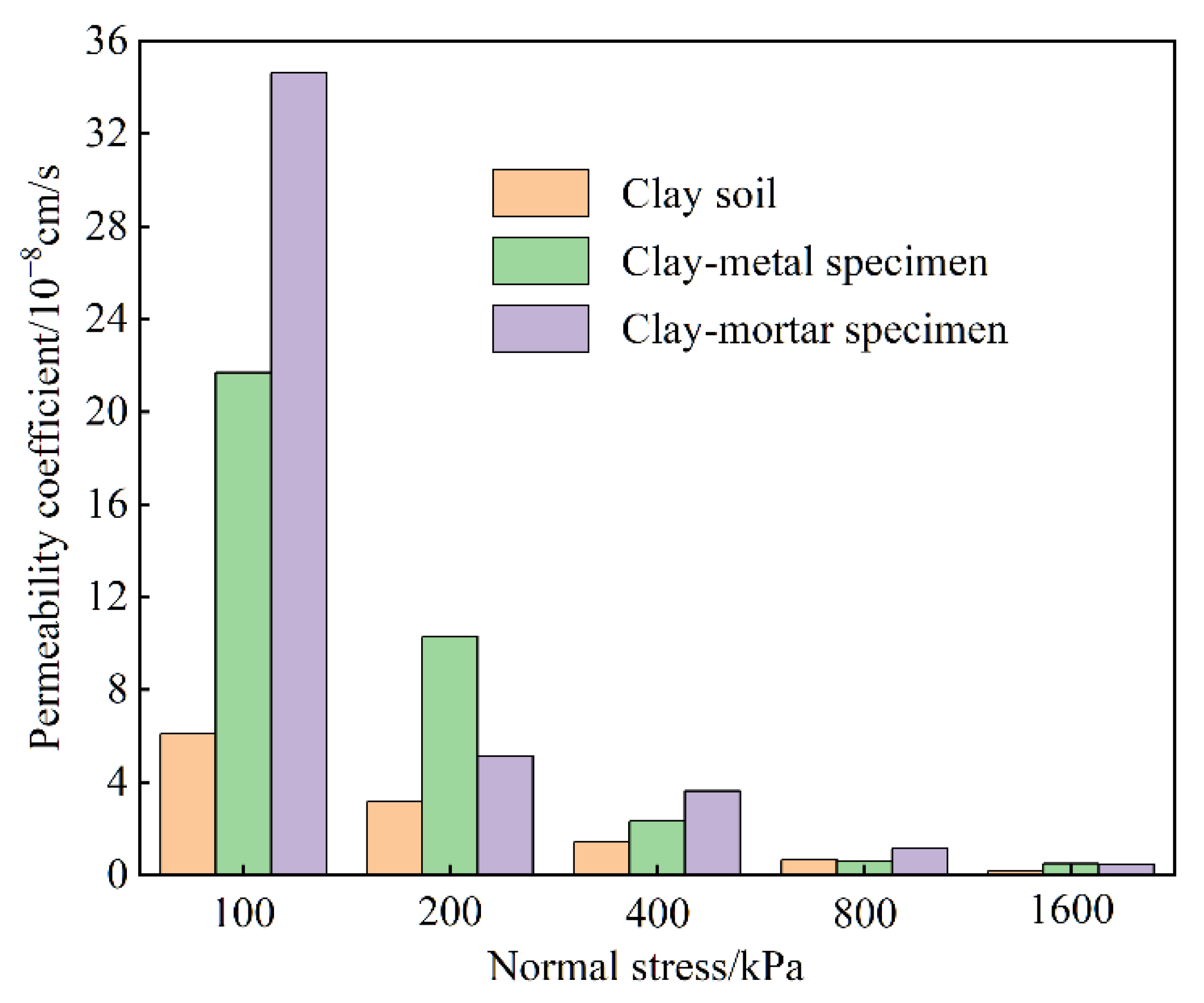
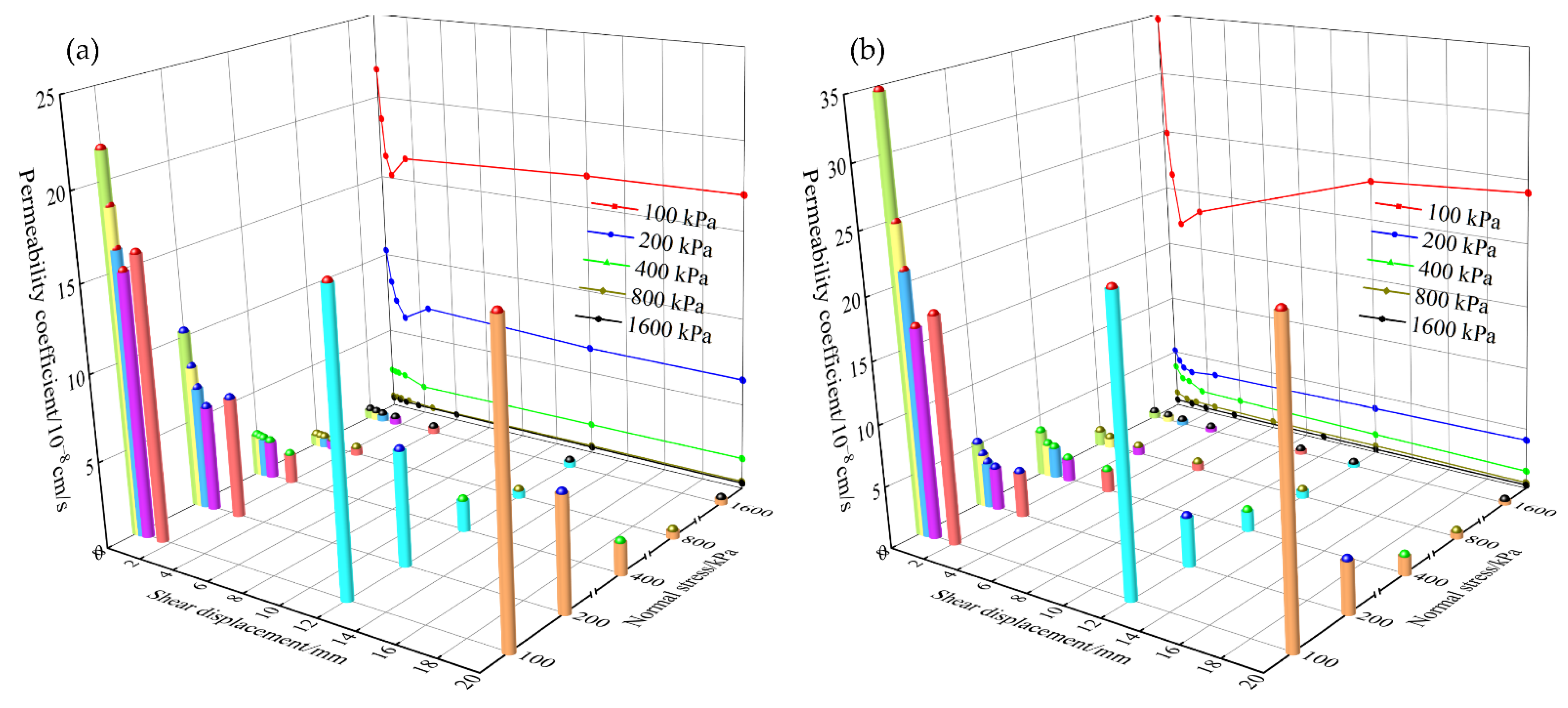
3.2.3. Overall Permeability Coefficient after Shear Deformation
4. Discussion
5. Conclusions
- Both the peak and residual shear strength of clay–structure interface increase as the normal stress increases. The shear strength and peak displacement values of clay soil, clay–mortar interface, and clay–metal interface decrease successively.
- The evolution laws of overall specimen permeability coefficient are different under low and high normal stress. The effect of interface roughness on the specimen permeability is remarkable under low normal stress.
- The overall permeability coefficient after shear deformation is less than its initial value under all test schemes. There is no seepage failure phenomenon on the clay–structure interface after large shear deformation in our tests.
- The evolution of both shear dilation and interface pore in the shear process controls clay–structure specimen permeability evolution with shear deformation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ma, H.; Chi, F. Major Technologies for Safe Construction of High Earth-Rockfill Dams. Engineering 2016, 2, 498–509. [Google Scholar] [CrossRef]
- Wu, Y.; Zhang, B.; Yu, Y.; Zhang, Z. Consolidation Analysis of Nuozhadu High Earth-Rockfill Dam Based on the Coupling of Seepage and Stress-Deformation Physical State. Int. J. Geomech. 2016, 16, 4015085. [Google Scholar] [CrossRef]
- Lei, H.; Wu, Y.; Yu, Y.; Zhang, B.; Lv, H. Influence of Shear on Permeability of Clayey Soil. Int. J. Geomech. 2016, 16, 4016010. [Google Scholar] [CrossRef]
- Liu, Q.; Wu, Y.; Li, Q.; Yu, Y.; Lv, H. Modified Model for Hydraulic Conductivity of Clayey Soil under Shear. Int. J. Geomech. 2019, 19, 6019015. [Google Scholar] [CrossRef]
- Wang, G.; Wei, L.; Wei, X.; Zhang, J. Progress of study on hydraulic failure mechanism of clay core-wall in high earth-rockfill dam. Water Resour. Hydro. Eng. 2019, 50, 58–65. [Google Scholar]
- Deng, G.; Huangfu, Z.; Wu, Y.; Zhang, Y.; Chen, H.; Zhang, Y.; Yang, Y. Development and prospect of deformation compatibility control of earth core embankment dams. J. Hydro. Eng. 2020, 39, 1–16. [Google Scholar] [CrossRef]
- Wang, X. Discussion on some problems observed in high earth-rockfill dams. Chin. J. Geotech. Eng. 2018, 40, 203–222. [Google Scholar] [CrossRef]
- Sherard, J.L. Lessons from the Teton dam failure. Eng. Geol. 1987, 24, 239–256. [Google Scholar] [CrossRef]
- Seed, H.B.; Duncan, J.M. The failure of teton dam. Eng. Geol. 1987, 24, 173–205. [Google Scholar] [CrossRef]
- Penman, A.D.M. On the embankment dam. Geotechnique 1986, 36, 303–348. [Google Scholar] [CrossRef] [Green Version]
- Foster, M.; Fell, R.; Spannagle, M. The statistics of embankment dam failures and accidents. Can. Geotech. J. 2000, 37, 1000–1024. [Google Scholar] [CrossRef]
- Wang, X.; Cheng, H.; Yan, P.; Zhang, J.; Ding, Y. The influence of roughness on cyclic and post-cyclic shear behavior of red clay-concrete interface subjected to up to 1000 cycles. Constr. Build. Mater. 2021, 273, 121718. [Google Scholar] [CrossRef]
- Taha, A.; Fall, M. Shear Behavior of Sensitive Marine Clay-Concrete Interfaces. J. Geotech. Geoenviron. 2013, 139, 644–650. [Google Scholar] [CrossRef]
- Maghsoodi, S.; Cuisinier, O.; Masrouri, F. Thermal effects on mechanical behaviour of soil-structure interface. Can. Geotech. J. 2020, 57, 32–47. [Google Scholar] [CrossRef] [Green Version]
- Li, C.; Kong, G.; Liu, H.; Abuel-Naga, H. Effect of temperature on behaviour of red clay-structure interface. Can. Geotech. J. 2019, 56, 126–134. [Google Scholar] [CrossRef]
- Yavari, N.; Tang, A.M.; Pereira, J.; Hassen, G. Effect of temperature on the shear strength of soils and soil/structure interface. Can. Geotech. J. 2016, 53, 1186–1194. [Google Scholar] [CrossRef]
- Xie, Q.; Liu, J.; Han, B.; Li, H.; Li, Y.; Jiang, Z. Experimental investigation of interfacial erosion on culvert-soil interface in earth dams. Soils Found. 2019, 59, 671–686. [Google Scholar] [CrossRef]
- Xie, Q.; Liu, J.; Han, B.; Li, H.; Li, Y.; Li, X. Experimental and Numerical Investigation of Bottom Outlet Leakage in Earth-Fill Dams. J. Perform. Constr. Fac. 2019, 33, 4019037. [Google Scholar] [CrossRef]
- Xie, Q.; Liu, J.; Han, B.; Li, H.; Li, Y.; Li, X. Critical Hydraulic Gradient of Internal Erosion at the Soil-Structure Interface. Processes 2018, 6, 92. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.J.; Park, J.M.; Shin, J.H. Flow behaviour and piping potential at the soil-structure interface. Géotechnique 2019, 69, 79–84. [Google Scholar] [CrossRef]
- Wang, G.; You, K.; Wei, X.; Zhang, J. Experimental study on permeability of shear bands in compacted clay. Chin. J. Geotech. Eng. 2019, 41, 1530–1537. [Google Scholar] [CrossRef]
- Wei, X.; Zou, T.; Wang, G. Experimental study on permeability of clay during coupled compression and shear. Chin. J. Rock Mech. Eng. 2017, 36, 3561–3568. [Google Scholar] [CrossRef]
- Enayat, M.; Shoaei, G.; Nikudel, M.R. A Conceptual Model and Evaluating Experiments for Studying the Effect of Soil Deformation on Its Permeability. Shock Vib. 2021, 2021, 6652223. [Google Scholar] [CrossRef]
- Chang, D.S.; Zhang, L.M. Critical Hydraulic Gradients of Internal Erosion under Complex Stress States. J. Geotech. Geoenviron. 2013, 139, 1454–1467. [Google Scholar] [CrossRef]
- Lei, H.; Bian, F.; Yu, Y.; Sun, X. Experimental study of permeability of clayey soil during process of large shear deformation. Rock Soil Mech. 2010, 31, 1130–1133. [Google Scholar] [CrossRef]
- Wang, G.; Wei, L.; Wei, X.; Zhang, J. Permeability evolution of compacted clay during triaxial compression. Rock Soil Mech. 2020, 41, 32–38. [Google Scholar] [CrossRef]
- Lei, H.; Liu, Z.; Yu, Y.; Lu, H. Experimental study of seepage characteristics of clayey soil-structure interface under large shear deflormation. Rock Soil Mech. 2011, 32, 1040–1044. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, X.; Zhan, Z.; Yu, Y.; Lu, H. Analysis on seepage mechanism at soil-structure interface under shear. J. Hydro. Eng. 2021, 40, 113–121. [Google Scholar] [CrossRef]
- Luo, Y.; Jin, X.; Li, X.; Zhan, M.; Sheng, J. A New Apparatus for Evaluation of Contact Erosion at the Soil-Structure Interface. Geotech. Test. J. 2013, 36, 20120094. [Google Scholar] [CrossRef]
- Luo, Y.; Zhan, M.; Sheng, J.; Wu, Q. Hydro-mechanical coupling mechanism on joint of clay core-wall and concrete cut-off wall. J. Cent. South Univ. 2013, 20, 2578–2585. [Google Scholar] [CrossRef]
- Wang, S.; Chen, J.; Sheng, J.; Luo, Y. Laboratory investigation of stress state and grain composition affecting internal erosion in soils containing a suspended cut-off wall. KSCE J. Civ. Eng. 2016, 20, 1283–1293. [Google Scholar] [CrossRef]
- Wang, S.; Chen, J.; Luo, Y.; Sheng, J. Experiments on internal erosion in sandy gravel foundations containing a suspended cutoff wall under complex stress states. Nat. Hazards 2014, 74, 1163–1178. [Google Scholar] [CrossRef]
- Deng, G.; Zhang, Y.; Zhang, Y.; Zhan, Z.; Yang, J.; Lu, J.; Cao, X. Impermeability characteristics of junctional zone between compacted broadly graded clayey soil and hard surface. Chin. J. Geotech. Eng. 2021, 43, 1631–1639. [Google Scholar] [CrossRef]
- Zhang, Y.; Deng, G.; Zhang, Y.; Lu, J.; Cao, X. Experimental study on permeability characteristics of junctional zone between widely graded clay and concrete. Water Resour. Hydro. Eng. 2021, 52, 182–190. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, J.; Xiao, Y.; Li, J. Effect of roughness on shear behavior of red clay-concrete interface in large-scale direct shear tests. Can. Geotech. J. 2015, 52, 1122–1135. [Google Scholar] [CrossRef]
- ASTM D2487-17; Standard Practice for Classification of Soils for Engineering Purposes (Unified Soil Classification System). ASTM International: West Conshohocken, PA, USA, 2006. [CrossRef]
- ASTM D5321-02; Standard Test Method for Determining the Coefficient of Soil and Geosynthetic or Geosynthetic and Geosynthetic Friction by the Direct Shear Method. ASTM International: West Conshohocken, PA, USA, 2002. [CrossRef]
- Daniel, D.E. State-of-the-Art: Laboratory Hydraulic Conductivity Tests for Saturated Soils. ASTM Spec. Tech. Publ. 1994, 1142, 30. [Google Scholar] [CrossRef]

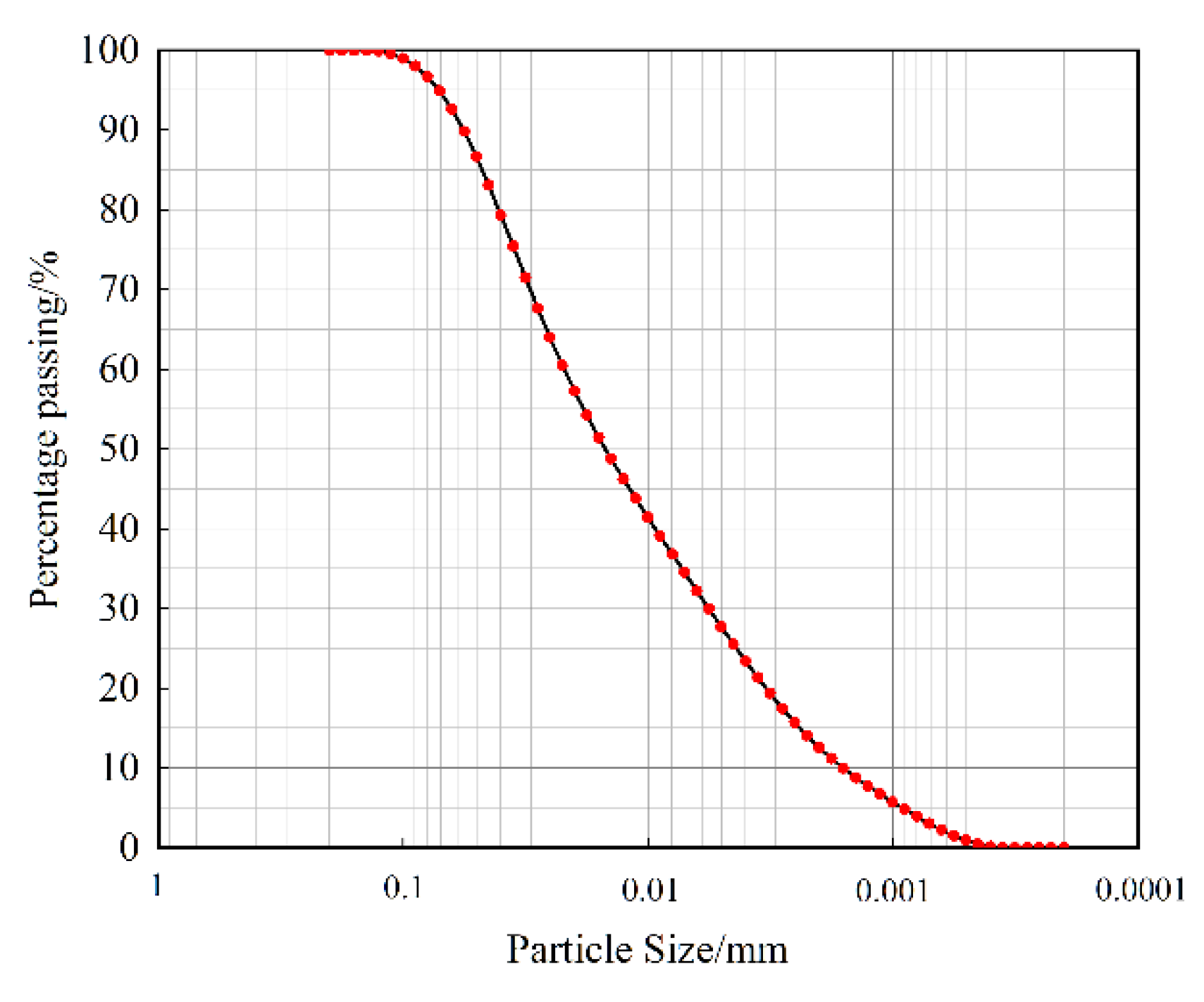


| Liquid Limit | Plastic Limit | Plasticity Index | Specific Gravity | Optimum Moisture Content | Maximum Dry Density |
|---|---|---|---|---|---|
| 31.0 | 15.7 | 15.3 | 2.725 | 15.6 | 1.83 |
| Type | Normal Stress (kPa) | Shear Displacement Control Point (mm) | Seepage Pressure (kPa) | Structure Rod |
|---|---|---|---|---|
| Rotary shear test | 100, 200, 400, 800, 1600 | 0~20 | - | Metal rod |
| Mortar rod | ||||
| Shear-seepage test | 100 | 0, 0.15, 0.3, 0.6, 1.5, 12, 20 | 40 | Metal rod |
| 200 | 0, 0.25, 0.5, 1, 2.5, 12, 20 | 80 | ||
| 400 | 0, 0.2, 0.4, 0.8, 2, 12, 20 | 160 | ||
| 800 | 0, 0.25, 0.5, 1, 2.5, 12, 20 | 320 | ||
| 1600 | 0, 0.4, 0.8, 1.6, 4, 12, 20 | 640 | ||
| 100 | 0, 0.2, 0.4, 0.8, 2, 12, 20 | 40 | Mortar rod | |
| 200 | 0, 0.25, 0.5, 1, 2.5, 12, 20 | 80 | ||
| 400 | 0, 0.4, 0.8, 1.6, 4, 12, 20 | 160 | ||
| 800 | 0, 0.6, 1.2, 2.4, 6, 12, 20 | 320 | ||
| 1600 | 0, 0.9, 1.8, 3.6, 9, 12, 20 | 640 |
| Clay soil | 56.8 | 29.7 | - | - |
| Clay–metal interface | 25.3 | 15.6 | 24.2 | 11.3 |
| Clay–mortar interface | 26.5 | 24.7 | 15.4 | 24.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tan, J.; Shen, Z.; Xu, L.; Zhang, H.; He, Y. Experimental Investigation on Seepage Characteristics of Clay–Structure Interface after Shear Deformation. Materials 2022, 15, 3802. https://doi.org/10.3390/ma15113802
Tan J, Shen Z, Xu L, Zhang H, He Y. Experimental Investigation on Seepage Characteristics of Clay–Structure Interface after Shear Deformation. Materials. 2022; 15(11):3802. https://doi.org/10.3390/ma15113802
Chicago/Turabian StyleTan, Jiacheng, Zhenzhong Shen, Liqun Xu, Hongwei Zhang, and Yingming He. 2022. "Experimental Investigation on Seepage Characteristics of Clay–Structure Interface after Shear Deformation" Materials 15, no. 11: 3802. https://doi.org/10.3390/ma15113802
APA StyleTan, J., Shen, Z., Xu, L., Zhang, H., & He, Y. (2022). Experimental Investigation on Seepage Characteristics of Clay–Structure Interface after Shear Deformation. Materials, 15(11), 3802. https://doi.org/10.3390/ma15113802







