Numerical Analysis of the Forming Mechanism of Exit Burrs in Metal Milling under Ice Boundary Constraint
Abstract
:1. Introduction
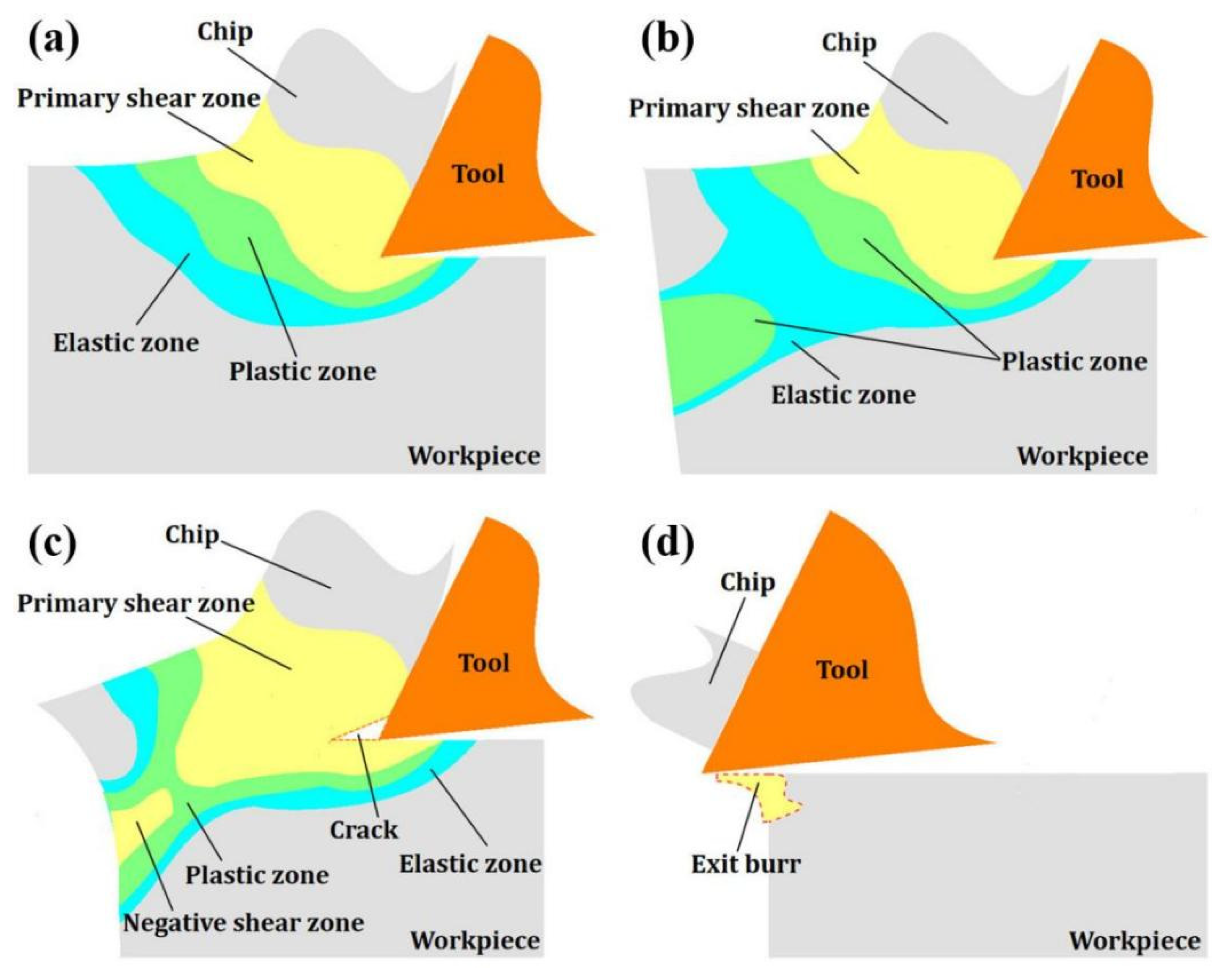
2. Analysis of the Formation Process of Exit Burr
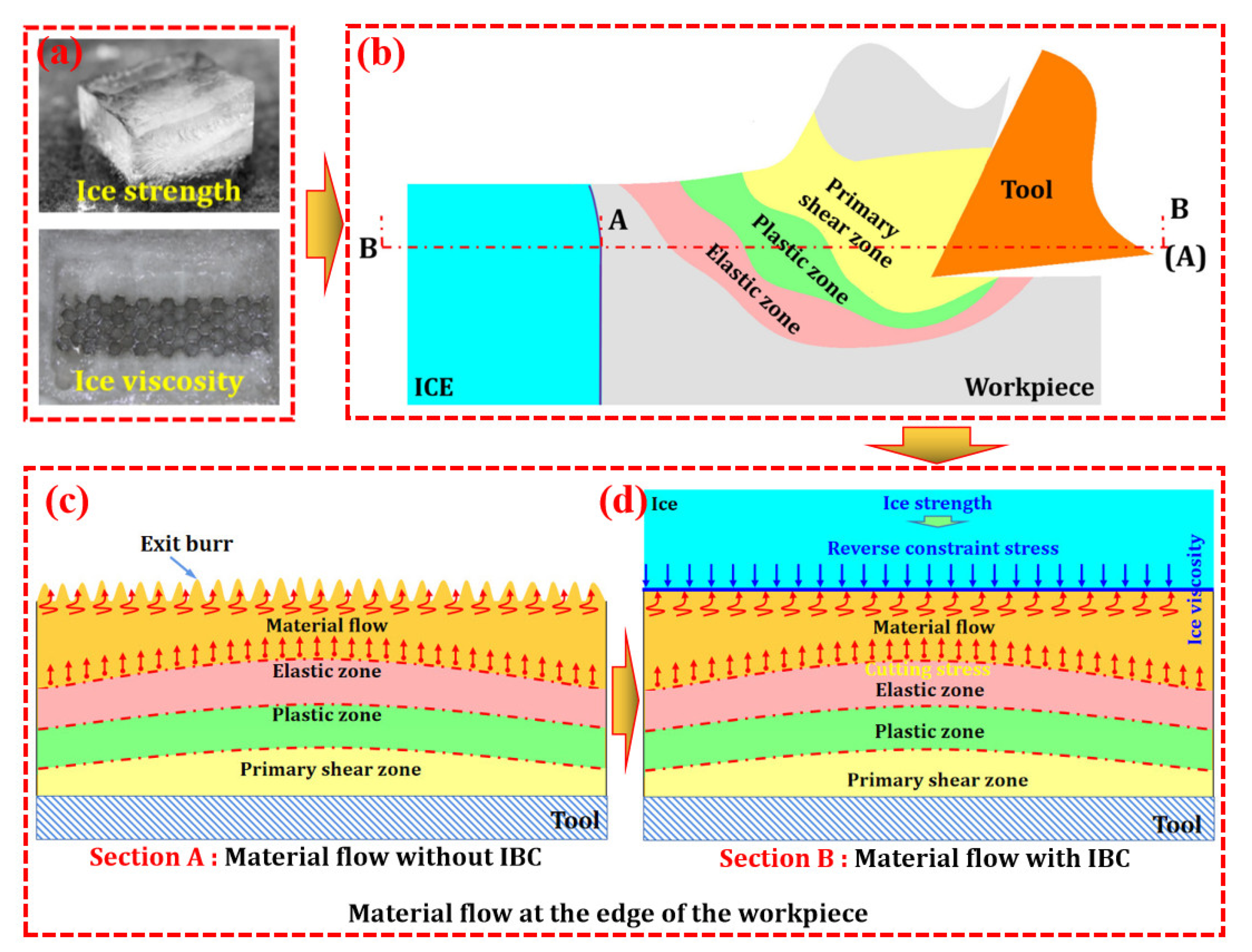
3. The Principle of the Ice Boundary Constraint (IBC) Method
4. Numerical Analysis
4.1. Material Constitutive Model
4.2. Friction Coefficient
4.3. Cryogenic Cooling Environment
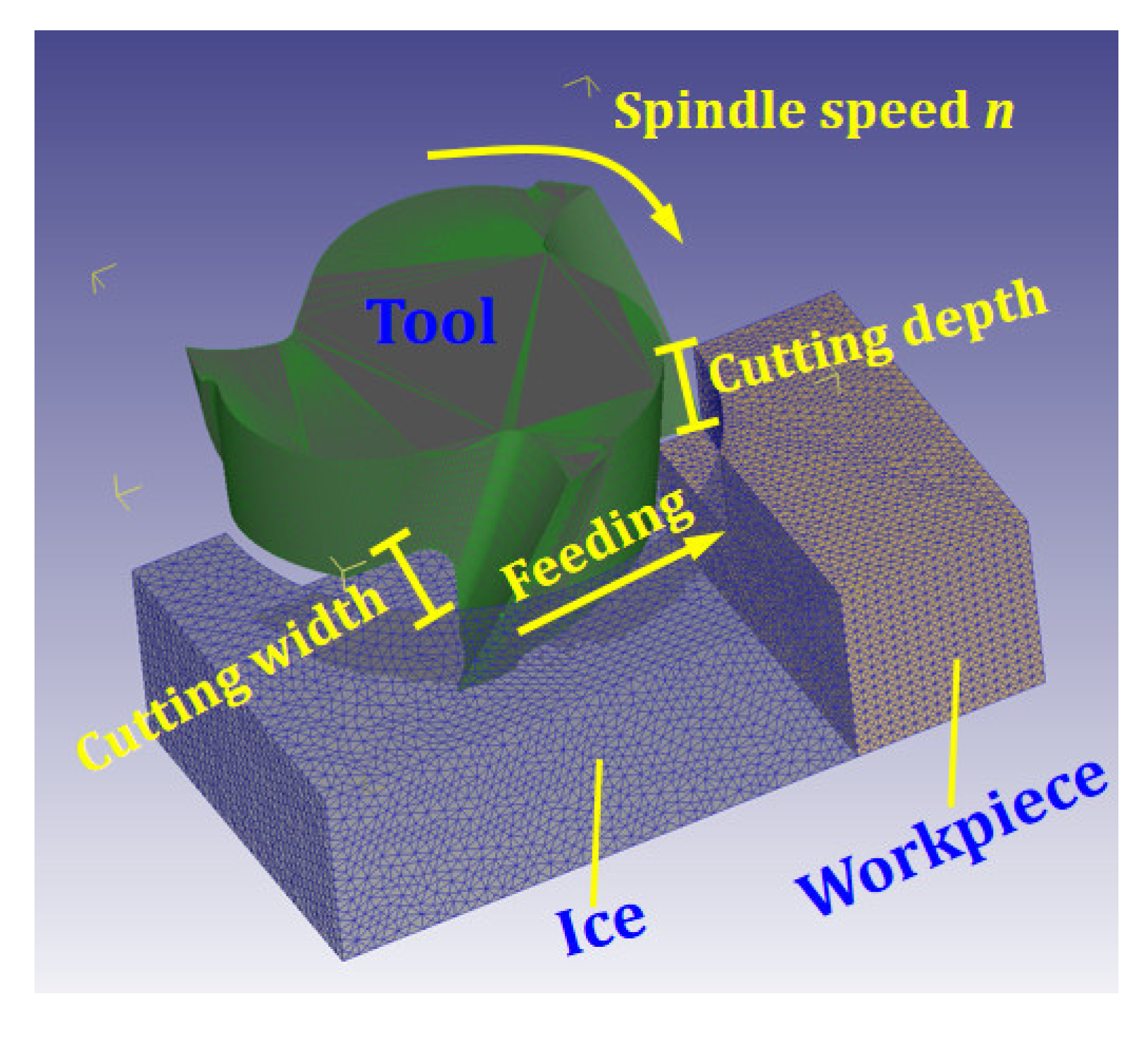
4.4. Cutting Simulation
4.5. Simulation Results Analysis
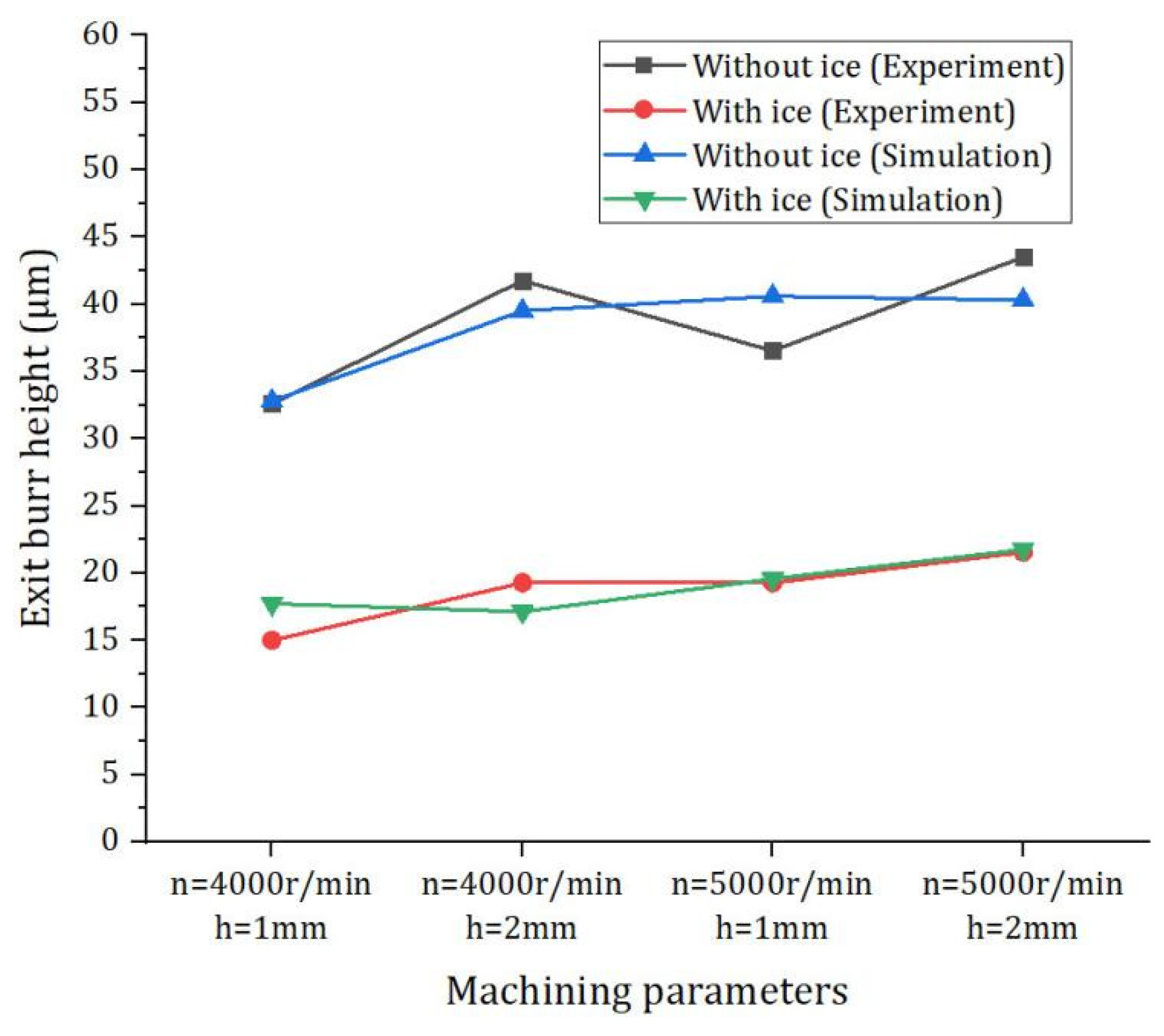
5. Experimental Verification
6. Conclusions
- (1)
- According to the simulation results, it can be seen that IBC can effectively suppress the flow of material caused by cutting stress and reduce the plastic bending deformation at the edge of the workpiece, thereby avoiding the formation of negative shear zone and, ultimately, suppressing the generation of exit burrs.
- (2)
- Under conventional machining, the edge topography of the workpiece changes significantly with the change in cutting parameters. However, the suppressing effect of IBC on exit burrs is less affected by cutting parameters.
- (3)
- The experimental results show that IBC can effectively suppress the formation of exit burrs, thereby improving the edge quality of the part. The comparison results show that the height of exit burr is reduced by 51.4% on average.
- (4)
- The finite element model is verified by the milling experiments. By comparing the simulation results with the experimental results, it can be seen that the finite element model can effectively predict exit burr height in milling experiments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| IBC | Ice boundary constraint | Kronecker symbol | |
| FEM | Finite element model | Lame coefficient | |
| AA2024 | Aluminum alloy 2024 | Equivalent plastic strain | |
| J-C | Johnson–cook | Hydrostatic stress | |
| Flow stress | Failure strain | ||
| Plastic strain | Initial failure strain | ||
| Plastic strain rate | LN2 | Liquid nitrogen | |
| Equivalent plastic strain | Friction coefficient | ||
| Material tempurature | Friction between the tool and the machined surface | ||
| Room tempurature | Normal stress | ||
| Melting tempurature | Heat dissipation density | ||
| Johnson–Cook constitutive constants | Tool temperature | ||
| Increment of the equivalent plastic strain | Average convective heat transfer coefficient | ||
| Equivalent strain of fracture | Effective cooling strength | ||
| Johnson–Cook damage constants for Aluminum alloy 2024 | Heat transfer coefficient of air | ||
| Ice constants | Prandtl number | ||
| Hydrostatic stress | Reynolds number | ||
| Octahedral shear stress | Actual heat transfer coefficient | ||
| Stress increment | Compensation coefficient |
References
- Régnier, T.; Fromentin, G.; D’Acunto, A.; Outeiro, J.; Marcon, B.; Crolet, A. Phenomenological Study of Multivariable Effects on Exit Burr Criteria During Orthogonal Cutting of AlSi Alloys Using Principal Components Analysis. J. Manuf. Sci. Eng. 2018, 140, 101006. [Google Scholar] [CrossRef]
- Aurich, J.C.; Dornfeld, D.; Arrazola, P.J.; Franke, V.; Leitz, L.; Min, S. Burrs—Analysis, control and removal. CIRP Ann. 2009, 58, 519–542. [Google Scholar] [CrossRef]
- Niknam, S.A.; Davoodi, B.; Davim, J.P.; Songmene, V. Mechanical deburring and edge-finishing processes for aluminum parts—A review. Int. J. Adv. Manuf. Technol. 2017, 95, 1101–1125. [Google Scholar] [CrossRef]
- Niknam, S.A.; Songmene, V. Milling burr formation, modeling and control: A review. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2015, 229, 893–909. [Google Scholar] [CrossRef]
- Rana, A.; Dongre, G.; Joshi, S.S. Analytical modeling of exit Burr in drilling of Ti6Al4V alloy. Sadhana Acad. Proc. Eng. Sci. 2019, 44, 133. [Google Scholar] [CrossRef]
- Zhang, X.; Yu, T.; Wang, W.; Zhao, J. Improved analytical prediction of burr formation in micro end milling. Int. J. Mech. Sci. 2018, 151, 461–470. [Google Scholar] [CrossRef]
- Pang, X.; Zhang, J.; Yin, X.; Zhang, B.; Deng, W. Analytical and experimental investigation of improved burr morphology prediction at the top edge in metal machining. Int. J. Adv. Manuf. Technol. 2020, 108, 1343–1355. [Google Scholar] [CrossRef]
- Hassan, A.A.; Soo, S.L.; Aspinwall, D.K.; Arnold, D.; Dowson, A. An analytical model to predict interlayer burr size following drilling of CFRP-metallic stack assemblies. CIRP Ann. 2020, 69, 109–112. [Google Scholar] [CrossRef]
- Luan, Y.; Lu, X.; Hou, P.; Liang, S.Y. Characteristics and Mechanism of Top Burr Formation in Micro-Milling LF21. J. Manuf. Sci. Eng. 2021, 143, 1–16. [Google Scholar] [CrossRef]
- Olsson, M.; Persson, H.; Agmell, M.; Bushlya, V.; Stahl, J.-E. FE simulation and experimental verification of side-flow and burr formation in machining of oxygen-free copper. Procedia CIRP 2018, 72, 1427–1432. [Google Scholar] [CrossRef]
- Yadav, A.K.; Kumar, M.; Bajpai, V.; Singh, N.K.; Singh, R.K. FE modeling of burr size in high- speed micro-milling of Ti6Al4V. Precis. Eng. J. Int. Soc. Precis. Eng. Nanotechnol. 2017, 49, 287–292. [Google Scholar] [CrossRef]
- Franczyk, E.; Slusarczyk, L.; Zebala, W. Drilling Burr Minimization by Changing Drill Geometry. Materials 2020, 13, 3207. [Google Scholar] [CrossRef] [PubMed]
- da Silva, L.C.; da Mota, P.R.; da Silva, M.B.; Sales, W.F.; Machado, A.R.; Jackson, M.J. Burr height minimization using the response surface methodology in milling of PH 13-8 Mo stainless steel. Int. J. Adv. Manuf. Technol. 2016, 87, 3485–3496. [Google Scholar] [CrossRef]
- Wu, F.; Liu, Z.; Guo, B.; Sun, Y.; Chen, J. Research on the burr-free interrupted cutting model of metals. J. Mater. Process. Technol. 2021, 295, 117190. [Google Scholar] [CrossRef]
- Gaitonde, V.N.; Karnik, S.R. Selection of optimal process parameters for minimizing burr size in drilling using Taguchi’s quality loss function approach. J. Braz. Soc. Mech. Sci. Eng. 2012, 34, 238–245. [Google Scholar] [CrossRef]
- Liu, Q.; Xu, J.; Yu, H. Experimental Study on the Influence of Tool Wear on the Cutting Process of Ti6Al4V. J. Phys. Conf. Ser. 2021, 1838, 012026. [Google Scholar] [CrossRef]
- Akula, S.; Nayak, S.N.; Bolar, G.; Managuli, V. Comparison of conventional drilling and helical milling for hole making in Ti6Al4V titanium alloy under sustainable dry condition. Manuf. Rev. 2021, 8, 12. [Google Scholar] [CrossRef]
- Kizhakken, V.; Mathew, J. Modeling of burr thickness in micro-end milling of Ti6Al4V. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2018, 233, 1087–1102. [Google Scholar] [CrossRef]
- Asad, M.; Ijaz, H.; Khan, M.A.A.; Khan, M.; Mabrouki, T.; Rashid, M.U. Comparative analyses and investigations of chamfered and honed-edge tool geometries on tool wear, chip morphology, residual stresses and end-burr formation. J. Manuf. Process. 2022, 80, 196–209. [Google Scholar] [CrossRef]
- Derradji-Aouat, A. Multi-surface failure criterion for saline ice in the brittle regime. Cold Reg. Sci. Technol. 2003, 36, 47–70. [Google Scholar] [CrossRef]







| Properties | AA2024 | Carbide Tool | Ice |
|---|---|---|---|
| Density (kg/m3) | 2700 | 7900 | 917 |
| Elastic modulus (Gpa) | 73 | 640 | 6.05 |
| Poisson’s ratio | 0.33 | 0.22 | 0.35 |
| Specific heat (J/kg K) | 0.557T + 877.6 | 220 | 2100 |
| Thermal conductivity (W/m K) | 0.247T + 114.4 (25 < T < 300) | 75.4 | 2 |
| Blade Number | Rake Angle | Tool Clearance | Blade Angle | Cutting-Edge Radius | Tool Coating | |
|---|---|---|---|---|---|---|
| Tool | 3 | 12° | 14° | 62° | 0.2 mm | No |
| A (Mpa) | B (Mpa) | C | n | m | |
|---|---|---|---|---|---|
| AA2024 | 352 | 440 | 0.0083 | 0.42 | 1 |
| D1 | D2 | D3 | D4 | D5 | |
|---|---|---|---|---|---|
| AA2024 | 0.13 | 0.13 | −1.5 | 0.011 | 0 |
| Ice | 22.39 | 2.06 | −0.023 |
| Workpiece | Length | Width | High | Materials |
|---|---|---|---|---|
| 8 | 4 | 4 | AA2024 | |
| Ice | Length | Width | High | Initial temperature |
| 8 | 11 | 4 | −20 °C | |
| Process | Radial depth of cut/h | Axial depth of cut/l | Spindle speed/n | Tool materials |
| 1 mm, 2 mm | 2 mm | 4000 r/min 5000 r/min | Tungsten steel carbide |
| Without IBC | With IBC | |
|---|---|---|
| Difference 1 | As the tool moves to the edge, the edge of the workpiece produces the plastic deformation zone and the primary shear zone in sequence. | As the cutting progresses, no plastic deformation zone and negative shear zone are generated at the edge of the workpiece. |
| Difference 2 | There is obvious bending deformation at the edge of the workpiece. | Only slight deformations occur at the edge of the workpiece. |
| Difference 3 | In the primary shear zone, a clear crack is produced along the cutting line. | No crack is generated in the primary shear zone. |
| No | Spindle Speed (n) (r/min) | Radial Depth of Cut (h) (mm) | Axial Depth of Cut (l) (mm) | Condition |
|---|---|---|---|---|
| 1 | 4000 | 1 | 2 | Without IBC |
| 2 | 4000 | 1 | 2 | With IBC |
| 3 | 4000 | 2 | 2 | Without IBC |
| 4 | 4000 | 2 | 2 | With IBC |
| 5 | 5000 | 1 | 2 | Without IBC |
| 6 | 5000 | 1 | 2 | With IBC |
| 7 | 5000 | 2 | 2 | Without IBC |
| 8 | 5000 | 2 | 2 | With IBC |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Xiong, W.; Ding, G.; Li, P.; Zhu, Z.; Liu, H. Numerical Analysis of the Forming Mechanism of Exit Burrs in Metal Milling under Ice Boundary Constraint. Materials 2022, 15, 5546. https://doi.org/10.3390/ma15165546
Wang C, Xiong W, Ding G, Li P, Zhu Z, Liu H. Numerical Analysis of the Forming Mechanism of Exit Burrs in Metal Milling under Ice Boundary Constraint. Materials. 2022; 15(16):5546. https://doi.org/10.3390/ma15165546
Chicago/Turabian StyleWang, Chengxin, Wentao Xiong, Guo Ding, Pengchao Li, Zhixiang Zhu, and Haibo Liu. 2022. "Numerical Analysis of the Forming Mechanism of Exit Burrs in Metal Milling under Ice Boundary Constraint" Materials 15, no. 16: 5546. https://doi.org/10.3390/ma15165546
APA StyleWang, C., Xiong, W., Ding, G., Li, P., Zhu, Z., & Liu, H. (2022). Numerical Analysis of the Forming Mechanism of Exit Burrs in Metal Milling under Ice Boundary Constraint. Materials, 15(16), 5546. https://doi.org/10.3390/ma15165546






