A Model to Calculate the Current–Temperature Relationship of Insulated and Jacketed Cables
Abstract
:1. Introduction
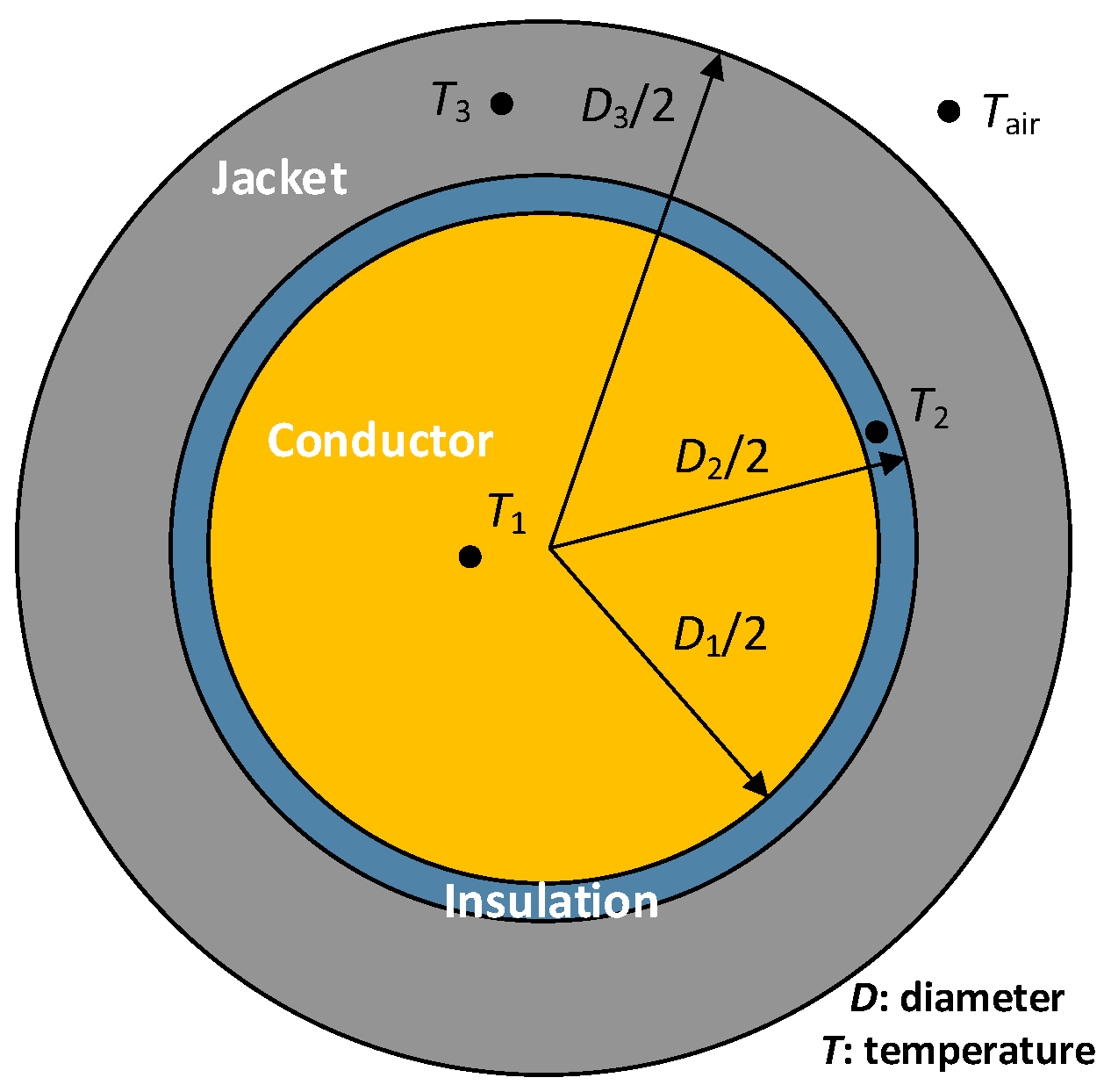
2. Transient Thermal Model of the Insulated and Jacketed Cable
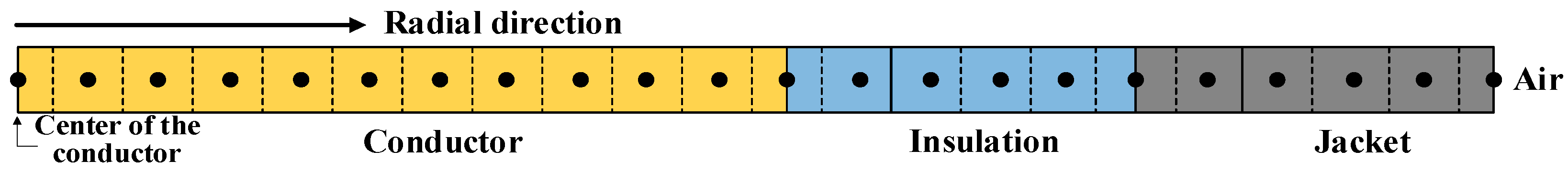
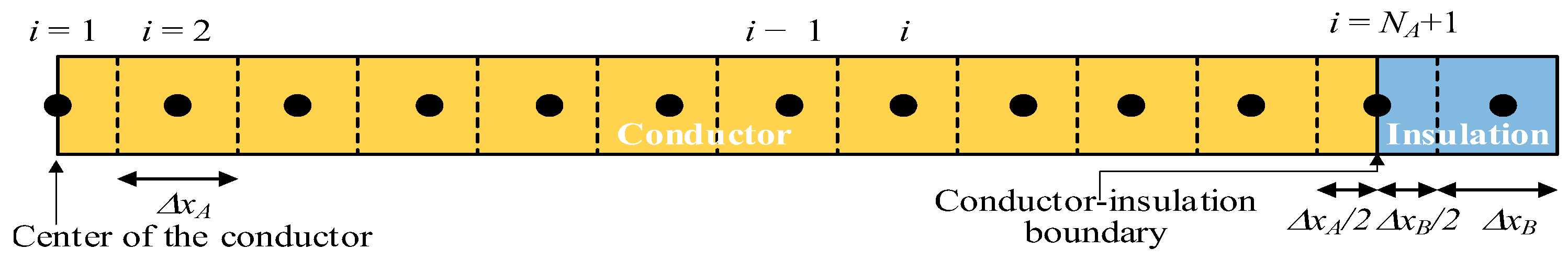
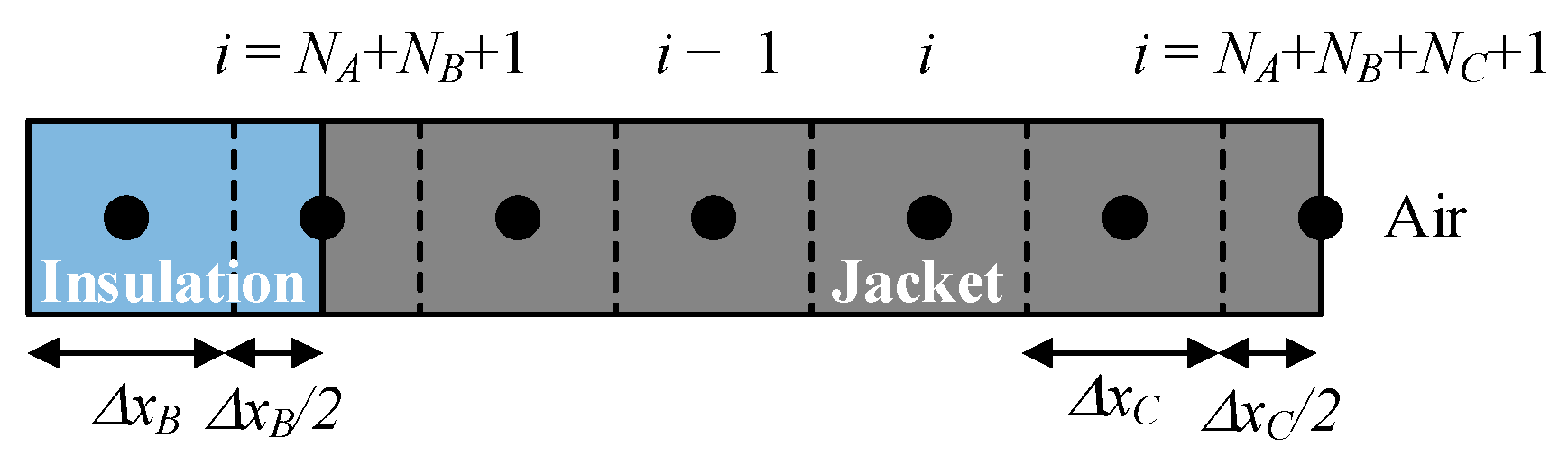
2.1. Domain Discretization and TDMA Formulation
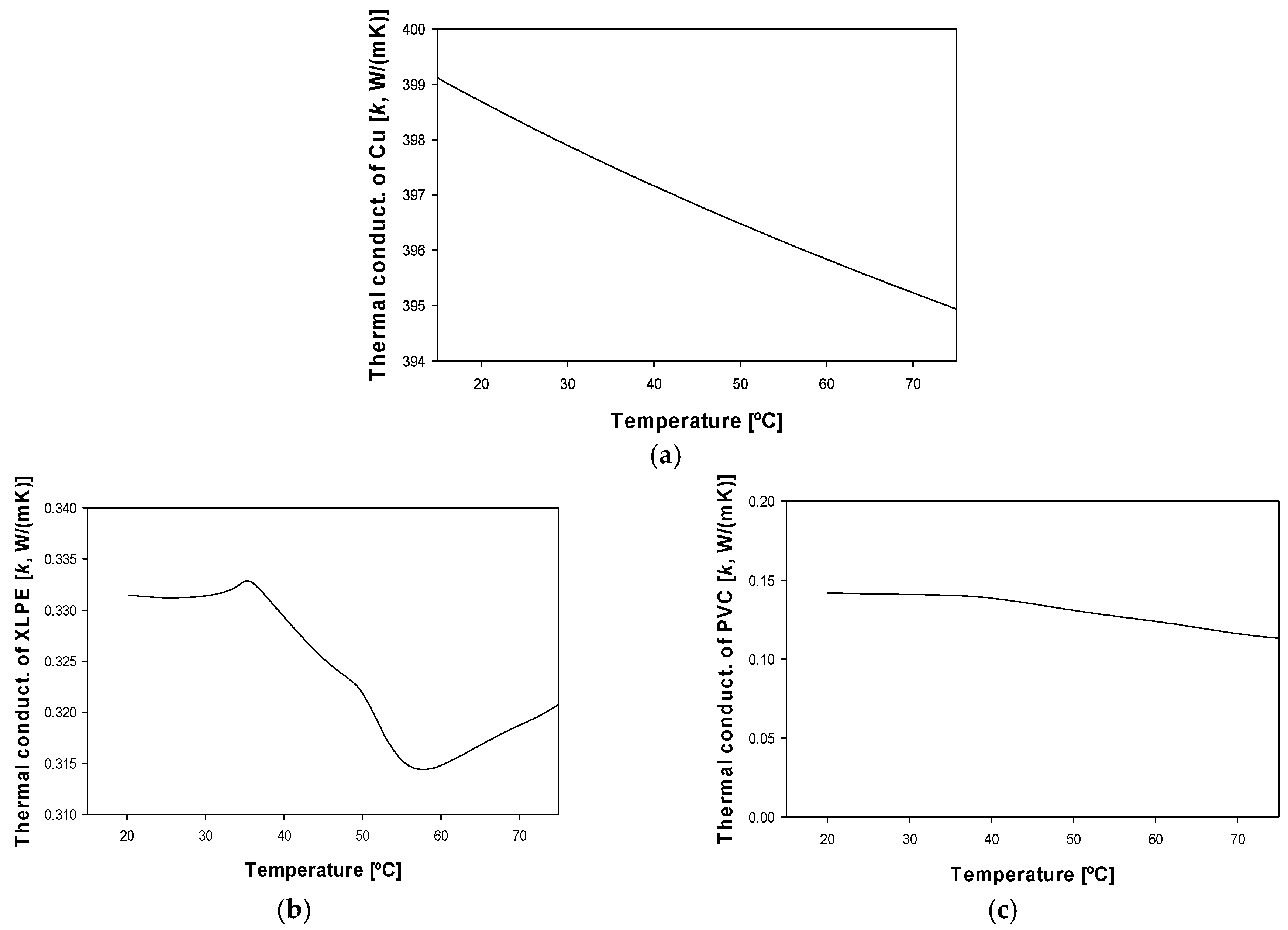
2.2. Conductor Discretization
2.3. Inner Insulation Discretization
2.4. Outer Jacket Discretization
3. Experimental
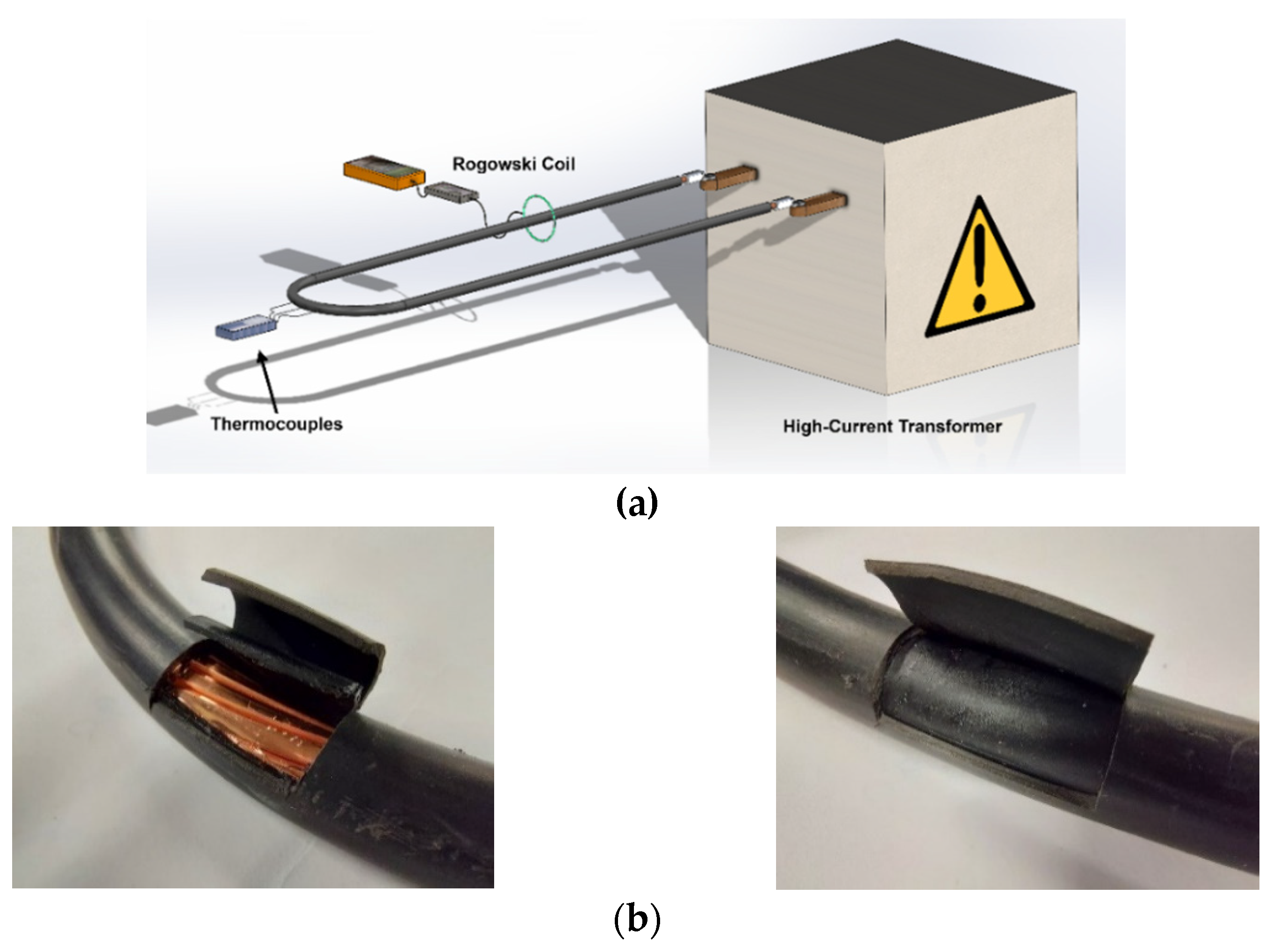
3.1. Experimental Setup
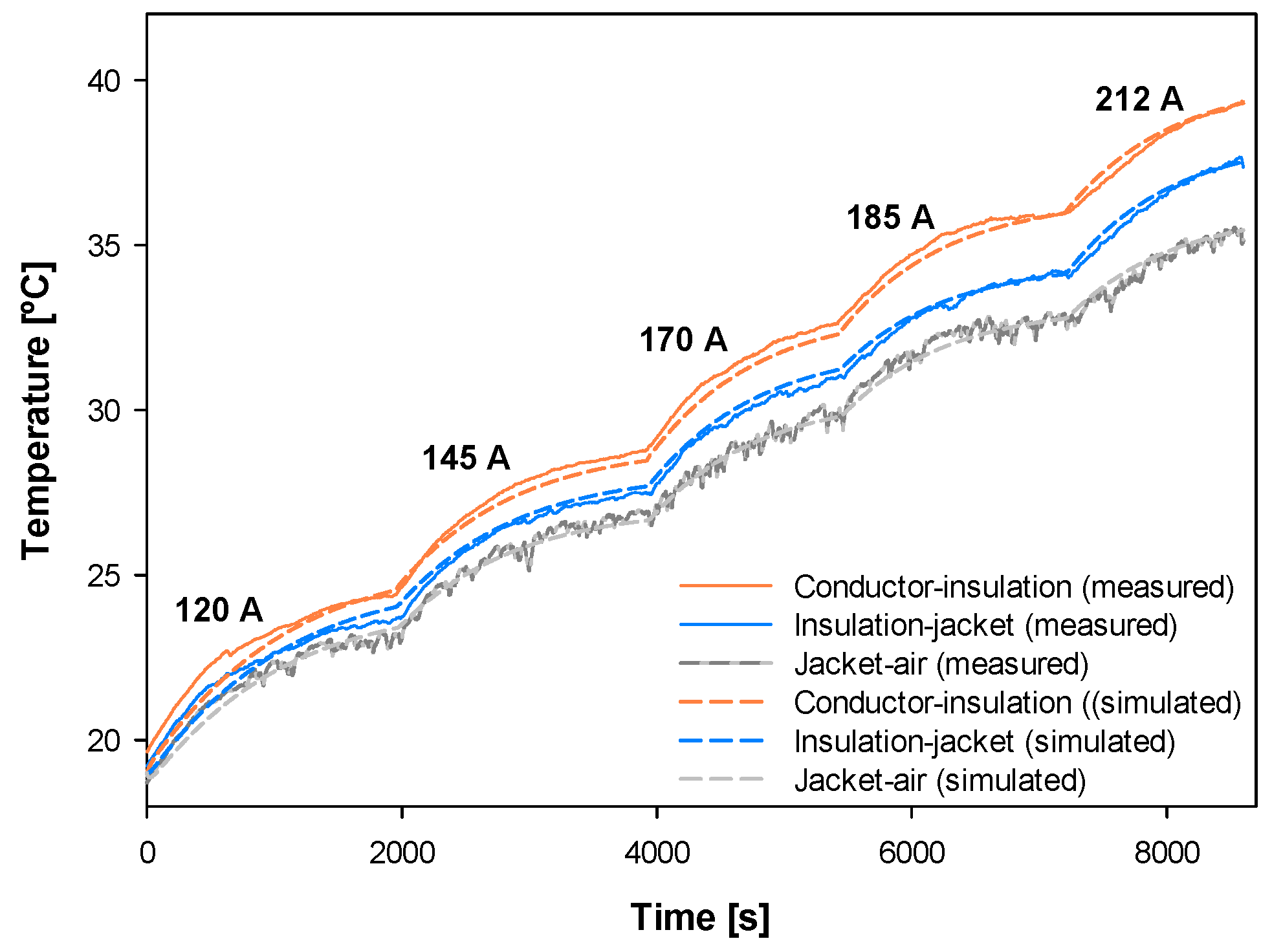
3.2. Experimental Results
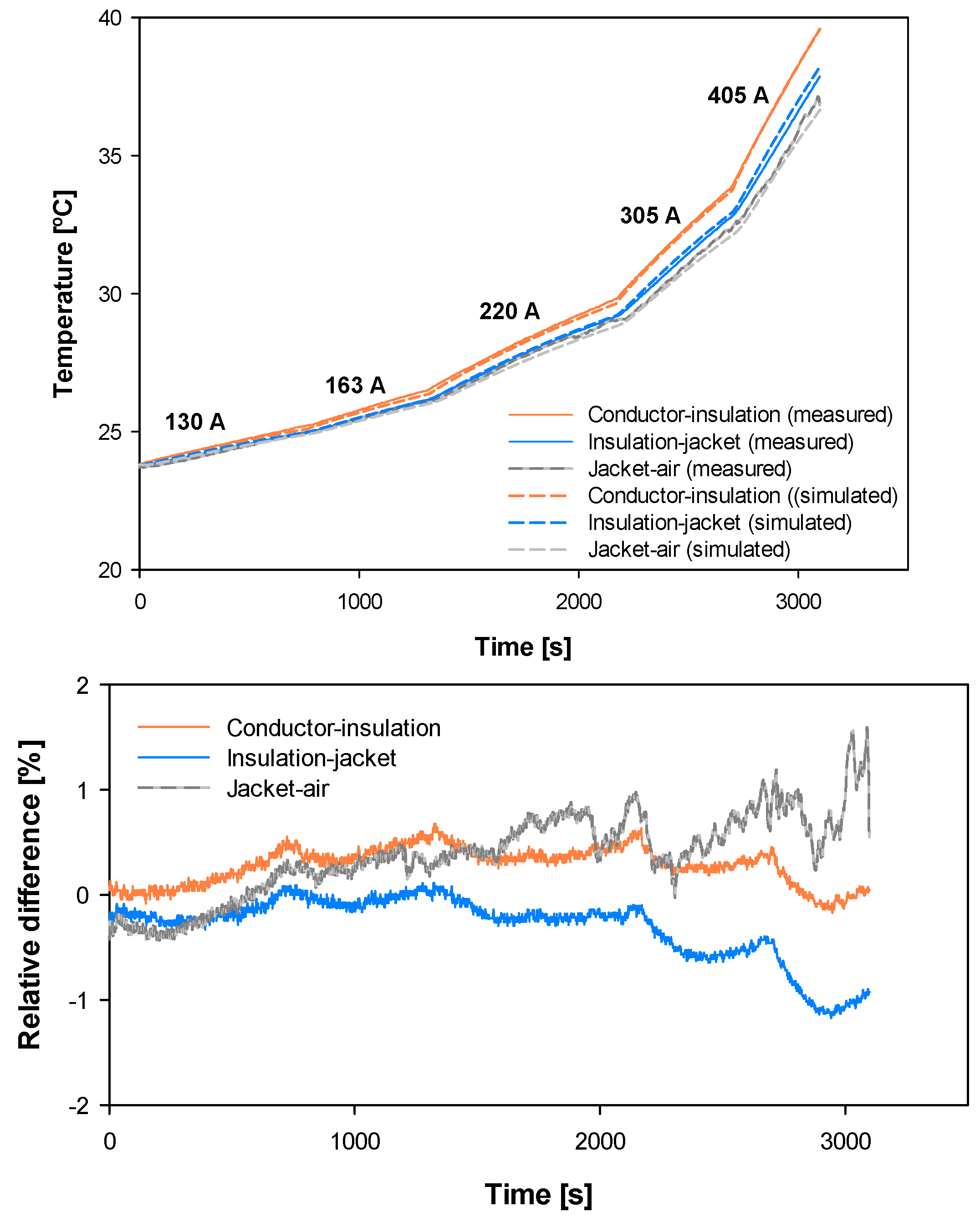
3.3. Additional Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gu, L.; Mo, W.; Wang, Y.; Fan, G.; Liu, H.; Wang, P.; Jia, S.; Xiu, S.; Zhao, L.; Chu, B. Analysis of Short-time Withstand Current and Peak Withstand Current Test of 500kV AC Fault Current Limiter. In Proceedings of the 2021 IEEE 4th International Electrical and Energy Conference (CIEEC), Wuhan, China, 28–30 May 2021. [Google Scholar] [CrossRef]
- Dorraki, N.; Niayesh, K. An Experimental Study of Short-Circuit Current Making Operation of Air Medium-Voltage Load Break Switches. IEEE Trans. Power Deliv. 2022. [Google Scholar] [CrossRef]
- Elsayed, A.M.; Shaheen, A.M.; Alharthi, M.M.; Ghoneim, S.S.M.; El-Sehiemy, R.A. Adequate operation of hybrid AC/MT-HVDC power systems using an improved multi- objective marine predators optimizer. IEEE Access 2021, 9, 51065–51087. [Google Scholar] [CrossRef]
- IEC 60287-1-1:2006; Electric Cables-Calculation of the Current Rating-Part 1-1: Current Rating Equations (100% Load Factor) and Calculation of Losses-General. IEC: Geneva, Switzerland, 2006; pp. 1–136.
- IEC 60853-1:1985; Calculation of the Cyclic and Emergency Current Rating of Cables. Part 1: Cyclic Rating Factor for Cables up to and Including 18/30(36) kV. IEC: Geneva, Switzerland, 1985; pp. 1–39.
- IEC 60986:2000; Short-Circuit Temperature Limits of Electric Cables with Rated Voltages from 6 kV (Um = 7.2 kV) up to 30 kV (Um = 36 kV). IEC: Geneva, Switzerland, 2000; pp. 1–19.
- IEC TR 62095:2003; Electric Cables-Calculations for Current Ratings-Finite Element Method. IEC: Geneva, Switzerland, 2003; pp. 1–69.
- Callender, G.; Goddard, K.F.; Dix, J.; Lewin, P.L. A Flexible Model to Calculate Buried Cable Ampacity in Complex Environments. IEEE Trans. Power Deliv. 2021, 37, 2007–2015. [Google Scholar] [CrossRef]
- Liu, G.; Xu, Z.; Ma, H.; Hao, Y.; Wang, P.; Wu, W.; Xie, Y.; Guo, D. An improved analytical thermal rating method for cables installed in short-conduits. Int. J. Electr. Power Energy Syst. 2020, 123, 106223. [Google Scholar] [CrossRef]
- Bustamante, S.; Mínguez, R.; Arroyo, A.; Manana, M.; Laso, A.; Castro, P.; Martinez, R. Thermal behaviour of medium-voltage underground cables under high-load operating conditions. Appl. Therm. Eng. 2019, 156, 444–452. [Google Scholar] [CrossRef]
- Rasoulpoor, M.; Mirzaie, M.; Mirimani, S.M. Thermal assessment of sheathed medium voltage power cables under non-sinusoidal current and daily load cycle. Appl. Therm. Eng. 2017, 123, 353–364. [Google Scholar] [CrossRef]
- Abomailek, C.; Capelli, F.; Riba, J.-R.; Casals-Torrens, P. Transient thermal modelling of substation connectors by means of dimensionality reduction. Appl. Therm. Eng. 2017, 111, 562–572. [Google Scholar] [CrossRef]
- Abomailek, C.; Riba, J.-R.; Capelli, F.; Moreno-Eguilaz, M. Fast electro-thermal simulation of short-circuit tests. IET Gener. Transm. Distrib. 2017, 11, 2124–2129. [Google Scholar] [CrossRef]
- Neher, J.H.; McGrath, M.H. The Calculation of the Temperature Rise and Load Capability of Cable Systems. Trans. Am. Inst. Electr. Eng. Part III Power Appar. Syst. 1957, 76, 752–764. [Google Scholar] [CrossRef]
- Yenchek, M.R.; Cole, G.P. Thermal modeling of portable power cables. IEEE Trans. Ind. Appl. 1997, 33, 72–79. [Google Scholar] [CrossRef]
- Brito Filho, J.P. Heat transfer in bare and insulated electrical wires with linear temperature-dependent resistivity. Appl. Therm. Eng. 2017, 112, 881–887. [Google Scholar] [CrossRef]
- Shekhar, A.; Feng, X.; Hebner, R.; Gattozzi, A.; Strank, S.; Mor, A.; Ramirez-Elizondo, L.; Bauer, P. Thermal modelling and experimental validation for research on medium voltage DC cables. In Proceedings of the 2017 IEEE Power & Energy Society General Meeting, Chicago, IL, USA, 16–20 July 2017; pp. 1–5. [Google Scholar] [CrossRef]
- Echavarren, F.; Rouco, L.; Gonzalez, A. Dynamic thermal modeling of insulated cables. In Cigré Session 2012; Cigré: Paris, France, 2012; pp. 1–8. [Google Scholar]
- Yang, L.; Qiu, W.; Huang, J.; Hao, Y.; Fu, M.; Hou, S.; Li, L. Comparison of Conductor-Temperature Calculations Based on Different Radial-Position-Temperature Detections for High-Voltage Power Cable. Energies 2018, 11, 117. [Google Scholar] [CrossRef]
- Aras, F.; Biçen, Y. Thermal modelling and analysis of high-voltage insulated power cables under transient loads. Comput. Appl. Eng. Educ. 2013, 21, 516–529. [Google Scholar] [CrossRef]
- Tkachenko, V.A.; Kropotin, O.V.; Shepelev, A.O.; Kropotin, V.O. Mathematical model of cable power line with XLPE insulation with underground installation. Omsk Sci. Bull. 2018, 137–141. [Google Scholar] [CrossRef]
- Henke, A.; Frei, S. Transient Temperature Calculation in a Single Cable Using an Analytic Approach. J. Fluid Flow Heat Mass Transf. 2020, 7, 58–65. [Google Scholar] [CrossRef]
- Rezk, K.; Forsberg, J. A fast running numerical model based on the implementation of volume forces for prediction of pressure drop in a fin tube heat exchanger. Appl. Math. Model. 2014, 38, 5822–5835. [Google Scholar] [CrossRef]
- Wang, X.; Jiang, Y. Model reduction of discrete-time bilinear systems by a Laguerre expansion technique. Appl. Math. Model. 2016, 40, 6650–6662. [Google Scholar] [CrossRef]
- Cigré Working Group 22.12. Thermal Behaviour of Overhead Conductors; Cigré: Paris, France, 2002. [Google Scholar]
- IEEE Std 738-2012; IEEE Standard for Calculating the Current-Temperature of Bare Overhead Conductors. IEEE: New York, NY, USA, 2012.
- Riba, J.-R. Analysis of formulas to calculate the AC resistance of different conductors’ configurations. Electr. Power Syst. Res. 2015, 127, 93–100. [Google Scholar] [CrossRef] [Green Version]
- Banerjee, B. An evaluation of plastic flow stress models for the simulation of high-temperature and high-strain-rate deformation of metals. Acta Mater. 2005, 58, 6810–6827. [Google Scholar] [CrossRef]
- Lee, K.Y.; Yang, J.S.; Choi, Y.S.; Park, D.H. Specific heat and thermal conductivity measurement of XLPE insulator and semiconducting materials. In Proceedings of the 2006 IEEE 8th International Conference on Properties & Applications of Dielectric Materials, Bali, Indonesia, 26–30 June 2006; pp. 805–809. [Google Scholar] [CrossRef]
- Alford, S.; Dole, M. Specific Heat of Synthetic High Polymers. VI. A Study of the Glass Transition in Polyvinyl Chloride. J. Am. Chem. Soc. 1955, 77, 4774–4777. [Google Scholar] [CrossRef]
- Abu-Eishah, S.I. Correlations for the Thermal Conductivity of Metals as a Function of Temperature. Int. J. Thermophys. 2001, 22, 1855–1868. [Google Scholar] [CrossRef]
- Qi, X.; Boggs, S. Thermal and mechanical properties of EPR and XLPE cable compounds. IEEE Electr. Insul. Mag. 2006, 22, 19–24. [Google Scholar] [CrossRef]
- Kok, M.; Demirelli, K.; Aydogdu, Y. Thermophysical Properties of Blend of Poly (Vinyl Chloride) With Poly (Isobornyl Acrylate). Int. J. Sci. Technol. 2008, 3, 37–42. [Google Scholar]
- Datta, B.N. Numerical Linear Algebra and Applications, 2nd ed.; SIAM: Philadelphia, PA, USA, 2010; ISBN 0898717655. [Google Scholar]
- Li, X.J.; Yang, J.; Yan, B.Q.; Zheng, X. Insulated Cable Temperature Calculation and Numerical Simulation. MATEC Web Conf. 2018, 175, 03014. [Google Scholar] [CrossRef]
- Beňa, Ľ.; Gáll, V.; Kanálik, M.; Kolcun, M.; Margitová, A.; Mészáros, A.; Urbanský, J. Calculation of the overhead transmission line conductor temperature in real operating conditions. Electr. Eng. 2021, 103, 769–780. [Google Scholar] [CrossRef]
- Hong, S.S.; Yang, Y.C.; Hsu, T.S.; Tseng, K.S.; Hsu, Y.F.; Wu, Y.R.; Jiang, J.A. Internet of Things-Based Monitoring for HV Transmission Lines: Dynamic Thermal Rating Analysis with Microclimate Variables. In Proceedings of the 2020 8th International Electrical Engineering Congress (iEECON), Chiang Mai, Thailand, 4–6 March 2020. [Google Scholar] [CrossRef]
- ANSI/NEMA CC 1-2018; Electric Power Connection for Substation. ANSI: New York, NY, USA, 2009.
- IEC 60512-5-2:2002; Connectors for Electronic Equipment-Tests and Measurements-Part 5-2: Current-Carrying Capacity Tests-Test 5b: Current-Temperature Derating. IEC: Geneva, Switzerland, 2002; pp. 1–13.
- IEC 61238-1-1:2018; Compression and Mechanical Connectors for Power Cables-Part 1-1: Test Methods and Requirements for Compression and Mechanical Connectors for Power Cables for Rated Voltages up to 1 kV (Um = 1.2 kV) Tested on Non-Insulated Conductors. IEC: Geneva, Switzerland, 2018; pp. 1–89.




| Characteristic | Value |
|---|---|
| Designation | Barrinax U-1000 R2V |
| Rated voltage [kVRMS] | 0.6/1.0 |
| Max voltage [kVRMS] | 1.2 |
| Max continuous service temperature [°C] | 90 |
| Short circuit temperature [°C] | 250 |
| Inner insulation material | XLPE |
| Inner insulation wall thickness [mm] | 1.1 |
| Outer jacket material | PVC |
| Outer jacket wall thickness [mm] | 1.5 |
| Effective copper cross section [mm2] | 70 |
| Outer conductor diameter [mm] | 9.5 |
| Copper resistivity 20 °C [Ohm·m] | 1.85 × 10−8 |
| Temperature coefficient of resistivity [K−1] | 0.0043 |
| Number of strands [-] | 14 |
| Per unit length mass of the conductor [kg/m] | 0.584 |
| Ambient temperature [°C] | 19 |
| Step | Current (ARMS) | Duration (s) |
|---|---|---|
| #1 | 120 | 1950 |
| #2 | 145 | 1950 |
| #3 | 170 | 1500 |
| #4 | 185 | 1850 |
| #5 | 212 | 1350 |
| Characteristic | Value |
|---|---|
| Designation | H07RN-F TITANEX 1 × 150 |
| Rated voltage [kVRMS] | 0.6/1.0 |
| Max voltage [kVRMS] | 1.2 |
| Max continuous service temperature [°C] | 90 |
| Short circuit temperature [°C] | 250 |
| Inner insulation wall thickness [mm] | 2.0 |
| Outer jacket wall thickness [mm] | 2.5 |
| Effective copper cross section [mm2] | 150 |
| Outer conductor diameter [mm] | 15 |
| Copper resistivity 20 °C [Ohm·m] | 1.85 × 10−8 |
| Flexibility class | 5 |
| Per unit length mass of the conductor [kg/m] | 1.74 |
| Ambient temperature [°C] | 23.5 |
| Step | Current (ARMS) | Duration (s) |
|---|---|---|
| #1 | 130 | 766 |
| #2 | 163 | 554 |
| #3 | 220 | 850 |
| #4 | 305 | 530 |
| #5 | 405 | 400 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Riba, J.-R.; Llauradó, J. A Model to Calculate the Current–Temperature Relationship of Insulated and Jacketed Cables. Materials 2022, 15, 6814. https://doi.org/10.3390/ma15196814
Riba J-R, Llauradó J. A Model to Calculate the Current–Temperature Relationship of Insulated and Jacketed Cables. Materials. 2022; 15(19):6814. https://doi.org/10.3390/ma15196814
Chicago/Turabian StyleRiba, Jordi-Roger, and Jordi Llauradó. 2022. "A Model to Calculate the Current–Temperature Relationship of Insulated and Jacketed Cables" Materials 15, no. 19: 6814. https://doi.org/10.3390/ma15196814
APA StyleRiba, J.-R., & Llauradó, J. (2022). A Model to Calculate the Current–Temperature Relationship of Insulated and Jacketed Cables. Materials, 15(19), 6814. https://doi.org/10.3390/ma15196814







