Numerical Simulation of Failure Behavior of Brittle Heterogeneous Rock under Uniaxial Compression Test
Abstract
1. Introduction
2. Materials and Methods
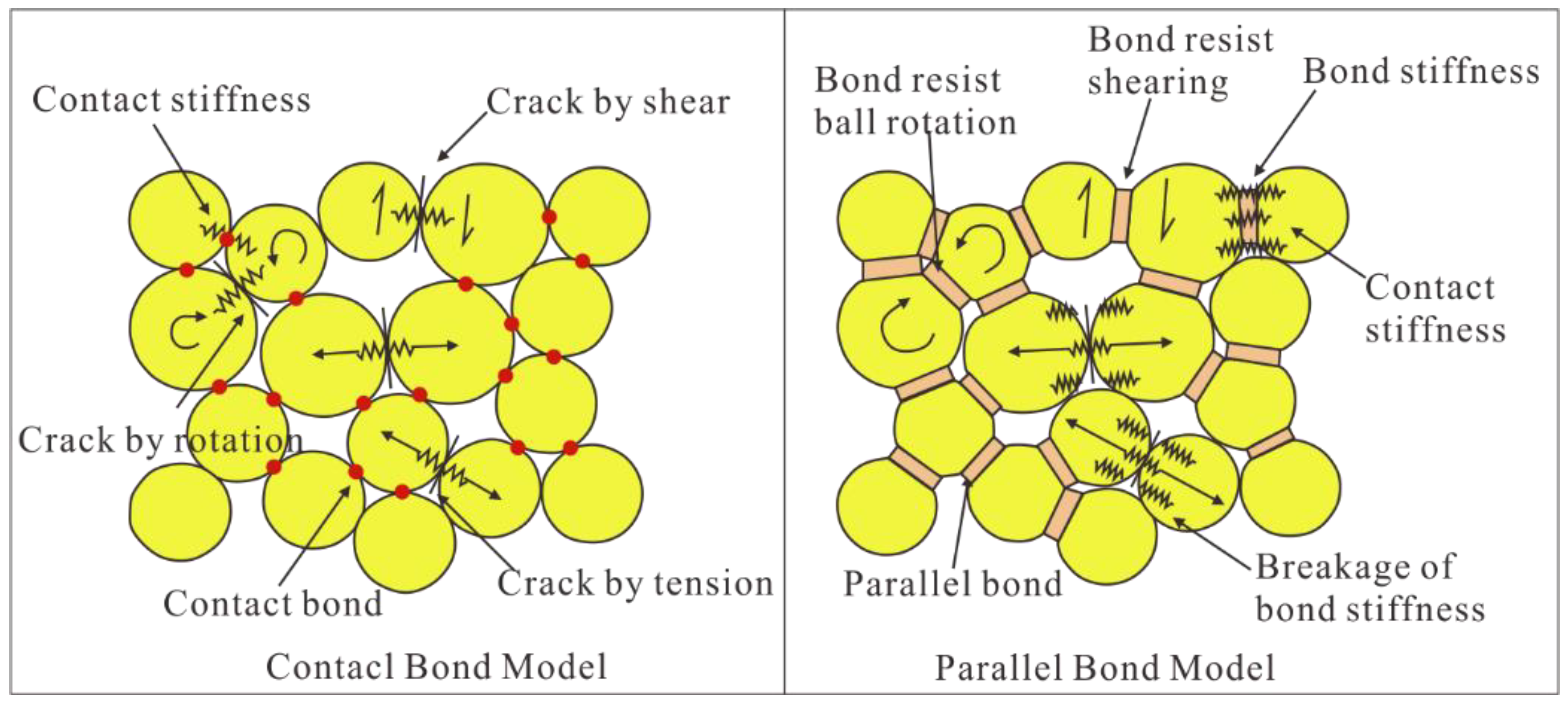
2.1. Brief Description of PFC2D
2.2. Calibration of Micro-Parameters
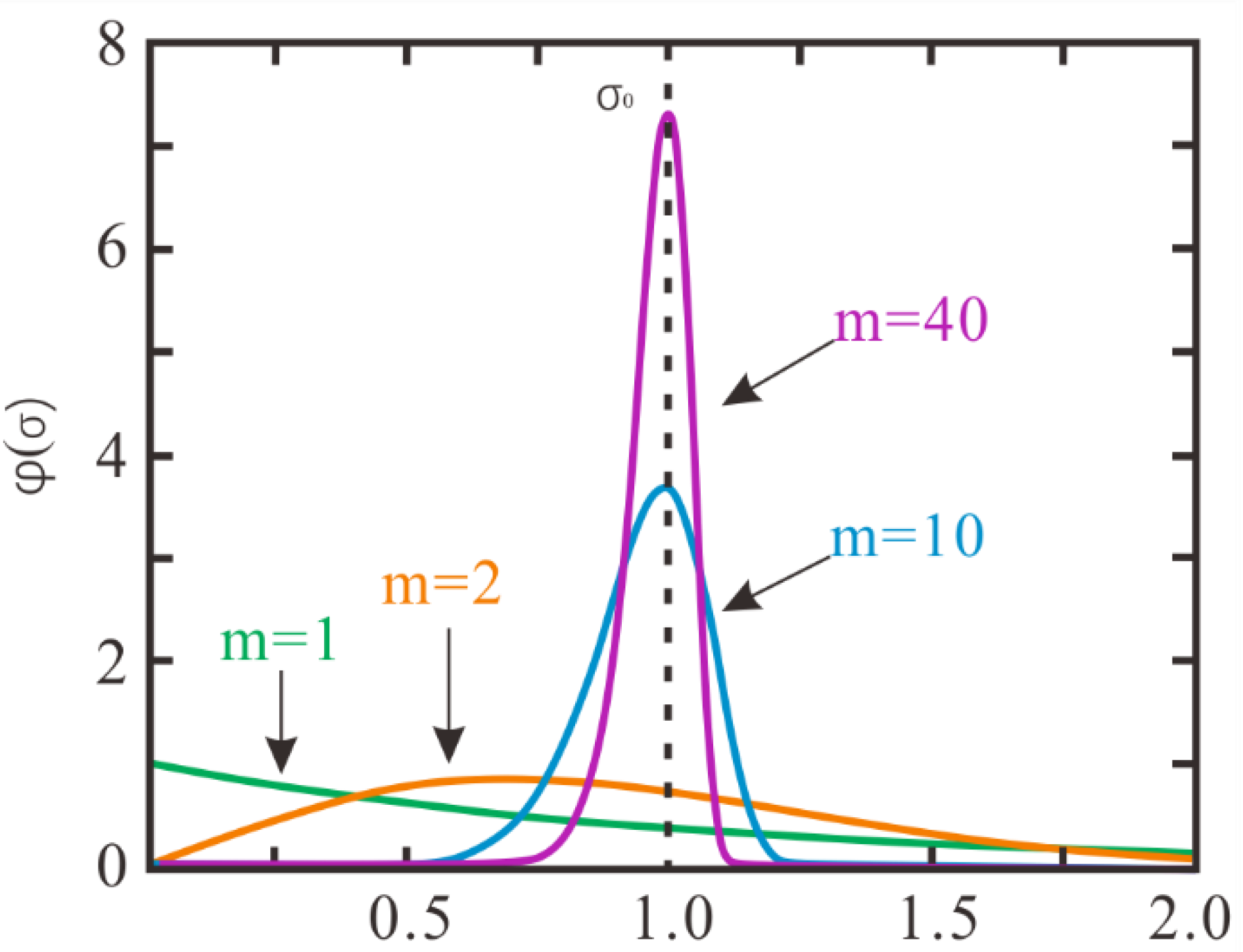
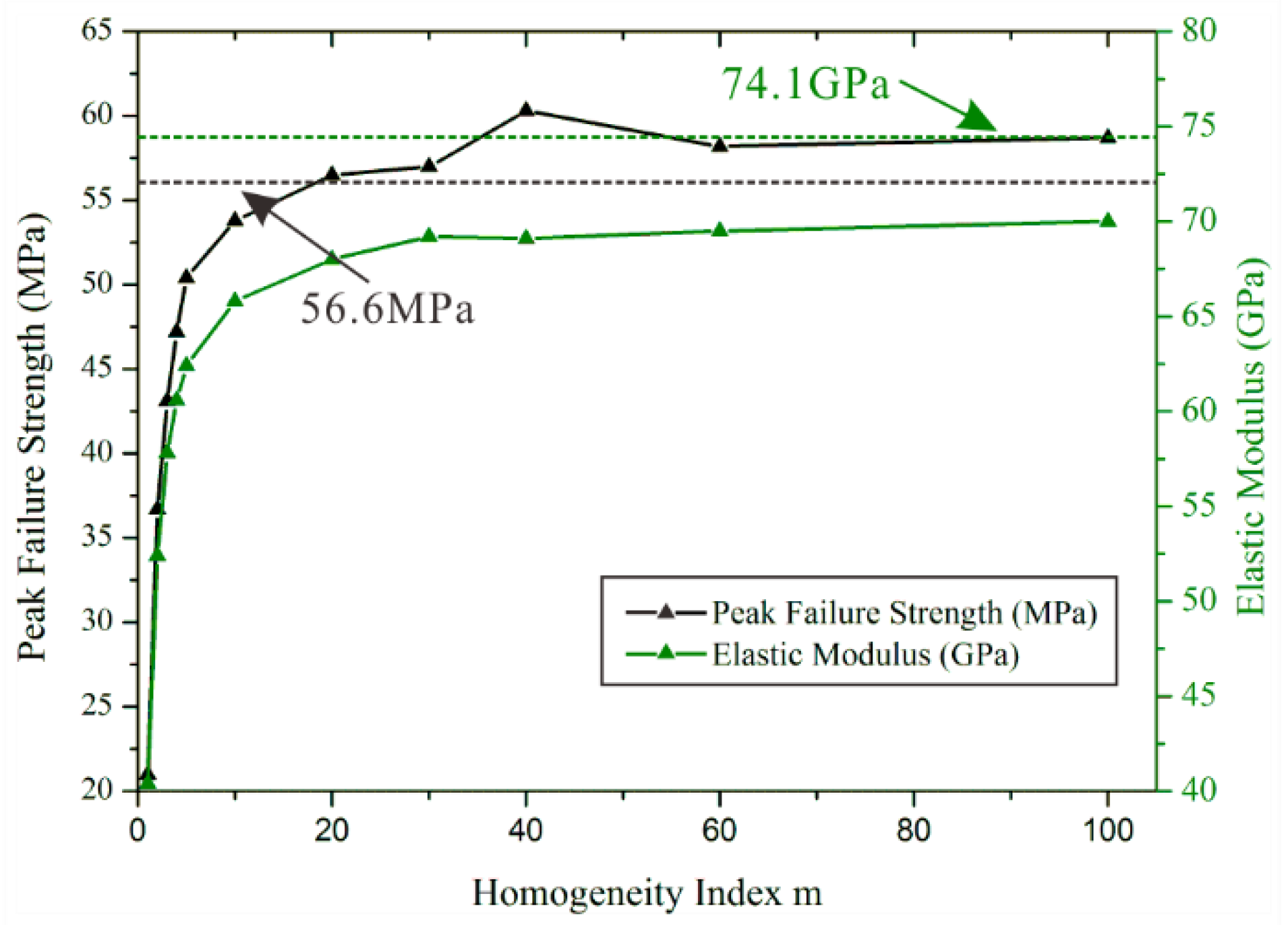
2.3. Achieving Heterogeneity with Weibull Distribution
3. The Optimal Combination of Micro-Parameters
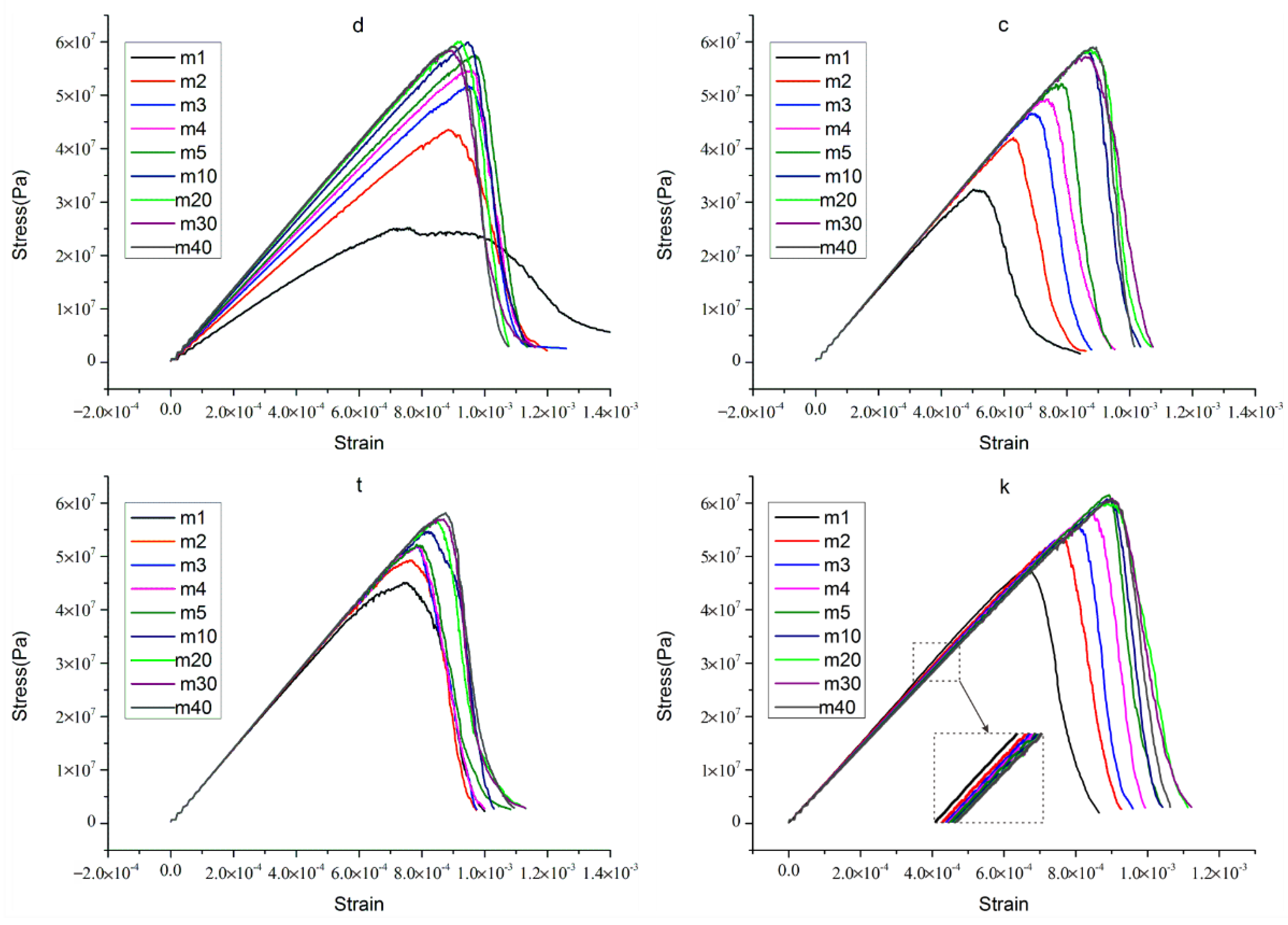
3.1. Build Different Micro-Parameter Combinations
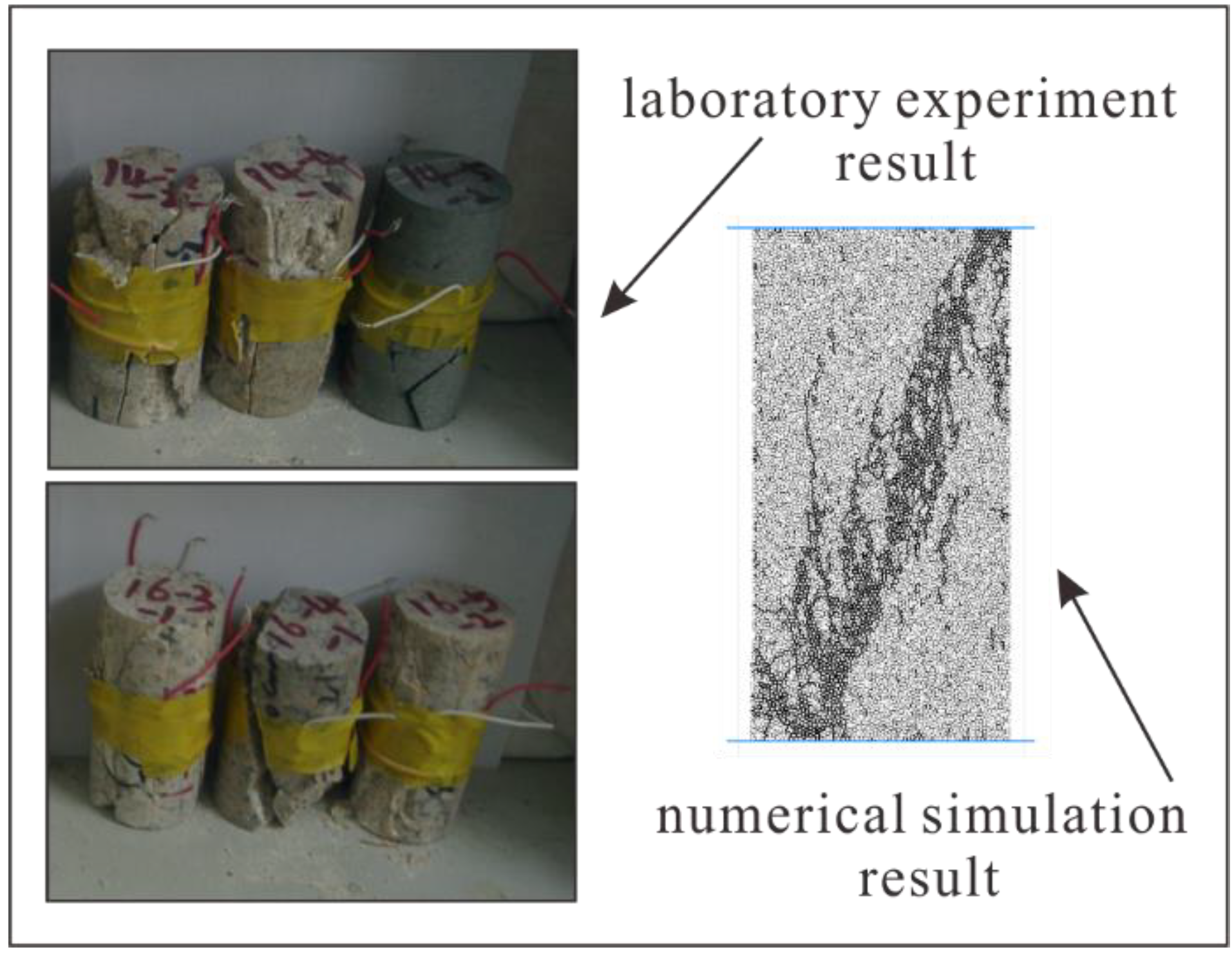
3.2. The Uniaxial Compressive Test Results
4. Numerical Simulation Result of Uniaxial Compression Test
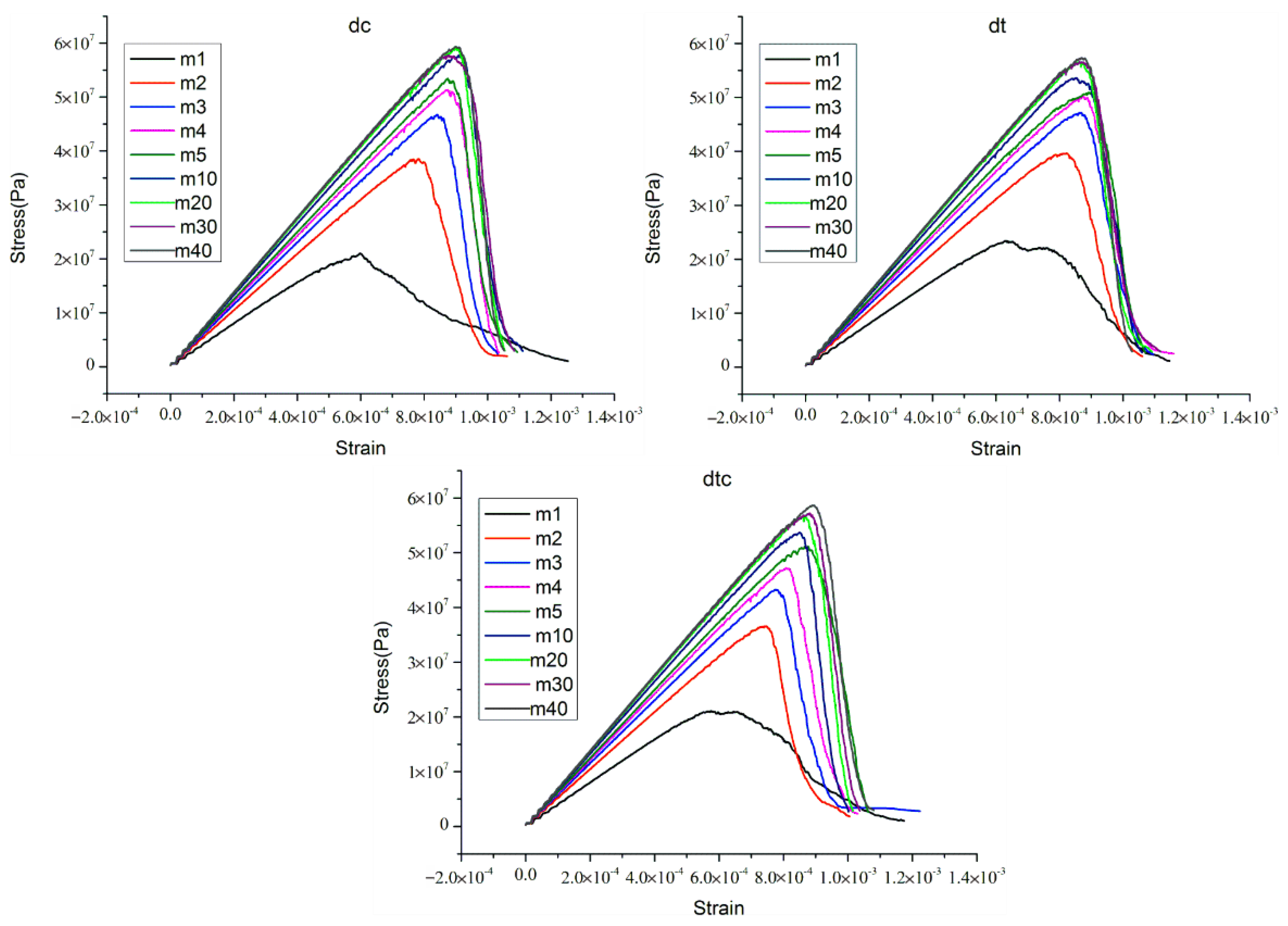
4.1. Stress-Strain Curve Results
4.2. Failure Development of Heterogeneous Rock Specimen
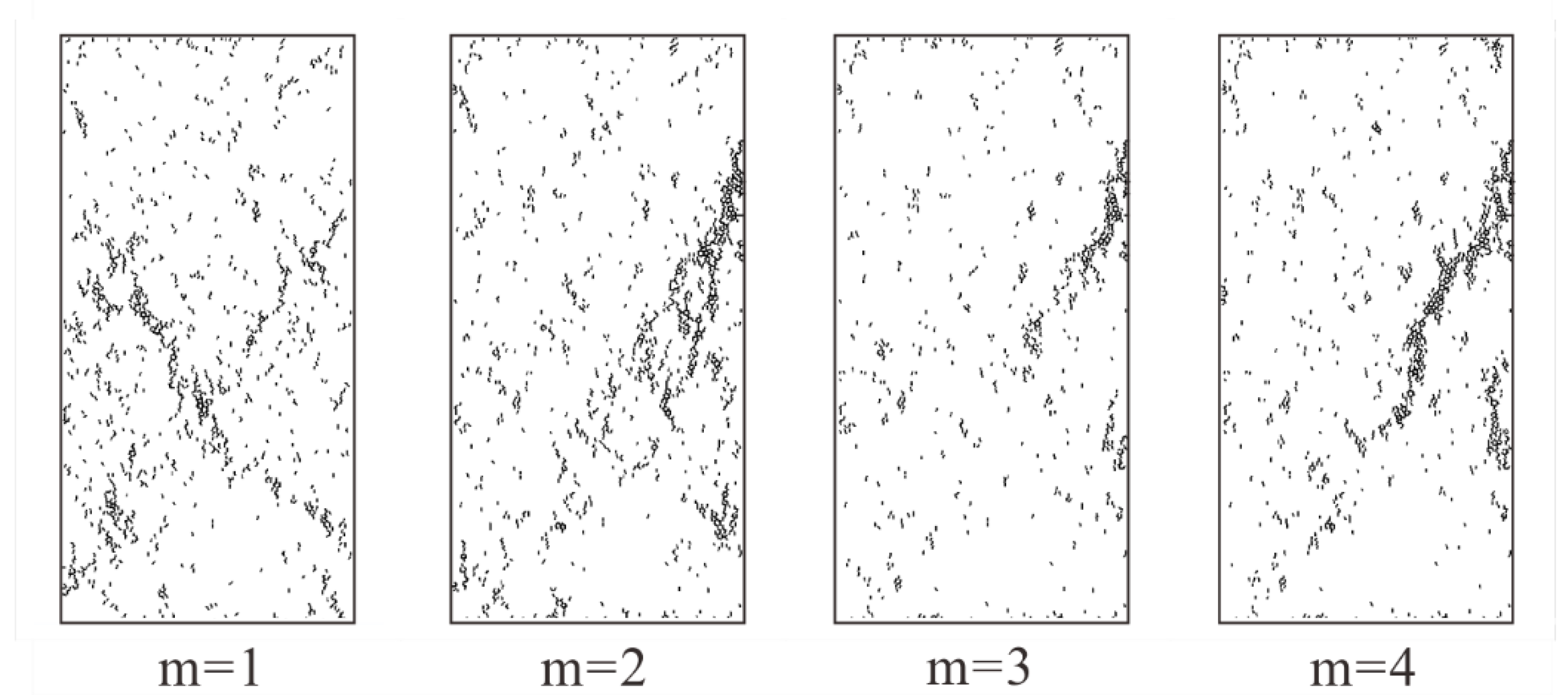
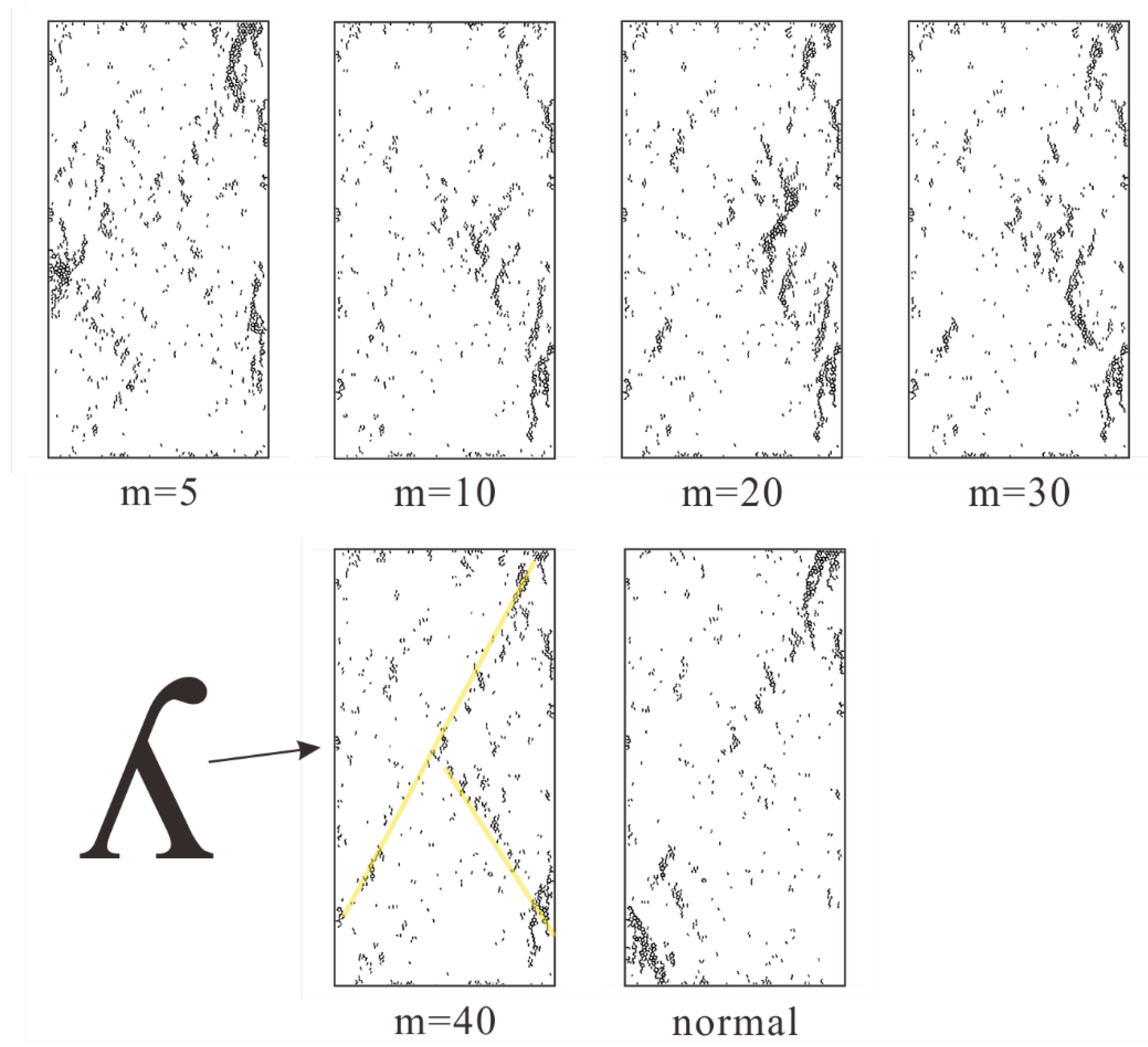
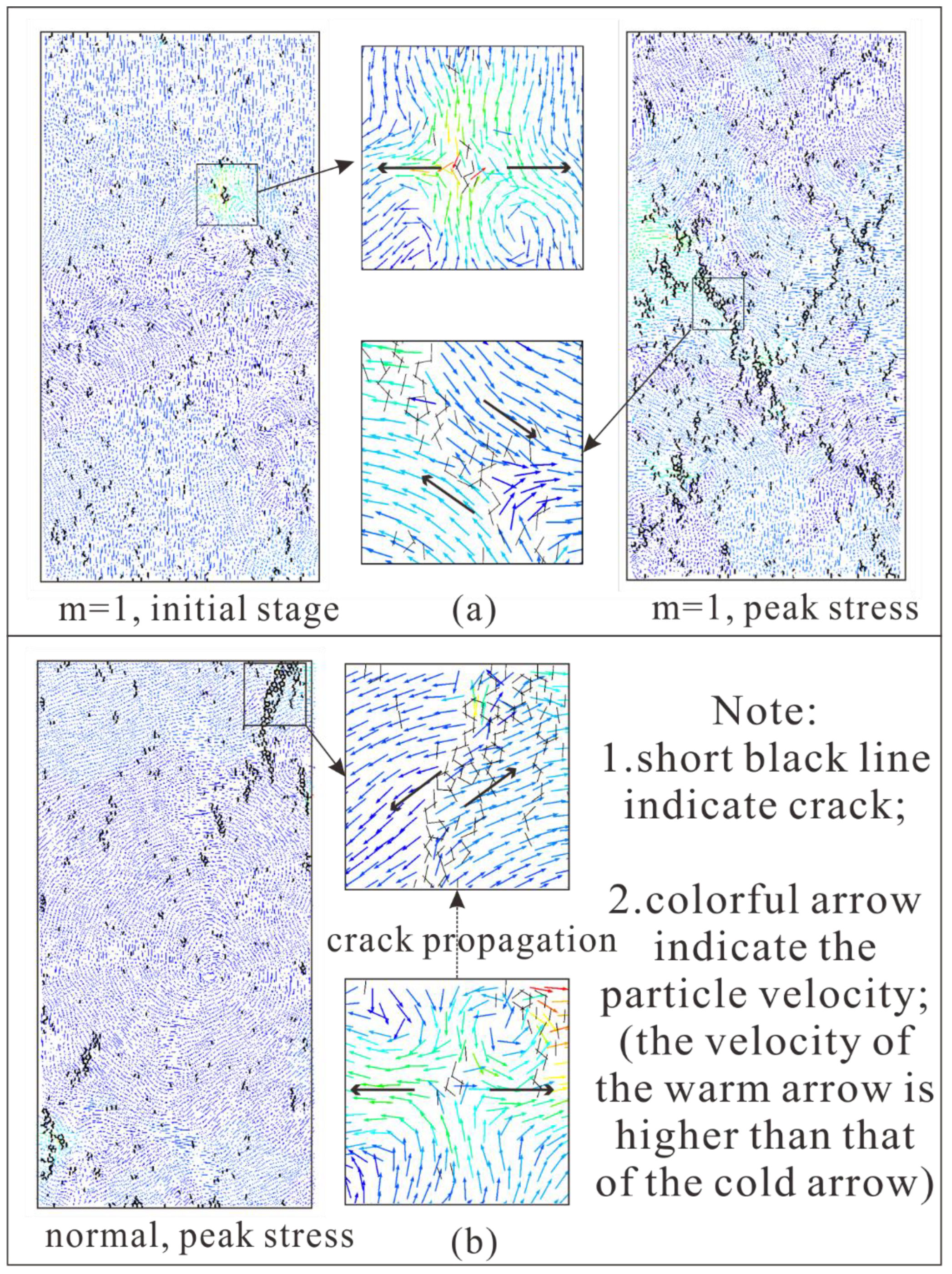
4.2.1. The Crack Distribution of Specimen under Peak Stress
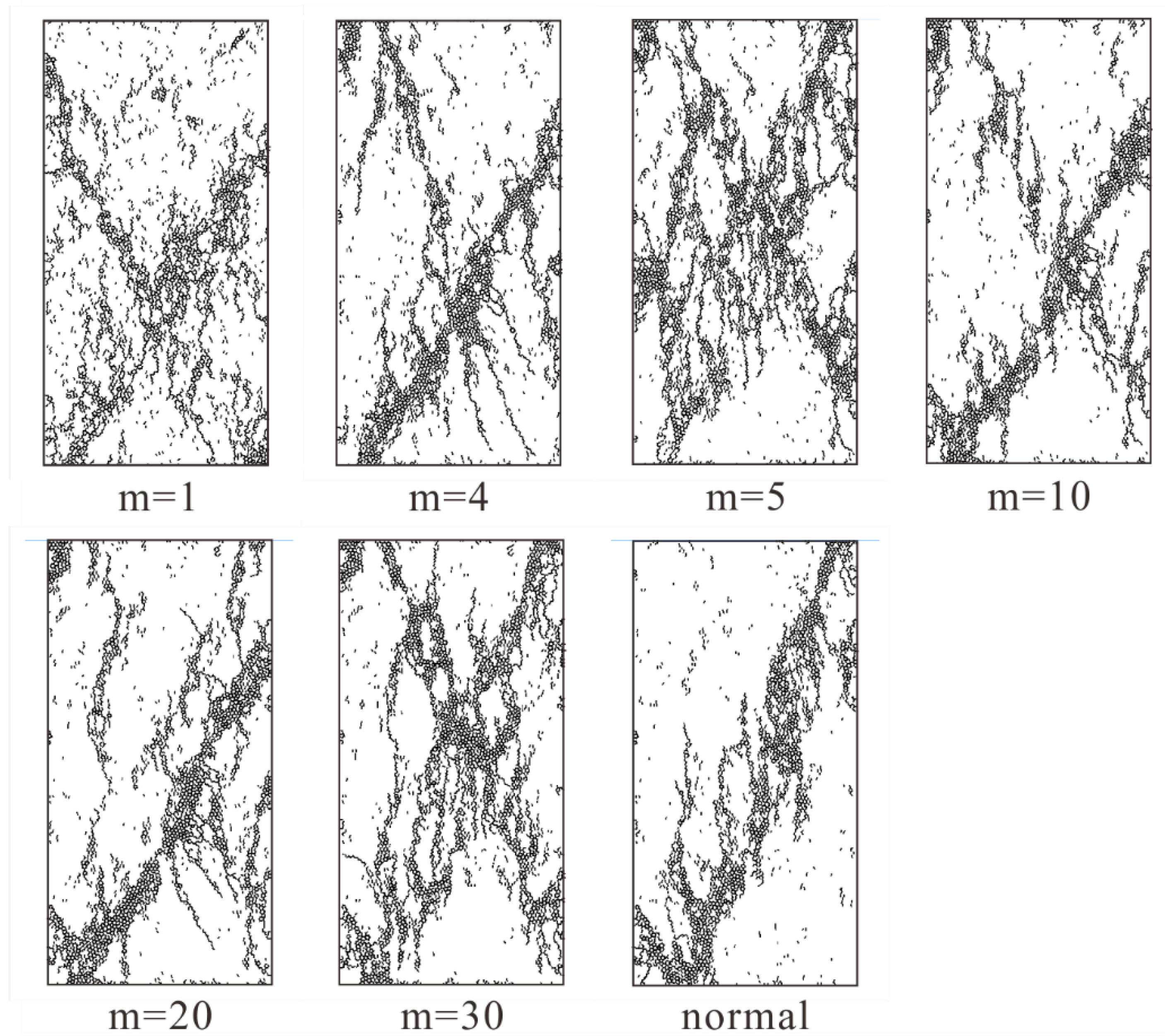
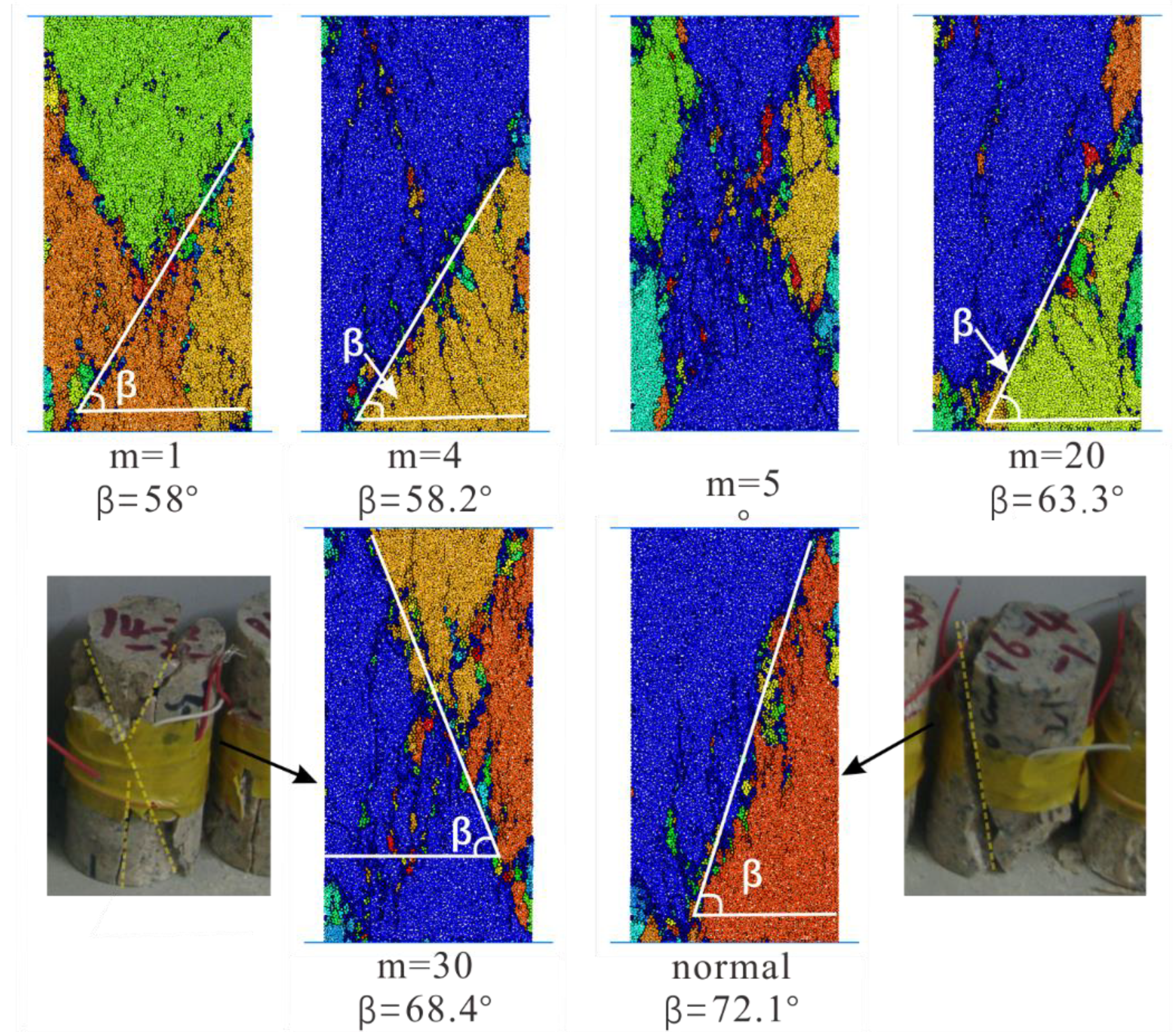
4.2.2. The Failure Mode
5. Discussion
5.1. The Forming and Evolution of Cracks
5.2. The Stress-Strain Curve
5.3. Expand the Application
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kranz, R.L. Microcracks in rocks—A review. Tectonophysics 1983, 100, 449–480. [Google Scholar] [CrossRef]
- Hamdi, E.; Lafhaj, Z. Microcracking based rock classification using ultrasonic and porosity-parameters and multivariate analysis methods. Eng. Geol. 2013, 167, 27–36. [Google Scholar] [CrossRef]
- Griffith, A.A. The Phenomena of Rupture and Flow in Solids. Philos. Trans. R. Soc. Lond. 1920, 221, 163–198. [Google Scholar]
- Åkesson, U.; Lindqvist, J.E.; Schouenborg, B.; Grelk, B. Relationship between microstructure and bowing properties of calcite marble claddings. Bull. Eng. Geol. Environ. 2006, 65, 73–79. [Google Scholar] [CrossRef]
- Zhang, X.P.; Wong, L.N.Y. Cracking Processes in Rock-Like Material Containing a Single Flaw Under Uniaxial Compression: A Numerical Study Based on Parallel Bonded-Particle Model Approach. Rock Mech. Rock Eng. 2012, 45, 711–737. [Google Scholar] [CrossRef]
- Debecker, B.; Vervoort, A. Two-dimensional discrete element simulations of the fracture behaviour of slate. Int. J. Rock Mech. Min. Sci. 2013, 61, 161–170. [Google Scholar] [CrossRef]
- Tang, C.A.; Liu, H.; Lee, P.K.K.; Tsui, Y.; Tham, L.G. Numerical studies of the influence of microstructure on rock failure in uniaxial compression—Part I: Effect of heterogeneity. Int. J. Rock Mech. Min. Sci. 2000, 37, 555–569. [Google Scholar] [CrossRef]
- Fan, W.C.; Yang, H.; Jiang, X.L.; Cao, P. Experimental and numerical investigation on crack mechanism of folded flawed rock-like material under uniaxial compression. Eng. Geol. 2021, 291, 106210. [Google Scholar] [CrossRef]
- Saadat, M.; Taheri, A. Modelling Microcracking Behaviour of Precracked Granite Using Grain Based Distinct Element Model. Rock Mech. Rock Eng. 2019, 52, 4669–4692. [Google Scholar] [CrossRef]
- Chmel, A.; Shcherbakov, I. A comparative acoustic emission study of compression and impact fracture in granite. Int. J. Rock Mech. Min. Sci. 2013, 64, 56–59. [Google Scholar] [CrossRef]
- Meng, F.Z.; Song, J.; Yue, Z.F.; Zhou, H.; Wang, X.S.; Wang, Z.Q. Failure mechanisms and damage evolution of hard rock joints under high stress: Insights from PFC2D modeling. Eng. Anal. Bound. Elem. 2022, 135, 394–411. [Google Scholar] [CrossRef]
- Zhai, T.Q.; Zhu, J.B.; Zhou, C.T.; Yang, T. Experimental investigation of the effect of thermal treatment on shear characteristics of healed rock joints. Int. J. Rock Mech. Min. Sci. 2022, 152, 105074. [Google Scholar] [CrossRef]
- Lindquist, W.B.; Venkatarangan, A. Pore and throat size distributions measured from synchrotron X-ray tomographic images of Fontainebleau sandstones. J. Geophys. Res. 2000, 105, 21509–21527. [Google Scholar] [CrossRef]
- Yun, T.S.; Jeong, Y.J.; Kim, K.Y.; Min, K.B. Evaluation of rock anisotropy using 3D X-ray computed tomography. Eng. Geol. 2013, 163, 11–19. [Google Scholar] [CrossRef]
- Zou, C.J.; Wong, L.N.Y. Experimental studies on cracking processes and failure in marble under dynamic loading. Eng. Geol. 2014, 173, 19–31. [Google Scholar] [CrossRef]
- Fan, X.; Kulatilake, P.H.S.W.; Chen, X. Mechanical behavior of rock-like jointed blocks with multi-non-persistent joints under uniaxial loading: A particle mechanics approach. Eng. Geol. 2015, 190, 17–32. [Google Scholar] [CrossRef]
- Saharan, M.R.; Mitri, H.S. Numerical Procedure for Dynamic Simulation of Discrete Fractures Due to Blasting. Rock Mech. Rock Eng. 2008, 41, 641–670. [Google Scholar] [CrossRef]
- Li, G.; Ma, F.S.; Guo, J.; Zhao, H.J.; Liu, G. Study on deformation failure mechanism and support technology of deep soft rock roadway. Eng. Geol. 2020, 264, 105262. [Google Scholar] [CrossRef]
- Blair, S.C.; Cook, N.G.W. Analysis of Compressive Fracture in Rock Using Statistical Techniques: Part I. A Non-linear Rule-based Model. Int. J. Rock Mech. Min. Sci. 1998, 35, 837–848. [Google Scholar] [CrossRef]
- Zhang, Y.Z.; Zhong, W.L.; Yao, J.G. Dislocation theory solution and boundary element calculation of rock strata movement. J. China Coal Soc. 1987, 2, 21–31. [Google Scholar]
- Darvishi, A.; Ataei, M.; Rafiee, R. Investigating the effect of simultaneous extraction of two longwall panels on a maingate gateroad stability using numerical modeling. Int. J. Rock Mech. Min. Sci. 2020, 126, 104172. [Google Scholar] [CrossRef]
- Tian, Z.N.; Li, S.H.; Liu, X.Y.; Liu, B.S. Research on deformable calculation method based on three-dimensional block discrete element. Chin. J. Rock Mech. Eng. 2008, 27, 2832–2840. [Google Scholar]
- Jing, L. Formulation of discontinuous deformation analysis (DDA)an implicit discrete element model for block systems. Eng. Geol. 1998, 49, 371–381. [Google Scholar] [CrossRef]
- Jiang, M.J.; Yan, H.B.; Zhu, H.H.; Utili, S. Modeling shear behavior and strain localization in cemented sands by two-dimensional distinct element method analyses. Comput. Geotech. 2011, 38, 14–29. [Google Scholar] [CrossRef]
- Behbahani, S.S.; Moarefvand, P.; Ahangari, K.; Goshtasbi, K. Unloading scheme of Angooran mine slope by discrete-element modeling. Int. J. Rock Mech. Min. Sci. 2013, 64, 220–227. [Google Scholar] [CrossRef]
- Yang, S.Q.; Huang, Y.H.; Jing, H.W.; Liu, X.R. Discrete element modeling on fracture coalescence behavior of red sandstone containing two unparallel fissures under uniaxial compression. Eng. Geol. 2014, 178, 28–48. [Google Scholar] [CrossRef]
- Tang, P.; Chen, G.Q.; Huang, R.Q.; Zhou, J. Brittle failure of rockslides linked to the rock bridge length effect. Landslides 2020, 17, 793–803. [Google Scholar] [CrossRef]
- Cao, W.G.; Fang, Z.L.; Tang, X.J. Research on Statistical Constitutive Model of Rock Damage Softening. Chin. J. Rock Mech. Eng. 1998, 17, 628–633. [Google Scholar]
- Liu, H.Y.; Roquete, M.; Kou, S.Q.; Lindqvist, P.A. Characterization of rock heterogeneity and numerical verification. Eng. Geol. 2004, 72, 89–119. [Google Scholar] [CrossRef]
- Li, H.; Yang, C.H.; Ding, X.L.; William, N.T.; Yin, H.W.; Zhang, S.N. Weibull linear parallel bond model (WLPBM) for simulating micro-mechanical characteristics of heterogeneous rocks. Eng. Anal. Bound. Elem. 2019, 108, 82–94. [Google Scholar] [CrossRef]
- Potyondy, D.O.; Cundall, P.A. A bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2004, 41, 1329–1364. [Google Scholar] [CrossRef]
- Potyondy, D.O. Simulating stress corrosion with a bonded-particle model for rock. Int. J. Rock Mech. Min. Sci. 2007, 44, 677–691. [Google Scholar] [CrossRef]
- PFC Manual; Version 5.0; Itasca Consulting Group Inc.: Minneapolis, MN, USA, 2018.
- Zhang, Y.L.; Shao, J.F.; Saxcéb, G.D.; Shia, C.; Liu, Z.B. Study of deformation and failure in an anisotropic rock with a three dimensional discrete element model. Int. J. Rock Mech. Min. Sci. 2019, 120, 17–28. [Google Scholar] [CrossRef]
- Cho, N.; Martin, C.D.; Sego, D.C. A clumped particle model for rock. Int. J. Rock Mech. Min. Sci. 2007, 44, 997–1010. [Google Scholar] [CrossRef]
- Shi, C.; Yang, W.K.; Yang, J.X.; Chen, X. Calibration of microscaled mechanical parameters of granite based on a bondedparticle model with 2D particle flow code. Granul. Matter 2019, 21, 38. [Google Scholar] [CrossRef]
- Yoon, J. Application of experimental design and optimization to PFC model calibration in uniaxial compression simulation. Int. J. Rock Mech. Min. Sci. 2007, 44, 871–889. [Google Scholar] [CrossRef]
- Zhao, G.Y.; Dai, B.; Ma, C. Effect of mesoscopic parameters on macroscopic properties in parallel bonding model. Chin. J. Rock Mech. Eng. 2012, 31, 1491–1498. [Google Scholar]
- Yang, B.D.; Jiao, Y.; Lei, S.T. A study on the effects of microparameters on macroproperties for specimens created by bonded particles. Eng. Comput. 2006, 23, 607–631. [Google Scholar] [CrossRef]
- Li, H.; Yang, J.; Han, Y.; Yang, C.H.; Daemen, J.J.K.; Li, P. Weibull grain-based model (W-GBM) for simulating heterogeneous mechanical characteristics of salt rock. Eng. Anal. Bound. Elem. 2019, 108, 227–243. [Google Scholar] [CrossRef]
- Weibull, W. A Statistical Distribution Function of Wide Applicability. J. Appl. Mech. 1951, 13, 293–297. [Google Scholar] [CrossRef]
- Li, X.; Huai, Z.; Konietzky, H.; Li, X.B.; Wang, Y. A numerical study of brittle failure in rocks with distinct microcrack characteristics. Int. J. Rock Mech. Min. Sci. 2018, 106, 289–299. [Google Scholar] [CrossRef]
- He, S.J. Calibration Method and Sensitivity Analysis of Micromechanic Parameters for Limestone Based on Particle Flow. Master’s Thesis, Shandong University, Jinan, China, 2018. [Google Scholar]
- Xu, Z.H.; Wang, Z.Y.; Wang, W.Y.; Lin, P.; Wu, J. An integrated parameter calibration method and sensitivity analysis of microparameters on mechanical behavior of transversely isotropic rocks. Comput. Geotech. 2022, 142, 104573. [Google Scholar] [CrossRef]
- Xu, X.M.; Ling, D.S.; Chen, Y.M.; Huang, B. Correlation of microscopic and macroscopic elastic constants of granular materials based on linear contact model. Chin. J. Geotech. Eng. 2010, 32, 991–998. [Google Scholar]
- Su, H.; Dang, C.H.; Li, Y.J. Study of numerical simulation of acoustic emission in rock of inhomogeneity. Rock Soil Mech. 2011, 32, 1886–1890. [Google Scholar]
- Xu, Z.H.; Wang, W.Y.; Lin, P.; Xiong, Y.; Liu, Z.Y.; He, S.J. A parameter calibration method for PFC simulation: Development and a case study of limestone. Geomech. Eng. 2020, 22, 97–108. [Google Scholar]
- Coulomb, C.A. Sur une application des regles de maximus et minimus a quelques problemes de statique relatifs a l’architecture. Acad. R. Sci. Mem. Math. Phys. Divers. Savans 1773, 7, 343–382. [Google Scholar]
- Wang, X.B. Numerical simulation of failure processes and acoustic emissions of rock specimens with different strengths. Chin. J. Eng. 2008, 30, 837–843. [Google Scholar]
- Zhang, D.C.; Ranjith, P.G.; Perera, M.S.A. The brittleness indices used in rock mechanics and their application in shale hydraulic.fracturing: A review. J. Pet. Sci. Eng. 2016, 143, 158–170. [Google Scholar] [CrossRef]
- Zhou, H.; Chen, J.; Lu, J.J.; Jiang, Y.; Meng, F.Z. A New Rock Brittleness Evaluation Index Based on the Internal Friction Angle and Class I Stress–Strain Curve. Rock Mech. Rock Eng. 2018, 51, 2309–2316. [Google Scholar] [CrossRef]
- Tutluoğlu, L.; Öge, İ.F.; Karpuz, C. Relationship Between Pre-failure and Post-failure Mechanical Properties of Rock Material of Different Origin. Rock Mech. Rock Eng. 2015, 48, 121–141. [Google Scholar] [CrossRef]




| Micro-Parameters | Value |
|---|---|
| Minimum limit value of radius () (mm) | 0.3 |
| Ratio of upper and lower limits of radius (/) | 1.67 |
| Porosity, φ | 0.1 |
| Density | 2580 |
| Young’s modulus of the particle, (GPa) | 36 |
| Ratio of normal to shear stiffness of the particle, / | 1.5 |
| Particle friction coefficient (μ) | 0.58 |
| Young’s modulus of the parallel bond, (GPa) | 36 |
| Ratio of normal to shear stiffness of the parallel bond,/ | 1.5 |
| Parallel-bond tensile strength (), (MPa) | 15 |
| Parallel-bond cohension (), (MPa) | 28 |
| Parallel-bond friction angle () (°) | 30 |
| Laboratory Experiment Results | Numerical Simulation Results | |
|---|---|---|
| Density, ρ (g/cm3) | 2663 | 2580 |
| Yong’s modulus, E (GPa) | 77.56 | 74.1 |
| Uniaxial compressive strength, UCS (MPa) | 56.74 | 56.6 |
| Poisson’s ratio, ν | 0.2 | 0.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Ma, F.; Guo, J.; Zhou, T.; Song, Y.; Li, F. Numerical Simulation of Failure Behavior of Brittle Heterogeneous Rock under Uniaxial Compression Test. Materials 2022, 15, 7035. https://doi.org/10.3390/ma15197035
Liu J, Ma F, Guo J, Zhou T, Song Y, Li F. Numerical Simulation of Failure Behavior of Brittle Heterogeneous Rock under Uniaxial Compression Test. Materials. 2022; 15(19):7035. https://doi.org/10.3390/ma15197035
Chicago/Turabian StyleLiu, Jia, Fengshan Ma, Jie Guo, Tongtong Zhou, Yewei Song, and Fangrui Li. 2022. "Numerical Simulation of Failure Behavior of Brittle Heterogeneous Rock under Uniaxial Compression Test" Materials 15, no. 19: 7035. https://doi.org/10.3390/ma15197035
APA StyleLiu, J., Ma, F., Guo, J., Zhou, T., Song, Y., & Li, F. (2022). Numerical Simulation of Failure Behavior of Brittle Heterogeneous Rock under Uniaxial Compression Test. Materials, 15(19), 7035. https://doi.org/10.3390/ma15197035





