Selected Shear Models Based on the Analysis of the Critical Shear Crack for Slender Concrete Beams without Shear Reinforcement
Abstract
:1. Introduction
2. Overview of Selected Theoretical Models
2.1. Muttoni and Ruiz 2008 [11]
2.2. Zhang et al., 2014 [12]
2.3. Yang 2014 [13]
2.4. Cladera et al., 2016 [14]
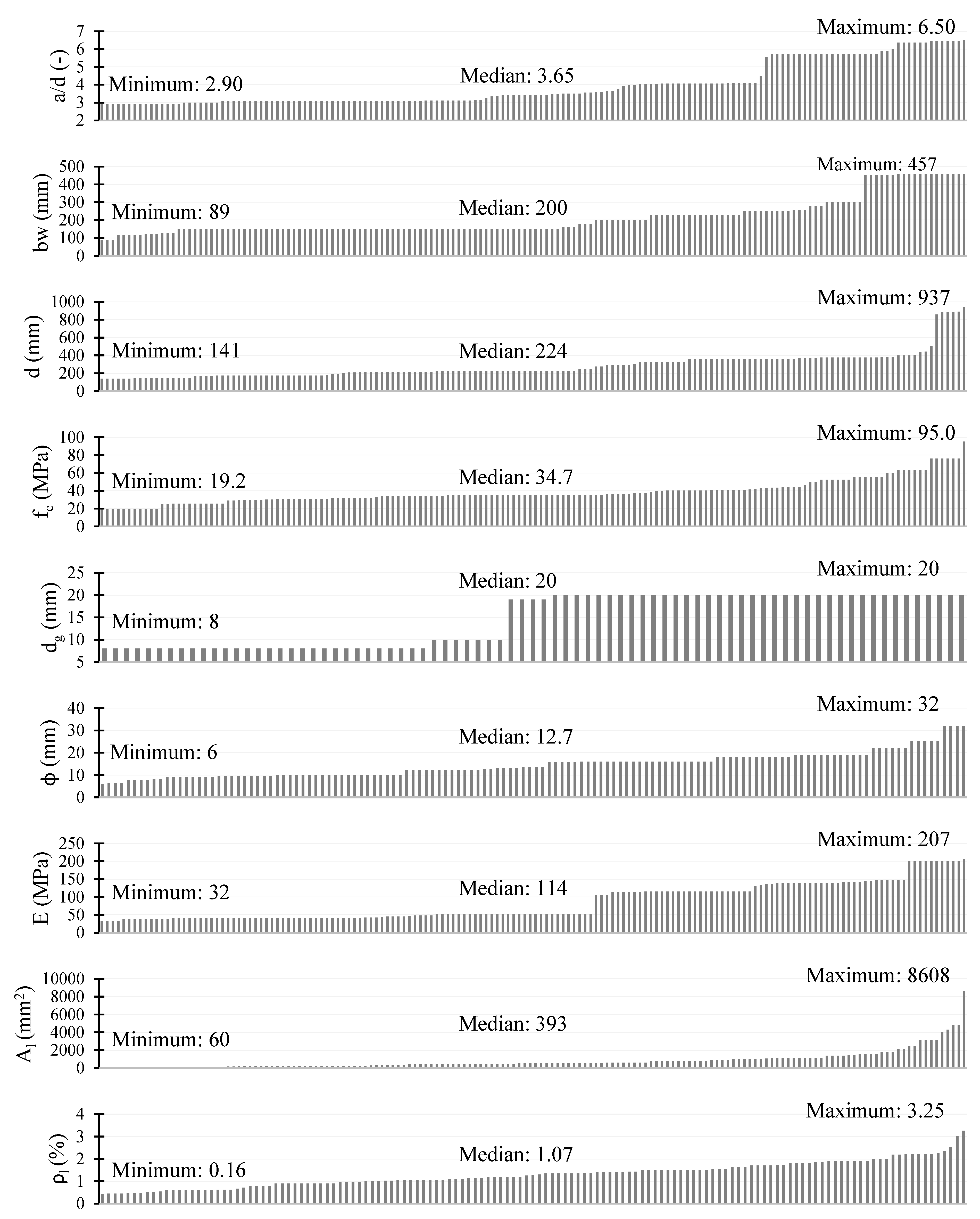
3. Test Database
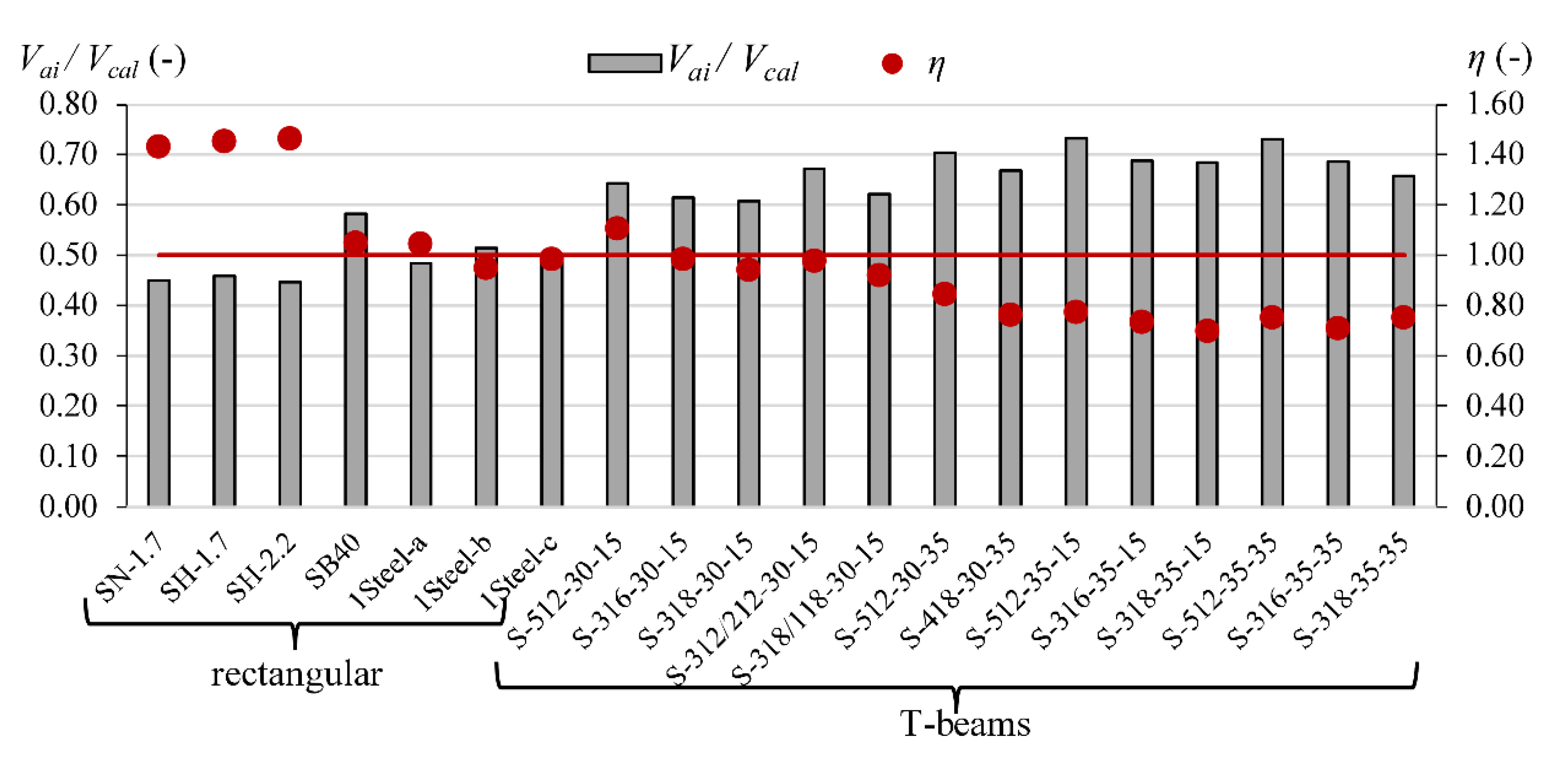
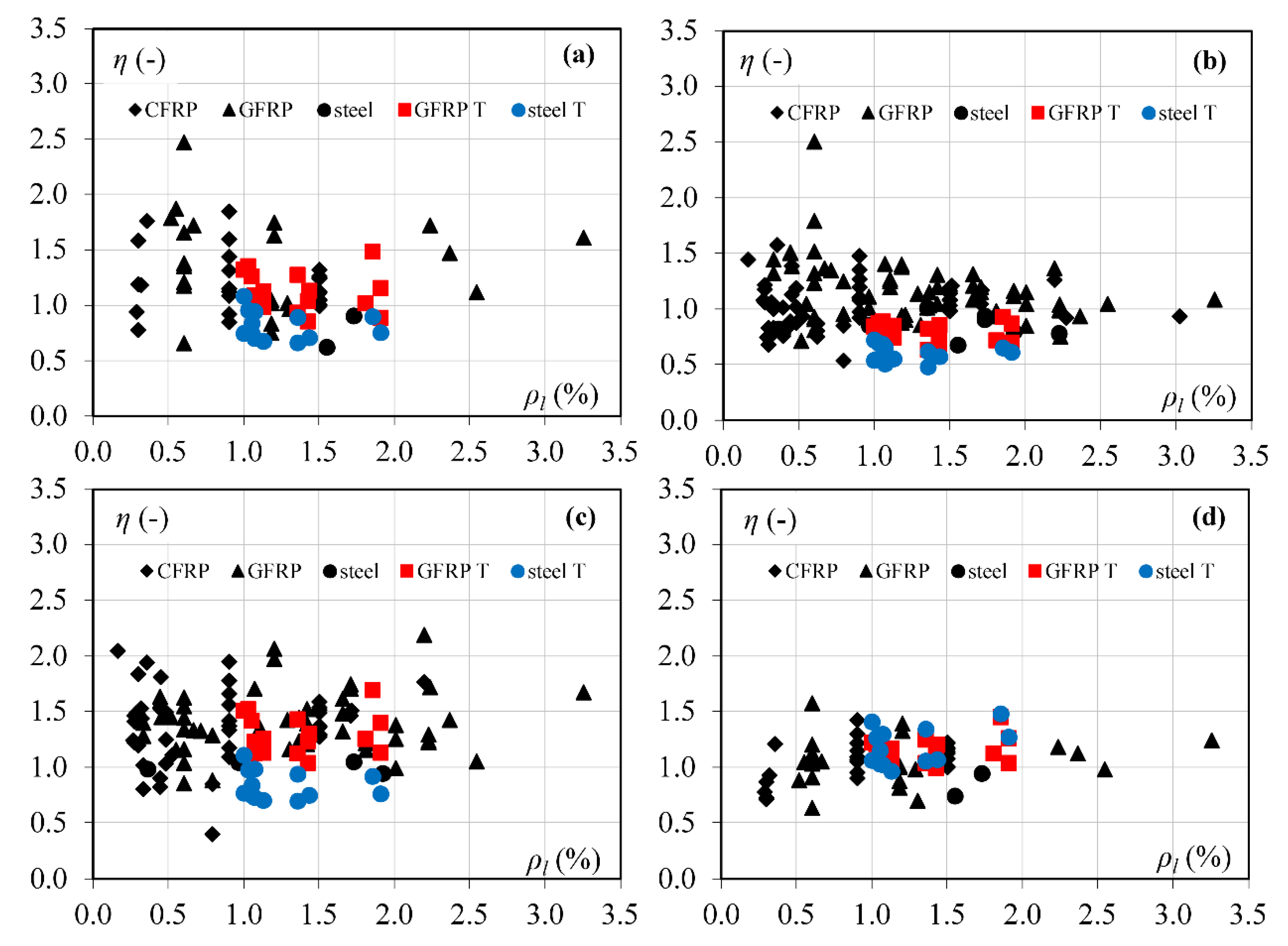
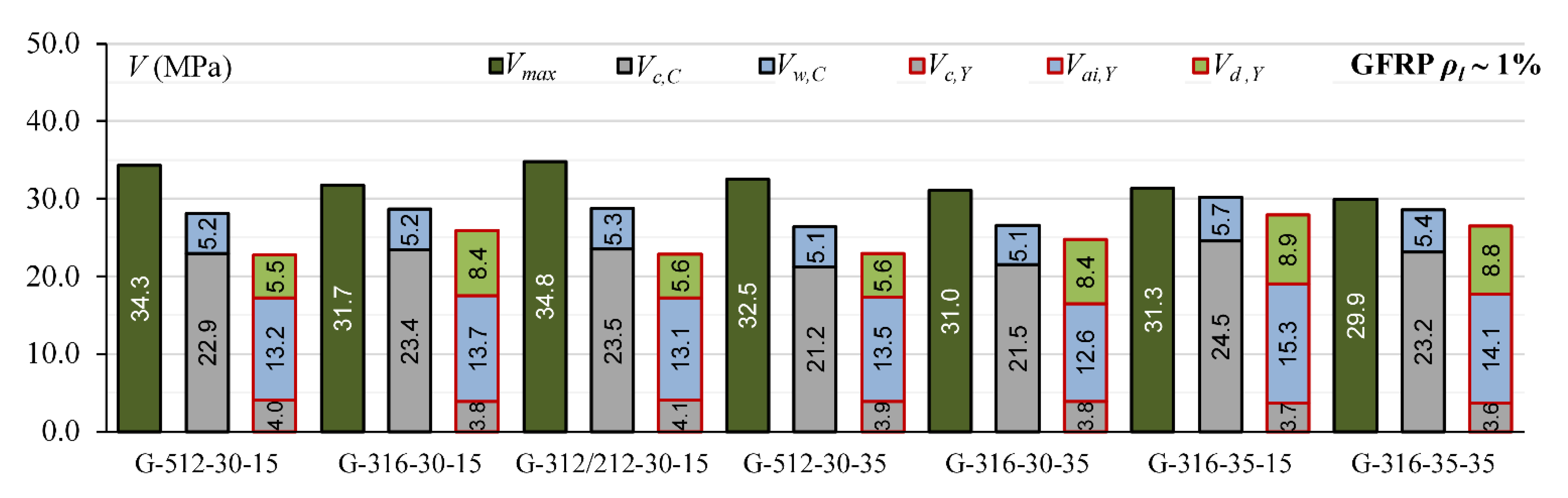
4. Results and Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| a | the distance from the support to the loading force [mm] |
| bw | the width of web in T-beams and the width in rectangular beams [mm] |
| d | the effective depth [mm] |
| dg | the maximum aggregate size [mm] |
| fc | the concrete compressive strength [MPa] |
| fctm | the mean concrete tensile strength [MPa] |
| hf | the height of flange in T-beams [mm] |
| n | the number of bars in longitudinal reinforcement [-] |
| x | the neutral axis depth [mm] |
| Al | the cross section longitudinal reinforcement [mm2] |
| E | the modulus of elasticity of longitudinal reinforcement [MPa] |
| Ec | the modulus of elasticity of concrete [MPa] |
| M | the bending moment in the critical section [kNm] |
| V | the shear force in critical section [kN] |
| ε | the strain in concrete [-] |
| the longitudinal reinforcement ratio [%] | |
| the diameter of longitudinal reinforcement [mm] |
Appendix A
| Specimen/Symbol | a/d | bw(mm) | d(mm) | fc(MPa) | dg (mm) | Type | ϕ (mm) | E (GPa) | Al (mm2) | ρl (%) | Vmax (kN) | [MR] | [Z] | [Y] | [C] | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Tureyen and Frosch [32] | V-A-1 | 3.4 | 457 | 360 | 40.3 | - | AFRP | - | 47.1 | 1579 | 1.0 | 115.6 | - | 105.5 | - | - |
| V-A-2 | 3.4 | 457 | 360 | 42.6 | - | AFRP | - | 130.0 | 3159 | 1.9 | 178.3 | - | 224.6 | - | - | |
| Zhao et al. [33] | I-No.1 | 3.00 | 150 | 250 | 34.3 | - | CFRP | - | 105.0 | 568 | 1.5 | 45.0 | - | 37.4 | - | - |
| II-No.6 | 3.00 | 150 | 250 | 34.3 | - | CFRP | - | 105.0 | 1136 | 3.0 | 46.0 | - | 49.1 | - | - | |
| IV-No.15 | 3.00 | 150 | 250 | 34.3 | - | CFRP | - | 105.0 | 852 | 2.3 | 40.5 | - | 44.0 | - | - | |
| El- Sayed et al. [34] | CN-1.7 | 3.10 | 250 | 326 | 43.6 | - | CFRP | 12.7 | 134 | 1393 | 1.7 | 124.5 | - | 106.3 | 82.4 | - |
| CH-1.7 | 3.10 | 250 | 326 | 63.0 | - | CFRP | 15.9 | 135 | 1390 | 1.7 | 130.0 | - | 124.8 | 88.8 | - | |
| CH-2.2 | 3.10 | 250 | 326 | 63.0 | - | CFRP | 15.9 | 135 | 1787 | 2.2 | 174.0 | - | 138.0 | 98.6 | - | |
| Jin et al. [35] | C-L-18-R1-1,2 | 3.10 | 200 | 215.5 | 33.6 | - | CFRP | 9 | 146.2 | 127 | 0.3 | 25.8 | - | 25.9 | 25.4 | - |
| C-L-18-R2-1,2 | 3.10 | 150 | 215.5 | 33.6 | - | CFRP | 9 | 146.2 | 127 | 0.4 | 18.9 | - | 22.1 | 22.9 | - | |
| C-L-18-R3-1,2 | 3.10 | 150 | 213.5 | 33.6 | - | CFRP | 13 | 147.9 | 265 | 0.8 | 15.3 | - | 28.6 | 38.6 | - | |
| C-L-27-R1-1,2 | 3.10 | 200 | 215.5 | 40.3 | - | CFRP | 9 | 146.2 | 127 | 0.3 | 23.2 | - | 27.9 | 28.9 | - | |
| C-L-27-R2-1,2 | 3.10 | 150 | 215.5 | 40.3 | - | CFRP | 9 | 146.2 | 127 | 0.4 | 21.1 | - | 23.8 | 23.4 | - | |
| C-L-27-R3-1,2 | 3.10 | 150 | 213.5 | 40.3 | - | CFRP | 13 | 147.9 | 265 | 0.8 | 26.2 | - | 30.8 | 30.7 | - | |
| Razaqpur et al. [36] | B1 | 3.50 | 300 | 200 | 52.3 | 20 | CFRP | 9.5 | 114 | 213 | 0.4 | 64.0 | 36.4 | 40.7 | 33.0 | 52.9 |
| B2 | 3.50 | 300 | 300 | 52.3 | 20 | CFRP | 9.5 | 114 | 284 | 0.3 | 61.0 | 51.6 | 57.8 | 39.9 | 65.8 | |
| B4 | 3.50 | 300 | 500 | 52.3 | 20 | CFRP | 9.5 | 114 | 425 | 0.3 | 68.0 | 72.6 | 91.8 | 45.7 | 87.4 | |
| B3 | 3.50 | 300 | 400 | 52.3 | 20 | CFRP | 9.5 | 114 | 354 | 0.3 | 55.0 | 70.8 | 74.8 | 46.1 | 77.2 | |
| Razaqpur et al. [37] | BA3 | 3.56 | 200 | 225 | 40.5 | - | CFRP | 8 | 145 | 201 | 0.4 | 47.0 | - | 34.0 | 26.0 | - |
| BA4 | 4.50 | 200 | 225 | 40.5 | - | CFRP | 8 | 145 | 201 | 0.4 | 38.5 | - | 34.0 | 25.3 | - | |
| Ashour and Kara [38] | B-300-2 | 3.60 | 200 | 276.117 | 29.8 | - | CFRP | 7.5 | 141.44 | 88 | 0.2 | 32.9 | - | 22.8 | 16.1 | - |
| B-300-4 | 3.60 | 200 | 276.117 | 29.8 | - | CFRP | 7.5 | 141.44 | 177 | 0.3 | 32.9 | - | 31.4 | 22.9 | - | |
| Olivito and Zuccarello [39] | Series I-1 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 19.5 | 18.0 | 18.1 | 14.6 | 18.4 |
| Series I-2 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 20.0 | 17.8 | 18.1 | 14.6 | 18.4 | |
| Series I-3 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 20.0 | 17.8 | 18.1 | 14.6 | 18.4 | |
| Series I-4 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 16.6 | 19.6 | 18.1 | 15.2 | 18.4 | |
| Series I-5 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 17.6 | 19.0 | 18.1 | 15.0 | 18.4 | |
| Series II-1 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 26.0 | 20.9 | 22.3 | 17.5 | 21.3 | |
| Series II-2 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 24.0 | 21.6 | 22.3 | 17.7 | 21.3 | |
| Series II-3 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 23.1 | 22.0 | 22.3 | 17.8 | 21.3 | |
| Series II-4 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 23.0 | 22.0 | 22.3 | 17.8 | 21.3 | |
| Series II-5 | 5.71 | 150 | 175 | 19.2 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 24.2 | 21.6 | 22.3 | 17.6 | 21.3 | |
| Series III-1 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 29.9 | 16.2 | 20.2 | 15.3 | 21.3 | |
| Series III-2 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 27.3 | 17.1 | 20.2 | 15.3 | 21.0 | |
| Series III-3 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 25.6 | 17.8 | 20.2 | 15.4 | 21.0 | |
| Series III-4 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 24.2 | 18.4 | 20.2 | 15.5 | 21.0 | |
| Series III-5 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 236 | 0.9 | 22.2 | 19.3 | 20.2 | 15.7 | 21.0 | |
| Series IV-1 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 29.7 | 22.5 | 25.0 | 18.7 | 21.0 | |
| Series IV-2 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 28.7 | 22.8 | 25.0 | 18.7 | 24.5 | |
| Series IV-3 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 24.5 | 24.6 | 25.0 | 19.2 | 24.5 | |
| Series IV-4 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 28.4 | 22.9 | 25.0 | 18.7 | 24.5 | |
| Series IV-5 | 5.71 | 150 | 175 | 25.6 | 20 | CFRP | 10 | 115 | 393 | 1.5 | 24.7 | 24.5 | 25.0 | 19.2 | 24.5 | |
| Ashour and Kara [38] | B-200-2 | 5.90 | 200 | 169.918 | 24.7 | - | CFRP | 7.5 | 141.44 | 88 | 0.3 | 17.6 | - | 16.3 | 14.2 | |
| B-200-4 | 5.90 | 200 | 169.918 | 24.7 | - | CFRP | 7.5 | 141.44 | 177 | 0.5 | 20.8 | - | 22.2 | 18.7 | ||
| Razaqpur et al. [36] | B6 | 6.00 | 300 | 400 | 52.3 | 20 | CFRP | 9.5 | 114 | 354 | 0.3 | 62.0 | 39.2 | 74.8 | 33.8 | 71.2 |
| B5 | 6.50 | 300 | 400 | 52.3 | 20 | CFRP | 9.5 | 114 | 354 | 0.3 | 51.0 | 43.0 | 74.8 | 34.5 | 70.4 | |
| Gross et al. [40] | 8-2-1 | 6.36 | 127 | 143 | 55.0 | - | CFRP | 6.3 | 139.0 | 60 | 0.3 | 14.3 | - | 12.2 | 10.1 | - |
| 8-2-2 | 6.36 | 127 | 143 | 55.0 | - | CFRP | 6.3 | 139.0 | 60 | 0.3 | 12.9 | - | 12.2 | 10.5 | - | |
| 8-2-3 | 6.36 | 127 | 143 | 55.0 | - | CFRP | 6.3 | 139.0 | 60 | 0.3 | 14.7 | - | 12.2 | 10.1 | - | |
| 11-2-1 | 6.36 | 89 | 143 | 76.0 | - | CFRP | - | 139.0 | 60 | 0.4 | 8.8 | - | 11.5 | - | - | |
| 11-2-2 | 6.36 | 89 | 143 | 76.0 | - | CFRP | - | 139.0 | 60 | 0.4 | 11.7 | - | 11.5 | - | - | |
| 11-2-3 | 6.36 | 89 | 143 | 76.0 | - | CFRP | - | 139.0 | 60 | 0.4 | 8.9 | - | 11.5 | - | - | |
| 8-3-1 | 6.45 | 159 | 141 | 55.0 | - | CFRP | 9.5 | 139.0 | 130 | 0.5 | 19.8 | - | 19.5 | 15.9 | - | |
| 8-3-2 | 6.45 | 159 | 141 | 55.0 | - | CFRP | 9.5 | 139.0 | 130 | 0.5 | 23.1 | - | 19.5 | 15.5 | - | |
| 8-3-3 | 6.45 | 159 | 141 | 55.0 | - | CFRP | 9.5 | 139.0 | 130 | 0.5 | 17.0 | - | 19.5 | 16.5 | - | |
| 11-3-1 | 6.45 | 121 | 141 | 76.0 | - | CFRP | - | 139.0 | 130 | 0.6 | 14.3 | - | 19.0 | - | - | |
| 11-3-2 | 6.45 | 121 | 141 | 76.0 | - | CFRP | - | 139.0 | 130 | 0.6 | 15.3 | - | 19.0 | - | - | |
| 11-3-3 | 6.45 | 121 | 141 | 76.0 | - | CFRP | - | 139.0 | 130 | 0.6 | 16.6 | - | 19.0 | - | - | |
| Niewels [41] | Q-A-3L | 2.93 | 300 | 444 | 43.3 | 8 | GFRP | 32 | 43.968 | 4021 | 3.3 | 149.0 | 92.5 | 137.6 | 89.3 | 119.9 |
| El-Sayed et al. [34] | GN-1.7 | 3.10 | 250 | 326 | 43.6 | - | GFRP | 15.9 | 42 | 1390 | 1.7 | 77.5 | - | 65.0 | 45.4 | = |
| GH-1.7 | 3.10 | 250 | 326 | 63.0 | - | GFRP | 15.9 | 42 | 1390 | 1.7 | 87.0 | - | 75.8 | 50.0 | = | |
| GH-2.2 | 3.10 | 250 | 326 | 63.0 | - | GFRP | 15.9 | 42 | 1787 | 2.2 | 115.5 | - | 84.8 | 52.8 | = | |
| Steiner et al. [42] | A1 | 3.1 | 457 | 889 | 29.6 | - | GFRP | - | 41.0 | 2413 | 0.6 | 159.0 | - | 172.1 | - | - |
| Jin et al. [35] | G-L-18-R1-1,2 | 3.10 | 200 | 215.5 | 33.6 | - | GFRP | 9 | 41.0 | 127 | 0.3 | 20.7 | - | 14.3 | 14.8 | - |
| G-L-18-R2-1,2 | 3.10 | 150 | 215.5 | 33.6 | - | GFRP | 9 | 41.0 | 127 | 0.4 | 18.6 | - | 12.3 | 11.7 | - | |
| G-L-18-R3-1,2 | 3.10 | 150 | 213.5 | 33.6 | - | GFRP | 13 | 40.0 | 265 | 0.8 | 15.3 | - | 16.0 | 17.3 | - | |
| G-L-27-R1-1,2 | 3.10 | 200 | 215.5 | 40.3 | - | GFRP | 9 | 41.0 | 127 | 0.3 | 20.4 | - | 15.4 | 15.9 | - | |
| G-L-27-R2-1,2 | 3.10 | 150 | 215.5 | 40.3 | - | GFRP | 9.00 | 41.0 | 127 | 0.4 | 20.0 | - | 13.3 | 12.2 | - | |
| G-L-27-R3-1,2 | 3.10 | 150 | 213.5 | 40.3 | - | GFRP | 13.00 | 40.0 | 265 | 0.8 | 21.5 | - | 17.2 | 16.7 | - | |
| Matta et al. [43] | S3-0.24-1B | 3.10 | 114 | 292 | 40.6 | 19 | GFRP | - | 48.2 | 393 | 1.2 | 22.0 | 26.5 | 23.5 | - | 25.2 |
| S3-0.24-2B | 3.10 | 114 | 292 | 40.6 | 19 | GFRP | - | 48.2 | 393 | 1.2 | 20.6 | 27.6 | 23.5 | - | 25.2 | |
| S6-0.24-1B | 3.10 | 229 | 146 | 40.6 | 19 | GFRP | - | 48.2 | 395 | 1.2 | 33.0 | 31.6 | 23.6 | - | 32.6 | |
| S6-0.24-2B | 3.10 | 229 | 146 | 40.6 | 19 | GFRP | - | 48.2 | 395 | 1.2 | 32.5 | 31.9 | 23.6 | - | 32.6 | |
| Matta and Nanni [44] | S1-1 | 3.11 | 457 | 883 | 29.5 | 20 | GFRP | 32 | 40.7 | 2413 | 0.6 | 154.1 | 93.0 | 170.1 | 99.5 | 128.2 |
| S3-1 | 3.11 | 114 | 294 | 59.7 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 15.2 | 23.2 | 18.9 | 17.7 | 24.1 | |
| S3-2 | 3.11 | 114 | 294 | 32.1 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 19.3 | 14.3 | 14.7 | 13.4 | 17.5 | |
| S3-3 | 3.11 | 114 | 294 | 32.1 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 18.1 | 15.0 | 14.7 | 13.5 | 17.5 | |
| S6-1 | 3.11 | 229 | 147 | 59.7 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 28.6 | 24.4 | 18.9 | 27.5 | 31.6 | |
| S6-2 | 3.11 | 229 | 147 | 32.1 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 36.8 | 14.9 | 14.7 | 22.7 | 23.4 | |
| S6-3 | 3.11 | 229 | 147 | 32.1 | 20 | GFRP | 16 | 40.8 | 201 | 0.6 | 26.3 | 19.1 | 14.7 | 22.6 | 23.4 | |
| S1B-1 | 3.12 | 457 | 880 | 29.5 | 20 | GFRP | 32 | 40.7 | 4825 | 1.2 | 220.7 | 126.2 | 234.0 | 107.1 | 158.7 | |
| S1B-2 | 3.12 | 457 | 880 | 30.7 | 20 | GFRP | 32 | 41.4 | 4825 | 1.2 | 216.2 | 132.8 | 239.6 | 109.6 | 163.1 | |
| Ashour [45] | Beam 3 | 3.14 | 150 | 212 | 28.9 | - | GFRP | 12 | 32 | 226 | 0.7 | 17.5 | - | 13.0 | 13.2 | 11.3 |
| Beam 9 | 3.14 | 150 | 212 | 50.2 | - | GFRP | 12 | 32 | 339 | 1.1 | 27.5 | - | 19.6 | 16.1 | 18.1 | |
| Bentz et al. [46] | L05-0 | 3.26 | 450 | 937 | 46.0 | 10 | GFRP | 25.4 | 37 | 2152 | 0.5 | 135.0 | 75.5 | 190.5 | 93.2 | 152.7 |
| M05-0 | 3.48 | 450 | 438 | 35.0 | 10 | GFRP | 25.4 | 37 | 1076 | 0.5 | 86.0 | 45.9 | 82.3 | 74.5 | 82.6 | |
| L20-0 | 3.56 | 450 | 857 | 36.0 | 10 | GFRP | 25.4 | 37 | 8608 | 2.2 | 232.0 | 134.6 | 309.2 | 135.2 | 196.8 | |
| M20-0 | 3.77 | 450 | 405 | 35.0 | 10 | GFRP | 25.4 | 37 | 4304 | 2.4 | 138.0 | 94.0 | 148.0 | 96.8 | 123.2 | |
| S05-0 | 3.93 | 450 | 194 | 35.0 | 10 | GFRP | 12.7 | 37 | 580 | 0.7 | 54.5 | 31.6 | 40.0 | 40.9 | 51.9 | |
| S20-0 | 4.05 | 450 | 188 | 35.0 | 10 | GFRP | 25.4 | 37 | 2152 | 2.5 | 74.0 | 66.2 | 71.0 | 70.3 | 75.8 | |
| Guadagnini et al. [47] | GB43 | 3.36 | 150 | 223 | 40.3 | 20 | GFRP | 13.5 | 45 | 429 | 1.3 | 27.2 | 26.7 | 24.0 | 19.1 | 27.7 |
| Tureyen and Frosch [32] | V-G1-1 | 3.4 | 457 | 360 | 39.7 | - | GFRP | - | 40.5 | 1579 | 1.0 | 108.9 | - | 97.9 | - | - |
| V-G2-1 | 3.4 | 457 | 360 | 39.8 | - | GFRP | - | 37.6 | 1579 | 1.0 | 95.4 | - | 94.7 | - | - | |
| V-G1-2 | 3.4 | 457 | 360 | 42.2 | - | GFRP | - | 32.0 | 3159 | 1.9 | 138.0 | - | 123.5 | - | - | |
| V-G2-2 | 3.4 | 457 | 360 | 42.5 | - | GFRP | - | 37.0 | 3159 | 1.9 | 153.7 | - | 132.1 | - | - | |
| Imjai [48] | TB6B | 3.49 | 150 | 220 | 95.0 | 10 | GFRP | 13.5 | 45 | 429 | 1.3 | 29.1 | 30.2 | 34.0 | 25.1 | 41.6 |
| Duranovic et al. [49] | GB2 | 3.65 | 150 | 210 | 38.1 | - | GFRP | 13.5 | 45 | 429 | 1.4 | 26.0 | - | 22.6 | 18.0 | - |
| GB6 | 3.65 | 150 | 210 | 32.9 | - | GFRP | 13.5 | 45 | 429 | 1.4 | 22.0 | - | 21.3 | 17.8 | - | |
| Ashour [45] | Beam 1 | 3.97 | 150 | 168 | 28.9 | - | GFRP | 6 | 38 | 113 | 0.4 | 12.5 | - | 9.0 | 8.6 | - |
| Beam 7 | 3.97 | 150 | 168 | 50.2 | - | GFRP | 12 | 32 | 339 | 1.3 | 17.5 | - | 17.3 | 15.5 | - | |
| Yost et al. [50] | 1FRP-a | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.1 | 39.1 | - | 30.9 | 28.9 | - |
| 1FRP-b | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.1 | 38.5 | - | 30.9 | 28.9 | - | |
| 1FRP-c | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.1 | 36.8 | - | 30.9 | 29.0 | - | |
| 2FRP-a | 4.06 | 178 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.4 | 28.1 | - | 26.9 | 23.3 | - | |
| 2FRP-b | 4.06 | 178 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.4 | 35.0 | - | 26.9 | 23.0 | - | |
| 2FRP-c | 4.06 | 178 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 567 | 1.4 | 32.1 | - | 26.9 | 23.1 | - | |
| 3FRP-a | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 851 | 1.7 | 40.0 | - | 37.0 | 30.4 | - | |
| 3FRP-b | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 851 | 1.7 | 48.6 | - | 37.0 | 30.1 | - | |
| 3FRP-c | 4.06 | 229 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 851 | 1.7 | 44.7 | - | 37.0 | 30.1 | - | |
| 4FRP-a | 4.06 | 279 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 1134 | 1.8 | 43.8 | - | 46.9 | 38.0 | - | |
| 4FRP-b | 4.06 | 279 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 1134 | 1.8 | 45.9 | - | 46.9 | 37.8 | - | |
| 4FRP-c | 4.06 | 279 | 225 | 34.7 | - | GFRP | 19 | 40.336 | 1134 | 1.8 | 46.1 | - | 46.9 | 37.7 | - | |
| 5FRP-a | 4.08 | 254 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.0 | 37.7 | - | 44.5 | 37.9 | - | |
| 5FRP-b | 4.08 | 254 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.0 | 51.0 | - | 44.5 | 37.0 | - | |
| 5FRP-c | 4.08 | 254 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.0 | 46.6 | - | 44.5 | 37.1 | - | |
| 6FRP-a | 4.08 | 229 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.2 | 43.5 | - | 42.0 | 33.7 | - | |
| 6FRP-b | 4.08 | 229 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.2 | 41.8 | - | 42.0 | 33.8 | - | |
| 6FRP-c | 4.08 | 229 | 224 | 34.7 | - | GFRP | 22 | 40.336 | 1140 | 2.2 | 41.3 | - | 42.0 | 33.8 | - | |
| El-Sayed et al. [34] | SN-1.7 | 3.10 | 250 | 326 | 43.6 | - | steel | 16 | 200 | 1407 | 1.7 | 144.5 | - | 124.7 | 101.1 | - |
| SH-1.7 | 3.10 | 250 | 326 | 63.0 | - | steel | 16 | 200 | 1407 | 1.7 | 160.0 | - | 146.5 | 110.3 | - | |
| SH-2.2 | 3.10 | 250 | 326 | 63.0 | - | steel | 16 | 200 | 1810 | 2.2 | 184.0 | - | 161.0 | 125.7 | - | |
| Guadagnini et al. [47] | SB40 | 3.35 | 150 | 224 | 43.4 | 20 | steel | 12 | 207 | 452 | 1.3 | 45.3 | 50.2 | 48.3 | 43.2 | 47.8 |
| Tureyen and Frosch [32] | V-S-1 | 3.4 | 457 | 360 | 40.9 | - | steel | - | 199.8 | 1579 | 1.0 | 180.5 | - | 198.6 | - | - |
| V-S-2 | 3.4 | 457 | 360 | 41.3 | - | steel | - | 200 | 3159 | 1.9 | 205.2 | - | 261.6 | - | - | |
| V-D-2 | 3.4 | 457 | 360 | 43.6 | - | steel | - | 200 | 592 | 0.4 | 135.7 | - | 134.3 | - | - | |
| Yost et al. [50] | 1Steel-a | 4.03 | 229 | 227 | 34.7 | - | steel | 16 | 200 | 804 | 1.5 | 60.7 | - | 70.7 | 58.2 | - |
| 1Steel-b | 4.03 | 229 | 227 | 34.7 | - | steel | 16 | 200 | 804 | 1.5 | 56.3 | - | 70.7 | 59.3 | - | |
| 1Steel-c | 4.03 | 229 | 227 | 34.7 | - | steel | 16 | 200 | 804 | 1.5 | 58.0 | - | 70.7 | 58.9 | - | |
| Olivito and Zuccarello [39] | S-1 | 5.56 | 150 | 180 | 19.2 | 20 | steel | - | 200 | 340 | 1.3 | 18.1 | 29.1 | 26.7 | - | 24.4 |
| Kotynia and Kaszubska [28] | G-512-30-15 | 2.90 | 150 | 379 | 30.10 | 8 | GFRP | 12 | 50.5 | 565 | 0.99 | 34.27 | 25.92 | 40.81 | 22.73 | 28.09 |
| G-316-30-15 | 2.92 | 150 | 377 | 31.10 | 8 | GFRP | 16 | 50.5 | 603 | 1.07 | 31.75 | 29.13 | 42.49 | 25.91 | 28.63 | |
| G-318-30-15 | 2.93 | 150 | 376 | 31.10 | 8 | GFRP | 18 | 50.5 | 763 | 1.35 | 38.57 | 30.24 | 46.93 | 26.97 | 30.86 | |
| G-416-30-15 | 2.92 | 150 | 377 | 30.50 | 8 | GFRP | 16 | 50.5 | 804 | 1.42 | 34.77 | 33.57 | 47.67 | 28.25 | 31.52 | |
| G-418-30-15 | 2.93 | 150 | 376 | 31.10 | 8 | GFRP | 18 | 50.5 | 1018 | 1.80 | 38.14 | 37.61 | 53.33 | 30.41 | 33.97 | |
| G-312/212-30-15 | 2.99 | 150 | 367.8 | 32.30 | 8 | GFRP | 12 | 50.5 | 565 | 1.02 | 34.78 | 25.66 | 39.83 | 22.87 | 28.76 | |
| G-318/118-30-15 | 3.00 | 150 | 367 | 32.30 | 8 | GFRP | 18 | 50.5 | 1018 | 1.85 | 47.72 | 32.14 | 51.50 | 28.19 | 32.88 | |
| G-512-30-35 | 3.06 | 150 | 359 | 31.10 | 8 | GFRP | 12 | 50.5 | 565 | 1.05 | 32.47 | 25.82 | 36.47 | 22.90 | 26.37 | |
| G-316-30-35 | 3.08 | 150 | 357 | 30.50 | 8 | GFRP | 16 | 50.5 | 603 | 1.13 | 31.01 | 27.64 | 36.51 | 24.77 | 26.56 | |
| G-318-30-35 | 3.09 | 150 | 356 | 30.50 | 8 | GFRP | 18 | 50.5 | 763 | 1.43 | 34.42 | 30.57 | 40.28 | 26.54 | 28.60 | |
| G-418-30-35 | 3.09 | 150 | 356 | 30.10 | 8 | GFRP | 18 | 50.5 | 1018 | 1.91 | 39.41 | 34.19 | 45.25 | 28.26 | 31.23 | |
| G-316-35-15 | 2.92 | 150 | 377 | 37.05 | 8 | GFRP | 16 | 50.5 | 603 | 1.07 | 31.31 | 32.04 | 48.35 | 27.92 | 30.24 | |
| G-318-35-15 | 2.93 | 150 | 376 | 37.05 | 8 | GFRP | 18 | 50.5 | 763 | 1.35 | 33.76 | 36.13 | 53.43 | 30.15 | 32.56 | |
| G-416-35-15 | 2.92 | 150 | 377 | 36.02 | 8 | GFRP | 16 | 50.5 | 804 | 1.42 | 32.43 | 38.13 | 53.97 | 31.17 | 32.66 | |
| G-316-35-35 | 3.08 | 150 | 357 | 35.00 | 8 | GFRP | 16 | 50.5 | 603 | 1.13 | 29.90 | 30.34 | 40.26 | 26.48 | 28.59 | |
| G-418-35-35 | 3.09 | 150 | 356 | 35.00 | 8 | GFRP | 18 | 50.5 | 1018 | 1.91 | 35.14 | 39.56 | 50.43 | 31.17 | 33.85 | |
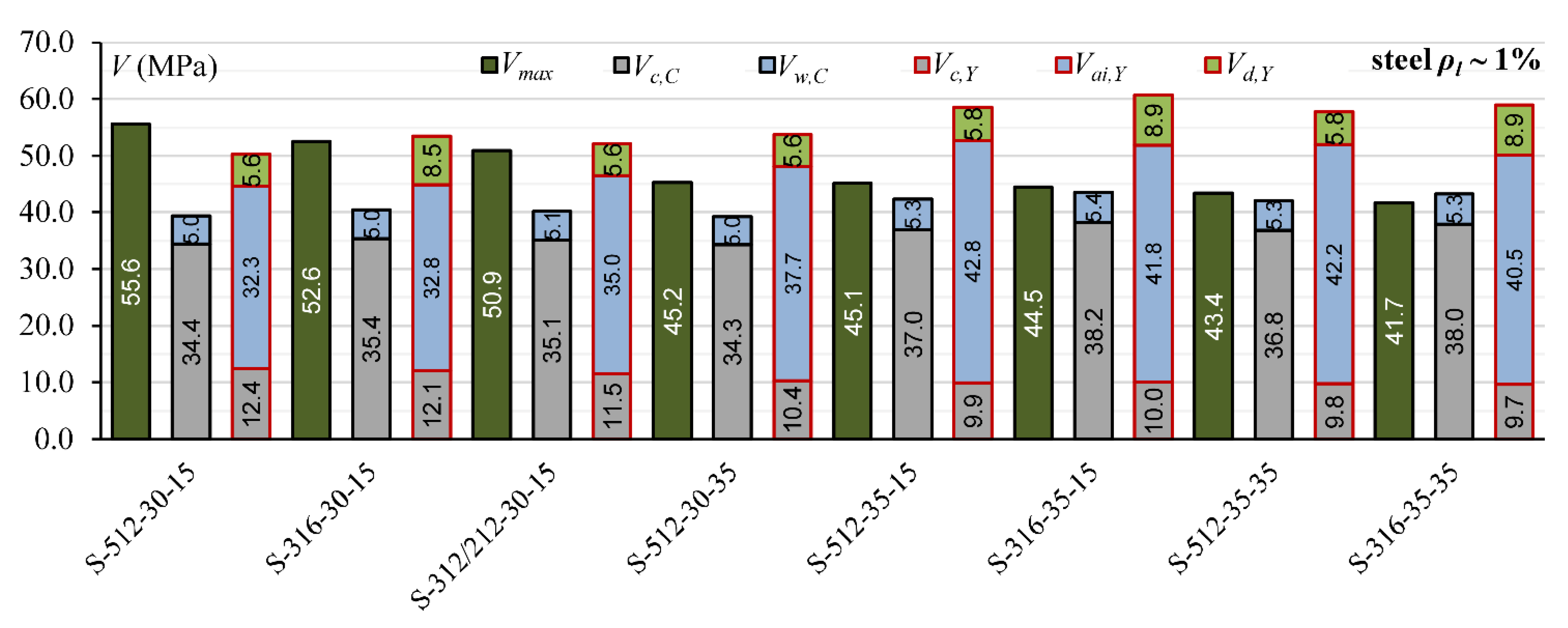
| Kotynia and Kaszubska [28] | S-512-30-15 | 2.90 | 150 | 379 | 31.10 | 8 | steel | 12 | 201 | 565 | 0.99 | 55.59 | 51.48 | 76.64 | 50.25 | 39.38 |
| S-316-30-15 | 2.92 | 150 | 377 | 32.30 | 8 | steel | 16 | 201 | 603 | 1.07 | 52.59 | 55.68 | 79.88 | 53.42 | 40.42 | |
| S-318-30-15 | 2.93 | 150 | 376 | 33.80 | 8 | steel | 18 | 201 | 763 | 1.35 | 56.10 | 62.81 | 90.37 | 59.59 | 41.77 | |
| S-312/212-30-15 | 2.99 | 150 | 367.8 | 32.30 | 8 | steel | 12 | 201 | 565 | 1.02 | 50.93 | 53.13 | 72.70 | 52.09 | 40.20 | |
| S-318/118-30-15 | 3.00 | 150 | 367 | 33.80 | 8 | steel | 18 | 201 | 1018 | 1.85 | 61.79 | 68.51 | 94.37 | 67.21 | 41.64 | |
| S-512-30-35 | 3.06 | 150 | 359 | 31.10 | 8 | steel | 12 | 201 | 565 | 1.05 | 45.24 | 53.90 | 66.21 | 53.67 | 39.16 | |
| S-418-30-35 | 3.09 | 150 | 356 | 33.80 | 8 | steel | 18 | 201 | 1018 | 1.91 | 52.94 | 70.18 | 86.62 | 69.58 | 41.66 | |
| S-512-35-15 | 2.90 | 150 | 379 | 34.95 | 8 | steel | 12 | 201 | 565 | 0.99 | 45.14 | 60.21 | 83.90 | 58.50 | 42.36 | |
| S-316-35-15 | 2.92 | 150 | 377 | 36.33 | 8 | steel | 16 | 201 | 603 | 1.07 | 44.52 | 63.55 | 87.47 | 60.67 | 43.55 | |
| S-318-35-15 | 2.93 | 150 | 376 | 37.35 | 8 | steel | 18 | 201 | 763 | 1.35 | 47.04 | 70.69 | 97.62 | 67.35 | 44.55 | |
| S-512-35-35 | 3.06 | 150 | 359 | 35.00 | 8 | steel | 12 | 201 | 565 | 1.05 | 43.40 | 58.10 | 72.27 | 57.74 | 42.13 | |
| S-316-35-35 | 3.08 | 150 | 357 | 36.33 | 8 | steel | 16 | 201 | 603 | 1.13 | 41.72 | 61.90 | 75.09 | 59.03 | 43.31 | |
| S-318-35-35 | 3.09 | 150 | 356 | 36.33 | 8 | steel | 18 | 201 | 763 | 1.43 | 46.89 | 66.23 | 81.85 | 62.60 | 43.70 |
References
- FIB Task Group 9.3. FRP Reinforcement in RC Structures. Bulletin No. 40, 2007, p. 160. Available online: https://re.public.polimi.it/handle/11311/661443 (accessed on 28 September 2007).
- JSCE. Recommendation for Design and Construction of Concrete Structures Using Continuous Fiber Reinforcing Materials; Japan Soc. of Civil Engineers: Tokyo, Japan, 1997; Volume 23. [Google Scholar]
- ACI 440.1R-15; Guide for the Design and Construction of Structural Concrete Reinforced with FRP Bars. American Concrete Institute: Farmington Hills, MI, USA, 2015; p. 88. [CrossRef] [Green Version]
- CAN/CSA-S806-12; Design and Construction of Building Structures with Fibre-Reinforced Polymers. Canadian Standards Association: Toronto, ON, Canada, 2012; p. 206.
- ISIS-M03-07; Reinforcing Concrete Structures with Fiber Reinforced Polymers. Canadian Network of Centers of Excellence on Intelligent Sensing for Innovative Structures. ISIS Canada: Winnipeg, MB, Canada, 2007.
- Kaszubska, M. Analysis of the Flexural Reinforcement on the Shear Strength of the Concrete Beams without Transverse Reinforcement. Ph.D. Thesis, Lodz University of Technology, Lodz, Poland, December 2018. [Google Scholar]
- Taylor, H.P.J. Investigation of the Dowel Shear Forces Carried by Tensile Steel in Reinforced Concrete Beams; Technical Report no. TRA 431; Cement and Concrete Association: London, UK, November 1969. [Google Scholar]
- Taylor, H.P.J. Investigation of the Forces Carried Across Cracks in Reinforced Concrete Beams in Shear by Interlock of Aggregate; Technical Report No. 42.77; Cement and Concrete Association: London, UK, 1970. [Google Scholar]
- Tottori, S.; Wakui, H. Shear capacity of RC and PC beams using FRP reinforcement. Proc. Aci Sp Detroit 1993, 138, 615–632. [Google Scholar] [CrossRef]
- Marí, A.; Bairán, J.; Cladera, A.; Oller, E.; Ribas, C. Shear-flexural strength mechanical model for the design and assessment of reinforced concrete beams. Struct. Infrastruct. Eng. 2015, 11, 1399–1419. [Google Scholar] [CrossRef]
- Muttoni, A.; Ruiz, M.F. Shear strength of members without transverse reinforcement as function of critical shear crack width. ACI Struct. J. 2008, 105, 163–172. [Google Scholar] [CrossRef]
- Zhang, T.; Oehlers, D.J.; Visintin, P. Shear Strength of FRP RC Beams and One-Way Slabs without Stirrups. J. Compos. Constr. 2014, 18, 5. [Google Scholar] [CrossRef]
- Yang, Y. Shear Behaviour of Reinforced Concrete Members without Shear Reinforcement, a New Look at an Old Problem. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, April 2014. [Google Scholar]
- Cladera, A.; Marí, A.; Bairán, J.M.; Ribas, C.; Oller, E.; Duarte, N. The compression chord capacity model for the shear design and assessment of reinforced and prestressed concrete beams. Struct. Concr. 2016, 17, 1017–1032. [Google Scholar] [CrossRef]
- Kani, M.W.; Huggins, M.W.; Wittkopp, R.R. Kani on Shear in Reinforced Concrete; Deptartment of Civil Engineering, University of Toronto: Toronto, ON, Canada, 1979. [Google Scholar]
- Lucas, W.; Oehlers, D.J.; Ali, M. Formulation of a Shear Resistance Mechanism for Inclined Cracks in RC Beams. J. Struct. Eng. 2011, 137, 12. [Google Scholar] [CrossRef]
- ACI 363. State-of-the-Art Report on High-Strength Concrete (ACI 363R-92). ACI J. Proc. 1992, 363, 92. [Google Scholar]
- Bažant, Z.P.; Ohtsubo, H. Stability conditions for propagation of a system of cracks in a brittle solid. Mech. Res. Commun. 1977, 4, 353–366. [Google Scholar] [CrossRef]
- Walraven, J. Aggregate Interlock: A Theoretical and Experimental Analysis. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, October 1980. [Google Scholar]
- Baumann, T.; Rüsch, H. Versuche zum Studium der Verdübelungswirkung der Biegezugbewehrung eines Stahlbetonbalkens (Heft 210); Ernst and Sohn: Berlin, Germany, 1970. [Google Scholar]
- Mörsch, E. Concrete-Steel Construction; Engineering News Publishing Company: London, UK, 1909. [Google Scholar]
- Marí, A.; Cladera, A.; Bairán García, J.M.; Oller, E.; Ribas, C. Shear-flexural strength mechanical model for the design and assessment of reinforced concrete beams subjected to point or distributed loads. Struct. Infrastruct. Eng. 2014, 8, 337–353. [Google Scholar] [CrossRef] [Green Version]
- Cladera, A.; Marí, A.; Ribas, C.; Bairán, J.; Oller, E. Predicting the shear-flexural strength of slender reinforced concrete T and I shaped beams. Eng. Struct. 2015, 101, 386–398. [Google Scholar] [CrossRef]
- Szczech, D.; Kotynia, R. Effect of shear reinforcement ratio on the shear capacity of GFRP reinforced concrete beams. Arch. Civ. Eng. 2021, 67, 1. [Google Scholar]
- Szczech, D.; Kotynia, R. Shear Tests on GFRP Reinforced Concrete Beams. In Proceedings of the MATEC Web of Conferences EDP Sciences, Lodz, Poland, 21–23 October 2020; Volume 323. [Google Scholar] [CrossRef]
- Kaszubska, M.; Kotynia, R.; Barros, J.A.O. Influence of Longitudinal GFRP Reinforcement Ratio on Shear Capacity of Concrete Beams without Stirrups. Procedia Eng. 2017, 193, 361–368. [Google Scholar] [CrossRef]
- Kaszubska, M.; Kotynia, R.; Barros, J.A.O.; Baghi, H. Shear behavior of concrete beams reinforced exclusively with longitudinal glass fiber reinforced polymer bars: Experimental research. Struct. Concr. 2017, 19, 152–161. [Google Scholar] [CrossRef] [Green Version]
- Kotynia, R.; Kaszubska, M. Research of the flexural reinforcement effect on the shear strength of concrete beams without transverse reinforcement. Report 2020, 23, 164. [Google Scholar]
- EN 1992-1-1; Eurocode 2: Design of Concrete Structures. Part 1: General Rules and Rules for Buildings. British Standard Institution: London, UK, 2004.
- Zhang, T.; Visintin, P.; Oehlers, D.J. Shear strength of RC beams without web reinforcement. Aust. J. Struct. Eng. 2016, 17, 87–96. [Google Scholar] [CrossRef] [Green Version]
- Kupfer, H. Erweiterung der Mörsch’schen Fachwerkanalogie mit Hilfe des Prinzips vom Minimum der Formänderungsarbeit; CEB Bulletin d’information: Paris, France, 1964. [Google Scholar]
- Tureyen, A.K.; Frosch, R.J. Shear tests of FRP-reinforced concrete beams without stirrups. ACI Struct. J. 2002, 99, 427–434. [Google Scholar] [CrossRef]
- Zhao, W.; Maruyama, K.; Suzuki, H. Shear behaviour of concrete beams reinforced by FRP rods as longitudinal and shear reinforcement. In Proceedings of the Non-Metallic (FRP) Reinforcement for Concrete Structures, Second International RILEM Symposium, Rilem, Ghent, 23–25 August 1995. [Google Scholar]
- El-Sayed, A.K.; El-Salakawy, E.; Benmokrane, B. Shear Capacity of High-Strength Concrete Beams Reinforced with FRP Bars. ACI Struct. J. 2006, 103, 383–389. [Google Scholar]
- Jin, M.H.; Jang, H.S.; Kim, C.H.; Baek, D.I. Concrete Shear Strength of Lightweight Concrete BEAM REINFORCED with FRP bar. In Proceedings of the APFIS, Seoul, Korea; 9–11 December 2009. [Google Scholar]
- Razaqpur, A.; Shedid, G.M.; Isgor, B. Shear Strength of Fiber-Reinforced Polymer Reinforced Concrete Beams Subject to Unsymmetric Loading. J. Compos. Constr. 2011, 15, 500–512. [Google Scholar] [CrossRef]
- Razaqpur, A.G.; Isgor, B.O.; Greenaway, S.; Selley, A. Concrete Contribution to the Shear Resistance of Fiber Reinforced Polymer Reinforced Concrete Members. J. Compos. Constr. 2004, 8, 452–460. [Google Scholar] [CrossRef]
- Ashour, A.F.; Kara, I.F. Shear Capacity of FRP Reinforced Concrete Beams. In Proceedings of the FRPRCS-11, Guimarães, Portugal; 26–28 June 2013. [Google Scholar]
- Olivito, R.S.; Zuccarello, F.A. On the shear behaviour of concrete beams reinforced by carbon fibre-reinforced polymer bars: An experimental investigation by means of acoustic emission technique. Strain 2010, 46, 5. [Google Scholar] [CrossRef]
- Gross, S.P.; Dinehart, D.W.; Yost, J.R.; Theisz, P.M. Experimental tests of high-strength concrete beams reinforced with CFRP bars. In Proceedings of the 4th International Conference on Advanced Composite Materials in Bridges and Structures (ACMBS-4), Calgary, AB, Canada; 20–23 July 2004. [Google Scholar]
- Niewels, J. Zum Tragverhalten von Betonbauteilen mit Faserverbundkunststoff-Bewehrung. Ph.D Thesis, Rheinisch-Westfälische Technische Hochschule Aachen, Aachen, Germany, November 2008. [Google Scholar]
- Steiner, S.; El-Sayed, A.K.; Benmokrane, B. Shear Behaviour of Large-Size Beams Reinforced with Glass FRP Bars. In Proceedings of the Annual Conference—Canadian Society for Civil Engineering, Quebec, Canada, 10–13 June 2008. [Google Scholar]
- Matta, F.; El-Sayed, A.K.; Nanni, A.; Benmokrane, B. Size effect on concrete shear strength in beams reinforced with fiber-reinforced polymer bars. ACI Struct. J. 2013, 110, 617–628. [Google Scholar]
- Matta, F.; Nanni, A. Scaling of strength of FRP reinforced concrete beams without shear reinforcement. In Proceedings of the Fourth International Conference on FRP Composites in Civil Engineering (CICE2008), Zurich, Shwitzerland, 22–24 July 2008. [Google Scholar]
- Ashour, A.F. Flexural and shear capacities of concrete beams reinforced with GFRP bars. Constr. Build. Mater. 2006, 20, 1005–1015. [Google Scholar] [CrossRef]
- Bentz, E.C.; Massam, L.; Collins, M.P. Shear Strength of Large Concrete Members with FRP Reinforcement. J. Compos. Constr. 2010, 14, 637–646. [Google Scholar] [CrossRef]
- Guadagnini, M.; Pilakoutas, K.; Waldron, P. Shear Resistance of FRP RC Beams: Experimental Study. J. Compos. Constr. 2006, 10, 464–473. [Google Scholar] [CrossRef]
- Imjai, T. Design and Analysis of Curved FRP Composites as Shear Reinforcement for Concrete Structures. Ph.D. Thesis, The University of Sheffield, Sheffield, UK, 2007. [Google Scholar]
- Duranovic, N.; Pilakoutas, K.; Waldron, P. Tests on Concrete Beams Reinforced with Glass Fibre Reinforced Plastic Bars. In Proceedings of the Third International Symposium on Non-Metallic (FRP) Reinforcement for Concrete Structures (FRPRCS-3), Sapporo, Japan, 14–16 October 1997. [Google Scholar]
- Yost, J.R.; Gross, S.P.; Dinehart, D.W. Shear Strength of Normal Strength Concrete Beams Reinforced with Deformed GFRP Bars. J. Compos. Constr. 2001, 5, 268–275. [Google Scholar] [CrossRef]








| Muttoni and Ruiz [11] | Zhang et al. [12] | Yang [13] | Cladera et al. [14] | |
|---|---|---|---|---|
| Number of specimens | 79 | 158 | 134 | 79 |
| ηmin | 0.62 | 0.48 | 0.40 | 0.63 |
| ηmax | 2.47 | 2.50 | 2.19 | 1.57 |
| ηm | 1.16 | 1.01 | 1.31 | 1.09 |
| median | 1.11 | 1.01 | 1.32 | 1.09 |
| ση | 0.35 | 0.27 | 0.31 | 0.18 |
| COV | 0.30 | 0.27 | 0.24 | 0.17 |
| Muttoni and Ruiz [11] | Zhang et al. [12] | Yang [13] | Cladera et al. [14] | |
|---|---|---|---|---|
| CFRP-reinforced rectangular beams | ||||
| Number of specimens | 26 | 56 | 47 | 26 |
| ηmin | 0.78 | 0.54 | 0.40 | 0.71 |
| ηmax | 1.85 | 1.57 | 2.04 | 1.42 |
| ηm | 1.20 | 1.04 | 1.38 | 1.06 |
| ση | 0.26 | 0.20 | 0.32 | 0.17 |
| COV | 0.22 | 0.20 | 0.23 | 0.16 |
| GFRP-reinforced rectangular beams | ||||
| Number of specimens | 22 | 60 | 51 | 22 |
| ηmin | 0.65 | 0.71 | 0.86 | 0.63 |
| ηmax | 2.47 | 2.50 | 2.19 | 1.57 |
| ηm | 1.37 | 1.16 | 1.39 | 1.05 |
| ση | 0.43 | 0.28 | 0.27 | 0.21 |
| COV | 0.31 | 0.24 | 0.19 | 0.20 |
| GFRP-reinforced T-beams | ||||
| Number of specimens | 16 | 16 | 16 | 16 |
| ηmin | 0.85 | 0.60 | 1.04 | 0.99 |
| ηmax | 1.48 | 0.93 | 1.69 | 1.45 |
| ηm | 1.12 | 0.78 | 1.30 | 1.15 |
| ση | 0.18 | 0.10 | 0.18 | 0.11 |
| COV | 0.16 | 0.13 | 0.14 | 0.10 |
| GFRP-reinforced beams (T-section and rectangular) | ||||
| Number of specimens | 38 | 76 | 67 | 38 |
| ηmin | 0.65 | 0.60 | 0.86 | 0.63 |
| ηmax | 2.47 | 2.50 | 2.19 | 1.57 |
| ηm | 1.26 | 1.08 | 1.37 | 1.10 |
| ση | 0.37 | 0.29 | 0.25 | 0.19 |
| COV | 0.29 | 0.27 | 0.18 | 0.17 |
| steel-reinforced rectangular beams | ||||
| Number of specimens | 2 | 11 | 7 | 2 |
| ηmin | - | 0.68 | 0.95 | - |
| ηmax | - | 1.16 | 1.46 | - |
| ηm | - | 0.93 | 1.20 | - |
| ση | - | 0.15 | 0.22 | - |
| COV | - | 0.16 | 0.19 | - |
| steel-reinforced T-beams | ||||
| Number of specimens | 13 | 13 | 13 | 13 |
| ηmin | 0.67 | 0.48 | 0.70 | 0.96 |
| ηmax | 1.08 | 0.73 | 1.11 | 1.48 |
| ηm | 0.82 | 0.61 | 0.84 | 1.19 |
| ση | 0.12 | 0.07 | 0.13 | 0.16 |
| COV | 0.15 | 0.12 | 0.15 | 0.14 |
| steel-reinforced beams (T-section and rectangular) | ||||
| Number of specimens | 15 | 24 | 20 | 15 |
| ηmin | 0.62 | 0.48 | 0.70 | 0.74 |
| ηmax | 1.08 | 1.16 | 1.46 | 1.48 |
| ηm | 0.81 | 0.75 | 0.97 | 1.14 |
| ση | 0.13 | 0.20 | 0.24 | 0.19 |
| COV | 0.16 | 0.26 | 0.24 | 0.17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaszubska, M.; Kotynia, R. Selected Shear Models Based on the Analysis of the Critical Shear Crack for Slender Concrete Beams without Shear Reinforcement. Materials 2022, 15, 8259. https://doi.org/10.3390/ma15228259
Kaszubska M, Kotynia R. Selected Shear Models Based on the Analysis of the Critical Shear Crack for Slender Concrete Beams without Shear Reinforcement. Materials. 2022; 15(22):8259. https://doi.org/10.3390/ma15228259
Chicago/Turabian StyleKaszubska, Monika, and Renata Kotynia. 2022. "Selected Shear Models Based on the Analysis of the Critical Shear Crack for Slender Concrete Beams without Shear Reinforcement" Materials 15, no. 22: 8259. https://doi.org/10.3390/ma15228259
APA StyleKaszubska, M., & Kotynia, R. (2022). Selected Shear Models Based on the Analysis of the Critical Shear Crack for Slender Concrete Beams without Shear Reinforcement. Materials, 15(22), 8259. https://doi.org/10.3390/ma15228259







