Long-Term Stability of TiS2–Alkylamine Hybrid Materials
Abstract
1. Introduction
2. Materials and Methods
2.1. Synthesis
2.2. Experimental Characterization
2.3. Computational Methods
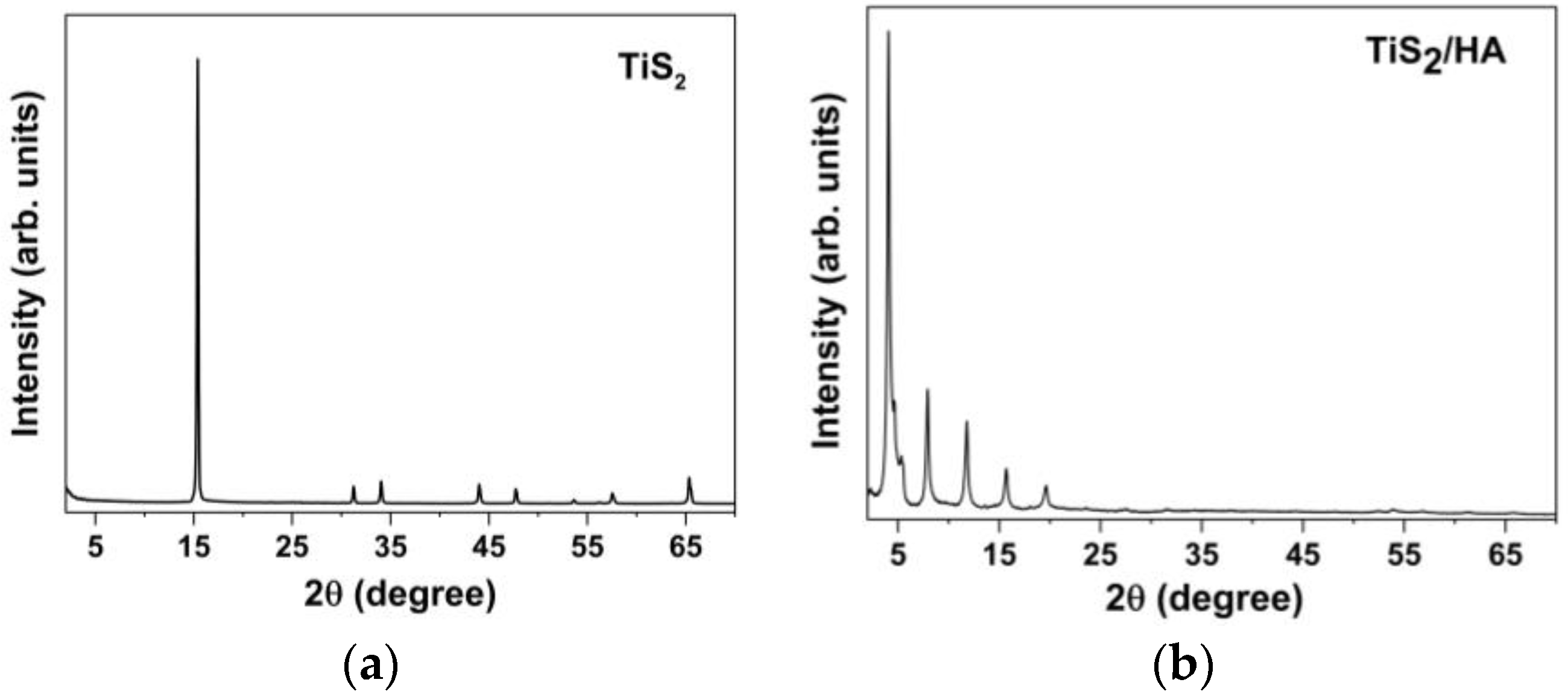
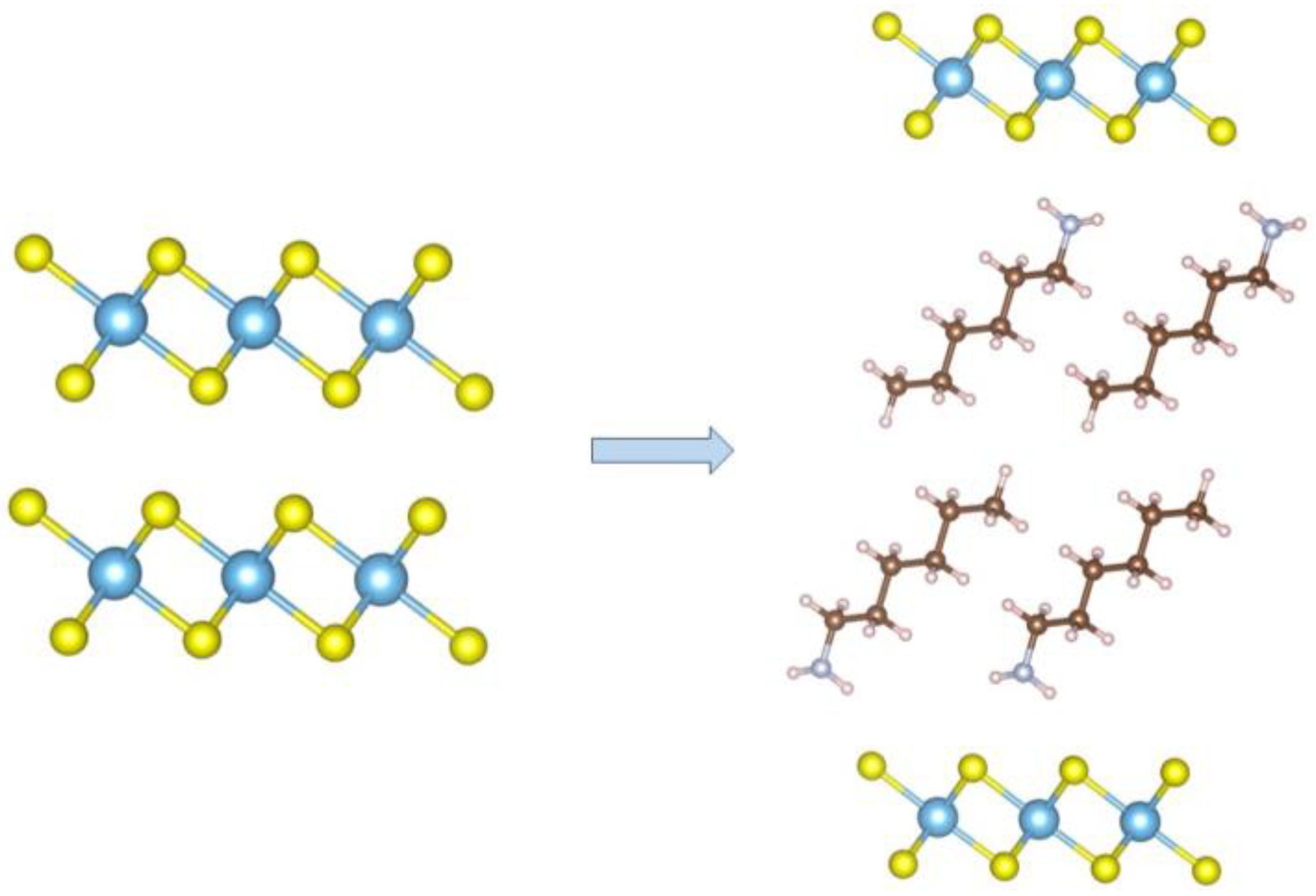
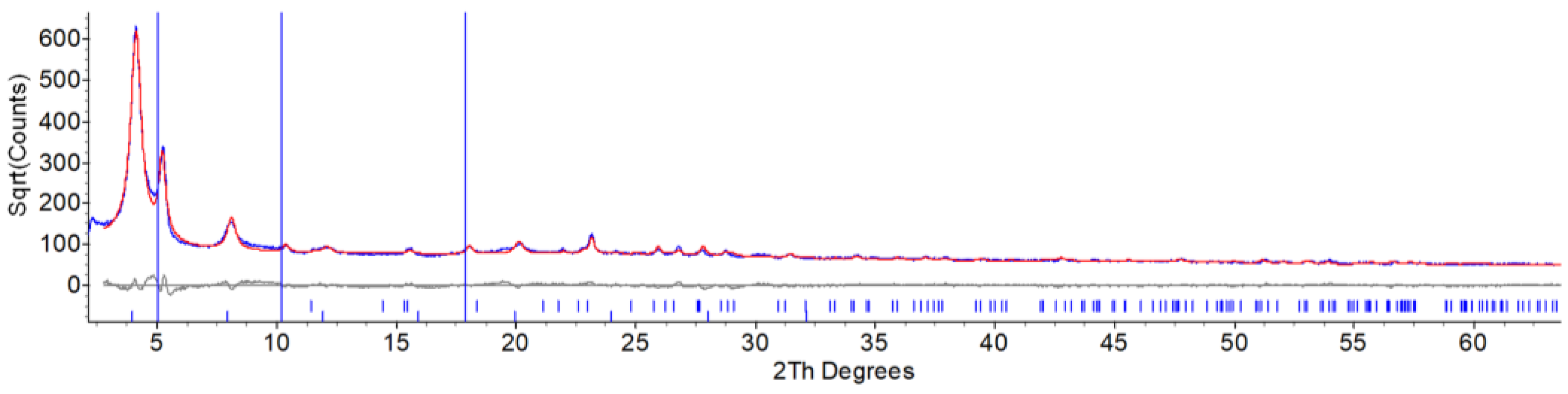
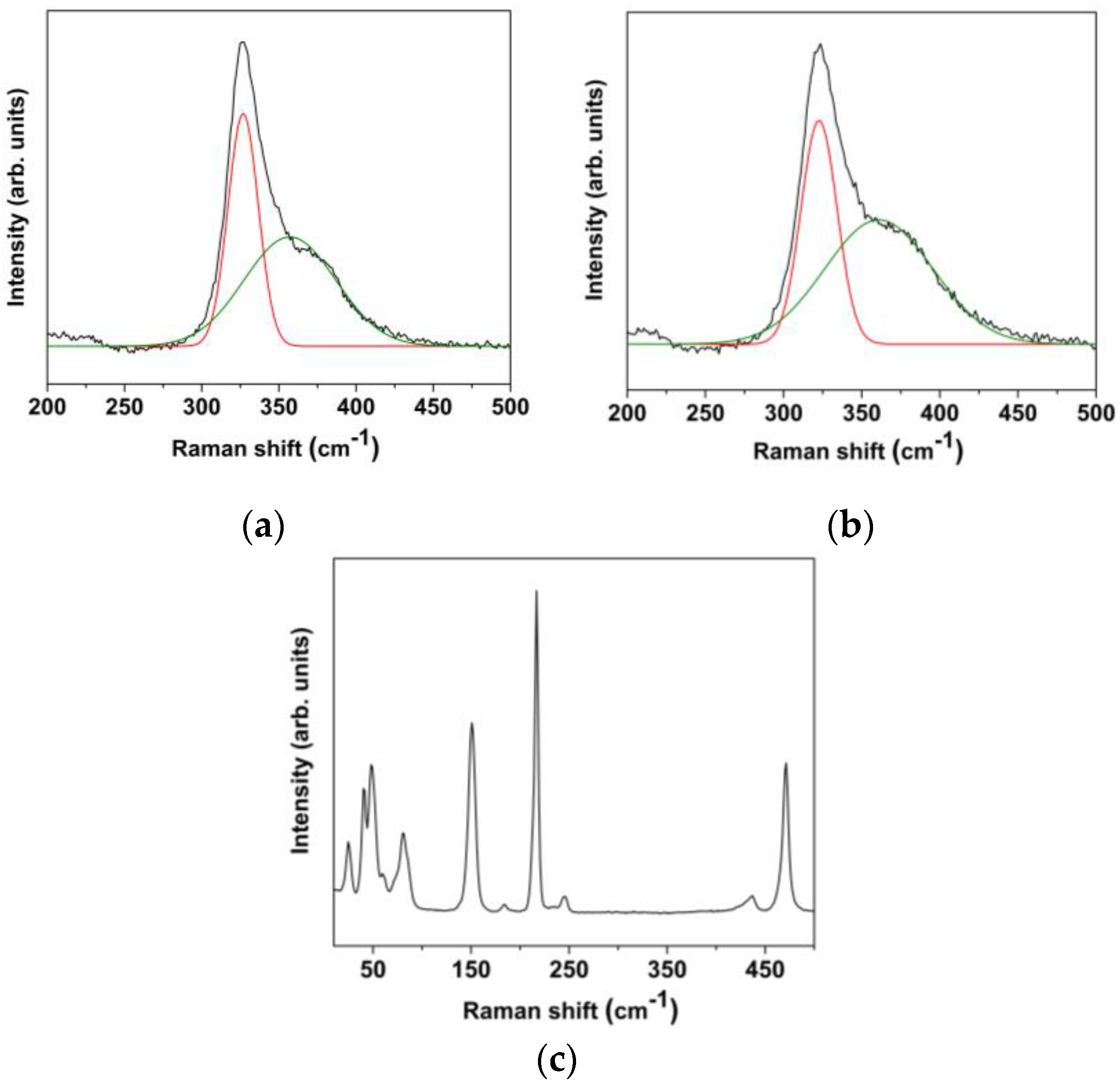
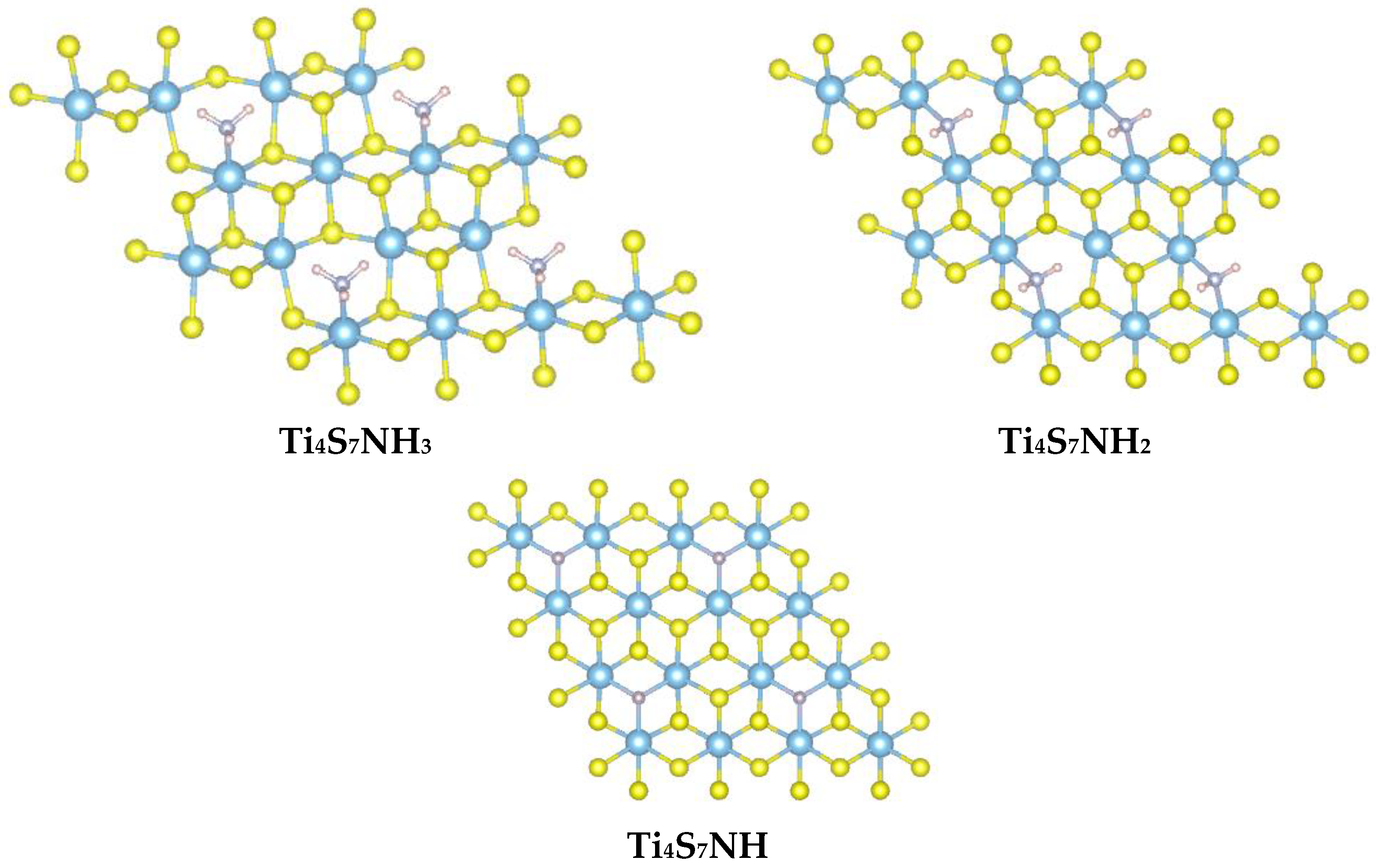
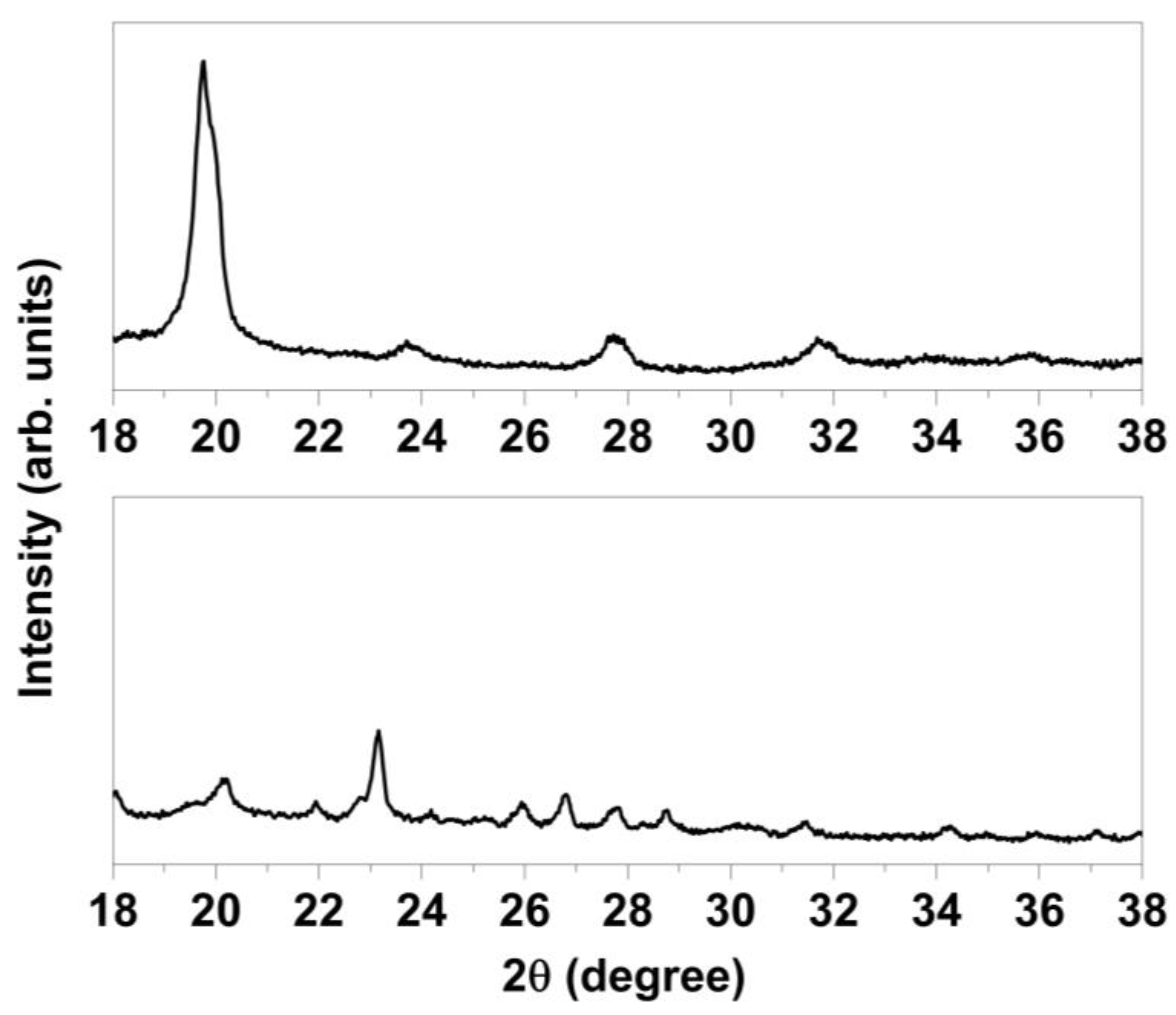
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Newby, S.; Mirihanage, W.; Fernando, A. Recent Advancements in Thermoelectric Generators for Smart Textile Application. Mater. Today Commun. 2022, 33, 104585. [Google Scholar] [CrossRef]
- Sun, W.; Liu, W.-D.; Liu, Q.; Chen, Z.G. Advances in Thermoelectric Devices for Localized Cooling. Chem. Eng. J. 2022, 450, 138389. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, W.; Yang, J.; Li, S. Recent Advances of Layered Thermoelectric Materials. Adv. Sustain. Syst. 2018, 2, 1800046. [Google Scholar] [CrossRef]
- Gayner, C.; Kar, K.K. Recent Advances in Thermoelectric Materials. Prog. Mater. Sci. 2016, 83, 330–382. [Google Scholar] [CrossRef]
- Cao, T.; Shi, X.L.; Chen, Z.G. Advances in the Design and Assembly of Flexible Thermoelectric Device. Prog. Mater. Sci. 2022, 131, 101003. [Google Scholar] [CrossRef]
- Choi, J.; Gordon, M.P.; Yuan, P.; Kang, H.; Zaia, E.W.; Urban, J.J.; Li, L.; Lu, N.; Liu, M.; Xu, H.; et al. Organic Thermoelectric Materials; Lin, Z., He, M., Eds.; The Royal Society of Chemistry: London, UK, 2020; pp. 1–316. ISBN 978-1-78801-470-0. [Google Scholar]
- Wang, H.; Yu, C. Organic Thermoelectrics: Materials Preparation, Performance Optimization, and Device Integration. Joule 2019, 3, 53–80. [Google Scholar] [CrossRef]
- Cowen, L.M.; Atoyo, J.; Carnie, M.J.; Baran, D.; Schroeder, B.C. Review—Organic Materials for Thermoelectric Energy Generation. ECS J. Solid State Sci. Technol. 2017, 6, N3080–N3088. [Google Scholar] [CrossRef]
- Champier, D. Thermoelectric Generators: A Review of Applications. Energy Convers. Manag. 2017, 140, 167–181. [Google Scholar] [CrossRef]
- Jin, H.; Li, J.; Iocozzia, J.; Zeng, X.; Wei, P.; Yang, C.; Li, N.; Liu, Z.; He, J.H.; Zhu, T.; et al. Hybride Organisch-anorganische Thermoelektrische Materialien Und Baueinheiten. Angew. Chem. 2019, 131, 15348–15370. [Google Scholar] [CrossRef]
- Huang, Y.; Liang, J.; Wang, C.; Yin, S.; Fu, W.; Zhu, H.; Wan, C. Hybrid Superlattices of Two-Dimensional Materials and Organics. Chem. Soc. Rev. 2020, 49, 6866–6883. [Google Scholar] [CrossRef]
- Tchitchekova, D.S.; Ponrouch, A.; Verrelli, R.; Broux, T.; Frontera, C.; Sorrentino, A.; Bardé, F.; Biskup, N.; Arroyo-De Dompablo, M.E.; Palacín, M.R. Electrochemical Intercalation of Calcium and Magnesium in TiS2: Fundamental Studies Related to Multivalent Battery Applications. Chem. Mater. 2018, 30, 847–856. [Google Scholar] [CrossRef]
- Tian, R.; Wan, C.; Wang, Y.; Wei, Q.; Ishida, T.; Yamamoto, A.; Tsuruta, A.; Shin, W.; Li, S.; Koumoto, K. A Solution-Processed TiS2/Organic Hybrid Superlattice Film towards Flexible Thermoelectric Devices. J. Mater. Chem. A 2017, 5, 564–570. [Google Scholar] [CrossRef]
- Wan, C.; Tian, R.; Azizi, A.B.; Huang, Y.; Wei, Q.; Sasai, R.; Wasusate, S.; Ishida, T.; Koumoto, K. Flexible Thermoelectric Foil for Wearable Energy Harvesting. Nano Energy 2016, 30, 840–845. [Google Scholar] [CrossRef]
- Guo, Z.; Sun, F.; Yuan, W. Chemical Intercalations in Layered Transition Metal Chalcogenides: Syntheses, Structures, and Related Properties. Cryst. Growth Des. 2017, 17, 2238–2253. [Google Scholar] [CrossRef]
- Jung, Y.; Zhou, Y.; Cha, J.J. Intercalation in Two-Dimensional Transition Metal Chalcogenides. Inorg. Chem. Front. 2016, 3, 452–463. [Google Scholar] [CrossRef]
- Commins, P.; Dippenaar, A.B.; Li, L.; Hara, H.; Haynes, D.A.; Naumov, P. Mechanically Compliant Single Crystals of a Stable Organic Radical. Chem. Sci. 2021, 12, 6188–6193. [Google Scholar] [CrossRef]
- Wan, C.; Wang, Y.; Wang, N.; Norimatsu, W.; Kusunoki, M.; Koumoto, K. Development of Novel Thermoelectric Materials by Reduction of Lattice Thermal Conductivity. Sci. Technol. Adv. Mater. 2010, 11, 044306. [Google Scholar] [CrossRef]
- Wan, C.; Tian, R.; Kondou, M.; Yang, R.; Zong, P.; Koumoto, K. Ultrahigh Thermoelectric Power Factor in Flexible Hybrid Inorganic-Organic Superlattice. Nat. Commun. 2017, 8, 1024. [Google Scholar] [CrossRef]
- Wan, C.; Gu, X.; Dang, F.; Itoh, T.; Wang, Y.; Sasaki, H.; Kondo, M.; Koga, K.; Yabuki, K.; Snyder, G.J.; et al. Flexible N-Type Thermoelectric Materials by Organic Intercalation of Layered Transition Metal Dichalcogenide TiS2. Nat. Mater. 2015, 14, 622–627. [Google Scholar] [CrossRef]
- Trost, B.M. Atom Economy—A Challenge for Organic Synthesis: Homogeneous Catalysis Leads the Way. Angew. Chem. Int. Ed. English 1995, 34, 259–281. [Google Scholar] [CrossRef]
- Coelho, A.A. TOPAS and TOPAS-Academic: An Optimization Program Integrating Computer Algebra and Crystallographic Objects Written in C++. J. Appl. Crystallogr. 2018, 51, 210–218. [Google Scholar] [CrossRef]
- Giannozzi, P.; Baroni, S.; Bonini, N.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Chiarotti, G.L.; Cococcioni, M.; Dabo, I.; et al. QUANTUM ESPRESSO: A Modular and Open-Source Software Project for Quantum Simulations of Materials. J. Phys. Condens. Matter 2009, 21, 395502. [Google Scholar] [CrossRef] [PubMed]
- Giannozzi, P.; Andreussi, O.; Brumme, T.; Bunau, O.; Nardelli, M.B.; Calandra, M.; Car, R.; Cavazzoni, C.; Ceresoli, D.; Cococcioni, M. Advanced capabilities for materials modelling with Quantum ESPRESSO. J. Phys. Condens. Matter 2017, 29, 465901. [Google Scholar] [CrossRef] [PubMed]
- Prandini, G.; Marrazzo, A.; Castelli, I.E.; Mounet, N.; Marzari, N. Precision and Efficiency in Solid-State Pseudopotential Calculations. npj Comput. Mater. 2018, 4, 72. [Google Scholar] [CrossRef]
- Lejaeghere, K.; Bihlmayer, G.; Björkman, T.; Blaha, P.; Blügel, S.; Blum, V.; Caliste, D.; Castelli, I.E.; Clark, S.J.; Dal Corso, A.; et al. Reproducibility in Density Functional Theory Calculations of Solids. Science 2016, 351, 6280. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Ruzsinszky, A.; Csonka, G.I.; Vydrov, O.A.; Scuseria, G.E.; Constantin, L.A.; Zhou, X.; Burke, K. Restoring the Density-Gradient Expansion for Exchange in Solids and Surfaces. Phys. Rev. Lett. 2008, 100, 136406. [Google Scholar] [CrossRef] [PubMed]
- Hubbard, J. Electron Correlations in Narrow Energy Bands. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1963, 276, 238–257. [Google Scholar] [CrossRef]
- Grimme, S.; Ehrlich, S.; Goerigk, L. Effect of the Damping Function in Dispersion Corrected Density Functional Theory. J. Comput. Chem. 2011, 32, 1456–1465. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A Consistent and Accurate Ab Initio Parametrization of Density Functional Dispersion Correction (DFT-D) for the 94 Elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- Bunn, C.W. The crystal structure of long-chain normal paraffin hydrocarbons. The “shape” of the <CH2 group. Trans. Faraday Soc. 1939, 35, 482–491. [Google Scholar] [CrossRef]
- Mikuriya, M.; Taniguchi, K.; Koyama, Y.; Watanabe, H.; Yoshioka, D.; Mitsuhashi, R.; Asato, E. Crystal Structure of S8 Molecule from Thiourea. X-ray Struct. Anal. Online 2020, 36, 1–2. [Google Scholar] [CrossRef]
- Grey, I.E.; Bordet, P.; Wilson, N.C. Structure of the Amorphous Titania Precursor Phase of N-Doped Photocatalysts. RSC Adv. 2021, 11, 8619–8627. [Google Scholar] [CrossRef]
- Sherrell, P.C.; Sharda, K.; Grotta, C.; Ranalli, J.; Sokolikova, M.S.; Pesci, F.M.; Palczynski, P.; Bemmer, V.L.; Mattevi, C. Thickness-Dependent Characterization of Chemically Exfoliated TiS2 Nanosheets. ACS Omega 2018, 3, 8655–8662. [Google Scholar] [CrossRef] [PubMed]
- Dużyńska, A.; Judek, J.; Wilczyński, K.; Zberecki, K.; Łapińska, A.; Wróblewska, A.; Zdrojek, M. Temperature-Induced Phonon Behavior in Titanium Disulfide (TiS2) Nanosheets. J. Raman Spectrosc. 2019, 50, 1114–1119. [Google Scholar] [CrossRef]
- Lin, C.; Zhu, X.; Feng, J.; Wu, C.; Hu, S.; Peng, J.; Guo, Y.; Peng, L.; Zhao, J.; Huang, J.; et al. Hydrogen-Incorporated TiS2 Ultrathin Nanosheets with Ultrahigh Conductivity for Stamp-Transferrable Electrodes. J. Am. Chem. Soc. 2013, 135, 5144–5151. [Google Scholar] [CrossRef] [PubMed]
- Shima, T.; Luo, G.; Hu, S.; Luo, Y.; Hou, Z. Experimental and Computational Studies of Dinitrogen Activation and Hydrogenation at a Tetranuclear Titanium Imide/Hydride Framework. J. Am. Chem. Soc. 2019, 141, 2713–2720. [Google Scholar] [CrossRef]
- Tang, W.; Sanville, E.; Henkelman, G. A Grid-Based Bader Analysis Algorithm without Lattice Bias. J. Phys. Condens. Matter 2009, 21, 084204. [Google Scholar] [CrossRef]
- Henkelman, G.; Arnaldsson, A.; Jónsson, H. A Fast and Robust Algorithm for Bader Decomposition of Charge Density. Comput. Mater. Sci. 2006, 36, 354–360. [Google Scholar] [CrossRef]
- Wang, Z.; Jin, J.; Zheng, Y.; Zhang, X.; Zhu, Z.; Zhou, Y.; Cui, X.; Li, J.; Shang, M.; Zhao, X.; et al. Achieving Efficient and Stable Perovskite Solar Cells in Ambient Air Through Non-Halide Engineering. Adv. Energy Mater. 2021, 11, 2102169. [Google Scholar] [CrossRef]

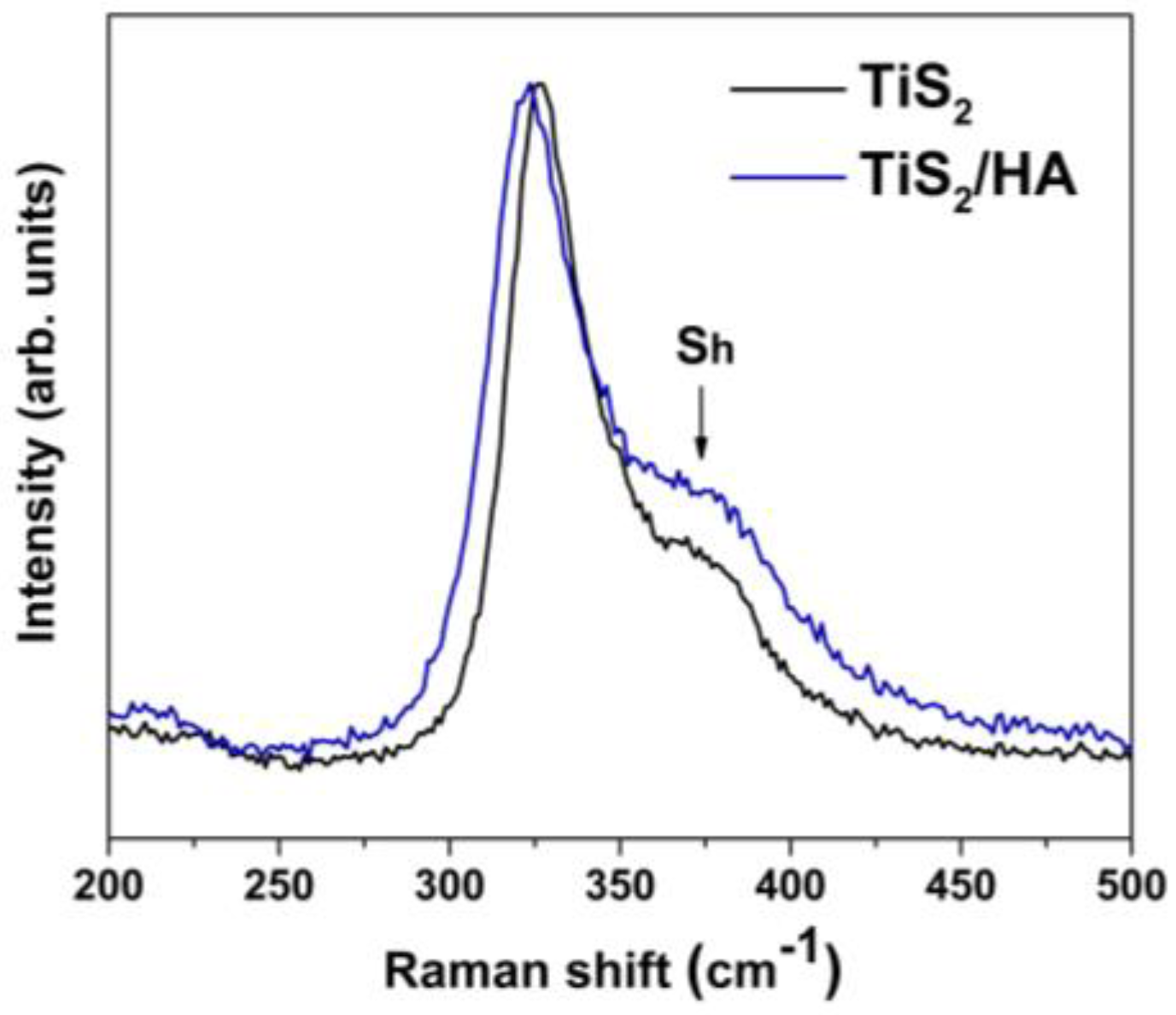
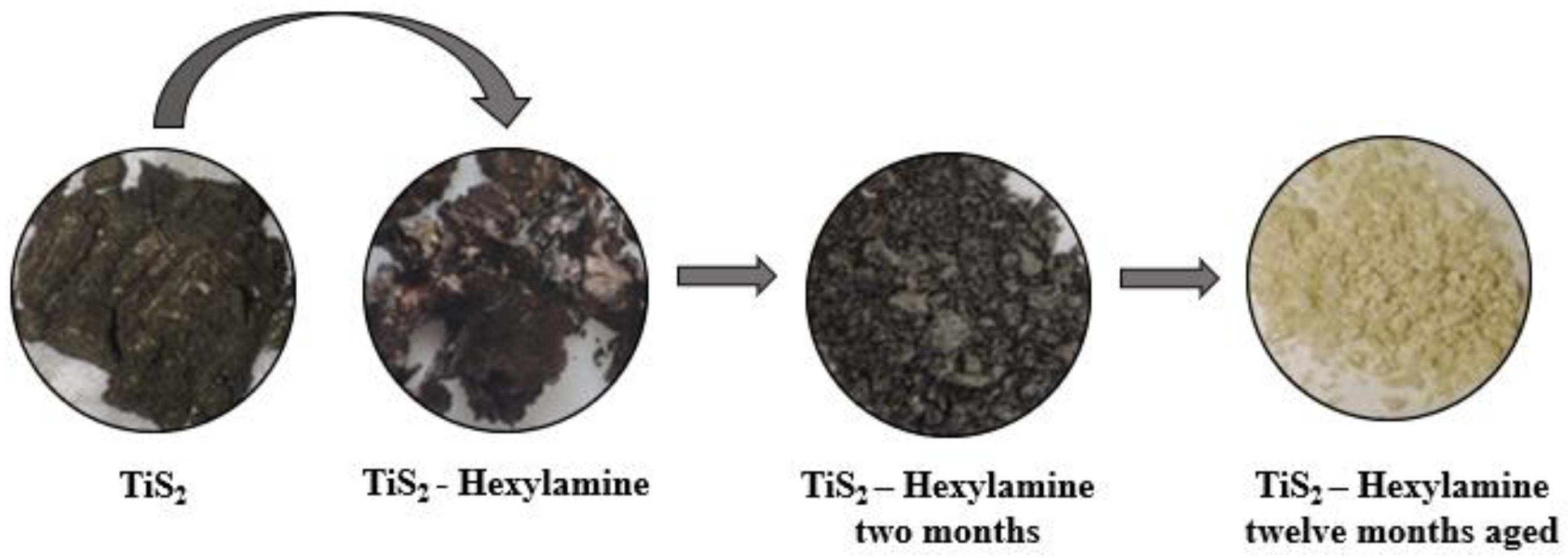

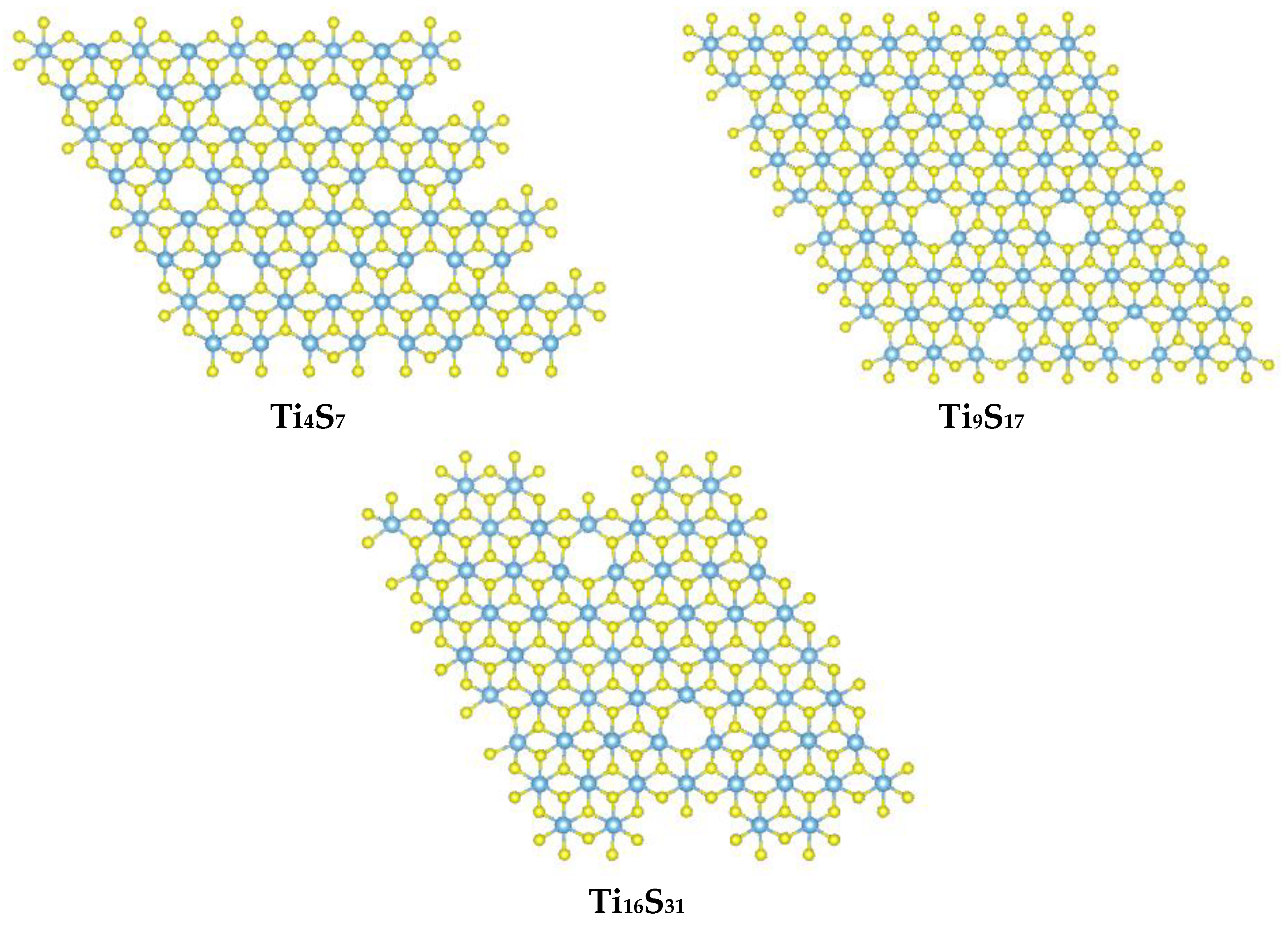
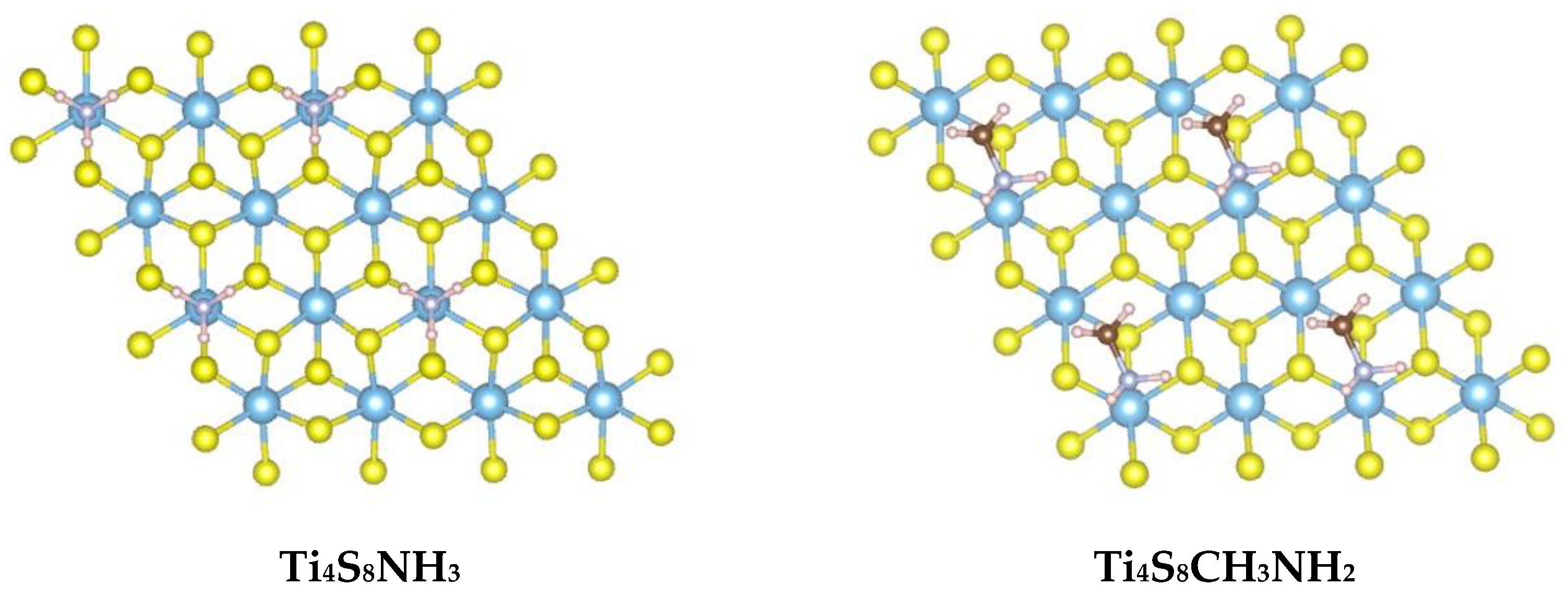
| Mode | Raman Shift (cm−1) |
|---|---|
| Eu | 162.45 |
| Eg | 233.10 |
| A1g | 321.23 |
| A2u | 361.10 |
| Reaction | ΔHr (eV) |
|---|---|
| Ti4S8 → Ti4S7 + S | 3.14 |
| Ti9S18 → Ti9S17 + S | 2.71 |
| Ti16S32 → Ti16S31 + S | 2.43 |
| Reaction | ΔHr (eV) |
|---|---|
| Ti4S8 + NH4+ + OH− → Ti4S8NH3 + H2O | −2.93 |
| Ti4S8 + CH3NH3+ + OH− → Ti4S8CH3NH2 + H2O | −1.75 |
| Reaction | ΔHr (eV) |
|---|---|
| Ti4S8NH3 → Ti4S7NH3 + S | 1.51 |
| Ti4S8NH3 → Ti4S7NH2 + S + ½ H2 | 1.47 |
| Ti4S8NH3 → Ti4S7NH + S + H2 | 1.76 |
| Ti4S8NH3 + ½ O2 → Ti4S7NH + S + H2O | −0.83 |
| Ti4S8 | Ti4S7NH3 | Ti4S7NH2 | Ti4S7NH | |
|---|---|---|---|---|
| Ti (10) | 10.23 | 10.2721 | 10.1974 | 10.1304 |
| S (8) | 6.89 | 6.9950 | 6.9530 | 6.9218 |
| N (7) | - | 6.2964 | 6.3866 | 6.4353 |
| H (1) | - | 0.5500 | 0.5760 | 0.5900 |
| Total Ti/S | 96.00 | 90.05 | 89.46 | 88.97 |
| Total ammonia | - | 7.95 | 7.54 | 7.03 |
| Total | 96.00 | 98.00 | 97.00 | 96.00 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ursi, F.; Virga, S.; Garcìa-Espejo, G.; Masciocchi, N.; Martorana, A.; Giannici, F. Long-Term Stability of TiS2–Alkylamine Hybrid Materials. Materials 2022, 15, 8297. https://doi.org/10.3390/ma15238297
Ursi F, Virga S, Garcìa-Espejo G, Masciocchi N, Martorana A, Giannici F. Long-Term Stability of TiS2–Alkylamine Hybrid Materials. Materials. 2022; 15(23):8297. https://doi.org/10.3390/ma15238297
Chicago/Turabian StyleUrsi, Federica, Simone Virga, Gonzalo Garcìa-Espejo, Norberto Masciocchi, Antonino Martorana, and Francesco Giannici. 2022. "Long-Term Stability of TiS2–Alkylamine Hybrid Materials" Materials 15, no. 23: 8297. https://doi.org/10.3390/ma15238297
APA StyleUrsi, F., Virga, S., Garcìa-Espejo, G., Masciocchi, N., Martorana, A., & Giannici, F. (2022). Long-Term Stability of TiS2–Alkylamine Hybrid Materials. Materials, 15(23), 8297. https://doi.org/10.3390/ma15238297






