High-Frequency ac Susceptibility of Iron-Based Superconductors
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
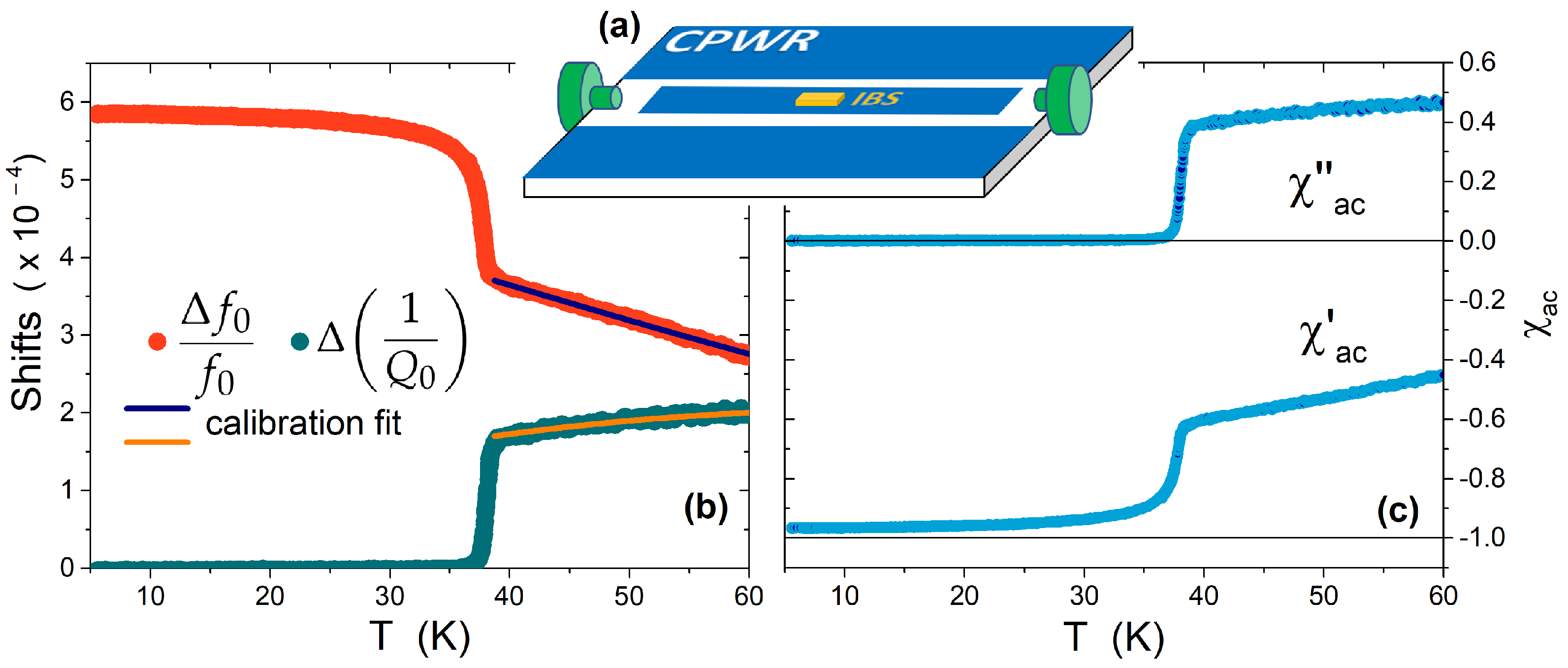
2.2. The CPWR Method
2.3. Calibration
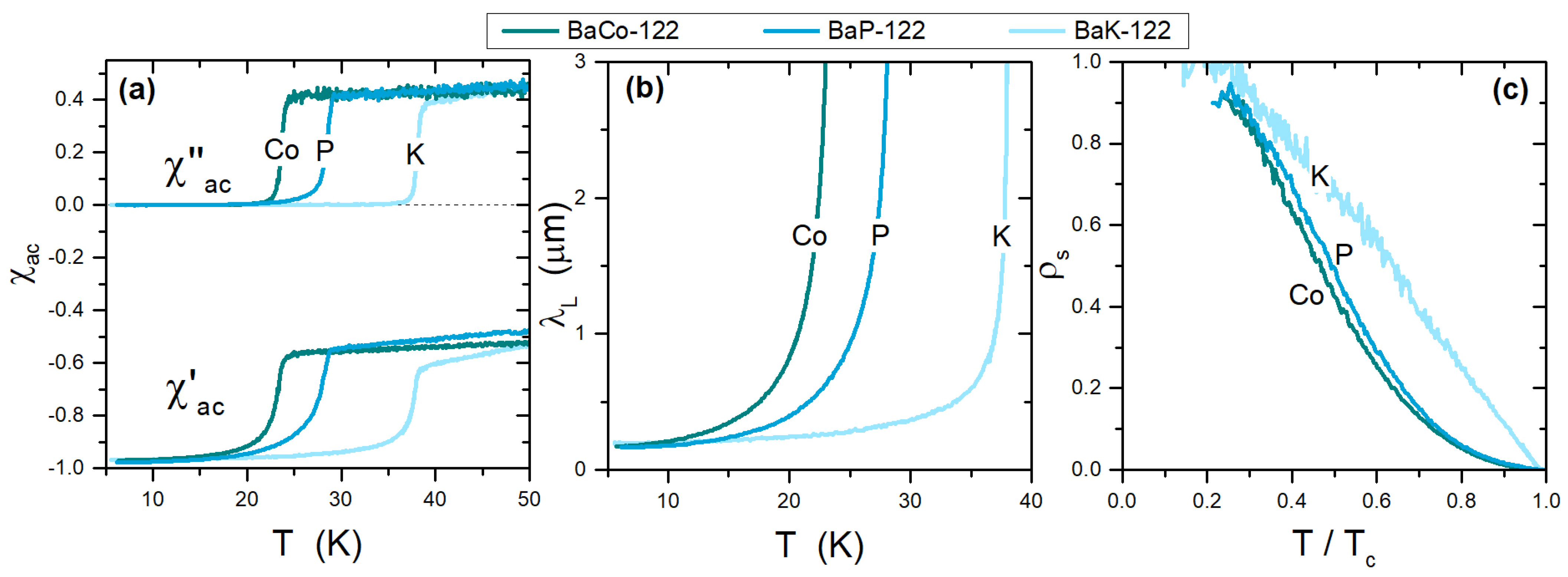
2.4. Penetration Depths and Superfluid Density
2.5. Irradiation Experiments
3. Results and Discussion
3.1. Comparison among Different IBS Families
3.2. Effects of Different Kinds of Doping in Ba-122
3.3. Effects of Proton Irradiation
3.4. Flux-Pinning Effects
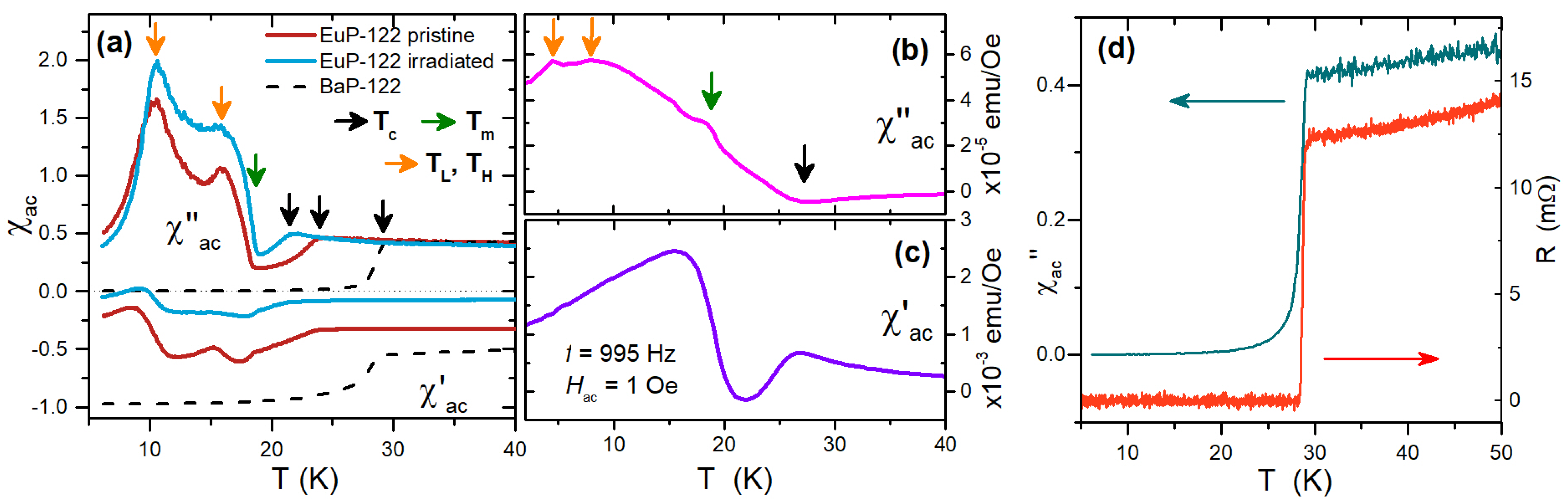
3.5. The Ferromagnetic Superconductor EuP-122
3.6. Comparison with Other Techniques
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kamihara, Y.; Hiramatsu, H.; Hirano, M.; Kawamura, R.; Yanagi, H.; Kamiya, T.; Hosono, H. Iron-Based Layered Superconductor: LaOFeP. J. Am. Chem. Soc. 2006, 128, 10012–10013. [Google Scholar] [CrossRef] [PubMed]
- Pyon, S.; Mori, H.; Tamegai, T.; Awaji, S.; Kito, H.; Ishida, S.; Yoshida, Y.; Kajitani, H.; Koizumi, N. Fabrication of small superconducting coils using (Ba,A)Fe2As2 (A: Na, K) round wires with large critical current densities. Supercond. Sci. Technol. 2021, 34, 105008. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, D.; Wei, S.; Wang, Y.; Wang, C.; Zhang, Z.; Yao, H.; Zhang, X.; Liu, F.; Liu, H.; et al. First performance test of the iron-based superconducting racetrack coils at 10 T. Supercond. Sci. Technol. 2021, 34, 035021. [Google Scholar] [CrossRef]
- Paglione, J.; Greene, R.L. High-temperature superconductivity in iron-based materials. Nat. Phys. 2010, 6, 645. [Google Scholar] [CrossRef] [Green Version]
- Mazin, I.I.; Singh, D.J.; Johannes, M.D.; Du, M.H. Unconventional Superconductivity with a Sign Reversal in the Order Parameter of LaFeAsO1-xFx. Phys. Rev. Lett. 2008, 101, 057003. [Google Scholar] [CrossRef] [Green Version]
- Chubukov, A.V.; Efremov, D.V.; Eremin, I. Magnetism, superconductivity, and pairing symmetry in iron-based superconductors. Phys. Rev. B 2008, 78, 134512. [Google Scholar] [CrossRef] [Green Version]
- Stanev, V.; Kang, J.; Tesanovic, Z. Spin fluctuation dynamics and multiband superconductivity in iron pnictides. Phys. Rev. B 2008, 78, 184509. [Google Scholar] [CrossRef] [Green Version]
- Hirschfeld, P.J.; Korshunov, M.M.; Mazin, I.I. Gap symmetry and structure of Fe-based superconductors. Rep. Prog. Phys. 2011, 74, 124508. [Google Scholar] [CrossRef] [Green Version]
- Takahashi, H.; Okada, T.; Imai, Y.; Kitagawa, K.; Matsubayashi, K.; Uwatoko, Y.; Maeda, A. Investigation of the superconducting gap structure in SrFe2(As0.7P0.3)2 by magnetic penetration depth and flux flow resistivity analysis. Phys. Rev. B 2012, 86, 144525. [Google Scholar] [CrossRef] [Green Version]
- Schilling, M.B.; Baumgartner, A.; Gorshunov, B.; Zhukova, E.S.; Dravin, V.A.; Mitsen, K.V.; Efremov, D.V.; Dolgov, O.V.; Iida, K.; Dressel, M.; et al. Tracing the s± symmetry in iron pnictides by controlled disorder. Phys. Rev. B 2016, 93, 174515. [Google Scholar] [CrossRef] [Green Version]
- Ghigo, G.; Torsello, D.; Ummarino, G.A.; Gozzelino, L.; Tanatar, M.A.; Prozorov, R.; Canfield, P.C. Disorder-Driven Transition from s± to s++ Superconducting Order Parameter in Proton Irradiated Ba(Fe1-xRhx)2As2 Single Crystals. Phys. Rev. Lett. 2018, 121, 107001. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pompeo, N.; Torokhtii, K.; Alimenti, A.; Silva, E. A method based on a dual frequency resonator to estimate physical parameters of superconductors from surface impedance measurements in a magnetic field. Measurement 2021, 184, 109937. [Google Scholar] [CrossRef]
- Silva, E.; Lezzerini, A.; Lanucara, M.; Sarti, S.; Marcon, R. A cavity system for the measurement of the surface resistance at 48 GHz in high-superconductors. Meas. Sci. Technol. 1998, 9, 275–282. [Google Scholar] [CrossRef]
- Ghigo, G.; Ummarino, G.A.; Gozzelino, L.; Gerbaldo, R.; Laviano, F.; Torsello, D.; Tamegai, T. Effects of disorder induced by heavy-ion irradiation on (Ba1-xKx)Fe2As2 single crystals, within the three-band Eliashberg s± wave model. Sci. Rep. 2017, 7, 13029. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ghigo, G.; Torsello, D.; Gerbaldo, R.; Gozzelino, L.; Laviano, F.; Tamegai, T. Effects of heavy-ion irradiation on the microwave surface impedance of (Ba1-xKx)Fe2As2 single crystals. Supercond. Sci. Technol. 2018, 31, 034006. [Google Scholar] [CrossRef]
- Chen, D.X.; Navau, C.; Del-Valle, N.; Sanchez, A. Determination of London penetration depth from ac susceptibility measurements of a square superconducting thin film. Phys. C Supercond. 2014, 500, 9–13. [Google Scholar] [CrossRef]
- Mancusi, D.; Galluzzi, A.; Pace, S.; Polichetti, M. Effects of the third harmonic demagnetization field on the ac losses of a granular FeSeTe superconductor in ac field. J. Supercond. Nov. Magn. 2018, 31, 2011–2018. [Google Scholar] [CrossRef]
- Gömöry, F. Characterization of high-temperature superconductors by AC susceptibility measurements. Supercond. Sci. Technol. 1997, 10, 523. [Google Scholar] [CrossRef]
- Polichetti, M.; Adesso, M.G.; Pace, S. Response of glass and liquid phases in the vortex lattice to an external AC magnetic field at different frequencies. Phys. A Stat. Mech. Its Appl. 2004, 339, 119–124. [Google Scholar] [CrossRef] [Green Version]
- Galluzzi, A.; Buchkov, K.; Tomov, V.; Nazarova, E.; Leo, A.; Grimaldi, G.; Pace, S.; Polichetti, M. Mixed state properties analysis in AC magnetic field of strong pinning Fe(Se,Te) single crystal. Supercond. Sci. Technol. 2020, 33, 094006. [Google Scholar] [CrossRef]
- Mancusi, D.; Galluzzi, A.; Pace, S.; Polichetti, M. Demagnetization harmonic effects on the magnetization of granular systems on a macroscopic scale: The superconducting case. J. Phys. Condens. Matter 2017, 29, 425701. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Buchkov, K.; Galluzzi, A.; Mancusi, D.; Nazarova, E.; Pace, S.; Polichetti, M. Harmonic AC magnetic susceptibility analysis of FeSe crystals with composite morphology. Phys. Scr. 2019, 94, 085804. [Google Scholar] [CrossRef]
- Taen, T.; Tsuchiya, Y.; Nakajima, Y.; Tamegai, T. Superconductivity at Tc∼14K in single-crystalline FeTe0.61Se0.39. Phys. Rev. B 2009, 80, 092502. [Google Scholar] [CrossRef] [Green Version]
- Taen, T.; Ohtake, F.; Akiyama, H.; Inoue, H.; Sun, Y.; Pyon, S.; Tamegai, T.; Kitamura, H. Pair-breaking effects induced by 3-MeV proton irradiation in Ba1-xKxFe2As2. Phys. Rev. B 2013, 88, 224514. [Google Scholar] [CrossRef]
- Nakajima, Y.; Taen, T.; Tsuchiya, Y.; Tamegai, T.; Kitamura, H.; Murakami, T. Suppression of the critical temperature of superconducting Ba(Fe1-xCox)2As2 by point defects from proton irradiation. Phys. Rev. B 2010, 82, 220504. [Google Scholar] [CrossRef]
- Park, A.; Pyon, S.; Sun, Y.; Veshchunov, I.; Chen, J.; Ito, N.; Suwa, T.; Tamegai, T.; Kitamura, H.; Ichinose, A. Quasiparticle scattering in 3 MeV proton irradiated BaFe2(As 0.67P0.33)2. Phys. Rev. B 2018, 98, 054512. [Google Scholar] [CrossRef]
- Meier, W.R.; Kong, T.; Kaluarachchi, U.S.; Taufour, V.; Jo, N.H.; Drachuck, G.; Böhmer, A.E.; Saunders, S.M.; Sapkota, A.; Kreyssig, A.; et al. Anisotropic thermodynamic and transport properties of single-crystalline CaKFe4As4. Phys. Rev. B 2016, 94, 064501. [Google Scholar] [CrossRef] [Green Version]
- Yi, X.; Li, M.; Xing, X.; Meng, Y.; Zhao, C.; Shi, Z. Single crystal growth and effects of Ni doping on the novel 12442-type iron-based superconductor RbCa2Fe4As4F2. New J. Phys. 2020, 22, 073007. [Google Scholar] [CrossRef]
- Xu, X.; Jiao, W.H.; Zhou, N.; Li, Y.K.; Chen, B.; Cao, C.; Dai, J.; Bangura, A.F.; Cao, G. Electronic nematicity revealed by torque magnetometry in EuFe2(As1-xPx)2. Phys. Rev. B 2014, 89, 104517. [Google Scholar] [CrossRef] [Green Version]
- Ghigo, G.; Laviano, F.; Gerbaldo, R.; Gozzelino, L. Tuning the response of YBCO microwave resonators by heavy-ion patterned micro-channels. Supercond. Sci. Technol. 2012, 25, 115007. [Google Scholar] [CrossRef]
- Petersan, P.J.; Anlage, S.M. Measurement of resonant frequency and quality factor of microwave resonators: Comparison of methods. J. Appl. Phys. 1998, 84, 3392–3402. [Google Scholar] [CrossRef] [Green Version]
- Chen, L.F.; Ong, C.K.; Neo, C.P.; Varadan, V.V.; Varadan, V.K. Microwave Electronics: Measurement and Materials Characterization; Wiley: Hoboken, NJ, USA, 2004. [Google Scholar]
- Ghigo, G.; Torsello, D.; Gozzelino, L.; Tamegai, T.; Veshchunov, I.S.; Pyon, S.; Jiao, W.; Cao, G.H.; Grebenchuk, S.Y.; Golovchanskiy, I.A.; et al. Microwave analysis of the interplay between magnetism and superconductivity in EuFe2(As1-xPx)2 single crystals. Phys. Rev. Res. 2019, 1, 033110. [Google Scholar] [CrossRef] [Green Version]
- Ghigo, G.; Torsello, D. Microwave Analysis of Unconventional Superconductors with Coplanar-Resonator Techniques; Springer: Berlin/Heidelberg, Germany, in press.
- Landau, L.D.; Bell, J.; Kearsley, M.; Pitaevskii, L.; Lifshitz, E.; Sykes, J. Electrodynamics of Continuous Media; Elsevier: Amsterdam, The Netherlands, 2013; Volume 8. [Google Scholar]
- Ghigo, G.; Ummarino, G.A.; Gozzelino, L.; Tamegai, T. Penetration depth of Ba1-xKxFe2As2 single crystals explained within a multiband Eliashberg s± approach. Phys. Rev. B 2017, 96, 014501. [Google Scholar] [CrossRef]
- Torsello, D.; Ummarino, G.A.; Gerbaldo, R.; Gozzelino, L.; Ghigo, G. Eliashberg Analysis of the Electrodynamic Response of Ba(Fe1-xRhx)2As2 Across the s± to s++ Order Parameter Transition. J. Supercond. Nov. Magn. 2020, 33, 2319–2324. [Google Scholar] [CrossRef]
- Torsello, D.; Ummarino, G.A.; Gozzelino, L.; Tamegai, T.; Ghigo, G. Comprehensive Eliashberg analysis of microwave conductivity and penetration depth of K-, Co-, and P-substituted BaFe2As2. Phys. Rev. B 2019, 99, 134518. [Google Scholar] [CrossRef] [Green Version]
- Torsello, D.; Ummarino, G.; Bekaert, J.; Gozzelino, L.; Gerbaldo, R.; Tanatar, M.; Canfield, P.; Prozorov, R.; Ghigo, G. Tuning the Intrinsic Anisotropy with Disorder in the CaKFe4As4 Superconductor. Phys. Rev. Appl. 2020, 13, 064046. [Google Scholar] [CrossRef]
- Wang, Z.C.; Liu, Y.; Wu, S.Q.; Shao, Y.T.; Ren, Z.; Cao, G.H. Giant anisotropy in superconducting single crystals of CsCa2Fe4As4F2. Phys. Rev. B 2019, 99, 144501. [Google Scholar] [CrossRef] [Green Version]
- Pyon, S.; Taya, S.; Kobayashi, Y.; Takahashi, A.; Li, W.; Taen, T.; Wang, T.; Mu, G.; Kitamura, H.; Ichinose, A.; et al. Critical Current Density and Vortex Dynamics in Pristine and Irradiated KCa2Fe4As4F2. Materials 2021, 14, 5283. [Google Scholar] [CrossRef]
- Torsello, D.; Cho, K.; Joshi, K.R.; Ghimire, S.; Ummarino, G.A.; Nusran, N.M.; Tanatar, M.A.; Meier, W.R.; Xu, M.; Bud’ko, S.L.; et al. Analysis of the London penetration depth in Ni-doped CaKFe4As4. Phys. Rev. B 2019, 100, 094513. [Google Scholar] [CrossRef] [Green Version]
- Torsello, D.; Piatti, E.; Ummarino, G.; Yi, X.; Xing, X.; Shi, Z.; Ghigo, G.; Daghero, D. Nodal multigap superconductivity in the anisotropic iron-based compound RbCa2Fe4As4F2. npj Quantum Mater. 2022, 7, 10. [Google Scholar] [CrossRef]
- Kim, H.; Martin, C.; Gordon, R.T.; Tanatar, M.A.; Hu, J.; Qian, B.; Mao, Z.Q.; Hu, R.; Petrovic, C.; Salovich, N.; et al. London penetration depth and superfluid density of single-crystalline Fe1+y(Te1-xSex) and Fe1+y(Te1-xSx). Phys. Rev. B 2010, 81, 180503. [Google Scholar] [CrossRef] [Green Version]
- Klein, T.; Braithwaite, D.; Demuer, A.; Knafo, W.; Lapertot, G.; Marcenat, C.; Rodière, P.; Sheikin, I.; Strobel, P.; Sulpice, A.; et al. Thermodynamic phase diagram of Fe(Se0.5Te0.5) single crystals in fields up to 28 tesla. Phys. Rev. B 2010, 82, 184506. [Google Scholar] [CrossRef] [Green Version]
- Bendele, M.; Weyeneth, S.; Puzniak, R.; Maisuradze, A.; Pomjakushina, E.; Conder, K.; Pomjakushin, V.; Luetkens, H.; Katrych, S.; Wisniewski, A.; et al. Anisotropic superconducting properties of single-crystalline FeSe0.5Te0.5. Phys. Rev. B 2010, 81, 224520. [Google Scholar] [CrossRef] [Green Version]
- Kasahara, S.; Shibauchi, T.; Hashimoto, K.; Ikada, K.; Tonegawa, S.; Okazaki, R.; Shishido, H.; Ikeda, H.; Takeya, H.; Hirata, K.; et al. Evolution from non-Fermi- to Fermi-liquid transport via isovalent doping in BaFe2(As1-xPx)2 superconductors. Phys. Rev. B 2010, 81, 184519. [Google Scholar] [CrossRef]
- Torsello, D.; Gerbaldo, R.; Gozzelino, L.; Laviano, F.; Takahashi, A.; Park, A.; Pyon, S.; Ichinose, A.; Tamegai, T.; Ghigo, G. Twofold role of columnar defects in iron based superconductors. Supercond. Sci. Technol. 2020, 33, 094012. [Google Scholar] [CrossRef]
- Torsello, D.; Gozzelino, L.; Gerbaldo, R.; Tamegai, T.; Ghigo, G. Scaling laws for ion irradiation effects in iron-based superconductors. Sci. Rep. 2021, 11, 5818. [Google Scholar] [CrossRef] [PubMed]
- Balatsky, A.V.; Vekhter, I.; Zhu, J.X. Impurity-induced states in conventional and unconventional superconductors. Rev. Mod. Phys. 2006, 78, 373–433. [Google Scholar] [CrossRef] [Green Version]
- Kim, J.; Haberkorn, N.; Graf, M.J.; Usov, I.; Ronning, F.; Civale, L.; Nazaretski, E.; Chen, G.F.; Yu, W.; Thompson, J.D.; et al. Magnetic penetration-depth measurements of a suppressed superfluid density of superconducting Ca0.5Na0.5Fe2As2 single crystals by proton irradiation. Phys. Rev. B 2012, 86, 144509. [Google Scholar] [CrossRef] [Green Version]
- Torsello, D.; Gerbaldo, R.; Gozzelino, L.; Tanatar, M.A.; Prozorov, R.; Canfield, P.C.; Ghigo, G. Electrodynamic response of Ba (Fe1-xRhx)2As2 across the s± to s++ order parameter transition. Eur. Phys. J. Spec. Top. 2019, 228, 719–723. [Google Scholar] [CrossRef]
- Pompeo, N.; Torokhtii, K.; Alimenti, A.; Sylva, G.; Braccini, V.; Silva, E. Pinning properties of FeSeTe thin film through multifrequency measurements of the surface impedance. Supercond. Sci. Technol. 2020, 33, 114006. [Google Scholar] [CrossRef]
- Nowik, I.; Felner, I.; Ren, Z.; Cao, G.H.; Xu, Z.A. Coexistence of ferromagnetism and superconductivity: Magnetization and Mössbauer studies of EuFe2(As1-xPx)2. J. Phys. Condens. Matter 2011, 23, 065701. [Google Scholar] [CrossRef] [PubMed]
- Pogrebna, A.; Mertelj, T.; Vujičić, N.; Cao, G.; Xu, Z.; Mihailovic, D. Coexistence of ferromagnetism and superconductivity in iron based pnictides: A time resolved magnetooptical study. Sci. Rep. 2015, 5, 7754. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Veshchunov, I.; Vinnikov, L.Y.; Stolyarov, V.; Zhou, N.; Shi, Z.; Xu, X.; Grebenchuk, S.Y.; Baranov, D.S.; Golovchanskiy, I.; Pyon, S.; et al. Visualization of the magnetic flux structure in phosphorus-doped EuFe2As2 single crystals. JETP Lett. 2017, 105, 98–102. [Google Scholar] [CrossRef] [Green Version]
- Stolyarov, V.S.; Veshchunov, I.S.; Grebenchuk, S.Y.; Baranov, D.S.; Golovchanskiy, I.A.; Shishkin, A.G.; Zhou, N.; Shi, Z.; Xu, X.; Pyon, S.; et al. Domain Meissner state and spontaneous vortex-antivortex generation in the ferromagnetic superconductor EuFe2(As0.79P0.21)2. Sci. Adv. 2018, 4, eaat1061. [Google Scholar] [CrossRef] [Green Version]
- Vinnikov, L.Y.; Veshchunov, I.S.; Sidel’nikov, M.S.; Stolyarov, V.S.; Egorov, S.V.; Skryabina, O.V.; Jiaod, W.; Cao, G.; Tamegai, T. Direct Observation of Vortex and Meissner Domains in a Ferromagnetic Superconductor EuFe2(As0.79P0.21)2 Single Crystal. JETP Lett. 2019, 109, 521–524. [Google Scholar] [CrossRef]
- Ghigo, G.; Torsello, D.; Gerbaldo, R.; Gozzelino, L.; Pyon, S.; Veshchunov, I.S.; Tamegai, T.; Cao, G.H. Effects of proton irradiation on the magnetic superconductor EuFe2(As 1-xPx)2. Supercond. Sci. Technol. 2020, 33, 094011. [Google Scholar] [CrossRef]




| Material | x | Label | Preparation Method | Ref. |
|---|---|---|---|---|
| FeSeTe | 0.61 | SeTe-11 | Slow cooling—low T annealing | [23] |
| BaKFeAs | 0.42 | BaK-122 | FeAs self-flux | [24] |
| Ba(FeCo)As | 0.075 | BaCo-122 | FeAs/CoAs self-flux | [25] |
| BaFe(AsP) | 0.33 | BaP-122 | BaAs/BaP flux | [26] |
| CaKFeAs | − | CaK-1144 | High-T solution growth | [27] |
| RbCaFeAsF | − | Rb-12442 | RbAs self-flux | [28] |
| EuFe(AsP) | 0.20 | EuP-122 | Self-flux | [29] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghigo, G.; Fracasso, M.; Gerbaldo, R.; Gozzelino, L.; Laviano, F.; Napolitano, A.; Cao, G.-H.; Graf, M.J.; Prozorov, R.; Tamegai, T.; et al. High-Frequency ac Susceptibility of Iron-Based Superconductors. Materials 2022, 15, 1079. https://doi.org/10.3390/ma15031079
Ghigo G, Fracasso M, Gerbaldo R, Gozzelino L, Laviano F, Napolitano A, Cao G-H, Graf MJ, Prozorov R, Tamegai T, et al. High-Frequency ac Susceptibility of Iron-Based Superconductors. Materials. 2022; 15(3):1079. https://doi.org/10.3390/ma15031079
Chicago/Turabian StyleGhigo, Gianluca, Michela Fracasso, Roberto Gerbaldo, Laura Gozzelino, Francesco Laviano, Andrea Napolitano, Guang-Han Cao, Michael J. Graf, Ruslan Prozorov, Tsuyoshi Tamegai, and et al. 2022. "High-Frequency ac Susceptibility of Iron-Based Superconductors" Materials 15, no. 3: 1079. https://doi.org/10.3390/ma15031079
APA StyleGhigo, G., Fracasso, M., Gerbaldo, R., Gozzelino, L., Laviano, F., Napolitano, A., Cao, G.-H., Graf, M. J., Prozorov, R., Tamegai, T., Shi, Z., Xing, X., & Torsello, D. (2022). High-Frequency ac Susceptibility of Iron-Based Superconductors. Materials, 15(3), 1079. https://doi.org/10.3390/ma15031079










