Current Research and Challenges in Bitumen Emulsion Manufacturing and Its Properties
Abstract
:1. Introduction
2. Bitumen Emulsion Composition and Classification
2.1. Overview
2.2. Bitumen
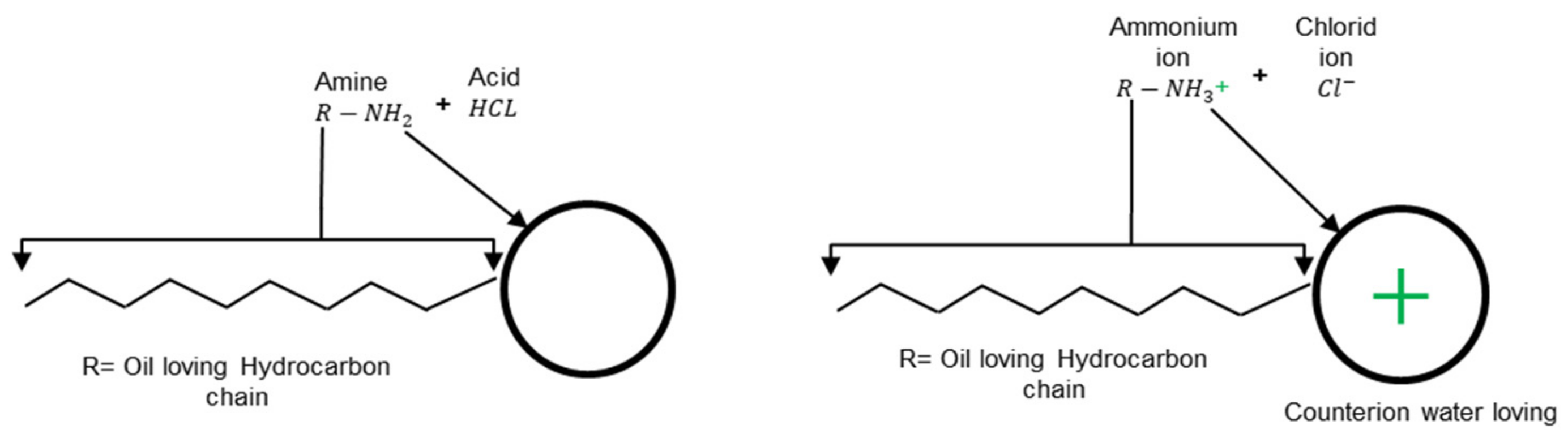
2.3. Surfactant
2.4. Bitumen Emulsion Classification
3. Bitumen Emulsion Properties
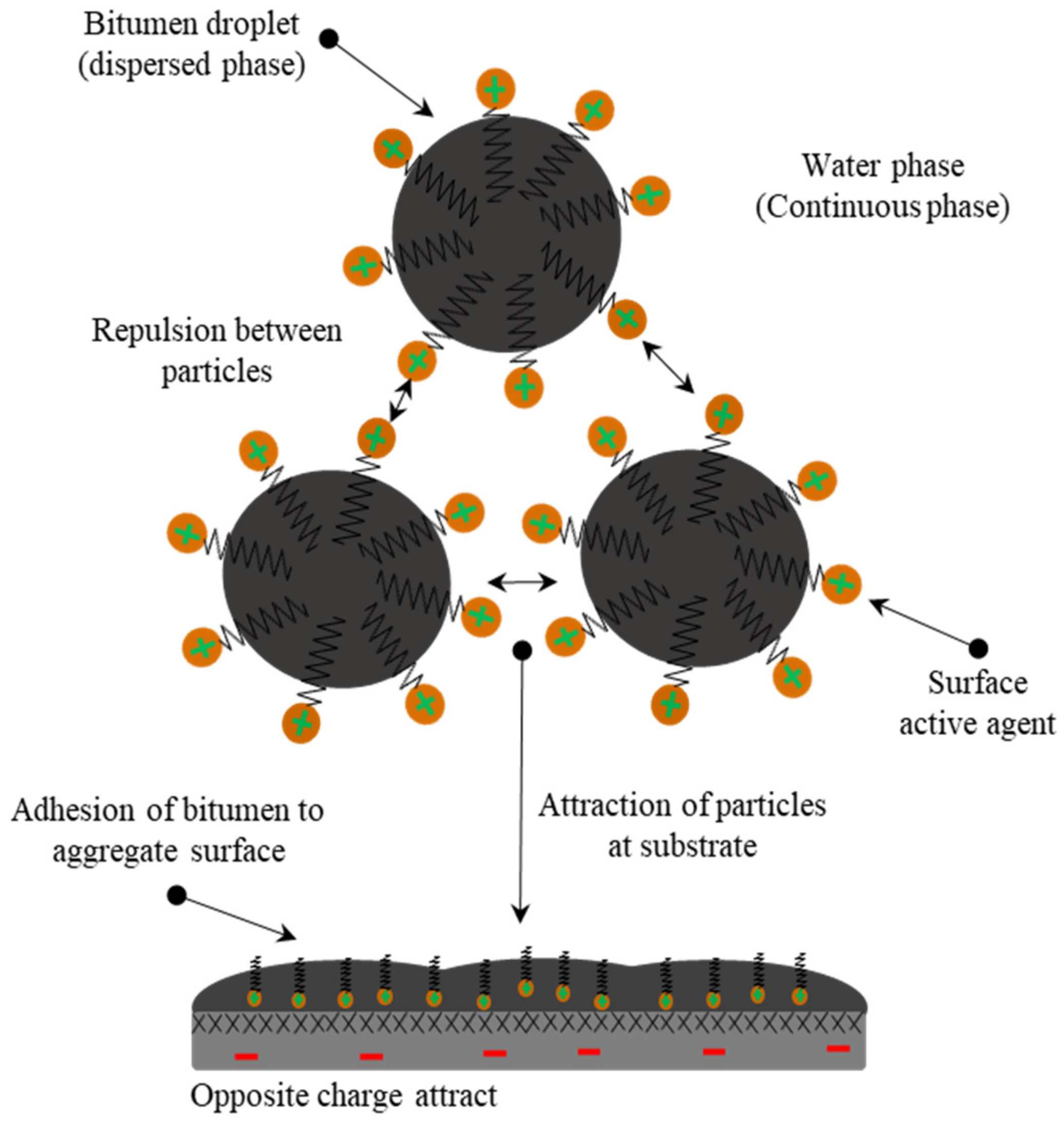
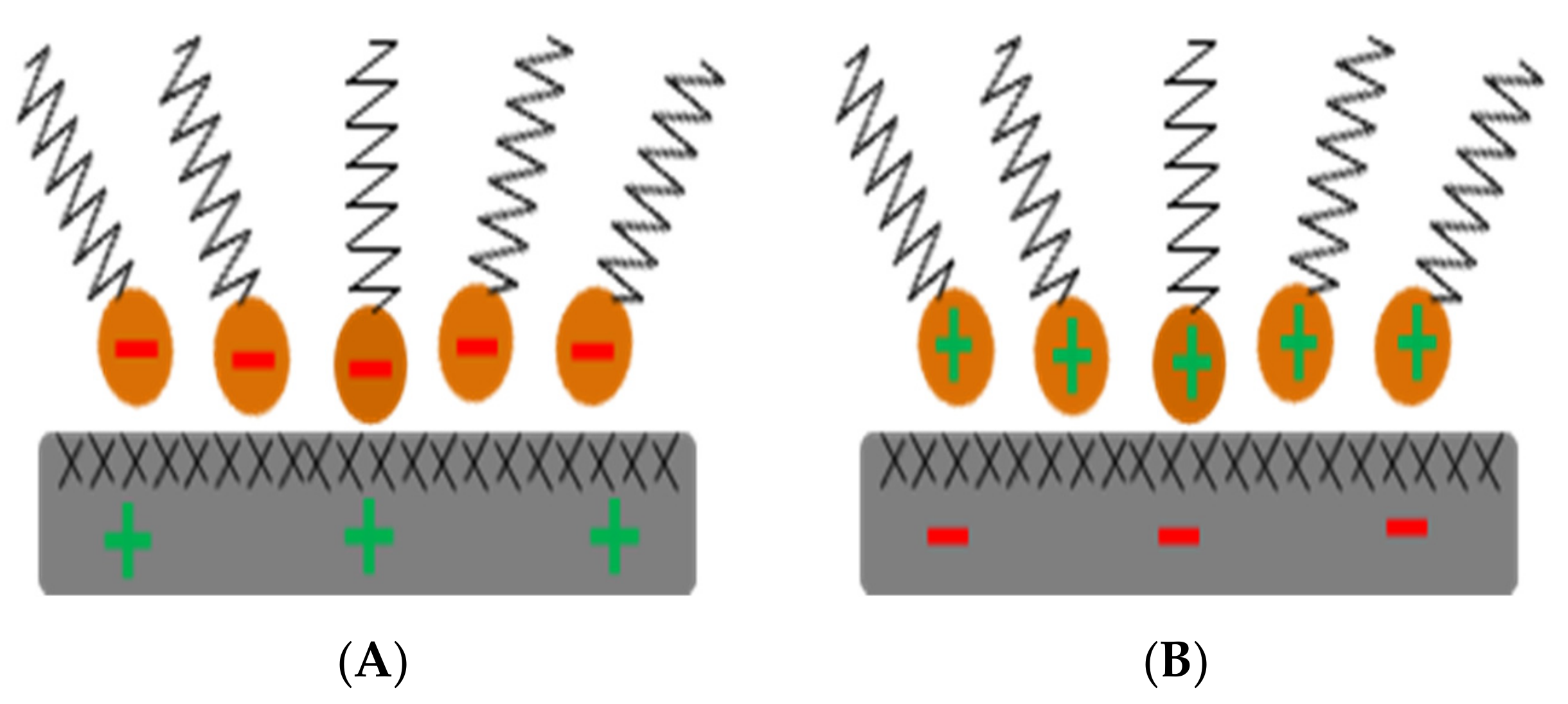
3.1. Bitumen Emulsion Stability
3.2. Bitumen Emulsions Viscosity
3.3. Bitumen Emulsion Breaking
3.3.1. Surfactant Adsorption Breaking Mechanism
3.3.2. Water Evaporation Breaking Mechanism
3.3.3. pH Change Breaking Mechanism
3.4. Bitumen Emulsion Adhesivity
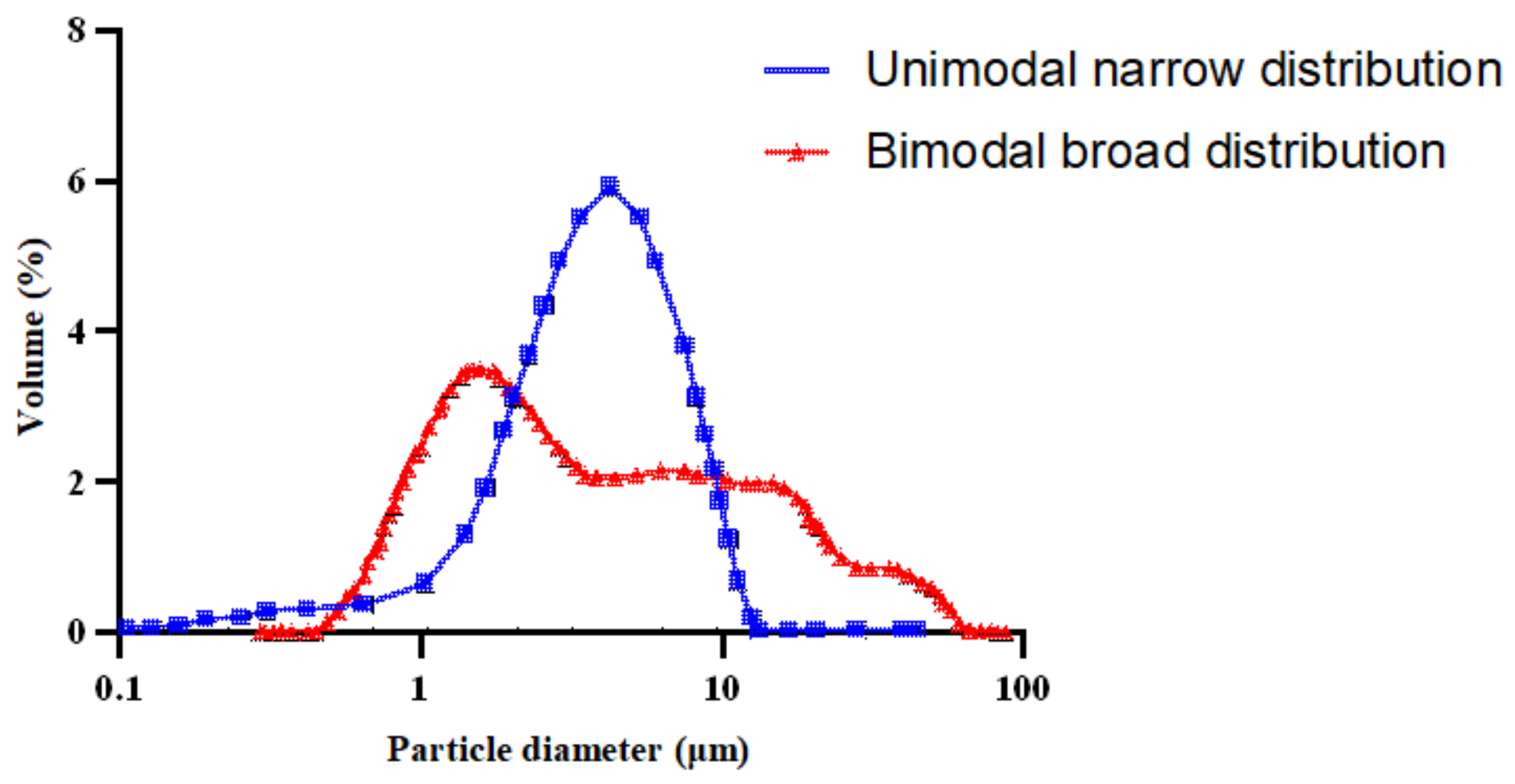
3.5. Droplet Size and Droplet Size Distribution
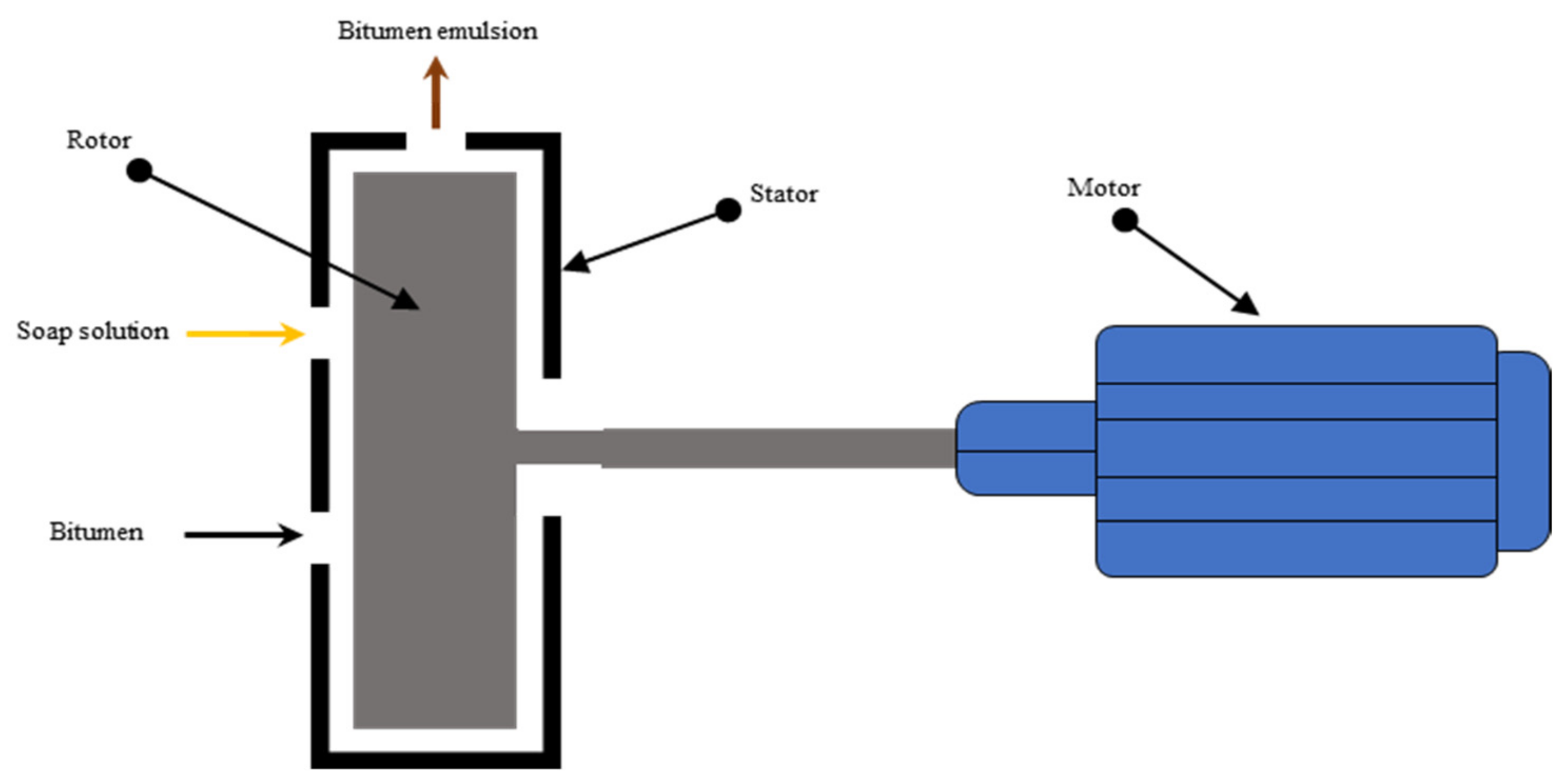
4. Bitumen Emulsion Manufacture
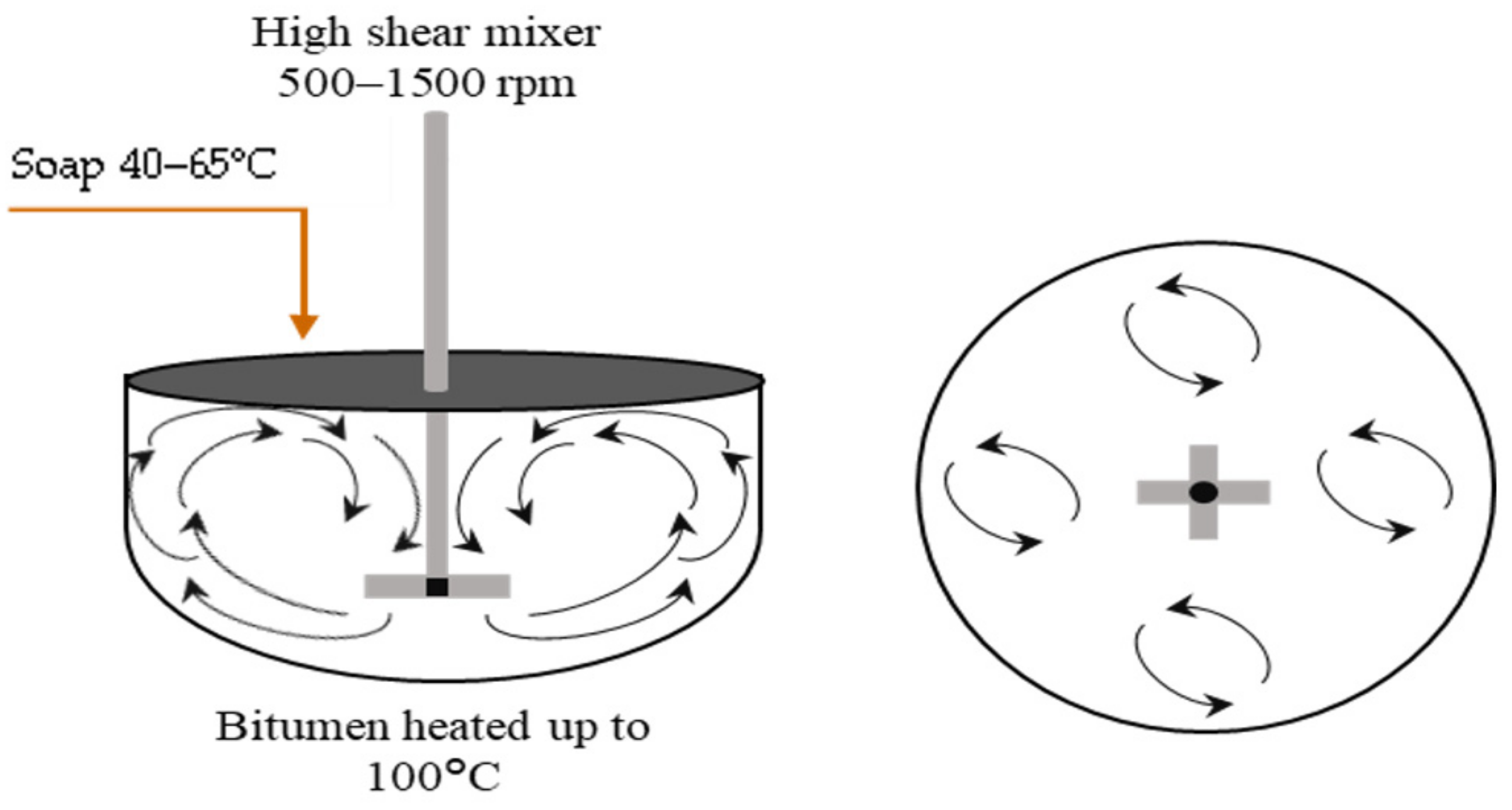
5. Emulsification Temperature
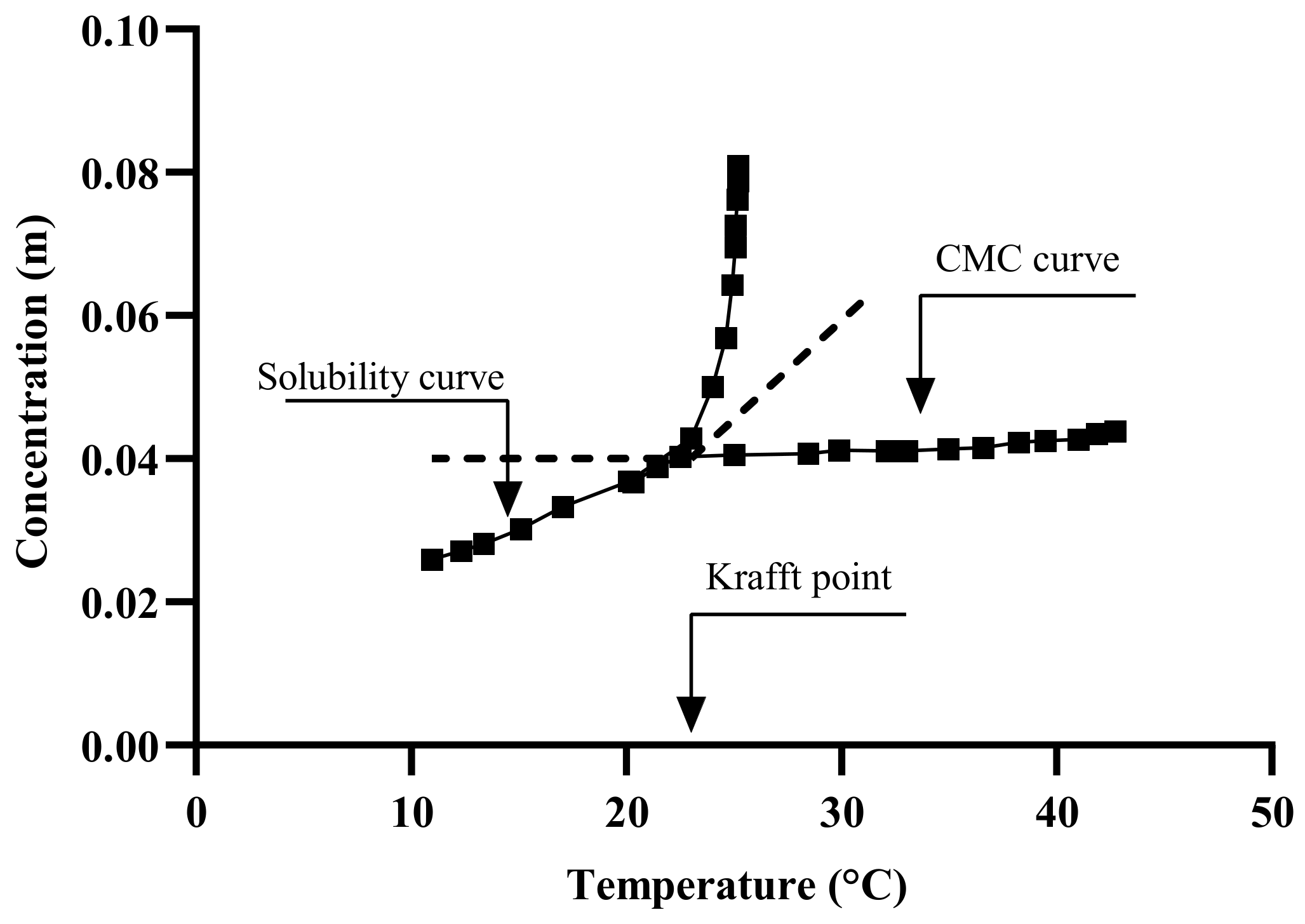
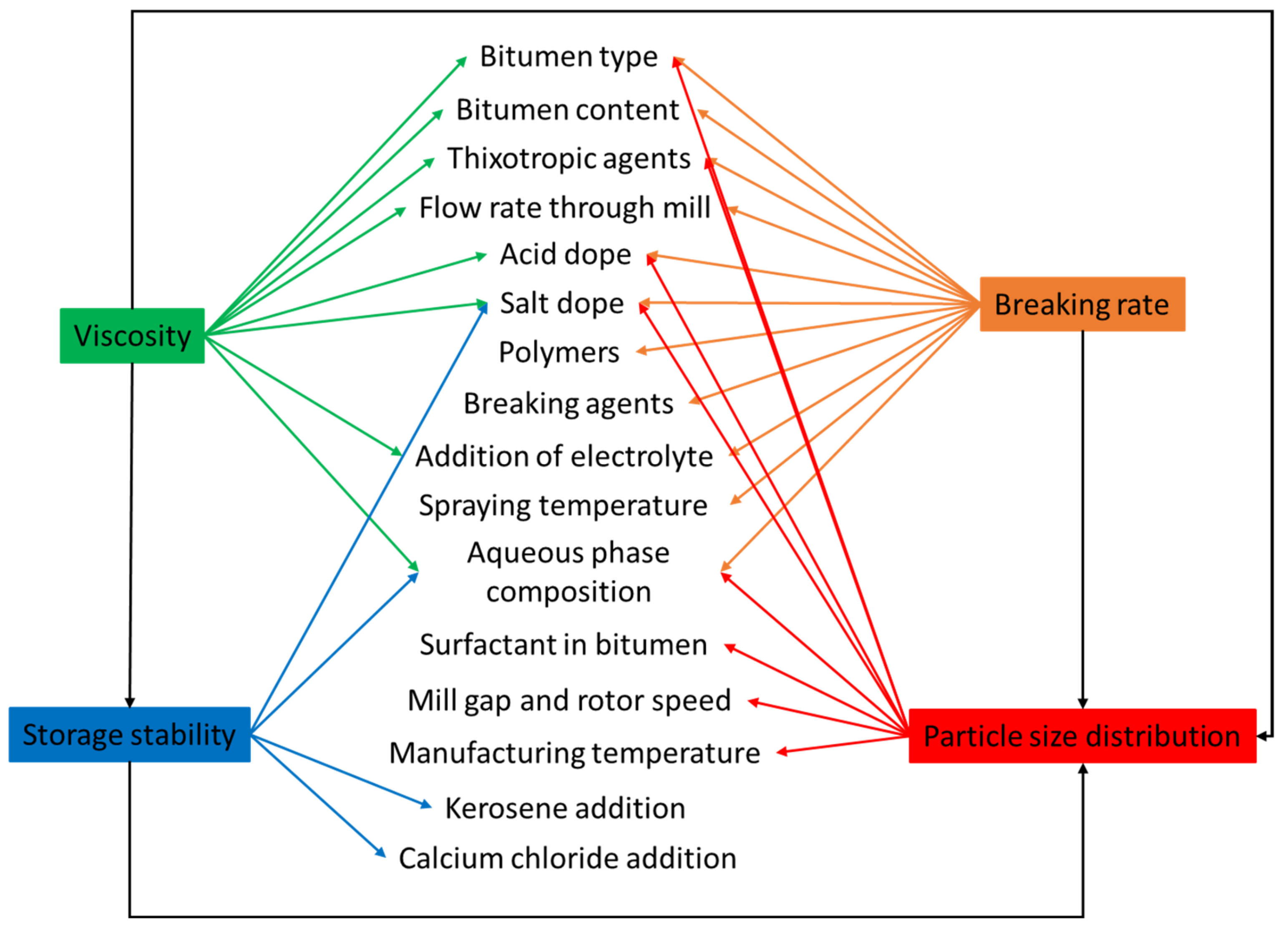
6. Bitumen Emulsion Formulation-Related Parameters
7. Bitumen Emulsion Formulation Tools
7.1. The Hydrophilic–Lipophilic Balance (HLB) Concept
7.2. Winsor’s R-Ratio
7.3. The Phase Inversion Temperature (PIT) Concept
7.4. The Cohesive Energy Ratio (CER) Concept
7.5. Hydrophilic–Lipophilic Deviation (HLD)
7.5.1. Terms Characterizing for HLD Equation
7.5.2. HLD Emulsion Formulation Diagram
8. Conclusions
- The physicochemical formulation of the surfactant-bitumen-water system is discussed extensively.
- Bitumen emulsification processes were identified as a colloid mill and the HIPR method. However, the colloid mill method could not be used for stiffer bitumen, while the HIPR approach may produce a concentrated emulsion with an average droplet size of 1 μm.
- In the formulation process, several parameters, such as materials fractions, mill speed, pH of soap phase, and using the HIPR method, can have a significant impact on specific emulsion properties such as droplet size distribution.
- Bitumen aggregate affinity can be improved by optimizing bitumen emulsion production parameters. For example, adding some salt will increase the viscosity, which in turn affects the adhesion work between the aggregate and the bitumen.
- When utilizing fatty acids or amine ionic surfactants, formulation acidity dependence is a critical parameter to consider (using HCl or NaCl for soap preparation).
- This comprehensive review gathered scientific literature to help in producing bitumen emulsion with the required properties for specific applications using scientific and pre-established approaches. Various formulation tools were presented and reviewed. HLD could be used to formulate and analyze the bitumen emulsification process, as well as anticipate the resulting emulsion characteristics, which can offer an insight into pavement performance to the mix designer. This can be helpful to use the ultimate potential of bitumen emulsion.
- Although some theoretical formulation tools have been described in this paper, experimental work is required to initiate the use of these theories such as salinity scans, perturbation experiments, etc.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nikolaides, A.F. Bituminous Mixtures & Pavements VI; CRC Press: London, UK, 2015. [Google Scholar] [CrossRef]
- Autelitano, F.; Giuliani, F. Analytical assessment of asphalt odor patterns in hot mix asphalt production. J. Clean. Prod. 2018, 172, 1212–1223. [Google Scholar] [CrossRef]
- Gorman, J.L.; Crawford, R.J.; Harding, I.H. Bitumen emulsions in road construction—A review. Road Transp. Res. 2004, 13, 25. [Google Scholar]
- Read, J.; Whiteoak, D. The Shell Bitumen Handbook; Thomas Telford: London, UK, 2003. [Google Scholar] [CrossRef]
- James, A. Overview of asphalt emulsion. In Asphalt Emulsion Technology; Transportation Research Board: Washington, DC, USA, 2006; pp. 1–15. [Google Scholar]
- Brennan, M.J.; O’Flaherty, C.A. Materials used in road pavements. In Highways; CRC Press: London, UK, 2002; 118p. [Google Scholar]
- Hunter, R.N. Bituminous Mixtures in Road Construction; Thomas Telford: London, UK, 1994. [Google Scholar]
- Simpson, W.C.; Griffin, R.L.; Miles, T.K. Relationship of asphalt properties to chemical constitution. J. Chem. Eng. Data 1961, 6, 426–429. [Google Scholar] [CrossRef]
- Girdler, R.B. Constitution of asphaltenes and related studies. Assoc. Asphalt Paving Technol. Proc. 1965, 34, 45–79. [Google Scholar]
- Salou, M.; Siffert, B.; Jada, A. Interfacial characteristics of petroleum bitumens in contact with acid water. Fuel 1998, 77, 343–346. [Google Scholar] [CrossRef]
- Al-Sabagh, A.M.; Zaki, N.N.; Badawi, A.M. Effect of binary surfactant mixtures on the stability of asphalt emulsions. J. Chem. Technol. Biotechnol. 1997, 69, 350–356. [Google Scholar] [CrossRef]
- Lu, X.; Sjövall, P.; Soenen, H. Structural and chemical analysis of bitumen using time-of-flight secondary ion mass spectrometry (TOF-SIMS). Fuel 2017, 199, 206–218. [Google Scholar] [CrossRef]
- Lesueur, D. The colloidal structure of bitumen: Consequences on the rheology and on the mechanisms of bitumen modification. Adv. Colloid Interface Sci. 2009, 145, 42–82. [Google Scholar] [CrossRef]
- Jada, A.; Salou, M. Effects of the asphaltene and resin contents of the bitumens on the water–bitumen interface properties. J. Pet. Sci. Eng. 2002, 33, 185–193. [Google Scholar] [CrossRef]
- Corbett, L.W. Composition of asphalt based on generic fractionation, using solvent deasphaltening, elution-adsorption chromatography, and densimetric characterization. Anal. Chem. 1969, 41, 576–579. [Google Scholar] [CrossRef]
- Ur-Rahman, S.; Dwivedi, M.C. Preparation of anionic asphalt emulsion by partial sulphonation. Pet. Sci. Technol. 1997, 15, 715–727. [Google Scholar] [CrossRef]
- Gibb, J.M. Evaluation of Resistance to Permanent in the Design of Bituminous Mixtures. Ph.D. Thesis, University of Nottingham, Nottingham, UK, 1996. [Google Scholar]
- Sjoblom, J. Emulsions and Emulsion Stability: Surfactant Science; Series/61; CRC Press: London, UK, 2005. [Google Scholar] [CrossRef]
- Myers, D. Surfactant Science and Technology; John Wiley & Sons: Hoboken, NJ, USA, 2020. [Google Scholar]
- Salou, M.; Siffert, B.; Jada, A. Study of the stability of bitumen emulsions by application of DLVO theory. Colloids Surf. A 1998, 142, 9–16. [Google Scholar] [CrossRef]
- Schilling, P. Emulsifiers for Bituminous Emulsions. U.S. Patent No. 4,547,224, 15 October 1985. [Google Scholar]
- Ignatavicius, S.; Kavanagh, A.; Colleran, D.; Brennan, M.; Newell, S. The use Anionic Bitumen Emulsions in Pavements—A state of the art review. In Proceedings of the 7th Eurasphalt and Eurobitume Congress, Online, 15–17 June 2021. [Google Scholar]
- Schilling, P.; Crews, E. Anionic Bituminous Emulsions with Improved Adhesion. U.S. Patent No. 4990590A, 30 June 1998. [Google Scholar]
- Schilling, P.; Schreuders, H.G. Viscosifiers for Asphalt Emulsions. U.S. Patent No. 4859245, 22 August 1989. [Google Scholar]
- Egan, R.R. The preparation and properties of amines and cationic surfactants from fatty acids. J. Am. Oil Chem. Soc. 1968, 45, 481–486. [Google Scholar] [CrossRef]
- EN 13808:2013; Bitumen and Bituminous Binders—Framework for Specifying Cationic Bituminous Emulsions. European Comission: Brussels, Belgium, 2013.
- Forschungsgesellschaft für Straßen- und Verkehrswessen (FGSV). Technische Lieferbedingungen für Bitumenemulsionen TL BE-StB 15. 2015. Available online: https://www.fgsv-verlag.de/tl-be-stb (accessed on 4 December 2021).
- Kilpatrick, P.K. Water-in-crude oil emulsion stabilization: Review and unanswered questions. Energy Fuels 2012, 26, 4017–4026. [Google Scholar] [CrossRef]
- Schuster, D. Encyclopedia of Emulsion Technology; CRC Press: London, UK, 1996. [Google Scholar]
- Braham, A. Introduction to asphalt emulsions (certificate 1). In Pavement Maintenance and Rehabilitation Professional Development Courses; University of Arkansas: Fayetteville, AR, USA, 2017. [Google Scholar]
- Sjoblom, J. Encyclopedic Handbook of Emulsion Technology; CRC Press: London, UK, 2001; 736p, Available online: https://books.google.com/books/about/Encyclopedic_Handbook_of_Emulsion_Techno.html?hl=de&id=Q4wwWbQTivUC (accessed on 7 July 2021).
- Urbina-Villalba, G. An algorithm for emulsion stability simulations: Account of flocculation, coalescence, surfactant adsorption and the process of ostwald ripening. Int. J. Mol. Sci. 2009, 10, 761–804. [Google Scholar] [CrossRef] [Green Version]
- Pinto, I.; Buss, A. ζ Potential as a measure of asphalt emulsion stability. Energy Fuels 2020, 34, 2143–2151. [Google Scholar] [CrossRef]
- Furlong, S.; James, A.; Kalinowski, E.; Thompson, M. Water enclosed within the droplets of bitumen emulsions and its relation to viscosity changes during storage. Colloids Surf. A 1999, 152, 147–153. [Google Scholar] [CrossRef]
- Boussad, K.; Lasseur, C.; Lombardi, B.K. La distribution granulométrique des émulsions bitume: Un paramètre clé pour ajuster leur viscosité. Rev. Gen. 1995, 1, 72–73. [Google Scholar]
- Sherman, P. Industrial rheology with particular reference to foods, pharmaceuticals, and cosmetics. In Industrial Rheology with Particular Reference to Foods, Pharmaceuticals, and Cosmetics; Academic Press: Cambridge, MA, USA, 1970. [Google Scholar]
- Lesueur, D.; Ezzarougui, M.; Hervé, G.; Odie, L. The Rheological Properties of Bitumen Emulsions Part II—Experimental Characterization. Road Mater. Pavement Des. 2003, 4, 169–184. [Google Scholar]
- Tausk, R.J.M.; Wilson, P.N. Colloid chemical studies on bitumen-in-water emulsions part I. Absorption of water in the bitumen droplets and other factors affecting emulsion viscosity. Colloids Surf. 1981, 2, 71–80. [Google Scholar] [CrossRef]
- Vladisavljevic, G.T.; Schubert, H. Preparation and analysis of oil-in-water emulsions with a narrow droplet size distribution using Shirasu-porous-glass (SPG) membranes. Desalination 2002, 144, 167–172. [Google Scholar] [CrossRef]
- Rámirez, M.; Bullón, J.; Andérez, J.; Mira, I.; Salager, J.-L. Drop size distribution bimodality and its effect on O/W emulsion viscosity. J. Dispers. Sci. Technol. 2002, 23, 309–321. [Google Scholar] [CrossRef]
- Alade, O.S.; Mahmoud, M.; Al Shehri, D.; Mokheimer, E.M.A.; Sasaki, K.; Ohashi, R.; Kamal, M.S.; Muhammad, I.; Al-Nakhli, A. Experimental and numerical studies on production scheme to improve energy efficiency of bitumen production through insitu oil-in-water (O/W) emulsion. Energy 2021, 244, 122700. [Google Scholar] [CrossRef]
- Salager, J.L. Surfactant-oil-water systems near the affinity inversion part II: Viscosity of emulsified systems. J. Dispers. Sci. Technol. 1983, 4, 161–173. [Google Scholar] [CrossRef]
- Samanos, J. Method for Preparing a Bitumen Emulsion, Corresponding Bitumen Emulsion and Use Thereof. U.S. Patent No. 6576050B1, 10 June 2003. [Google Scholar]
- Gorman, J.L.; Crawford, R.J.; Stannard, P.; Harding, I.H. The role of aggregate surface chemistry in bitumen emulsion—Aggregate interactions. Road Transp. Res. 1998, 7, 3. [Google Scholar]
- Hagen, A.P.; Lee, W.D.; Jones, T.M. Asphalt-aggregate interactions characterized by zeta potential and retained strength measurements for natural and organosilane-treated aggregates. Transp. Res. Rec. 1996, 1535, 111–116. [Google Scholar] [CrossRef]
- Lyklema, J. Adsorption at solid-liquid interfaces with special reference to emulsion systems. Colloids Surf. A 1994, 91, 25–38. [Google Scholar] [CrossRef]
- Castillo, G.L.; Poirier, P.A.J.; Bourrell, M. Breaking of an asphalt emulsion on a mineral aggregate: Phenomenology, modelling and optimization. In Proceedings of the First World Congress on Emulsion, Paris, France, 19–22 October 1993; pp. 19–22. [Google Scholar]
- Rodríguez-Valverde, M.A.; Ramón-Torregrosa, P.A.; Páez-Dueñas, M.A.; Cabrerizo-Vílchez, M.A.; Hidalgo-Álvarez, R. Imaging techniques applied to characterize bitumen and bituminous emulsions. Adv. Colloid Interface Sci. 2008, 136, 93–108. [Google Scholar] [CrossRef]
- Needham, D. Developments in Bitumen Emulsion Mixtures for Roads. 1996. Available online: http://eprints.nottingham.ac.uk/11101/ (accessed on 3 August 2021).
- Thanaya, I.N.A. Improving the Performance Emulsion of Cold Bituminous (CBEMS) Incorporating Waste. Ph.D. Thesis, University of Leeds, Leeds, UK, 2003. [Google Scholar]
- Poirier, J.E.; Bourrel, M.; Castillo, P.; Chambu, C.; Kbala, M. Asphalt emulsions: Experimental study of the cationic surfactant adsorption at the asphalt-water interface. Trends Colloid. Interface Sci. 1989, 3, 106–111. [Google Scholar]
- Saadoon, T.; Garcia, A.; Gómez-Meijide, B. Dynamics of water evaporation in cold asphalt mixtures. Mater. Des. 2017, 107, 2411–2502. [Google Scholar] [CrossRef]
- Al-Mohammedawi, A.; Mollenhauer, K. A study on the influence of the chemical nature of fillers on rheological and fatigue behavior of bitumen emulsion. Mastic. Mater. 2020, 13, 4627. [Google Scholar] [CrossRef]
- Shekhovtsova, S.; Korotkov, A.; Vysotskaya, M. Method of forecasting the effectiveness of cationic bitumen emulsions. Mag. Civ. Eng. 2018, 78, 91–100. [Google Scholar] [CrossRef]
- Tang, F.; Xu, G.; Ma, T.; Kong, L. Study on the effect of demulsification speed of emulsified asphalt based on surface characteristics of aggregates. Materials 2018, 11, 1488. [Google Scholar] [CrossRef] [Green Version]
- Shaw, D. Introduction to Colloid and Surface Chemistry; Buttenworth-Heinemann: Burlington, MA, USA, 1992. [Google Scholar]
- Izzi, N.; Yusoff, M.; Chailleux, E.; Airey, G.D. A comparative study of the influence of shift factor equations on master curve construction. Int. J. Pavement Res. Technol. 2011, 4, 324. [Google Scholar]
- Shi, H.; Xu, T.; Jiang, R. Combustion mechanism of four components separated from asphalt binder. Fuel 2017, 192, 18–26. [Google Scholar] [CrossRef]
- Brown, E.R.; Kandhal, P.S.; Roberts, F.L.; Kim, Y.R.; Lee, D.-Y.; Kennedy, T.W. Hot Mix Asphalt Materials, Mixture Design, and Construction; NAPA Research and Education Foundation: Lanham, MD, USA, 2009. [Google Scholar]
- Johannes, P.T. Development of an Improved Mixture Design Framework for Slurry Seals and Micro-Surfacing Treatments. Ph.D. Thesis, University of Wisconsin—Madison, Madison, WI, USA, 2014. [Google Scholar]
- Kong, L. Exploration of molecular dynamics for the adsorption of anionic emulsifier on the main chemical composition surface of aggregate. Constr. Build. Mater. 2021, 292, 123210. [Google Scholar] [CrossRef]
- Hu, S.-G.; Wang, T.; Wang, F.-Z.; Liu, Z.-C. Adsorption behaviour between cement and asphalt emulsion in cement–asphalt mortar. Adv. Cem. Res. 2009, 21, 11–14. [Google Scholar] [CrossRef]
- Loeber, L.; Mueller, G.; Héritier, B.; Jolivet, T.; Malot, M. An attempt to the understanding on the mechanism of film formation of a bituminous emulsion on mineral substrate. In Proceedings of the 2nd Eurasphalt Eurobitume Congress, Barcelona, Spain, 20–22 September 2000; Available online: https://trid.trb.org/view/674031 (accessed on 7 July 2021).
- Micaelo, R.; Guerra, A.; Quaresma, L.; Cidade, M.T. Study of the effect of filler on the fatigue behaviour of bitumen-filler mastics under DSR testing. Constr. Build. Mater. 2017, 155, 228–238. [Google Scholar] [CrossRef]
- Wu, X.; Czarnecki, J.; Hamza, N.; Masliyah, J. Interaction forces between bitumen droplets in water. Langmuir 1999, 15, 5244–5250. [Google Scholar] [CrossRef]
- Laroche, I.; Wu, X.; Masliyah, J.H.; Czarnecki, J. Dynamic and static interactions between bitumen droplets in water. J. Colloid Interface Sci. 2002, 250, 316–326. [Google Scholar] [CrossRef]
- Liu, J.; Xu, Z.; Masliyah, J. Colloidal forces between bitumen surfaces in aqueous solutions measured with atomic force microscope. Colloids Surf. A 2005, 260, 217–228. [Google Scholar] [CrossRef]
- Liu, J.; Zhou, Z.; Xu, Z.; Masliyah, J. Bitumen–clay interactions in aqueous media studied by zeta potential distribution measurement. J. Colloid Interface Sci. 2002, 252, 409–418. [Google Scholar] [CrossRef]
- Liu, J.; Xu, Z.; Masliyah, J. Studies on bitumen-silica interaction in aqueous solutions by atomic force microscopy. Langmuir 2003, 19, 3911–3920. [Google Scholar] [CrossRef]
- Liu, J.; Xu, Z.; Masliyah, J. Interaction between bitumen and fines in oil sands extraction system: Implication to bitumen recovery. Can. J. Chem. Eng. 2004, 82, 655–666. [Google Scholar] [CrossRef]
- Long, J.; Xu, Z.; Masliyah, J.H. Adhesion of single polyelectrolyte molecules on silica, mica, and bitumen surfaces. Langmuir 2006, 22, 1652–1659. [Google Scholar] [CrossRef]
- Zhao, H.; Long, J.; Masliyah, J.H.; Xu, Z. Effect of divalent cations and surfactants on silica−bitumen interactions. Ind. Eng. Chem. Res. 2006, 45, 7482–7490. [Google Scholar] [CrossRef]
- Long, J.; Zhang, L.; Xu, Z.; Masliyah, J.H. Colloidal interactions between Langmuir−Blodgett bitumen films and fine solid particles. Langmuir 2006, 22, 8831–8839. [Google Scholar] [CrossRef] [PubMed]
- Isacsson, U. A compilation of laboratory methods for studying stability of bitumen emulsions. Mater. Struct. 1985, 18, 228–236. [Google Scholar] [CrossRef]
- Ensley, E.K.; Petersen, J.C.; Robertson, R.E. Asphalt–Aggregate bonding energy measurements by microcalorimetric methods. Thermochim. Acta 1984, 77, 95–107. [Google Scholar] [CrossRef]
- Nowell, D.V.; Powell, M.W. Determination of adhesion in bitumen-mineral systems by heat-of-immersion calorimetry. J. Therm. Anal. 1991, 37, 2109–2124. [Google Scholar] [CrossRef]
- Letoffe, J.M.; Claudy, P.; Planche, J.P.; Germanaud, L. Characterization of Bitumen-Aggregate Interaction by Isothermal Microcalorimetry. Thermochim. Acta 1992, 210, 27–40. [Google Scholar] [CrossRef]
- Evans, M.B.; Nowell, D.V.; Powell, M.W. Determination of adhesion in bitumenmineral systems by heat-of-immersion calorimetry. J. Therm. Anal. 1993, 40, 121–131. [Google Scholar] [CrossRef]
- Lu, J.R. The composition and structure of sodium dodecyl sulfate-dodecanol mixtures adsorbed at the air-water interface: A neutron reflection study. J. Colloid Interface Sci. 1995, 174, 441–455. [Google Scholar] [CrossRef]
- Conboy, J.C.; Messmer, M.C.; Richmond, G.L. Effect of alkyl chain length on the conformation and order of simple ionic surfactants adsorbed at the D2O/CCl4 interface as studied by sum-frequency vibrational spectroscopy. Langmuir 1998, 14, 6722–6727. [Google Scholar] [CrossRef]
- Kunieda, H.; Ozawa, K.; Aramaki, K.; Nakano, A.; Solans, C. Formation of microemulsions in mixed ionic-nonionic surfactant systems. Langmuir 1998, 14, 260–263. [Google Scholar] [CrossRef]
- Penfold, J. Structure and composition of the mixed monolayer of hexadecyltrimethylammonium bromide and benzyl alcohol adsorbed at the air/water interface. Langmuir 1998, 2139–2144. [Google Scholar] [CrossRef]
- Penfold, J.; Staples, E.; Tucker, I.; Soubiran, L.; Creeth, A.; Hubbard, J. Adsorption of di-chain cationic and non-ionic surfactant mixtures at the air/water interface. Phys. Chem. Chem. Phys. 2000, 2, 5230–5234. [Google Scholar] [CrossRef]
- Bumajdad, A. Interfacial compositions and phase structures in mixed surfactant microemulsions. Langmuir 1999, 15, 5271–5278. [Google Scholar] [CrossRef]
- McKenna, C.E.; Knock, M.M.; Bain, C.D. First-order phase transition in mixed monolayers of hexadecyltrimethylammonium bromide and tetradecane at the air−water interface. Langmuir 2000, 16, 5853–5855. [Google Scholar] [CrossRef]
- Jin, S.R.; Zhang, K.; Pang, J.X.; Song, S.S. Synthesis and application research of op-10/cationic surfactant composite asphalt emulsifier. Appl. Mech. Mater. 2013, 364, 664–668. [Google Scholar] [CrossRef]
- Tan, Y.; Ouyang, J.; Lv, J.; Li, Y. Effect of emulsifier on cement hydration in cement asphalt mortar. Constr. Build. Mater. 2013, 47, 159–164. [Google Scholar] [CrossRef]
- Heukelom, W.; Wijga, P.W. Bitumen Testing; Koninklijke Shell Laboratorium: Amsterdam, The Netherlands, 1973. [Google Scholar]
- Majidzadeh, K.; Brovold, F.N. Effect of Water on Bitumen–Aggregate Mixtures; University of Florida: Gainesville, FL, USA, 1966. [Google Scholar]
- Baldi-Sevilla, A.; Aguiar-Moya, J.P.; Vargas-Nordcbeck, A.; Loria-Salazar, L. Effect of aggregate–bitumen compatibility on moisture susceptibility of asphalt mixtures. Road Mater. Pavement Des. 2017, 18, 318–328. [Google Scholar] [CrossRef]
- Goodarzi, F.; Zendehboudi, S. A comprehensive review on emulsions and emulsion stability in chemical and energy industries. Can. J. Chem. Eng. 2019, 97, 281–309. [Google Scholar] [CrossRef] [Green Version]
- Gupta, A.; Eral, H.B.; Hatton, T.A.; Doyle, P.S. Nanoemulsions: Formation, properties and applications. Soft Matter 2016, 12, 2826–2841. [Google Scholar] [CrossRef] [Green Version]
- Tartaro, G.; Mateos, H.; Schirone, D.; Angelico, R.; Palazzo, G. Microemulsion microstructure(s): A tutorial review. Nanomaterials 2020, 10, 1657. [Google Scholar] [CrossRef]
- Tadros, T.F. Rheology of Dispersions: Principles and Applications; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Querol, N.; Barreneche, C.; Cabeza, L.F. Method for controlling mean droplet size in the manufacture of phase inversion bituminous emulsions. Colloids Surf. A 2017, 527, 49–54. [Google Scholar] [CrossRef] [Green Version]
- Schroën, K.; de Ruiter, J.; Berton-Carabin, C. The importance of interfacial tension in emulsification: Connecting scaling relations used in large scale preparation with microfluidic measurement methods. Chem. Eng. 2020, 4, 63. [Google Scholar] [CrossRef]
- Maindarkar, S.; Dubbelboer, A.; Meuldijk, J.; Hoogland, H.; Henson, M. Prediction of emulsion drop size distributions in colloid mills. Chem. Eng. Sci. 2014, 118, 114–125. [Google Scholar] [CrossRef] [Green Version]
- Baumgardner, G.L. Asphalt emulsion manufacturing today and tomorrow. Asph. Emuls. Technol. 2006, 2006, 16–25. [Google Scholar]
- You, L.; Dai, Q.; You, Z.; Zhou, X.; Washko, S. Stability and rheology of asphalt-emulsion under varying acidic and alkaline levels. J. Clean. Prod. 2020, 256, 120417. [Google Scholar] [CrossRef]
- Liu, Z.G.; Zong, L.; Le Zhao, L.; Xie, X.M. Preparation and storage stability of asphalt emulsions made from modified lignin cationic asphalt emulsifiers. Appl. Mech. Mater. 2013, 357, 781–785. [Google Scholar] [CrossRef]
- Ouyang, J.; Sun, Y.; Zarei, S. Fabrication of solvent-free asphalt emulsion prime with high penetrative ability. Constr. Build. Mater. 2020, 230, 117020. [Google Scholar] [CrossRef]
- Gutierrez, X.; Silva, F.; Chirinos, M.; Leiva, J.; Rivas, H. Bitumen-in-water emulsions: An overview on formation, stability, and rheological properties. J. Dispers. Sci. Technol. 2002, 23, 405–418. [Google Scholar] [CrossRef]
- Hunter, R.N.; Self, A.; Read, J.; Hobson, E. The Shell Bitumen Handbook; ICE Publishing: London, UK, 2015. [Google Scholar]
- Kumasaka, J.; Ueda, R. Effect of emulsification process conditions on the properties of water-in-bitumen emulsion. J. Jpn. Assoc. Pet. Technol. 2017, 82, 74–84. [Google Scholar]
- Dresin, J.-M.; Picard, N.; Siaud, B.; Stock, C. Bitumen Emulsions, Method for Obtaining Them and Compositions Containing Same. U.S. Patent No. 6451885B1, 17 September 2002. [Google Scholar]
- Calabrese, R.V. Assessment of rotor-stator mixing devices. In Proceedings of the 17th Biennial North American Mixing Conference (Mixing XVII), Banff, AB, Canada, 15–20 August 1999. [Google Scholar]
- Tadros, T.F. Emulsion formation, stability, and rheology. In Emulsion Formation and Stability; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Gingras, J.-P.; Tanguy, P.A.; Mariotti, S.; Chaverot, P. Effect of process parameters on bitumen emulsions. Chem. Eng. Process. Process Intensif. 2005, 44, 979–986. [Google Scholar] [CrossRef]
- Asphalt Institute; Asphalt Emulsion Manufacturers Association. A Basic Asphalt Emulsion Manual; Federal Highway Administration, Department of Transportation: Wahington, DC, USA, 1997.
- Hooleran, G. Analysis of emulsion stability and asphalt compatibility. In Proceedings of the 1999 International Symposium on Asphalt Emulsion Technology, Washington, DC, USA, 11–14 November 1999. [Google Scholar]
- Lissant, K.J.; Mayhan, K.G. A study of medium and high internal phase ratio water/polymer emulsions. J. Colloid Interface Sci. 1973, 42, 201–208. [Google Scholar] [CrossRef]
- Lissant, K.J. Emulsions and emulsion technology. Soil Sci. 1975, 120, 160. [Google Scholar] [CrossRef]
- Lesueur, D.; Herrero, L.; Hurtado, J.; Potti, J.J.; Rodríguez-Valverde, M.A. Taylor-made bitumen emulsions manufacturing using the High Internal Phase Ratio method. In Proceedings of the 4th World Congress on Emulsion, Lyon, France, 3–6 October 2006; Volume 475. [Google Scholar]
- Aronson, M.P.; Petko, M.F. High Internal Phase Emulsions. U.S. Patent No. 4606913-A, 19 August 1986. [Google Scholar]
- Gingras, J.-P.; Fradette, L.; Tanguy, P.; Jorda, E. Concentrated bitumen-in-water emulsification in coaxial mixers. Ind. Eng. Chem. Res. 2007, 46, 1818–1825. [Google Scholar] [CrossRef]
- Rivas, H.; Silva, F.; Gutierrez, X.; Nunez, G. Bitumen in water bimodal emulsions stabilized by natural surfactants. J. Dispers. Sci. Technol. 2002, 23, 405–418. [Google Scholar]
- Querol, N.; Barreneche, C.; Cabeza, L.F. Storage stability of bimodal emulsions vs. monomodal emulsions. Appl. Sci. 2017, 7, 1267. [Google Scholar] [CrossRef] [Green Version]
- Arenas-Calderon, E.; Sadtler, V.; Marchal, P.; Choplin, L.; Delfosse, F.; Maze, M. Preparation of highly concentrated bitumen emulsions by catastrophic phase inversion: Follow-up of the emulsification process. Colloids Surf. A 2014, 458, 25–31. [Google Scholar] [CrossRef]
- Dicharry, C.; Diaz, J.; Torré, J.-P.; Ricaurte, M. Influence of the carbon chain length of a sulfate-based surfactant on the formation of CO2, CH4 and CO2–CH4 gas hydrates. Chem. Eng. Sci. 2016, 152, 736–745. [Google Scholar] [CrossRef] [Green Version]
- Dölle, S.; Lechner, B.; Park, J.H.; Schymura, S.; Lagerwall, J.P.F.; Scalia, G. Utilizing the Krafft phenomenon to generate ideal micelle-free surfactant-stabilized nanoparticle suspensions. Angew. Chem. Int. Ed. 2012, 51, 3254–3257. [Google Scholar] [CrossRef]
- Makavipour, F.; Pashley, R.M.; Rahman, A.F. Low-level arsenic removal from drinking water. Glob. Chall. 2019, 3, 1700047. [Google Scholar] [CrossRef] [Green Version]
- Moon, T.L.; Blanzat, M.; Labadie, L.; Perez, E.; Rico-Lattes, I. Synthesis and physicochemical study of new surfactants derived from carboxylic acid sugars. J. Dispers. Sci. Technol. 2001, 22, 167–176. [Google Scholar] [CrossRef]
- Malik, N.A.; Ali, A. Krafft temperature and thermodynamic study of interaction of glycine, diglycine, and triglycine with hexadecylpyridinium chloride and hexadecylpyridinium bromide: A conductometric approach. J. Mol. Liq. 2016, 213, 213–220. [Google Scholar] [CrossRef]
- Kunieda, H.; Shinoda, K. Krafft points, critical micelle concentrations, surface tension, and solubilizing power of aqueous solutions of fluorinated surfactants. J. Phys. Chem. 1976, 80, 2468–2470. [Google Scholar] [CrossRef]
- Islam, M.N.; Sharker, K.K.; Sarker, K.C. Salt-induced modulation of the Krafft temperature and critical micelle concentration of benzyldimethylhexadecylammonium chloride. J. Surfactants Deterg. 2015, 18, 651–659. [Google Scholar] [CrossRef]
- Roy, J.C.; Islam, M.N.; Aktaruzzaman, G. The effect of NaCl on the Krafft temperature and related behavior of cetyltrimethylammonium bromide in aqueous solution. J. Surfactants Deterg. 2014, 17, 231–242. [Google Scholar] [CrossRef]
- Lesueur, D. Polymer modified bitumen emulsions (PMBEs). In Polymer Modified Bitumen; Elsevier: Amsterdam, The Netherlands, 2011; pp. 25–42. [Google Scholar]
- Abd El-Rahman, A.M.M.; El-Shafie, M.; Abo-Shanab, Z.L.; El-Kholy, S.A. Modifying asphalt emulsion with different types of polymers for surface treatment applications. Pet. Sci. Technol. 2017, 35, 1473–1480. [Google Scholar] [CrossRef]
- Shafii, M.A.; Rahman, M.Y.A.; Ahmad, J. Polymer modified asphalt emulsion. Int. J. Civ. Environ. Eng. 2011, 11, 43–49. [Google Scholar]
- Yuan, Y.-Q.; Zou, X.-W.; Xiong, P.-F. Effects of temperature on the emulsification in surfactant-water-oil systems. Int. J. Mod. Phys. B 2003, 17, 2773–2780. [Google Scholar] [CrossRef]
- Schramm, L.L.; Stasiuk, E.N.; Marangoni, D.G. Surfactants and their applications. Annu. Rep. Sect. C 2003, 99, 3–48. [Google Scholar] [CrossRef]
- James, S.; Logaraj, A. Emulsification d’Hydrocarbures a Haut Point de Ramollissement [Emulsification of High Softening Point Hydrocarbons]. Available online: https://e-asphalt.com/wp-content/uploads/2019/04/CME-02.pdf (accessed on 29 January 2022).
- Pang, J.; Du, S.; Chang, R.; Pei, Q.; Cui, D. Effect of emulsifier content on the rheological properties of asphalt emulsion residues. J. Appl. Polym. Sci. 2015, 132, 15. [Google Scholar] [CrossRef]
- Miljković, M.; Radenberg, M.; Fang, X.; Lura, P. Influence of emulsifier content on cement hydration and mechanical performance of bitumen emulsion mortar. Mater. Struct. 2017, 50, 185. [Google Scholar] [CrossRef]
- Xiao, J.J.; Jiang, W. Study the influence of soap solution’s pH value on the modified asphalt emulsion performance. Appl. Mech. Mater. 2013, 253, 312–316. [Google Scholar] [CrossRef]
- Cui, D.; Pang, J. The effect of ph on the properties of a cationic bitumen emulsifier. Tenside Surfactants Deterg. 2017, 54, 386–392. [Google Scholar] [CrossRef]
- Boucard, L.; Schmitt, V.; Farcas, F.; Gaudefroy, V. Bitumen emulsions formulation and destabilisation process relationship: Influence of salts addition. Road Mater. Pavement Des. 2015, 16 (Suppl. S1), 330–348. [Google Scholar] [CrossRef]
- Kong, L.; Luo, W.; Feng, B.; Quan, X. Influence of emulsifier on surface mass transfer based on molecular dynamics simulations. Front. Mater. 2020, 7, 1. [Google Scholar] [CrossRef] [Green Version]
- Ziari, H.; Keymanesh, M.R.; Zalnezhad, H. Effect of emulsifying agent on rheological properties of bitumen emulsion modified with different techniques of adding SBR latex polymer. Road Mater. Pavement Des. 2020, 23, 639–655. [Google Scholar] [CrossRef]
- Griffin, W.C. Classification of surface-active agents by HLB. J. Soc. Cosmet. Chem. 1949, 1, 311–326. [Google Scholar]
- Pasquali, R.C.; Sacco, N.; Bregni, C. The studies on hydrophilic-lipophilic balance (HLB): Sixty years after William C. Griffin’s pioneer work (1949–2009). Lat. Am. J. Pharm. 2009, 28, 313–317. [Google Scholar]
- Kunieda, H.; Shinoda, K. Evaluation of the hydrophile-lipophile balance (HLB) of nonionic surfactants. I. Multisurfactant systems. J. Colloid Interface Sci. 1985, 107, 107–121. [Google Scholar] [CrossRef]
- Reham, S.S.; Masjuki, H.H.; Kalam, M.A.; Shancita, I.; Fattah, I.M.R.; Ruhul, A.M. Study on stability, fuel properties, engine combustion, performance and emission characteristics of biofuel emulsion. Renew. Sustain. Energy Rev. 2015, 52, 1566–1579. [Google Scholar] [CrossRef]
- Kondo, S. Effect of the hydrophilic-lipophilic balance (HLB) of surfactants included in the post-CMP cleaning chemicals on porous SiOC direct CMP. In Proceedings of the 2007 IEEE International Interconnect Technology Conference, Burlingame, CA, USA, 3–6 June 2007; pp. 172–174. [Google Scholar]
- Murphy, M.; Jordaan, G.J.; Modise, T.; Dryburgh, R.C. The influence of the characteristics of emulsifying agents on the stabilisation of granular materials using nano-silane modified bitumen emulsions. In Proceedings of the 12th Conference of Asphalt Pavements for Southern Africa (CAPSA 2019), Sun City, South Africa, 13–16 October 2019. [Google Scholar]
- Kunieda, H. Correlation between the formation of a lamellar liquid crystalline phase and the hydrophile-lipophile balance (HLB) of surfactants. J. Colloid Interface Sci. 1986, 114, 378–385. [Google Scholar] [CrossRef]
- Al-Sabagh, A.M. The relevance HLB of surfactants on the stability of asphalt emulsion. Colloids Surf. A 2002, 204, 73–83. [Google Scholar] [CrossRef]
- Barril, X.; Muñoz, J.; Luque, F.J.; Orozco, M. Simplified descriptions of the topological distribution of hydrophilic/hydrophobic characteristics of molecules. Phys. Chem. Chem. Phys. 2000, 2, 4897–4905. [Google Scholar] [CrossRef]
- Needs, A. The HLB SYSTEM: A Time-Saving Guide to Emulsifier Selection Time-Saving Guide to Emulsifier Selection; ICI Americas Inc.: New Castle, DE, USA, 1976. [Google Scholar]
- Gadhave, A. Determination of hydrophilic-lipophilic balance value. Int. J. Sci. Res. 2014, 3, 573–575. [Google Scholar]
- Royer, M.; Nollet, M.; Catte, M.; Collinet, M.; Pierlot, C. Towards a new universal way to describe the required hydrophilic lipophilic balance of oils using the phase inversion temperature of C10E4/n-octane/water emulsions. Colloids Surf. A 2018, 536, 165–171. [Google Scholar] [CrossRef]
- Davies, J.T. A quantitative kinetic theory of emulsion type, I. Physical chemistry of the emulsifying agent. In Gas/Liquid and Liquid/Liquid Interface, Proceedings of the 2nd International Congress of Surface Activity, London, UK, 1 January 1957; Butterworths: London, UK, 1957; Volume 1, pp. 426–438. [Google Scholar]
- Sheng, J.J. Modern Chemical Enhanced Oil Recovery: Theory and Practice; Gulf Professional Publishing: Houston, TX, USA, 2010. [Google Scholar]
- Marquez, R.; Forgiarini, A.M.; Fernández, J.; Langevin, D.; Salager, J. New interfacial rheology characteristics measured using a spinning-drop Rheometer at the optimum formulation of a simple surfactant–oil–water system. J. Surfactants Deterg. 2018, 21, 611–623. [Google Scholar] [CrossRef]
- Winsor, P.A. Solvent Properties of Amphiphilic Compounds; Butterworths Scientific Publications: London, UK, 1954. [Google Scholar]
- Nguyen, T.T.L.; Edelen, A.; Neighbors, B.; Sabatini, D.A. Biocompatible lecithin-based microemulsions with rhamnolipid and sophorolipid biosurfactants: Formulation and potential applications. J. Colloid Interface Sci. 2010, 348, 498–504. [Google Scholar] [CrossRef] [PubMed]
- Salager, J.-L.; Antón, R.E.; Sabatini, D.A.; Harwell, J.H.; Acosta, E.J.; Tolosa, L.I. Enhancing solubilization in microemulsions—State of the art and current trends. J. Surfactants Deterg. 2005, 8, 3–21. [Google Scholar] [CrossRef]
- Arai, H.; Shinoda, K. The effect of mixing of oils and of nonionic surfactants on the phase inversion temperatures of emulsions. J. Colloid Interface Sci. 1967, 25, 396–400. [Google Scholar] [CrossRef]
- Shinoda, K.; Saito, H. The stability of O/W type emulsions as functions of temperature and the HLB of emulsifiers: The emulsification by PIT-method. J. Colloid Interface Sci. 1969, 30, 258–263. [Google Scholar] [CrossRef]
- Tadros, T.; Izquierdo, P.; Esquena, J.; Solans, C. Formation and stability of nano-emulsions. Adv. Colloid Interface Sci. 2004, 108, 303–318. [Google Scholar] [CrossRef]
- Shinoda, K. The comparison between the PIT system and the HLB-value system to emulsifier selection. In Proceedings of the 5th International Congress of Surface Activity, Barcelona, Spain, 9–13 September 1969; Volume 2, pp. 275–283. [Google Scholar]
- McClements, D.J. Edible nanoemulsions: Fabrication, properties, and functional performance. Soft Matter 2011, 7, 2297–2316. [Google Scholar] [CrossRef] [Green Version]
- Solans, C.; Solé, I. Nano-emulsions: Formation by low-energy methods. Curr. Opin. Colloid Interface Sci. 2012, 17, 246–254. [Google Scholar] [CrossRef]
- Roger, K.; Cabane, B.; Olsson, U. Emulsification through surfactant hydration: The PIC process revisited. Langmuir 2011, 27, 604–611. [Google Scholar] [CrossRef]
- Shinoda, K.; Saito, H. The effect of temperature on the phase equilibria and the types of dispersions of the ternary system composed of water, cyclohexane, and nonionic surfactant. J. Colloid Interface Sci. 1968, 26, 70–74. [Google Scholar] [CrossRef]
- Walstra, P.; Smulders, P. Modern Aspects of Emulsion Science; Royal Society of Chemistry: London, UK, 1998; p. 56. [Google Scholar]
- Lee, G.W.J.; Tadros, T.F. Formation and stability of emulsions produced by dilution of emulsifiable concentrates. Part I. An investigation of the dispersion on dilution of emulsifiable concentrates containing cationic and non-ionic surfactants. Colloids Surf. 1982, 5, 105–115. [Google Scholar] [CrossRef]
- Beerbower, A. Application of the cohesive energy ratio (CER) concept to anionic emulsifiers. Am. Cosmet. Perfum. 1972, 87, 85. [Google Scholar]
- Holtzscherer, C.; Candau, F. Application of the cohesive energy ratio concept (CER) to the formation of polymerizable microemulsions. Colloids Surf. 1988, 29, 411–423. [Google Scholar] [CrossRef]
- Rosen, M.J.; Kunjappu, J.T. Surfactants and Interfacial Phenomena; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Bouton, F.; Durand, M.; Nardello-Rataj, V.; Serry, M.; Aubry, J.-M. Classification of terpene oils using the fish diagrams and the Equivalent Alkane Carbon (EACN) scale. Colloids Surf. A 2009, 338, 142–147. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Morgan, C.; Poindexter, L.; Fernandez, J. Application of the hydrophilic–lipophilic deviation concept to surfactant characterization and surfactant selection for enhanced oil recovery. J. Surfactants Deterg. 2019, 22, 983–999. [Google Scholar] [CrossRef]
- Witthayapanyanon, A.; Harwell, J.H.; Sabatini, D.A. Hydrophilic–lipophilic deviation (HLD) method for characterizing conventional and extended surfactants. J. Colloid Interface Sci. 2008, 325, 259–266. [Google Scholar] [CrossRef]
- Budhathoki, M.; Hsu, T.-P.; Lohateeraparp, P.; Roberts, B.L.; Shiau, B.-J.; Harwell, J.H. Design of an optimal middle phase microemulsion for ultra high saline brine using hydrophilic lipophilic deviation (HLD) method. Colloids Surf. A 2016, 488, 36–45. [Google Scholar] [CrossRef]
- Wang, S. Design of extended surfactant-only EOR formulations for an ultrahigh salinity oil field by using hydrophilic lipophilic deviation (HLD) approach: From laboratory screening to simulation. Fuel 2019, 254, 115698. [Google Scholar] [CrossRef]
- Salager, J.L.; Morgan, J.C.; Schechter, R.S.; Wade, W.H.; Vasquez, E. Optimum formulation of surfactant/water/oil systems for minimum interfacial tension or phase behavior. Soc. Pet. Eng. J. 1979, 19, 107–115. [Google Scholar] [CrossRef]
- Bourrel, M.; Salager, J.L.; Schechter, R.S.; Wade, W.H. A correlation for phase behavior of nonionic surfactants. J. Colloid Interface Sci. 1980, 75, 451–461. [Google Scholar] [CrossRef]
- Antón, R.E.; Garcés, N.; Yajure, A. A correlation for three-phase behavior of cation1C surfactant-oil-water systems. J. Dispers. Sci. Technol. 1997, 18, 539–555. [Google Scholar] [CrossRef]
- Salager, J.L.; Antón, R.; Briceno, M.; Choplin, L.; Marquez, L.; Pizzino, A.; Rodriguez, M.P. The emergence of formulation engineering in emulsion making—Transferring know-how from research laboratory to plant. Polym. Int. 2003, 52, 471–478. [Google Scholar] [CrossRef]
- Salager, J.-L.; Marquez, N.; Graciaa, A.; Lachaise, J. Partitioning of ethoxylated octylphenol surfactants in microemulsion−oil−water systems: Influence of temperature and relation between partitioning coefficient and physicochemical formulation. Langmuir 2000, 16, 5534–5539. [Google Scholar] [CrossRef]
- Nawavimarn, P.; Rongsayamanont, W.; Subsanguan, T.; Luepromchai, E. Bio-based dispersants for fuel oil spill remediation based on the Hydrophilic-Lipophilic Deviation (HLD) concept and Box-Behnken design. Environ. Pollut. 2021, 285, 117378. [Google Scholar] [CrossRef]
- Abbott, S. Surfactant Science: Principles and Practice; DEStech Publications: Lancaster, PA, USA, 2015; ISBN 9781605954844. [Google Scholar]
- Acosta, E.J.; Bhakta, A.S. The HLD-NAC model for mixtures of ionic and nonionic surfactants. J. Surfactants Deterg. 2009, 12, 7–19. [Google Scholar] [CrossRef]
- Panumonwatee, G.; Charoensaeng, A.; Arpornpong, N. Application of Hydrophilic–lipophilic deviation equations to the formulation of a mixed-surfactant washing agent for crude rice bran oil removal from spent bleaching earth. J. Surfactants Deterg. 2021, 24, 949–962. [Google Scholar] [CrossRef]
- Salager, J.-L.; Antón, R.E.; Anderez, J.M.; Aubry, J.-M. Formulation des micro-émulsions par la méthode HLD. Tech. Ing. 2001, 157, 1–20. [Google Scholar]
- Chen, C.; Shen, H.; Harwell, J.H.; Shiau, B.-J. Characterizing oil mixture and surfactant mixture via hydrophilic-lipophilic deviation (HLD) principle: An insight in consumer products development. Colloids Surf. A 2021, 634, 127599. [Google Scholar] [CrossRef]
- Skauge, A.; Fotland, P. Effect of pressure and temperature on the phase behavior of microemulsions. SPE Reserv. Eng. 1990, 5, 601–608. [Google Scholar] [CrossRef]
- Schirone, D.; Tartaro, G.; Gentile, L.; Palazzo, G. An HLD framework for cationic ammonium surfactants. JCIS Open 2021, 4, 100033. [Google Scholar] [CrossRef]
- Salager, J.-L.; Antón, R.; Bullón, J.; Forgiarini, A.; Marquez, R. How to use the normalized hydrophilic-lipophilic deviation (HLDN) concept for the formulation of equilibrated and emulsified surfactant-oil-water systems for cosmetics and pharmaceutical products. Cosmetics 2020, 7, 57. [Google Scholar] [CrossRef]
- Acosta, E.J.; Yuan, J.S.; Bhakta, A.S. The characteristic curvature of ionic surfactants. J. Surfactants Deterg. 2008, 11, 145–158. [Google Scholar] [CrossRef]
- Kumar, P.; Mittal, K.L. Handbook of Microemulsion Science and Technology; CRC Press: London, UK, 1999. [Google Scholar]
- Najjar, R. Microemulsions: An Introduction to Properties and Applications; BoD—Books on Demand: Norderstedt, Germany, 2012. [Google Scholar]
- Kittithammavong, V.; Charoensaeng, A.; Khaodhiar, S. A Normalized HLD (HLDN) Tool for Optimal Salt-Concentration Prediction of Microemulsions. Appl. Sci. 2021, 11, 9151. [Google Scholar] [CrossRef]
- Kiran, S.K.; Acosta, E.J.; Moran, K. Evaluating the hydrophilic–lipophilic nature of asphaltenic oils and naphthenic amphiphiles using microemulsion models. J. Colloid Interface Sci. 2009, 336, 304–313. [Google Scholar] [CrossRef]
- Salager, J.-L.; Forgiarini, A.; Marquez, L.; Pena, A.; Pizzino, A.; Rodriguez, M.P.; Rondon-Gonzalez, M. Using emulsion inversion in industrial processes. Adv. Colloid Interface Sci. 2004, 108, 259–272. [Google Scholar] [CrossRef]
- Salager, J.-L. Emulsion phase inversion phenomena. In Emulsions and Emulsion Stability; CRC Press: London, UK, 2005; pp. 205–246. [Google Scholar]




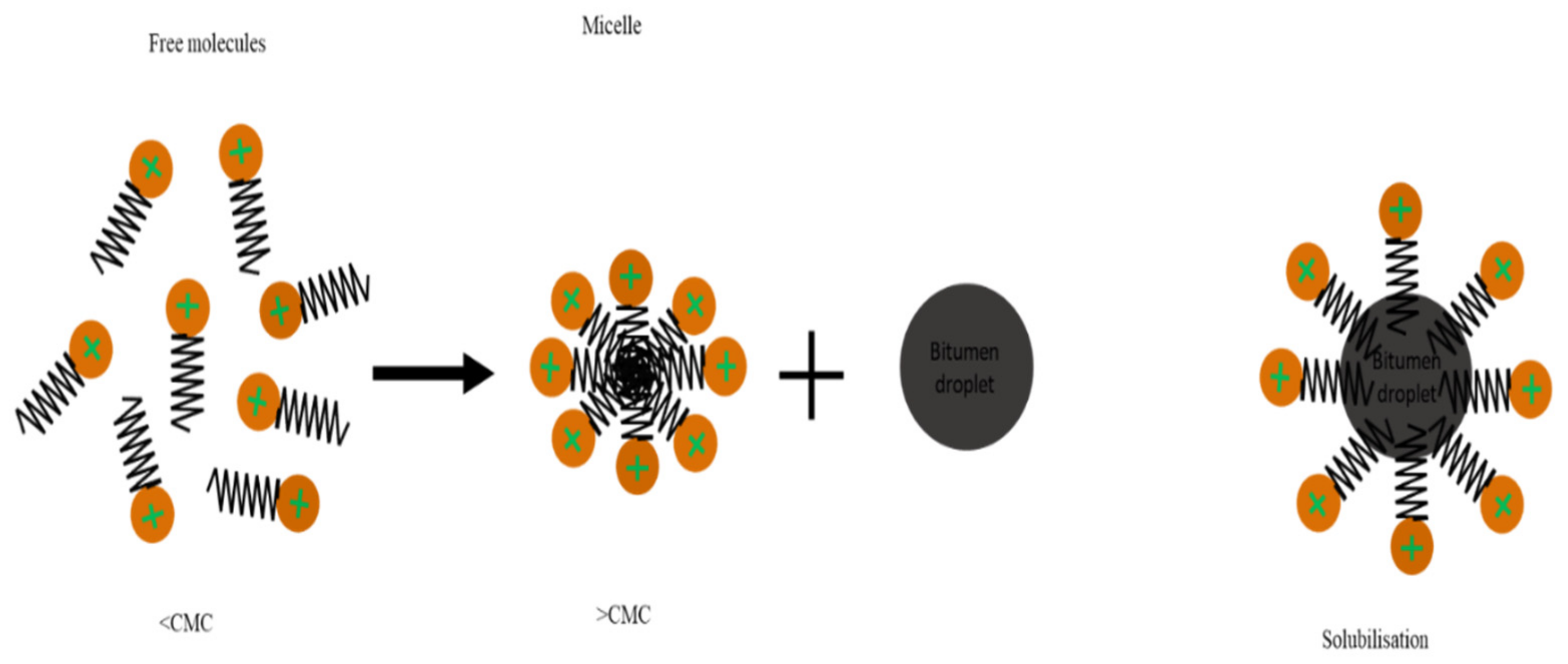
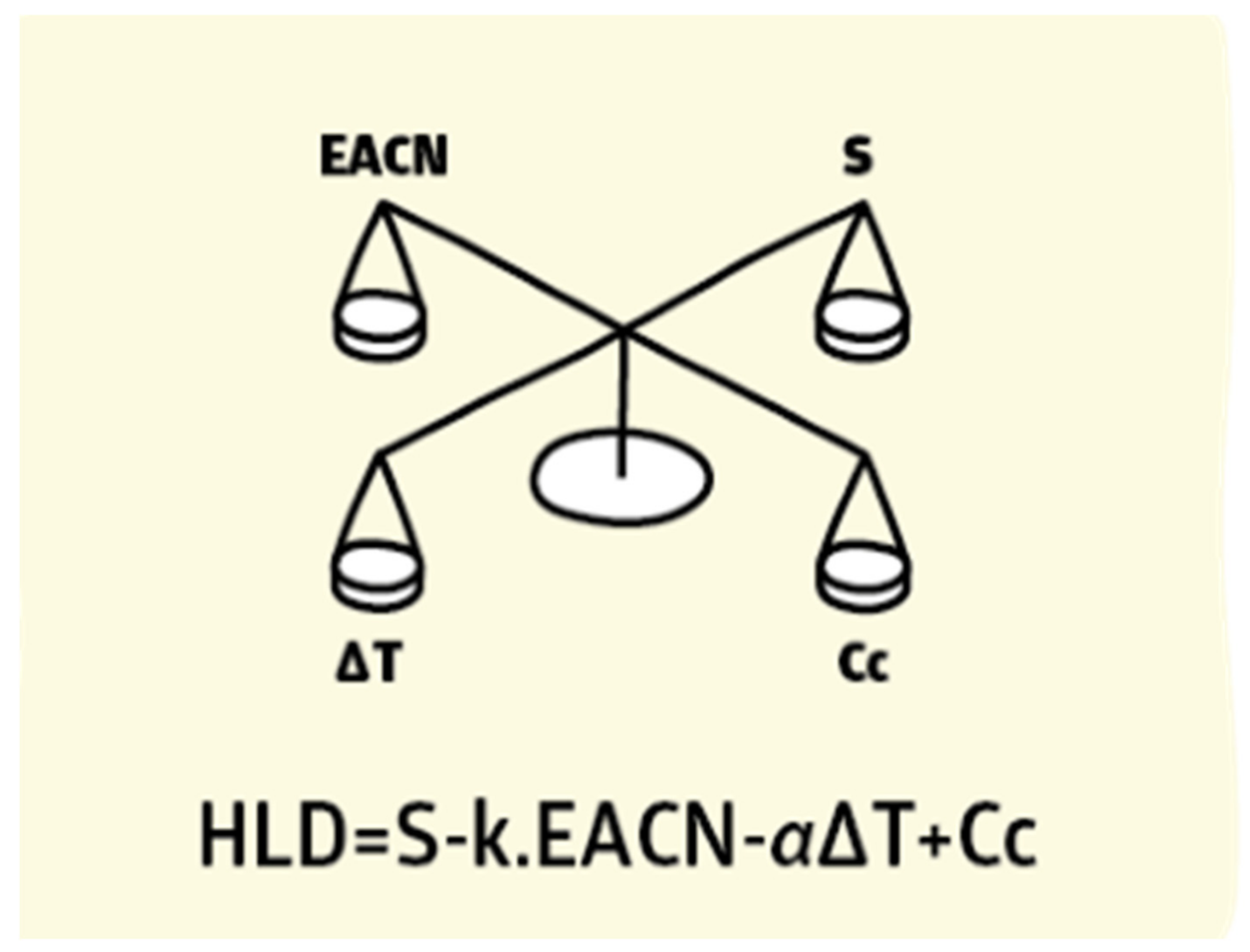
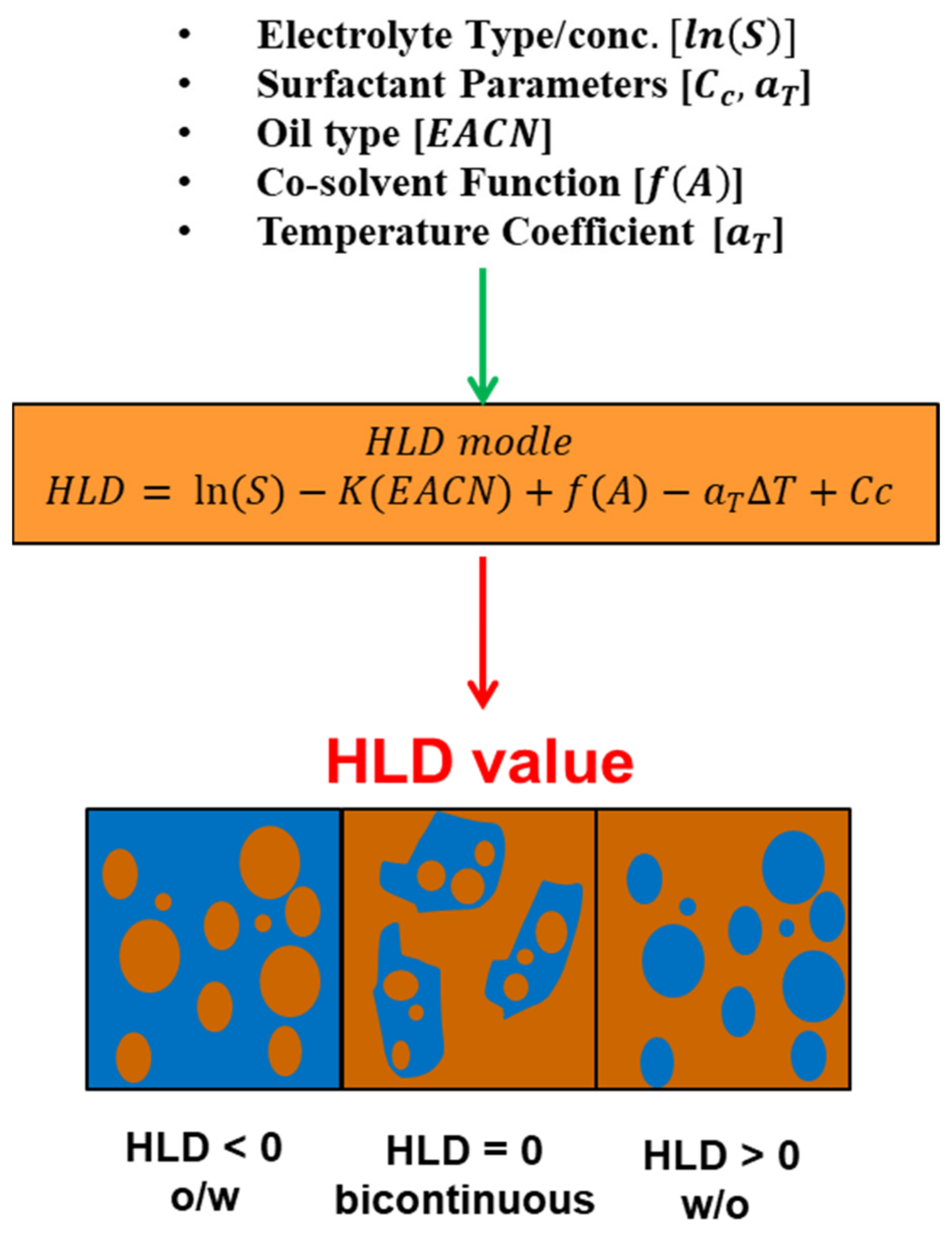
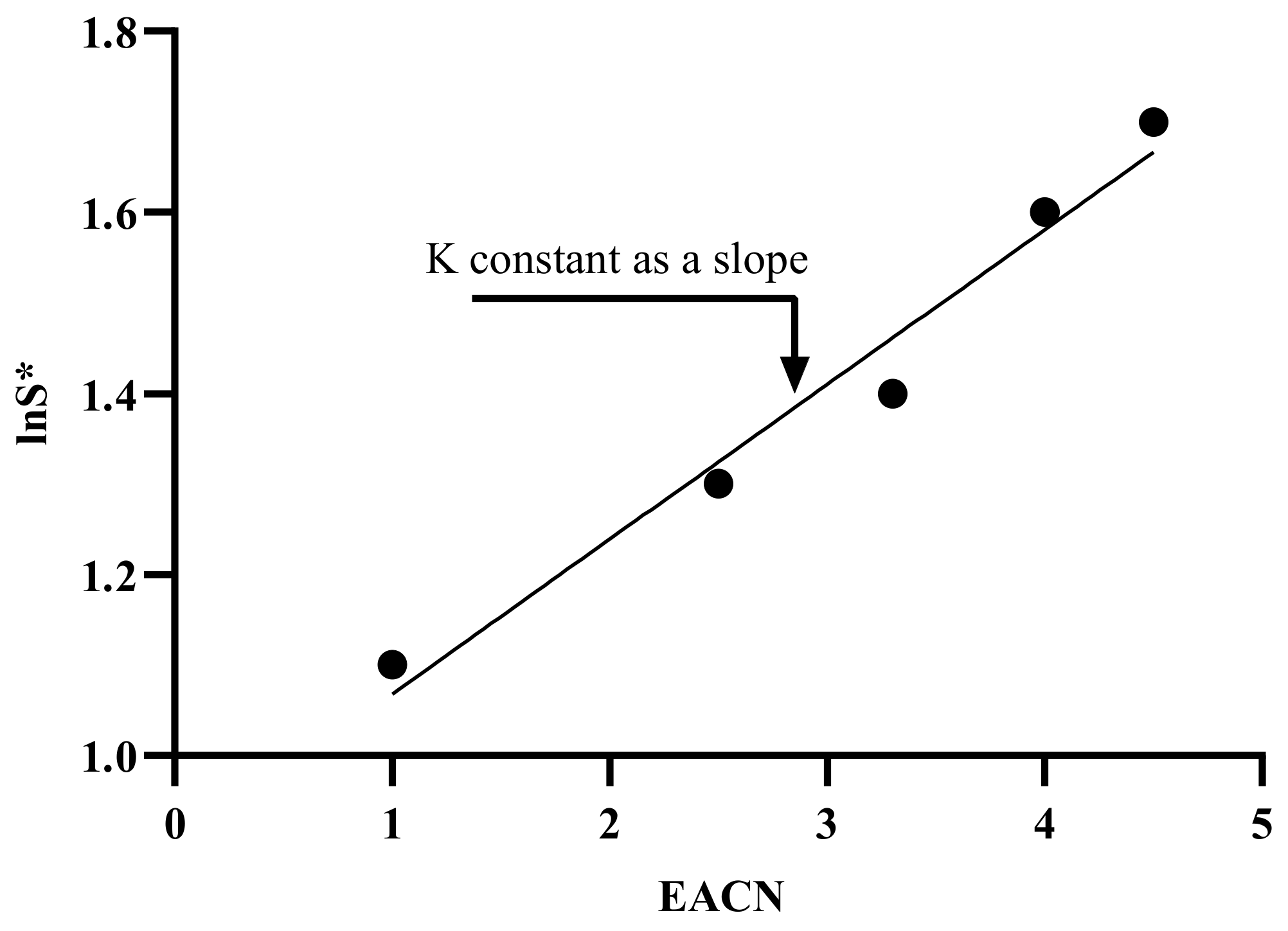

| Symbol | Description | Standard |
|---|---|---|
| C | Cationic bitumen emulsion | EN 1430 |
| Two digit number | Bitumen content as a% by mass | EN 1428 EN 1431 |
| Type of binder | ||
| B | Bitumen grade | EN 12591 |
| P | Addition of polymers | EN 14023 |
| F | Addition of more than 3% by mass of flux | |
| 2 to 10 | Breaking value | EN 13808 |
| Study | Variable | Conclusion |
|---|---|---|
| Pang et al. [133] | Surfactant content | Upsurging the surfactant content increases the viscosity of the emulsion. |
| Miljković et al. [134] | Cationic surfactant content | The surfactant content affects the cement hydration kinetics, emulsion rheology, and water binding, which are linked to mechanical performance cold mix. |
| Ouyang et al. [101] | Surfactant content | Higher surfactant content in prime coat emulsion resulted in higher aggregate base interlocking. |
| Xiao and Jiang [135] | The pH of the aqueous phase | pH values are correlated with surfactants and affect the resulting final emulsion properties. |
| Cui and Pang [136] | The pH of the aqueous phase | The interfacial tension property is dependent on the pH value of the aqueous phase. |
| Boucard et al. [137] | Electrolyte type | The addition of an electrolyte promotes flocculation, while the electrolyte NaOH promotes coalescence regardless of the dispersed phase (bitumen or silicone oil). |
| Baumgardner [98] | Ionic exchange | Ion exchange should take place, enabling the emulsion to retain its properties. |
| Baumgardner [98] | Colloid mill parameters | Input and output temperatures as well as the milling mechanical variables all have a significant impact on the properties of the final bitumen emulsions. |
| Kong et al. [138] | Anionic surfactant structure | During the mass transfer process, the SDBS and its isomers were adsorbed on the calcium carbonate surface and produced an aggregate structure. Na ions exhibited no evident aggregation behavior in the surfactant’s polar head during this phase. |
| Ziari et al. [139] | Surfactant type and emulsification method | The surfactant type and manufacturing technique seemed to have an impact on the mechanical characteristics of the mix, such as permanent deformation performance at elevated temperatures, fatigue cracking performance at intermediate temperatures, and some other mechanical properties. |
| Tan et al. [87] | Surfactant type and its content | The surfactant has a considerable retarding impact on cement hydration, which is related to the surfactant kinds and doses. |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Mohammedawi, A.; Mollenhauer, K. Current Research and Challenges in Bitumen Emulsion Manufacturing and Its Properties. Materials 2022, 15, 2026. https://doi.org/10.3390/ma15062026
Al-Mohammedawi A, Mollenhauer K. Current Research and Challenges in Bitumen Emulsion Manufacturing and Its Properties. Materials. 2022; 15(6):2026. https://doi.org/10.3390/ma15062026
Chicago/Turabian StyleAl-Mohammedawi, Ahmed, and Konrad Mollenhauer. 2022. "Current Research and Challenges in Bitumen Emulsion Manufacturing and Its Properties" Materials 15, no. 6: 2026. https://doi.org/10.3390/ma15062026
APA StyleAl-Mohammedawi, A., & Mollenhauer, K. (2022). Current Research and Challenges in Bitumen Emulsion Manufacturing and Its Properties. Materials, 15(6), 2026. https://doi.org/10.3390/ma15062026






