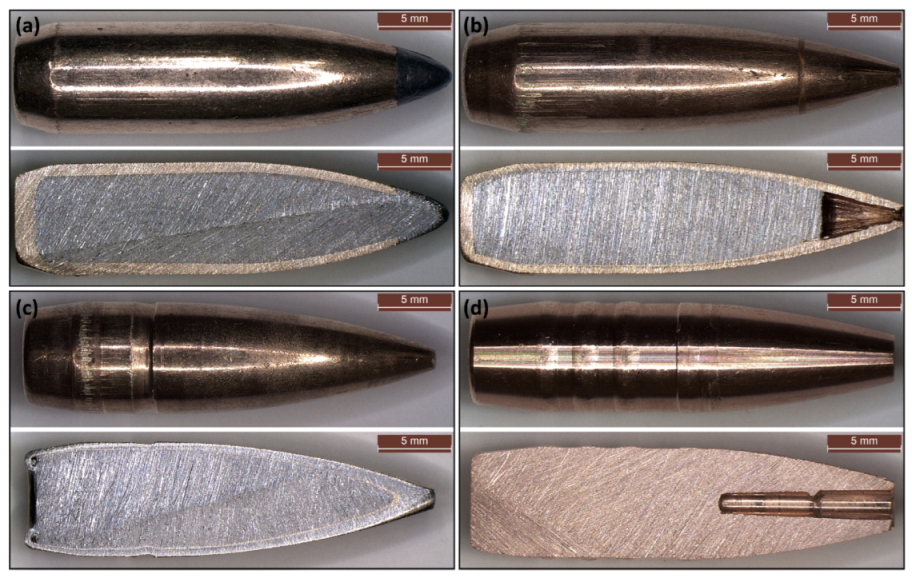
Figure 1.
External geometry and longitudinal section of the bullets used for experimentation; (a) Soft Point, (b) Hollow Point, (c) Full Metal Jacket and (d) Monolithic.
Figure 1.
External geometry and longitudinal section of the bullets used for experimentation; (a) Soft Point, (b) Hollow Point, (c) Full Metal Jacket and (d) Monolithic.
Figure 2.
Metallographic images of the metal plates used for experimentation, made of (a) Creusabro® 8000 steel and (b) Durostat® 400 steel. Both steel grades form martensite and bainite with carbides finely dispersed in the metal (etching agent: Nital2—Magnification: 500×). This type of investigation could not reveal the small amount of residual austenite prescribed for Creusabro® 8000 steel.
Figure 2.
Metallographic images of the metal plates used for experimentation, made of (a) Creusabro® 8000 steel and (b) Durostat® 400 steel. Both steel grades form martensite and bainite with carbides finely dispersed in the metal (etching agent: Nital2—Magnification: 500×). This type of investigation could not reveal the small amount of residual austenite prescribed for Creusabro® 8000 steel.
Figure 3.
Geometry of the specimens used for the tensile tests. Proportional specimens obtained in compliance with the UNI EN ISO 6892-1:2020 standard were used [
20].
Figure 3.
Geometry of the specimens used for the tensile tests. Proportional specimens obtained in compliance with the UNI EN ISO 6892-1:2020 standard were used [
20].
Figure 4.
Engineering stress/strain curves for Creusabro® 8000 steel (red lines) and Durostat® 400 steel (black lines) plates used for experimentation.
Figure 4.
Engineering stress/strain curves for Creusabro® 8000 steel (red lines) and Durostat® 400 steel (black lines) plates used for experimentation.
Figure 5.
Scheme of the camera settings (top view).
Figure 5.
Scheme of the camera settings (top view).
Figure 6.
Scheme of the camera settings (front view).
Figure 6.
Scheme of the camera settings (front view).
Figure 7.
Error on position vs. observer-reference distance. The worst-case scenario is identified for the Monolithic bullets, for which the distance from the trajectory and the reference plane is 400 mm: the apparent speed in such cases is 15.1% higher than the real speed.
Figure 7.
Error on position vs. observer-reference distance. The worst-case scenario is identified for the Monolithic bullets, for which the distance from the trajectory and the reference plane is 400 mm: the apparent speed in such cases is 15.1% higher than the real speed.
Figure 8.
Overview (left) and detail (right) of the finite element model of the plate highlighting the choice and progressive increase in the loaded elements characterizing the three arbitrary load distributions: 100 mm2 distribution (grey), 200 mm2 distribution (yellow), and 400 mm2 distribution (light blue); the three circles represent the area-equivalent circular extensions corresponding to the minimum and maximum limit of the compression area, and the external limit of the sliding area as experimentally observed on the impacted plates, respectively (Figures 12 and 13). Only a quarter of the plate was modeled, with orthogonal symmetry conditions.
Figure 8.
Overview (left) and detail (right) of the finite element model of the plate highlighting the choice and progressive increase in the loaded elements characterizing the three arbitrary load distributions: 100 mm2 distribution (grey), 200 mm2 distribution (yellow), and 400 mm2 distribution (light blue); the three circles represent the area-equivalent circular extensions corresponding to the minimum and maximum limit of the compression area, and the external limit of the sliding area as experimentally observed on the impacted plates, respectively (Figures 12 and 13). Only a quarter of the plate was modeled, with orthogonal symmetry conditions.
Figure 9.
Stress field convergence. Comparison between the maximum principal stress field at 0.05 ms as predicted by the 2.5 mm model (left) and the 1.25 mm model (right). The difference in peak stress is less than 1%. No significant differences are visible. The stress wave is axially symmetric despite the squared shape of the arbitrary loaded surface.
Figure 9.
Stress field convergence. Comparison between the maximum principal stress field at 0.05 ms as predicted by the 2.5 mm model (left) and the 1.25 mm model (right). The difference in peak stress is less than 1%. No significant differences are visible. The stress wave is axially symmetric despite the squared shape of the arbitrary loaded surface.
Figure 10.
Maximum principal stress waves comparison at 50 mm from the origin. The predictions obtained by the higher nodal density model (red) and by the lower nodal density model (blue) are almost impossible to distinguish, even after 0.4 ms, which corresponds to around ten times the load application time. The maximum difference in peak stress is 10% after 0.23 ms, therefore 0.19 mm after the end of the loading time. This demonstrates that the stress prediction is consistent.
Figure 10.
Maximum principal stress waves comparison at 50 mm from the origin. The predictions obtained by the higher nodal density model (red) and by the lower nodal density model (blue) are almost impossible to distinguish, even after 0.4 ms, which corresponds to around ten times the load application time. The maximum difference in peak stress is 10% after 0.23 ms, therefore 0.19 mm after the end of the loading time. This demonstrates that the stress prediction is consistent.
Figure 11.
Residual deformation fields at 20 ms after the impacts [mm]. Comparison between the 2.5 mm shell model (left) and the 1.25 mm shell model (right). The predictions are equivalent; the maximum difference is less than 0.5% at the peak: 0.03 mm over 7.3 mm of maximum deformation.
Figure 11.
Residual deformation fields at 20 ms after the impacts [mm]. Comparison between the 2.5 mm shell model (left) and the 1.25 mm shell model (right). The predictions are equivalent; the maximum difference is less than 0.5% at the peak: 0.03 mm over 7.3 mm of maximum deformation.
Figure 12.
Front and rear surfaces of the Durostat® 400 plates impacted by the bullets. The impacted areas show the marks of the interaction with the bullets, which can be distinguished in a central area where the interaction appears to be more compressive, and a peripheral area where the radial marks show the effects of the sliding of the bullet debris. The sliding marks are evident from a diameter of approximately 11 to 16 mm (roughly 100 mm2 to 200 mm2), to a diameter of 20 to 25 mm around the epicenter (around 400 mm2). The detailed front view of the FMJ effects shows the melted filler mixed with parts of the jacket, still blocked inside the crater.
Figure 12.
Front and rear surfaces of the Durostat® 400 plates impacted by the bullets. The impacted areas show the marks of the interaction with the bullets, which can be distinguished in a central area where the interaction appears to be more compressive, and a peripheral area where the radial marks show the effects of the sliding of the bullet debris. The sliding marks are evident from a diameter of approximately 11 to 16 mm (roughly 100 mm2 to 200 mm2), to a diameter of 20 to 25 mm around the epicenter (around 400 mm2). The detailed front view of the FMJ effects shows the melted filler mixed with parts of the jacket, still blocked inside the crater.
Figure 13.
Front and rear surfaces of the Creusabro® 8000 plates impacted by the bullets. The impacted areas show the marks of the interaction with the bullets, that can be distinguished in a central area where the interaction appears to be more compressive, and a peripheral area where the radial marks show the effects of the sliding of the bullet debris. The sliding marks are evident from a diameter of approximately 11 to 16 mm (roughly 100 mm2 to 200 mm2), to a diameter of 20 to 25 mm around the epicenter (around 400 mm2).
Figure 13.
Front and rear surfaces of the Creusabro® 8000 plates impacted by the bullets. The impacted areas show the marks of the interaction with the bullets, that can be distinguished in a central area where the interaction appears to be more compressive, and a peripheral area where the radial marks show the effects of the sliding of the bullet debris. The sliding marks are evident from a diameter of approximately 11 to 16 mm (roughly 100 mm2 to 200 mm2), to a diameter of 20 to 25 mm around the epicenter (around 400 mm2).
Figure 14.
Partial penetration of FMJ bullet material through the first plate of Durostat® 400. Back of the first plate showing the penetration of some melted filler material.
Figure 14.
Partial penetration of FMJ bullet material through the first plate of Durostat® 400. Back of the first plate showing the penetration of some melted filler material.
Figure 15.
The figure shows ten frames of the first 0.3 ms after the impact for the combination FMJ-Creusabro® 8000, taken from the footage recorded by the perspective camera (Camera 2). The first frames (a–c) show the crushing of the bullet at its first contact with the target, allowing the appreciation of a spark caused by the high hardness of the plate. Fragments are then projected radially from the impact point starting from small particles (c–f), with slightly bigger fragments later ricocheting in annular patterns (f–j). Frames shown here are processed to show the parts of interest, filtering out the noise otherwise preventing the appreciation of the bullet fragmentation.
Figure 15.
The figure shows ten frames of the first 0.3 ms after the impact for the combination FMJ-Creusabro® 8000, taken from the footage recorded by the perspective camera (Camera 2). The first frames (a–c) show the crushing of the bullet at its first contact with the target, allowing the appreciation of a spark caused by the high hardness of the plate. Fragments are then projected radially from the impact point starting from small particles (c–f), with slightly bigger fragments later ricocheting in annular patterns (f–j). Frames shown here are processed to show the parts of interest, filtering out the noise otherwise preventing the appreciation of the bullet fragmentation.
Figure 16.
The figure shows a large fragment of the Full Metal Jacket bouncing back from the impact area of the Creusabro® 8000 plate. The shot is therefore non-compliant with the bullet-splash definition. The fragment translates with perpendicular speed measured at 4.4 ± 2% m/s. Mild spinning can be appreciated. The three frames shown here (a–c), extracted from the second camera footage, were processed to isolate the fragment from the dust created after the impact: for each frame, only the fragment and the dent on the plate are visible; an approximate dimensional reference for the dent and the fragment is reported in frame (a). The fragment has a diameter of around 7.5 mm and thickness of about 4 mm, with the shape similar to the bulge created on the plate.
Figure 16.
The figure shows a large fragment of the Full Metal Jacket bouncing back from the impact area of the Creusabro® 8000 plate. The shot is therefore non-compliant with the bullet-splash definition. The fragment translates with perpendicular speed measured at 4.4 ± 2% m/s. Mild spinning can be appreciated. The three frames shown here (a–c), extracted from the second camera footage, were processed to isolate the fragment from the dust created after the impact: for each frame, only the fragment and the dent on the plate are visible; an approximate dimensional reference for the dent and the fragment is reported in frame (a). The fragment has a diameter of around 7.5 mm and thickness of about 4 mm, with the shape similar to the bulge created on the plate.
Figure 17.
Load histories estimated according to Equation (8), applied to the impacts on Durostat® 400. The SP, HP and MONO bullets have similar force peaks around 220–230 kN. The FMJ reaches instead 307 kN force. The duration of the impulses ranges from 36 to 47 µs.
Figure 17.
Load histories estimated according to Equation (8), applied to the impacts on Durostat® 400. The SP, HP and MONO bullets have similar force peaks around 220–230 kN. The FMJ reaches instead 307 kN force. The duration of the impulses ranges from 36 to 47 µs.
Figure 18.
Load histories estimated according to Equation (8), applied to the impacts on Creusabro® 8000. The SP reaches 194 kN peak force, MONO reaches 243 kN, HP reaches 260 kN, and FMJ reaches 281 kN. The durations of the impulses range from 39 µs for FMJ to 49 µs for SP.
Figure 18.
Load histories estimated according to Equation (8), applied to the impacts on Creusabro® 8000. The SP reaches 194 kN peak force, MONO reaches 243 kN, HP reaches 260 kN, and FMJ reaches 281 kN. The durations of the impulses range from 39 µs for FMJ to 49 µs for SP.
Figure 19.
Residual displacements caused by the Soft Point bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) is near the maximum experimental value (blue dotted line), around 4 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) is near the minimum experimental value, around 3 mm. The predicted apex displacement for the 400 mm2 distribution (light blue line) is around 1 mm lower than the minimum experimental value. The radial extension of the bulge is underestimated. Over 35 mm radial distance from the apex the three load distributions are impossible to distinguish.
Figure 19.
Residual displacements caused by the Soft Point bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) is near the maximum experimental value (blue dotted line), around 4 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) is near the minimum experimental value, around 3 mm. The predicted apex displacement for the 400 mm2 distribution (light blue line) is around 1 mm lower than the minimum experimental value. The radial extension of the bulge is underestimated. Over 35 mm radial distance from the apex the three load distributions are impossible to distinguish.
Figure 20.
Soft Point bullet on Durostat® 400 plates: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (109%), while the 200 mm2 and 400 mm2 give 82% and 55%. The three distributions provide more consistent predictions in terms of bulge volume and slope, all between 51 and 68%.
Figure 20.
Soft Point bullet on Durostat® 400 plates: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (109%), while the 200 mm2 and 400 mm2 give 82% and 55%. The three distributions provide more consistent predictions in terms of bulge volume and slope, all between 51 and 68%.
Figure 21.
Residual displacements caused by the Hollow Point bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) of about 1 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) coincides with the maximum experimental value, around 3.4 mm. The predicted apex displacement for the 400 mm2 distribution (light blue line) is almost coincident with the minimum experimental value. The radial extension of the bulge is slightly underestimated. Over 25 mm radial distance from the apex the three load distributions are almost impossible to distinguish.
Figure 21.
Residual displacements caused by the Hollow Point bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) of about 1 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) coincides with the maximum experimental value, around 3.4 mm. The predicted apex displacement for the 400 mm2 distribution (light blue line) is almost coincident with the minimum experimental value. The radial extension of the bulge is slightly underestimated. Over 25 mm radial distance from the apex the three load distributions are almost impossible to distinguish.
Figure 22.
Validation for HP on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (119%), while the 100 mm2 and 400 mm2 give 157% and 80%. The three distributions provide more consistent predictions in terms of bulge volume and slope, all between 45% and 67%.
Figure 22.
Validation for HP on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (119%), while the 100 mm2 and 400 mm2 give 157% and 80%. The three distributions provide more consistent predictions in terms of bulge volume and slope, all between 45% and 67%.
Figure 23.
Residual displacements caused by the Full Metal Jacket bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) underestimates the experimental range (blue and red dotted lines) of about 2.5 mm. The overall bulge extension is significantly underestimated. For radial distance over 25 mm from the apex, the three load distributions are almost impossible to distinguish.
Figure 23.
Residual displacements caused by the Full Metal Jacket bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) underestimates the experimental range (blue and red dotted lines) of about 2.5 mm. The overall bulge extension is significantly underestimated. For radial distance over 25 mm from the apex, the three load distributions are almost impossible to distinguish.
Figure 24.
Validation for FMJ on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (63%), while the 200 mm2 and 400 mm2 give 48% and 30%. The three distributions provide consistent predictions in terms of bulge volume around 30–33%. In slope, the estimate increases as the distribution area, from 17.5% to 40%.
Figure 24.
Validation for FMJ on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (63%), while the 200 mm2 and 400 mm2 give 48% and 30%. The three distributions provide consistent predictions in terms of bulge volume around 30–33%. In slope, the estimate increases as the distribution area, from 17.5% to 40%.
Figure 25.
Residual displacements caused by the Monolithic bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) is almost coincident with the maximum experimental value (blue dotted line). The predicted apex displacement for the 200 mm2 distribution (yellow line) coincides with the minimum experimental value. The radial extension of the bulge is slightly underestimated. For radial distances over 35 mm from the apex, the three load distributions are impossible to distinguish.
Figure 25.
Residual displacements caused by the Monolithic bullet on Durostat® 400 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) is almost coincident with the maximum experimental value (blue dotted line). The predicted apex displacement for the 200 mm2 distribution (yellow line) coincides with the minimum experimental value. The radial extension of the bulge is slightly underestimated. For radial distances over 35 mm from the apex, the three load distributions are impossible to distinguish.
Figure 26.
Validation for MONO on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (113%), while the 200 mm2 and 400 mm2 give 83% and 54%. The bulge volume predictions decrease from 76% to 63% as the area increases. The slope predictions are more consistent, between 57% and 67%.
Figure 26.
Validation for MONO on Durostat® 400: validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (113%), while the 200 mm2 and 400 mm2 give 83% and 54%. The bulge volume predictions decrease from 76% to 63% as the area increases. The slope predictions are more consistent, between 57% and 67%.
Figure 27.
Residual displacements caused by the Soft Point bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) slightly exceeds the maximum experimental value (blue dotted line). The predicted apex displacement for the 200 mm2 distribution (yellow line) is in the middle of the experimental range. The predicted apex displacement for the 400 mm2 distribution (light blue line) underestimates the minimum experimental value of about 0.5 mm. The radial extension of the bulge is slightly underestimated. Over 35 mm radial distance from the apex the three load distributions are impossible to distinguish.
Figure 27.
Residual displacements caused by the Soft Point bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) slightly exceeds the maximum experimental value (blue dotted line). The predicted apex displacement for the 200 mm2 distribution (yellow line) is in the middle of the experimental range. The predicted apex displacement for the 400 mm2 distribution (light blue line) underestimates the minimum experimental value of about 0.5 mm. The radial extension of the bulge is slightly underestimated. Over 35 mm radial distance from the apex the three load distributions are impossible to distinguish.
Figure 28.
Validation for SP on Creusabro® 8000. Validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (98%), while the 100 mm2 and 400 mm2 give 128% and 66%. The bulge volume predictions decrease from 81% to 56% as the area increases. The slope predictions are more consistent, between 80% and 88%.
Figure 28.
Validation for SP on Creusabro® 8000. Validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (98%), while the 100 mm2 and 400 mm2 give 128% and 66%. The bulge volume predictions decrease from 81% to 56% as the area increases. The slope predictions are more consistent, between 80% and 88%.
Figure 29.
Residual displacements caused by the Hollow Point bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) of about 0.2 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) is in the middle of the experimental range. The predicted apex displacement for the 400 mm2 distribution (light blue line) is 0.5 lower than the minimum experimental value. The radial extension of the bulge is slightly underestimated. For radial distances over 25 mm from the apex, the three load distributions are impossible to distinguish.
Figure 29.
Residual displacements caused by the Hollow Point bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) of about 0.2 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) is in the middle of the experimental range. The predicted apex displacement for the 400 mm2 distribution (light blue line) is 0.5 lower than the minimum experimental value. The radial extension of the bulge is slightly underestimated. For radial distances over 25 mm from the apex, the three load distributions are impossible to distinguish.
Figure 30.
Validation for HP on Creusabro® 8000. Validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (98%), while the 100 mm2 and 400 mm2 give 125% and 63%. The bulge volume predictions decrease from 75% to 63% as the area increases. The slope predictions increase from 60% (100 mm2) to 80% (200 and 400 mm2).
Figure 30.
Validation for HP on Creusabro® 8000. Validation of the calculated residual displacement fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (98%), while the 100 mm2 and 400 mm2 give 125% and 63%. The bulge volume predictions decrease from 75% to 63% as the area increases. The slope predictions increase from 60% (100 mm2) to 80% (200 and 400 mm2).
Figure 31.
Residual displacements caused by the Full Metal Jacket bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) underestimates the experimental (blue and red dotted lines) range of about 2 mm. The overall bulge extension is significantly underestimated. For radial distances over 15 mm from the apex, the three load distributions are difficult to distinguish.
Figure 31.
Residual displacements caused by the Full Metal Jacket bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) underestimates the experimental (blue and red dotted lines) range of about 2 mm. The overall bulge extension is significantly underestimated. For radial distances over 15 mm from the apex, the three load distributions are difficult to distinguish.
Figure 32.
Validation for FMJ on Creusabro® 8000. Validation of the calculated residual displacements fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (67%), while the 200 mm2 and 400 mm2 give 49% and 32%. The bulge volume predictions are consistent between 22% and 25%. The slope predictions increase from 9% (100 mm2) to 19% (400 mm2).
Figure 32.
Validation for FMJ on Creusabro® 8000. Validation of the calculated residual displacements fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 100 mm2 distribution gives the best estimation (67%), while the 200 mm2 and 400 mm2 give 49% and 32%. The bulge volume predictions are consistent between 22% and 25%. The slope predictions increase from 9% (100 mm2) to 19% (400 mm2).
Figure 33.
Residual displacements caused by the Monolithic bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) by 0.3 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) almost coincides with the middle range of the experimental values (blue and red dotted lines). The predicted apex displacement for the 400 mm2 distribution (light blue line) coincides with the minimum experimental value. The radial extension of the bulge is well estimated. For radial distances over 35 mm from the apex, the three load distributions are impossible to distinguish.
Figure 33.
Residual displacements caused by the Monolithic bullet on Creusabro® 8000 plates. The predicted apex displacement for the 100 mm2 distribution (grey line) exceeds the maximum experimental value (blue dotted line) by 0.3 mm. The predicted apex displacement for the 200 mm2 distribution (yellow line) almost coincides with the middle range of the experimental values (blue and red dotted lines). The predicted apex displacement for the 400 mm2 distribution (light blue line) coincides with the minimum experimental value. The radial extension of the bulge is well estimated. For radial distances over 35 mm from the apex, the three load distributions are impossible to distinguish.
Figure 34.
Validation for MONO on Creusabro® 8000. Validation of the calculated residual displacements fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (107%), while the 100 mm2 and 400 mm2 give 141% and 70%. The bulge volume predictions decrease with the area, from 113% to 76%. The slope predictions are more consistent, between 109% and 123%.
Figure 34.
Validation for MONO on Creusabro® 8000. Validation of the calculated residual displacements fields in terms of apex displacement (blue), total bulge volume (red), and slope at 50 mm (yellow). In abscissa the loaded area of the three arbitrary distributions. In terms of apex displacement, the 200 mm2 distribution gives the best estimation (107%), while the 100 mm2 and 400 mm2 give 141% and 70%. The bulge volume predictions decrease with the area, from 113% to 76%. The slope predictions are more consistent, between 109% and 123%.
Table 1.
Main data of the bullets used for experimentation as provided by the manufacturers. The nominal muzzle velocity is the expected velocity at the exit of the firearm. The nominal energy is the expected kinetic energy of the bullets at the exit of the firearm.
Table 1.
Main data of the bullets used for experimentation as provided by the manufacturers. The nominal muzzle velocity is the expected velocity at the exit of the firearm. The nominal energy is the expected kinetic energy of the bullets at the exit of the firearm.
| Bullet Type | Cal. | Bullet
Mass [g] | Nominal Muzzle Velocity [m/s] | Nominal
Energy [J] |
|---|
| Soft Point (SP) | 308 Win | 11.7 | 870 | 4428 |
| Hollow Point (HP) | 308 Win | 10.9 | 800 | 3485 |
| Full Metal Jacket (FMJ) | 308 Win | 9.5 | 865 | 3570 |
| Monolithic (MONO) | 308 Win | 9.6 | 860 | 3550 |
Table 2.
Chemical analyses of Creusabro® 8000 steel (wt%).
Table 2.
Chemical analyses of Creusabro® 8000 steel (wt%).
| | C | S | P | Si | Mn | Ni | Cr | Mo |
|---|
| Metal Plate | 0.21 | 0.002 | 0.009 | 0.74 | 1.18 | 0.49 | 0.70 | 0.26 |
| Steel Mill Specs | 0.28
Max | 0.005
Max | 0.018
Max | Unspecified | 1.60
Max | 1.00
Max | 1.60
Max | 0.40
Max |
Table 3.
Chemical analyses Durostat® 400 steel (wt%).
Table 3.
Chemical analyses Durostat® 400 steel (wt%).
| | C | S | P | Si | Mn | Al | Cr | Mo | B | Ti |
|---|
| Metal Plate | 0.10 | 0.002 | 0.008 | 0.16 | 2.08 | 0.03 | 0.26 | <0.01 | 0.001 | 0.021 |
| Steel Mill Specs | 0.18
Max | 0.010
Max | 0.025
Max | 0.60
Max | 2.10
Max | 0.02
Min | 1.00
Max | 0.50
Max | 0.005
Max | 0.050
Max |
Table 4.
Summary of tensile tests results for the Creusabro® 8000 steel and Durostat® 400 plates used for experimentation.
Table 4.
Summary of tensile tests results for the Creusabro® 8000 steel and Durostat® 400 plates used for experimentation.
| | E [Gpa] | YS [Mpa] | UTS [Mpa] | YS/UTS | Ag% | A% |
|---|
| Creusabro® 8000 | 210 | 1190 | 1735 | 0.68 | 5 | 11 |
| Durostat® 400 | 210 | 1100 | 1290 | 0.85 | 3 | 11 |
Table 5.
Data acquisition: camera 1.
Table 5.
Data acquisition: camera 1.
| Shot | Resolution [px] | Sampling Frequency [fps] |
|---|
| 1 | 256 × 256 | 39,000 |
| 2 | 320 × 128 | 50,000 |
| 3 | 320 × 128 | 50,000 |
| 4 | 320 × 128 | 50,000 |
| 5 | 320 × 128 | 50,000 |
| 6 | 320 × 128 | 50,000 |
| 7 | 320 × 128 | 40,000 |
| 8 | 320 × 128 | 40,000 |
Table 6.
Data acquisition: camera 2.
Table 6.
Data acquisition: camera 2.
| Shot | Resolution [px] | Sampling Frequency [fps] |
|---|
| 1 | 320 × 256 | 33,000 |
| 2 | 320 × 152 | 48,000 |
| 3 | 320 × 152 | 48,000 |
| 4 | 320 × 152 | 48,000 |
| 5 | 320 × 152 | 48,000 |
| 6 | 320 × 152 | 48,000 |
| 7 | 320 × 152 | 48,000 |
| 8 | 320 × 152 | 48,000 |
Table 7.
Table of the constitutive parameters associated with the Creusabro® 8000 and Durostat® 400 models associated with the plates: RO = density; E = Young Modulus; PR = Poisson Ratio; SIGY = Yield Stress; ETAN = linear hardening modulus; EPPF = initial necking strain; C, p = rate effect parameters according to the Cowper–Symonds model; EPPFR = ultimate failure strain.
Table 7.
Table of the constitutive parameters associated with the Creusabro® 8000 and Durostat® 400 models associated with the plates: RO = density; E = Young Modulus; PR = Poisson Ratio; SIGY = Yield Stress; ETAN = linear hardening modulus; EPPF = initial necking strain; C, p = rate effect parameters according to the Cowper–Symonds model; EPPFR = ultimate failure strain.
| | RO
[kg/m3] | E
[GPa] | PR | SIGY [MPa] | ETAN [MPa] | EPPF | C
[s−1] | p | EPPFR |
|---|
| Creusabro® 8000 | 7800 | 210 | 0.33 | 1190 | 11,891.7 | 0.058 | 396,500 | 3.0745 | 0.11 |
| Durostat® 400 | 7800 | 210 | 0.33 | 1100 | 5759.6 | 0.044 | 396,500 | 3.0745 | 0.11 |
Table 8.
Estimated impact velocities.
Table 8.
Estimated impact velocities.
| Shot | Bullet | Material | Impact Speed
[m/s] | Maximum Error [%] |
|---|
| 1 | Soft Point (SP) | Durostat® 400 | 642 | 1.1 |
| 2 | Hollow Point (HP) | Durostat® 400 | 645 | 8.5 * |
| 3 | Full Metal Jacket (FMJ) | Durostat® 400 | 769 | 1.2 |
| 4 | Monolithic (MONO) | Durostat® 400 | 738 | 3.5 |
| 5 | Soft Point (SP) | Creusabro® 8000 | 607 | 1.2 |
| 6 | Hollow Point (HP) | Creusabro® 8000 | 702 | 5.7 |
| 7 | Full Metal Jacket (FMJ) | Creusabro® 8000 | 736 | 2.9 |
| 8 | Monolithic (MONO) | Creusabro® 8000 | 749 | 2.8 |
Table 9.
Average velocities at muzzle and at 100 m and relative losses for each bullet type. Averages are computed over a total of four shots per bullet type.
Table 9.
Average velocities at muzzle and at 100 m and relative losses for each bullet type. Averages are computed over a total of four shots per bullet type.
| Bullet | [m/s]
| [m/s]
| Loss [%] |
|---|
| Soft Point (SP) | 763 | 620 | 18.7 |
| Hollow Point (HP) | 814 | 727 | 10.7 |
| Full Metal Jacket (FMJ) | 843 | 731 | 13.3 |
| Monolithic (MONO) | 837 | 741 | 11.5 |
Table 10.
Density values associated with the bullet materials for the four bullet types so that the volume integration of the density fields correspond to the nominal bullets’ masses.
Table 10.
Density values associated with the bullet materials for the four bullet types so that the volume integration of the density fields correspond to the nominal bullets’ masses.
| Bullet | [kg/m3]
| [kg/m3]
| Bullet Mass [g] |
|---|
| Soft Point (SP) | 8730 | 12,640 | 11.66 |
| Hollow Point (HP) | 8730 | 12,280 | 10.89 |
| Full Metal Jacket (FMJ) | 8730 | 11,325 | 9.53 |
| Monolithic (MONO) | 9425 | - | 9.59 |
Table 11.
Validation comparisons for Durostat® 400. Validation percentages are calculated as numerical simulation results obtained for the 100 mm2 distribution divided by the experimental results.
Table 11.
Validation comparisons for Durostat® 400. Validation percentages are calculated as numerical simulation results obtained for the 100 mm2 distribution divided by the experimental results.
| | SP | HP | FMJ * | MONO |
|---|
| Validation in Bulge Volume | 64.22% | 53.31% | 31.69% | 76.12% |
| Validation in Apex Displacement | 108.90% | 156.92% | 63.47% | 112.62% |
| Experimental Slope @ 50 mm | −2.00% | −2.00% | −4.00% | −2.00% |
| Numerical Slope @ 50 mm | −1.20% | −0.91% | −0.70% | −1.15% |
| Validation in Slope @ 50 mm | 60.19% | 45.26% | 17.50% | 57.43% |
Table 12.
Validation comparisons for Creusabro® 8000. Validation percentages are calculated as numerical simulation results obtained for the 100 mm2 distribution divided by the experimental results.
Table 12.
Validation comparisons for Creusabro® 8000. Validation percentages are calculated as numerical simulation results obtained for the 100 mm2 distribution divided by the experimental results.
| | SP | HP | FMJ * | MONO |
|---|
| Validation in Bulge Volume | 75.48% | 74.98% | 21.95% | 105.05% |
| Validation in Apex Displacement | 127.73% | 125.00% | 66.62% | 141.36% |
| Experimental Slope @ 50 mm | −1.50% | −1.50% | −4.50% | −1.00% |
| Numerical Slope @ 50 mm | −1.22% | −0.90% | −0.39% | −1.13% |
| Validation in Slope @ 50 mm | 81.11% | 60.00% | 8.69% | 113.26% |