A Three-Dimensional Vibration Theory for Ultralight Cellular Sandwich Plates Subjected to Linearly Varying In-Plane Distributed Loads
Abstract
:1. Introduction
2. Formulation
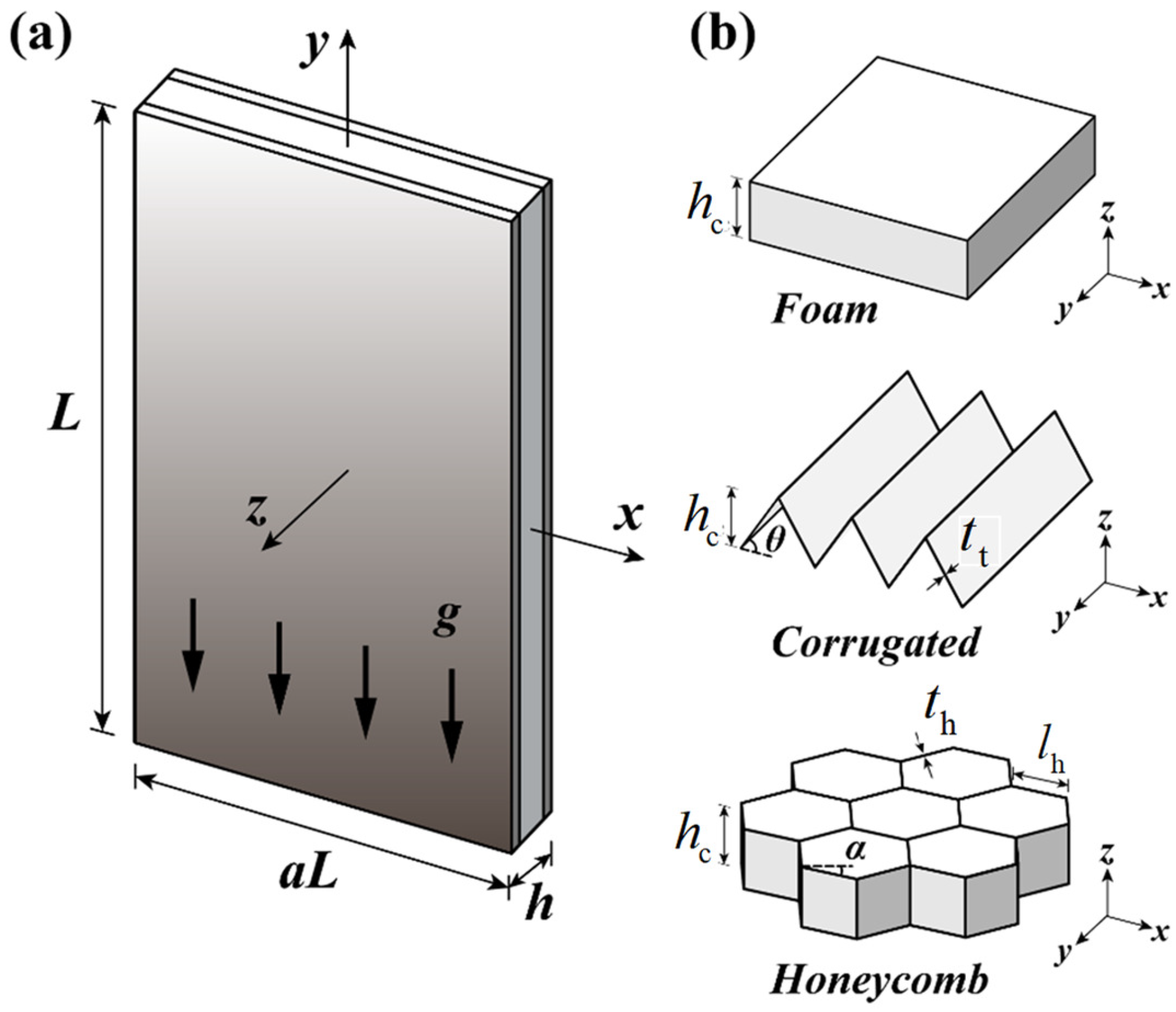
2.1. Problem Description
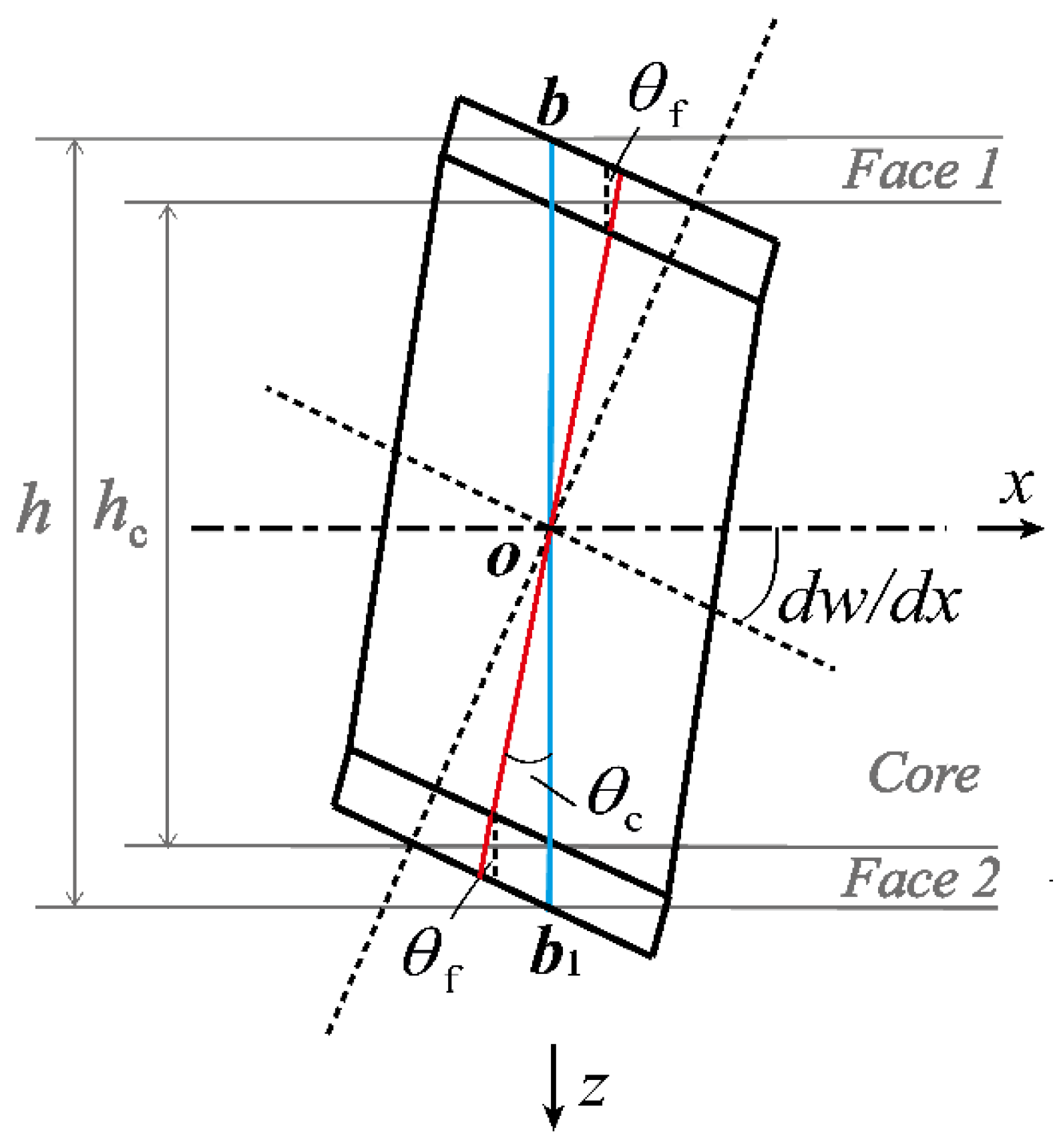
2.2. Kinematics and Constitutive Equations
- a.
- Face sheet layer 1 :
- b.
- Sandwich core layer :
- c.
- Face sheet layer 2 :
2.3. Energy Formulation
- Strain energy:
- Potential energy generated from IPDLs:
- Kinetic energy:
3. Solution Procedures and Validation
3.1. Solution Procedure
3.2. Validation
4. Results and Discussion
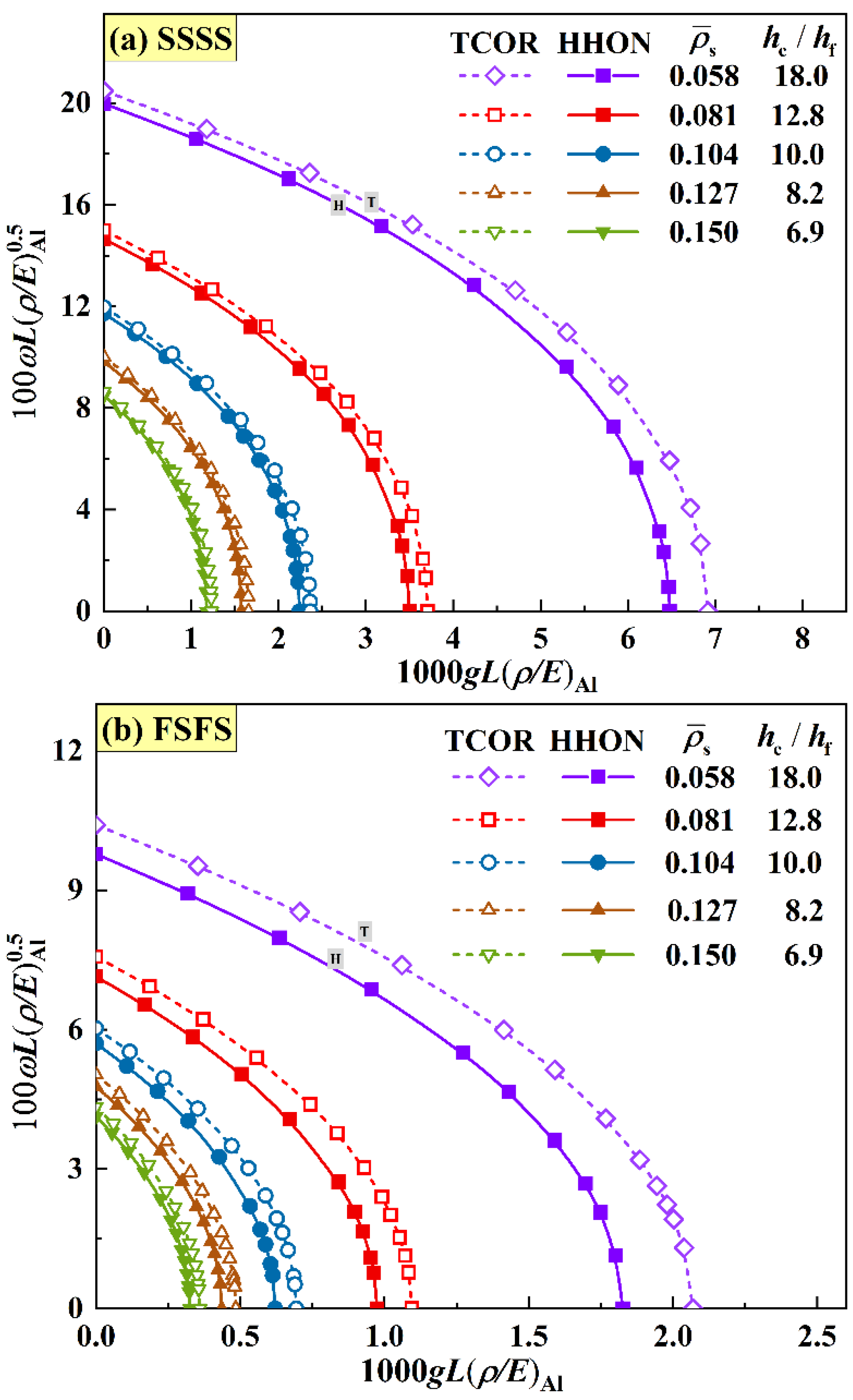
4.1. Effect of Boundary Condition (BC)
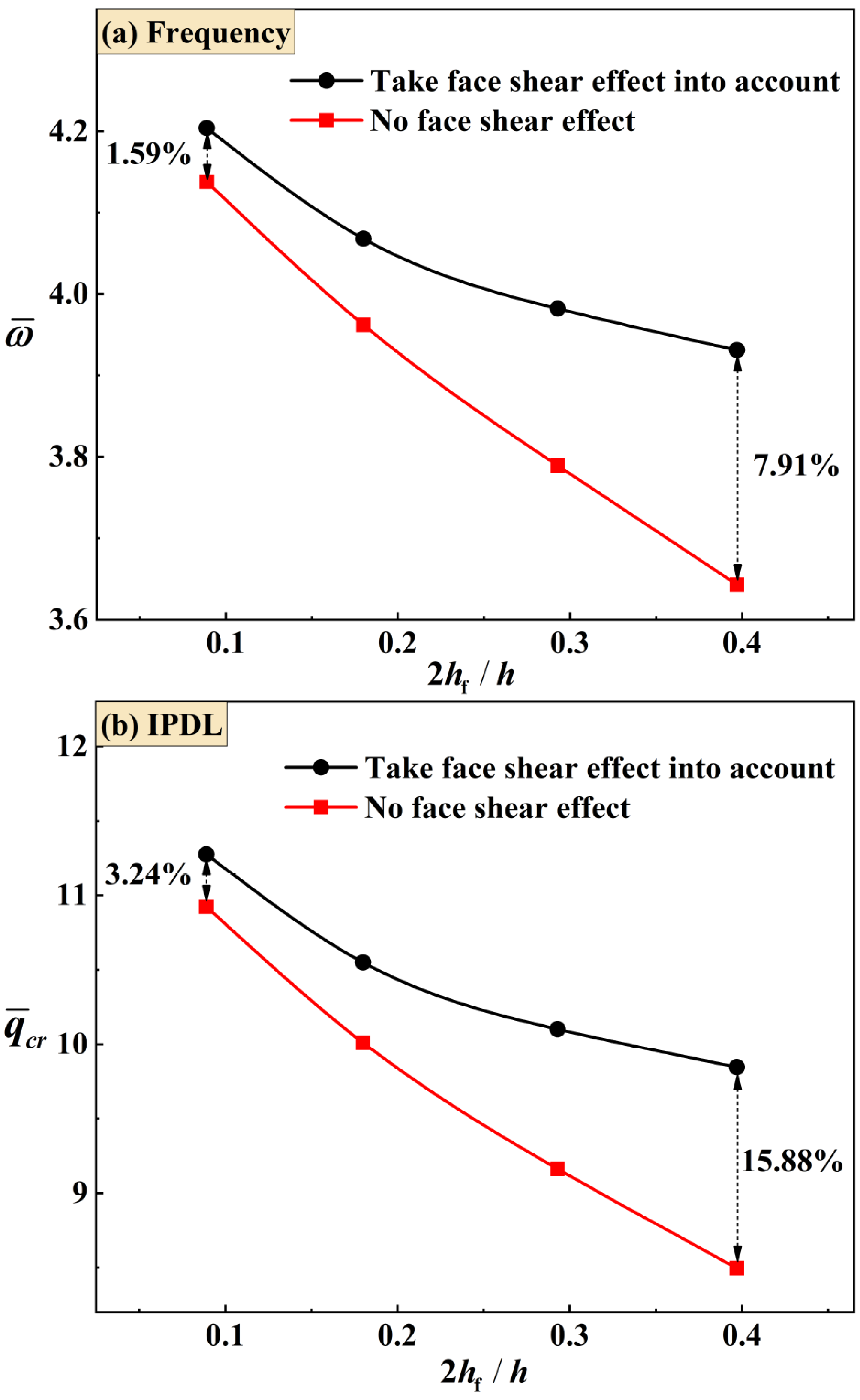
4.2. Effect of Geometric Parameters
4.3. Comparison between Metal and Composite Face Sheets
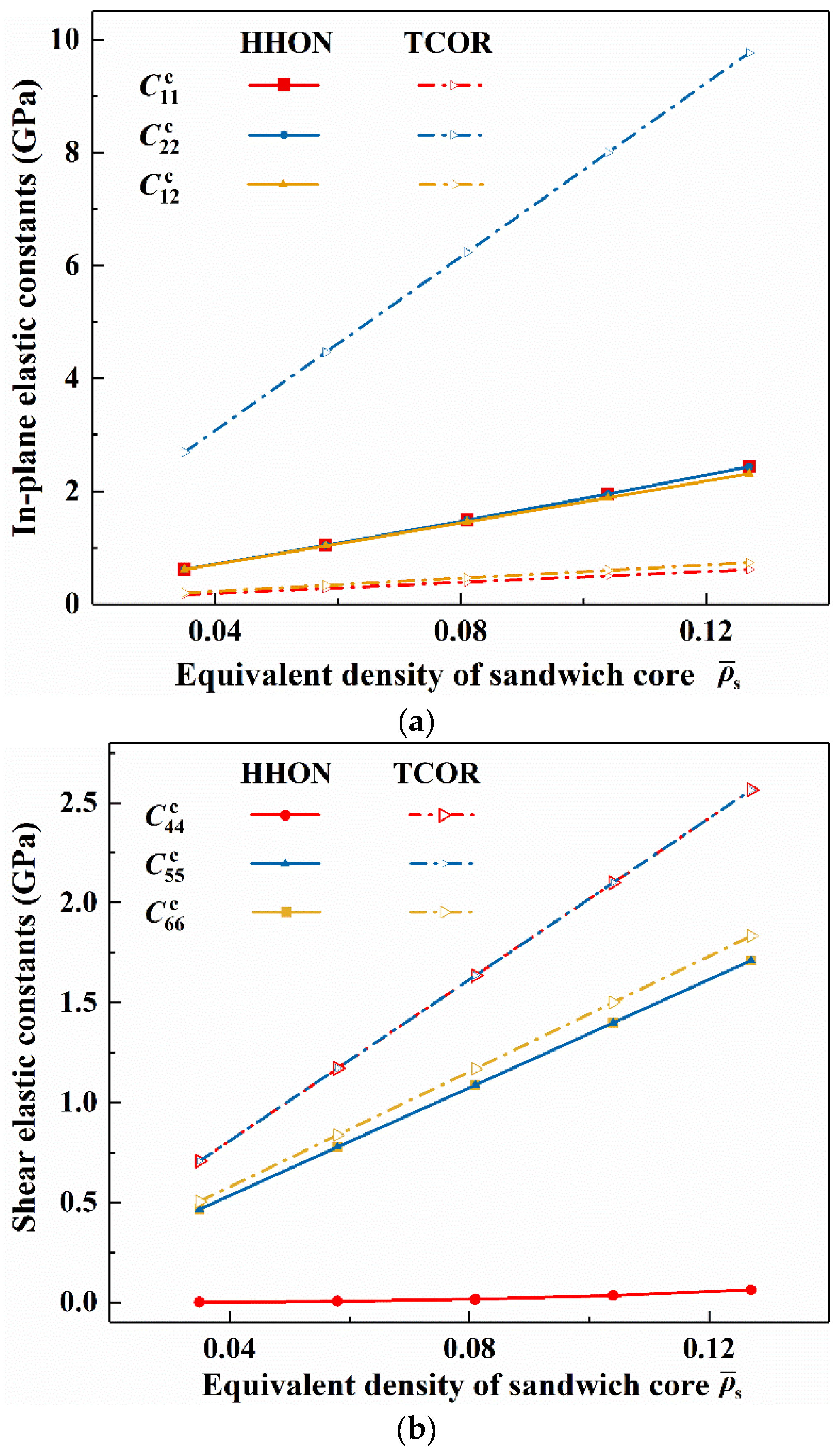
4.4. Effect of Sandwich Core Type
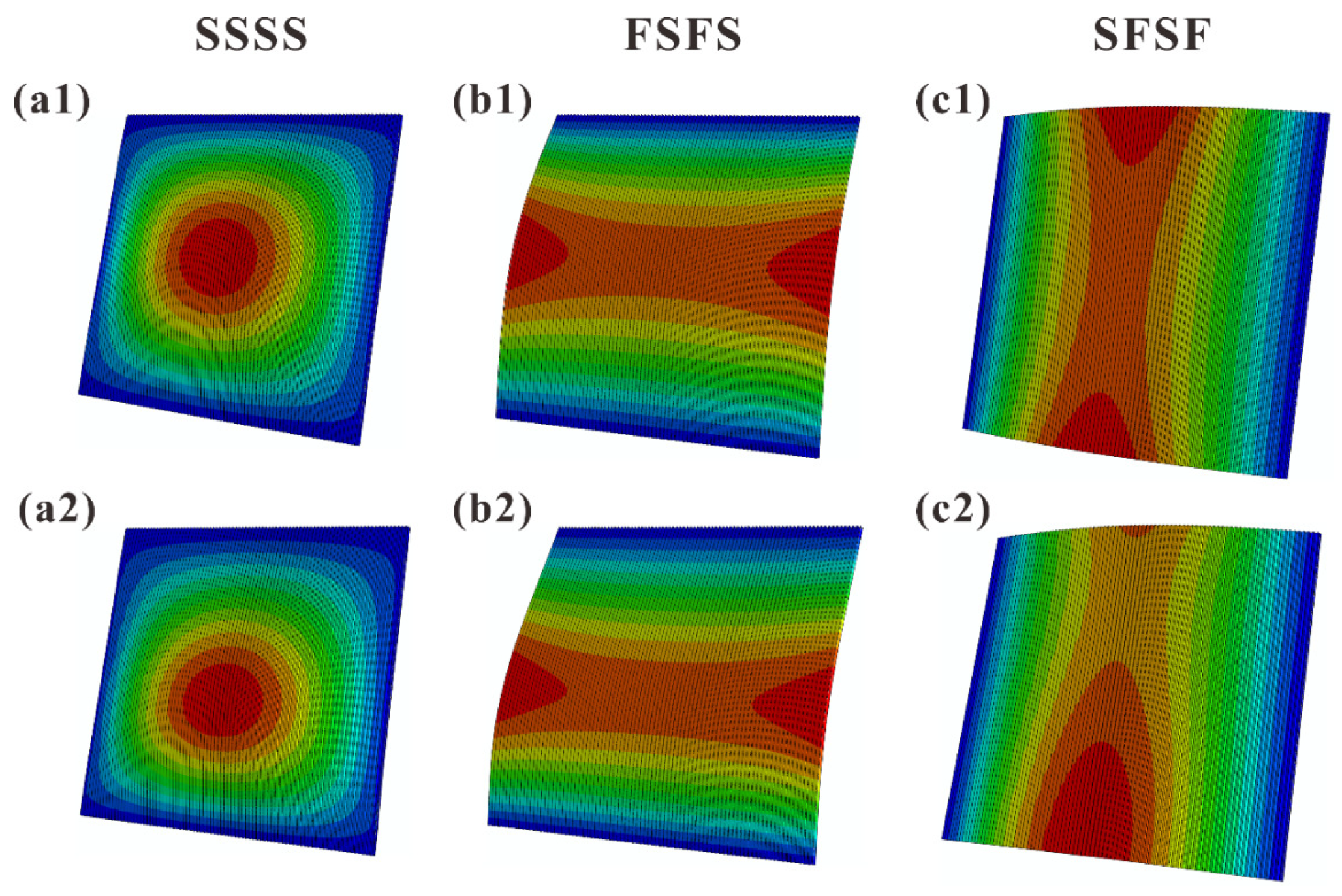
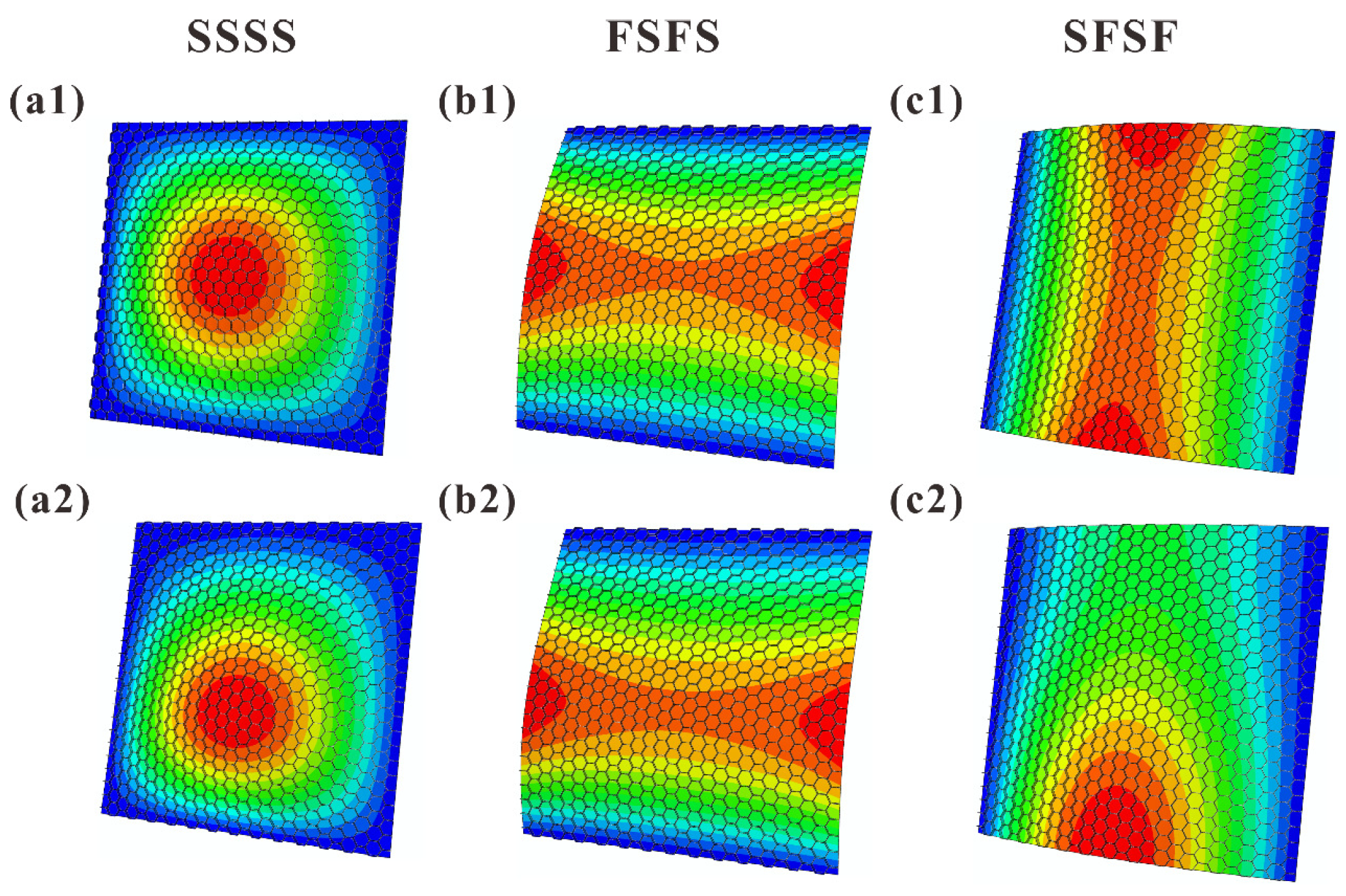
4.5. Comparison of Modal Shapes
5. Conclusions
- (1)
- The fundamental frequency and critical buckling IPDL of a sandwich plate are both much higher than a monolithic plate with equal mass. As the sandwich core, TCOR and HHON are more efficient in enhancing the structural stability than the foam.
- (2)
- For TCOR metal sandwich plates, the frequency and critical buckling IPDL are not sensitive to the inclination angle of corrugation. However, the frequency and critical buckling IPDL of both TCOR and HHON sandwich plates are quite sensitive to either core density or core thickness.
- (3)
- The frequency versus IPDL curves and the vibration modal shapes are quite different for sandwich plates under different BCs (i.e., SSSS, SFSF, and FSFS). Especially for the case under SFSF BC, the vibration modal may shift as the IPDL is increased.
- (4)
- Using fiber-reinforced composite face sheets in lieu of metal face sheets enhances the performance of the HHON sandwich plate in terms of fundamental frequency and critical buckling IPDL. However, such enhancement occurs only under partial BCs with specific types of fiber stacking.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Equivalent Elastic Constants of Sandwich Cores
Appendix B. Elements of Structural Matrix K
Appendix C. Elements of Mass Matrix M
References
- Siddhardha, K. Autonomous reduced-gravity enabling quadrotor test-bed: Design, modelling and flight test analysis. Aerosp. Sci. Technol. 2019, 86, 64–77. [Google Scholar] [CrossRef]
- Zhao, Z.; Zhao, H.; Chang, Z.; Feng, X. Analysis of bending and buckling of pre-twisted beams: A bioinspired study. Acta Mech. Sin. 2014, 30, 507–515. [Google Scholar] [CrossRef]
- Buffone, C.; Grishaev, V.; Glushchuk, A. Experimental investigation of liquid retention in a cyclone evaporator under variable gravity conditions. Appl. Therm. Eng. 2016, 99, 235–243. [Google Scholar] [CrossRef]
- Yao, Z.; Zhao, R.; Zega, V.; Corigliano, A. A metaplate for complete 3D vibration isolation. Eur. J. Mech. A Solids 2020, 84, 104016. [Google Scholar] [CrossRef]
- Bahaadini, R.; Saidi, A.R. Stability analysis of thin-walled spinning reinforced pipes conveying fluid in thermal environment. Eur. J. Mech. A Solids 2018, 72, 298–309. [Google Scholar] [CrossRef]
- Simons, D.A.; Leissa, A.W. Vibration of rectangular cantilever plates subjected to in-plane acceleration loads. J. Sound Vib. 1971, 17, 407–422. [Google Scholar] [CrossRef]
- Saxena, I.F.; Guzman, N.; Hui, K.; Mal, A.K. Disbond detection in a composite honeycomb structure of an aircraft vertical stabilizer by fiber Bragg grating detecting guided ultrasound waves. J. Mech. Eng. Sci. 2017, 231, 3001–3010. [Google Scholar] [CrossRef]
- Xu, Q.; Liu, J. An improved dynamic model for silicone material beam with large deformation. Acta Mech. Sin. 2018, 34, 744–753. [Google Scholar] [CrossRef]
- Wang, T.M.; Sussaman, J.M. Elastic stability of a simply supported plate under linearly variable compressive stresses. AIAA J. 1967, 5, 1362–1364. [Google Scholar] [CrossRef]
- Han, B.; Li, F.H.; Zhang, Q.C.; Chen, C.Q.; Lu, T.J. Stability and initial post-buckling analysis of a standing sandwich beam under terminal force and self-weight. Arch. Appl. Mech. 2016, 86, 1063–1082. [Google Scholar] [CrossRef]
- Fauconneau, G.; Marangoni, R.D. Natural frequencies and elastic stability of a simply-supported rectangular plate under linearly varying compressive loads. Int. J. Solids Struct. 1971, 7, 473–493. [Google Scholar] [CrossRef]
- Brown, C.J. Elastic buckling of plates subjected to distributed tangential loads. Comput. Struct. 1991, 41, 151–1565. [Google Scholar] [CrossRef]
- Wang, C.M.; Xiang, Y.; Wang, C.Y. Buckling of standing vertical plates under body forces. Int. J. Struct. Stab. Dyn. 2002, 2, 151–161. [Google Scholar] [CrossRef]
- Wang, C.Y. Buckling of a heavy standing plate with top load. Thin Walled Struct. 2010, 48, 127–133. [Google Scholar] [CrossRef]
- Bodaghi, M.; Saidi, A.R. Buckling behavior of standing laminated Mindlin plates subjected to body force and vertical loading. Compos. Struct. 2011, 93, 538–547. [Google Scholar] [CrossRef]
- Yu, L.H.; Wang, C.Y. Fundamental frequency of a standing heavy plate with vertical simply-supported edges. J. Sound Vib. 2009, 321, 1–7. [Google Scholar] [CrossRef]
- Yu, L.H.; Wang, C.Y. Vibration of a standing plate with simply supported vertical sides and weakened by a horizontal hinge. Thin Walled Struct. 2011, 49, 899–901. [Google Scholar] [CrossRef]
- Wang, W.B.; Yang, X.H.; Han, B.; Zhang, Q.C.; Wang, X.F.; Lu, T.J. Analytical design of effective thermal conductivity for fluid-saturated prismatic cellular metal honeycombs. Theor. Appl. Mech. Lett. 2016, 6, 69–75. [Google Scholar] [CrossRef]
- Han, B.; Yue, Z.S.; Wu, H.; Zhang, Q.; Lu, T.J. Superior compressive performance of hierarchical origami-corrugation metallic sandwich structures based on selective laser melting. Compos. Struct. 2022, 300, 116181. [Google Scholar] [CrossRef]
- Tang, Y.F.; Li, F.H.; Xin, F.; Lu, T.J. Heterogeneously perforated honeycomb-corrugation hybrid sandwich panel as sound absorber. Mater. Des. 2017, 134, 502–512. [Google Scholar] [CrossRef]
- Wu, H.X.; Liu, Y.; Zhang, X.C. In-plane crushing behavior and energy absorption design of composite honeycombs. Acta Mech. Sin. 2018, 34, 1108–1123. [Google Scholar] [CrossRef]
- Wang, X.; Li, X.; Yue, Z.S.; Zhang, Q.C.; Du, S.F.; Yang, Z.K.; Han, B.; Lu, T.J. Optimal design of metallic corrugated sandwich panels with polyurea-metal laminate face sheets for simultaneous vibration attenuation and structural stiffness. Compos. Struct. 2021, 256, 112994. [Google Scholar] [CrossRef]
- Li, F.H.; Han, B.; Zhang, Q.C.; Jin, F.; Lu, T.J. Buckling of a standing corrugated sandwich plate subjected to body force and terminal load. Thin Walled Struct. 2018, 127, 688–699. [Google Scholar] [CrossRef]
- Volmir, A.S. Stability of sandwich plates and shells. In Stability of Deformable S.; Nauka Publishing House: Moscow, Russia, 1967; Chapter 19. (In Russian) [Google Scholar]
- Liew, K.M.; Pan, Z.Z.; Zhang, L.W. An overview of layerwise theories for composite laminates and structures: Development, numerical implementation and application. Compos. Struct. 2019, 216, 240–259. [Google Scholar] [CrossRef]
- Sayyad, A.S.; Ghugal, Y.M. Bending, buckling and free vibration of laminated composite and sandwich beams: Acritical review of literature. Compos. Struct. 2017, 171, 486–504. [Google Scholar] [CrossRef]
- Iurlaro, L.; Gherlone, M.; Di Sciuva, M.; Tessler, A. Assessment of the refined zigzag theory for bending, vibration, and buckling of sandwich plates: A comparative study of different theories. Compos. Struct. 2013, 106, 777–792. [Google Scholar] [CrossRef]
- Akif, K.; Mehmet, D.; Timon, R. A novel mixed finite element formulation based on the refined zigzag theory for the stress analysis of laminated composite plates. Compos. Struct. 2021, 267, 113886. [Google Scholar]
- Lekhnitskii, S.G. Strength calculation of composite beams. Vestn. Inzhen Tekhnikov 1935, 9, 137–148. [Google Scholar]
- Di Sciuva, M. Bending, vibration and buckling of simply supported thick multilayered orthortopic plates. J. Sound Vib. 1986, 105, 425–442. [Google Scholar] [CrossRef]
- Tessler, A.; Di Sciuva, M.; Gherlone, M. A refined zigzag beam theory for composite and sandwich beams. J. Compos. Mater. 2009, 43, 1051–1081. [Google Scholar] [CrossRef]
- Iurlaro, L.; Ascione, A.; Gherlone, M. Free vibration analysis of sandwich beams using the refined zigzag theory: An experimental assessment. Meccanica 2015, 50, 2525–2535. [Google Scholar] [CrossRef]
- Iurlaro, L.; Gherlone, M.; Di Sciuva, M. Bending and free vibration analysis of functionally graded sandwich plates using the refined zigzag theory. J. Sandw. Struct. Mater. 2014, 16, 669–699. [Google Scholar] [CrossRef]
- Nguyen, S.N.; Lee, J.; Cho, M. Atriangular finite element using Laplace transform for viscoelastic laminated composite plates based on efficient higher-order zigzag theory. Compos. Struct. 2016, 155, 223–244. [Google Scholar] [CrossRef]
- Dorduncu, M. Stress analysis of sandwich plates with functionally graded cores using peridynamic differential operator and refined zigzag theory. Thin Walled Struct. 2020, 146, 106468. [Google Scholar] [CrossRef]
- Di Sciuva, M.; Sorrenti, M. Bending, free vibration and buckling of functionally graded carbon nanotube-reinforced sandwich plates, using the extended refined zigzag theory. Compos. Struct. 2019, 227, 111324. [Google Scholar] [CrossRef]
- Han, B.; Hui, W.W.; Zhang, Q.C.; Zhao, Z.Y.; Jin, F.; Zhang, Q.; Lu, T.J.; Lu, B.H. A refined quasi-3D zigzag beam theory for free vibration and stability analysis of multilayered composite beams subjected to thermomechanical loading. Compos. Struct. 2018, 204, 620–633. [Google Scholar] [CrossRef]
- Allen, H.G. Analysis and Design of Structural Sandwich Panels; Pergamon Press: Oxford, UK, 1969. [Google Scholar]
- Lou, J.; Ma, L.; Wu, L.Z. Free vibration analysis of simply supported sandwich beams with lattice truss core. Mater. Sci. Eng. B 2012, 177, 1712–1716. [Google Scholar] [CrossRef]
- Wang, C.M.; Aung, T.M. Plastic buckling analysis of thick plates using p-Ritz method. Int. J. Solids Struct. 2007, 44, 6239–6255. [Google Scholar] [CrossRef]
- Li, M.; Wu, L.Z.; Ma, L. Structural response of all-composite pyramidal truss core sandwich columns in end compression. Compos. Struct. 2011, 93, 1964–1972. [Google Scholar] [CrossRef]
- Han, B.; Qin, K.K.; Zhang, Q.C.; Zhang, Q.; Lu, T.J.; Lu, B.H. Free vibration and buckling of foam-filled composite corrugated sandwich plates under thermal loading. Compos. Struct. 2017, 172, 173–189. [Google Scholar] [CrossRef]
- Malek, S.; Gibson, L. Effective elastic properties of periodic HHONs. Mech. Mater. 2015, 91, 226–240. [Google Scholar] [CrossRef]
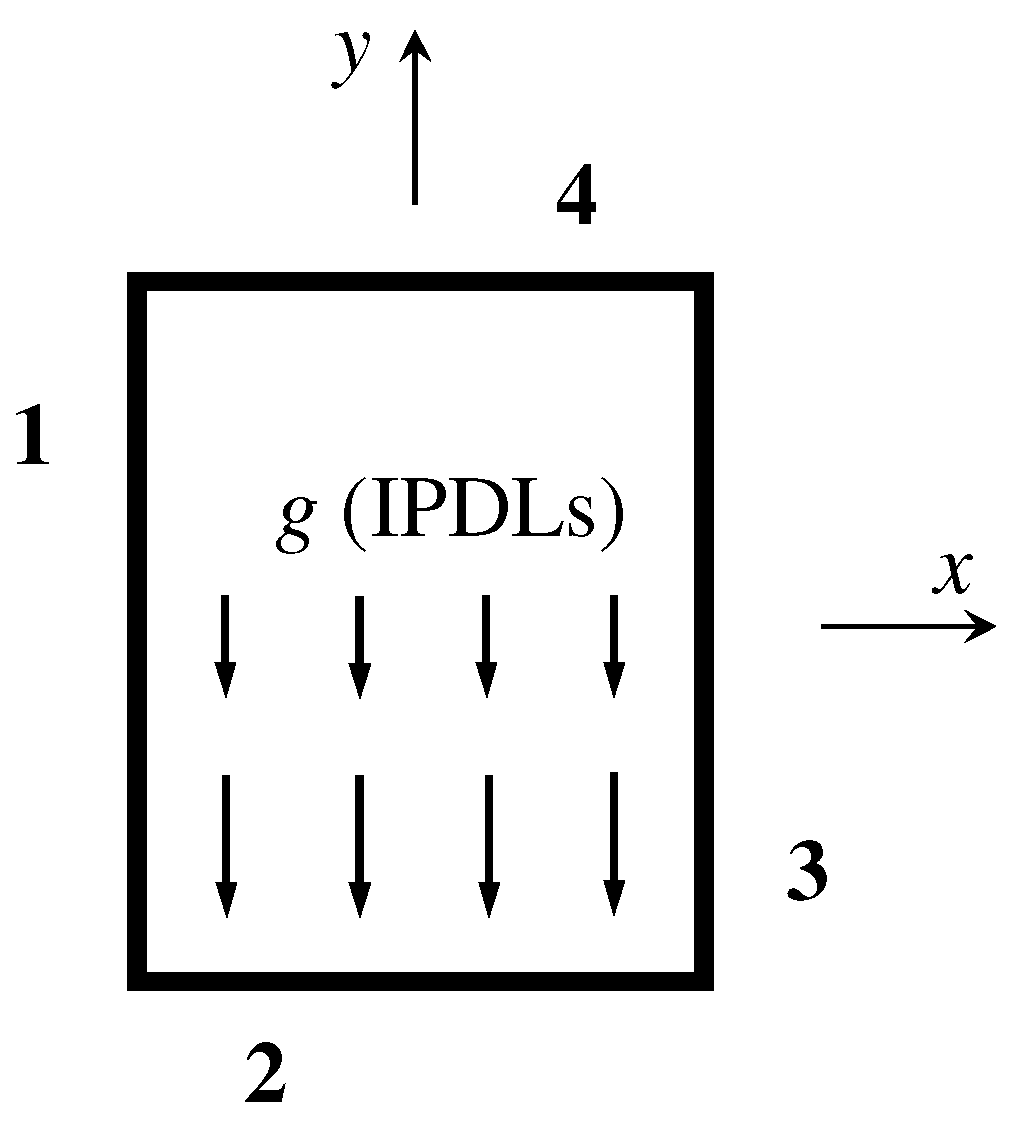






| BC | |||
|---|---|---|---|
| SSSS (1234) | 0,0,1,1 | 1,1,0,0 | 1,1,1,1 |
| FSFS (1234) | 0,0,1,1 | 0,0,0,0 | 0,0,1,1 |
| SFSF (1234) | 0,0,0,0 | 1,1,0,0 | 1,1,0,0 |
| SCFF a (1234 b) | 0,0,0,1 | 0,1,0,1 | 0,1,0,1 |
| Case | Method | SSSF | SFSF | SCSF | SSSS |
|---|---|---|---|---|---|
| FE* (L/h = 40) | 40.208 | 38.168 | 40.692 | 47.859 | |
| FE (L/h = 100) | 40.526 | 38.353 | 41.011 | 48.414 | |
| Present study (L/h = 40) | 40.998 | 38.776 | 41.486 | 49.098 | |
| Present study (L/h = 100) | 41.165 | 38.913 | 41.667 | 49.308 | |
| Yu et al. [16,17] | 41.204 | 38.950 | 41.706 | 49.351 | |
| FE (L/h = 40) | 195.646 | 87.064 | 280.818 | 195.858 | |
| FE (L/h = 100) | 203.092 | 93.052 | 287.700 | 203.372 | |
| Present study (L/h = 40) | 210.304 | 99.528 | 299.924 | 210.583 | |
| Present study (L/h = 100) | 213.167 | 101.303 | 305.095 | 213.468 | |
| Wang et al. [13,14] | 213.72 | -- | 306.09 | 214.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, F.-H.; Han, B.; Zhang, A.-H.; Liu, K.; Wang, Y.; Lu, T.-J. A Three-Dimensional Vibration Theory for Ultralight Cellular Sandwich Plates Subjected to Linearly Varying In-Plane Distributed Loads. Materials 2023, 16, 4086. https://doi.org/10.3390/ma16114086
Li F-H, Han B, Zhang A-H, Liu K, Wang Y, Lu T-J. A Three-Dimensional Vibration Theory for Ultralight Cellular Sandwich Plates Subjected to Linearly Varying In-Plane Distributed Loads. Materials. 2023; 16(11):4086. https://doi.org/10.3390/ma16114086
Chicago/Turabian StyleLi, Fei-Hao, Bin Han, Ai-Hua Zhang, Kai Liu, Ying Wang, and Tian-Jian Lu. 2023. "A Three-Dimensional Vibration Theory for Ultralight Cellular Sandwich Plates Subjected to Linearly Varying In-Plane Distributed Loads" Materials 16, no. 11: 4086. https://doi.org/10.3390/ma16114086
APA StyleLi, F.-H., Han, B., Zhang, A.-H., Liu, K., Wang, Y., & Lu, T.-J. (2023). A Three-Dimensional Vibration Theory for Ultralight Cellular Sandwich Plates Subjected to Linearly Varying In-Plane Distributed Loads. Materials, 16(11), 4086. https://doi.org/10.3390/ma16114086






