Influence of Composite Structure on Temperature Distribution—An Analysis Using the Finite Difference Method
Abstract
:1. Introduction
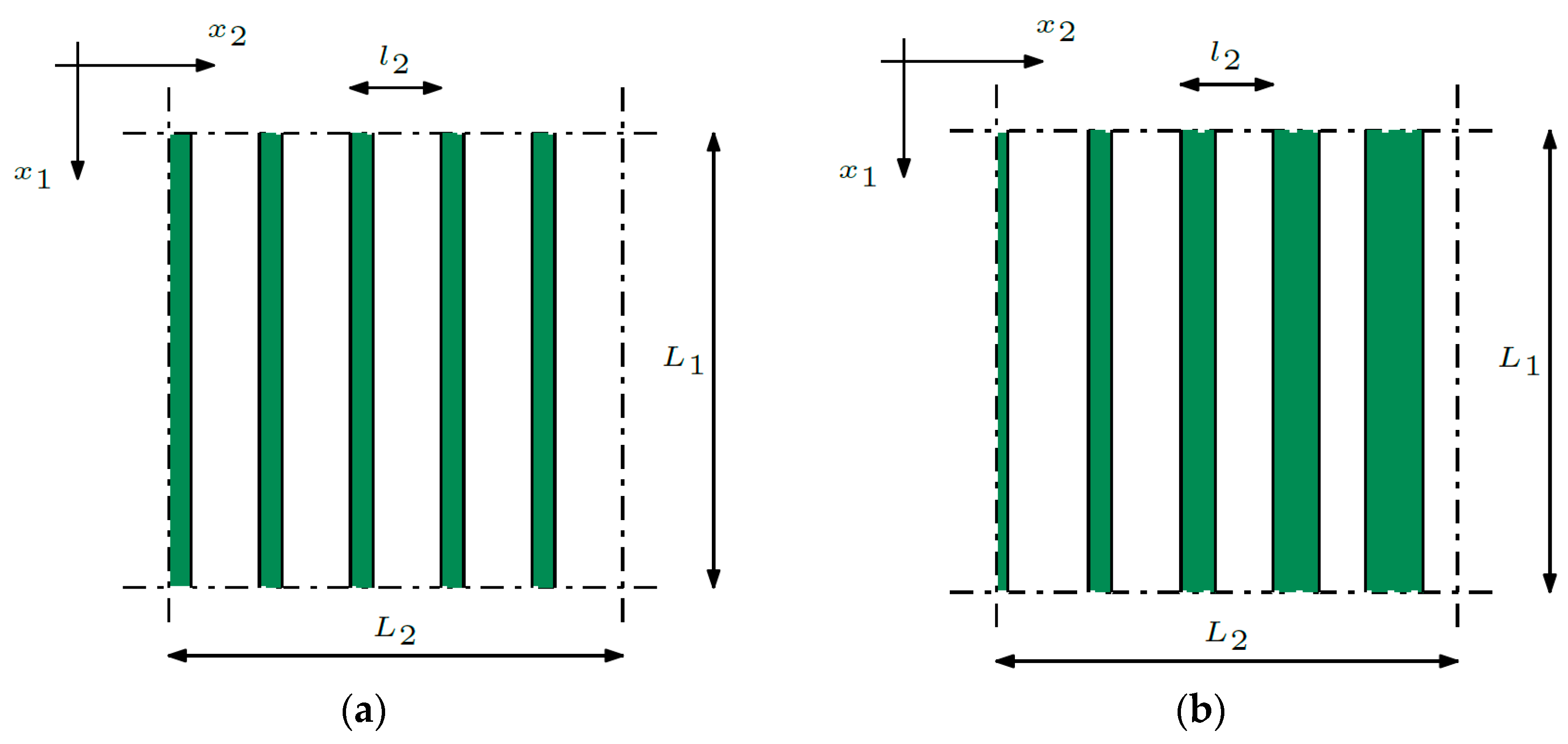
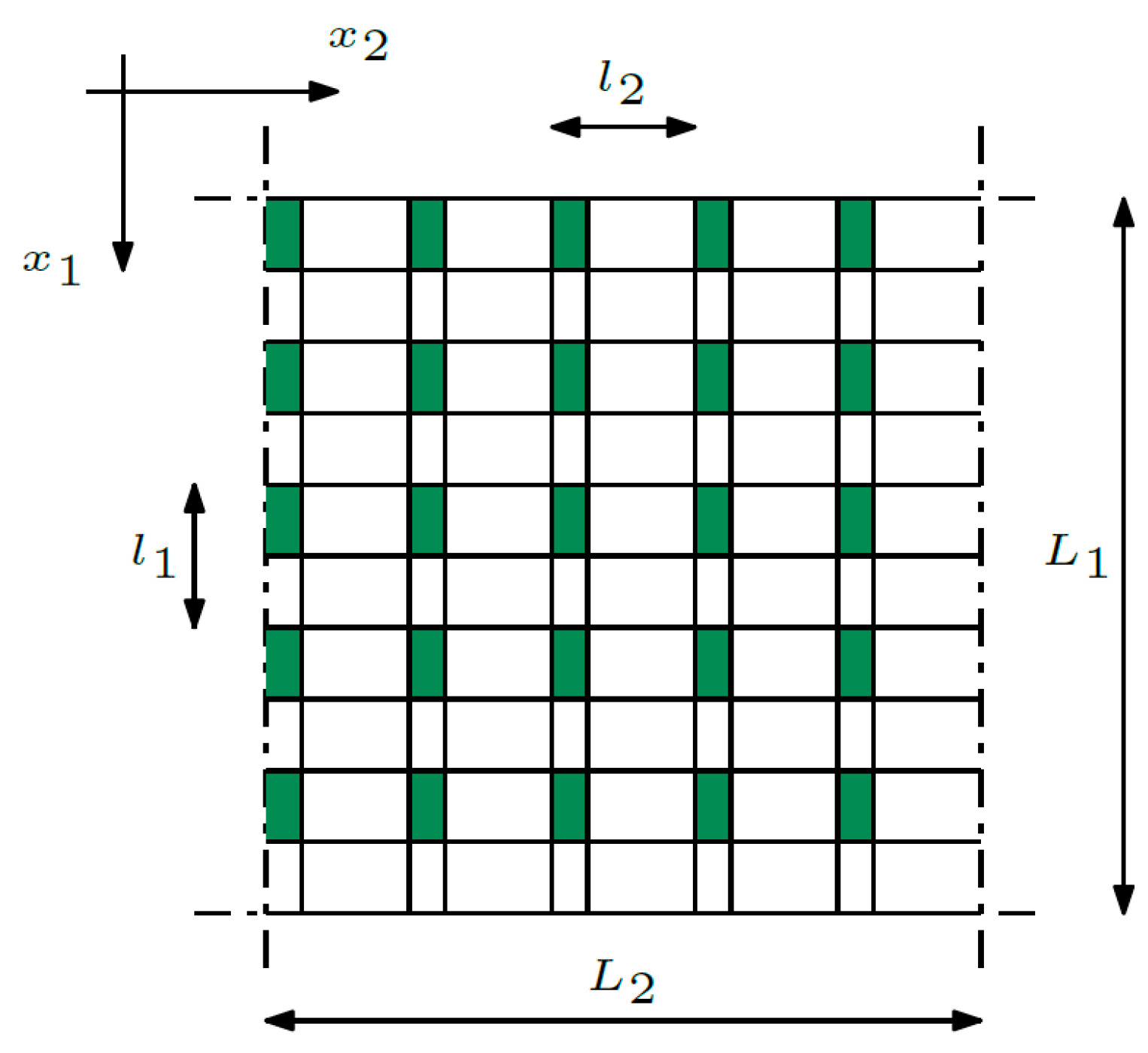
2. Various Composite Structures of Interest
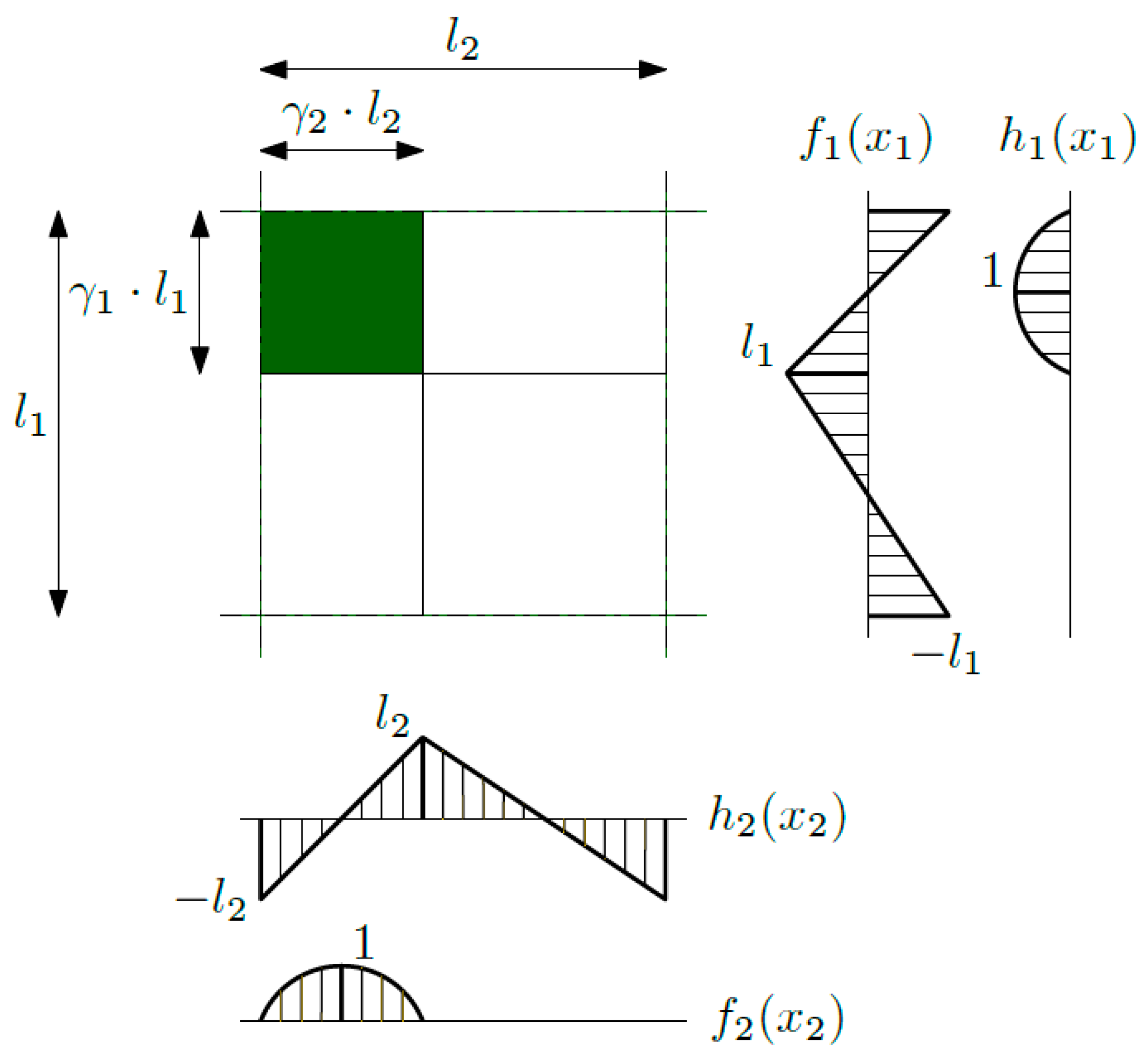
3. Tolerance Averaging Technique
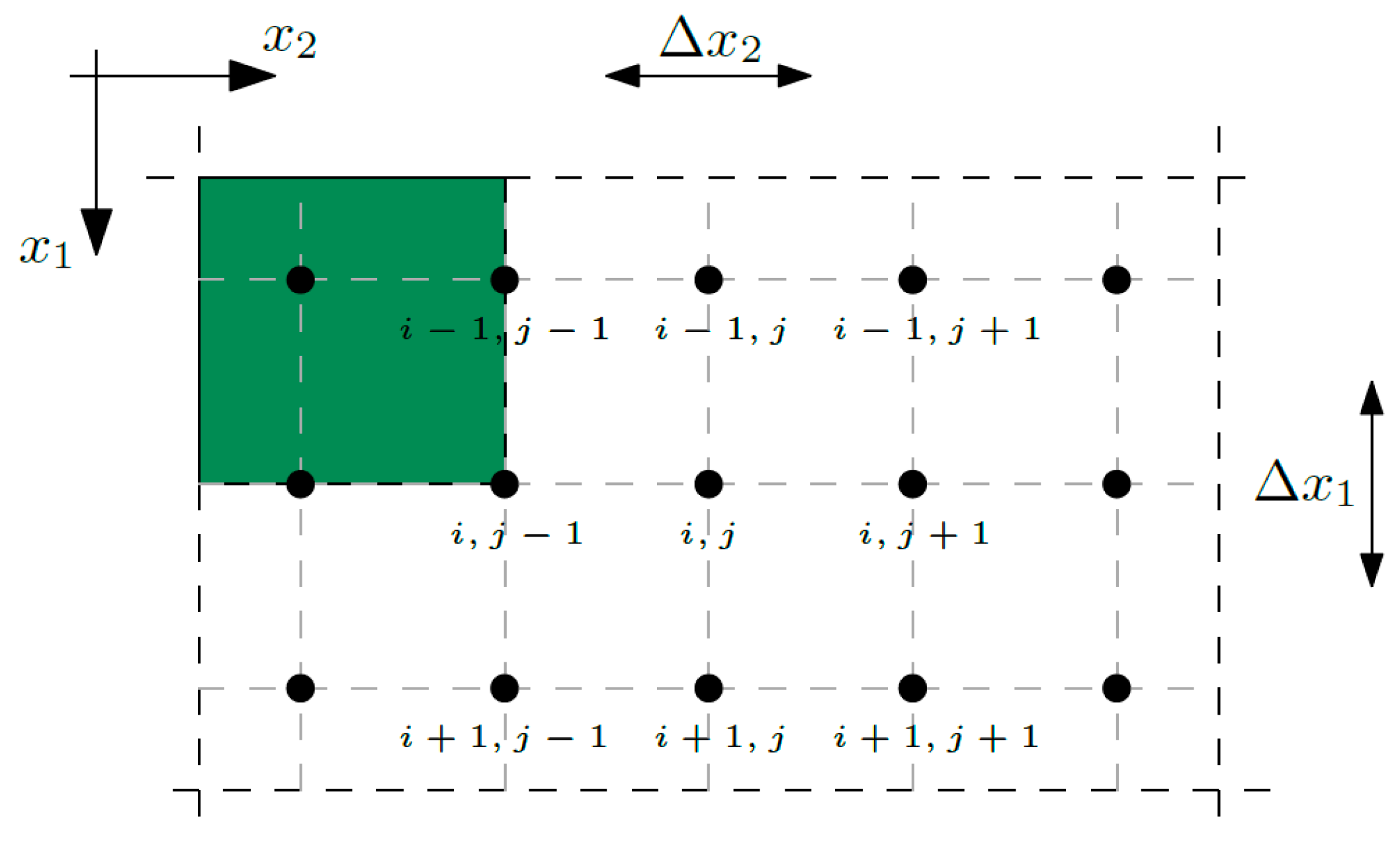
4. Finite Difference Method
5. Results
5.1. Periodic and Biperiodic Structure
5.2. Periodic and Functionally Graded Structure
5.3. Convergence Study
5.3.1. Biperiodic Structure
5.3.2. Periodic Structure
5.3.3. Functionally Graded Structure
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Santos, H.; Mota Soares, C.M.; Mota Soares, C.A.; Reddy, J.N. A semi-analytical finite element model for the analysis of cylindrical shells made of functionally graded materials under thermal shock. Compos. Struct. 2008, 86, 9–21. [Google Scholar] [CrossRef]
- Sadowski, T.; Ataya, S.; Nakonieczny, K. Thermal analysis of layered FGM cylindrical plates subjected to sudden cooling process at one side. Comparison of two applied methods for problem solution. Comput. Mater. Sci. 2009, 45, 624–632. [Google Scholar] [CrossRef]
- Bensoussan, A.; Lions, J.L.; Papanicolay, G. Asymptotic Analysis for Periodic Structures, 1st ed.; North-Holland: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Matysiak, S.J.; Nagórko, W. Microlocal parameters in the modelling of microperiodic plates. Ing. Arch. 1989, 59, 434–444. [Google Scholar] [CrossRef]
- Matysiak, S.J.; Perkowski, D.M. On heat conduction in periodically stratified composites with slant layering to boundaries. Therm. Sci. 2015, 19, 83–94. [Google Scholar] [CrossRef]
- Aboudi, J.; Pindera, M.J.; Arnold, S.M. A coupled higher-order theory for functionally graded composites with martial homogenization. Compos. Eng. 1995, 5, 771–792. [Google Scholar] [CrossRef]
- Aboudi, J.; Pindera, M.J.; Arnold, S.M. Higher-order theory for functionally graded materials. Compos. Part B Eng. 1999, 30, 777–832. [Google Scholar] [CrossRef]
- Sladek, J.; Sladek, V.; Zhang, C.H. Transient heat conduction analysis in functionally graded materials by the meshless local boundary integral equation method. Comput. Mater. Sci. 2003, 28, 494–504. [Google Scholar] [CrossRef]
- Woźniak, C.; Wierzbicki, E. Averaging Techniques in Thermomechanics of Composite Solids. Tolerance Averaging versus Homogenization, 1st ed.; Publishing House of Częstochowa University of Technology: Częstochowa, Poland, 2000. [Google Scholar]
- Jędrysiak, J. Termomechanika Laminatów, płyt i Powłok o Funkcyjnej Gradacji Własności, 1st ed.; Publishing House of Łódź University of Technology: Łódź, Poland, 2010. [Google Scholar]
- Domagalski, Ł. Comparison of the natural vibration frequencies of timoshenko and bernoulli periodic beams. Materials 2021, 14, 7628. [Google Scholar] [CrossRef]
- Jędrysiak, J. Theoretical Tolerance Modelling of Dynamics and Stability for Axially Functionally Graded (AFG) Beams. Materials 2023, 16, 2096. [Google Scholar] [CrossRef]
- Kaźmierczak-Sobińska, M.; Jędrysiak, J. Free Vibrations of Microstructured Functionally Graded Plate Band with Clamped Edges. Vib. Phys. Syst. 2021, 32, 2021216. [Google Scholar]
- Tomczyk, B.; Bagdasaryan, V.; Gołąbczak, M.; Litawska, A. A new combined asymptotic-tolerance model of thermoelasticity problems for thin biperiodic cylindrical shells. Compos. Mater. 2023, 309, 116708. [Google Scholar] [CrossRef]
- Tomczyk, B.; Gołąbczak, M.; Litawska, A.; Gołąbczak, A. Extended tolerance modelling of dynamic problems for thin uniperiodic cylindrical shells. Contin. Mech. Thermodyn. Link Is Disabl. 2023, 35, 183–210. [Google Scholar] [CrossRef]
- Ostrowski, P.; Jędrysiak, J. Dependence of temperature fluctuations on randomized material properties in two-component pe-riodic laminate. Compos. Struct. 2021, 257, 113171. [Google Scholar] [CrossRef]
- Nowacki, W. Elasticity Theory; National Science Publishing House: Warszawa, Poland, 1970. (In Polish) [Google Scholar]
- Domagalski, Ł. Free and forced large amplitude vibrations of periodically inhomogeneous slender beams. Arch. Civ. Mech. Eng. 2018, 18, 1506–1519. [Google Scholar] [CrossRef]
- Marczak, J. The tolerance modelling of vibrations of periodic sandwich structures—Comparison of simple modelling approaches. Eng. Struct. 2021, 234, 111845. [Google Scholar] [CrossRef]
- Tomczyk, B.; Gołąbczak, M. Tolerance and asymptotic modelling of dynamic thermoelasticity problems for thin micro-periodic cylindrical shells. Meccanica 2020, 55, 2391–2411. [Google Scholar] [CrossRef]
- Tomczyk, B.; Bagdasaryan, V.; Gołąbczak, M.; Litawska, A. Stability of thin micro-periodic cylindrical shells; extended tolerance modelling. Compos. Struct. 2020, 253, 112743. [Google Scholar] [CrossRef]
- Jędrysiak, J. Tolerance modelling of vibrations and stability for periodic slender visco-elastic beams on a foundation with damping. Revisiting. Materials 2020, 13, 3939. [Google Scholar] [CrossRef]
- Pazera, E. Heat transfer in periodically laminated structures-third type boundary conditions. Int. J. Comput. Methods 2021, 18, 2041011. [Google Scholar] [CrossRef]
- Tomczyk, B.; Bagdasaryan, V.; Gołąbczak, M.; Litawska, A. On the modelling of stability problems for thin cylindrical shells with two-directional micro-periodic structure. Compos. Struct. 2021, 275, 114495. [Google Scholar] [CrossRef]
- Kubacka, E.; Ostrowski, P. Heat conduction issue in biperiodic composite using Finite Difference Method. Compos. Struct. 2021, 261, 113310. [Google Scholar] [CrossRef]
- Kubacka, E.; Ostrowski, P. A Finite Difference Algorithm Applied to the Averaged Equations of the Heat Conduction Issue in Biperiodic Composites—Robin Boundary Conditions. Materials 2021, 14, 6329. [Google Scholar] [CrossRef] [PubMed]
- Jędrysiak, J.; Kaźmierczak-Sobińska, M. Theoretical analysis of buckling for functionally graded thin plates with microstructure resting on an elastic foundation. Materials 2020, 13, 4031. [Google Scholar] [CrossRef]
- Pazera, E.; Jędrysiak, J. Thermomechanical analysis of functionally graded laminates using tolerance approach. AIP Conf. Proc. 2018, 1922, 140001. [Google Scholar]
- Marczak, J. Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core. Materials 2022, 15, 7611. [Google Scholar] [CrossRef] [PubMed]

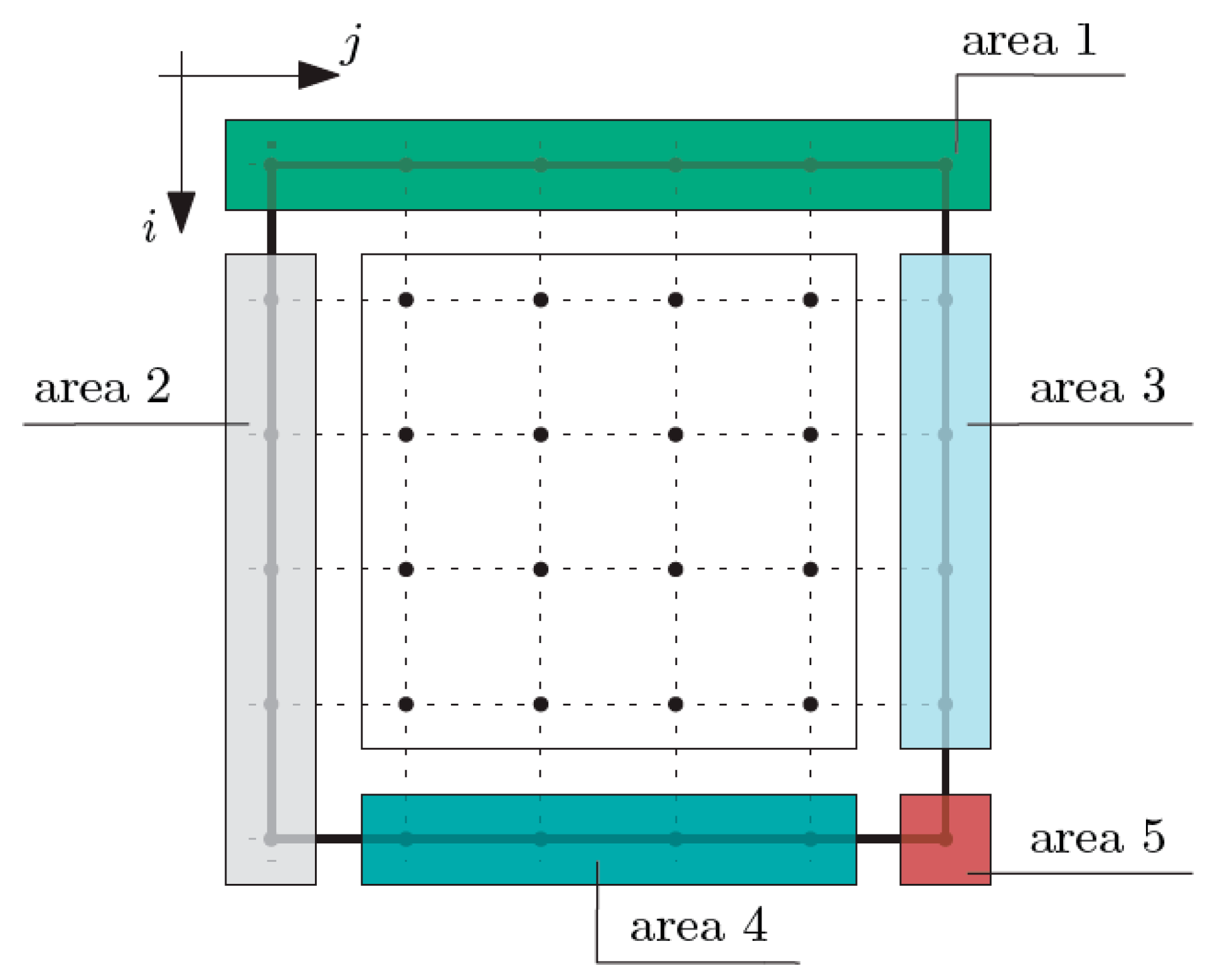
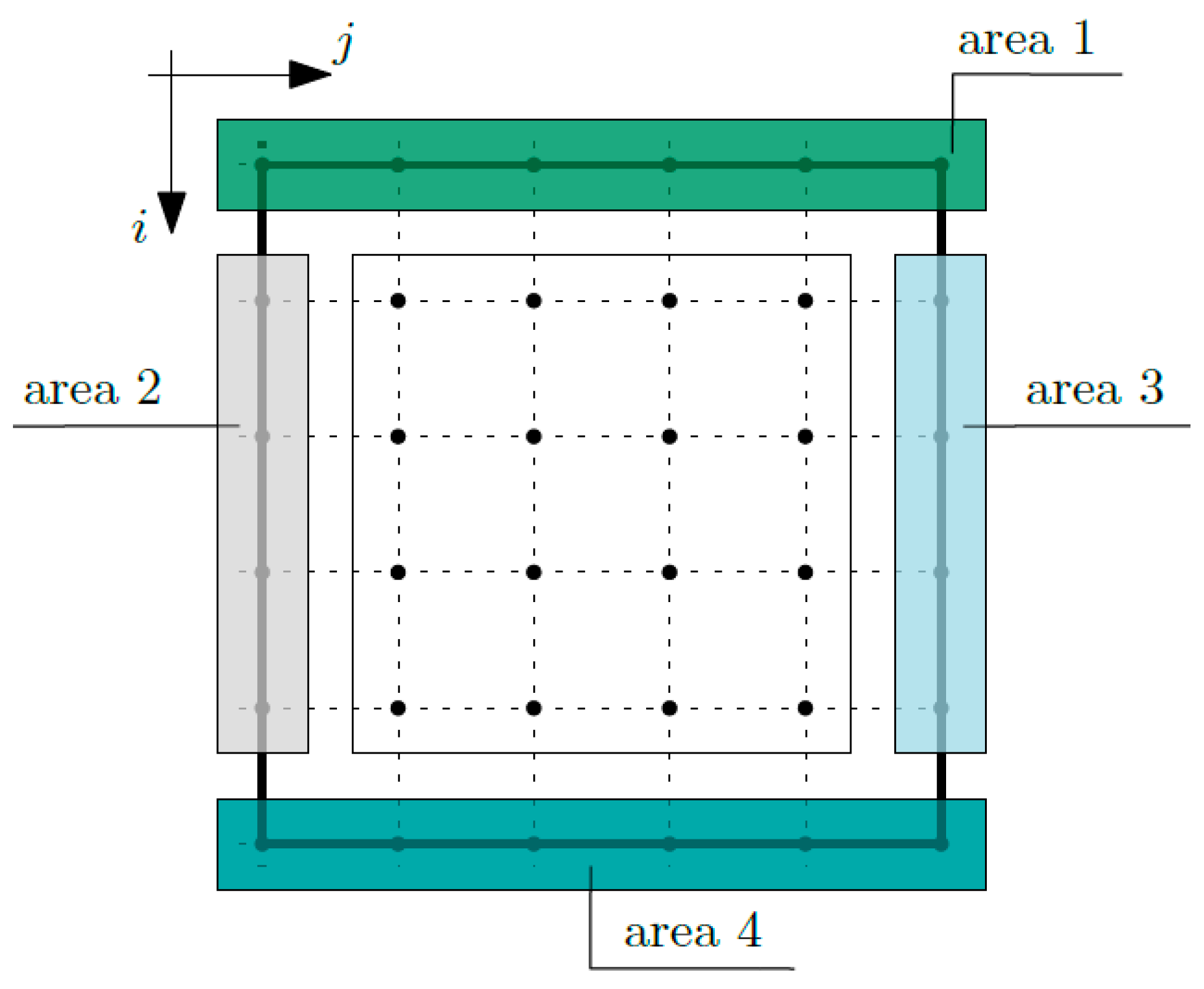
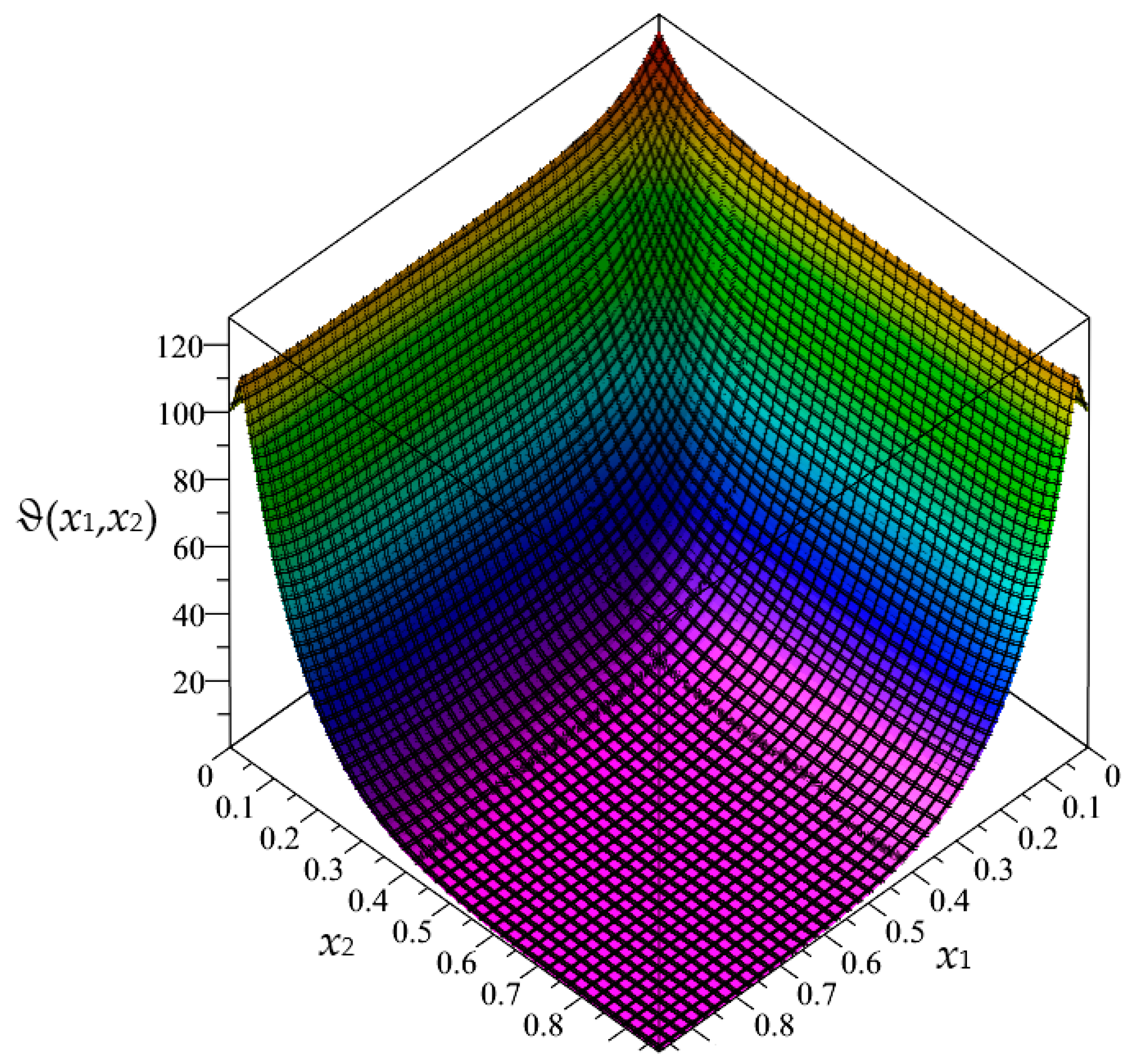


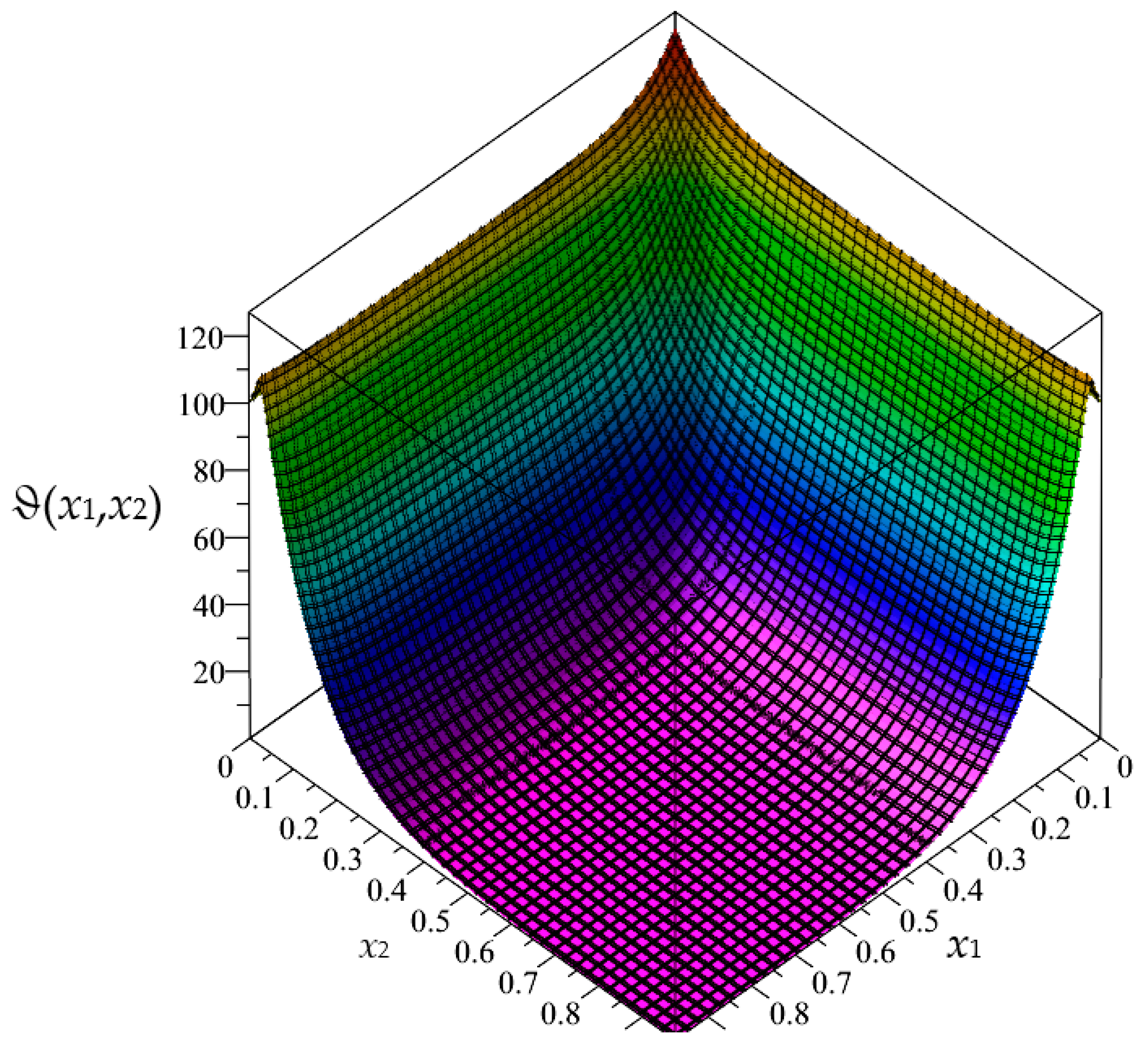
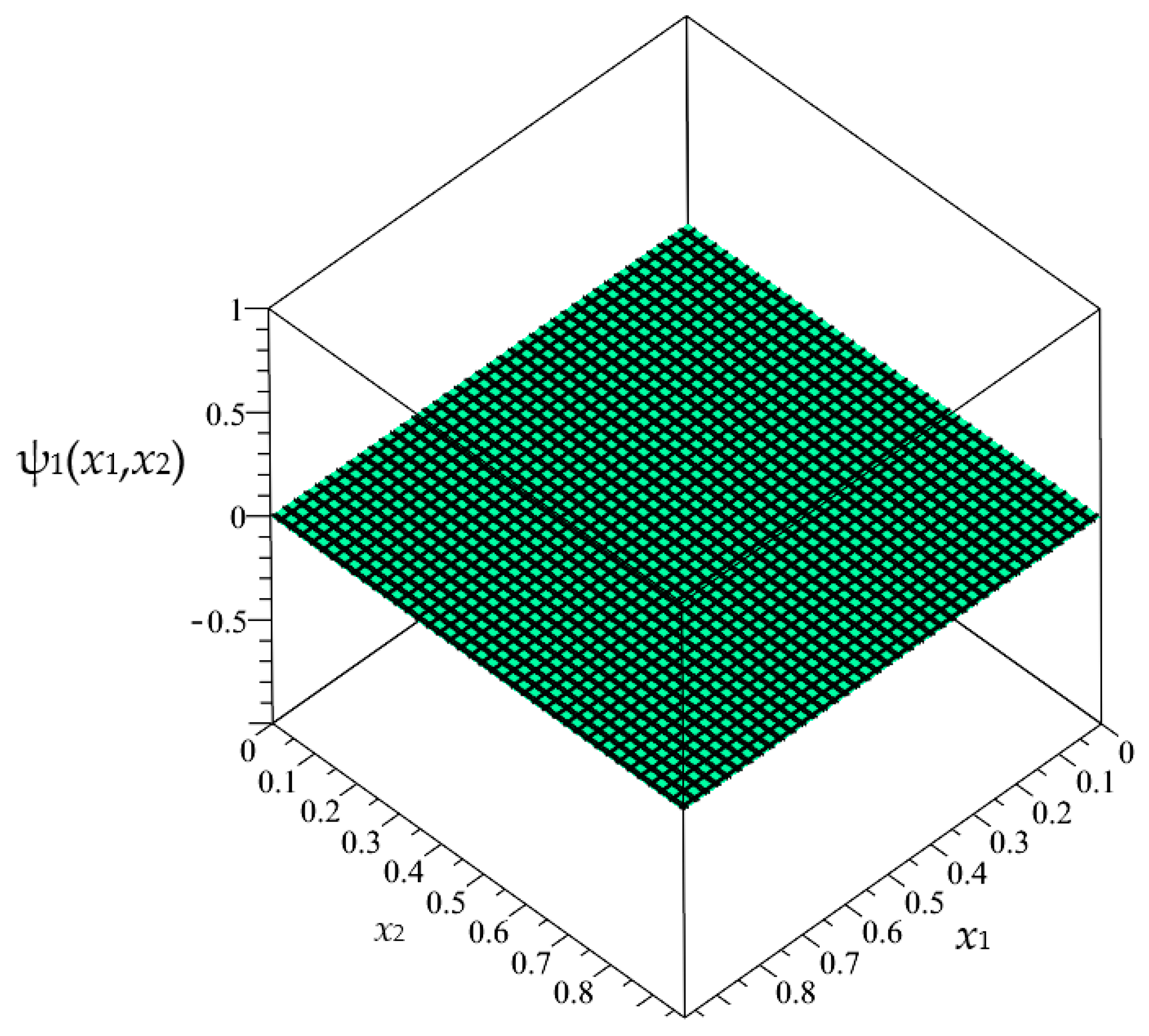
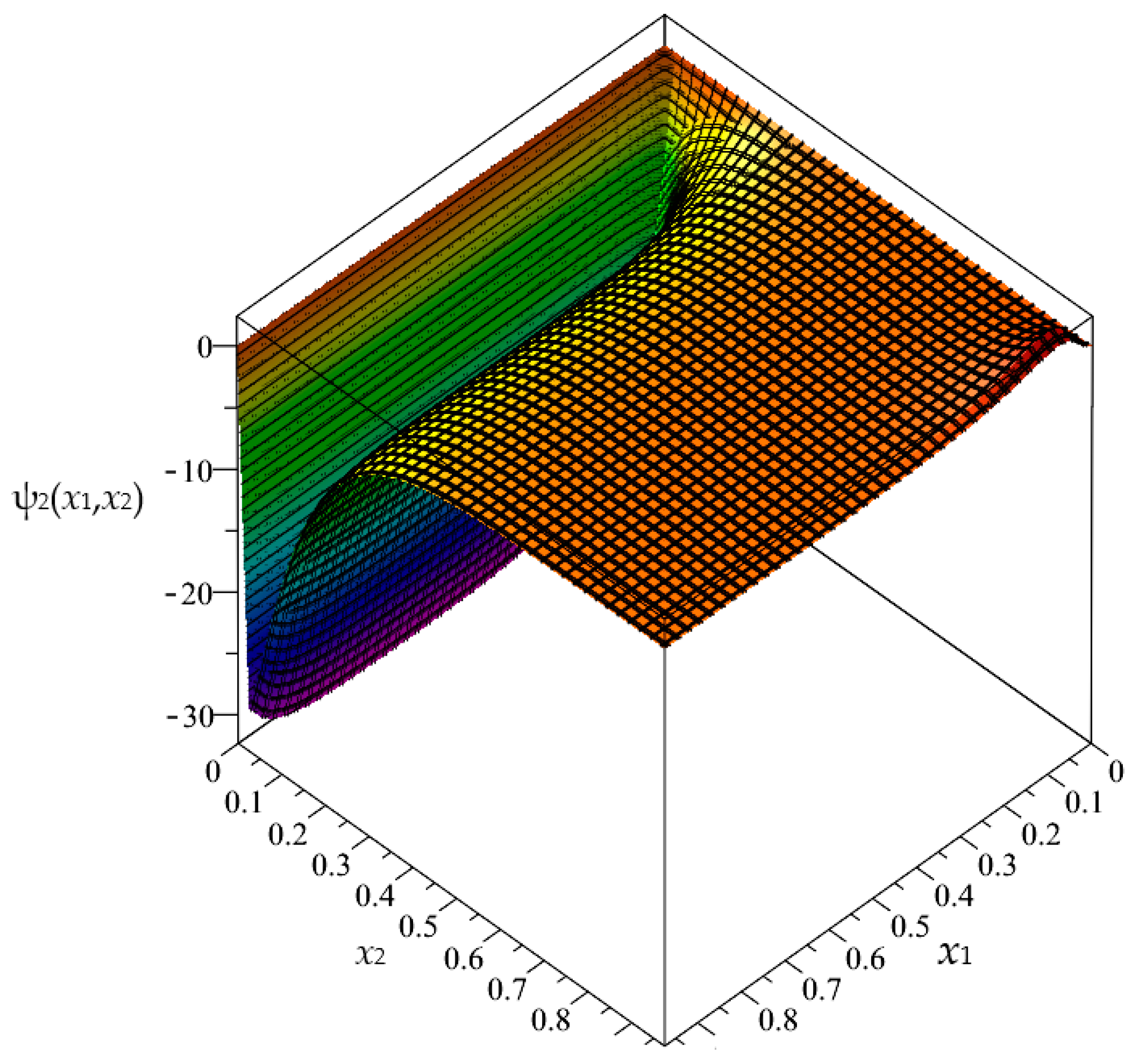

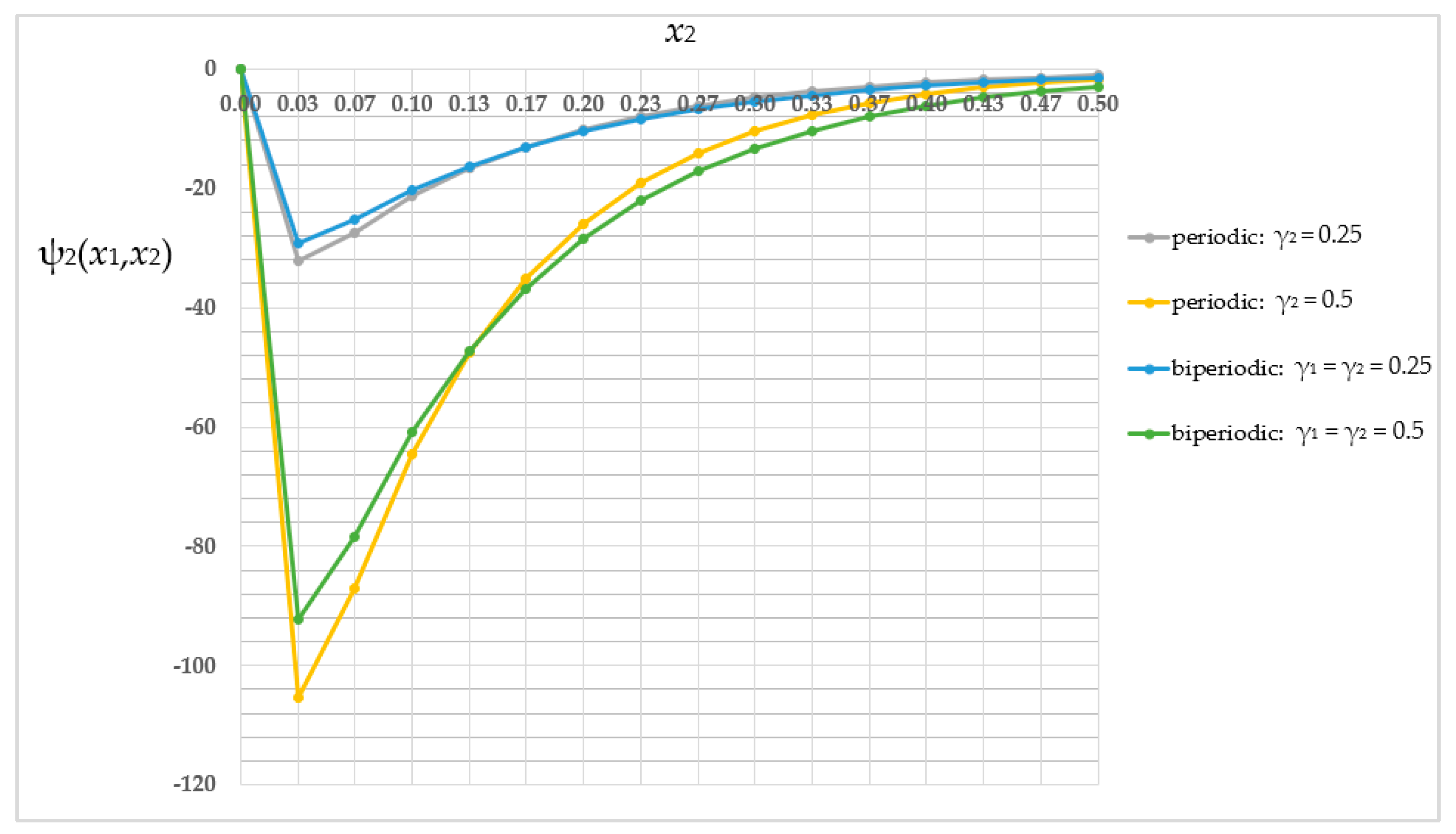
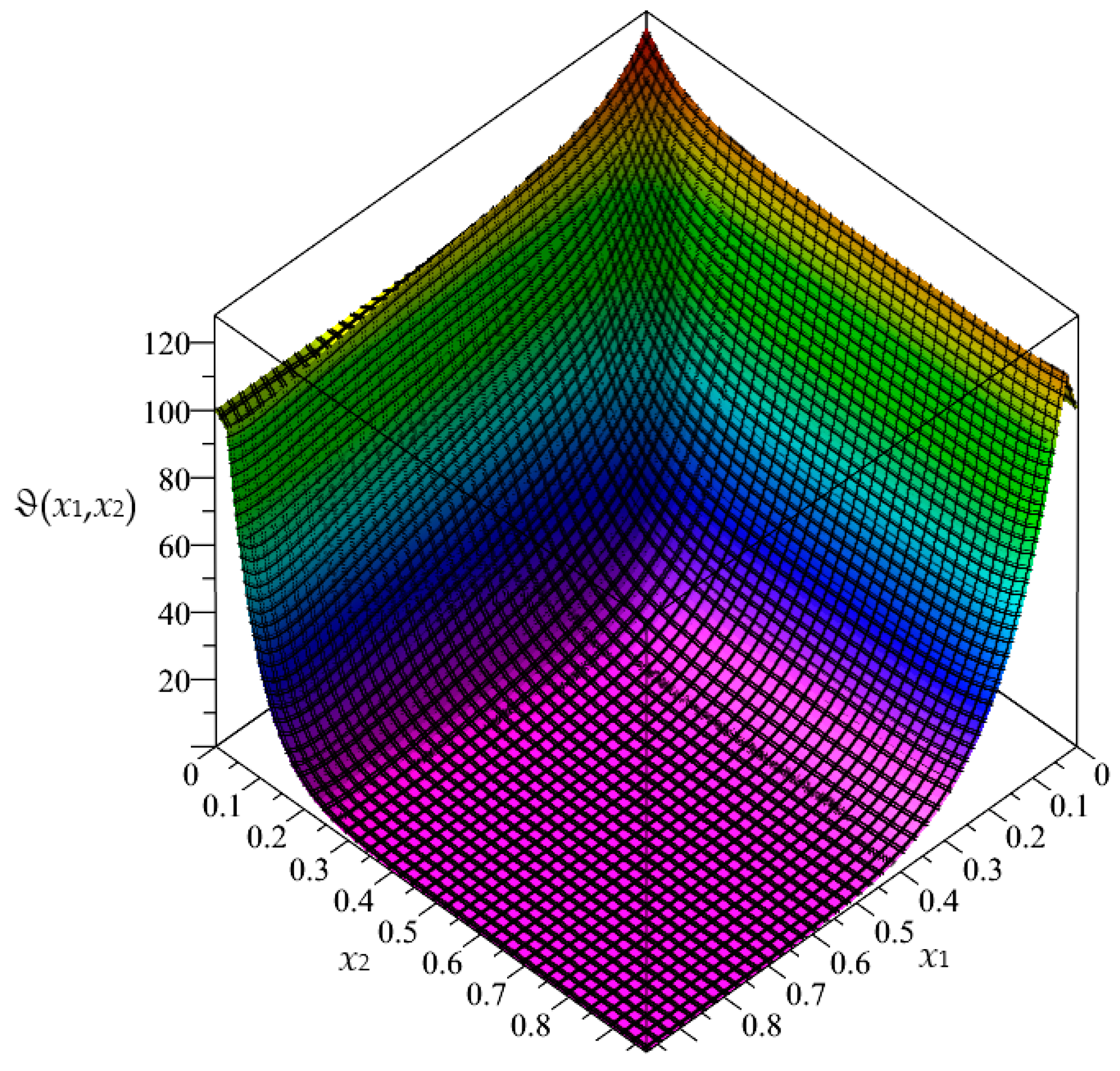


| i | Uniform Structure First Material | Uniform Structure Second Material | Periodic Structure γ2 = 0.25 | Periodic Structure γ2 = 0.5 | Biperiodic Structure γ1 = γ2 = 0.25 | Biperiodic Structure γ1 = γ2 = 0.5 |
|---|---|---|---|---|---|---|
| 5 | 21.76 | 60.25 | 52.18 | 43.42 | 58.12 | 51.14 |
| 6 | 13.49 | 49.26 | 41.04 | 32.48 | 47.05 | 39.93 |
| 7 | 8.36 | 40.35 | 32.33 | 24.33 | 38.16 | 31.24 |
| 8 | 5.19 | 33.15 | 25.53 | 18.25 | 31.03 | 24.49 |
| 9 | 3.22 | 27.31 | 20.21 | 13.71 | 25.31 | 19.25 |
| 10 | 2.00 | 22.59 | 16.06 | 10.33 | 20.73 | 15.19 |
| 11 | 1.25 | 18.77 | 12.82 | 7.81 | 17.05 | 12.04 |
| 12 | 0.78 | 15.68 | 10.28 | 5.93 | 14.10 | 9.59 |
| 13 | 0.49 | 13.18 | 8.31 | 4.53 | 11.73 | 7.70 |
| 14 | 0.31 | 11.16 | 6.77 | 3.49 | 9.84 | 6.23 |
| 15 | 0.20 | 9.52 | 5.56 | 2.71 | 8.32 | 5.09 |
| 16 | 0.13 | 8.21 | 4.62 | 2.13 | 7.11 | 4.21 |
| 17 | 0.09 | 7.14 | 3.89 | 1.70 | 6.14 | 3.52 |
| 18 | 0.06 | 6.28 | 3.32 | 1.38 | 5.36 | 2.99 |
| 19 | 0.04 | 5.58 | 2.88 | 1.14 | 4.74 | 2.58 |
| 20 | 0.03 | 5.02 | 2.53 | 0.97 | 4.24 | 2.27 |
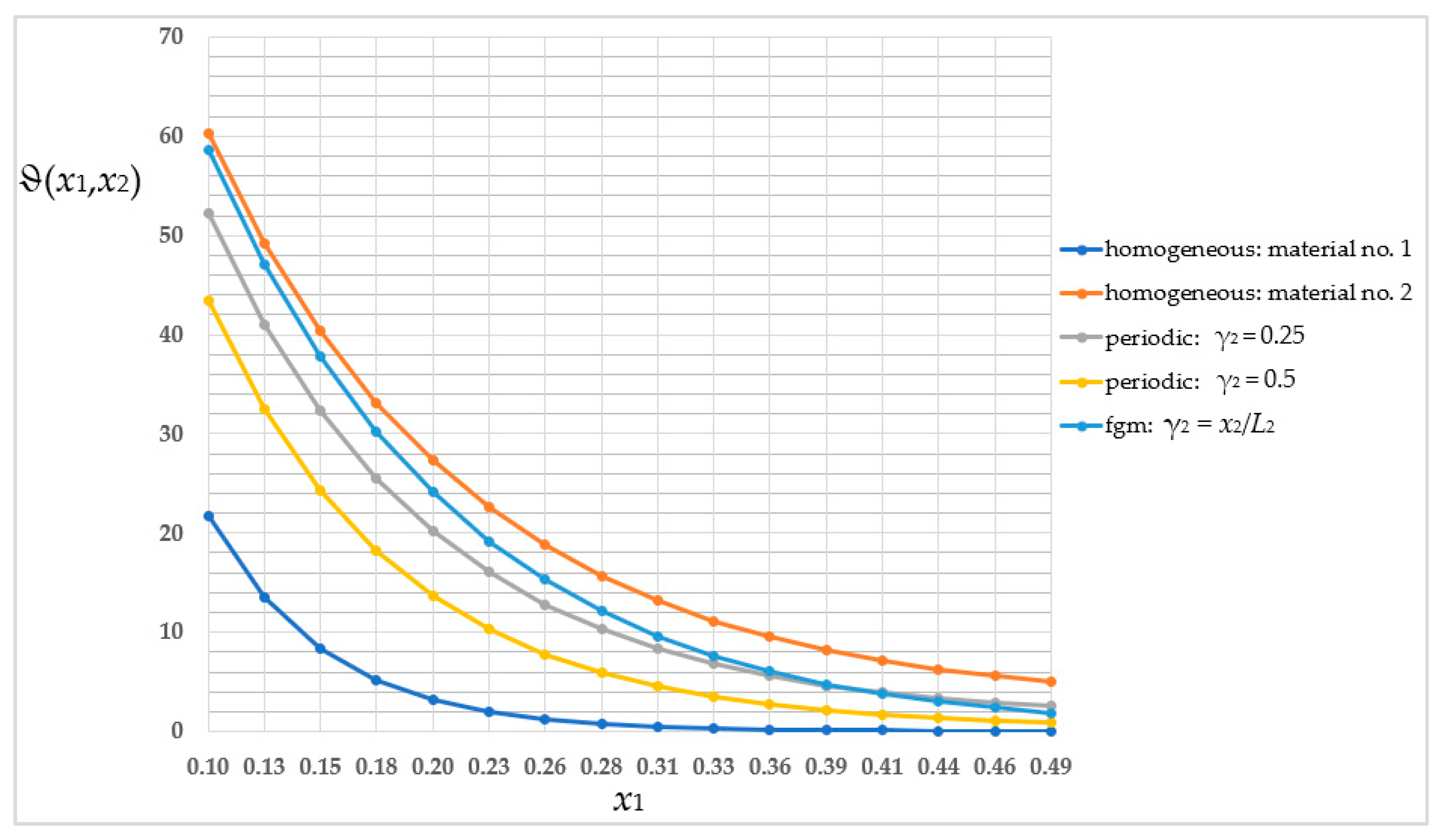
| i | Uniform Structure First Material | Uniform Structure Second Material | Periodic Structure γ2 = 0.25 | Periodic Structure γ2 = 0.5 | FGM Structure γ2 = x2/L2 |
|---|---|---|---|---|---|
| 5 | 21.76 | 60.25 | 52.18 | 43.42 | 58.62 |
| 6 | 13.49 | 49.26 | 41.04 | 32.48 | 47.12 |
| 7 | 8.36 | 40.35 | 32.33 | 24.33 | 37.78 |
| 8 | 5.19 | 33.15 | 25.53 | 18.25 | 30.21 |
| 9 | 3.22 | 27.31 | 20.21 | 13.71 | 24.11 |
| 10 | 2.00 | 22.59 | 16.06 | 10.33 | 19.20 |
| 11 | 1.25 | 18.77 | 12.82 | 7.81 | 15.26 |
| 12 | 0.78 | 15.68 | 10.28 | 5.93 | 12.12 |
| 13 | 0.49 | 13.18 | 8.31 | 4.53 | 9.60 |
| 14 | 0.31 | 11.16 | 6.77 | 3.49 | 7.61 |
| 15 | 0.20 | 9.52 | 5.56 | 2.71 | 6.02 |
| 16 | 0.13 | 8.21 | 4.62 | 2.13 | 4.76 |
| 17 | 0.09 | 7.14 | 3.89 | 1.70 | 3.77 |
| 18 | 0.06 | 6.28 | 3.32 | 1.38 | 2.98 |
| 19 | 0.04 | 5.58 | 2.88 | 1.14 | 2.37 |
| 20 | 0.03 | 5.02 | 2.53 | 0.97 | 1.88 |
| Number of Intervals Δx1 and Δx2 | ϑ(x1,x2) | Δϑ(x1,x2) | ψ1(x1,x2) | Δψ1(x1,x2) | ψ2(x1,x2) | Δψ2(x1,x2) |
|---|---|---|---|---|---|---|
| 40 | 3.838 | - | −0.5271 | - | −0.5308 | - |
| 42 | 3.801 | 0.037 | −0.5217 | 0.0054 | −0.5257 | 0.0051 |
| 44 | 3.770 | 0.031 | −0.5175 | 0.0042 | −0.5216 | 0.0041 |
| 46 | 3.743 | 0.027 | −0.5137 | 0.0038 | −0.5180 | 0.0036 |
| 48 | 3.719 | 0.024 | −0.5102 | 0.0035 | −0.5148 | 0.0032 |
| 50 | 3.697 | 0.022 | −0.5072 | 0.0030 | −0.5119 | 0.0029 |
| 52 | 3.677 | 0.020 | −0.5046 | 0.0026 | −0.5092 | 0.0027 |
| 54 | 3.659 | 0.018 | −0.5021 | 0.0025 | −0.5068 | 0.0024 |
| 56 | 3.642 | 0.017 | −0.4998 | 0.0023 | −0.5046 | 0.0022 |
| 58 | 3.627 | 0.015 | −0.4976 | 0.0022 | −0.5026 | 0.0020 |
| 60 | 3.613 | 0.014 | −0.4955 | 0.0021 | −0.5008 | 0.0018 |
| Number of Intervals Δx1 and Δx2 | ϑ(x1,x2) | Δϑ(x1,x2) | ψ2(x1,x2) | Δψ2(x1,x2) |
|---|---|---|---|---|
| 40 | 2.261 | - | −0.3533 | - |
| 42 | 2.237 | 0.024 | −0.3496 | 0.0037 |
| 44 | 2.216 | 0.021 | −0.3463 | 0.0033 |
| 46 | 2.197 | 0.019 | −0.3434 | 0.0029 |
| 48 | 2.180 | 0.017 | −0.3408 | 0.0026 |
| 50 | 2.165 | 0.015 | −0.3385 | 0.0023 |
| 52 | 2.151 | 0.014 | −0.3364 | 0.0021 |
| 54 | 2.139 | 0.012 | −0.3345 | 0.0019 |
| 56 | 2.128 | 0.011 | −0.3328 | 0.0017 |
| 58 | 2.118 | 0.010 | −0.3312 | 0.0016 |
| 60 | 2.109 | 0.009 | −0.3297 | 0.0015 |
| Number of Intervals Δx1 and Δx2 | ϑ(x1,x2) | Δϑ(x1,x2) | ψ2(x1,x2) | Δψ2(x1,x2) |
|---|---|---|---|---|
| 40 | 2.157 | - | −0.8139 | - |
| 42 | 2.134 | 0.023 | −0.8059 | 0.0080 |
| 44 | 2.113 | 0.021 | −0.7988 | 0.0071 |
| 46 | 2.095 | 0.018 | −0.7925 | 0.0063 |
| 48 | 2.079 | 0.016 | −0.7869 | 0.0056 |
| 50 | 2.064 | 0.015 | −0.7818 | 0.0051 |
| 52 | 2.051 | 0.013 | −0.7772 | 0.0046 |
| 54 | 2.039 | 0.012 | −0.7731 | 0.0041 |
| 56 | 2.028 | 0.011 | −0.7693 | 0.0038 |
| 58 | 2.018 | 0.010 | −0.7659 | 0.0034 |
| 60 | 2.009 | 0.009 | −0.7627 | 0.0032 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kubacka, E.; Ostrowski, P. Influence of Composite Structure on Temperature Distribution—An Analysis Using the Finite Difference Method. Materials 2023, 16, 5193. https://doi.org/10.3390/ma16145193
Kubacka E, Ostrowski P. Influence of Composite Structure on Temperature Distribution—An Analysis Using the Finite Difference Method. Materials. 2023; 16(14):5193. https://doi.org/10.3390/ma16145193
Chicago/Turabian StyleKubacka, Ewelina, and Piotr Ostrowski. 2023. "Influence of Composite Structure on Temperature Distribution—An Analysis Using the Finite Difference Method" Materials 16, no. 14: 5193. https://doi.org/10.3390/ma16145193





