Verification of Composite Beam Theory with Finite Element Model for Pretensioned Concrete Members with Prestressing FRP Tendons
Abstract
:1. Introduction
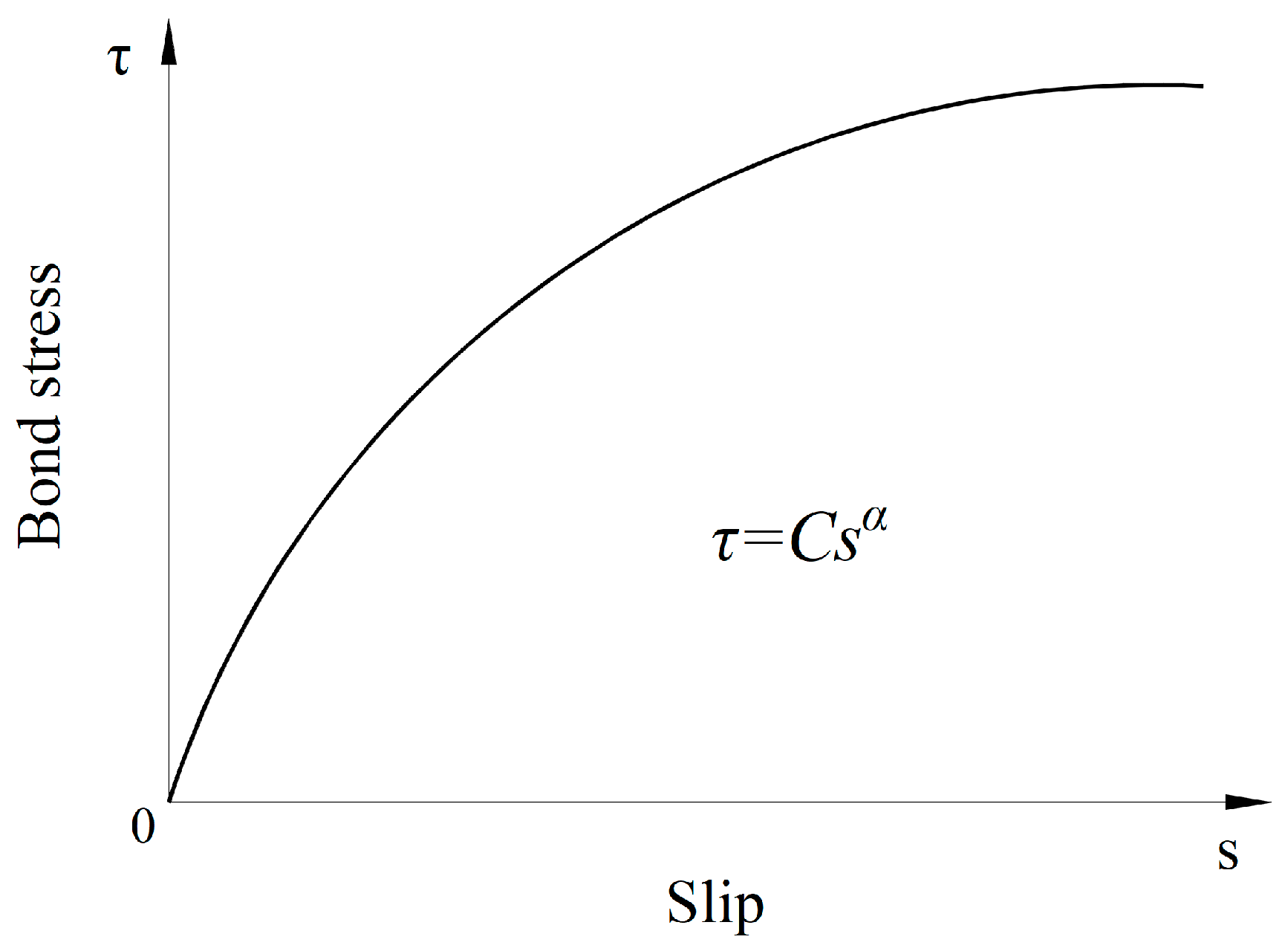
2. Background of the Bond Mechanism
3. Analytical Solution for FRP-Strengthening Concrete Members
3.1. General Approach of Composite Beam Theory
- (1)
- Linear elastic constitutive behavior and small displacement are applied to each component in the developed analytical models;
- (2)
- FRP’s bending resistance is negligible compared to that provided by the concrete member;
- (3)
- Slender beams are considered and therefore Euler–Bernoulli beam theory can be applied to each component of a composite structure, and, therefore, the shear deformation is neglected through the cross section of the concrete beam and FRP components, respectively.
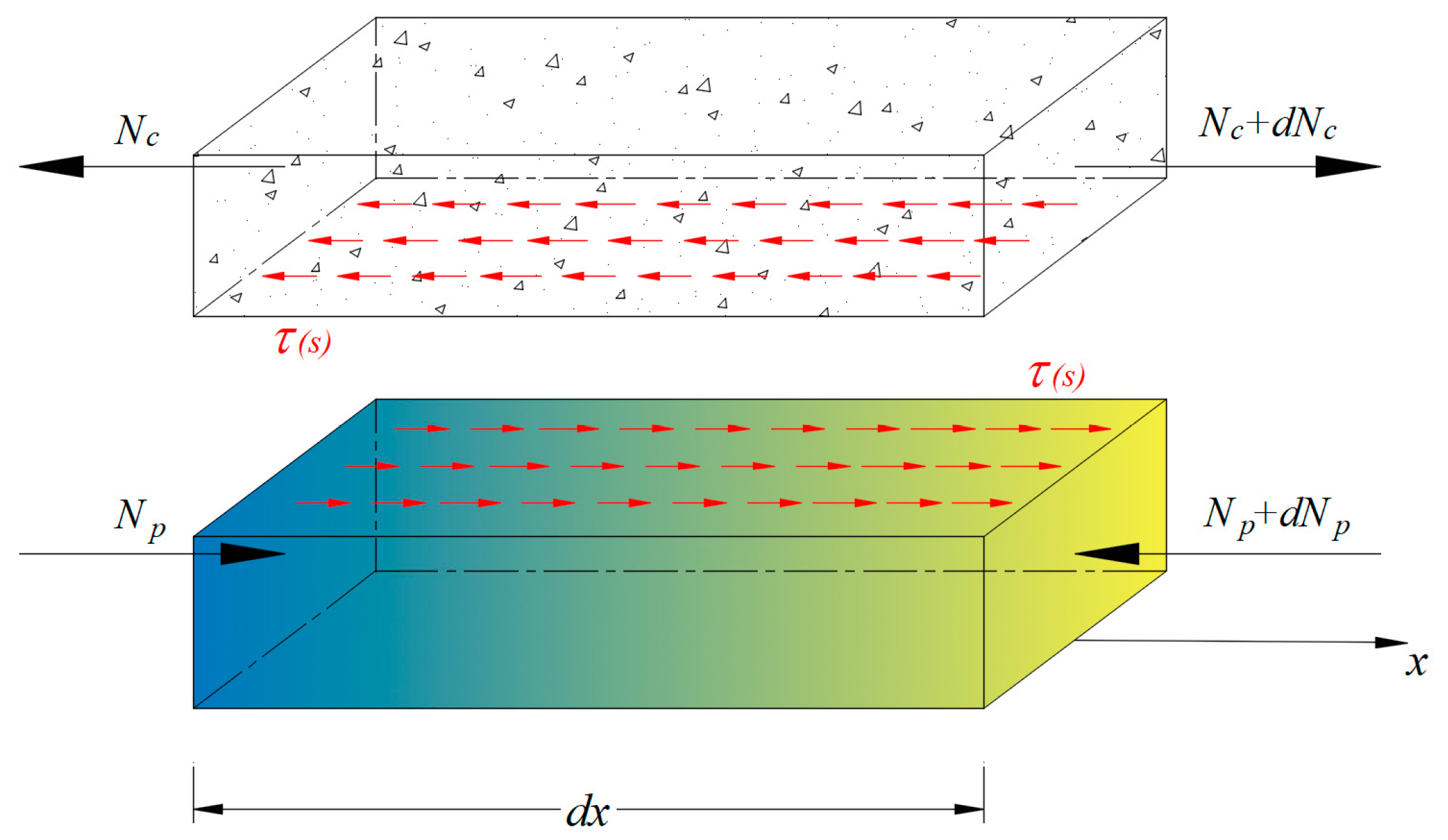
3.1.1. Axial Force Equilibrium
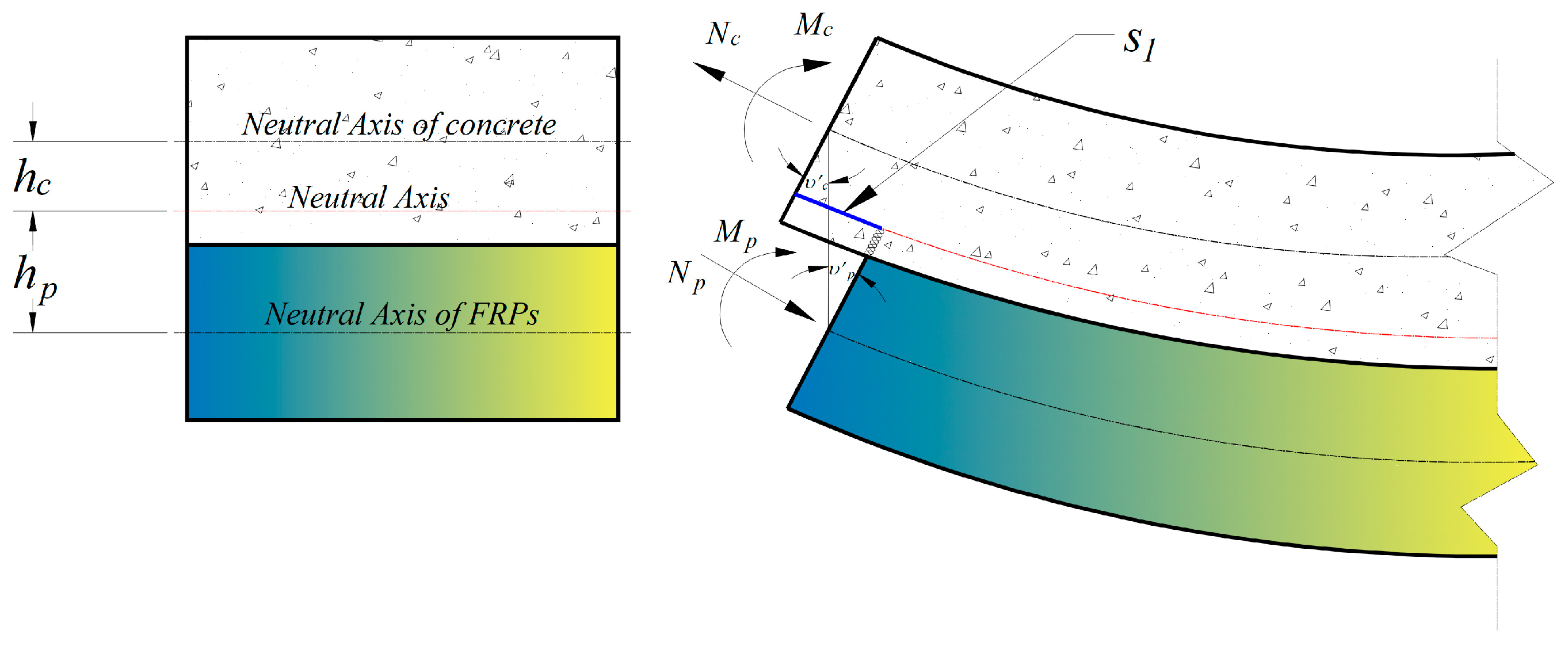
3.1.2. Bending Moment Equilibrium
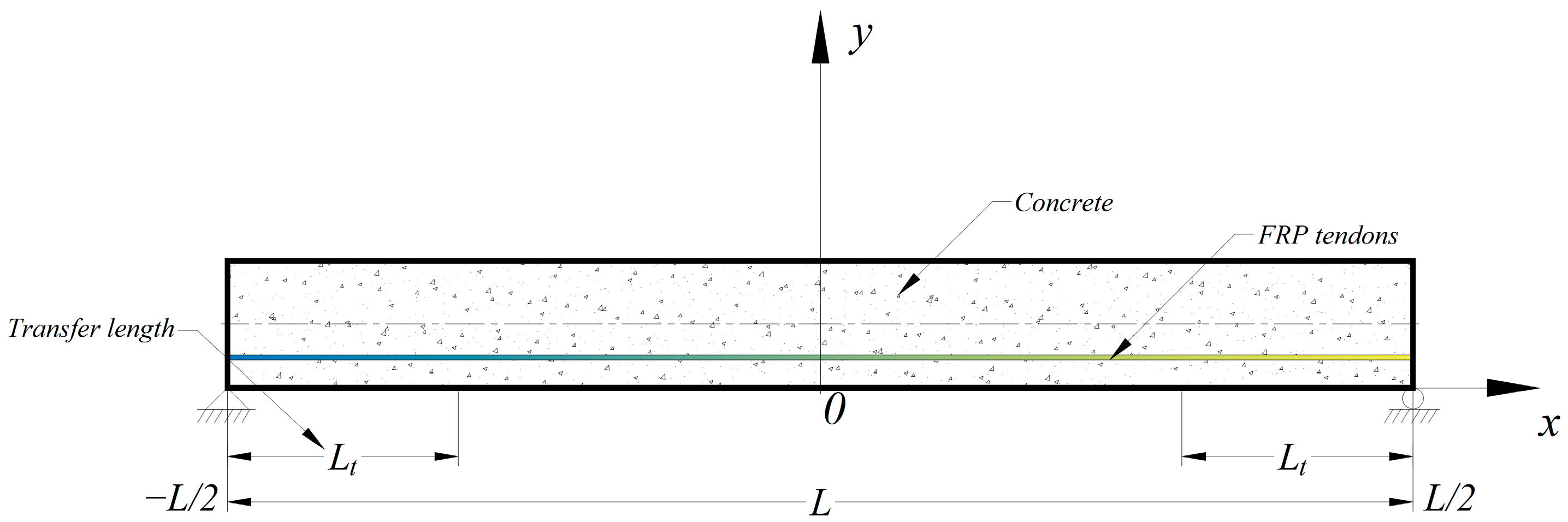
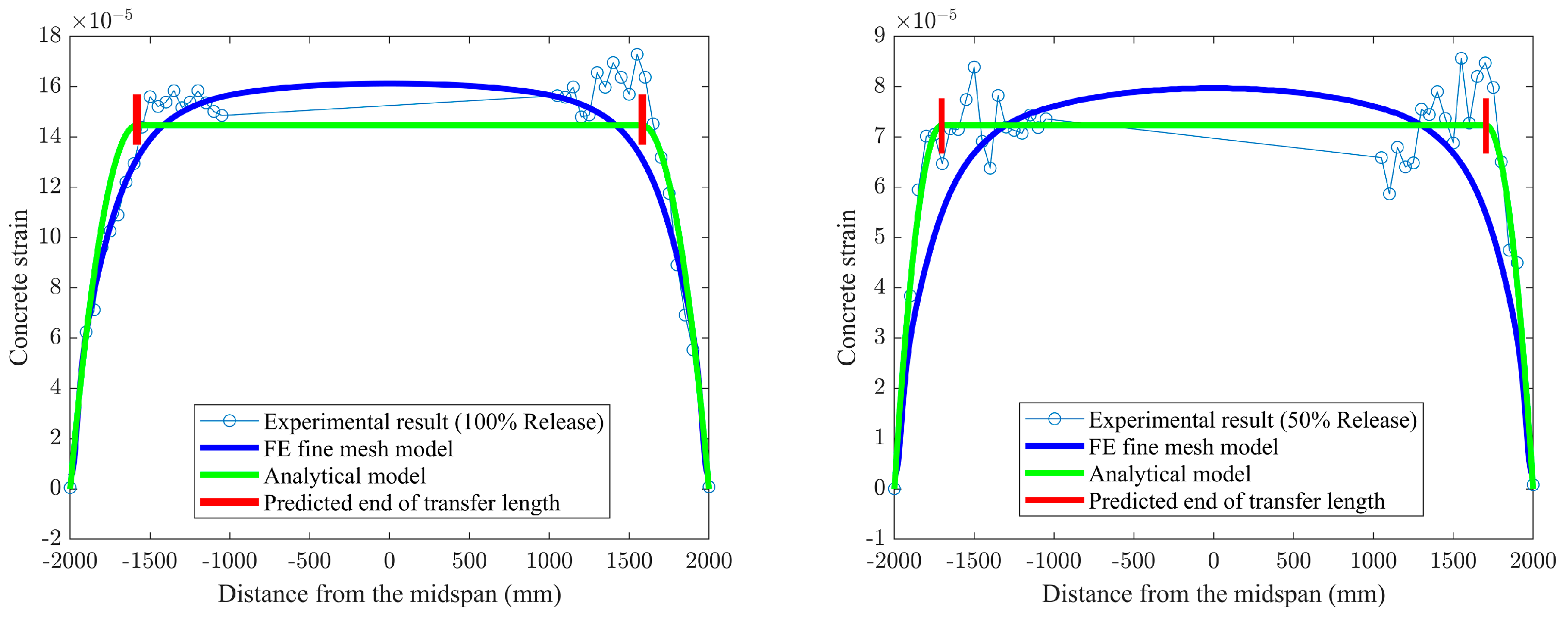
3.2. Predictions of Transfer Length for Prestressed FRP Tendons Application
4. Numerical Implementation with Finite Element Modeling
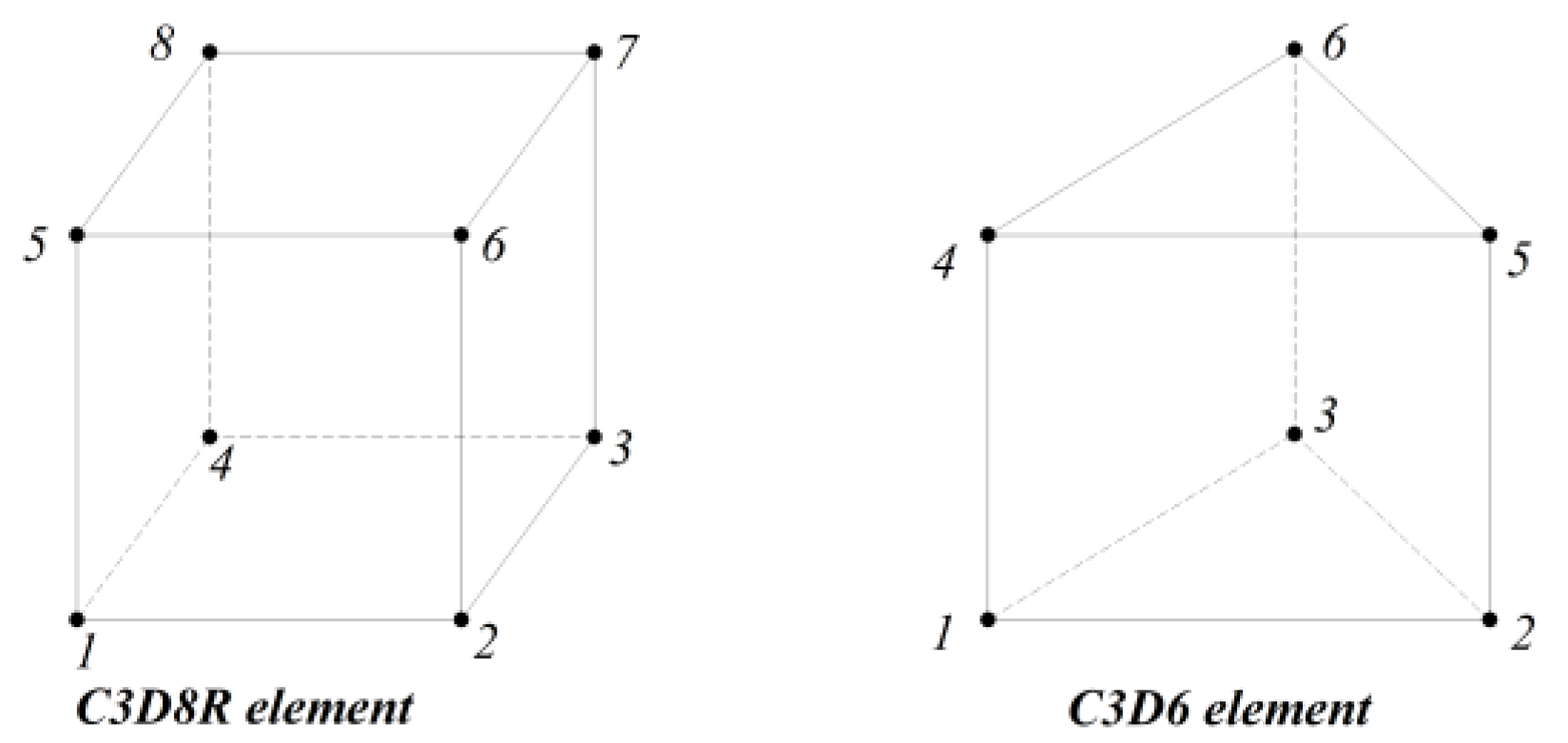
4.1. Finite Element Modeling of Pretensioned RC Beams with FRP Tendons
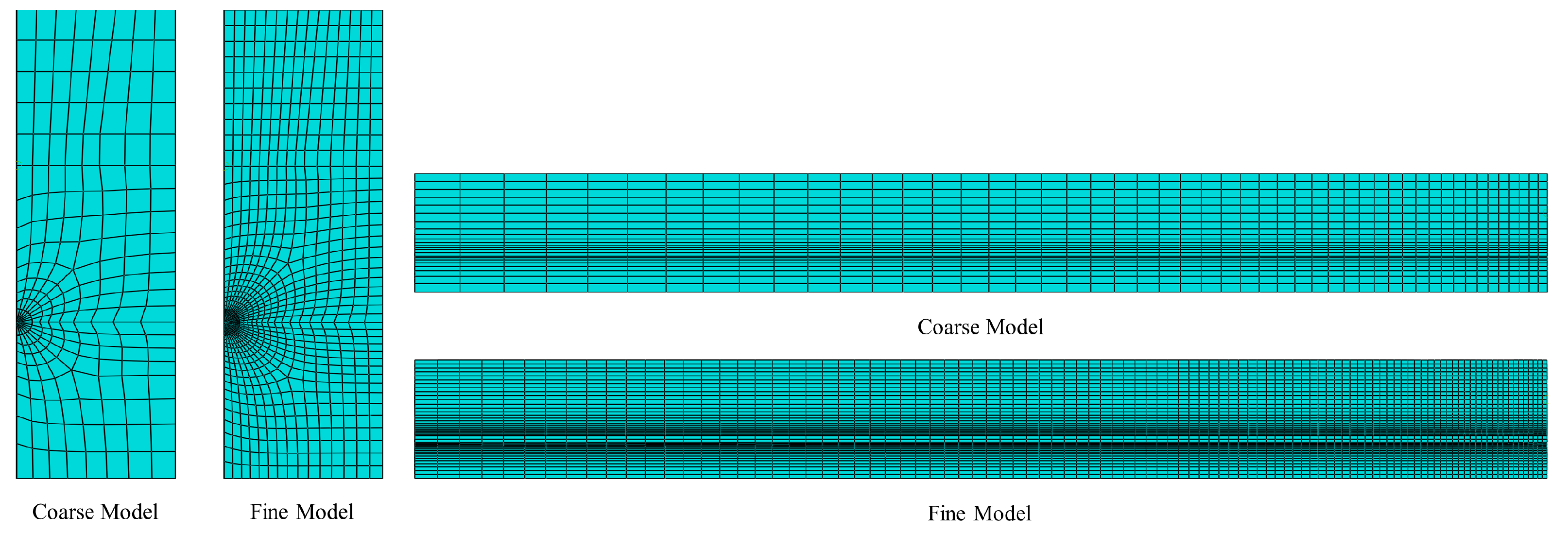
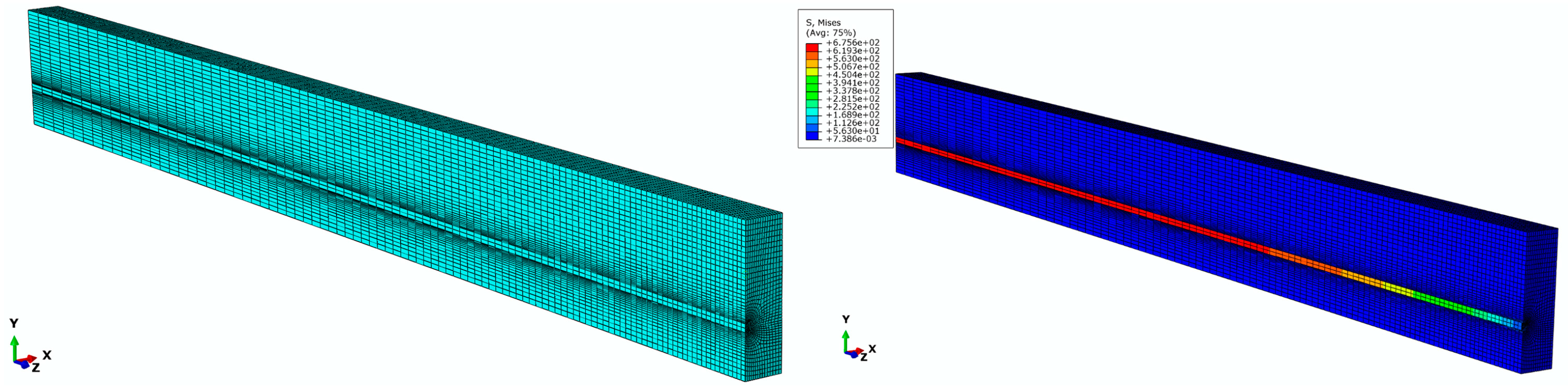
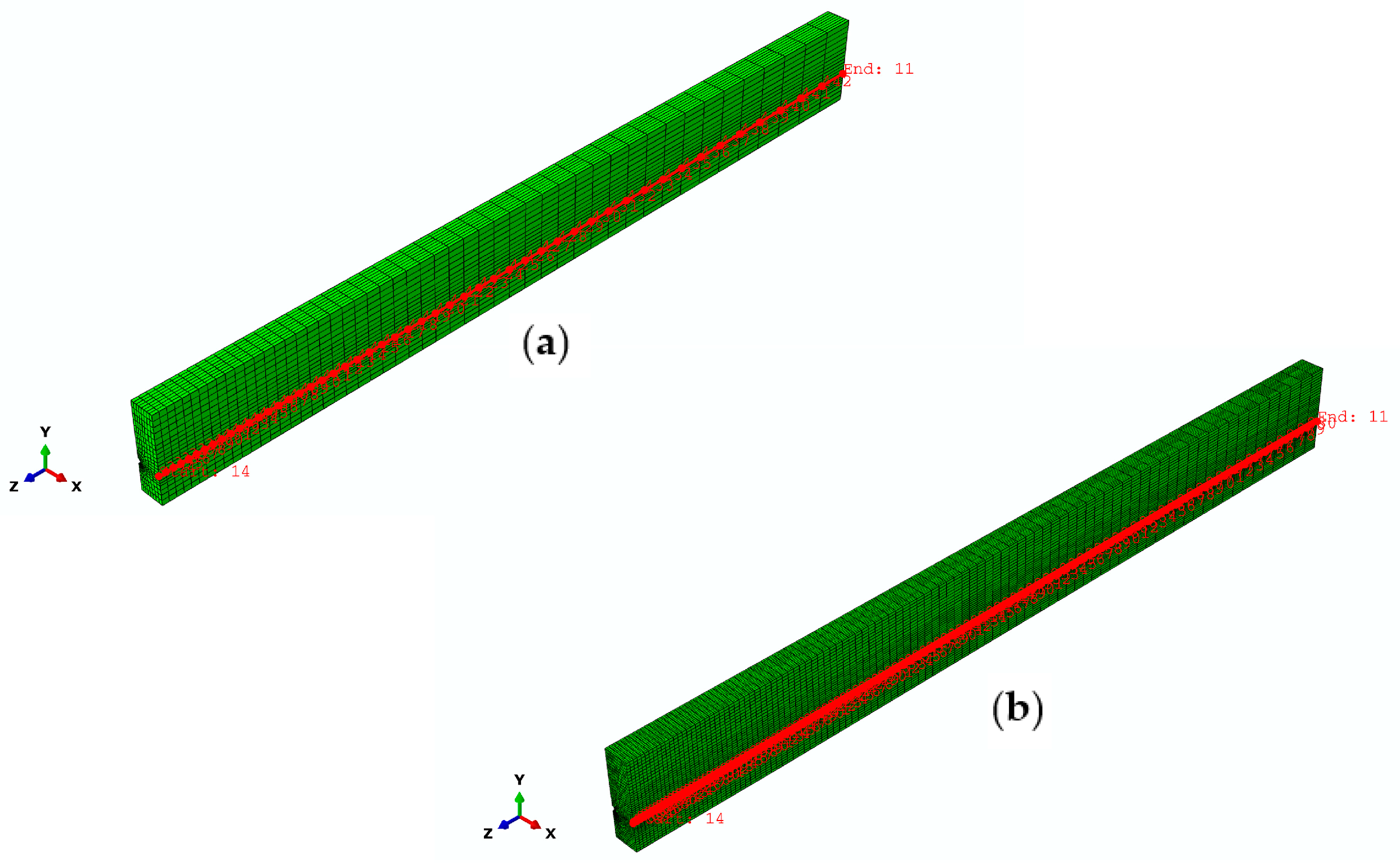
4.2. Convergence Analysis and Verification of FE Model
5. Comparison and Discussion
6. Conclusions
- A general form of the governing equations has been presented specifically for FRP-strengthening concrete members in terms of composite beam theory. Associating with the knowledge of the local bond stress–slip relationship between FRP and concrete, the closed-form solution can be solved under corresponding boundary conditions;
- Comparisons with the experimental data demonstrate good agreement, which indicates that the proposed FE model with fine mesh is acceptable. The measured transfer length for high pretension agrees with the prediction from the fine FE model with the friction coefficient within a 10% range. The consistency between the FE model results and the previously developed analytical solutions demonstrate that theoretical results using composite beam theory are superior to that of the numerical simulation;
- Although friction plays a key role in the interaction between concrete and prestressed tendons, the slip-prediction comparisons show that if the adhesion and mechanical interlocking are ignored, the bond behavior cannot be accurately evaluated;
- The transfer-length prediction is strongly dependent on the adopted function form of the bond–slip relationship between the concrete and FRP tendons in the analytical model using composite beam theory. For the analytical solution of the mechanical behavior of concrete members strengthened with FRP in terms of partially composite action, the most critical issue is to have knowledge of the local bond–slip relationship in the interface region.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wu, J.; Zhu, Y.; Li, C. Experimental Investigation of Fatigue Capacity of Bending-Anchored CFRP Cables. Polymers 2023, 15, 2483. [Google Scholar] [CrossRef]
- Lu, Z.; Li, W.; Zeng, X.; Pan, Y. Durability of BFRP bars and BFRP reinforced seawater sea-sand concrete beams immersed in water and simulated seawater. Constr. Build. Mater. 2023, 363, 129845. [Google Scholar] [CrossRef]
- Xian, G.; Guo, R.; Li, C.; Hong, B. Mechanical properties of carbon/glass fiber reinforced polymer plates with sandwich structure exposed to freezing-thawing environment: Effects of water immersion, bending loading and fiber hybrid mode. Mech. Adv. Mater. Struct. 2023, 30, 814–834. [Google Scholar] [CrossRef]
- Eligehausen, R.; Popov, E.P.; Bertero, V.V. Local Bond Stress-Slip Relationships of Deformed Bars under Generalized Excitations; Report No. UCB/EERC 83-23; University of California: Berkeley, CA, USA, 1983. [Google Scholar]
- Nanni, A.; Tanigaki, M.; Hasuo, K. Bond anchorage of pretensioned FRP tendon at force release. J. Struct. Eng. 1992, 118, 2837–2854. [Google Scholar] [CrossRef]
- Lees, J.M.; Burgoyne, C.J. Transfer bond stresses generated between FRP tendons and concrete. Mag. Concr. Res. 1999, 51, 229–239. [Google Scholar] [CrossRef]
- Focacci, F.; Nanni, A.; Bakis, C.E. Local bond-slip relationship for FRP reinforcement in concrete. J. Compos. Constr. 2000, 4, 24–31. [Google Scholar] [CrossRef]
- Den Uijl, J.A. Bond modelling of prestressing strand. Spec. Publ. 1998, 180, 145–170. [Google Scholar]
- Zhang, W.; Liu, X.; Huang, Y.; Tong, M.-N. Reliability-based analysis of the flexural strength of concrete beams reinforced with hybrid BFRP and steel rebars. Arch. Civ. Mech. Eng. 2022, 22, 171. [Google Scholar] [CrossRef]
- Yiqun, H.; Zhang, W.; Liu, X. Assessment of Diagonal Macrocrack-Induced Debonding Mechanisms in FRP-Strengthened RC Beams. J. Compos. Constr. 2022, 26, 04022056. [Google Scholar] [CrossRef]
- Huang, H.; Huang, M.; Zhang, W.; Guo, M.; Liu, B. Progressive collapse of multistory 3D reinforced concrete frame structures after the loss of an edge column. Struct. Infrastruct. Eng. 2022, 18, 249–265. [Google Scholar] [CrossRef]
- Lee, Y.J.; Boothby, T.E.; Bakis, C.E.; Nanni, A. Slip modulus of FRP sheets bonded to concrete. J. Compos. Constr. 1999, 3, 161–167. [Google Scholar] [CrossRef]
- De Lorenzis, L.; Miller, B.; Nanni, A. Bond of FRP laminates to concrete. ACI Mater. J. 2001, 98, 256–264. [Google Scholar]
- Nakaba, K.; Kanakubo, T.; Furuta, T.; Yoshizawa, H. Bond behavior between fiber-reinforced polymer laminates and concrete. Struct. J. 2001, 98, 359–367. [Google Scholar]
- Dai, J.; Ueda, T.; Sato, Y. Development of the nonlinear bond stress–slip model of fiber reinforced plastics sheet–concrete interfaces with a simple method. J. Compos. Constr. 2005, 9, 52–62. [Google Scholar] [CrossRef]
- Lu, X.Z.; Teng, J.G.; Ye, L.P.; Jiang, J.J. Bond–slip models for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 920–937. [Google Scholar] [CrossRef]
- Lu, X.Z.; Ye, L.P.; Teng, J.G.; Jiang, J.J. Meso-scale finite element model for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 564–575. [Google Scholar] [CrossRef]
- Guo, Z.G.; Cao, S.Y.; Sun, W.M.; Lin, X.Y. Experimental study on bond stress-slip behaviour between FRP sheets and concrete. In Proceedings of the International Symposium on Bond Behaviour of FRP in Structures, Hong Kong, China, 7–9 December 2005; FRP in Construction; pp. 77–84. [Google Scholar]
- Zhou, Y.W.; Wu, Y.F.; Yun, Y. Analytical modeling of the bond-slip relationship at FRP-concrete interfaces for adhesively-bonded joints. Compos. Part B Eng. 2010, 41, 423–433. [Google Scholar] [CrossRef]
- Biscaia, H.C.; Chastre, C.; Silva, M.A. Linear and nonlinear analysis of bond-slip models for interfaces between FRP composites and concrete. Compos. Part B Eng. 2013, 45, 1554–1568. [Google Scholar] [CrossRef]
- Ko, H.; Matthys, S.; Palmieri, A.; Sato, Y. Development of a simplified bond stress-slip model for bonded FRP-concrete interfaces. Constr. Build. Mater. 2014, 68, 142–157. [Google Scholar] [CrossRef]
- Zhang, L.; Teng, J.G. Finite element prediction of interfacial stresses in structural members bonded with a thin plate. Eng. Struct. 2010, 32, 459–471. [Google Scholar] [CrossRef]
- Sha, X.; Davidson, J.S. Analysis of transfer length for prestressed FRP tendons in pretensioned concrete using composite beam theory. Compos. Struct. 2019, 208, 665–677. [Google Scholar] [CrossRef]
- Granholm, H. On Composite Beams and Columns with Particular Regard to Nailed Timber Structures; Chalmers University of Technology: Gothenburg, Sweden, 1949. [Google Scholar]
- Newmark, N.M.; Siess, C.P.; Viest, I.M. Test and analysis of composite beams with incomplete interaction. Proc. Soc. Exp. Stress Anal. 1951, 9, 75–92. [Google Scholar]
- Bai, F.; Davidson, J.S. Analysis of partially composite foam insulated concrete sandwich structures. Eng. Struct. 2015, 91, 197–209. [Google Scholar] [CrossRef]
- Sha, X.; Davidson, J.S. Analysis of Interfacial Stresses in Concrete Beams Strengthened by Externally Bonded FRP Laminates Using Composite Beam Theory. Compos. Struct. 2020, 19, 112235. [Google Scholar] [CrossRef]
- Arab, A.A.; Badie, S.S.; Manzari, M.T. A methodological approach for finite element modeling of pretensioned concrete members at the release of pretensioning. Eng. Struct. 2011, 33, 1918–1929. [Google Scholar] [CrossRef]
- Abdelatif, A.O.; Owen, J.S.; Hussein, M.F. Modelling the prestress transfer in pre-tensioned concrete elements. Finite Elem. Anal. Des. 2015, 94, 47–63. [Google Scholar] [CrossRef]
- Yapar, O.; Basu, P.K.; Nordendale, N. Accurate finite element modeling of pretensioned prestressed concrete beams. Eng. Struct. 2015, 101, 163–178. [Google Scholar] [CrossRef]
- Khin, M.; Tanaka, M.; Venkataramana, K.; Harada, T. Experimental Study on Friction Factor for Fiber Reinforced Polymer Tendons in Pretensioned Prestressed Concrete Members. Spec. Publ. 1999, 188, 856–864. [Google Scholar]
- Hibbit, K. ABAQUS/Standard User’s Manual, Version; Sorensen Inc.: Syracuse, UT, USA, 2002; Volume 5. [Google Scholar]
- Rabbat, B.G.; Kaar, P.H.; Russell, H.G.; Bruce, R.N., Jr. Fatigue tests of prestressed girders with blanketed and draped strands. Transp. Res. Rec. 1978, 665, 13–21. [Google Scholar]
- Janney, J.R. Nature of bond in pre-tensioned prestressed concrete. J. Proc. 1954, 50, 717–736. [Google Scholar]
- du Béton, F.I. Bond of reinforcement in concrete: State-of-art report. Bulletin 2000, 10, 160–167. [Google Scholar]
- AASHTO. AASHTO LRFD Bridge Design Specifications and Commentary; AASHTO: Washington, DC, USA, 2012. [Google Scholar]
- CEB-FIP M. 90; Design of Concrete Structures. CEB-FIP-Model-Code 1990. British Standards Institute: London, UK, 1993.
- Russo, G.; Zingone, G.; Romano, F. Analytical solution for bond-slip of reinforcing bars in RC joints. J. Struct. Eng. 1990, 116, 336–355. [Google Scholar] [CrossRef]
- Malvar, L.J. Bond Stress-Slip Characteristics of FRP Rebars; Naval Facilities Engineering Service Center: Port Hueneme, CA, USA, 1994. [Google Scholar]
- Soroushian, P.; Choi, K.B.; Park, G.H.; Aslani, F. Bond of deformed bars to concrete: Effects of confinement and strength of concrete. Mater. J. 1991, 88, 227–232. [Google Scholar]
- Nanni, A.; Utsunomiya, T.; Yonekura, H.; Tanigaki, M. Transmission of prestressing force to concrete by bonded fiber reinforced plastic tendons. ACI Struct. J. 1992, 89, 335–344. [Google Scholar]
- Nie, J.; Cai, C.S. Steel–concrete composite beams considering shear slip effects. J. Struct. Eng. 2003, 129, 495–506. [Google Scholar] [CrossRef]




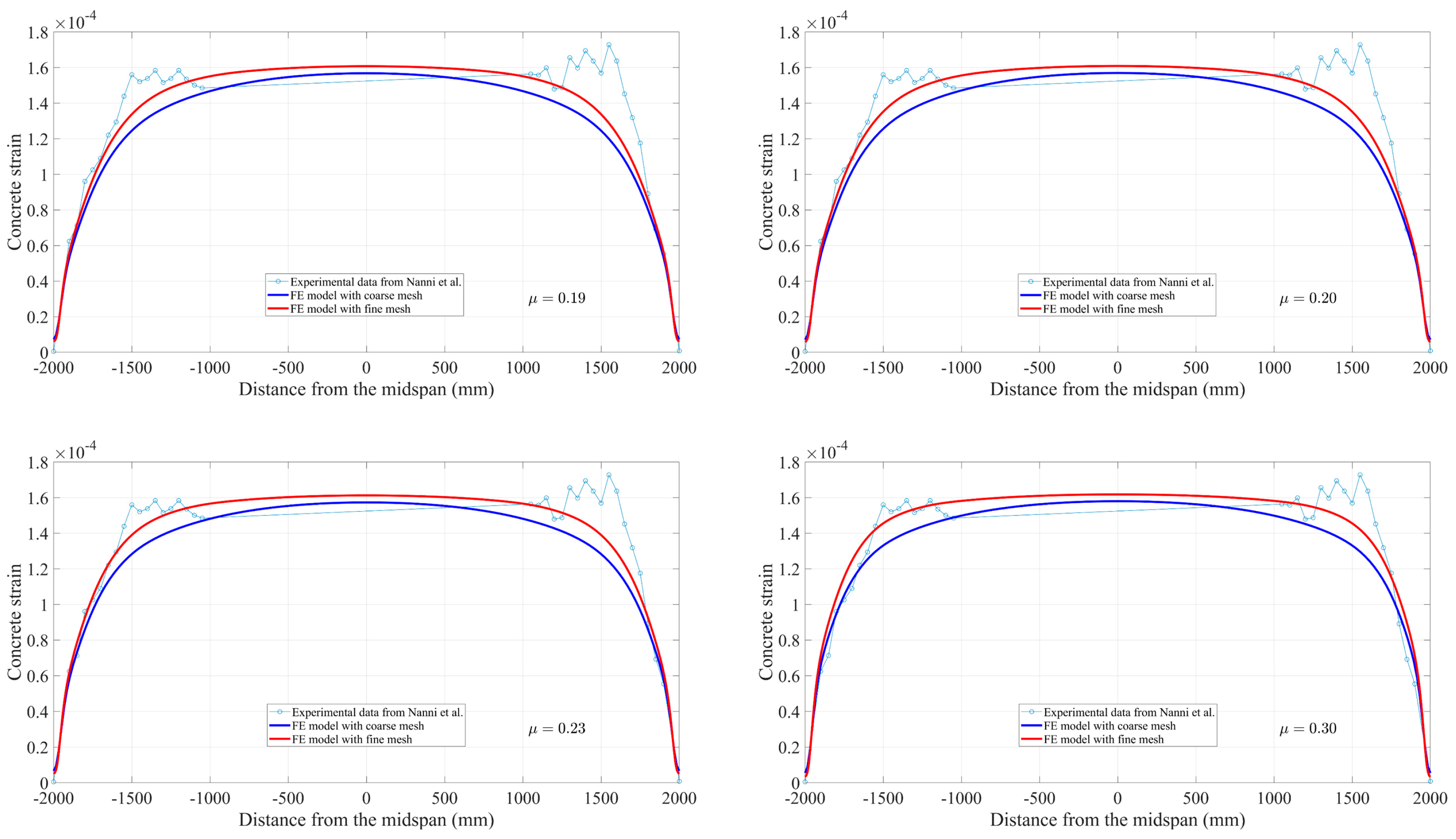

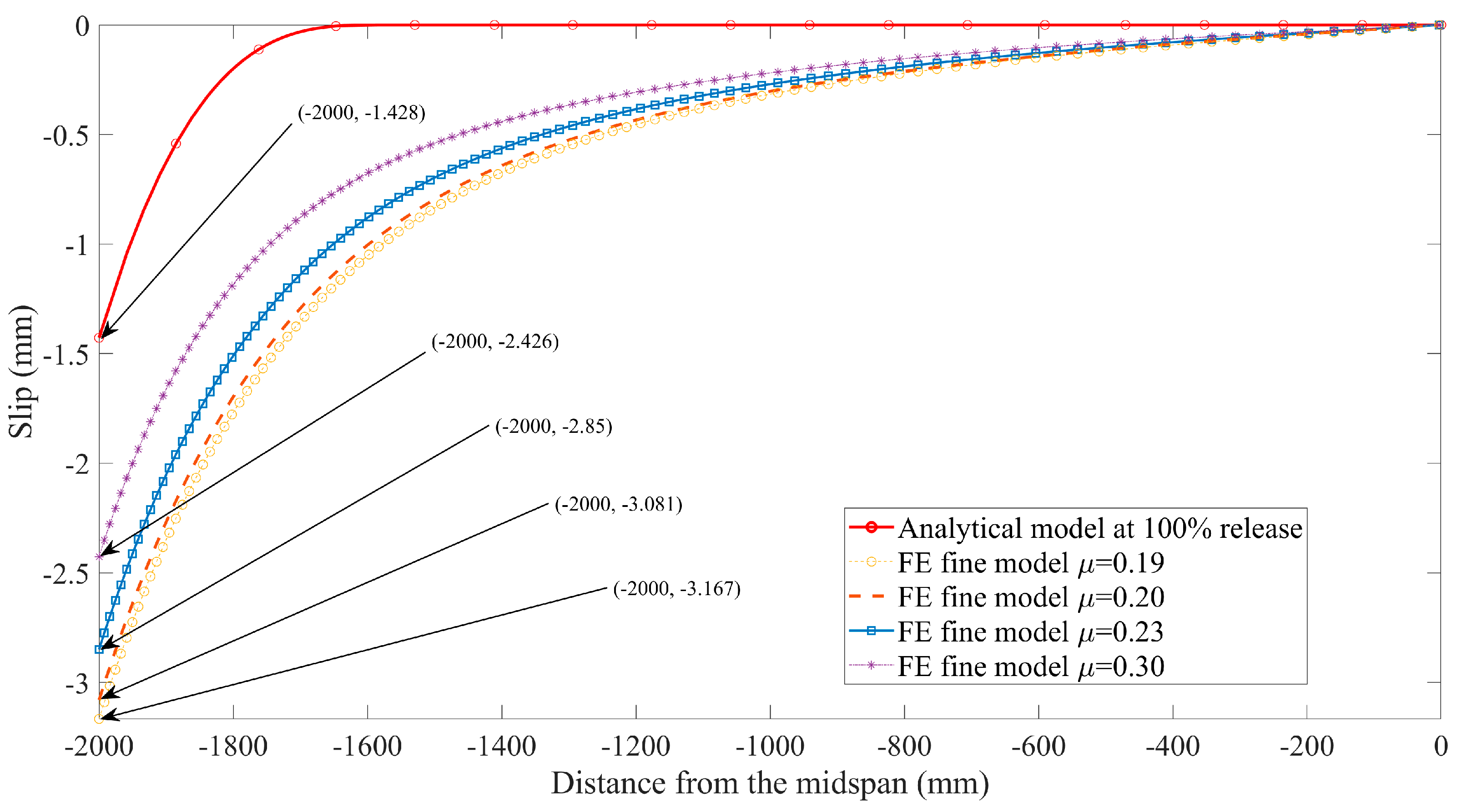
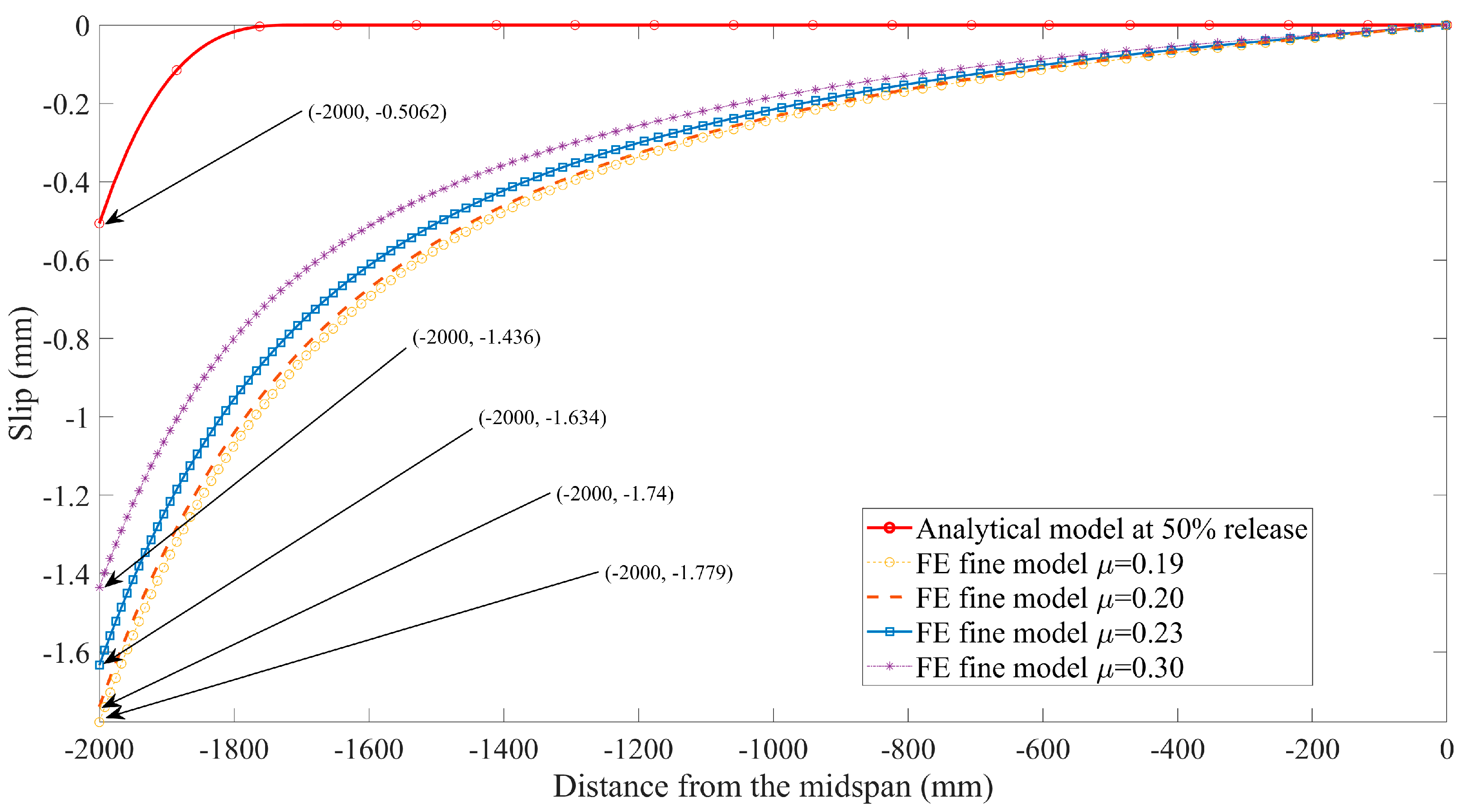

| FRP Tendons | Concrete | Friction Coefficient |
|---|---|---|
| Vinylon FRP rods | HEM | 0.23 |
| Carbon FRP strands | HEM | 0.30 |
| Vinylon FRP rods | HEM and mortar | 0.20 |
| Carbon FRP strands | HEM and mortar | 0.19 |
| Part | Modulus of Elasticity (MPa) | Poisson’s Ratio | Density (tonne/mm3) |
|---|---|---|---|
| Concrete | 25,070 | 0.20 | 2.4 × 10−9 |
| AFRP tendons | 67,600 | 0.35 | 1.4 × 10−9 |
| Beam Group | Measured (mm) [41] | Predicted by FE Model (mm) | ||||
|---|---|---|---|---|---|---|
| High pretension (100% force release) | 450 | Fine | 670 | 651 | 597 | 496 |
| Coarse | 939 | 939 | 893 | 806 | ||
| Low pretension (50% force release) | 400 | Fine | 967 | 943 | 897 | 831 |
| Coarse | 1192 | 1192 | 1192 | 1138 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sha, X.; Davidson, J.S. Verification of Composite Beam Theory with Finite Element Model for Pretensioned Concrete Members with Prestressing FRP Tendons. Materials 2023, 16, 6376. https://doi.org/10.3390/ma16196376
Sha X, Davidson JS. Verification of Composite Beam Theory with Finite Element Model for Pretensioned Concrete Members with Prestressing FRP Tendons. Materials. 2023; 16(19):6376. https://doi.org/10.3390/ma16196376
Chicago/Turabian StyleSha, Xin, and James S. Davidson. 2023. "Verification of Composite Beam Theory with Finite Element Model for Pretensioned Concrete Members with Prestressing FRP Tendons" Materials 16, no. 19: 6376. https://doi.org/10.3390/ma16196376
APA StyleSha, X., & Davidson, J. S. (2023). Verification of Composite Beam Theory with Finite Element Model for Pretensioned Concrete Members with Prestressing FRP Tendons. Materials, 16(19), 6376. https://doi.org/10.3390/ma16196376







