Comparison of Grain-Growth Mean-Field Models Regarding Predicted Grain Size Distributions
Abstract
:1. Introduction
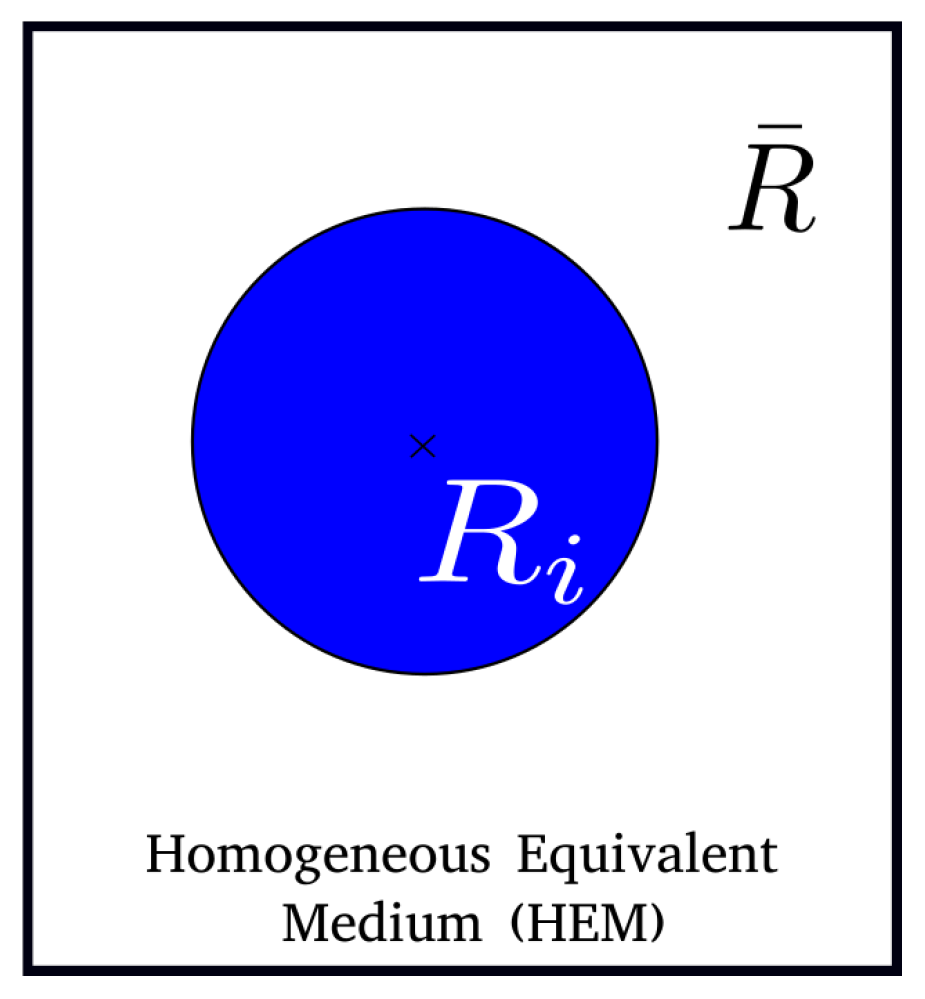
2. Mean-Field Models
2.1. Burke and Turnbull Model
2.2. Hillert Model
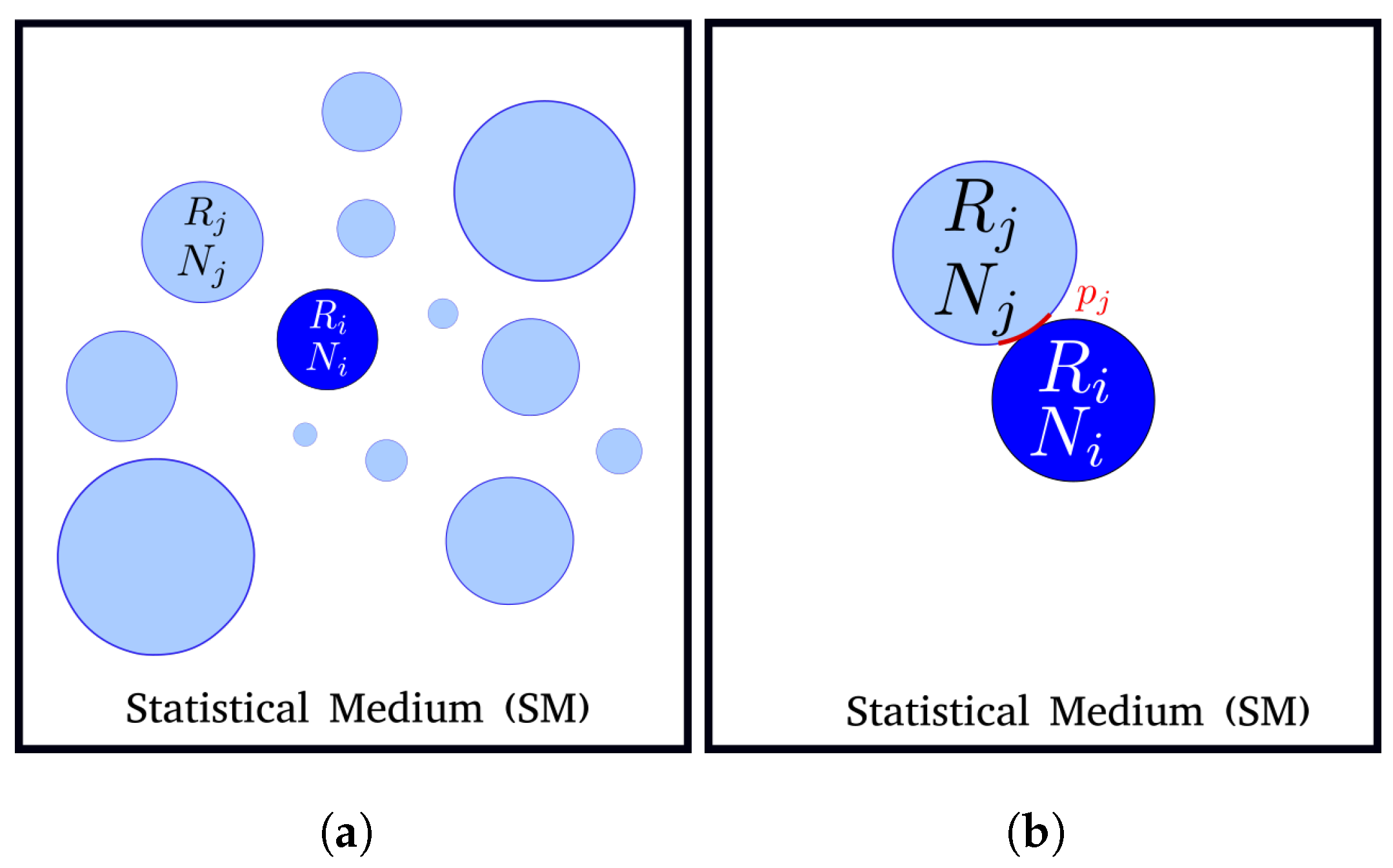
2.3. Abbruzzese et al. Model
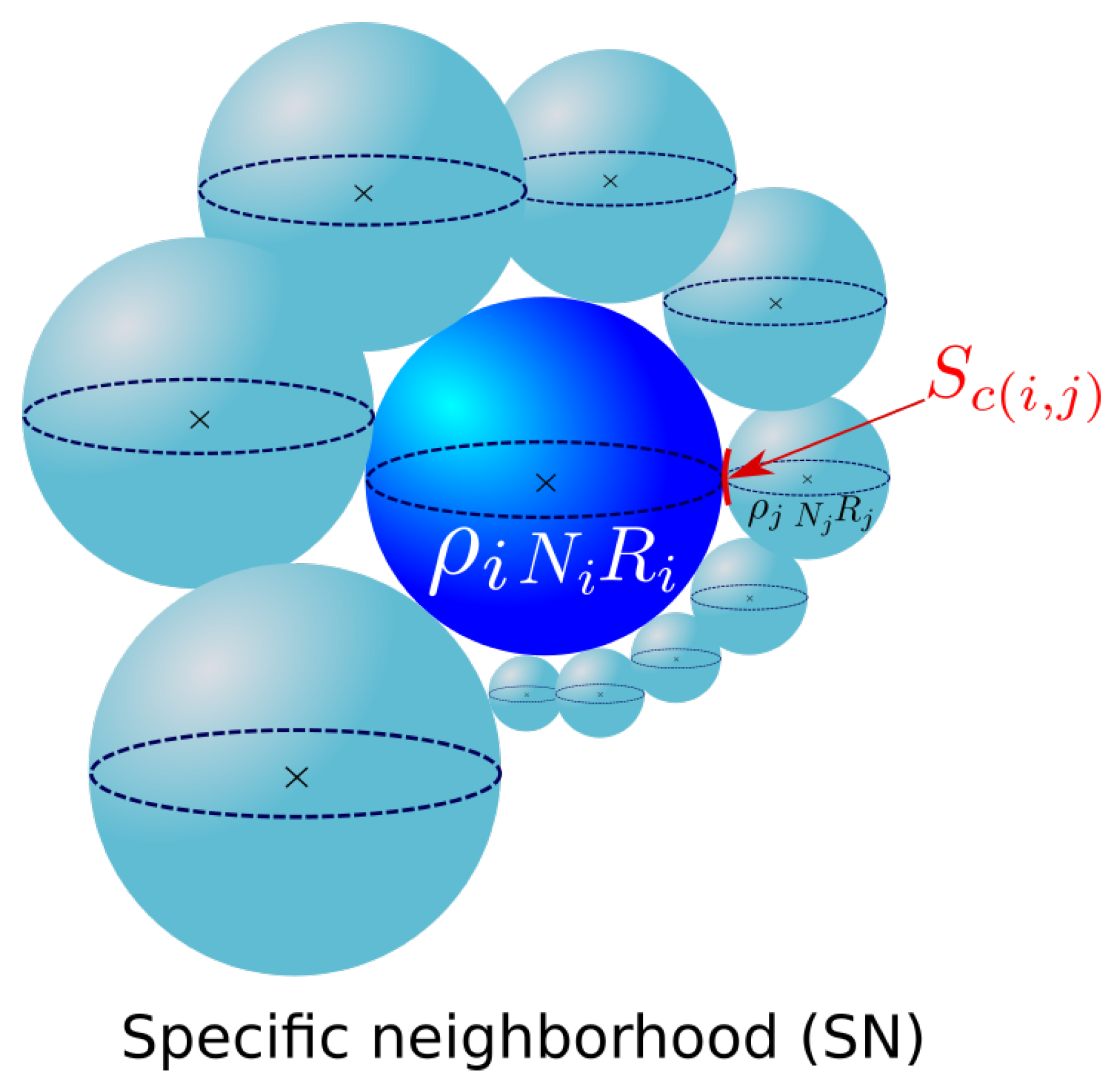
2.4. Maire et al. Model
3. Input Data for Mean-Field Modeling
3.1. Material-Dependent Model Parameters Acquisition
Experimental Data
3.2. Use of Saltykov Algorithm to Obtain a 3D GSD
3.2.1. GB Mobility Parameter Identification
A First Approximation Using the Classical B&T Law
Refined Identification
Model-Dependence of Reduced Mobility
4. Results and Discussion
4.1. Numerical Parameters
4.1.1. Convergence Study Concerning the Number of Grain Classes Introduced in the Model
4.1.2. Different Spatial Dimensions Considered to Define the Contact Probability
Description of the Spatial Dimensions
Impact on the Distribution Results
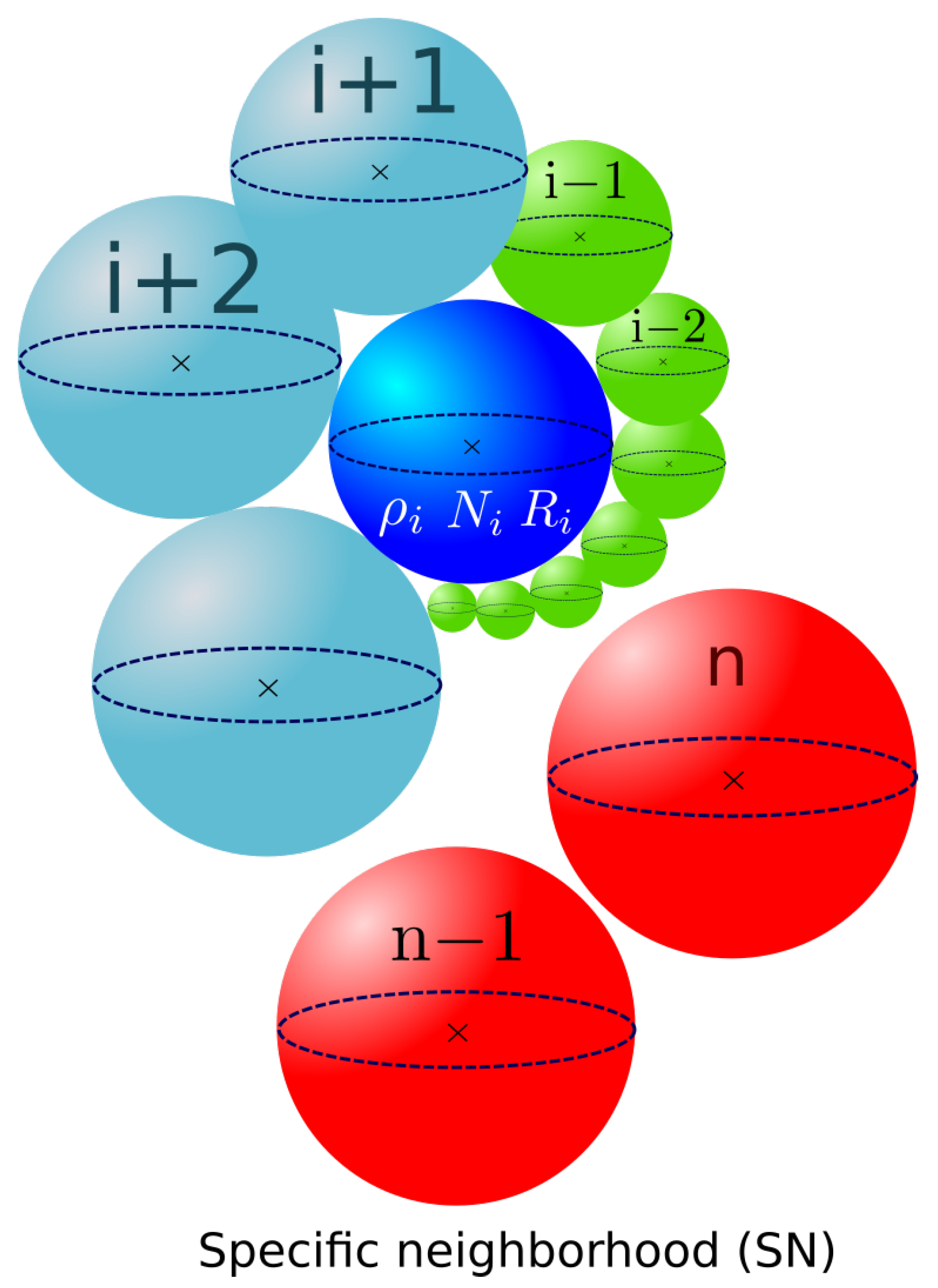
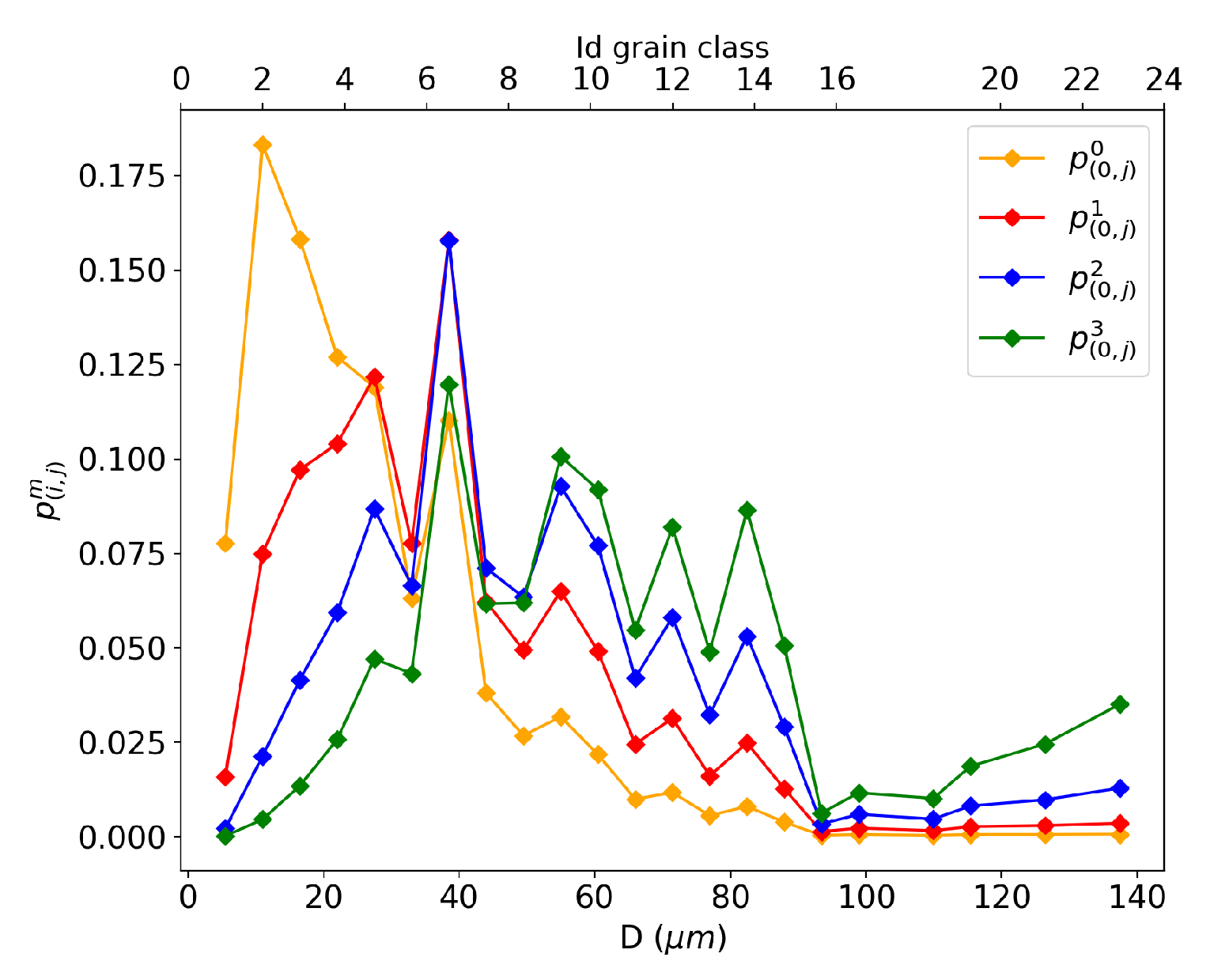
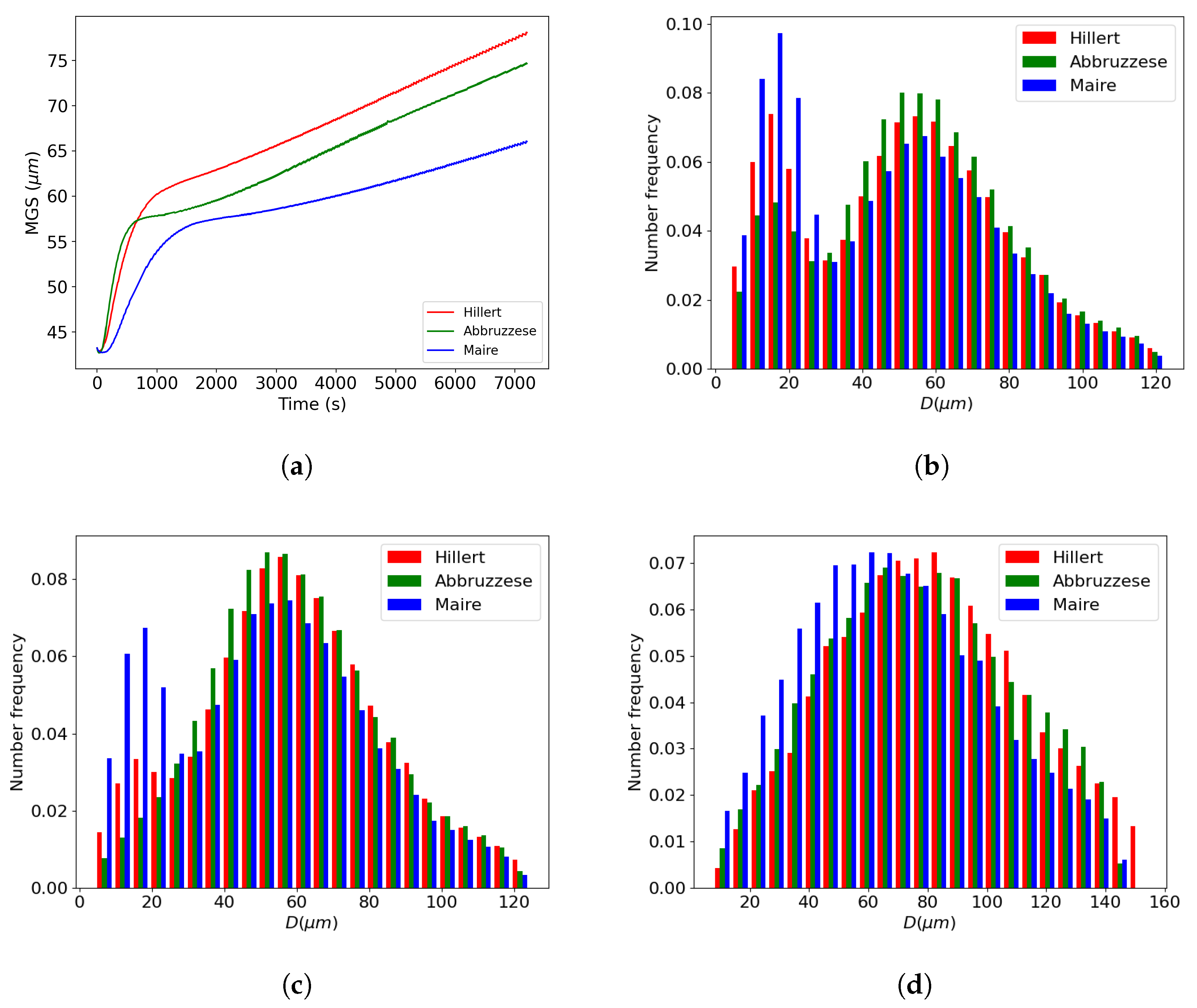
4.1.3. Impact of the Selection Order of Grain Classes on the Neighborhood Construction
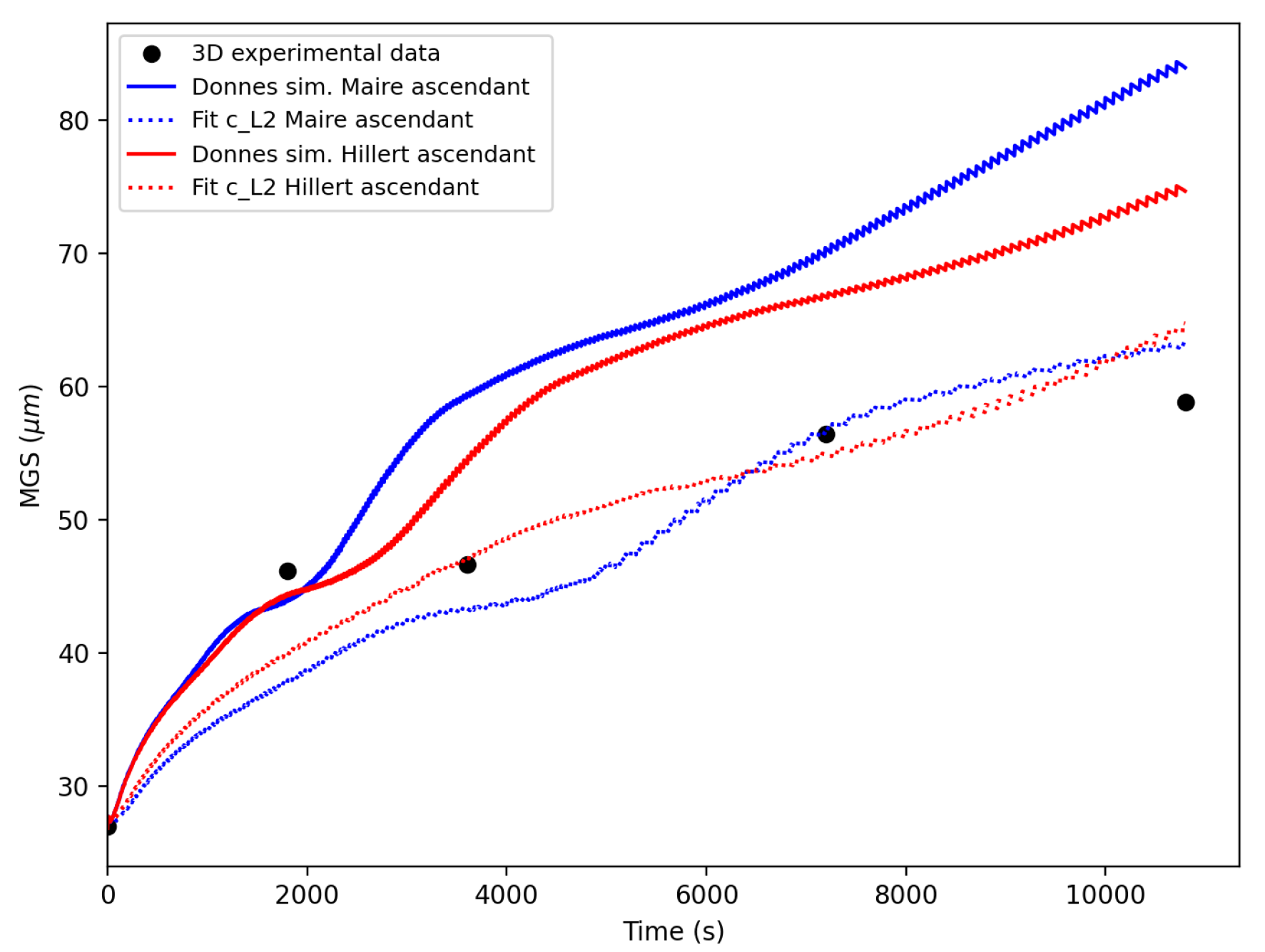
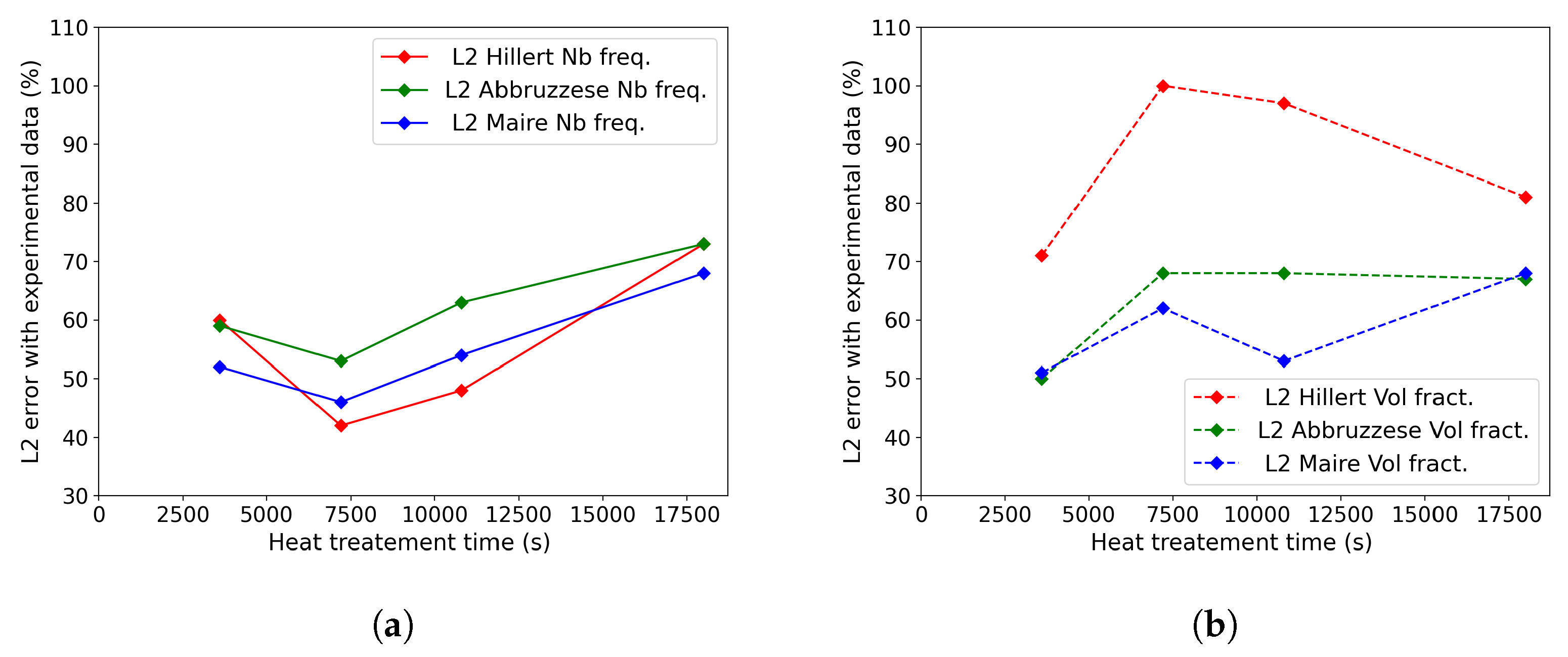
4.2. Comparison of Mean-Field Models Using Different Initial Microstructures
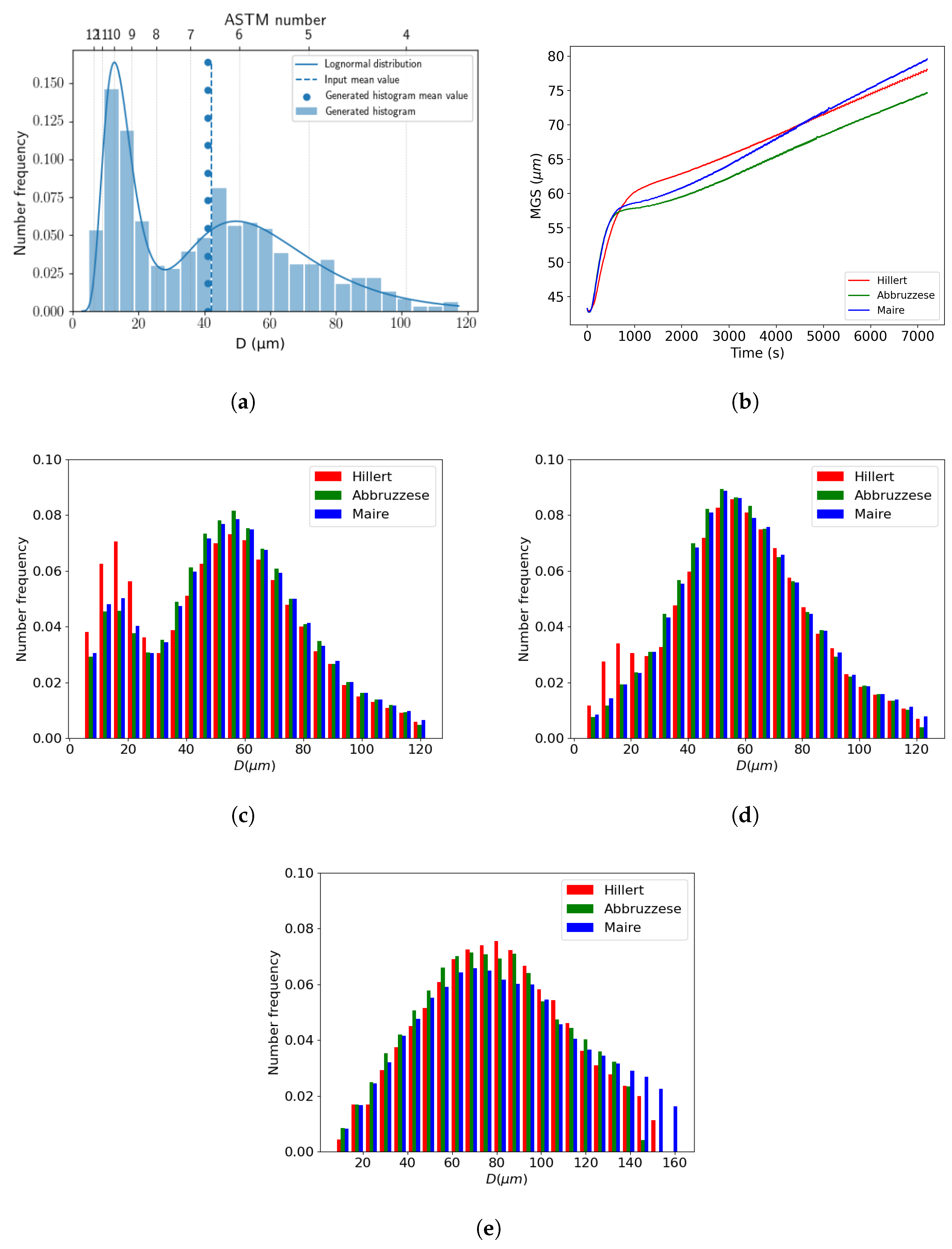
4.2.1. Comparison of Mean-Field Models with a Monomodal Initial Microstructure
4.2.2. Comparison of Mean-Field Models on Bimodal Initial Microstructure
Impact of the Selection Order of Neighborhood Construction on the Distribution Prediction
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rollett, A.; Rohrer, G.S.; Humphreys, J. Recrystallization and Related Annealing Phenomena; Newnes: Oxford, UK, 2017. [Google Scholar]
- Avrami, M. Kinetics of Phase Change. I. General Theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Johnson, W.; Mehl, R. Reaction kinetics in processes of nucleation and growth. Trans. Am. Inst. Min. Engin. 1939, 135, 416–442. [Google Scholar]
- Bernacki, M.; Chastel, Y.; Coupez, T.; Logé, R. Level set framework for the numerical modelling of primary recrystallization in polycrystalline materials. Scr. Mater. 2008, 58, 1129–1132. [Google Scholar] [CrossRef]
- Hallberg, H. Approaches to Modeling of Recrystallization. Metals 2011, 1, 16–48. [Google Scholar] [CrossRef]
- Hillert, M. On the theory of normal and abnormal grain growth. Acta Metall. 1965, 13, 227–238. [Google Scholar] [CrossRef]
- Abbruzzese, G.; Heckelmann, I.; Lücke, K. Statistical theory of two-dimensional grain growth—I. The topological foundation. Acta Metall. Mater. 1992, 40, 519–532. [Google Scholar] [CrossRef]
- Montheillet, F.; Lurdos, O.; Damamme, G. A grain scale approach for modeling steady-state discontinuous dynamic recrystallization. Acta Mater. 2009, 57, 1602–1612. [Google Scholar] [CrossRef]
- Cram, D.; Zurob, H.; Brechet, Y.; Hutchinson, C. Modelling discontinuous dynamic recrystallization using a physically based model for nucleation. Acta Mater. 2009, 57, 5218–5228. [Google Scholar] [CrossRef]
- Bernard, P.; Bag, S.; Huang, K.; Logé, R. A two-site mean field model of discontinuous dynamic recrystallization. Mater. Sci. Eng. A 2011, 528, 7357–7367. [Google Scholar] [CrossRef]
- Favre, J.; Fabrègue, D.; Piot, D.; Tang, N.; Koizumi, Y.; Maire, E.; Chiba, A. Modeling Grain Boundary Motion and Dynamic Recrystallization in Pure Metals. Metall. Mater. Trans. A 2013, 44, 5861–5875. [Google Scholar] [CrossRef]
- Maire, L.; Fausty, J.; Bernacki, M.; Bozzolo, N.; Micheli, P.D.; Moussa, C. A new topological approach for the mean field modeling of dynamic recrystallization. Mater. Des. 2018, 146, 194–207. [Google Scholar] [CrossRef]
- Burke, J.; Turnbull, D. Recrystallization and grain growth. Prog. Metal Phys. 1952, 3, 220–292. [Google Scholar] [CrossRef]
- Lücke, K.; Heckelmann, I.; Abbruzzese, G. Statistical theory of two-dimensional grain growth—II. Kinetics of grain growth. Acta Metall. Mater. 1992, 40, 533–542. [Google Scholar] [CrossRef]
- Beltran, O.; Huang, K.; Logé, R. A mean field model of dynamic and post-dynamic recrystallization predicting kinetics, grain size and flow stress. Comput. Mater. Sci. 2015, 102, 293–303. [Google Scholar] [CrossRef]
- Flipon, B.; Bozzolo, N.; Bernacki, M. A simplified intragranular description of dislocation density heterogeneities to improve dynamically recrystallized grain size predictions. Materialia 2022, 26, 101585. [Google Scholar] [CrossRef]
- Bachmann, F.; Hielscher, R.; Schaeben, H. Texture Analysis with MTEX—Free and Open Source Software Toolbox. Solid State Phenom. 2010, 160, 63–68. [Google Scholar] [CrossRef]
- Saltykov, S. Stereometric Metallography; Metallurgizdat: Moscow, Russia, 1958. [Google Scholar]
- Tucker, J.C.; Chan, L.H.; Rohrer, G.S.; Groeber, M.A.; Rollett, A.D. Comparison of grain size distributions in a Ni-based superalloy in three and two dimensions using the Saltykov method. Scr. Mater. 2012, 66, 554–557. [Google Scholar] [CrossRef]
- Lopez-Sanchez, M.; Llana-Fúnez, S. An extension of the Saltykov method to quantify 3D grain size distributions in mylonites. J. Struct. Geol. 2016, 93, 146–161. [Google Scholar] [CrossRef]
- Di Schino, A.; Kenny, J.M.; Salvatori, I.; Abbruzzese, G. Modelling primary recrystallization and grain growth in a low nickel austenitic stainless steel. J. Mater. Sci. 2001, 36, 593–601. [Google Scholar] [CrossRef]
- Rohrer, G.S. “Introduction to Grains, Phases, and Interfaces—An Interpretation of Microstructure,” Trans. AIME, 1948, vol. 175, pp. 15–51, by CS Smith. Metall. Mater. Trans. A 2010, 41, 1063–1100. [Google Scholar] [CrossRef]
- Kohara, S.; Parthasarathi, M.N.; Beck, P.A. Anisotropy of Boundary Mobility. J. Appl. Phys. 1958, 29, 1125–1126. [Google Scholar] [CrossRef]
- ASTM E112-96; Standard Test Methods for Determining Average Grain Size. ASTM International: West Conshohocken, PA, USA, 2004.
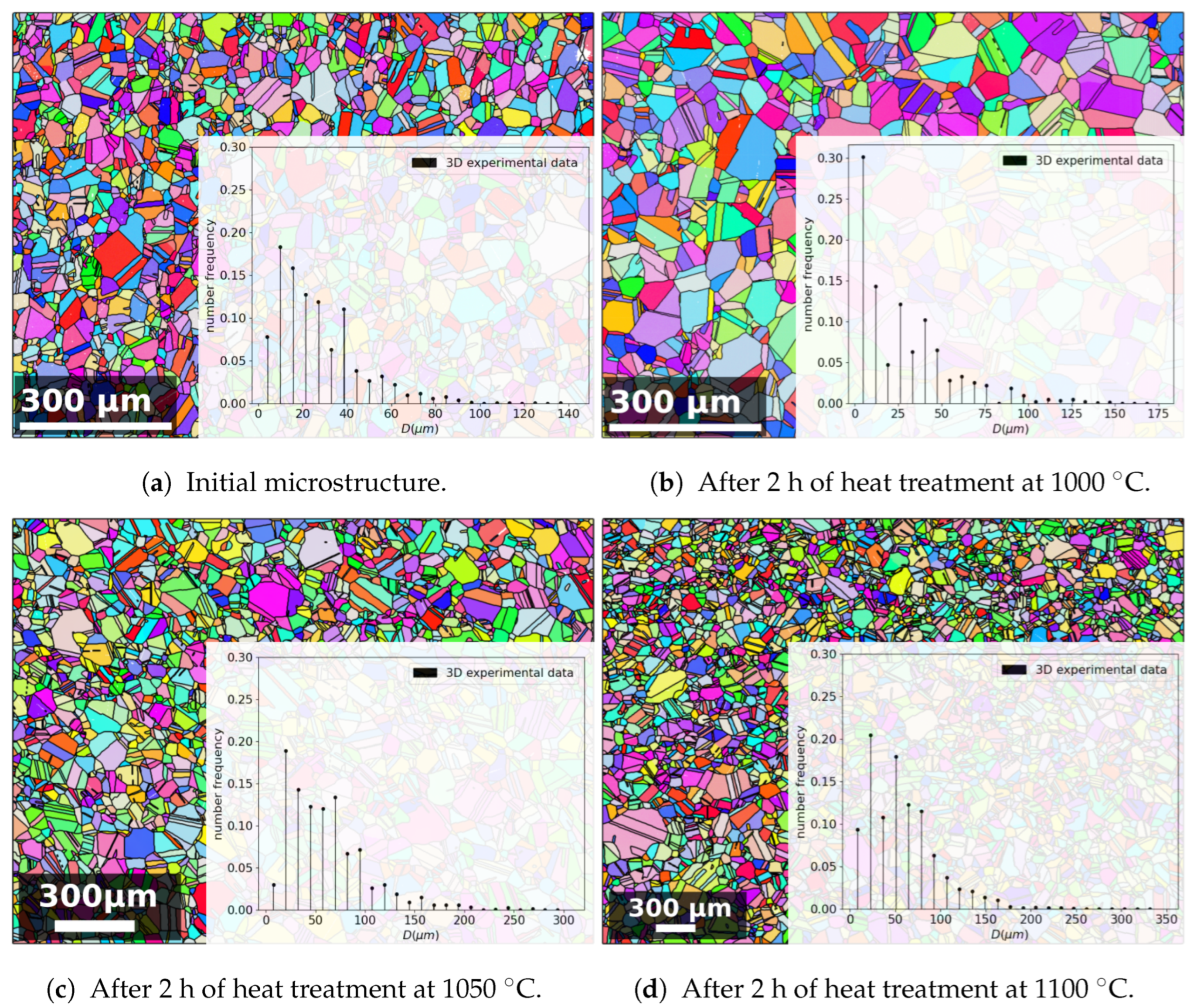







| 1000 °C | 1050 °C | 1100 °C |
|---|---|---|
| 30 min | 30 min | 30 min |
| 1 h | 1 h | 1 h |
| 2 h | 2 h | 2 h |
| 3 h | 3 h | 3 h |
| 5 h | 5 h | 5 h |
| T = 1000 °C | T = 1050 °C | T = 1100 °C | |||||||
|---|---|---|---|---|---|---|---|---|---|
| (m) | × (mm × mm) | #G | (m) | × (mm × mm) | #G | (m) | × (mm × mm) | #G | |
| Initial | 1.49 | 1.1 × 0.85 | 980 | 1.49 | 1.1 × 0.85 | 980 | 1.49 | 1.1 × 0.85 | 980 |
| 30 min | 2.5 | 2 × 1.4 | 2654 | 1.13 | 1 × 0.7 | 534 | 3.3 | 3.7 × 2.8 | 3509 |
| 1 h | 2.5 | 2 × 1.4 | 2078 | 3 | 3 × 2.2 | 1964 | 3.3 | 3.7 × 2.8 | 3590 |
| 2 h | 1.13 | 1 × 0.7 | 456 | 3 | 3 × 2.2 | 1154 | 3.77 | 3.7 × 2.8 | 2208 |
| 3 h | 1.13 | 1 × 0.7 | 468 | 1.13 | 1 × 0.7 | 300 | 3.77 | 3.7 × 2.8 | 2263 |
| 5 h | 1.13 | 1 × 0.7 | 243 | 1.13 | 1 × 0.7 | 133 | 3.77 | 3.7 × 2.8 | 2304 |
| Temperature | 1000 °C | 1050 °C | 1100 °C |
|---|---|---|---|
| (ms) | 2.30 × 10 | 1.08 × 10 | 1.10 × 10 |
| Model | Hillert | Abbruzzese | Maire |
|---|---|---|---|
| (ms) | 1.08 × 10 | 1.27 × 10 | 1.10 × 10 |
| Sorting Order | Ascending | Descending | Shuffle |
|---|---|---|---|
| (ms) | 2.19 × 10 | 5.00 × 10 | 2.30 × 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roth, M.; Flipon, B.; Bozzolo, N.; Bernacki, M. Comparison of Grain-Growth Mean-Field Models Regarding Predicted Grain Size Distributions. Materials 2023, 16, 6761. https://doi.org/10.3390/ma16206761
Roth M, Flipon B, Bozzolo N, Bernacki M. Comparison of Grain-Growth Mean-Field Models Regarding Predicted Grain Size Distributions. Materials. 2023; 16(20):6761. https://doi.org/10.3390/ma16206761
Chicago/Turabian StyleRoth, Marion, Baptiste Flipon, Nathalie Bozzolo, and Marc Bernacki. 2023. "Comparison of Grain-Growth Mean-Field Models Regarding Predicted Grain Size Distributions" Materials 16, no. 20: 6761. https://doi.org/10.3390/ma16206761
APA StyleRoth, M., Flipon, B., Bozzolo, N., & Bernacki, M. (2023). Comparison of Grain-Growth Mean-Field Models Regarding Predicted Grain Size Distributions. Materials, 16(20), 6761. https://doi.org/10.3390/ma16206761






