The Effects of Hybrid Steel/Basalt Fibers on the Durability of Concrete Pavement against Freeze–Thaw Cycles
Abstract
:1. Introduction
2. Experimental Procedure
2.1. Raw Materials
2.2. Mix Proportion and Experimental Design
2.3. Test Procedures
2.3.1. Mass Loss Tests
2.3.2. Abrasion Resistance Tests
2.3.3. Compressive Strength Tests
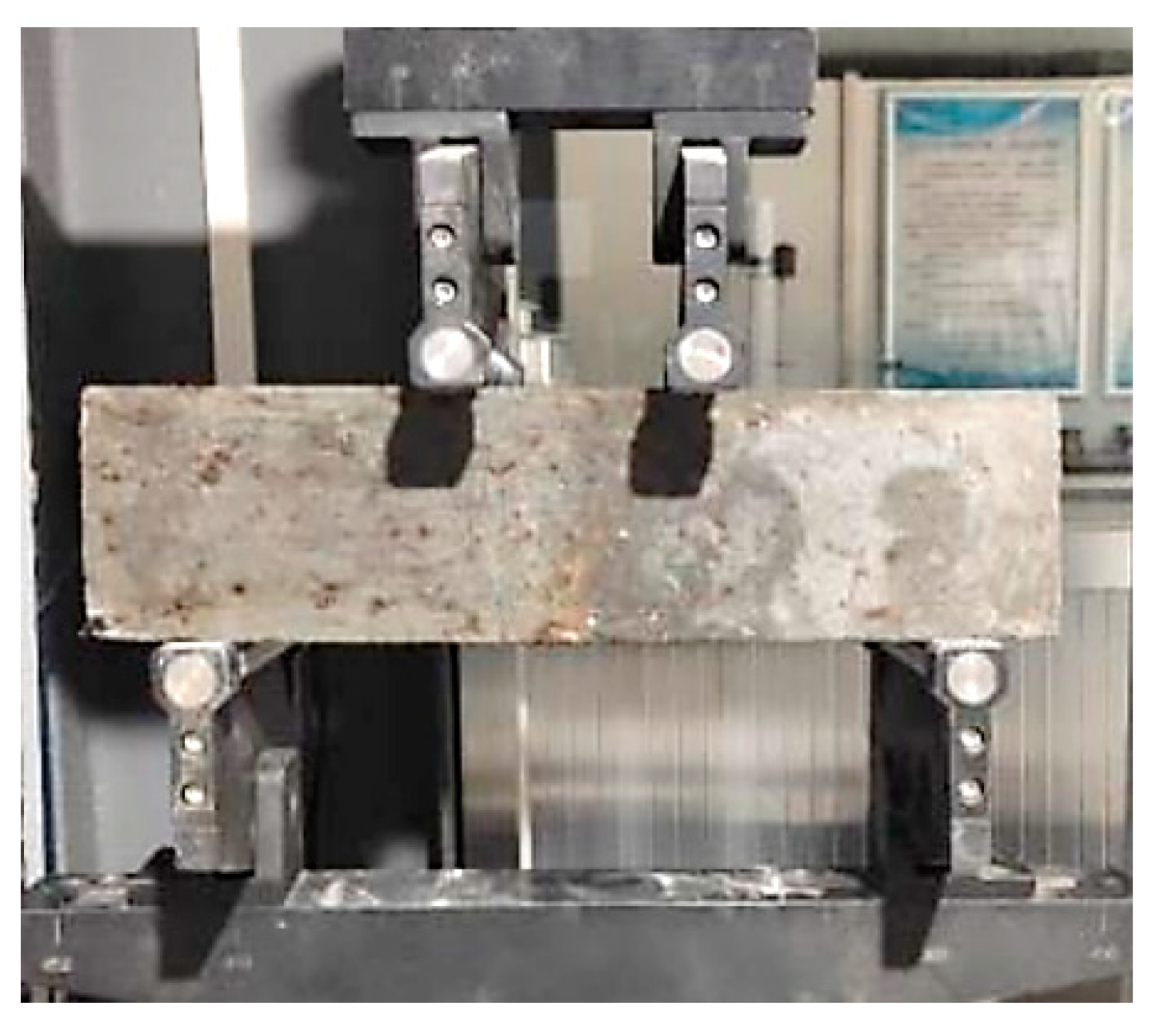
2.3.4. Flexural Strength Tests
2.3.5. Thickness of the Damaged Layer and Relative Dynamic Modulus of Elasticity Tests
2.3.6. Micro-Hardness Tests
2.3.7. SEM Tests
3. Results and Discussion
3.1. Macroscopic Results
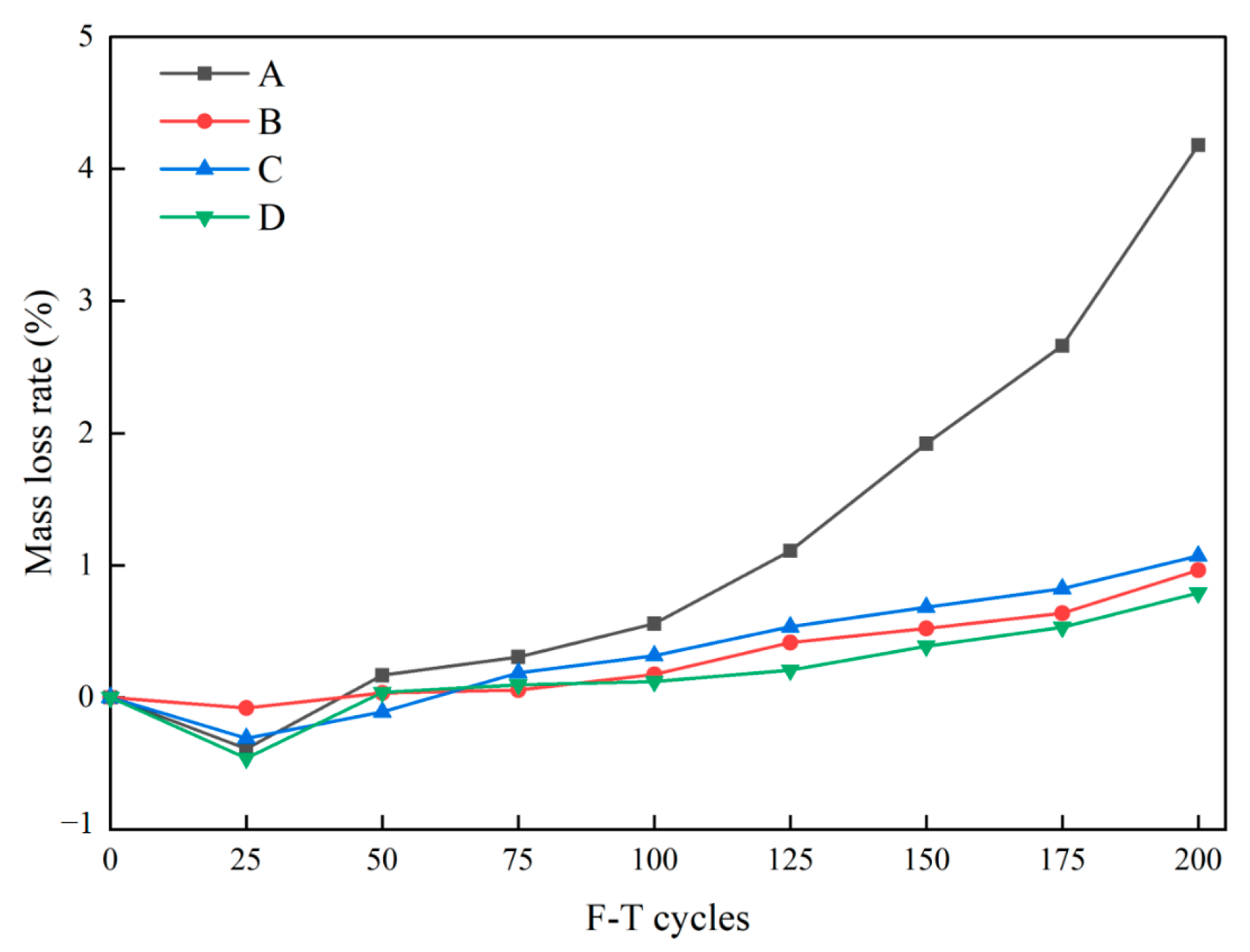
3.1.1. Surface Deterioration and Mass Loss
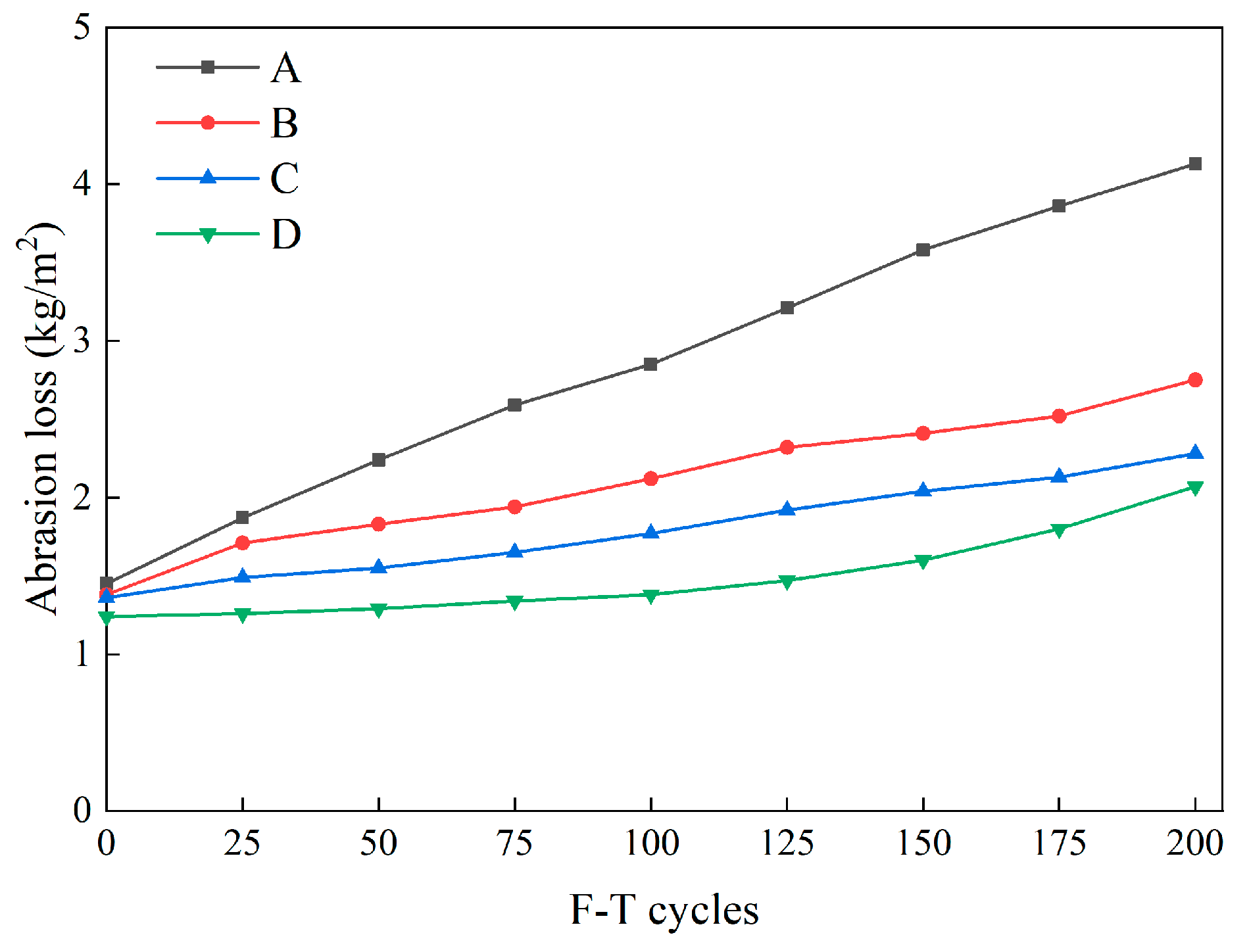
3.1.2. Abrasion Resistance
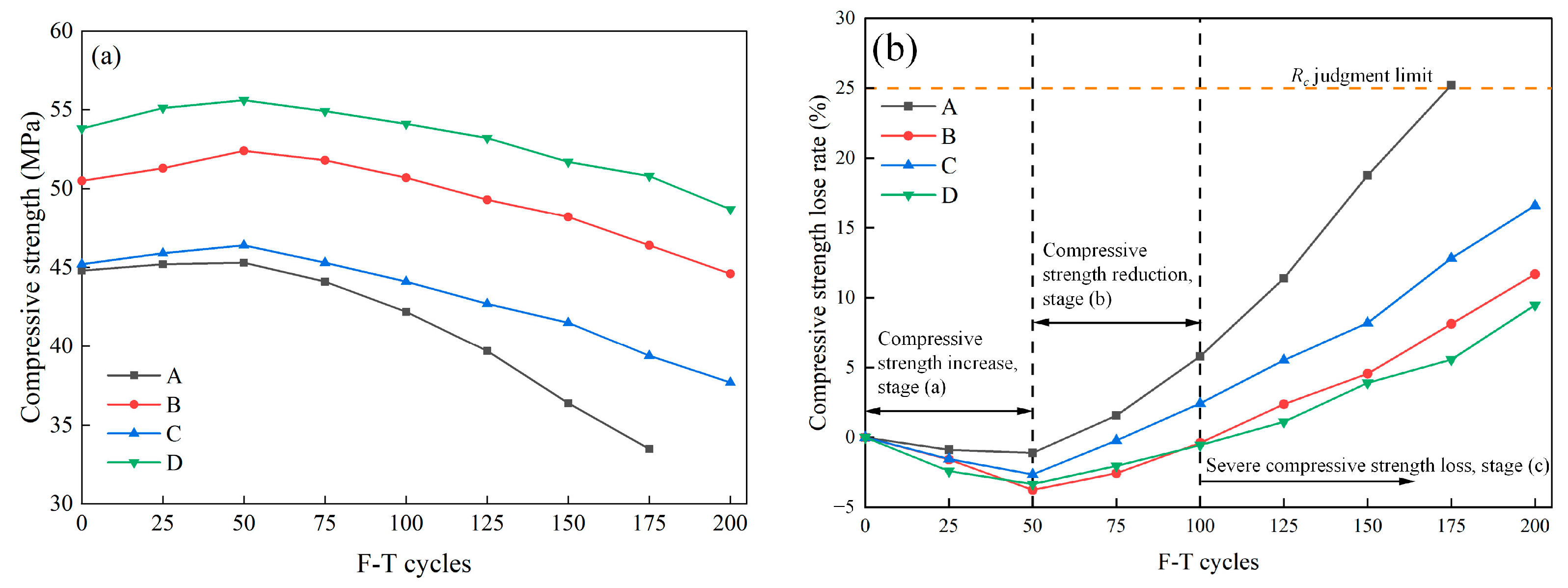
3.1.3. Compressive Strength Loss
3.1.4. Flexural Strength Loss
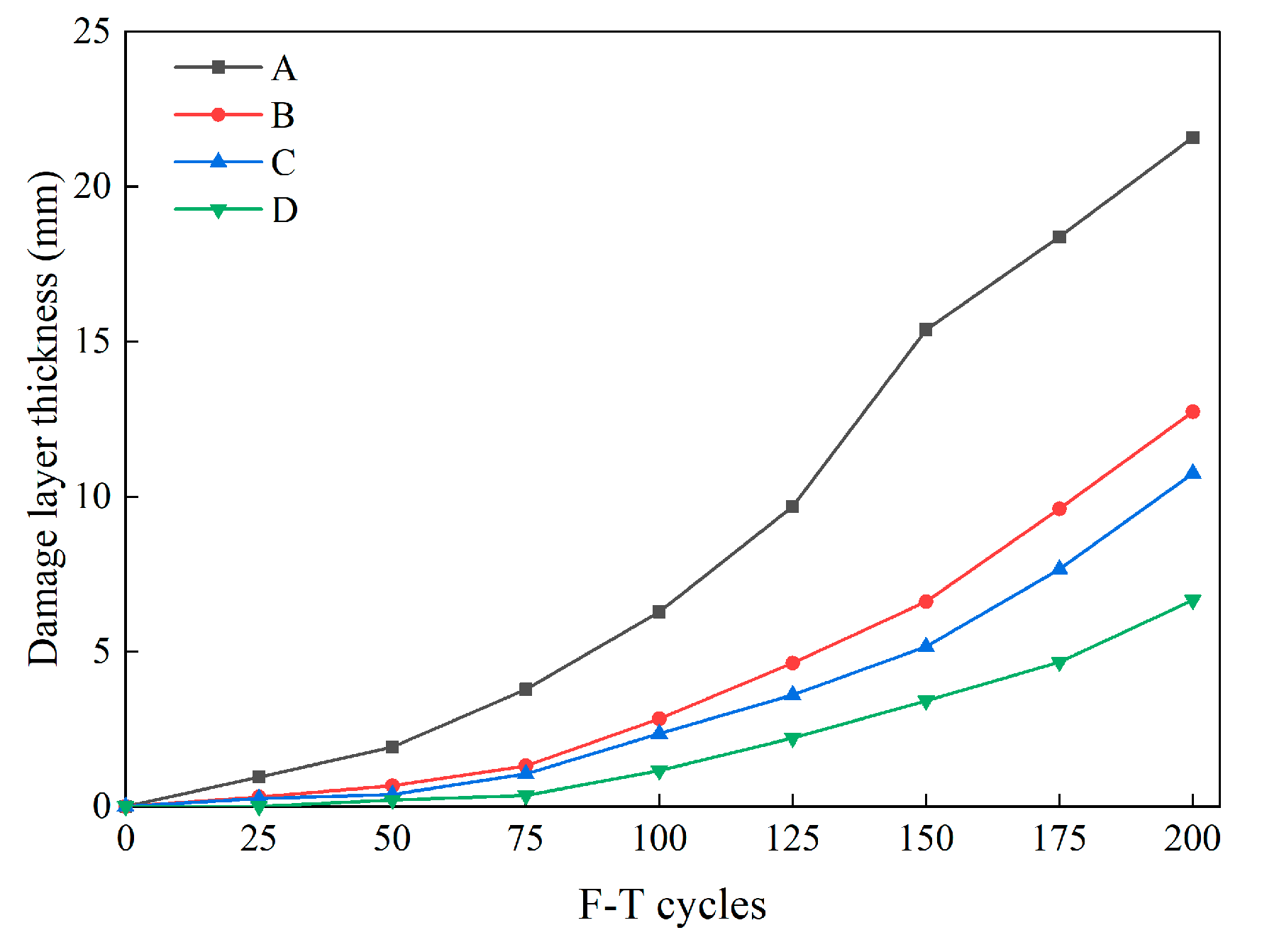
3.1.5. Damaged Layer Thickness
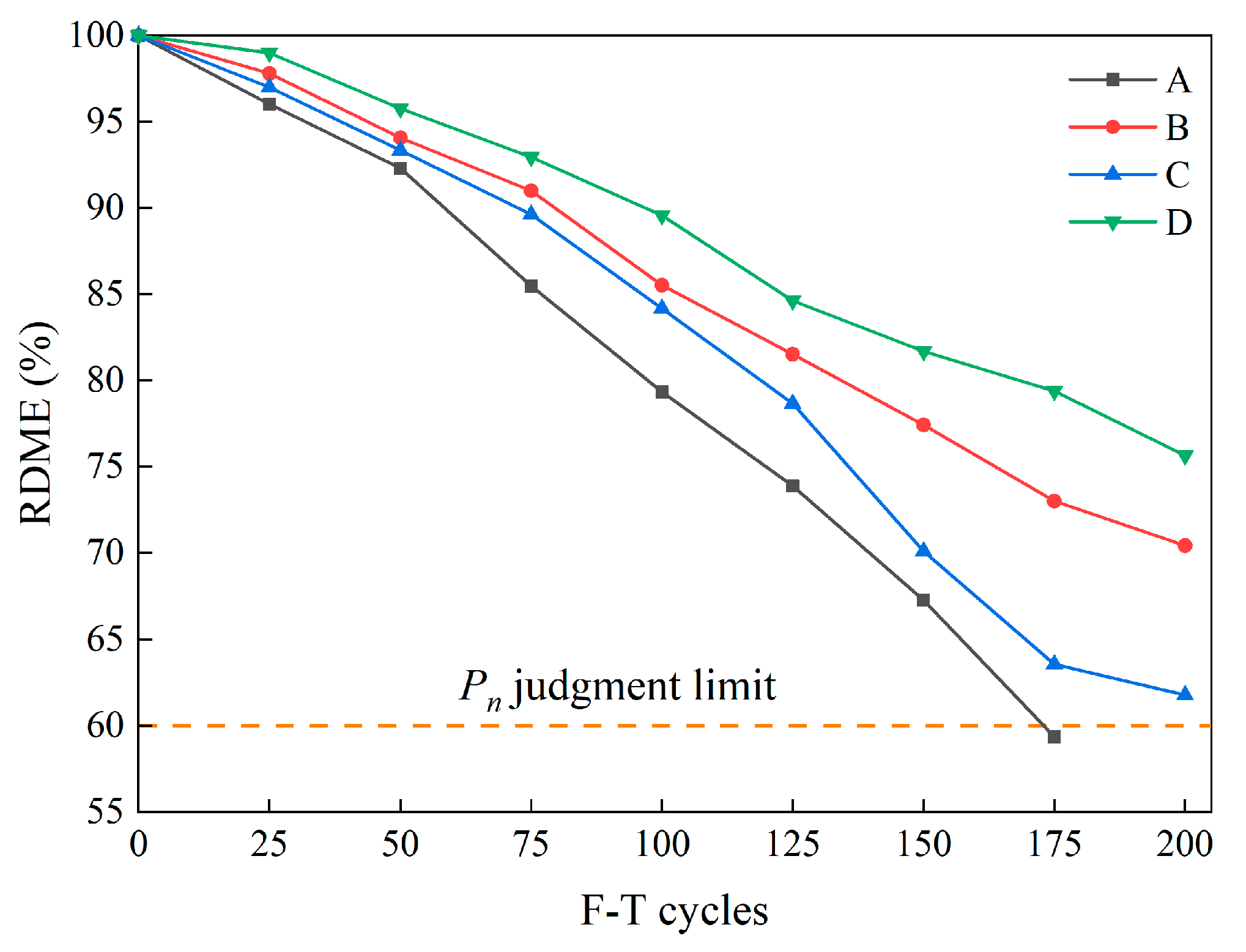
3.1.6. Relative Dynamic Modulus of Elasticity
3.2. Microscopic Results
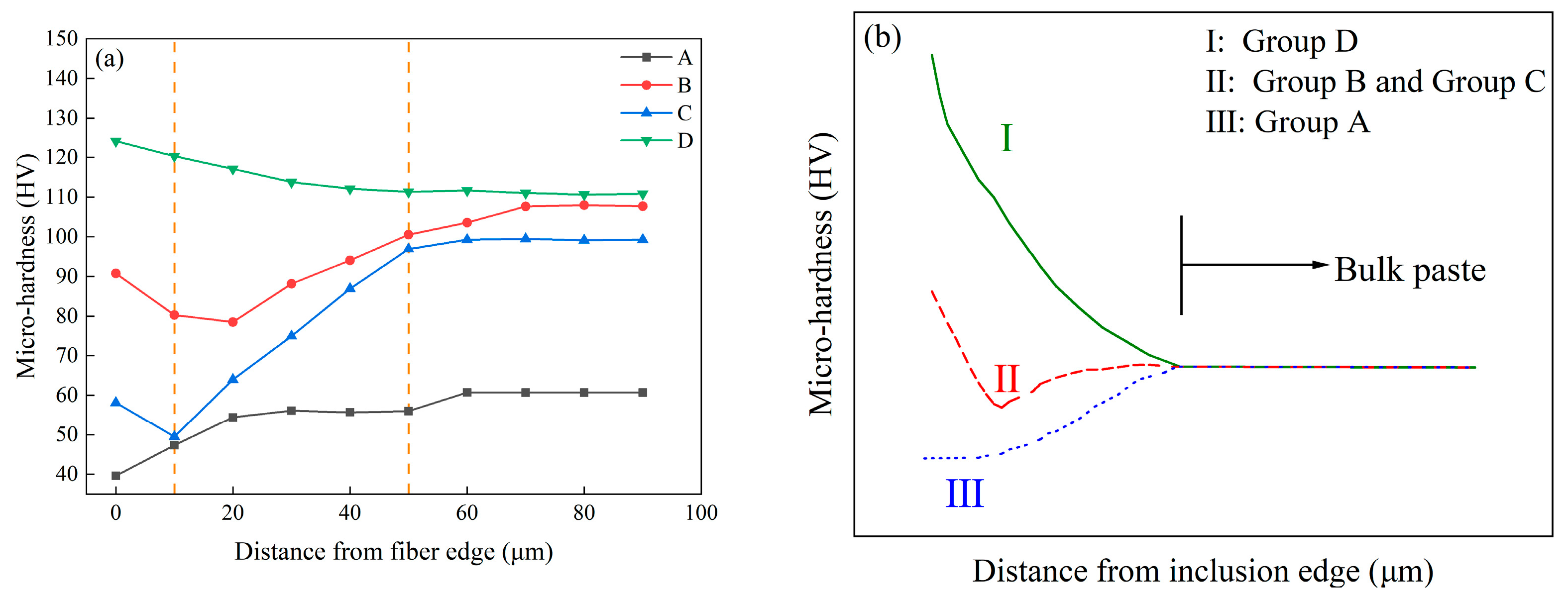
3.2.1. Micro-Hardness Test Results
3.2.2. SEM Observations
4. Grey–Markov Model of F-T Damage
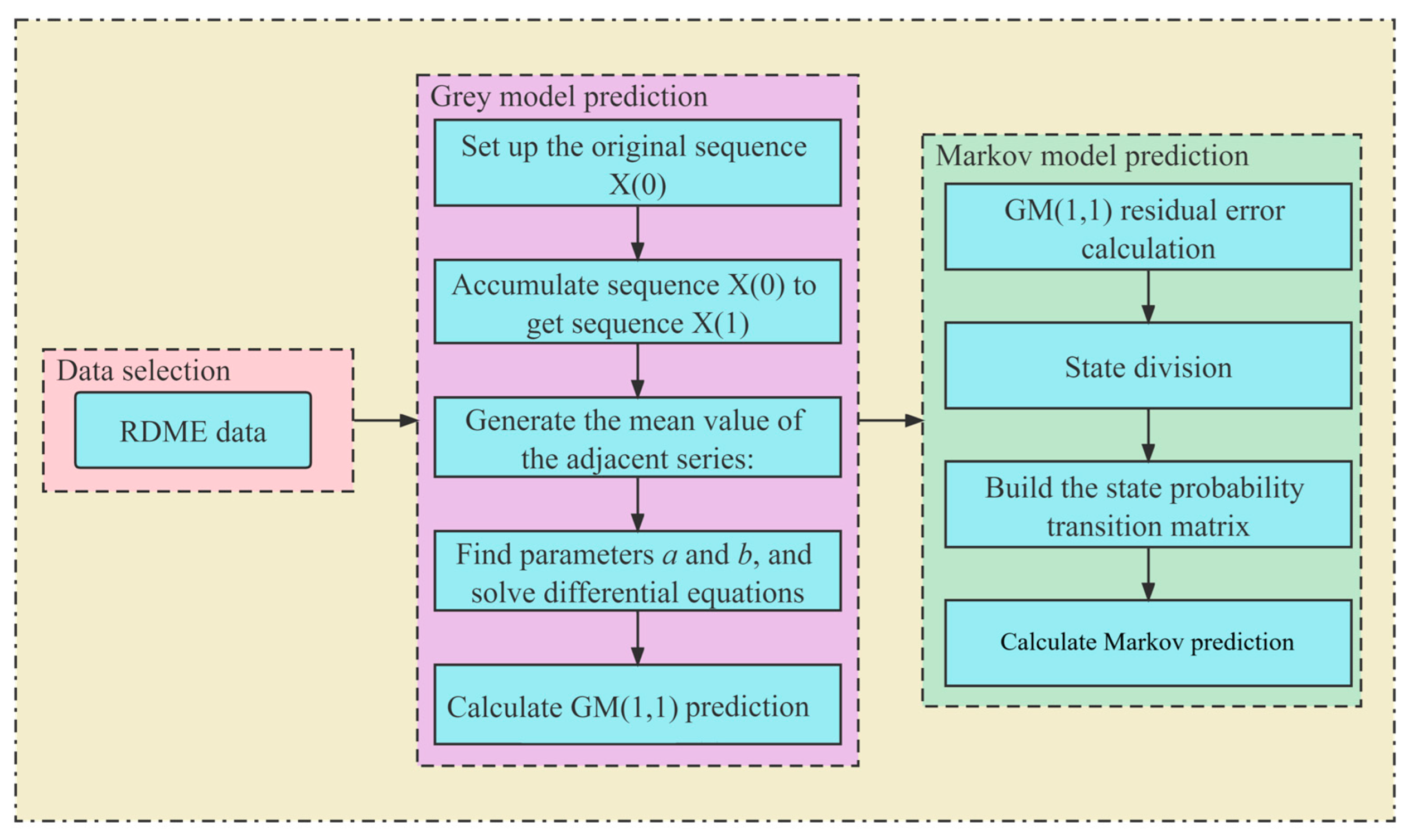
4.1. Grey Theory
4.2. Markov Chain Correction of Grey Model Errors
4.3. Analysis of Prediction Results
5. Conclusions
- (1)
- F-T cycling exerts detrimental effects on the durability characteristics of concrete. Compressive strength, flexural strength, abrasion resistance, and RDME typically exhibit a declining trend with increasing F-T cycles, while the mass loss and damage layer thickness increase with the augmentation of F-T cycles. A copious assembly of randomly dispersed hybrid SFs/BFs within the concrete matrix engenders a three-dimensional constraining framework, thereby efficaciously enhancing the F-T durability of the concrete.
- (2)
- The SEM analysis reveals that the fibers dissipate the energy required for crack propagation by means of friction with the cementitious matrix, as well as the pull-out energy and fracture energy of the fibers, thereby serving to toughen and impede crack propagation, consequently enhancing F-T resistance. The microhardness test results indicate that the ITZ strength is lowest in the control group, whereas in the HFRC, the impact of F-T cycles on the ITZ is relatively minimal due to the robust bonding between fibers and the surrounding matrix.
- (3)
- A Grey–Markov model, built upon the results obtained from the RDME test, is formulated to predict the service life of each group of specimens. The hybrid method affects the concrete’s service life. Under F-T cycles, the predicted life of each group in the sequence is Group D > Group B > Group C > Group A.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lv, Z.; Yuan, S.; Lin, H. Analytical stress solution for cold region tunnels with unequal ground stress and support delay under different frost heave conditions of surrounding rock. Cold Reg. Sci. Technol. 2023, 206, 103742. [Google Scholar] [CrossRef]
- Lou, W.; Zhang, Y.; Gu, Y.; Luo, G.; Bai, H.; Huang, M.; Virk, M.S.; Bian, R. An improved rigid rod method for wind-induced swing response prediction of heavily iced transmission lines. J. Wind. Eng. Ind. Aerodyn. 2023, 235, 105358. [Google Scholar] [CrossRef]
- Chen, B.; He, M.; Huang, Z.; Wu, Z. Long-term field test and numerical simulation of foamed polyurethane insulation on concrete dam in severely cold region. Constr. Build. Mater. 2019, 212, 618–634. [Google Scholar] [CrossRef]
- Swainson, I.P.; Schulson, E.M. A neutron diffraction study of ice and water within a hardened cement paste during freeze-thaw. Cem. Concr. Res. 2001, 31, 1821–1830. [Google Scholar] [CrossRef]
- Rong, X.; Li, L.; Zheng, S.; Wang, F.; Huang, W.; Zhang, Y.; Lu, D. Freeze-thaw damage model for concrete considering a nonuniform temperature field. J. Build. Eng. 2023, 72, 106747. [Google Scholar] [CrossRef]
- Niu, F.; He, J.; Jiang, H.; Jiao, C. Damage constitutive model for concrete under the coupling action of freeze-thaw cycles and load based on homogenization theory. J. Build. Eng. 2023, 76, 107152. [Google Scholar] [CrossRef]
- Alguhi, H.; Tomlinson, D. Crack behaviour and flexural response of steel and chopped glass fibre-reinforced concrete: Experimental and analytical study. J. Build. Eng. 2023, 75, 106914. [Google Scholar] [CrossRef]
- Mao, J.; Liang, N.; Liu, X.; Zhong, Z.; Zhou, C. Investigation on early-age cracking resistance of basalt-polypropylene fiber reinforced concrete in restrained ring tests. J. Build. Eng. 2023, 70, 106155. [Google Scholar] [CrossRef]
- Khan, M.; Cao, M.; Ali, M. Cracking behaviour and constitutive modelling of hybrid fibre reinforced concrete. J. Build. Eng. 2020, 30, 101272. [Google Scholar] [CrossRef]
- Zhang, Z.; Yang, F.; Liu, J.; Wang, S. Eco-friendly high strength, high ductility engineered cementitious composites (ECC) with substitution of fly ash by rice husk ash. Cem. Concr. Res. 2020, 137, 106200. [Google Scholar] [CrossRef]
- Haneef, T.; Kumari, K.; Mukhopadhyay, C.; Venkatachalapathy; Purnachandra, B.; Jayakumar, T. Influence of fly ash and curing on cracking behavior of concrete by acoustic emission technique. Constr. Build. Mater. 2013, 44, 342–350. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, Z.; He, J.; Shi, X. High-strength engineered cementitious composites with nanosilica incorporated: Mechanical performance and autogenous self-healing behavior. Cem. Concr. Compos. 2023, 135, 104837. [Google Scholar] [CrossRef]
- Guler, S.; Akbulut, Z.F. Effect of freeze-thaw cycles on strength and toughness properties of new generation 3D/4D/5D steel fiber-reinforced concrete. J. Build. Eng. 2022, 51, 104239. [Google Scholar] [CrossRef]
- Han, J.; Zhang, W.; Liu, Y. Experimental Study on freeze-thaw resistance of steel fiber-reinforced hydraulic concrete with two-grade aggregate. J. Build. Eng. 2022, 60, 105181. [Google Scholar] [CrossRef]
- Zeng, W.; Ding, Y.; Zhang, Y.; Dehn, F. Effect of steel fiber on the crack permeability evolution and crack surface topography of concrete subjected to freeze-thaw damage. Cem. Concr. Res. 2020, 138, 106230. [Google Scholar] [CrossRef]
- Aliha, M.R.M.; Karimi, H.; Abedi, M. The role of mix design and short glass fiber content on mode-I cracking characteristics of polymer concrete. Constr. Build. Mater. 2022, 317, 126139. [Google Scholar] [CrossRef]
- Zhang, Z.; Gao, Y.; Qin, F.; Sun, F.; Huang, Y. Mechanical properties of sustainable high strength ECC with substitution of cement by limestone powder. Case Stud. Constr. Mater. 2023, 19, e02434. [Google Scholar] [CrossRef]
- Shen, L.; Yao, X.; Luzio, G.D.; Jiang, M.; Han, Y. Mix optimization of hybrid steel and polypropylene fiber-reinforced concrete for anti-thermal spalling. J. Build. Eng. 2023, 63, 105409. [Google Scholar] [CrossRef]
- Shaikh, F.U.A.; Taweel, M. Compressive strength and failure behaviour of fibre reinforced concrete at elevated temperatures. Adv. Concr. Constr. 2015, 3, 283–293. [Google Scholar] [CrossRef]
- Guler, S.; Akbulut, Z. Workability, physical & mechanical properties of the cement mortars strengthened with metakaolin and steel/basalt fibers exposed to freezing-thawing periods. Constr. Build. Mater. 2023, 394, 132100. [Google Scholar]
- Zhang, N.; Zhou, J.; Ma, G. Dynamic Properties of Strain-Hardening Cementitious Composite Reinforced with Basalt and Steel Fibers. Int. J. Concr. Struct. Mater. 2020, 14, 44–58. [Google Scholar] [CrossRef]
- Khan, M.; Cao, M.; Ali, M. Effect of basalt fibers on mechanical properties of calcium carbonate whisker-steel fiber reinforced concrete. Constr. Build. Mater. 2018, 192, 742–753. [Google Scholar] [CrossRef]
- Khan, M.; Cao, M.; Xie, C.; Ali, M. Effectiveness of hybrid steel-basalt fiber reinforced concrete under compression. Case Stud. Constr. Mater. 2022, 16, e00941. [Google Scholar] [CrossRef]
- Afroz, M.; Venkatesan, S.; Patnaikuni, I. Effects of hybrid fibers on the development of high volume fly ash cement composite. Constr. Build. Mater. 2019, 215, 984–997. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, Q.; Li, V.C. Multiple-scale investigations on self-healing induced mechanical property recovery of ECC. Cem. Concr. Compos. 2019, 103, 293–302. [Google Scholar] [CrossRef]
- Liu, D.; Yu, J.; Qin, F.; Zhang, K.; Zhang, Z. Mechanical performance of high-strength engineering cementitious composites (ECC) with hybriding PE and steel fibers. Case Stud. Constr. Mater. 2023, 18, e01961. [Google Scholar] [CrossRef]
- Ma, K.; Feng, K.; Wang, Z.; Long, G.; Xie, Y.; Li, W. Mechanical properties and crack evolution of SCC with macro-crack under freeze-thaw cycles. J. Build. Eng. 2023, 69, 106323. [Google Scholar] [CrossRef]
- Liu, X.; Yan, Z.; Wang, D.; Zhao, R.; Niu, D.; Wang, Y. Corrosion cracking behavior of reinforced concrete under freeze-thaw cycles. J. Build. Eng. 2023, 64, 105610. [Google Scholar] [CrossRef]
- JTG D40-2011; Specifications for Design of Highway Cement Concrete Pavement. China Building Industry Press: Beijing, China, 2011.
- Yu, J.; Qiao, H.; Zhu, F.; Wang, X. Research on Damage and Deterioration of Fiber Concrete under Acid Rain Environment Based on GM(1,1)-Markov. Materials 2021, 14, 6326. [Google Scholar] [CrossRef]
- JTG 3420-2020; Testing Methods of Cement and Concrete for Highway Engineering. China Ministry of Transport Press: Beijing, China, 2020.
- Yu, J.; Liu, D.; Zhang, Z. Durability and life prediction analysis of recycled aggregate concrete with ceramic waste powder under freeze-thaw conditions based on impact-echo method and Grey-Markov model. Front. Mater. 2022, 9, 1060294. [Google Scholar] [CrossRef]
- Liu, D.; Tu, Y.; Sas, G.; Elfgren, L. Freeze-thaw damage evaluation and model creation for concrete exposed to freeze-thaw cycles at early-age. Constr. Build. Mater. 2021, 312, 125352. [Google Scholar] [CrossRef]
- Xia, D.; Yu, S.; Yu, J.; Feng, C.; Li, B.; Zheng, Z.; Wu, H. Damage characteristics of hybrid fiber reinforced concrete under the freeze-thaw cycles and compound-salt attack. Case Stud. Constr. Mater. 2023, 18, e01814. [Google Scholar] [CrossRef]
- Qiao, Y.; Sun, W.; Jiang, J. Damage process of concrete subjected to coupling fatigue load and freeze/thaw cycles. Constr. Build. Mater. 2015, 93, 806–811. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhang, H.; Zhu, K.; Tang, Z.; Zhang, H. Deterioration mechanism on Micro-structure of unsaturated polyester resin modified concrete for bridge deck pavement under salty Freeze-thaw cycles. Constr. Build. Mater. 2023, 368, 130366. [Google Scholar] [CrossRef]
- Borosnyói, A.; Erdélyi, A. De-Icing Salt Scaling Damage Kinetics of Fibre Reinforced Concretes Made with High Bond Crimped Steel Fibres. Period. Polytech. Civ. Eng. 2015, 59, 279–286. [Google Scholar] [CrossRef]
- Yoo, D.Y.; Park, J.J.; Kim, S.W. Fiber pullout behavior of HPFRCC: Effects of matrix strength and fiber type. Compos. Struct. 2017, 174, 263–276. [Google Scholar] [CrossRef]
- Igarashi, S.; Bentur, A.; Mindess, S. Microhardness testing of cementitious materials. Adv. Cem. Based Mater. 1996, 4, 48–57. [Google Scholar] [CrossRef]
- Yuan, X.; Dai, M.; Li, M.; Liu, F. Study of the Freeze-Thaw Resistance for Composite Fiber Recycled Concrete with Sulphate Attack Exposure. Buildings 2023, 13, 1037. [Google Scholar] [CrossRef]
- Horszczaruk, E.; Brzozowski, P. Abrasion resistance and mechanical strength of underwater repair concrete curing under hydrostatic pressure. Constr. Build. Mater. 2023, 394, 132256. [Google Scholar] [CrossRef]
- Alahmad, S.; Toumi, A.; Verdier, J.; Francois, R. Effect of crack opening on carbon dioxide penetration in cracked mortar samples. Mater. Struct. 2008, 42, 559–566. [Google Scholar] [CrossRef]
- Nia, A.; Hedayatian, M.; Nili, M.; Afrough, V. An experimental and numerical study on how steel and polypropylene fibers affect the impact resistance in fiber-reinforced concrete. Int. J. Impact Eng. 2012, 46, 62–73. [Google Scholar]
- Jabeen, G.; Yang, X.; Luo, P.; Rahim, S. Application of Grey-Markov Chain Model in Software Reliability Prediction. J. Comput. 2019, 30, 14–27. [Google Scholar]
- Yang, X.; Wang, S.; Peng, Y.; Chen, J.; Meng, L. Short-term photovoltaic power prediction with similar-day integrated by BP-AdaBoost based on the Grey-Markov model. Electr. Power Syst. Res. 2023, 215, 108966. [Google Scholar] [CrossRef]








| Chemical Composition | SiO2 | Al2O3 | CaO | Fe2O3 | MgO | MnO | K2O | IL | TiO2 |
|---|---|---|---|---|---|---|---|---|---|
| Cement | 26.7 | 11.5 | 48.9 | 4.9 | 3.0 | 0.4 | 1.6 | 1.8 | 1.2 |
| Fly ash | 46.4 | 29.9 | 9.4 | 6.9 | 1.9 | 0.2 | 1.5 | 2.4 | 1.4 |
| Types | Length (mm) | Diameter (μm) | Density (g/cm3) | Elastic Modulus (GPa) | Tensile Modulus (GPa) |
|---|---|---|---|---|---|
| SF | 30 | 50 | 7.8 | 200 | 1.2 |
| BF | 12 | 20 | 2.7 | 100 | 4.5 |
| Samples | A | B | C | D |
|---|---|---|---|---|
| Cement/(kg·m−3) | 400 | 400 | 400 | 400 |
| Fly ash/(kg·m−3) | 100 | 100 | 100 | 100 |
| Coarse aggregate/(kg·m−3) | 1165 | 1165 | 1165 | 1165 |
| Fine aggregate/(kg·m−3) | 635 | 635 | 635 | 635 |
| Water /(kg·m−3) | 200 | 200 | 200 | 200 |
| Water reducer/(kg·m−3) | 4.5 | 4.5 | 4.5 | 4.5 |
| SF/% (by volume fraction) | / | 2.0 | / | 2.0 |
| BF/%(by volume fraction) | / | / | 0.1 | 0.1 |
| Test Project | Specimen Dimension/mm |
|---|---|
| Mass loss | 100 × 100 × 100 |
| Abrasion resistance | 150 × 150 × 150 |
| Compressive strength test | 100 × 100 × 100 |
| Flexural strength test | 400 × 100 × 100 |
| Damaged layer thickness | 400 × 100 × 100 |
| Relative dynamic modulus of elasticity | 400 × 100 × 100 |
| Samples | a | b | R2 | Expected Service Life (Time) |
|---|---|---|---|---|
| A | 0.077 | 109.810 | 0.9791 | 175 |
| B | 0.048 | 105.972 | 0.9932 | 280 |
| C | 0.068 | 110.108 | 0.9889 | 205 |
| D | 0.039 | 105.395 | 0.9942 | 350 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, J.; Yi, Z.; Zhang, Z.; Liu, D.; Ran, J. The Effects of Hybrid Steel/Basalt Fibers on the Durability of Concrete Pavement against Freeze–Thaw Cycles. Materials 2023, 16, 7137. https://doi.org/10.3390/ma16227137
Yu J, Yi Z, Zhang Z, Liu D, Ran J. The Effects of Hybrid Steel/Basalt Fibers on the Durability of Concrete Pavement against Freeze–Thaw Cycles. Materials. 2023; 16(22):7137. https://doi.org/10.3390/ma16227137
Chicago/Turabian StyleYu, Jianqiao, Zijing Yi, Zhigang Zhang, Dawei Liu, and Junxin Ran. 2023. "The Effects of Hybrid Steel/Basalt Fibers on the Durability of Concrete Pavement against Freeze–Thaw Cycles" Materials 16, no. 22: 7137. https://doi.org/10.3390/ma16227137
APA StyleYu, J., Yi, Z., Zhang, Z., Liu, D., & Ran, J. (2023). The Effects of Hybrid Steel/Basalt Fibers on the Durability of Concrete Pavement against Freeze–Thaw Cycles. Materials, 16(22), 7137. https://doi.org/10.3390/ma16227137







