Pyrolysis Kinetic Behavior and Thermodynamic Analysis of PET Nonwoven Fabric
Abstract
:1. Introduction
2. Experimental
2.1. Materials and PET Feedstock Preparation
2.2. Thermogravimetric Measurements
2.3. Chemical Analysis of the Generated Vapor Pyrolysis Compounds
2.4. Pyrolysis Kinetics and Thermodynamic Analysis of PET Membranes
3. Results and Discussion
3.1. Elemental and Proximate Analysis
3.2. Thermogravimetric Analysis
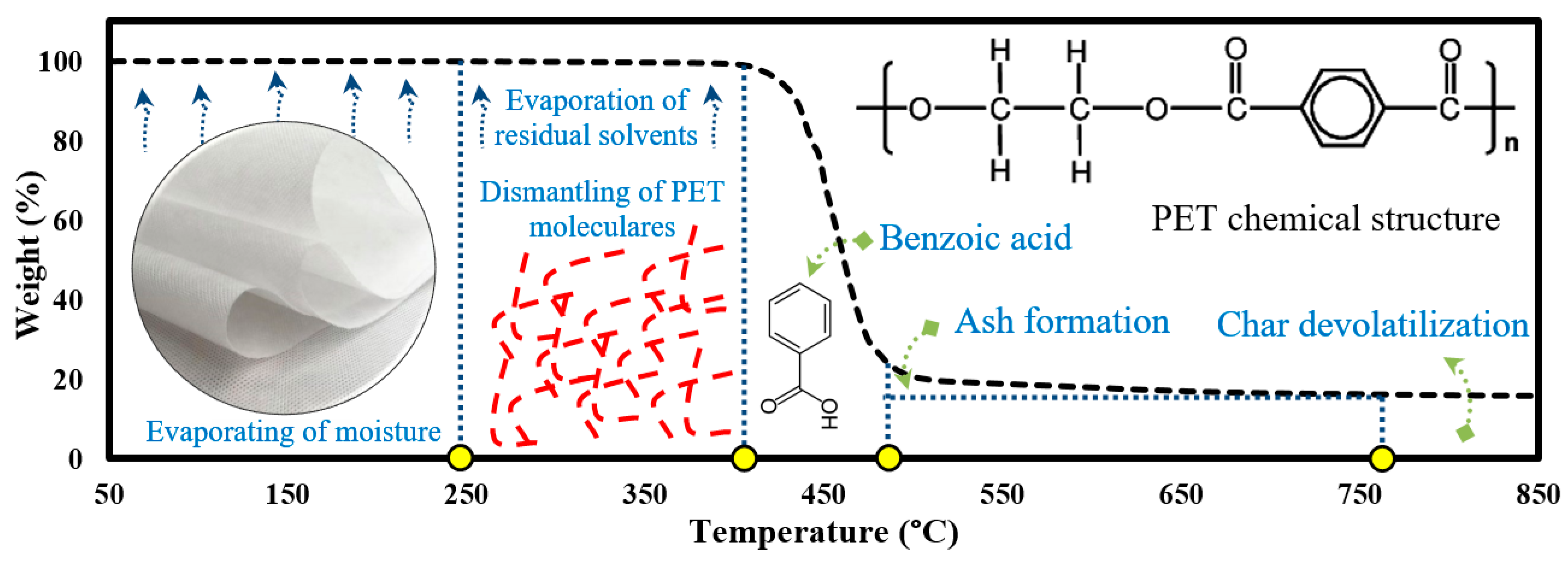
3.3. Mechanism of PET Membrane Pyrolysis
3.4. Chemical Analysis of the Synthesized Volatile Products Using TG-FTIR
3.5. Chemical Analysis of the Synthesized Chemical Compounds Using GC–MS
3.6. Kinetic and Thermodynamic Analysis of PET Fabric Pyrolysis
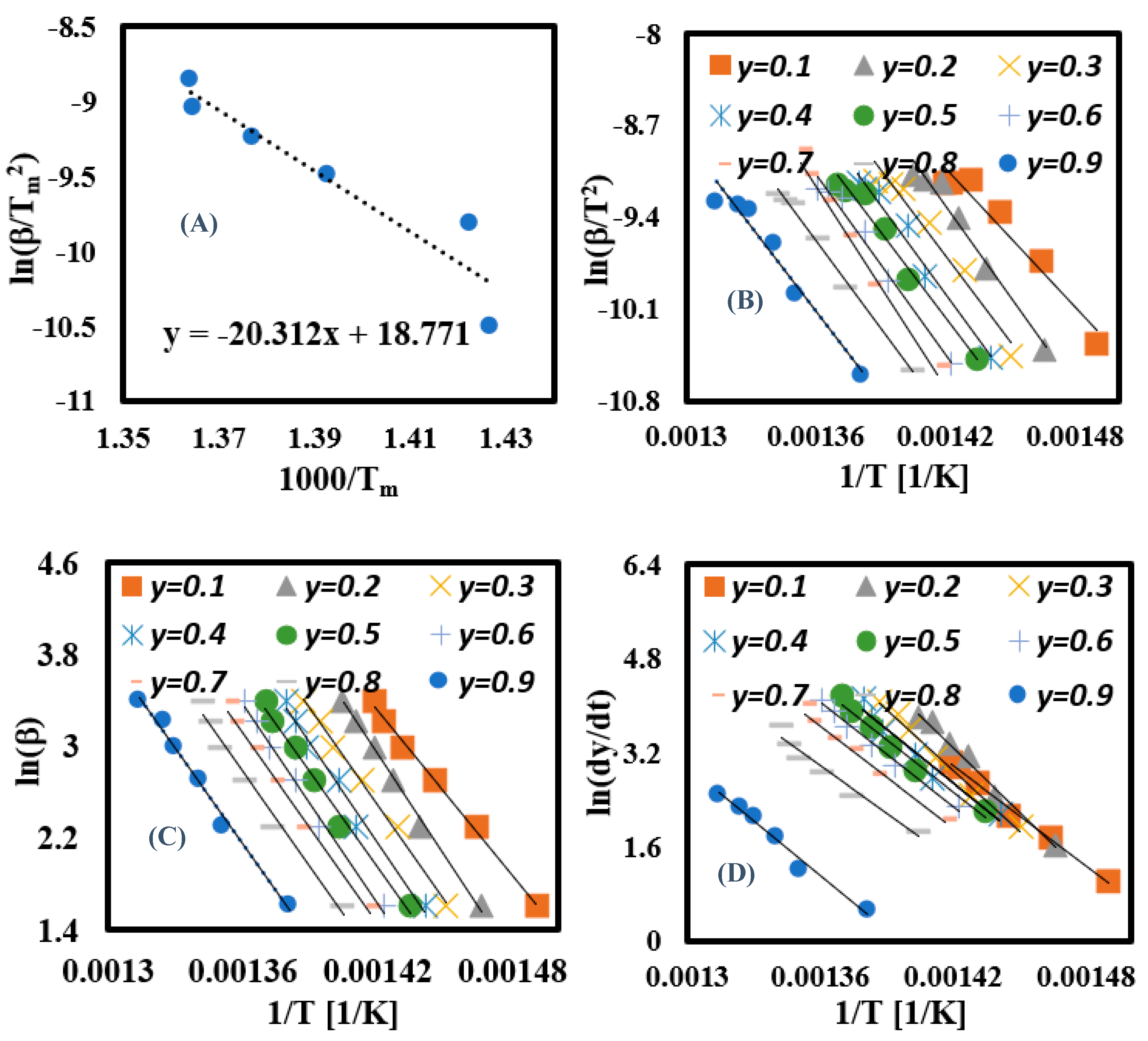
3.6.1. Activation Energy for the Entire Thermochemical Process
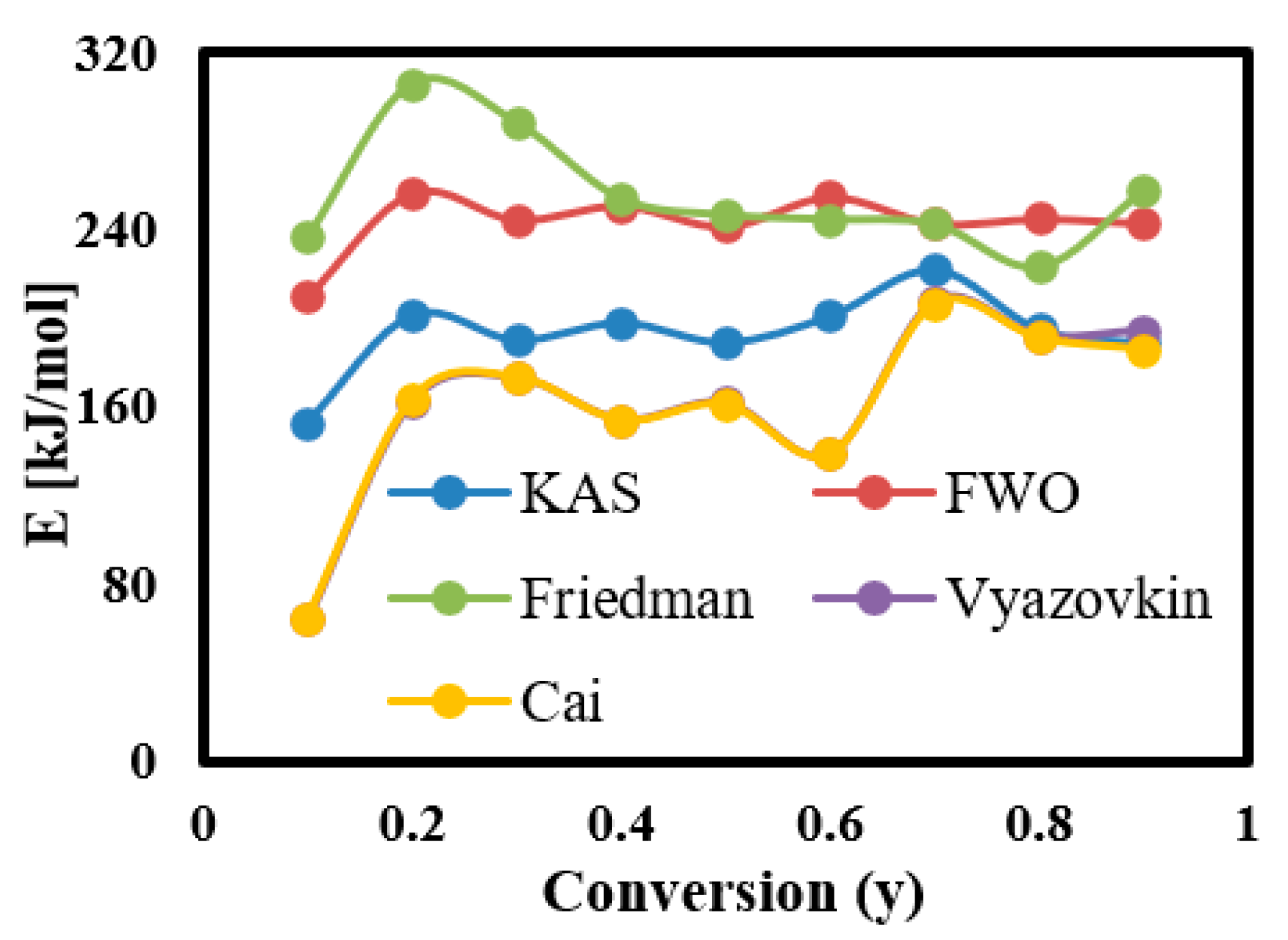
3.6.2. Calculation of Ea for Each Conversion Rate Using Linear Kinetic Methods
3.6.3. Calculation of Ea for Each Conversion Rate Using Nonlinear Kinetic Methods
3.6.4. Analysis of the Fitted TGA-DTG Curves Using DAEM and IPR
3.6.5. Thermodynamic Analysis
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wang, L.; Kahrizi, M.; Lu, P.; Wei, Y.; Yang, H.; Yu, Y.; Wang, L.; Li, Y.; Zhao, S. Enhancing water permeability and antifouling performance of thin–film composite membrane by tailoring the support layer. Desalination 2021, 516, 115193. [Google Scholar] [CrossRef]
- Yousef, S.; Subadra, S.P.; Tuckute, S.; Baltušnikas, A.; Lukošiūtė, S.-I.; Arafa, E.L.; Mohamed, A. Finite element analysis of fibreglass and carbon fabrics reinforced polyethersulfone membranes. Mater. Today Commun. 2022, 31, 103682. [Google Scholar] [CrossRef]
- Wang, S.; Li, T.; Chen, C.; Chen, S.; Liu, B.; Crittenden, J. Non-woven PET fabric reinforced and enhanced the performance of ultrafiltration membranes composed of PVDF blended with PVDF-g-PEGMA for industrial applications. Appl. Surf. Sci. 2018, 435, 1072–1079. [Google Scholar] [CrossRef]
- Ursino, C.; Ounifi, I.; Di Nicolò, E.; Cheng, X.; Shao, L.; Zhang, Y.; Drioli, E.; Criscuoli, A.; Figoli, A. Development of non-woven fabric-based ECTFE membranes for direct contact membrane distillation application. Desalination 2021, 500, 114879. [Google Scholar] [CrossRef]
- El-Badawy, T.; Othman, M.H.D.; Adam, M.R.; Ismail, A.; Rahman, M.A.; Jaafar, J.; Usman, J.; Mamah, S.C.; Raji, Y.O. The influence of pretreatment step on hollow braided PET fabric as a potential membrane substrate. Mater. Today Proc. 2020, 46, 1990–1997. [Google Scholar] [CrossRef]
- Wu, H.; Zhao, H.; Lin, Y.; Liu, X.; Yao, H.; Yu, L.; Wang, H.; Wang, X. Fabrication of polysulfone membrane with sponge-like structure by using different non-woven fabrics. Sep. Purif. Technol. 2022, 297, 121553. [Google Scholar] [CrossRef]
- Xia, L.; Zhang, Q.; Zhuang, X.; Zhang, S.; Duan, C.; Wang, X.; Cheng, B. Hot-Pressed Wet-Laid Polyethylene Terephthalate Nonwoven as Support for Separation Membranes. Polymers 2019, 11, 1547. [Google Scholar] [CrossRef]
- Kamakshi; Kumar, R.; Saraswat, V.K.; Kumar, M.; Awasthi, K.; Stamm, M. Hydrogen gas separation with controlled selectivity via efficient and cost effective block copolymer coated PET membranes. Int. J. Hydrogen Energy 2017, 42, 19977–19983. [Google Scholar] [CrossRef]
- Xiong, Q.; Chen, H.; Tian, Q.; Yue, X.; Qiu, F.; Zhang, T.; Wang, A.-B. Waste PET derived Janus fibrous membrane for efficient oil/water emulsions separation. J. Environ. Chem. Eng. 2022, 10, 108459. [Google Scholar] [CrossRef]
- Yi, K.; Huang, J.; Li, X.; Li, S.; Pang, H.; Liu, Z.; Zhang, W.; Liu, S.; Liu, C.; Shu, W. Long-term impacts of polyethylene terephthalate (PET) microplastics in membrane bioreactor. J. Environ. Manag. 2022, 323, 116234. [Google Scholar] [CrossRef]
- Jin, X.; Wang, H.; Jin, X.; Wang, H.; Chen, L.; Wang, W.; Lin, T.; Zhu, Z. Preparation of keratin/PET nanofiber membrane and its high adsorption performance of Cr(VI). Sci. Total. Environ. 2020, 710, 135546. [Google Scholar] [CrossRef] [PubMed]
- Petit, C.; Delouya, G.; Taussky, D.; Barkati, M.; Lambert, C.; Beauchemin, M.-C.; Clavel, S.; Mok, G.; Paré, A.-S.G.; Nguyen, T.-V.; et al. PSMA-PET/CT-Guided Intensification of Radiation Therapy for Prostate Cancer (PSMAgRT): Findings of Detection Rate, Effect on Cancer Management, and Early Toxicity from a Phase 2 Randomized Controlled Trial. Int. J. Radiat. Oncol. 2023, 116, 779–787. [Google Scholar] [CrossRef] [PubMed]
- Qureshi, M.S.; Oasmaa, A.; Pihkola, H.; Deviatkin, I.; Tenhunen, A.; Mannila, J.; Minkkinen, H.; Pohjakallio, M.; Laine-Ylijoki, J. Pyrolysis of plastic waste: Opportunities and challenges. J. Anal. Appl. Pyrolysis 2020, 152, 104804. [Google Scholar] [CrossRef]
- Huysveld, S.; Ragaert, K.; Demets, R.; Nhu, T.; Civancik-Uslu, D.; Kusenberg, M.; Van Geem, K.; De Meester, S.; Dewulf, J. Technical and market substitutability of recycled materials: Calculating the environmental benefits of mechanical and chemical recycling of plastic packaging waste. Waste Manag. 2022, 152, 69–79. [Google Scholar] [CrossRef]
- Ragaert, K.; Delva, L.; Van Geem, K. Mechanical and chemical recycling of solid plastic waste. Waste Manag. 2017, 69, 24–58. [Google Scholar] [CrossRef] [PubMed]
- Chawla, S.; Varghese, B.S.; Chithra, A.; Hussain, C.G.; Keçili, R.; Hussain, C.M. Environmental impacts of post-consumer plastic wastes: Treatment technologies towards eco-sustainability and circular economy. Chemosphere 2022, 308, 135867. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Effect of aluminum leaching pretreatment on catalytic pyrolysis of metallised food packaging plastics and its linear and nonlinear kinetic behaviour. Sci. Total. Environ. 2022, 844, 157150. [Google Scholar] [CrossRef]
- Lin, S.; Ali, M.U.; Zheng, C.; Cai, Z.; Wong, M.H. Toxic chemicals from uncontrolled e-waste recycling: Exposure, body burden, health impact. J. Hazard. Mater. 2022, 426, 127792. [Google Scholar] [CrossRef]
- Kim, N.-K.; Lee, S.-H.; Park, H.-D. Current biotechnologies on depolymerization of polyethylene terephthalate (PET) and repolymerization of reclaimed monomers from PET for bio-upcycling: A critical review. Bioresour. Technol. 2022, 363, 127931. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Zakarauskas, K.; Striūgas, N. A new sustainable strategy for oil, CH4 and aluminum recovery from metallised food packaging plastics waste using catalytic pyrolysis over ZSM-5 zeolite catalyst. Thermochim. Acta 2022, 713, 179223. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Zakarauskas, K.; Jančauskas, A.; Striūgas, N. An eco-friendly strategy for recovery of H2-CH4-rich syngas, benzene-rich tar and carbon nanoparticles from surgical mask waste using an updraft gasifier system. Energy Sources Part A Recover. Util. Environ. Eff. 2023, 45, 5063–5080. [Google Scholar] [CrossRef]
- Guan, M.; Zhao, S.; Shi, J.; Jin, H.; Wei, W. Experimental study on the gasification characteristics of polyethylene terephthalate (PET) microplastics in supercritical H2O/CO2 environment. J. Clean. Prod. 2023, 385, 135661. [Google Scholar] [CrossRef]
- Choi, M.-J.; Jeong, Y.-S.; Kim, J.-S. Air gasification of polyethylene terephthalate using a two-stage gasifier with active carbon for the production of H2 and CO. Energy 2021, 223, 120122. [Google Scholar] [CrossRef]
- Atashi, F.; Gholizadeh, M.; Ataei, F. Pyrolysis analysis of polyethylene terephthalate: Effects of carrier gases (N2, He, and Ar) and zeolite catalyst (A4) on yield. J. Chem. Technol. Biotechnol. 2022, 97, 3395–3405. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Mohamed, A.; Abdelnaby, M.A. Pyrolysis kinetic behavior and TG-FTIR-GC–MS analysis of end-life ultrafiltration polymer nanocomposite membranes. Chem. Eng. J. 2022, 428, 131181. [Google Scholar] [CrossRef]
- Dhahak, A.; Hild, G.; Rouaud, M.; Mauviel, G.; Burkle-Vitzthum, V. Slow pyrolysis of polyethylene terephthalate: Online monitoring of gas production and quantitative analysis of waxy products. J. Anal. Appl. Pyrolysis 2019, 142, 104664. [Google Scholar] [CrossRef]
- Straka, P.; Bičáková, O.; Šupová, M. Slow pyrolysis of waste polyethylene terephthalate yielding paraldehyde, ethylene glycol, benzoic acid and clean fuel. Polym. Degrad. Stab. 2022, 198, 109900. [Google Scholar] [CrossRef]
- Liu, Y.; Fu, W.; Liu, T.; Zhang, Y.; Li, B. Microwave pyrolysis of polyethylene terephthalate (PET) plastic bottle sheets for energy recovery. J. Anal. Appl. Pyrolysis 2022, 161, 105414. [Google Scholar] [CrossRef]
- Niksiar, A.; Faramarzi, A.H.; Sohrabi, M. Kinetic study of polyethylene terephthalate (PET) pyrolysis in a spouted bed reactor. J. Anal. Appl. Pyrolysis 2015, 113, 419–425. [Google Scholar] [CrossRef]
- Kaban, A.P.S.; Rahmat, N.G.; Fatriansyah, J.F. Kinetics of catalytic pyrolysis of polyethylene terephthalate (PET) plastic polymer with zeolite. AIP Conf. Proc. 2020, 2262, 050007. [Google Scholar] [CrossRef]
- Al-Salem, S.M.; Lettieri, P. Kinetics of polyethylene terephthalate (PET) and polystyrene (PS) dynamic pyrolysis. Int. J. Chem. Mol. Eng. 2010, 4, 402–410. [Google Scholar]
- Brems, A.; Baeyens, J.; Beerlandt, J.; Dewil, R. Thermogravimetric pyrolysis of waste polyethylene-terephthalate and polystyrene: A critical assessment of kinetics modelling. Resour. Conserv. Recycl. 2011, 55, 772–781. [Google Scholar] [CrossRef]
- Dubdub, I.; Alhulaybi, Z. Catalytic Pyrolysis of PET Polymer Using Nonisothermal Thermogravimetric Analysis Data: Kinetics and Artificial Neural Networks Studies. Polymers 2022, 15, 70. [Google Scholar] [CrossRef]
- Ramamoorthy, M.; Pisal, A.A.; Rengasamy, R.S.; Rao, A.V. In-situ synthesis of silica aerogel in polyethylene terephthalate fibre nonwovens and their composite properties on acoustical absorption behavior. J. Porous Mater. 2018, 25, 179–187. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Praspaliauskas, M.; Abdelnaby, M.A. Pyrolysis Kinetic Behaviour of Glass Fibre-Reinforced Epoxy Resin Composites Using Linear and Nonlinear Isoconversional Methods. Polymers 2021, 13, 1543. [Google Scholar] [CrossRef]
- Yousef, S.; Tonkonogovas, A.; Mohamed, A. Performance of sponge and finger-like structures of PSF/PET membranes in hydrogen separation industry. J. Ind. Eng. Chem. 2023, 125, 232–241. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Zakarauskas, K.; Striūgas, N. Recovery of styrene-rich oil and glass fibres from fibres-reinforced unsaturated polyester resin end-of-life wind turbine blades using pyrolysis technology. J. Anal. Appl. Pyrolysis 2023, 173, 106100. [Google Scholar] [CrossRef]
- Yousef, S.; Kiminaitė, I.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Recovery of phenol and acetic acid from glass fibre reinforced thermoplastic resin using catalytic pyrolysis process on ZSM-5 zeolite catalyst and its kinetic behaviour. Thermochim. Acta 2022, 715, 179293. [Google Scholar] [CrossRef]
- Yousef, S.; Kiminaitė, I.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Catalytic pyrolysis kinetic behaviour of glass fibre-reinforced epoxy resin composites over ZSM-5 zeolite catalyst. Fuel 2022, 315, 123235. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Praspaliauskas, M.; Abdelnaby, M.A. Pyrolysis kinetic behaviour, TG-FTIR, and GC/MS analysis of cigarette butts and their components. Biomass Convers. Biorefinery 2022. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Pyrolysis kinetic behaviour and TG-FTIR-GC–MS analysis of Coronavirus Face Masks. J. Anal. Appl. Pyrolysis 2021, 156, 105118. [Google Scholar] [CrossRef] [PubMed]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Thermal decomposition of CNTs and graphene-reinforced glass fibers/epoxy and their kinetics. Biomass Convers. Biorefinery 2022. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Influence of carbon black filler on pyrolysis kinetic behaviour and TG-FTIR-GC–MS analysis of glass fibre reinforced polymer composites. Energy 2021, 233, 121167. [Google Scholar] [CrossRef]
- Yousef, S.; Eimontas, J.; Striūgas, N.; Abdelnaby, M.A. Pyrolysis kinetic behaviour and thermodynamic analysis of waste wind turbine blades (carbon fibres/unsaturated polyester resin). Energy Sources Part A Recover. Util. Environ. Eff. 2023, 45, 10505–10522. [Google Scholar] [CrossRef]
- Duan, Y.; Duan, L.; Wang, J.; Anthony, E.J. Observation of simultaneously low CO, NOx and SO2 emission during oxy-coal combustion in a pressurized fluidized bed. Fuel 2019, 242, 374–381. [Google Scholar] [CrossRef]
- Huang, X.; Yin, H.; Zhang, H.; Mei, N.; Mu, L. Pyrolysis characteristics, gas products, volatiles, and thermo–kinetics of industrial lignin via TG/DTG–FTIR/MS and in–situ Py–PI–TOF/MS. Energy 2022, 259, 125062. [Google Scholar] [CrossRef]
- Saha, B.; Ghoshal, A. Thermal degradation kinetics of poly(ethylene terephthalate) from waste soft drinks bottles. Chem. Eng. J. 2005, 111, 39–43. [Google Scholar] [CrossRef]
- Martín-Gullón, I.; Esperanza, M.; Font, R. Kinetic model for the pyrolysis and combustion of poly-(ethylene terephthalate) (PET). J. Anal. Appl. Pyrolysis 2001, 58–59, 635–650. [Google Scholar] [CrossRef]
- Alongi, J.; Camino, G.; Malucelli, G. Heating rate effect on char yield from cotton, poly(ethylene terephthalate) and blend fabrics. Carbohydr. Polym. 2013, 92, 1327–1334. [Google Scholar] [CrossRef]
- Huang, J.; Meng, H.; Luo, X.; Mu, X.; Xu, W.; Jin, L.; Lai, B. Insights into the thermal degradation mechanisms of polyethylene terephthalate dimer using DFT method. Chemosphere 2022, 291, 133112. [Google Scholar] [CrossRef]
- Nasution, F.; Husin, H.; Mahidin; Abnisa, F.; Yani, F.T.; Maulinda, L.; Ahmadi. Conversion of pyrolysis vapors derived from non-biodegradable waste plastics (PET) into valuable fuels using nickel-impregnated HZSM5-70 catalysts. Energy Convers. Manag. 2022, 273, 116440. [Google Scholar] [CrossRef]
- Feng, B.; Zhao, C.; Chen, B.; Zhuo, W.; Liu, Y.; Wang, L.; He, L.; Tang, F. Application of synthetic benzoic acid technology in environmental radiocarbon monitoring. J. Environ. Radioact. 2020, 216, 106188. [Google Scholar] [CrossRef] [PubMed]
- Hernández-Fuentes, C.; Ruiz-Guerrero, R.; Morales-Ramírez, A.d.J.; Molina-Maldonado, P.; Medina-Velazquez, D.Y. New Mononuclear Complex of Europium(III) and Benzoic Acid: From Synthesis and Crystal Structure Solution to Luminescence Emission. Crystals 2020, 10, 674. [Google Scholar] [CrossRef]
- Volli, V.; Varma, R.; Pradhan, D.; Panda, A.K.; Singh, R.K.; Shu, C.-M. Thermal degradation behaviour, kinetics, and thermodynamics of Bombax Malabarica seeds through TG-FTIR and Py-GC/MS analysis. Sustain. Energy Technol. Assess. 2023, 57, 103150. [Google Scholar] [CrossRef]
- Jia, W.; Wang, X.; Shi, L. Endogenous benzoic acid interferes with the signatures of amino acids and thiol compounds through perturbing N-methyltransferase, glutamate-cysteine ligase, and glutathione S-transferase activity in dairy products. Food Res. Int. 2022, 161, 111857. [Google Scholar] [CrossRef]
- Park, S.-Y.; Yoo, M.-Y.; Paik, H.-D.; Lim, S.-D. Production of benzoic acid as a natural compound in fermented skim milk using commercial cheese starter. J. Dairy Sci. 2017, 100, 4269–4275. [Google Scholar] [CrossRef]
- Diaz-Silvarrey, L.S.; McMahon, A.; Phan, A.N. Benzoic acid recovery via waste poly(ethylene terephthalate) (PET) catalytic pyrolysis using sulphated zirconia catalyst. J. Anal. Appl. Pyrolysis 2018, 134, 621–631. [Google Scholar] [CrossRef]
- Osman, A.I.; Farrell, C.; Al-Muhtaseb, A.H.; Al-Fatesh, A.S.; Harrison, J.; Rooney, D.W. Pyrolysis kinetic modelling of abundant plastic waste (PET) and in-situ emission monitoring. Environ. Sci. Eur. 2020, 32, 112. [Google Scholar] [CrossRef]
- Vyazovkin, S. Modern Isoconversional Kinetics: From Misconceptions to Advances. In Handbook of Thermal Analysis and Calorimetry; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar] [CrossRef]
- Cai, J.; Chen, S. A new iterative linear integral isoconversional method for the determination of the activation energy varying with the conversion degree. J. Comput. Chem. 2009, 30, 1986–1991. [Google Scholar] [CrossRef]
- Gajera, B.; Tyagi, U.; Sarma, A.K.; Jha, M.K. Pyrolysis of cattle manure: Kinetics and thermodynamic analysis using TGA and artificial neural network. Biomass Convers. Biorefinery 2023. [Google Scholar] [CrossRef]






| Equation No. | Method | Expressions |
|---|---|---|
| (3) | Kissinger | = − |
| (4) | KAS | = − |
| (5) | FWO | = − 5.335 − |
| (6) | Friedman | = |
| (7) | Vyazovkin | |
| (8) | Cai | |
| (9) | DAEM | = − |
| (10) | IPR | |
| (11) | Dev.% | (%) = |
| (12) | ΔH | ΔH = Ea RTm |
| (13) | ΔG | ΔG = Ea + RTm |
| (14) | ΔS | ΔS = |
| Elemental Analysis (wt.%) | Proximate Analysis (wt.%) | ||
|---|---|---|---|
| Nitrogen (N) | <0.01 | Moisture | 0.27 ± 0.04 |
| Carbon (C) | 62.318 ± 0.035 | Volatiles | 79.92 ± 0.28 |
| Hydrogen (H) | 4.221 ± 0.024 | Fixed Carbon | 17.89 ± 0.12 |
| Sulfur (S) | <0.01 | Ash | 1.92 ± 0.01 |
| Oxygen (O) | 33.46 ± 0.021 | ||
| Pyrolysis Parameters | 5 °C/min | 10 °C/min | 15 °C/min | 20 °C/min | 25 °C/min | 30 °C/min |
|---|---|---|---|---|---|---|
| Ti (°C) | 362 | 367 | 375 | 385 | 394 | 396 |
| Tm (°C) | 429 | 445 | 451 | 453 | 456 | 460 |
| Tf (°C) | 484 | 498 | 510 | 516 | 519 | 521 |
| Rmax (%/min) | 10 | 19.4 | 28.9 | 39.2 | 50 | 65.9 |
| Di (% min−1 °C−3) | 1.95 × 10−6 | 3.05 × 10−6 | 4.27 × 10−6 | 5.35 × 10−6 | 6.63 × 10−6 | 8.82 × 10−6 |
| 33 | 39 | 40 | 42 | 42 | 41 | |
| T5 | 393.3 | 413.2 | 404.9 | 416.5 | 423.7 | 424.7 |
| T30 | 420.9 | 436.6 | 433.5 | 444.1 | 446.7 | 450.5 |
| THRI | 200.8 | 209.3 | 206.8 | 212.2 | 214.4 | 215.7 |
| 5 °C/min | 10 °C/min | 15 °C/min | 20 °C/min | 25 °C/min | 30 °C/min | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Time (min.) | GC Compounds | Area (%) | Time (min.) | GC Compounds | Area (%) | Time (min.) | GC Compounds | Area (%) | Time (min.) | GC Compounds | Area (%) | Time (min.) | GC Compounds | Area (%) | Time (min.) | GC Compounds | Area (%) |
| 1.181 | Carbon dioxide | 2.12 | 1.187 | Carbon dioxide | 2.17 | 1.187 | Carbon dioxide | 2.59 | 1.181 | Carbon dioxide | 1.90 | 1.194 | Carbon dioxide | 3.21 | 1.187 | Carbon dioxide | 3.66 |
| 1.239 | Acetaldehyde | 7.40 | 1.245 | Acetaldehyde | 8.46 | 1.239 | Acetaldehyde | 9.46 | 1.239 | Acetaldehyde | 6.07 | 1.246 | Acetaldehyde | 9.89 | 1.239 | Acetaldehyde | 9.60 |
| 12.177 | Benzoic acid | 74.90 | 11.628 | N-[5-(m-Tolyl)-1,3,4-thiadiazol-2-1326yl]benzimidic acid | 1.02 | 2.158 | Benzene | 0.67 | 11.531 | Vinyl benzoate | 2.70 | 2.164 | Benzene | 0.81 | 2.151 | Benzene | 0.85 |
| 15.030 | 2-n-Propoxyamphetamine | 1.32 | 12.190 | Benzoic acid | 71.82 | 11.602 | Benzenebutanoic acid, .gamma.-oxo- | 1.84 | 12.197 | Benzoic acid | 60.60 | 11.602 | Vinyl benzoate | 4.40 | 11.556 | Vinyl benzoate | 5.63 |
| 17.514 | 2,4-Dimethylamphetamine | 0.60 | 14.965 | 2-n-Propoxyamphetamine | 0.98 | 12.203 | Benzoic acid | 73.40 | 14.894 | 4-Ethylbenzoic acid | 2.28 | 12.223 | Benzoic acid | 68.88 | 12.216 | Benzoic acid | 62.53 |
| 19.176 | Acetic acid, 2-(1-methyl-2-oxohydrazino)-, N′-[(E)-(2-hydroxyphenyl) methylidene]hydrazide, N-oxide | 0.39 | 17.488 | (3,4,5,6-Tetrahydro-2H-[2,3′]bipyridinyl-1-yl)acetic acid hydrazide | 1.79 | 14.965 | 4-Ethylbenzoic acid | 1.26 | 17.424 | 4-Methyl-2,6-dihydroxyquinoline | 1.66 | 14.959 | 4-Ethylbenzoic acid | 1.60 | 17.449 | 2-t-Butyl-1-methyl-3-phenyl-imidazolidin-4-one | 4.18 |
| 21.046 | 2-Fluorenamine | 0.60 | 18.523 | Phthalic acid, 2-hexyl ester | 0.93 | 17.482 | 2-t-Butyl-1-methyl-3-phenyl-imidazolidin-4-one | 1.90 | 21.150 | Octadecanal | 3.72 | 17.456 | Hydantoin, 5-ethyl-5-phenyl-, (.+/-.)- | 3.99 | 19.028 | Cyclododecene, 1-methyl- | 1.93 |
| 22.527 | N-Dodecylmethylamine | 1.20 | 21.039 | 9-Methyl-9H-carbazole | 0.91 | 18.530 | Terephthalic monohydroxamic acid | 0.72 | 21.777 | Cyclononasiloxane, octadecamethyl- | 2.05 | 17.986 | Terephthalic monohydroxamic acid | 2.39 | 20.127 | 16-Octadecenal | 1.58 |
| 23.581 | Benzoic acid, 2-propenyl ester | 9.48 | 22.514 | L-Alanine-4-nitroanilide | 0.96 | 19.054 | Benzyl alcohol, .alpha.-(1-aminoethyl)-m-hydroxy-, (-)- | 0.42 | 22.152 | Oxirane, heptadecyl- | 2.45 | 23.556 | Benzamide, N-hydroxy-N-(2-hydroxy-3-phenoxypropyl)- | 2.81 | 20.237 | 1,1,1,5,7,7,7-Heptamethyl-3,3-bis(trimethylsiloxy)tetrasiloxane | 0.85 |
| 24.222 | Phenol, 2-[(N,N-dimethylamino)methyl]-3,5-dimethyl- | 0.40 | 23.569 | Benzoic acid, 3,3,5-trimethyl-6-oxo-2-phenyl-3,6-dihydro-2H-pyran-4-yl ester | 7.35 | 19.992 | Phosphinic fluoride, diphenyl- | 0.39 | 22.469 | 4,4′-(Hexafluoroisopropylidene)diphenol | 8.48 | 26.092 | Benzonitrile, m-phenethyl- | 1.29 | 21.169 | Hexadecanal | 1.67 |
| 26.085 | 2-Amino-1-(o-methoxyphenyl)propane | 1.60 | 26.091 | Azetidine, 1-benzyl-3,3-dimethyl-2-phenyl- | 2.38 | 21.046 | Dimethyl-(2-thioxo-[1,2,3]dioxaphosphinan-2-yl)-amine | 0.55 | 23.097 | Oleyl alcohol, methyl ether | 2.26 | 27.340 | Tetrasiloxane, decamethyl- | 0.74 | 22.159 | Hexadecanal | 0.97 |
| 27.340 | 1-Propene, 3-(2-cyclopentenyl)-2-methyl-1,1-diphenyl- | 1.23 | 22.527 | 1-Methyl-2-phenoxyethylamine | 0.77 | 23.194 | Cyclodecasiloxane, eicosamethyl- | 1.71 | 22.495 | 4,4′-(Hexafluoroisopropylidene)diphenol | 2.59 | ||||||
| 23.575 | Butanedioic acid, 2,3-bis(benzoyloxy)-, [S-(R*,R*)]- | 3.68 | 24.009 | Oxirane, hexadecyl- | 1.44 | 23.109 | Oxirane, heptadecyl- | 1.16 | |||||||||
| 26.091 | Benzonitrile, m-phenethyl- | 1.60 | 24.481 | Hexasiloxane, tetradecamethyl- | 1.64 | 23.543 | 1,2-Ethanediol, dibenzoate | 1.78 | |||||||||
| 27.340 | 1H-Indole, 5-methyl-2-phenyl- | 0.75 | 25.729 | Cyclononasiloxane, octadecamethyl- | 1.05 | 26.091 | Azetidine, 1-benzyl-3,3-dimethyl-2-phenyl- | 1.00 | |||||||||
| KAS | FWO | Friedman | Vyazovkin | Cai | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ea (KJ/mol) | R2 | A (1/s) | Ea (KJ/mol) | R2 | A (1/s) | Ea (KJ/mol) | R2 | A (1/s) | Ea (kJ/mol) | R2 | Ea (KJ/mol) | R2 | |
| 0.1 | 153.19 | 0.95 | 8 × 108 | 209.51 | 1.00 | 1.65 × 1018 | 237.09 | 0.99 | 7.63 × 1020 | 64.35 | 0.89 | 64.83 | 0.86 |
| 0.2 | 201.87 | 0.97 | 4 × 1011 | 256.76 | 1.00 | 6.03 × 1020 | 305.76 | 1.00 | 3.23 × 1025 | 162.31 | 0.98 | 163.88 | 0.98 |
| 0.3 | 190.31 | 0.96 | 2 × 1010 | 244.48 | 1.00 | 2.35 × 1019 | 288.20 | 0.99 | 4.81 × 1023 | 172.97 | 0.99 | 173.58 | 0.99 |
| 0.4 | 198.31 | 0.98 | 3 × 1010 | 250.98 | 0.99 | 2.72 × 1019 | 254.06 | 0.96 | 4.72 × 1021 | 154.16 | 0.97 | 153.98 | 0.97 |
| 0.5 | 189.29 | 0.98 | 3 × 109 | 241.40 | 0.99 | 3 × 1018 | 246.86 | 0.98 | 1.03 × 1020 | 161.89 | 0.97 | 161.32 | 0.97 |
| 0.6 | 201.33 | 0.98 | 1 × 1010 | 254.89 | 0.99 | 1.34 × 1019 | 244.86 | 0.99 | 3.98 × 1019 | 138.67 | 0.89 | 138.57 | 0.87 |
| 0.7 | 222.50 | 0.98 | 2 × 1011 | 242.81 | 0.99 | 1.08 × 1018 | 242.71 | 0.98 | 1.39 × 1019 | 207.41 | 0.92 | 206.92 | 0.91 |
| 0.8 | 195.03 | 1.00 | 2 × 109 | 244.97 | 0.98 | 8.94 × 1017 | 223.43 | 0.95 | 2.27 × 1017 | 191.77 | 0.82 | 191.29 | 0.81 |
| 0.9 | 188.80 | 0.96 | 3 × 108 | 242.84 | 0.99 | 2.7 × 1017 | 257.90 | 0.98 | 8.42 × 1018 | 194.42 | 0.80 | 186.18 | 0.81 |
| Avg. | 193.40 | 0.97 | 8 × 1010 | 243.18 | 0.99 | 7.49 × 1019 | 255.65 | 0.98 | 3.64 × 1024 | 160.88 | 0.91 | 160.06 | 0.91 |
| y | The Activation Energy (kJ/mol) | The Activation Energy (kJ/mol) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Vyazovkin | Cai | ||||||||
| Initial Value | First Iteration | Second Iteration | Third Iteration | Fourth Iteration | First Iteration | Second Iteration | Third Iteration | Fourth Iteration | |
| 0.1 | 200 | 62.24 | 62.19 | 64.35 | 64.35 | 64.93 | 64.35 | 64.83 | 64.83 |
| 0.2 | 200 | 156.91 | 156.39 | 162.31 | 162.31 | 162.36 | 161.82 | 163.88 | 163.88 |
| 0.3 | 200 | 166.59 | 166.70 | 172.97 | 172.97 | 172.37 | 172.48 | 173.58 | 173.58 |
| 0.4 | 200 | 149.04 | 148.72 | 154.16 | 154.16 | 154.21 | 153.88 | 153.98 | 153.98 |
| 0.5 | 200 | 156.50 | 156.13 | 161.89 | 161.89 | 161.93 | 161.55 | 161.32 | 161.32 |
| 0.6 | 200 | 133.93 | 133.84 | 138.67 | 138.67 | 138.58 | 138.48 | 138.57 | 138.57 |
| 0.7 | 200 | 200.40 | 199.73 | 207.41 | 207.41 | 207.35 | 206.66 | 206.92 | 206.92 |
| 0.8 | 200 | 185.36 | 184.87 | 191.77 | 191.77 | 191.79 | 191.29 | 191.29 | 191.29 |
| 0.9 | 200 | 179.83 | 179.35 | 194.42 | 194.42 | 186.07 | 185.57 | 186.18 | 186.18 |
| Avg. | 200 | 154.53 | 154.21 | 160.88 | 160.88 | 159.95 | 159.56 | 160.06 | 160.06 |
| Model | DAEM | IPR |
|---|---|---|
| E1 | 243.15 | 222.17 |
| A1 | 4.20 × 1016 | 1.66 × 1012 |
| E2 | 301.51 | 284.76 |
| A2 | 4.62 × 1016 | 1.77 × 1013 |
| KAS | FWO | Friedman | |||||||
|---|---|---|---|---|---|---|---|---|---|
| ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | |
| 0.1 | 151 | 176 | 214.7 | 207 | 183 | 294.9 | 231 | 133 | 329.2 |
| 0.2 | 196 | 223 | 278.9 | 251 | 154 | 357.2 | 300 | 140 | 427.0 |
| 0.3 | 184 | 229 | 262.5 | 239 | 161 | 339.7 | 282 | 147 | 402.0 |
| 0.4 | 192 | 234 | 273.8 | 245 | 167 | 349.0 | 248 | 140 | 353.4 |
| 0.5 | 183 | 239 | 261.0 | 236 | 170 | 335.3 | 241 | 155 | 343.1 |
| 0.6 | 195 | 244 | 278.1 | 249 | 175 | 354.5 | 239 | 158 | 340.3 |
| 0.7 | 217 | 248 | 308.3 | 237 | 177 | 337.3 | 237 | 162 | 337.2 |
| 0.8 | 189 | 247 | 269.2 | 239 | 181 | 340.4 | 218 | 167 | 309.7 |
| 0.9 | 183 | 252 | 260.3 | 237 | 186 | 337.3 | 252 | 180 | 358.8 |
| Avg. | 188 | 232 | 267.4 | 238 | 173 | 338.4 | 250 | 154 | 355.6 |
| KAS | FWO | Friedman | |||||||
|---|---|---|---|---|---|---|---|---|---|
| ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | ΔH (kJ/mol) | ΔG (kJ/mol) | ΔS (J/mol K) | |
| 0.1 | 151 | 130 | 205.6 | 207 | 182 | 282.3 | 231 | 129 | 315.0 |
| 0.2 | 196 | 180 | 266.8 | 251 | 150 | 341.8 | 300 | 133 | 408.6 |
| 0.3 | 184 | 150 | 251.1 | 238 | 158 | 325.0 | 282 | 141 | 384.7 |
| 0.4 | 192 | 160 | 262.0 | 245 | 163 | 333.9 | 248 | 135 | 338.1 |
| 0.5 | 183 | 137 | 249.7 | 235 | 167 | 320.8 | 241 | 151 | 328.3 |
| 0.6 | 195 | 157 | 266.1 | 249 | 171 | 339.2 | 239 | 155 | 325.5 |
| 0.7 | 216 | 196 | 295.0 | 237 | 175 | 322.7 | 237 | 159 | 322.6 |
| 0.8 | 189 | 141 | 257.6 | 239 | 178 | 325.6 | 217 | 165 | 296.3 |
| 0.9 | 183 | 123 | 249.1 | 237 | 183 | 322.7 | 252 | 177 | 343.3 |
| Avg. | 188 | 153 | 255.9 | 238 | 170 | 323.8 | 250 | 149 | 340.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yousef, S.; Eimontas, J.; Striūgas, N.; Mohamed, A.; Ali Abdelnaby, M. Pyrolysis Kinetic Behavior and Thermodynamic Analysis of PET Nonwoven Fabric. Materials 2023, 16, 6079. https://doi.org/10.3390/ma16186079
Yousef S, Eimontas J, Striūgas N, Mohamed A, Ali Abdelnaby M. Pyrolysis Kinetic Behavior and Thermodynamic Analysis of PET Nonwoven Fabric. Materials. 2023; 16(18):6079. https://doi.org/10.3390/ma16186079
Chicago/Turabian StyleYousef, Samy, Justas Eimontas, Nerijus Striūgas, Alaa Mohamed, and Mohammed Ali Abdelnaby. 2023. "Pyrolysis Kinetic Behavior and Thermodynamic Analysis of PET Nonwoven Fabric" Materials 16, no. 18: 6079. https://doi.org/10.3390/ma16186079
APA StyleYousef, S., Eimontas, J., Striūgas, N., Mohamed, A., & Ali Abdelnaby, M. (2023). Pyrolysis Kinetic Behavior and Thermodynamic Analysis of PET Nonwoven Fabric. Materials, 16(18), 6079. https://doi.org/10.3390/ma16186079







