Anelastic Effects in Fe–Ga and Fe–Ga-Based Alloys: A Review
Abstract
:1. Introduction
- -
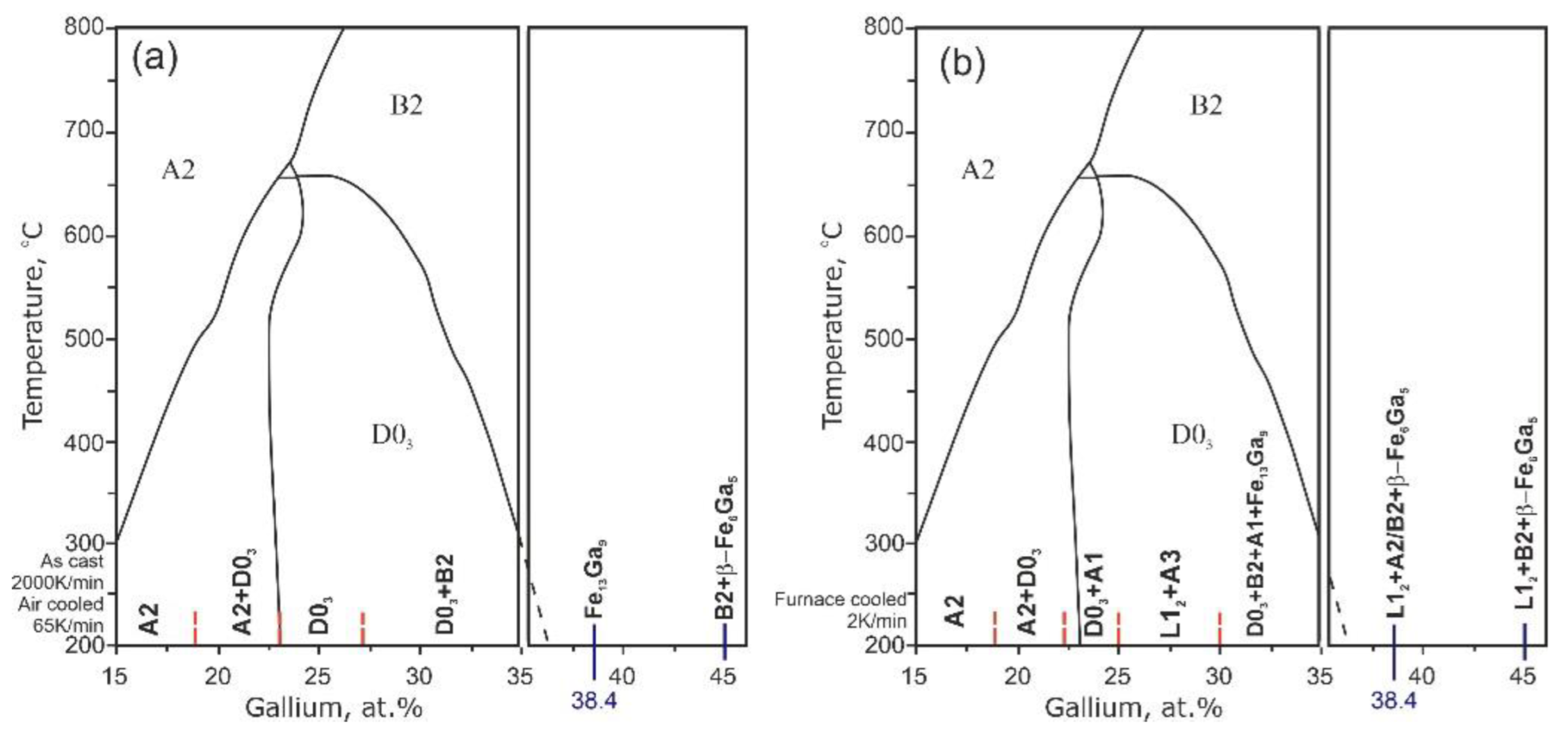
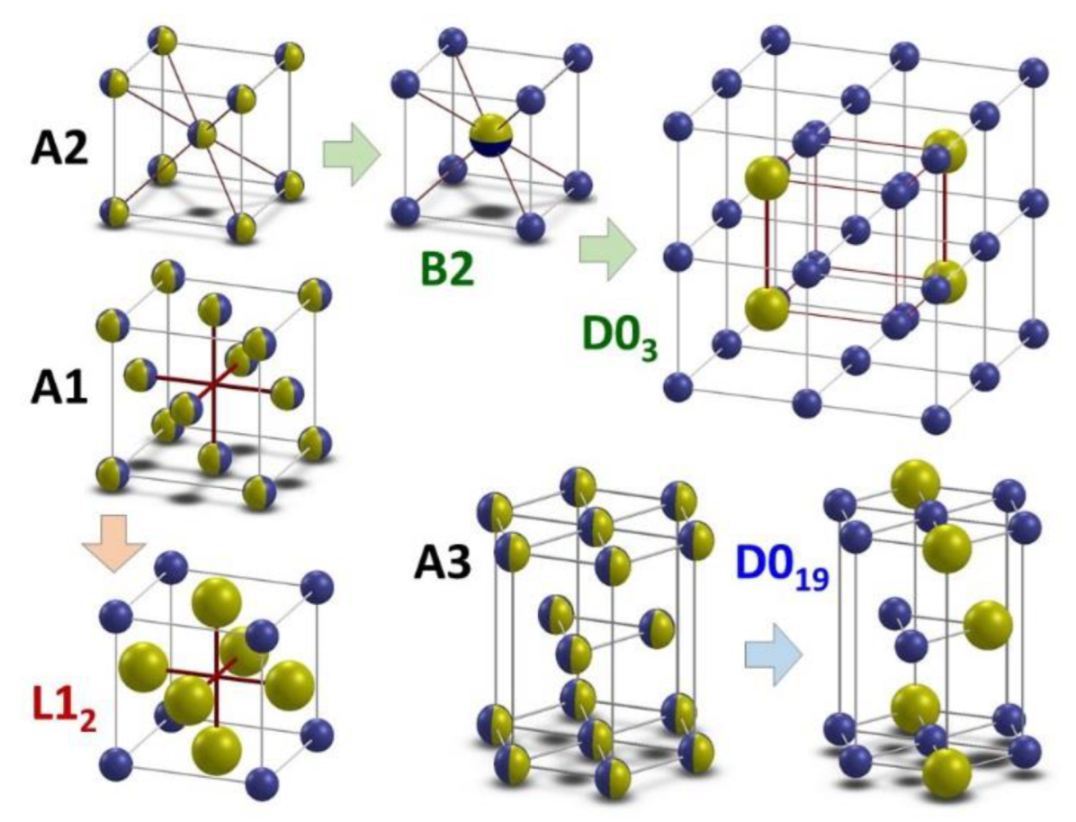
- The A1—has an γ-Fe-type structure with Fe and Ga (or Al) atoms randomly distributed, sp. gr. Fm3m (N225);
- -
- The A2—has an α-Fe-type structure with Fe and Ga (or Al) atoms randomly distributed, sp. gr. Im3m (N229);
- -
- The A3—has a Mg-type structure with randomly distributed Ga atoms, sp. gr. P63/mmc (N194);
- -
- The L12—has an Cu3Au-type structure with Fe and Ga atoms partially ordered, sp. gr. Fm3m (result of A1 phase ordering) (N226);
- -
- The B2—has a CsCl-type structure with Fe and Me atoms partially ordered, sp. gr. Pm3m (result of A2 phase ordering) (N221);
- -
- The D03—has a BiF3-type structure with Fe and Me atoms partially ordered, sp. gr. Fm3m (result of A2 phase ordering) (225);
- -
- The D019—has a MgCd3-type structure with Fe and Ga/Ge atoms partially ordered, sp. gr. P63/mmc (result of A3 phase ordering) (N194).
- -
- -
- In Fe–(26–27)Al alloys, the A2 → B2 → D03 transitions take place.

2. Fundamentals of Anelasticity
- (i)
- In frequency-dependent IF tests (FDIF), at a fixed temperature (τ is a constant in Equation (2) for a given fixed temperature), the frequency f is varied over a few orders of magnitude. This method allows direct measurements of Q−1 and E spectra vs. ω·τ, as introduced by Equation (2) and shown in Figure 4a [101]. The results can also be presented as a function of f (f = ω/2π, as τ is a constant for a chosen temperature of measurements).
- (ii)
- Most of the existing mechanical spectroscopy set-ups (e.g., vibrating reeds, torsion pendula) allow measurements of Q−1 as a function of temperature (T) but not frequency, i.e., to measure temperature-dependent internal friction (TDIF) (Figure 4b). The temperature dependence (e.g., for jumps of atoms) is typically described by the Arrhenius equation:
3. Anelastic Effects in Binary Fe–Ga and Ternary Fe–Ga-Based Alloys
3.1. Anelasticity of Fe–Ga Alloys with Ga < 24 at.%
- -
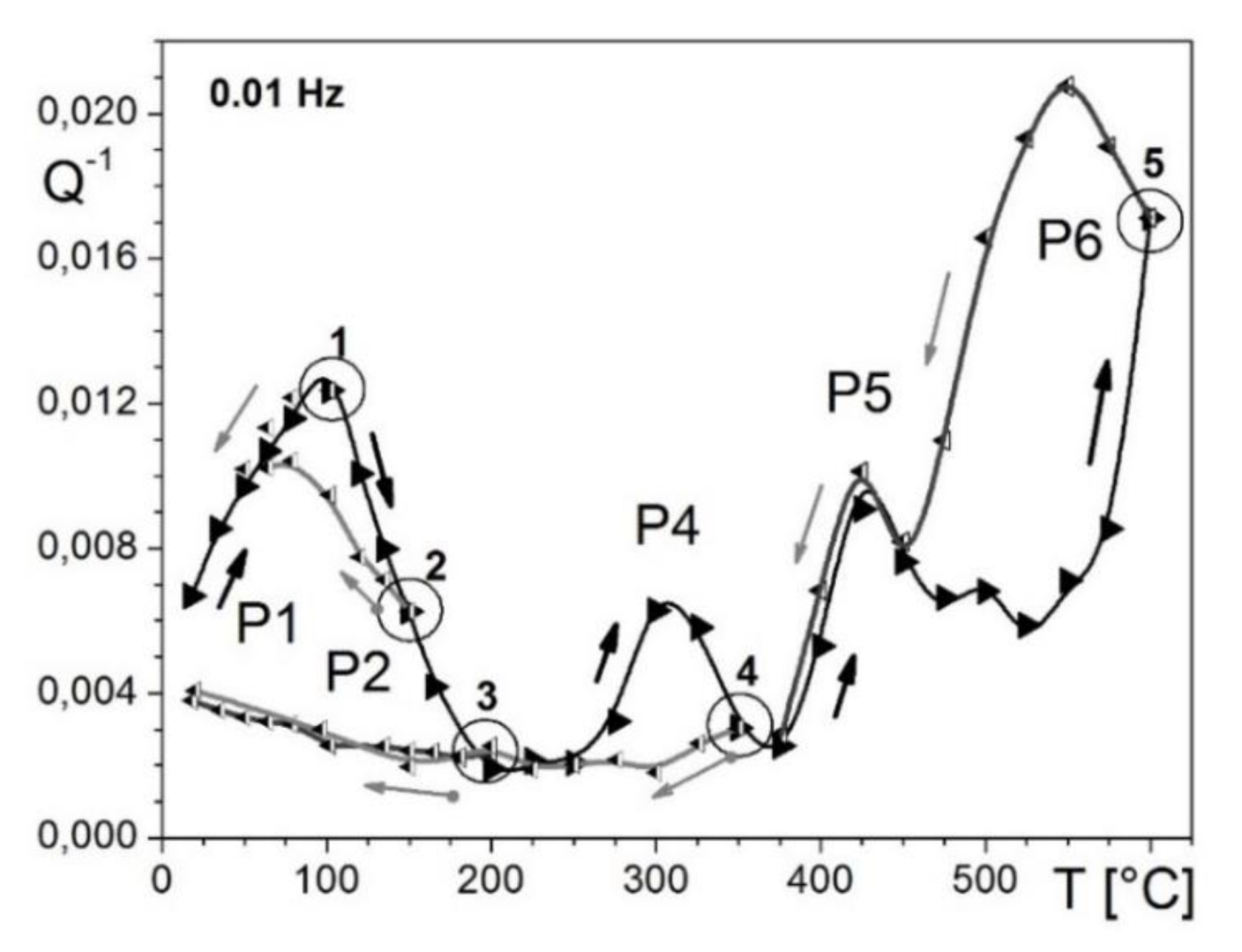
- A total of 2 broad low-temperature peaks (−80–20 °C, f ≈ 230 Hz and 2 kHz) with activation energy (H) of about 1 eV were tentatively explained as Hasiguti-type relaxations of dislocations with neighboring self-lattice defects. At the same time, authors would not exclude that these effects may correspond to the β and γ relaxations in bcc structures, i.e., kink pair formation in a0{111}/2 screw dislocations on {110} and {112} slip planes (these peaks were recorded using TDIF tests, and, therefore, they are not shown in Figure 6). Similar peaks were also recorded in Fe–Al alloys [70];
- -
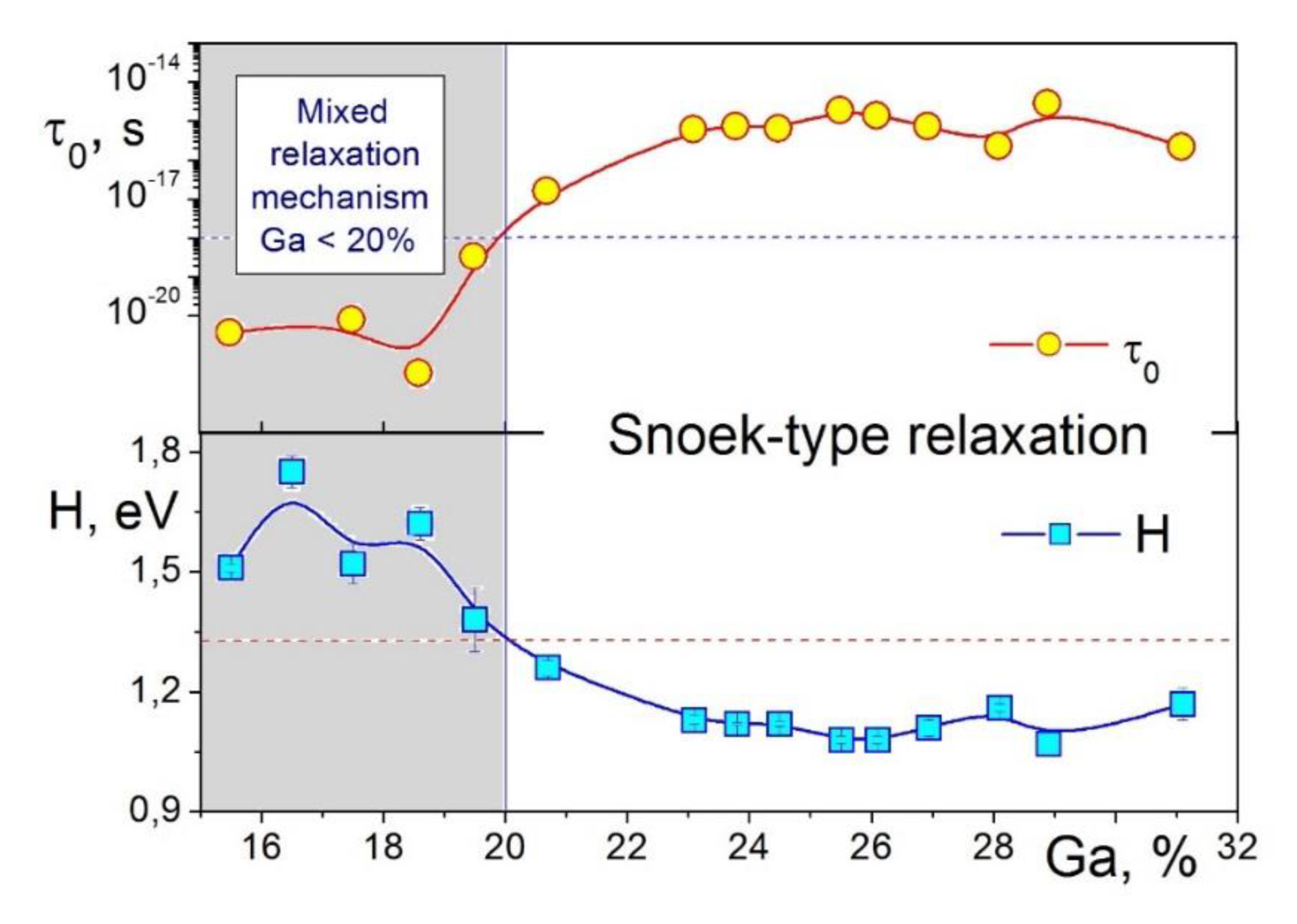
- Double-headed peaks (P1 and P2) in the temperature range between 50 and 200 °C (dependent on measuring frequency). The peak’s activation energy was estimated from Arrhenius plot as HP1 = 0.92–1.08 eV and HP2 = 1.0–1.14 eV and characteristic relaxation time (τ0) between 10−15 and 10−16 s (different setups, TDIF tests). These values obtained from FDIF are similar but not the same: H1 = 1.1 eV, τ01 = 10−16 s (P1 effect) and H2 = 1.04 eV, τ02 = 6 × 10−14 s (P2 effect). A similar peak with the activation parameters H1 ≈ 0.87 eV and τ0 ≈ 10−16 s is recorded in Fe–3Ga alloy. These peaks were attributed to the Snoek type relaxation, i.e., stress-induced carbon atom (C) jumps in Fe-C-Fe and Fe(6-n)-C-Ga(n) positions (here, n is number of Ga atoms in the first coordinate shell of C atom). Similar peaks were also recorded and well resolved in Fe–Al and Fe–Al-Si alloys [80,81];
- -
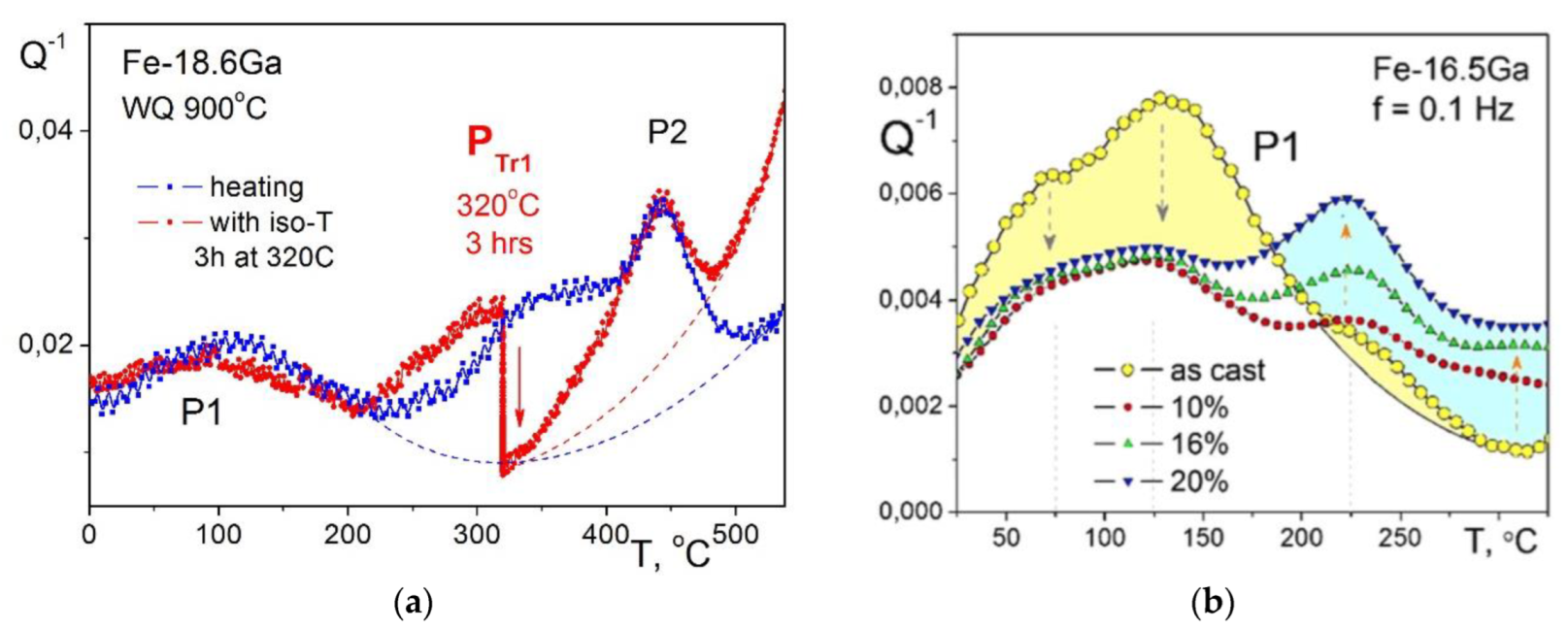
- The P3 effect above 200 °C was observed only in TDIF tests but never in FDIF tests. This fact underlines that this P3 peak is the transient effect which takes place only at heating due to a structural transition. Similar peaks are denoted below as PTr. It was proposed that the nature of this P3 (or PTr1) peak is due to annihilation of thermal vacancies and ordering (the P3 is not shown in Figure 6, where only results at fixed temperatures are used). Later effects of annihilation of thermal vacancies and ordering were confirmed by positron annihilation and neutron diffraction methods [22,36,59];
- -
- The P4 peak recorded by FDIF tests, similar to the P3 peak at TDIF curves, is unstable with respect to heating and disappears after annealing at 350 °C. The nature of this peak was not explained. We cannot exclude that the P3 and P4 peaks have similar natures, both of them disappearing after heating. Nevertheless, the P4 peak, in contrast with the P3 peak, is a thermally activated effect: its position at FDIF curves depends on measuring temperature in agreement with the ω × τ = 1 rule (see Equation (2a));
- -
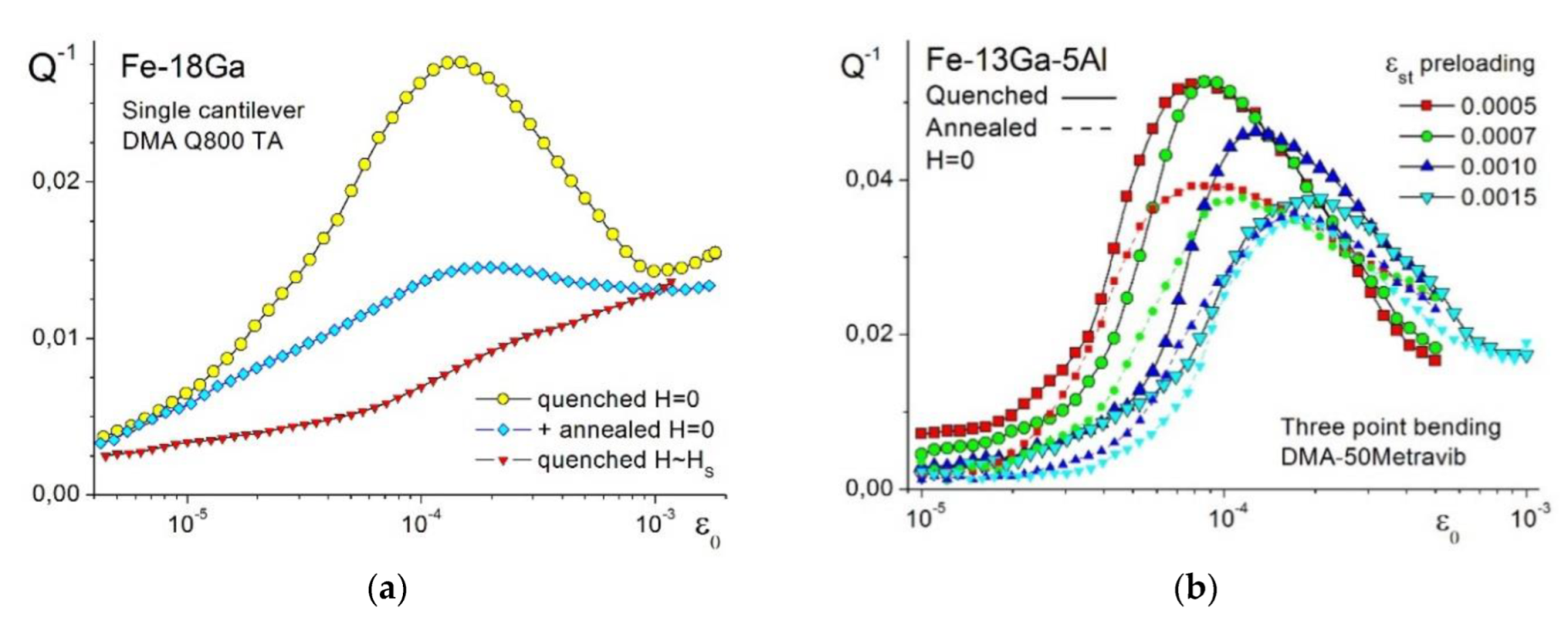
- The thermally activated P5 peak at about 450 °C (Figure 6) is stable with respect to heating. Activation parameters of the peak are: τ05 ≈ 10−17 s and H5 = 2.5 eV. According to its activation energy and influence of quenching this peak corresponds to Zener relaxation;
- -
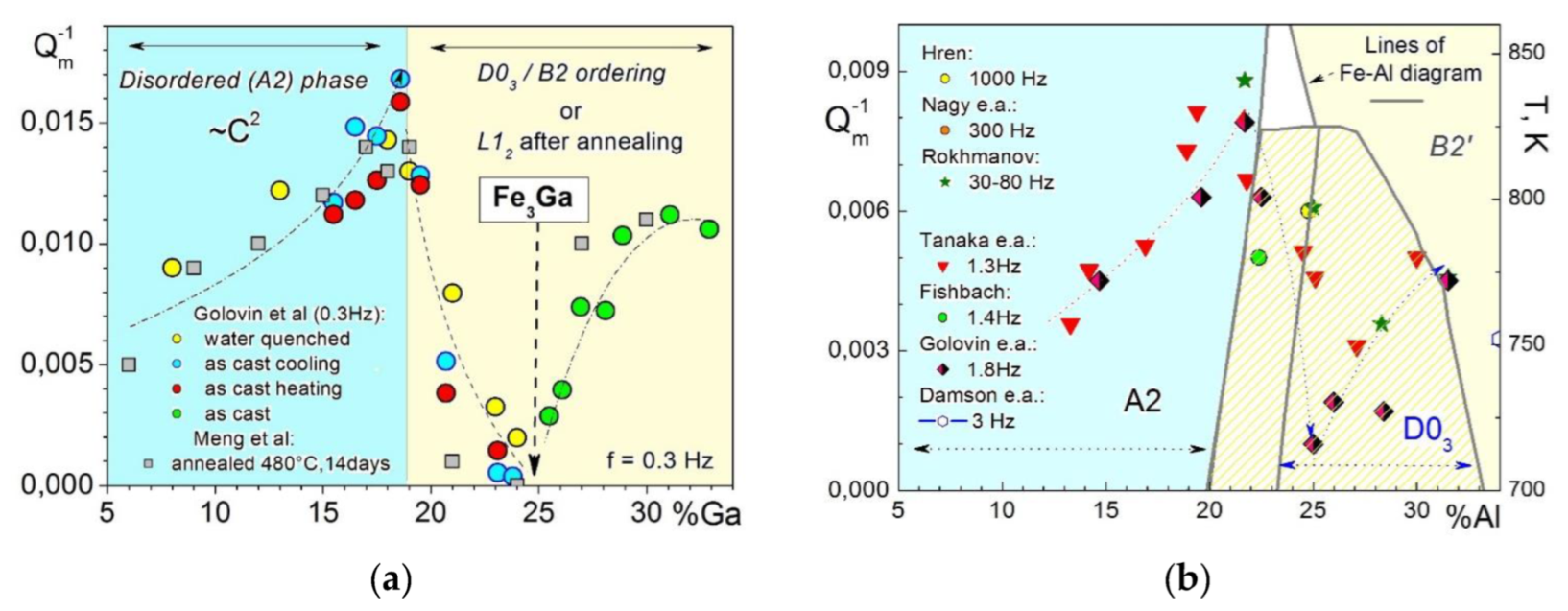
- The P6 peak appears after annealing at about 500 °C and increases strongly with the annealing temperature. Activation parameters (τ06 ≈ 2 × 10−16 s and H6 = 2.7 eV) suggest a grain boundary mechanism of this peak. Later, a similar peak was reported for Fe–27Ga (2.8 eV) [46,47], and, most recently, the interpretation of this effect as a grain boundary peak was confirmed for Fe–30Ga alloy with activation parameters: τ0 ≈ 10−12 s and H ≈ 2.1 eV [61].
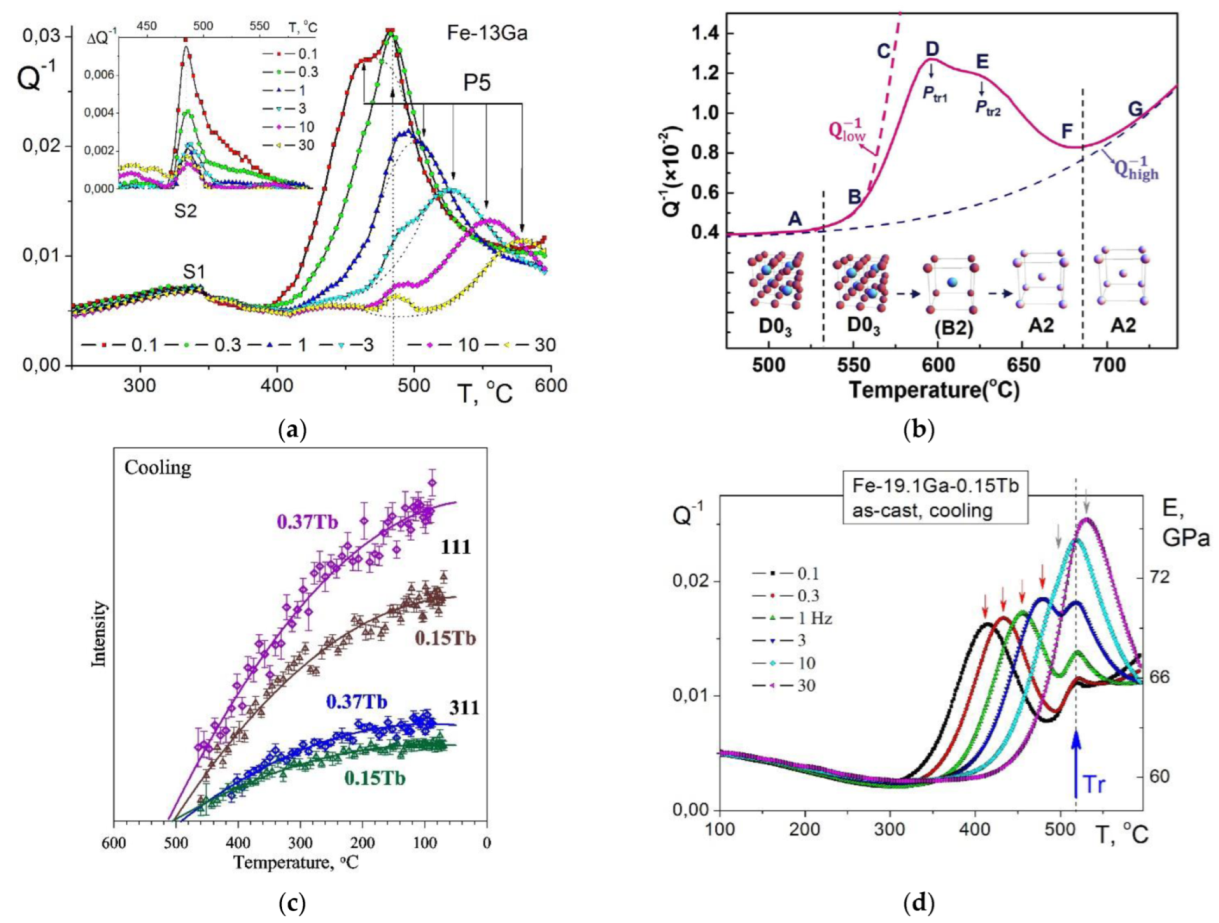
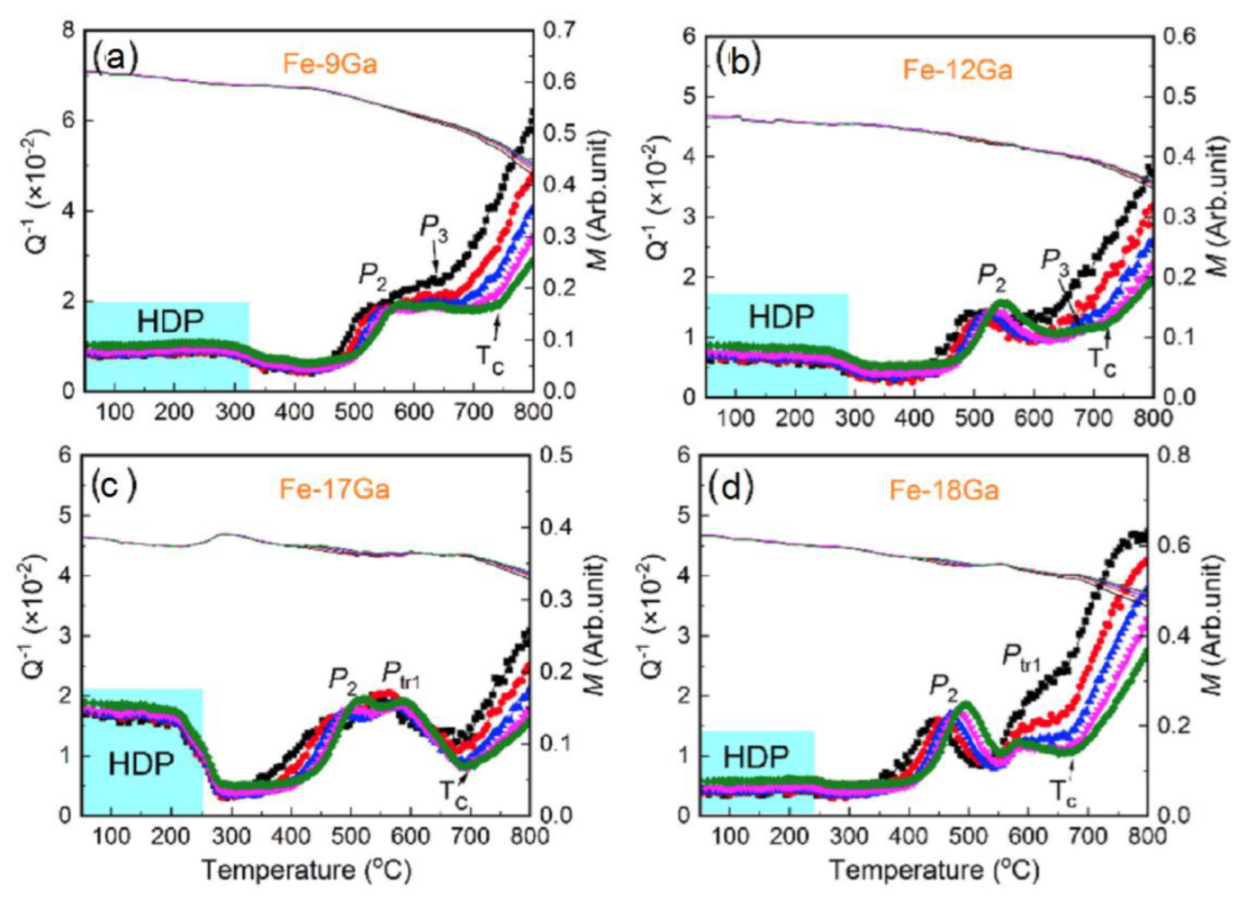
3.2. Anelasticity of Fe–Ga Alloys with Ga > 24 at.%
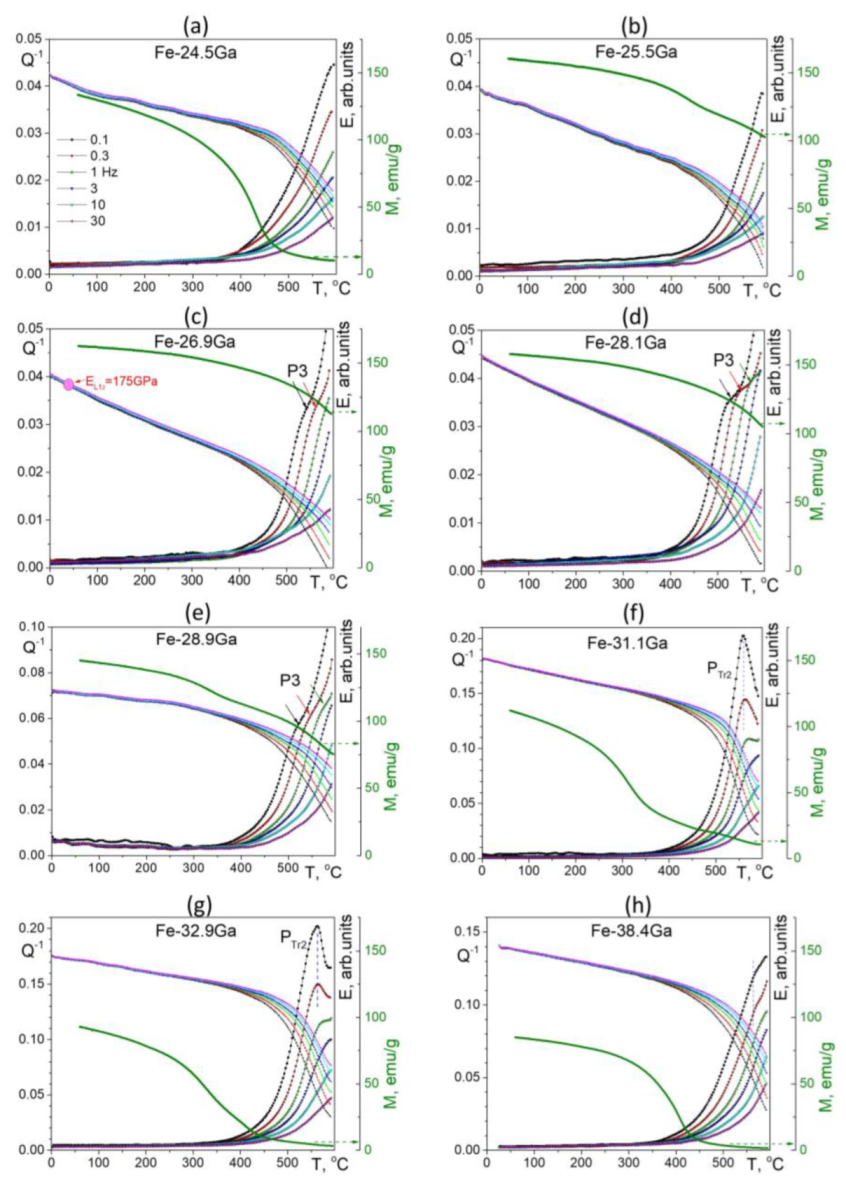
3.2.1. Fe–Ga Alloys with 23 < Ga at.% < 29
- -
- Two thermally activated peaks and transient effect, accompanied by sharp increase in modulus;
- -
- The P1 peak height decreases, and the P2 peak height increases. Activation energies (values rounded to tenths) of P1 and P2 peaks are given in Table 3;
- -
- The relaxation strength of the P2 (Zener) peak depends on %Ga in alloys and is discussed below;
- -
- The total height of transient peaks (peak plus background) is approximately constant within accuracy of the measurements 0.05 < QTr−1 < 0.06. High temperature background increases with increase in Ga content leading to non-monotonous height of PTr vs. Ga content.
- Transient Anelasticity
- Thermally Activated Anelastic Effects
- (1)
- The P1 effect can be caused, at least partly, by the γ relaxation. This relaxation effect is due to the kink pair formation on screw dislocations in bcc metals or alloys with the activation energy Hγ = 2HK(screw) + HM(screw) − 2kBT, where HK(screw) and HM(screw) are the energies of formation and motion of a single kink on screw dislocation, correspondingly [111]. The main disagreement of the experimental results with the existing theory of the γ-relaxation theory [111,141,142] is in extremely fast values of the characteristic relaxation time (Table 3). The apparent experimental values fit better to the point defect relaxation, i.e., to the Snoek-type effect, while the dislocation related relaxation has slower relaxation time;
- (2)
- The P1 effect can be also caused by reorientation of pairs of vacancies under applied cyclic stress. Similar ideas were proposed for Fe–Al [143] and Fe–Cr alloys [144]. The arguments in favor of this hypothesis are a decrease in the P1 peak effect after annealing, which leads to a drastic decrease in vacancy concentration in Fe–Ga alloys [22], and the experimental values of characteristic relaxation times, which are in favor of the point defect relaxation.
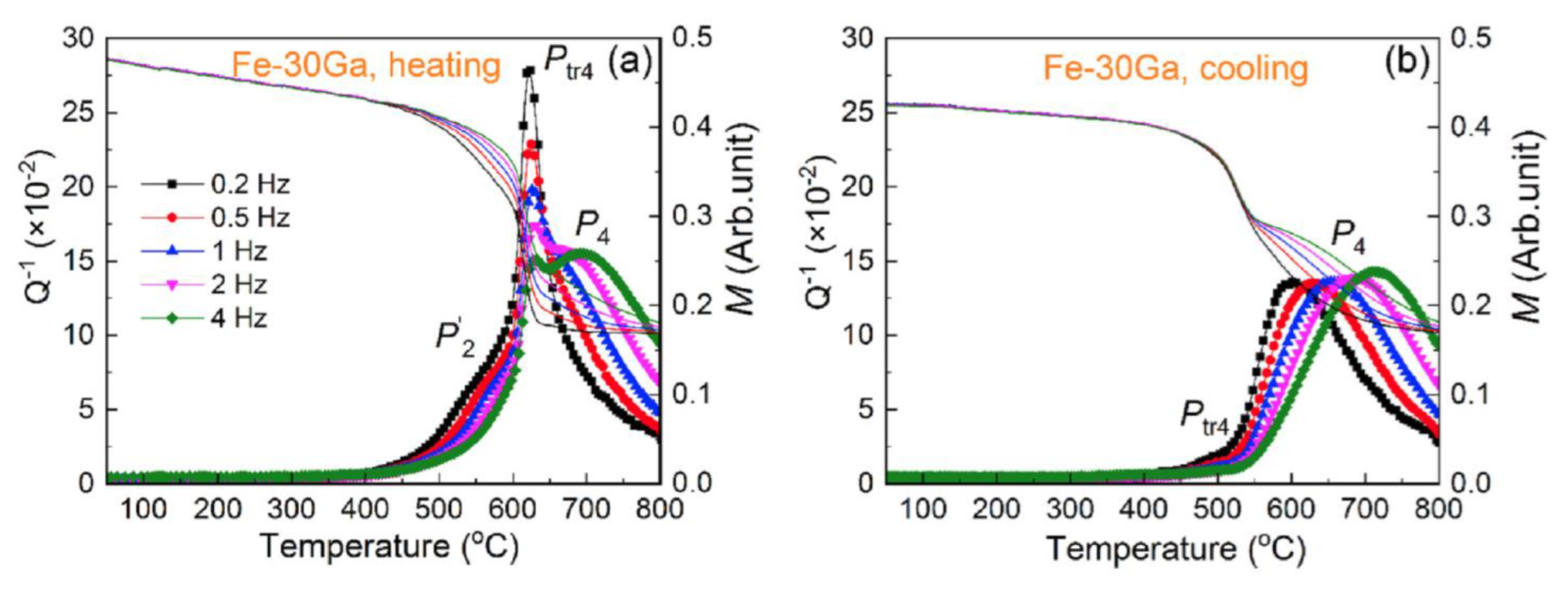
3.2.2. Fe–Ga Alloys Ga > 30 at.%
4. Summary
- i.
- Several papers report intermediate steps for the D03 to L12 transition, which includes the appearance of the m-D03, D022, L60, etc., nano or micro precipitations, and the twinning and local displacive character of this transition. In all cases, the appearance of these phases has a local character, and their volume fraction is very small to change the interpretation of anelastic effects discussed in this paper. Independent of the details of the transition mechanism, the related anelastic strain comes from the lattice deformation when the material is transformed. Phase transitions in the studied alloys are carefully evaluated by real-time neutron diffraction and discussed in this review;
- ii.
- Moreover, above-mentioned local phases were reported only by either XRD or TEM methods, which study the surface of the samples. Several papers clearly demonstrated that the bulk and surface structure of Fe–Ga samples is rather different [13,148,149]. This is another reason why we exclude discussion on the details of the surface structure of Fe–Ga alloys as all anelastic effects were measured only on a bulk sample.
- The Zener effect in the alloys with the same composition but different structure (bcc or fcc);
- Local mechanisms of Zener and Snoek effects in ternary Fe–Ga–Al alloys;
- There are inconsistencies between significantly higher magnetostriction in Fe–Ga alloys compared with Fe–Al alloys and damping capacity of alloys of these systems.
Funding
Acknowledgments
Conflicts of Interest
References
- Clark, A.E.; Hathaway, K.B.; Wun-Fogle, M.; Restorff, J.B.; Lograsso, T.A.; Keppens, V.M.; Petculescu, G.; Taylor, R.A. Extraordinary Magnetoelasticity and Lattice Softening in Bcc Fe-Ga Alloys. J. Appl. Phys. 2003, 93, 8621–8623. [Google Scholar] [CrossRef] [Green Version]
- Srisukhumbowornchai, N.; Guruswamy, S. Large magnetostriction in directionally solidified FeGa and FeGaAl alloys. J. Appl. Phys. 2001, 90, 5680–5688. [Google Scholar] [CrossRef] [Green Version]
- Lograsso, T.A.; Summers, E.M. Detection and quantification of D03 chemical order in Fe-Ga alloys using high resolution X-ray diffraction. Mater. Sci. Eng. A 2006, 416, 240–245. [Google Scholar] [CrossRef]
- Ishimoto, M.; Numakura, H.; Wuttig, M. Magnetoelastic damping in Fe–Ga solid-solution alloys. Mater. Sci. Eng. A 2006, 442, 195–198. [Google Scholar] [CrossRef]
- Smith, G.W.; Birchak, J.R. Effect of internal stress distribution on magnetomechanical damping. J. Appl. Phys. 1968, 39, 2311–2315. [Google Scholar] [CrossRef]
- Golovin, I.S. Damping mechanisms in high damping metals. Key Eng. Mater. 2006, 319, 225–230. [Google Scholar] [CrossRef]
- Ruffoni, M.P.; Pascarelli, S.; Grössinger, R.; Turtelli, R.S.; Bormio-Nunes, C.; Pettifer, R.F. Direct measurement of intrinsic atomic scale magnetostriction. Phys. Rev. Lett. 2008, 101, 147202. [Google Scholar] [CrossRef]
- Ikeda, O.; Kainuma, R.; Ohinuma, I.; Fukamichi, K.; Ishida, K. Phase equilibria and stability of ordered b.c.c. phases in the Fe-rich portion of the Fe–Ga system. J. Alloys Compd. 2002, 347, 198–205. [Google Scholar] [CrossRef]
- Xing, Q.; Du, Y.; McQueeney, R.; Lograsso, T. Structural investigations of Fe–Ga alloys: Phase relations and magnetostrictive behavior. Acta Mater. 2008, 56, 4536–4546. [Google Scholar] [CrossRef]
- Golovin, I.S.; Balagurov, A.M. Structure Induced Anelasticity in Iron Intermetallic Compounds and Alloys; Materials Research Forum LLC: Millersville, PA, USA, 2018; 256p. [Google Scholar]
- Golovin, I.S.; Palacheva, V.V.; Mohamed, A.K.; Balagurov, A.M. Structure and Properties of Fe-Ga Alloys as Promising Materials for Electronics. Phys. Met. Metallogr. 2020, 121, 851–893. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Golovin, I.S. Neutron scattering in studies of Fe-based functional alloys (Fe-Ga, Fe-Al). Uspekhi Fiz. Nauk. Russ. Acad. Sci. 2021, 64, 702–721. [Google Scholar]
- Sumnikov, S.V.; Bobrikov, I.A.; Golovin, I.S.; Balagurov, A.M. Bulk vs. surface structural phases in Fe-27Ga alloy. J. Alloys Compd. 2022, 928, 167116. [Google Scholar] [CrossRef]
- Gödecke, T.; Köster, W. Über den aufbau des systems Eisen-Gallium zwichen 10 und 50 at.%Ga und dessen abhängigkeit von der wärmebehandlung. Z. Met. 1977, 68, 758–764. [Google Scholar]
- Kubaschewski, O. Fe-Ga Iron-Gallium. In IRON-Binary Phase Diagrams; Springer: Berlin/Heidelberg, Germany, 1982; pp. 38–43. [Google Scholar]
- Mohamed, A.K.; Palacheva, V.V.; Cheverikin, V.V.; Zanaeva, E.N.; Cheng, W.C.; Kulitckii, V.; Divinski, S.; Wilde, G.; Golovin, I.S. The Fe-Ga phase diagram: Revisited. JALCOM 2020, 846, 156486. [Google Scholar] [CrossRef]
- Mohamed, A.K.; Palacheva, V.V.; Cheverikin, V.V.; Vershinina, T.N.; Balagurov, A.M.; Muralikrishna, G.M.; Esakkiraja, N.; Divinski, S.V.; Wilde, G.; Golovin, I.S. Low-temperature metastable-to-equilibrium phase transitions in Fe-Ga alloys. Intermetallics 2022, 145, 107540. [Google Scholar] [CrossRef]
- Mouas, M.; Fazel, N.; Gasser, F.; Golovin, I.; Gasser, J.-G. Kinetics of the L12 ↔ D019 transition for Fe3Ga-type alloy determined by in situ electrical resistivity. Mater. Lett. 2023, 334, 133731. [Google Scholar] [CrossRef]
- Golovin, I.; Balagurov, A.; Bobrikov, I.; Sumnikov, S.; Mohamed, A. Cooling rate as a tool of tailoring structure of Fe-(9–33%)Ga alloys. Intermetallics 2019, 114, 106610. [Google Scholar] [CrossRef]
- Golovin, I.; Mohamed, A.; Bobrikov, I.; Balagurov, A. Time-Temperature-Transformation from metastable to equilibrium structure in Fe-Ga. Mater. Lett. 2020, 263, 127257. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Samoylova, N.Y.; Bobrikov, I.A.; Sumnikov, S.V.; Golovin, I.S. The first- and second-order isothermal phase transitions in Fe3Ga-type compounds. Acta Crystallogr. Sect. B Struct. Sci. 2019, 75, 1024–1033. [Google Scholar] [CrossRef] [Green Version]
- Dubov, L.; Shtotsky, Y.; Akmalova, Y.; Funtikov, Y.; Palacheva, V.; Bazlov, A.; Golovin, I. Ordering processes in Fe-Ga alloys studied by positron annihilation lifetime spectroscopy. Mater. Lett. 2016, 171, 46–49. [Google Scholar] [CrossRef]
- Balagurov, A.; Sumnikov, S.; Cifre, J.; Palacheva, V.; Chubov, D.; Golovin, I. In situ study of order-disorder transitions and anelasticity in Fe-26Al alloy. J. Alloys Compd. 2023, 932, 167663. [Google Scholar] [CrossRef]
- Golovin, I.; Balagurov, A.M.; Palacheva, V.; Bobrikov, I.; Zlokazov, V. In situ neutron diffraction study of bulk phase transitions in Fe-27Ga alloys. Mater. Des. 2016, 98, 113–119. [Google Scholar] [CrossRef]
- Leineweber, A.; Becker, H.; Boev, A.; Bobrikov, I.; Balagurov, A.; Golovin, I. Fe13Ga9 intermetallic in bcc-base Fe–Ga alloy. Intermetallics 2021, 131, 107059. [Google Scholar] [CrossRef]
- Vershinina, T.; Bobrikov, I.; Sumnikov, S.; Boev, A.; Balagurov, A.; Mohamed, A.; Golovin, I. Crystal structure and phase composition evolution during heat treatment of Fe-45Ga alloy. Intermetallics 2021, 131, 107110. [Google Scholar] [CrossRef]
- Vershinina, T.; Samoylova, N.; Sumnikov, S.; Balagurov, A.; Palacheva, V.; Golovin, I. Comparative study of structures and phase transitions in Fe–(31−35) at%Ga alloys by in situ neutron diffraction. J. Alloys Compd. 2023, 934, 167967. [Google Scholar] [CrossRef]
- Guruswamy, S.; Mungsantisuk, P.; Corson, R.; Srisukhumbowornchai, N. Rare-earth free Fe–Ga based magnetostrictive alloys for actuators and sensor. Trans. Indian Inst. Met. 2004, 57, 315–323. [Google Scholar] [CrossRef]
- Fang, M.L.; Zhu, J.; Gao, X.X. Damping capacity of Fe-Ga alloys. Adv. Mater. Res. 2011, 228–229, 937–941. [Google Scholar]
- Golovin, I.S.; Rivière, A. Mechanisms of anelasticity in Fe-13Ga alloy. Intermetallics 2011, 19, 453–459. [Google Scholar] [CrossRef]
- Golovin, I.S.; Belamri, Z.; Hamana, D. Internal friction, dilatometric and calorimetric study of anelasticity in Fe-13 at. %Ga and Fe-8 at. % Al-3 at. %Ga alloys. J. Alloys Compd. 2011, 509, 8165–8170. [Google Scholar] [CrossRef]
- Fang, M.; Zhu, J.; Golovin, I.S.; Li, J.; Yuan, C.; Gao, X. Internal friction in (Fe80Ga20)99.95(NbC)0.05 alloy at elevated temperatures. Intermetallics 2012, 29, 133–139. [Google Scholar] [CrossRef]
- Golovin, I.; Cifre, J. Structural mechanisms of anelasticity in Fe–Ga-based alloys. J. Alloys Compd. 2014, 584, 322–326. [Google Scholar] [CrossRef]
- Golovin, I.; Palacheva, V.; Zadorozhnyy, V.; Zhu, J.; Jiang, H.; Cifre, J.; Lograsso, T. Influence of composition and heat treatment on damping and magnetostrictive properties of Fe–18%(Ga + Al) alloys. Acta Mater. 2014, 78, 93–102. [Google Scholar] [CrossRef] [Green Version]
- Emdadi, A.A.; Cifre, J.; Dementeva, O.Y.; Golovin, I.S. Effect of heat treatment on ordering and functional properties of the Fe–19Ga alloy. J. Alloys Compd. 2015, 619, 58–65. [Google Scholar] [CrossRef]
- Golovin, I.S.; Dubov, L.; Funtikov, Y.V.; Palacheva, V.; Cifre, J.; Hamana, D. Study of Ordering and Properties in Fe-Ga Alloys With 18 and 21 at. pct Ga. Met. Mater. Trans. A 2015, 46, 1131–1139. [Google Scholar] [CrossRef]
- Golovin, I.; Palacheva, V.; Bazlov, A.; Cifre, J.; Pons, J. Structure and anelasticity of Fe3Ga and Fe3(Ga,Al) type alloys. J. Alloys Compd. 2015, 644, 959–967. [Google Scholar] [CrossRef]
- Golovin, I.S.; Palacheva, V.V.; Emdadi, A.A.; Zadorozhnyy, M.Y.; Bazlov, A.I.; Gorshenkov, M.V.; Pozdniakov, A.V.; Savchenko, E.S.; Cifre, J.; Barbin, R.; et al. Structure and properties of high damping Fe-Ga based alloy. Met. Mater. 2015, 53, 267–274. [Google Scholar] [CrossRef] [Green Version]
- Golovin, I.S. Anelasticity of Fe-Ga based alloys. Mater. Des. 2015, 88, 577–587. [Google Scholar] [CrossRef]
- Golovin, I.; Palacheva, V.; Bazlov, A.; Cifre, J.; Nollmann, N.; Divinski, S.; Wilde, G. Diffusionless nature of D03 → L12 transition in Fe3Ga alloys. J. Alloys Compd. 2016, 656, 897–902. [Google Scholar] [CrossRef]
- Golovin, I.; Balagurov, A.M.; Bobrikov, I.; Palacheva, V.; Cifre, J. Phase transition induced anelasticity in Fe–Ga alloys with 25 and 27%Ga. J. Alloys Compd. 2016, 675, 393–398. [Google Scholar] [CrossRef]
- Golovin, I.; Balagurov, A.M.; Bobrikov, I.; Cifre, J. Structure induced anelasticity in Fe3Me (Me = Al, Ga, Ge) alloys. J. Alloys Compd. 2016, 688, 310–319. [Google Scholar] [CrossRef]
- Boyer, S.A.; Gerland, M.; Rivière, A.; Cifre, J.; Palacheva, V.V.; Mikhaylovskaya, A.V.; Golovin, I.S. Anelasticity of the Fe-Ga alloys in the range of Zener relaxation. J. Alloys Compd. 2018, 730, 424–433. [Google Scholar] [CrossRef]
- Emdadi, A.; Palacheva, V.V.; Balagurov, M.; Bobrikov, I.A.; Cheverikin, V.V.; Cifre, J.; Golovin, I.S. Tb-dependent phase transitions in Fe-Ga functional alloys. Intermetallics 2018, 93, 55–62. [Google Scholar] [CrossRef]
- Emdadi, A.; Palacheva, V.; Cheverikin, V.; Divinski, S.; Wilde, G.; Golovin, I. Structure and magnetic properties of Fe-Ga alloys doped by Tb. J. Alloys Compd. 2018, 758, 214–223. [Google Scholar] [CrossRef]
- Golovin, I.; Balagurov, A.; Emdadi, A.; Palacheva, V.; Bobrikov, I.; Cheverikin, V.; Zanaeva, E.; Mari, D. Phase transitions in Fe-27Ga alloys: Guidance to develop functionality. Intermetallics 2018, 100, 20–26. [Google Scholar] [CrossRef]
- Golovin, I.S.; Palacheva, V.V.; Emdadi, A.; Mari, D.; Heintz, A.; Balagurov, A.M.; Bobrikov, I.A. Anelasticity of Phase Transitions and Magnetostriction in Fe-(27-28%)Ga Alloys. Mater. Res. 2018, 21 (Suppl. S2), e20170906. [Google Scholar] [CrossRef] [Green Version]
- Golovin, I.; Balagurov, A.; Cheng, W.; Cifre, J.; Burdin, D.; Bobrikov, I.; Palacheva, V.; Samoylova, N.Y.; Zanaeva, E. In situ studies of atomic ordering in Fe-19Ga type alloys. Intermetallics 2018, 105, 6–12. [Google Scholar] [CrossRef]
- Golovin, I.S.; Palacheva, V.V.; Cifre, J.; Jiang, C. Mechanical spectroscopy of Fe-Ga alloys at elevated temperatures. J. Alloys Compd. 2019, 785, 1257–1263. [Google Scholar] [CrossRef]
- Golovin, I.; Palacheva, V.; Mari, D.; Vuillème, G.; Balagurov, A.; Bobrikov, I.; Cifre, J.; Sinning, H.-R. Mechanical spectroscopy as an in situ tool to study first and second order transitions in metastable Fe-Ga alloys. J. Alloys Compd. 2019, 790, 1149–1156. [Google Scholar] [CrossRef]
- Golovin, I.; Mohamed, A.; Palacheva, V.; Cheverikin, V.; Pozdnyakov, A.; Korovushkin, V.; Balagurov, A.; Bobrikov, I.; Fazel, N.; Mouas, M.; et al. Comparative study of structure and phase transitions in Fe-(25–27)%Ga alloys. J. Alloys Compd. 2019, 811, 152030. [Google Scholar] [CrossRef]
- Sun, M.; Wu, Y.; Jiang, W.; Liu, W.; Wang, X.; Gao, Y.; Liu, R.; Hao, T.; Wen, W.; Fang, Q. Effect of La addition on high-temperature order-disorder phase transformation in Fe−18Ga alloy. Intermetallics 2019, 111, 106496. [Google Scholar] [CrossRef]
- Sun, M.; Wang, X.; Jiang, W.; Wang, H.; Gao, Y.; Hao, T.; Zhang, T.; Wang, L.; Fang, Q.; Liu, C. Correlation between thermal history and high-temperature order–disorder phase transition in Fe–18Ga alloy. Mater. Lett. 2019, 239, 147–150. [Google Scholar] [CrossRef]
- Sun, M.; Balagurov, A.; Bobrikov, I.; Wang, X.; Wen, W.; Golovin, I.S.; Fang, Q. High damping in Fe-Ga-La alloys: Phenomenological model for magneto-mechanical hysteresis damping and experiment. J. Mater. Sci. Technol. 2021, 72, 69–80. [Google Scholar] [CrossRef]
- Qiao, R.; Gou, J.; Yang, T.; Zhang, Y.; Liu, F.; Hu, S.; Ma, T. Enhanced damping capacity of ferromagnetic Fe-Ga alloys by introducing structural defects. J. Mater. Sci. Technol. 2021, 84, 173–181. [Google Scholar] [CrossRef]
- Sun, M.; Li, L.; Liu, C.; Liu, X.; Jiang, W.; Lei, Y.; Gao, Y.; Cheng, Z.; Wang, X.; Sheng, Z.; et al. Ultra-high anisotropic magneto-mechanical damping in Fe-18at.%Ga single crystals. Scr. Mater. 2022, 212, 114552. [Google Scholar] [CrossRef]
- Li, L.; Sun, M.; Jing, K.; Gao, Y.; Jiang, W.; Wang, H.; Fang, Q.; Wang, X. Effects of Al addition on damping and mechanical properties of Fe–Ga alloy sheet. J. Mater. Res. Technol. 2022, 19, 3592–3602. [Google Scholar] [CrossRef]
- Sun, M.; Huanga, W.; Lia, L.; Jiang, W.; Gao, R.; Wen, W.; Hao, T.; Wang, X.; Fang, Q. Behavior and mechanism of quench-induced internal friction peak in Fe-18 at.%Ga alloy. J. Alloys Compd. 2021, 856, 158178. [Google Scholar] [CrossRef]
- Golovin, I.; Palacheva, V.; Mohamed, A.; Cifre, J.; Dubov, L.; Samoylova, N.; Balagurov, A. Mechanical spectroscopy of atomic ordering in Fe-(16−21)Ga-RE alloys. J. Alloys Compd. 2021, 864, 158819. [Google Scholar] [CrossRef]
- Golovin, I.S.; Mohamed, A.K.; Palacheva, V.V.; Cifre, J.; Samoylova, N.Y.; Balagurov, A.M. Mechanical spectroscopy of phase transitions in Fe-(23–38)Ga-RE alloys. J. Alloys Compd. 2021, 874, 159882. [Google Scholar] [CrossRef]
- Sun, M.; Li, L.; Jiang, W.; Gao, Y.; Wang, H.; Wang, X.; Fang, Q. Internal friction phenomena in a wide temperature range up to 800oC in long-term annealed Fe-(0–30) at.%Ga alloys. J. Alloys Compd. 2021, 887, 161444. [Google Scholar] [CrossRef]
- Li, L.; Gao, Y.X.; Sun, M.; Jing, K.; Zhuang, Z.; Wang, X.P.; Jiang, W.B.; Fang, Q.F. Effect of phase composition on the internal friction and magnetostriction in the L12/D03 biphase Fe-27Ga alloys. J. Alloys Compd. 2022, 895, 162661. [Google Scholar] [CrossRef]
- Li, L.; Gao, Y.X.; Zhuang, Z.; Sun, M.; Jiang, W.B.; Wang, H.; Fang, Q.F.; Wang, X.P. Study on high damping L12/D03 biphase Fe-27Ga alloys with near-zero magnetostriction and its damping mechanism. J. Alloys Compd. 2022, 924, 166554. [Google Scholar] [CrossRef]
- Chang, H.; Jen, S.; Liao, Y.; Chang, F.; Chiu, C.; Cifre, J.; Chubov, D.; Golovin, I. Magneto-mechanical and damping properties in Fe79.9Al20-xGaxTb0.1 alloys. J. Alloys Compd. 2022, 927, 166894. [Google Scholar] [CrossRef]
- Birčáková, Z.; Milyutin, V.; Kollár, P.; Fáberová, M.; Bureš, R.; Füzer, J.; Neslušan, M.; Vorobiov, S.; Batkova, M. Magnetic characteristics and core loss separation in magnetostrictive FeGa and FeGaRE (RE = Tb, Y) alloys. Intermetallics 2022, 151, 107744. [Google Scholar] [CrossRef]
- Golovin, I.S.; Neuhäuser, H.; Rivière, A.; Strahl, A. Anelastisity of Fe-Al alloys, revisited. Intermetallics 2004, 12, 125–150. [Google Scholar] [CrossRef]
- Golovin, I.S.; Blanter, M.S.; Pozdova, T.V.; Tanaka, K.; Magalas, L.B. Effect of substitutional ordering on the carbon Snoek relaxation in Fe-Al-C alloys. Phys. Status Solidi (A) 1998, 168, 403–416. [Google Scholar] [CrossRef]
- Golovin, I.S.; Pozdova, T.V.; Rokhmanov, N.Y.; Mukherji, D. Relaxation Mechanisms in Fe-Al-C Alloys. Metall. Mater. Trans. A 2003, 34, 255–266. [Google Scholar] [CrossRef]
- Golovin, I.S.; Golovina, S.B.; Strahl, A.; Neuhäuser, H.; Pavlova, T.S.; Golovin, S.A.; Schaller, R. Anelasticity of Fe3Al. Scr. Mater. 2004, 50, 1187–1192. [Google Scholar] [CrossRef]
- Strahl, A.; Golovina, S.B.; Golovin, I.S.; Neuhäuser, H. On dislocation-related internal friction in Fe-22 to 31 at.% Al. J. Alloys Compd. 2004, 370, 268–273. [Google Scholar] [CrossRef]
- Golovin, I.S.; Divinski, S.V.; Čížek, J.; Procházka, I.; Stein, F. Study of atoms diffusivity and related relaxation phenomena in Fe3Al-(Ti,Nb)-C alloys. Acta Mater. 2005, 53, 2581–2594. [Google Scholar] [CrossRef]
- Golovin, I.S.; Rivière, A. Mechanical spectroscopy of the Zener relaxation in Fe-22Al and Fe-26Al alloys. Intermetallics 2006, 14, 570–577. [Google Scholar] [CrossRef]
- Pavlova, T.S.; Golovin, I.S.; Sinning, H.-R.; Golovin, S.A.; Siemers, C. Internal friction in Fe-Al-Si alloys at elevated temperatures. Intermetallics 2006, 14, 1238–1249. [Google Scholar] [CrossRef]
- Golovin, I.S.; Strahl, A.; Neuhäuser, H. Anelastic relaxations and structure of ternary Fe-Al-Me alloys with Me = Co, Cr, Ge, Mn, Nb, Si, Ta, Ti, Zr. Int. J. Mater. Res. (Form. Z. Metallkd.) 2006, 42, 1078–1092. [Google Scholar] [CrossRef]
- Golovin, I.S.; Rivière, A. Zener relaxation in ordered-disordered Fe-(22–28%)Al alloys. Mater. Sci. Eng. A 2006, 442, 86–91. [Google Scholar] [CrossRef]
- Golovin, I.S.; Pavlova, T.S.; Golovina, S.B.; Sinning, H.-R.; Golovin, S.A. Effect of severe plastic deformation of Fe-26at.%Al and titanium on internal friction. Mater. Sci. Eng. A 2006, 442, 165–169. [Google Scholar] [CrossRef]
- Strahl, A.; Golovin, I.S.; Neuhäuser, H.; Golovina, S.B.; Sinning, H.-R. Influence of Al concentration on parameters of the short and long range carbon diffusion in Fe-Al alloys. Mater. Sci. Eng. A 2006, 442, 128–132. [Google Scholar] [CrossRef]
- Lambri, O.; Pérez-Landazábal, J.I.; Cuello, G.; Cano, J.; Recarte, V.; Golovin, I.S. Mechanical Spectroscopy and Neutron Diffraction Studies in Fe-Al-Si Alloys. Solid State Phenom. 2008, 137, 91–98. [Google Scholar] [CrossRef]
- Golovin, I.S.; Rivière, A. Mechanical spectroscopy of Fe-25Al-Cr alloys in medium temperature range. Solid State Phenom. 2008, 137, 99–108. [Google Scholar] [CrossRef]
- Golovin, I.S.; Jäger, S.; Semin, V.; Serzhantova, G.; Sinning, H.; Sokolova, O.A.; Stein, F.; Golovin, S. Snoek-Type and Zener Relaxation in Fe-Si-Al Alloys. Solid State Phenom. 2008, 137, 69–82. [Google Scholar] [CrossRef]
- Sinning, H.-R.; Golovin, I.S.; Strahl, A.; Sokolova, O.A.; Sazonova, T. Interactions between solute atoms in Fe–Si–Al–C alloys as studied by mechanical spectroscopy. Mater. Sci. Eng. A 2009, 521–522, 63–66. [Google Scholar] [CrossRef]
- Simas, P.; San Juan, J.; Nó, M.L. High-temperature internal friction in a Fe-38 at.% Al intermetallic. Mater. Sci. Eng. A 2009, 521–522, 73–76. [Google Scholar] [CrossRef]
- Simas, P.; San Juan, J.; Nó, M.L. High-temperature relaxation analysis in a fine-grain B2 FeAl intermetallic. Intermetallics 2010, 18, 1348–1352. [Google Scholar] [CrossRef]
- Lambri, O.; Pérez-Landazábal, J.; Recarte, V.; Cuello, G.; Golovin, I. Order controlled dislocations and grain boundary mobility in Fe–Al–Cr alloys. J. Alloys Compd. 2012, 537, 117–122. [Google Scholar] [CrossRef]
- Emdadi, A.; Nartey, M.A.; Xu, Y.G.; Golovin, I.S. Study of damping capacity of Fe–5.4Al–0.05Ti alloy. J. Alloys Compd. 2015, 653, 460–467. [Google Scholar] [CrossRef]
- Golovin, I.S.; Emdadi, A.; Balagurov, A.M.; Bobrikov, I.A.; Cifre, J.; Zadorozhnyy, Μ.Y.; Rivière, A. Anelasticity of iron-aluminide Fe3Al type single and polycrystals. J. Alloys Compd. 2018, 746, 660–669. [Google Scholar] [CrossRef]
- Chang, H.; Jen, S.; Liao, Y.; Tseng, D.; Hsieh, H.; Chang, W.; Chiu, C.; Cifre, J.; Chubov, D.; Golovin, I. Enhancement of the magneto-mechanical properties in directional solidified Fe80Al20 alloys by doping Tb. J. Alloys Compd. 2022, 893, 162262. [Google Scholar] [CrossRef]
- Udovenko, V.A.; Chudakov, I.B.; Polyakova, N.A. Mechanism for the High Damping State in Alloys of the Fe-Al System. In M3D III, Mechanics and Mechanisms of Material Damping, ASTM STP 1304; Wolfenden, A., Kinra, V., Eds.; ASTM: Philadelphia, PA, USA, 1997; pp. 204–213. [Google Scholar]
- Chudakov, I.; Polyakova, N.A.; Mackushev, S.; Udovenko, V. On the Formation of High Damping State in Fe-Al and Fe-Cr Alloys. Solid State Phenom. 2008, 137, 109–118. [Google Scholar] [CrossRef]
- Mishnev, P.A.; Uglov, V.A.; Zhilenko, S.V.; Chudakov, I.B. Analysis of Specific Properties and Features of Application of New Industrial High-Damping Steel. Mater. Sci. Forum 2018, 931, 608–613. [Google Scholar] [CrossRef]
- Coulomb, C.A. Recherches Theoretiques et Experimentales sur la Force de Torsion et sur l’elasticite Des Fils Metals; Academie Royale des Sciences: Paris, France, 1784. (In French) [Google Scholar]
- Hook, R. Lectures De Potentia Restitutiva, or of Springs, Explaining the Power of Springing Bodies; John Martyn: Oxford, UK, 1678. [Google Scholar]
- Zener, C. Elasticity and Anelasticity of Metals; University of Chicago Press: Chicago, IL, USA, 1948. [Google Scholar]
- Mason, W.P. Physical Acoustics and Properties of Solids; Van Nostrand: Princeton, NJ, USA, 1958. [Google Scholar]
- Krishtal, M.A.; Pigusov, Y.V.; Golovin, S.A. Internal Friction in Metals and Alloys; Metallurgizdat: Moscow, Russia, 1964. (In Russian) [Google Scholar]
- Nowick, A.S.; Berry, B.S. Anelastic Relaxation in Crystalline Solids; Academic Press: New York, NY, USA, 1972. [Google Scholar]
- De Batist, R. Internal Friction of Structural Defects in Crystalline Solids; North Holland Publ Comp: Amsterdam, The Netherlands, 1972. [Google Scholar]
- Postnikov, V.S. Internal Friction in Metals; Metallurgiya: Moscow, Russia, 1974. (In Russian) [Google Scholar]
- Lakes, R.S. Viscoelastic Solids; CRC Press: Boca Raton, FL, USA, 1999. [Google Scholar]
- Schaller, R.; Fantozzi, G.; Gremaud, G. (Eds.) Mechanical Spectroscopy Q–1 2001 with Applications to Materials Science; Trans Tech Publications: Zurich, Switzerland, 2001. [Google Scholar]
- Blanter, M.S.; Golovin, I.S.; Neuhäuser, H.; Sinning, H.-R. Internal Friction in Metallic Materials. A Handbook; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Ngai, K.L. Relaxation and Diffusion in Complex Systems; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Fang, Q.; Wang, X.; Wu, X.; Lu, H. The Basic Principles and Applications of Internal Friction and Mechanical Spectroscopy; Shanghai Jiaotong University Press: Shanghai, China, 2014. (In Chinese) [Google Scholar]
- Kohlrausch, R. Theorie des elektrischen Rückstandes in der Leidener Flasche. R. Phys. Chem. 1854, 91, 179–214. [Google Scholar]
- Snoek, J. Effect of small quantities of carbon and nitrogen on the elastic and plastic properties of iron. Physica 1941, 8, 711–733. [Google Scholar] [CrossRef]
- Smithells, K.J. Metals Reference Book: Elastic Properties and Damping Capacity, 5th ed.; Elsevier: London, UK; Boston, MA, USA, 1976; pp. 975–1006. [Google Scholar]
- Blanter, M.S.; Pigusov, Y.V. (Eds.) Internal Friction Method in Physical Metallurgy Researches; Metallurgiya: Moscow, Russia, 1991. (In Russian) [Google Scholar]
- Blanter, M.S.; Golovin, I.S. Internal Friction. In Encyclopedia of Iron, Steel and Their Alloys; Colás, R., Totten, G.E., Eds.; Taylor and Francis: New York, NY, USA, 2015; pp. 1852–1870. [Google Scholar]
- Koiwa, M. Theory of the Snoek effect in ternary b.c.c. alloys I. General theory. Philos. Mag. 1971, 24, 81–106. [Google Scholar] [CrossRef]
- Kê, T.-S. Experimental Evidence of the Viscous Behavior of Grain Boundaries in Metals. Phys. Rev. 1947, 71, 533–546. [Google Scholar] [CrossRef]
- Benoit, W. Thermodynamics of 2nd and 3rd order transitions. In Mechanical Spectroscopy Q-1 2001 with Applications to Materials Science; Schaller, R., Fantozzi, G., Gremaud, G., Eds.; Trans Tech Publications: Zurich, Switzerland, 2001; pp. 341–360. [Google Scholar]
- Fantozzi, G. Ferroelectricity. In Mechanical Spectroscopy Q-1 2001 with Applications to Materials Science; Schaller, R., Fantozzi, G., Gremaud, G., Eds.; Trans Tech Publications: Zurich, Switzerland, 2001; pp. 361–381. [Google Scholar]
- Scheil, E.; Müller, J.; Die. Dämpfung mechanischer Schwingungen wdhrend der Martensitbildung in Eisen-Nickel-Legierungen. Arch. Eisenhutt. 1956, 27, 801–805. [Google Scholar]
- Delorme, J.F.; Gobin, P.F. Internal friction and microdeformation associated with the martensitic transformations in metallic solids. Metaux-Corros. -Ind. 1973, 573, 185–222. [Google Scholar]
- San Juan, J.; Perez-Saez, R.P. Transitory effects. In Mechanical Spectroscopy Q-1 2001 with Applications to Materials Science; Schaller, R., Fantozzi, G., Gremaud, G., Eds.; Trans Tech Publications: Zurich, Switzerland, 2001; pp. 416–437. [Google Scholar]
- James, D.W. High damping metals for engineering applications. Mater. Sci. Eng. 1969, 4, 1–8. [Google Scholar] [CrossRef]
- Cochardt, A. Magnetoelastic Internal Friction. In Magnetic Properties of Metals and Alloys; Pergamon Press: Tarrytown, NY, USA, 1958; pp. 328–363. [Google Scholar]
- Golovin, I.S. Mechanisms of Linear Anelasticity in Fe–M and Fe–Al–M (M = Ga, Ge) Alloys. Phys. Met. Metallogr. 2013, 114, 1018–1030. [Google Scholar] [CrossRef]
- Sun, M.; Wang, X.; Wang, L.; Wang, H.; Jiang, W.; Liu, W.; Hao, T.; Gao, R.; Gao, Y.; Zhang, T.; et al. High-temperature order-disorder phase transition in Fe-18Ga alloy evaluated by internal friction method. J. Alloys Compd. 2018, 750, 669–676. [Google Scholar] [CrossRef]
- Kissinger, H.E. Reaction Kinetics in Differential Thermal Analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Bobrikov, I.A.; Sumnikov, S.V.; Golovin, I.S. Antiphase domains or dispersed clusters? Neutron diffraction study of coherent atomic ordering in Fe3Al-type alloys. Acta Mater. 2018, 153, 45–52. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Bobrikov, I.A.; Sumnikov, S.V.; Golovin, I.S. Dispersed clusters in (Fe,Cr)3Al alloys: Neutron time-of-flight diffraction study. Phys. Rev. Mater. 2019, 3, 013608. [Google Scholar] [CrossRef]
- Balagurov, A.; Bobrikov, I.; Golovin, I. Effects of Ordering in Fe–xAl Alloys. JETP Lett. 2019, 110, 585–591. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Bobrikov, I.A.; Sumnikov, S.V.; Golovin, I.S. Cluster-Like Structure of Fe-Based Alloys with Enhanced Magnetostriction. J. Surf. Investig. X-ray Synchrotron Neutron Tech. 2020, 14, S11–S14. [Google Scholar] [CrossRef]
- Chubov, D.G.; Palacheva, V.V.; Cifre, J.; Chang, H.W.; Jen, S.U.; Balagurov, A.M.; Golovin, I.S. Mechanisms of anelastic relaxation in ternary Fe-20(Al+Ga) alloys. Submitted.
- Balagurov, A.M.; Bobrikov, I.A.; Sumnikov, S.V.; Yerzhanov, B.; Chubov, D.G.; Palacheva, V.V.; Golovin, I.S. Structures and phase transitions in Fe-Ga-Al alloys. Phys. Solid State 2022, 64, 1837–1845. [Google Scholar] [CrossRef]
- Golovin, I.; Chubov, D.; Berezner, A.; Shcherbakov, A.; Schlagel, D.; Chang, H. Magnetostriction and damping of forced vibrations in Fe-Mo single and polycrystal alloys. J. Alloys Compd. 2022, 925, 166786. [Google Scholar] [CrossRef]
- Smith, G.W.; Birchak, J.R. Internal stress distribution theory of magnetomechanical hysteresis and extension to include effects of magnetic field and applied stress. J. Appl. Phys. 1969, 40, 5174–5178. [Google Scholar] [CrossRef]
- Chudakov, I.B.; Golovin, I.S. The Effect of Crystalline and Magnetic Structure on Magneto-mechanical Damping of Fe-Cr-Based alloys. Mech. Mech. Mater. Damping ASTM STP 1997, 1304, 162–178. [Google Scholar]
- Beshers, D.; Vunni, G. Topological hysteresis as a model for Rayleigh damping. Mater. Sci. Eng. A 2006, 442, 191–194. [Google Scholar] [CrossRef]
- Palacheva, V.; Emdadi, A.; Emeis, F.; Bobrikov, I.; Balagurov, A.; Divinski, S.; Wilde, G.; Golovin, I. Phase transitions as a tool for tailoring magnetostriction in intrinsic Fe-Ga composites. Acta Mater. 2017, 130, 229–239. [Google Scholar] [CrossRef]
- Zhang, Y.; Gou, J.; Yang, T.; Ke, Y.; Ma, T. Non-equilibrium time-temperature-transformation diagram for enhancing magnetostriction of Fe-Ga alloys. Acta Mater. 2023, 244, 118548. [Google Scholar] [CrossRef]
- Okamoto, H. Fe–Ga (Iron–Gallium). In Phase Diagrams of Binary Iron Alloys; Okamoto, H., Ed.; ASM International: Materials Park, OH, USA, 1993; pp. 51–147. [Google Scholar]
- Bras, J.; Couderc, J.; Fagot, M.; Ferre, J. Transformation ordered-disordered solution in Fe–Ga. J. Acta Metall. 1977, 25, 1077–1084. [Google Scholar] [CrossRef]
- Vershinina, T.N.; Bobrikov, I.A.; Sumnikov, S.V.; Balagurov, A.M.; Mohamed, A.K.; Golovin, I.S. Structure evolution of as-cast metastable Fe-38Ga alloy towards equilibrium. J. Alloys Compd. 2021, 889, 161782. [Google Scholar] [CrossRef]
- Khachaturyan, A.G.; Viehland, D. Structurally Heterogeneous Model of Extrinsic Magnetostriction for Fe-Ga and Similar. Magnetic Alloys: Part I. Decompos. Confin. Displac. Transform./Met. Mater. Trans. 2007, A38, 2308–2316. [Google Scholar]
- Khachaturyan, A.; Viehland, D. Structurally Heterogeneous Model of Extrinsic Magnetostriction for Fe-Ga and Similar Magnetic Alloys: Part II. Giant Magnetostriction and Elastic Softening. Metall. Mater. Trans. 2007, A38, 2317–2328. [Google Scholar] [CrossRef]
- Balagurov, A.M.; Bobrikov, I.A.; Golovin, I.S.; Cheverikin, V.V.; Golovin, S.A. Stabilization of bcc-born phases in Fe-27Ga by adding Tb: Comparative in situ neutron diffraction study. Mater. Lett. 2016, 181, 67–70. [Google Scholar] [CrossRef]
- Golovin, I.S.; Balagurov, A.M.; Palacheva, V.V.; Emdadi, A.; Bobrikov, I.A.; Churyumov, A.I.; Cheverikin, V.V.; Pozdniakov, A.V.; Mikhaylovskaya, A.V.; Golovin, S.A. Influence of Tb on structure and properties of Fe-19%Ga and Fe-27%Ga alloys. J. Alloys Compd. 2017, 707, 51–56. [Google Scholar] [CrossRef]
- Golovin, I.S.; Golovina, S.B. Effect of alloying α-Fe with aluminum, silicon, cobalt, and germanium on the Snoek relaxation parameters. Phys. Met. Metallogr. 2006, 102, 593–603. [Google Scholar] [CrossRef]
- Seeger, A. The mechanism of the β-relaxation in high-purity bcc metals. Philos. Mag. Lett. 2003, 83, 107–115. [Google Scholar] [CrossRef]
- Seeger, A. Dislocation relaxation processes in bcc metals: B versus g relaxation. Phil. Mag. Lett. 2004, 84, 79–86. [Google Scholar] [CrossRef]
- Schaefer, H.E.; Damson, B.; Weller, M.; Arzt, E.; George, E.P. Thermal vacancies and high-temperature me-chanical properties of FeAl. Phys. Status Solidi (A) 1997, 160, 531–540. [Google Scholar] [CrossRef]
- Konstantinović, M.J.; Minov, B.; Renterghem, W.V. Carbon Distribution in Ferrit-ic-Martensitic Fe-Cr-C Alloys. Mater. Res. 2018, 21 (Suppl. S2), e20170886. [Google Scholar] [CrossRef] [Green Version]
- Girifalco, L.A. Vacancy concentration and diffusion in order-disorder alloys. J. Phys. Chem. Solid. 1964, 24, 323–333. [Google Scholar] [CrossRef]
- Eggersmann, M.; Mehrer, H. Diffusion in intermetallic phases of the Fe-Al system. Philos. Mag. A 2000, 80, 1219–1244. [Google Scholar] [CrossRef]
- Salamon, M.; Mehrer, H. Interdiffusion, Kirkendall effect, and Al self-diffusion in Fe-Al alloys. Z. Met. 2005, 96, 4–16. [Google Scholar]
- Bobrikov, I.A.; Samoylova, N.Y.; Sumnikov, S.V.; Ivanshina, O.Y.; Korneeva, E.A.; Balagurov, A.M.; Golovin, I.S. Temperature evolution of Fe–27Ga structure: Comparison of in situ X-ray and neutron diffraction studies. J. Appl. Crystallogr. 2020, 53, 1343–1352. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, P.; Gou, J.; Liu, X.; Yang, T.; You, C.; Ma, T. Depth-dependent decomposition and property of large magnetostriction Fe-Ga alloys. Appl. Surf. Sci. 2021, 569, 151059. [Google Scholar] [CrossRef]






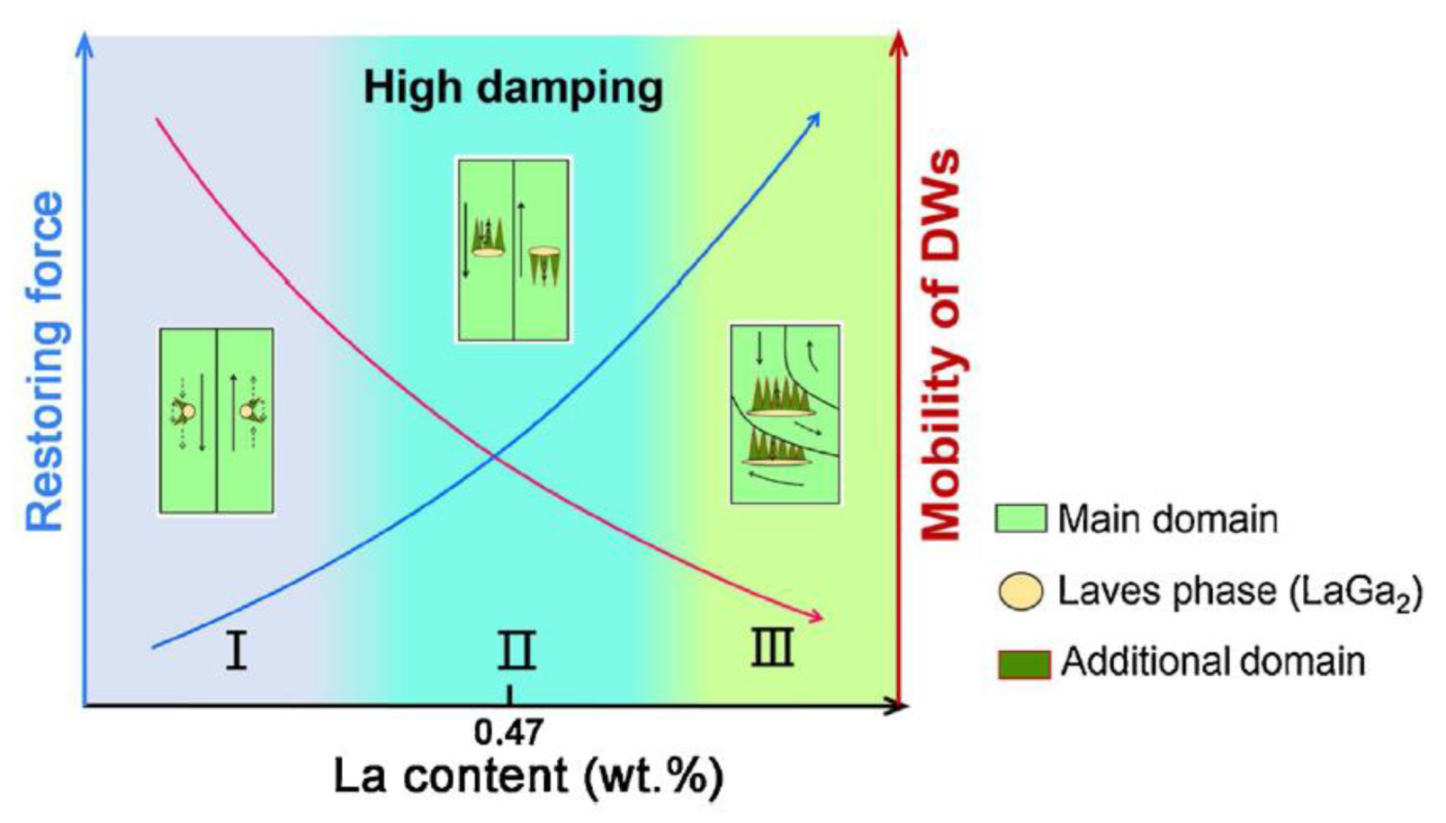


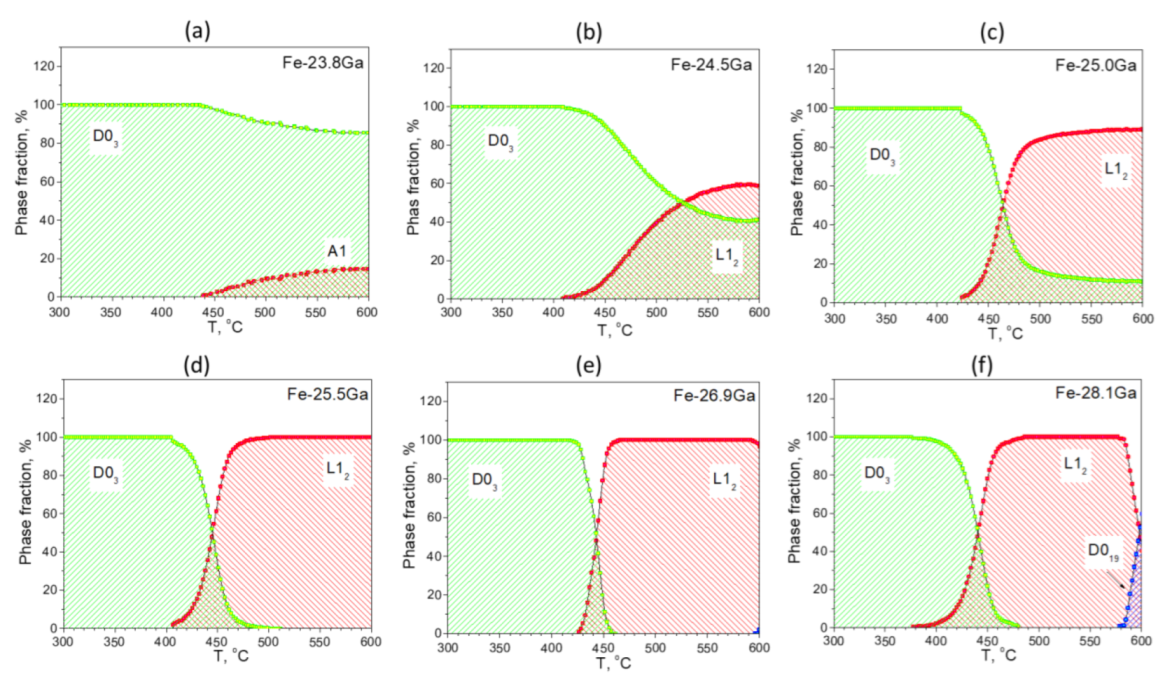
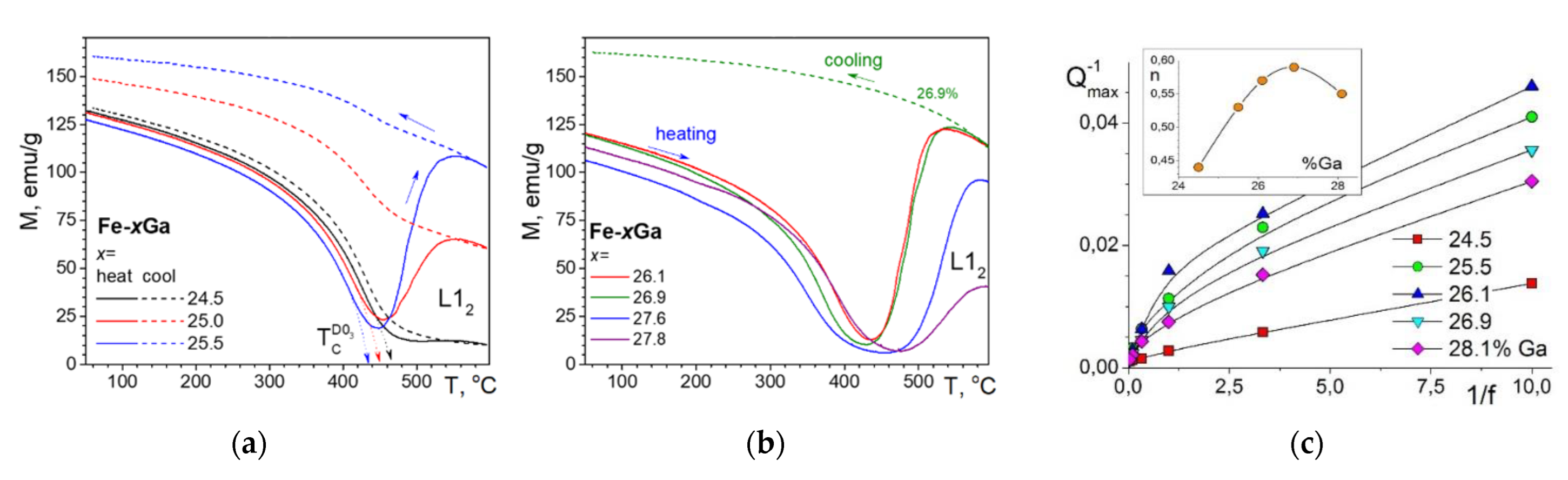
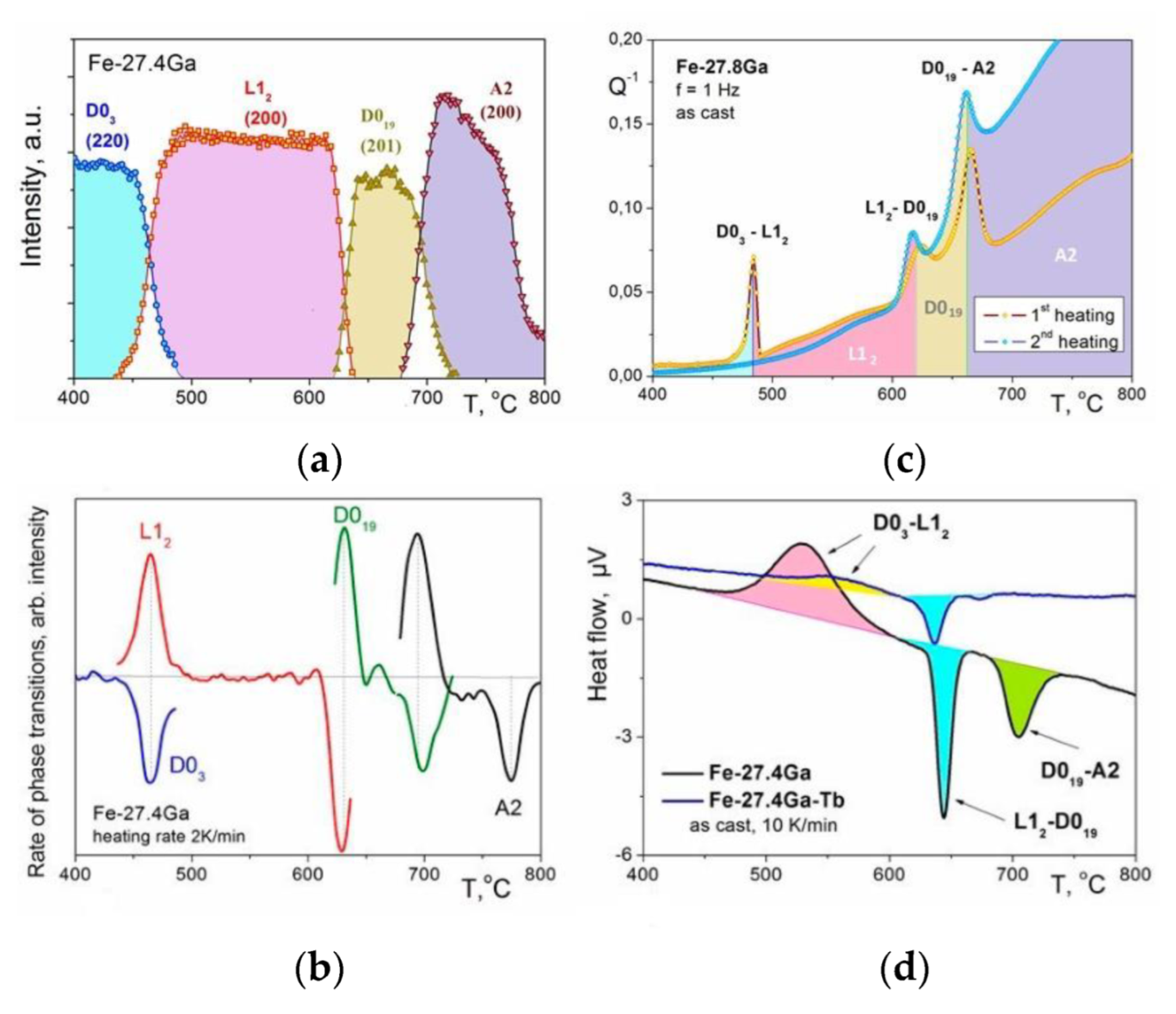





| Alloy | H, eV | τ0, s | βτ (1 Hz) |
|---|---|---|---|
| Fe–15.5Ga | 2.49 ± 0.05 | 4 × 10−18 | 1.6 |
| Fe–16.5Ga | 2.56 ± 0.02 | 9 × 10−19 | 1.7 |
| Fe–17.5Ga | 2.39 ± 0.05 | 8 × 10−18 | 1.9 |
| Fe–18.6Ga | 2.56 ± 0.02 | 6 × 10−19 | 2.1 |
| Fe–19.5Ga | 2.60 ± 0.06 | 9 × 10−19 | 2.2 |
| Fe–20.7Ga | 2.38 ± 0.02 | 5 × 10−18 | 2.0 |
| Alloy | P1 (Snoek-Type) | P2 (Zener) | ||
|---|---|---|---|---|
| H, eV | τ0, s | (H), eV | τ0, s | |
| Fe–18Ga–0.2La | HDP plateau instead of peak | 2.40 ± 0.20 | 8 × 10−18 | |
| Fe–18.6Ga–0.1Dy | 1.2 ± 0.2 | 7 × 10−17 | 2.60 ± 0.10 | 4 × 10−19 |
| Fe–19.5Ga–0.15Tb | unclear | 2.24 ± 0.06 | 6 × 10−17 | |
| Fe–18.5Ga–0.2Er | 1.2 ± 0.1 | 8 × 10−18 | 2.70 ± 0.10 | 7 × 10−20 |
| Fe–18.7Ga–0.1Yb | 1.1 ± 0.1 | 4 × 10−15 | 2.50 ± 0.10 | 9 × 10−19 |
| Ga, at.% | 23.1 | 23.8 | 24.5 | 25.5 | 26.9 | 28.1 | 28.9 | 31.1 | |
| Snoek | H, eV | 1.1 | 1.1 | 1.1 | 1.1 | 1.1 | 1.2 | 1.1 | 1.2 |
| τ0, s | 10−15 | 10−15 | 10−15 | 10−15 | 10−15 | 10−16 | 10−15 | 10−16 | |
| Zener | H, eV | 2.2 | 1.9 | 2.3 | 2.4 | 1.7 | 1.8 | 1.8 | 1.4 |
| τ0, s | 10−16 | 10−15 | - | - | 10−15 | 10−15 | 10−16 | 10−16 |
| PTr1 D03 → L12 | PTr2 L12 → D019 | PTr3 D019 → A2(B2) | |
|---|---|---|---|
| 0.35 | 0.18 | 0.29 | |
| 0.41 | 0.22 | 0.44 |
| Transition | Second-Order Transition Disorder (D03 to A2) | First-Order Irreversible Transition (D03 to L12) | First-Order Irreversible Transition: From D03 + Fe13Ga9 to L12 + Fe6Ga5 |
|---|---|---|---|
| Range of n | n ≈ 0.7–0.9 | n ≈ 0.6 (average) | n ≈ 0.2–0.7 |
| % Ga | 16–20% | 24–28% | 29–33% or 38% |
| Fe–16.5Ga | 0.91 | - | - |
| Fe–17.5Ga | 0.90 | - | - |
| Fe–18.6Ga | 0.69 | - | - |
| Fe–19.5Ga | 0.71 | - | - |
| Fe–25.0Ga | - | 0.57 | - |
| Fe–25.5Ga | - | 0.56 | - |
| Fe–26.1Ga | - | 0.62 | - |
| Fe–26.9Ga | - | 0.52 | - |
| Fe–27.3Ga | - | 0.63 | - |
| Fe–27.6Ga | - | 0.68 | - |
| Fe–28.1Ga | - | 0.61 | - |
| Fe–28.9Ga | - | - | 0.25 |
| Fe–31.1Ga | - | - | 0.69 |
| Fe–32.9Ga | - | - | 0.68 |
| Fe–38.4Ga | - | - | 0.21 |
| Alloy | D03/B2 | Fe13Ga9 | L12 | α-Fe6Ga5 |
|---|---|---|---|---|
| Fe–31.1Ga | 25–850 | (415)–570, | 470–650 | 570–670 |
| Fe–32.9Ga | 25–850 | 25 (390)–570 | 480–650 | 570–670 |
| Fe–34.4Ga | 25–850 | 25 (350)–580 | 500–670 | 560–700 |
| Fe–38.4Ga | 25–850 | 25–570 | 570–660 | 570–720 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Golovin, I.S. Anelastic Effects in Fe–Ga and Fe–Ga-Based Alloys: A Review. Materials 2023, 16, 2365. https://doi.org/10.3390/ma16062365
Golovin IS. Anelastic Effects in Fe–Ga and Fe–Ga-Based Alloys: A Review. Materials. 2023; 16(6):2365. https://doi.org/10.3390/ma16062365
Chicago/Turabian StyleGolovin, Igor S. 2023. "Anelastic Effects in Fe–Ga and Fe–Ga-Based Alloys: A Review" Materials 16, no. 6: 2365. https://doi.org/10.3390/ma16062365
APA StyleGolovin, I. S. (2023). Anelastic Effects in Fe–Ga and Fe–Ga-Based Alloys: A Review. Materials, 16(6), 2365. https://doi.org/10.3390/ma16062365





