Structural and Thermoelectric Properties of Gd2−2xSr1+2xMn2O7 Double-Layered Manganites
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Structural and Morphological Analysis
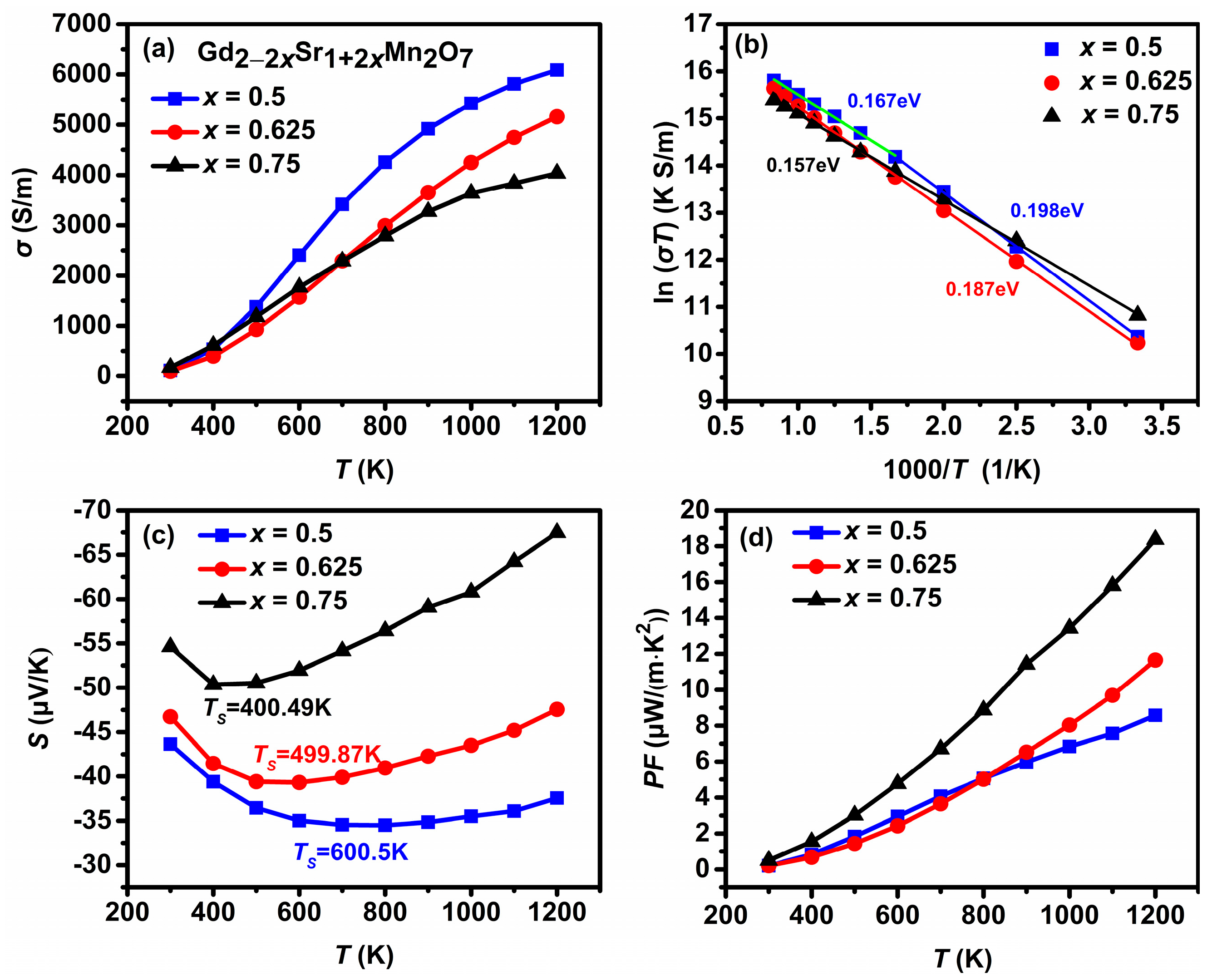
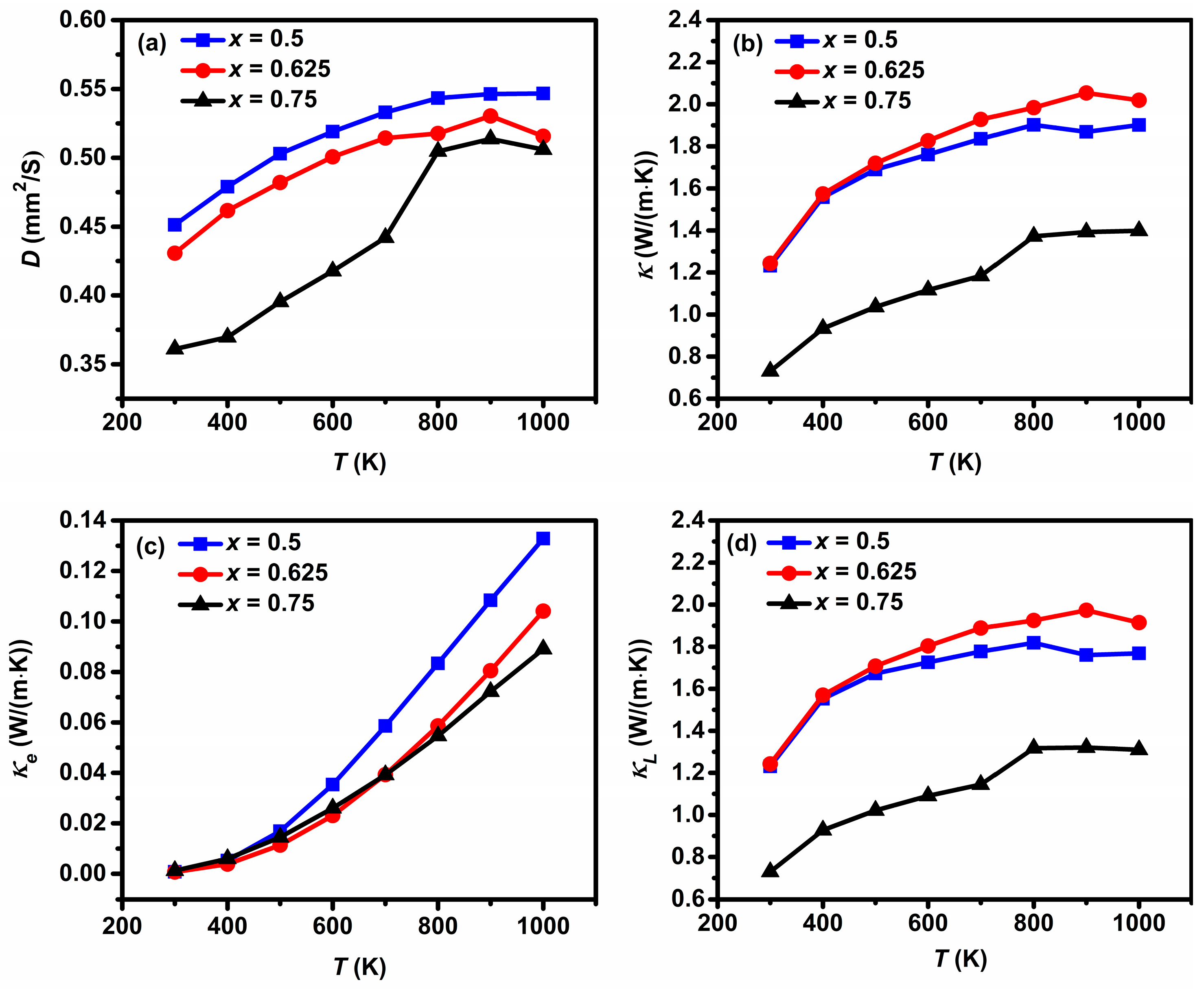
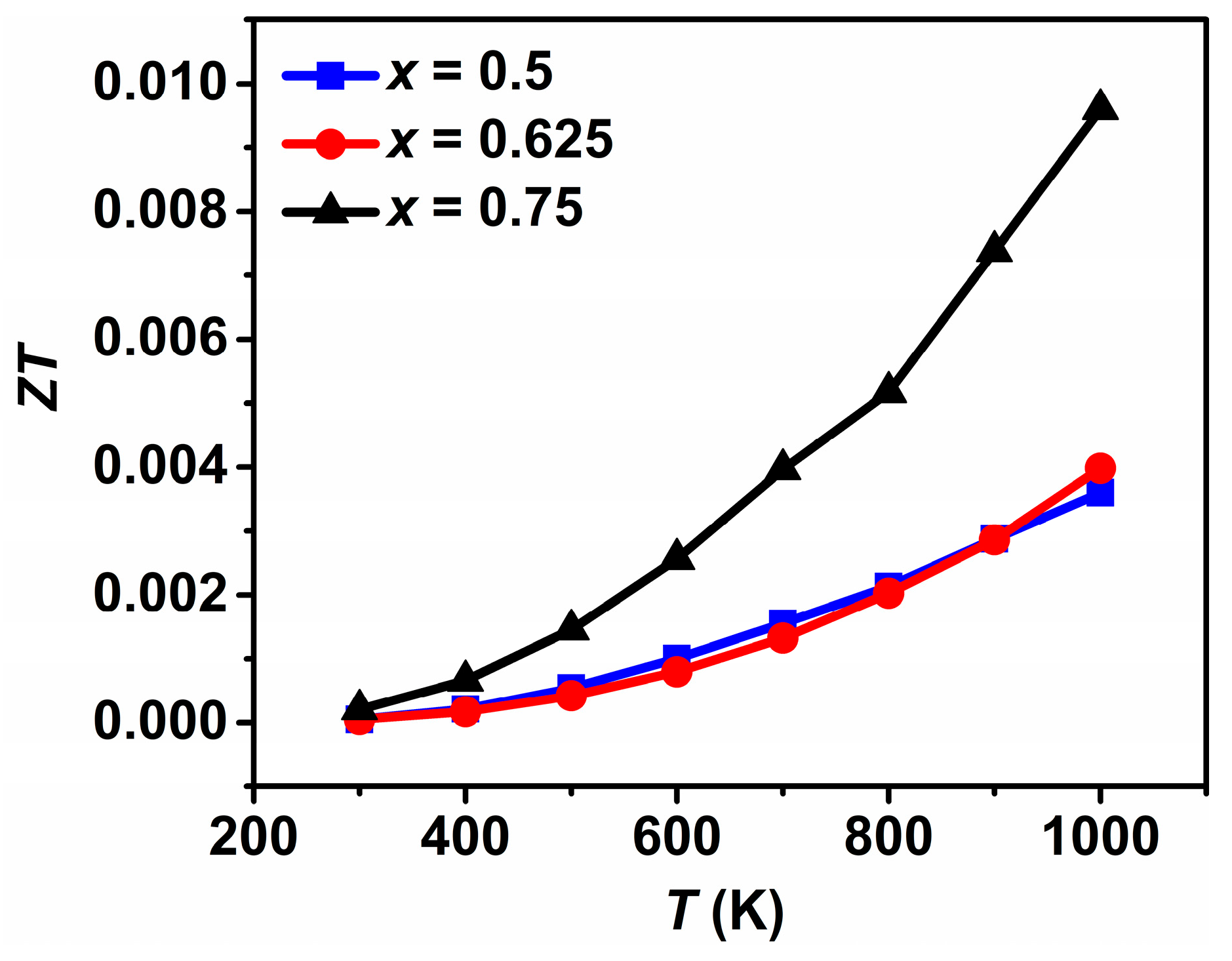
3.2. Thermoelectric Properties
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Terasaki, I.; Sasago, Y.; Uchinokura, K. Large thermoelectric power in NaCo2O4 single crystals. Phys. Rev. B 1997, 56, R12685–R12687. [Google Scholar] [CrossRef]
- Fergus, J.W. Oxide materials for high temperature thermoelectric energy conversion. J. Eur. Ceram. Soc. 2012, 32, 525–540. [Google Scholar] [CrossRef]
- Yin, Y.N.; Tudu, B.; Tiwari, A. Recent advances in oxide thermoelectric materials and modules. Vacuum 2017, 146, 356–374. [Google Scholar] [CrossRef]
- Zhao, L.-D.; He, J.; Berardan, D.; Lin, Y.; Li, J.-F.; Nan, C.-W.; Dragoe, N. BiCuSeO oxyselenides: New promising thermoelectric materials. Energy Environ. Sci. 2014, 7, 2900–2924. [Google Scholar] [CrossRef]
- Megha, A.; Sourav, J.S.; Mani, R.; Tanmoy, M. High performance (ZT>1) n-type oxide thermoelectric composites from earth abundant materials. Nano Energy 2021, 84, 105905. [Google Scholar] [CrossRef]
- Wang, H.; Su, W.; Liu, J.; Wang, C. Recent development of n-type perovskite thermoelectrics. J. Mater. 2016, 2, 225–236. [Google Scholar] [CrossRef] [Green Version]
- Walia, S.; Balendhran, S.; Nili, H.; Zhuiykov, S.; Rosengarten, G.; Wang, Q.H.; Bhaskaran, M.; Sriram, S.; Strano, M.S.; Kalantar-zadeh, K. Transition metal oxides—Thermoelectric properties. Prog. Mater Sci. 2013, 58, 1443–1489. [Google Scholar] [CrossRef] [Green Version]
- Kimura, T.; Tokura, Y. Layered Magnetic Manganites. Annu. Rev. Mater. Sci. 2000, 30, 451–474. [Google Scholar] [CrossRef]
- Dawley, N.M.; Pek, E.K.; Lee, C.-H.; Ragasa, E.J.; Xiong, X.; Lee, K.; Phillpot, S.R.; Chernatynskiy, A.V.; Cahill, D.G.; Schlom, D.G. Thermal conductivity of the n = 1–5 and 10 members of the (SrTiO3)nSrO Ruddlesden–Popper superlattices. Appl. Phys. Lett. 2021, 118, 091904. [Google Scholar] [CrossRef]
- Wang, Y.F.; Lee, K.H.; Ohta, H.; Koumoto, K. Fabrication and thermoelectric properties of heavily rare-earth metal-doped SrO(SrTiO3)n (n=1, 2) ceramics. Ceram. Int. 2008, 34, 849–852. [Google Scholar] [CrossRef]
- Toyoda, T.; Sasaki, N.; Shimada, K.; Okube, M.; Sasaki, S. High-Temperature Thermoelectric Property of Layered La2−2xCa1+2xMn2O7 Manganites (0.75 ≤x ≤ 1.0). Jpn. J. Appl. Phys. 2011, 50, 041101. [Google Scholar] [CrossRef]
- Liu, C.-J.; Bhaskar, A.; Yuan, J.J. High-temperature transport properties of Ca0.98RE0.02MnO3−δ (RE=Sm, Gd, and Dy). Appl. Phys. Lett. 2011, 98, 214101. [Google Scholar] [CrossRef]
- Sun, R.R.; Qin, X.Y.; Li, L.L.; Li, D.; Zhang, J.; Zhang, Y.S.; Tang, C.J. The effects of elements doping on transport and thermoelectric properties of Sr3Ti2O7. J. Phys. Chem. Solids 2014, 75, 629–637. [Google Scholar] [CrossRef]
- Wang, Y.F.; Lee, K.H.; Hyuga, H.; Kita, H.; Ohta, H.; Koumoto, K. Enhancement of thermoelectric performance in rare earth-doped Sr3Ti2O7 by symmetry restoration of TiO6 octahedra. J. Electroceram. 2010, 24, 76–82. [Google Scholar] [CrossRef]
- Mitchell, J.F.; Argyriou, D.N.; Berger, A.; Gray, K.E.; Osborn, R.; Welp, U. Spin, Charge, and Lattice States in Layered Magnetoresistive Oxides. J. Phys. Chem. B 2001, 105, 10731–10745. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, F.; Zhang, P.; Sun, X.; Yan, Q. Magnetic and electric properties of layered perovskites Nd2−2xSr1+2xMn2O7 (x=0.3–0.5). J. Magn. Magn. Mater. 1998, 190, 166–170. [Google Scholar] [CrossRef]
- Moritomo, Y.; Asamitsu, A.; Kuwahara, H.; Tokura, Y. Giant magnetoresistance of manganese oxides with a layered perovskite structure. Nature 1996, 380, 141–144. [Google Scholar] [CrossRef]
- Kimura, T.; Tomioka, Y.; Kuwahara, H.; Asamitsu, A.; Tamura, M.; Tokura, Y. Interplane Tunneling Magnetoresistance in a Layered Manganite Crystal. Science 1996, 274, 1698–1701. [Google Scholar] [CrossRef]
- Hur, N.H.; Kim, J.-T.; Yoo, K.H.; Park, Y.K.; Park, J.-C. Effect of lanthanide ions on the magnetotransport properties in layered Sr1.6R1.4Mn2O7 (R=La, Pr, Nd, Gd). Phys. Rev. B 1998, 57, 10740–10744. [Google Scholar] [CrossRef]
- Asano, H.; Hayakawa, J.; Matsui, M. Two-dimensional ferromagnetic ordering and magnetoresistance in the layered perovskite La2−2xCa1+2xMn2O7. Phys. Rev. B 1997, 56, 5395–5403. [Google Scholar] [CrossRef]
- Battle, P.D.; Green, M.A.; Laskey, N.S.; Kasmir, N.; Millburn, J.E.; Spring, L.E.; Sullivan, S.P.; Rosseinsky, M.J.; Vente, J.F. Control of electronic properties by lanthanide size and manganese oxidation state in the MnIII/MnIV Ruddlesden-Popper phases Ln2−xSr1+xMn2O7. J. Mater. Chem. 1997, 7, 977–988. [Google Scholar] [CrossRef]
- He, L.; Qin, N.; Wei, J.; Li, M.; Song, Y.; Yan, J. Metastable phase diagram of the Gd2O3–SrO–CoOx ternary system. Int. J. Mater. Res. 2022, 113, 508–519. [Google Scholar] [CrossRef]
- Wei, J.X.; Yin, Y.; Yan, J.L. Structural and thermoelectric properties of GdxSr2−xCoO4 layered perovskites. Ceram. Int. 2021, 47, 19835–19842. [Google Scholar] [CrossRef]
- Argyriou, D.N.; Mitchell, J.F.; Potter, C.D.; Bader, S.D.; Kleb, R.; Jorgensen, J.D. Unconventional magnetostriction in layered La1.2Sr1.8Mn2O7: Evidence for spin-lattice coupling above TC. Phys. Rev. B 1997, 55, R11965–R11968. [Google Scholar] [CrossRef]
- Kumar, B.; Tiwari, J.K.; Chauhan, H.C.; Ghosh, S. Multiple magnetic phase transitions with different universality classes in bilayer La1.4Sr1.6Mn2O7 manganite. Sci. Rep. 2021, 11, 21184. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Physica B 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Battle, P.D.; Green, M.A.; Laskey, N.S.; Millburn, J.E.; Murphy, L.; Rosseinsky, M.J.; Sullivan, S.P.; Vente, J.F. Layered Ruddlesden-Popper manganese oxides: Synthesis and cation ordering. Chem. Mater. 1997, 9, 552–559. [Google Scholar] [CrossRef]
- Ruck, K.; Dorr, K.; Nenkov, K.; Muller, K.H.; Krabbes, G.; Schupp, B.; Khristov, M. Magnetic and transport properties of Nd0.2La1.8−2xSr1+2xMn2O7 (x=0.5, 0.4, 0.3) and La1.5Sr1.5Mn2O7. J. Phys. Condens. Matter 2001, 13, 1571–1583. [Google Scholar] [CrossRef]
- Zhu, Y.; Wang, C.; Su, W.; Li, J.; Liu, J.; Du, Y.; Mei, L. High-temperature thermoelectric performance of Ca0.96Dy0.02RE0.02MnO3 ceramics (RE=Ho, Er, Tm). Ceram. Int. 2014, 40, 15531–15536. [Google Scholar] [CrossRef]
- Nag, A.; Shubha, V. Oxide Thermoelectric Materials: A Structure–Property Relationship. J. Electron. Mater. 2014, 43, 962–977. [Google Scholar] [CrossRef] [Green Version]
- Lan, J.; Lin, Y.-H.; Fang, H.; Mei, A.; Nan, C.-W.; Liu, Y.; Xu, S.; Peters, M. High-Temperature Thermoelectric Behaviors of Fine-Grained Gd-Doped CaMnO3 Ceramics. J. Am. Ceram. Soc. 2010, 93, 2121–2124. [Google Scholar] [CrossRef]
- Nakamae, S.; Colson, D.; Forget, A.; Legros, I.; Marucco, J.F.; Ayache, C.; Ocio, M. Thermoelectric power of hole-doped manganites: La2−2xSr1+2xMn2O7 (0.3 ≤x ≤0.5). Phys. Rev. B 2001, 63, 092407. [Google Scholar] [CrossRef]
- Tanwar, K.; Anjum, F.; Shukla, A.K.; Maiti, T. Role of structural distortion on thermoelectric aspects of heavily Sr2+ doped GdMnO3. J. Appl. Phys. 2018, 124, 094902. [Google Scholar] [CrossRef]
- Cutler, M.; Leavy, J.F.; Fitzpatrick, R.L. Electronic Transport in Semimetallic Cerium Sulfide. Phys. Rev. 1964, 133, A1143–A1152. [Google Scholar] [CrossRef]
- Wang, C.; Shi, L.; Xu, X.; Zhou, S.; Zhao, J.; Guo, Y.; Liu, H.; He, L.; Cai, X.; Xu, G. High-temperature thermoelectric characteristics of B-site substituted Yb0.1Ca0.9Mn1−xNbxO3 system (0≤x≤0.1). Appl. Phys. A 2013, 112, 1003–1009. [Google Scholar] [CrossRef]
- Kanas, N.; Singh, S.P.; Rotan, M.; Desissa, T.D.; Grande, T.; Wiik, K.; Norby, T.; Einarsrud, M.-A. Thermoelectric Properties of Ca3Co2−xMnxO6 (x = 0.05, 0.2, 0.5, 0.75, and 1). Materials 2019, 12, 497. [Google Scholar] [CrossRef] [Green Version]


| x = 0.5 | x = 0.625 | x = 0.75 | |
|---|---|---|---|
| a (Å) | 3.82705 (5) | 3.83642 (7) | 3.83446 (10) |
| c (Å) | 20.0030 (3) | 19.8882 (4) | 19.9048 (6) |
| V (Å3) | 292.971 (7) | 292.72 (1) | 292.66 (1) |
| ρx (g/cm3) * | 6.342 | 6.123 | 5.842 |
| d (g/cm3) | 5.9716 | 5.6942 | 5.5009 |
| % T. D. | 94.2 | 93.0 | 94.2 |
| Occ. Gd1/Sr1 | 0.141/0.859 | 0.176/0.824 | 0.131/0.869 |
| zGd2/Sr2 | 0.31729 (4) | 0.31714 (4) | 0.31677 (5) |
| Occ. Gd2/Sr2 | 0.466/0.534 | 0.306/0.694 | 0.150/0.850 |
| zMn | 0.09766 (8) | 0.09698 (9) | 0.0975 (1) |
| zO2 | 0.1996 (3) | 0.1973 (3) | 0.1966 (4) |
| zO3 | 0.0989 (2) | 0.0969 (2) | 0.0959 (3) |
| dMn-O1 (Å) | 1.9535 (18) | 1.9288 (18) | 1.9417 (24) |
| dMn-O2 (Å) | 2.0391 (63) | 1.9952 (62) | 1.9716 (83) |
| dMn-O3 (Å) | 1.9137 (1) | 1.91821 (4) | 1.9175 (1) |
| Rp (%) | 10.9 | 10.7 | 12.8 |
| Rwp (%) | 12.7 | 12.7 | 15.2 |
| Rexp (%) | 7.38 | 7.09 | 6.69 |
| EDS Composition (at.%) | |||||
|---|---|---|---|---|---|
| x | Nominal Formula | Gd | Sr | Mn | EDS Formula |
| 0.5 | GdSr2Mn2O7 | 20.77 ± 3.53 | 40.02 ± 1.48 | 39.21 ± 3.28 | Gd1.038Sr2.001Mn1.961O7 |
| 0.625 | Gd0.75Sr2.25Mn2O7 | 14.07 ± 4.12 | 46.25 ± 4.28 | 39.68 ± 1.92 | Gd0.703Sr2.313Mn1.984O7 |
| 0.75 | Gd0.5Sr2.5Mn2O7 | 12.64 ± 3.79 | 46.49 ± 3.67 | 40.87 ± 1.67 | Gd0.632Sr2.325Mn2.043O7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qin, N.; Pang, Y.; Xu, Z.; Chen, X.; Yan, J. Structural and Thermoelectric Properties of Gd2−2xSr1+2xMn2O7 Double-Layered Manganites. Materials 2023, 16, 2548. https://doi.org/10.3390/ma16072548
Qin N, Pang Y, Xu Z, Chen X, Yan J. Structural and Thermoelectric Properties of Gd2−2xSr1+2xMn2O7 Double-Layered Manganites. Materials. 2023; 16(7):2548. https://doi.org/10.3390/ma16072548
Chicago/Turabian StyleQin, Nailing, Yehai Pang, Zhengbing Xu, Xiyong Chen, and Jialin Yan. 2023. "Structural and Thermoelectric Properties of Gd2−2xSr1+2xMn2O7 Double-Layered Manganites" Materials 16, no. 7: 2548. https://doi.org/10.3390/ma16072548
APA StyleQin, N., Pang, Y., Xu, Z., Chen, X., & Yan, J. (2023). Structural and Thermoelectric Properties of Gd2−2xSr1+2xMn2O7 Double-Layered Manganites. Materials, 16(7), 2548. https://doi.org/10.3390/ma16072548







