Investigating Electromechanical Buckling Response of FG-GPL-Reinforced Piezoelectric Doubly Curved Shallow Shells Embedded in an Elastic Substrate
Abstract
1. Introduction
2. Problem Formulation
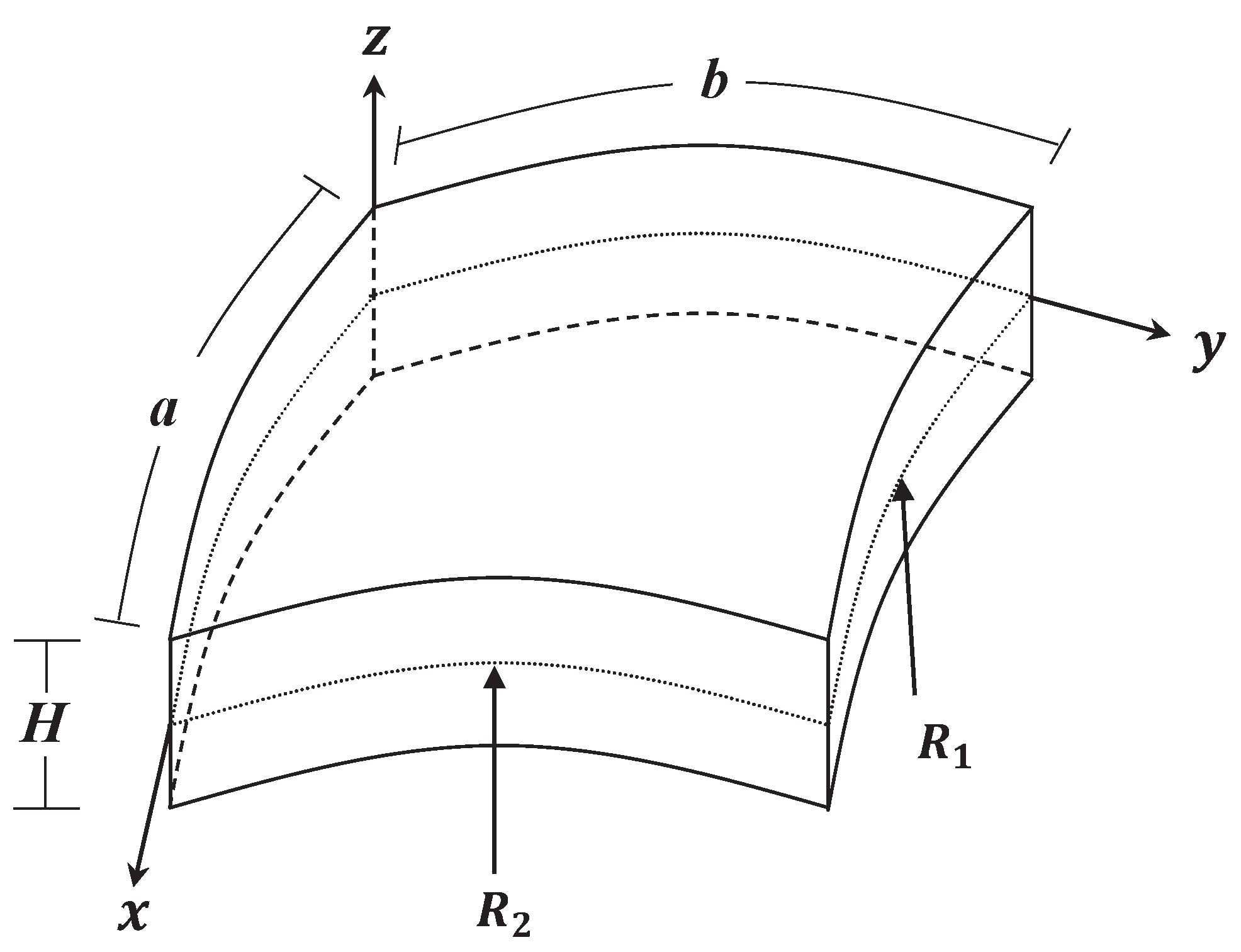
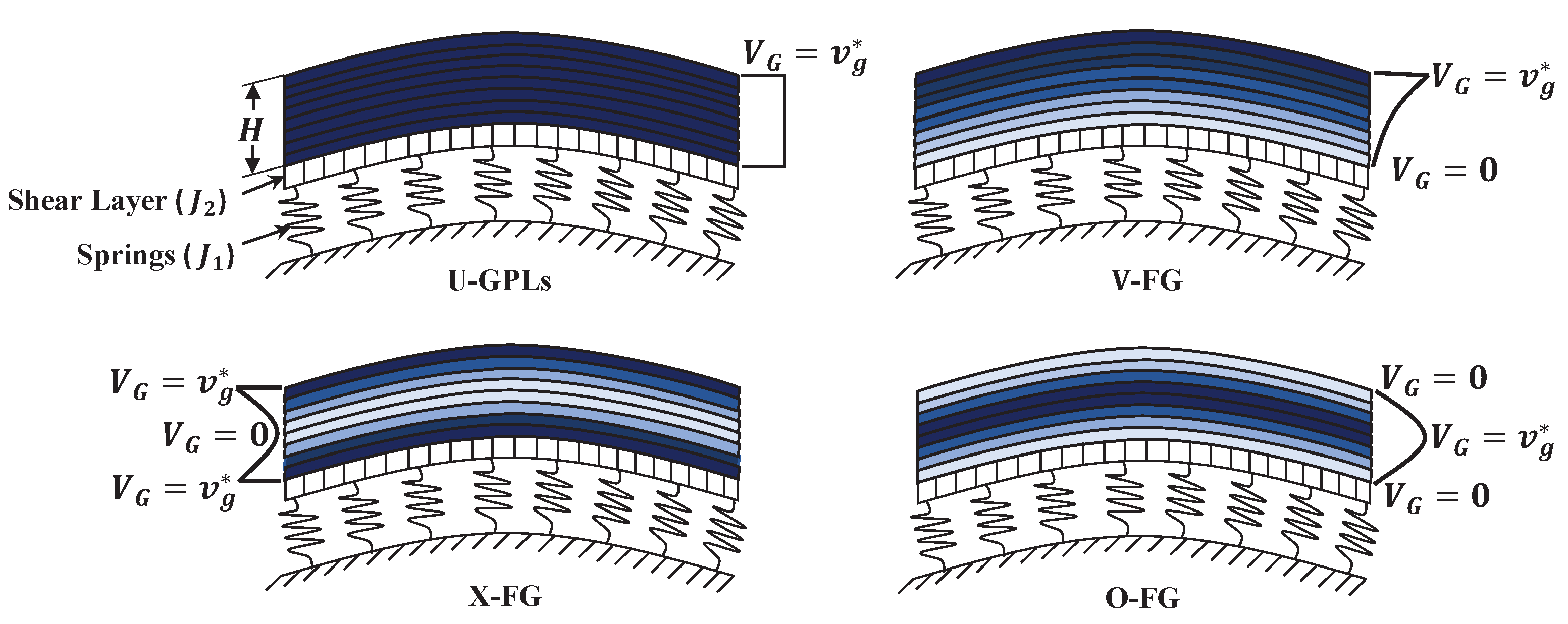
2.1. Shell Configuration
2.2. Displacement Field
2.3. Constitutive Relations
3. Governing Equations
4. Solution Procedure
5. Numerical Anaylsis and Discussions
6. Conclusions
- The GPL weight fraction and GPL distribution types significantly impact the stiffness as well as the dynamical characteristics of structures of the GPLs/piezoelectric nanocomposite doubly curved shallow shells. The GPLs improve high-strength and multifunctional nanocomposite materials. The results emphasize that the U-GPL type has the best mechanical characteristics, while the O-FG type has the weakest stiffness.
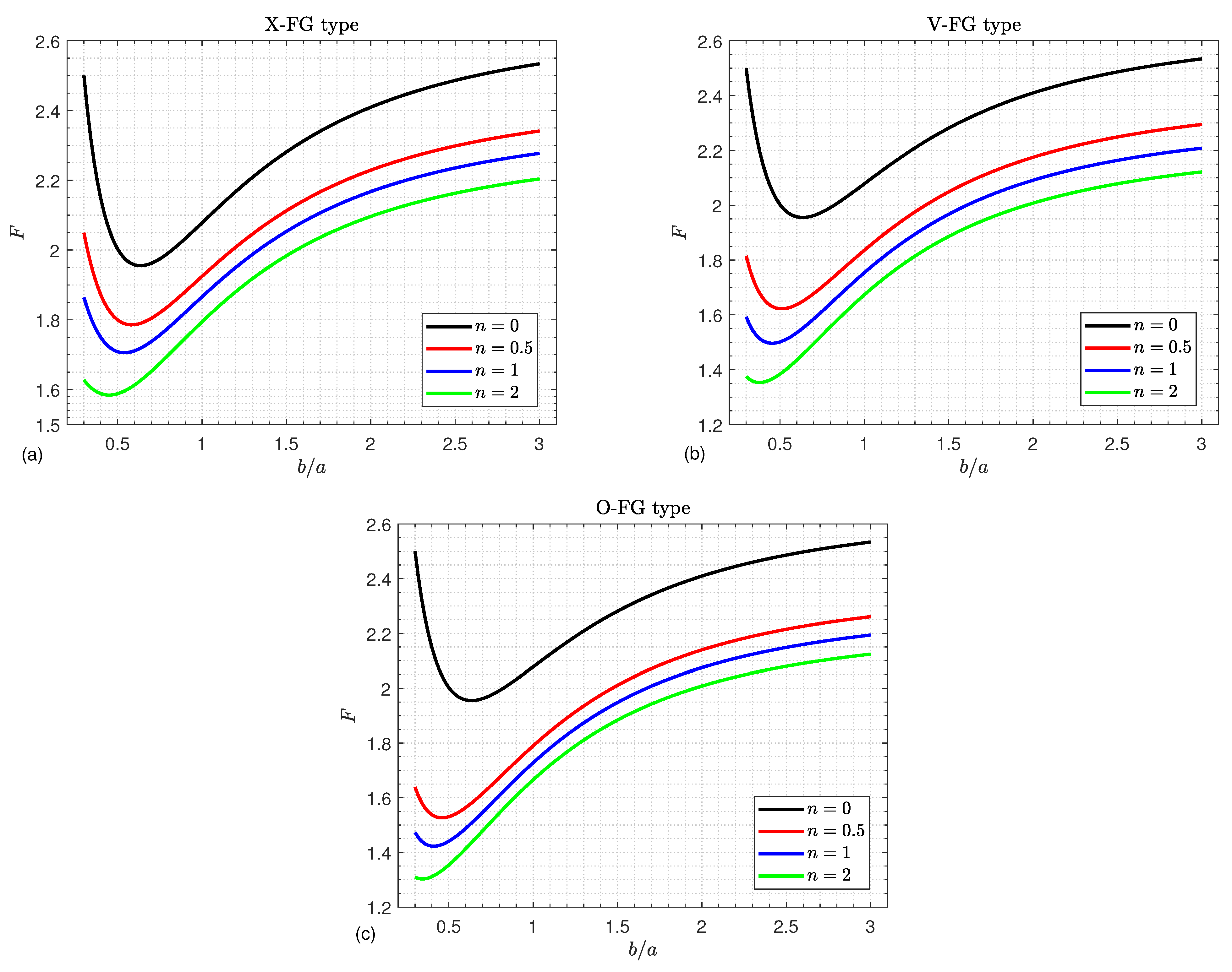
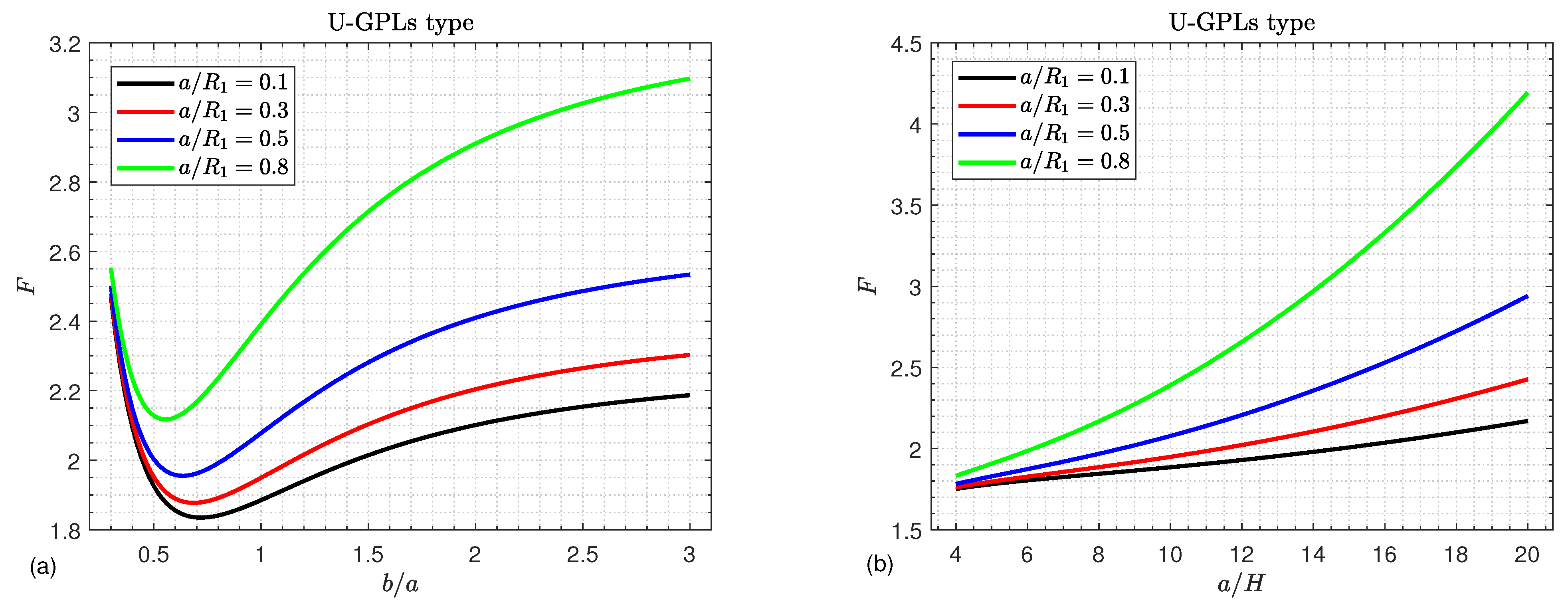
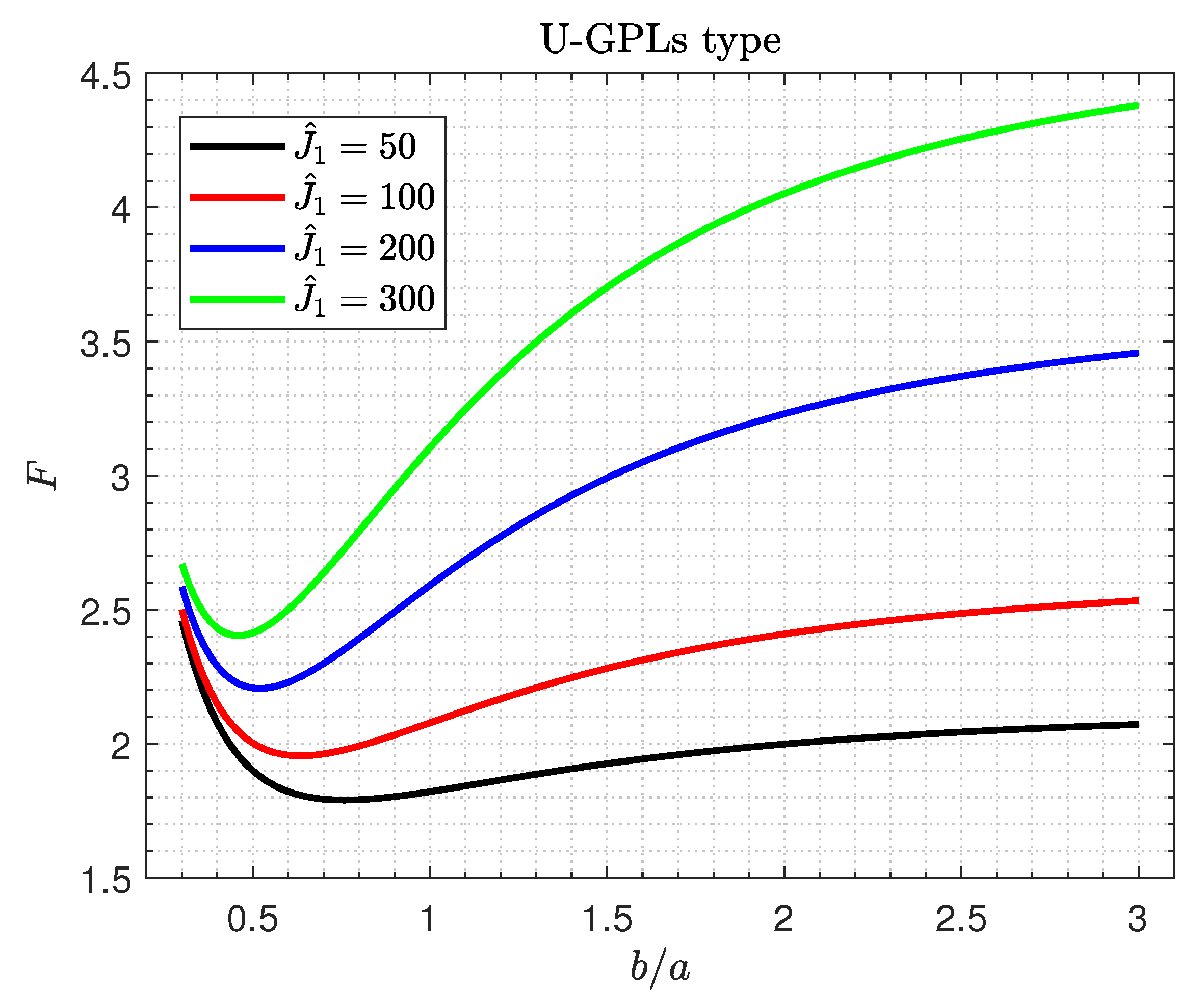
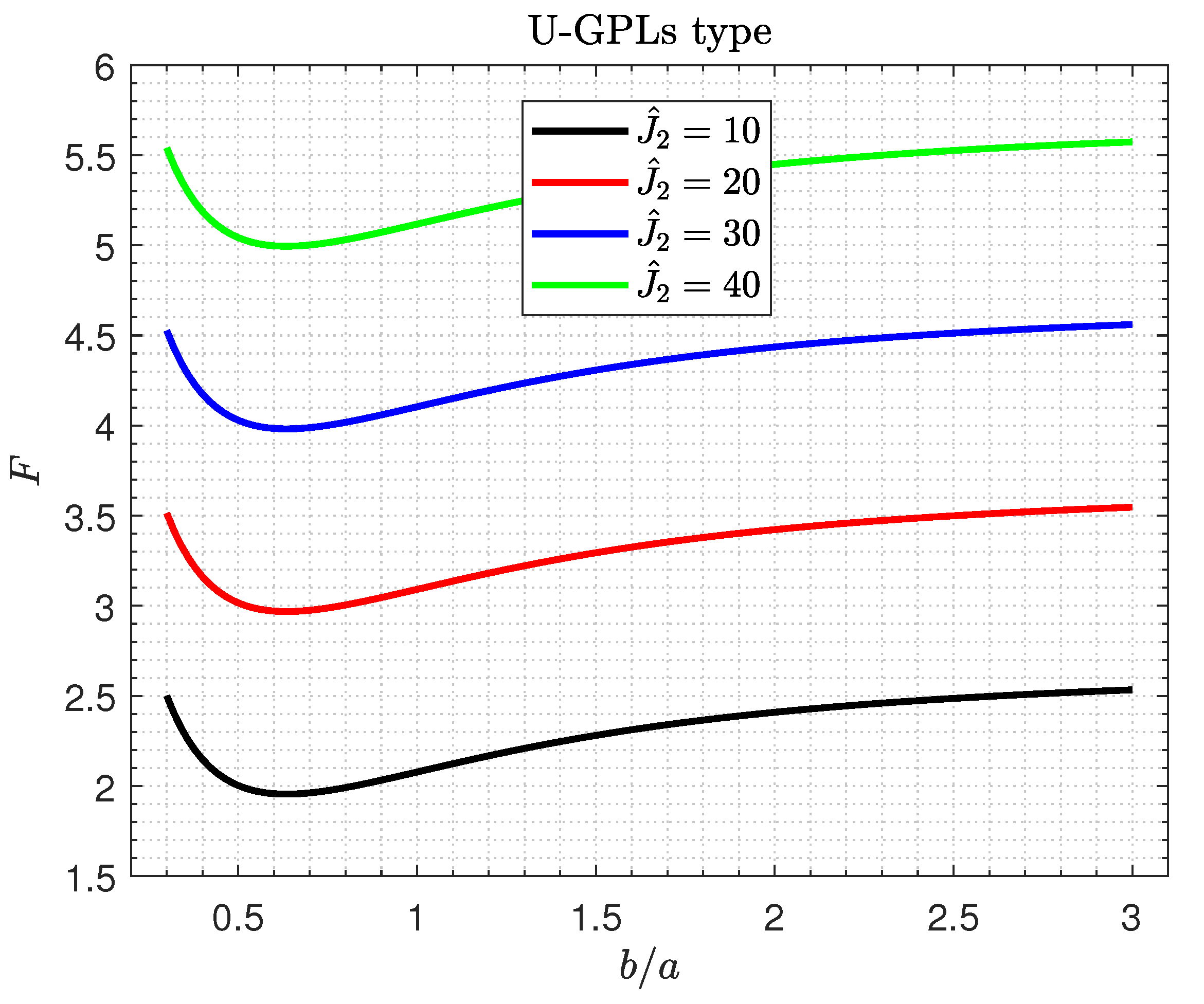
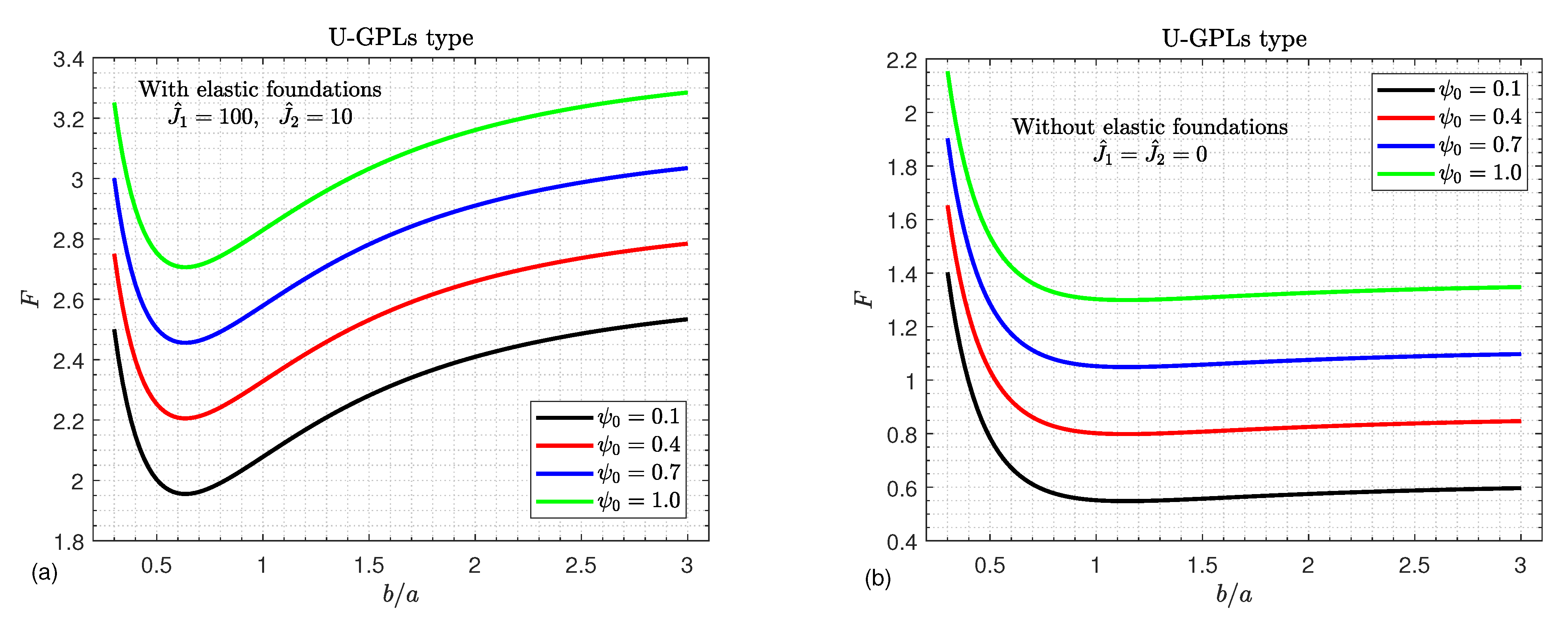
- An increase in the elastic stiffness and the aspect ratio leads to an increase in the critical buckling load.
- The sensitivity performance of the critical buckling load of GPLs/piezoelectric nanocomposite doubly curved shallow shells without elastic foundations is reduced by increasing the external electric voltage.
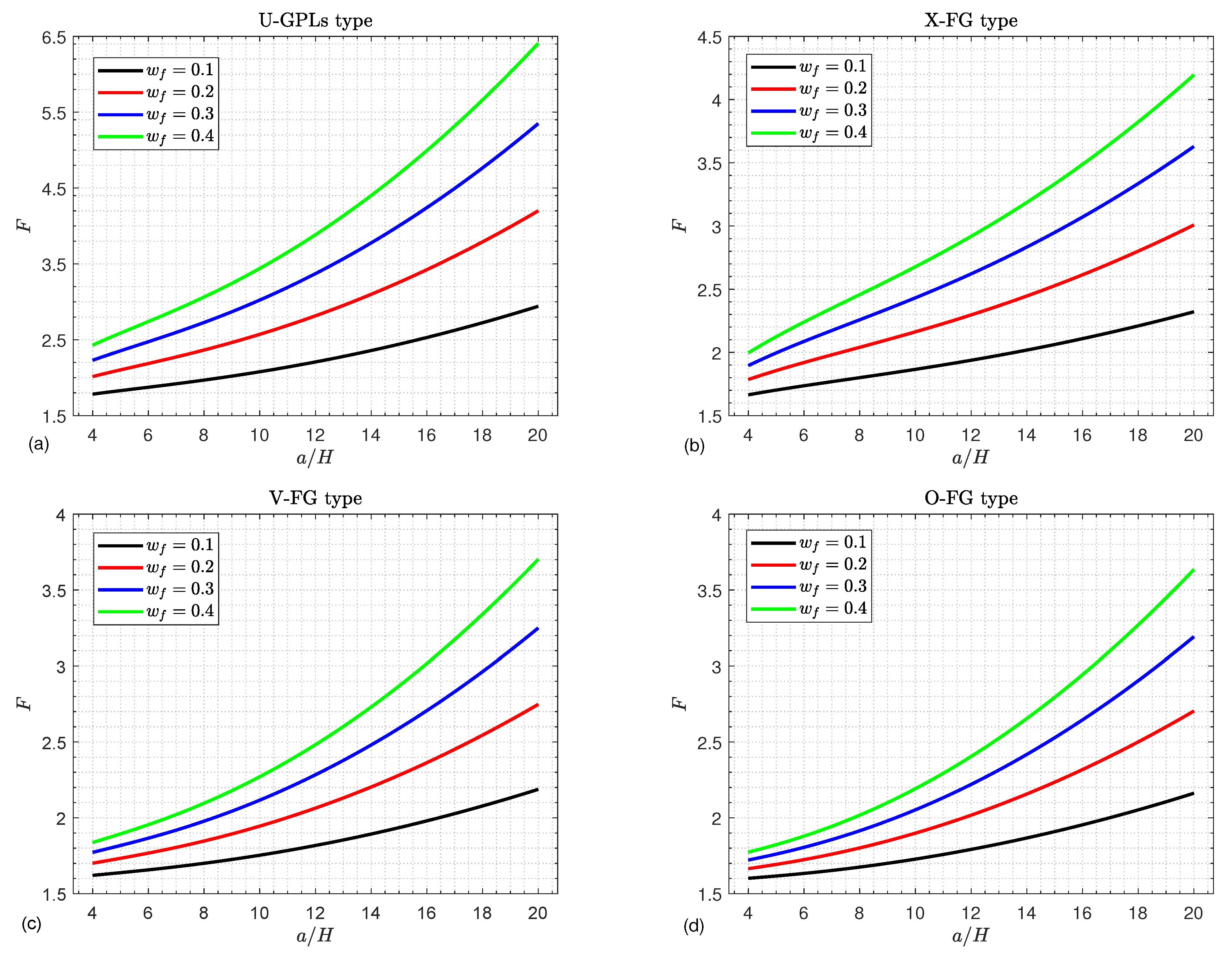
- The critical buckling loads noticeably depend on the dimensions of the shells. They increase as the shallowness ratio and the side-to-thickness ratio increase. Moreover, for small values of the shallowness ratio, the buckling load F may be independent of it.
- Increasing the graphene weight fraction enhances the plate stiffness and this leads to a noticeable increase in the critical buckling load.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Salehi-Khojin, A.; Jalili, N. Buckling of boron nitride nanotube reinforced piezoelectric polymeric composites subject to combined electro-thermo-mechanical loadings. Compos. Sci. Technol. 2008, 68, 1489–1501. [Google Scholar] [CrossRef]
- Sun, J.; Xu, X.; Lim, C.W.; Zhou, Z.; Xiao, S. Accurate thermo-electro-mechanical buckling of shear deformable piezoelectric fiber-reinforced composite cylindrical shells. Compos. Struct. 2016, 141, 221–231. [Google Scholar] [CrossRef]
- Tan, P.; Tong, L. Modeling for the electro-magneto-thermo-elastic properties of piezoelectric-magnetic fiber reinforced composites. Compos. Part A Appl. Sci. Manuf. 2002, 33, 631–645. [Google Scholar] [CrossRef]
- Potts, J.R.; Dreyer, D.R.; Bielawski, C.W.; Ruoff, R.S. Graphene-based polymer nanocomposites. Polymer 2011, 52, 5–25. [Google Scholar] [CrossRef]
- Abazid, M.A. The nonlocal strain gradient theory for hygrothermo-electromagnetic effects on buckling, vibration and wave propagation in piezoelectromagnetic nanoplates. Inter. J. Appl. Mech. 2019, 11, 1950067. [Google Scholar] [CrossRef]
- Sobhy, M. Piezoelectric bending of GPL-reinforced annular and circular sandwich nanoplates with FG porous core integrated with sensor and actuator using DQM. Arch. Civil Mech. Eng. 2021, 21, 78. [Google Scholar] [CrossRef]
- Polley, C.; Distler, T.; Detsch, R.; Lund, H.; Springer, A.; Boccaccini, A.R.; Seitz, H. 3D printing of piezoelectric barium titanate-hydroxyapatite scaffolds with interconnected porosity for bone tissue engineering. Materials 2020, 13, 1773. [Google Scholar] [CrossRef]
- Abouelregal, A.E.; Ahmad, H.; Yao, S.W. Functionally graded piezoelectric medium exposed to a movable heat flow based on a heat equation with a memory-dependent derivative. Materials 2020, 13, 3953. [Google Scholar] [CrossRef] [PubMed]
- Toron, B.; Szperlich, P.; Koziol, M. SbSI composites based on epoxy resin and cellulose for energy harvesting and sensors—The influence of SBSI nanowires conglomeration on piezoelectric properties. Materials 2020, 13, 902. [Google Scholar] [CrossRef]
- Wu, C.C.M.; Kahn, M.; Moy, W. Piezoelectric ceramics with functional gradients: A new application in material design. J. Am. Ceram. Soc. 1996, 79, 809–812. [Google Scholar] [CrossRef]
- El Harti, K.; Rahmoune, M.; Sanbi, M.; Saadani, R. Bentaleb, M.; Rahmoune, M. Dynamic control of euler bernoulli FG porous beam under thermal loading with bonded piezoelectric materials. Ferroelectrics 2020, 558, 104–116. [Google Scholar] [CrossRef]
- Mallek, H.; Jrad, H.; Wali, M.; Dammak, F. Nonlinear dynamic analysis of piezoelectric-bonded FG-CNTR composite structures using an improved FSDT theory. Eng. Comp. 2021, 37, 1389–1407. [Google Scholar] [CrossRef]
- Sobhy, M.; Al Mukahal, F.H.H. Magnetic control of vibrational behavior of smart FG sandwich plates with honeycomb core via a quasi-3D plate theory. Adv. Eng. Mater. 2023. [Google Scholar] [CrossRef]
- Garg, A.; Chalak, H.D.; Belarbi, M.O.; Zenkour, A.M. Hygro-thermo-mechanical based bending analysis of symmetric and unsymmetric power-law, exponential and sigmoidal FG sandwich beams. Mech. Advan Mater. Struct. 2021, 29, 4523–4545. [Google Scholar] [CrossRef]
- Meyer, J.C.; Geim, A.K.; Katsnelson, M.I.; Novoselov, K.S.; Booth, T.J.; Roth, S. The structure of suspended graphene sheets. Nature 2007, 446, 60–63. [Google Scholar] [CrossRef]
- Rafiee, M.A.; Rafiee, J.; Yu, Z.Z.; Koratkar, N. Buckling resistant graphene nanocomposites. Appl. Phys. Lett. 2009, 95, 223103. [Google Scholar] [CrossRef]
- Sobhy, M.; Abazid, M.A. Dynamic and instability analyses of FG graphene-reinforced sandwich deep curved nanobeams with viscoelastic core under magnetic field effect. Compo Part B Eng. 2019, 174, 106966. [Google Scholar] [CrossRef]
- Abazid, M.A. 2D magnetic field effect on the thermal buckling of metal foam nanoplates reinforced with FG-GPLs lying on pasternak foundation in humid environment. Euro. Phys. J. Plus 2020, 135, 910. [Google Scholar] [CrossRef]
- Abbasipour, M.; Khajavi, R.; Yousefi, A.A.; Yazdanshenas, M.E.; Razaghian, F. The piezoelectric response of electrospun PVDF nanofibers with graphene oxide, graphene, and halloysite nanofillers: A comparative study. J. Mater. Sci. Mater. Elect. 2017, 28, 15942–15952. [Google Scholar] [CrossRef]
- Liao, Y.; Li, Z.; Xia, W. Size-dependent structural behaviors of crumpled graphene sheets. Carbon 2021, 174, 148–157. [Google Scholar] [CrossRef]
- Maity, N.; Mandal, A.; Nandi, A.K. Hierarchical nanostructured polyaniline functionalized graphene/poly (vinylidene fluoride) composites for improved dielectric performances. Polymer 2016, 103, 83–97. [Google Scholar] [CrossRef]
- Olabi, A.G.; Abdelkareem, M.A.; Wilberforce, T.; Sayed, E.T. Application of graphene in energy storage device—A review. Renew. Sustain. Energy Rev. 2021, 135, 110026. [Google Scholar] [CrossRef]
- Mao, J.J.; Zhang, W. Linear and nonlinear free and forced vibrations of graphene reinforced piezoelectric composite plate under external voltage excitation. Compos. Struct. 2018, 203, 551–565. [Google Scholar] [CrossRef]
- Mao, J.J.; Zhang, W. Buckling and post-buckling analyses of functionally graded graphene reinforced piezoelectric plate subjected to electric potential and axial forces. Compos. Struct. 2019, 216, 392–405. [Google Scholar] [CrossRef]
- Sobhani, E.; Avcar, M. Natural frequency analysis of imperfect GNPRN conical shell, cylindrical shell, and annular plate structures resting on Winkler-Pasternak Foundations under arbitrary boundary conditions. Eng. Anal. Bound. Elem. 2022, 144, 145–164. [Google Scholar] [CrossRef]
- Salmani, R.; Gholami, R.; Ansari, R.; Fakhraie, M. Analytical investigation on the nonlinear postbuckling of functionally graded porous cylindrical shells reinforced with graphene nanoplatelets. Euro. Phys. J. Plus 2021, 136, 53. [Google Scholar] [CrossRef]
- Wang, M.; Xu, Y.-G.; Qiao, P.; Li, Z.-M. Buckling and free vibration analysis of shear deformable graphene-reinforced composite laminated plates. Compos. Struct. 2022, 280, 114854. [Google Scholar] [CrossRef]
- Wang, Y.; Feng, C.; Santiuste, C.; Zhao, Z.; Yang, J. Buckling and postbuckling of dielectric composite beam reinforced with graphene platelets (gpls). Aerosp. Sci. Technol. 2019, 91, 208–218. [Google Scholar] [CrossRef]
- Wang, Y.; Feng, C.; Yang, J.; Zhou, D.; Liu, W. Static response of functionally graded graphene platelet–reinforced composite plate with dielectric property. J. Intell. Mater. Syst. Struct. 2020, 31, 2211–2228. [Google Scholar] [CrossRef]
- Duc, N.D.; Quan, T.Q.; Laut, V.D. Nonlinear dynamic analysis and vibration of shear deformable piezoelectric fgm double curved shallow shells under damping-thermo-electro-mechanical loads. Compos. Struct. 2015, 125, 29–40. [Google Scholar] [CrossRef]
- Kiani, Y.; Akbarzadeh, A.H.; Chen, Z.T.; Eslami, M.R. Static and dynamic analysis of an fgm doubly curved panel resting on the pasternak-type elastic foundation. Compos. Struct. 2012, 94, 2474–2484. [Google Scholar] [CrossRef]
- Amabili, M. A new third-order shear deformation theory with non-linearities in shear for static and dynamic analysis of laminated doubly curved shells. Compos. Struct. 2015, 128, 260–273. [Google Scholar] [CrossRef]
- Sobhy, M. Magneto-electro-thermal bending of fg-graphene reinforced polymer doubly-curved shallow shells with piezoelectromagnetic faces. Compos. Struct. 2018, 203, 844–860. [Google Scholar] [CrossRef]
- Esmaeili, H.R.; Kiani, Y. Vibrations of graphene platelet reinforced composite doubly curved shells subjected to thermal shock. Mech. Based Des. Struct. Mach. 2022. [Google Scholar] [CrossRef]
- Ngoc, V.; Hoangc, V.; Tien, N.D.; Ninh, D.G.; Thang, V.T.; Truong, D.V. Nonlinear dynamics of functionally graded graphene nanoplatelet reinforced polymer doubly-curved shallow shells resting on elastic foundation using a micromechanical model. J. Sandw. Struct. Mater. 2021, 23, 3250–3279. [Google Scholar]
- Karimiasl, M.; Ebrahimi, F.; Akgöz, B. Buckling and post-buckling responses of smart doubly curved composite shallow shells embedded in sma fiber under hygro-thermal loading. Compos. Struct. 2019, 223, 110988. [Google Scholar] [CrossRef]
- Salehipour, H.; Emadi, S.; Tayebikhorami, S.; Shahmohammadi, M.A. A semi-analytical solution for dynamic stability analysis of nanocomposite/fibre-reinforced doubly-curved panels resting on the elastic foundation in thermal environment. Eur. Phys. J. Plus 2022, 137, 2. [Google Scholar] [CrossRef]
- Feng, S.; Kitipornchai, C.; Yang, J. Nonlinear free vibration of functionally graded polymer composite beams reinforced with graphene nanoplatelets (gpls). Eng. Struct. 2017, 140, 110–119. [Google Scholar] [CrossRef]
- Song, S.; Kitipornchai, M.; Yang, J. Free and forced vibrations of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Struct. 2017, 159, 579–588. [Google Scholar] [CrossRef]
- Halpin, J.C.; Kardos, J.L. The halpin-tsai equations: A review. Polym. Eng. Sci. 1976, 16, 344–352. [Google Scholar]
- Sobhy, M.; Zenkour, A.M. Vibration analysis of functionally graded graphene platelet-reinforced composite doubly-curved shallow shells on elastic foundations. Steel Compos. Struct. 2019, 33, 195–208. [Google Scholar]
- Al Mukahal, F.H.H.; Sobhy, M. Wave propagation and free vibration of fg graphene platelets sandwich curved beam with auxetic core resting on viscoelastic foundation via dqm. Arch. Civ. Mech. Eng. 2022, 22, 12. [Google Scholar] [CrossRef]
- Sobhy, M. Analytical buckling temperature prediction of fg piezoelectric sandwich plates with lightweight core. Mater. Res. Express 2021, 8, 095704. [Google Scholar] [CrossRef]
- Sobhy, M. Stability analysis of smart FG sandwich plates with auxetic core. Int. J. Appl. Mech. 2021, 13, 2150093. [Google Scholar] [CrossRef]
- Oktem, A.S.; Mantari, J.L.; Soares, C.G. Static response of functionally graded plates and doubly-curved shells based on a higher order shear deformation theory. Eur. J. -Mech.-A/Solids 2012, 36, 163–172. [Google Scholar] [CrossRef]
- Reddy, J.N.; Liu, C.F. A higher-order shear deformation theory of laminated elastic shells. Int. J. Eng. Sci. 1985, 23, 319–330. [Google Scholar] [CrossRef]
- Zhang, S.; Xia, R.; Lebrun, L.; Anderson, D.; Shrout, T.R. Piezoelectric materials for high power, high temperature applications. Mater. Lett. 2005, 59, 3471–3475. [Google Scholar] [CrossRef]
- Meyers, C.A.; Hyer, M.W. Thermal buckling and postbuckling of symmetrically laminated composite plates. J. Therm. Stress. 1991, 14, 519–540. [Google Scholar] [CrossRef]
- Shariat, B.A.S.; Eslami, M.R. Buckling of thick functionally graded plates under mechanical and thermal loads. Compos. Struct. 2007, 78, 433–439. [Google Scholar] [CrossRef]
- Sobhy, M. Size-dependent hygro-thermal buckling of porous fgm sandwich microplates and microbeams using a novel four-variable shear deformation theory. Int. J. Appl. Mech. 2020, 12, 2050017. [Google Scholar] [CrossRef]
- Matsunaga, H. Vibration and stability of thick simply supported shallow shells subjected to in-plane stresses. J. Sound Vib. 1999, 225, 41–60. [Google Scholar] [CrossRef]
- Matsunaga, H. Free vibration and stability of functionally graded shallow shells according to a 2D higher-order deformation theory. Compos. Struct. 2008, 84, 132–146. [Google Scholar] [CrossRef]
| Load Type | Ref. [51] | Present | ||
|---|---|---|---|---|
| Uniaxial | 0 | 0 | 3.7412 | 3.7866 |
| 0.2 | 0.2 | 4.1630 | 4.2350 | |
| 0.2 | 0 | 3.8391 | 3.8987 | |
| 0.2 | −0.2 | 3.7100 | 3.7866 | |
| Biaxial | 0 | 0 | 1.8706 | 1.8933 |
| 0.2 | 0.2 | 2.0815 | 2.1175 | |
| 0.2 | 0 | 1.9195 | 1.9493 | |
| 0.2 | −0.2 | 1.8550 | 1.8933 |
| Type | Ref. [52] | Present | ||||
|---|---|---|---|---|---|---|
| CST | FSDT | HST | ||||
| 2 | Plate | 0 | 0.64040 | 0.34790 | 0.35810 | 0.37947 |
| Sph. | 0.5 | 0.64460 | 0.36270 | 0.36790 | 0.40480 | |
| 1 | 0.65560 | 0.40230 | 0.39480 | 0.48079 | ||
| Cyl. | 0.5 | 0.61430 | 0.34440 | 0.35310 | 0.38580 | |
| 1 | 0.56850 | 0.33560 | 0.34030 | 0.40480 | ||
| Hyp. | 0.5 | 0.56440 | 0.32200 | 0.33110 | 0.37947 | |
| 5 | Plate | 0 | 0.13570 | 0.11300 | 0.11400 | 0.11808 |
| Sph. | 0.5 | 0.15610 | 0.13430 | 0.13410 | 0.14341 | |
| 1 | 0.21190 | 0.19270 | 0.18950 | 0.21940 | ||
| Cyl. | 0.5 | 0.13890 | 0.11680 | 0.11740 | 0.12441 | |
| 1 | 0.14760 | 0.12710 | 0.12690 | 0.14341 | ||
| Hyp. | 0.5 | 0.12840 | 0.10700 | 0.10790 | 0.11808 | |
| 10 | Plate | 0 | 0.03557 | 0.03372 | 0.03381 | 0.03423 |
| Sph. | 0.5 | 0.05921 | 0.05774 | 0.05720 | 0.05956 | |
| 1 | 0.12420 | 0.12260 | 0.12160 | 0.13555 | ||
| Cyl. | 0.5 | 0.04104 | 0.03924 | 0.03924 | 0.04056 | |
| 1 | 0.05625 | 0.05457 | 0.05435 | 0.05956 | ||
| Hyp. | 0.5 | 0.03381 | 0.03205 | 0.03214 | 0.03423 | |
| Uniaxial | Biaxial | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| U-GPLs | X-FG | V-FG | O-FG | U-GPLs | X-FG | V-FG | O-FG | |||
| 5 | 0.5 | 1 | 6.4683 | 6.1167 | 5.9348 | 5.8803 | 1.9903 | 1.8821 | 1.8261 | 1.0691 |
| 0.5 | −1 | 6.2759 | 6.0204 | 5.8394 | 5.7840 | 1.9311 | 1.8524 | 1.7968 | 1.0516 | |
| 0.5 | 0 | 6.3507 | 6.0578 | 5.8764 | 5.8215 | 1.9541 | 1.8640 | 1.8081 | 1.0585 | |
| 0 | 0 | 6.2425 | 6.0037 | 5.8232 | 5.7673 | 1.9208 | 1.8473 | 1.7918 | 1.0486 | |
| 10 | 0.5 | 1 | 10.1233 | 8.0287 | 7.7431 | 7.6875 | 3.1149 | 2.4704 | 2.3825 | 1.3977 |
| 0.5 | −1 | 6.6440 | 6.2881 | 6.0095 | 5.9469 | 2.0443 | 1.9348 | 1.8491 | 1.0813 | |
| 0.5 | 0 | 6.9433 | 6.4378 | 6.1582 | 6.0966 | 2.1364 | 1.9809 | 1.8948 | 1.1085 | |
| 0 | 0 | 6.5104 | 6.2212 | 5.9437 | 5.8801 | 2.0032 | 1.9142 | 1.8288 | 1.0691 | |
| 20 | 0.5 | 1 | 21.7943 | 13.9199 | 13.5845 | 13.5413 | 6.7059 | 4.2831 | 4.1798 | 2.4621 |
| 0.5 | −1 | 7.8771 | 6.9575 | 6.6422 | 6.5788 | 2.4237 | 2.1408 | 2.0438 | 1.1961 | |
| 0.5 | 0 | 9.0743 | 7.5564 | 7.2385 | 7.1777 | 2.7921 | 2.3250 | 2.2272 | 1.3050 | |
| 0 | 0 | 7.3426 | 6.6901 | 6.3773 | 6.3114 | 2.2593 | 2.0585 | 1.9622 | 1.1475 | |
| 30 | 0.5 | 1 | 26.9927 | 16.5649 | 16.2176 | 16.1786 | 8.3054 | 5.0969 | 4.9900 | 2.9416 |
| 0.5 | −1 | 9.9048 | 8.0162 | 7.6922 | 7.6299 | 3.0476 | 2.4665 | 2.3668 | 1.3873 | |
| 0.5 | 0 | 12.5985 | 9.3638 | 9.0350 | 8.9775 | 3.8765 | 2.8812 | 2.7800 | 1.6323 | |
| 0 | 0 | 8.7023 | 7.4147 | 7.0946 | 7.0283 | 2.6776 | 2.2814 | 2.1829 | 1.2779 | |
| 40 | 0.5 | 1 | 36.2940 | 21.2738 | 20.9154 | 20.8847 | 11.1674 | 6.5458 | 6.4355 | 3.7972 |
| 0.5 | −1 | 12.7400 | 9.4903 | 9.1618 | 9.1012 | 3.9200 | 2.9201 | 2.8190 | 1.6548 | |
| 0.5 | 0 | 17.5287 | 11.8859 | 11.5502 | 11.4969 | 5.3934 | 3.6572 | 3.5539 | 2.0903 | |
| 0 | 0 | 10.6021 | 8.4208 | 8.0981 | 8.0317 | 3.2622 | 2.5910 | 2.4917 | 1.4603 | |
| Uniaxial | Biaxial | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| U-GPLs | X-FG | V-FG | O-FG | U-GPLs | X-FG | V-FG | O-FG | |||
| 0.1 | 0.5 | 1 | 7.4137 | 6.6731 | 6.3923 | 6.3319 | 2.2811 | 2.0533 | 1.9669 | 1.9483 |
| 0.5 | −1 | 6.6440 | 6.2881 | 6.0095 | 5.9469 | 2.0443 | 1.9348 | 1.8491 | 1.8298 | |
| 0.5 | 0 | 6.9433 | 6.4378 | 6.1582 | 6.0966 | 2.1364 | 1.9809 | 1.8948 | 1.8759 | |
| 0 | 0 | 6.5104 | 6.2212 | 5.9437 | 5.8801 | 2.0032 | 1.9142 | 1.8288 | 1.8093 | |
| 0.2 | 0.5 | 1 | 9.0369 | 7.6087 | 7.0641 | 6.9572 | 2.7806 | 2.3411 | 2.1736 | 2.1407 |
| 0.5 | −1 | 7.5612 | 6.8805 | 6.3438 | 6.2290 | 2.3265 | 2.1171 | 1.9519 | 1.9166 | |
| 0.5 | 0 | 8.1351 | 7.1637 | 6.6233 | 6.5122 | 2.5031 | 2.2042 | 2.0379 | 2.0037 | |
| 0 | 0 | 7.3050 | 6.7541 | 6.2212 | 6.1026 | 2.2477 | 2.0782 | 1.9142 | 1.8777 | |
| 0.3 | 0.5 | 1 | 10.5266 | 8.4577 | 7.6661 | 7.5214 | 3.2389 | 2.6024 | 2.3588 | 2.3143 |
| 0.5 | −1 | 8.3942 | 7.4157 | 6.6401 | 6.4794 | 2.5828 | 2.2818 | 2.0431 | 1.9937 | |
| 0.5 | 0 | 9.2235 | 7.8210 | 7.0379 | 6.8846 | 2.8380 | 2.4065 | 2.1655 | 2.1183 | |
| 0 | 0 | 8.0240 | 7.2348 | 6.4669 | 6.2985 | 2.4689 | 2.2261 | 1.9898 | 1.9380 | |
| 0.4 | 0.5 | 1 | 11.9005 | 9.2328 | 8.2095 | 8.0336 | 3.6617 | 2.8409 | 2.5260 | 2.4719 |
| 0.5 | −1 | 9.1558 | 7.9025 | 6.9049 | 6.7033 | 2.8172 | 2.4315 | 2.1246 | 2.0626 | |
| 0.5 | 0 | 10.2232 | 8.4198 | 7.4103 | 7.2207 | 3.1456 | 2.5907 | 2.2801 | 2.2217 | |
| 0 | 0 | 8.6793 | 7.6715 | 6.6861 | 6.4724 | 2.6706 | 2.3605 | 2.0573 | 1.9915 | |
| 0.5 | 0.5 | 1 | 13.1732 | 9.9438 | 8.7028 | 8.5011 | 4.0533 | 3.0596 | 2.6778 | 2.6157 |
| 0.5 | −1 | 9.8561 | 8.3477 | 7.1431 | 6.9050 | 3.0327 | 2.5685 | 2.1979 | 2.1246 | |
| 0.5 | 0 | 11.1461 | 8.9684 | 7.7470 | 7.5257 | 3.4296 | 2.7595 | 2.3837 | 2.3156 | |
| 0 | 0 | 9.2803 | 8.0706 | 6.8832 | 6.6279 | 2.8555 | 2.4833 | 2.1179 | 2.0394 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al Mukahal, F.H.H.; Abazid, M.A.; Sobhy, M. Investigating Electromechanical Buckling Response of FG-GPL-Reinforced Piezoelectric Doubly Curved Shallow Shells Embedded in an Elastic Substrate. Materials 2023, 16, 2975. https://doi.org/10.3390/ma16082975
Al Mukahal FHH, Abazid MA, Sobhy M. Investigating Electromechanical Buckling Response of FG-GPL-Reinforced Piezoelectric Doubly Curved Shallow Shells Embedded in an Elastic Substrate. Materials. 2023; 16(8):2975. https://doi.org/10.3390/ma16082975
Chicago/Turabian StyleAl Mukahal, Fatemah H. H., Mohammad Alakel Abazid, and Mohammed Sobhy. 2023. "Investigating Electromechanical Buckling Response of FG-GPL-Reinforced Piezoelectric Doubly Curved Shallow Shells Embedded in an Elastic Substrate" Materials 16, no. 8: 2975. https://doi.org/10.3390/ma16082975
APA StyleAl Mukahal, F. H. H., Abazid, M. A., & Sobhy, M. (2023). Investigating Electromechanical Buckling Response of FG-GPL-Reinforced Piezoelectric Doubly Curved Shallow Shells Embedded in an Elastic Substrate. Materials, 16(8), 2975. https://doi.org/10.3390/ma16082975







