Electrostatically Interacting Wannier Qubits in Curved Space
Abstract
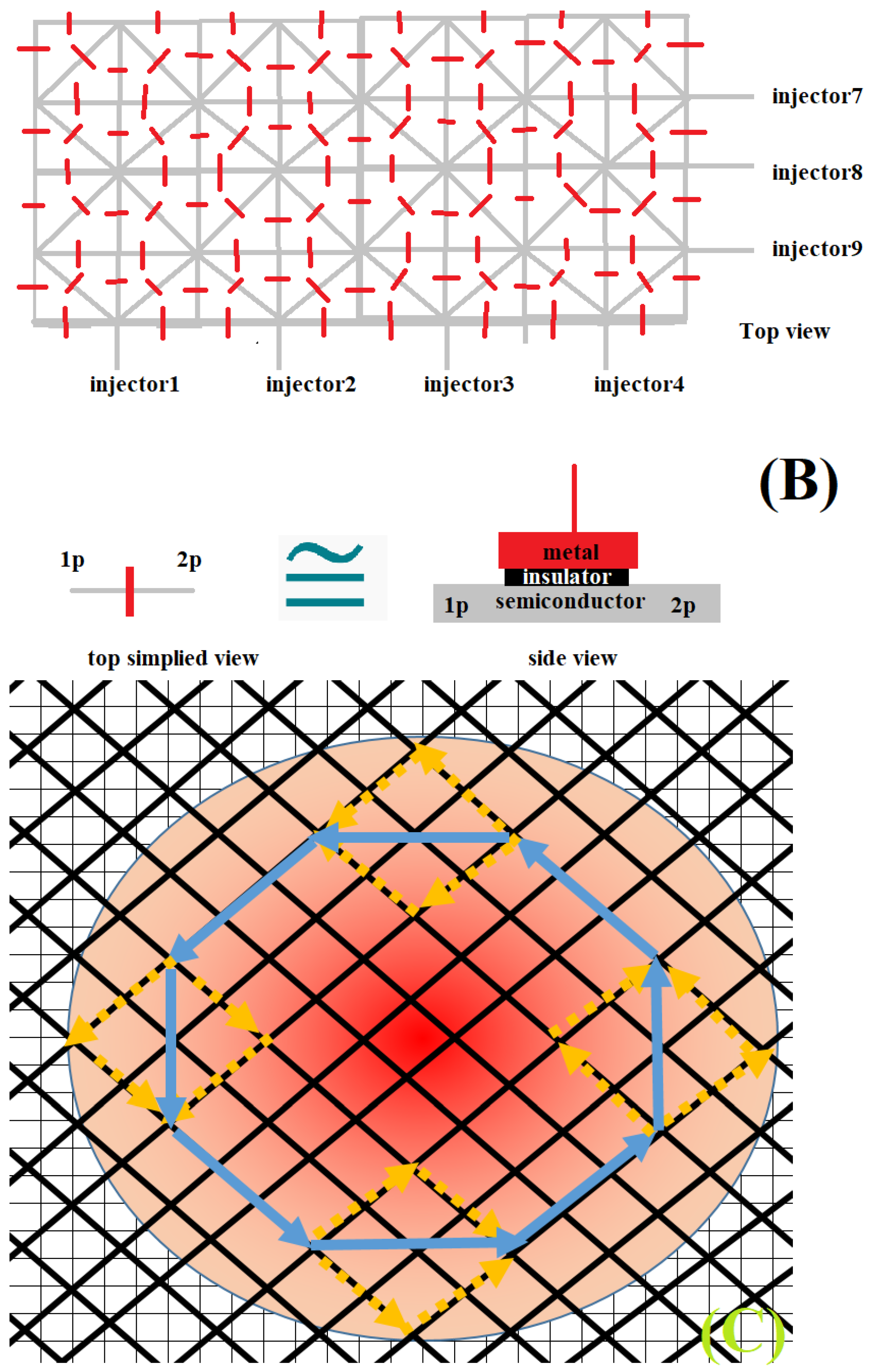
1. Classical vs. Quantum Picture in Programmable Matter
2. Philosophy Behind Charged-Based Classical and Quantum Logic
| Modality | Superconducting | Trapped Ion | Photonic | Neutral Atom | Silicon Spin |
|---|---|---|---|---|---|
| # Qubits | 127Q | 32Q | 20 Photons: 216 Qumode | 100Q | 2Q |
| T2 Lifetime | Short: 15 s–256 s | Long: 0.2 s–50 s | Short: 150 s | Long: 0.2 s–10 s | Mixed: 1 s–0.5 s |
| 2Q Gate Fidelity | High: 99–99.7% | High: 98.5–99.92% | Promising: 98% | Promising: 97.4% | Promising: 90–98% |
| Gate Speed | Fast: 10 ns–196 ns | Mixed: 1 s–3 ms | Very Fast: 1 ns | Medium: 1 s | Fast: 0.8–80 ns |
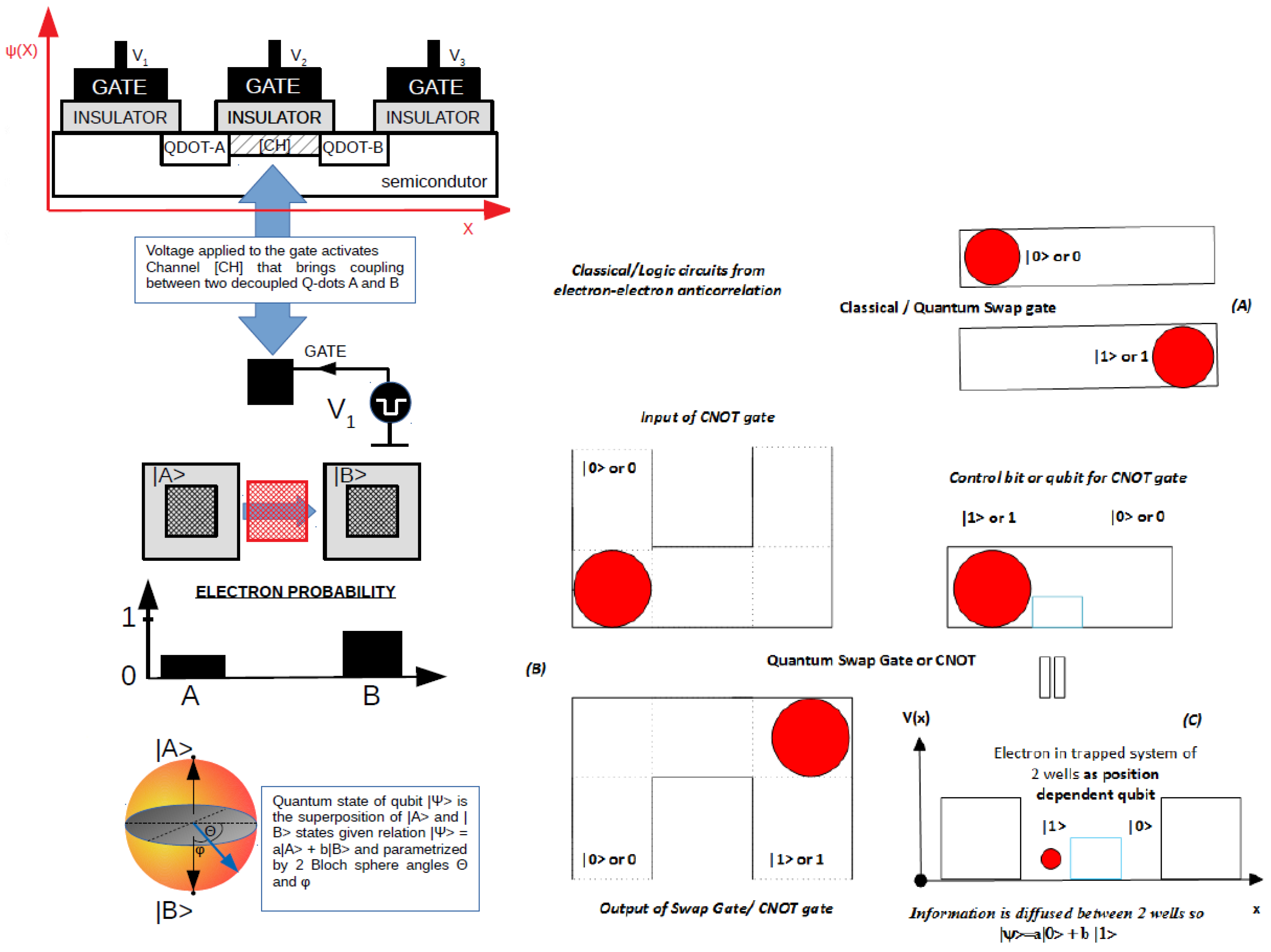
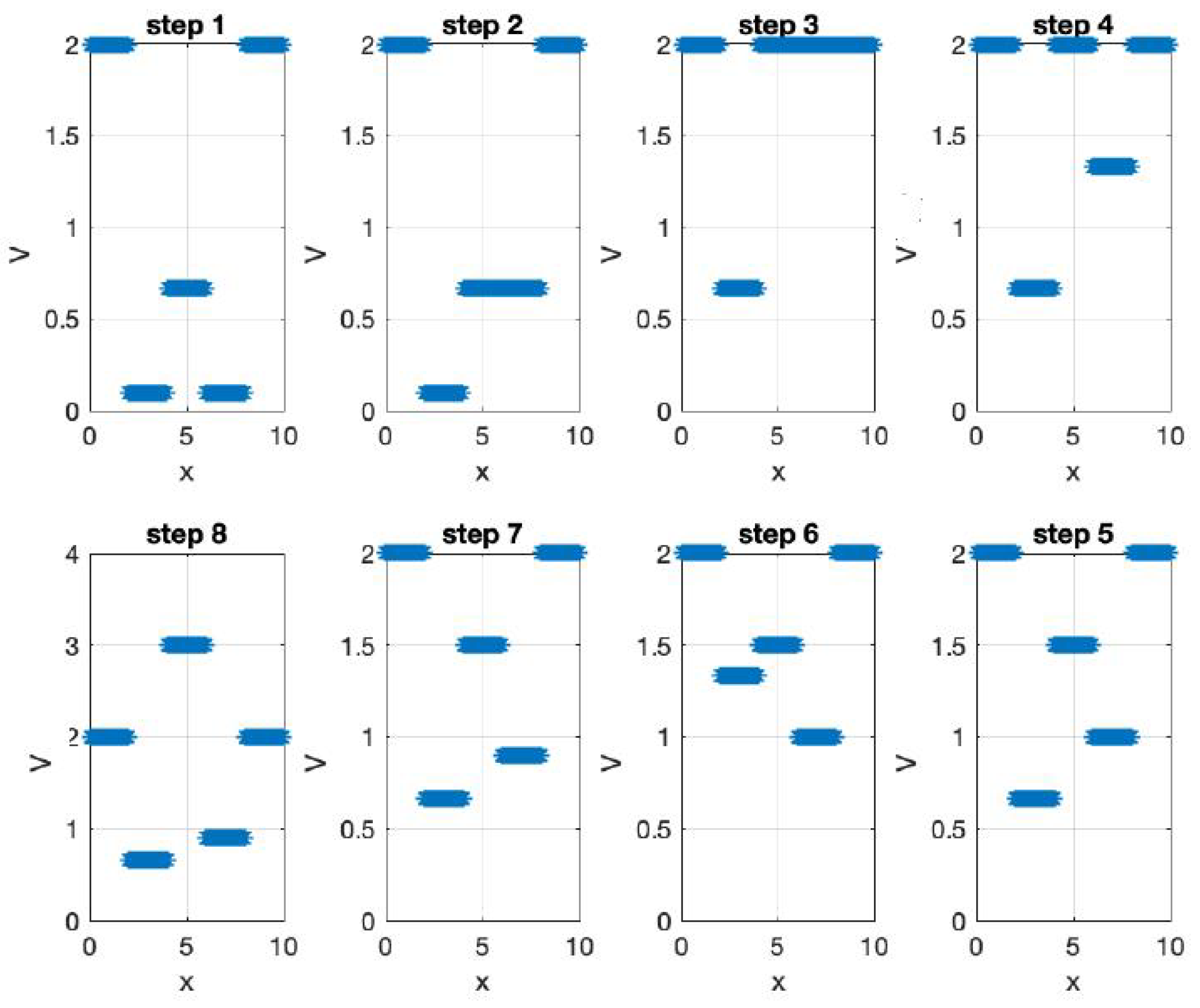
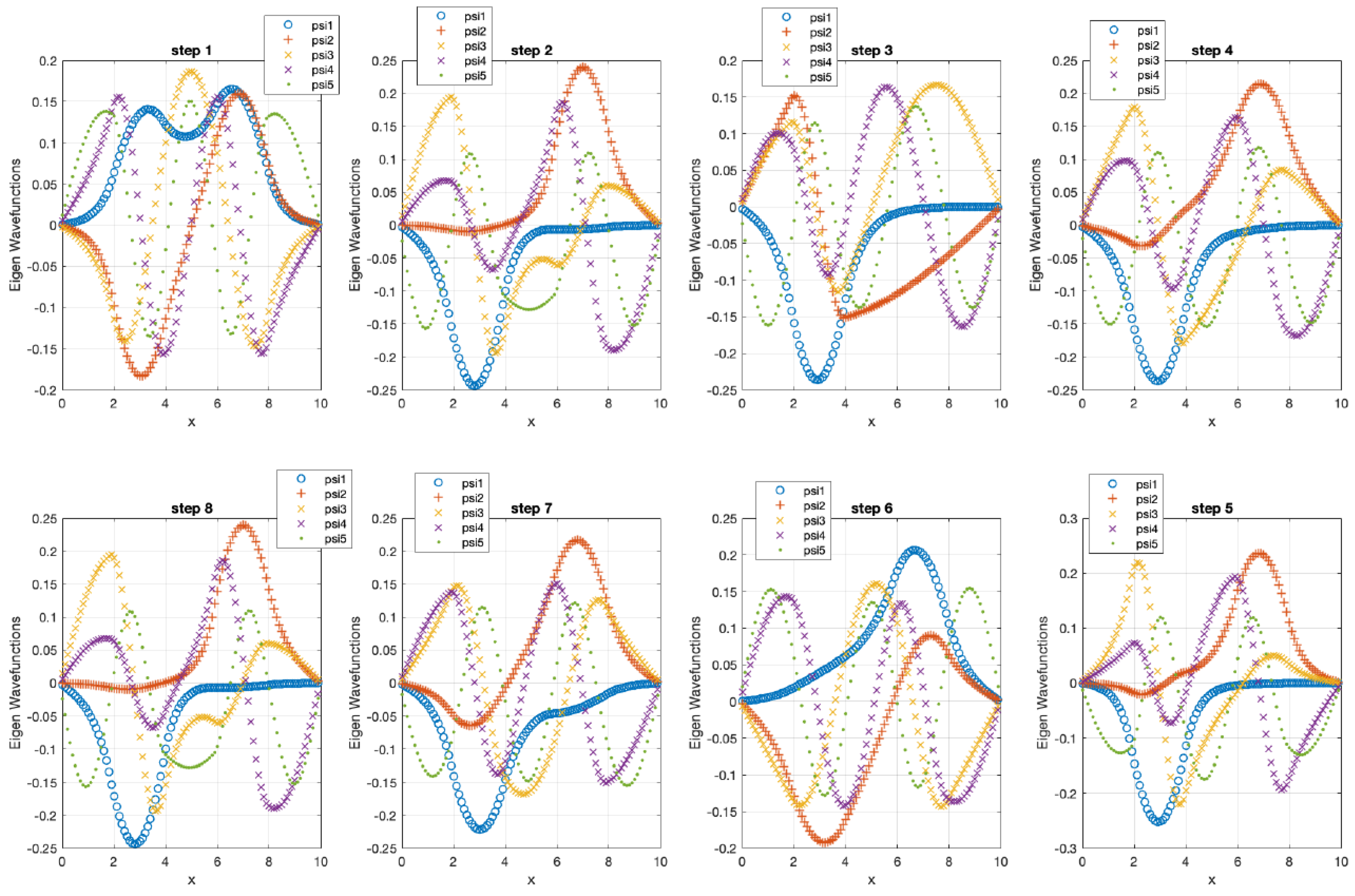

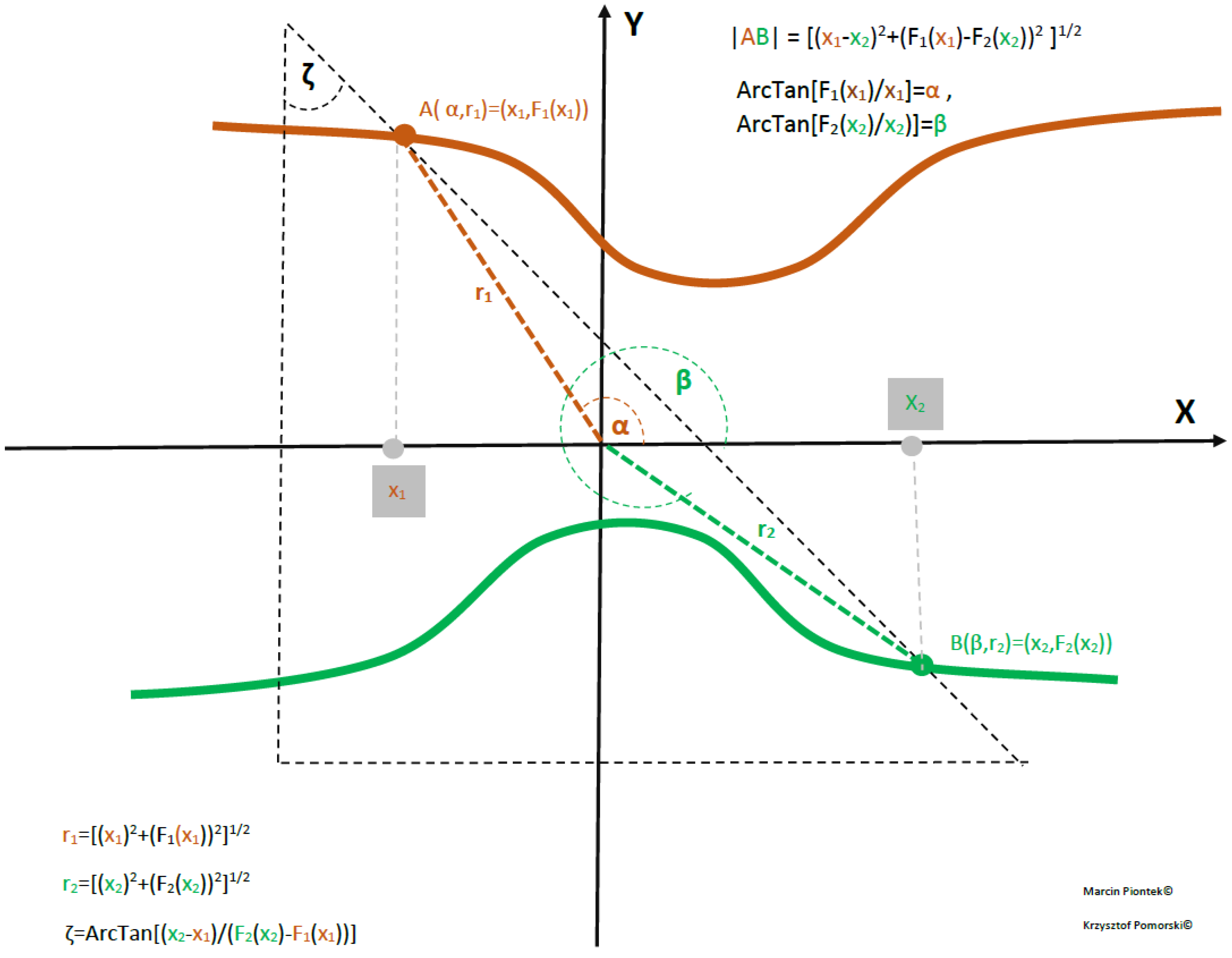
3. From Schrödinger to Wannier Functions
3.1. Equivalence of Wannier and Schrödinger Formalism
3.2. Rabi Oscillations in the Tight-Binding Model
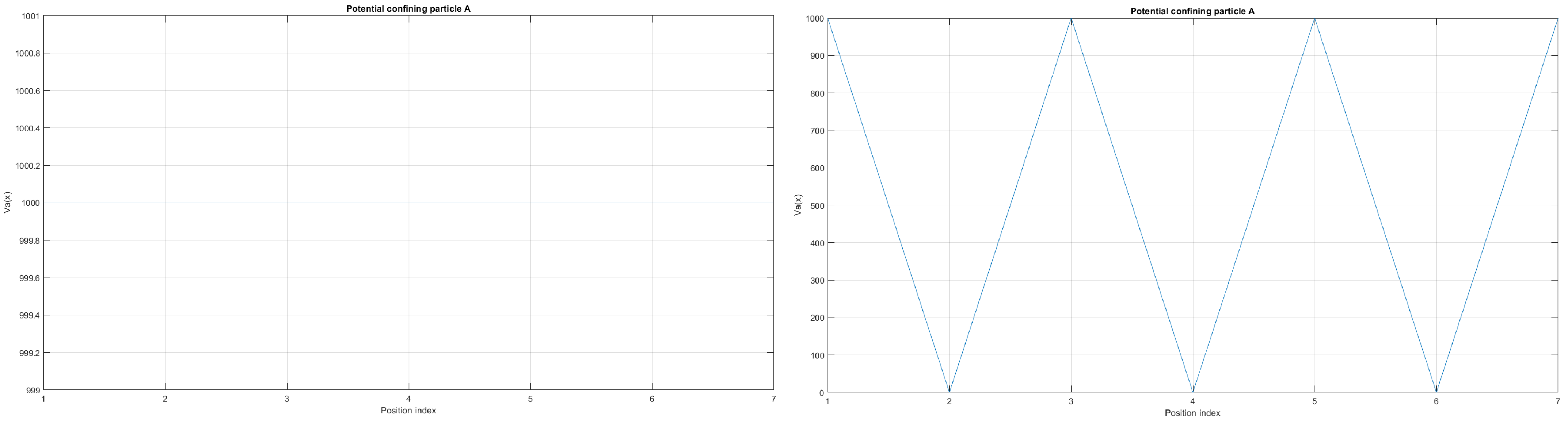
4. Open Curvy Loops Confining a Single Electron in Cartesian Coordinates in Schrödinger Formalism
4.1. Case of Deformed Curvy Wannier Qubit
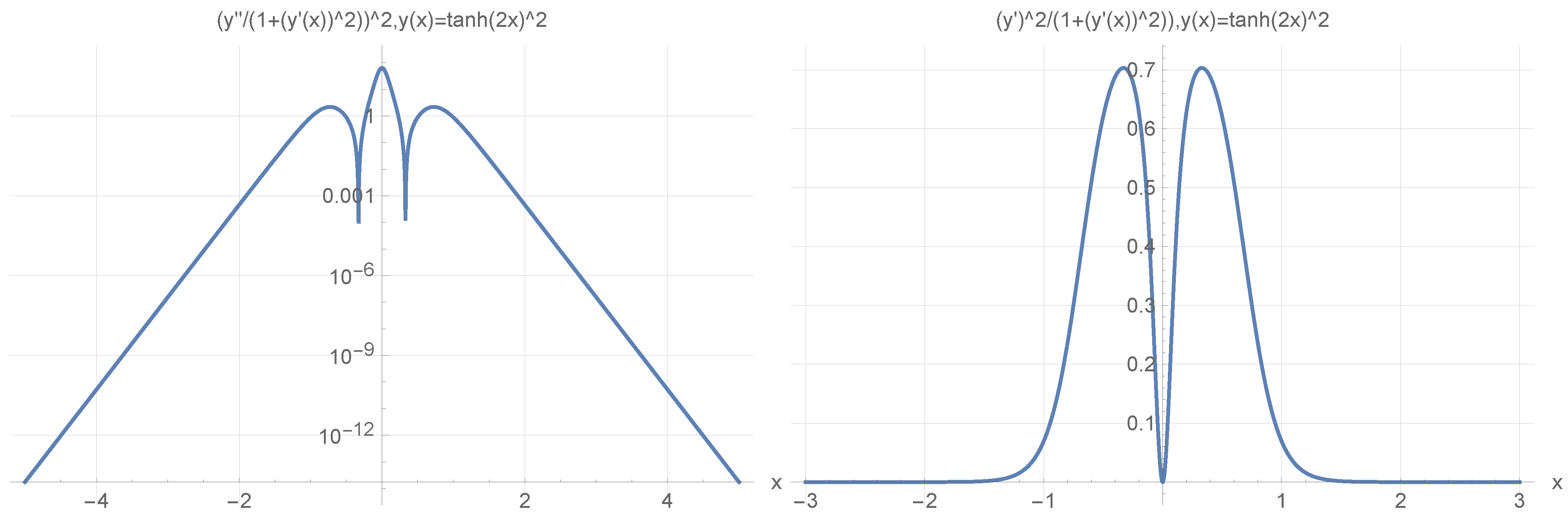
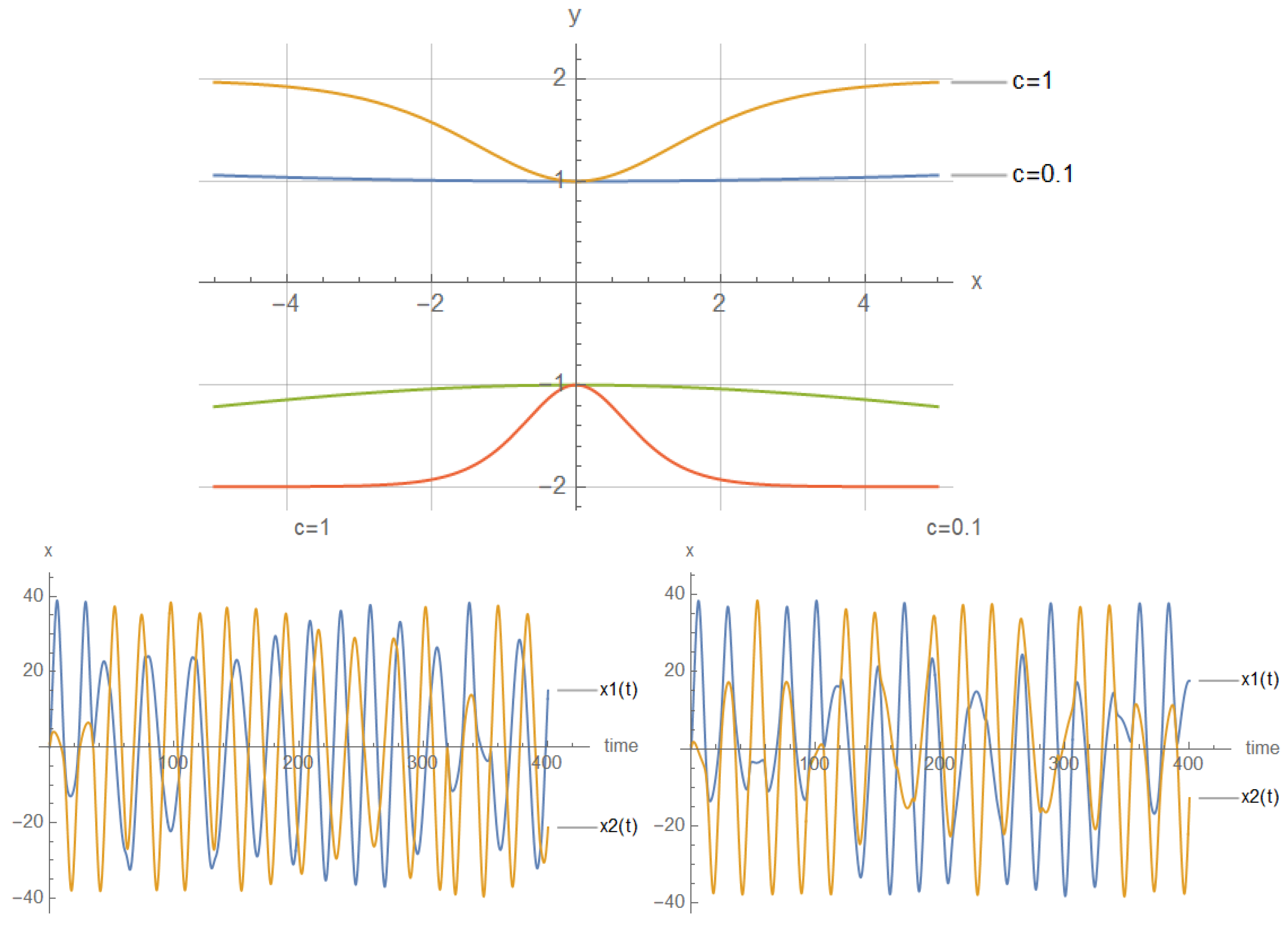
4.2. Derivation of Geometric Aharonov–Bohm Effect
Case of Ginzburg–Landau and Schrödinger Equations in Curved Space with Zero Vector Potential
4.3. Case of GL and Schrödinger Equations in Curved Space with Non-Zero Vector Potential
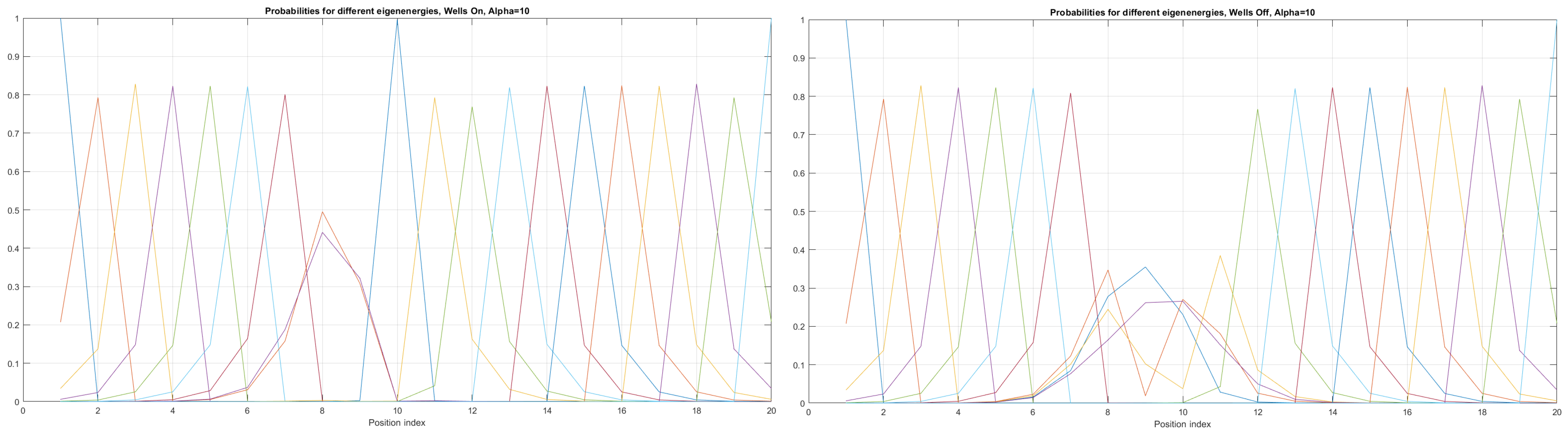
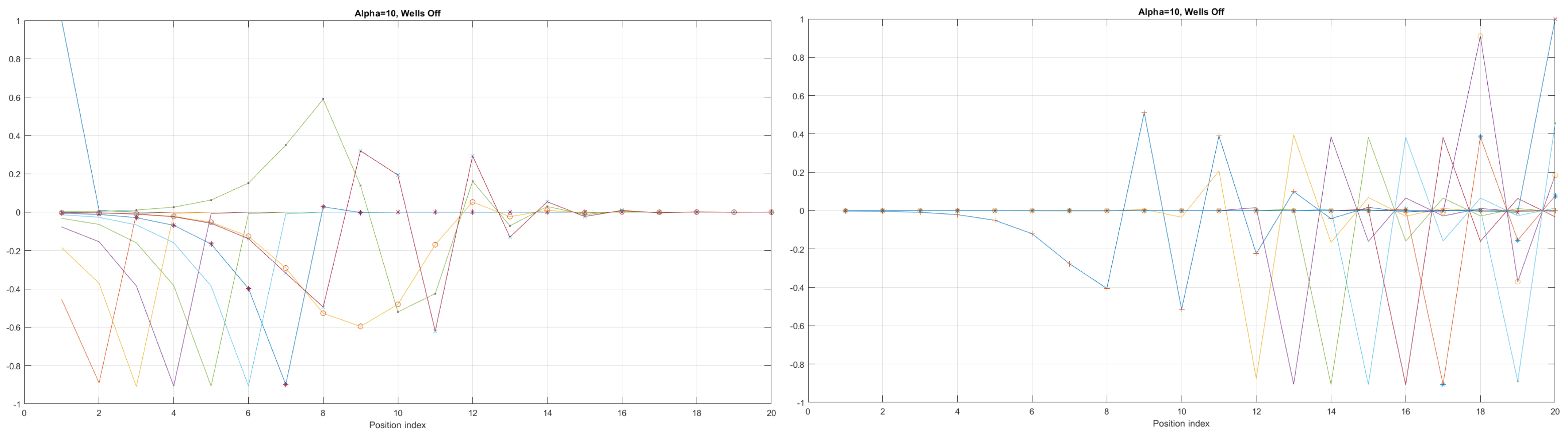
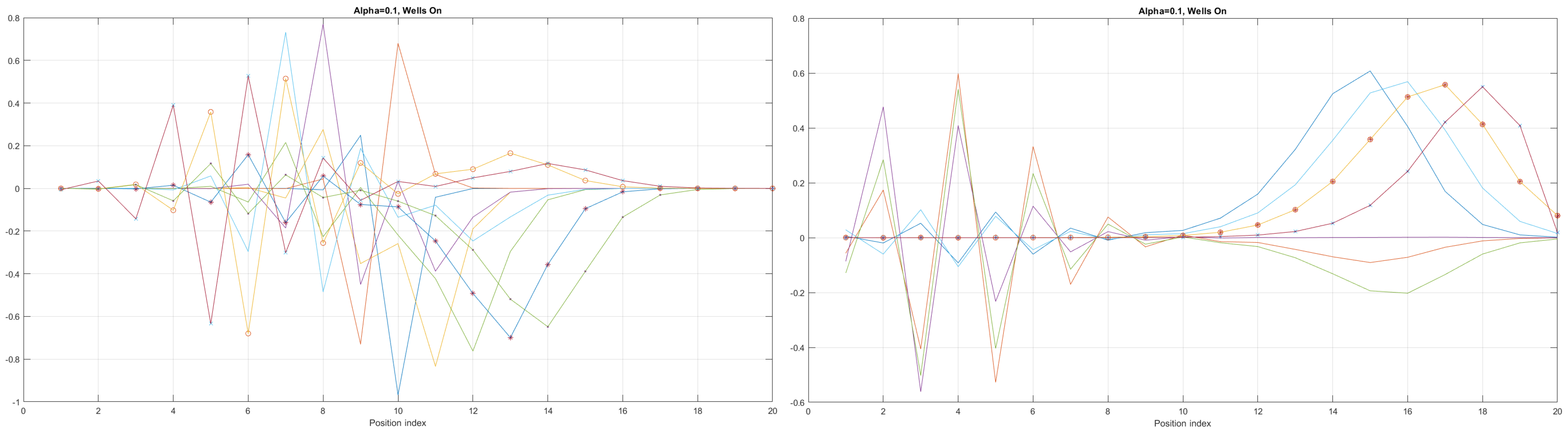
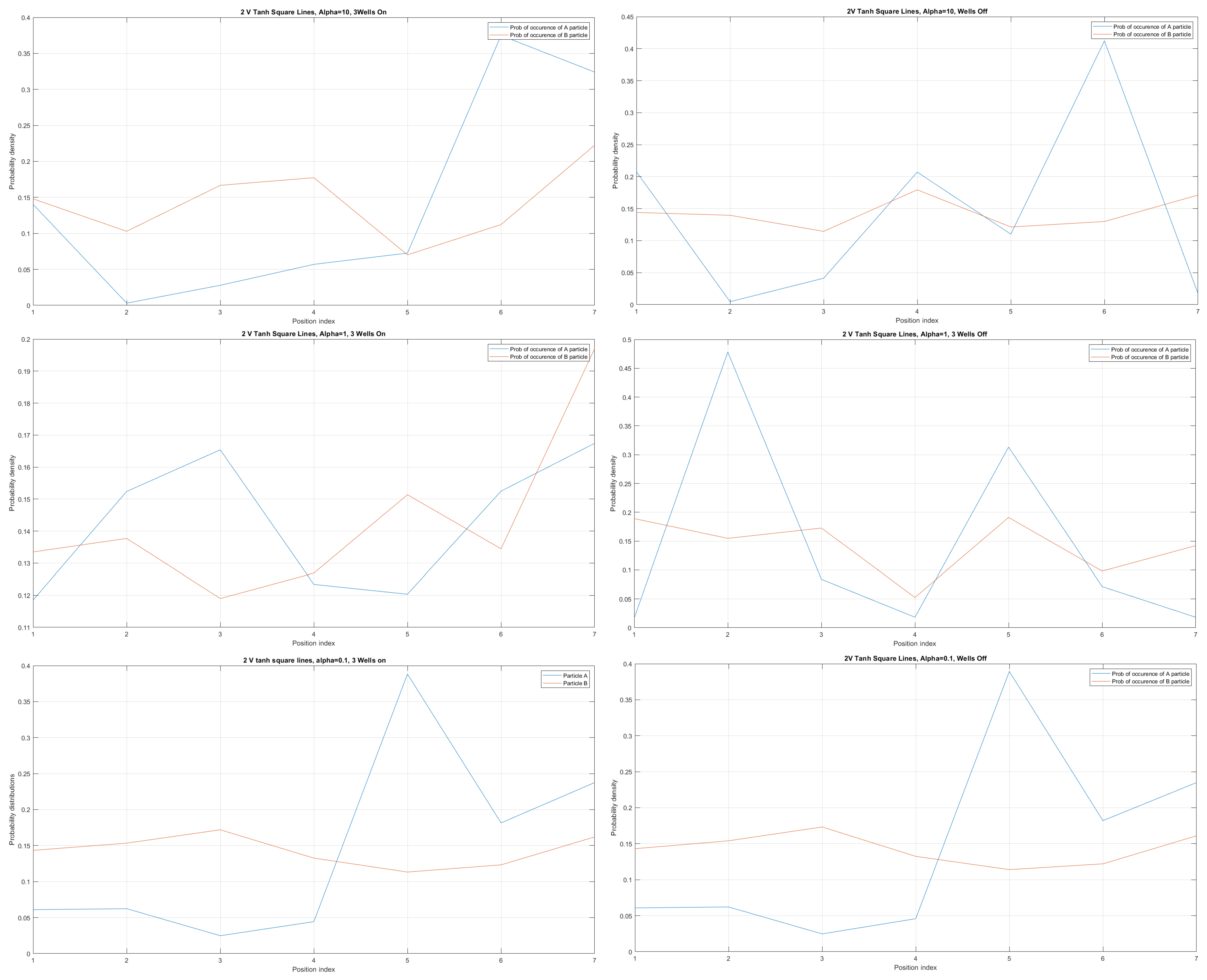
5. Case of Two Electrostatically Interacting Single-Electron Lines in Schrödinger Formalism
6. Classical Description of a Physical System Implementing a Wannier Qubit Swap Gate
7. Conclusions and Further Perspectives
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Giounanlis, P.; Blokhina, E.; Pomorski, K.; Leipold, D.R.; Staszewski, R.B. Modeling of Semiconductor Electrostatic Qubits Realized Through Coupled Quantum Dots. IEEE Access 2019, 7, 49262–49278. [Google Scholar] [CrossRef]
- Pomorski, K.; Giounanlis, P.; Blokhina, E.; Leipold, D.; Peczkowski, P.; Staszewski, R.B. From two types of electrostatic position-dependent semiconductor qubits to quantum universal gates and hybrid semiconductor-superconducting quantum computer. In Proceedings of the Superconductivity and Particle Accelerators, Kraków, Poland, 27–29 November 2018; Volume 11054. [Google Scholar]
- Ridene, S. Novel T-shaped GaSb/InAsN quantum wire for mid-infrared laser applications. Phys. Lett. A 2017, 381, 3324–3331. [Google Scholar] [CrossRef]
- Ridene, S. Mid-infrared emission in InxGaAs1-x/GaAs T-shaped quantum wire lasers and its indium composition dependence. Infrared Phys. Technol. 2018, 89, 218–222. [Google Scholar] [CrossRef]
- Likharev, K.K. Single-Electron Devices and Their Applications. Proc. IEEE 1999, 87, 606–632. [Google Scholar] [CrossRef]
- Fujisawa, T.; Hayashi, T.; Cheong, H.D.; Jeong, Y.H.; Hirayama, Y. Rotation and phase-shift operations for a charge qubit in a double quantum dot. Phys. E Low-Dimens. Syst. Nanostruct. 2004, 21, 10461052. [Google Scholar] [CrossRef]
- Petersson, K.D.; Petta, J.R.; Lu, H.; Gossard, A.C. Quantum coherence in a one-electron semiconductor charge qubit. Phys. Rev. Lett. 2010, 105, 246804. [Google Scholar] [CrossRef]
- Leipold, D. Controlled Rabi Oscillations as Foundation for Entangled Quantum Aperture Logic. In Proceedings of the Seminar at UC Berkley Quantum Labs, Berkeley, CA, USA, 25 July 2018; UC Berkley Quantum Labs: Berkeley, CA, USA, 2018. [Google Scholar]
- MacQuarrie, E.R.; Neyens, S.F.; Dodson, J.P.; Corrigan, J.; Thorgrimsson, B.; Holman, N.; Palma, M.; Edge, L.F.; Friesen, M.; Coppersmith, S.N.; et al. Progress toward a capacitively mediated CNOT between two charge qubits in Si/SiGe. npj Quantum Inf. 2020, 6, 81. [Google Scholar] [CrossRef]
- Weichselbaum, A.; Ulloa, S.E. Charge qubits and limitations of electrostatic quantum gates. Phys. Rev. A 2004, 70, 342–346. [Google Scholar] [CrossRef]
- Lee, N.; Tsuchiya, R.; Shinkai, G.; Kanno, Y.; Mine, T.; Takahama, T.; Mizokuchi, R.; Kodera, T.; Hisamoto, D.; Mizuno, H. Enhancing electrostatic coupling in silicon quantum dot array by dual gate oxide thickness for large-scale integration. Appl. Phys. Lett. 2020, 116, 162106. [Google Scholar] [CrossRef]
- Pauka, S.J.; Das, K.; Kalra, R.; Moini, A.; Yang, Y.; Trainer, M.; Bousquet, A.; Cantaloube, C.; Dick, N.; Gardner, G.C.; et al. A cryogenic CMOS chip for generating control signals for multiple qubits. Nat. Electron. 2021, 4, 64–70. [Google Scholar] [CrossRef]
- Pomorski, K. Seminars on Quantum Technologies at YouTube Channel: Quantum Hardware Systems. 2020. Available online: https://www.youtube.com/watch?v=Bhj_ZF36APw (accessed on 16 May 2020).
- Pomorski, K.; Giounanlis, P.; Blokhina, E.; Leipold, D.; Staszewski, R.B. Analytic view on Coupled Single-Electron Lines. Semicond. Sci. Technol. 2019, 34, 125015. [Google Scholar] [CrossRef]
- Bashir, I.; Asker, M.; Cetintepe, C.; Leipold, D.; Esmailiyan, A.; Wang, H.; Siriburanon, T.; Giounanlis, P.; Blokhina, E.; Pomorski, K.; et al. A Mixed-Signal Control Core for a Fully Integrated Semiconductor Quantum Computer System-on-Chip. In Proceedings of the ESSCIRC 2019—IEEE 45th European Solid State Circuits Conference (ESSCIRC), Cracow, Poland, 23–26 September 2019; pp. 125–128. [Google Scholar] [CrossRef]
- Pomorski, K.D.; Peczkowski, P.; Staszewski, R.B. Analytical solutions for N interacting electron system confined in graph of coupled electrostatic semiconductor and superconducting quantum dots in tight-binding model. Cryogenics 2020, 109, 103117. [Google Scholar] [CrossRef]
- Xu, H.Q. Method of calculations for electron transport in multiterminal quantum systems based on real-space lattice models. Phys. Rev. B 2002, 66, 165305. [Google Scholar] [CrossRef]
- Liang, Z.X.; Hu, B.B.; Wu, B. Interaction Effects on Wannier Functions of a Bose-Einstein Condensate in an Optical Lattice and Implications for Bose-Hubbard Model. arXiv 2009, arXiv:0903.4058. [Google Scholar]
- Marzari, N.; Yates, R.J.; Souza, I.; Vanderbilt, D. Maximally localized Wannier functions: Theory and applications. Rev. Mod. Phys. 2012, 84, 1419. [Google Scholar] [CrossRef]
- Spalek, J. Wstep do Fizyki Materii Skondensowanej; PWN: Warszaw, Poland, 2015. [Google Scholar]
- Aharonov, Y.; Bohm, D. Significance of Electromagnetic Potentials in the Quantum Theory. Phys. Rev. 1959, 115, 485. [Google Scholar] [CrossRef]
- Available online: https://quantumtech.blog/2022/10/20/quantum-computing-modalities-a-qubit-primer-revisited/ (accessed on 20 October 2022).
- Pomorski, K. Analytical Solutions for N-Electron Interacting System Confined in Graph of Coupled Electrostatic Semiconductor and Superconducting Quantum Dots in Tight-Binding Model with Focus on Quantum Information Processing. In Nanomaterials and Nanocomposites, Nanostructure Surfaces, and Their Applications; Springer Proceedings in Physics; Springer: Cham, Switzerland, 2023; pp. 67–165. [Google Scholar]
- Pomorski, K. Analytical View on Non-Invasive Measurement of Moving Charge by Position Dependent Semiconductor Qubit. In Proceedings of the Future Technologies Conference 2020, Vancouver, BC, Canada, 5–6 November 2020; Advances in Intelligent Systems and Computing Book Series. Springer: New York, NY, USA, 2020; Volume 1289, pp. 31–53. [Google Scholar]
- Pomorski, K. Fundamental description of Wannier qubits of any topology in semiconductor by analytical and numerical computations. arXiv 2021, arXiv:2103.05869. [Google Scholar]
- Szafran, B. Paired electron motion in interacting chains of quantum dots. Phys. Rev. B 2020, 101, 075306. [Google Scholar] [CrossRef]
- Pomorski, K. Analytical view on tunnable electrostatic Quantum Swap Gate in tight-binding model. arXiv 2020, arXiv:2001.02513. [Google Scholar]
- da Costa, R.C.T. Quantum mechanics of a constrained particle. Phys. Rev. A 1981, 23, 1982. [Google Scholar] [CrossRef]
- Pomorski, K. Equivalence between classical epidemic model and non-dissipative and dissipative quantum tight-binding model. arXiv 2020, arXiv:2012.09923. [Google Scholar]
- Pomorski, K. Analytic view on N body interaction in electrostatic quantum gates and decoherence effects in tight-binding model. Int. J. Quantum Inf. 2021, 19, 2141001. [Google Scholar] [CrossRef]
- Jinbin, L.; Yue, Y.; Dudarev, A.M. Interaction broadening of Wannier functions and Mott transitions in atomic BEC. New J. Phys. 2006, 8, 140. [Google Scholar]
- Moodie, J.C.; Long, M.W. An exact power series representation of the Baker–Campbell–Hausdorff formula. J. Phys. A Math. Theor. 2020, 54, 015208. [Google Scholar] [CrossRef]
- Casas, F.; Murua, A.; Nadinic, M. Efficient computation of the Zassenhaus formula. Comput. Phys. Commun. 2012, 183, 2386–2391. [Google Scholar] [CrossRef]
- Wang, J.; Sciarrino, F.; Laing, A.; Thompson, M.G. Integrated photonic quantum technologies. Nat. Photonics 2020, 14, 273–284. [Google Scholar] [CrossRef]
- Pomorski, K.; Staszewski, R.B. Towards quantum internet and non-local communication in position-based qubits. AIP Conf. Proc. 2020, 2241, 020030. [Google Scholar]
- Jaynes, E.T.; Cummings, F.W. Comparison of quantum and semiclassical radiation theories with application to the beam maser. Proc. IEEE 1963, 51, 89–109. [Google Scholar] [CrossRef]
- Exirifarda, Q.; Karimia, E. Schrödinger equation in a general curved space-time geometry. arXiv 2021, arXiv:2105.13896. [Google Scholar]
- Du, L.; Wang, Y.L.; Liang, G.H.; Kang, G.Z.; Zong, H.S. Schrödinger Equation of a Particle on a Rotating Curved Surface. Chin. Phys. Lett. 2016, 33, 030301. [Google Scholar] [CrossRef][Green Version]
- Zhang, C.; Chen, T.; Wang, X.; Xue, Z. Microwave driven geometric quantum computation on semiconductor charge qubits. Adv. Quantum Technol. 2021, 4, 2100011. [Google Scholar] [CrossRef]
- Duan, L.M.; Cirac, J.I.; Zoller, P. Geometric Manipulation of Trapped Ions for Quantum Computation. arXiv 2001, arXiv:0111086v1. [Google Scholar]
- Stepien, L.T. Bogomolny equations for the BPS Skyrme models with impurity. New J. Phys. 2020, 140, 154. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pomorski, K. Electrostatically Interacting Wannier Qubits in Curved Space. Materials 2024, 17, 4846. https://doi.org/10.3390/ma17194846
Pomorski K. Electrostatically Interacting Wannier Qubits in Curved Space. Materials. 2024; 17(19):4846. https://doi.org/10.3390/ma17194846
Chicago/Turabian StylePomorski, Krzysztof. 2024. "Electrostatically Interacting Wannier Qubits in Curved Space" Materials 17, no. 19: 4846. https://doi.org/10.3390/ma17194846
APA StylePomorski, K. (2024). Electrostatically Interacting Wannier Qubits in Curved Space. Materials, 17(19), 4846. https://doi.org/10.3390/ma17194846






