Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging
Abstract
:1. Introduction
2. Fundamentals of Biaxial Alternate Forging
3. Results and Discussion
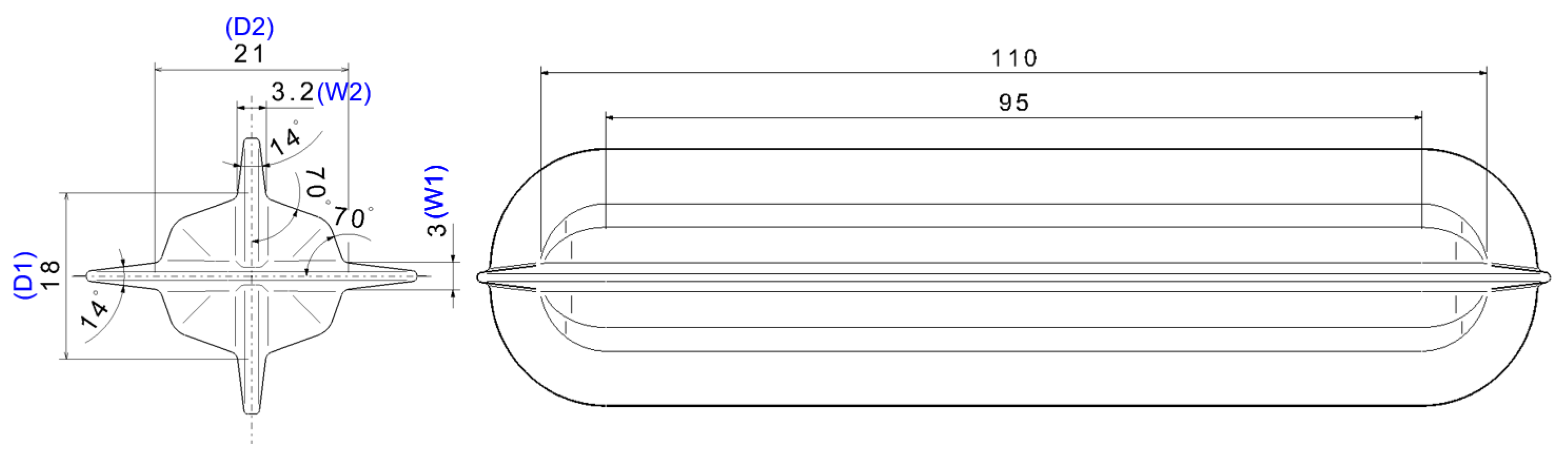
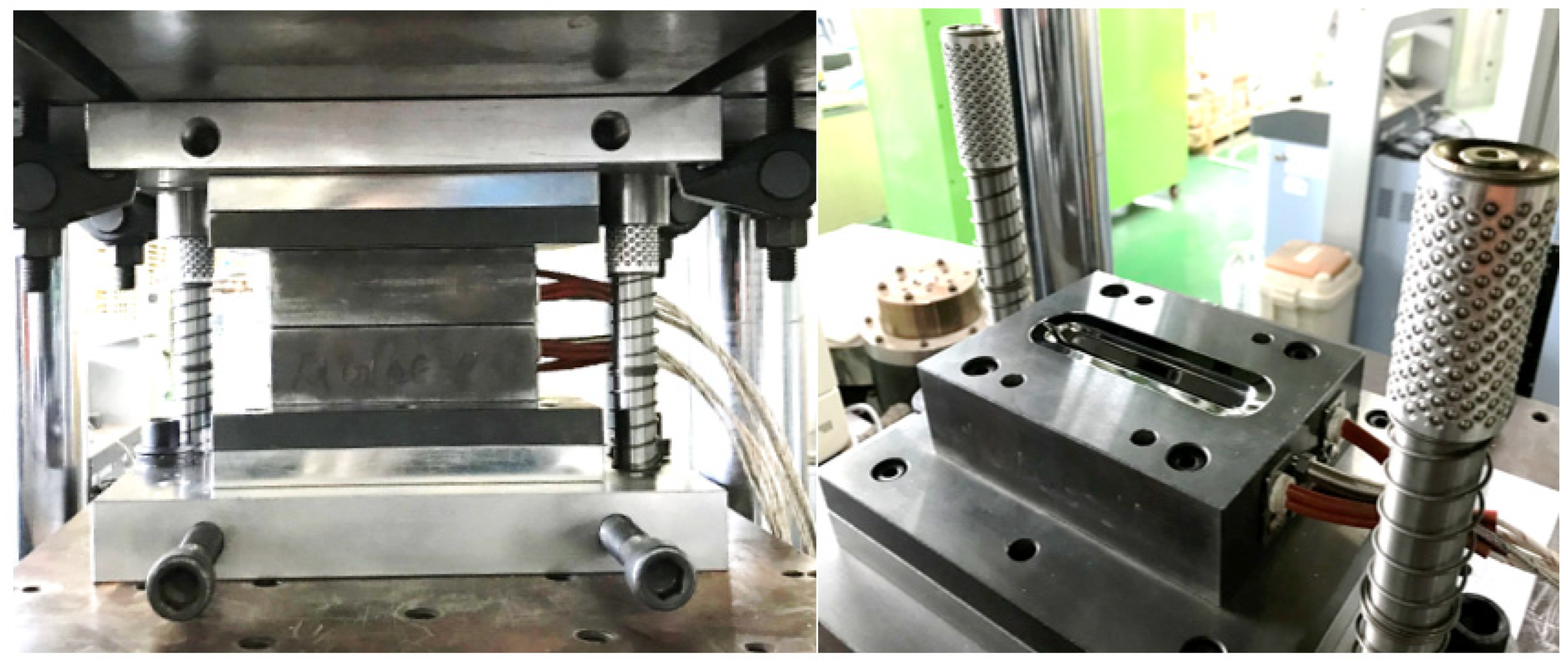
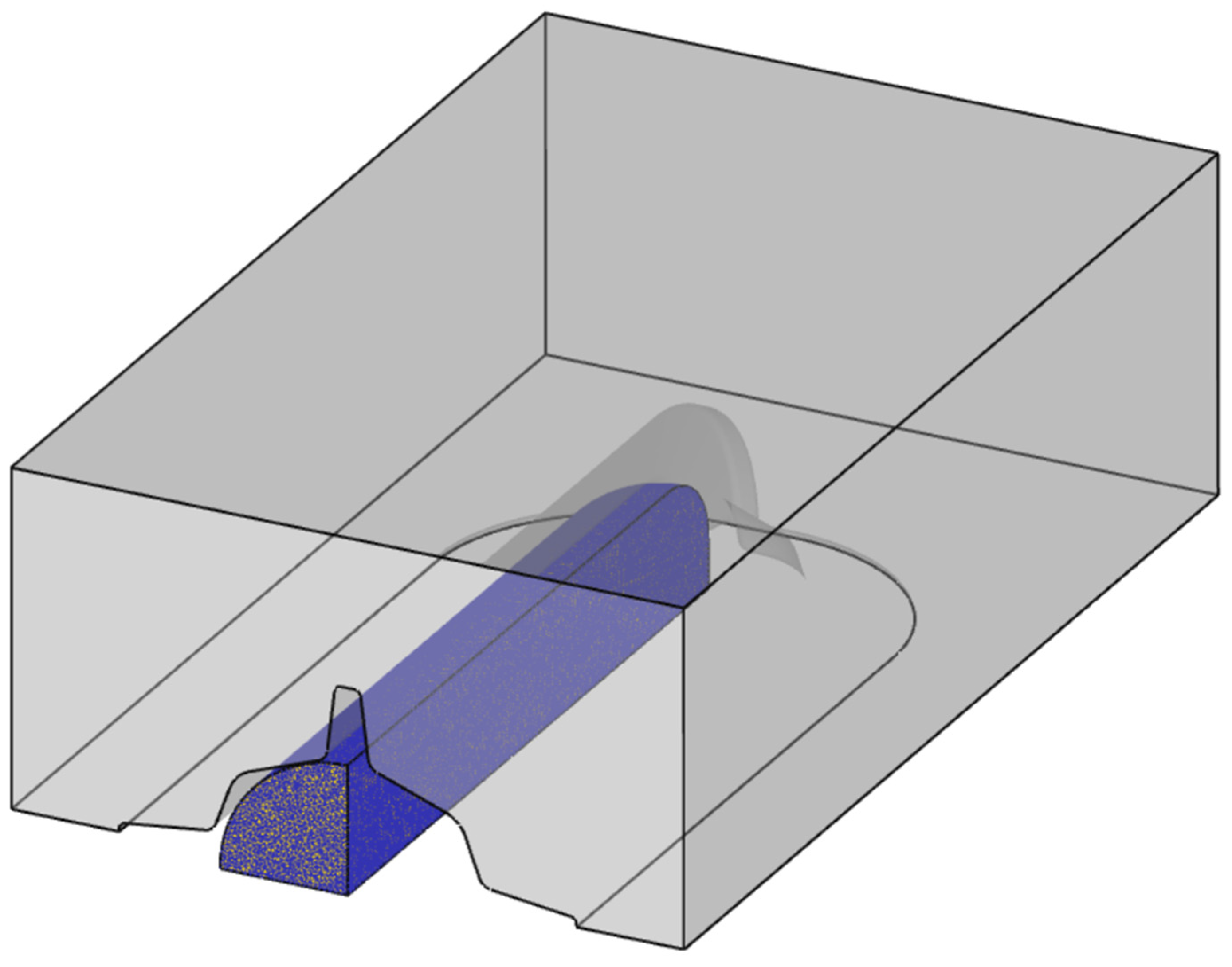
3.1. Finite Element Analysis Conditions
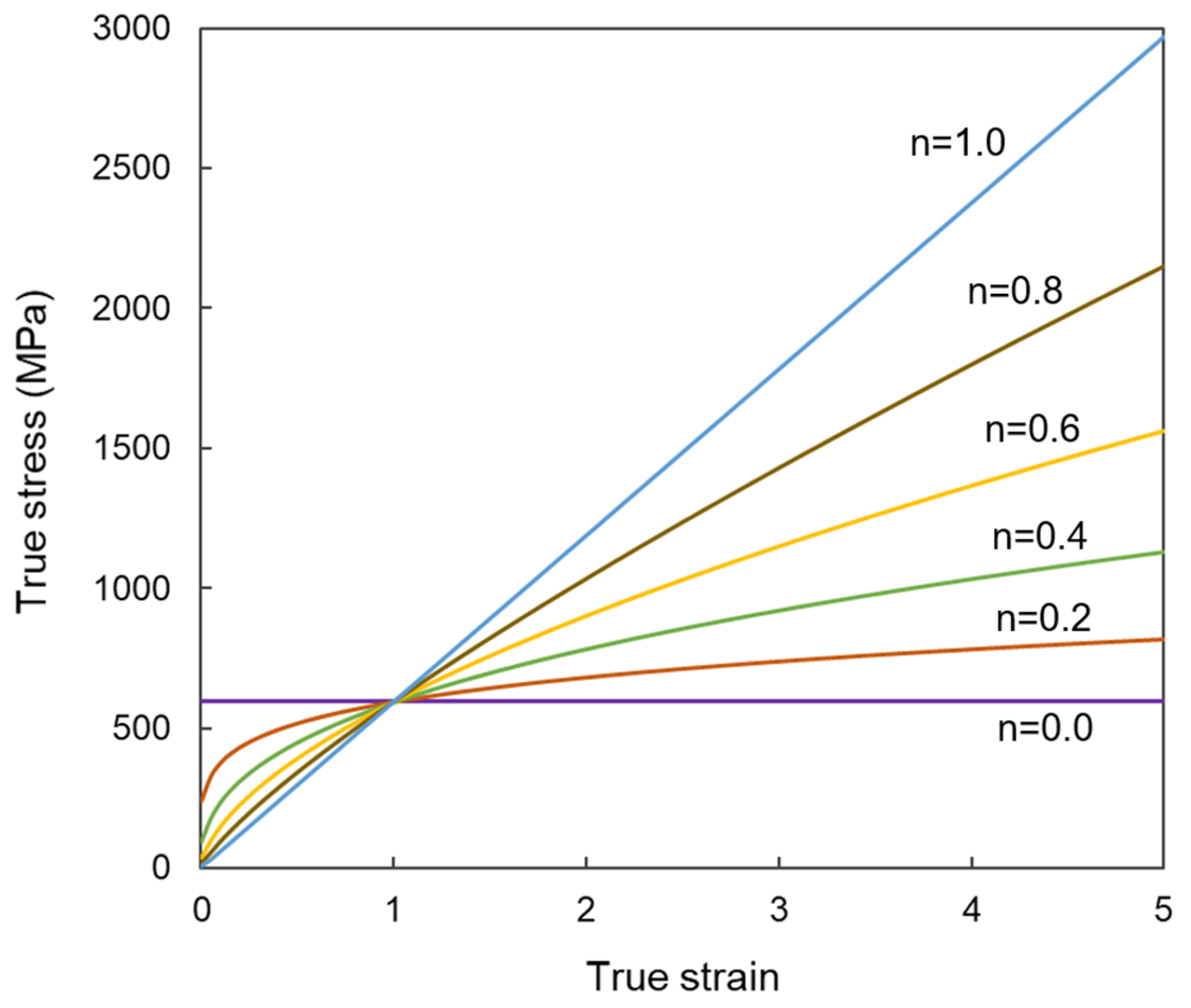
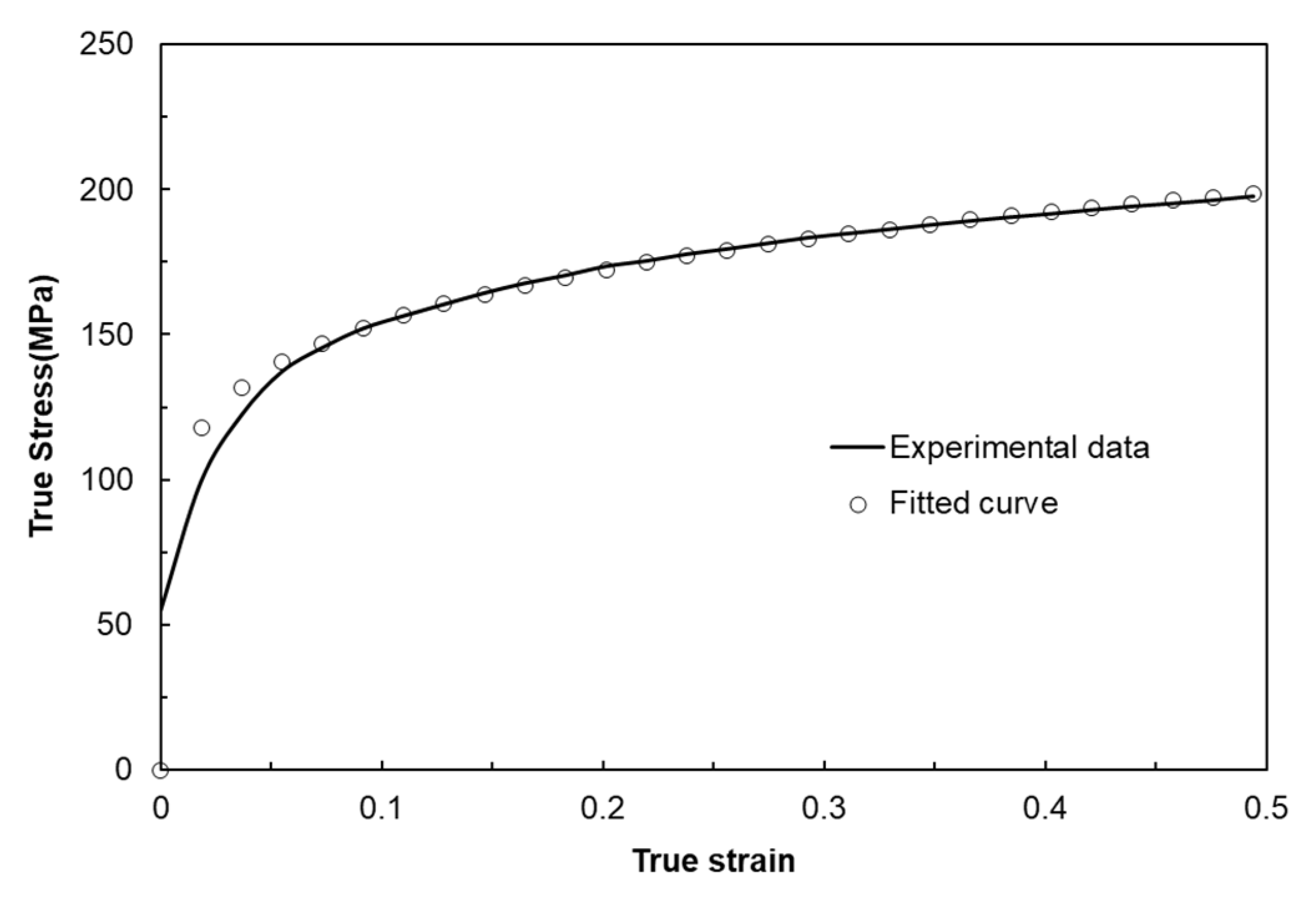
3.2. Flow Stress for Material
3.3. Finite Element Analysis Results
3.3.1. Results of Deformation Analysis Depending on Strain-Hardening Exponent
3.3.2. Estimations of Average Effective Strain Depending on Forged Passes
4. Biaxial Alternating Forging of 6061 Al Alloy
5. Conclusions
- As a result of the finite element analysis on biaxial alternate forging, it was confirmed that the strain distribution and amount of the effective strain accumulated inside the workpiece per forging pass varied depending on the strain hardening exponent, which changes the deformation behavior of the workpiece.
- The calculation of the strain distribution in the area within the diameter 4 mm of the reduced cross-section in the tensile specimen showed that the average effective strains after eight passes were approximately 440% and 300% when the strain-hardening exponent is 0 and 0.2, respectively, indicating that the biaxial alternate forging is suitable for an SPD process.
- The average effective strain of the 6061 aluminum alloy within the diameter of 6 mm from the core of the workpiece after eight passes of forging was found to be approximately 264%. This indicates that it can impose a large strain that significantly exceeds the elongation of the raw material.
- As a result of the tensile test on the forged 6061 aluminum alloy workpieces, the strength gradually increased without a significant reduction in elongation after two passes. The optical microstructures in the center of the forged workpieces showed the distribution of deformed grains and twins with no recrystallization throughout all the forging passes.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Azushima, A.; Kopp, R.; Korhonen, A.; Yang, D.; Micari, F.; Lahoti, G.D.; Groche, P.; Yanagimoto, J.; Tsuji, N.; Rosochowski, A.; et al. Severe Plastic Deformation (SPD) Processes for Metals. CIRP Ann.-Manuf. Technol. 2008, 57, 716. [Google Scholar] [CrossRef]
- Zhang, H.; He, Z.; Gao, W. Effect of Surface Severe Plastic Deformation on Microstructure and Hardness of Al Alloy Sheet with Enhanced Precipitation. Mater. Lett. 2023, 333, 133632. [Google Scholar] [CrossRef]
- Rogachev, S.O.; Zavodov, A.V.; Naumova, E.A.; Chernenok, T.V.; Lukina, E.A.; Zadorozhnyy, M.Y. Improvement of Strength-Ductility Balance of Al-Ca-Mn-Fe Alloy by Severe Plastic Deformation. Mater. Lett. 2023, 349, 134797. [Google Scholar] [CrossRef]
- Manjunath, G.K.; Bhat, K.U.; Kumar, G.V.P. Dry Sliding Wear Behaviour of Al-5Zn-2Mg Alloy Processed by Severe Plastic Deformation. Mater. Today Proc. 2021, 46, 9232–9238. [Google Scholar] [CrossRef]
- Parimi, A.K.; Robi, P.S.; Dwivedy, S.K. Severe Plastic Deformation of Copper and Al-Cu Alloy Using Multiple Channel-Die Compression. Mater. Des. 2011, 32, 1948–1956. [Google Scholar] [CrossRef]
- Zha, M.; Li, Y.; Mathiesen, R.H.; Bjørge, R.; Roven, H.J. Achieve High Ductility and Strength in an Al-Mg Alloy by Severe Plastic Deformation Combined with Inter-Pass Annealing. Mater. Sci. Eng. A 2014, 598, 141–146. [Google Scholar] [CrossRef]
- Zhu, Z.; Han, J.; Gao, C.; Liu, M.; Song, J.; Wang, Z.; Li, H. Microstructures and Mechanical Properties of Al-Li 2198-T8 Alloys Processed by Two Different Severe Plastic Deformation Methods: A Comparative Study. Mater. Sci. Eng. A 2017, 681, 65–73. [Google Scholar] [CrossRef]
- Rogachev, S.O.; Naumova, E.A.; Vasileva, E.S.; Magurina, M.Y.; Sundeev, R.V.; Veligzhanin, A.A. Structure and Mechanical Properties of Al-Ca Alloys Processed by Severe Plastic Deformation. Mater. Sci. Eng. A 2019, 767, 138410. [Google Scholar] [CrossRef]
- Naumova, E.A.; Rogachev, S.O.; Sundeev, R.V. Effect of Severe Plastic Deformations on Structure Features and Mechanical Behavior of Al4Ca Intermetallic in Al-18% Ca Alloy. J. Alloys Compd. 2021, 854, 157117. [Google Scholar] [CrossRef]
- Zhao, S.; Meng, C.; Mao, F.; Hu, W.; Gottstein, G. Influence of Severe Plastic Deformation on Dynamic Strain Aging of Ultrafine Grained Al-Mg Alloys. Acta Mater. 2014, 76, 54–67. [Google Scholar] [CrossRef]
- Mohammadi, A.; Enikeev, N.A.; Murashkin, M.Y.; Arita, M.; Edalati, K. Developing Age-Hardenable Al-Zr Alloy by Ultra-Severe Plastic Deformation: Significance of Supersaturation, Segregation and Precipitation on Hardening and Electrical Conductivity. Acta Mater. 2021, 203, 116503. [Google Scholar] [CrossRef]
- Moradpour, M.; Khodabakhshi, F.; Mohebpour, S.R.; Eskandari, H.; Haghshenas, M. Finite Element Modeling and Experimental Validation of CGP Classical and New Cross Routes for Severe Plastic Deformation of an Al-Mg Alloy. J. Manuf. Process. 2019, 37, 348–361. [Google Scholar] [CrossRef]
- Liu, M.P.; Sun, S.C.; Roven, H.J.; Yu, Y.D.; Zhang, Z.; Murashkin, M.; Valiev, R.Z. Deformation defects and electron irradiation effect in a nanostructured Al–Mg aluminum alloy processed by severe plastic deformation. Trans. Nonferrous Met. Soc. China 2012, 22, 1810–1816. [Google Scholar] [CrossRef]
- Shin, Y.-C.; Ha, S.-H.; Kim, B.-H.; Yoon, Y.-O.; Lim, S.-H.; Choi, H.-J.; Kim, S.K.; Hyun, S.-K. Forgeability Assessment of Al-6 wt%Mg Alloy Using Biaxial Alternate Forging. J. Mater. Process. Technol. 2020, 286, 116822. [Google Scholar] [CrossRef]
- Shin, Y.-C.; Ha, S.-H.; Yoon, Y.-O.; Kim, S.K.; Choi, H.-J.; Hyun, K.-H.; Hyun, S.-K. Microstructure Evolution and Tensile Properties of Al-Mg Alloy Containing a High Content of Mg by Biaxial Alternative Forging. Trans. Mater. Process. 2019, 28, 361–367. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Islamgaliev, R.K.; Alexandrov, I.V. Bulk Nanostructured Materials from Severe Plastic Deformation. Prog. Mater. Sci. 2000, 45, 103–189. [Google Scholar] [CrossRef]
- Markushev, M.; Nugmanov, D.; Sitdikov, O.; Vinogradov, A. Structure, Texture and Strength of Mg-5.8Zn-0.65Zr Alloy after Hot-to-Warm Multi-Step Isothermal Forging and Isothermal Rolling to Large Strains. Mater. Sci. Eng. A 2018, 709, 330–338. [Google Scholar] [CrossRef]
- Davis, J.R. Aluminum and Aluminum Alloys; ASM International: Novelty, OH, USA, 1993. [Google Scholar]
- ASTM: B557M-10; Standard Test Methods for Tension Testing Wrought and Cast Aluminum- and Magnesium-Alloy Products (Metric). ASTM International: West Conshohocken, PA, USA, 2010.
- Valberg, H.S. Applied Metal Forming: Including FEM Analysis; Cambridge University Press: New York, NY, USA, 2010; p. 122. ISBN 978-0-521-51823-9. [Google Scholar]
- Marciniak, Z.; Duncan, J.L.; Hu, S.J. Mechanics of Sheet Metal Forming; Butterworth-Heinemann: Oxford, UK, 2002; pp. 36–38. ISBN 978-0-750-65300-8. [Google Scholar]
- Hosford, W.F.; Caddell, R.M. Metal Forming: Mechanics and Metallurgy; Cambridge University Press: New York, NY, USA, 2007; p. 34. ISBN 978-0-521-88121-0. [Google Scholar]
- Awasthi, A.; Saxena, K.K.; Dwivedi, R.K.; Buddhi, D.; Mohammed, K.A. Design and Analysis of ECAP Processing for Al6061 Alloy: A Microstructure and Mechanical Property Study. Int. J. Interact. Des. Manuf. 2023, 17, 2309–2321. [Google Scholar] [CrossRef]
- Sakai, T.; Belyakov, A.; Kaibyshev, R.; Miura, H.; Jonas, J.J. Dynamic and Post-Dynamic Recrystallization under Hot, Cold and Severe Plastic Deformation Conditions. Prog. Mater. Sci. 2014, 60, 130–207. [Google Scholar] [CrossRef]



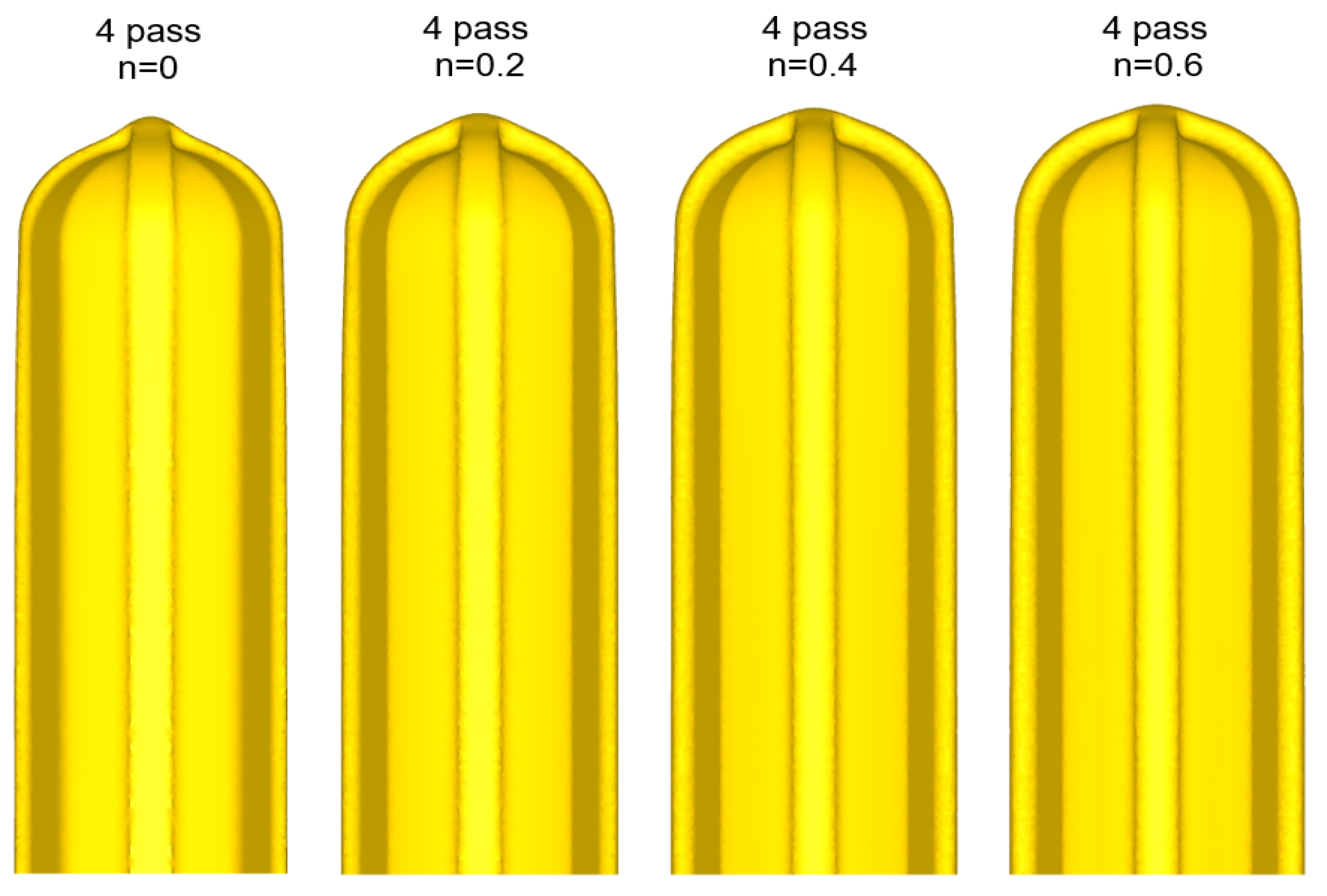

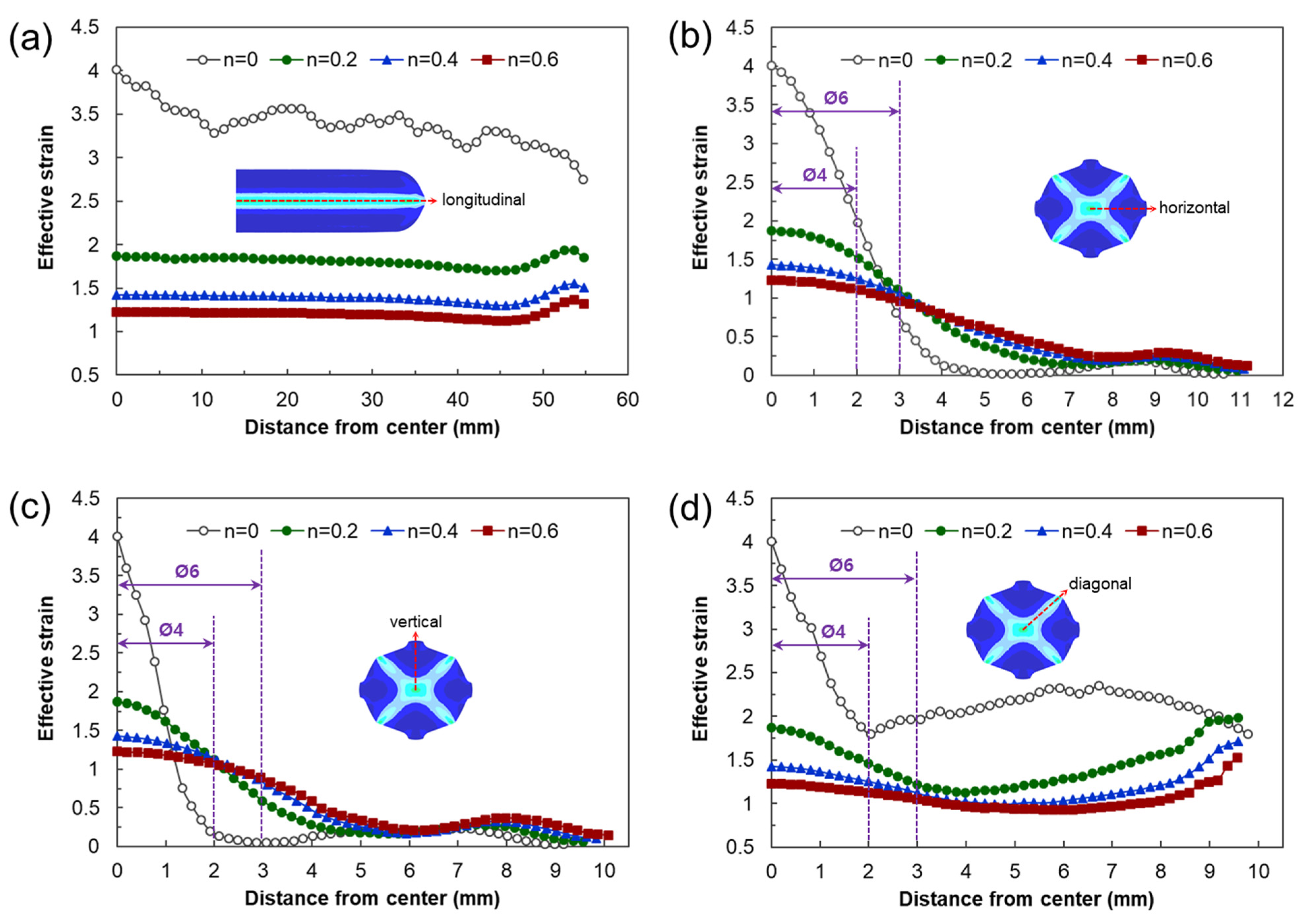

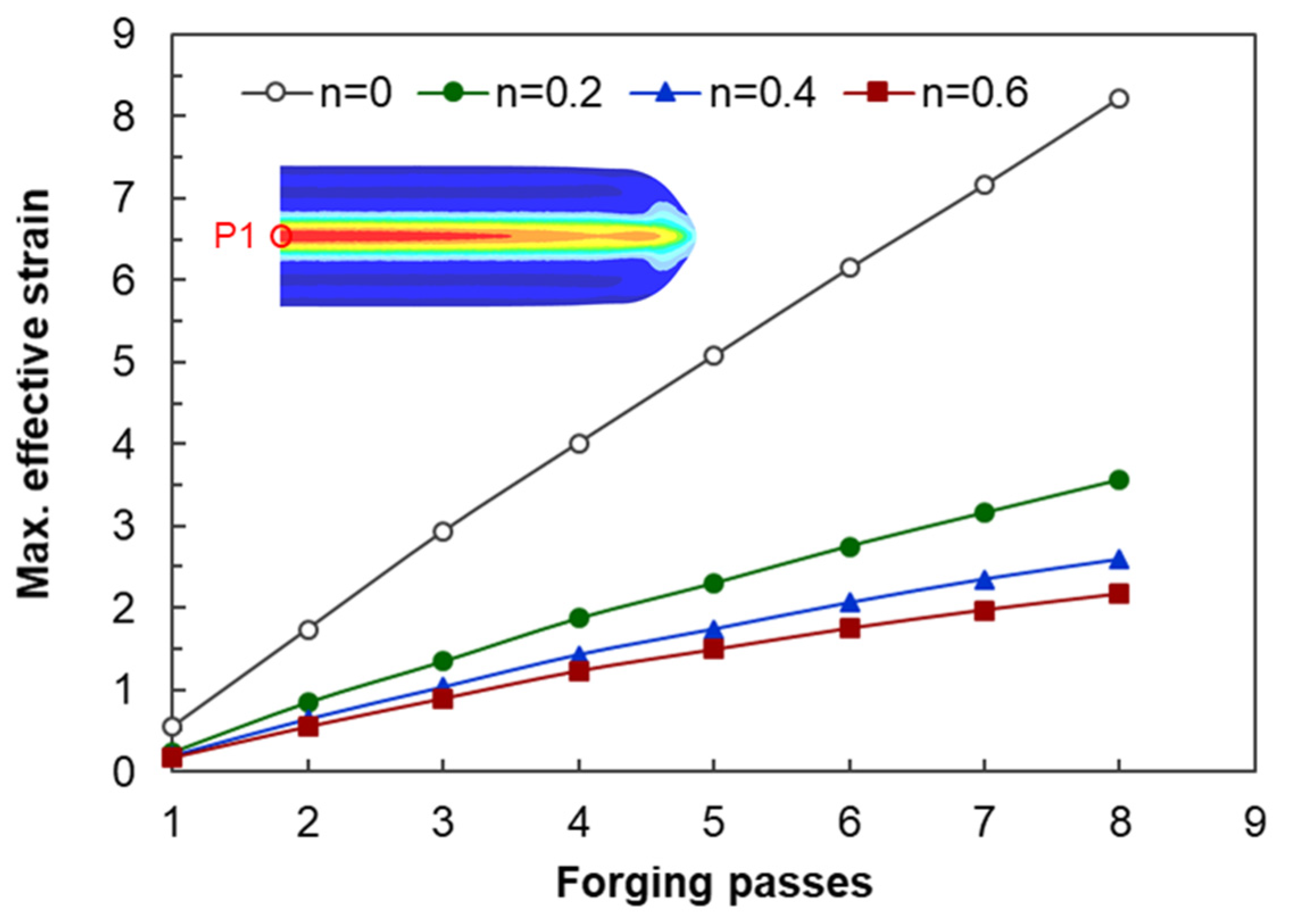

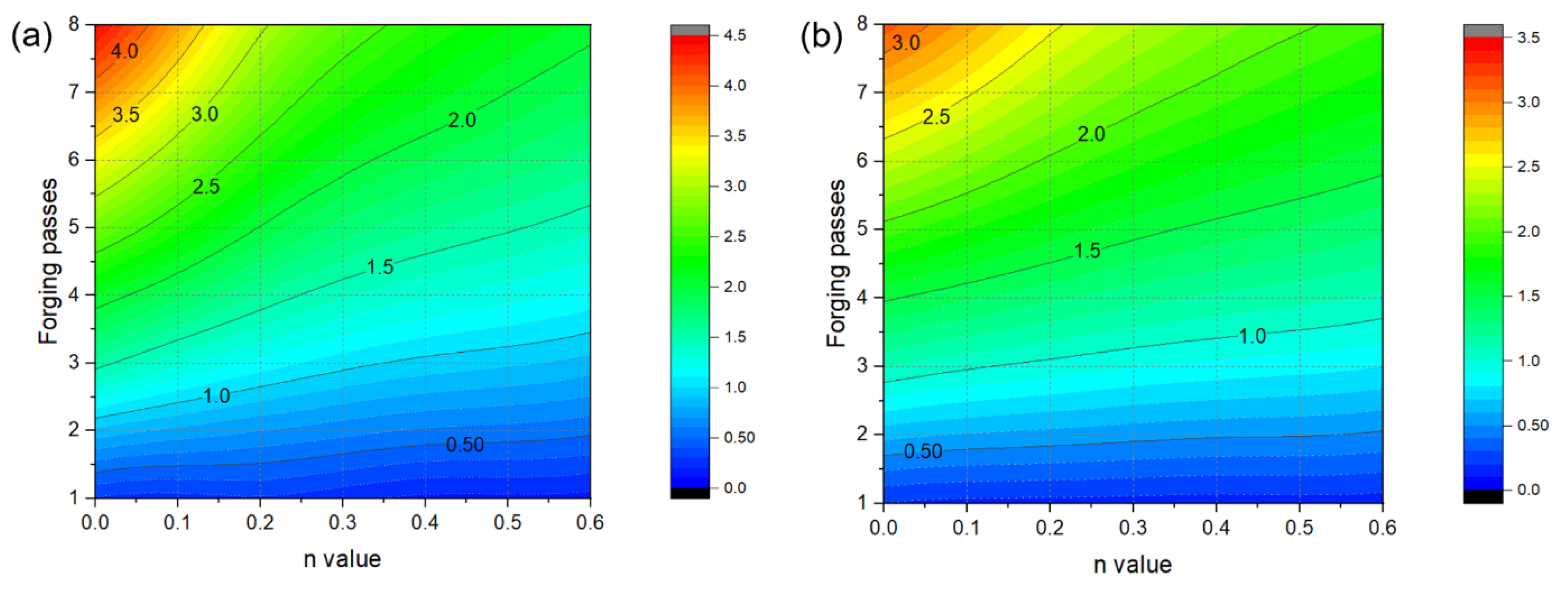





| Forging Passes | ||||||||
|---|---|---|---|---|---|---|---|---|
| D4 Specimen | D6 Specimen | |||||||
| A | B | C | D | A | B | C | D | |
| 1 | 4.4994 | −4.1337 | 0.6468 | 0.2887 | −0.1035 | 0.2315 | −0.1900 | 0.1977 |
| 2 | −0.1765 | 0.8638 | −1.0399 | 0.8733 | −0.1513 | 0.3860 | −0.4381 | 0.6375 |
| 3 | −2.2025 | 3.7803 | −2.6383 | 1.5559 | −0.5462 | 0.9872 | −0.9052 | 1.1059 |
| 4 | −2.3728 | 4.4439 | −3.3914 | 2.1069 | −0.2585 | 0.8547 | −1.1574 | 1.5202 |
| 5 | −4.0397 | 7.0479 | −4.9670 | 2.7320 | −0.5020 | 1.4089 | −1.7096 | 1.9485 |
| 6 | −5.5281 | 9.3960 | −6.3917 | 3.3037 | −0.8550 | 2.1942 | −2.3873 | 2.3689 |
| 7 | −6.7134 | 11.422 | −7.7897 | 3.8821 | −0.9281 | 2.6342 | −2.9525 | 2.7644 |
| 8 | −8.9278 | 14.736 | −9.6048 | 4.4453 | −1.7251 | 4.0135 | −3.8606 | 3.1622 |
| Pass | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| Avg. effective strain (%) | 17.3 | 57.7 | 98.6 | 135.8 | 171.2 | 204.3 | 236.0 | 264.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ha, S.-H.; Shin, Y.-C. Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging. Materials 2024, 17, 968. https://doi.org/10.3390/ma17050968
Ha S-H, Shin Y-C. Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging. Materials. 2024; 17(5):968. https://doi.org/10.3390/ma17050968
Chicago/Turabian StyleHa, Seong-Ho, and Young-Chul Shin. 2024. "Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging" Materials 17, no. 5: 968. https://doi.org/10.3390/ma17050968
APA StyleHa, S.-H., & Shin, Y.-C. (2024). Deformation Behavior and Microstructure of 6061 Aluminum Alloy Processed by Severe Plastic Deformation Using Biaxial Alternate Forging. Materials, 17(5), 968. https://doi.org/10.3390/ma17050968










