Next Article in Journal
Dynamic Performance of a Steel Road Sign with Multi-Material Electronic Signboard Under Mining-Induced Tremors from Different Mining Areas: Experimental and Numerical Research
Next Article in Special Issue
Recovery of Valuable Materials Based on Pb and Zn in the Hydrometallurgical Processing of Copper Shaft Furnace Dust
Previous Article in Journal
Influence of Carbon Nanotube Addition on Microstructure and Microwave Heating Performance of Polycarbosilane-Based Silicon Carbide
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Materials
Volume 18
Issue 7
10.3390/ma18071453
materials-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Synthesis, Structural and Magnetic Properties of BiFeO3 Substituted with Ag

by
Maria Čebela
1,2,*,
Pavla Šenjug
2,
Dejan Zagorac
1,3,
Igor Popov
4,5,
Jelena Zagorac
1,3,
Milena Rosić
1,* and
Damir Pajić
2,*
1
“Vinča” Institute of Nuclear Sciences, National Institute of the Republic of Serbia, University of Belgrade, Mike Petrovića Alasa 12-14, 11351 Belgrade, Serbia
2
Faculty of Science, Department of Physics, University of Zagreb, Bijenička Cesta 32, 10000 Zagreb, Croatia
3
Center of Excellence “CextremeLab”, “Vinča” Institute of Nuclear Sciences, National Institute of the Republic of Serbia, University of Belgrade, Mike Petrovića Alasa 12-14, 11351 Belgrade, Serbia
4
Institute for Multidisciplinary Research, University of Belgrade, Kneza Višeslava 1, 11030 Belgrade, Serbia
5
Institute of Physics, University of Belgrade, Pregrevica 118, 11080 Belgrade, Serbia
*
Authors to whom correspondence should be addressed.
Materials 2025, 18(7), 1453; https://doi.org/10.3390/ma18071453
Submission received: 20 February 2025 / Revised: 12 March 2025 / Accepted: 22 March 2025 / Published: 25 March 2025
(This article belongs to the Special Issue Advances in Process Metallurgy and Metal Recycling)
Browse Figures
Versions Notes

Abstract

:
Here, we report the hydrothermal synthesis of BFO (bismuth ferrite) and Bi1−xAgxFeO3 (x = 0.01, 0.02) ultrafine nanopowders. The diffraction patterns show that all obtained particles belong to the R3c space group. On top of that, crystal structure prediction has been accomplished using bond valence calculations (BVCs). Several promising perovskite structures have been proposed together with experimentally observed modifications of BFO as a function of silver doping. Magnetization measurements were performed on BFO, both pure and substituted with 1% and 2% of Ag. The addition of Ag in BFO did not affect the Neel temperature, TN = 630 K for all samples; instead, the influence of Ag was observed in the increase in the value and irreversibility of magnetization, which are usual characteristics of weak ferromagnetism. Our calculations based on density functional theory (DFT) are in agreement with the experimental finding of enhanced magnetization upon Ag doping of antiferromagnetic BFO, which is assigned to the perturbation of magnetic-type interactions between Fe atoms by Ag substitutional doping. Additionally, electronic and magnetic properties were studied for all phases predicted by the BVCs study. DFT predicted half-metallicity in the γ phase of BFO, which may be of great interest for further study and potential applications.

1. Introduction

After many years of practical and scientific interests, perovskite-type bismuth ferrites still attract enormous attention. This is due to their ferroelectric and antiferromagnetic arrangement in only one phase [1,2,3]. Due to the variety of their unique behaviors, they have attracted a wide range of applications such as optoelectronic and spintronic devices, data storage, chemical resistant sensors and photocatalytic activities [4,5,6,7,8,9]. Many examinations have been conducted on the substitution of bismuth ions in BiFeO3 by alkaline earth, transition and rare earth metals like Sr2+, Mn3+, Nb3+ and La3+ to name just a few of the numerous examples [10,11,12]. Perovskites with mixed metal oxides provide an important class of electric, ferroelectric, catalytic and magnetic materials [13]. From the magnetic and ferroelectric viewpoint, the nanosized ferrite particles with appropriate shapes usually display qualitatively new properties concerning their bulk counterparts, such as changes in magnetic transition temperatures, the emergence of superparamagnetism, size dependence of the saturation magnetization and coercivity, etc. [14,15].
Under standard conditions, the known modification of bismuth ferrite crystallizes in the perovskite structure type (α) and possesses a rhombohedral space group R3c (no. 161) [16,17,18,19].
The location of residual porosity, microstructure, particle size, grain growth behavior and grain boundary characteristics in sintered specimens play a crucial role in determining the electrical and magnetic properties of BiFeO3 [20,21,22,23]. Notably, introducing dopants in BiFeO3 materials can significantly impact their structure and properties. Recent studies have successfully fabricated various morphologies of Ag-substituted BiFeO3, including thin films [24,25,26,27], nanofilms [28], nanoparticles [29,30], nanopowders [31,32], nanocrystalline BFO [33] and bulk/polycrystalline ceramics [34,35,36,37].
The development of the secondary phase, weak electromagnetic coupling and weak magnetization are only some of the reasons for the limited technological application of bismuth ferrites. Doping is used to increase its multiferroic properties. Perovskite-type bismuth ferrites have the general formula of ABO3, which is complicated by doping with various elements in appropriate stoichiometric ratios. Doping leads to a charge imbalance in the system, which can be adjusted by creating oxygen vacancies, charge disproportionality of Fe3+ and Fe5+ or partial exchange of Fe3+ into Fe4+ [13]. The element we use as a dopant in this perovskite structure will occupy the A or B site or both of them (amphoteric) depending on its ionic radius, where if it is larger, it will occupy the A site, and if it is smaller, it will occupy the B site [38]. Silver ions are used as a dopant, and since they are much larger than Fe cations, Ag will occupy the A sites. If it were not so, partial substitution of Fe for Ag would affect the change in Fe valence. The perovskite structures are governed by the Goldschmidt tolerance factor f = (rA + rO)/ √ 2 (rB + rO) [39]. The stress in the structure is due to the mismatch between the equilibrium lengths of the A-O and B-O bonds. When the Goldschmidt tolerance factor is f = 1, it represents an unstressed cubic lattice. If f > 1 or f < 1, relaxed deformation occurs by distorting the structure from its ideal cubic shape. The Goldschmidt tolerance factor is 0.9309, which leads us to conclude that the observed nanometric structure could be stable.
In this study, we investigated the effects of Ag doping on the structural, electrical and magnetic properties of BiFeO3. To advance the understanding of BiFeO3’s magnetic behavior, we complemented experimental magnetization measurements with ab initio theoretical calculations. A central focus of this study was the intricate spin cycloid, a fundamental feature governing the multiferroic properties of BiFeO3, and the development of strategies for its precise control. The investigation further extended to a detailed analysis of the structure–property relationship under Ag doping, providing new insights into the role of compositional tuning. Additionally, predictive modeling enabled the identification of novel perovskite structures alongside a comprehensive evaluation of their electronic and magnetic properties. By integrating high-resolution synthesis, XRD characterization, magnetic analysis and theoretical predictions, this work offers a profound understanding of the impact of Ag substitution on the structural and magnetic evolution of BiFeO3, establishing a robust framework for tailoring its functional properties.

2. Materials and Methods

2.1. Synthesis and Structural Characterization of Bi1−xAgxFeO3 (x = 0.01, 0.02) Nanopowders

Nanocrystalline powders of Bi1−xAgxFeO3 were synthesized using the hydrothermal method, a well-established technique recognized for its energy efficiency, cost-effectiveness and ability to yield high-purity products with controlled morphology. High-purity precursors, including bismuth nitrate (Bi(NO3)3·5H2O), silver nitrate (AgNO3), iron nitrate (Fe(NO3)3·9H2O) and potassium hydroxide (KOH), were employed to ensure the reproducibility and phase purity of the final material. The synthesis involved dissolving stoichiometric amounts of Bi(NO3)3·5H2O, AgNO3 and Fe(NO3)3·9H2O in 40 mL of an 8 M KOH solution, followed by vigorous stirring for 30 min to promote homogeneous mixing and precursor reactivity. The resulting solution was then transferred into a Teflon-lined stainless steel autoclave and subjected to hydrothermal treatment at 200 °C under autogenous pressure for 6 h, facilitating crystallization under controlled conditions. Following the reaction, the system was cooled naturally to ambient temperature, and the precipitated powders were carefully collected. To eliminate residual reactants and potential secondary phases, the powders underwent multiple washing cycles involving centrifugation with distilled water. Subsequently, they were dispersed in ethanol and subjected to ultrasonication for 60 min to break up agglomerates and ensure uniform particle distribution. Finally, the purified powders were dried via controlled evaporation of ethanol using a heated mortar maintained at 60 °C, yielding nanocrystalline Bi1−xAgxFeO3 with well-defined structural characteristics [40].
Multiple syntheses of bismuth ferrite doped with two distinct concentrations of Ag were successfully performed, yielding phase-pure samples in each case. This consistency underscores the high precision and reproducibility of the synthesis process. For magnetic characterization, a representative sample was carefully selected for each doping level to ensure that the measured properties accurately reflect the intrinsic magnetic behavior of the doped bismuth ferrite system.
The structure of the obtained powder was determined by X-ray powder diffraction on a Rigaku ULTIMA IV XRPD diffractometer (Tokyo, Japan) with Cu Kα1,2 radiations, at room temperature. Data for structural refinement were taken in the 2θ range 10–110°, with a step width of 0.02° 2θ and 5 s per step. The refinement was performed with the FullProf (version 5.90) [41] computer program which adopts the Rietveld calculation method. In the present approach, the grain size broadening was represented by a Lorentzian function, and strain broadening by a Gaussian function.

2.2. Magnetic Measurements

Magnetization was measured on the MPMS 5 SQUID magnetometer (Quantum Design Inc., San Diego, CA, USA) in a wide temperature range, from 2 K to 720 K, and in fields up to 5T. The high temperatures were realized using an oven option, which enabled the measurements at the high temperatures. To measure the temperature dependence of magnetization or the so-called zero-field-cooled (ZFC) and field-cooled (FC) magnetization curves of the compounds with the high magnetic phase transition temperature, such as pure BFO (T N ~630 K), correctly through the entire temperature range, it was necessary to use the following procedure. The samples were first cooled in zero field till 2 K and measured with the standard setup (pellet in the plastic straw) until T = 400 K; then, the samples were taken out and put on a quartz tube holder of the oven option and inserted back for the measurements on the high temperatures. After completing the ZFC curve, the FC magnetization was measured while cooling from 720 K to room temperature, where again the sample was removed and put in a standard setup for the continuation of the FC curve. For all the compounds, M(T) was measured in the constant field of 1 kOe. In addition to the M(T) measurements, the field dependence was measured at temperatures of 4 K and 300 K on a standard setup.

2.3. Crystal Structure Prediction (CSP) and Bond Valence Calculations (BVC)

Our general approach and methods of crystal structure prediction (CSP) and identification of structure candidates have been given in detail elsewhere [42,43,44,45], especially including the multidisciplinary approach and previous successful applications on perovskites [46,47,48,49]. The Structure Prediction Diagnostic Software (SPuDS V2.21.05.11) [49] was used to generate potential perovskite-related structure candidates in a silver-doped BiFeO3 chemical system. The SPuDS program is based on bond valence calculations (BVCs) and is used to predict the crystal structures of perovskites, including those distorted by tilting of symmetric octahedra or caused by Jahn–Teller distortions [46,47,48,49]. The stability of the predicted perovskite-related structures is determined using the global instability index (GII). The GII is obtained by comparing the calculated bond valence sums and the ideal formal valences, and it directly corresponds to the amount of the Ag quantity in BiFeO3 and the different Glazer tilt systems. The symmetry of the novel predicted structures was analyzed with the algorithms SFND [50] and RGS [51], all implemented in the KPLOT version 3 program [52]. The predicted structures were visualized using the VESTA 4.6.0 software [53].

2.4. Density Functional Theory (DFT) Calculations

We utilized SIESTA 5.2 [54] implementation of DFT to determine the atomic structure, electronic and magnetic properties of investigated crystal phases. Valence electrons were modeled using norm-conserving Troullier–Martins pseudopotentials [55], incorporating partial core corrections. A double-zeta basis set was utilized, and calculations for potentials and charge density were performed on a real-space grid with a mesh cutoff energy of 350 Ry, ensuring total energy convergence within 0.1 meV per unit cell during self-consistency procedures. Generalized-gradient approximation with Coulomb interaction potential, GGA+U, [56,57,58,59] was employed. At the same time, we fixed the Hubbard Ueff = 3.8 eV for Fe 3d orbital and Ueff = 0.95 for Fe 4s orbital in all calculations, which was successfully applied in our previous calculations [40]. The crystals were modeled using conventional unit cells that contain 30 atoms (for α- and R-type structures) or 40 atoms (for β-, γ-, T1- and T2-type structures). The Brillouin zone was sampled by following k-point grids using the Monkhorst–Pack sampling: 8 × 8 × 4 (α-type structure), 6 × 6 × 6 (β -type structure), 8 × 8 × 8 (γ-type structure), 8 × 8 × 4 (R-type structure), 6 × 8 × 6 (T1- and T2-type structures). Atomic structures were relaxed using the conjugate gradient method, where the structures were considered optimized when the maximal force on atoms dropped below 0.04 eV/Angstrom. We examined a ferromagnetic (FM) configuration along with three antiferromagnetic configurations labelled AFM-a, AFM-c and AFM-g by setting up initial atomic spin moments on Fe atoms, which were allowed to relax in self-consistent cycles.

3. Results

3.1. X-Ray Powder Diffraction (XRPD) and Rietveld Refinement

The most common way to clarify the structural changes caused by Ag doping is using Rietveld refinement. This procedure requires a structural model that is an approximation of the actual structure. The starting structural model for the rhombohedral R3cH (no.161) space group using a hexagonal setting was built up with crystallographic data reported in [60].
The structure refinement was carried out on a FullProf program [61] which adopts the Rietveld calculation method. A pseudo-Voigt function was chosen as a profile function among profiles in the refinement program. The refinement of the background was performed using linear interpolation between 56 selected points. The best fit between the calculated and observed X-ray diffraction pattern is shown in Figure 1a,b, where all allowed Bragg reflections are shown by vertical bars. Inspecting the difference between the experimental and calculated profiles indicates good agreement. Besides the difference curve (blue line), the good refinement is proven by the R (reliability) factors that have values between 1.7 and 2.7.
The crystal structures of Bi1−xAgxFeO3 (x = 0.01, 0.02) are shown in Figure 2.
The results of the Rietveld refinement, including unit cell parameters, atomic positions, and average bond distances, are given in Table 1. According to [62], the ionic radii of the Ag+ cation in coordination VI is 1.15 Å, and for the Bi3+ in the same coordination is 1.03 Å. Silver, instead of bismuth in the crystal lattice, will increase the unit cell parameters. In Table 1, we can follow the effect of entering silver in the structure of BiFeO3 by following values of the unit cell parameters, unit cell volume, and bond lengths. As expected, both investigated compounds have higher values for those parameters in comparison with undoped BiFeO3. Furthermore, we can see the difference between Bi0.98Ag0.02FeO3 where 2% of silver in the structure further increases the above-mentioned parameters in Bi0.98Ag0.02FeO3 if compared with Bi0.99Ag0.01FeO3. The changes in the crystal structure indicate the presence of silver in the structure, while the values of the occupation factors during Rietveld refinement were fixed to the nominal compositions of the powders.

3.2. CSP of Silver-Doped BiFeO3 Perovskites

A crystal structure prediction study was carried out in a bismuth ferrite system as a function of Ag concentration. Calculations were accomplished using bond valence calculations (BVCs) and SPuDS code to find possible additional structure candidates besides experimentally observed α-phase with perovskite structure type. Ten additional perovskite-related structure candidates were predicted, five with 0.1% silver in BFO and five with 0.2% Ag in BFO. Moreover, we predicted 0.5% Ag in BFO and generated an additional five structure candidates. These predicted structures are ranked according to the global instability index (GII) and tilt system and summarized in Table 2 (full structural data are presented in the Supporting Information).
The most stable BiFeO3 perovskite structure with silver doping, according to the GII criterion (Table 2), is the β-type in the space group Pnma. This is in agreement with previous experimental and theoretical data where the β-type structure was found in doped bismuth ferrite [30,31,36,40,62]. The GII ranging of the β-type structure increased from 0.02601 to 0.03542 at 2% Ag doping in BFO, indicating less stability with a higher level of silver dopant in BFO. The predicted β modification in the 2% Ag-doped BFO is shown in Figure 3a.
On the other hand, the γ-type of structure is the candidate with the highest level of the GII index, but the GII index is reduced with the increase in the Ag dopant, indicating that increasing silver concentration could stabilize this phase. This will be further confirmed later on in our DFT calculations (See Section 3.3). The gamma phase shows the highest cubic symmetry (Pm-3m (no. 221) space group) where the iron atoms are coordinated by six O atoms forming a perfect octahedron (Figure 3b). The γ-type modification was found in the experiments and theoretical studies in pure BiFeO3 at high temperatures, [6,63,64] and was also found in our previous research on pure BFO [40], indicating the possible existence of the gamma phase in the silver-doped bismuth ferrite.
The next three predicted silver-doped structures in BFO, the R-, T1- and T2-type, are almost the same according to the GII index regardless of silver concentration, indicating the same possibility of synthesis between the beta and the gamma phase. The R-type appears in the rhombohedral R-3c (no. 167) space group (Figure 4a), structurally related to the experimentally observed alpha phase, making the R-type a bit more favorable in silver-doped bismuth ferrite. This concurs with previous experimental and theoretical research on pure BiFeO3 where high-temperature rhombohedral modification of BiFeO3 was identified [65,66]. On the other hand, both tetragonal T1 and T2 structures are closely related, where T2 modification appears as a polytype of T1 [67] (Figure 4b,c). Still, both phases are easily distinguishable by symmetry, where the T1 type appears in the P4/mbm (no. 127) space group, and the T2 type shows I4/mcm (no. 140) space group. Previous literature data on various tetragonal modifications in pure BiFeO3 at high temperatures [6,40,68,69] indicate that both T1- and T2-phases have a high potential for experimental synthesis in silver-doped BiFeO3. In order to explore higher doping levels of Ag in BFO, we predicted these perovskite structure candidates in the Bi0.95Ag0.05FeO3 compound. However, very small changes in structure and unit cell parameters were detected, implying that larger amounts of silver do not have a significant impact on the crystal structure of BFO (full structural data are presented in the Supporting Information).

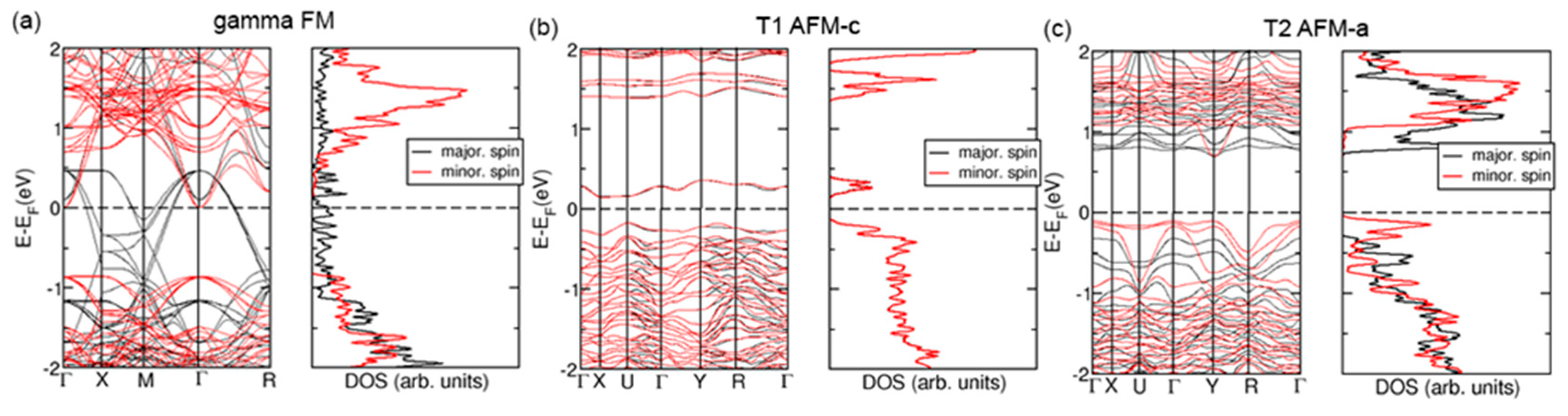
3.3. Electronic and Magnetic Properties—Density Functional Theory Study

Using the structures predicted by the BVCs method, we analyzed the electronic and magnetic properties of the doped materials. To investigate the doped systems, we substituted one Bi atom with one Ag atom per conventional unit cell. This corresponds to doping of 12.5% (1/8) for α- and R-type structures and 16.6% (1/6) for remaining structural types. Unit cells that correspond to smaller, closer to experimental, doping levels are significantly larger than the unit cells which we used in the DFT calculations. Larger unit cells and the correspondingly larger number of atoms per unit cell significantly increase the number of degrees of freedom, which made geometry optimizations difficult and, for most phases, unachievable for the quantum method; atomic forces or even electron density could not converge below desired tolerance levels. However, differences between bond lengths of the doped materials predicted by the BVCs method for doping levels of 5% and 12.5% do not exceed 0.26%, which fairly justifies the utilization of the smaller unit cells. Additionally, the BVCs study indicated the stabilization of a studied crystal, particularly the γ phase, by an increase in Ag doping. Our DFT calculations, as will be shown below, confirmed this indication for a significantly larger doping concentration than that considered in BVCs. We proceeded with DFT calculations in the following steps. First, using the conjugate gradient method, we optimized the atomic structure of the undoped crystals. The optimization was performed with atomic spins initially set up in a ferromagnetic FM, or one of the three antiferromagnetic spin orders, AFM-a, AFM-c or AFM-g. The spin orders were determined by initial spins on Fe atoms. Second, we substituted one Bi atom with one Ag atom in the optimized undoped structure. After setting up the atomic spins to the previously obtained values in undoped structures, the conjugate gradient optimization was conducted for the doped structures. Table 3 shows the energy released upon the substitution of one Bi atom by one Ag atom in the unit cell. It is calculated from the expression Edop = E(doped) + E(Bi) − E(undoped) − E(Ag), where E(doped) is the energy of the doped crystal, E(undoped) is the energy of the pristine crystal and E(Bi) and E(Ag) are energies of isolated Bi and Ag atoms. For the crystals with theoretically considered high levels of Ag doping, our DFT calculations indicate that only three phases can be substitutionally doped: γ, T1 and T2 with ground states having FM, AFM-c and AFM-a spin textures, respectively. Note that, e.g., molecular dynamics simulations (MDSs) would be essential to study the thermodynamic stability of these phases at finite temperatures, from, for example, the Lindemann index. However, MDSs are very “expensive” for investigated systems, in which atomic forces and density matrices were difficult to converge in self-consistent field iterations even in the conjugate gradient structural optimizations (essentially presenting quasi-dynamics of atomic structures at the absolute zero).
The spins were allowed to relax during self-consistent field iterations. For all investigated phases, the initial spin configurations were retained, while the obtained values of atomic spins are listed in Table S1 of the provided Supplementary Material. Two conclusions can be derived from Table S1:
  • Doping reduces the magnetization in the FM-ordered systems of all crystals by around 5% except for the R phase, for which magnetization is reduced by 7%.
  • In undoped systems, spins are perfectly compensated in AFM magnetic orders, whereas Ag doping induces net spins on Fe and O atoms in AFM magnetic textures.
Next, we show the electronic structure of the three systems with theoretically feasible high Ag doping, ferromagnetic γ-type structure, T1-type structure with AFM-c and T2-type structure with AFM-a spin textures, which are in ground electronic states according to our DFT calculations. Electronic band structures (BSs) and electronic density of states (DOS) for these phases are represented in Figure 5 in Panels (a), (b) and (c), respectively. The band structure of the ferromagnetic γ-type structure has mixed features of metal, semi-metal and half-metal. A band with a relatively large dispersion of about 2 eV starts at the center of the Brillouin zone (Γ-point at Γ-X segment) and crosses the Fermi level close to the M point. At the segment M-Γ-R, the bottom of the conduction band is placed below the top of the valence band, indicating the semi-metallic nature of the crystal. The majority spin characterizes the energy range from around −1 eV below the Fermi level to the Fermi level, indicating the half-metallic nature of the material in the γ-type structure. The T1 phase is nearly perfectly compensated antiferromagnet with AFM-c spin texture. It is also a narrow-gap semiconductor, with a direct gap of around 0.5 eV. In contrast, the T2-type structure with AFM-a spin texture is a semiconductor with a band gap of about 0.8 eV. Its valence band is characterized primarily by minority spin, whereas the conduction band has predominantly majority spin. The T2 phase has an indirect band gap, with the top of the valence band at the Γ-point and the bottom of the condition band at the Y point. We obtained electronic BS and DOS also for α, β and R phases and all combinations of spin textures. These are included in Figures S1 and S2 of the Supplementary Material.

3.4. Magnetic Properties

The temperature dependence of magnetization measured on the pellets of pure BFO, and BFO with 1% and 2% of Ag is shown in Figure 6. It can be seen that the incorporation of Ag increases the value and the irreversibility of magnetization but does not change the temperature of the magnetic phase transition (TN = 630 K). Our DFT calculations are in agreement with the experimental finding of magnetization increase by Ag doping in AFM spin orders, with spins localized at Fe and O atoms. While pure BFO is known to be an antiferromagnet with the spin cycloid magnetic order [70], often, the weak ferromagnetic moment can be found due to the microstructure of the samples. Very rarely, it is synthesized in monocrystal form, which would show the peak in the magnetization at the transition temperature, and more often it is found to be in the form of nanoparticles, where the non-compensated surface spins and the breaking of spin spiral periodicity (period 62 nm) attribute to the appearance of the magnetic moment. The substitution of nonmagnetic Ag enhances the development of the weak ferromagnetic moment, and the irreversibility of the magnetization increases as it disturbs the antiferromagnetic interaction of the Fe ions. A similar effect is also found in the substitution with other elements [71,72,73].
A peculiar behavior is observed in pure BFO and BFO with 1% of Ag, where the crossing of ZFC and FC curves is present around 280 K and 250 K, respectively. Such behavior was already observed and can be attributed to different origins such as the competition of the interactions between Fe in different magnetic sublattices spin freezing in surface layers of small particles and other types of canting in the antiferromagnetic spin cycloids, etc. [74,75]. Only the former origin was attainable by our DFT calculations since we modeled the systems as infinite crystals (i.e., without surfaces), and we performed collinear spin calculations that do not take into account the spin canting in an antiferromagnet.
From the field dependence of magnetization at 4 K and 300 K (Figure 7), additional conclusions can be drawn in the same direction. The substitution of Ag increases the value of magnetization, which is expressed in the larger changes at the lowest fields and the additional appearance of the S-shape is superimposed on the antiferromagnetic linear dependence, which is characteristic of the weak ferromagnetic behavior. The pronounced weak ferromagnetic hysteresis is found in the BFO with 2% of Ag also at room temperature, while 0% Ag and 1% Ag curves resemble much more the shape of antiferromagnetic MH loops. This shows that even the addition of only 2% of a nonmagnetic substituent can produce a weak ferromagnetic response at ambient temperatures, thereby opening the possibilities of developing such materials towards eventual applications in different multiferroic devices.

4. Conclusions

A comprehensive multidisciplinary study was conducted to investigate the impact of doping BiFeO3 with Ag atoms. Nanocrystalline powders of BiFeO3 and Bi1−xAgxFeO3 (with x = 0.01 and 0.02) were synthesized using the hydrothermal method. Samples doped with 1 and 2 atomic percent (at. %) of Ag were characterized using powder X-ray diffraction, and the structure refinement was carried out on a FullProf program, which adopts the Rietveld calculation method. The doped BiFeO3 samples, regardless of the Ag concentration, exhibited the α-phase structure in the space group R3cH. To explore additional possible structures in Ag-doped BiFeO3, bond valence calculations (BVCs) were employed for structure prediction. As a result, several structure candidates, including α-, β-, γ-, R-, T1 and T2 structures, were predicted in various Ag-doped BiFeO3 systems using BVCs. We studied the electronic and magnetic properties of these structures using DFT. Our DFT results are in agreement with the experimental finding of magnetization increase upon Ag doping in antiferromagnetically ordered structures and the possibility to dope the γ phase with a high Ag dopant concentration. Additionally, DFT calculations predict the semiconducting properties of antiferromagnetic T1 and T2 phases. More importantly, the half-metallic property of the γ-type structure that DFT calculations predicted, as well as the induced weak ferromagnetism with light doping, may be of great interest for further research due to its potential physical consequences and applications in future nanodevices.

Supplementary Materials

The following supporting information can be downloaded at: https://www.mdpi.com/article/10.3390/ma18071453/s1, Figure S1: Electronic band structure and density of states for all combinations of alpha, beta, gamma and R phases on one hand and FM, AFM-a, AFM-c and AFM-g spin orders on the other hand; Figure S2: Electronic band structure and density of states for all combinations of T1 and T2 phases on one hand and FM, AFM-a, AFM-c and AFM-g spin orders on the other hand. Table S1: Calculated values of the global instability index (GII) and tilt system of the most promising Bi0.99Ag0.01FeO3 modifications using the bond valence calculations (BVCs) method.; Table S2: Structural data of the most promising Bi0.99Ag0.01FeO3 perovskite modifications were calculated using the BVCs method; Table S3: Calculated values of the global instability index (GII) and tilt system of the most promising Bi0.98Ag0.02FeO3 modifications using the bond valence calculations (BVCs) method; Table S4: Structural data of the most promising Bi0.98Ag0.02FeO3 perovskite modifications were calculated using the BVCs method; Table S5 Atomic spins (in units of Bohr magneton) were obtained by DFT calculations for each of the undoped or Ag-doped phases for FM, AFM-a, AFM-c and AFM-g spin textures. For undoped crystals, the Ag atom is not present and so spin on the Ag atom is not available (N/A). Data for Alpha and R phases in AFM-c and AFM-g spin configurations are not available as well, as these results could not converge.

Author Contributions

Conceptualization, M.Č. and D.P.; methodology, M.Č, D.Z., I.P. and J.Z.; software, D.Z. and I.P.; validation, M.R. and D.P.; formal analysis, P.Š. and J.Z.; investigation, D.Z., P.Š., J.Z. and M.R.; writing—original draft preparation, I.P. and M.Č.; writing—review and editing, M.Č., M.R. and D.P.; visualization, D.Z., P.Š. and J.Z.; supervision, D.P. All authors have read and agreed to the published version of the manuscript.

Funding

This research was funded by the Ministry of Science, Technological Development and Innovation of the Republic of Serbia, based on Contract no. 451-03-136/2025-03/200017 (“Vinča” Institute of Nuclear Sciences—National Institute of the Republic of Serbia, University of Belgrade), 451-03-66/2024-03/200053 (Institute for Multidisciplinary Research, University of Belgrade), and by the Croatian Science Foundation, Zagreb, Croatia [project IP-2022-10-6321]; M. Č. acknowledges the support from the Bilateral Project Between Serbia and Austria (OeAD-GmbH-Project no. RS 24/2022) 337-00-577/2021-09/6. DFT calculations were performed using computational resources at the Center of Surface and Nanoanalytics, Johannes Kepler University, Linz, Austria. We thank Kurt Hingerl for providing the computational resources at JKU. P.Š. and D.P. acknowledge support from the CeNIKS, Grant No. KK.01.1.1.02.0013 co-financed by the Croatian Government and the European Union through the European Regional Development Fund—Competitiveness and Cohesion Operational Programme.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

The original contributions presented in this study are included in the article/Supplementary Material. Further inquiries can be directed to the corresponding authors.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Monem, A.M.A.; Abd-Elmohssen, N.; El-Bahnasawy, H.H.; Makram, N.; Sedeek, K. Comparative studies by X-ray diffraction, Raman, vibrating sample magnetometer and Mössbauer spectroscopy of pure, Sr doped and Sr, Co co-doped BiFeO3 ceramic synthesized via tartaric acid-assisted technique. Ceram. Int. 2023, 49, 15213–15220. [Google Scholar] [CrossRef]
  2. Jin, C.; Geng, W.; Wang, L.; Han, W.; Zheng, D.; Hu, S.; Ye, M.; Xu, Z.; Ji, Y.; Zhao, J.; et al. Tuning ferroelectricity, and ferromagnetism in BiFeO3/BiMnO3 superlattices. Nanoscale 2020, 12, 9810–9816. [Google Scholar] [CrossRef]
  3. Cheong, S.W.; Mostovoy, M. Multiferroics: A magnetic twist for ferroelectricity. Nat. Mater. 2007, 6, 13–20. [Google Scholar] [CrossRef] [PubMed]
  4. Haruna, A.; Abdulkadir, I.; Idris, S.O. Photocatalytic activity and doping effects of BiFeO3 nanoparticles in model organic dyes. Heliyon 2020, 6, e03237. [Google Scholar] [CrossRef]
  5. Freitas, V.F.; Dias, G.S.; Protzek, O.A.; Montanher, D.Z.; Catellani, I.B.; Silva, D.M.; C’otica, L.F.; dos Santos, I.A. Structural phase relations in perovskite–structured BiFeO3-based multiferroic compounds. J. Adv. Ceram. 2013, 2, 103–111. [Google Scholar] [CrossRef]
  6. Selbach, S.M.; Tybell, T.; Einarsrudand, T.M.; Grande, T. Size-dependent properties of multiferroic BiFeO3 nanoparticles. Chem. Mater. 2007, 19, 6478–6484. [Google Scholar] [CrossRef]
  7. Yakout, S.M. Spintronics and innovative memory devices: A review on advances in magnetoelectric BiFeO3. J. Supercond. Nov. Magnetism. 2021, 34, 317–338. [Google Scholar] [CrossRef]
  8. Rizwan, S.; Awan, S.; Irfan, S. Room-temperature ferromagnetism in Gd and Sn co-doped bismuth ferrite nanoparticles and co-doped BiFeO3/MXene (Ti3C2) nanohybrids of spintronics applications. Ceram. Int. 2020, 46 Pt A, 29011–29021. [Google Scholar] [CrossRef]
  9. Neogi, S.; Ghosh, R. Behavioral remodelling of perovskite BiFeO3 by Ag-doping strategies for enhanced and ppb level acetone sensing performance: An advanced enlightenment for selectivity and sensing mechanism. Appl Mater Today 2022, 29, 101611. [Google Scholar] [CrossRef]
  10. Xu, H.; Xu, J.; Li, H.; Zhang, W.; Zhang, Y.; Zhai, Z. Highly sensitive ethanol and acetone gas sensors with reduced working temperature based on Sr-doped BiFeO3 nanomaterial. J. Mater. Res. Technol. 2022, 17, 1955–1963. [Google Scholar] [CrossRef]
  11. Liu, H.; Sun, Y. Defect chemistry for Mn-doped and Nb-doped BiFeO3-based ceramics. J. Phys. Chem. Solids 2022, 170, 110951. [Google Scholar] [CrossRef]
  12. Aishwarya, K.; Navamathavan, R. Effect of grain size and orthorhombic phase of La-doped BiFeO3 on thermoelectric properties. J. Alloys Compd. 2023, 947, 169452. [Google Scholar] [CrossRef]
  13. Nazeer, Z.; Bibi, I.; Majid, F.; Kamal, S.; Ghafoor, A.; Ali, A.; Kausar, A.; Elqahtani, Z.M.; Alwadai, N.; Iqbal, M. Microemulsion synthesis of Ga and Sr doped BiFeO3 nanoparticles and evaluation of their ferroelectric, optical, dielectric and photocatalytic properties. Phys. B 2023, 657, 414788. [Google Scholar] [CrossRef]
  14. Anwar, A.; Basith, M.A.; Choudhury, S. From bulk to nano: A comparative investigation of structural, ferroelectric and magnetic properties of Sm and Ti co-doped BiFeO3 multiferroics. Mater. Res. Bull. 2019, 111, 93–101. [Google Scholar] [CrossRef]
  15. Chowdhury, S.S.; Kamal, A.H.M.; Hossain, R.; Hasan, M.; Islam, M.F.; Ahmmad, B.; Basith, M.A. Dy doped BiFeO3: A bulk ceramic with improved multiferroic properties compared to nano counterparts. Ceram. Int. 2017, 43, 9191–9199. [Google Scholar] [CrossRef]
  16. Moreau, J.M.; Michel, C.; Gerson, R.; James, W.J. Ferroelectric BiFeO3 X-ray and neutron diffraction study. J. Phys. Chem. Solids 1971, 32, 1315–1320. [Google Scholar] [CrossRef]
  17. Kubel, F.; Schmid, H. Structure of a ferroelectric and ferroelastic monodomain crystal of the perovskite BiFeO3. Acta Crystallog. B 1990, 46, 698–702. [Google Scholar] [CrossRef]
  18. Wang, J.; Neaton, J.B.; Zheng, H.; Nagarajan, V.; Ogale, S.B.; Liu, B.; Viehland, D.; Vaithyanathan, V.; Schlom, D.G.; Waghmare, U.V.; et al. Epitaxial BiFeO3 Multiferroic Thin Film Heterostructures. Science 2003, 299, 1719–1722. [Google Scholar] [CrossRef] [PubMed]
  19. Feng, H. Magnetism and electronic properties of BiFeO3 under lower pressure. J. Magn. Magn. Mater. 2010, 322, 3755–3759. [Google Scholar] [CrossRef]
  20. Catalan, G.; Scott, J.F. Physics and Applications of Bismuth Ferrite. Adv. Mater. 2009, 21, 2463–2485. [Google Scholar] [CrossRef]
  21. Martin, L.W.; Crane, S.P.; Chu, Y.H.; Holcomb, M.B.; Gajek, M.; Huijben, M.; Yang, C.H.; Balke, N.; Ramesh, R. Multiferroics and magnetoelectrics: Thin films and nanostructures. J. Phys.-Condens. Mat. 2008, 20, 434220. [Google Scholar] [CrossRef]
  22. Wu, J.; Fan, Z.; Xiao, D.; Zhu, J.; Wang, J. Multiferroic bismuth ferrite-based materials for multifunctional applications: Ceramic bulks, thin films and nanostructures. Prog. Mater. Sci. 2016, 84, 335–402. [Google Scholar] [CrossRef]
  23. Park, J.-G.; Le, M.D.; Jeong, J.; Lee, S. Structure and spin dynamics of multiferroic BiFeO3. J. Phys.-Condens. Mat. 2014, 26, 433202. [Google Scholar] [CrossRef]
  24. Wang, Y.; Wang, Y.; Wei, M.; Zhang, J.; Zhang, Y. Role of Ho Doping in Magnetization Mechanism of BiFeO3 Thin Films. J. Supercond. Nov. Magn. 2019, 32, 3495–3501. [Google Scholar] [CrossRef]
  25. Liu, Y.Q.; Wang, Y.J.; Zhang, J.; Gao, M.; Zhang, Y.J.; Wei, M.B.; Yang, J.H. Effect of Ho substitution on the structure and magnetic property of BiFeO3 prepared by sol-gel method. Mater. Sci. Semicond. Process. 2015, 40, 787–795. [Google Scholar] [CrossRef]
  26. Sharif, S.; Murtaza, G.; Meydan, T.; Williams, P.I.; Cuenca, J.; Hashimdeen, S.H.; Shaheen, F.; Ahmad, R. Structural, surface morphology, dielectric and magnetic properties of holmium doped BiFeO3 thin films prepared by pulsed laser deposition. Thin Solid Films 2018, 662, 83–89. [Google Scholar] [CrossRef]
  27. Hou, P.; Liu, B.; Guo, Z.; Zhou, P.; Wang, B.; Zhao, L. Effect of Ho doping on the crystal structure, surface morphology and magnetic property of BiFeO3 thin films prepared via the sol-gel technology. J. Alloys Compd. 2019, 775, 59–62. [Google Scholar] [CrossRef]
  28. Yun, Q.; Bai, A.; Zhao, S. Lattice distortion of holmium doped bismuth ferrite nanofilms. J. Rare Earth 2014, 32, 884–889. [Google Scholar] [CrossRef]
  29. Islam, M.R.; Zubair, M.A.; Galib, R.H.; Hoque, M.S.B.; Tomko, J.A.; Aryana, K.; Basak, A.K.; Hopkins, P.E. Vacancy-Induced Temperature-Dependent Thermal and Magnetic Properties of Holmium-Substituted Bismuth Ferrite Nanoparticle Compacts. ACS Appl. Mater. Interfaces 2022, 14, 25886–25897. [Google Scholar] [CrossRef]
  30. Chaturvedi, S.; Bag, R.; Sathe, V.; Kulkarni, S.; Singh, S. Holmium induced enhanced functionality at room temperature and structural phase transition at high temperature in bismuth ferrite nanoparticles. J. Mater. Chem. C 2016, 4, 780–792. [Google Scholar] [CrossRef]
  31. Stojadinović, B.; Dohčević-Mitrović, Z.; Stepanenko, D.; Rosić, M.; Petronijević, I.; Tasić, N.; Ilić, N.; Matović, B.; Stojanović, B. Dielectric and ferroelectric properties of Ho-doped BiFeO3 nanopowders across the structural phase transition. Ceram. Int. 2017, 43, 16531–16538. [Google Scholar] [CrossRef]
  32. Kuang, D.; Yang, F.; Wang, W.; Yang, Z. Effects of Ho3+ and transition metal ion doping on optical and magnetic properties of BiFeO3 nanopowders. J. Mater. Sci.-Mater. Electron. 2018, 29, 4041–4047. [Google Scholar] [CrossRef]
  33. Thakuria, P.; Joy, P.A. High room temperature ferromagnetic moment of Ho substituted nanocrystalline BiFeO3. Appl. Phys. Lett. 2010, 97, 162504. [Google Scholar] [CrossRef]
  34. Jeon, N.; Rout, D.; Kim, I.W.; Kang, S.-J.L. Enhanced multiferroic properties of single-phase BiFeO3 bulk ceramics by Ho doping. Appl. Phys. Lett. 2011, 98, 072901. [Google Scholar] [CrossRef]
  35. Pradhan, S.K.; Das, J.; Rout, P.P.; Mohanta, V.R.; Das, S.K.; Samantray, S.; Sahu, D.R.; Huang, J.L.; Verma, S.; Roul, B.K. Effect of holmium substitution for the improvement of multiferroic properties of BiFeO3. J. Phys. Chem. Solids 2010, 71, 1557–1564. [Google Scholar] [CrossRef]
  36. Van Minh, N.; Quan, N.G. Structural, optical and electromagnetic properties of Bi1−xHoxFeO3 multiferroic materials. J. Alloys Compd. 2011, 509, 2663–2666. [Google Scholar] [CrossRef]
  37. Muneeswaran, M.; Lee, S.H.; Kim, D.H.; Jung, B.S.; Chang, S.H.; Jang, J.-W.; Choi, B.C.; Jeong, J.H.; Giridharan, N.V.; Venkateswaran, C. Structural, vibrational, and enhanced magneto-electric coupling in Ho-substituted BiFeO3. J. Alloys Compd. 2018, 750, 276–285. [Google Scholar] [CrossRef]
  38. Li, X.; Zhang, Y.; Kang, W.; Yan, Z.; Shen, Y.; Huo, J. Anti-perovskite nitrides and oxides: Properties and preparation. Comp. Mater. Sci. 2023, 225, 112188. [Google Scholar] [CrossRef]
  39. Goldschmidt, V.M. Die gesetze der krystallochemie. Naturwissenschaften 1926, 14, 477–485. [Google Scholar] [CrossRef]
  40. Čebela, M.; Zagorac, D.; Batalović, K.; Radaković, J.; Stojadinović, B.; Spasojević, V.; Hercigonja, R. BiFeO3 perovskites: A multidisciplinary approach to multiferroics. Ceram. Int. 2017, 43, 1256–1264. [Google Scholar] [CrossRef]
  41. Rodriguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B 1993, 192, 55–69. [Google Scholar] [CrossRef]
  42. Zagorac, D.; Schön, J.C. Energy landscapes of pure and doped ZnO: From bulk crystals to nanostructures. Front. Nanosci. 2022, 21, 151–193. [Google Scholar] [CrossRef]
  43. Zagorac, J.; Zagorac, D.; Šrot, V.; Ranđelović, M.; Pejić, M.; van Aken, P.A.; Matović, B.; Schön, J.C. Synthesis, Characterization, and Electronic Properties of ZnO/ZnS Core/Shell Nanostructures Investigated Using a Multidisciplinary Approach. Materials 2023, 16, 326. [Google Scholar] [CrossRef]
  44. Buyer, C.; Grossholz, H.; Wolf, S.; Zagorac, D.; Zagorac, J.; Schön, J.C.; Schleid, T. Crystal-Structure Prediction and Experimental Investigation of the Polymorphic Lanthanum Fluoride Selenides LaFSe and La2F4Se. Cryst. Growth Des. 2022, 22, 7133–7142. [Google Scholar] [CrossRef]
  45. Pejić, M.; Zagorac, D.; Zagorac, J.; Matović, B.; Schön, J.C. Structure prediction via global energy landscape exploration of the ternary rare-earth compound LaOI. Z.. Anorg. Allg. Chem. 2022, 648, e202200308. [Google Scholar] [CrossRef]
  46. Zagorac, J.; Zagorac, D.; Zarubica, A.; Schön, J.C.; Djuris, K.; Matovic, B. Prediction of possible CaMnO3 modifications using an ab initio minimization data-mining approach. Acta Crystallogr. B Struct. Sci. Cryst. Eng. Mater. 2014, 70, 809–819. [Google Scholar] [CrossRef]
  47. Rosić, M.; Zagorac, D.; Milivojević, D.; Paunović, N.; Zagorac, J.; Dohčević-Mitrović, Z.; Matović, B. Theoretical and experimental study of octahedral tilting of Ca1-xGdxMnO3 (x = 0.05, 0.1, 0.15, 0.2) nanometric powders. J. Alloys Compd. 2016, 678, 219–227. [Google Scholar] [CrossRef]
  48. Lufaso, M.W.; Woodward, P.M. Prediction of the crystal structures of perovskites using the software program SPuDS. Acta Crystallogr. B 2001, 57, 725–738. [Google Scholar] [CrossRef]
  49. Lufaso, M.W.; Woodward, P.M. Jahn-Teller distortions, cation ordering and octahedral tilting in perovskites. Acta Crystallogr. B 2004, 60, 10–20. [Google Scholar] [CrossRef]
  50. Hundt, R.; Schön, J.C.; Hannemann, A.; Jansen, M. Determination of symmetries and idealized cell parameters for simulated structures. J. Appl. Crystallogr. 1999, 32, 413–416. [Google Scholar] [CrossRef]
  51. Hannemann, A.; Hundt, R.; Schön, J.C.; Jansen, M. A New Algorithm for Space-Group Determination. J. Appl. Crystallogr. 1998, 31, 922–928. [Google Scholar] [CrossRef]
  52. Hundt, R. KPLOT: A Program for Plotting and Analysing Crystal Structures; Technicum Scientific Publishing: Stuttgart, Germany, 2016. [Google Scholar]
  53. Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
  54. Soler, J.M.; Artacho, E.; Gale, J.D.; Garcia, A.; Junquera, J.; Ordejon, P.; Sanchez-Portal, D. The SIESTA Method for Ab initio Order-N Materials Simulation. J. Phys. Condens. Matter. 2002, 14, 2745. [Google Scholar] [CrossRef]
  55. Troullier, N.; Martins, J.L. Efficient Psudopotentials for Plane-wave Calculations. Phys. Rev. B 1991, 43, 1993–2006. [Google Scholar] [CrossRef]
  56. Liechtenstein, A.I.; Anisimov, V.I.; Zaanen, J. Density-functional theory and strong interactions: Orbital ordering in Mott-Hubbard insulators. Phys. Rev. B 1995, 52, R5467–R5470. [Google Scholar] [CrossRef]
  57. Anisimov, V.I.; Zaanen, J.; Andersen, O.K. Band theory and Mott insulators: Hubbard U instead of Stoner I. Phys. Rev. B 1991, 44, 943–954. [Google Scholar] [CrossRef]
  58. Vladimir, I.A.; Aryasetiawan, F.; Lichtenstein, A.I. First-principles calculations of the electronic structure and spectra of strongly correlated systems: The LDA+ U method. J. Phys.-Condens. Matter 1997, 9, 767. [Google Scholar] [CrossRef]
  59. Ivanov, S.A.; Nordblad, P.; Tellgren, R.; Ericsson, T.; Korchagina, S.K.; Rybakova, L.F.; Hewat, A. Influence of PbZrO3 doping on the structural and magnetic properties of BiFeO3. Solid State Sci. 2008, 10, 1875–1885. [Google Scholar] [CrossRef]
  60. Roisnel, T.; Rodriguez-Carvajal, J. WinPLOTR: A Windows tool for powder diffraction pattern analysis. Mater. Sci. Forum 2001, 378, 118–123. [Google Scholar] [CrossRef]
  61. Shannon, R. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. A 1976, 32, 751–767. [Google Scholar] [CrossRef]
  62. Chaturvedi, S.; Sarkar, I.; Shirolkar, M.M.; Jeng, U.-S.; Yeh, Y.-Q.; Rajendra, R.; Ballav, N.; Kulkarni, S. Probing bismuth ferrite nanoparticles by hard x-ray photoemission: Anomalous occurrence of metallic bismuth. Appl. Phys. Lett. 2014, 105, 102910. [Google Scholar] [CrossRef]
  63. Palai, R.; Katiyar, R.S.; Schmid, H.; Tissot, P.; Clark, S.J.; Robertson, J.; Redfern, S.A.T.; Catalan, G.; Scott, J.F. β phase and γ−β metal-insulator transition in multiferroic BiFeO3. Phys. Rev. B 2008, 77, 014110. [Google Scholar] [CrossRef]
  64. Haumont, R.; Kreisel, J.; Bouvier, P.; Hippert, F. Phonon anomalies and the ferroelectric phase transition in multiferroic BiFeO3. Phys. Rev. B 2006, 73, 132101. [Google Scholar] [CrossRef]
  65. Selbach, S.M.; Tybell, T.; Einarsrud, M.-A.; Grande, T. The Ferroic Phase Transitions of BiFeO3. Adv. Mater. 2008, 20, 3692–3696. [Google Scholar] [CrossRef]
  66. Munro, J.M.; Akamatsu, H.; Padmanabhan, H.; Liu, V.S.; Shi, Y.; Chen, L.-Q.; VanLeeuwen, B.K.; Dabo, I.; Gopalan, V. Discovering minimum energy pathways via distortion symmetry groups. Phys. Rev. B 2018, 98, 085107. [Google Scholar] [CrossRef]
  67. Zagorac, D.; Zagorac, J.; Schön, J.C.; Stojanović, N.; Matović, B. ZnO/ZnS (hetero)structures: Ab initio investigations of polytypic behaviour of mixed ZnO and ZnS compounds. Acta Crystallogr. B 2018, 74, 628–642. [Google Scholar] [CrossRef]
  68. Ravindran, P.; Vidya, R.; Kjekshus, A.; Fjellvåg, H.; Eriksson, O. Theoretical investigation of magnetoelectric behaviour in BiFeO3. Phys. Rev. B 2006, 74, 224412. [Google Scholar] [CrossRef]
  69. Diéguez, O.; González-Vázquez, O.E.; Wojdeł, J.C.; Íñiguez, J. First-principles predictions of low-energy phases of multiferroic BiFeO3. Phys. Rev. B 2011, 83, 094105. [Google Scholar] [CrossRef]
  70. Lu, J.; Günther, A.; Schrettle, F.; Mayr, F.; Krohns, S.; Lunkenheimer, P.; Pimenov, A.; Travkin, V.D.; Mukhin, A.A.; Loidl, A. On the room temperature multiferroic BiFeO3: Magnetic, dielectric and thermal properties. Eur. Phys. J. B 2010, 75, 451–460. [Google Scholar] [CrossRef]
  71. Li, Y.; Liu, L.; Wang, D.; Zhang, H.; He, X.; Li, Q. The Study of Magnetic Properties for Non-Magnetic Ions Doped BiFeO3. Materials 2021, 14, 4061. [Google Scholar] [CrossRef]
  72. Luković Golić, D.; Radojković, A.; Dapčević, A.; Pajić, D.; Dragović, J.; Torić, F.; Ćirković, J.; Branković, G.; Branković, Z. Change in structural, ferroelectric, and magnetic properties of bismuth ferrite induced by doping with gadolinium. Ceram. Int. 2019, 45, 19158–19165. [Google Scholar] [CrossRef]
  73. Radojković, A.; Luković Golić, D.; Ćirković, J.; Marinković Stanojević, Z.; Pajić, D.; Torić, F.; Dapčević, A.; Vulić, P.; Branković, Z.; Branković, G. Tuning of BiFeO3 multiferroic properties by light doping with Nb. Ceram. Int. 2018, 44, 16739–16744. [Google Scholar] [CrossRef]
  74. Suchomski, C.; Reitz, C.; Pajic, D.; Jaglicic, Z.; Djerdj, I.; Brezesinski, T. Large-Pore Mesoporous Ho3Fe5O12 Thin Films with a Strong Room-Temperature Perpendicular Magnetic Anisotropy by Sol-Gel Processing. Chem. Mater. 2014, 26, 2337–2343. [Google Scholar] [CrossRef]
  75. Englich, J.; Lütgemeier, H.; Pieper, M.W.; Jülich, K.F.A.; Germany, W.; Nekvasil, V.; Novák, P. Umbrella structure in Ho substituted YIG–NMR study. Solid State Commun. 1985, 56, 825–828. [Google Scholar] [CrossRef]
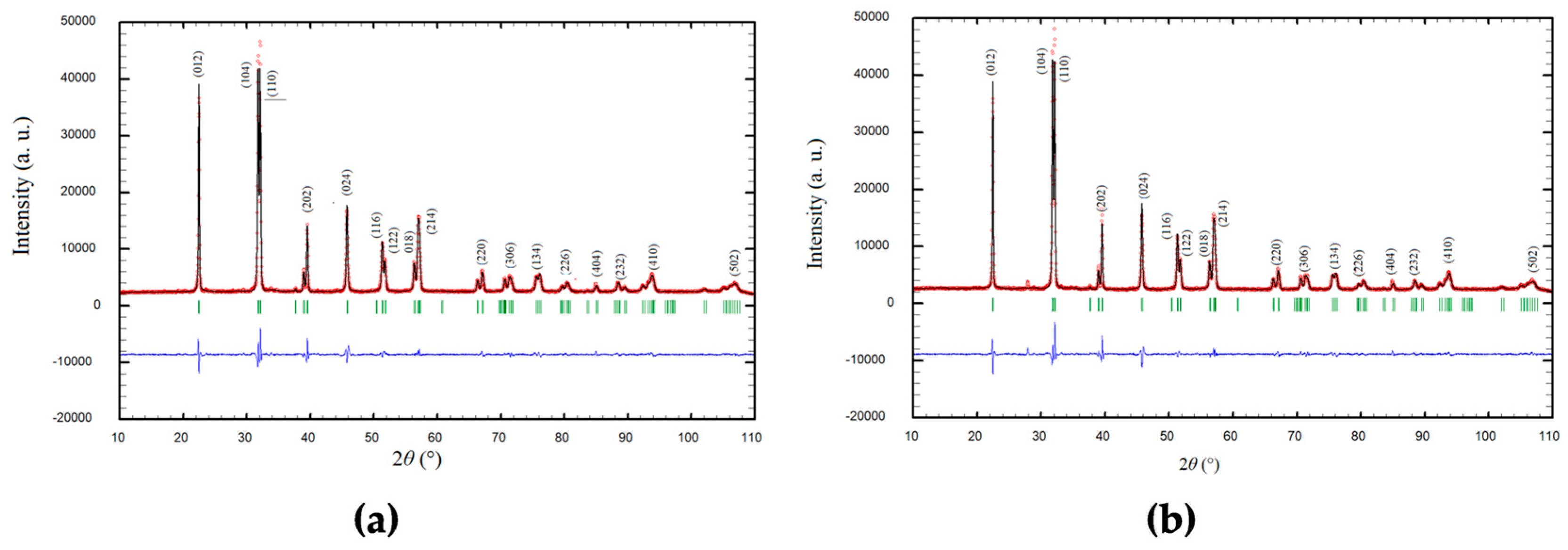
Materials 18 01453 g001
Figure 1. The structural refinement patterns of (a) Bi0.99Ag0.01FeO3 and (b) Bi0.98Ag0.02FeO3. A difference (observed–calculated) plot is shown beneath (blue line). Green stick marks above the different data indicate the reflection position. Red color denotes the experimental diffraction pattern.
Figure 1. The structural refinement patterns of (a) Bi0.99Ag0.01FeO3 and (b) Bi0.98Ag0.02FeO3. A difference (observed–calculated) plot is shown beneath (blue line). Green stick marks above the different data indicate the reflection position. Red color denotes the experimental diffraction pattern.
Materials 18 01453 g001
Materials 18 01453 g002
Figure 2. The refined silver-doped α-BiFeO3 structure in the rhombohedral R3cH (no.161) space group using a hexagonal setting. Green spheres correspond to Bi atoms, while red color corresponds to silver doping, blue atoms correspond to oxygen and orange atoms correspond to iron, represented by Fe-O octahedra.
Figure 2. The refined silver-doped α-BiFeO3 structure in the rhombohedral R3cH (no.161) space group using a hexagonal setting. Green spheres correspond to Bi atoms, while red color corresponds to silver doping, blue atoms correspond to oxygen and orange atoms correspond to iron, represented by Fe-O octahedra.
Materials 18 01453 g002
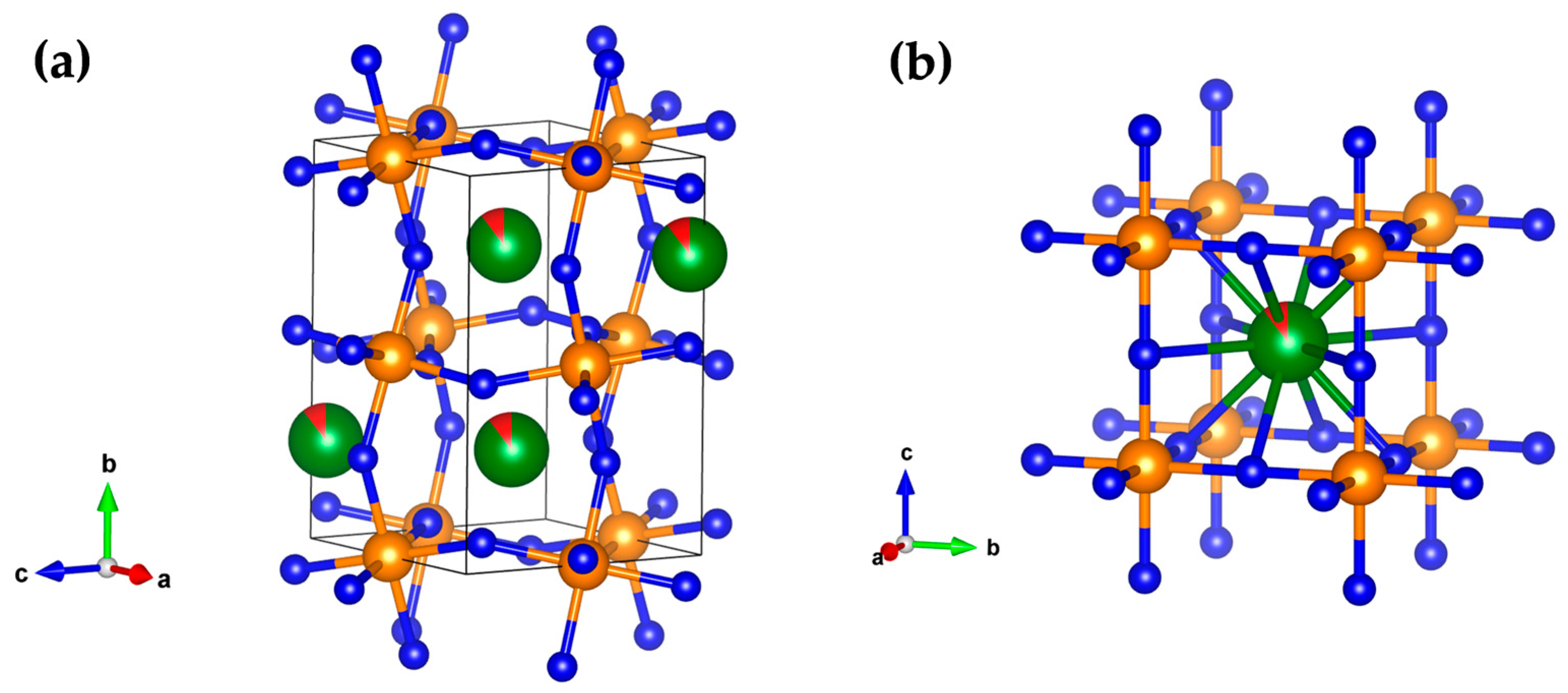
Materials 18 01453 g003
Figure 3. Visualization of the predicted: (a) β-type of structure in the space group Pnma (no. 62); (b) γ-type in the cubic Pm-3m (no. 221) space group, in the Bi0.98Ag0.02FeO3 compound.
Figure 3. Visualization of the predicted: (a) β-type of structure in the space group Pnma (no. 62); (b) γ-type in the cubic Pm-3m (no. 221) space group, in the Bi0.98Ag0.02FeO3 compound.
Materials 18 01453 g003
Materials 18 01453 g004
Figure 4. Visualization of the predicted: (a) R-type in the rhombohedral R-3c (no. 167) space group; (b) T1-type structure appearing in the space group P4/mbm (no. 127); (c) T2-type appearing in the I4/mcm (no. 140) space group in the Bi0.98Ag0.02FeO3 compound.
Figure 4. Visualization of the predicted: (a) R-type in the rhombohedral R-3c (no. 167) space group; (b) T1-type structure appearing in the space group P4/mbm (no. 127); (c) T2-type appearing in the I4/mcm (no. 140) space group in the Bi0.98Ag0.02FeO3 compound.
Materials 18 01453 g004
Materials 18 01453 g005
Figure 5. Electronic band structure (BS) and density of states (DOS) for ferromagnetic γ phase (a), T1 phase with AFM-c spin texture (b) and T2 phase with AFM-a spin texture (c). The figure on the left side of each panel represents BS, while the right-hand side figure of each panel shows DOS. The vertical scale of DOS figures matches the ones of BS. Features of majority spins are depicted by black lines, while those of minority spins are in red. Dash lines indicate Fermi’s energy.
Figure 5. Electronic band structure (BS) and density of states (DOS) for ferromagnetic γ phase (a), T1 phase with AFM-c spin texture (b) and T2 phase with AFM-a spin texture (c). The figure on the left side of each panel represents BS, while the right-hand side figure of each panel shows DOS. The vertical scale of DOS figures matches the ones of BS. Features of majority spins are depicted by black lines, while those of minority spins are in red. Dash lines indicate Fermi’s energy.
Materials 18 01453 g005
Materials 18 01453 g006
Figure 6. The temperature dependence of magnetization was measured in the magnetic field of 1 kOe on the pellets of pure BFO (black circles), BFO with 1% Ag (red rectangles) and 2% Ag (blue triangles). The empty symbols represent the ZFC, and the full symbols FC magnetization curves.
Figure 6. The temperature dependence of magnetization was measured in the magnetic field of 1 kOe on the pellets of pure BFO (black circles), BFO with 1% Ag (red rectangles) and 2% Ag (blue triangles). The empty symbols represent the ZFC, and the full symbols FC magnetization curves.
Materials 18 01453 g006
Materials 18 01453 g007
Figure 7. Field dependence of magnetization at 4 K and 300 K (inset), measured on the pellets of pure BFO (black circles), BFO with 1% Ag (red rectangles) and 2% Ag (blue triangles).
Figure 7. Field dependence of magnetization at 4 K and 300 K (inset), measured on the pellets of pure BFO (black circles), BFO with 1% Ag (red rectangles) and 2% Ag (blue triangles).
Materials 18 01453 g007
Table 1. Refined structure parameters for Bi0.99Ag0.01FeO3 and Bi0.98Ag0.02FeO3 in comparison with pure BiAgFeO3.
Table 1. Refined structure parameters for Bi0.99Ag0.01FeO3 and Bi0.98Ag0.02FeO3 in comparison with pure BiAgFeO3.
SampleStructure ParametersDistances
Bi0.99Ag0.01FeO3a = 5.57708(6)
c = 13.8667(3)
V = 373.52 Å3
Bi/Ag 0 0 0
Fe 0 0 0.2170(2)
O 0.570(1) 0.027(1) 0.9512(5)		3 × (Bi-O) = 2.5651(19) Å
3 × (Bi-O) = 2.227(6) Å
Average = 2.3963 Å
3 × (Fe-O) = 2.002(4) Å
3 × (Fe-O) = 2.045(5) Å
Average = 2.0231 Å
Bi0.98Ag0.02FeO3a = 5.57765(6)
c = 13.8682(3)
V = 373.64 Å3
Bi/Ag 0 0 0
Fe 0 0 0.2208(2)
O 0.562(2) 0.022(1) 0.9533(6)		3 × (Bi-O) = 2.586(3) Å
3 × (Bi-O) = 2.262(8) Å
Average = 2.4244 Å
3 × (Fe-O) = 1.963(5) Å
3 × (Fe-O) = 2.075(7) Å
Average = 2.0191 Å
BiFeO3 [1]a = 5.5767(3)
c = 13.8639(8)
V = 373.40 Å3
Bi 0 0 0
Fe 0 0 0.2208(2)
O 0.4478(4) 0.0196(5) 0.9522(2)		3 × (Bi-O) = 2.533(3) Å
3 × (Bi-O) = 2.265(3) Å
Average = 2.3989 Å
3 × (Fe-O) = 1.957(5) Å
3 × (Fe-O) = 2.101(7) Å
Average = 2.0289 Å
Table 2. Computed values of the GII and tilt system of the most promising predicted structure candidates in the (a) Bi0.99Ag0.01FeO3; (b) Bi0.98Ag0.02FeO3 system. Calculations were carried out using the BVCs method.
Table 2. Computed values of the GII and tilt system of the most promising predicted structure candidates in the (a) Bi0.99Ag0.01FeO3; (b) Bi0.98Ag0.02FeO3 system. Calculations were carried out using the BVCs method.
CompositionBi0.99Ag0.01FeO3
ModificationSpace GroupTilt SystemGII
β-typePnmaa−b+a−0.02601
R-typeR-3ca−a−a−0.13907
T1-typeP4/mbma0a0c−0.14216
T2-typeI4/mcma0a0c−0.14216
γ-typePm-3ma0a0a00.76254
Bi0.98Ag0.02FeO3
β-typePnmaa−b+a−0.03542
R-typeR-3ca−a−a−0.14136
T1-typeP4/mbma0a0c−0.14433
T2-typeI4/mcma0a0c−0.14433
γ-typePm-3ma0a0a00.75986
Table 3. Energy (eV/unit cell) for substitution of one Bi atom by one Ag atom in the unit cell. The doping energy is calculated using the following formula: Edop = E(doped) + E(Bi) − E(undoped) − E(Ag). The fields marked in blue indicate the doping energies in the ground states.
Table 3. Energy (eV/unit cell) for substitution of one Bi atom by one Ag atom in the unit cell. The doping energy is calculated using the following formula: Edop = E(doped) + E(Bi) − E(undoped) − E(Ag). The fields marked in blue indicate the doping energies in the ground states.
PhaseFMAFM-aAFM-cAFM-g
α0.1032.351N/AN/A
β1.3571.6391.7372.077
γ−0.3500.1270.7041.531
R0.1021.623N/AN/A
T1−0.383−0.294−0.609−0.133
T2−0.068−0.3531.456−0.073
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Čebela, M.; Šenjug, P.; Zagorac, D.; Popov, I.; Zagorac, J.; Rosić, M.; Pajić, D. Synthesis, Structural and Magnetic Properties of BiFeO3 Substituted with Ag. Materials 2025, 18, 1453. https://doi.org/10.3390/ma18071453

AMA Style

Čebela M, Šenjug P, Zagorac D, Popov I, Zagorac J, Rosić M, Pajić D. Synthesis, Structural and Magnetic Properties of BiFeO3 Substituted with Ag. Materials. 2025; 18(7):1453. https://doi.org/10.3390/ma18071453

Chicago/Turabian Style

Čebela, Maria, Pavla Šenjug, Dejan Zagorac, Igor Popov, Jelena Zagorac, Milena Rosić, and Damir Pajić. 2025. "Synthesis, Structural and Magnetic Properties of BiFeO3 Substituted with Ag" Materials 18, no. 7: 1453. https://doi.org/10.3390/ma18071453

APA Style

Čebela, M., Šenjug, P., Zagorac, D., Popov, I., Zagorac, J., Rosić, M., & Pajić, D. (2025). Synthesis, Structural and Magnetic Properties of BiFeO3 Substituted with Ag. Materials, 18(7), 1453. https://doi.org/10.3390/ma18071453

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop