Influence of Preheating on the Microstructure of a Hot Extruded Nickel-Based Superalloy
Abstract
1. Introduction
2. Materials and Experiments
3. Results
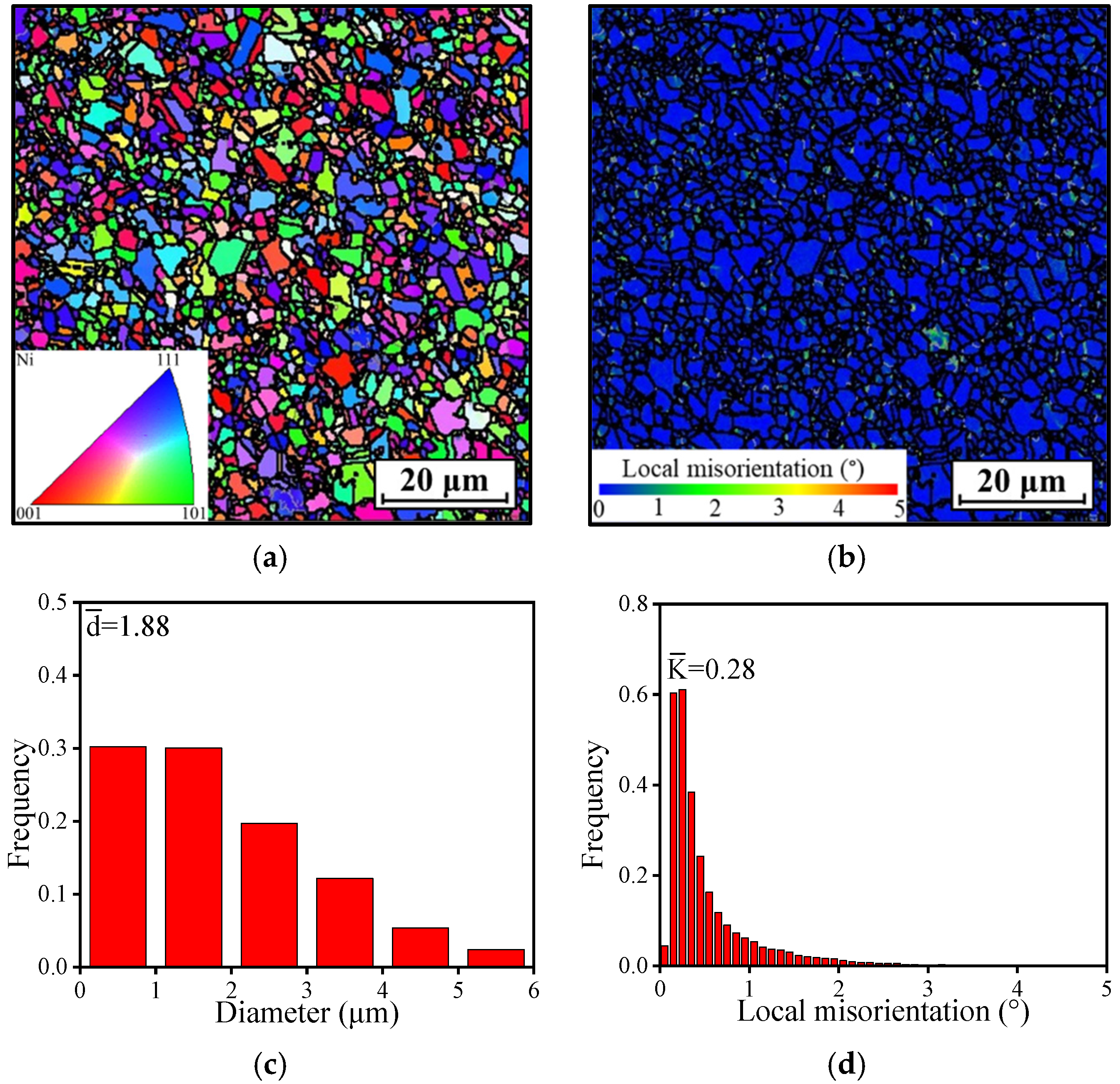
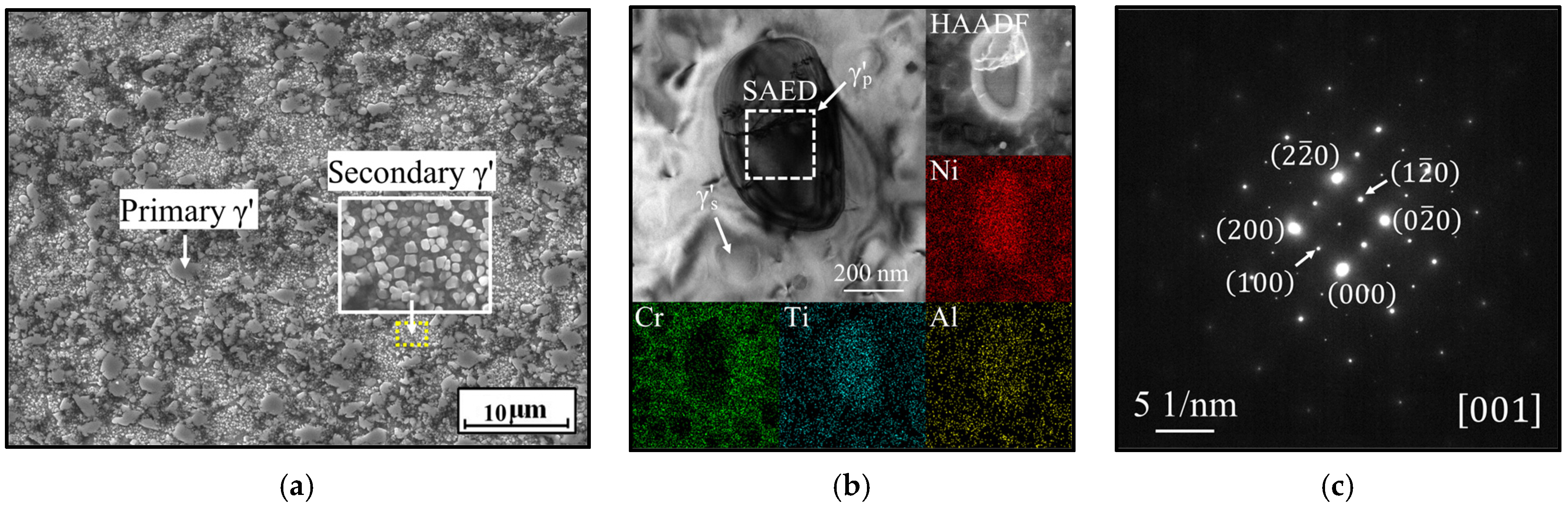
3.1. Microstructures of Extruded Alloy
3.2. Microstructure Evolution During Preheating
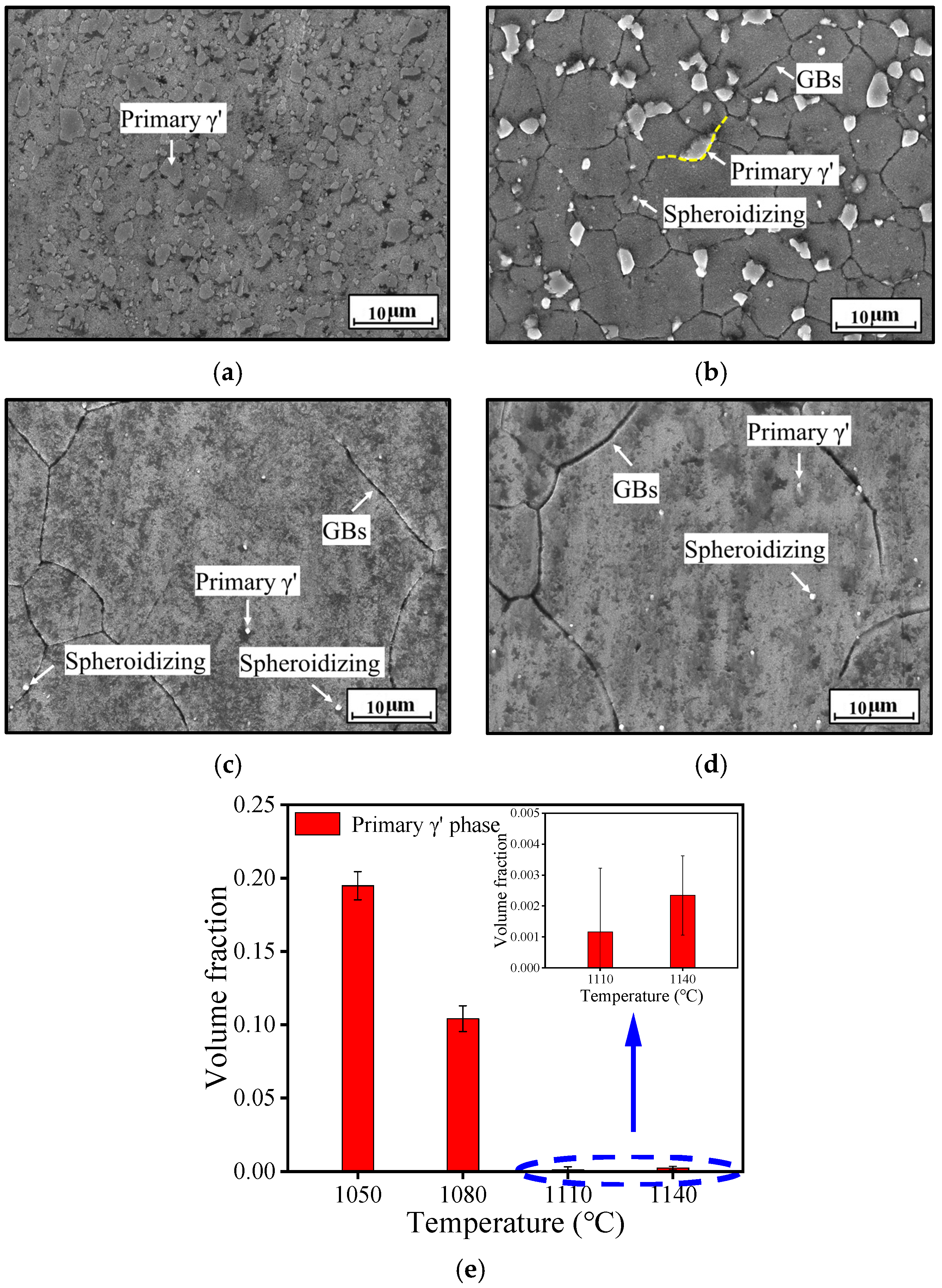
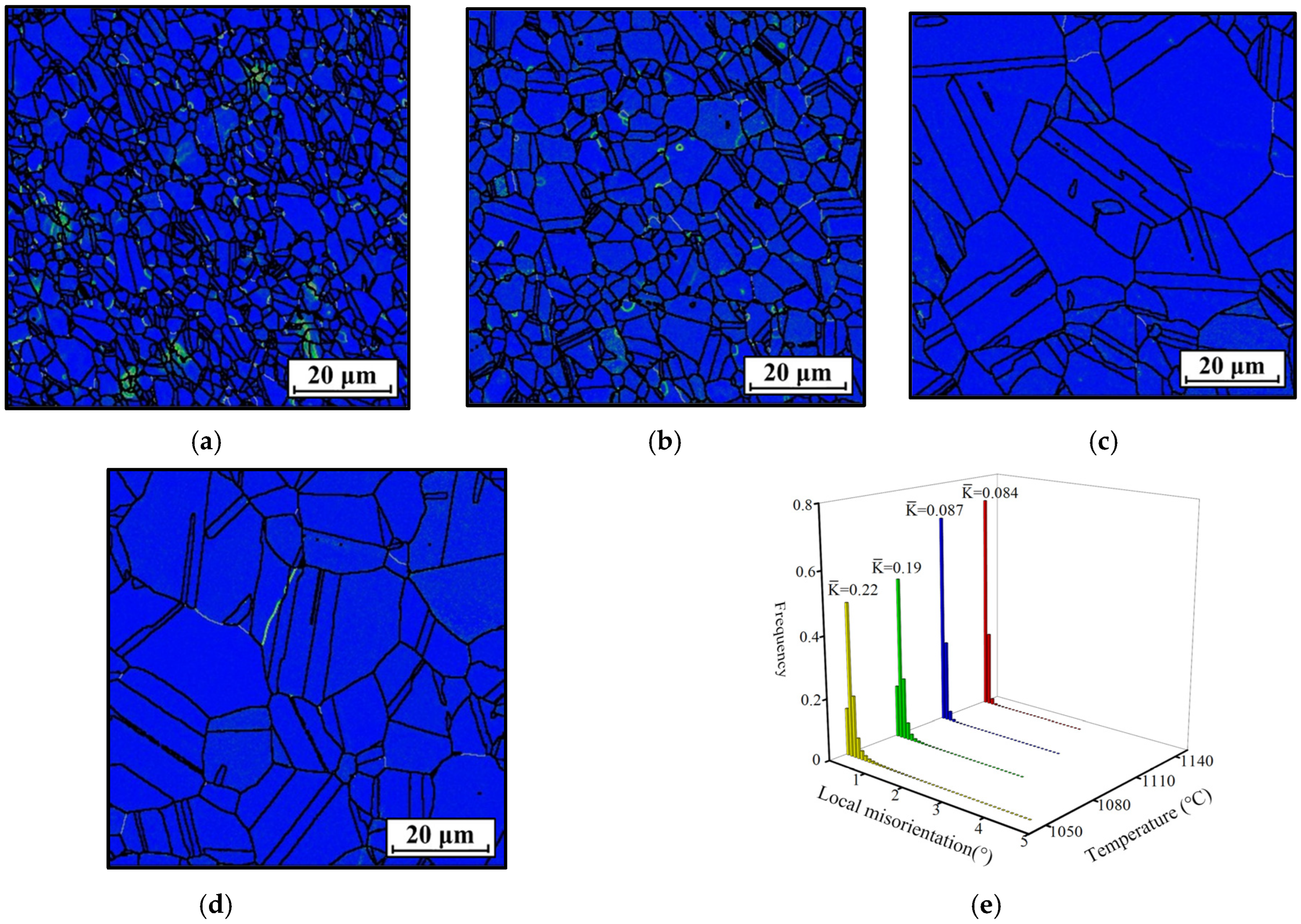
3.2.1. Effect of Heating Temperature
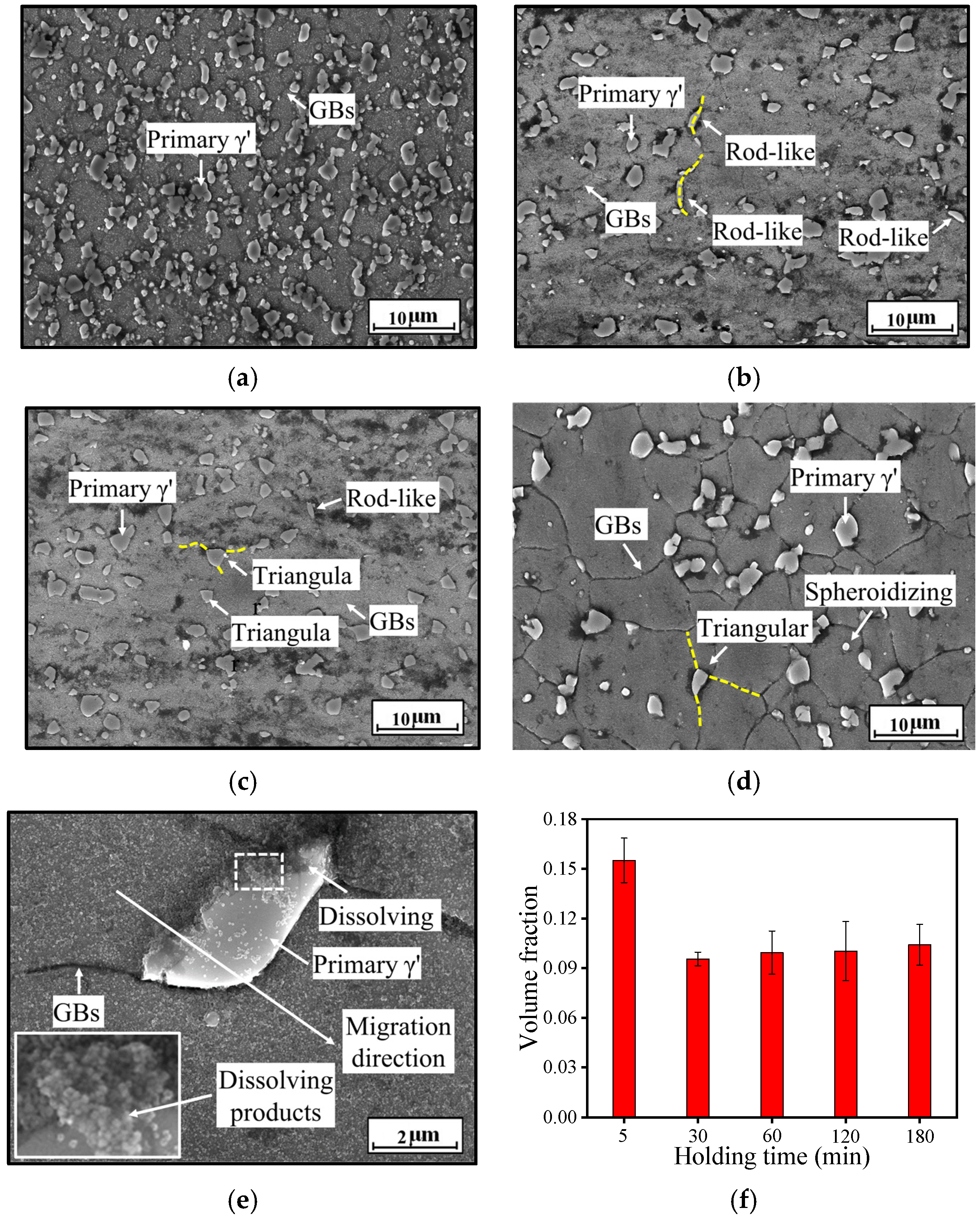
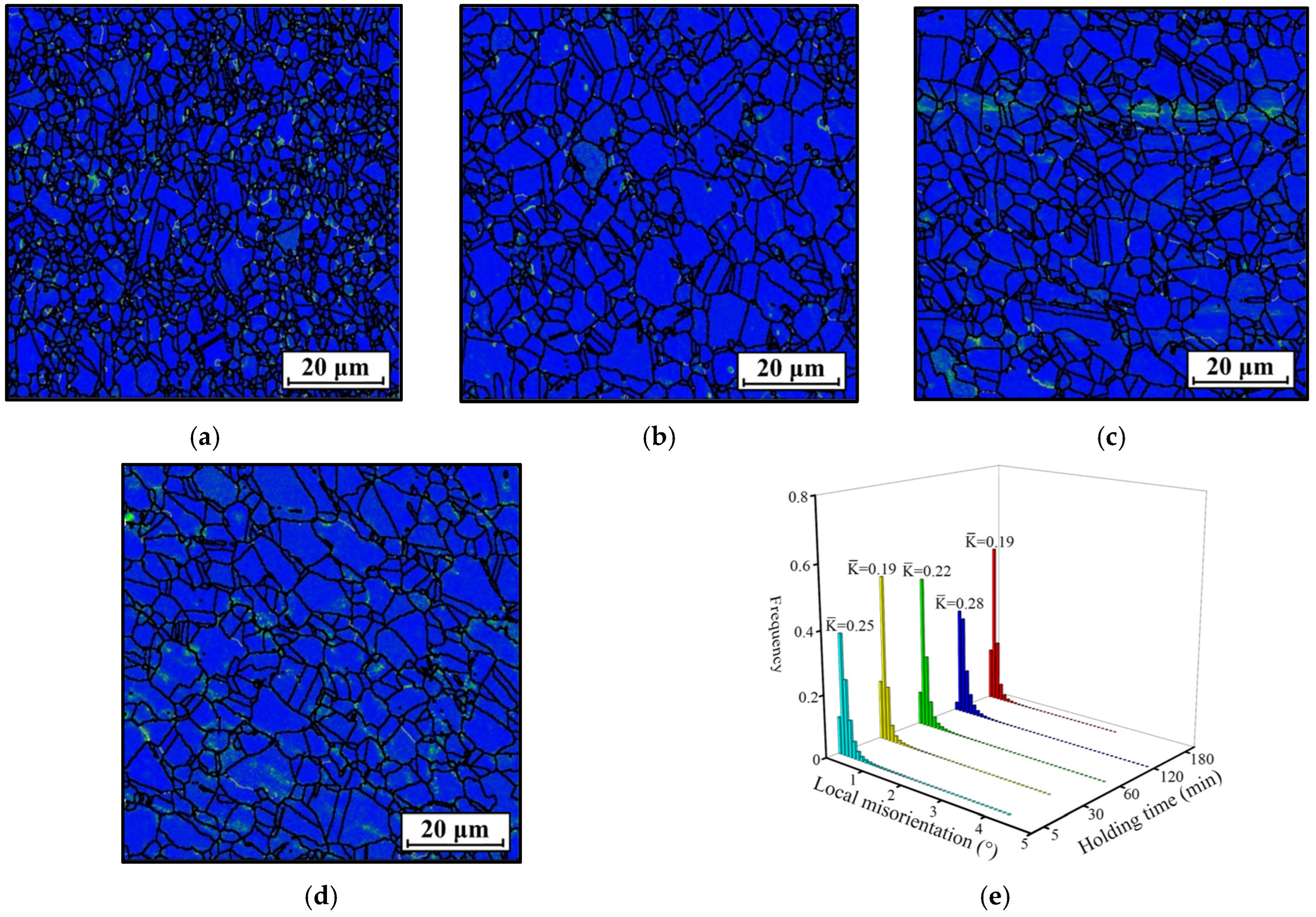
3.2.2. Effect of Holding Time
4. Discussion
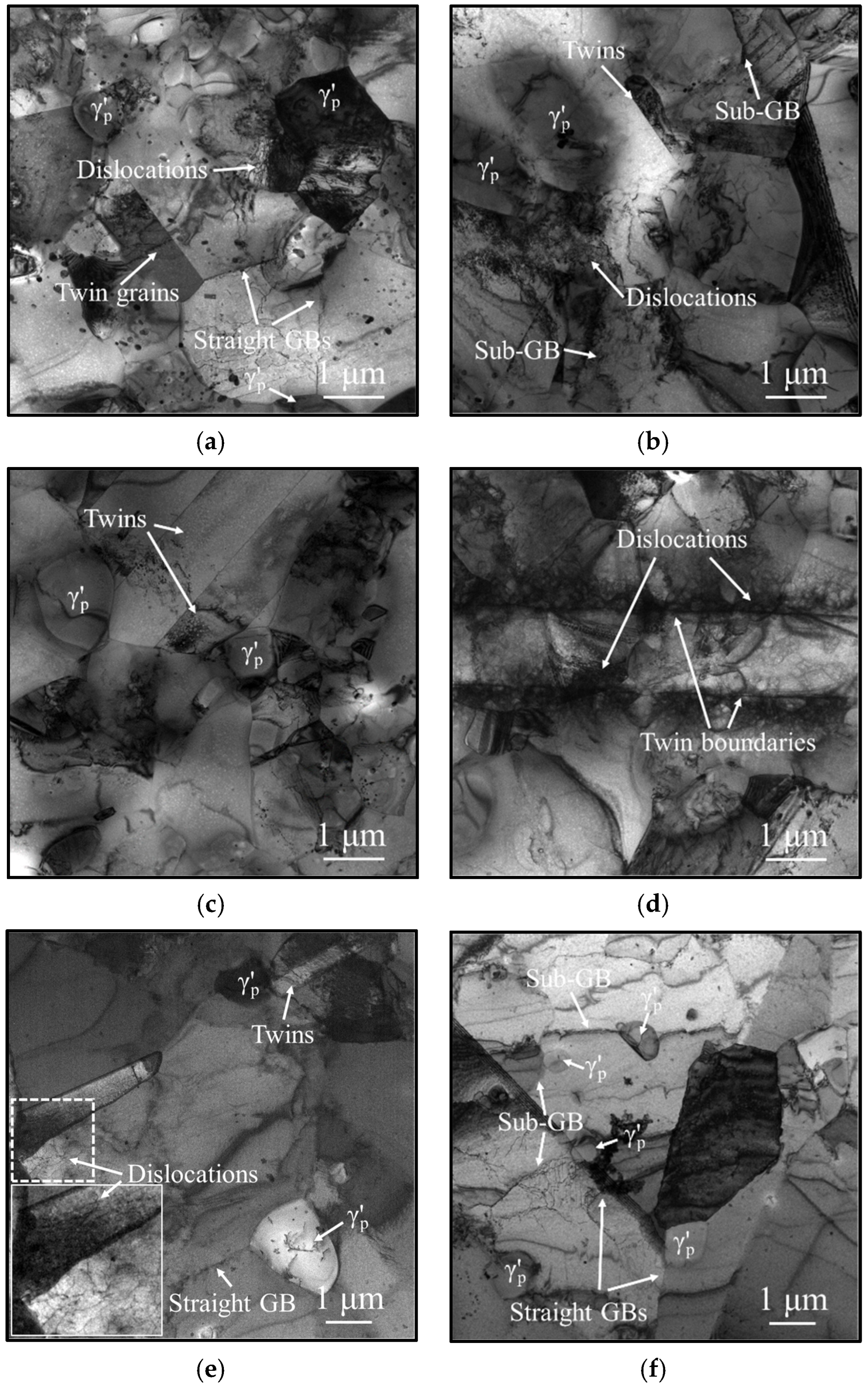
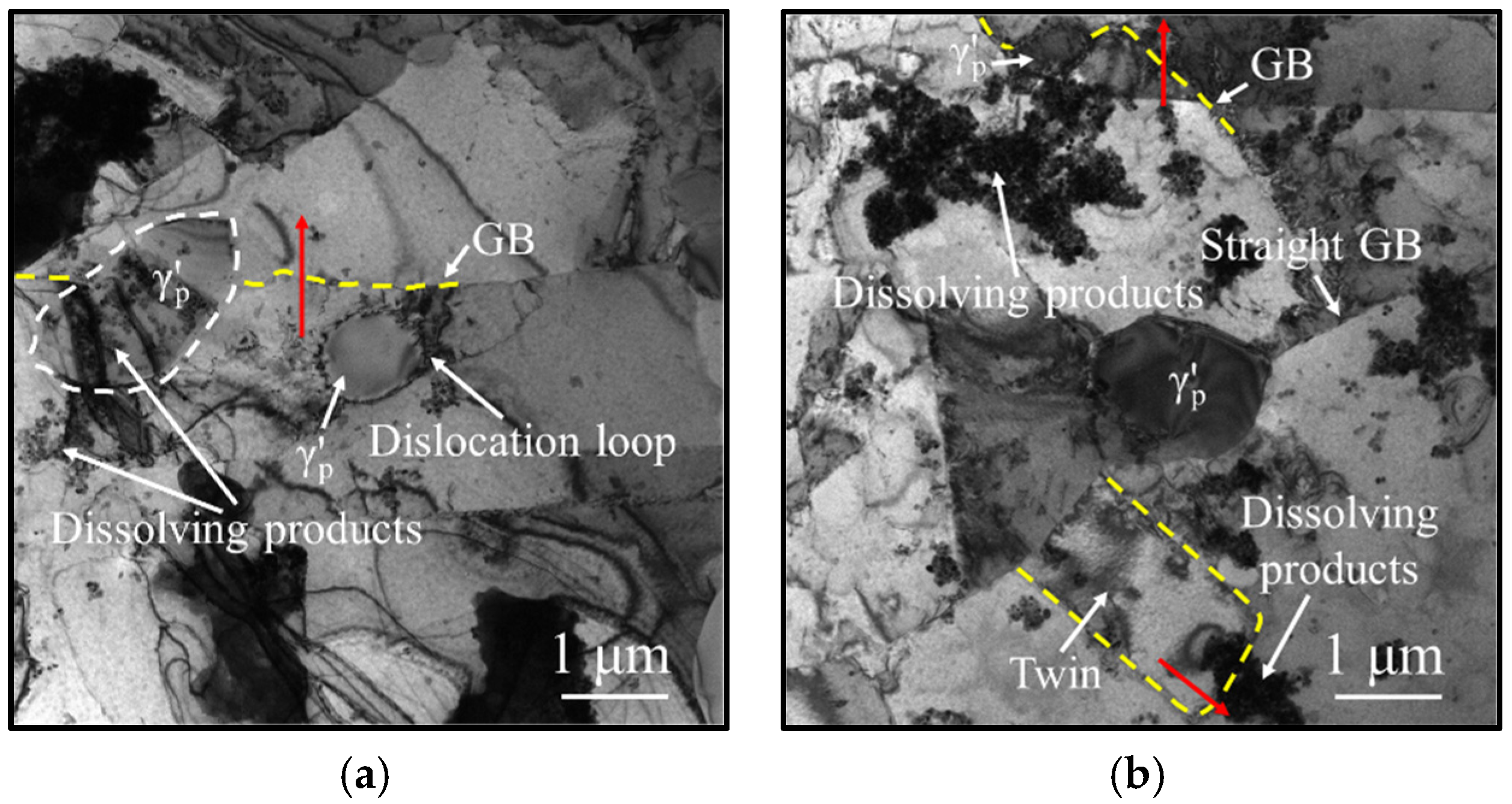
4.1. Interaction Mechanism Between the γ′p Phase and the Grain Structure
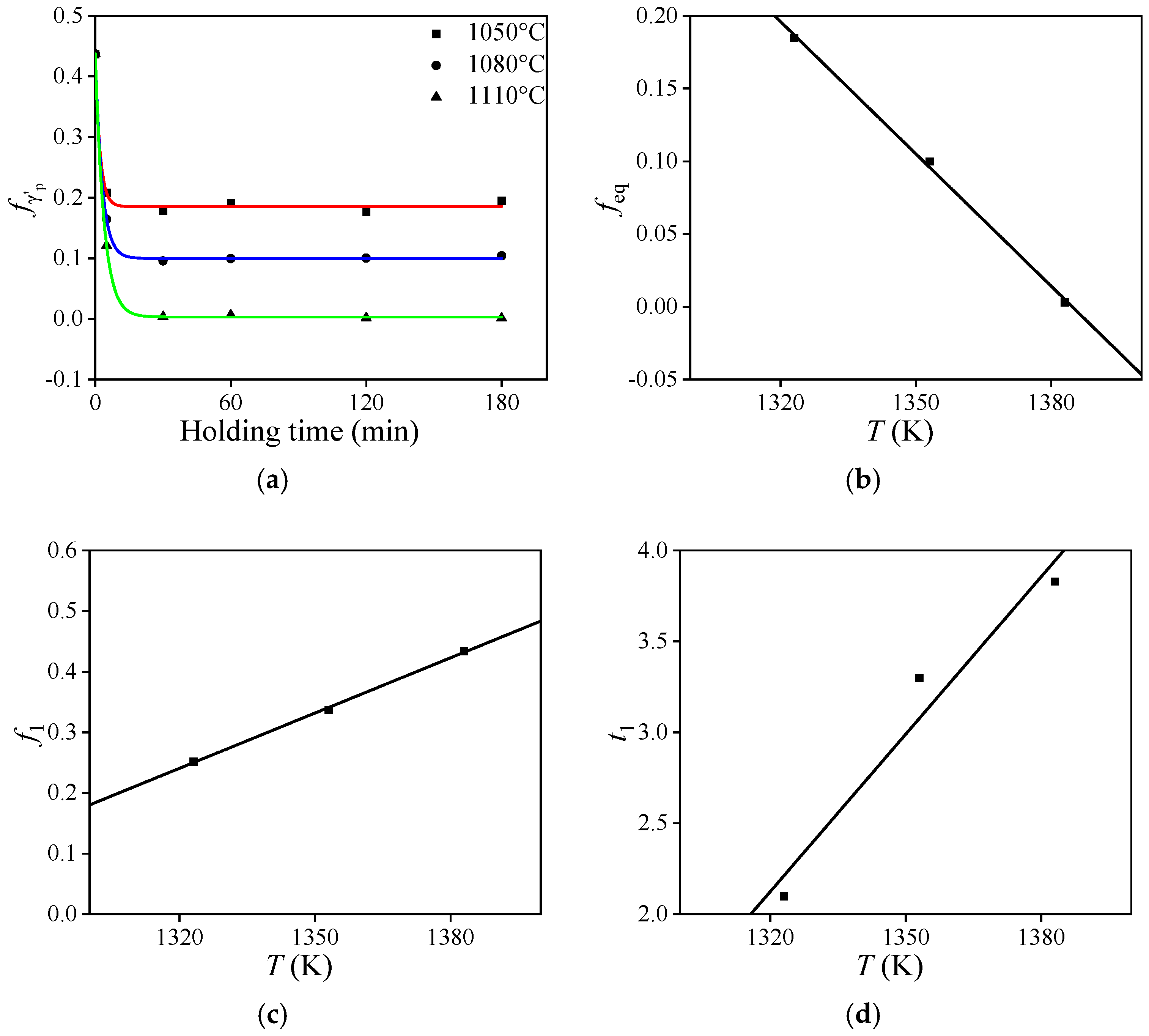
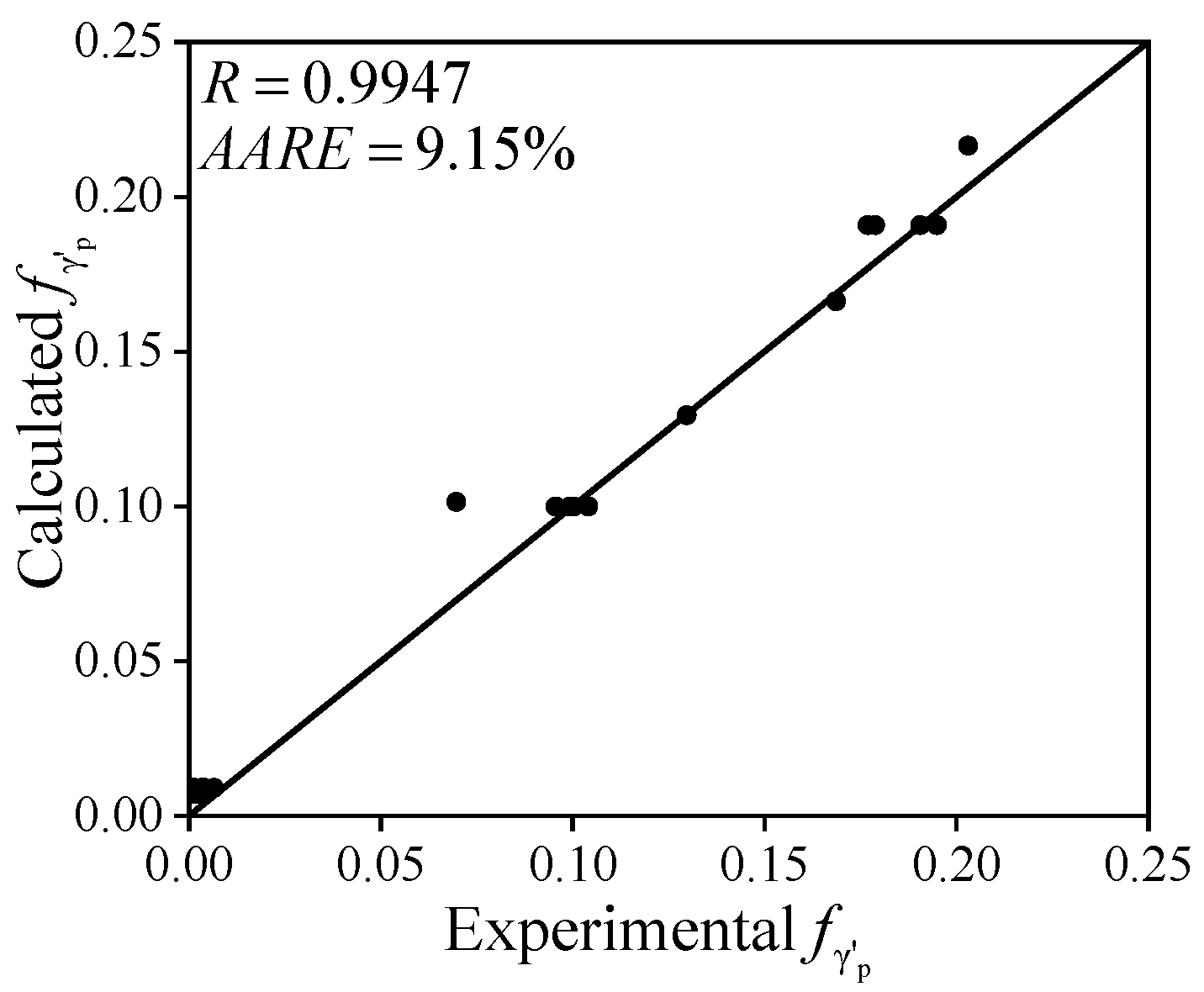
4.2. γ′p Phase Dissolution Kinetics Models
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Babu, K.A.; Mandal, S.; Kumar, A.; Athreya, C.N.; de Boer, B.; Sarma, V.S. Characterization of hot deformation behaviour of alloy 617 through kinetic analysis, dynamic material modeling and microstructural studies. Mater. Sci. Eng. A 2016, 664, 177–187. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, J.Y.; Wang, B.; Yao, C.G. Comparative study of IN600 superalloy produced by two powder metallurgy technologies: Argon atomizing and plasma rotating electrode process. Vacuum 2018, 156, 302–309. [Google Scholar] [CrossRef]
- Liu, F.F.; Chen, J.Y.; Dong, J.X.; Zhang, M.C.; Yao, Z.H. The hot deformation behaviors of coarse, fine and mixed grain for Udimet 720Li superalloy. Mater. Sci. Eng. A 2016, 651, 102–115. [Google Scholar] [CrossRef]
- Schulz, B.; Leitner, T.; Hafok, M.; Primig, S. Advancements in processing of Ni-based superalloys by microstructure engineering via discontinuous γ′ break-down. Materialia 2023, 31, 101873. [Google Scholar] [CrossRef]
- Kumar, S.S.S.; Raghu, T.; Bhattacharjee, P.P.; Rao, G.A.; Borah, U. Constitutive modeling for predicting peak stress characteristics during hot deformation of hot isostatically processed nickel-base superalloy. J. Mater. Sci. 2015, 50, 6444–6456. [Google Scholar] [CrossRef]
- Jia, J.; Zhang, Y.W.; Tao, Y.; Yan, T.; Ji, H.Y. Effects of solution temperature on tensile properties of a high γ′ volume fraction P/M superalloy. Materials 2022, 15, 5528. [Google Scholar] [CrossRef]
- Tan, G.; Li, H.Z.; Wang, Y.; Qiao, S.C.; Yang, L.; Huang, Z.Q.; Cheng, T.W.; Zhao, Z.X. Effect of Zener-Hollomon parameter on microstructure evolution of a HEXed PM nickel-based superalloy. J. Alloys Compd. 2021, 874, 159889. [Google Scholar] [CrossRef]
- Gardner, S.; Li, W.; Coleman, M.; Johnston, R. The effects of thermomechanical history on the microstructure of a nickel-base superalloy during forging. Mater. Sci. Eng. A 2016, 668, 263–270. [Google Scholar] [CrossRef]
- Wu, Y.T.; Li, C.; Xia, X.C.; Liang, H.Y.; Qi, Q.Q.; Liu, Y.C. Precipitate coarsening and its effects on the hot deformation behavior of the recently developed γ’-strengthened superalloys. J. Mater. Sci. Technol. 2021, 67, 95–104. [Google Scholar]
- Janeiro, I.S.; Franchet, J.M.; Cormier, J.; Bozzolo, N. Dynamic precipitation during forging of a γ-γ′ nickel-based superalloy. Metall. Mater. Trans. A 2023, 54, 2052–2070. [Google Scholar] [CrossRef]
- Eriksson, E.; Hanning, F.; Andersson, J.; Colliander, M.H. Meta-dynamic recrystallization in the Ni-based superalloy Haynes 282. Metals 2023, 13, 1335. [Google Scholar] [CrossRef]
- Sajjadi, S.A.; Nategh, S. A high temperature deformation mechanism map for the high performance Ni-base superalloy GTD-111. Mater. Sci. Eng. A 2001, 307, 158–164. [Google Scholar]
- Yang, Q.M.; Lin, Y.C.; Chen, M.S.; Chen, Z.J. Modeling dynamic recrystallization behavior in a novel HIPed P/M superalloy during high-temperature deformation. Materials 2022, 15, 4030. [Google Scholar] [CrossRef]
- Zhu, J.C.; Lin, Y.C.; Yang, Q.M.; Chen, Z.J.; Naseri, M. A novel dynamic recrystallization kinetics model based on particle-stimulated nucleation of the HEXed FGH4113A alloy during hot deformation. Mater. Charact. 2024, 217, 114459. [Google Scholar]
- Wen, H.N.; Jin, J.S.; Tang, X.F.; Wang, X.Y.; Yang, H.Y.; Zhang, Y.D.; Zhang, M.; Deng, L.; Wei, Q.S.; Chen, J.Z.; et al. Machine learning-assisted constitutive modeling of a novel powder metallurgy superalloy. Int. J. Mech. Sci. 2023, 260, 108654. [Google Scholar]
- Chen, Z.J.; Lin, Y.C.; He, D.G.; Lou, Y.M.; Chen, M.S. A unified dislocation density-based model for an aged polycrystalline Ni-based superalloy considering the coupled effects of complicate deformation mechanisms and initial δ phase. Mater. Sci. Eng. A 2021, 827, 142062. [Google Scholar]
- Li, J.; An, X.H.; Wang, T.; Zhu, E.; Li, F.G. Thermal deformation behavior and dynamic recrystallization mechanism of GH4065A alloy considering the effect of γ’ phase. Intermetallics 2024, 75, 108545. [Google Scholar]
- Thornton, E.L.; Zannoun, H.; Vomero, C.; Caudill, D.; Schoop, J. A review of constitutive models and thermal properties for nickel-based superalloys across machining-specific regimes. J. Manuf. Sci. E-T ASME 2023, 145, 080801. [Google Scholar]
- Eriksson, E.; Hanning, F.; Andersson, J.; Colliander, M.H. Dynamic recrystallization during hot compression of Ni-based superalloy Haynes 282. J. Alloys Compd. 2023, 960, 170837. [Google Scholar]
- Li, H.Z.; Yang, L.; Wang, Y.; Tan, G.; Qiao, S.C.; Huang, Z.Q.; Liu, M.X. Thermal deformation and dynamic recrystallization of a novel HEXed P/M nickel-based superalloy. Mater. Charact. 2020, 163, 110285. [Google Scholar]
- Wang, M.J.; Sun, C.Y.; Fu, M.W.; Liu, Z.L.; Wang, C.H. Experimental investigations and constitutive modeling of the dynamic recrystallization behavior of Inconel 740 superalloy. Mater. Sci. Eng. A 2020, 793, 139939. [Google Scholar]
- Charpagne, M.A.; Franchet, J.M.; Bozzolo, N. Overgrown grains appearing during sub-solvus heat treatment in a polycrystalline γ-γ’ Nickel-based superalloy. Mater. Des. 2018, 144, 353–360. [Google Scholar]
- Wang, J.; Huang, H.L.; Xin, D.; Hu, J.C.; Zhang, H.; Ruan, J.J.; Zhou, X.; Zhang, S.Z.; Jiang, L. Dissolution behavior and kinetics of the γ’ precipitates within a novel powder metallurgy Ni-based superalloy. J. Mater. Res. Technol. 2024, 30, 1950–1961. [Google Scholar]
- Zhang, W.; Li, J.N.; Dong, R.F.; Chen, Z.S.; Li, J.; Zhou, X.; Wang, Q.Z.; Qu, J.L. Effect of heat treatment process parameters on the microstructure and properties of GH4720Li superalloy. Modelling Simul. Mater. Res. Express 2023, 10, 016514. [Google Scholar]
- Li, P.K.; Chen, L.; Bu, H.Y.; Zeng, Y.; Li, S.H.; Wang, C. Effect of cooling rate on the Morphological changes in the secondary precipitation in FGH97 nickel-based PM superalloy. Intermetallics 2024, 171, 108344. [Google Scholar] [CrossRef]
- Liu, Z.S.; Li, C.W.; Dong, A.P.; Gu, J.F. Effect of initial microstructure on the properties of K439B nickel-based superalloy during prolonged aging at 800 °C. Intermetallics 2024, 172, 108364. [Google Scholar]
- Cai, Y.F.; Zhang, Y.Y.; Li, J.K.; Yang, M.S.; Liu, J.T.; Liu, T. Effects of pre-precipitation treatment temperature on the microstructure and mechanical properties of nickel-based powder superalloy. Intermetallics 2023, 163, 108059. [Google Scholar]
- Quan, G.Z.; Zhang, P.; Ma, Y.Y.; Zhang, Y.Q.; Lu, C.L.; Wang, W.Y. Characterization of grain growth behaviors by BP-ANN and Sellars models for nickle-base superalloy and their comparisons. Trans. Nonferrous Met. Soc. China 2020, 30, 2435–2448. [Google Scholar] [CrossRef]
- Huang, H.L.; Zhang, H.F.; Hu, B.F.; Wang, H.; Liu, G.Q. Study on γ′ precipitation behavior in a nickel-based PM superalloy during interrupted continuous cooling. Intermetallics 2020, 116, 106659. [Google Scholar]
- Ji, Z.Y.; Jia, C.L.; Pan, X.Y.; Qiu, C.L. Grain growth kinetics and γ’ dissolution behavior of a novel disc superalloy GH4065A. JOM 2023, 75, 5884–5892. [Google Scholar] [CrossRef]
- Yang, Y.H.; Zhang, B.Y.; Chen, X.Q.; Wang, X.X.; Sun, J.S. Modeling and simulation of grain growth for FGH96 superalloy using a developed cellular automaton model. Model. Simul. Mater. Sci. Eng. 2024, 32, 055011. [Google Scholar]
- Liu, D.H.; Chai, H.R.; Yang, L.; Qiu, W.Q.; Guo, Z.H.; Wang, Z.L. Study on the dynamic recrystallization mechanisms of GH5188 superalloy during hot compression deformation. J. Alloys Compd. 2022, 895, 162565. [Google Scholar] [CrossRef]
- Momeni, A.; Ebrahimi, G.; Ezatpour, H. Modelling the yield point phenomena during deformation at elevated temperatures: Case study on Inconel 600. Philos. Mag. 2018, 98, 2543–2561. [Google Scholar]
- Zhu, L.H.; Wei, B.; Pan, H.; Xiao, L.; Guo, J.Z.; Ji, H.J. Dynamic deformation mechanism for distinct flow behaviors in FGH4113A superalloy with high γ′ content during isothermal compression at sub-/near-/super-solvus temperatures. Mater. Des. 2024, 241, 112890. [Google Scholar] [CrossRef]
- Cao, B.X.; Kong, H.J.; Ding, Z.Y.; Wu, S.W.; Luan, J.H.; Jiao, Z.B.; Lu, J.; Liu, C.T.; Yang, T. A novel L12-strengthened multicomponent Co-rich high-entropy alloy with both high γ’-solvus temperature and superior high-temperature strength. Scr. Mater. 2021, 199, 113826. [Google Scholar]
- Wen, H.N.; Jin, J.S.; Tang, X.F.; Wang, X.Y.; Yang, H.Y.; Zhang, Y.D.; Deng, L.; Gong, P.; Wei, Q.S.; He, Y.J.; et al. Systematic analysis of distinct flow characteristics and underlying microstructural evolution mechanisms of a novel fine-grained P/M nickel-based superalloy during isothermal compression. J. Mater. Sci. Technol. 2023, 162, 57–73. [Google Scholar]
- Zhang, H.K.; Ma, H.C.; Chang, T.X.; Zhang, Y.; Logé, R.E.; Zhang, Q.; Fang, X.W.; Huang, K. Deformation mechanisms of primary γ’ precipitates in nickel-based superalloy. Scr. Mater. 2023, 224, 115109. [Google Scholar]
- Li, Z.Q.; Wang, D.C.; Zhai, Y.W.; Jiang, C.; Zhou, L.Y.; Zhou, Z.G.; Wang, H.Z.; Zhang, Z.B.; Yan, L.; Wang, L.P.; et al. Microstructure and microtexture evolution characteristics of a powder metallurgy Ni-based superalloy during static recrystallization. J. Iron Steel Res. Int. 2024, 31, 2308–2325. [Google Scholar]
- Zhang, J.K.; Cao, Y.; Gong, Y.H.; Xiao, J.; Zhu, Y.L.; Luo, R.; He, Q.B.; Liu, Q. Revealing hot deformation behavior of Inconel X-750 superalloy: A novel hot processing map coupled with grain boundary engineering. J. Mater. Res. Technol. 2023, 25, 1245–1262. [Google Scholar]
- Song, K.; Aindow, M. Grain growth and particle pinning in a model Ni-based superalloy. Mater. Sci. Eng. A 2008, 479, 365–372. [Google Scholar]
- Qiao, S.C.; Li, N.; Gao, X.Y.; Wen, X.; Wang, F.Z.; Xia, Z.K.; Wang, Y.Q.; Jiang, J.Z.; Liu, S.; Yuan, C. Low plastic deformation: Its impact on the microstructural evolution during heat treatment of nickel-based wrought superalloy GH4698. Intermetallics 2025, 179, 108678. [Google Scholar]


| Co | Cr | Mo | W | Ti | Al | Nb | Ta | Hf | Ni |
|---|---|---|---|---|---|---|---|---|---|
| 19.1 | 12.9 | 4.1 | 4.1 | 3.7 | 3.0 | 1.3 | 1.0 | 0.2 | Bal. |
| Ni | Co | Ti | Al | Cr | Mo | Nb | Ta | W | Hf |
|---|---|---|---|---|---|---|---|---|---|
| 62.82 | 12.68 | 8.81 | 7.76 | 3.76 | 1.22 | 1.05 | 0.99 | 0.79 | 0.11 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, J.-C.; Lin, Y.-C.; Ling, Y.-H.; Li, S.-X.; Chen, Z.-J.; Qiu, Y.-L. Influence of Preheating on the Microstructure of a Hot Extruded Nickel-Based Superalloy. Materials 2025, 18, 1478. https://doi.org/10.3390/ma18071478
Zhu J-C, Lin Y-C, Ling Y-H, Li S-X, Chen Z-J, Qiu Y-L. Influence of Preheating on the Microstructure of a Hot Extruded Nickel-Based Superalloy. Materials. 2025; 18(7):1478. https://doi.org/10.3390/ma18071478
Chicago/Turabian StyleZhu, Jun-Cheng, Yong-Cheng Lin, Yun-Han Ling, Shu-Xin Li, Zi-Jian Chen, and Yu-Liang Qiu. 2025. "Influence of Preheating on the Microstructure of a Hot Extruded Nickel-Based Superalloy" Materials 18, no. 7: 1478. https://doi.org/10.3390/ma18071478
APA StyleZhu, J.-C., Lin, Y.-C., Ling, Y.-H., Li, S.-X., Chen, Z.-J., & Qiu, Y.-L. (2025). Influence of Preheating on the Microstructure of a Hot Extruded Nickel-Based Superalloy. Materials, 18(7), 1478. https://doi.org/10.3390/ma18071478






