Divergent Hydraulic Strategies Explain the Interspecific Associations of Co-Occurring Trees in Forest–Steppe Ecotone
Abstract
:1. Introduction
2. Materials and Methods
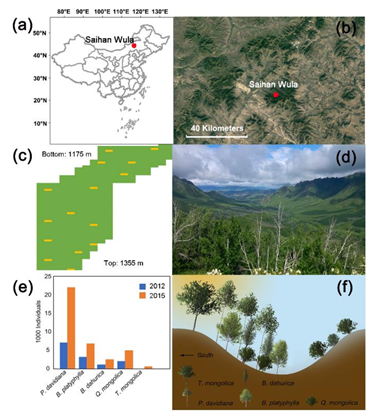
2.1. Site Description and Sampling Design
2.2. Interspecific Association Quantification
2.3. Hydraulic Traits Measurement
2.4. Statistical Analysis
3. Results
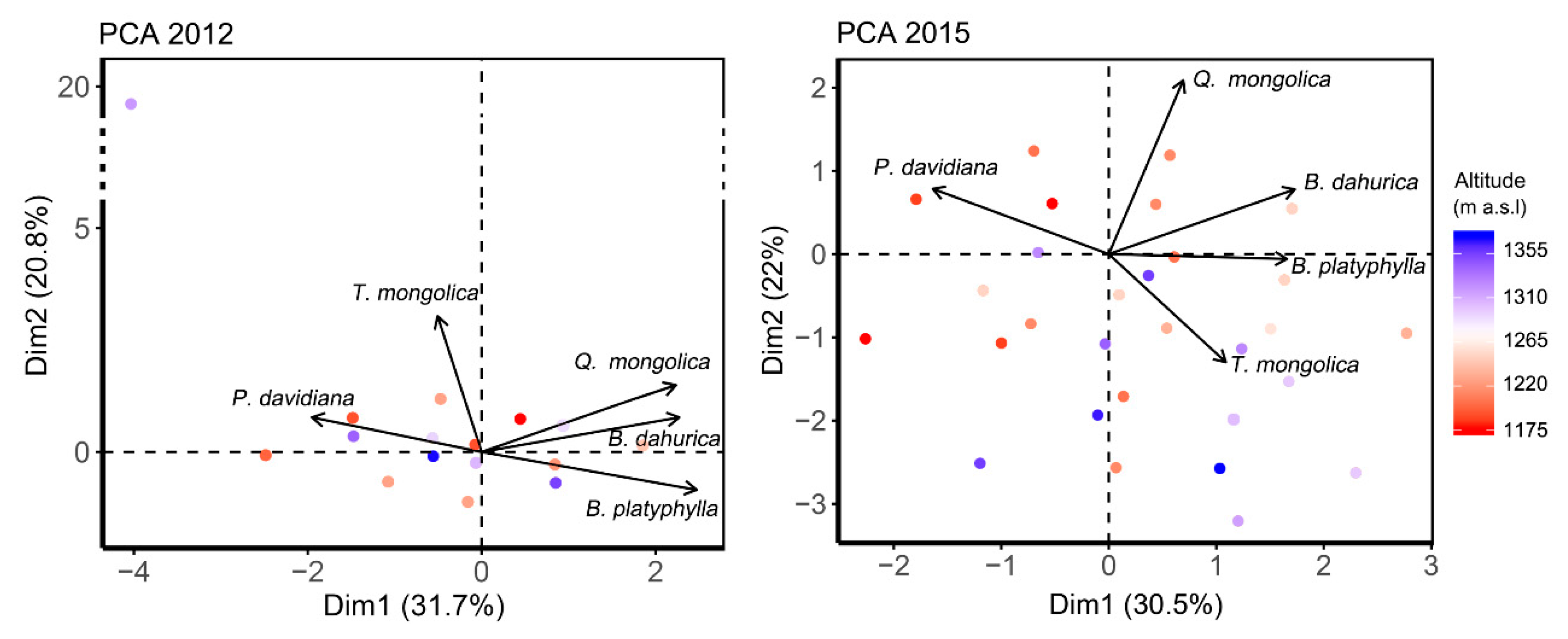
3.1. Interspecific Association among Species
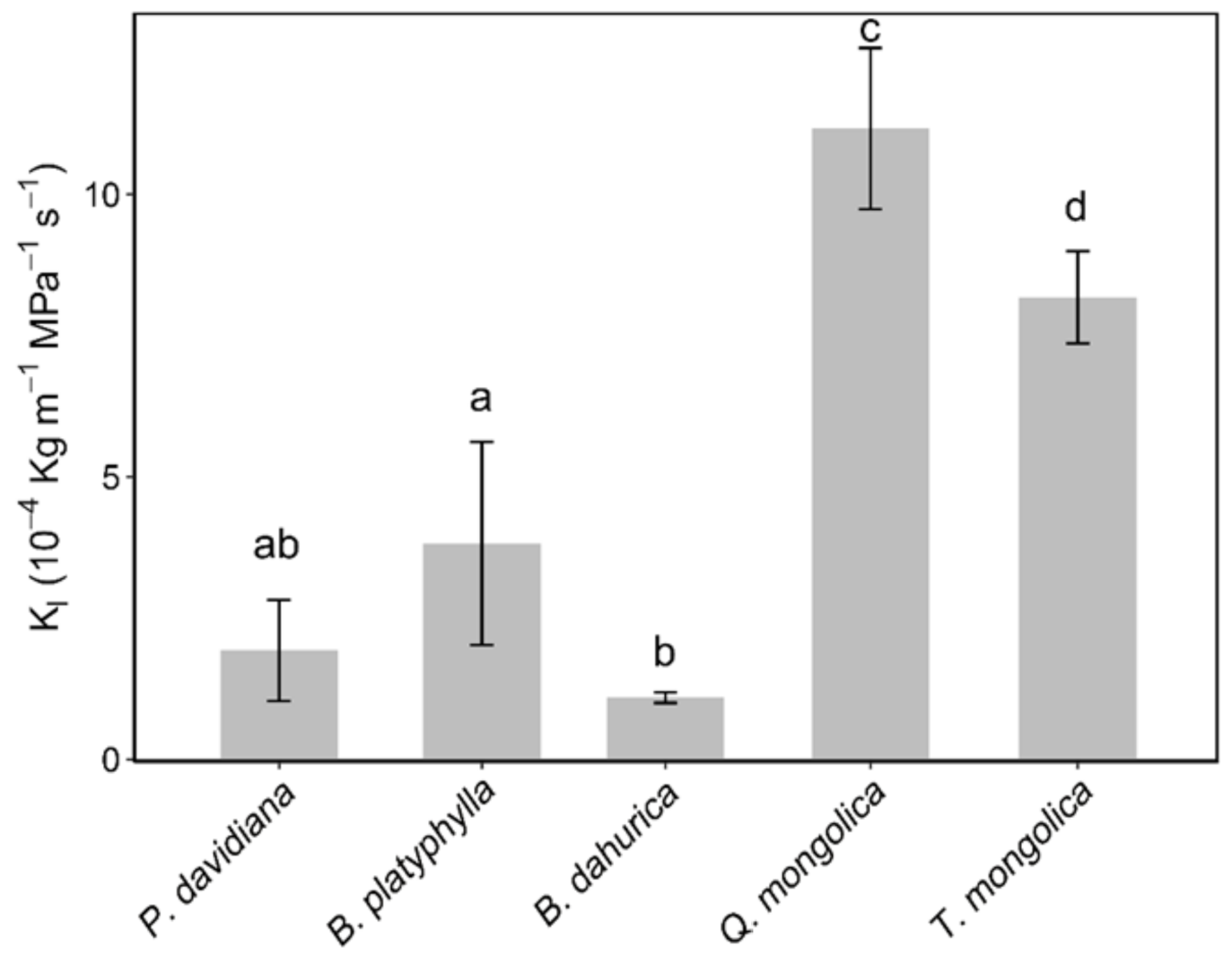
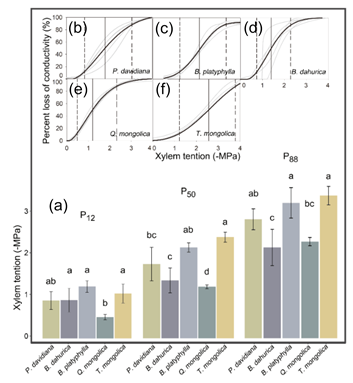
3.2. Hydraulic Safety and Efficiency Differences among Species
3.3. The Relationship between Interspecific Association and Species Hydraulic Strategy
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Rotenberg, E.; Yakir, D. Contribution of semi-arid forests to the climate system. Science 2010, 327, 451–454. [Google Scholar] [CrossRef]
- Xu, C.; Liu, H.; Anenkhonov, O.A.; Korolyuk, A.Y.; Sandanov, D.V.; Balsanova, L.D.; Naidanov, B.B.; Wu, X. Long-term forest resilience to climate change indicated by mortality, regeneration, and growth in semiarid southern Siberia. Glob. Chang. Biol. 2017, 23, 2370–2382. [Google Scholar] [CrossRef] [PubMed]
- Allen, C.D.; Macalady, A.K.; Chenchouni, H.; Bachelet, D.; McDowell, N.; Vennetier, M.; Kitzberger, T.; Rigling, A.; Breshears, D.D.; Hogg, E.H.; et al. A global overview of drought and heat-induced tree mortality reveals emerging climate change risks for forests. For. Ecol. Manag. 2010, 259, 660–684. [Google Scholar] [CrossRef] [Green Version]
- Erdos, L. The edge of two worlds: A new review and synthesis on Eurasian forest-steppes. Appl. Veg. Sci. 2018, 21, 345–362. [Google Scholar] [CrossRef]
- Chun-yu, Y.; Shao-fei, L.I.U.; Li-fei, Y.U. Interspecific associations of dominant tree species with restoration of a karst forest. J. Zhejiang AF Univ. 2010, 27, 44–50. [Google Scholar]
- Liu, Z.; Zhu, Y.; Wang, J.; Ma, W.; Meng, J. Species association of the dominant tree species in an old-growth forest and implications for enrichment planting for the restoration of natural degraded forest in subtropical China. Forests 2019, 10, 957. [Google Scholar] [CrossRef] [Green Version]
- Xie, T.; Tian-Zhen, J.U.; Shi, H.X.; Fan, Z.H.; Yang, G.K.; Zhang, S.Z. Interspecific association of rare and endangered Pinus bungeana community in Xiaolongshan of Gansu. Chin. J. Ecol. 2010, 29, 448–453. [Google Scholar]
- Liu, H.; Park Williams, A.; Allen, C.D.; Guo, D.; Wu, X.; Anenkhonov, O.A.; Liang, E.; Sandanov, D.V.; Yin, Y.; Qi, Z.; et al. Rapid warming accelerates tree growth decline in semi-arid forests of Inner Asia. Glob. Chang. Biol. 2013, 19, 2500–2510. [Google Scholar] [CrossRef]
- Nardini, A.; Pitt, F. Drought resistance of Quercus pubescens as a function of root hydraulic conductance, xylem embolism and hydraulic architecture. New Phytol. 1999, 143, 485–493. [Google Scholar] [CrossRef]
- Zimmermann, M.H. Hydraulic architecture of some diffuse-porous trees. Can. J. Bot. 1978, 56, 2286–2295. [Google Scholar] [CrossRef] [Green Version]
- Carvalho, E.C.D.; Martins, F.R.; Soares, A.A.; Oliveira, R.S.; Muniz, C.R.; Araujo, F.S. Hydraulic architecture of lianas in a semiarid climate: Efficiency or safety? Acta Bot. Bras. 2015, 29, 198–206. [Google Scholar] [CrossRef]
- Sperry, J.S.; Meinzer, F.C.; Mcculloh, K.A. Safety and efficiency conflicts in hydraulic architecture: Scaling from tissues to trees. Plant Cell Environ. 2008, 31, 632–645. [Google Scholar] [CrossRef] [PubMed]
- Kondoh, S.; Yahata, H.; Nakashizuka, T.; Kondoh, M. Interspecific variation in vessel size, growth and drought tolerance of broad-leaved trees in semi-arid regions of Kenya. Tree Physiol. 2006, 26, 899–904. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cruiziat, P.; Cochard, H.; Ameglio, T. Hydraulic architecture of trees: Main concepts and results. Ann. For. Sci. 2002, 59, 723–752. [Google Scholar] [CrossRef] [Green Version]
- Hao, G.Y.; Lucero, M.E.; Sanderson, S.C.; Zacharias, E.H.; Holbrook, N.M. Polyploidy enhances the occupation of heterogeneous environments through hydraulic related trade-offs in Atriplex canescens (Chenopodiaceae). New Phytol. 2013, 197, 970–978. [Google Scholar] [CrossRef]
- Choat, B.; Brodribb, T.J.; Brodersen, C.R.; Duursma, R.A.; Lopez, R.; Medlyn, B.E. Triggers of tree mortality under drought. Nature 2018, 558, 531–539. [Google Scholar] [CrossRef]
- Sperry, J.S.; Love, D.M. What plant hydraulics can tell us about responses to climate-change droughts. New Phytol. 2015, 207, 14–27. [Google Scholar] [CrossRef]
- Gebauer, R.L.E.; Schwinning, S.; Ehleringer, J.R. Interspecific competition and resource pulse utilization in a cold desert community. Ecology 2002, 83, 2602–2616. [Google Scholar] [CrossRef]
- Jiang, Z.H.; Ma, K.M.; Anand, M.; Zhang, Y.X. Interplay of temperature and woody cover shapes herb communities along an elevational gradient in a temperate forest in Beijing, China. Community Ecol. 2015, 16, 215–222. [Google Scholar] [CrossRef] [Green Version]
- Zeng, N.; Yao, H.X.; Zhou, M.; Zhao, P.W.; Dech, J.P.; Zhang, B.; Lu, X. Species-specific determinants of mortality and recruitment in the forest-steppe ecotone of northeast China. For. Chron. 2016, 92, 336–344. [Google Scholar] [CrossRef]
- Zhao, P.W.; Xu, C.Y.; Zhoul, M.; Zhang, B.; Ge, P.; Zeng, N.; Liu, H.Y. Rapid regeneration offsets losses from warming-induced tree mortality in an aspen dominated broad-leaved forest in northern China. PLoS ONE 2018, 13, e0195630. [Google Scholar] [CrossRef] [PubMed]
- Wheeler, J.K.; Huggett, B.A.; Tofte, A.N.; Rockwell, F.E.; Holbrook, N.M. Cutting xylem under tension or supersaturated with gas can generate PLC and the appearance of rapid recovery from embolism. Plant Cell Environ. 2013, 36, 1938–1949. [Google Scholar] [CrossRef] [PubMed]
- Venturas, M.D.; MacKinnon, E.D.; Jacobsen, A.L.; Pratt, R.B. Excising stem samples underwater at native tension does not induce xylem cavitation. Plant Cell Environ. 38, 1060–1068. [CrossRef] [PubMed]
- Zwieniecki, M.A.; Holbrook, N.M. Diurnal variation in xylem hydraulic conductivity in white ash (Fraxinus americana L.), red maple (Acer rubrum L,) and red spruce (Picea rubens Sarg,). Plant Cell Environ. 1998, 21, 1173–1180. [Google Scholar] [CrossRef] [Green Version]
- Alder, N.N.; Pockman, W.T.; Sperry, J.S.; Nuismer, S. Use of centrifugal force in the study of xylem cavitation. J. Exp. Bot. 1997, 48, 665–674. [Google Scholar] [CrossRef] [Green Version]
- Bartlett, M.K.; Klein, T.; Jansen, S.; Choat, B.; Sack, L. The correlations and sequence of plant stomatal, hydraulic, and wilting responses to drought. Proc. Natl. Acad. Sci. USA 2016, 113, 13098–13103. [Google Scholar] [CrossRef] [Green Version]
- Dulamsuren, C.; Hauck, M.; Leuschner, C. Recent drought stress leads to growth reductions in Larix sibirica in the western Khentey, Mongolia. Glob. Chang. Biol. 2010, 16, 3024–3035. [Google Scholar] [CrossRef]
- Seager, R.; Vecchi, G.A. Greenhouse warming and the 21st century hydroclimate of southwestern North America. Proc. Natl. Acad. Sci. USA 2010, 107, 21277–21282. [Google Scholar] [CrossRef] [Green Version]
- Piao, S.L.; Ciais, P.; Huang, Y.; Shen, Z.H.; Peng, S.S.; Li, J.S.; Zhou, L.P.; Liu, H.Y.; Ma, Y.C.; Ding, Y.H.; et al. The impacts of climate change on water resources and agriculture in China. Nature 2010, 467, 43–51. [Google Scholar] [CrossRef]
- Chesson, P.; Gebauer, R.L.E.; Schwinning, S.; Huntly, N.; Wiegand, K.; Ernest, M.S.K.; Sher, A.; Novoplansky, A.; Weltzin, J.F. Resource pulses, species interactions, and diversity maintenance in arid and semi-arid environments. Oecologia 2004, 141, 236–253. [Google Scholar] [CrossRef]
- Choat, B.; Jansen, S.; Brodribb, T.J.; Cochard, H.; Delzon, S.; Bhaskar, R.; Bucci, S.J.; Feild, T.S.; Gleason, S.M.; Hacke, U.G.; et al. Global convergence in the vulnerability of forests to drought. Nature 2012, 491, 752–755. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Landhausser, S.M.; Wachowski, J.; Lieffers, V.J. Transfer of live aspen root fragments, an effective tool for large-scale boreal forest reclamation. Can. J For. Res 2015, 45, 1056–1064. [Google Scholar] [CrossRef]
- Mitton, J.B.; Grant, M.C. Genetic variation and the natural history of quaking aspen. Bioscience 1996, 46, 25–31. [Google Scholar] [CrossRef] [Green Version]


| Species | Yates Correlation | Ochiai Index | |||||
|---|---|---|---|---|---|---|---|
| V2012 | χ22012 | V2015 | χ22015 | OI2012 | OI2015 | ||
| P. davidiana | B. platyphylla | −12,384 | 31.80 | −15,998 | 34.44 | 0.39 | 0.38 |
| P. davidiana | B. dahurica | −4816 | 2.74 | −11,818 | 12.74 | 0.52 | 0.50 |
| P. davidiana | Q. mongolica | −8944 | 10.07 | −1083 | 0.07 | 0.56 | 0.70 |
| P. davidiana | T. mongolica | 172 | 0.13 | −8227 | 14.45 | 0.05 | 0.21 |
| B. platyphylla | B. dahurica | 11,592 | 24.83 | 15,661 | 27.45 | 0.68 | 0.67 |
| B. platyphylla | Q. mongolica | 9036 | 15.09 | 2620 | 0.73 | 0.73 | 0.75 |
| B. platyphylla | T. mongolica | −420 | 0.65 | 2353 | 1.18 | 0.00 | 0.33 |
| B. dahurica | Q. mongolica | 17,693 | 36.26 | 15,246 | 20.70 | 0.64 | 0.64 |
| B. dahurica | T. mongolica | −239 | 0.01 | 8220 | 12.01 | 0.00 | 0.33 |
| Q. mongolica | T. mongolica | 223 | 0.02 | −2756 | 1.34 | 0.06 | 0.26 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, J.; Liu, H.; Xu, C.; Qi, Y.; Zhu, X.; Zhou, M.; Liu, B.; Wu, Y. Divergent Hydraulic Strategies Explain the Interspecific Associations of Co-Occurring Trees in Forest–Steppe Ecotone. Forests 2020, 11, 942. https://doi.org/10.3390/f11090942
Dai J, Liu H, Xu C, Qi Y, Zhu X, Zhou M, Liu B, Wu Y. Divergent Hydraulic Strategies Explain the Interspecific Associations of Co-Occurring Trees in Forest–Steppe Ecotone. Forests. 2020; 11(9):942. https://doi.org/10.3390/f11090942
Chicago/Turabian StyleDai, Jingyu, Hongyan Liu, Chongyang Xu, Yang Qi, Xinrong Zhu, Mei Zhou, Bingbing Liu, and Yiheng Wu. 2020. "Divergent Hydraulic Strategies Explain the Interspecific Associations of Co-Occurring Trees in Forest–Steppe Ecotone" Forests 11, no. 9: 942. https://doi.org/10.3390/f11090942
APA StyleDai, J., Liu, H., Xu, C., Qi, Y., Zhu, X., Zhou, M., Liu, B., & Wu, Y. (2020). Divergent Hydraulic Strategies Explain the Interspecific Associations of Co-Occurring Trees in Forest–Steppe Ecotone. Forests, 11(9), 942. https://doi.org/10.3390/f11090942







