Analysis of the Operating Parameters of Wood Transport Vehicles from the Point of View of Operational Reliability

Abstract

The aim of the research was to create a universal system for monitoring and evaluating the operating parameters of the haulage vehicles used for the haulage of wood with self-maintenance. The article presents partial results from the entire research. Data for research into the operational reliability of IVECO, SCANIA, and TATRA vehicles were obtained from the real-world operating conditions of two companies dealing with the mining/transportation process. Information from the operating conditions was obtained according to the test plan [n, R, t], according to which n objects were simultaneously tested, and the objects that were damaged during the tests were replaced with new ones; the tests ended after the test time t for each of the n positions. Based on the results and statistical analyses, it can be said that the best operational reliability is achieved by IVECO, followed by SCANIA, and only then by TATRA. The resolution of the above conclusions in operating conditions will contribute to the efficiency of the operation of the investigated facilities and the extension of the technical life of the means of transportation.

1. Introduction

Proper care for forests and forest ecosystems is important to ensure their functioning. It is important to take care of forest renewal as well as the extraction and processing of wood and wood material through modern technologies and the latest knowledge in the field of forest science and research [1,2]. The technologies used for the removal of wood from the removal point along public roads are very diverse. Road vehicles for the removal of wood for motor transport are divided according to the drive method into motor vehicles (driven by their own engine) and trailers (they do not have their own engine and are connected to motor vehicles). They depend on the form of transported wood (whole trees or their sections, shortened trunks, cuts of medium lengths, short cuts, etc.), the design of vehicles, and loading equipment [3]. Recently, forestry in Slovakia has had a very rapid tendency toward research at all levels. Increasing demands on the qualities of crop treatment in forestry increase the requirement for highly efficient and reliable forest equipment and technological devices to fulfill the requirements. Nowadays, the forest economy is based on the wide usage of forest machines and devices [4,5]. The transportation of wood and wood material also includes the removal of wood. The removal of wood follows on from the concentration of wood (export of wood and wood material). It is the transport of wood from the forest warehouse or from the removal point in the forest to the main handling or dispatch warehouse, or directly to the customer. Motor vehicles and trailers are used here. When hauling wood, hauling sets with the transport of wood in lengths over 6 m prevail [6]. The technologies used for the removal of wood from the removal point along public roads are very diverse. Road vehicles for the removal of wood for motor transport are divided according to the method of propulsion into motor vehicles (driven by their own engine) and trailers (they do not have their own engine and are connected to motor vehicles). They depend on the form of transported wood (whole trees or their sections, shortened trunks, cuts of medium lengths, short cuts, etc.), the design of vehicles, and loading equipment [7].

The aim of the paper is to evaluate a model of operational reliability for forest transportation vehicles. These machines are used for the transportation of wood, mainly on the read system. Testing is important for many reasons. The results of tests are often necessary for making decisions (from a quality point of view), evaluating reliability (e.g., maintenance), planning, and making choices. Adequate testing leads to high reliability and very good quality [5,8]. The main reason for paying attention to this area of reliability and maintenance is that, for every company, creating the most reliable system possible is a challenge and nowadays a common need. It is therefore necessary to be able to assess the reliability of all machinery and equipment and, in the event of deterioration in the characteristics of the means of transport monitored or stagnation, to be able to take appropriate steps to remedy this situation over time. The dependence of companies and people on technology is growing, and it is therefore necessary to ensure that the failure rate of used machinery and equipment is kept to a minimum or that machinery and equipment have maximum controlled maintenance based on real operating conditions [1,3].

The literature often equates reliability, in terms of meaning (and as a measure), with operational readiness. If a given object is composed of multiple subassemblies, then it is important to determine not only its reliability characteristics but also the impact of the reliability of individual subassemblies on the reliability of this specific object. The theory of reliability defines a system as an organized set of objects intended for the execution of specific tasks. The method of system element interconnections, which determines the impact of system failure depending on the failure of individual elements, is called the reliability structure of a system [9,10,11].

The purposeful processing of long-term documented maintenance data can provide plenty of information not only about a machine’s history but also about its maintenance system. The main objective of data analysis is to continually improve maintenance efficiency, which is closely related to improvements in dependability and overall productivity of the production equipment. Further examples of the evaluation of maintenance management data can be found in [12,13]. The best maintenance policy from the point of view of unit costs for this example is predictive maintenance. The benefit of the proposed mathematical models is not only the ability to compute the optimal interval of predetermined maintenance and the optimal diagnostic signal for predictive maintenance, but also the ability to provide quantitative proof that preventive predetermined the maintenance increases the operational reliability of machine objects. The decision lies with maintenance specialists as to whether they adopt and apply these models and methods for improving the maintenance effectiveness of industry production equipment [9,13,14,15]. Operational reliability prediction grows with the introduction of software and hardware innovations. For this reason, it is clearly necessary to say that Industry 4.0 has also affected this area of industry, which greatly affects the use of these machines and equipment in real operating conditions [4].

2. Materials and Methods

When evaluating operational reliability, the normal distribution (Gaussian bell) and Weibull distribution [16] are used based on the results achieved. One of the advantages of using the Weibull distribution is that the failure rate can have a rising, falling, or constant trend. Weibull distribution is flexible and adaptable for data over a wide range [2].

ANOVA is most often used in the evaluation of operational reliability. Analysis of variance examines the relationship between the dependent variable, “Y” and one or more factors (variables). Either a one-factor ANOVA or a multi-factor ANOVA [17] is used to evaluate the results. With multifactorial ANOVA, there is a greater possibility of testing dependencies (interactions) between factors and the complexity of hypotheses [18]. In the case of investigating the operational reliability of transport vehicles, a one-factor ANOVA is sufficient, since only one variable, Y, is always assessed against another variable parameter. The following studies were also devoted to similar research. [18,19,20].

The defined methodology for researching the operational reliability of transport vehicles is intended for monitoring the real operating conditions and selected indicators of operational reliability in accordance with the established test plan.

The following methodology was chosen for the collection, evaluation, and analysis of the operational reliability of forest means of transport for a simple evaluation of empirical information:

• Device passport processing (for each examined vehicle, a device passport with operating data for the monitored period was created)
• Using mathematical statistics for the definition of mathematical characteristics
• Creation of a histogram and a curve of cumulative relative frequency of time between failures or fault-free indicator
• Defining the disturbance intensity λ (t)
• Defining the mean time to failure T_S (h)
• Defining other indicators of operation depending on monitored kilometers, numbers of replaced parts, and economic indicators (costs)

The data for research into the operational reliability of IVECO, SCANIA, and TATRA vehicles were obtained from the real operating conditions of two companies dealing with the felling and transport processes in the monitored period (years: 2019–2022). Operational repairs and maintenance of vehicles for both companies were carried out in authorized service centers (

Table 1. List of investigated transport means.

Operator	Production	Model	Evidenc Number of Cars	Monitored Period	The Number of km at the Beginning of the Monitored Period	Number of km at the End of the Monitored Period	Number of Hours of Operation during Observation (h)
ŠLP TU vo Zvolene	IVECO TRAKKER	380T	ZV104BI	1 July 2017–14 September 2020	78,352	259,876	3784
		380T	ZV364BG	1 July 2017–14 September 2020	69,741	271,262	4232
		380T	ZV261AX	1 July 2017–14 September 2020	74,953	269,322	4025
	SCANIA	G490	ZV481DF	1 July 2017–14 September 2020	72,478	274,635	4309
		G490	ZV259DG	1 July 2017–14 September 2020	69,846	298,563	4852
		G490	ZV631CH	1 July 2017–14 September 2020	73,258	271,875	4136
Lesy SR, š.p.	TATRA PHOENIX	T 158 EKO	BB686GA	1 July 2017–14 September 2020	45,819	178,516	2564
		T158 EKO	BB68	1 July 2017–14 September 2020	48,741	169,852	2332
		T158 EKO	BB157HT	1 July 2017–14 September 2020	43,358	154,658	2175

).

Information from the operating conditions was obtained according to the test plan [n, R, t], according to which n objects were simultaneously tested, and the objects that were damaged during the tests were replaced with new ones; the tests ended after the test time t for each of the n positions.

Another, no less important, characteristic of reliability is the failure rate. Which represents the probability that a mechanical object that has not broken down at operational time “t” will break down immediately after operational time t [13]. This leads to an increase in machinery operating costs—too short a maintenance period results in an increase in maintenance costs; too long maintenance intervals lead to an increase in costs due to the poor technical condition of the production equipment.

A mathematical model of a non-renewable technical object that describes its reliability, understood as the ability to execute tasks under specific conditions and within a known time interval, is a non-negative and constant random variable T [21,22,23,24,25,26,27]. The basic measure of the reliability of an object R(t) within a time interval [0, t] is the object probability described by the following formula in Equation (1):

$$R\left(t\right)=P\left(T\ge t\right) for t\ge 0\left(1\right)$$

(1)

The reliability function of an object R(t) for each t ≥ 0 has a value equal to the probability of an event involving the failure-free operation of the object at least until t, which is the probability of an object being in a state of fitness until t. The function, which for each established t ≥ 0 adopts the value of the probability of an event that the object at moment t is damaged, is referred to as an unreliability function, described by the following formula in Equation (2):

$$F\left(t\right)=P\left(T<t\right)=1-R\left(t\right)$$

(2)

By evaluating the results from the operation of the equipment, the operator of the equipment receives an overview of the deployment of the forest means of transport in real operating conditions, such as the number of kilometers traveled during the monitored period as well as the hours of deployment of the equipment in operating conditions, the funds spent (costs) for repairs and maintenance, the number of replaced spare parts, as well as structural groups of the machine in a faulty condition. These data are presented in

Table 2. Basic information measured during the monitored period.

Producer	Number Plate of the Vehicle	The Number of km Driven during the Monitored Period	Total Time of Operation during the Monitored Period	The Number of Replaced Parts during the Monitored Period	Total Costs of Operating the Equipment during the Monitored Period	Number of Repairs
		[km]	[h]	[pcs]	[EUR]
TATRA	BB686GA	132,697	2564	232	55,013.10	44
	BB684GA	121,111	2332	191	44,099.90	41
	BB157HT	111,300	2175	167	35,020.50	33
SCANIA	ZV481DF	202,157	4309	161	42,249.59	34
	ZV259DG	228,717	4852	194	46,402.60	43
	ZV631CH	198,617	4136	231	54,692.91	44
IVECO	ZV104BI	181,524	3784	161	33,846.61	29
	ZV364BG	201,521	4232	190	38,596.93	36
	ZV261AX	194,369	4025	213	50,281.93	44

,

Table 3. Average values of monitored values converted to operational parameters at operators according to individual producers of transport vehicles.

Producer	The Average Number of Kilometers Driven during the Monitored Period	Average Time of Operation during the Monitored Period	Average Number of Replaced Parts during the Monitored Period	Average Costs of Operating the Equipment during the Monitored Period	Average Number of Repairs
	[km]	[h]	[pcs]	[EUR]
TATRA	121,702.67	2357.00	196.67	44,711.20	39.33
SCANIA	209,830.33	4432.11	195.33	47,781.70	40.33
IVECO	192,471.33	4013.67	188.00	40,908.50	36.33

,

Table 4. Basic information converted to economic parameters necessary for operators of monitored means of transportation according to individual produces.

Producer	Number Plate of the Vehicle	The Number of Kilometers Traveled per 1 h of Operation	Maintenance Costs for 1 h of Operation	Number of Kilometers Driven for 1 Repair	Number of Driving Hours per Repair	Maintenance Costs per 1 km of Travel
		[km]	[EUR]	[km]	[h]	[EUR]
TATRA	BB686GA	51.75	21.46	3015.84	58.27	0.41
	BB684GA	51.93	18.91	2953.93	56.88	0.36
	BB157HT	51.17	16.10	3372.73	65.91	0.31
SCANIA	ZV481DF	46.92	9.80	5945.79	126.74	0.21
	ZV259DG	47.14	9.56	5319.00	112.84	0.20
	ZV631CH	48.02	13.22	4514.02	94.00	0.28
IVECO	ZV104BI	47.97	8.94	6259.45	130.48	0.19
	ZV364BG	47.62	9.12	5597.81	117.56	0.19
	ZV261AX	48.29	12.49	4417.48	91.48	0.26

and

Table 5. Average values of the basic information of the monitored values converted to operational parameters for operators according to individual producers of transport vehicles.

Producer	Average Number of Kilometers Driven per 1 h of Operation	Average Repair and Maintenance Costs for 1 h of Operation	Average Number of Kilometers Driven for 1 Repair and Maintenance	Average Number of Driving Hours per Repair and Maintenance	Average Cost of Repairs and Maintenance per 1 km of Driving
	[km]	[EUR]	[km]	[h]	[EUR]
TATRA	51.63	18.97	1031.38	19.97	0.37
SCANIA	47.34	10.78	1778.22	36.63	0.23
IVECO	47.95	10.19	1631.11	36.82	0.21

.

3. Results and Discussion

In statistics, the mutual comparison of individual sample sets is important for the recognition of sample sets. This comparison enables the analysis of their variation (structure). This also defines the structure of the basic file in relation to the selected files of the researched means of transport, i.e., the reasons for obtaining measured data.

3.1. Failure Instensity λ(t)

The two-parameter Weibull distribution was used to determine the intensity of disturbances λ(t). The size parameter and the shape parameter b of the Weibull distribution were determined. The position parameter c of the Weibull distribution is equal to zero. The time interval is always t ≥ 0.

The relation of the Weibull distribution used to calculate the intensity of disturbances λ(t) is:

$$\lambda \left(t\right)=\frac{b}{a}·{\left(\frac{t}{a}\right)}^{b-1}$$

(3)

Based on empirical information (data collected in operating conditions) and using STATISTICA 12, the parameters of the Weibull distribution were calculated for the manufacturers of vehicles TATRA, SCANIA, and IVECO (

Table 6. Parameters of the Weibull distribution for the time interval between failures.

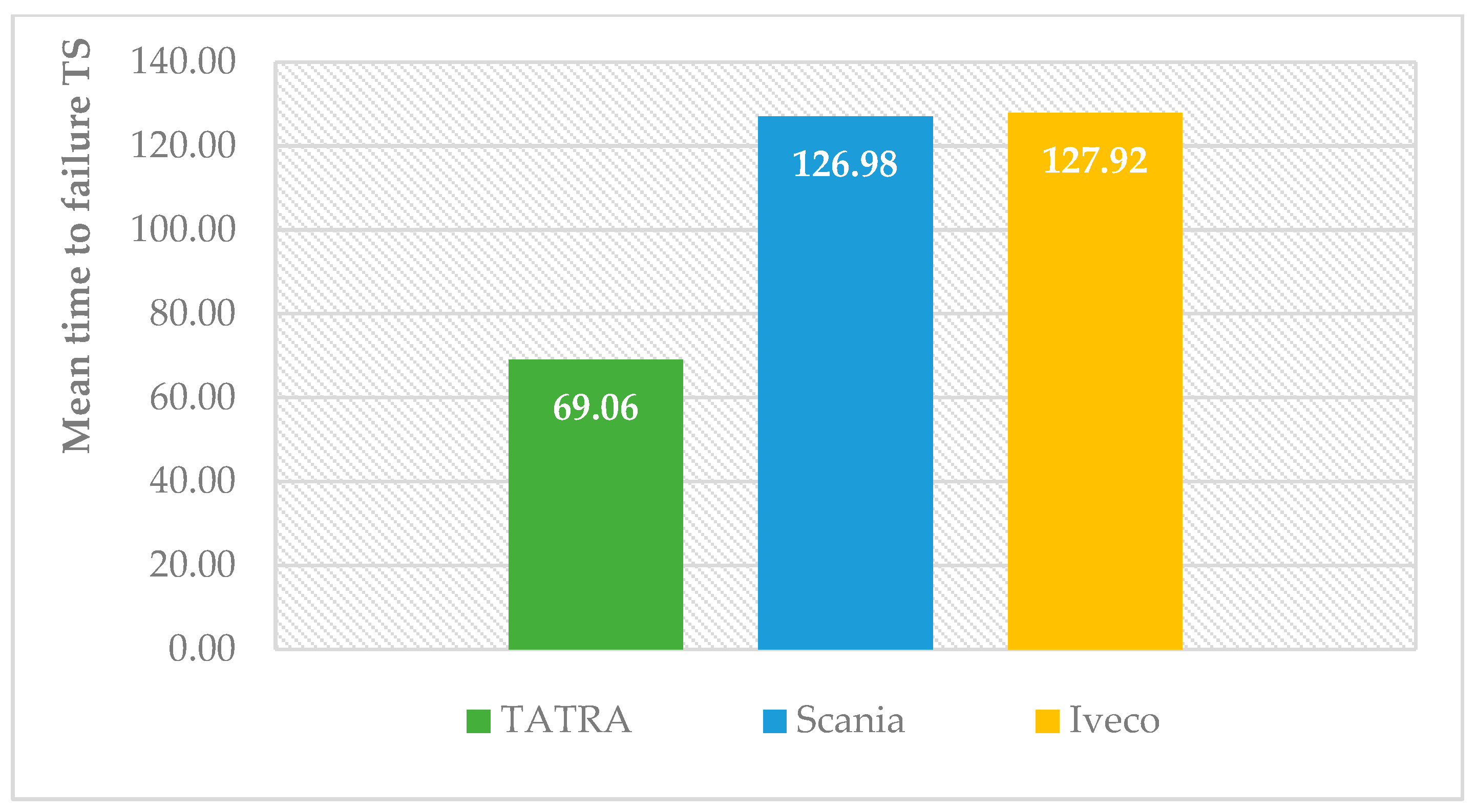
Producer	a (Parameter of Size)	b (Parameter Shape)	c (Parameter Position)	n (Number of Measurements)
TATRA	69.0616	2.3614	0	115
SCANIA	126.975	2.3908	0	118
IVECO	127.9213	2.2061	0	106

).

The intensity of faults λ(t) for the producers of the monitored vehicles is shown in Figure 1.

3.2. Mean Time to Failure T_S (h)

The mean time to T_S failure is one of the indicators of operational reliability. For all producers of the monitored means of transportation, the parameters of the Weibull distribution and the assumption given by the relationship are based on:

$$T_S=\overline{t}=a.\varsigma \left(1+\frac{1}{b}\right)\cong a$$

(4)

where:

• a is the size parameter of the Weibull distribution,
• b is the shape parameter of the Weibull distribution,
• $\varsigma$ is the gamma function (value determined in a table).

It is clear from the values for the average time to failure T_S that the best reliability (the parameter is the average time to failure) was achieved by vehicles manufactured by IVECO, followed by SCANIA, and then TATRA (Figure 2).

The results of the measurements were classified according to frequency into construction groups depending on the producer). The values are narrow as a basis for the use of a simple linear correlation of independence.

Subsequently, the expected values in cases of independence for the measured data were expressed (

Table 7. Expected values for measured data in case of independence.

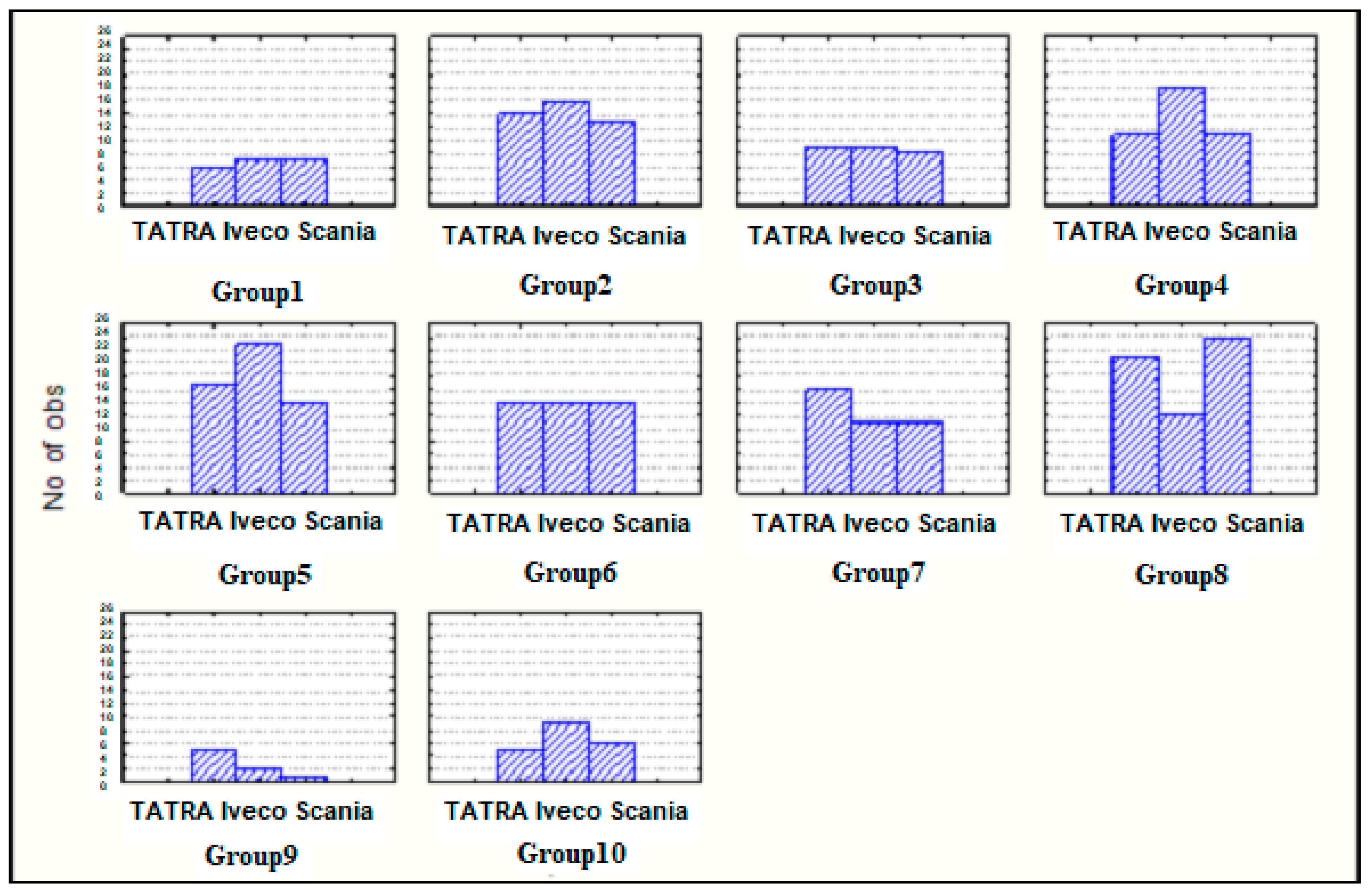
Construction Group	Producer			Total
	TATRA	SCANIA	IVECO
Cabin and sensors	6.78	6.95	6.26	20
Sensors	14.58	14.95	13.47	43
Engine	8.82	9.04	8.14	26
Engine and its cooling and lubrication	13.56	13.91	12.53	40
Gearboxes and gear mechanisms	18.31	18.78	16.91	54
Chassis	14.24	14.60	13.16	42
Hydraulic system of the machine	12.89	13.21	11.90	38
Hydraulic crane with a log grab	19.33	19.82	17.85	57
Trailer/semi-trailer	2.71	2.78	2.51	8
Crossbars	6.78	6.95	6.26	20
All construction groups altogether (10)	118	121	109	348

).

The measured values were compared against the expected values in independence for the measured data (

Table 8. Residuals of measured data against expected values for individual producers of transportation vehicles depending on the construction group of the monitored machines.

Construction Group	Producer			Total
	TATRA	SCANIA	IVECO
Cabin and sensors	−0.78	0.05	0.74	0
Sensors	−0.58	1.05	−0.47	0
Engine	0.18	−0.04	−0.14	0
Engine and its cooling and lubrication	−2.56	4.09	−1.53	0
Gearboxes and gear mechanisms	−1.31	4.22	−2.91	0
Chassis	−0.24	−0.60	0.84	0
Hydraulic system of the machine	3.11	−2.21	−0.90	0
Hydraulic crane with a log grab	1.67	−7.82	6.15	0
Trailer/semi-trailer	2.29	−0.78	−1.51	0
Crossbars	−1.78	2.05	−0.26	0
All construction groups altogether (10)	0	0	0	0

).

To achieve the result, a simple linear correlation in the form of the Pearson correlation chi-square test was used (

Table 9. Results of simple linear correlation—Pearson’s chi-square test.

	Chi-Square	df	p
Pearson Chi-square	14.47	df = 18	0.698

). This linear correlation was used because the variables were measured on an interval scale. The correlation coefficient does not depend on the scale on which the variables were measured, i.e., the division into groups does not depend on the producer of the vehicle. Three producers of hauling equipment and ten construction groups of the machine were correlated. The correlation coefficient came out the same. The correlation is high if the measured points in the plane can be translated by the method of least squares regression, from which the residuals are subsequently derived. The resulting correlation (agreement) for individual construction groups of transportation vehicles, depending on the producers, is 69.8%, i.e., the statement that the division into construction groups of the machine does not depend on the producer of the vehicle was confirmed.

Based on the measured results, histograms were made for individual construction groups, with a comparison of the frequency of events for individual producers (Figure 3).

The authors [14,18,28] devoted themselves to processing the issues of machine reliability, service time, and effective monitoring of operating parameters. S. Sankararaman, 2013 [29] proposes a computational methodology for quantifying the individual contributions of variability and uncertainty of distribution parameters to the overall uncertainty of a random variable. Robert B. Stone et al. (2005) [30] explore the utility of a new design methodology that allows an FMEA-style failure analysis to be performed during conceptual design. The functional failure design method (FFDM) guides designers to improved designs by predicting likely failure modes based on the intended functionality of the product. Reliability prediction deals with the evaluation of a design prior to the actual construction of the system. Although product reliability is not increased by the prediction process, the result of reliability prediction provides an early indication as to whether a design is likely to meet reliability goals, points to potential reliability problem areas in a new design or design modifications, and identifies components needing further testing. It is a tool to determine as early as possible whether the equipment will be reliable enough or whether it needs further improvement to function successfully for the company.

The system of organizing maintenance represents a significant internal source for increasing the reliability of machines and equipment. A positive result can be achieved through planning, management, improving the organization of work, and recording data, up to the management of spare parts. In other words, the maintenance system is a means for equipment to keep them in good technical condition or restore this good technical condition for the duration of their technical life or for the period that their operator can maintain considering the costs incurred [31]. Ormon et al. (2002) present three general procedures (using both simulation and analytical solution techniques) to predict system reliability and average mission cost. Procedures take into account both known and unknown failure rates and analysis at the component and subsystem levels.

As for the monitored means of transport, it is difficult to say which of the producers is most suitable for use in the operating conditions of Slovak forestry. Each forest means of transport has its pluses and minuses when deployed in real conditions. However, it is unequivocally possible to say whose operational reliability, through the intensity of failures, mean time to failure, and other monitored parameters, turned out to be the most reliable.

The research was conducted under real-world working conditions at a company running a wood processing business. This is a very important fact because the company follows all service requirements. The research results were obtained with the help of observed objects, software, and statistical methods. They are going to solve the problem of maintenance costs and create more effective operational conditions. The maintenance organization system is an important internal source for increasing the reliability of machinery and equipment. A positive result can be achieved through planning, management, improvement of work organization, data recording, and the management of spare parts [7]. In other words, a maintenance system is a means of maintaining or restoring equipment to good technical condition for the duration of its technical life or for a period that its operator can maintain in view of the costs incurred.

All manufacturers try to implement their own know-how and technologies, with which they try to reach their customers and operators of the given means of transport. Most of the mentioned manufacturer’s systems are usable only with certain limitations, and therefore the manual collection of operating data is still used for the evaluation of operation data. IVECO’s devices have an integrated ELEMENTS system in the upper ranges, which provides the possibility of planning preventive maintenance of the device, which maximizes the service life of the vehicle. The manufacturer of SCANIA vehicles provides its own service method for its vehicles with higher equipment. It is a system integrated into the vehicle so that shutdowns are planned and maximum operability is ensured. Vehicle operation data is accessible 24 h a day to the contractual service center on the digital platform, while know-how about the service vehicle is provided in real time. The manufacturer TATRA equips its vehicles with a system called the BBM superstructure module, with which the manufacturer tries to meet the ever-increasing requirements for the operational reliability of its vehicles.

4. Conclusions

Based on the results and statistical analyses, it can be said that IVECO achieves the best operational reliability, followed by SCANIA, and only then TATRA. Documenting and analyzing failure states of individual construction groups of means of transport, a percentage evaluation of the occurrence of failure states of individual construction groups of monitored equipment is calculated according to the manufacturers of TATRA, SCANIA, and IVECO. Considering the confirmed claim that the division of the machine into structural groups does not depend on the manufacturer of the means of transport, a percentage evaluation of failure states was also created for all monitored means of transport as a whole. Individual results are presented in the text.

The research into the operational reliability of the machines clearly shows that, with the IVECO brand, the failure rate of the machine ranges from 1–22% when monitoring the structural groups of the machine. Iveco has the highest failure rate with construction group no. 8—hydraulic crane with log grab (22%). With a small difference, the SCANIA machine shows a lower failure rate (19%), which was evaluated for the maximum failure rate in the group transmissions and transmission mechanisms (transmissions, couplings, shafts, joints, gearboxes, differentials, and gear systems). The lowest failure rate of the monitored machines was observed with the TATRA brand (18%). Problems occurred with the construction group of machine no. 8—a hydraulic crane with log grab. If the operators were to focus on the costs spent on the operation of these means of transport, they would spend the lowest funds on the means of transport produced by IVECO, followed by TATRA, and the most funds would be spent on the operation of SCANIA vehicles. If the parameter for the choice of use and operation was the number of replaced parts, then the smallest number of replaced parts was for tractors of IVECO vehicles, followed by SCANIA, and the highest number of replaced parts for the monitored period was for tractors of vehicles produced by TATRA. If the operators were to compare the number of repairs on transport vehicles, TATRA vehicles would have the least number of repairs, followed by SCANIA and IVECO vehicles, which would have the most repairs.