Elastic and Strength Properties of Heat-Treated Beech and Birch Wood
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Methods
3. Results
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| MS = 1663.7 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.947 | |||||
| Beech | 210 | 0.002 * | 0.002 * | ||||
| Birch | REF | 0.001 * | 0.001 * | 0.720 | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.466 | 0.673 | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.000 * | 0.000 * | 0.000 * | |
| MS = 3.0963 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.705 | |||||
| Beech | 210 | 0.746 | 0.934 | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.000 * | 0.965 | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.000 * | 0.037 | 0.043 | |
| MS = 5,622,000 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.459 | |||||
| Beech | 210 | 0.261 | 0.077 | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.008 * | 0.065 | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.004 * | 0.115 | 0.711 | |
| MS = 2,308,000 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.093 | |||||
| Beech | 210 | 0.002 * | 0.000 * | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.343 | 0.000 * | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.001 * | 0.093 | 0.013 | |
| MS = 279.08 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.154 | |||||
| Beech | 210 | 0.000 * | 0.000 * | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.000 * | 0.000 * | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.157 | 0.000 * | 0.000 * | |
| MS = 4.7429 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.000 * | |||||
| Beech | 210 | 0.000 * | 0.000 * | ||||
| Birch | REF | 0.386 | 0.001 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.001 * | 0.000 * | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.444 | 0.000 * | 0.000 * | |
| MS = 101.28 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.780 | |||||
| Beech | 210 | 0.000 * | 0.000 * | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.074 | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.383 | 0.010 * | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.243 | 0.470 | 0.053 | |
| MS = 127.42 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.629 | |||||
| Beech | 210 | 0.000 * | 0.000 * | ||||
| Birch | REF | 0.000 * | 0.000 * | 0.009 * | |||
| Birch | 165 | 0.000 * | 0.000 * | 0.121 | 0.245 | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.036 | 0.558 | 0.513 | |
| MS = 0.46655 | Beech | Beech | Beech | Birch | Birch | Birch | |
|---|---|---|---|---|---|---|---|
| DF = 174 | REF | 165 | 210 | REF | 165 | 210 | |
| Beech | REF | ||||||
| Beech | 165 | 0.000 * | |||||
| Beech | 210 | 0.000 * | 0.000 * | ||||
| Birch | REF | 0.026 | 0.000 * | 0.000 * | |||
| Birch | 165 | 0.000 * | 0.024 | 0.000 * | 0.000 * | ||
| Birch | 210 | 0.000 * | 0.000 * | 0.000 * | 0.000 * | 0.000 * | |
References
- Ministry of Agriculture. Zpráva o Stavu Lesa a Lesního Hospodářství ČR v Roce 2016; Report on the State of Forest and Forestry in the Czech Republic 2014; Ministry of Agriculture: Prague, Czech Republic, 2017.
- Bhuiyan, M.T.R.; Hirai, N.; Sobue, N. Changes of crystallinity in wood cellulose by heat treatment under dried and moist conditions. J. Wood Sci. 2000, 46, 431–436. [Google Scholar] [CrossRef]
- Bhuiyan, M.T.R.; Hirai, N.; Sobue, N. Effect of intermittent heat treatment on crystallinity in wood cellulose. J. Wood Sci. 2001, 47, 336–341. [Google Scholar] [CrossRef]
- Bhuiyan, M.T.R.; Hirai, N.; Sobue, N. Behavior of piezoelectric, dielectric, and elastic constants of wood during about 40 repeated measurements between 100 °C and 220 °C. J. Wood Sci. 2002, 48, 1–7. [Google Scholar] [CrossRef]
- Bhuiyan, M.T.R.; Hirai, N.; Sobue, N. Study of crystalline behavior of heat-treated wood cellulose during treatments in water. J. Wood Sci. 2005, 51, 42–47. [Google Scholar] [CrossRef]
- Bourgois, J.; Bartholin, M.C.; Guyonnet, R. Thermal treatment of wood: analysis of the obtained product. Wood Sci. Technol. 1989, 23, 303–310. [Google Scholar] [CrossRef]
- Cousins, W.J. Young’s modulus of hemicellulose as related to moisture content. Wood Sci. Technol. 1978, 12, 161–167. [Google Scholar] [CrossRef]
- Esteves, B.M.; Pereira, H.M. Wood modification by heat treatment: A review. BioResources 2009, 4, 370–404. [Google Scholar]
- Fengel, D.; Wegener, G. Wood: Chemistry, Ultrastructure, Reactions, 2nd ed.; Walter de Gruyter: Berlin, Germany, 1989; p. 613. ISBN 978-0899255934. [Google Scholar]
- Garrote, G.; Domínguez, H.; Parajó, J.C. Hydrothermal processing of lignocellulosic materials. Holz als Roh- und Werkstoff 1999, 57, 191–202. [Google Scholar] [CrossRef]
- Hill, C.A.S. Wood Modification: Chemical, Thermal and Other Processes; John Wiley & Sons: London, UK, 2006; p. 260. ISBN 978-0-470-02172-9. [Google Scholar]
- Hon, D.N.-S.; Shiraishi, N. Wood and Cellulosic Chemistry—Second Edition, Revised, and Expanded; Marcel Dekker: New York, NY, USA, 2001; p. 928. ISBN 978-0824700249. [Google Scholar]
- Kačíková, D.; Kačík, F. Chemické a Mechanické Zmeny Dreva pri Termickej Úprave (Chemical and Mechanical Changes of Wood Due to the Thermal Modification); Technical University in Zvolen: Zvolen, Slovakia, 2011. [Google Scholar]
- Kvietková, M.; Gašparík, M.; Gaff, M. Effect of thermal treatment on surface quality of beech wood after plane milling. BioResources 2015, 10, 4226–4238. [Google Scholar] [CrossRef]
- Navi, P.; Sandberg, D. Thermo-Hydro-Mechanical Wood Processing, 1st ed.; CRC Press: Lausanne, Switzerland, 2012; p. 280. ISBN 978-1439860427. [Google Scholar]
- Reinprecht, L.; Vidholdová, Z. Termodrevo—Príprava, Vlastnosti a Aplikácie (ThermoWood—Preparation, Properties and Applications); Technical University in Zvolen: Zvolen, Slovakia, 2008. [Google Scholar]
- Richardson, B.A. Wood Preservation, 2nd ed.; Taylor & Francis: London, UK; New York, NY, USA, 1993; p. 240. ISBN 978-0419174905. [Google Scholar]
- Sandberg, D.; Kutnar, A.; Mantanis, G. Wood modification technologies—A review. iForest 2017, 10, 895–908. [Google Scholar] [CrossRef]
- Shmulsky, R.; Jones, P.D. Forest Products and Wood Science, 6th ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2011; p. 496. ISBN 978-0813820743. [Google Scholar]
- Smith, I.; Landis, E.; Gong, M. Fracture and Fatigue in Wood, 1st ed.; John Wiley & Sons: Chichester, UK, 2003; p. 242. ISBN 978-0471487081. [Google Scholar]
- Stamm, J.A. Wood and Cellulose Science; Ronald Press Co.: New York, NY, USA, 1964; p. 549. ISBN 978-0826084958. [Google Scholar]
- Tsoumis, G.T. Science and Technology of Wood—Structure, Properties, Utilization, 1st ed.; Van Nostrand Reinhold: New York, NY, USA, 1991; p. 494. ISBN 9780442239855. [Google Scholar]
- Ugolev, B.; Gorbacheva, G.; Belkovskiy, S. Quantification of wood memory effect. In Wood the Best Material for Mankind; Arbora Publishers: Zvolen, Slovakia, 2013; pp. 31–37. ISBN 978-80-968868-6-9. [Google Scholar]
- Windeisen, E.; Strobel, C.; Wegener, G. Chemical changes during the production of thermo-treated beech wood. Wood Sci. Technol. 2007, 41, 523–536. [Google Scholar] [CrossRef]
- Viitaniemi, P.; Ranta-Maunus, A.; Jämsä, S.; Ek, P. Method for Processing of Wood at Elevated Temperatures. Patent EP-0759137 VTT, 11 May 1995. [Google Scholar]
- Kubojima, Y.; Okano, T.; Ohta, M. Bending strength and toughness of heat-treated wood. J. Wood Sci. 2000, 46, 8–15. [Google Scholar] [CrossRef]
- Kubojima, Y.; Okano, T.; Ohta, M. Vibrational properties of heat-treated green wood. J. Wood Sci. 2000, 46, 63–67. [Google Scholar] [CrossRef]
- Tiryaki, S. Investigating The Relationship Between Some Mechanical Properties and Weight Loss in Heat Treated Woods. J. Polytech. 2015, 18, 149–154. [Google Scholar] [CrossRef]
- Holeček, T.; Gašparík, M.; Lagaňa, R.; Borůvka, V.; Oberhofnerová, E. Measuring the modulus of elasticity of thermally treated spruce wood using the ultrasound and resonance methods. BioResources 2017, 12, 819–838. [Google Scholar] [CrossRef]
- Poncsak, S.; Kocaefe, D.; Bouazara, M.; Pichette, A. Effect of high temperature treatment on the mechanical properties of birch (Betula papyrifera). Wood Sci. Technol. 2006, 40, 647–663. [Google Scholar] [CrossRef]
- Candelier, K.; Thevenon, M.-F.; Petrissans, A.; Dumarcay, S.; Gerardin, P.; Petrissans, M. Control of wood thermal treatment and its effects on decay resistance: A review. Ann. For. Sci. 2016, 73, 571–583. [Google Scholar] [CrossRef]
- Xie, Y.; Liu, Y.; Sun, Y. Heat-treated wood and its development in Europe. J. For. Res. 2002, 13, 224–230. [Google Scholar] [CrossRef]
- Chung, H.; Park, Y.; Yang, S.-Y.; Kim, H.; Han, Y.; Chang, Y.-S.; Yeo, H. Effect of heat treatment temperature and time on sound absorption coefficient of Larix kaempferi wood. J. Wood Sci. 2017, 63, 575–579. [Google Scholar] [CrossRef]
- Asseva, R.; Serkov, B.; Sivenkov, A. Fire Behavior and Fire Protection in Timber Buildings, 1st ed.; Springer: Dordrecht, The Netherlands, 2014; p. 290. ISBN 978-94-007-7459-9. [Google Scholar]
- Reinprecht, L. Wood Deterioration, Protection and Maintenance, 1st ed.; John Wiley & Sons: Oxford, UK, 2016; p. 376. ISBN 978-1119106531. [Google Scholar]
- Reinprecht, L.; Vidholdová, Z. Termodrevo (ThermoWood); Šmíra-Print: Ostrava, Czech Republic, 2011; p. 290. ISBN 978-80-87427-05-7. [Google Scholar]
- Borůvka, V.; Zeidler, A.; Holeček, T. Comparison of Stiffness and Strength Properties of Untreated and Heat-Treated Wood of Douglas Fir and Alder. BioResources 2015, 10, 8281–8294. [Google Scholar] [CrossRef]
- Wagenführ, R. Holzatlas, 5th ed.; Fachbuchverlag: Leipzig, Germany, 2000; p. 707. ISBN 978-3446213906. [Google Scholar]
- Požgaj, A.; Chovanec, D.; Kurjatko, S.; Babiak, M. Štruktúra a Vlastnosti Dreva (Structure and Properties of Wood), 1st ed.; Príroda: Bratislava, Slovakia, 1993; p. 485. ISBN 80-07-00600-1. [Google Scholar]
- Bodig, J.; Jayne, B.A. Mechanics of Wood and Wood Composites, 1st ed.; Van Nostrand Reinhold: New York, NY, USA, 1982; p. 712. ISBN 0442008228. [Google Scholar]
- ČSN 49 0103. Drevo. Zisťovanie Vlhkosti pri Fyzikálnych a Mechanických Skúškach (Wood. Determination of Moisture Content at Physical and Mechanical Testing); Office for Standardization and Measurement: Prague, Czech Republic, 1 December 1979. [Google Scholar]
- ČSN 49 0108. Drevo. Zisťovanie Hustoty (Wood. Determination of the Density); Czech Standards Institute: Prague, Czech Republic, 1 March 1993. [Google Scholar]
- ČSN 49 0115. Drevo. Zisťovanie Medze Pevnosti v Statickom Ohybe (Wood. Determination of Ultimate Strength in Flexure Tests); Office for Standardization and Measurement: Prague, Czech Republic, 1 December 1979. [Google Scholar]
- ČSN 49 0116. Drevo. Metóda Zisťovania Modulu Pružnosti pri Statickom Ohybe (Wood. Determination of the Modulus of Elasticity in Static Bending); Office for Standardization and Measurement: Prague, Czech Republic, 1 January 1982. [Google Scholar]
- ČSN 49 0117. Drevo. Rázová Húževnatosť v Ohybe (Wood. Impact Strength in Flexure); Office for Standardization and Measurement: Prague, Czech Republic, 1 January 1980. [Google Scholar]
- ČSN 64 0128. Zkoušky Tuhých Nekovových Materiálů. Tvrdost Podle Brinella (Testing of Rigid Non-Metallic Materials. Hardness According to Brinell); Czech Office for Standardization: Prague, Czech Republic, 1 May 1953. [Google Scholar]
- ČSN EN 1534 (492124). Dřevěné podlahoviny—Stanovení Odolnosti Proti Vtisku—Metoda zkoušení (Wood Flooring—Determination of Resistence to Indentation—Test Method); Czech Office for Standards, Metrology and Testing: Prague, Czech Republic, 1 May 2011. [Google Scholar]
- FAKOPP Ultrasonic Timer User’s Guide. Available online: http://fakopp.com/docs/products/ultrasonic/UltrasonicGuide.pdf (accessed on 19 February 2018).
- Dinwoodie, J.M. Timber: Its Nature and Behavior, 2nd ed.; CRC Press: New York, NY, USA, 2000; p. 272. ISBN 978-0419235804. [Google Scholar]
- ITA (International Thermowood Association). Thermowood Handbook; International Thermowood Association: Helsinki, Finland, 2003; Available online: http://vanhoorebeke.com/docs/Thermowood handboek.pdf (accessed on 19 February 2018).
- Johansson, D. Heat Treatment of Solid Wood (Effects on Absorption, Strength and Colour). Ph.D. Thesis, Luleå University of Technology, Luleå, Sweden, 2008. [Google Scholar]
- Zobel, B.J.; Buijtenen, J.P. Wood Variation: Its Causes and Control, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1989; p. 363. ISBN 978-3-642-74071-8. [Google Scholar]
- Zobel, B.J.; Sprague, J.R. Juvenile Wood in Forest Trees, 1st ed.; Springer: Berlin/Heidelberg, Germany, 1998; p. 304. ISBN 978-3-642-72128-1. [Google Scholar]
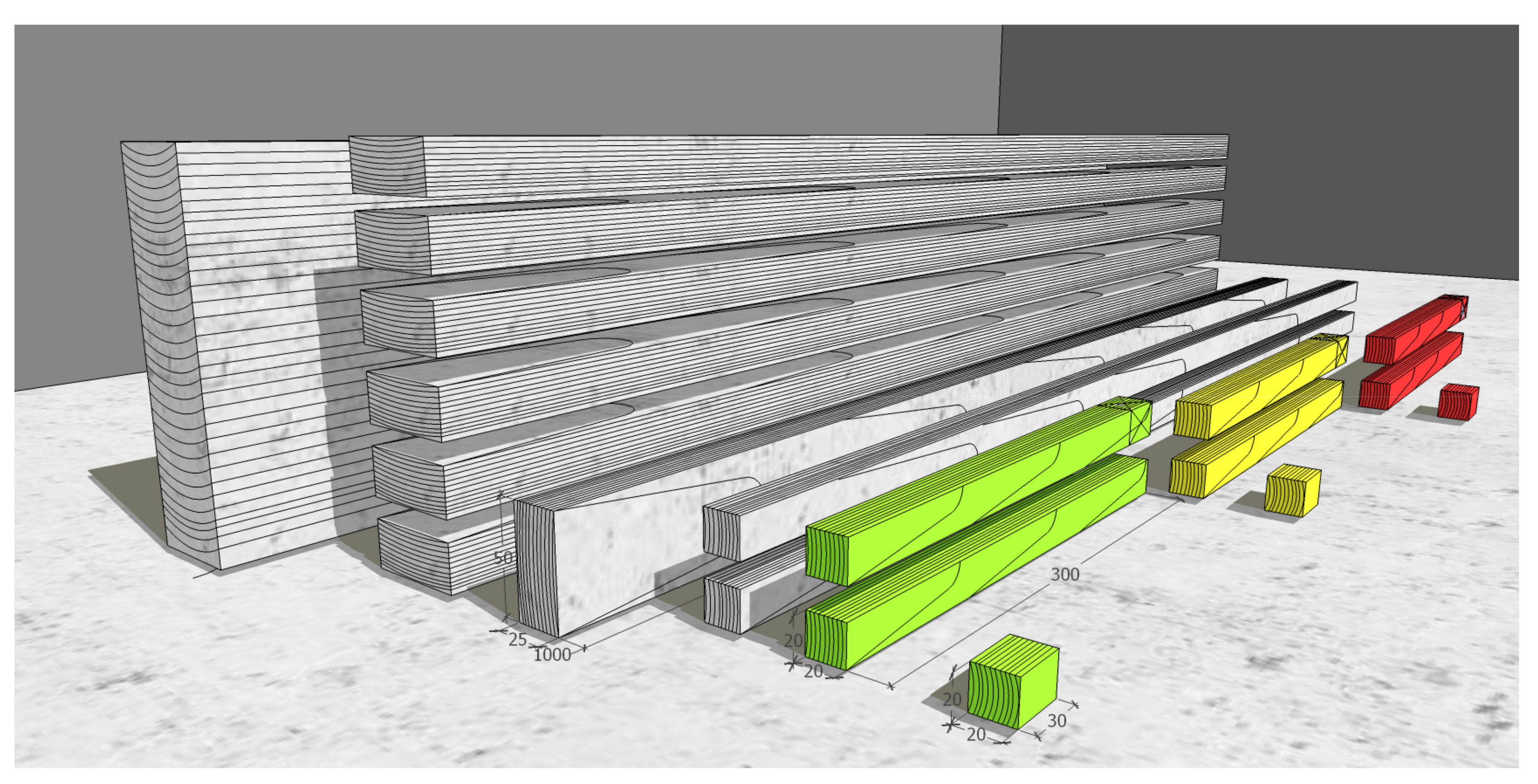

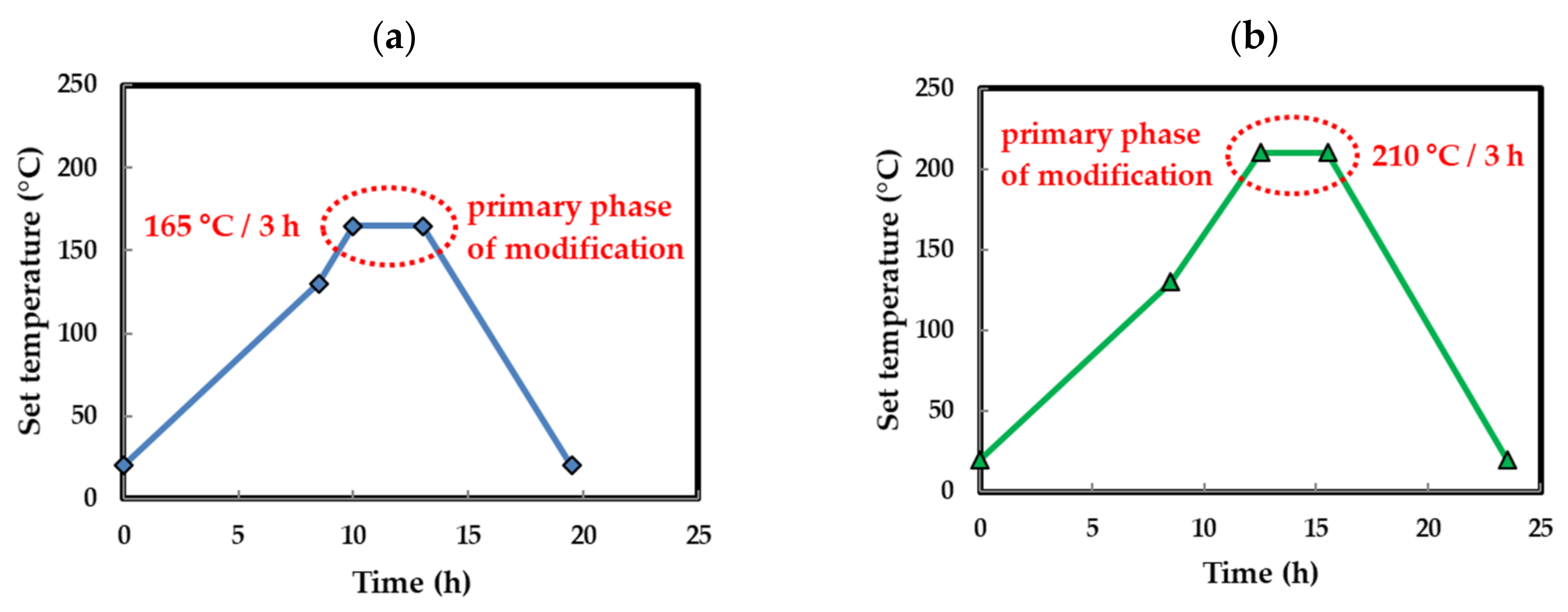



| Technical Parameters | |
|---|---|
| Filling capacity of furnace | 0.38 m3 |
| Maximum load capacity | 150 kg |
| Maximum reachable temperature | 300 °C |
| Maximum working temperature | 250 °C |
| Power consumption | 3 kWh |
| Wood Species 1 | ||||||
|---|---|---|---|---|---|---|
| Beech | Birch | |||||
| Min. | Mean | Max. | Min. | Mean | Max. | |
| Density (kg/m3) | 540 | 720 | 910 | 510 | 650 | 830 |
| Static modulus of elasticity (MPa) | 10,000 | 16,000 | 18,000 | 14,500 | 16,500 | |
| Bending strength (MPa) | 74 | 123 | 210 | 76 | 147 | 155 |
| Impact bending strength (J/cm2) | 3.0 | 10.0 | 19.0 | 4.5 | 10.0 | 13.0 |
| Hardness LR/LT (MPa) | 34 | 22 | 27 | |||
| Properties | Heat Treatment Degree | Minimum | Mean | Maximum | Std.Dev. | Coef.Var. (%) |
|---|---|---|---|---|---|---|
| Density | REF | 622 | 676 | 729 | 27 | 4.0 |
| (kg/m3) | 165 | 615 | 676 | 742 | 33 | 4.8 |
| 210 | 579 | 643 | 802 | 53 | 8.3 | |
| Annual | REF | 1.1 | 2.1 | 3.4 | 0.4 | 19.3 |
| ring width | 165 | 1.3 | 1.9 | 3.0 | 0.4 | 22.2 |
| (mm) | 210 | 1.0 | 2.0 | 4.3 | 0.7 | 34.1 |
| Dynamic | REF | 10,107 | 12,986 | 16,583 | 1639 | 12.6 |
| modulus of elasticity | 165 | 10,077 | 13,439 | 16,959 | 1878 | 14.0 |
| (MPa) | 210 | 8779 | 12,297 | 18,925 | 2281 | 18.5 |
| Static | REF | 8735 | 10,056 | 11,142 | 740 | 7.4 |
| modulus of elasticity | 165 | 8909 | 10,715 | 12,703 | 1070 | 10.0 |
| (MPa) | 210 | 7007 | 8830 | 11,072 | 1053 | 11.9 |
| Modulus of rupture- | REF | 86.7 | 102.7 | 117.3 | 8.1 | 7.9 |
| bending strength | 165 | 75.4 | 108.8 | 130.2 | 14.6 | 13.5 |
| (MPa) | 210 | 27.9 | 42.0 | 62.5 | 9.0 | 21.5 |
| Toughness-impact | REF | 4.1 | 8.4 | 16.4 | 2.7 | 32.6 |
| bending strength | 165 | 2.5 | 6.1 | 10.0 | 1.9 | 30.6 |
| (J/cm2) | 210 | 0.3 | 1.6 | 3.9 | 0.9 | 59.3 |
| Hardness LR | REF | 14.5 | 41.5 | 60.8 | 11.0 | 26.4 |
| (MPa) | 165 | 14.8 | 40.7 | 57.3 | 9.9 | 24.4 |
| 210 | 13.3 | 26.1 | 53.8 | 11.4 | 43.9 | |
| Hardness LT | REF | 35.8 | 53.8 | 61.8 | 7.3 | 13.6 |
| (MPa) | 165 | 32.0 | 55.2 | 65.5 | 7.5 | 13.6 |
| 210 | 20.3 | 40.9 | 64.3 | 12.2 | 29.8 | |
| Specific strength- | REF | 127.8 | 152.0 | 168.5 | 11.5 | 7.6 |
| MOR/Density | 165 | 110.2 | 160.7 | 181.0 | 18.0 | 11.2 |
| (kN·m/kg) | 210 | 39.3 | 65.5 | 90.6 | 13.5 | 20.6 |
| Specific strength- | REF | 54.4 | 79.5 | 93.8 | 10.2 | 12.8 |
| Hardness LT/Density | 165 | 51.4 | 81.4 | 92.6 | 9.5 | 11.7 |
| (kN·m/kg) | 210 | 32.5 | 63.3 | 94.3 | 16.6 | 26.2 |
| Moisture content | REF | 11.6 | 12.0 | 12.4 | 0.2 | 1.5 |
| (%) | 165 | 8.3 | 9.2 | 11.0 | 0.5 | 5.9 |
| 210 | 4.5 | 5.9 | 7.4 | 0.8 | 13.0 |
| Properties | Heat Treatment Degree | Minimum | Mean | Maximum | Std.Dev. | Coef.Var. (%) |
|---|---|---|---|---|---|---|
| Density | REF | 493 | 639 | 696 | 42 | 6.5 |
| (kg/m3) | 165 | 501 | 634 | 721 | 43 | 6.7 |
| 210 | 467 | 588 | 691 | 42 | 7.2 | |
| Annual | REF | 3.3 | 7.5 | 13.6 | 2.5 | 33.5 |
| ring width | 165 | 2.1 | 7.6 | 14.7 | 2.8 | 36.5 |
| (mm) | 210 | 2.7 | 6.6 | 10.9 | 1.9 | 29.5 |
| Dynamic | REF | 4824 | 9485 | 17,585 | 2998 | 31.6 |
| modulus of elasticity | 165 | 6482 | 10,676 | 15,749 | 2385 | 22.3 |
| (MPa) | 210 | 3307 | 10,449 | 15,997 | 2764 | 26.5 |
| Static | REF | 1464 | 6829 | 10,407 | 2138 | 31.3 |
| modulus of elasticity | 165 | 1264 | 8458 | 10,965 | 1956 | 23.1 |
| (MPa) | 210 | 3178 | 7487 | 10,231 | 1628 | 21.8 |
| Modulus of rupture- | REF | 8.1 | 67.7 | 101.2 | 24.1 | 35.6 |
| bending strength | 165 | 8.5 | 85.1 | 127.0 | 25.1 | 29.5 |
| (MPa) | 210 | 8.9 | 35.9 | 62.1 | 10.0 | 27.8 |
| Toughness-impact | REF | 1.6 | 7.9 | 13.4 | 3.6 | 46.0 |
| bending strength | 165 | 0.3 | 3.4 | 7.1 | 1.8 | 52.2 |
| (J/cm2) | 210 | 0.5 | 1.1 | 2.6 | 0.5 | 46.2 |
| Hardness LR | REF | 13.2 | 21.2 | 44.6 | 6.1 | 28.9 |
| (MPa) | 165 | 14.7 | 28.4 | 62.3 | 12.9 | 45.4 |
| 210 | 14.2 | 23.1 | 41.7 | 7.4 | 32.2 | |
| Hardness LT | REF | 16.2 | 32.8 | 52.0 | 12.7 | 38.6 |
| (MPa) | 165 | 16.7 | 36.4 | 70.6 | 12.3 | 33.8 |
| 210 | 14.2 | 34.5 | 65.7 | 13.9 | 40.4 | |
| Specific strength- | REF | 12.3 | 106.6 | 150.5 | 37.9 | 35.6 |
| MOR/Density | 165 | 12.4 | 135.1 | 213.5 | 40.7 | 30.1 |
| (kN·m/kg) | 210 | 15.6 | 61.5 | 109.3 | 18.3 | 29.8 |
| Specific strength- | REF | 27.1 | 50.7 | 76.7 | 17.9 | 35.2 |
| Hardness LT/Density | 165 | 27.2 | 57.1 | 103.6 | 17.7 | 31.1 |
| (kN·m/kg) | 210 | 28.6 | 58.1 | 98.0 | 21.7 | 37.3 |
| Moisture content | REF | 10.0 | 12.4 | 13.5 | 0.7 | 5.5 |
| (%) | 165 | 4.9 | 8.8 | 10.7 | 1.1 | 13.1 |
| 210 | 3.7 | 4.4 | 5.2 | 0.3 | 7.5 |
| Heat Treatment Degree | Beech | Birch | |
|---|---|---|---|
| Density | 165/REF | 0 | −1 |
| 210/REF | −5 | −8 | |
| Dynamic modulus of elasticity | 165/REF | 3 | 13 |
| 210/REF | −5 | 10 | |
| Static modulus of elasticity | 165/REF | 7 | 24 |
| 210/REF | −12 | 10 | |
| Modulus of rupture-bending strength | 165/REF | 6 | 26 |
| 210/REF | −59 | −47 | |
| Toughness-impact bending strength | 165/REF | −28 | −56 |
| 210/REF | −81 | −86 | |
| Hardness LR | 165/REF | −2 | 34 |
| 210/REF | −37 | 9 | |
| Hardness LT | 165/REF | 3 | 11 |
| 210/REF | −24 | 5 | |
| Moisture content | 165/REF | −23 | −29 |
| 210/REF | −51 | −64 |
| Wood Species | Mannans | Xylans |
|---|---|---|
| Beech | 0.9 | 19.0 |
| Birch | 3.2 | 24.9 |
| Pine | 12.4 | 7.6 |
| Fir | 10.0 | 5.2 |
| 210/REF | |||
|---|---|---|---|
| Density | MOR | Toughness | |
| Beech | 5 | 59 | 81 |
| Birch | 8 | 47 | 86 |
| Douglas Fir | 4 | 8 | 34 |
| Alder | 10 | 45 | 63 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Borůvka, V.; Zeidler, A.; Holeček, T.; Dudík, R. Elastic and Strength Properties of Heat-Treated Beech and Birch Wood. Forests 2018, 9, 197. https://doi.org/10.3390/f9040197
Borůvka V, Zeidler A, Holeček T, Dudík R. Elastic and Strength Properties of Heat-Treated Beech and Birch Wood. Forests. 2018; 9(4):197. https://doi.org/10.3390/f9040197
Chicago/Turabian StyleBorůvka, Vlastimil, Aleš Zeidler, Tomáš Holeček, and Roman Dudík. 2018. "Elastic and Strength Properties of Heat-Treated Beech and Birch Wood" Forests 9, no. 4: 197. https://doi.org/10.3390/f9040197
APA StyleBorůvka, V., Zeidler, A., Holeček, T., & Dudík, R. (2018). Elastic and Strength Properties of Heat-Treated Beech and Birch Wood. Forests, 9(4), 197. https://doi.org/10.3390/f9040197






