Microdosimetric Investigation and a Novel Model of Radiosensitization in the Presence of Metallic Nanoparticles
Abstract
1. Introduction
2. Materials and Methods
2.1. Generalized Formulation of the Theory of Dual Radiation Action (TDRA)
2.2. The Application of TDRA to NP Radiosensitization
2.3. A Novel Phenomenological Model—Bomb Model
2.4. The Nanoparticles and the Cell Models
2.5. MC Simulation of Irradiation on NP and Secondary Electrons Transport
2.6. Postprocessing of the Results from the MC Simulations
3. Results
3.1. Parameters λ and μ
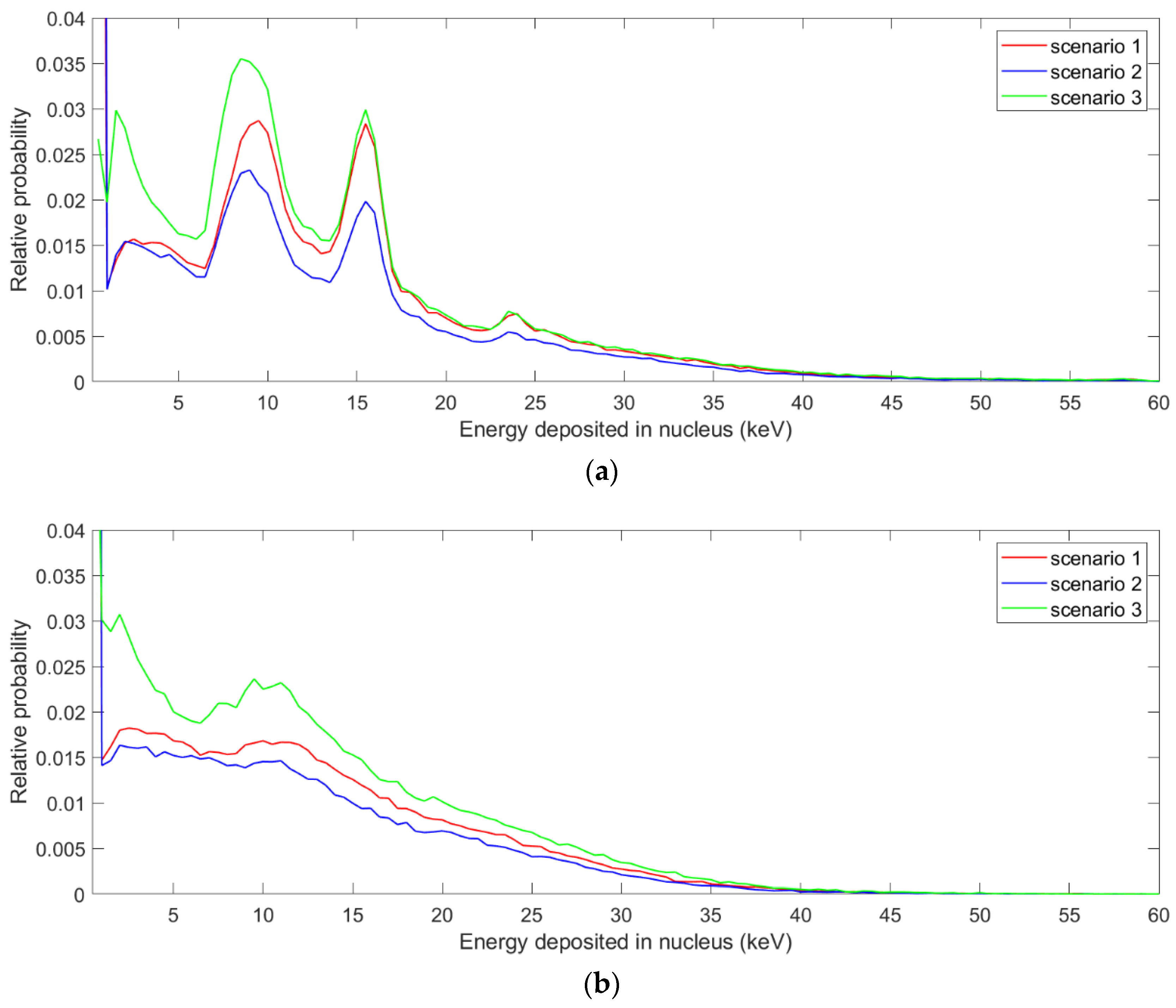
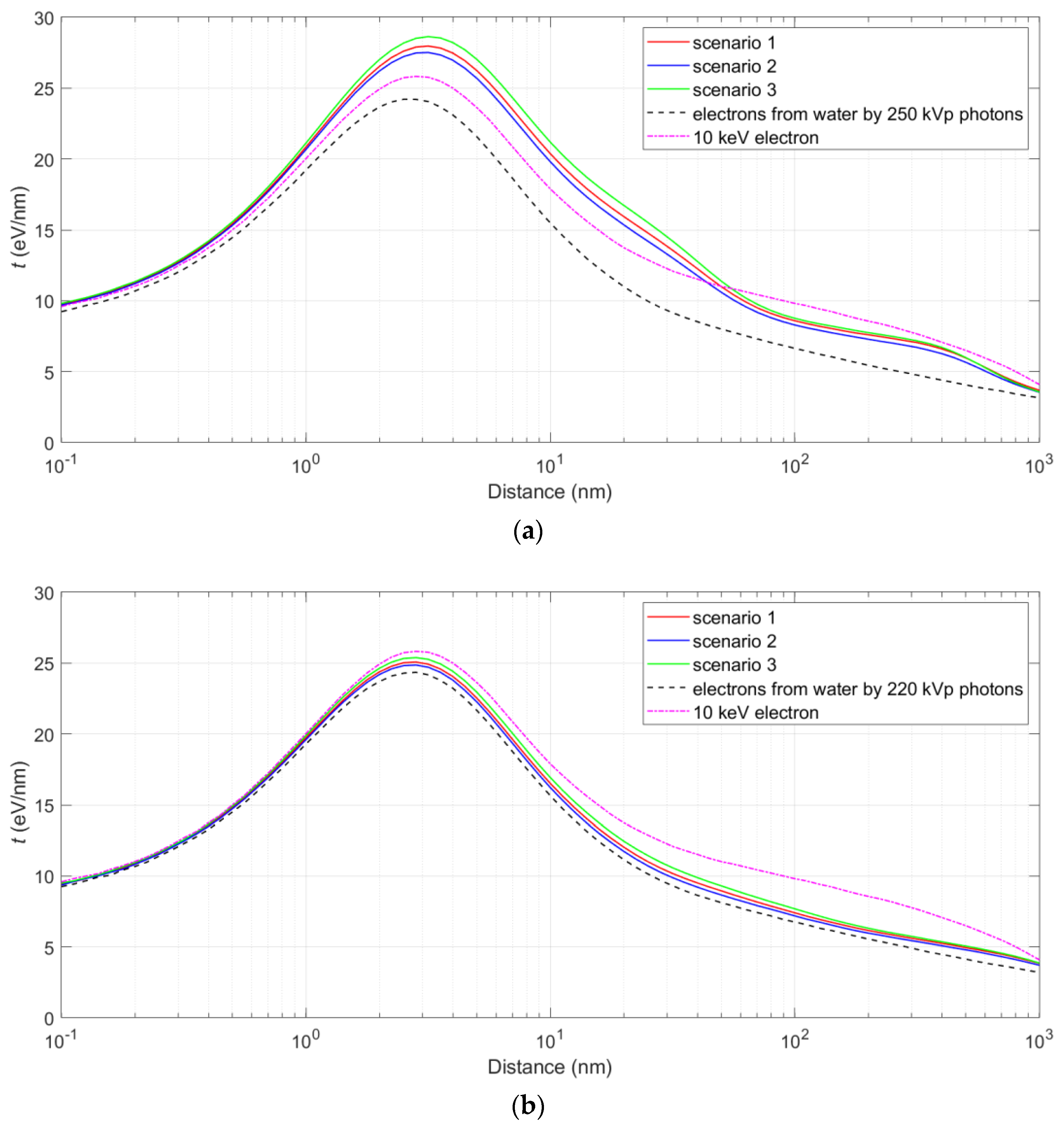
3.2. The Spectrum of Energy Deposited in the Nucleus and the Proximity Function
3.3. Parameter a of the Distance Model, and the Changes in α and RBE
3.4. Predictions by the Bomb Model for the Radiosensitization
4. Discussion
4.1. Study Limitations and Implications of the Microdosimetric Investigation
4.2. Implications of the Bomb Model
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Checklist Item # | Item Name | Description | References |
|---|---|---|---|
| 2, 3 | Code, version/release date | Geant4, v.10.07.p02/released on 14 June 2021 | Ref. [41] http://geant4.web.cern.ch/ accessed on (10 December 2021) |
| 4, 17 | Validation | The general Geant4 framework has been validated extensively. | https://geant-val.cern.ch/ accessed on (10 December 2021) |
| 5 | Timing | All simulations were performed on an Intel® Xeon(R) CPU E5-2690 v2, with a 64GB memory. In Step 1, each simulation took about 7500 s for 2 × 109 histories. In Step 2, each took about 1800 s for 2 × 104 histories. In Step 3, each took about 1800 s for 5 × 105 histories. In Step 4, each took about 2700 s for 2 × 106 histories. | |
| 8 | Source description | The spectra of the parallel beams of 105 kVp, 220 kVp, 250 kVp were generated using SpekCalc. Elekta 6 MV spectrum presented by Sheikh-Bagheri et al. was used for the 6MV source. | Refs. [65,66] |
| 9 | Cross-sections | Steps 1 and 3, Livermore package incorporated in Geant4; Step 2: Geant4-DNA option 2. Step 4: Livermore package incorporated in Geant4 was used for photon and electron transport in the NPs, and Geant4-DNA option 2 was used for electron transport in water. | Refs. [42,43,44,45,67] |
| 10 | Transport parameters | Steps 1 and 3, the minimum threshold of secondary particle production was used (250 eV); Step 2: tracking cut was set to 7.4 eV; Step 4: tracking cut was set to 7.4 eV for the transport of electrons in water; the minimum threshold of secondary particle production (250 eV) and lowest electron energy of 7.4 eV were used for the transport of electrons in the NPs. | |
| 11 | VRT and/or AEIT | Step 1: Geometrical importance sampling was used for the region near the water cylinder center; Step 2: Neither VRT nor AEIT was used; Step 3: physics-based biasing was used to amplify the Compton scattering and photo-electric interaction cross-sections, secondary electrons and photons were killed upon generation; Step 4: the same physics-based biasing as in Step 3 was used for the transport of photons in the NPs. | Ref. [68] |
| 12 | Scored quantities | Step 1: number and phase-space data of photons entering the NP-representing sphere, electrons spectrum in the sphere and dose near the sphere; Step 2: the energy deposition of electrons in water; Step 3: the number of ionizations in an NP and the number of photons entering the NP; Step 4: the number of ionizations and the energy deposition of secondary electrons in the nucleus. | Ref. [69] |
| 13, 18 | # of histories/statistical uncertainty | To achieve <2% relative uncertainty for the quantities to calculate, 2 × 109, 2 × 104, 5 × 106, and 2 × 106 histories were used for the simulations in Steps 1, 2, 3, and 4, respectively. | |
| 14 | Statistical methods | The history-by-history method was used. | Ref. [70] |
| 15, 16 | Postprocessing | See Section 2.6 for details. |
References
- Lechtman, E.; Chattopadhyay, N.; Cai, Z.; Mashouf, S.; Reilly, R.; Pignol, J.P. Implications on clinical scenario of gold nanoparticle radiosensitization in regards to photon energy, nanoparticle size, concentration and location. Phys. Med. Biol. 2011, 56, 4631–4647. [Google Scholar] [CrossRef] [PubMed]
- McMahon, S.J.; Hyland, W.B.; Muir, M.F.; Coulter, J.A.; Jain, S.; Butterworth, K.T.; Schettino, G.; Dickson, G.R.; Hounsell, A.R.; O’Sullivan, J.M.; et al. Biological consequences of nanoscale energy deposition near irradiated heavy atom nanoparticles. Sci. Rep. 2011, 1, 18. [Google Scholar] [CrossRef] [PubMed]
- Butterworth, K.T.; McMahon, S.J.; Currell, F.J.; Prise, K.M. Physical basis and biological mechanisms of gold nanoparticle radiosensitization. Nanoscale 2012, 4, 4830–4838. [Google Scholar] [CrossRef]
- Luchette, M.; Korideck, H.; Makrigiorgos, M.; Tillement, O.; Berbeco, R. Radiation dose enhancement of gadolinium-based AGuIX nanoparticles on HeLa cells. Nanomed. Nanotechnol. Biol. Med. 2014, 10, 1751–1755. [Google Scholar] [CrossRef]
- Taupin, F.; Flaender, M.; Delorme, R.; Brochard, T.; Mayol, J.-F.; Arnaud, J.; Perriat, P.; Sancey, L.; Lux, F.; Barth, R.F.; et al. Gadolinium nanoparticles and contrast agent as radiation sensitizers. Phys. Med. Biol. 2015, 60, 4449–4464. [Google Scholar] [CrossRef]
- Rosa, S.; Connolly, C.; Schettino, G.; Butterworth, K.T.; Prise, K.M. Biological mechanisms of gold nanoparticle radiosensitization. Cancer Nanotechnol. 2017, 8, 2. [Google Scholar] [CrossRef]
- Balagurumoorthy, P.; Xu, X.; Wang, K.; Adelstein, S.J.; Kassis, A.I. Effect of distance between decaying125I and DNA on Auger-electron induced double-strand break yield. Int. J. Radiat. Biol. 2012, 88, 998–1008. [Google Scholar] [CrossRef] [PubMed]
- Kellerer, A.M. 2—Fundamentals of Microdosimetry. In The Dosimetry of Ionizing Radiation; Kase, K.R., BjÄRngard, B.E., Attix, F.H., Eds.; Academic Press: Orlando, FL, USA, 1985; pp. 77–162. [Google Scholar]
- Kellerer, A.M.; Rossi, H.H. The theory of dual radiation action. Curr. Top. Radiat. Res. Q. 1972, 8, 85–158. [Google Scholar]
- Rossi, H.H.; Zaider, M. Compound dual radiation action. I. General aspects. Radiat. Res. 1992, 132, 178–183. [Google Scholar] [CrossRef] [PubMed]
- Wang, C.K.; Zhang, X. Compound dual radiation action theory for 252Cf brachytherapy. Radiat. Prot. Dosim. 2004, 110, 801–806. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.; Zhang, H.; Wang, Z.; Min, X.; Liu, Y.; Wu, Z.; Sun, C.; Hu, B. Chromosomal aberrations in the bone marrow cells of mice induced by accelerated 12C6+ ions. Mutat. Res. Mol. Mech. Mutagen. 2011, 716, 20–26. [Google Scholar] [CrossRef]
- Hawkins, R.B. A microdosimetric-kinetic theory of the dependence of the RBE for cell death on LET. Med. Phys. 1998, 25, 1157–1170. [Google Scholar] [CrossRef] [PubMed]
- Hofmann, W.; Li, W.B.; Friedland, W.; Miller, B.W.; Madas, B.; Bardiès, M.; Balásházy, I. Internal microdosimetry of alpha-emitting radionuclides. Radiat. Environ. Biophys. 2019, 59, 29–62. [Google Scholar] [CrossRef]
- Kyriakou, I.; Tremi, I.; Georgakilas, A.G.; Emfietzoglou, D. Microdosimetric investigation of the radiation quality of low-medium energy electrons using Geant4-DNA. Appl. Radiat. Isot. 2021, 172, 109654. [Google Scholar] [CrossRef]
- Francis, Z.; Villagrasa, C.; Clairand, I. Simulation of DNA damage clustering after proton irradiation using an adapted DBSCAN algorithm. Comput. Methods Progr. Biomed. 2011, 101, 265–270. [Google Scholar] [CrossRef]
- Kellerer, A.M.; Rossi, H.H. A Generalized Formulation of Dual Radiation Action1. Radiat. Res. 2012, 178, AV204–AV213. [Google Scholar] [CrossRef] [PubMed]
- Scholz, M.; Kraft, G. Calculation of Heavy Ion Inactivation Probabilities Based on Track Structure, X-ray Sensitivity and Target Size. Radiat. Prot. Dosim. 1994, 52, 29–33. [Google Scholar] [CrossRef]
- Elsässer, T.; Scholz, M. Cluster Effects within the Local Effect Model. Radiat. Res. 2007, 167, 319–329. [Google Scholar] [CrossRef] [PubMed]
- Hawkins, R.B. A Microdosimetric-Kinetic Model for the Effect of Non-Poisson Distribution of Lethal Lesions on the Variation of RBE with LET. Radiat. Res. 2003, 160, 61–69. [Google Scholar] [CrossRef]
- Tobias, C.A. The repair-misrepair model in radiobiology: Comparison to other models. Radiat. Res. 1985, 104 (Suppl. 8), S77–S95. [Google Scholar] [CrossRef]
- Curtis, S.B. Lethal and Potentially Lethal Lesions Induced by Radiation—A Unified Repair Model. Radiat. Res. 1986, 106, 252. [Google Scholar] [CrossRef]
- Carlson, D.J.; Stewart, R.D.; Semenenko, V.A.; Sandison, G.A. Combined Use of Monte Carlo DNA Damage Simulations and Deterministic Repair Models to Examine Putative Mechanisms of Cell Killing. Radiat. Res. 2008, 169, 447–459. [Google Scholar] [CrossRef]
- Goodhead, D.T. Saturable repair models of radiation action in mammalian cells. Radiat. Res. 1985, 104 (Suppl. 8), S58–S67. [Google Scholar] [CrossRef]
- Lechtman, E.; Mashouf, S.; Chattopadhyay, N.; Keller, B.M.; Lai, P.; Cai, Z.; Reilly, R.M.; Pignol, J.-P. A Monte Carlo-based model of gold nanoparticle radiosensitization accounting for increased radiobiological effectiveness. Phys. Med. Biol. 2013, 58, 3075–3087. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; McMahon, S.; Paganetti, H.; Schuemann, J. Biological modeling of gold nanoparticle enhanced radiotherapy for proton therapy. Phys. Med. Biol. 2015, 60, 4149–4168. [Google Scholar] [CrossRef] [PubMed]
- Stewart, R.D.; Carlson, D.J.; Butkus, M.P.; Hawkins, R.; Friedrich, T.; Scholz, M. A comparison of mechanism-inspired models for particle relative biological effectiveness (RBE). Med. Phys. 2018, 45, e925–e952. [Google Scholar] [CrossRef]
- Abolfath, R.M.; Carlson, D.J.; Chen, Z.J.; Nath, R. A molecular dynamics simulation of DNA damage induction by ionizing radiation. Phys. Med. Biol. 2013, 58, 7143–7157. [Google Scholar] [CrossRef] [PubMed]
- Taschereau, R.; Roy, R.; Pouliot, J. A comparison of methods to calculate biological effectiveness (RBE) from Monte Carlo simulations. Med. Dosim. 2003, 28, 21–26. [Google Scholar] [CrossRef]
- Chithrani, D.B.; Jelveh, S.; Jalali, F.; Van Prooijen, M.; Allen, C.; Bristow, R.; Hill, R.P.; Jaffray, D. Gold Nanoparticles as Radiation Sensitizers in Cancer Therapy. Radiat. Res. 2010, 173, 719–728. [Google Scholar] [CrossRef]
- Miladi, I.; Aloy, M.-T.; Armandy, E.; Mowat, P.; Kryza, D.; Magné, N.; Tillement, O.; Lux, F.; Billotey, C.; Janier, M.; et al. Combining ultrasmall gadolinium-based nanoparticles with photon irradiation overcomes radioresistance of head and neck squamous cell carcinoma. Nanomed. Nanotechnol. Biol. Med. 2015, 11, 247–257. [Google Scholar] [CrossRef]
- Liu, W.; Deacon, J.; Yan, H.; Sun, B.; Liu, Y.; Hegan, D.; Li, Q.; Coman, D.; Parent, M.; Hyder, F.; et al. Tumor-targeted pH-low insertion peptide delivery of theranostic gadolinium nanoparticles for image-guided nanoparticle-enhanced radiation therapy. Transl. Oncol. 2020, 13, 100839. [Google Scholar] [CrossRef]
- Detappe, A.; Kunjachan, S.; Rottmann, J.; Robar, J.; Tsiamas, P.; Korideck, H.; Tillement, O.; Berbeco, R. AGuIX nanoparticles as a promising platform for image-guided radiation therapy. Cancer Nanotechnol. 2015, 6, 4. [Google Scholar] [CrossRef] [PubMed]
- Sancey, L.; Lux, F.; Kotb, S.; Roux, S.; Dufort, S.; Bianchi, A.; Crémillieux, Y.; Fries, P.; Coll, J.-L.; Rodriguez-Lafrasse, C.; et al. The use of theranostic gadolinium-based nanoprobes to improve radiotherapy efficacy. Br. J. Radiol. 2014, 87, 20140134. [Google Scholar] [CrossRef]
- Chithrani, D.; Ghazani, A.A.; Chan, W.C.W. Determining the Size and Shape Dependence of Gold Nanoparticle Uptake into Mammalian Cells. Nano Lett. 2006, 6, 662–668. [Google Scholar] [CrossRef] [PubMed]
- Falzone, N.; Lee, B.Q.; Able, S.; Malcolm, J.; Terry, S.; Alayed, Y.; Vallis, K.A. Targeting Micrometastases: The Effect of Heterogeneous Radionuclide Distribution on Tumor Control Probability. J. Nucl. Med. 2018, 60, 250–258. [Google Scholar] [CrossRef]
- Borle, A.B. Kinetic Analyses of Calcium Movements in HeLa Cell Cultures. J. Gen. Physiol. 1969, 53, 57–69. [Google Scholar] [CrossRef]
- Monier, K.; Armas, J.C.G.; Etteldorf, S.; Ghazal, P.; Sullivan, K. Annexation of the interchromosomal space during viral infection. Nature 2000, 2, 661–665. [Google Scholar] [CrossRef] [PubMed]
- Jiang, R.-D.; Shen, H.; Piao, Y.-J. The morphometrical analysis on the ultrastructure of A549 cells. Rom. J. Morphol. Embryol. Rev. Roum. Morphol. Embryol. 2010, 51, 663–667. [Google Scholar]
- Štefančíková, L.; Porcel, E.; Eustache, P.; Li, S.; Salado, D.; Marco, S.; Guerquin-Kern, J.-L.; Réfrégiers, M.; Tillement, O.; Lux, F.; et al. Cell localisation of gadolinium-based nanoparticles and related radiosensitising efficacy in glioblastoma cells. Cancer Nanotechnol. 2014, 5, 6. [Google Scholar] [CrossRef] [PubMed]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.; et al. GEANT4-a simulation toolkit. Nucl. Instrum. Meth. A 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Incerti, S.; Ivanchenko, A.; Karamitros, M.; Mantero, A.; Moretto, P.; Tran, N.H.; Mascialino, B.; Champion, C.; Ivanchenko, V.; Bernal, M.A.; et al. Comparison ofGEANT4very low energy cross section models with experimental data in water. Med. Phys. 2010, 37, 4692–4708. [Google Scholar] [CrossRef]
- Incerti, S.; Baldacchino, G.; Bernal, M.; Capra, R.; Champion, C.; Francis, Z.; Guèye, P.; Mantero, A.; Mascialino, B.; Moretto, P.; et al. The Geant4-DNA project. Int. J. Modeling Simul. Sci. Comput. 2010, 1, 157–178. [Google Scholar] [CrossRef]
- Incerti, S.; Kyriakou, I.; Bernal, M.A.; Bordage, M.C.; Francis, Z.; Guatelli, S.; Ivanchenko, V.; Karamitros, M.; Lampe, N.; Lee, S.B.; et al. Geant4-DNA example applications for track structure simulations in liquid water: A report from the Geant4-DNA Project. Med. Phys. 2018, 45, e722–e739. [Google Scholar] [CrossRef]
- Bernal, M.A.; Bordage, M.C.; Brown, J.M.; Davidkova, M.; Delage, E.; el Bitar, Z.; Enger, S.A.; Francis, Z.; Guatelli, S.; Ivanchenko, V.N.; et al. Track structure modeling in liquid water: A review of the Geant4-DNA very low energy extension of the Geant4 Monte Carlo simulation toolkit. Phys. Med. 2015, 31, 861–874. [Google Scholar] [CrossRef]
- Incerti, S.; Kyriakou, I.; Bordage, M.C.; Guatelli, S.; Ivanchenko, V.; Emfietzoglou, D. Track structure simulations of proximity functions in liquid water using the Geant4-DNA toolkit. J. Appl. Phys. 2019, 125, 104301. [Google Scholar] [CrossRef]
- Wozny, A.-S.; Aloy, M.-T.; Alphonse, G.; Magné, N.; Janier, M.; Tillement, O.; Lux, F.; Beuve, M.; Rodriguez-Lafrasse, C. Gadolinium-based nanoparticles as sensitizing agents to carbon ions in head and neck tumor cells. Nanomed. Nanotechnol. Biol. Med. 2017, 13, 2655–2660. [Google Scholar] [CrossRef] [PubMed]
- Rima, W.; Sancey, L.; Aloy, M.-T.; Armandy, E.; Alcantara, G.B.; Epicier, T.; Malchère, A.; Joly-Pottuz, L.; Mowat, P.; Lux, F.; et al. Internalization pathways into cancer cells of gadolinium-based radiosensitizing nanoparticles. Biomaterials 2013, 34, 181–195. [Google Scholar] [CrossRef] [PubMed]
- Rashkow, J.T.; Patel, S.C.; Tappero, R.; Sitharaman, B. Quantification of single-cell nanoparticle concentrations and the distribution of these concentrations in cell population. J. R. Soc. Interface 2014, 11, 20131152. [Google Scholar] [CrossRef]
- Rothen-Rutishauser, B.; A Kuhn, D.; Ali, Z.; Gasser, M.; Amin, F.; Parak, W.J.; Vanhecke, D.; Fink, A.; Gehr, P.; Brandenberger, C. Quantification of gold nanoparticle cell uptake under controlled biological conditions and adequate resolution. Nanomedicine 2014, 9, 607–621. [Google Scholar] [CrossRef]
- Wéra, A.-C.; Heuskin, A.-C.; Riquier, H.; Michiels, C.; Lucas, S. Low-LET Proton Irradiation of A549 Non-small Cell Lung Adenocarcinoma Cells: Dose Response and RBE Determination. Radiat. Res. 2013, 179, 273–281. [Google Scholar] [CrossRef]
- Taggart, L.E.; McMahon, S.; Butterworth, K.; Currell, F.; Schettino, G.; Prise, K. Protein disulphide isomerase as a target for nanoparticle-mediated sensitisation of cancer cells to radiation. Nanotechnology 2016, 27, 215101. [Google Scholar] [CrossRef] [PubMed]
- Howard, D.; Sebastian, S.; Le, Q.V.-C.; Thierry, B.; Kempson, I. Chemical Mechanisms of Nanoparticle Radiosensitization and Radioprotection: A Review of Structure-Function Relationships Influencing Reactive Oxygen Species. Int. J. Mol. Sci. 2020, 21, 579. [Google Scholar] [CrossRef] [PubMed]
- Simonet, S.; Rodriguez-Lafrasse, C.; Beal, D.; Gerbaud, S.; Malesys, C.; Tillement, O.; Lux, F.; Fayyad-Kazan, H.; Rachidi, W.; Ardail, D. Gadolinium-Based Nanoparticles Can Overcome the Radioresistance of Head and Neck Squamous Cell Carcinoma Through the Induction of Autophagy. J. Biomed. Nanotechnol. 2020, 16, 111–124. [Google Scholar] [CrossRef] [PubMed]
- Goodhead, D.T.; Thacker, J.; Cox, R. Effects of Radiations of Different Qualities on Cells: Molecular Mechanisms of Damage and Repair. Int. J. Radiat. Biol. 1993, 63, 543–556. [Google Scholar] [CrossRef]
- Nikjoo, H.; Lindborg, L. RBE of low energy electrons and photons. Phys. Med. Biol. 2010, 55, R65–R109. [Google Scholar] [CrossRef]
- Chmelevsky, D.; Kellerer, A.M.; Terrissol, M.; Patau, J.P. Proximity Functions for Electrons up to 10 keV. Radiat. Res. 1980, 84, 219. [Google Scholar] [CrossRef][Green Version]
- Lux, F.; Sancey, L.; Bianchi, A.; Crémillieux, Y.; Roux, S.; Tillement, O. Gadolinium-based nanoparticles for theranostic MRI-radiosensitization. Nanomedicine 2015, 10, 1801–1815. [Google Scholar] [CrossRef]
- Huo, S.; Jin, S.; Ma, X.; Xue, X.; Yang, K.; Kumar, A.; Wang, P.C.; Zhang, J.; Hu, Z.; Liang, X.-J. Ultrasmall Gold Nanoparticles as Carriers for Nucleus-Based Gene Therapy Due to Size-Dependent Nuclear Entry. ACS Nano 2014, 8, 5852–5862. [Google Scholar] [CrossRef]
- Jain, S.; Coulter, J.; Hounsell, A.R.; Butterworth, K.; McMahon, S.; Hyland, W.B.; Muir, M.F.; Dickson, G.R.; Prise, K.; Currell, F.; et al. Cell-Specific Radiosensitization by Gold Nanoparticles at Megavoltage Radiation Energies. Int. J. Radiat. Oncol. 2011, 79, 531–539. [Google Scholar] [CrossRef]
- Butterworth, K.T.; Coulter, J.A.; Jain, S.; Forker, J.; McMahon, S.J.; Schettino, G.; Prise, K.M.; Currell, F.J.; Hirst, D.G. Evaluation of cytotoxicity and radiation enhancement using 1.9 nm gold particles: Potential application for cancer therapy. Nanotechnology 2010, 21, 295101. [Google Scholar] [CrossRef] [PubMed]
- Kotb, S.; Detappe, A.; Lux, F.; Appaix, F.; Barbier, E.; Tran, V.-L.; Plissonneau, M.; Gehan, H.; Lefranc, F.; Rodriguez-Lafrasse, C.; et al. Gadolinium-Based Nanoparticles and Radiation Therapy for Multiple Brain Melanoma Metastases: Proof of Concept before Phase I Trial. Theranostics 2016, 6, 418–427. [Google Scholar] [CrossRef]
- Stewart, C.; Konstantinov, K.; McKinnon, S.; Guatelli, S.; Lerch, M.; Rosenfeld, A.; Tehei, M.; Corde, S. First proof of bismuth oxide nanoparticles as efficient radiosensitisers on highly radioresistant cancer cells. Phys. Med. 2016, 32, 1444–1452. [Google Scholar] [CrossRef]
- Sechopoulos, I.; Rogers, D.W.O.; Bazalova-Carter, M.; Bolch, W.E.; Heath, E.C.; McNitt-Gray, M.F.; Sempau, J.; Williamson, J.F. RECORDS: Improved Reporting of montE CarlO RaDiation transport Studies: Report of the AAPM Research Committee Task Group 268. Med. Phys. 2017, 45, e1–e5. [Google Scholar] [CrossRef]
- Poludniowski, G.; Landry, G.; Deblois, F.; Evans, P.; Verhaegen, F. SpekCalc: A program to calculate photon spectra from tungsten anode x-ray tubes. Phys. Med. Biol. 2009, 54, N433–N438. [Google Scholar] [CrossRef]
- Sheikh-Bagheri, D.; Rogers, D.W.O. Monte Carlo calculation of nine megavoltage photon beam spectra using the BEAM code. Med. Phys. 2002, 29, 391–402. [Google Scholar] [CrossRef]
- Geant4—Physics Reference Manual. Release 10.7. 2020. Available online: https://geant4-userdoc.web.cern.ch/UsersGuides/PhysicsReferenceManual/BackupVersions/V10.7/fo/PhysicsReferenceManual.pdf (accessed on 10 December 2021).
- Geant4—Book for Application Developers. Release 10.7. 2020. Available online: https://geant4-userdoc.web.cern.ch/UsersGuides/ForApplicationDeveloper/BackupVersions/V10.7/fo/BookForApplicationDevelopers.pdf (accessed on 10 December 2021).
- Williamson, J.F. Monte Carlo evaluation of kerma at a point for photon transport problems. Med. Phys. 1987, 14, 567–576. [Google Scholar] [CrossRef]
- Verhaegen, F.; Seco, J. Monte Carlo Techniques in Radiation Therapy; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]


| NP | Photon Beam | λ (Photons per Gy per NP) | μ (Ionizations per Photon) | (Ionizations per Gy per NP) |
|---|---|---|---|---|
| AGuIX | 250 kVp | 0.168 ± 0.003 | (3.00 ± 0.06) × 10−7 | (5.04 ± 0.14) × 10−8 |
| 50 nm AuNP | 105 kVp | 46.1 ± 0.9 | (6.41 ± 0.13) × 10−4 | (2.96 ± 0.08) × 10−2 |
| 220 kVp | 47.7 ± 1.0 | (4.09 ± 0.08) × 10−4 | (1.95 ± 0.05) × 10−2 | |
| 137Cs (660 keV) | 5.97 ± 0.12 | (8.96 ± 0.18) × 10−6 | (5.35 ± 0.15) × 10−5 | |
| 6 MV | 2.63 ± 0.05 | (4.63 ± 0.09) × 10−6 | (1.22 ± 0.03) × 10−5 |
| NP | Scenarios of NP Distribution | Concentration (# per Cell) | Δξ (Gy) | Δαcal/α a | Δαexp/α | RBE at 2 Gy | DER b |
|---|---|---|---|---|---|---|---|
| AGuIX (3 nm) | 1 | 6.06 × 108 | 0.027–0.078 | 0.034 | 1.7–11 | 1.016–1.020 | 1.025 |
| 6.06 × 109 | 0.27–0.78 | 0.34 | 1.15–1.19 | 1.25 | |||
| 2 | 6.06 × 108 | 0.020–0.059 | 0.025 | 1.012–1.015 | 1.019 | ||
| 6.06 × 109 | 0.20–0.59 | 0.25 | 1.12–1.15 | 1.19 | |||
| 3 | 6.06 × 108 | 0.031–0.089 | 0.038 | 1.017–1.022 | 1.027 | ||
| 6.06 × 109 | 0.31–0.89 | 0.38 | 1.16–1.21 | 1.27 | |||
| 50 nm AuNP | 1 | 6000 | 0.93 | 0.25 | 1.35 | 1.31 | 1.24 |
| 18,000 | 2.8 | 0.76 | 1.76 | 1.71 | |||
| 2 | 6000 | 0.75 | 0.21 | 1.25 | 1.20 | ||
| 18,000 | 2.3 | 0.62 | 1.64 | 1.59 | |||
| 3 | 6000 | 1.20 | 0.33 | 1.38 | 1.30 | ||
| 18,000 | 3.6 | 0.98 | 1.93 | 1.91 |
| Cell and NP | Irradiation Photons | # of NPs per Cell | α without NPs (Gy−1) | α with NPs (Gy−1) | p1 | Survival Fraction (SF) at 2Gy without NPs | Survival Fraction (SF) at 2Gy with NPs | RBE at 2Gy |
|---|---|---|---|---|---|---|---|---|
| SQ20B, AGuIX | 250 kVp | 6.06 × 108 | 0.04 | 0.5 | 0.015 a | 0.76 | 0.33 | 2.17 |
| A549, AGuIX | 1.66 × 107 | 0.332 ± 0.045 [51] | 0.349 ± 0.054 b | 0–5.6 × 10−2 | 0.48 | 0.46 | 1.04 | |
| 1.32 × 109 | 0.488 ± 0.063 b | (2.34 ± 0.67) × 10−3 | 0.35 | 1.37 | ||||
| Hela, AuNP | 105 kVp | 6000 | 0.237 ± 0.005 | 0.528 ± 0.007 | (1.64 ± 0.04) × 10−3 | 0.53 | 0.28 | 1.69 |
| 220 kVp | 6000 | 0.150 ± 0.004 | 0.352 ± 0.005 | (1.73 ± 0.05) × 10−3 | 0.63 | 0.42 | 1.56 | |
| 137Cs (660 keV) | 6000 | 0.119 ± 0.013 | 0.259 ± 0.011 | 0.436 ± 0.055 | 0.67 | 0.53 | 1.39 | |
| 6 MV | 6000 | 0.110 ± 0.008 | 0.191 ± 0.002 | 1.11 ± 0.12 | 0.71 | 0.60 | 1.35 |
| References | NP Type and Concentration | Radiation (Photons) | Cell Type | Change in α (Gy−1) | Change in β (Gy−2) |
|---|---|---|---|---|---|
| Chithrani et al. [30] | 50 nm Gold NP, 6000 NPs per cell, | 105 kVp | HeLa | 0.237 to 0.528 | 0.041 to 0.054 |
| 220 kVp | 0.150 to 0.352 | 0.041 to 0.041 | |||
| 137Cs (660 keV) | 0.119 to 0.259 | 0.040 to 0.030 | |||
| 6 MVp | 0.110 to 0.191 | 0.029 to 0.031 | |||
| Jain et al. [60] | 1.9 nm Gold NP, 12 μM | 160 kVp | MDA-MB-231 | 0.019 to 0.091 | 0.052 to 0.093 |
| 6 MV | 0.002 to 0.104 | 0.079 to 0.098 | |||
| 15 MV | 0.083 to 0.061 | 0.059 to 0.121 | |||
| Butterworth et al. [61] | 1.9 nm Gold NP, 10 μg/mL−1 | 160 kVp | AGO-1552B | 0.25 to 0.30 | 0.04 to 0.05 |
| Astro | 0.37 to 0.40 | 0.08 to 0.09 | |||
| DU-145 | 0.03 to 0.05 | 0.04 to 0.04 | |||
| L132 | 0.12 to 0.11 | 0.03 to 0.03 | |||
| MCF-7 | 0.46 to 0.28 | 0.02 to 0.07 | |||
| MDA-231-MB | 0.09 to 0.15 | 0.03 to 0.03 | |||
| PC-3 | 0.12 to 0.29 | 0.06 to 0.03 | |||
| T98G | 0.04 to 0.14 | 0.03 to 0.02 | |||
| 1.9 nm Gold NP, 100 μg/ml | AGO-1552B | 0.25 to 0.68 | 0.04 to <0.04 | ||
| Astro | 0.37 to 0.23 | 0.08 to 0.16 | |||
| DU-145 | 0.03 to 0.04 | 0.04 to 0.04 | |||
| L132 | 0.12 to 0.05 | 0.03 to 0.04 | |||
| MCF-7 | 0.46 to 0.24 | 0.02 to 0.08 | |||
| MDA-231-MB | 0.09 to 0.27 | 0.03 to 0.02 | |||
| PC-3 | 0.12 to 0.21 | 0.06 to 0.03 | |||
| T98G | 0.04 to 0.06 | 0.03 to 0.02 | |||
| Stefancikova et al. [40] | AGuIX, 0.5 mM | 1.25 MV | U87 | 0.4 to 0.71 | 0.03 to 0 |
| Miladi et al. [31] | AGuIX, 0.6 mM AGuIX | 250 kVp | SQ20B | 0.04 to 0.5 | 0.05 to 0.03 |
| FaDu | 0.01 to 0.2 | 0.08 to 0.07 | |||
| Cal33 | −0.05 to 0.07 | 0.08 to 0.11 | |||
| AGuIX, 0.4 mM AGuIX | SQ20B | 0.04 to 0.15 | 0.05 to 0.05 | ||
| Kotb et al. [62] | AGuIX, 0.6 mg/L AGuIX | 220 kVp | B16F10 | 0.056 to 0.275 | 0.025 to 0.022 |
| Stewart et al. [63] | Bi2O3 NP, 50 μg/mL | 125 kVp | 9 L gliosarcoma cell | 0.075 to 0.355 | 0.017 to 0 |
| 10 MV | 0.150 to 0.256 | 0.013 to 0.009 | |||
| Wozny et al. [47] | AGuIX, 0.8 mg/mL AGuIX | 250 kVp | SQ20B | 0.07 to 0.19 | 0.03 to 0.04 |
| Simonet et al. [54] | AGuIX, 0.8 mM Gd | 250 kVp | SQ20B J.L. | 0.1593 to 0.2357 | 0.0079 to 0.0088 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, H.; Carlson, D.J.; Abolfath, R.; Liu, W. Microdosimetric Investigation and a Novel Model of Radiosensitization in the Presence of Metallic Nanoparticles. Pharmaceutics 2021, 13, 2191. https://doi.org/10.3390/pharmaceutics13122191
Yan H, Carlson DJ, Abolfath R, Liu W. Microdosimetric Investigation and a Novel Model of Radiosensitization in the Presence of Metallic Nanoparticles. Pharmaceutics. 2021; 13(12):2191. https://doi.org/10.3390/pharmaceutics13122191
Chicago/Turabian StyleYan, Huagang, David J. Carlson, Ramin Abolfath, and Wu Liu. 2021. "Microdosimetric Investigation and a Novel Model of Radiosensitization in the Presence of Metallic Nanoparticles" Pharmaceutics 13, no. 12: 2191. https://doi.org/10.3390/pharmaceutics13122191
APA StyleYan, H., Carlson, D. J., Abolfath, R., & Liu, W. (2021). Microdosimetric Investigation and a Novel Model of Radiosensitization in the Presence of Metallic Nanoparticles. Pharmaceutics, 13(12), 2191. https://doi.org/10.3390/pharmaceutics13122191







