Impact of Powder Properties on the Rheological Behavior of Excipients
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Material Characterization
2.2.1. Shape
2.2.2. Laser Diffraction
2.2.3. Moisture Content
2.2.4. Specific Surface Area
2.2.5. Bulk and Tapped Density
2.2.6. Ring Shear Testing
2.2.7. Charge Density
2.2.8. True Density and Heckel Testing
2.3. Preparation of the Blends
2.4. Rotating Drum Method
2.5. Multivariate Analyses
2.6. Tableting
2.7. Tablet Testing
3. Results and Discussion
3.1. Raw Material Characterization
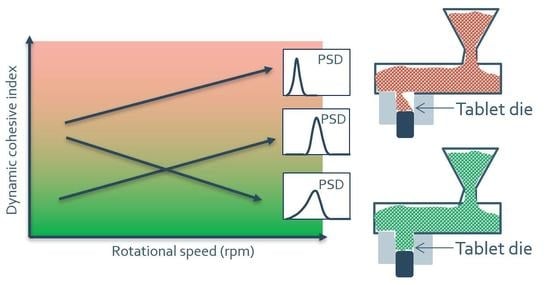
3.2. Cohesive Index as a Function of Rotational Speed—From Static to Dynamic Regimes
3.3. Multivariate Analyses to Reveal Drivers for the Rheological Index
3.4. Variation of the Amount of Fines
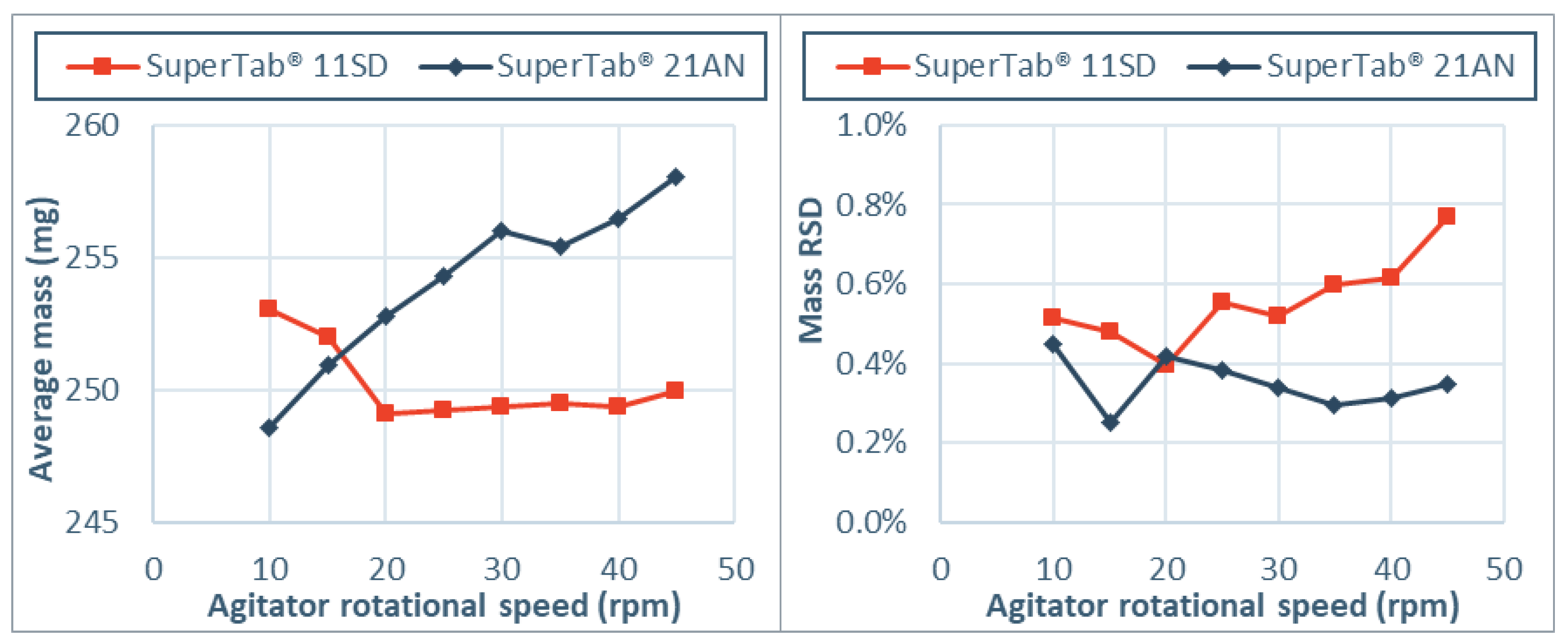
3.5. Correlating Rheological Behavior to Tableting Performance
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Krantz, M.; Zhang, H.; Zhu, J. Characterization of Powder Flow: Static and Dynamic Testing. Powder Technol. 2009, 194, 239–245. [Google Scholar] [CrossRef]
- Pernenkil, L.; Cooney, C.L. A Review on the Continuous Blending of Powders. Chem. Eng. Sci. 2006, 61, 720–742. [Google Scholar] [CrossRef]
- Prescott, J.K.; Barnum, R.A. On Powder Flowability. Pharm. Technol. 2000, 24, 60–85. [Google Scholar]
- Blackshields, C.A.; Crean, A.M. Continuous Powder Feeding for Pharmaceutical Solid Dosage Form Manufacture: A Short Review. Pharm. Dev. Technol. 2018, 23, 554–560. [Google Scholar] [CrossRef] [PubMed]
- Puckhaber, D.; Eichler, S.; Kwade, A.; Finke, J.H. Impact of Particle and Equipment Properties on Residence Time Distribution of Pharmaceutical Excipients in Rotary Tablet Presses. Pharmaceutics 2020, 12, 283. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peeters, E.; De Beer, T.; Vervaet, C.; Remon, J.P. Reduction of Tablet Weight Variability by Optimizing Paddle Speed in the Forced Feeder of a High-Speed Rotary Tablet Press. Drug Dev. Ind. Pharm. 2015, 41, 530–539. [Google Scholar] [CrossRef] [Green Version]
- Schwedes, J. Review on Testers for Measuring Flow Properties of Bulk Solids. Granul. Matter 2003, 5, 1–43. [Google Scholar] [CrossRef]
- Zhou, Y.C.; Xu, B.H.; Yu, A.B.; Zulli, P. An Experimental and Numerical Study of the Angle of Repose of Coarse Spheres. Powder Technol. 2002, 125, 45–54. [Google Scholar] [CrossRef]
- Ileleji, K.E.; Zhou, B. The Angle of Repose of Bulk Corn Stover Particles. Powder Technol. 2008, 187, 110–118. [Google Scholar] [CrossRef]
- Saker, A.; Cares-Pacheco, M.G.; Marchal, P.; Falk, V. Powders Flowability Assessment in Granular Compaction: What about the Consistency of Hausner Ratio? Powder Technol. 2019, 354, 52–63. [Google Scholar] [CrossRef]
- Saw, H.Y.; Davies, C.E.; Paterson, A.H.J.; Jones, J.R. Correlation between Powder Flow Properties Measured by Shear Testing and Hausner Ratio. Procedia Eng. 2015, 102, 218–225. [Google Scholar] [CrossRef] [Green Version]
- Zhou, X.; Nauka, E.; Narang, A.; Mao, C. Flow Function of Pharmaceutical Powders at Low-Stress Conditions Can Be Inferred Using a Simple Flow-Through-Orifice Device. J. Pharm. Sci. 2020, 109, 2009–2017. [Google Scholar] [CrossRef]
- Nalluri, V.R.; Kuentz, M. Flowability Characterisation of Drug-Excipient Blends Using a Novel Powder Avalanching Method. Eur. J. Pharm. Biopharm. 2010, 74, 388–396. [Google Scholar] [CrossRef]
- Pirard, S.L.; Lumay, G.; Vandewalle, N.; Pirard, J.P. Motion of Carbon Nanotubes in a Rotating Drum: The Dynamic Angle of Repose and a Bed Behavior Diagram. Chem. Eng. J. 2009, 146, 143–147. [Google Scholar] [CrossRef]
- Freeman, R. Measuring the Flow Properties of Consolidated, Conditioned and Aerated Powders-A Comparative Study Using a Powder Rheometer and a Rotational Shear Cell. Powder Technol. 2007, 174, 25–33. [Google Scholar] [CrossRef]
- Koynov, S.; Glasser, B.; Muzzio, F. Comparison of Three Rotational Shear Cell Testers: Powder Flowability and Bulk Density. Powder Technol. 2015, 283, 103–112. [Google Scholar] [CrossRef]
- Shah, R.B.; Tawakkul, M.A.; Khan, M.A. Comparative Evaluation of Flow for Pharmaceutical Powders and Granules. AAPS PharmSciTech 2008, 9, 250–258. [Google Scholar] [CrossRef] [Green Version]
- Stavrou, A.G.; Hare, C.; Hassanpour, A.; Wu, C.Y. Investigation of Powder Flowability at Low Stresses: Influence of Particle Size and Size Distribution. Powder Technol. 2020, 364, 98–114. [Google Scholar] [CrossRef]
- Wu, C.Y.; Dihoru, L.; Cocks, A.C.F. The Flow of Powder into Simple and Stepped Dies. Powder Technol. 2003, 134, 24–39. [Google Scholar] [CrossRef]
- Mendez, R.; Muzzio, F.; Velazquez, C. Study of the Effects of Feed Frames on Powder Blend Properties during the Filling of Tablet Press Dies. Powder Technol. 2010, 200, 105–116. [Google Scholar] [CrossRef]
- Mehrotra, A.; Chaudhuri, B.; Faqih, A.; Tomassone, M.S.; Muzzio, F.J. A Modeling Approach for Understanding Effects of Powder Flow Properties on Tablet Weight Variability. Powder Technol. 2009, 188, 295–300. [Google Scholar] [CrossRef]
- Lee, W.B.; Widjaja, E.; Heng, P.W.S.; Chan, L.W. The Effect of Rotation Speed and Particle Size Distribution Variability on Mixability: An Avalanche Rheological and Multivariate Image Analytical Approach. Int. J. Pharm. 2020, 579. [Google Scholar] [CrossRef]
- Lumay, G.; Boschini, F.; Traina, K.; Bontempi, S.; Remy, J.C.; Cloots, R.; Vandewalle, N. Measuring the Flowing Properties of Powders and Grains. Powder Technol. 2012, 224, 19–27. [Google Scholar] [CrossRef]
- Boschini, F.; Delaval, V.; Traina, K.; Vandewalle, N.; Lumay, G. Linking Flowability and Granulometry of Lactose Powders. Int. J. Pharm. 2015, 494, 312–320. [Google Scholar] [CrossRef] [PubMed]
- Geldart, D. Types of Gas Fhidization. Powder Technol. 1973, 7, 285–292. [Google Scholar] [CrossRef]
- Aulton, M.E.; Taylor, K.M.G. Powder flow. In Aulton’s Pharmaceutics E-Book: The Design and Manufacture of Medicines; Churchill Livingstone (Elsevier): Edinburgh, Scotland, 2013; pp. 189–200. ISBN 978-0702042904. [Google Scholar]
- Goh, H.P.; Heng, P.W.S.; Liew, C.V. Comparative Evaluation of Powder Flow Parameters with Reference to Particle Size and Shape. Int. J. Pharm. 2018, 547, 133–141. [Google Scholar] [CrossRef]
- Fu, X.; Huck, D.; Makein, L.; Armstrong, B.; Willen, U.; Freeman, T. Effect of Particle Shape and Size on Flow Properties of Lactose Powders. Particuology 2012, 10, 203–208. [Google Scholar] [CrossRef]
- Rajchenbach, J. Flow in Powders: From Discrete Avalanches to Continuous Regime. Phys. Rev. Lett. 1990, 65, 2221–2224. [Google Scholar] [CrossRef]
- Castellanos, A.; Valverde, J.M.; Pérez, A.T.; Ramos, A.; Watson, P.K. Flow Regimes in Fine Cohesive Powders. Phys. Rev. Lett. 1999, 82, 1156–1159. [Google Scholar] [CrossRef]
- Alexander, A.W.; Chaudhuri, B.; Faqih, A.M.; Muzzio, F.J.; Davies, C.; Tomassone, M.S. Avalanching Flow of Cohesive Powders. Powder Technol. 2006, 164, 13–21. [Google Scholar] [CrossRef]
- Neveu, A.; Janssen, P.; Lumay, G. The Flowability of Lactose Powders to Optimise Tableting Processes. ONdrugDelivery 2020, 2020, 58–62. [Google Scholar]
- Francia, V.; Yahia, L.A.A.; Ocone, R.; Ozel, A. From Quasi-Static to Intermediate Regimes in Shear Cell Devices: Theory and Characterisation. KONA Powder Part. J. 2021, 38, 3–25. [Google Scholar] [CrossRef]
- Tardos, G.I.; McNamara, S.; Talu, I. Slow and Intermediate Flow of a Frictional Bulk Powder in the Couette Geometry. Powder Technol. 2003, 131, 23–39. [Google Scholar] [CrossRef]
- Amidon, G.E.; Secreast, P.J.; Mudie, D. Particle, Powder, and Compact Characterization. Dev. Solid Oral Dos. Forms 2009, 163–186. [Google Scholar] [CrossRef]
- Shi, H.; Lumay, G.; Luding, S. Stretching the Limits of Dynamic and Quasi-Static Flow Testing on Cohesive Limestone Powders. Powder Technol. 2020, 367, 183–191. [Google Scholar] [CrossRef]
- Allenspach, C.; Timmins, P.; Lumay, G.; Holman, J.; Minko, T. Loss-in-Weight Feeding, Powder Flow and Electrostatic Evaluation for Direct Compression Hydroxypropyl Methylcellulose ( HPMC ) to Support Continuous Manufacturing. Int. J. Pharm. 2021, 596, 120259. [Google Scholar] [CrossRef] [PubMed]
- Hickey, A.J.; Mansour, H.M.; Telko, M.J.; Xu, Z.; Smyth, H.D.C.; Mulder, T.; McLean, R.; Langridge, J.; Papadopoulos, D. Physical Characterization of Component Particles Included in Dry Powder Inhalers. II. Dynamic Characteristics. J. Pharm. Sci. 2007, 96, 1302–1319. [Google Scholar] [CrossRef]
- Molerus, O.; Nywlt, M. The Influence of the Fine Particle Content of the Flow Behaviour of Bulk Materials. Powder Technol. 1984, 37, 145–154. [Google Scholar] [CrossRef]
- Pillitteri, S.; Opsomer, E.; Lumay, G.; Vandewalle, N. How Size Ratio and Segregation Affect the Packing of Binary Granular Mixtures. Soft Matter 2020, 16, 9094–9100. [Google Scholar] [CrossRef]
- Kojima, T.; Elliott, J.A. Incipient Flow Properties of Two-Component Fine Powder Systems and Their Relationships with Bulk Density and Particle Contacts. Powder Technol. 2012, 228, 359–370. [Google Scholar] [CrossRef]







| Characterization Technique | Physical Property | Abbreviation | Range of Values | Unit |
|---|---|---|---|---|
| Visual observation by scanning electron microscopy | Shape | Shape | - | - |
| Particle size distribution (PSD) by laser diffraction | 10% cumulative undersize of volumetric PSD | ×10 | 3.0–77.6 | μm |
| 50% cumulative undersize of volumetric PSD | ×50 | 18.3–243 | μm | |
| 90% cumulative undersize of volumetric PSD | ×90 | 49.4–406 | μm | |
| Span of the volumetric PSD* | Span | 1.22–2.83 | - | |
| Karl fisher titration | Total moisture content | KF | 0.1–5.8 | %w/w |
| Thermogravimetric balance | Loss on drying | LOD | 0.0–9.3 | %w/w |
| Brunauer–Emmett–Teller analysis with Krypton | Specific surface area | SSA | 0.1–5.1 | m2/g |
| Graduated cylinder | Bulk density | BD | 0.35–0.79 | g/mL |
| Tapped density | TD | 0.49–0.98 | g/mL | |
| Hausner ratio | HR | 1.15–1.60 | - | |
| Ring shear cell tester | Flow function coefficient at 4 kPa pre-consolidation pressure | ffc | 2.4–17.3 | - |
| Electric charge analyzer | Initial charge density | q0 | −1.5–0.2 | nC/g |
| Final charge density | qf | −1.5–0.2 | nC/g | |
| Tribo-charging density variation | Δq | −5.0–0.1 | nC/g | |
| Gas pycnometer | True density | TrD | 1.52–1.58 | g/mL |
| Heckel testing by compaction simulation | Yield pressure at 0.01 mm/s—slow | PyS | 78–229 | MPa |
| Yield pressure at 300 mm/s—fast | PyF | 80–236 | MPa | |
| Strain Rate Sensitivity | SRS | 0–48 | % | |
| Rotating drum | Rheological index | RI | −0.54–0.86 | rpm−1 |
| Grade | Abbreviation | Type | Shape | ×10 (µm) | ×50 (µm) | ×90 (µm) | Span | Bulk Density (g/mL) | Hausner Ratio (-) | ffc @4 kPa (-) |
|---|---|---|---|---|---|---|---|---|---|---|
| Lactopress® anhydrous | LP anh | Anhydrous lactose | Shards | 16.5 | 133 | 323 | 2.30 | 0.69 | 1.28 | 7.5 |
| SuperTab® 21 AN | 21 AN | Anhydrous lactose | Shards | 24.1 | 180 | 387 | 2.02 | 0.72 | 1.27 | 7.7 |
| SuperTab® 22 AN | 22 AN | Anhydrous lactose | Shards | 47.0 | 203 | 359 | 1.54 | 0.68 | 1.17 | 15 |
| SuperTab® 24 AN | 24 AN | (Granulated) anhydrous lactose | Granular | 37.0 | 121 | 298 | 2.15 | 0.54 | 1.25 | 13 |
| Pharmatose® 80 M | 80 M | Lactose monohydrate (sieved) | Tomahawk | 76.6 | 242 | 406 | 1.36 | 0.79 | 1.19 | 13 |
| Pharmatose® 150 M | 150 M | Lactose monohydrate (milled) | Tomahawk/fines | 7.4 | 68.4 | 189 | 2.66 | 0.72 | 1.36 | 3.8 |
| Pharmatose® 200 M | 200 M | Lactose monohydrate (milled) | Tomahawk/fines | 4.4 | 37.7 | 111 | 2.83 | 0.62 | 1.58 | 3.7 |
| Pharmatose® 450 M | 450 M | Lactose monohydrate (milled) | Fines | 3.0 | 18.3 | 49.4 | 2.54 | 0.50 | 1.60 | 2.4 |
| SuperTab® 30 GR | 30 GR | Modified lactose monohydrate | Granular | 38.3 | 126 | 297 | 2.05 | 0.63 | 1.24 | 17 |
| SuperTab® 11 SD | 11 SD | Modified lactose monohydrate | Spherical | 44.0 | 119 | 223 | 1.51 | 0.63 | 1.19 | 17 |
| SuperTab® 14 SD | 14 SD | Modified lactose monohydrate | Spherical | 47.7 | 124 | 227 | 1.44 | 0.62 | 1.15 | 14 |
| SuperTab® 50 ODT | 50 ODT | Modified lactose monohydrate | Spherical | 30.9 | 106 | 199 | 1.58 | 0.71 | 1.17 | 13 |
| Pharmacel® 101 | MCC101 | Microcrystalline cellulose | Spherical/Fibers | 20.0 | 62.2 | 137 | 1.89 | 0.34 | 1.45 | 5.9 |
| Pharmacel® 102 | MCC102 | Microcrystalline cellulose | Spherical/Fibers | 29.9 | 86.9 | 200 | 1.95 | 0.33 | 1.39 | 7.0 |
| Pharmacel® sMCC90 | sMCC90 | Microcrystalline cellulose, co-processed with silicon dioxide | Spherical/Fibers | 29.4 | 102 | 233 | 1.99 | 0.38 | 1.32 | 9.0 |
| Primojel® | PJ | Superdisintegrant | Spherical | 21.1 | 42.3 | 72.6 | 1.22 | 0.79 | 1.21 | 12 |
| Primellose® | PL | Superdisintegrant | Fibers | 24.4 | 54.4 | 114 | 1.65 | 0.55 | 1.35 | 7.4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Janssen, P.H.M.; Depaifve, S.; Neveu, A.; Francqui, F.; Dickhoff, B.H.J. Impact of Powder Properties on the Rheological Behavior of Excipients. Pharmaceutics 2021, 13, 1198. https://doi.org/10.3390/pharmaceutics13081198
Janssen PHM, Depaifve S, Neveu A, Francqui F, Dickhoff BHJ. Impact of Powder Properties on the Rheological Behavior of Excipients. Pharmaceutics. 2021; 13(8):1198. https://doi.org/10.3390/pharmaceutics13081198
Chicago/Turabian StyleJanssen, Pauline H. M., Sébastien Depaifve, Aurélien Neveu, Filip Francqui, and Bastiaan H. J. Dickhoff. 2021. "Impact of Powder Properties on the Rheological Behavior of Excipients" Pharmaceutics 13, no. 8: 1198. https://doi.org/10.3390/pharmaceutics13081198
APA StyleJanssen, P. H. M., Depaifve, S., Neveu, A., Francqui, F., & Dickhoff, B. H. J. (2021). Impact of Powder Properties on the Rheological Behavior of Excipients. Pharmaceutics, 13(8), 1198. https://doi.org/10.3390/pharmaceutics13081198