Numerical Investigation of the Particle Dynamics in a Rotorgranulator Depending on the Properties of the Coating Liquid
Abstract
:1. Introduction
2. Model Description
2.1. CFD Modeling
2.2. DEM Modeling
2.2.1. Contact Forces
2.2.2. Capillary and Viscous Forces
3. Simulation Setup
3.1. Geometry of the Fluidized Bed Rotor Granulator
3.2. Simulation Setup
4. Results
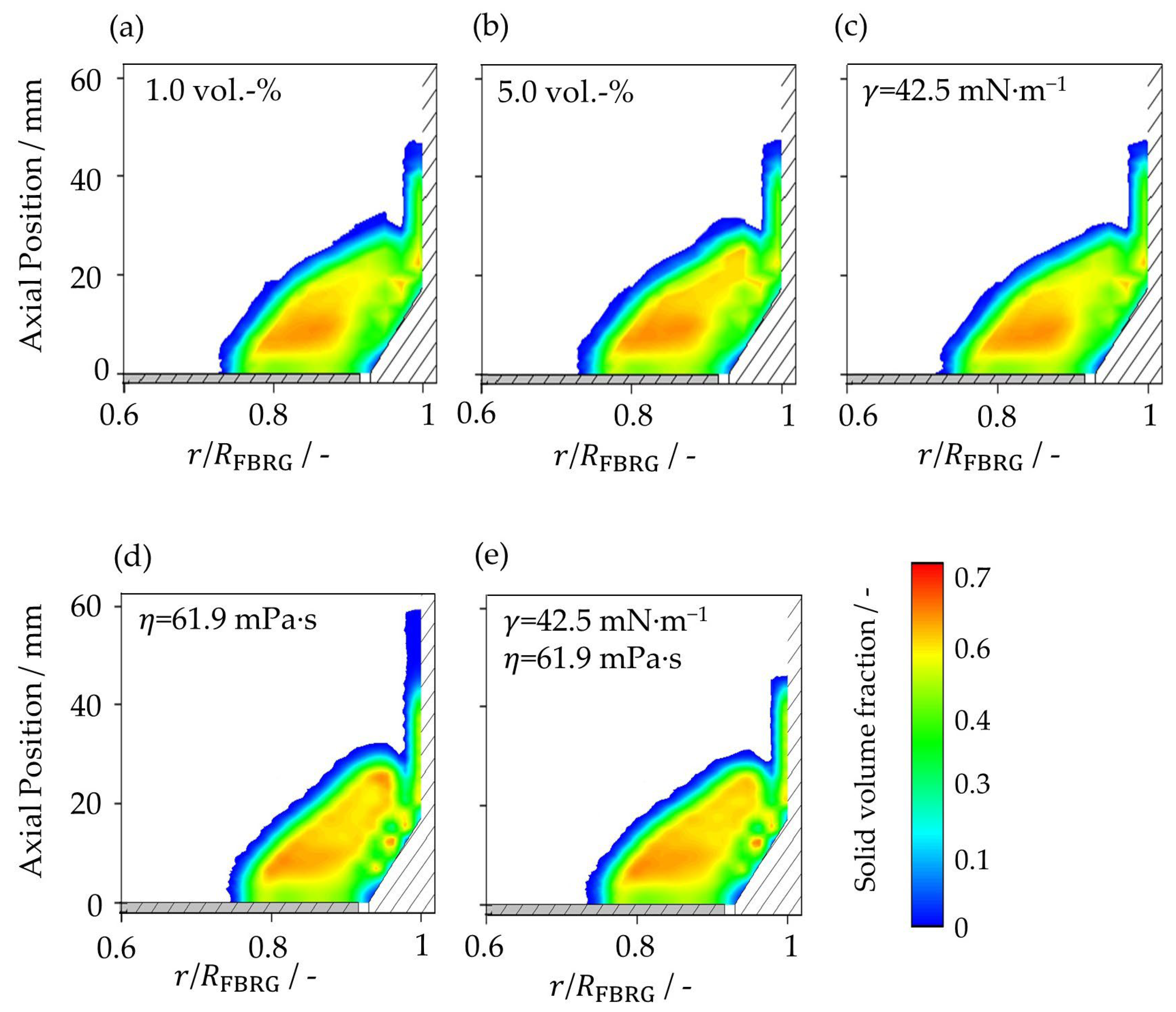
4.1. Particle Solid Volume Fraction
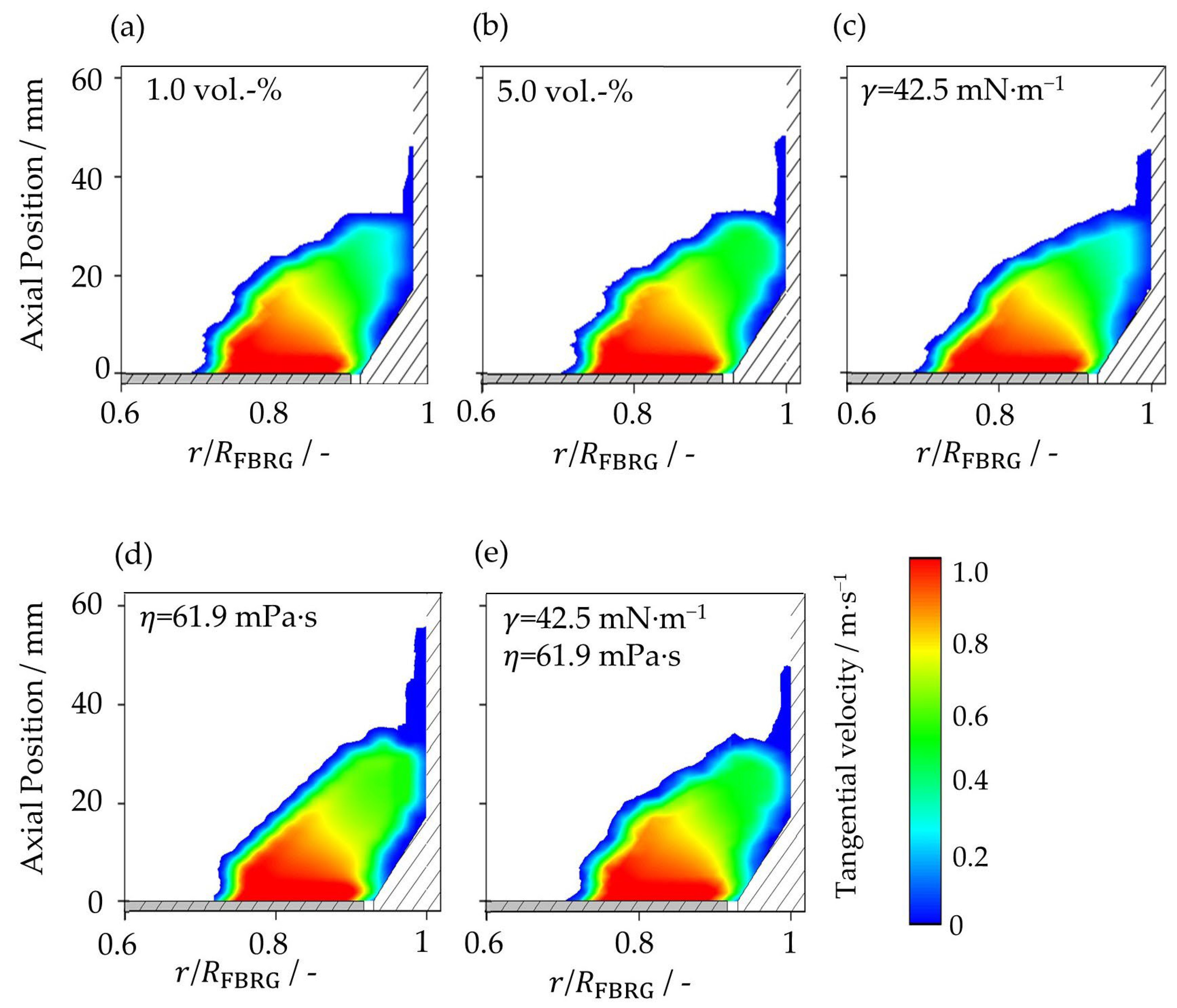
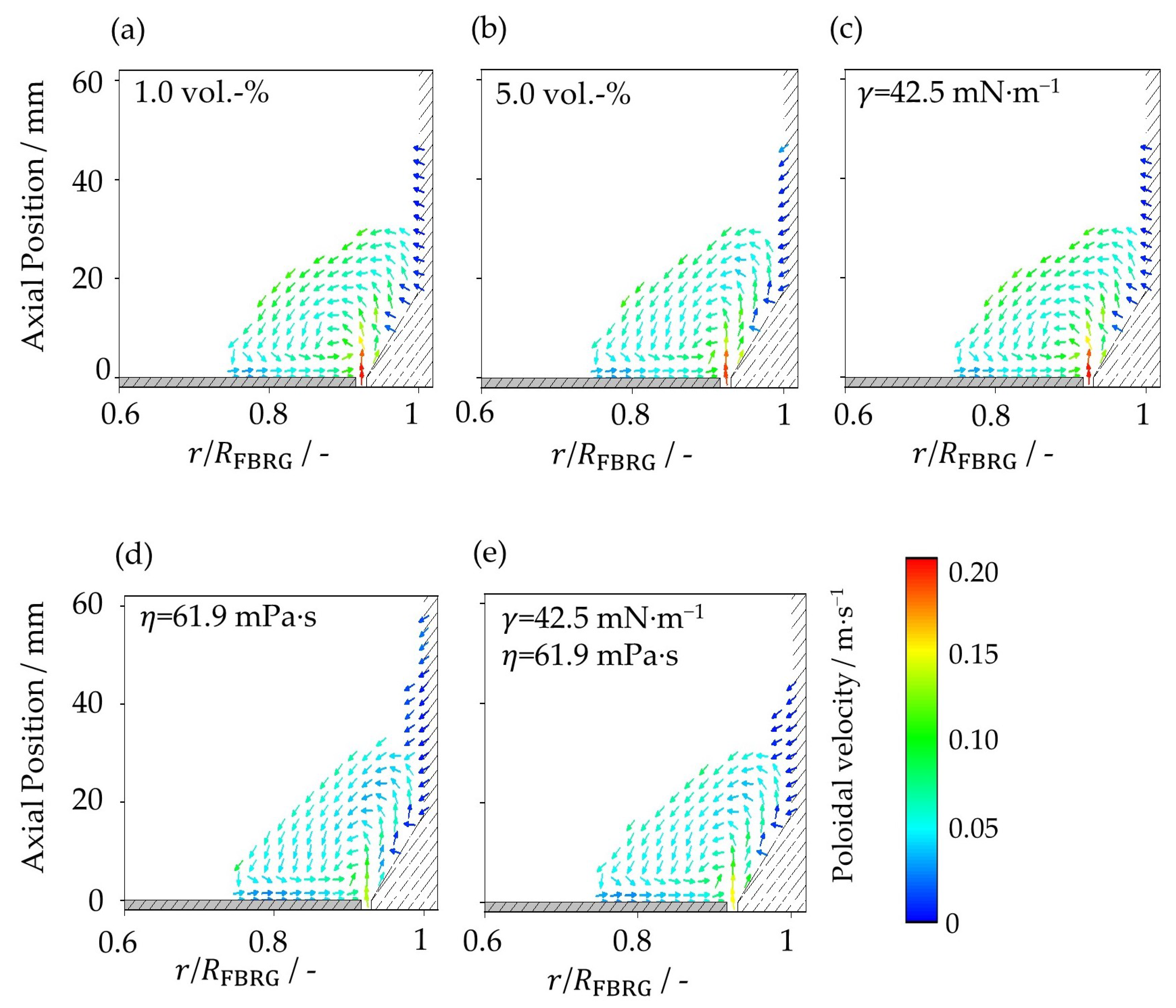
4.2. Particle Velocity
4.3. Rotational Particle Velocity
4.4. Particle Contacts
5. Conclusions
- Increasing the viscosity to the value of a 6 mass-% PHARMACOAT® 606 coating solution results in a denser particle bed. In addition, the particle rotation velocities and the particle movement in the poloidal plane are reduced.
- A reduced liquid loading in the bed as well as a reduced surface tension of the coating liquid lead to lower capillary forces, and thus, to increased particle movement.
- The fraction of high contact velocities increases at low liquid loading or low surface tension, while it decreases at high viscosity. On the other hand, the average contact force increases significantly with high viscosity.
- Based on the proportional dependence of capillary force on surface tension or viscosity force on viscosity, it was found that an increase in viscosity leads to an increase in aggregate size, whereas a reduction in surface tension results in a decrease.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mörl, L.; Heinrich, S.; Peglow, M. Chapter 2 Fluidized bed spray granulation. In Granulation; Elsevier: Amsterdam, The Netherlands, 2007; pp. 21–188. ISBN 9780444518712. [Google Scholar]
- Tsotsas, E.; Mujumdar, A.S. Modern Drying Technology; Wiley: Hoboken, NJ, USA, 2011; Volume 3, ISBN 9783527315581. [Google Scholar]
- Korakianiti, E.S.; Rekkas, D.M.; Dallas, P.P.; Choulis, N.H. Sequential optimization of a pelletization process in a fluid bed rotor granulator. J. Drug Deliv. Sci. Technol. 2004, 14, 207–214. [Google Scholar] [CrossRef]
- Neuwirth, J.; Antonyuk, S.; Heinrich, S.; Jacob, M. CFD–DEM study and direct measurement of the granular flow in a rotor granulator. Chem. Eng. Sci. 2013, 86, 151–163. [Google Scholar] [CrossRef]
- Grohn, P.; Lawall, M.; Oesau, T.; Heinrich, S.; Antonyuk, S. CFD-DEM Simulation of a Coating Process in a Fluidized Bed Rotor Granulator. Processes 2020, 8, 1090. [Google Scholar] [CrossRef]
- Langner, M.; Kitzmann, I.; Ruppert, A.-L.; Wittich, I.; Wolf, B. In-line particle size measurement and process influences on rotary fluidized bed agglomeration. Powder Technol. 2020, 364, 673–679. [Google Scholar] [CrossRef]
- Jacob, M. Chapter 9 Granulation equipment. In Granulation; Elsevier: Amsterdam, The Netherlands, 2007; pp. 417–476. ISBN 9780444518712. [Google Scholar]
- Grohn, P.; Oesau, T.; Heinrich, S.; Antonyuk, S. Investigation of the influence of wetting on the particle dynamics in a fluidized bed rotor granulator by MPT measurements and CFD-DEM simulations. Powder Technol. 2022, 408, 117736. [Google Scholar] [CrossRef]
- Vuppala, M.K.; Parikh, D.M.; Bhagat, H.R. Application of Powder-Layering Technology and Film Coating for Manufacture of Sustained-Release Pellets Using a Rotary Fluid Bed Processor. Drug Dev. Ind. Pharm. 1997, 23, 687–694. [Google Scholar] [CrossRef]
- Kristensen, J.; Schaefer, T.; Kleinebudde, P. Direct pelletization in a rotary processor controlled by torque measurements. II: Effects of changes in the content of microcrystalline cellulose. AAPS PharmSci 2000, 2, 45. [Google Scholar] [CrossRef] [Green Version]
- Gu, L.; Liew, C.V.; Heng, P.W.S. Wet spheronization by rotary processing—A multistage single-pot process for producing spheroids. Drug Dev. Ind. Pharm. 2004, 30, 111–123. [Google Scholar] [CrossRef]
- Deen, N.G.; van Sint Annaland, M.; van der Hoef, M.A.; Kuipers, J.A.M. Review of discrete particle modeling of fluidized beds. Chem. Eng. Sci. 2007, 62, 28–44. [Google Scholar] [CrossRef]
- Fries, L.; Dosta, M.; Antonyuk, S.; Heinrich, S.; Palzer, S. Moisture Distribution in Fluidized Beds with Liquid Injection. Chem. Eng. Technol. 2011, 34, 1076–1084. [Google Scholar] [CrossRef]
- Salikov, V.; Heinrich, S.; Antonyuk, S.; Sutkar, V.S.; Deen, N.G.; Kuipers, J.A.M. Investigations on the spouting stability in a prismatic spouted bed and apparatus optimization. Adv. Powder Technol. 2015, 26, 718–733. [Google Scholar] [CrossRef]
- Breuninger, P.; Weis, D.; Behrendt, I.; Grohn, P.; Krull, F.; Antonyuk, S. CFD–DEM simulation of fine particles in a spouted bed apparatus with a Wurster tube. Particuology 2019, 42, 114–125. [Google Scholar] [CrossRef]
- Sutkar, V.S.; Deen, N.G.; Salikov, V.; Antonyuk, S.; Heinrich, S.; Kuipers, J.A.M. Experimental and numerical investigations of a pseudo-2D spout fluidized bed with draft plates. Powder Technol. 2015, 270, 537–547. [Google Scholar] [CrossRef] [Green Version]
- Heinrich, S.; Dosta, M.; Antonyuk, S. Multiscale Analysis of a Coating Process in a Wurster Fluidized Bed Apparatus. In Mesoscale Modeling in Chemical Engineering Part I; Elsevier: Amsterdam, The Netherlands, 2015; pp. 83–135. ISBN 9780128012475. [Google Scholar]
- Muguruma, Y.; Tanaka, T.; Tsuji, Y. Numerical simulation of particulate flow with liquid bridge between particles (simulation of centrifugal tumbling granulator). Powder Technol. 2000, 109, 49–57. [Google Scholar] [CrossRef]
- Weis, D.; Evers, M.; Thommes, M.; Antonyuk, S. DEM simulation of the mixing behavior in a spheronization process. Chem. Eng. Sci. 2018, 192, 803–815. [Google Scholar] [CrossRef]
- Weis, D.; Grohn, P.; Evers, M.; Thommes, M.; García, E.; Antonyuk, S. Implementation of formation mechanisms in DEM simulation of the spheronization process of pharmaceutical pellets. Powder Technol. 2021, 378, 667–679. [Google Scholar] [CrossRef]
- Grohn, P.; Schaedler, L.; Atxutegi, A.; Heinrich, S.; Antonyuk, S. CFD-DEM Simulation of Superquadric Cylindrical Particles in a Spouted Bed and a Rotor Granulator. Chem. Ing. Tech. 2023, 95, 244–255. [Google Scholar] [CrossRef]
- Neuwirth, J. Charakterisierung und Diskrete-Partikel-Modellierung des Strömungs- und Dispersionsverhaltens im Rotorgranulator, 1st ed.; Cuvillier Verlag: Göttingen, Germany, 2017; ISBN 9783736994768. [Google Scholar]
- Israelachvili, J.N. Intermolecular and Surface Forces; Elsevier: Amsterdam, The Netherlands, 2011; ISBN 9780123919274. [Google Scholar]
- Lian, G.; Thornton, C.; Adams, M.J. Discrete particle simulation of agglomerate impact coalescence. Chem. Eng. Sci. 1998, 53, 3381–3391. [Google Scholar] [CrossRef]
- Popov, V.L. (Ed.) Contact Mechanics and Friction: Physical Principles and Applications, 1st ed.; Springer: Berlin, Germany, 2010; ISBN 978-3-642-10802-0. [Google Scholar]
- Grohn, P.; Oesau, T.; Heinrich, S.; Antonyuk, S. Investigation of the influence of impact velocity and liquid bridge volume on the maximum liquid bridge length. Adv. Powder Technol. 2022, 33, 103630. [Google Scholar] [CrossRef]
- Moukalled, F. The Finite Volume Method in Computational Fluid Dynamics: An Advanced Introduction with OpenFOAM® and Matlab, 1st ed.; Springer: Cham, Switzerland, 2016; ISBN 9783319168746. [Google Scholar]
- Hoomans, B.P.B.; Kuipers, J.A.M.; Briels, W.J.; van Swaaij, W.P.M. Discrete particle simulation of bubble and slug formation in a two-dimensional gas-fluidised bed: A hard-sphere approach. Chem. Eng. Sci. 1996, 51, 99–118. [Google Scholar] [CrossRef]
- Goniva, C.; Kloss, C.; Deen, N.G.; Kuipers, J.A.M.; Pirker, S. Influence of rolling friction on single spout fluidized bed simulation. Particuology 2012, 10, 582–591. [Google Scholar] [CrossRef]
- Kanitz, M.; Grabe, J. The influence of the void fraction on the particle migration: A coupled computational fluid dynamics–discrete element method study about drag force correlations. Int. J. Numer. Anal. Methods Geomech. 2021, 45, 45–63. [Google Scholar] [CrossRef]
- Zhao, J.; Shan, T. Coupled CFD–DEM simulation of fluid–particle interaction in geomechanics. Powder Technol. 2013, 239, 248–258. [Google Scholar] [CrossRef]
- Di Felice, R. The voidage function for fluid-particle interaction systems. Int. J. Multiph. Flow 1994, 20, 153–159. [Google Scholar] [CrossRef]
- Zhou, Z.Y.; Kuang, S.B.; Chu, K.W.; Yu, A.B. Discrete particle simulation of particle–fluid flow: Model formulations and their applicability. J. Fluid Mech. 2010, 661, 482–510. [Google Scholar] [CrossRef]
- Hesse, R.; Krull, F.; Antonyuk, S. Experimentally calibrated CFD-DEM study of air impairment during powder discharge for varying hopper configurations. Powder Technol. 2020, 372, 404–419. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D.L. A discrete numerical model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Crowe, C.T.; Schwarzkopf, J.D.; Sommerfeld, M.; Tsuji, Y. Multiphase Flows with Droplets and Particles; CRC Press: Boca Raton, FL, USA, 2011; ISBN 9780429106392. [Google Scholar]
- Salikov, V.; Antonyuk, S.; Heinrich, S.; Sutkar, V.S.; Deen, N.G.; Kuipers, J.A.M. Characterization and CFD-DEM modelling of a prismatic spouted bed. Powder Technol. 2015, 270, 622–636. [Google Scholar] [CrossRef] [Green Version]
- Lian, G.; Thornton, C.; Adams, M.J. A Theoretical Study of the Liquid Bridge Forces between Two Rigid Spherical Bodies. J. Colloid Interface Sci. 1993, 161, 138–147. [Google Scholar] [CrossRef]
- Mikami, T.; Kamiya, H.; Horio, M. Numerical simulation of cohesive powder behavior in a fluidized bed. Chem. Eng. Sci. 1998, 53, 1927–1940. [Google Scholar] [CrossRef]
- Rabinovich, Y.I.; Esayanur, M.S.; Moudgil, B.M. Capillary forces between two spheres with a fixed volume liquid bridge: Theory and experiment. Langmuir 2005, 21, 10992–10997. [Google Scholar] [CrossRef] [PubMed]
- Shi, D.; McCarthy, J.J. Numerical simulation of liquid transfer between particles. Powder Technol. 2008, 184, 64–75. [Google Scholar] [CrossRef]
- Pitois, O.; Moucheront, P.; Chateau, X. Rupture energy of a pendular liquid bridge. Eur. Phys. J. B 2001, 23, 79–86. [Google Scholar] [CrossRef]
- Antonyuk, S.; Heinrich, S.; Deen, N.; Kuipers, H. Influence of liquid layers on energy absorption during particle impact. Particuology 2009, 7, 245–259. [Google Scholar] [CrossRef]
- Gollwitzer, F.; Rehberg, I.; Kruelle, C.A.; Huang, K. Coefficient of restitution for wet particles. Phys. Rev. E Stat. Nonlin. Soft Matter Phys. 2012, 86, 11303. [Google Scholar] [CrossRef] [Green Version]
- Schmelzle, S.; Asylbekov, E.; Radel, B.; Nirschl, H. Modelling of partially wet particles in DEM simulations of a solid mixing process. Powder Technol. 2018, 338, 354–364. [Google Scholar] [CrossRef] [Green Version]
- Reynolds, O. IV. On the theory of lubrication and its application to Mr. Beauchamp tower’s experiments, including an experimental determination of the viscosity of olive oil. Phil. Trans. R. Soc. 1886, 177, 157–234. [Google Scholar] [CrossRef]
- Adams, M.J.; Perchard, V. The cohesive forces between particles with interstitial liquid. In Institution of Chemical Engineering Symposium; Institution of Chemical Engineers: Rugby, UK, 1985; pp. 147–160. [Google Scholar]
- Nase, S.T.; Vargas, W.L.; Abatan, A.A.; McCarthy, J.J. Discrete characterization tools for cohesive granular material. Powder Technol. 2001, 116, 214–223. [Google Scholar] [CrossRef]
- Tang, T.; He, Y.; Tai, T.; Wen, D. DEM numerical investigation of wet particle flow behaviors in multiple-spout fluidized beds. Chem. Eng. Sci. 2017, 172, 79–99. [Google Scholar] [CrossRef] [Green Version]
- Anand, A.; Curtis, J.S.; Wassgren, C.R.; Hancock, B.C.; Ketterhagen, W.R. Predicting discharge dynamics of wet cohesive particles from a rectangular hopper using the discrete element method (DEM). Chem. Eng. Sci. 2009, 64, 5268–5275. [Google Scholar] [CrossRef]
- Washino, K.; Miyazaki, K.; Tsuji, T.; Tanaka, T. A new contact liquid dispersion model for discrete particle simulation. Chem. Eng. Res. Des. 2016, 110, 123–130. [Google Scholar] [CrossRef]
- Weller, H.G.; Tabor, G.; Jasak, H.; Fureby, C. A tensorial approach to computational continuum mechanics using object-oriented techniques. Comput. Phys. 1998, 12, 620. [Google Scholar] [CrossRef]
- Issa, R.I. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Kloss, C.; Goniva, C.; Hager, A.; Amberger, S.; Pirker, S. Models, algorithms and validation for opensource DEM and CFD-DEM. Prog. Comput. Fluid Dyn. 2012, 12, 140–152. [Google Scholar] [CrossRef]
- Oesau, T.; Grohn, P.; Pietsch-Braune, S.; Antonyuk, S.; Heinrich, S. Novel approach for measurement of restitution coefficient by magnetic particle tracking. Adv. Powder Technol. 2022, 33, 103362. [Google Scholar] [CrossRef]
- Weis, D. Beschreibung des Sphäronisationsprozesses von Pharmazeutischen Pellets Mittels Numerischer Methoden. Ph.D. Thesis, TU Kaiserslautern, Kaiserslautern, Germany, 2021. [Google Scholar]






| Parameters of the Gas | Unit | Value |
|---|---|---|
| Fluid | - | air |
| Fluid temperature | °C | 20 |
| Fluid kinematic viscosity | kg∙m−1∙s−1 | |
| Fluid density | kg∙m−3 | 1.2 |
| Inlet gap velocity | m∙s−1 | 32 |
| Parameter | Unit | Value/Variation Range |
|---|---|---|
| Particle | - | ceramic cores coated with PVB |
| Particle bed mass | kg | 1.0 |
| Particle density | kg∙m−3 | 3485 |
| Particle diameter | mm | 2.8 |
| Young’s modulus particle | GPa | 1.69 |
| Young’s modulus walls | GPa | 3.0 |
| Poisson ratio | - | 0.3 |
| Restitution coefficient | - | 0.89 |
| Static friction particle–particle | - | 0.23 |
| Static friction particle–wall | - | 0.46 |
| Rolling friction particle–particle | - | 0.075 |
| Rolling friction particle–wall | - | 0.065 |
| Liquid volume on particle | vol-% | 1–5 |
| Surface tension | mN∙m−1 | 42.5–72.8 |
| Liquid dynamic viscosity | Pa∙s | 0.001–0.619 |
| Liquid density | kg∙m−3 | 1000 |
| Contact angle particle-particle | ° | 25 |
| Contact angle particle–wall/rotor | ° | 45 |
| Case | Liquid Properties |
|---|---|
| (a) 1.0 vol.-% | 1 vol.-%, = 72.8 mN∙m−1, = 1 mPas |
| (b) 5.0 vol.-% | 5 vol.-%, = 72.8 mN∙m−1, = 1 mPas |
| (c) = 42.5 mN∙m−1 | 5 vol.-%, = 42.5 mN∙m−1, = 1 mPas |
| (d) = 61.9 mPa∙s | 5 vol.-%, = 72.8 mN∙m−1, = 61.9 mPas |
| (e) = 42.5 mN∙m−1, = 61.9 mPa∙s | 5 vol.-%, = 42.5 mN∙m−1, = 61.9 mPas |
| Case | PRN in 1/s | RMP in % |
|---|---|---|
| (a) 1.0 vol.-% | 0.73 | 6.86 |
| (b) 5.0 vol.-% | 0.76 | 6.01 |
| (c) = 42.5 mN∙m−1 | 0.73 | 7.21 |
| (d) = 61.9 mPa∙s | 0.87 | 4.58 |
| (e) = 42.5 mN∙m−1, = 61.9 mPa∙s | 0.87 | 5.14 |
| Case | Contact Rates/- | Average Numbers of Contact Partners/- |
|---|---|---|
| (a) 1.0 vol.-% | 2.14 | 3.23 |
| (b) 5.0 vol.-% | 2.96 | 3.45 |
| (c) = 42.5 mN∙m−1 | 2.21 | 3.34 |
| (d) = 61.9 mPa∙s | 2.56 | 3.76 |
| (e) = 42.5 mN∙m−1, = 61.9 mPa∙s | 2.24 | 3.43 |
| Contact Partners | Contact Velocity/m∙s−1 | Normal Contact Force/mN | Tangential Contact Force/mN |
|---|---|---|---|
| (a) 1.0 vol.-% | |||
| P–P | 0.040 | 1.40 | 0.25 |
| P–W | 0.046 | 1.52 | 0.62 |
| P–R | 0.208 | 4.01 | 1.36 |
| (b) 5.0 vol.-% | |||
| P–P | 0.041 | 1.47 | 0.26 |
| P–W | 0.066 | 1.58 | 0.63 |
| P–R | 0.217 | 3.89 | 1.34 |
| (c) 𝛾 = 42.5 mN∙m−1 | |||
| P–P | 0.045 | 1.24 | 0.22 |
| P–W | 0.079 | 1.18 | 0.46 |
| P–R | 0.230 | 3.29 | 1.16 |
| (d) 𝜂 = 61.9 mPa∙s | |||
| P–P | 0.033 | 1.68 | 0.46 |
| P–W | 0.039 | 1.32 | 0.77 |
| P–R | 0.205 | 3.59 | 2.88 |
| (e) 𝛾 = 42.5 mN∙m−1, 𝜂 = 61.9 mPa∙s | |||
| P–P | 0.037 | 1.53 | 0.43 |
| P–W | 0.069 | 1.12 | 0.82 |
| P–R | 0.222 | 3.27 | 3.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grohn, P.; Heinrich, S.; Antonyuk, S. Numerical Investigation of the Particle Dynamics in a Rotorgranulator Depending on the Properties of the Coating Liquid. Pharmaceutics 2023, 15, 469. https://doi.org/10.3390/pharmaceutics15020469
Grohn P, Heinrich S, Antonyuk S. Numerical Investigation of the Particle Dynamics in a Rotorgranulator Depending on the Properties of the Coating Liquid. Pharmaceutics. 2023; 15(2):469. https://doi.org/10.3390/pharmaceutics15020469
Chicago/Turabian StyleGrohn, Philipp, Stefan Heinrich, and Sergiy Antonyuk. 2023. "Numerical Investigation of the Particle Dynamics in a Rotorgranulator Depending on the Properties of the Coating Liquid" Pharmaceutics 15, no. 2: 469. https://doi.org/10.3390/pharmaceutics15020469
APA StyleGrohn, P., Heinrich, S., & Antonyuk, S. (2023). Numerical Investigation of the Particle Dynamics in a Rotorgranulator Depending on the Properties of the Coating Liquid. Pharmaceutics, 15(2), 469. https://doi.org/10.3390/pharmaceutics15020469








