Temperature Dependence of the Complexation Mechanism of Celecoxib and Hydroxyl-β-cyclodextrin in Aqueous Solution
Abstract
:1. Introduction
2. Experimental
2.1. Materials
2.2. Phase Solubility Studies
2.3. Computational Method
3. Results and Discussion
3.1. Phase Solubility

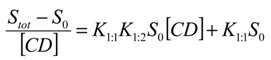
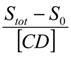 versus [CD] should yield a linear curve with an intercept of K1:1S0 and a slope of K1:1K1:2S0. Through this approach, the K1:1 and K1:2 for 333 °K samples were estimated to be approximately 6.5 and 12,370.0, respectively, indicating that the 1:2 complex was much more favored for samples that were heated to 333 °K.
versus [CD] should yield a linear curve with an intercept of K1:1S0 and a slope of K1:1K1:2S0. Through this approach, the K1:1 and K1:2 for 333 °K samples were estimated to be approximately 6.5 and 12,370.0, respectively, indicating that the 1:2 complex was much more favored for samples that were heated to 333 °K.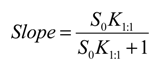


3.2. MD Simulations
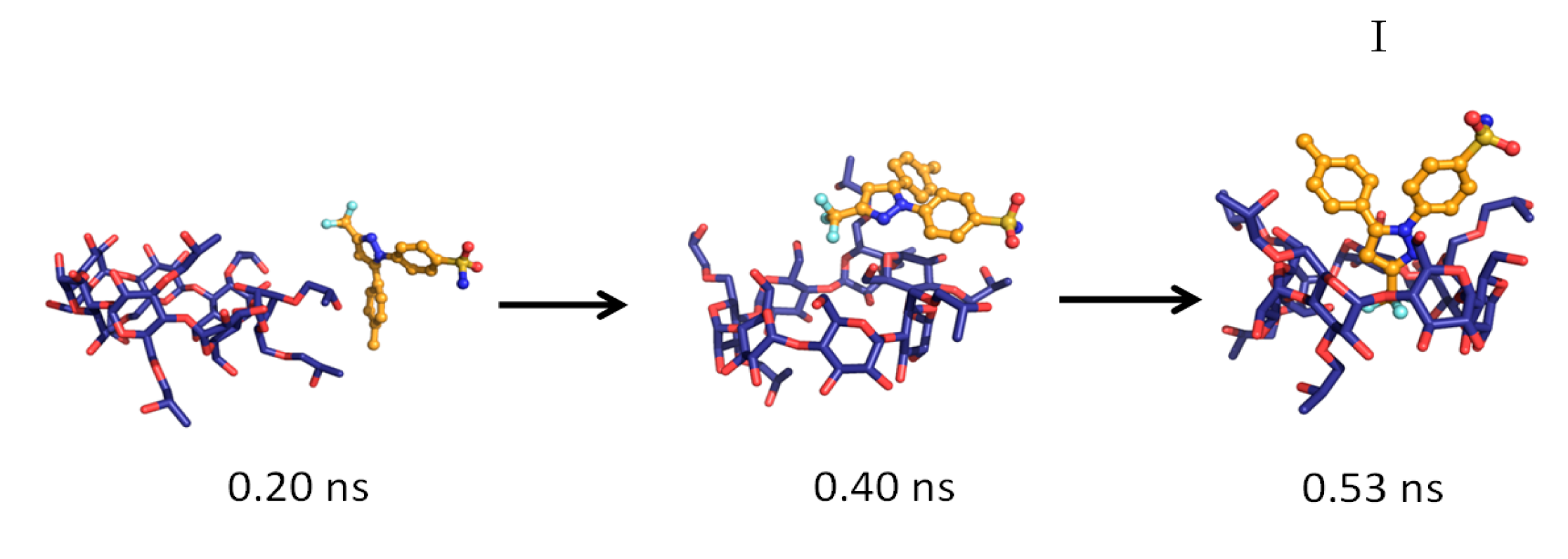


| Temperature | System a | Simulation Time (ns) | Complexation Structure Observed b | Time When the Final Structure Formed (ns) |
|---|---|---|---|---|
| 298 °K | 1 | 3.0 | I | 0.53 |
| 2 | 4.8 | IV | 1.10 | |
| 3 | 9.0 | I | 1.30 | |
| 333 °K | 1 | 8.0 | III (via II) | 0.48 |
| 2 | 5.0 | I | 1.50 | |
| 3 | 9.0 | IV | 1.60 |
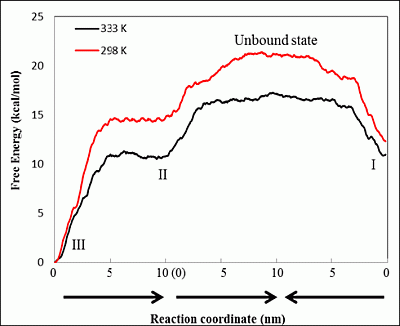
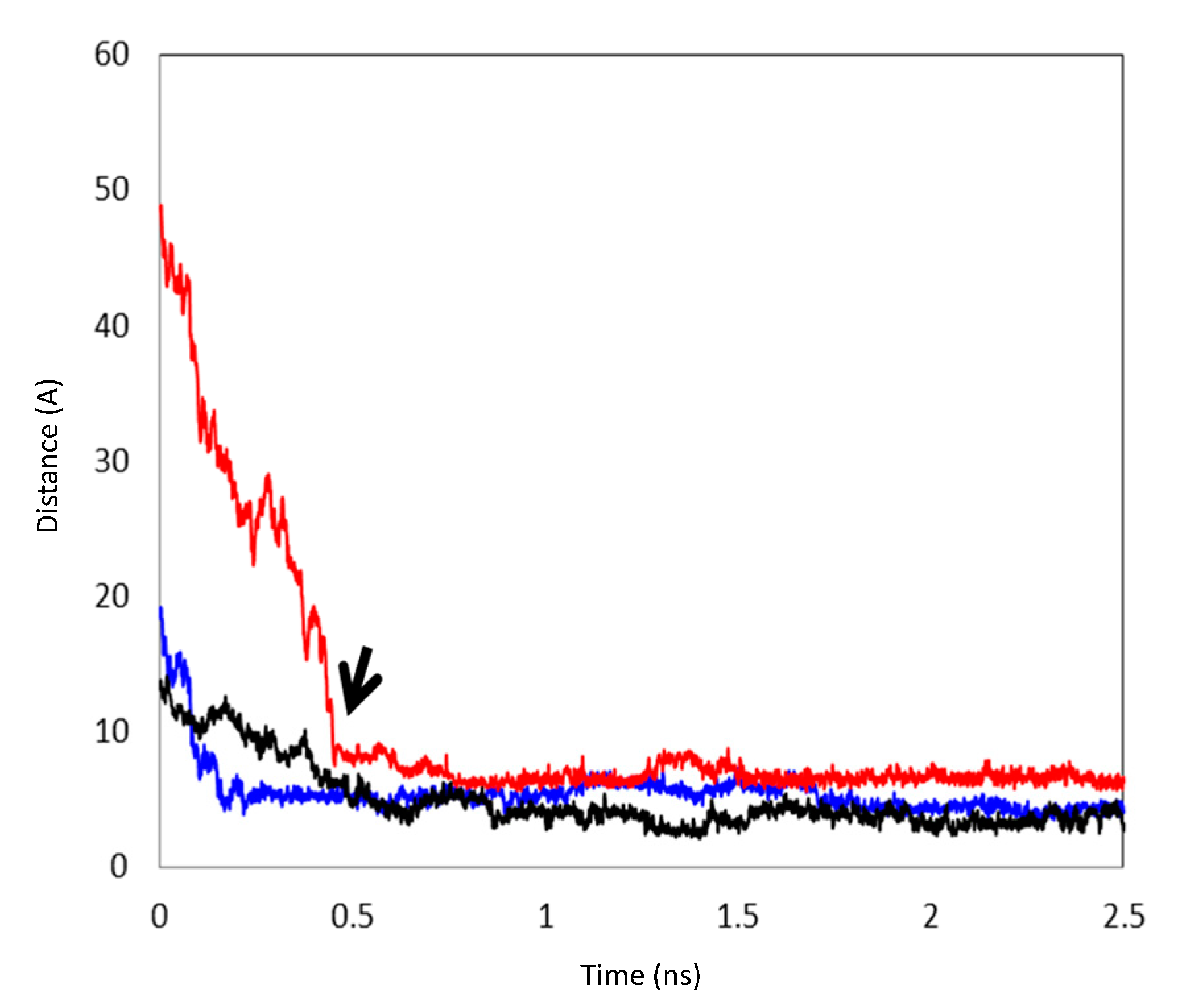
3.3. Steered MD Simulations

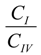 is the ratio between the equilibrium concentrations of I and IV, CI and CIV, respectively, which can also be considered as the probability ratio between the formation of these two complexes,
is the ratio between the equilibrium concentrations of I and IV, CI and CIV, respectively, which can also be considered as the probability ratio between the formation of these two complexes, 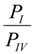 , given that the system started from the unbound state. Using Equation (6), the probability ratio between the formation of Complexes I and IV at 333 °K was estimated to be
, given that the system started from the unbound state. Using Equation (6), the probability ratio between the formation of Complexes I and IV at 333 °K was estimated to be 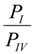 ≈ 269. In other words, the association complex (Complex IV) was less stable relative to Complex I and other inclusion complexation states (which presented still lower free energies). Therefore, it should not be a significant form of complexation in the system. The appearance of the association complex in the MD simulations was likely due to insufficient equilibration time of the MD runs. Because of the finding at 333 °K, we did not perform steered MD simulations on Complex IV at 298 °K assuming a similar instability of Complex IV at 298 °K.
≈ 269. In other words, the association complex (Complex IV) was less stable relative to Complex I and other inclusion complexation states (which presented still lower free energies). Therefore, it should not be a significant form of complexation in the system. The appearance of the association complex in the MD simulations was likely due to insufficient equilibration time of the MD runs. Because of the finding at 333 °K, we did not perform steered MD simulations on Complex IV at 298 °K assuming a similar instability of Complex IV at 298 °K.| kcal/mol | ∆GUnbound→I | ∆GUnbound→II | ∆GUnbound→III | ∆GUnbound→IV | ∆GII→I | |
|---|---|---|---|---|---|---|
| 298 °K | Average | −8.827 | −6.653 | −21.166 | – | −2.174 |
| SD | 0.062 | 0.115 | 0.124 | – | 0.054 | |
| 333 °K | Average | −6.249 | −6.473 | −17.164 | −2.548 | 0.223 |
| SD | 0.051 | 0.051 | 0.074 | 0.038 | 0.074 | |
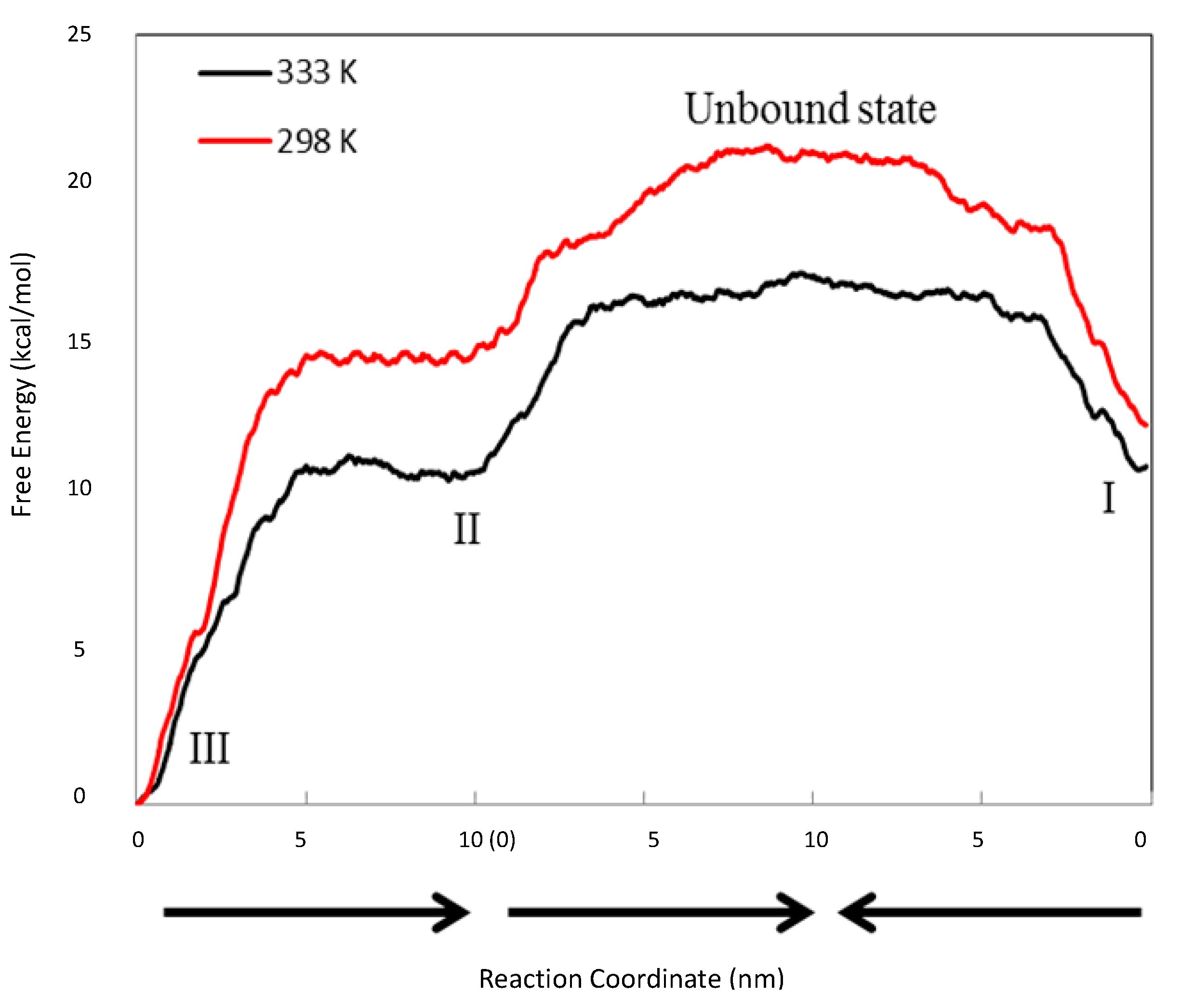
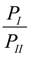 ≈ 39.4 at 298 K and
≈ 39.4 at 298 K and 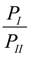 ≈ 0.71 at 333 °K, indicating that at 298 °K, the unbound state predominantly turned into Complex I initially, while at 333 °K, the chances to form Complexes I and II were almost even. Note that for a system starting from the unbound state, the first step is to form 1:1 complexes, i.e., Complexes I and II in this case, while the formation of a 1:2 complex needs to go through a 1:1 complex. Therefore, the probability ratio between Complexes I and II determines which 1:1 complex is likely to appear initially from the unbound state, irrespective of whether it is a metastable state.
≈ 0.71 at 333 °K, indicating that at 298 °K, the unbound state predominantly turned into Complex I initially, while at 333 °K, the chances to form Complexes I and II were almost even. Note that for a system starting from the unbound state, the first step is to form 1:1 complexes, i.e., Complexes I and II in this case, while the formation of a 1:2 complex needs to go through a 1:1 complex. Therefore, the probability ratio between Complexes I and II determines which 1:1 complex is likely to appear initially from the unbound state, irrespective of whether it is a metastable state.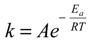 , where k is the reaction rate, A is the frequency factor and R is the gas constant. Assuming A is temperature independent, we may use the Arrhenius equation to estimate the reaction rate change upon temperature elevation (Equation (7)):
, where k is the reaction rate, A is the frequency factor and R is the gas constant. Assuming A is temperature independent, we may use the Arrhenius equation to estimate the reaction rate change upon temperature elevation (Equation (7)):
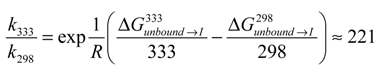
4. Conclusions
Author Contributions
Conflicts of Interest
References
- Stella, V.J.; Rajiwski, R.A. Cyclodextrins: Their future in drug formulation and delivery. Pharm. Res. 1997, 14, 556–567. [Google Scholar] [CrossRef]
- Loftson, T.; Brewster, M.E. Pharmaceutical applications of cyclodextrins. 1. Drug solubilization and stabilization. J. Pharm. Sci. 1996, 85, 1017–1025. [Google Scholar] [CrossRef]
- Uekema, K.; Hirayama, F.; Irie, T. Cyclodextrin drug carrier systems. Chem. Rev. 1998, 98, 2045–2076. [Google Scholar] [CrossRef]
- Connors, K.A. The stability of cyclodextrin complexes in solution. Chem. Rev. 1997, 97, 1325–1357. [Google Scholar] [CrossRef]
- Loftsson, T.; Magnusdottir, A.; Masson, M.; Sigurjonsdottir, H.F. Self-association and cyclodextrin solubilization of drugs. J. Pharm. Sci. 2002, 91, 2307–2316. [Google Scholar] [CrossRef]
- Cano, J.; Rodriguez, A.; Aicart, E.; Junquera, E. Temperature effect on the complex formation between tricyclic antidepressant drugs (amitriptyline or imipramine) and hydroxypropyl-β-cyclodextrin in water. J. Incl. Phenom. Macrocycl. Chem. 2007, 59, 279–285. [Google Scholar] [CrossRef]
- Junquera, E.; Romero, J.C.; Aicart, E. Behavior of tricyclic antidepressant in aqueous solution: Self-aggregation and association with β-cyclodextrin. Langmuir 2001, 17, 1826–1832. [Google Scholar] [CrossRef]
- De Sousa, F.B.; Denadai, A.M.L.; Iula, L.S.; Nascimento, C.S.; Fernandes, N.S.G.; Lima, A.C.; De Almeida, W.B.; Sinisterra, R.D. Supramolecular self-assembly of cyclodextrin and higher water soluble guest: Thermodynamics and topological studies. J. Am. Chem. Soc. 2008, 130, 8426–8436. [Google Scholar]
- Sun, T.; Shao, X.; Cai, W. Self-assembly behavior of β-cyclodextrin and imipramine. A free energy perturbation study. Chem. Phys. 2010, 371, 84–90. [Google Scholar]
- Ventura, C.A.; Giannone, I.; Paolino, D.; Pistarà, V.; Corsaro, A.; Puglisi, G. Preparation of celecoxib-dimethyl-β-cyclodextrin inclusion complex: Characterization and in vitro permeation study. Eur. J. Med. Chem. 2005, 40, 624–631. [Google Scholar] [CrossRef]
- Higuchi, T.; Connors, K.A. Phase-solubility techniques. Adv. Anal. Chem. Instrum. 1965, 4, 117–212. [Google Scholar]
- Materials Studio, Accelrys©. Available online: http://accelrys.com/ (accessed on June 2013).
- Sun, H. COMPASS: An ab initio force-field optimized for condensed-phase applicationsoverview with details on alkane and benzene compounds. J. Phys. Chem. B 1998, 102, 7338–7364. [Google Scholar]
- Izrailev, S.; Stepaniants, S.; Schulten, K. Applications of steered molecular dynamics to protein-ligand/membrane binding. Biophys. J. 1998, 74, A177. [Google Scholar]
- Case, D.A.; Cheatham, T.E.; Darden, T., III; Gohlke, H.; Luo, R.; Merz, K.M.; Onufriev, A., Jr.; Simmerling, C.; Wang, B.; Woods, R. The Amber biomolecular simulation programs. J. Comput. Chem. 2005, 26, 1668–1688. [Google Scholar]
- Wang, J.; Wolf, R.M.; Caldwell, J.W.; Kollman, P.A.; Case, D.A. Development and testing of a general amber force field. J. Comput. Chem. 2004, 25, 1157–1174. [Google Scholar] [CrossRef]
- Jorgensen, W.L.; Jenson, C. Temperature dependence of TIP3P, SPC, and TIP4P water from NPT monte carlo simulations: Seeking temperatures of maximum density. J. Comput. Chem. 1998, 19, 1179–1186. [Google Scholar] [CrossRef]
- Darden, T.A.; York, D.; Pedersen, L. Particle mesh ewald: An N log(N) method for Ewald sums in large systems. J. Chem. Phys. 1993, 98, 10089–10092. [Google Scholar]
- Jarzynski, C. Equilibrium free-energy differences from nonequilibrium measurements: A master-equation approach. Phys. Rev. E 1997, 56, 5018–5035. [Google Scholar] [CrossRef]
- Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 1997, 78, 2690–2693. [Google Scholar]
- Hummer, G.; Szabo, A. Free energy reconstruction from nonequilibrium single-molecule pulling experiments. Proc. Natl. Acad. Sci. USA 2001, 98, 3658–3661. [Google Scholar] [CrossRef]
- Jensen, M.O.; Park, S.; Tajkhorshid, E.; Schulten, K. Energetics of glycerol conduction through aquaglyceroporin GlpF. Proc. Natl. Acad. Sci. USA 2002, 99, 6731–6736. [Google Scholar] [CrossRef]
- Park, S.; Schulten, K. Calculating potentials of mean force from steered molecular dynamics simulations. J. Chem. Phys. 2004, 120, 5946–5961. [Google Scholar]
- Higuchi, T.; Kristiansen, H. Binding specificity between small organic solutes in aqueous solution: Classification of some solutes into two groups according to binding tendencies. J. Pharm. Sci. 1970, 59, 1601–1608. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Chiang, P.-C.; Shi, Y.; Cui, Y. Temperature Dependence of the Complexation Mechanism of Celecoxib and Hydroxyl-β-cyclodextrin in Aqueous Solution. Pharmaceutics 2014, 6, 467-480. https://doi.org/10.3390/pharmaceutics6030467
Chiang P-C, Shi Y, Cui Y. Temperature Dependence of the Complexation Mechanism of Celecoxib and Hydroxyl-β-cyclodextrin in Aqueous Solution. Pharmaceutics. 2014; 6(3):467-480. https://doi.org/10.3390/pharmaceutics6030467
Chicago/Turabian StyleChiang, Po-Chiang, Yue Shi, and Yong Cui. 2014. "Temperature Dependence of the Complexation Mechanism of Celecoxib and Hydroxyl-β-cyclodextrin in Aqueous Solution" Pharmaceutics 6, no. 3: 467-480. https://doi.org/10.3390/pharmaceutics6030467
APA StyleChiang, P.-C., Shi, Y., & Cui, Y. (2014). Temperature Dependence of the Complexation Mechanism of Celecoxib and Hydroxyl-β-cyclodextrin in Aqueous Solution. Pharmaceutics, 6(3), 467-480. https://doi.org/10.3390/pharmaceutics6030467