A Comparative Study of Coupled Preferential Crystallizers for the Efficient Resolution of Conglomerate-Forming Enantiomers
Abstract
:1. Introduction
2. Coupled Preferential Crystallizer Configurations
2.1. CPC-MSMPR Configuration
2.2. CPC Configuration
2.3. CPC-D Configuration
3. Model of the Coupled Crystallization Process
4. Results and Discussion
4.1. DL-threonine/H2O as Model System
4.2. Liquid and Solid Phase Mass Evolution
4.3. Effect of Feed Flow Rate on CPC-MSMPR Productivity and Yield
4.4. Effect of Liquid Phase Exchange Rate on CPC-MSMPR Productivity and Yield
4.5. Effect of Seed Mass on CPC-MSMPR Productivity and Yield
4.6. Productivity and Yield of CPC and CPC-D Configurations
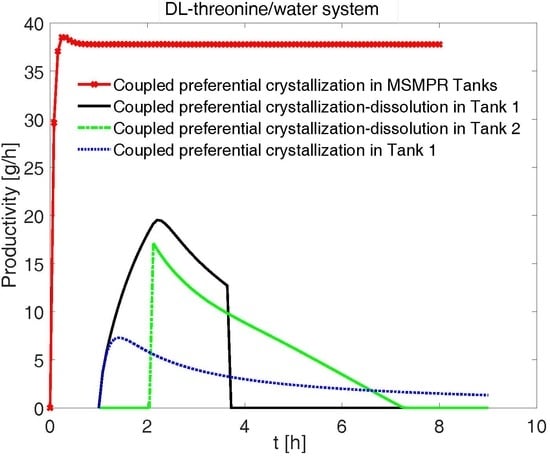
4.7. Comparison of Productivities and Yields for Various Configurations
4.8. Effect of Nucleation Kinetics on the Performances of Various Configurations
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Model Parameters
| Parameter | Symbol | Value | Units |
|---|---|---|---|
| constant for density | |||
| constant for density | |||
| constant for density | |||
| volume shape factor | 0.1222 | - | |
| density of solid threonine | 1250 | ||
| ideal gas constant | 8.314 | J (K mol) | |
| activation energy for growth | J mol | ||
| activation energy for secondary nucleation | J mol | ||
| dissolution rate constant | m s | ||
| growth rate constant | m s | ||
| growth exponent | g | - | |
| growth parameter (size-dependent term) | m | ||
| growth exponent (size-dependent term) | −0.4 | - | |
| secondary nucleation rate constant | m s | ||
| exponent for third moment | 3.0258 | - | |
| secondary nucleation exponent | - | ||
| primary nucleation rate constant | 1000 | s | |
| primary nucleation exponent | 1 | - | |
| nucleation induction time | 7200 | s | |
| slope of sigmoidal function | 0.01 | - | |
| solubility parameter | C | ||
| solubility parameter | - | ||
| solubility parameter | - | ||
| solubility parameter | - | ||
| mean seed size of enantiomer (L-threonine) | 27,95 | ||
| standard deviation of seed CSD | 0.3, 0.34 | - | |
| mean size of racemate | 9 | ||
| standard deviation of racemate CSD | 0.5 | - |
References
- Maier, N.M.; Franco, P.; Lindner, W. Separation of enantiomers: Needs, challenges, perspectives. J. Chromatogr. A 2001, 906, 3–33. [Google Scholar] [CrossRef]
- Heger, W.; Schmahl, H.J.; Klug, S.; Felies, A.; Nau, H.; Merker, H.J.; Neubert, D. Embryotoxic effects of thalidomide derivatives in the non-human primate Callithrix jacchus. IV. Teratogenicity of μg/kg doses of the EM12 enantiomers. Teratog. Carcinog. Mutagen. 1994, 14, 115–122. [Google Scholar] [CrossRef] [PubMed]
- Lin, G.Q.; You, Q.D.; Cheng, J.F. Chiral Drugs: Chemistry and Biological Action; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Lorenz, H.; Seidel-Morgenstern, A. Processes to Separate Enantiomers. Angew. Chem. Int. Ed. 2014, 53, 1218–1250. [Google Scholar] [CrossRef] [PubMed]
- Jacques, J.; Collet, A.; Wilen, S.H. Enantiomers, Racemates, and Resolutions; John Wiley & Sons: Hoboken, NJ, USA, 1981. [Google Scholar]
- Collet, A. Separation and purification of enantiomers by crystallisation methods. Enantiomer 1999, 4, 157–172. [Google Scholar]
- Beilles, S.; Cardinael, P.; Ndzié, E.; Petit, S.; Coquerel, G. Preferential crystallisation and comparative crystal growth study between pure enantiomer and racemic mixture of a chiral molecule: 5-Ethyl-5-methylhydantoin. Chem. Eng. Sci. 2001, 56, 2281–2294. [Google Scholar] [CrossRef]
- Coquerel, G. Preferential crystallization. In Novel Optical Resolution Technologies; Springer: Berlin/Heidelberg, Germany, 2007; pp. 1–51. [Google Scholar]
- Elsner, M.P.; Ziomek, G.; Seidel-Morgenstern, A. Efficient separation of enantiomers by preferential crystallization in two coupled vessels. AIChE J. 2009, 55, 640–649. [Google Scholar] [CrossRef]
- Elsner, M.P.; Ziomek, G.; Seidel-Morgenstern, A. Simultaneous preferential crystallization in a coupled batch operation mode. Part II: Experimental study and model refinement. Chem. Eng. Sci. 2011, 66, 1269–1284. [Google Scholar] [CrossRef]
- Eicke, M.J.; Levilain, G.; Seidel-Morgenstern, A. Efficient Resolution of Enantiomers by Coupling Preferential Crystallization and Dissolution. Part 2: A Parametric Simulation Study to Identify Suitable Process Conditions. Cryst. Growth Des. 2013, 13, 1638–1648. [Google Scholar] [CrossRef]
- Binev, D.; Seidel-Morgenstern, A.; Lorenz, H. Continuous Separation of Isomers in Fluidized Bed Crystallizers. Cryst. Growth Des. 2016, 16, 1409–1419. [Google Scholar] [CrossRef]
- Suwannasang, K.; Flood, A.E.; Coquerel, G. A Novel Design Approach to Scale-Up of the Temperature Cycle Enhanced-Deracemization Process: Coupled Mixed-Suspension Vessels. Cryst. Growth Des. 2016, 16, 6461–6467. [Google Scholar] [CrossRef]
- Viedma, C. Chiral Symmetry Breaking During Crystallization: Complete Chiral Purity Induced by Nonlinear Autocatalysis and Recycling. Phys. Rev. Lett. 2005, 94, 65504. [Google Scholar] [CrossRef] [PubMed]
- Iggland, M.; Mazzotti, M. A Population Balance Model for Chiral Resolution via Viedma Ripening. Cryst. Growth Des. 2011, 11, 4611–4622. [Google Scholar] [CrossRef]
- Xiouras, C.; Ter Horst, J.H.; Van Gerven, T.; Stefanidis, G.D. Coupling Viedma Ripening with Racemic Crystal Transformations: Mechanism of Deracemization. Cryst. Growth Des. 2017, 17, 4965–4976. [Google Scholar] [CrossRef]
- Subramanian, G. A Practical Approach to Chiral Separations by Liquid Chromatography; VCH: Weinheim, Germany, 1994. [Google Scholar]
- Schurig, V. Separation of enantiomers by gas chromatography. J. Chromatogr. A 2001, 906, 275–299. [Google Scholar] [CrossRef]
- Rajendran, A.; Paredes, G.; Mazzotti, M. Simulated moving bed chromatography for the separation of enantiomers. J. Chromatogr. A 2009, 1216, 709–738. [Google Scholar] [CrossRef] [PubMed]
- Keurentjes, J.; Nabuurs, L.; Vegter, E. Liquid membrane technology for the separation of racemic mixtures. J. Membr. Sci. 1996, 113, 351–360. [Google Scholar] [CrossRef]
- Lee, S.B.; Mitchell, D.T.; Trofin, L.; Nevanen, T.K.; Söderlund, H.; Martin, C.R. Antibody-based bio-nanotube membranes for enantiomeric drug separations. Science 2002, 296, 2198–2200. [Google Scholar] [CrossRef] [PubMed]
- Lorenz, H.; Sheehan, P.; Seidel-Morgenstern, A. Coupling of simulated moving bed chromatography and fractional crystallisation for efficient enantioseparation. J. Chromatogr. A 2001, 908, 201–214. [Google Scholar] [CrossRef]
- Ströhlein, G.; Schulte, M.; Strube, J. Hybrid Processes: Design Method for Optimal Coupling of Chromatography and Crystallization Units. Sep. Sci. Technol. 2003, 38, 3353–3383. [Google Scholar] [CrossRef]
- Amanullah, M.; Abel, S.; Mazzotti, M. Separation of Tröger’s Base Enantiomers through a Combination of Simulated Moving Bed Chromatography and Crystallization. Adsorption 2005, 11, 893–897. [Google Scholar] [CrossRef]
- Elsner, M.P.; Ziomek, G.; Seidel-Morgenstern, A. Simultaneous preferential crystallization in a coupled, batch operation mode—Part I: Theoretical analysis and optimization. Chem. Eng. Sci. 2007, 62, 4760–4769. [Google Scholar] [CrossRef]
- Levilain, G.; Eicke, M.J.; Seidel-Morgenstern, A. Efficient Resolution of Enantiomers by Coupling Preferential Crystallization and Dissolution. Part 1: Experimental Proof of Principle. Cryst. Growth Des. 2012, 12, 5396–5401. [Google Scholar] [CrossRef]
- Galan, K.; Eicke, M.J.; Elsner, M.P.; Lorenz, H.; Seidel-Morgenstern, A. Continuous Preferential Crystallization of Chiral Molecules in Single and Coupled Mixed-Suspension Mixed-Product-Removal Crystallizers. Cryst. Growth Des. 2015, 15, 1808–1818. [Google Scholar] [CrossRef]
- Qamar, S.; Galan, K.; Elsner, M.P.; Hussain, I.; Seidel-Morgenstern, A. Theoretical investigation of simultaneous continuous preferential crystallization in a coupled mode. Chem. Eng. Sci. 2013, 98, 25–39. [Google Scholar] [CrossRef]
- Köllges, T.; Vetter, T. Model-Based Analysis of Continuous Crystallization/Reaction Processes Separating Conglomerate Forming Enantiomers. Cryst. Growth Des. 2017, 17, 233–247. [Google Scholar] [CrossRef]
- Vetter, T.; Burcham, C.L.; Doherty, M.F. Separation of conglomerate forming enantiomers using a novel continuous preferential crystallization process. AIChE J. 2015, 61, 2810–2823. [Google Scholar] [CrossRef]
- Marchisio, D.L.; Pikturna, J.T.; Fox, R.O.; Vigil, R.D.; Barresi, A.A. Quadrature method of moments for population-balance equations. AIChE J. 2003, 49, 1266–1276. [Google Scholar] [CrossRef]
- Ferguson, S.; Ortner, F.; Quon, J.; Peeva, L.; Livingston, A.; Trout, B.L.; Myerson, A.S. Use of Continuous MSMPR Crystallization with Integrated Nanofiltration Membrane Recycle for Enhanced Yield and Purity in API Crystallization. Cryst. Growth Des. 2014, 14, 617–627. [Google Scholar] [CrossRef]
- Schaber, S.D.; Gerogiorgis, D.I.; Ramachandran, R.; Evans, J.M.B.; Barton, P.I.; Trout, B.L. Economic Analysis of Integrated Continuous and Batch Pharmaceutical Manufacturing: A Case Study. Ind. Eng. Chem. Res. 2011, 50, 10083–10092. [Google Scholar] [CrossRef]











| CPC-MSMPR | CPC | CPC-D | |||||
|---|---|---|---|---|---|---|---|
| Variable | Tank 1 | Tank 2 | Tank 1 | Tank 2 | Tank 1 | Tank 2 | |
| Liquid phase | g | g | g | g | g | g | |
| g | g | g | g | g | g | ||
| Solid phase | (L-threonine) | g | − | g | − | g | g |
| (D-threonine) | − | g | − | g | − | g | |
| Temperatures | |||||||
| Exchange rate | 80 mL min | 80 mL min | 80 mL min | ||||
| Feed rate | q | 80 mL min | 80 mL min | − | − | − | − |
| Removal rate | q | 80 mL min | 80 mL min | − | − | − | − |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Majumder, A.; Nagy, Z.K. A Comparative Study of Coupled Preferential Crystallizers for the Efficient Resolution of Conglomerate-Forming Enantiomers. Pharmaceutics 2017, 9, 55. https://doi.org/10.3390/pharmaceutics9040055
Majumder A, Nagy ZK. A Comparative Study of Coupled Preferential Crystallizers for the Efficient Resolution of Conglomerate-Forming Enantiomers. Pharmaceutics. 2017; 9(4):55. https://doi.org/10.3390/pharmaceutics9040055
Chicago/Turabian StyleMajumder, Aniruddha, and Zoltan K. Nagy. 2017. "A Comparative Study of Coupled Preferential Crystallizers for the Efficient Resolution of Conglomerate-Forming Enantiomers" Pharmaceutics 9, no. 4: 55. https://doi.org/10.3390/pharmaceutics9040055
APA StyleMajumder, A., & Nagy, Z. K. (2017). A Comparative Study of Coupled Preferential Crystallizers for the Efficient Resolution of Conglomerate-Forming Enantiomers. Pharmaceutics, 9(4), 55. https://doi.org/10.3390/pharmaceutics9040055